Resonant Adaptive MEMS Mirror
Abstract
:1. Introduction
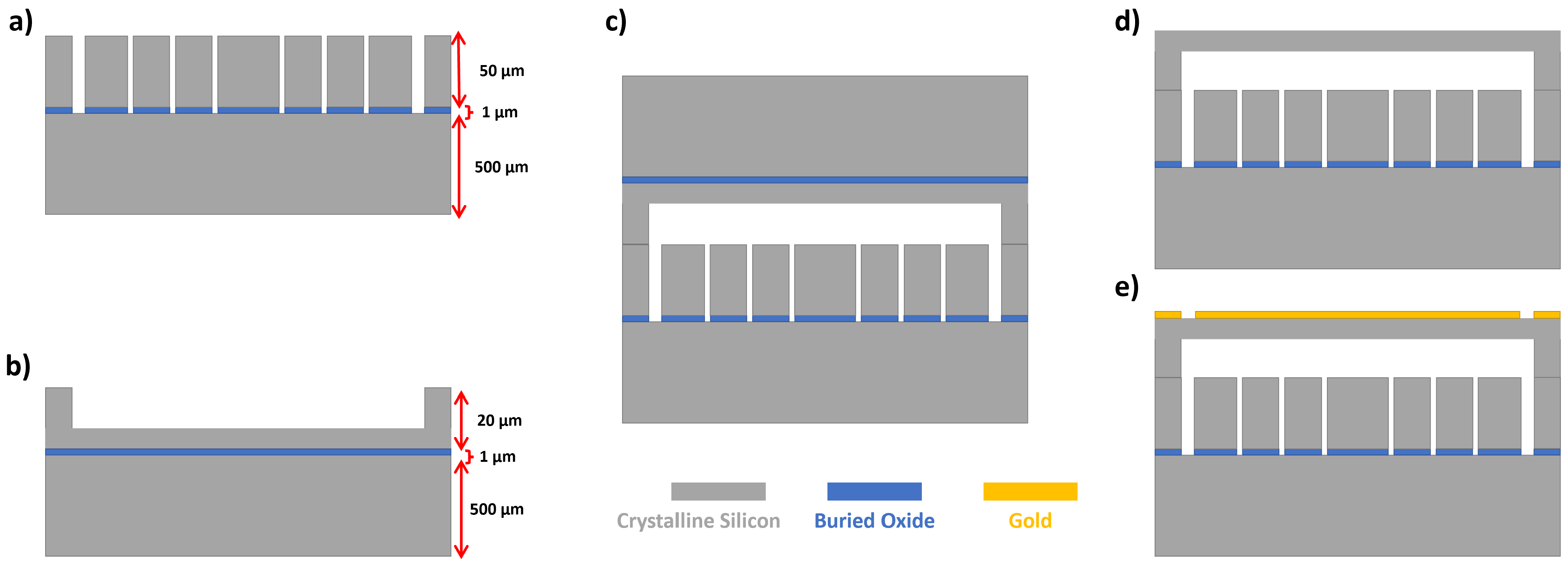
2. Mirror Design and Fabrication
2.1. Design

| Parameter | μm |
|---|---|
| Mirror radius, a | 800 |
| Mirror thickness, t | 10 |
| Beam length, l | 100 |
| Beam thickness, h | 10 |
| Beam width, w | 15 |
| Capacitive gap, d | 20 |
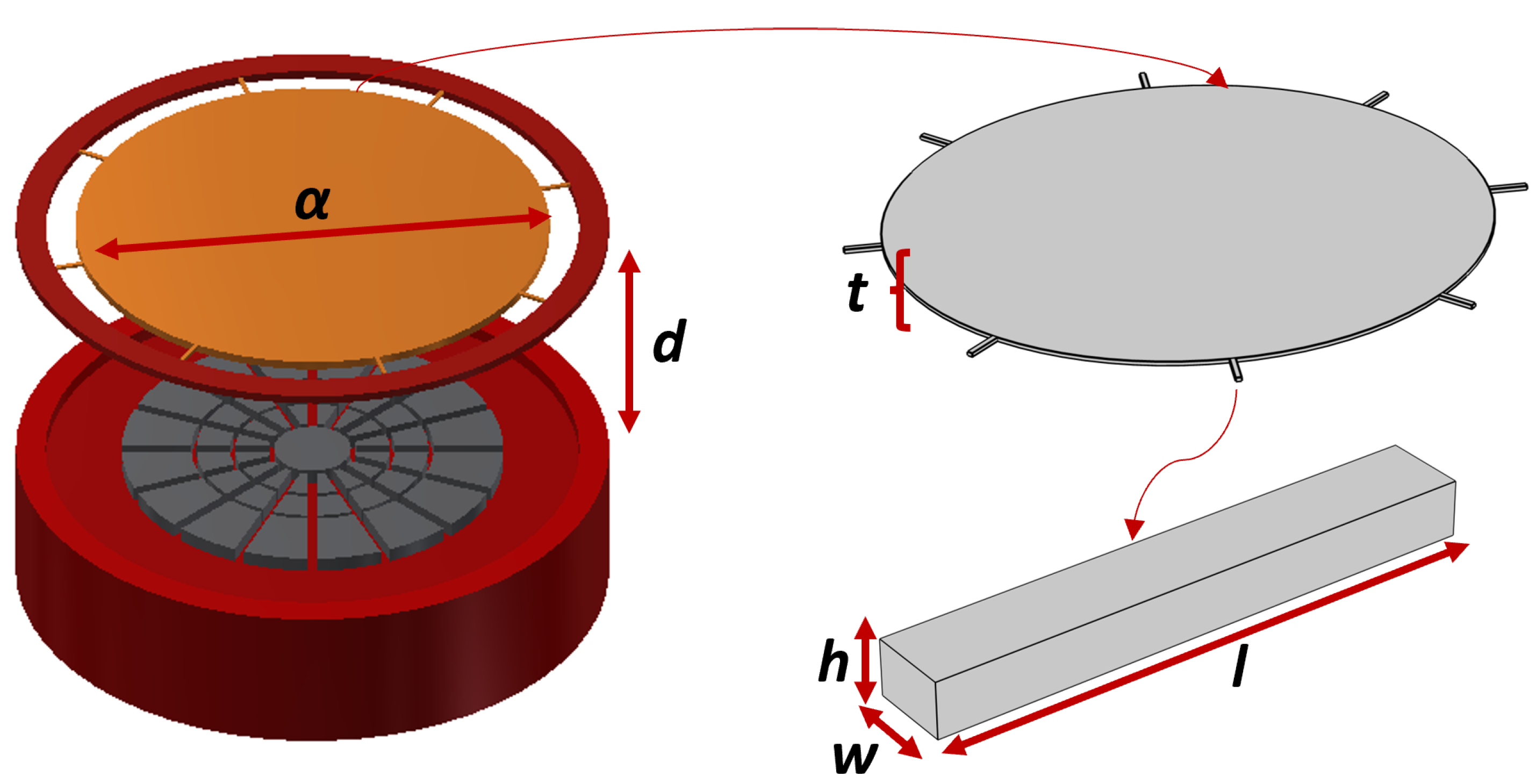
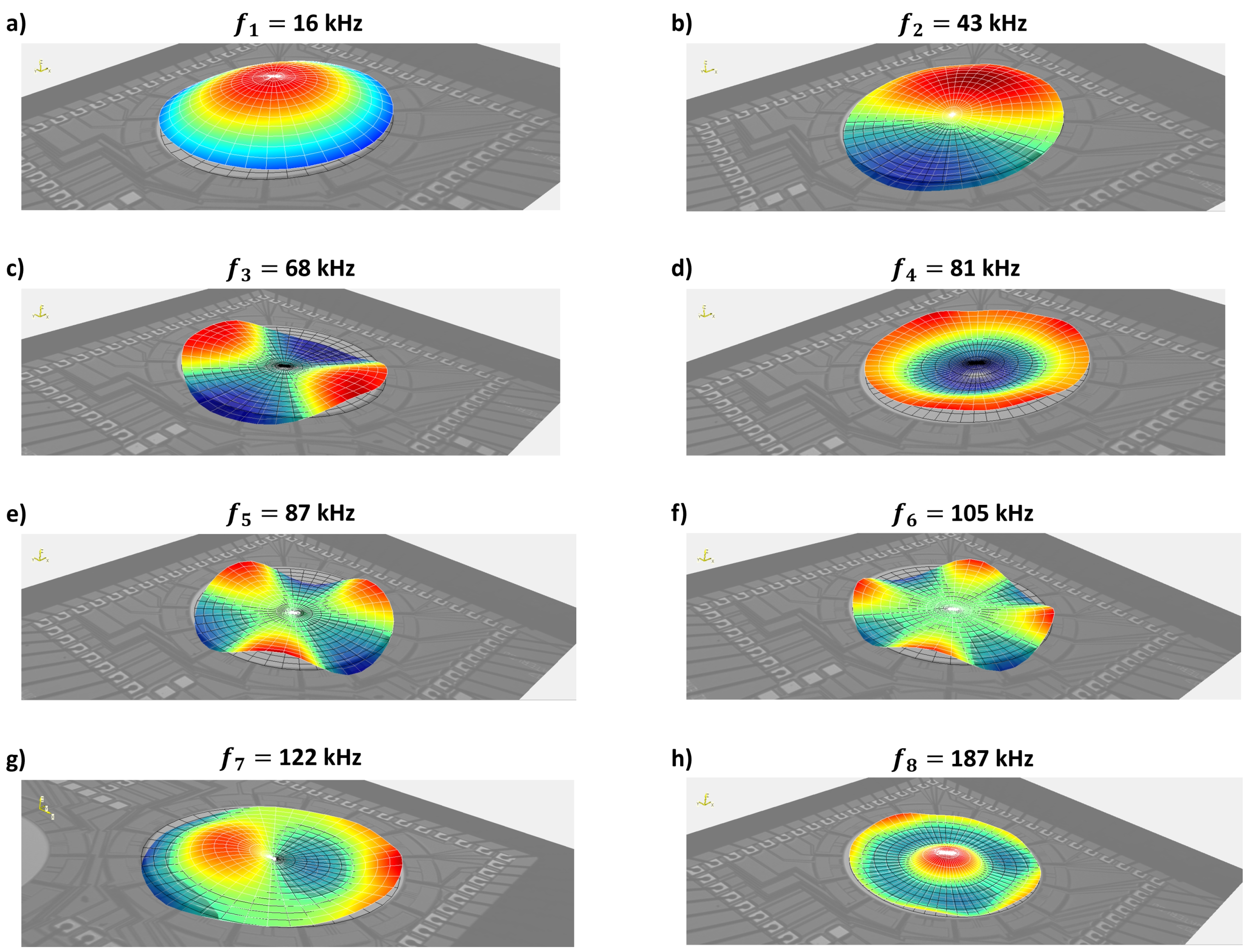
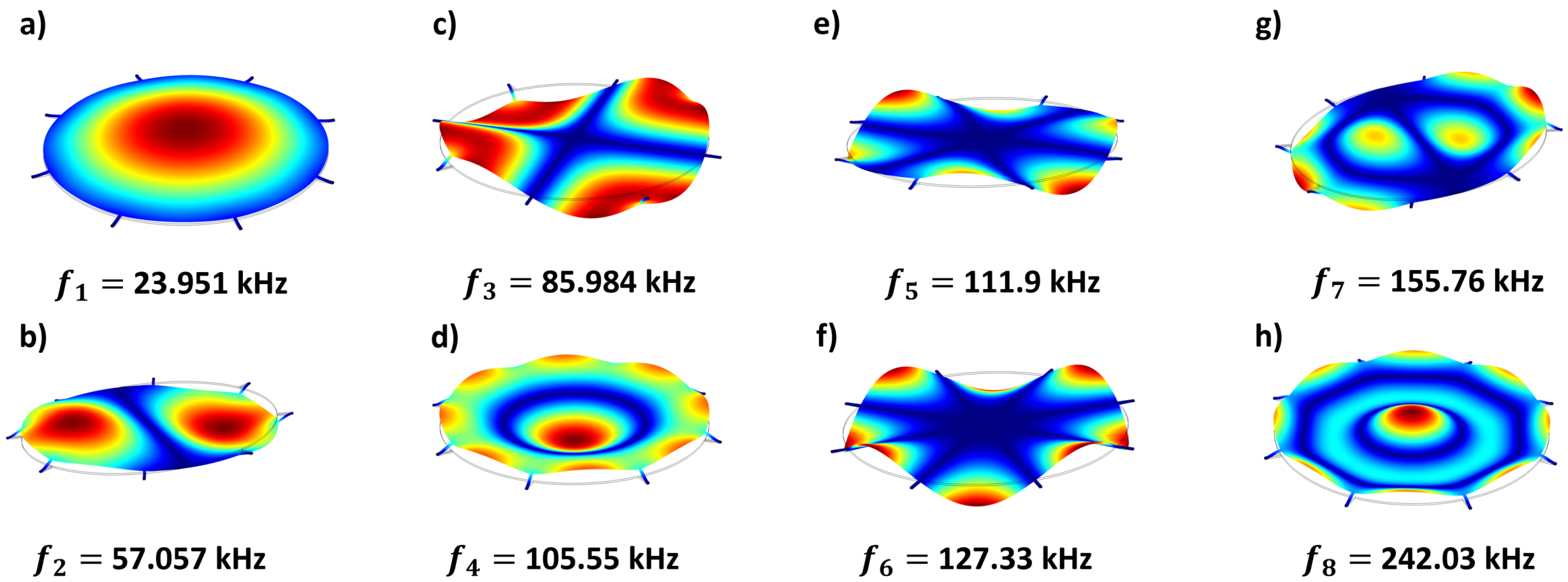
2.2. Predicted Mode Shapes
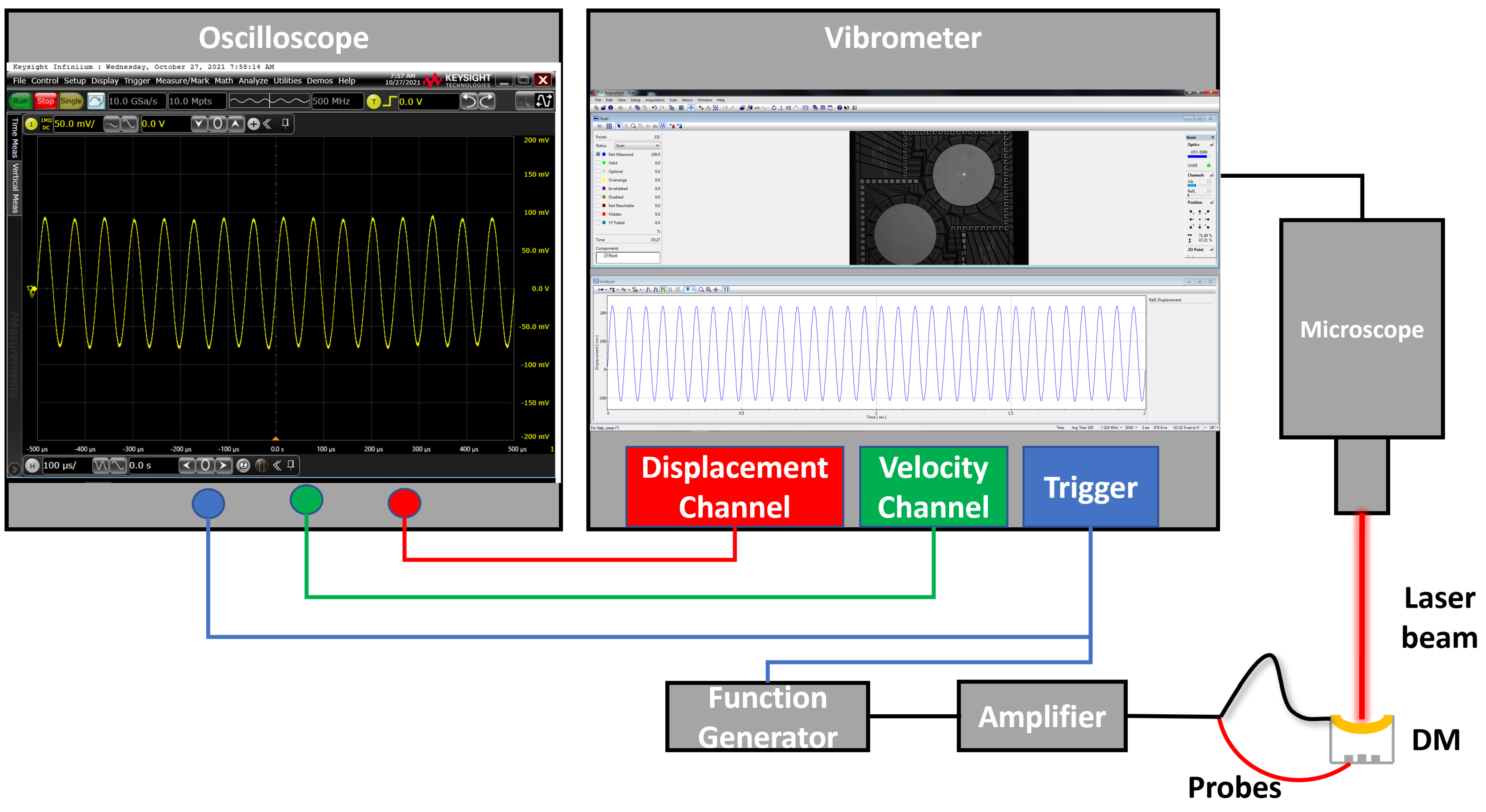
3. Experimental Characterization
4. Results and Discussion
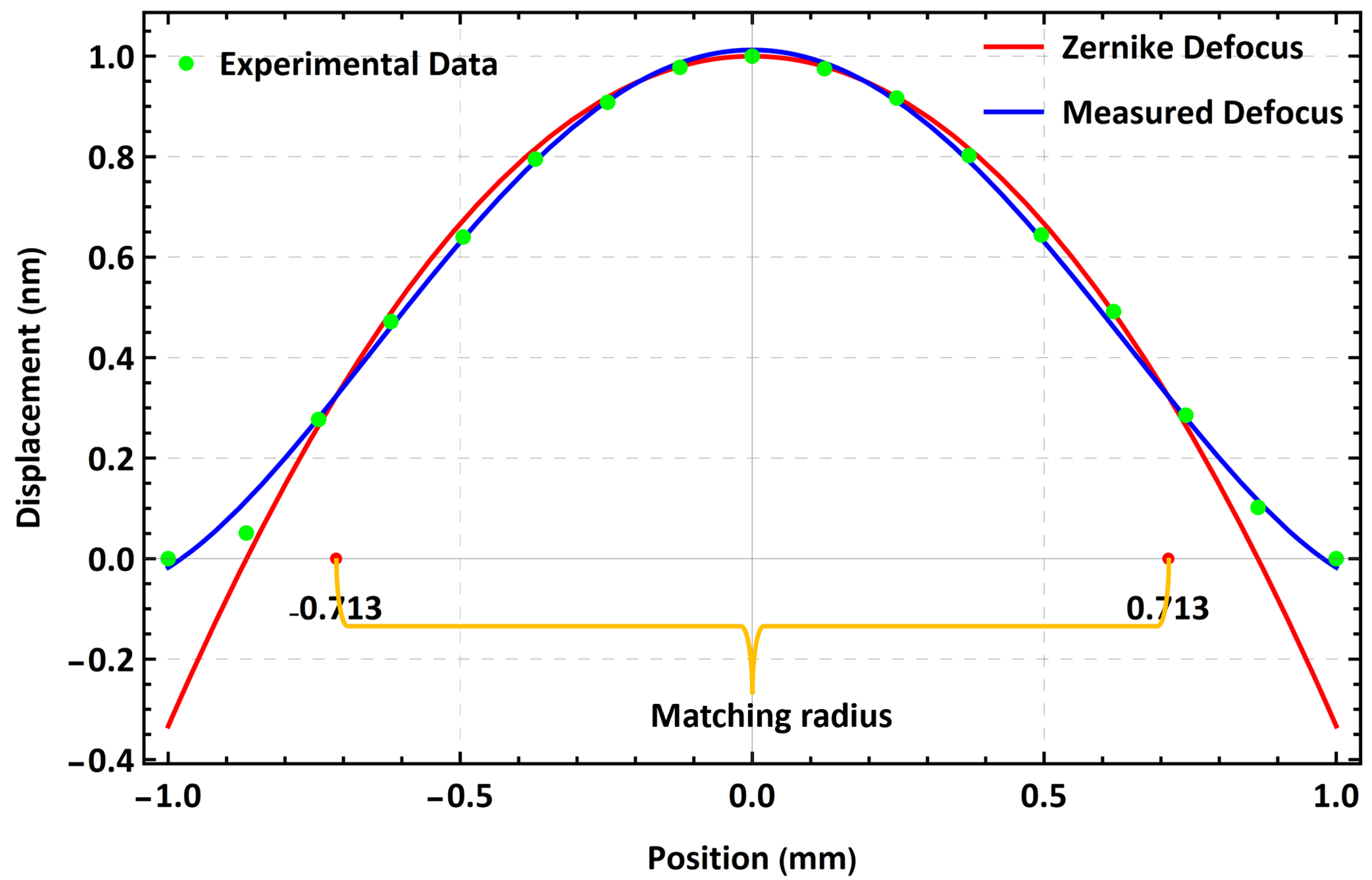
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Booth, M.J. Adaptive optics in microscopy. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2007, 365, 2829–2843. [Google Scholar] [CrossRef] [PubMed]
- Dai, G.M. Wavefront Optics for Vision Correction; SPIE Press: Bellingham, WC, USA, 2008; Volume 179. [Google Scholar]
- Booth, M.J. Adaptive optical microscopy: The ongoing quest for a perfect image. Light. Sci. Appl. 2014, 3, e165. [Google Scholar] [CrossRef]
- Beckers, J.M. Adaptive optics for astronomy: Principles, performance, and applications. Annu. Rev. Astron. Astrophys. 1993, 31, 13–62. [Google Scholar] [CrossRef]
- Davies, R.; Kasper, M. Adaptive optics for astronomy. Annu. Rev. Astron. Astrophys. 2012, 50, 305–351. [Google Scholar] [CrossRef]
- Ji, N. Adaptive optical fluorescence microscopy. Nat. Methods 2017, 14, 374–380. [Google Scholar] [CrossRef]
- Rodríguez, C.; Ji, N. Adaptive optical microscopy for neurobiology. Curr. Opin. Neurobiol. 2018, 50, 83–91. [Google Scholar] [CrossRef]
- Peinado, A.; Bendek, E.; Yokoyama, S.; Poskanzer, K.E. Deformable mirror-based axial scanning for two-photon mammalian brain imaging. Neurophotonics 2021, 8, 015003. [Google Scholar] [CrossRef]
- Roorda, A.; Duncan, J.L. Adaptive optics ophthalmoscopy. Annu. Rev. Vis. Sci. 2015, 1, 19–50. [Google Scholar] [CrossRef]
- Akyol, E.; Hagag, A.M.; Sivaprasad, S.; Lotery, A.J. Adaptive optics: Principles and applications in ophthalmology. Eye 2021, 35, 244–264. [Google Scholar] [CrossRef]
- Booth, M.J.; Neil, M.A.; Juškaitis, R.; Wilson, T. Adaptive aberration correction in a confocal microscope. Proc. Natl. Acad. Sci. USA 2002, 99, 5788–5792. [Google Scholar] [CrossRef]
- Lakshminarayanan, V.; Fleck, A. Zernike polynomials: A guide. J. Mod. Opt. 2011, 58, 545–561. [Google Scholar] [CrossRef]
- Tyson, R.K.; Frazier, B.W. Principles of Adaptive Optics; CRC Press: Boca Raton, FL, USA, 2022. [Google Scholar]
- Ealey, M.A.; Wellman, J.A. Deformable mirrors: Design fundamentals, key performance specifications, and parametric trades. In Active and Adaptive Optical Components; SPIE: Bellingham, WC, USA, 1992; Volume 1543, pp. 36–51. [Google Scholar]
- Bifano, T.G.; Perreault, J.; Mali, R.K.; Horenstein, M.N. Microelectromech. deformable mirrors. IEEE J. Sel. Top. Quantum Electron. 1999, 5, 83–89. [Google Scholar] [CrossRef]
- Madec, P.Y. Overview of deformable mirror technologies for adaptive optics and astronomy. In Adaptive Optics Systems III; SPIE: Bellingham, WC, USA, 2012; Volume 8447, p. 844705. [Google Scholar]
- Ealey, M.A.; Washeba, J.F. Continuous facesheet low voltage deformable mirrors. Opt. Eng. 1990, 29, 1191–1198. [Google Scholar] [CrossRef]
- Sinquin, J.C.; Lurçon, J.M.; Guillemard, C. Deformable mirror technologies for astronomy at CILAS. In Adaptive Optics Systems; Hubin, N., Max, C.E., Wizinowich, P.L., Eds.; SPIE: Bellingham, WC, USA, 2008; Volume 7015, pp. 151–162. [Google Scholar] [CrossRef]
- Biasi, R.; Gallieni, D.; Salinari, P.; Riccardi, A.; Mantegazza, P. Contactless thin adaptive mirror technology: Past, present, and future. In Adaptive Optics Systems II; Ellerbroek, B.L., Hart, M., Hubin, N., Wizinowich, P.L., Eds.; SPIE: Bellingham, WC, USA, 2010; Volume 7736, pp. 872–885. [Google Scholar] [CrossRef]
- Bifano, T. MEMS deformable mirrors. Nat. Photonics 2011, 5, 21–23. [Google Scholar] [CrossRef]
- Morgan, R.E.; Douglas, E.S.; Allan, G.W.; Bierden, P.; Chakrabarti, S.; Cook, T.; Egan, M.; Furesz, G.; Gubner, J.N.; Groff, T.D.; et al. MEMS deformable mirrors for space-based high-contrast imaging. Micromachines 2019, 10, 366. [Google Scholar] [CrossRef]
- Helmbrecht, M.A.; He, M.; Juneau, T.; Hart, M.; Doble, N. Segmented MEMS deformable-mirror for wavefront correction. In Optomechatronic Micro/Nano Devices and Components II; SPIE: Bellingham, WC, USA, 2006; Volume 6376, p. 63760D. [Google Scholar]
- Dagel, D.J.; Cowan, W.D.; Spahn, O.B.; Grossetete, G.D.; Grine, A.J.; Shaw, M.J.; Resnick, P.J.; Jokiel, B. Large-stroke MEMS deformable mirrors for adaptive optics. J. Microelectromech. Syst. 2006, 15, 572–583. [Google Scholar] [CrossRef]
- Stewart, J.B.; Bifano, T.G.; Cornelissen, S.; Bierden, P.; Levine, B.M.; Cook, T. Design and development of a 331-segment tip–tilt–piston mirror array for space-based adaptive optics. Sens. Actuators A Phys. 2007, 138, 230–238. [Google Scholar] [CrossRef]
- Kempf, C.J.; Helmbrecht, M.A.; Besse, M. Adaptive optics control system for segmented MEMS deformable mirrors. In Adaptive Optics IV; SPIE: Bellingham, WC, USA, 2010; Volume 7595, p. 75950M. [Google Scholar]
- Manzanera, S.; Helmbrecht, M.A.; Kempf, C.J.; Roorda, A. MEMS segmented-based adaptive optics scanning laser ophthalmoscope. Biomed. Opt. Express 2011, 2, 1204–1217. [Google Scholar] [CrossRef]
- Cornelissen, S.A.; Bierden, P.A.; Bifano, T.G.; Lam, C.L.V. 4096-element continuous face-sheet MEMS deformable mirror for high-contrast imaging. J. Micro/Nanolithogr. MEMS MOEMS 2009, 8, 1–8. [Google Scholar] [CrossRef]
- Nakazawa, K.; Sasaki, T.; Furuta, H.; Kamiya, J.; Kamiya, T.; Hane, K. Varifocal scanner using wafer bonding. J. Microelectromech. Syst. 2017, 26, 440–447. [Google Scholar] [CrossRef]
- Sasaki, T.; Kamada, T.; Hane, K. High-speed and large-amplitude resonant varifocal mirror. J. Robot. Mechatron. 2020, 32, 344–350. [Google Scholar] [CrossRef]
- Qi, B.; Himmer, A.P.; Gordon, L.M.; Yang, X.V.; Dickensheets, L.D.; Vitkin, I.A. Dynamic focus control in high-speed optical coherence tomography based on a microelectromechanical mirror. Opt. Commun. 2004, 232, 123–128. [Google Scholar] [CrossRef]
- Banerjee, K.; Rajaeipour, P.; Zappe, H.; Ataman, Ç. A 37-actuator polyimide deformable mirror with electrostatic actuation for adaptive optics microscopy. J. Micromech. Microeng. 2019, 29, 085005. [Google Scholar] [CrossRef]
- Younis, M.I. MEMS Linear and Nonlinear Statics and Dynamics; Springer: Berlin/Heidelberg, Germany, 2011; Volume 20. [Google Scholar]
- Algamili, A.S.; Khir, M.H.M.; Dennis, J.O.; Ahmed, A.Y.; Alabsi, S.S.; Hashwan, S.S.B.; Junaid, M.M. A review of actuation and sensing mechanisms in mems-based sensor devices. Nanoscale Res. Lett. 2021, 16, 1–21. [Google Scholar] [CrossRef]
- Guzmán, D.; de Cos Juez, F.J.; Lasheras, F.S.; Myers, R.; Young, L. Deformable mirror model for open-loop adaptive optics using multivariate adaptive regression splines. Opt. Express 2010, 18, 6492–6505. [Google Scholar] [CrossRef]
- Vogel, C.; Tyler, G.; Lu, Y.; Bifano, T.; Conan, R.; Blain, C. Modeling and parameter estimation for point-actuated continuous-facesheet deformable mirrors. JOSA A 2010, 27, A56–A63. [Google Scholar] [CrossRef]
- Zou, W.; Burns, S.A. High-accuracy wavefront control for retinal imaging with Adaptive-Influence-Matrix Adaptive Optics. Opt. Express 2009, 17, 20167–20177. [Google Scholar] [CrossRef]
- Huang, L.; Ma, X.; Bian, Q.; Li, T.; Zhou, C.; Gong, M. High-precision system identification method for a deformable mirror in wavefront control. Appl. Opt. 2015, 54, 4313–4317. [Google Scholar] [CrossRef]
- Haber, A.; Bifano, T. General approach to precise deformable mirror control. Opt. Express 2021, 29, 33741–33759. [Google Scholar] [CrossRef]
- Hampson, K.M.; Turcotte, R.; Miller, D.T.; Kurokawa, K.; Males, J.R.; Ji, N.; Booth, M.J. Adaptive optics for high-resolution imaging. Nat. Rev. Methods Prim. 2021, 1, 1–26. [Google Scholar] [CrossRef]
- Hishinuma, Y.; Yang, E.H. Piezoelectric unimorph microactuator arrays for single-crystal silicon continuous-membrane deformable mirror. J. Microelectromech. Syst. 2006, 15, 370–379. [Google Scholar] [CrossRef]
- Ma, J.; Liu, Y.; Chen, C.; Li, B.; Chu, J. Deformable mirrors based on piezoelectric unimorph microactuator array for adaptive optics correction. Opt. Commun. 2011, 284, 5062–5066. [Google Scholar] [CrossRef]
- Wapler, M.C.; Lemke, F.; Alia, G.; Wallrabe, U. Aspherical high-speed varifocal mirror for miniature catadioptric objectives. Opt. Express 2018, 26, 6090–6102. [Google Scholar] [CrossRef] [PubMed]
- Pribošek, J.; Bainschab, M.; Piot, A.; Moridi, M. Aspherical high-speed varifocal piezoelectric mems mirror. In Proceedings of the 2021 21st International Conference on Solid-State Sensors, Actuators and Microsystems (Transducers), Online, 20–25 June 2021; IEEE: Piscataway, NJ, USA, 2021; pp. 1088–1091. [Google Scholar]
- Cugat, O.; Basrour, S.; Divoux, C.; Mounaix, P.; Reyne, G. Deformable magnetic mirror for adaptive optics: Technological aspects. Sens. Actuators A Phys. 2001, 89, 1–9. [Google Scholar] [CrossRef]
- Hossain, M.M.; Lee, J.; Jung, D.; Kong, S.H. Focus-tunable micro-reflective lens: Design and fabrication feasibility with deformable micromirror. Solid-State Electron. 2019, 161, 107633. [Google Scholar] [CrossRef]
- Huang, L.; Xue, Q.; Yan, P.; Gong, M.; Li, T.; Feng, Z.; Ma, X. A thermo-field bimetal deformable mirror for wavefront correction in high power lasers. Laser Phys. Lett. 2013, 11, 015001. [Google Scholar] [CrossRef]
- Kasprzack, M.; Canuel, B.; Cavalier, F.; Day, R.; Genin, E.; Marque, J.; Sentenac, D.; Vajente, G. Performance of a thermally deformable mirror for correction of low-order aberrations in laser beams. Appl. Opt. 2013, 52, 2909–2916. [Google Scholar] [CrossRef]
- Morrison, J.; Imboden, M.; Little, T.D.; Bishop, D. Electrothermally actuated tip-tilt-piston micromirror with integrated varifocal capability. Opt. Express 2015, 23, 9555–9566. [Google Scholar] [CrossRef]
- Liu, Z.; Kocaoglu, O.P.; Miller, D.T. In-the-plane design of an off-axis ophthalmic adaptive optics system using toroidal mirrors. Biomed. Opt. Express 2013, 4, 3007–3030. [Google Scholar] [CrossRef]
- Chen, Z.; Mc Larney, B.; Rebling, J.; Deán-Ben, X.L.; Zhou, Q.; Gottschalk, S.; Razansky, D. High-Speed Large-Field Multifocal Illumination Fluorescence Microscopy. Laser Photonics Rev. 2020, 14, 1900070. [Google Scholar] [CrossRef]
- Micralyne, Inc. MicraGEM-Si Platform Design Handbook (Ver.1.3); Micralyne, Inc.: Edmonton, AB, Canada, 2016. [Google Scholar]
- Bifano, T.G.; Perreault, J.A.; Bierden, P.A. Micromachined deformable mirror for optical wavefront compensation. In High-Resolution Wavefront Control: Methods, Devices, and Applications II; Gonglewski, J.D., Vorontsov, M.A., Gruneisen, M.T., Eds.; SPIE: Bellingham, WC, USA, 2000; Volume 4124, pp. 7–14. [Google Scholar] [CrossRef]
- Boston Micromachines Inc. Low Actuator Count Deformable Mirrors. Available online: https://bostonmicromachines.com/wp-content/uploads/2021/09/Low-Actuator-Count-DM-2021_3.pdf (accessed on 18 June 2022).
- Iris AO Inc. PTT111 Deformable Mirror System. Available online: http://www.irisao.com/documents/irisao.ptt111.pdf (accessed on 18 June 2022).
- Boston Micromachines Inc. +K-DM Family. Available online: https://bostonmicromachines.com/wp-content/uploads/2020/04/BMC-K-Deformable-Mirrors.pdf (accessed on 18 June 2022).
- Xu, X.H.; Li, B.Q.; Feng, Y.; Chu, J.R. Design, fabrication and characterization of a bulk-PZT-actuated MEMS deformable mirror. J. Micromech. Microeng. 2007, 17, 2439. [Google Scholar] [CrossRef]
- Park, B.; Afsharipour, E.; Chrusch, D.; Shafai, C.; Andersen, D.; Burley, G. A low voltage and large stroke Lorentz force continuous deformable polymer mirror for wavefront control. Sens. Actuators A Phys. 2018, 280, 197–204. [Google Scholar] [CrossRef]

| Author | Actuation | Stroke (μm) | # Actuators | Surface | V (RMS) | Aperture (mm) |
|---|---|---|---|---|---|---|
| Bifano [52] | E.static | 2 | 140 | Cont. | 240 | 3.3 |
| BMC Inc. [53] | E.static | 1.5–5.5 | 137, 140 | Cont. | – | – |
| Helmbrecht [22] | E.static | 7.5 | 137, 111 | Segment | 125 | 3.5 |
| Iris AO Inc. [54] | E.static | 7 | 111 | Segment | – | 3.5 |
| Cornelissen [27] | E.static | 3 | 4096 | Cont. | 225 | 26.8 |
| BMC Inc. [55] | E.static | 1.5–5.5 | 2048–4092 | Cont. | – | 18.6–25 |
| Hishinuma [40] | PZT | 5 | 16 | Cont. | 50 | 2.5 |
| Xu [56] | PZT | 4.5 | 100 | Cont. | 100 | 30 |
| Park [57] | E.magnetic | 17 | 25 | Cont. | <1 | 12 |
| This work | E.static | 1.2 | 49 | Cont. | 106 | 1.6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kamel, A.; Kocer, S.; Mukhangaliyeva, L.; Saritas, R.; Gulsaran, A.; Elhady, A.; Basha, M.; Hajireza, P.; Yavuz, M.; Abdel-Rahman, E. Resonant Adaptive MEMS Mirror. Actuators 2022, 11, 224. https://doi.org/10.3390/act11080224
Kamel A, Kocer S, Mukhangaliyeva L, Saritas R, Gulsaran A, Elhady A, Basha M, Hajireza P, Yavuz M, Abdel-Rahman E. Resonant Adaptive MEMS Mirror. Actuators. 2022; 11(8):224. https://doi.org/10.3390/act11080224
Chicago/Turabian StyleKamel, Amr, Samed Kocer, Lyazzat Mukhangaliyeva, Resul Saritas, Ahmet Gulsaran, Alaa Elhady, Mohamed Basha, Parsin Hajireza, Mustafa Yavuz, and Eihab Abdel-Rahman. 2022. "Resonant Adaptive MEMS Mirror" Actuators 11, no. 8: 224. https://doi.org/10.3390/act11080224
APA StyleKamel, A., Kocer, S., Mukhangaliyeva, L., Saritas, R., Gulsaran, A., Elhady, A., Basha, M., Hajireza, P., Yavuz, M., & Abdel-Rahman, E. (2022). Resonant Adaptive MEMS Mirror. Actuators, 11(8), 224. https://doi.org/10.3390/act11080224








