Research on the Influence of Friction Pairs on the Output Characteristics of the Piezoelectric Ultrasonic Actuator
Abstract
:1. Introduction
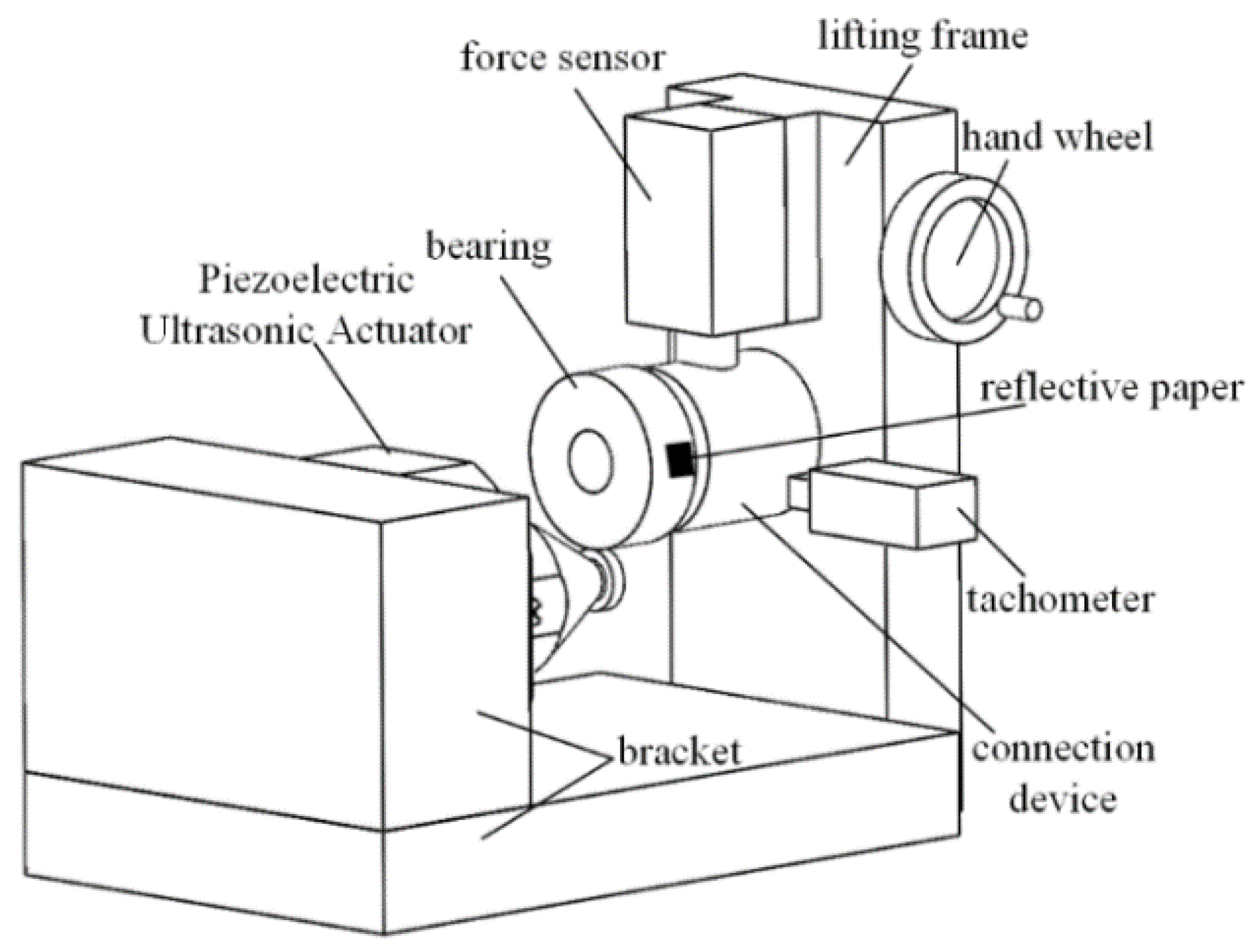
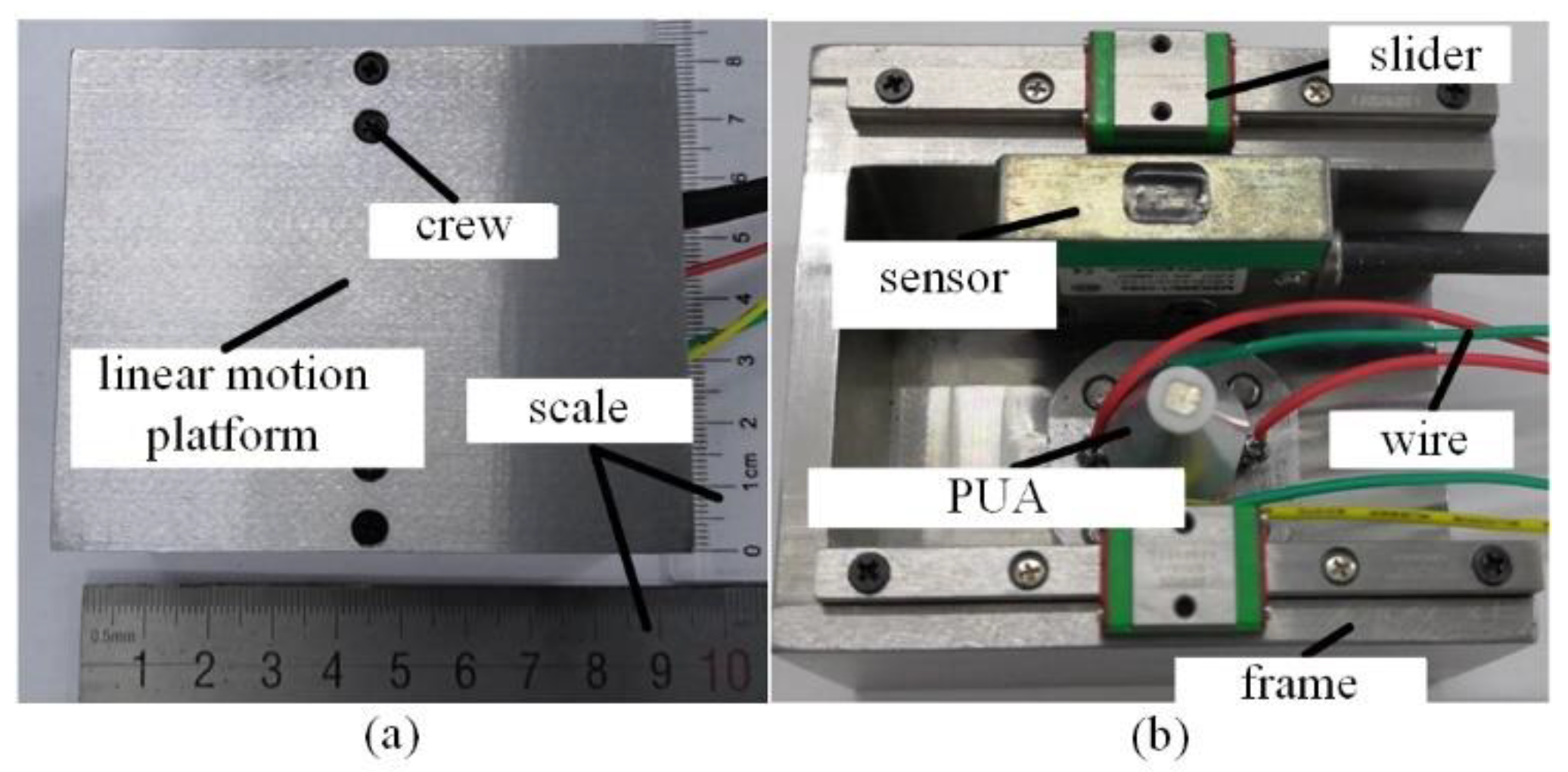
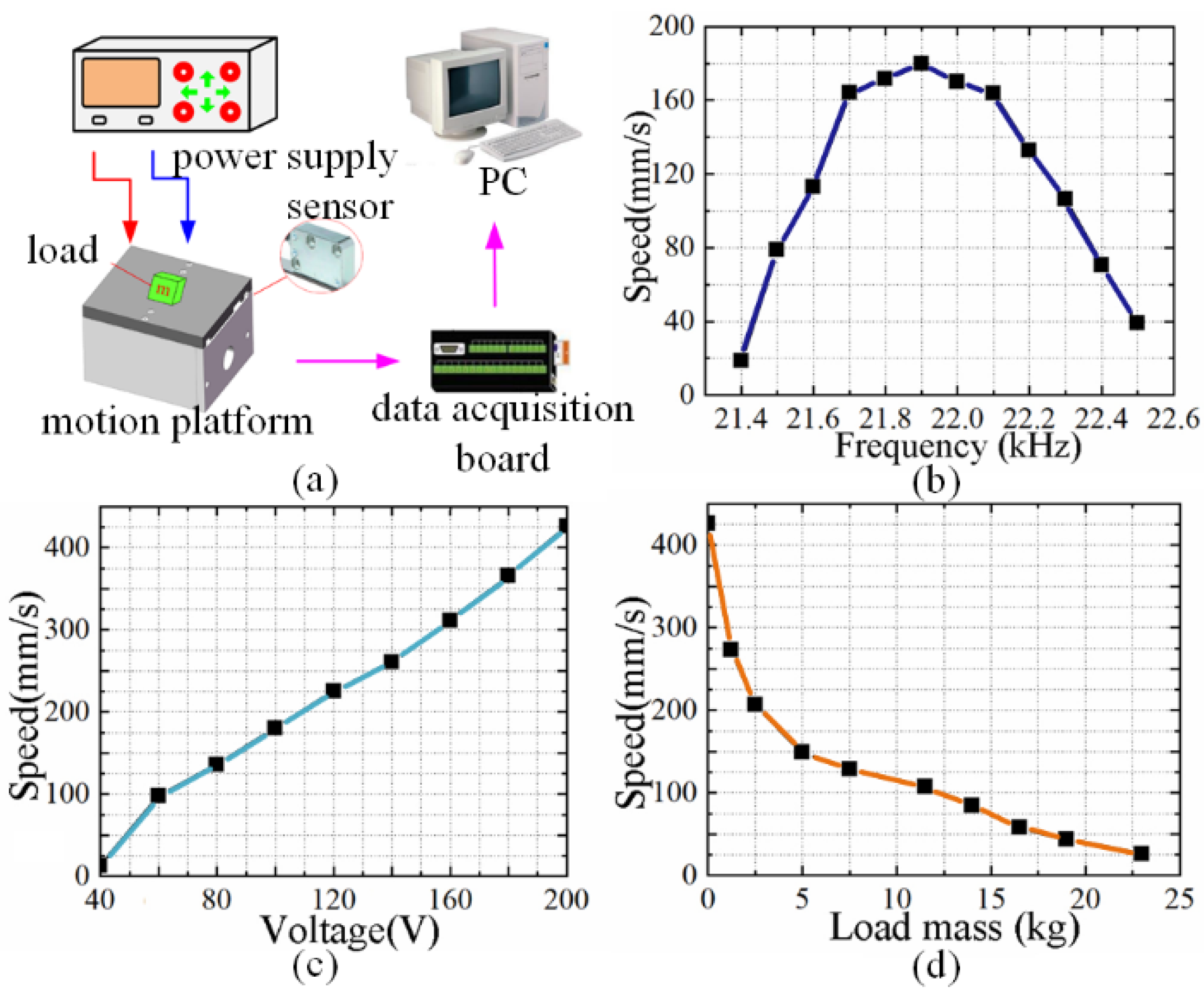
2. Experimental Platform Construction and Friction Pair Performance Test
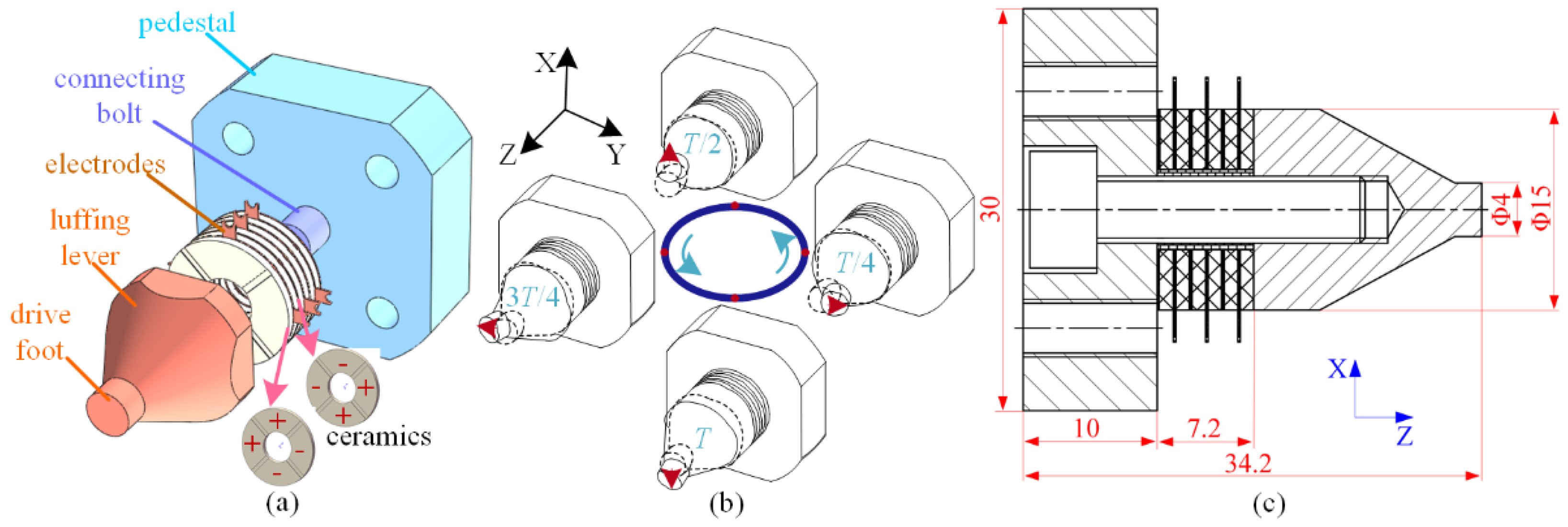
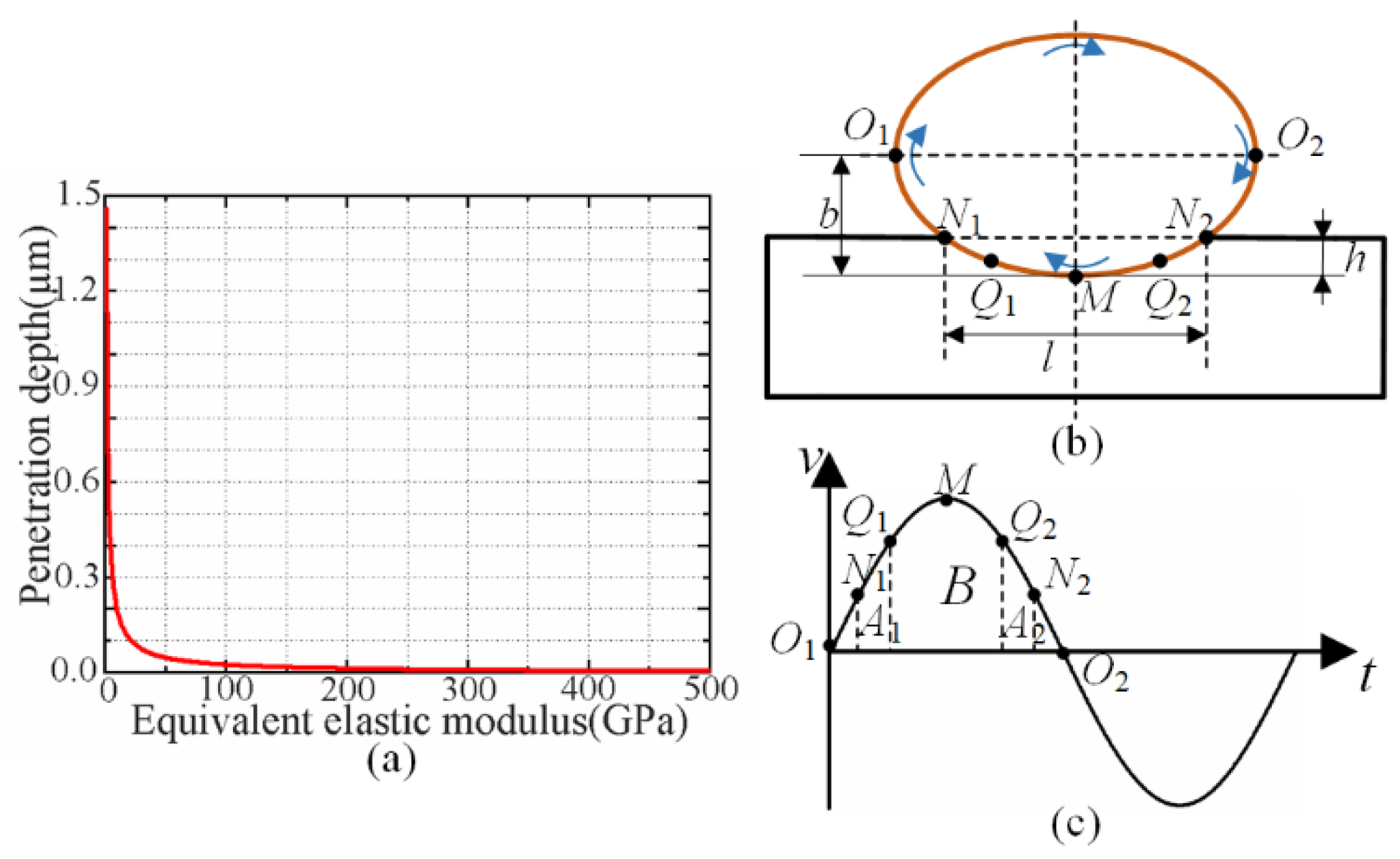
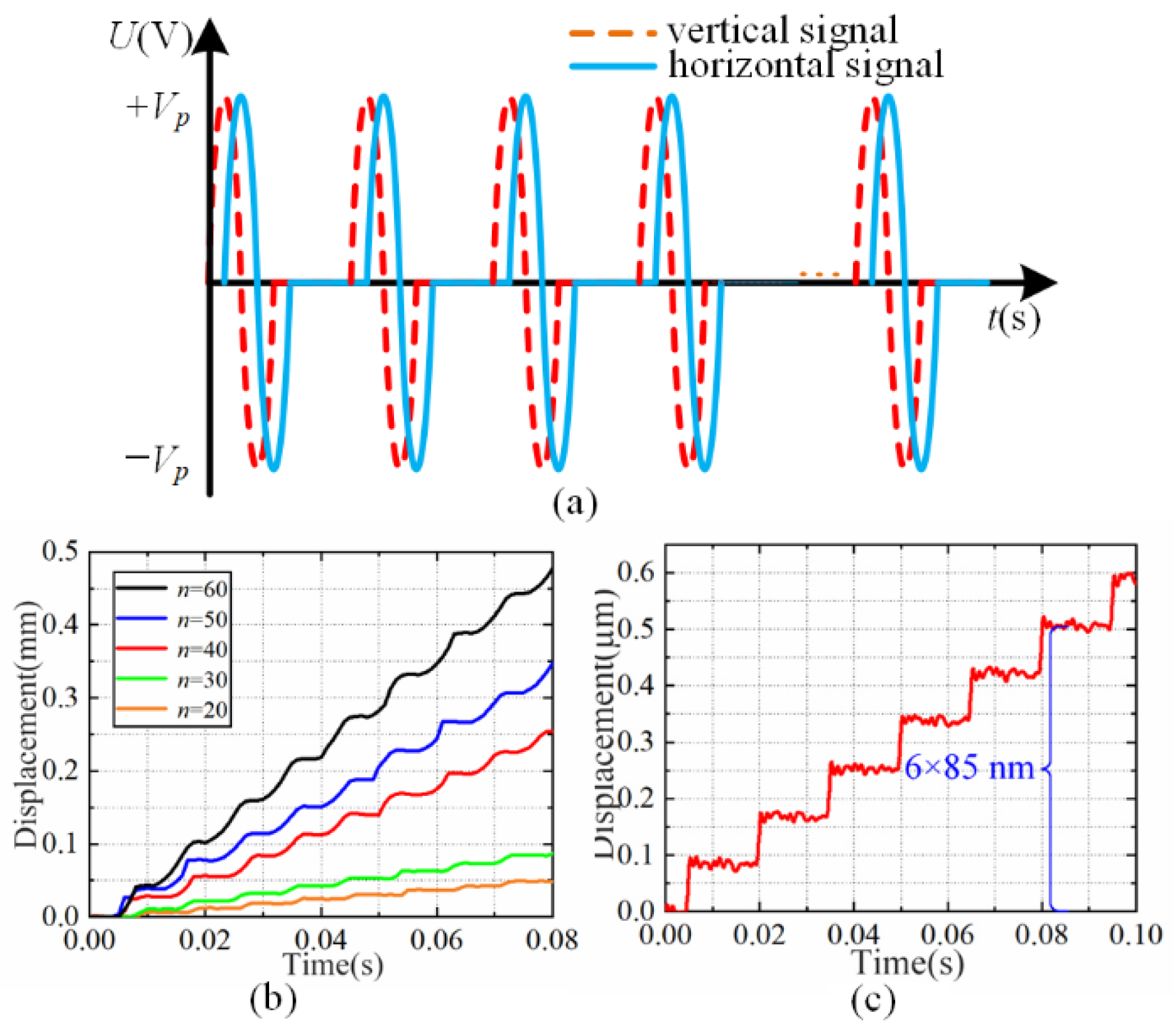
2.1. Configuration of the Actuator and Actuating Principle
- (1)
- t = T/4: The excitation voltage applied to the two vertical zones of the piezoelectric ceramics reaches the maximum positive value, and the bending displacement of the PUA along the negative direction of the Y axis reaches the maximum to press down the actuator.
- (2)
- t = T/2: The excitation voltage applied to the horizontal two regions of the piezoelectric ceramic reaches the maximum value, and the bending displacement of the PUA reaches the maximum along the positive direction of the X-axis. The driving foot moves along the positive X-axis of the actuator between t = T/4 and t = T/2.
- (3)
- t = 3T/4: The excitation voltage applied to the two vertical zones of the piezoelectric ceramics reaches the maximum negative value, the bending displacement of the PUA along the positive direction of the Y axis reaches the maximum and the driving foot leaves the actuator. The driving foot does not contact the mover between t = T/2 and t = 3T/4.
- (4)
- t = T: The excitation voltage applied to the left and right zones of the piezoelectric ceramics reaches the maximum negative value, and the bending displacement of the PUA along the negative direction of the X axis reaches the maximum. The driving foot does not contact the mover between t = 3T/4 and t = T.
2.2. Friction Pair Performance Test
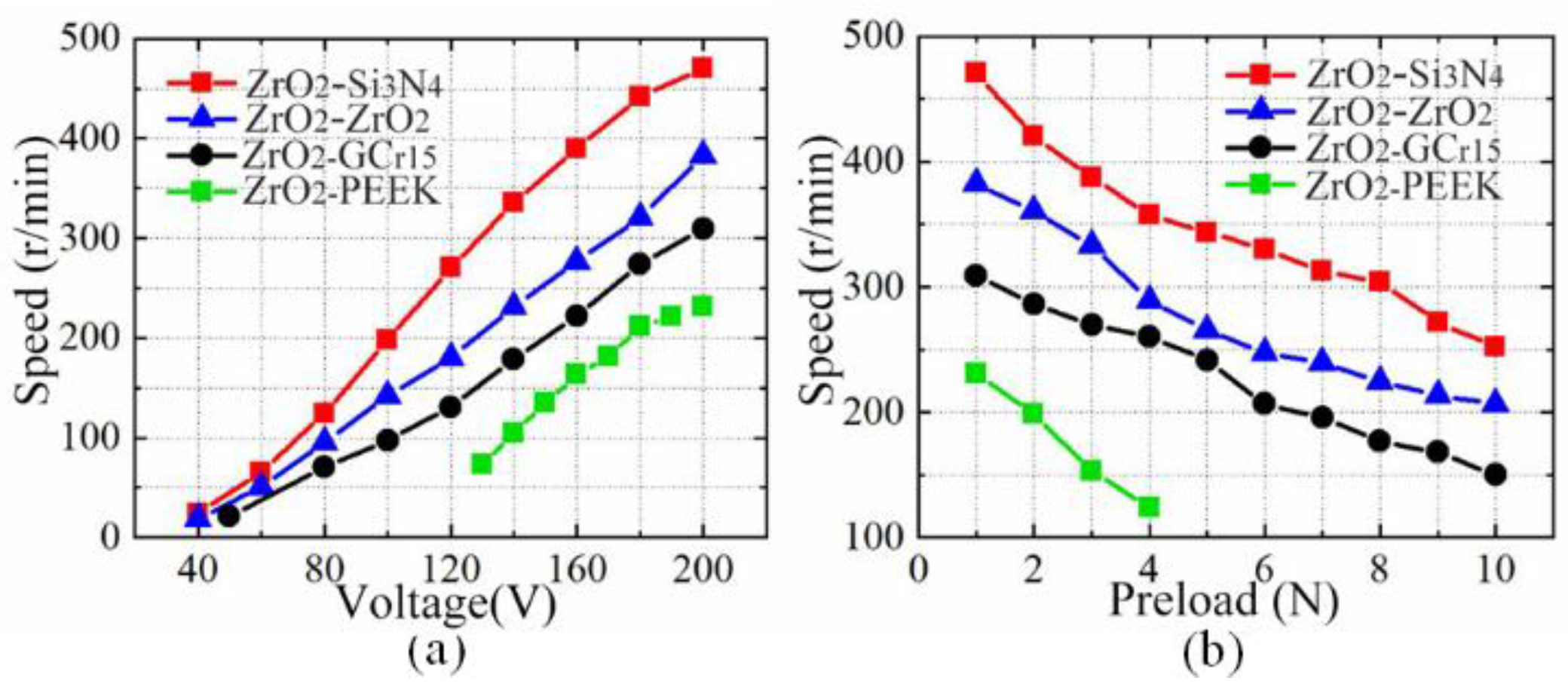
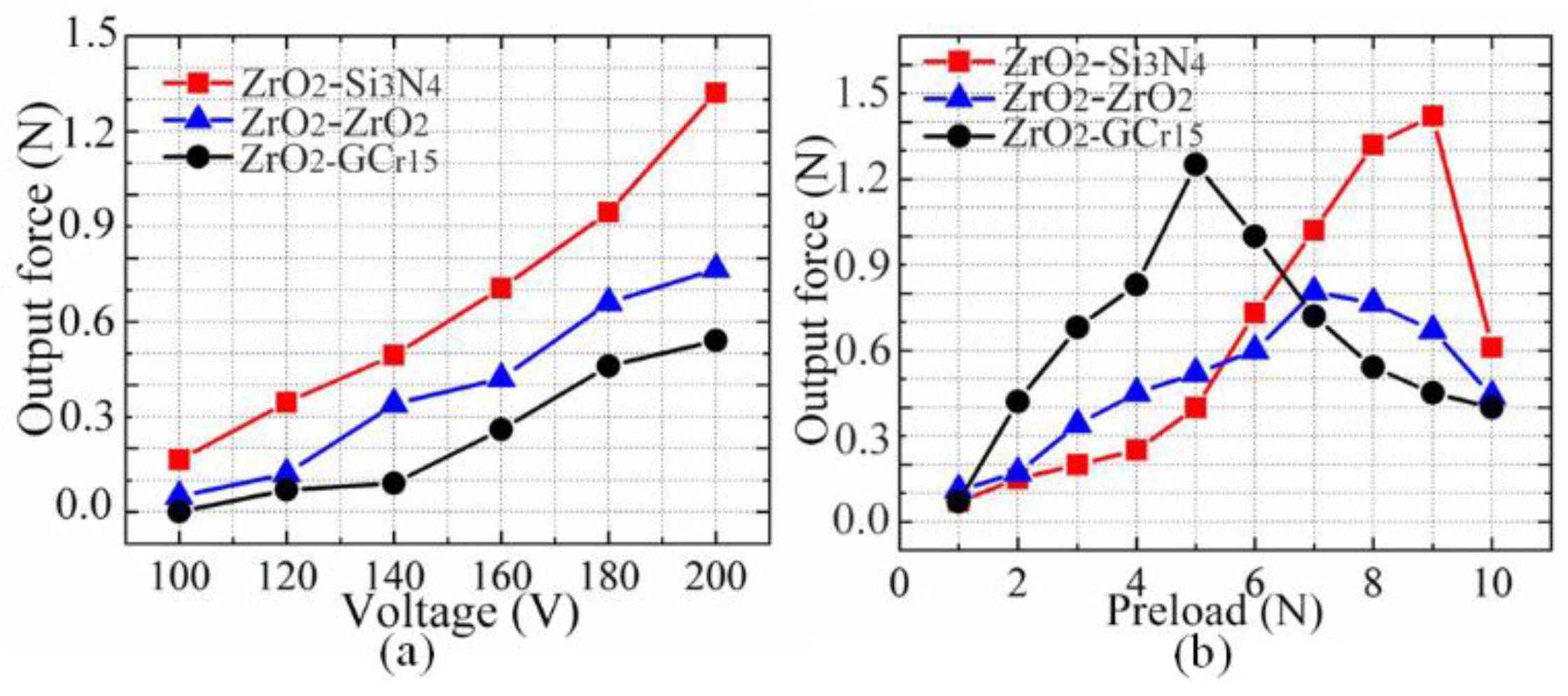
3. Discussion and Friction Material Selection
4. Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Shi, Y.L.; Lou, C.S.; Zhang, J. Investigation on a linear piezoelectric actuator based on stick-slip/scan excitation. Actuators 2021, 10, 39. [Google Scholar] [CrossRef]
- Tang, J.Z.; Wei, J.S.; Wang, Y.M.; Xu, Z.; Huang, H. A novel rotation-structure based stick-slip piezoelectric actuator with high consistency in forward and reverse motions. Actuators 2021, 10, 189. [Google Scholar] [CrossRef]
- Yu, H.P.; Liu, Y.X.; Tian, X.Q.; Zhang, S.J.; Liu, J.K. A precise rotary positioner driven by piezoelectric bimorphs: Design, analysis and experimental evaluation. Sens. Actuators A Phys. 2020, 313, 112197. [Google Scholar] [CrossRef]
- Wang, F.J.; Shi, B.C.; Tian, Y.L.; Huo, Z.C.; Zhao, X.Y.; Zhang, D.W. Design of a novel dual-axis micromanipulator with an asymmetric compliant structure. IEEE/ASME Trans. Mechatron. 2013, 24, 656–665. [Google Scholar] [CrossRef]
- Deng, J.; Liu, Y.X.; Li, J.; Zhang, S.J.; Li, K. Displacement linearity improving method of stepping piezoelectric platform based on leg wagging mechanism. IEEE Trans. Ind. Electron. 2022, 69, 6429–6432. [Google Scholar] [CrossRef]
- Deng, J.; Liu, S.H.; Liu, Y.X.; Wang, L.; Gao, X.; Li, K. A 2-DOF needle insertion device using inertial piezoelectric actuator. IEEE Trans. Ind. Electron. 2022, 69, 3918–3927. [Google Scholar] [CrossRef]
- Gao, X.; Deng, J.; Zhang, S.J.; Li, J.; Liu, Y.X. A compact 2-DOF micro/nano manipulator using single miniature piezoelectric tube actuator. IEEE Trans. Ind. Electron. 2022, 69, 3928–3937. [Google Scholar] [CrossRef]
- Ghenna, S.; Giraud, F.; Giraud-Audine, C.; Amberg, M. Vector control of piezoelectric transducers and ultrasonic actuators. IEEE Trans. Ind. Electron. 2018, 65, 4880–4888. [Google Scholar] [CrossRef]
- Liu, Y.X.; Li, J.; Deng, J.; Zhang, S.J.; Chen, W.S.; Xie, H.; Zhao, J. Arthropod-metamerism-inspired resonant piezoelectric millirobot. Adv. Intell. Syst. 2021, 3, 2100015. [Google Scholar] [CrossRef]
- Du, P.F.; Liu, Y.X.; Chen, W.S.; Zhang, S.J.; Deng, J. Fast and precise control for the vibration amplitude of an ultrasonic transducer based on fuzzy PID control. IEEE Trans. Ultrason. Ferroelect. Freq. Control 2012, 68, 2766–2774. [Google Scholar] [CrossRef]
- Ma, X.F.; Liu, Y.X.; Liu, J.K.; Deng, J. Crabbot: A pole-climbing robot driven by piezoelectric stack. IEEE Trans. Robot. 2021, 38, 765–778. [Google Scholar]
- Cheng, J.F.; Deng, J.; Liu, Y.X.; Zhang, S.J.; Lu, F.; Tian, X.Q.; Chen, W.S. Step consistency active control method for inertial piezoelectric actuator using embedded strain gauges. Rev. Sci. Instrum. 2021, 92, 25005. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Wang, H.R.; Cheng, T.H. Design and performance of a compact stick-slip type piezoelectric actuator based on right triangle flexible stator. Smart Mater. Struct. 2022, 31, 055013. [Google Scholar] [CrossRef]
- Kim, J.H.; Kim, S.H.; Kwak, Y.K. Development and optimization of 3-D bridge-type hinge mechanisms. Sens. Actuators A Phys. 2004, 116, 530–538. [Google Scholar] [CrossRef]
- Li, J.P.; Zhao, H.W.; Qu, X.T.; Qu, H.; Zhou, X.Q.; Fan, Z.Q.; Ma, Z.C.; Fu, H.S. Development of a compact 2-DOF precision piezoelectric positioning platform based on inchworm principle. Sens. Actuators A Phys. 2015, 222, 87–95. [Google Scholar] [CrossRef]
- Wang, Y.; Huang, W. A piezoelectric motor with two projections using two orthogonal flexural vibration modes. Sens. Actuator A Phys. 2016, 250, 170–176. [Google Scholar] [CrossRef]
- Makarem, S.; Delibas, B.; Koc, B. Data-driven tuning of PID controlled piezoelectric ultrasonic motor. Actuators 2021, 10, 148. [Google Scholar] [CrossRef]
- Li, H.; Tian, X.Q.; Shen, Z.H.; Li, K.; Liu, Y. A low-speed linear stage based on vibration trajectory control of a bending hybrid piezoelectric ultrasonic motor. Mech. Syst. Signal Process. 2019, 132, 523–534. [Google Scholar] [CrossRef]
- Liu, Y.X.; Wang, L.; Gu, Z.Z.; Quan, Q.Q.; Deng, J. Development of a two-dimensional linear piezoelectric stepping platform using longitudinal-bending hybrid actuators. IEEE Trans. Ind. Electron. 2019, 66, 3030–3040. [Google Scholar] [CrossRef]
- Liu, Y.X.; Yan, J.P.; Wang, L.; Chen, W.S. A two-DOF ultrasonic motor using a longitudinal-bending hybrid sandwich transducer. IEEE Trans. Ind. Electron. 2019, 66, 3041–3050. [Google Scholar] [CrossRef]
- Wallaschek, J. Contact mechanics of piezoelectric ultrasonic motors. Smart Mater. Struct. 1998, 7, 369–381. [Google Scholar] [CrossRef]
- Gao, J.; Altintas, Y. Development of a three-degree-of-freedom ultrasonic vibration tool holder for milling and drilling. IEEE/ASME Trans. Mechatron. 2012, 24, 1238–1247. [Google Scholar] [CrossRef]
- Qiu, W.; Mizuno, Y.; Nakamura, K. Tribological performance of ceramics in lubricated ultrasonic motors. Wear 2016, 352–353, 188–195. [Google Scholar] [CrossRef]
- Qu, J.J.; Zhang, Y.H.; Tian, X.; Li, J.B. Wear behavior of filled polymers for ultrasonic motor in vacuum environments. Wear 2015, 322, 108–116. [Google Scholar] [CrossRef]
- Gu, D.P.; Duan, C.S.; Fan, B.L.; Chen, S.W.; Yang, Y.L. Tribological properties of hybrid PTFE/Kevlar fabric composite in vacuum. Tribol. Int. 2016, 103, 423–431. [Google Scholar] [CrossRef]
- Liu, X.L.; Qiu, J.H.; Zhao, G. Improved energy conversion efficiency of the ultrasonic motor with surface texture. Ind. Lubr. Tribol. 2018, 70, 1729–1736. [Google Scholar] [CrossRef]
- Song, J.F.; Zhao, G.; Ding, Q.J.; Qiu, J.H. Reciprocating friction and wear of polyimide composites filled with solid lubricants. J. Polym. Eng. 2018, 38, 363–370. [Google Scholar] [CrossRef]
- Sun, F.Y.; Qu, J.J. Tribological properties of Ekonol composites friction material in traveling ware ultrasonic motor. Adv. Mat. Res. 2012, 322, 404–407. [Google Scholar] [CrossRef]
- Zhang, Y.H.; Fu, Y.H.; Hua, X.J.; Quan, L.; Qu, J.J. Characteristics and attenuation mechanism of linear standing-wave piezoelectric motors with ceramics-mated friction couples. Tribol. Int. 2021, 153, 106580. [Google Scholar] [CrossRef]
- Adachi, K.; Kato, K. Formation of smooth wear surfaces on alumina ceramics by embedding and tribo-sintering of fine wear particles. Wear 2000, 245, 84–91. [Google Scholar] [CrossRef]
- Olofsson, J.; Johansson, S.; Jacobson, S. Influence from humidity on the alumina friction drive system of an ultrasonic motor. Tribol. Int. 2009, 42, 1467–1477. [Google Scholar] [CrossRef]
- Gourgiotis, P.A.; Zisis, T.; Giannakopoulos, A.E.; Georgiadis, H.G. The hertz contact problem in couple-stress elasticity. Int. J. Solids Struct. 2019, 168, 228–237. [Google Scholar] [CrossRef]
- Izuhara, S.; Mashimo, T. Design and evaluation of a micro linear ultrasonic motor. Sens. Actuator A Phys. 2018, 278, 60–66. [Google Scholar] [CrossRef]
- Smithmaitrie, P.; Suybangdum, P.; Laoratanakul, P.; Muensit, N. Design and performance testing of an ultrasonic linear motor with dual piezoelectric actuators. IEEE Trans. Ultrason. Ferroelect. Freq. Control 2012, 59, 1033–1042. [Google Scholar] [CrossRef] [PubMed]
- Shi, Y.L.; Zhao, C.S. Simple new ultrasonic piezoelectric actuator for precision linear positioning. J. Electroceram. 2012, 28, 233–239. [Google Scholar] [CrossRef]

| Material Parameters | Si3N4 | ZrO2 | GCr15 | PEEK | 2A12 |
|---|---|---|---|---|---|
| Density ρ (g/cm3) | 3.2 | 6 | 7.8 | 1.32 | 2.81 |
| Elastic modulus E (GPa) | 320 | 210 | 208 | 3.8 | 72 |
| Poisson’s ratio | 0.26 | 0.3 | 0.3 | 0.4 | 0.33 |
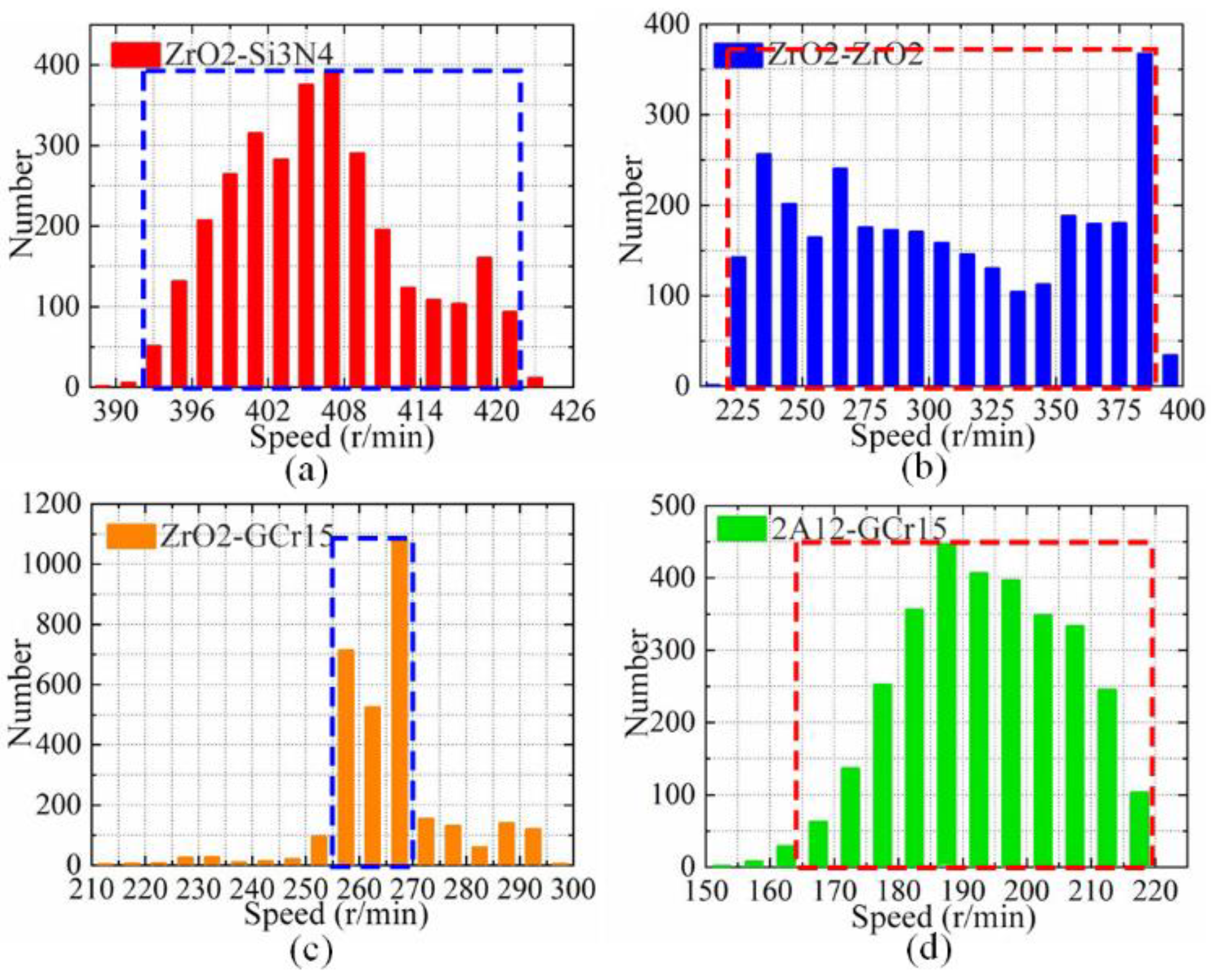
| Friction Pairs | Running Distance (m) | Initial Velocity (r/min) | Peak Velocity (r/min) | End Speed (r/min) |
|---|---|---|---|---|
| ZrO2-Si3N4 | 50 | 410 | 420 | 395 |
| ZrO2-ZrO2 | 40 | 355 | 390 | 228 |
| ZrO2-GCr15 | 25 | 285 | 295 | 258 |
| 2A12-Cr15 | 130 | 198 | 218 | 190 |
| Friction Pairs | Vm | SD | CSS | CV | S | K |
|---|---|---|---|---|---|---|
| ZrO2-Si3N4 | 406.06 | 7.01 | 1.53 × 105 | 0.01726 | 0.36572 | −0.50952 |
| ZrO2-ZrO2 | 307.39 | 53.29 | 88.5 × 105 | 0.17336 | 0.08296 | −1.36762 |
| ZrO2-GCr15 | 265.50 | 11.08 | 3.83 × 105 | 0.04173 | −0.03711 | 2.60159 |
| Friction Pair Material | ZrO2-Si3N4 | ZrO2-ZrO2 | ZrO2-GCr15 | ZrO2-PEEK |
|---|---|---|---|---|
| Equivalent elastic modulus E (GPa) | 126.8 | 105 | 104.5 | 3.73 |
| Friction coefficient | 0.13 | 0.11 | 0.13 | 0.14 |
| Parameters | This Work | The Motors in [33] | The Motors in [34] | The Motors in [35] |
|---|---|---|---|---|
| Total size (mm) | 30 × 30 × 34.2 | 2.6 × 2.6 × 2.2 | 85 × 6 × 1 | 55 × 26 × 14 |
| Voltage (Vp-p) | 100 | 150 | 60 | 400 |
| Speed (mm/s) | 179.8 | 140 | 176.2 | 159 |
| Load mass (kg) | 23 | / | / | / |
| Resolution (µm) | 0.085 | / | / | / |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deng, J.; Cheng, J.; Guan, Y.; Li, H.; Lu, F.; Chen, W. Research on the Influence of Friction Pairs on the Output Characteristics of the Piezoelectric Ultrasonic Actuator. Actuators 2022, 11, 212. https://doi.org/10.3390/act11080212
Deng J, Cheng J, Guan Y, Li H, Lu F, Chen W. Research on the Influence of Friction Pairs on the Output Characteristics of the Piezoelectric Ultrasonic Actuator. Actuators. 2022; 11(8):212. https://doi.org/10.3390/act11080212
Chicago/Turabian StyleDeng, Jie, Jianfei Cheng, Yuntian Guan, He Li, Fei Lu, and Weishan Chen. 2022. "Research on the Influence of Friction Pairs on the Output Characteristics of the Piezoelectric Ultrasonic Actuator" Actuators 11, no. 8: 212. https://doi.org/10.3390/act11080212
APA StyleDeng, J., Cheng, J., Guan, Y., Li, H., Lu, F., & Chen, W. (2022). Research on the Influence of Friction Pairs on the Output Characteristics of the Piezoelectric Ultrasonic Actuator. Actuators, 11(8), 212. https://doi.org/10.3390/act11080212






