Dual Synthetic Jets Actuator and Its Applications—Part II: Novel Fluidic Thrust-Vectoring Method Based on Dual Synthetic Jets Actuator
Abstract
1. Introduction
2. Materials and Methods
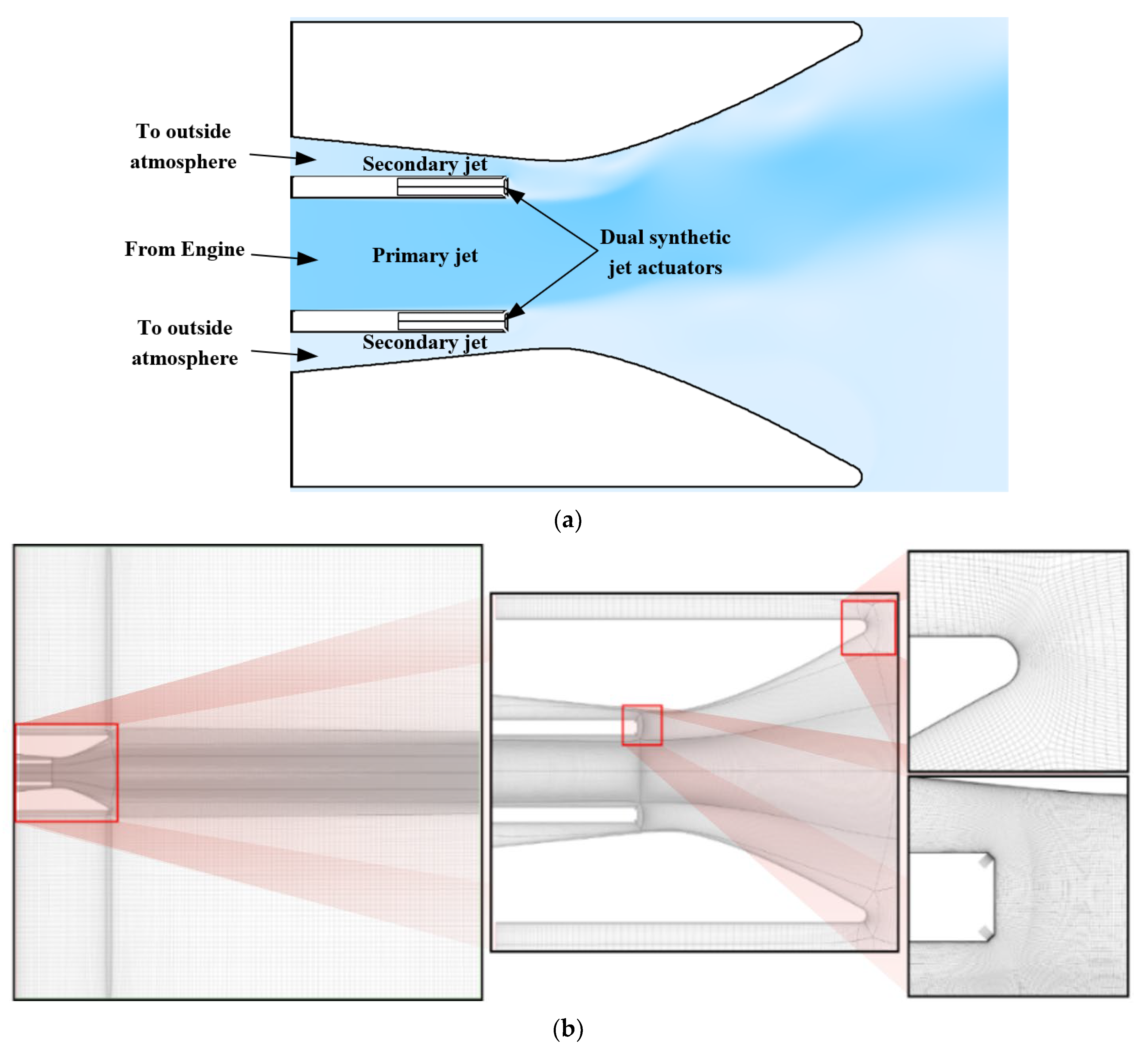
2.1. Method and Configuration
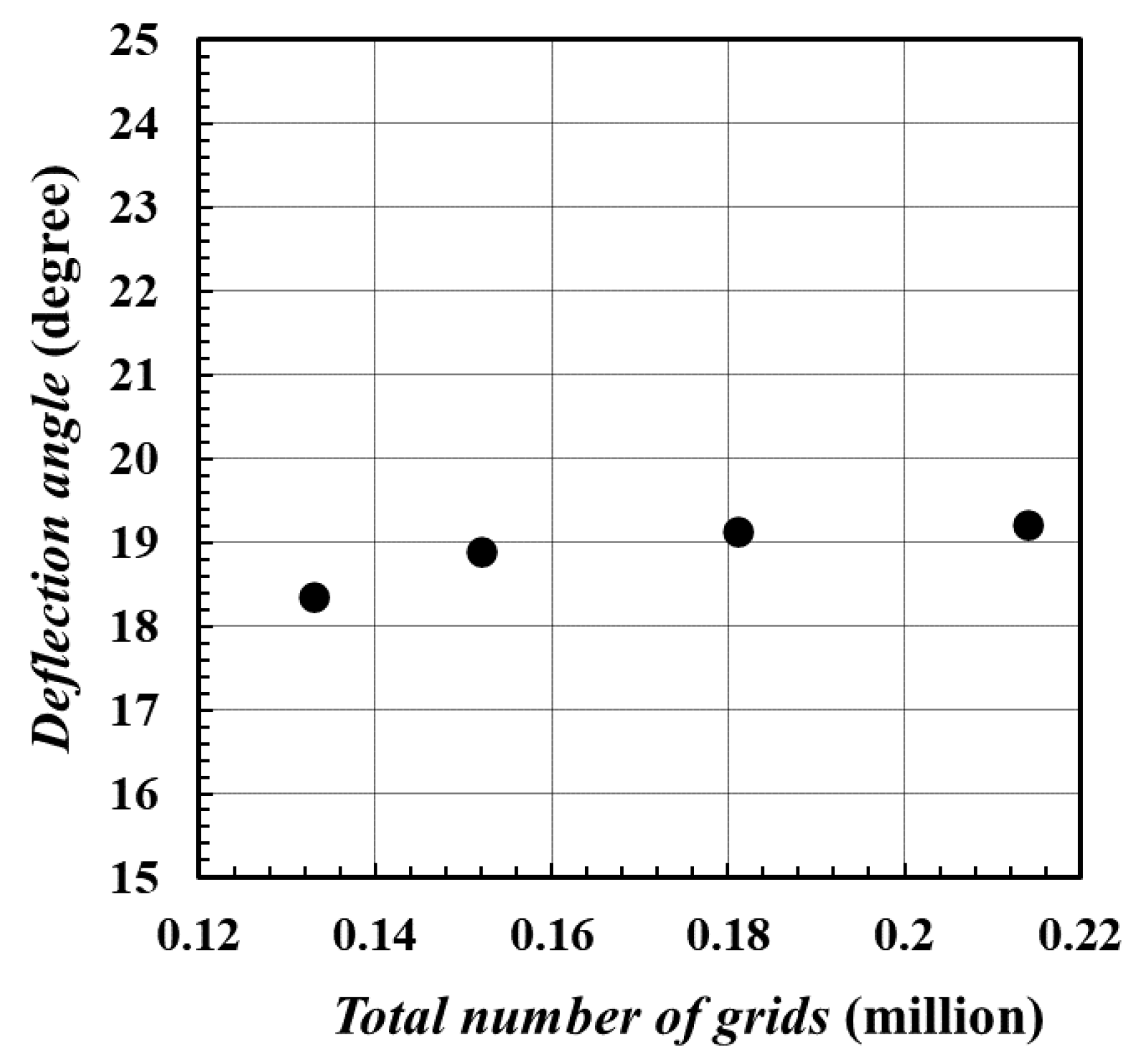
2.2. Simulation Setup
2.3. Relevant Definitions
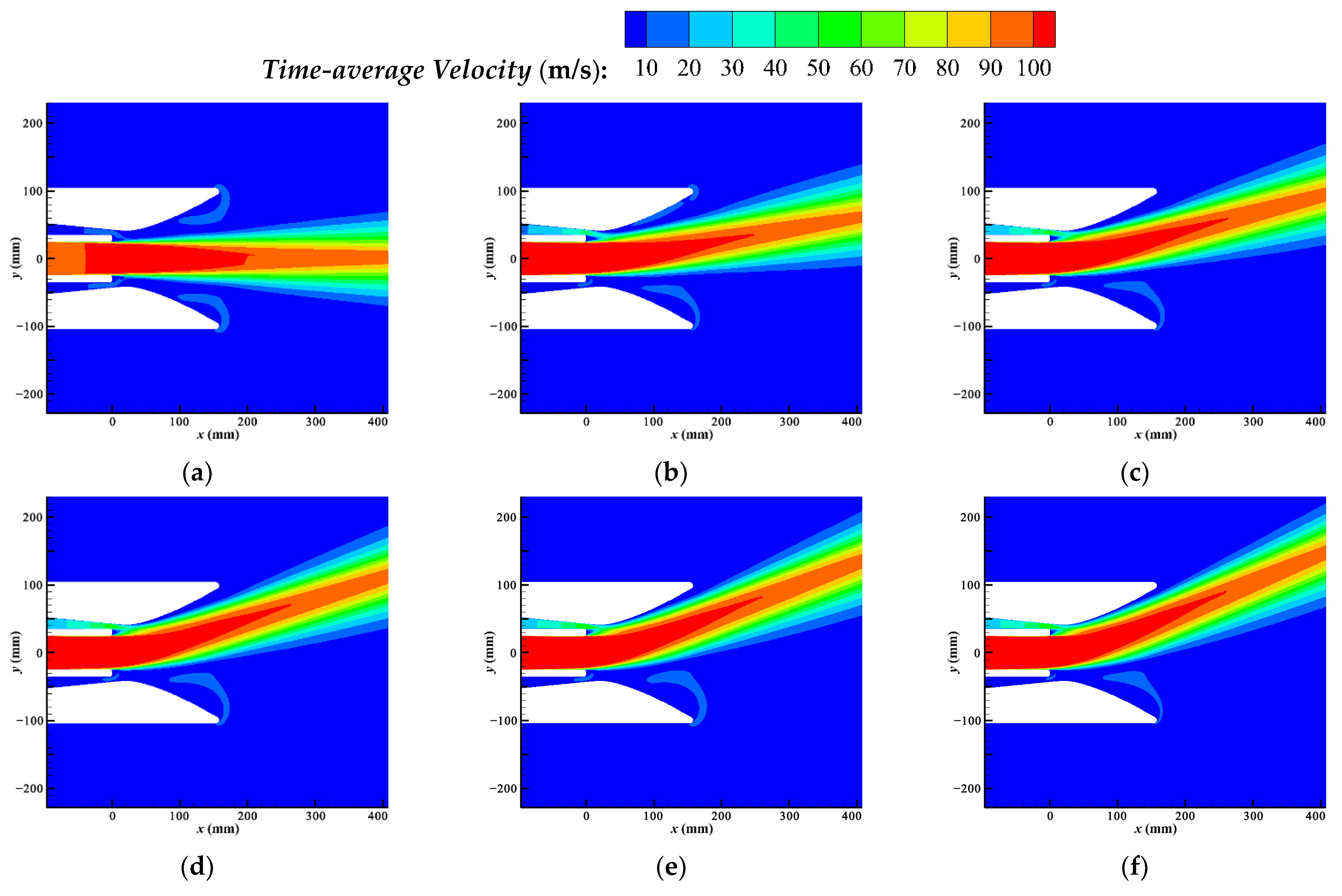
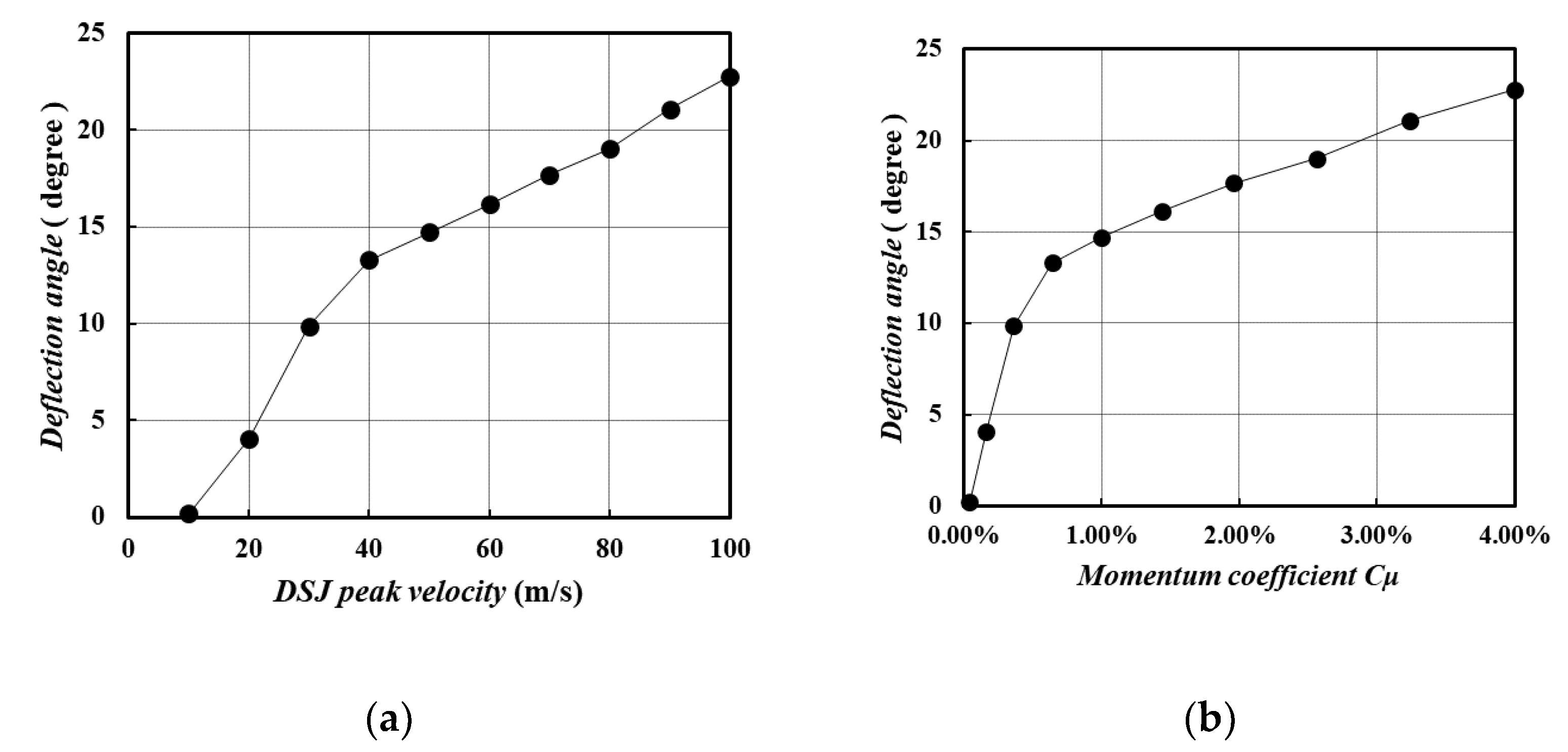
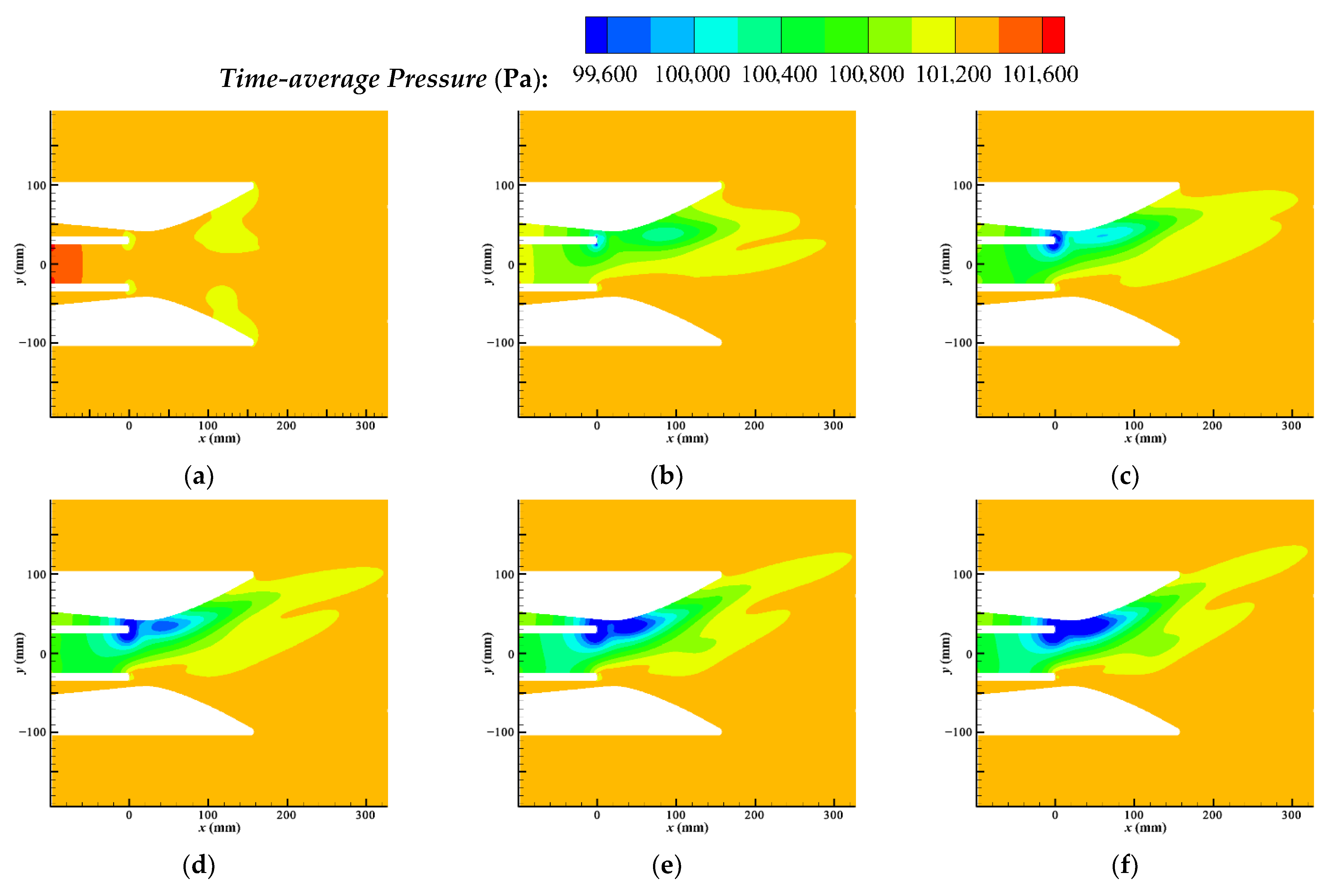
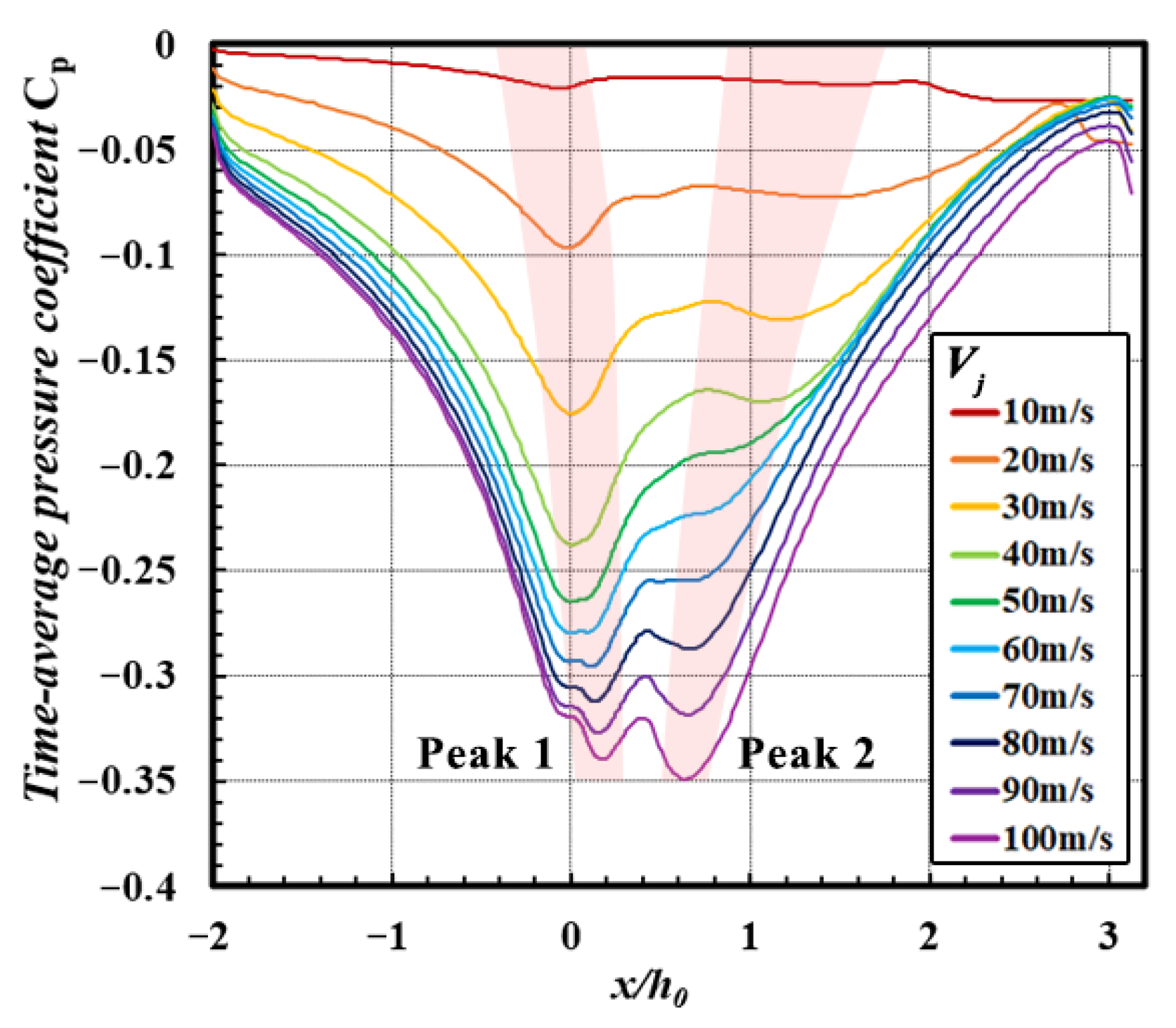
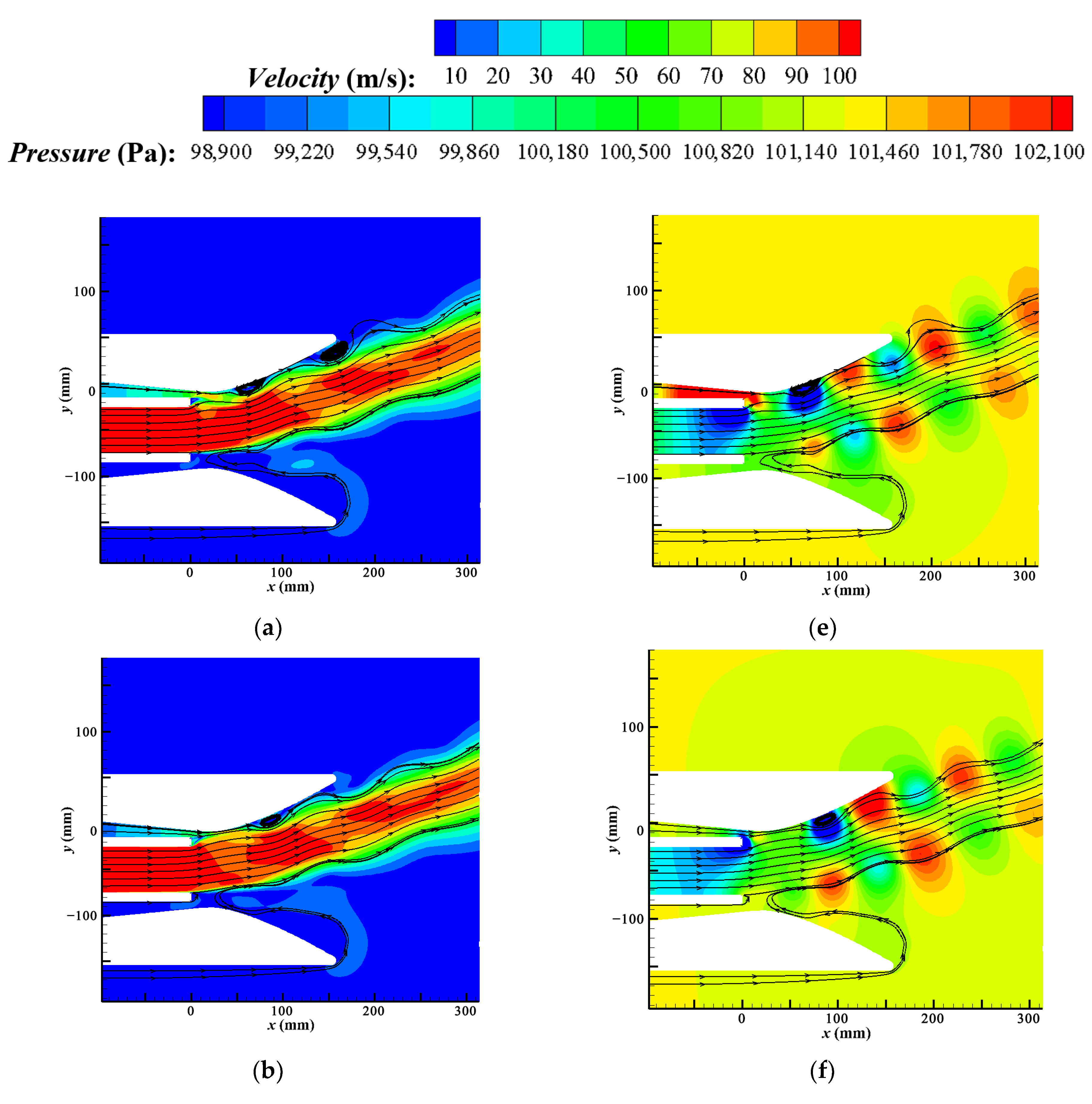
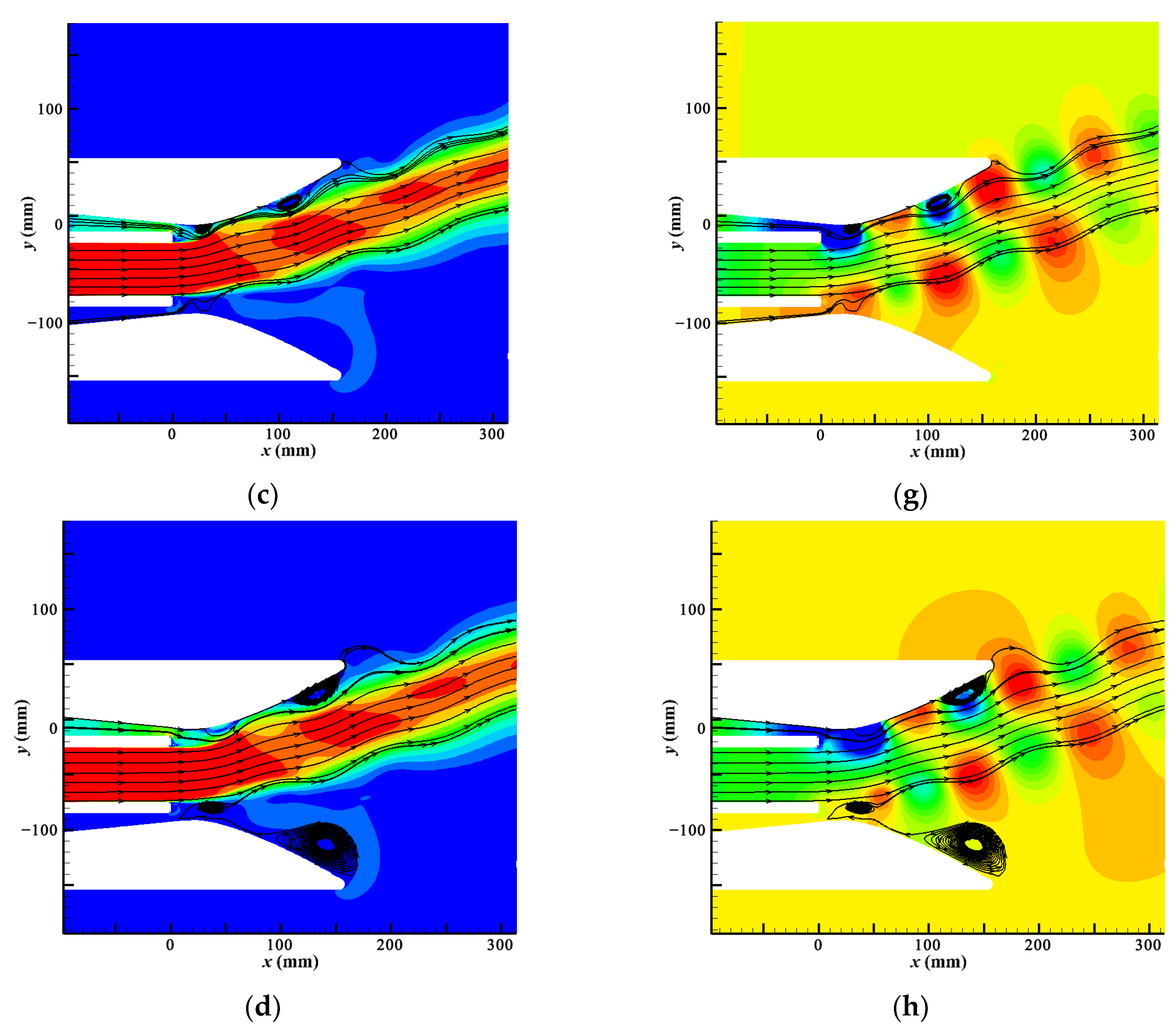
3. Results
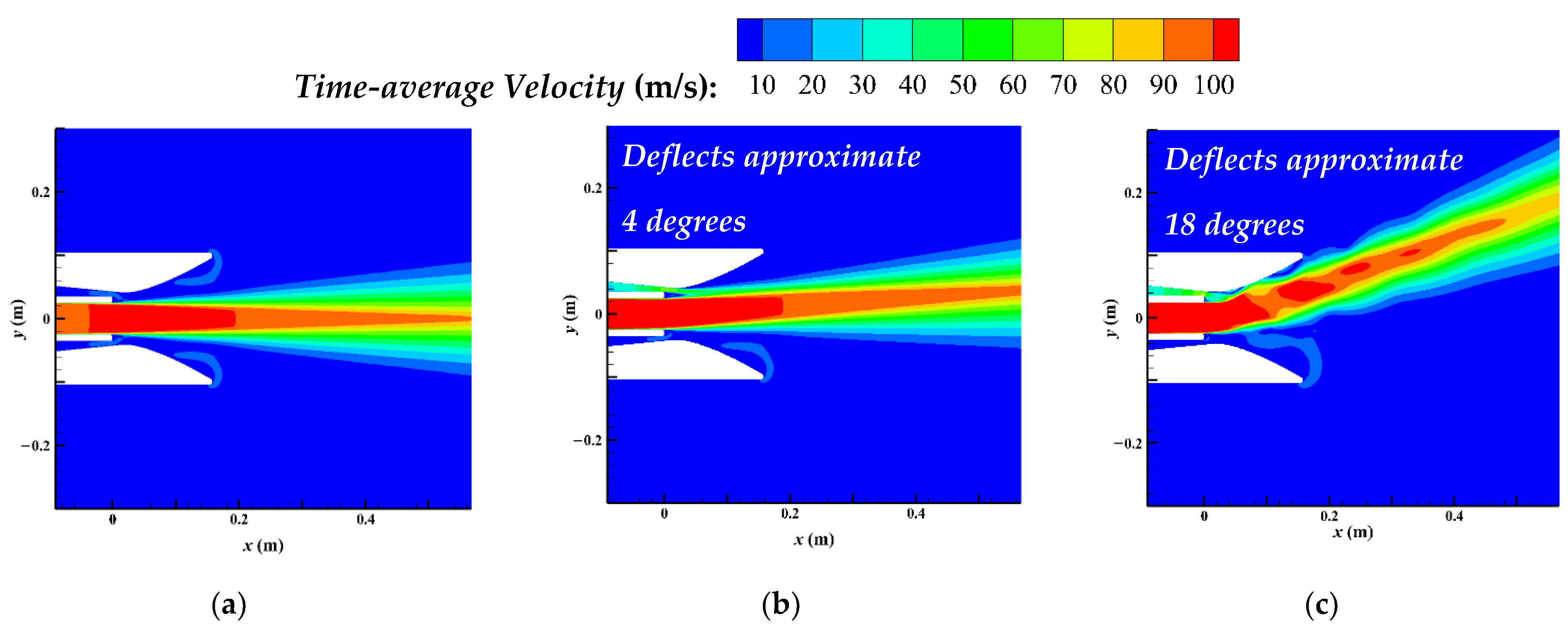
3.1. Control Characteristics of Thrust Vectoring
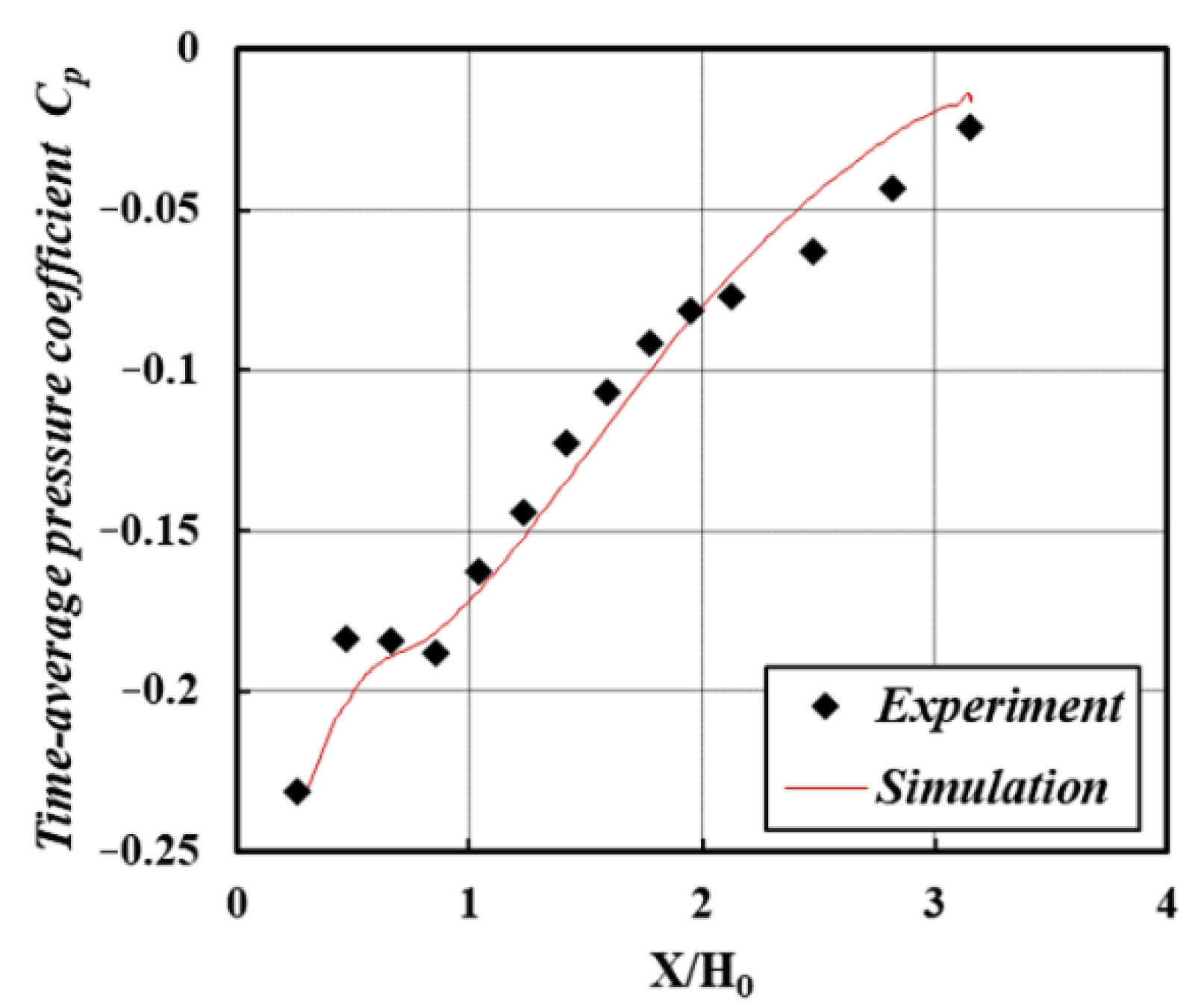
3.2. Pressure Characteristics of Flow Field
3.3. Periodic Evolution of Flow Field
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Deng, R.; Kim, H.D. A study on the thrust vector control using a bypass flow passage. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2015, 229, 1722–1729. [Google Scholar] [CrossRef]
- Miller, D.N.; Yagle, P.J.; Hamstra, J.W. Fluidic throat skewing for thrust vectoring in fixed-geometry nozzles. In Proceedings of the 37th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 11–14 January 1999; p. 365. [Google Scholar]
- Deere, K.A.; Berrier, B.L.; Flamm, J.D. A Computational Study of a Dual Throat Fluidic Thrust Vectoring Nozzle Concept. In Proceedings of the 41st AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Tucson, Arizona, 10–13 July 2005; p. 3502. [Google Scholar]
- Flamm, J.D. Experimental study of a nozzle using fluidic counterflow for thrust vectoring. In Proceedings of the 34th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, Cleveland, OH, USA, 13–15 July 1998; p. 3255. [Google Scholar]
- Mason, M.S.; Crowther, W.J. Fluidic thrust vectoring for low observable air vehicles. In Proceedings of the 2nd AIAA Flow Control Conference, Portland, OR, USA, 28 June–1 July 2004; p. 2210. [Google Scholar]
- Heo, J.Y.; Sung, H.G. Fluidic thrust vector control of supersonic jet using coflow injection. J. Propuls. Power 2012, 28, 858–861. [Google Scholar]
- Warsop, C.; Crowther, W.J.; Shearwood, T. NATO AVT-239: Flight demonstration of fluidic flight controls on the MAGMA subscale demonstrator aircraft. In Proceedings of the AIAA Scitech 2019 Forum, San Diego, CA, USA, 7–11 January 2019; p. 0282. [Google Scholar]
- Wen, X.; Zhou, K.W.; Liu, P.C.; Liu, Y.Z. Schlieren visualization of coflow fluidic thrust vectoring using sweeping jets. AIAA J. 2022, 60, 435–444. [Google Scholar] [CrossRef]
- Gu, R.; Xu, J.; Guo, S. Experimental and Numerical Investigations of a Bypass Dual Throat Nozzle. ASME J. Eng. Gas Turbines Power 2014, 136, 084501. [Google Scholar] [CrossRef]
- Xiao, Z.Y.; Gu, Y.S.; Jiang, X.; Chen, Z.B. A new fluidic thrust vectoring technique based on ejecting mixing effects. Acta Aeronaut. Astronaut. Sin. 2012, 33, 1967–1974. (In Chinese) [Google Scholar]
- Cao, Y.F.; Gu, Y.S.; Cheng, K.M.; Xiao, Z.Y.; Chen, Z.B.; He, K.F. Proportional control of jet deflection with passive secondary flow. Acta Aeronaut. Astronaut. Sin. 2015, 36, 757–763. (In Chinese) [Google Scholar]
- Cao, Y.F.; Gu, Y.S.; Han, J.X. Development and flight testing of a fluidic thrust vectoring demonstrator. Acta Aerodyn. Sin. 2019, 37, 593–599. (In Chinese) [Google Scholar]
- Gong, D.S.; Gu, Y.S.; Zhou, Y.H.; Shi, N.X. Control law of passive fluid thrust vector nozzle based on thermal jet of micro turbojet engine. Acta Aeronaut. Astronaut. Sin. 2020, 41, 123609. (In Chinese) [Google Scholar]
- Wen, X.; Tang, H.; Duan, F. Interaction of in-line twin synthetic jets with a separated flow. Phys. Fluids 2016, 28, 043602. [Google Scholar] [CrossRef]
- Wang, C.L.; Duan, F.; Tang, H. Active control of two-dimensional vortex-induced vibration of a circular cylinder using a pair of synthetic jets. In Symposium on Fluid-Structure-Sound Interactions and Control; Springer: Singapore, 2017. [Google Scholar]
- Luo, Z.B.; Zhao, Z.J.; Liu, J.F.; Deng, X.; Zheng, M.; Yang, H.; Chen, Q.; Li, S. Novel roll effector based on zero-mass-flux dual synthetic jets and its flight test. Chin. J. Aeronaut. 2022, 35, 1–5. [Google Scholar] [CrossRef]
- Smith, L.B.; Glezer, A. Vectoring and small-scale motions effected in free shear flows using synthetic jet actuators. In Proceedings of the 35th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 6–9 January 1997; p. 213. [Google Scholar]
- Smith, L.B.; Glezer, A. Jet vectoring using synthetic jets. J. Fluid Mech. 2002, 458, 1–34. [Google Scholar] [CrossRef]
- Pack, L.G.; Seifert, A. Periodic excitation for jet vectoring and enhanced spreading. J. Aircr. 2001, 38, 486–495. [Google Scholar] [CrossRef]
- Chiekh, M.B.; Jean-Christophe, B.; Miche, S. Synthetic jet control for flows in a diffuser: Vectoring, spreading and mixing enhancement. J. Turbul. 2003, 4, 032. [Google Scholar] [CrossRef]
- Luo, Z.B.; Xia, Z.X.; Liu, B. New generation of synthetic jet actuators. AIAA J. 2006, 44, 2418–2420. [Google Scholar] [CrossRef]
- Luo, Z.B.; Xia, Z.X.; Xie, Y.G. Jet vectoring control using a novel synthetic jet actuator. Chin. J. Aeronaut. 2007, 20, 193–201. [Google Scholar] [CrossRef][Green Version]
- Kim, J.Y.; Ghajar, A.F.; Tang, C.; Foutch, G.L. Comparison of near-wall treatment methods for high Reynolds number backward-facing step flow. Int. J. Comput. Fluid Dyn. 2005, 19, 493–500. [Google Scholar] [CrossRef]
- Luo, Z.B. Principle of Synthetic Jet and Dual Synthetic Jets, and their Applications in Jet Vectoring and Micro-Pump. Ph.D. Thesis, National University of Defense Technology, Changsha, China, 2006. (In Chinese). [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, J.-F.; Luo, Z.-B.; Deng, X.; Zhao, Z.-J.; Li, S.-Q.; Liu, Q.; Zhu, Y.-X. Dual Synthetic Jets Actuator and Its Applications—Part II: Novel Fluidic Thrust-Vectoring Method Based on Dual Synthetic Jets Actuator. Actuators 2022, 11, 209. https://doi.org/10.3390/act11080209
Liu J-F, Luo Z-B, Deng X, Zhao Z-J, Li S-Q, Liu Q, Zhu Y-X. Dual Synthetic Jets Actuator and Its Applications—Part II: Novel Fluidic Thrust-Vectoring Method Based on Dual Synthetic Jets Actuator. Actuators. 2022; 11(8):209. https://doi.org/10.3390/act11080209
Chicago/Turabian StyleLiu, Jie-Fu, Zhen-Bing Luo, Xiong Deng, Zhi-Jie Zhao, Shi-Qing Li, Qiang Liu, and Yin-Xin Zhu. 2022. "Dual Synthetic Jets Actuator and Its Applications—Part II: Novel Fluidic Thrust-Vectoring Method Based on Dual Synthetic Jets Actuator" Actuators 11, no. 8: 209. https://doi.org/10.3390/act11080209
APA StyleLiu, J.-F., Luo, Z.-B., Deng, X., Zhao, Z.-J., Li, S.-Q., Liu, Q., & Zhu, Y.-X. (2022). Dual Synthetic Jets Actuator and Its Applications—Part II: Novel Fluidic Thrust-Vectoring Method Based on Dual Synthetic Jets Actuator. Actuators, 11(8), 209. https://doi.org/10.3390/act11080209






