Pressure Fluctuation Characteristics Analysis of Centrifugal Pump as Turbine in Its Start-Up Process
Abstract
:1. Introduction
2. Research Methods
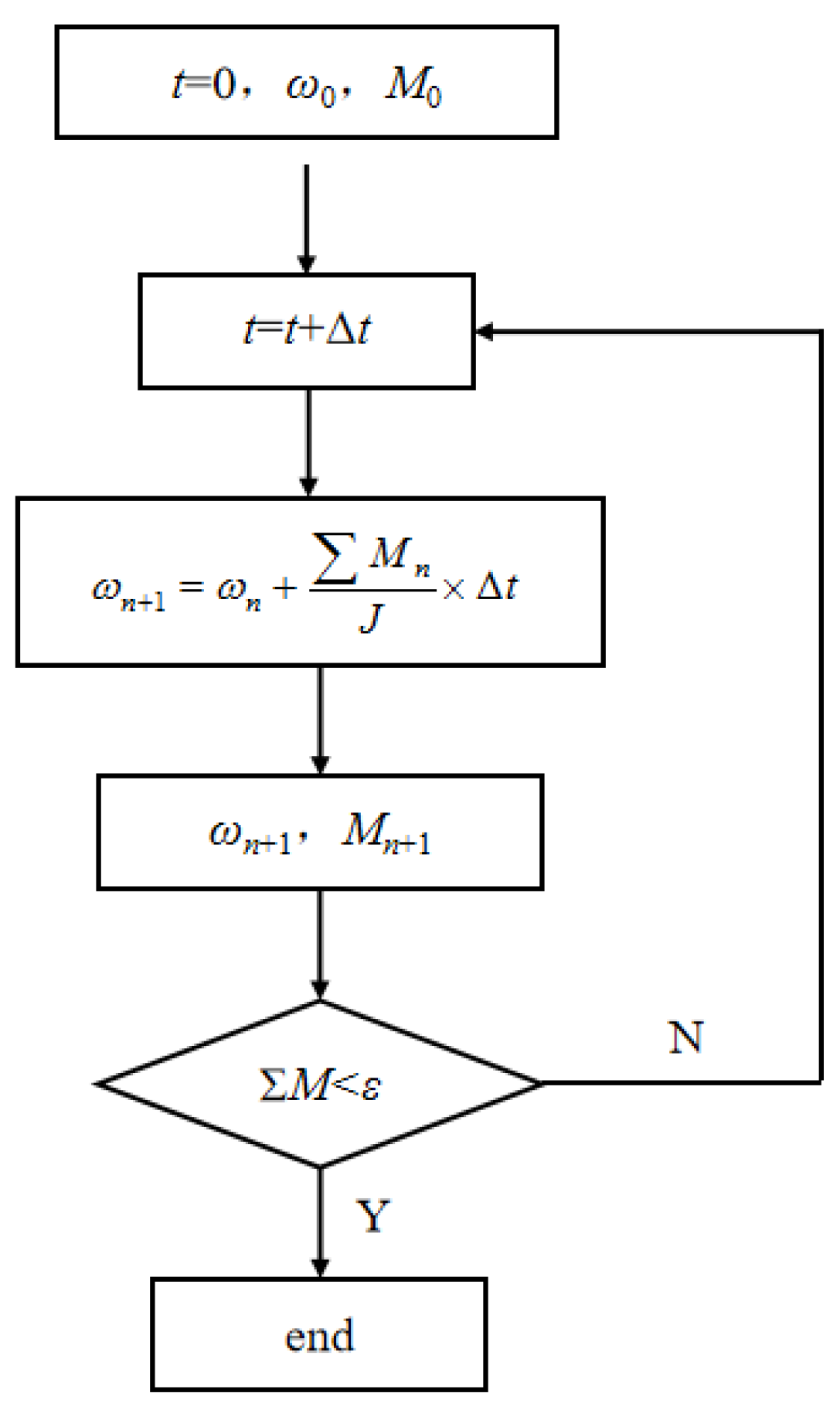
2.1. Rotating Speed Control Equation
2.2. Calculation Method
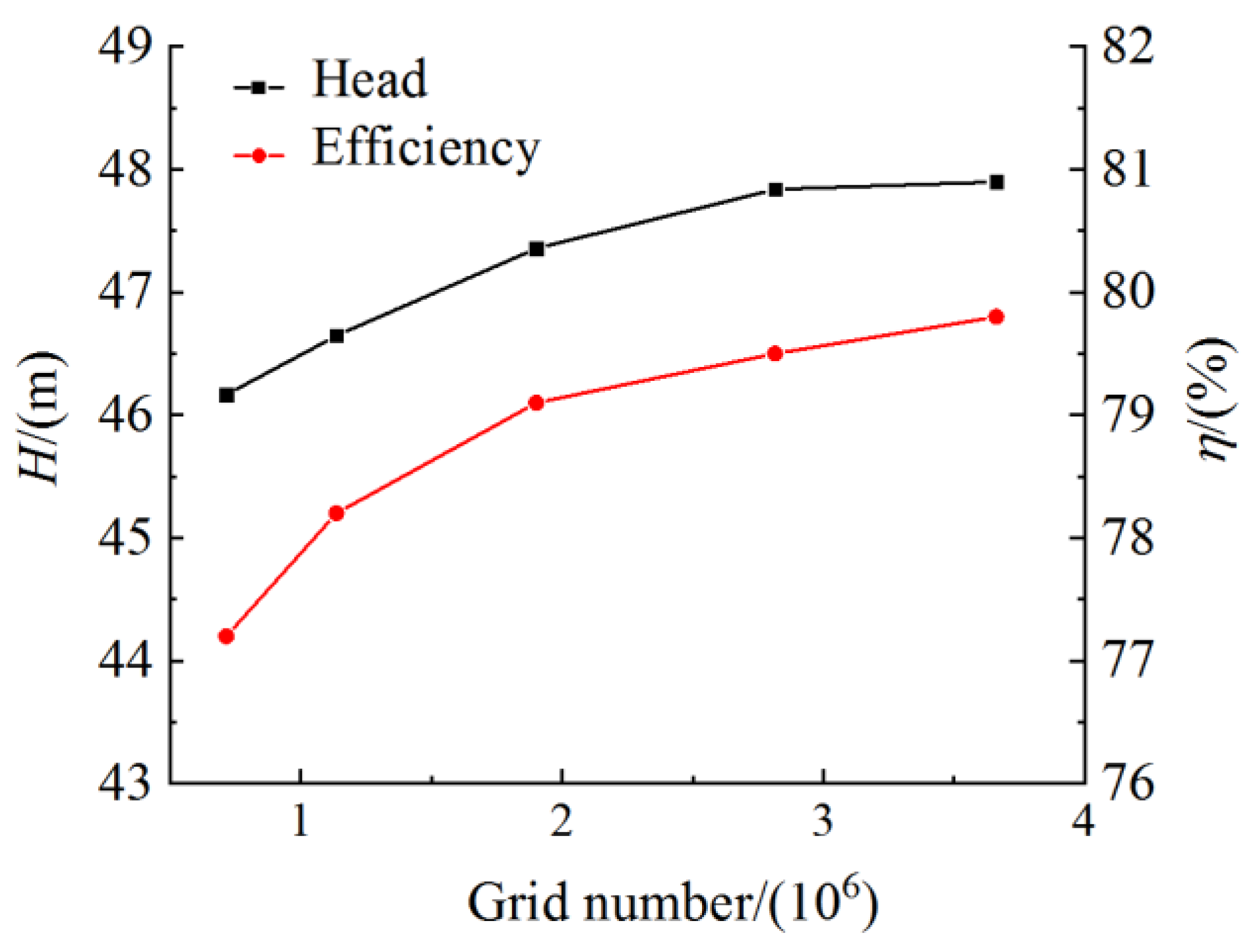
3. Numerical Simulation and Verification
3.1. Computational Model
3.2. Boundary Condition
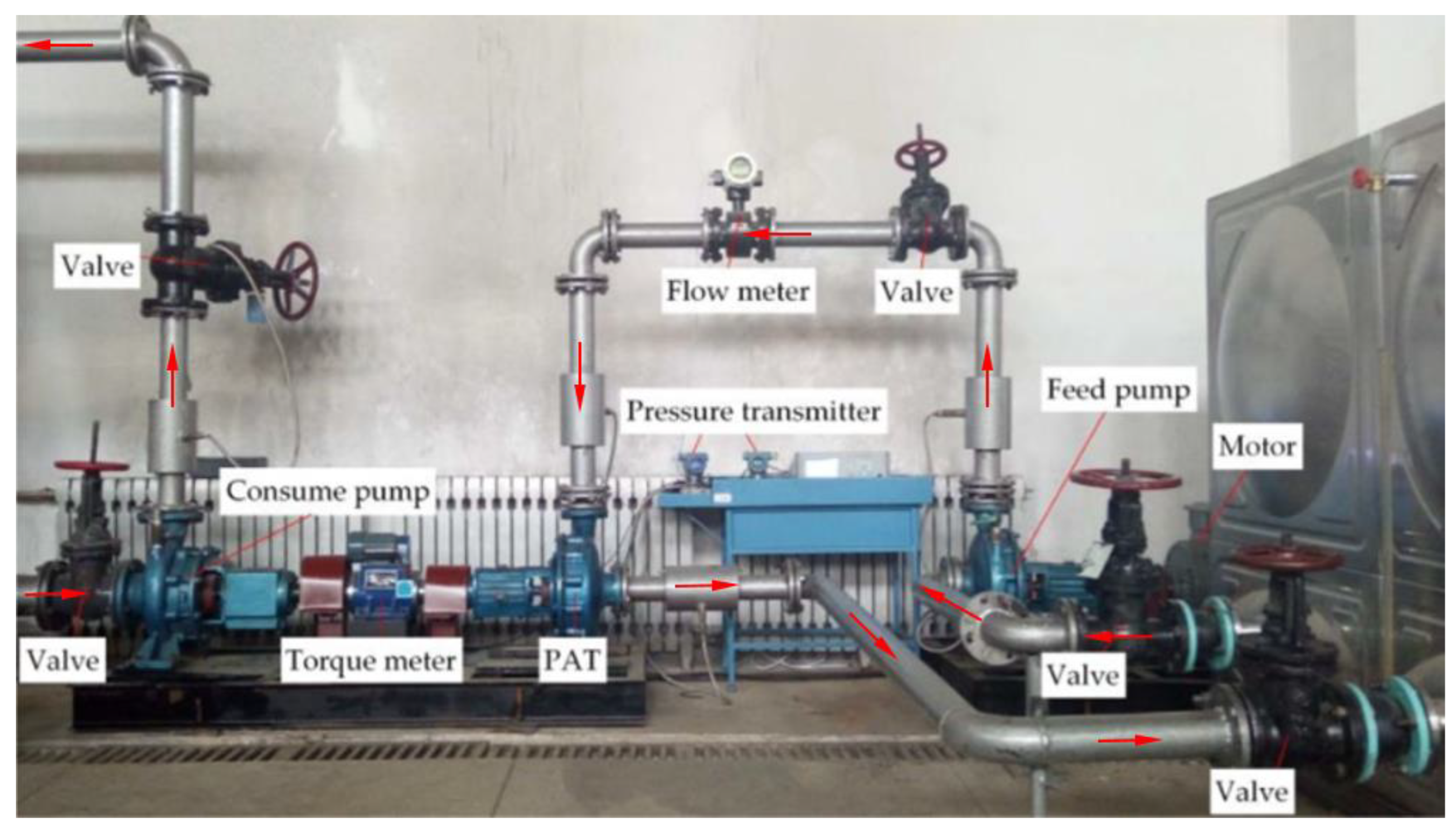
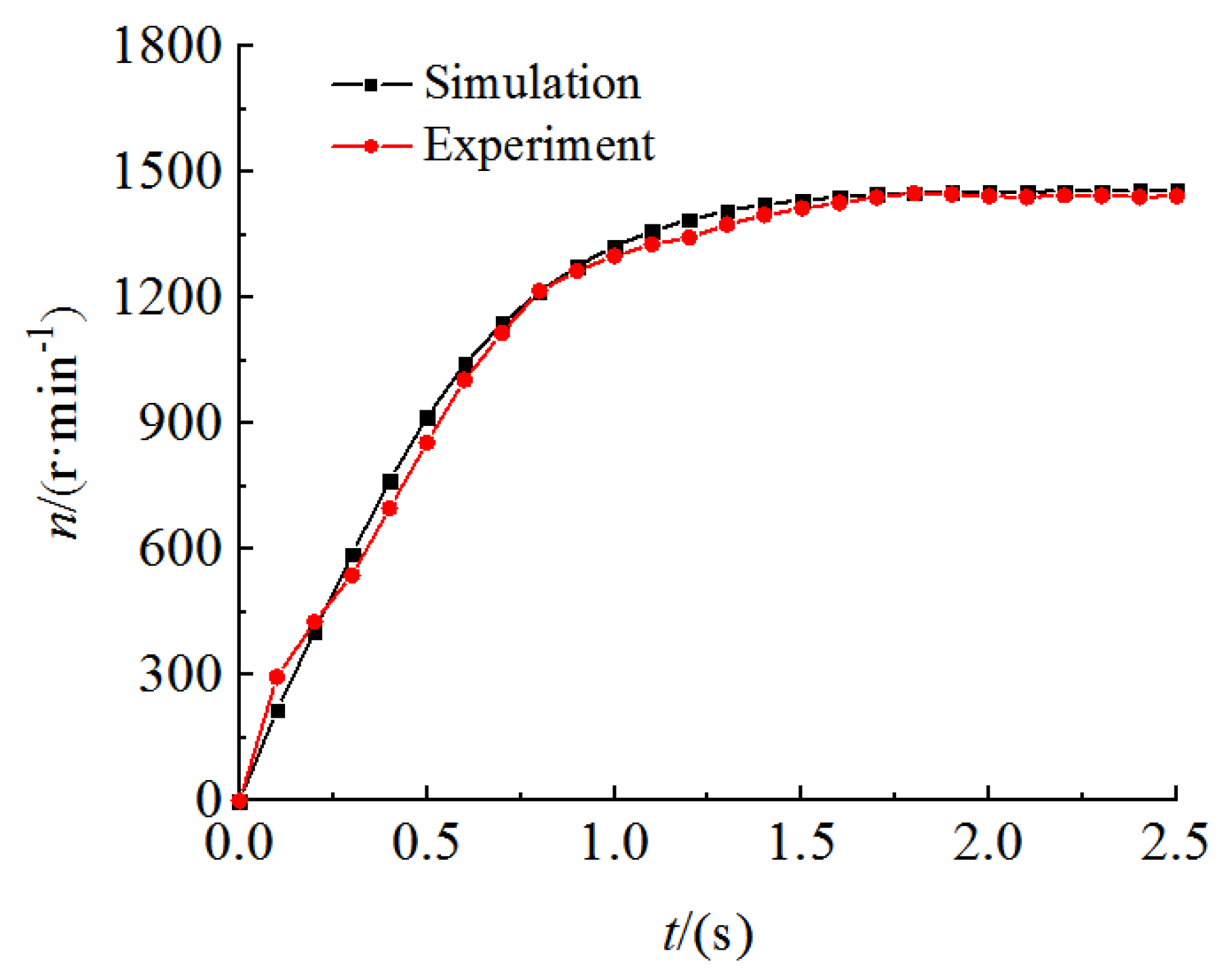
3.3. Experimental Verification
4. Results and Analysis
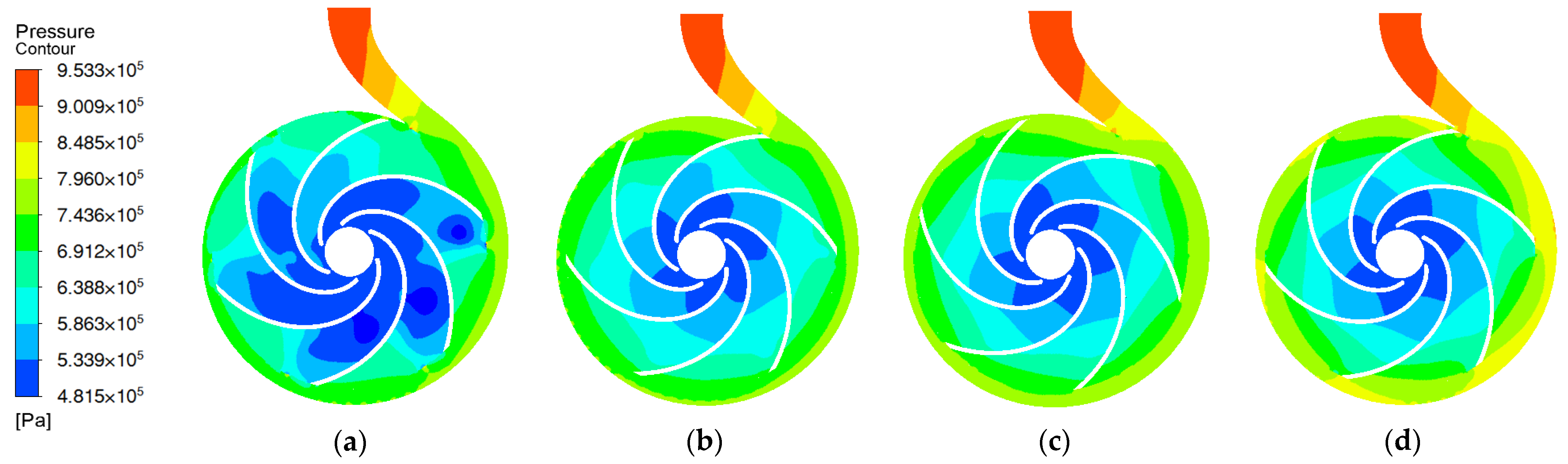
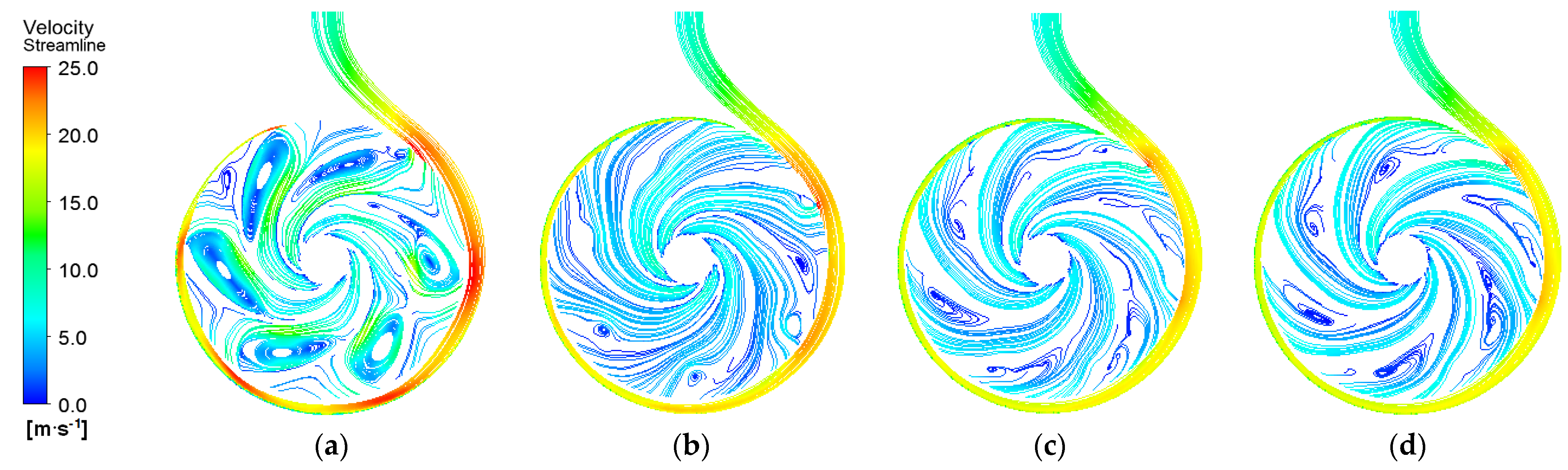
4.1. Evolution Analysis of Internal Flow Field
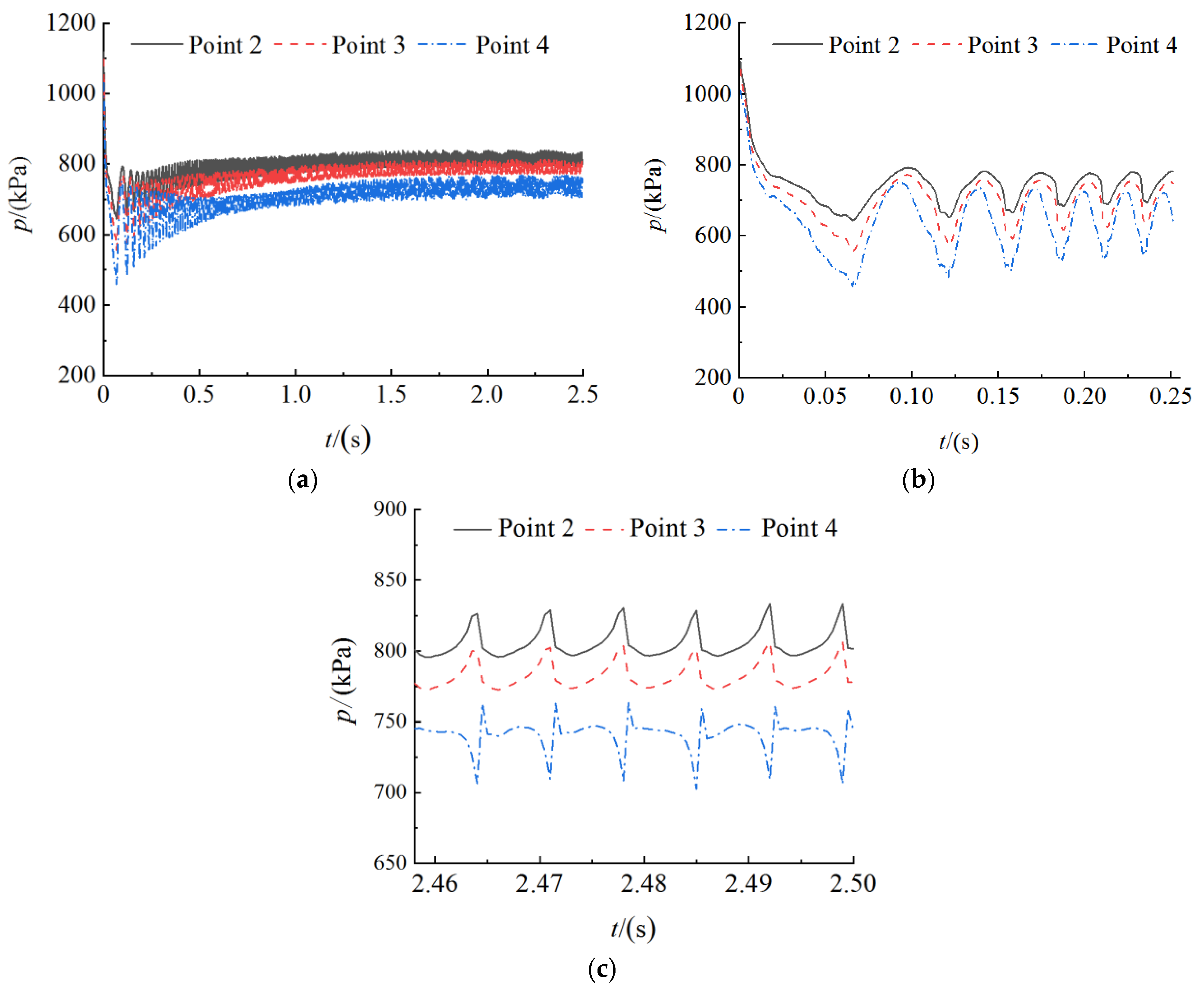
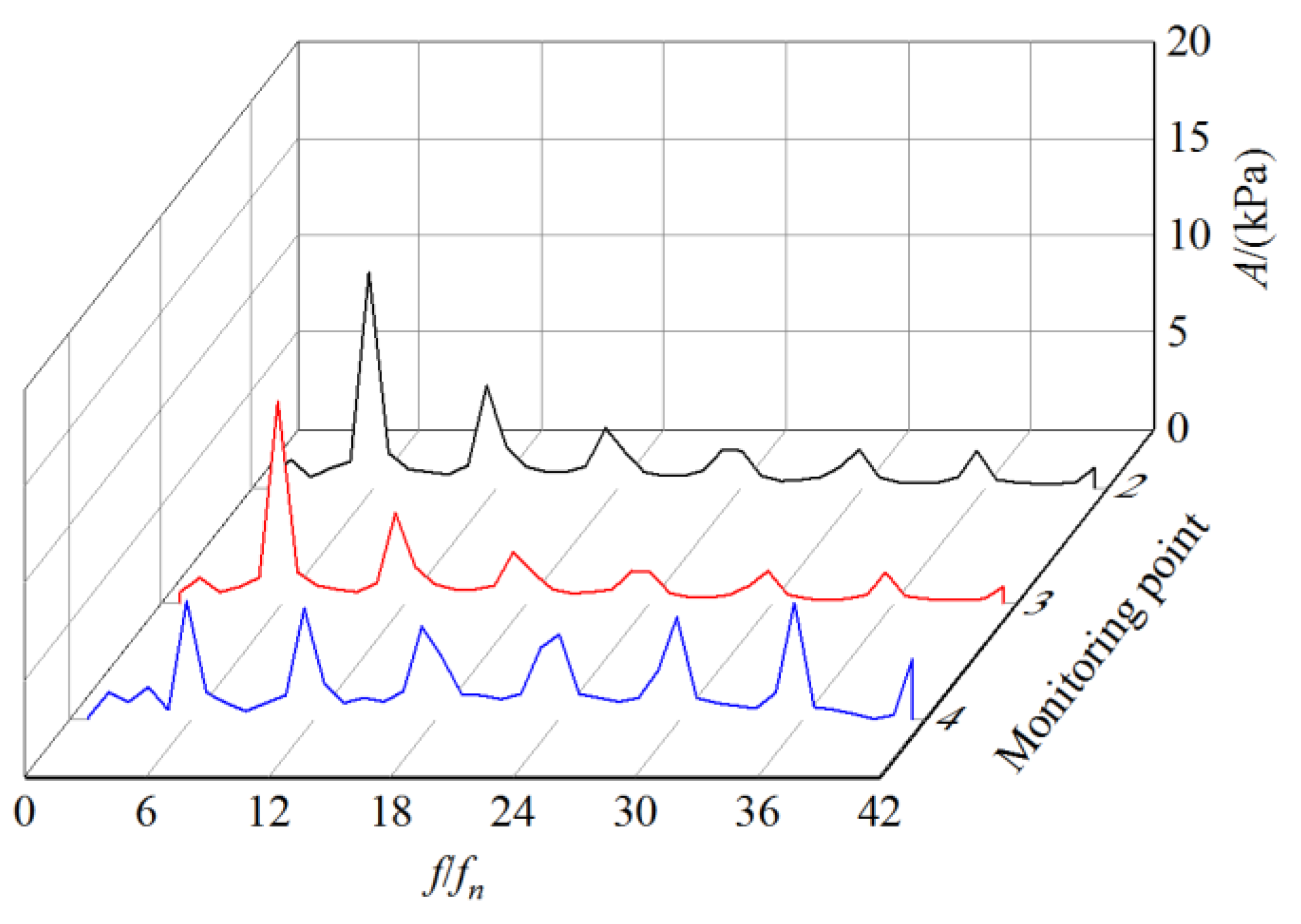
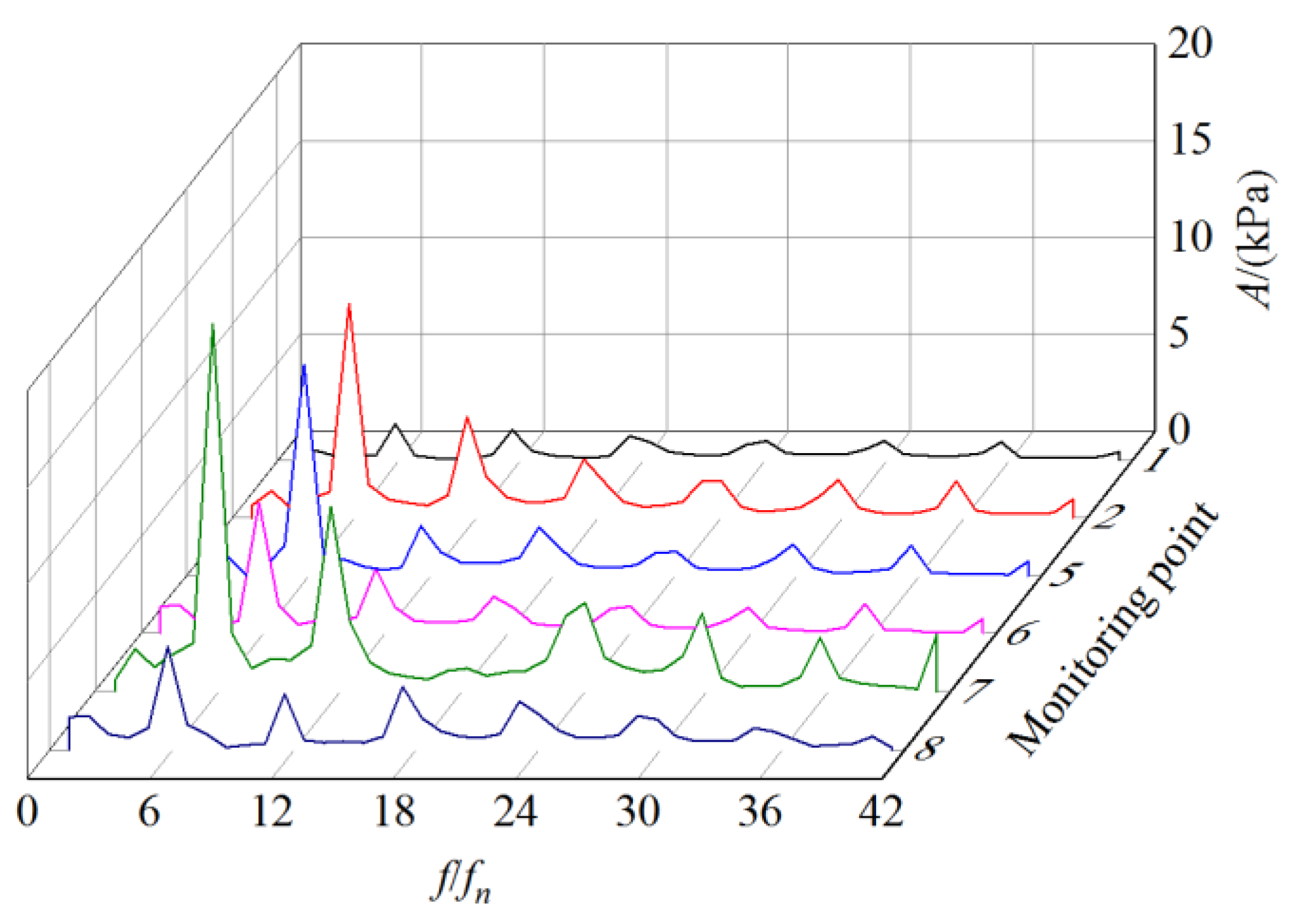
4.2. Analysis of Pressure Fluctuation at the Volute Radial Location
4.3. Analysis of Pressure Fluctuation at the Volute Circumferential Location
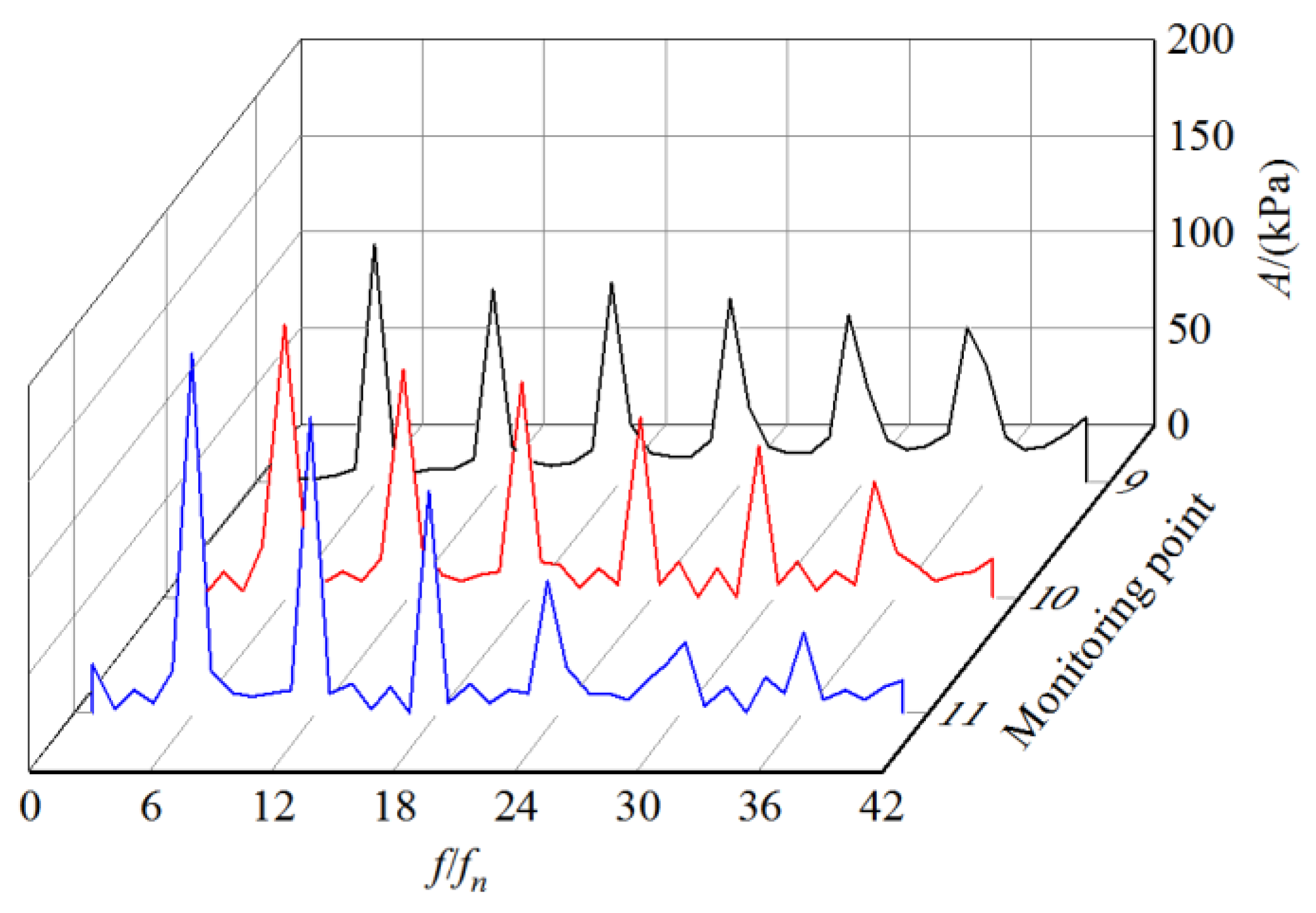
4.4. Analysis of Pressure Fluctuation in the Impeller
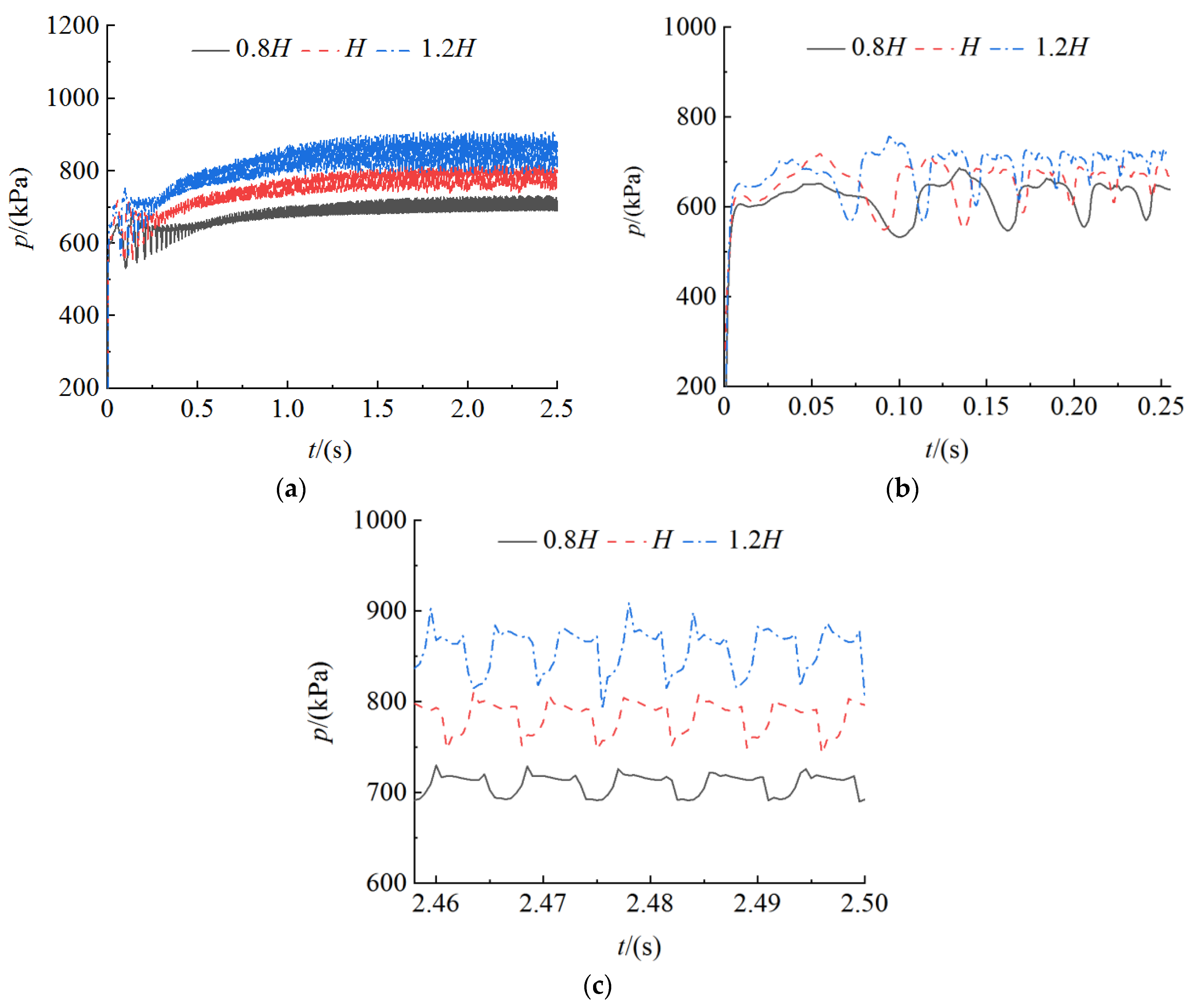
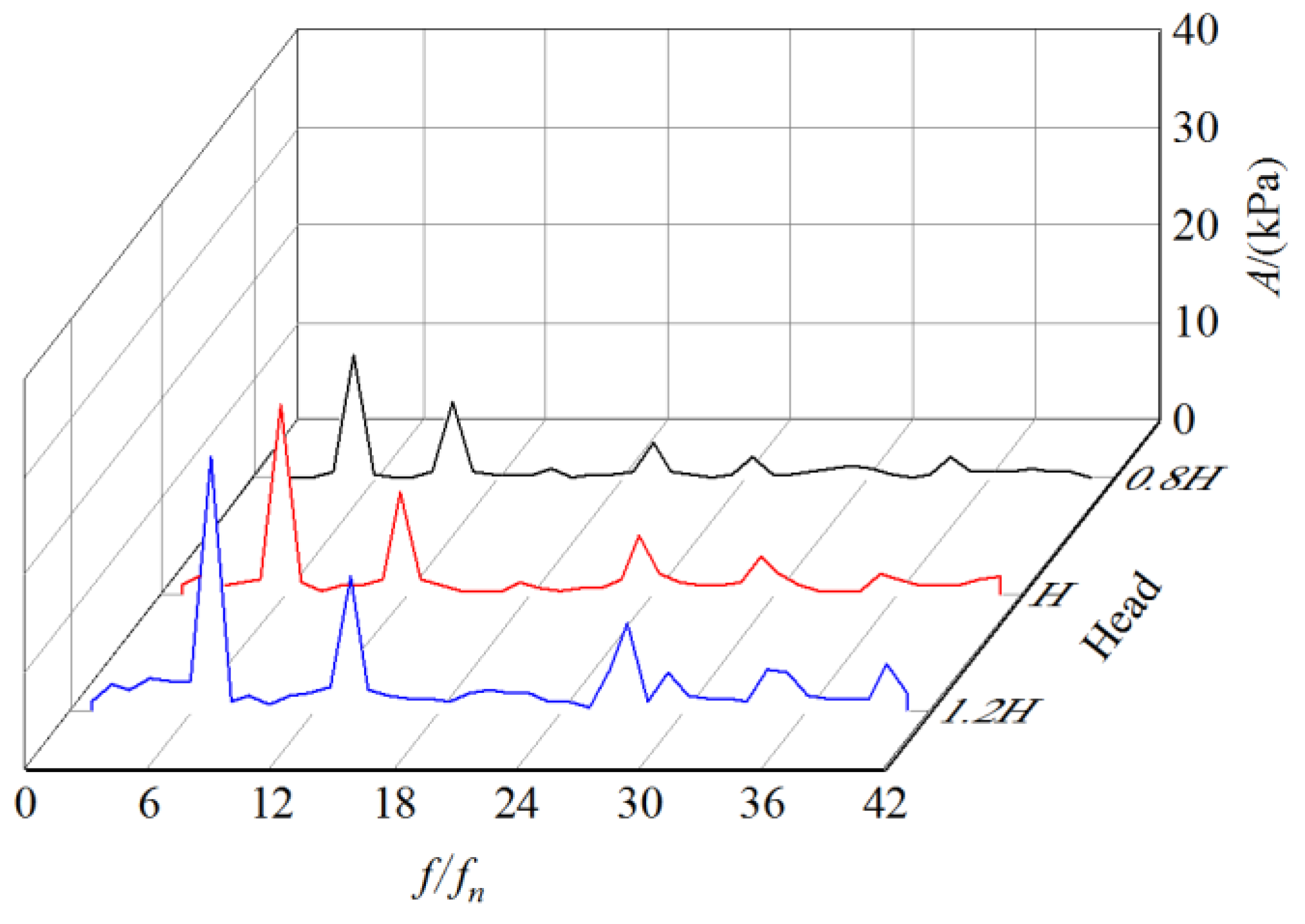
4.5. Analysis of Pressure Fluctuation at Various Head
5. Conclusions
- (1)
- At the beginning of start-up, there are a large number of low-pressure areas and strong vortexes in the impeller. With the increase in rotating speed, the pressure has a gradient distribution along the flow channel, and the vortexes rapidly decrease and are concentrated on the blade non-working face.
- (2)
- The pressure fluctuation amplitude is the largest at the initial start time. With the increase in rotating speed, the pressure fluctuation amplitude of the spiral part in the volute attenuates rapidly, but it attenuates slowly in the impeller. The number of pressure fluctuation at each position in one impeller rotation cycle is consistent with the blade number, and the dominant frequency of pressure fluctuation is 6 times that of the impeller rotation frequency.
- (3)
- With the decrease in radial dimension, the dominant frequency amplitude and time-averaged pressure of radial pressure fluctuation in the volute decrease gradually. The pressure fluctuation at the front end of the tongue is the largest in the volute. The most intense pressure fluctuation in the impeller occurs in the middle of the impeller towards the inner edge. The pressure fluctuation in the impeller is much larger than that in the volute. Due to the dynamic and static interference between the impeller and the volute, the secondary fluctuation occurs near the coupling surface in a period of pressure fluctuation.
- (4)
- With the increase in the head, the time required to complete the start-up decreases and the rotating speed increases after completing the start-up. Meanwhile, the number of pressure fluctuation at the same time, the fluctuation amplitude and the dominant frequency amplitude of pressure fluctuation increase during the start-up process.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, X.; Yang, J.; Shi, F. Status and prospect of study on energy recovery hydraulic turbines. J. Drain. Irrig. Mach. Eng. 2014, 32, 742–747. [Google Scholar]
- Binama, M.; Su, W.T.; Li, X.B.; Li, F.C.; Wei, X.Z.; An, S. Investigation on pump as turbine (PAT) technical aspects for micro hydropower schemes: A state of the art review. Renew. Sustain. Energy Rev. 2017, 79, 148–179. [Google Scholar] [CrossRef]
- Chen, T.; Zhang, Y.; Li, S. Instability of large-scale prototype Francis turbines of Three Gorges power station at part load. Proc. Inst. Mech. Eng. Part A J. Power Energy 2016, 230, 619–632. [Google Scholar] [CrossRef]
- Sanjay, V.; Rajesh, N. Investigations on pump running in turbine mode: A review of the state-of-the-art. Renew. Sustain. Energy Rev. 2014, 79, 842–868. [Google Scholar]
- Huang, S.; Qiu, G.; Su, X.; Chen, J.; Zou, W. Performance prediction of a centrifugal pump as turbine using rotor volute matching principle. Renew. Energy 2017, 108, 64–71. [Google Scholar] [CrossRef]
- Stefanizzi, M.; Torresi, M.; Fortunato, B. Experimental investigation and performance prediction modeling of a single stage centrifugal pump operating as turbine. Energy Procedia 2017, 126, 589–596. [Google Scholar] [CrossRef]
- Venturini, M.; Manservigi, L.; Alvisi, S.; Simani, S. Development of a physics-based model to predict the performance of pumps as turbines. Appl. Energy 2018, 231, 343–354. [Google Scholar] [CrossRef]
- Daniele, N.; Aonghus, M. A model for the extrapolation of the characteristic curves of pumps as turbines from a datum best efficiency point. Energy Convers. Manag. 2018, 174, 1–7. [Google Scholar]
- Yao, Z.; Wang, F.; Qu, L.; Xiao, R.; He, C.; Wang, M. Experimental investigation of time-frequency characteristics of pressure fluctuations in a double-suction centrifugal pump. ASME J. Fluids Eng. 2011, 133, 101303–101312. [Google Scholar] [CrossRef]
- Huang, X.; Liu, Z. Analysis of low frequency pressure fluctuations in vaneless centrifugal pump volute. J. Mech. Eng. 2014, 50, 170–176. [Google Scholar] [CrossRef]
- Jin, S.; Wang, Y.; Chang, S.; Su, Y. Pressure fluctuation of interior flow in mixed-flow pump. Trans. Chin. Soc. Agric. Mach. 2013, 44, 64–68. [Google Scholar]
- Zhang, D.; Wang, H.; Shi, W.; Pan, D.; Shao, P. Experimental investigation of pressure fluctuation with multiple flow rates in scaled axial flow pump. Trans. Chin. Soc. Agric. Mach. 2014, 45, 139–145. [Google Scholar]
- Feng, W.; Cheng, Q.; Guo, Z.; Pan, J. Characteristics of low frequency pressure fluctuation in axial flow pump with variable inlet guide vane. Trans. Chin. Soc. Agric. Mach. 2015, 46, 62–67. [Google Scholar]
- Sun, Y.; Zuo, Z.; Liu, S.; Wu, Y. Distribution of pressure fluctuations in prototype pump turbine at pump mode. Adv. Mech. Eng. 2014, 6, 923–937. [Google Scholar] [CrossRef]
- Li, D.; Gong, R.; Wang, H.; Xiang, G.; Wei, X.; Liu, Z. Dynamic analysis on pressure fluctuation in vaneless region of a pump turbine. Sci. China Technol. Sci. 2015, 58, 813–824. [Google Scholar] [CrossRef]
- Yang, S.; Kong, F.; Cheng, J.; Huang, Z.P.; Zhang, X.P. Reserch on unsteady pressure field within a hydraulic turbine volute. Eng. Mech. 2013, 30, 388–393. [Google Scholar]
- Yang, S.; Kong, F.; Qu, X.; Jiang, W.M. Influence of blade number on the performance and pressure fluctuations in a pump used as a turbine. J. Fluids Eng. 2012, 134, 124503–124512. [Google Scholar] [CrossRef]
- Yang, S.; Liu, H.; Kong, F.; Xia, B.; Tan, L.W. Effects of the radial gap between impeller tips and volute tongue influencing the performance and pressure fluctuations of pump as turbine. J. Fluids Eng. 2014, 136, 1–8. [Google Scholar] [CrossRef]
- Shi, G.; Yang, J.; Miao, S.; Li, T.L. Calculation of pressure fluctuation within volute of hydraulic turbine under different guide vane numbers. J. Aerosp. Power 2015, 30, 1228–1235. [Google Scholar]
- Shi, G.; Yang, J.; Miao, S.; Wang, X.H. Unsteady calculation of pressure fluctuations within hydraulic turbine under different entrance sections. J. Aerosp. Power 2016, 31, 659–668. [Google Scholar]
- Dai, C.; Kong, F.; Dong, L.; Feng, F. Analysis and experimental investigation of pressure fluctuation in draft tube of pump as turbine. J. Cent. South Univ. (Sci. Technol.) 2015, 46, 3131–3137. [Google Scholar]
- Shi, F.; Yang, J.; Wang, X. Analysis on characteristic of pressure fluctuation in hydraulic turbine with guide vane. Int. J. Fluid Mach. Syst. 2016, 9, 237–244. [Google Scholar] [CrossRef] [Green Version]
- Li, W.; Lu, D.; Ma, L.; Ji, L.; Wu, P. Experimental study on pressure vibration characteristics of mixed-flow pump during start-up. Trans. Chin. Soc. Agric. Eng. 2021, 37, 44–50. [Google Scholar]
- Fu, S.; Zheng, Y.; Kan, K.; Chen, H.; Han, X.; Liang, X.; Liu, H.; Tian, X. Numerical simulation and experimental study of transient characteristics in an axial flow pump during start-up. Renew. Energy 2020, 146, 1879–1887. [Google Scholar] [CrossRef]
- Chen, W.; Liu, Y.; Chen, L. Study on hydrodynamic performance of horizontal tidal turbine rotating passively based on UDF. Ocean Eng. 2018, 36, 119–126. [Google Scholar]
- Sun, K.; Li, Y.; Zhao, J. Transient starting performance analysis of vertical axis tidal turbine. Huazhong Univ. Sci. Technol. (Nat. Sci. Ed.) 2017, 45, 51–56. [Google Scholar]
- Untaroiu, A.; Wood, H.G.; Allaire, P.E.; Ribando, R.J. Investigation of self-starting capability of vertical axis wind turbines using a computational fluid dynamics approach. J. Sol. Energy Eng. 2011, 133, 125–134. [Google Scholar] [CrossRef]
- Su, X. Research on the Variable Rotation Speed and Transient Process of Centrifugal Pump as Turbine System. Ph.D. Thesis, South China University of Technology, Guangzhou, China, 2016. [Google Scholar]
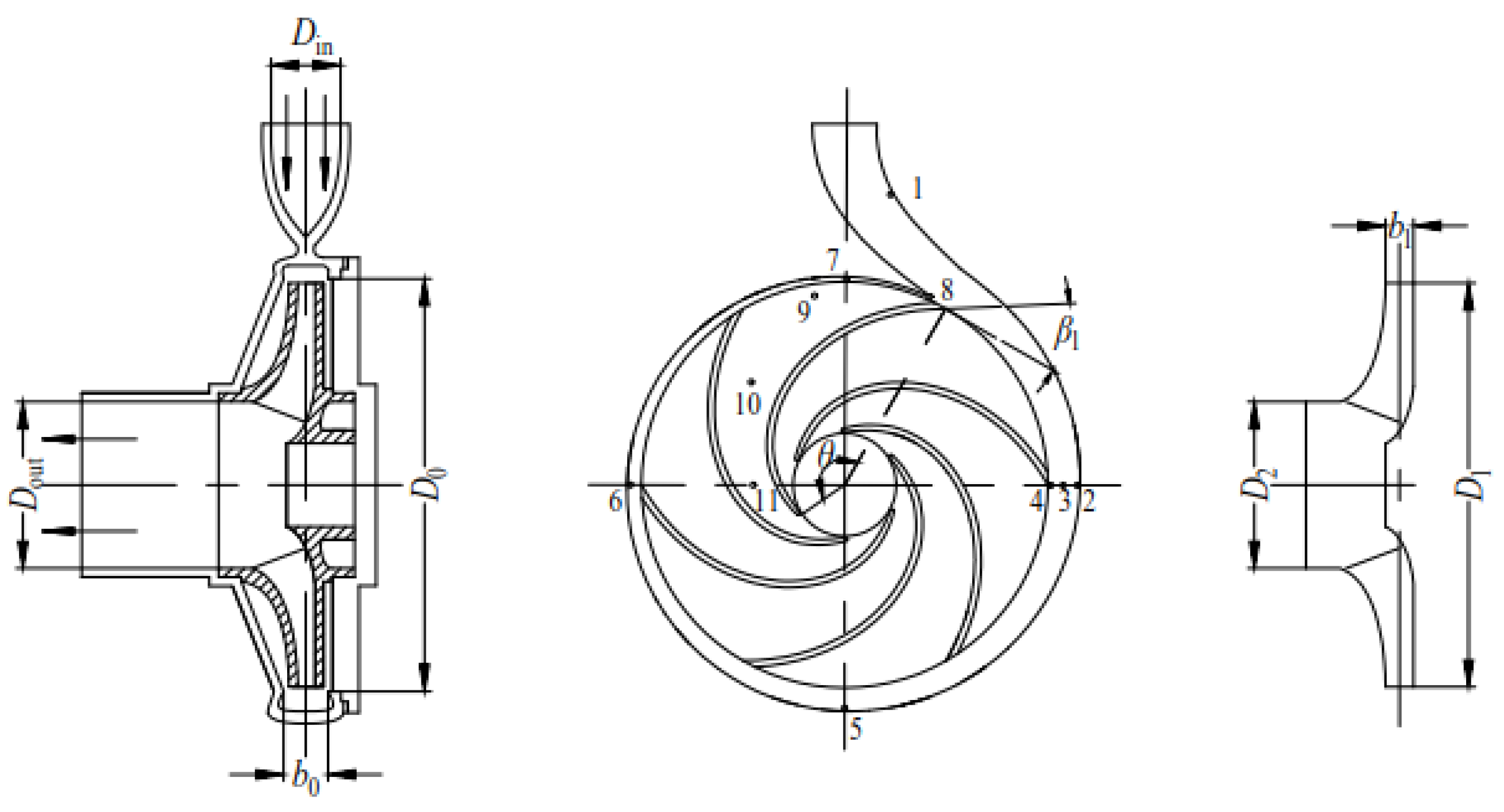





| Parameters | Value |
|---|---|
| Impeller inlet diameter D1/mm | 315 |
| Impeller outlet diameter D2/mm | 80 |
| Blade inlet width b1/mm | 10 |
| Number of blades z | 6 |
| Blade inlet offset angle β1 (°) | 32 |
| Blade wrap angle θ (°) | 150 |
| Volute inlet diameter Din/mm | 50 |
| Volute outlet width b0/mm | 24 |
| Base circle diameter D0/mm | 320 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chai, B.; Yang, J.; Wang, X.; Jiang, B. Pressure Fluctuation Characteristics Analysis of Centrifugal Pump as Turbine in Its Start-Up Process. Actuators 2022, 11, 132. https://doi.org/10.3390/act11050132
Chai B, Yang J, Wang X, Jiang B. Pressure Fluctuation Characteristics Analysis of Centrifugal Pump as Turbine in Its Start-Up Process. Actuators. 2022; 11(5):132. https://doi.org/10.3390/act11050132
Chicago/Turabian StyleChai, Baodui, Junhu Yang, Xiaohui Wang, and Bingxiao Jiang. 2022. "Pressure Fluctuation Characteristics Analysis of Centrifugal Pump as Turbine in Its Start-Up Process" Actuators 11, no. 5: 132. https://doi.org/10.3390/act11050132
APA StyleChai, B., Yang, J., Wang, X., & Jiang, B. (2022). Pressure Fluctuation Characteristics Analysis of Centrifugal Pump as Turbine in Its Start-Up Process. Actuators, 11(5), 132. https://doi.org/10.3390/act11050132






