Multi-Objective Optimization Design and Dynamic Performance Analysis of an Enhanced Radial Magnetorheological Valve with Both Annular and Radial Flow Paths
Abstract
:1. Introduction
2. Design and Development of an Enhanced Radial MR Valve
2.1. Principle and Structure Analysis
2.2. Mathematical Model of MR Valve
3. Flow Field Simulation of the Enhanced Radial MR Valve
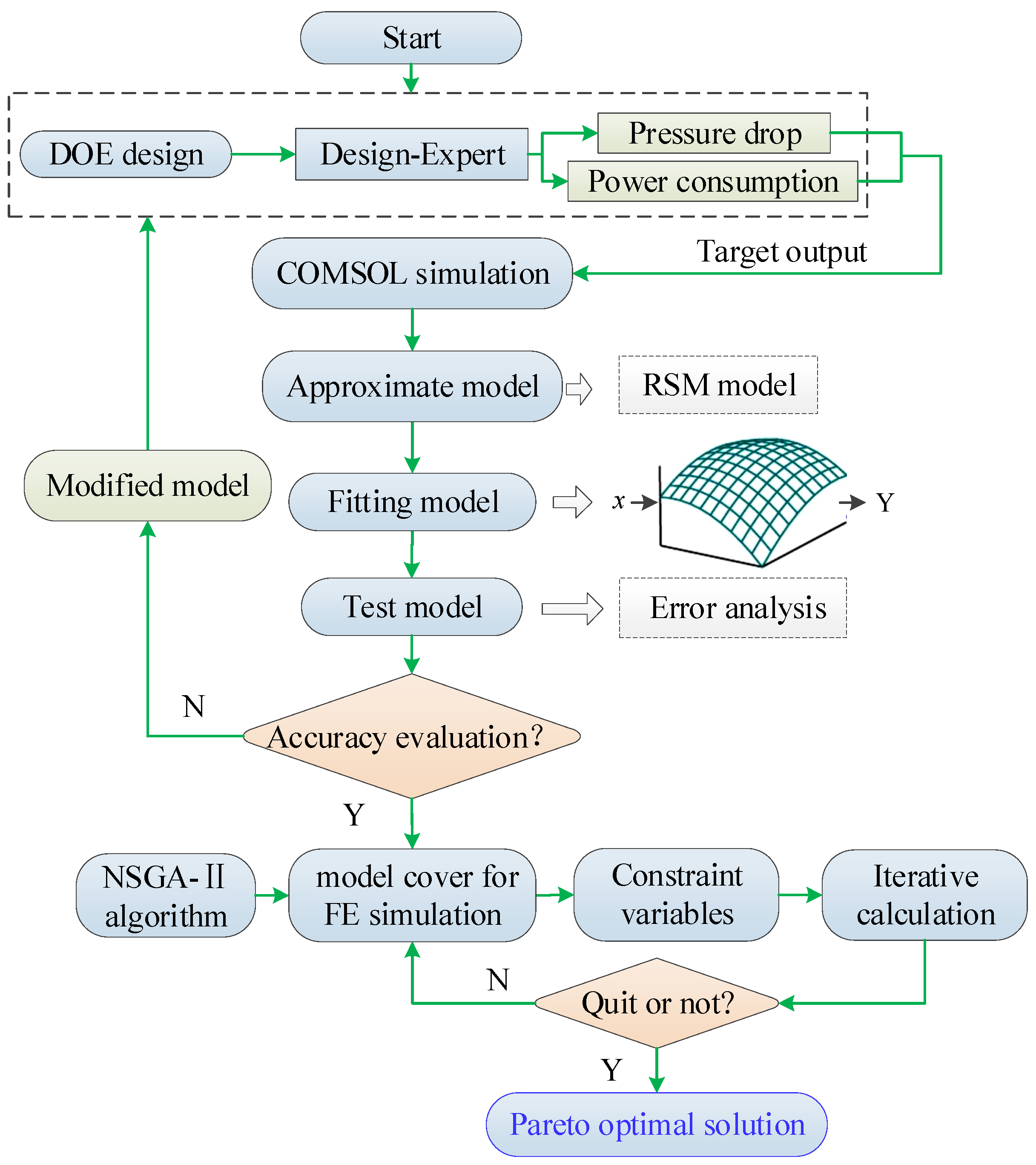
4. Multi-Objective Optimization Design of the Enhanced Radial MR Valve
4.1. Orthogonal Experimental Analysis
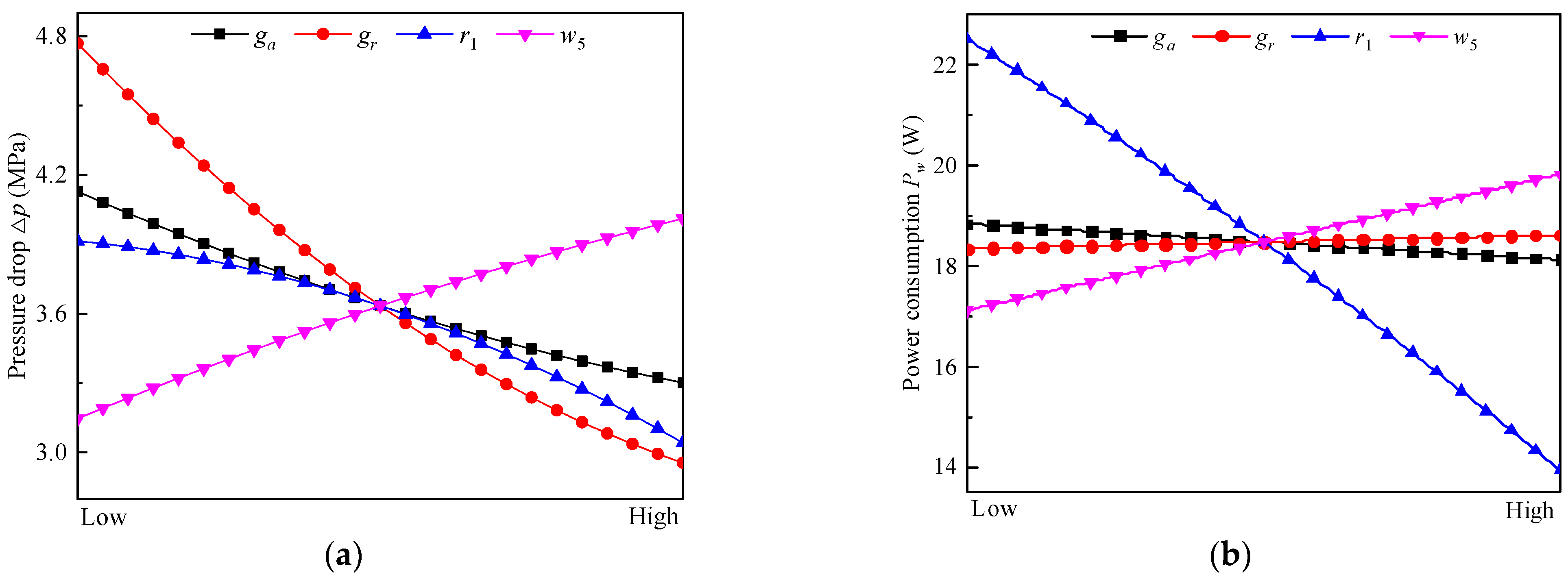
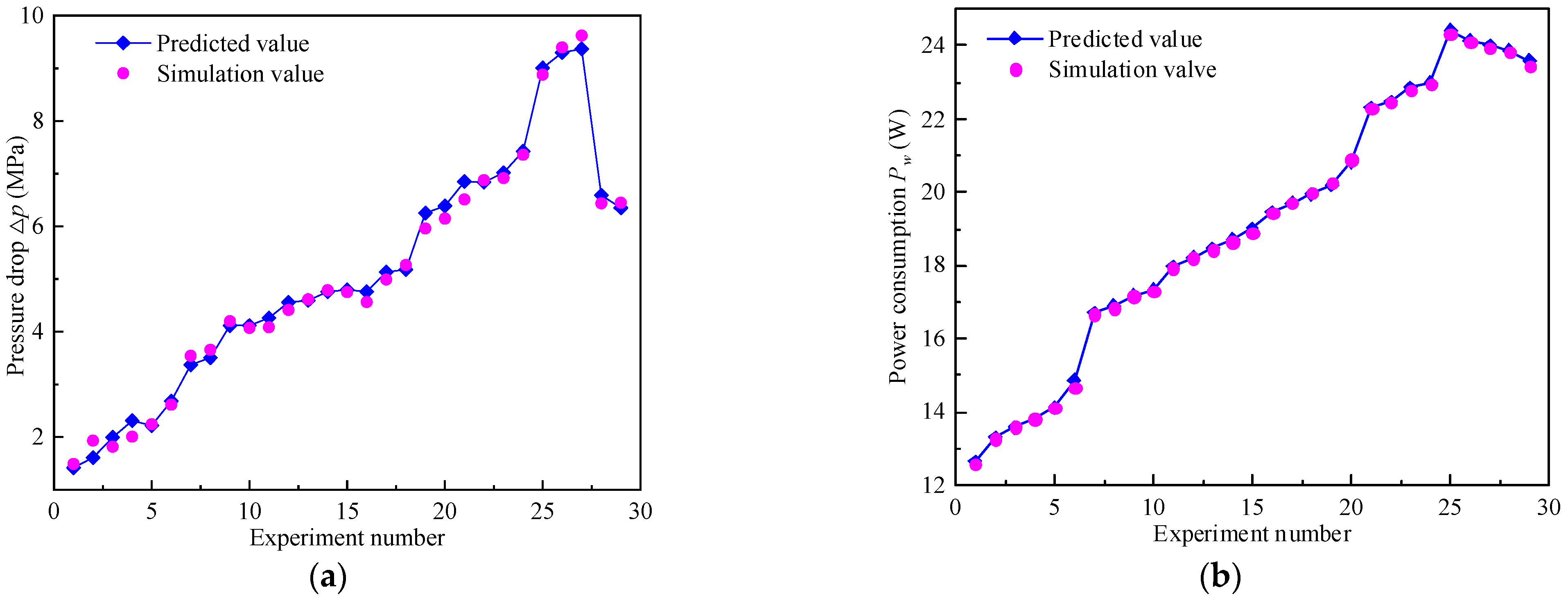
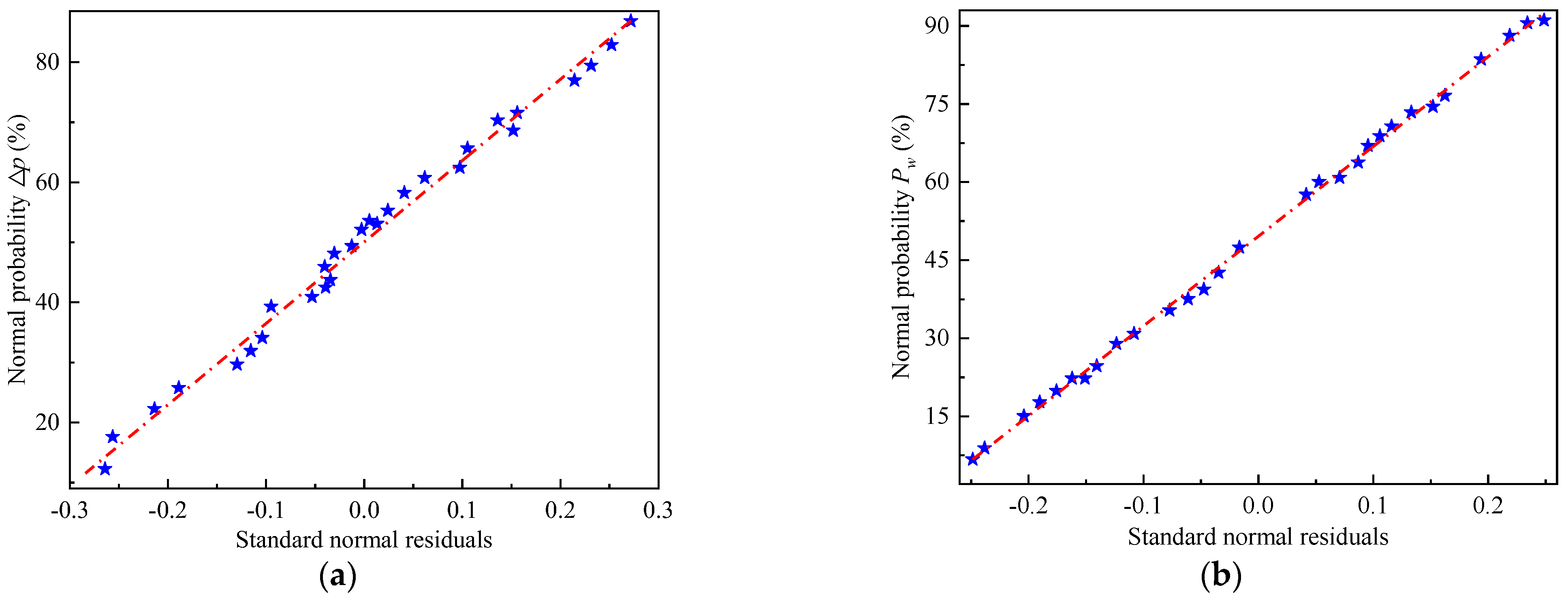
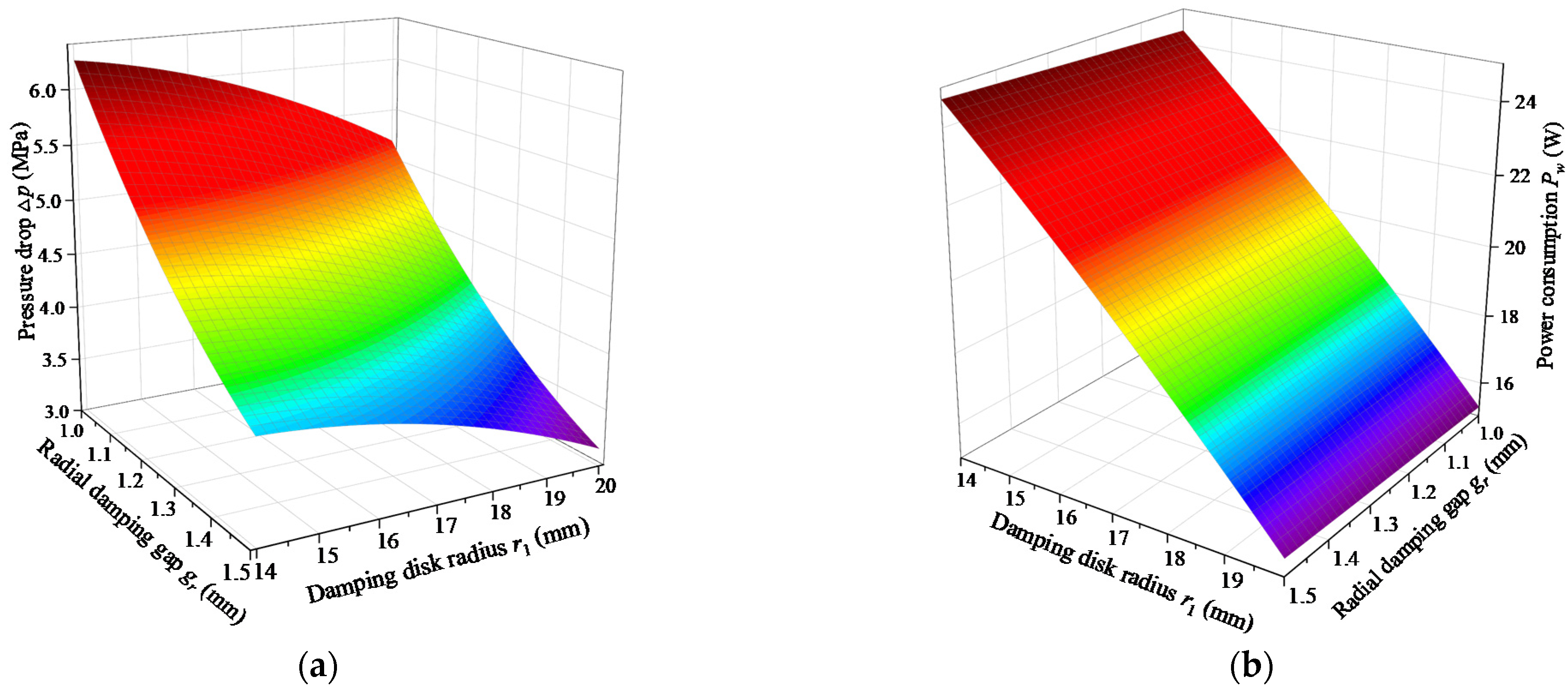
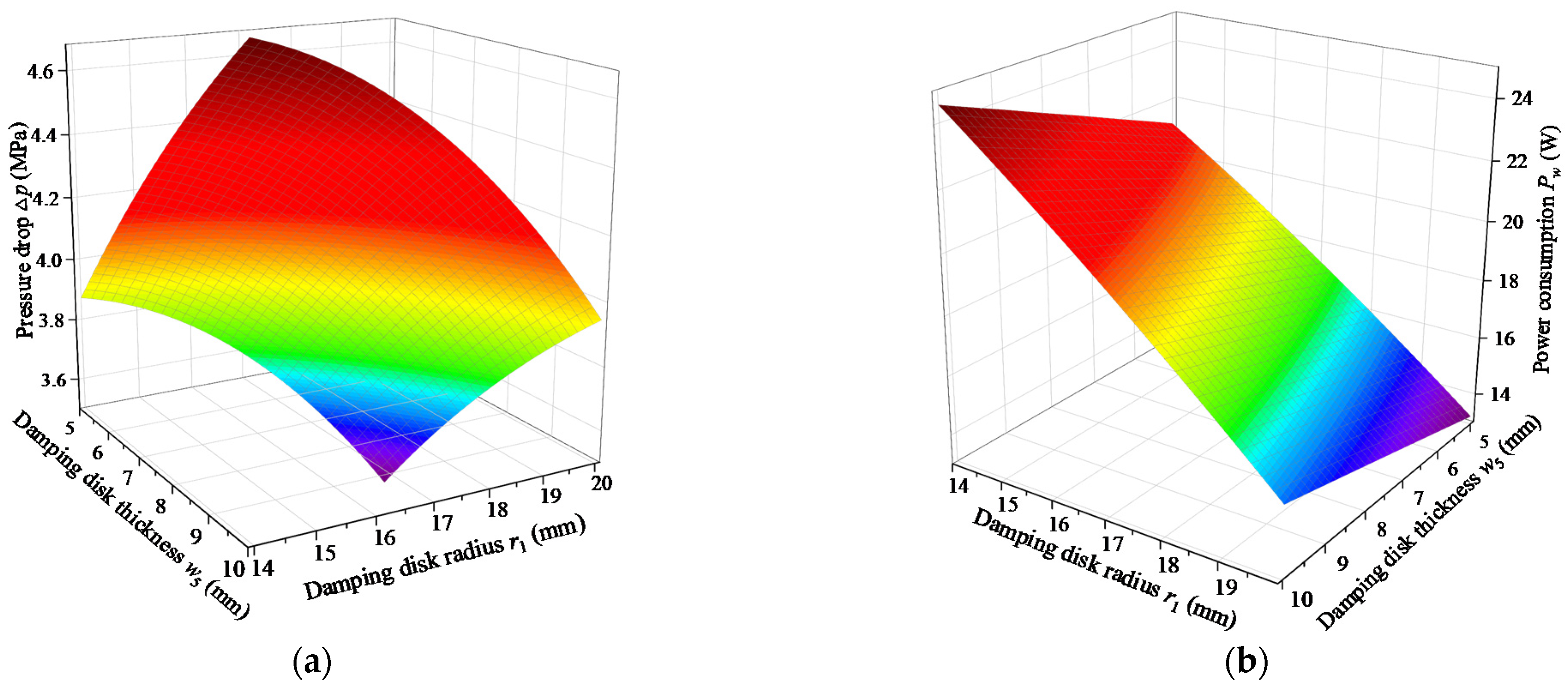
4.2. Construction of Approximate Model
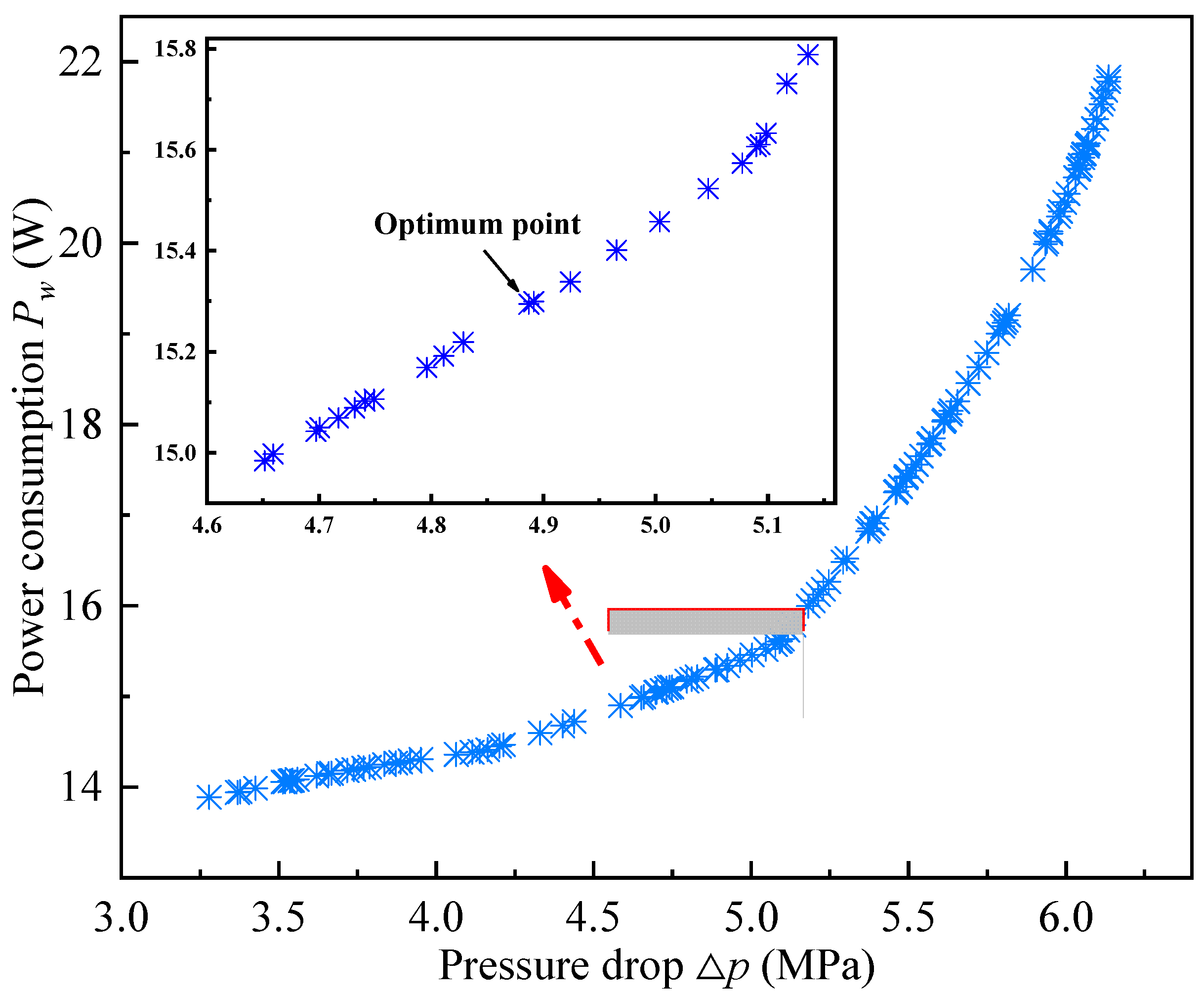
4.3. Multi-Objective Optimization Formulation
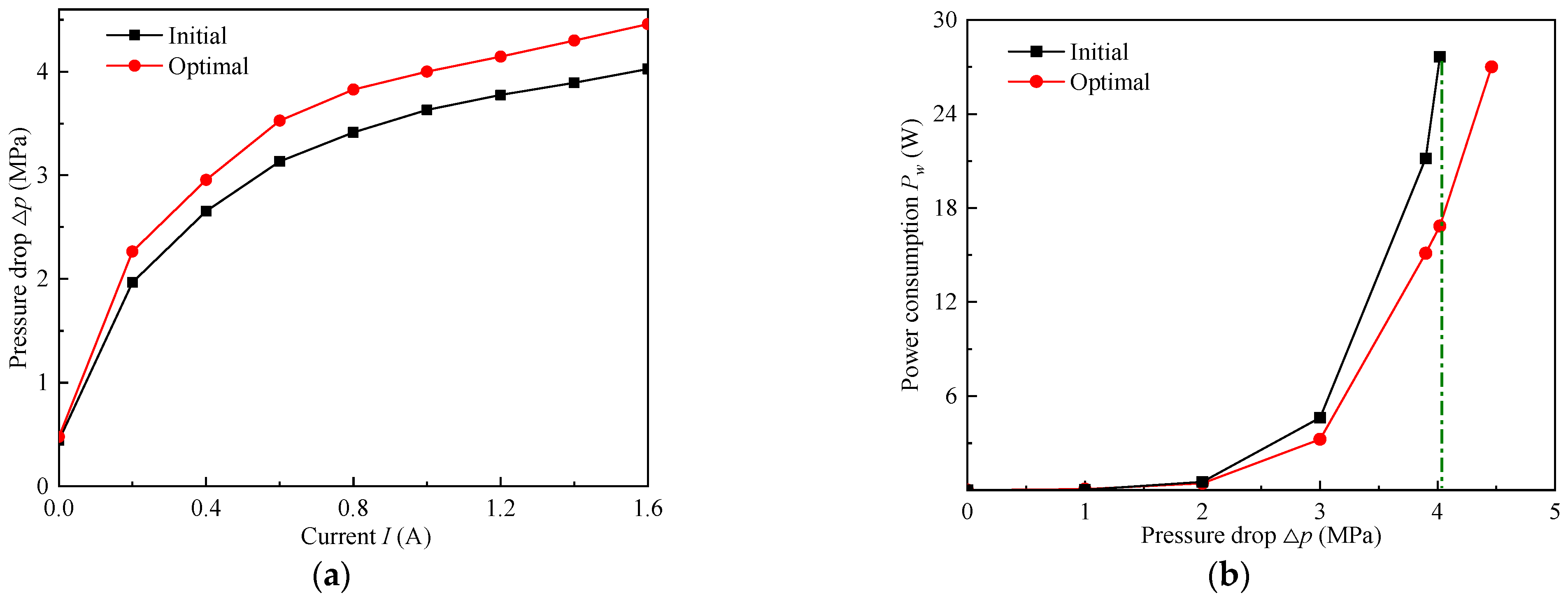
4.4. Analysis of Optimization Results
5. Performance Analysis of the Enhanced Radial MR Valve
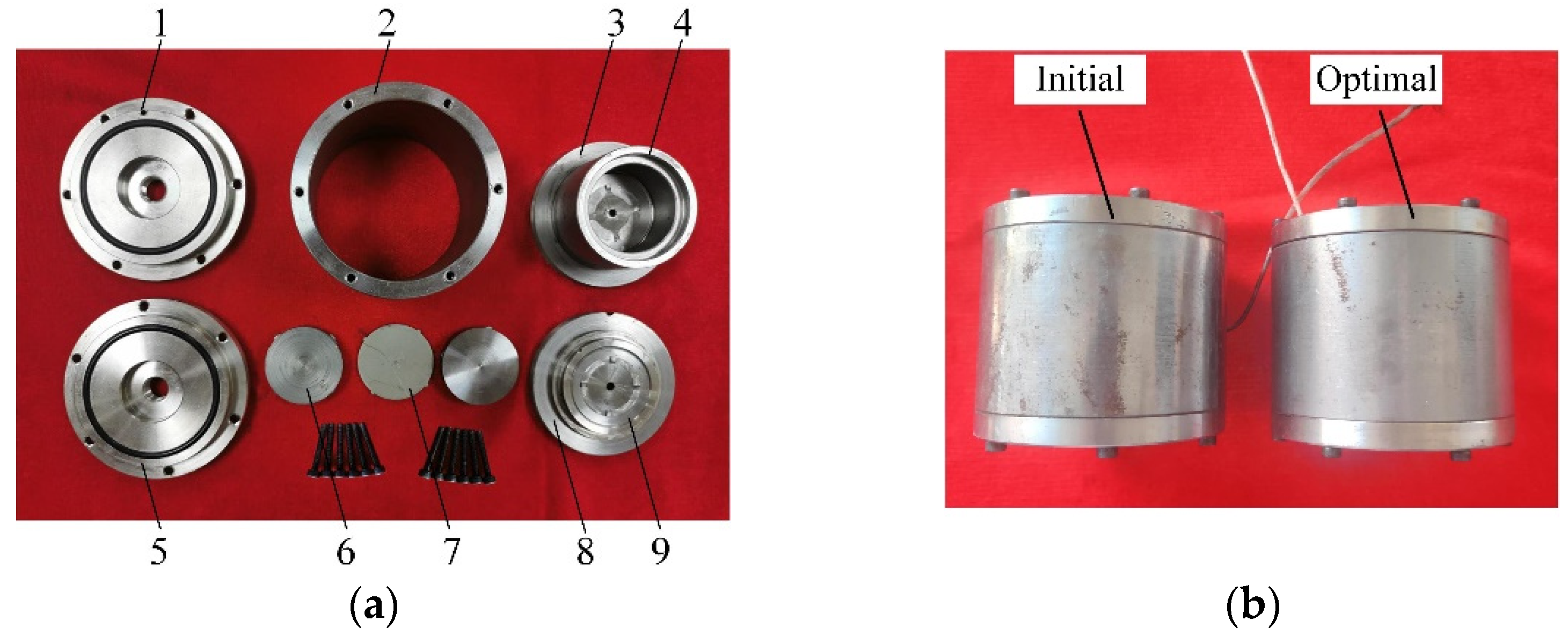
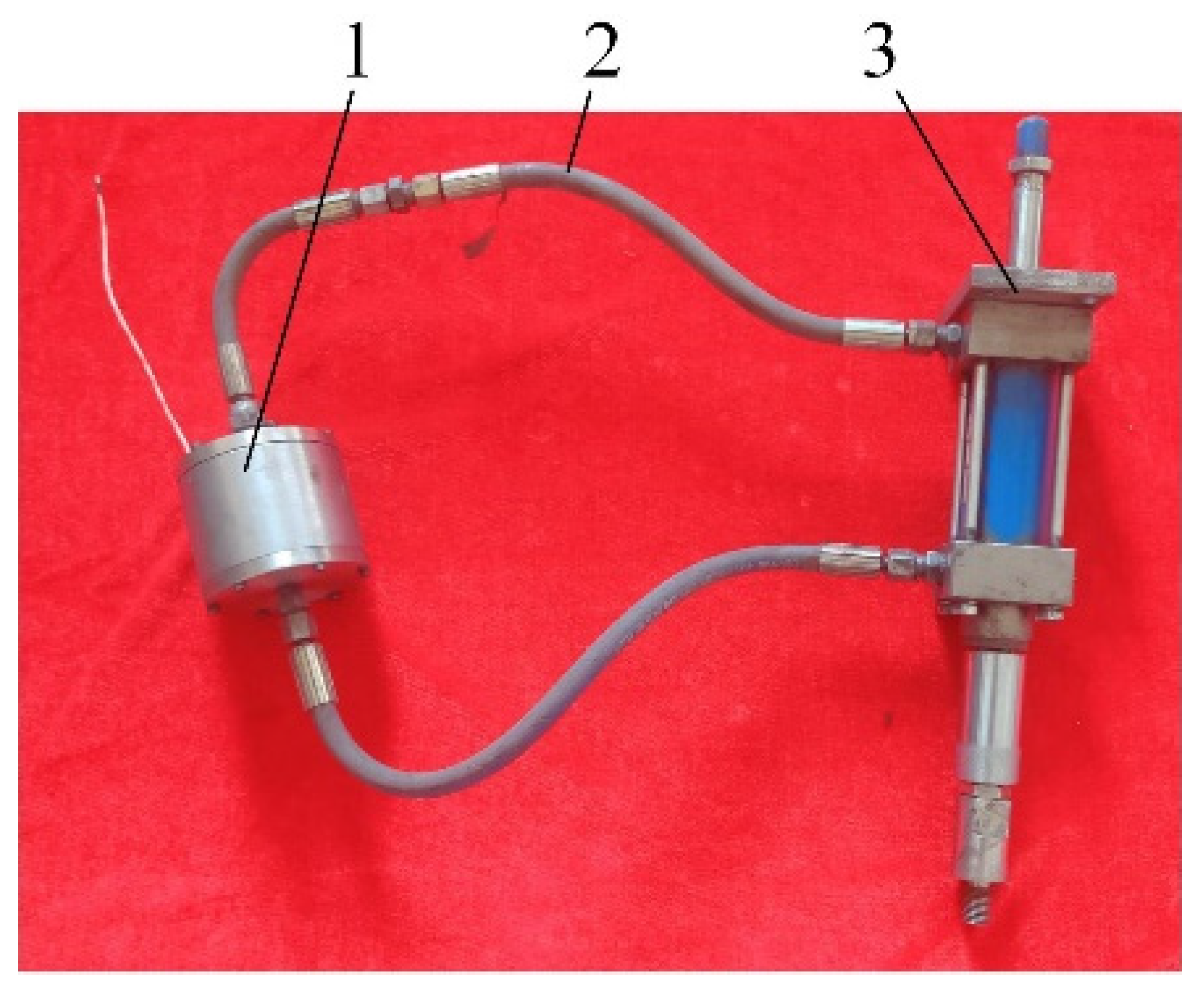
5.1. Prototype and Experimental Test Rig Setup
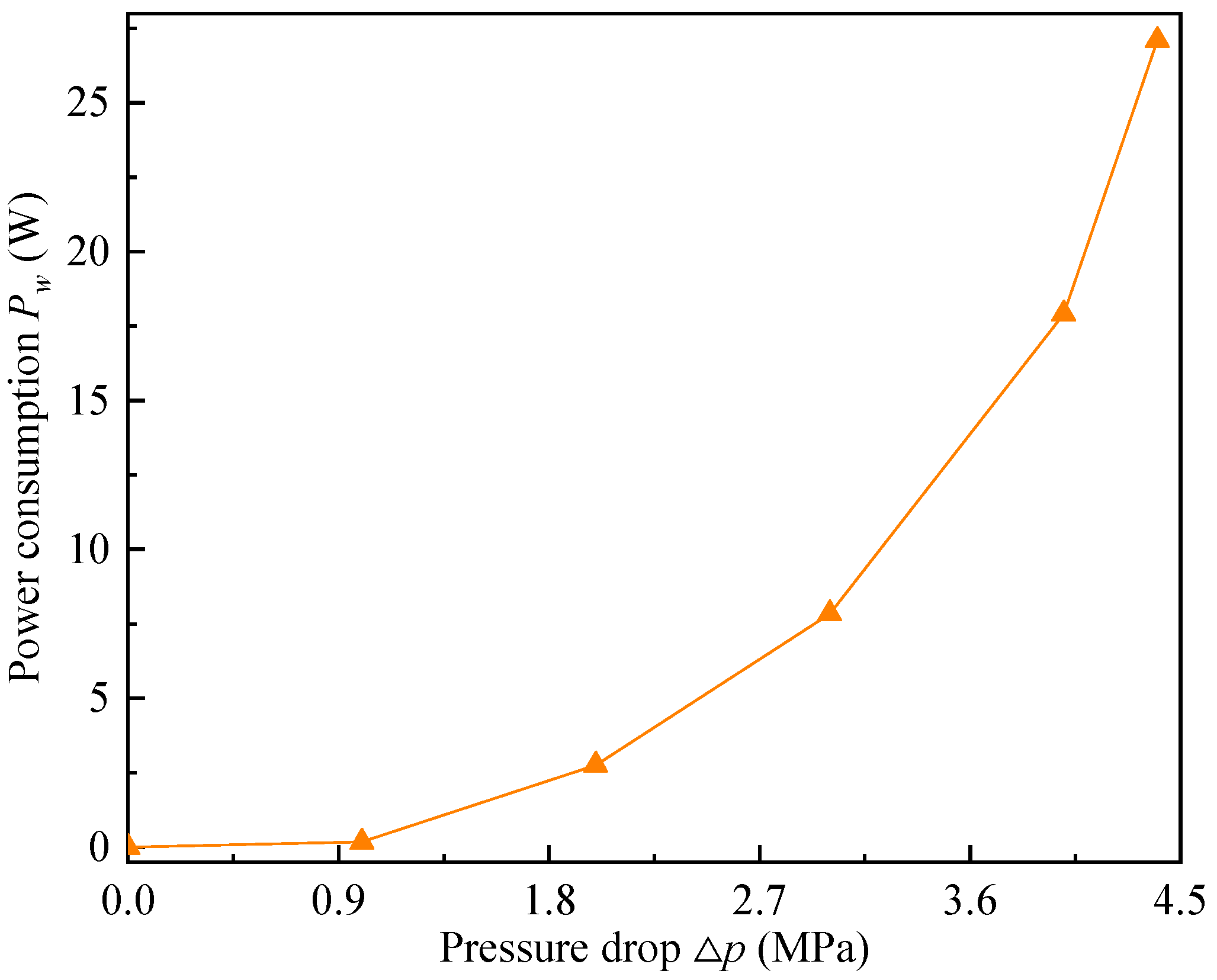
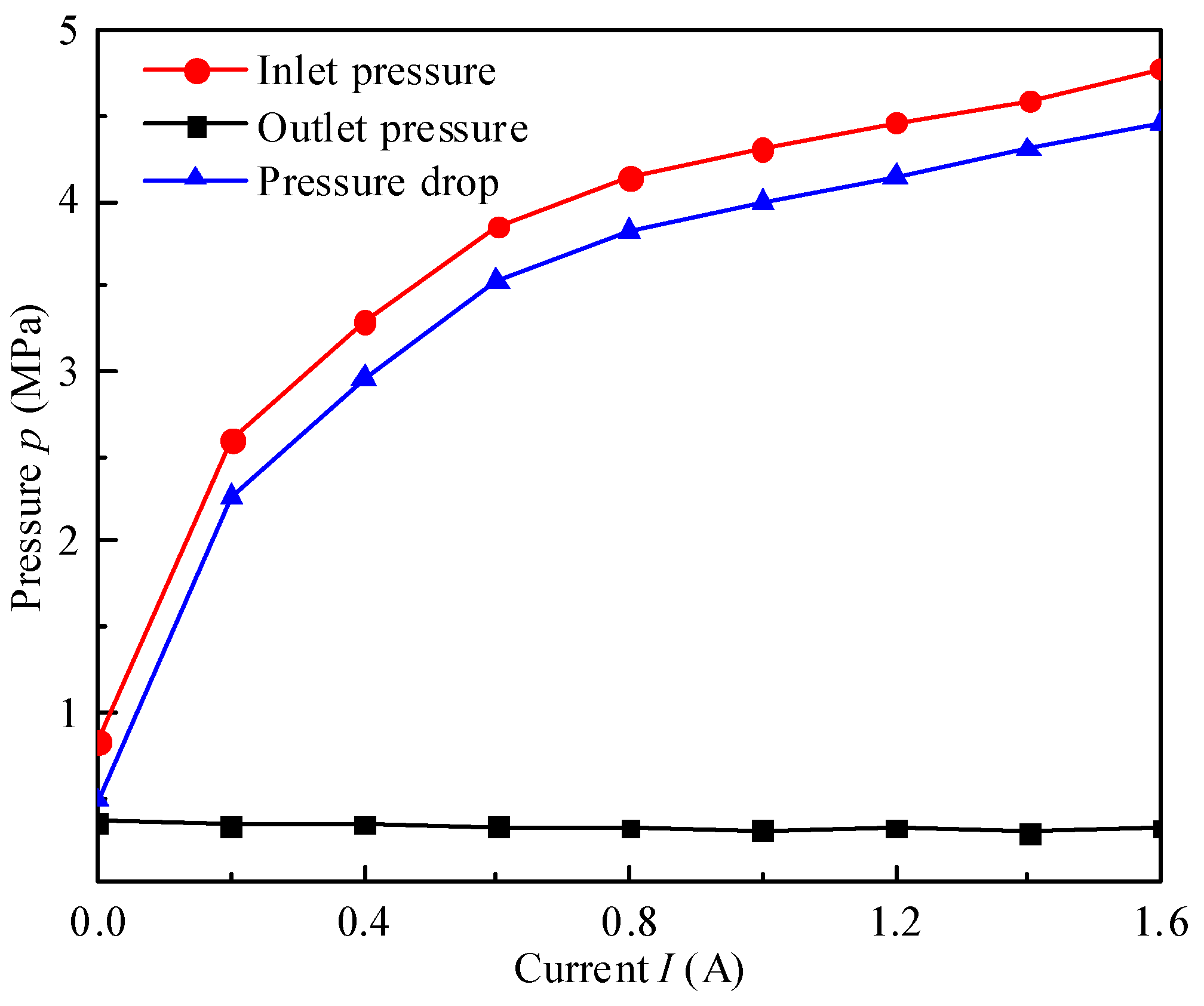
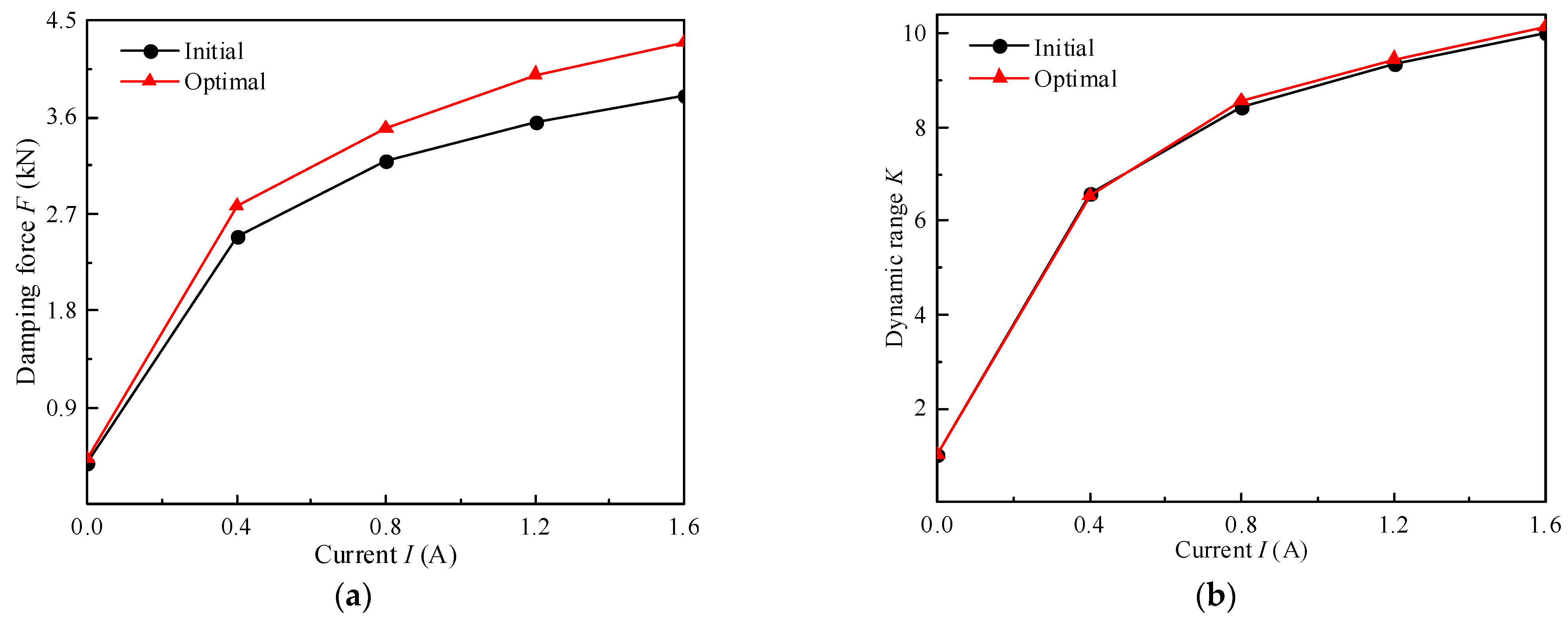
5.2. Experimental Analysis of Pressure Drop Performance
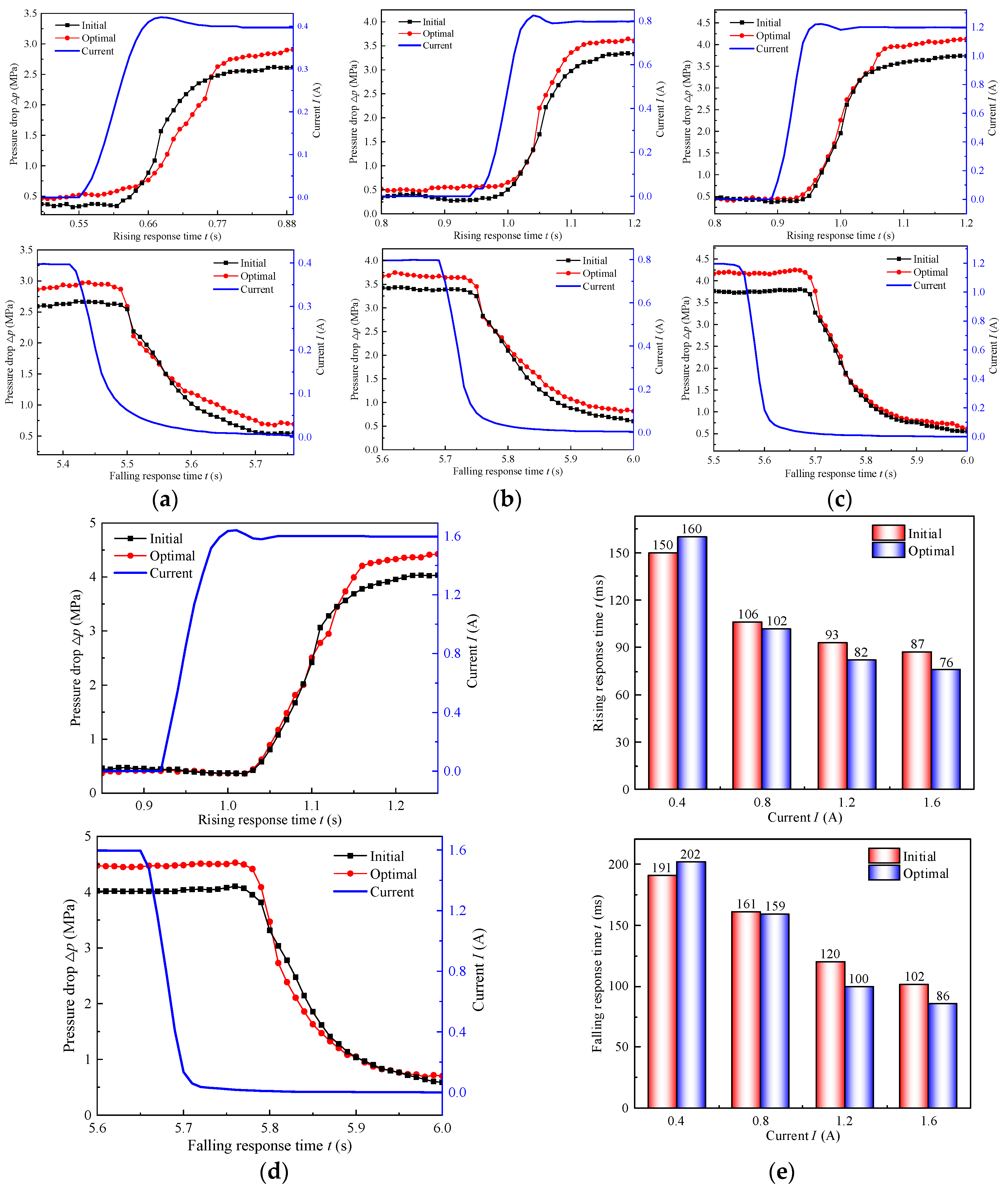
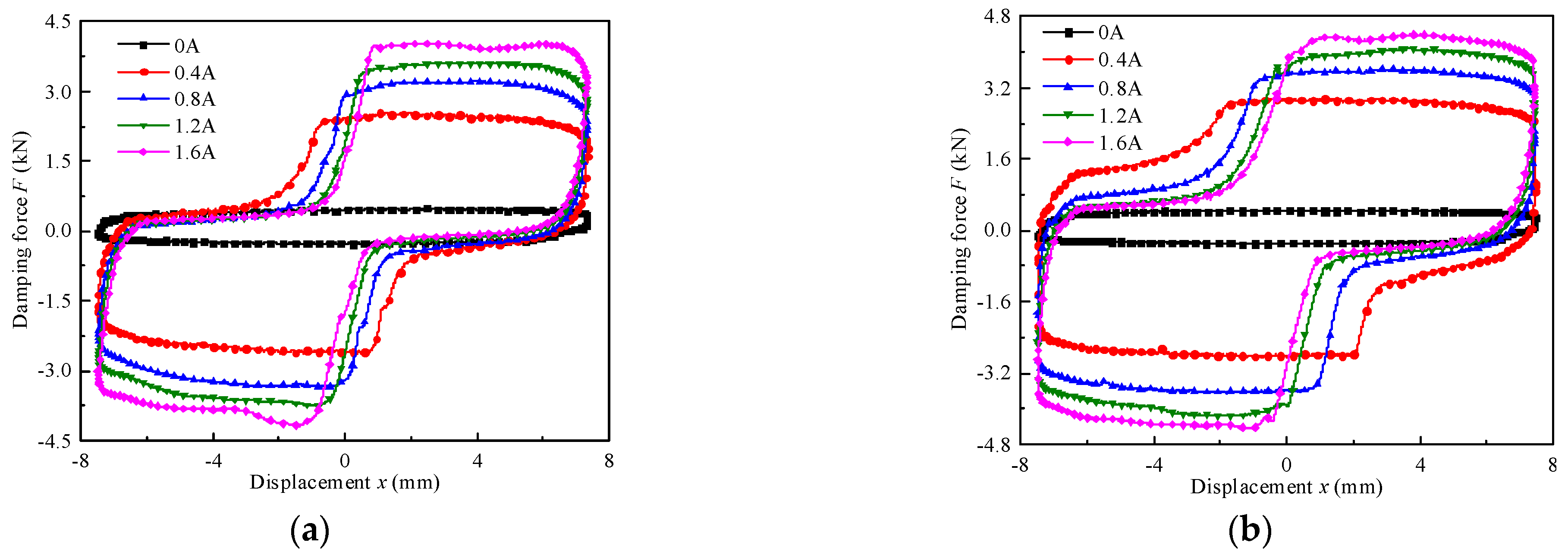
5.3. Experimental Analysis of Dynamic Response Time
6. Performance Analysis of the Enhanced Radial MR Valve Controlled Cylinder System
6.1. Test System of the Proposed MR Valve Controlled Cylinder System
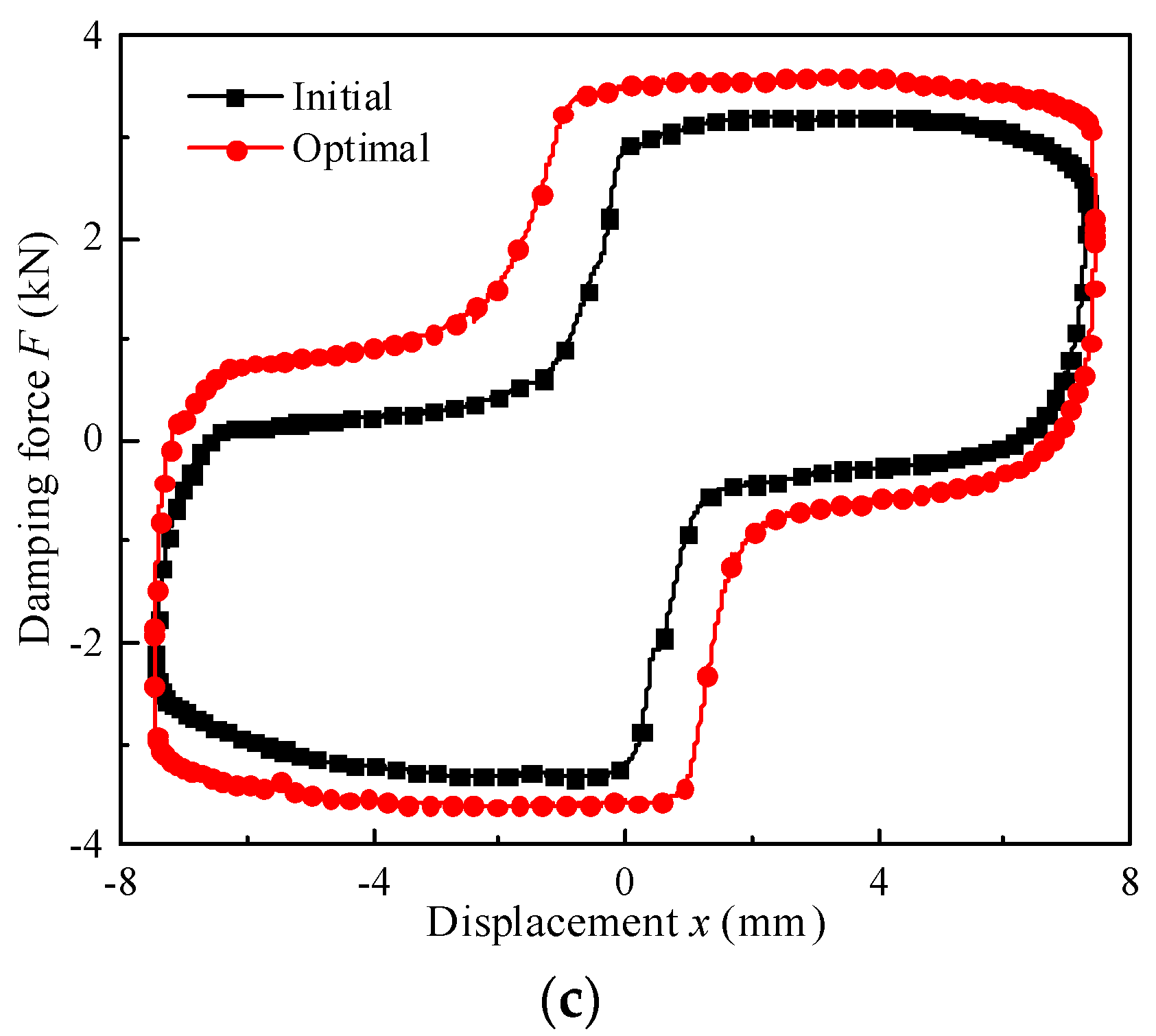
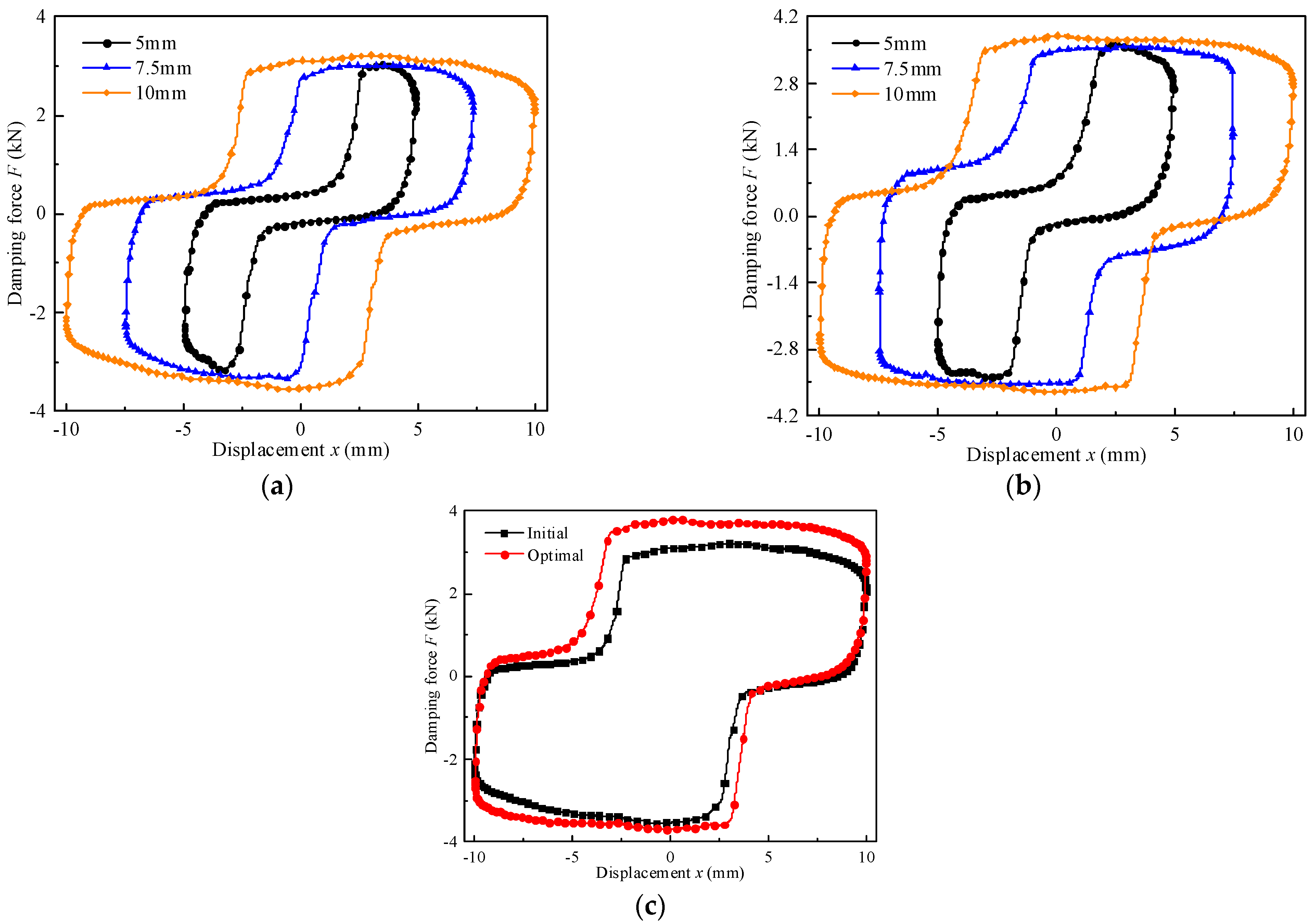
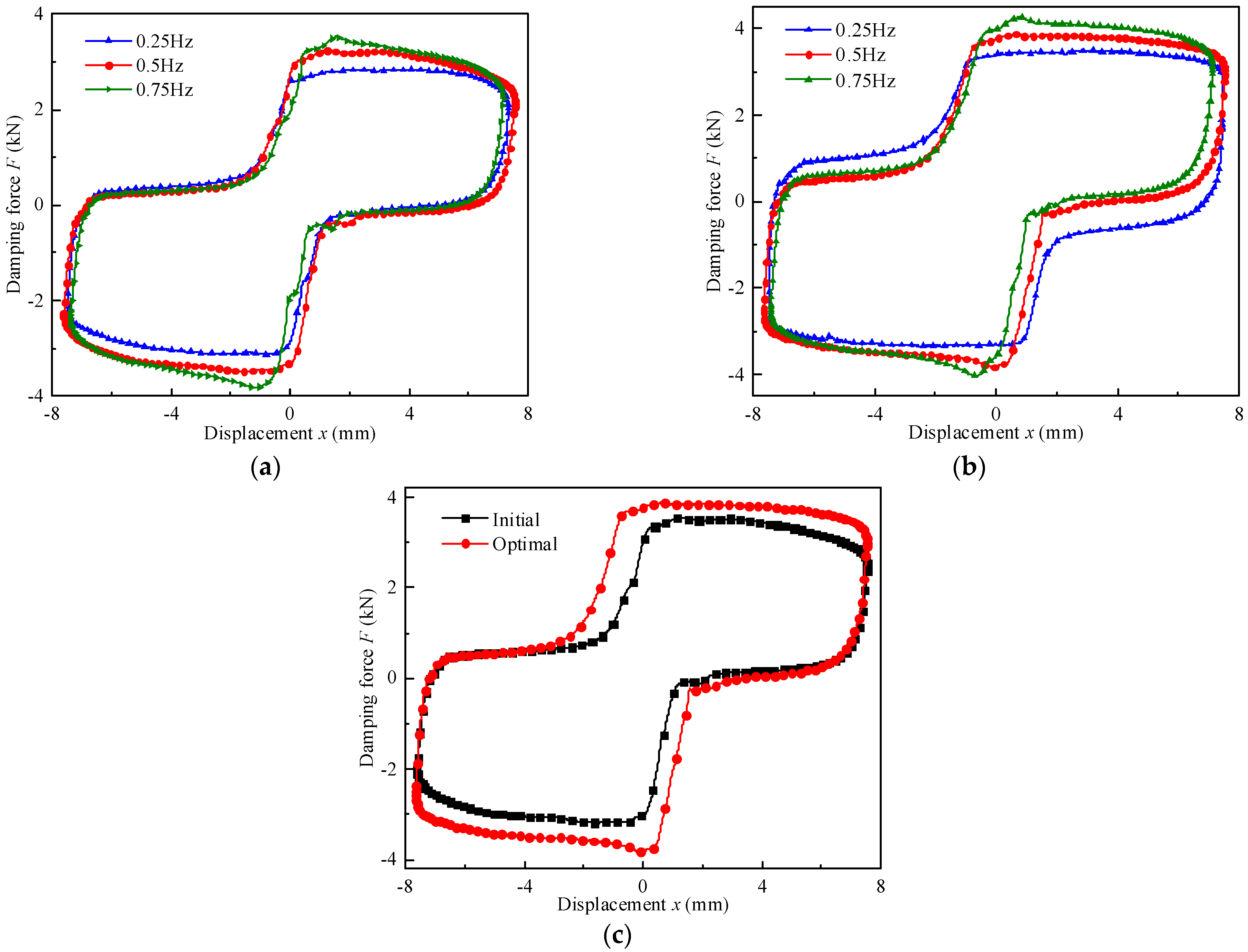
6.2. Dynamic Performance of the Initial and Optimal MR Valve Controlled Cylinder System
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Elsaady, W.; Oyadiji, S.O.; Nasser, A. A review on multi-physics numerical modelling in different applications of magnetorheological fluids. J. Intell. Mater. Syst. Struct. 2020, 31, 1855–1897. [Google Scholar] [CrossRef]
- Bose, H.; Rabindranath, R.; Ehrlich, J. Soft magnetorheological elastomers as new actuators for valves. J. Intell. Mater. Syst. Struct. 2012, 23, 989–994. [Google Scholar] [CrossRef]
- Arief, I.; Mukhopadhyay, P.K. Magnetorheology in CoNi nanoplatelet-based MRFs: Effect of platelet orientation and oscillatory shear. J. Magn. Magn. Mater. 2019, 479, 326–331. [Google Scholar] [CrossRef]
- Arief, I.; Mukhopadhyay, P.K. Two-step yielding in novel CoNi nanoplatelet-based magnetic fluids under oscillatory rheology. Mater. Lett. 2016, 167, 192–196. [Google Scholar] [CrossRef]
- Hu, G.L.; Long, M.; Yu, L.F.; Li, W.H. Design and performance evaluation of a novel magnetorheological valve with a tunable resistance gap. Smart Mater. Struct. 2014, 23, 127001. [Google Scholar] [CrossRef]
- Hu, G.L.; Zhang, J.W.; Zhong, F.; Yu, L.F. Performance evaluation of an improved radial magnetorheological valve and its application in the valve controlled cylinder system. Smart Mater. Struct. 2019, 28, 47003. [Google Scholar] [CrossRef]
- Bai, X.X.; Cai, F.; Chen, P. Resistor-capacitor (RC) operator-based hysteresis model for magnetorheological (MR) dampers. Mech. Syst. Signal Process. 2019, 117, 157–169. [Google Scholar] [CrossRef]
- Hu, G.L.; Yi, F.; Tong, W.; Yu, L.F. Development and evaluation of a MR damper with enhanced effective gap lengths. IEEE Access 2020, 8, 156347–156361. [Google Scholar] [CrossRef]
- Jamadar, M.-E.-H.; Desai, R.M.; Saini, R.S.T.; Kumar, H.; Joladarashi, S. Dynamic analysis of a quarter car model with semi-active seat suspension using a novel model for magneto-rheological (MR) damper. J. Vib. Eng. Technol. 2021, 9, 161–176. [Google Scholar] [CrossRef]
- Sun, S.S.; Ning, D.H.; Yang, J. Development of an MR seat suspension with self-powered generation capability. Smart Mater. Struct. 2017, 26, 085025. [Google Scholar] [CrossRef]
- Yu, Y.; Royel, S.; Li, Y.; Li, J.; Yousefi, A.M.; Gu, X.; Li, S.; Li, H. Dynamic modelling and control of shear-mode rotational MR damper for mitigating hazard vibration of building structures. Smart Mater. Struct. 2020, 29, 114006. [Google Scholar] [CrossRef]
- Andrade, R.M.; Filho, A.B.; Vimieiro, C.B.S.; Pinotti, M. Optimal design and torque control of an active magnetorheological prosthetic knee. Smart Mater. Struct. 2018, 27, 168–176. [Google Scholar] [CrossRef]
- Gao, F.; Liu, Y.; Liao, W. Optimal design of a magnetorheological damper used in smart prosthetic knees. Smart Mater. Struct. 2017, 26, 035034. [Google Scholar] [CrossRef]
- Fu, Q.; Wang, D.H.; Xu, L.; Yuan, G. A magnetorheological damper-based prosthetic knee and sliding mode tracking control method for an MRPK-based lower limb prosthesis. Smart Mater. Struct. 2017, 26, 045030. [Google Scholar] [CrossRef]
- Aydar, G.; Wang, X.J.; Gordaninejad, F. A novel two-way-controllable magneto-rheological fluid damper. Smart Mater. Struct. 2010, 19, 065024. [Google Scholar] [CrossRef]
- Sahin, H.; Gordaninejad, F.; Wang, X.; Liu, Y. Response time of magnetorheological fluids and magnetorheological valves under various flow conditions. J. Intell. Mater. Syst. Struct. 2012, 23, 949–957. [Google Scholar] [CrossRef]
- Hu, G.L.; Liao, M.K.; Li, W.H. Analysis of a compact annular-radial-orifice flow magnetorheological valve and evaluation of its performance. J. Intell. Mater. Syst. Struct. 2017, 28, 1322–1333. [Google Scholar] [CrossRef] [Green Version]
- Hu, G.L.; Zhou, F.; Liao, M.K.; Yu, L.F. Dynamic performance analysis of a compact annular-radial-orifice flow magnetorheological valve and its application in the valve controlled cylinder system. Actuators 2021, 10, 104. [Google Scholar] [CrossRef]
- Imaduddin, F.; Mazlan, S.A.; Zamzuri, H.; Yazid, I.I.M. Design and performance analysis of a compact magnetorheological valve with multiple annular and radial gaps. J. Intell. Mater. Syst. Struct. 2015, 26, 1038–1049. [Google Scholar] [CrossRef]
- Idris, M.H.; Imaduddin, F.; Mazlan, S.A.; Choi, S.B. A concentric design of a bypass magnetorheological fluid damper with a serpentine flux valve. Actuators 2020, 9, 16. [Google Scholar] [CrossRef] [Green Version]
- Bai, X.X.; Wereley, N.M.; Choi, Y.T. Magnetorheological energy absorber with dual concentric annular valves. J. Intell. Mater. Syst. Struct. 2016, 27, 944–958. [Google Scholar] [CrossRef]
- Yang, X.L.; Chen, Y.J.; Liu, Y.T.; Zhang, R.B. Modeling and experiments of an annular multi-channel magnetorheological valve. Actuators 2022, 11, 19. [Google Scholar] [CrossRef]
- Hu, G.L.; Zhong, F.; Zhang, H.Y.; Ding, R.Q. Structure optimization and performance analysis of a multiple radial magnetorheological valve. J. Beijing Inst. Technol. 2017, 26, 458–467. [Google Scholar]
- Nguyen, Q.H.; Choi, S.B.; Lee, Y.S. An analytical method for optimal design of MR valve structures. Smart Mater. Struct. 2009, 18, 095032. [Google Scholar] [CrossRef]
- Nguyen, Q.H.; Choi, S.B. Selection of magnetorheological brake types via optimal design considering maximum torque and constrained volume. Smart Mater. Struct. 2012, 21, 015012. [Google Scholar] [CrossRef]
- Keshav, M.; Chandramohan, S. Geometric optimization of magnetorheological valve using feedforward neural networks for distribution of magnetic flux density inside the valve. Smart Mater. Struct. 2019, 28, 105018. [Google Scholar] [CrossRef]
- Shamieh, H.; Sedaghati, R. Multi-objective design optimization and control of magnetorheological fluid brakes for automotive applications. Smart Mater. Struct. 2017, 26, 125012. [Google Scholar] [CrossRef]
- Naserimojarad, M.M.; Moallem, M.; Arzanpour, S. A comprehensive approach for optimal design of magnetorheological dampers. J. Intell. Mater. Syst. Struct. 2018, 29, 3648–3655. [Google Scholar] [CrossRef]
- Salloom, M.; Samad, Z. Magnetorheological directional control valve. Int. J. Adv. Manuf. Technol. 2012, 58, 279–292. [Google Scholar] [CrossRef]
- Paweł, M.; Sławomir, K.; Arkadiusz, M. Rotary shock-absorber with magnetorheological valves. Adv. Mater. Res. 2013, 628, 505–511. [Google Scholar]
- Kubík, M.; Macháček, O.; Strecker, Z.; Roupec, J.; Mazůrek, I. Design and testing of magnetorheological valve with fast force response time and great dynamic force range. Smart Mater. Struct. 2017, 26, 047002. [Google Scholar] [CrossRef]
- Yoo, J.H.; John, S.; Chaudhuri, A. A magnetorheological actuation system: Test and model. Smart Mater. Struct. 2008, 17, 025023. [Google Scholar]
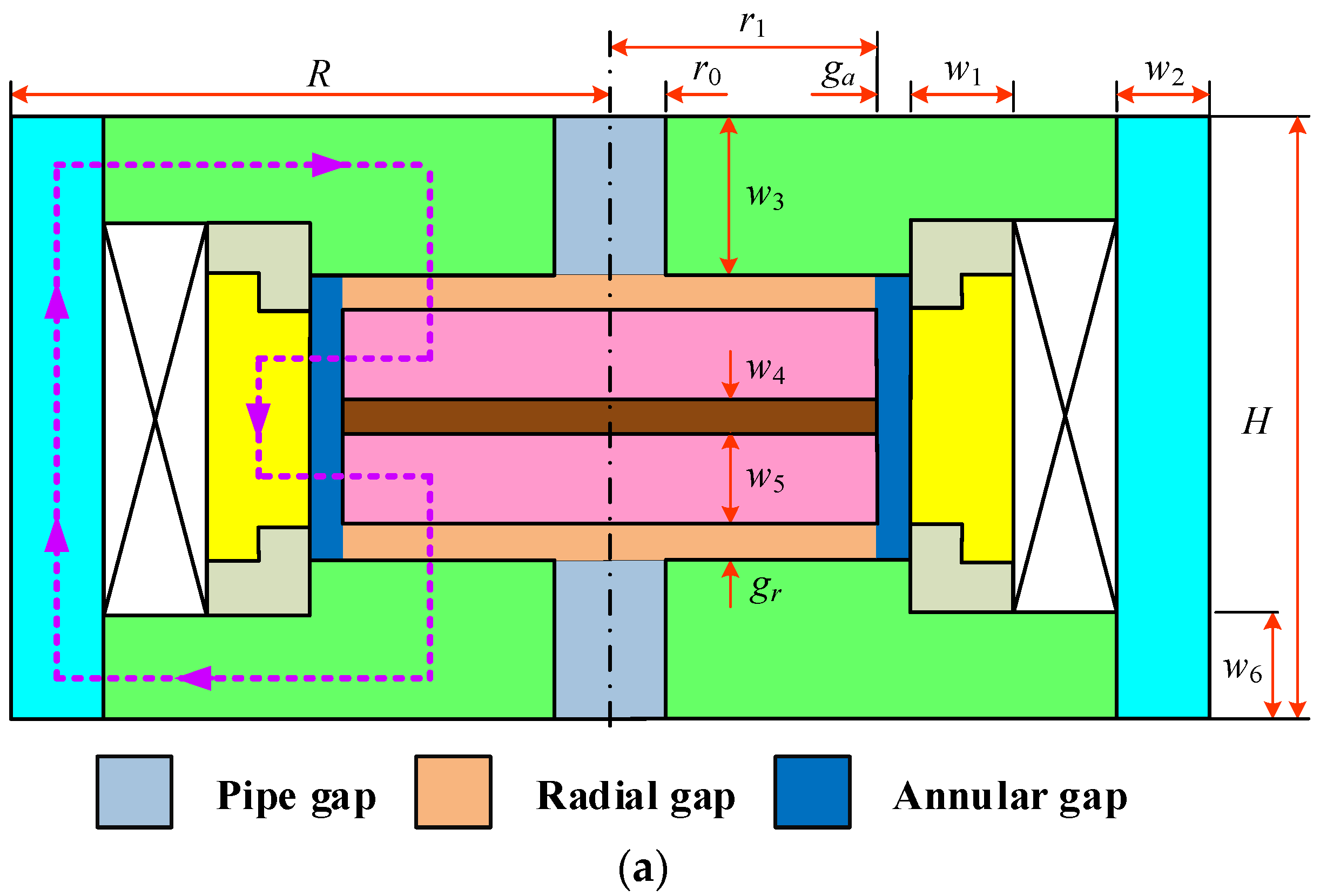
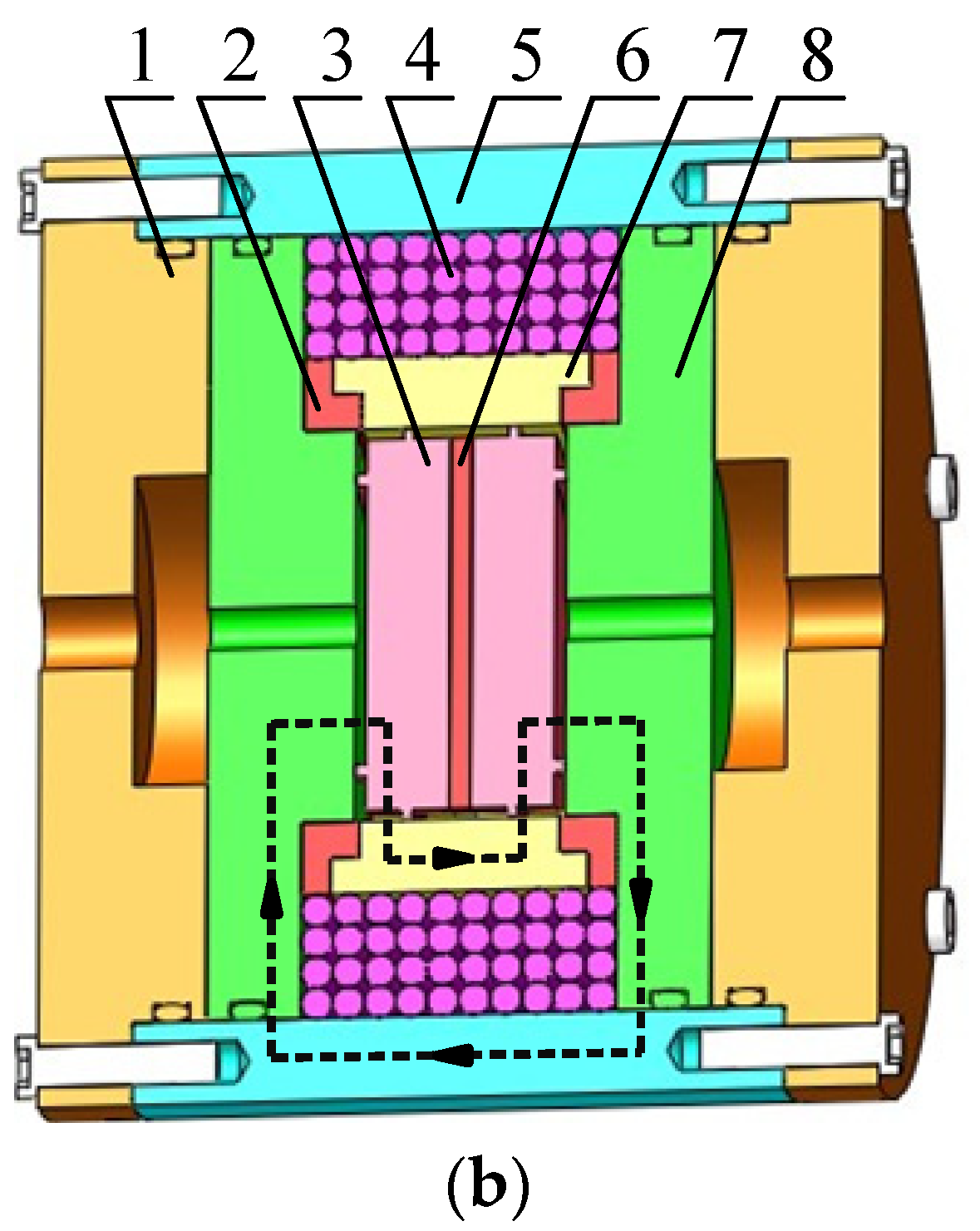
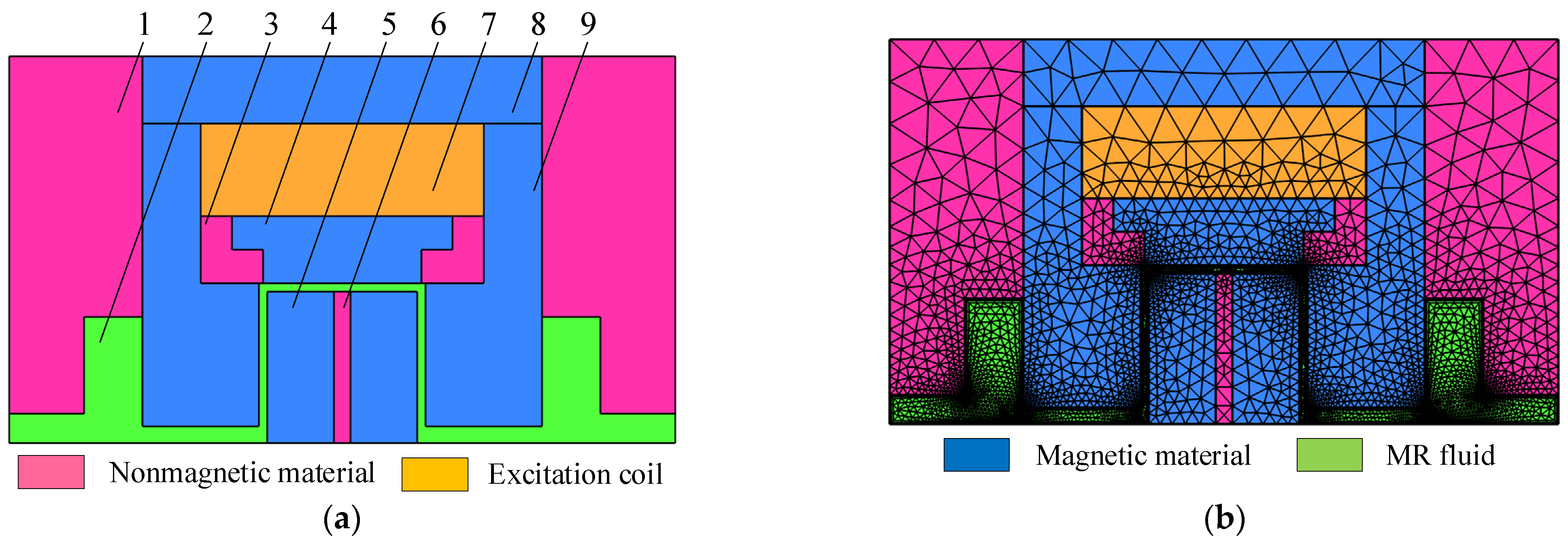








| Parameters | Values (mm) | Parameters | Values (mm) |
|---|---|---|---|
| Radius of center orifice r0 | 2 | Thickness of nonmagnetic disk w4 | 2 |
| Radius of damping disk r1 | 18 | Thickness of damping disk w5 | 8 |
| Radius of valve R | 46 | Width of winding groove w6 | 8 |
| Thickness of magnetic ring w1 | 8 | Radial damping gap gr | 1 |
| Thickness of valve body w2 | 8 | Annular damping gap ga | 1 |
| Thickness of positioning plate w3 | 14 | Length of valve H | 48 |
| Rang | Radial Damping Gap gr (mm) | Thickness of Damping Disk w5 (mm) | Annular Damping Gap ga (mm) | Radius of Damping Disk r1 (mm) |
|---|---|---|---|---|
| Min | 1 | 6 | 1 | 14 |
| Max | 1.5 | 10 | 1.5 | 20 |
| Fitting Index | RMSE | R2 | |||
|---|---|---|---|---|---|
| Model Type | Δp (MPa) | Pw (W) | Δp (MPa) | Pw (W) | |
| Response surface model | First order | 0.071 | 0.019 | 0.994 | 0.927 |
| Second order | 0.014 | 0.002 | 0.995 | 0.999 | |
| Third order | 0.019 | 0.003 | 0.994 | 0.999 | |
| gr (mm) | w5 (mm) | ga (mm) | r1 (mm) | Δp (MPa) | Pw (W) | ||
|---|---|---|---|---|---|---|---|
| Initial | 1 | 8 | 1 | 18 | 4.4 | 27.1 | |
| Optimal | 1 | 8.9 | 1.1 | 19.6 | 4.84 | 15.3 | |
| Current (A) | Rising Response Time (ms) | Falling Response time (ms) | ||
|---|---|---|---|---|
| Initial | Optimal | Initial | Optimal | |
| 0.4 | 150 | 160 | 191 | 202 |
| 0.8 | 106 | 102 | 161 | 156 |
| 1.2 | 93 | 82 | 120 | 100 |
| 1.6 | 87 | 76 | 102 | 86 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, J.; Zhou, F.; Wang, N.; Hu, G. Multi-Objective Optimization Design and Dynamic Performance Analysis of an Enhanced Radial Magnetorheological Valve with Both Annular and Radial Flow Paths. Actuators 2022, 11, 120. https://doi.org/10.3390/act11050120
Ren J, Zhou F, Wang N, Hu G. Multi-Objective Optimization Design and Dynamic Performance Analysis of an Enhanced Radial Magnetorheological Valve with Both Annular and Radial Flow Paths. Actuators. 2022; 11(5):120. https://doi.org/10.3390/act11050120
Chicago/Turabian StyleRen, Jiwen, Feng Zhou, Naibin Wang, and Guoliang Hu. 2022. "Multi-Objective Optimization Design and Dynamic Performance Analysis of an Enhanced Radial Magnetorheological Valve with Both Annular and Radial Flow Paths" Actuators 11, no. 5: 120. https://doi.org/10.3390/act11050120
APA StyleRen, J., Zhou, F., Wang, N., & Hu, G. (2022). Multi-Objective Optimization Design and Dynamic Performance Analysis of an Enhanced Radial Magnetorheological Valve with Both Annular and Radial Flow Paths. Actuators, 11(5), 120. https://doi.org/10.3390/act11050120






