High-Frequency Position Servo Control of Hydraulic Actuator with Valve Dynamic Compensation
Abstract
:1. Introduction
2. Valve Dynamics Compensation Strategy
- (1)
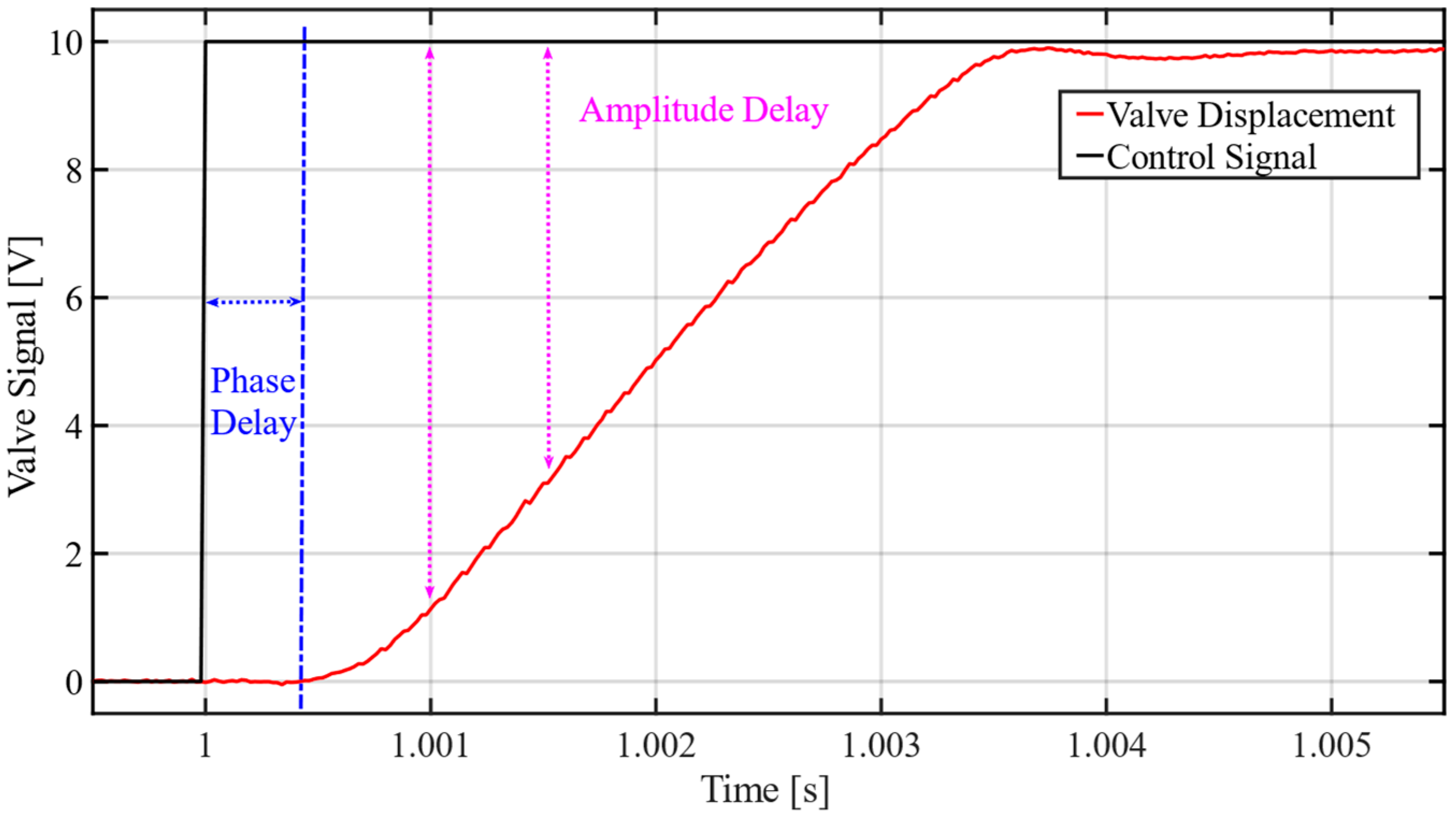
- The proportional valve dynamics are innovatively decoupled into phase delay and amplitude delay. Therefore, proportional valve dynamics can be compensated by phase delay compensation and amplitude delay compensation, respectively.
- (2)
- Proportional valve dynamics are compensated in a backstepping controller without increasing system order. Valve phase delay can be compensated by trajectory transformation strategy because the desired engine lifts are known in advance. Valve amplitude delay can be compensated by feedback of integral flow. The paper innovatively proposed feedback of integral flow instead of instantaneous flow. Compared with gain of instantaneous flow error, integral flow error gain is smaller, which can achieve smaller tracking errors. The experiment results in Section 5 can verify this conclusion.
3. Problem Statement and System Modeling
4. Controller Design
4.1. Desired Engine Valve Lifts Transformation
4.2. Backstepping Controller Design with Integral Flow Error Feedback
4.3. Stability Analysis
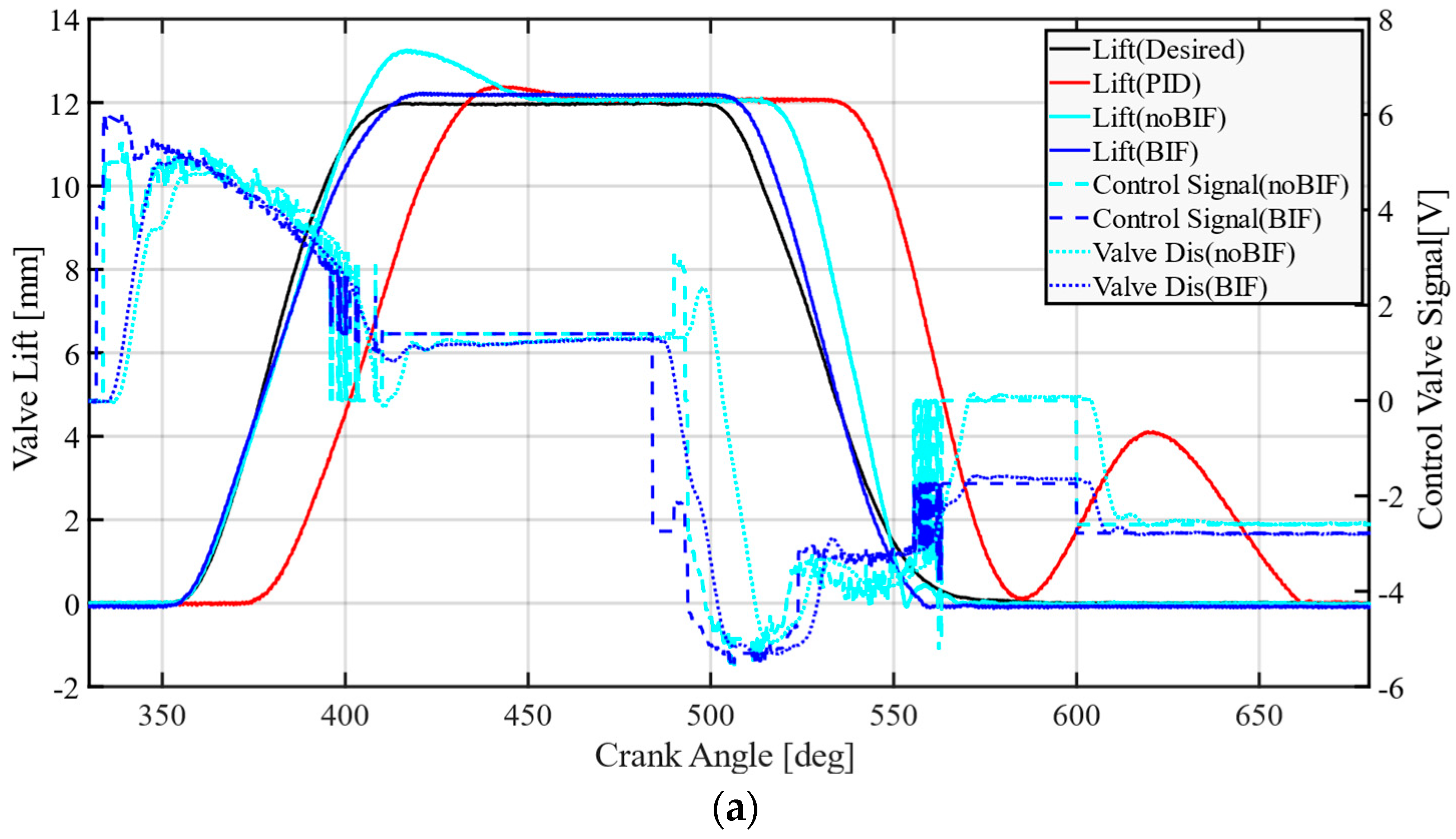
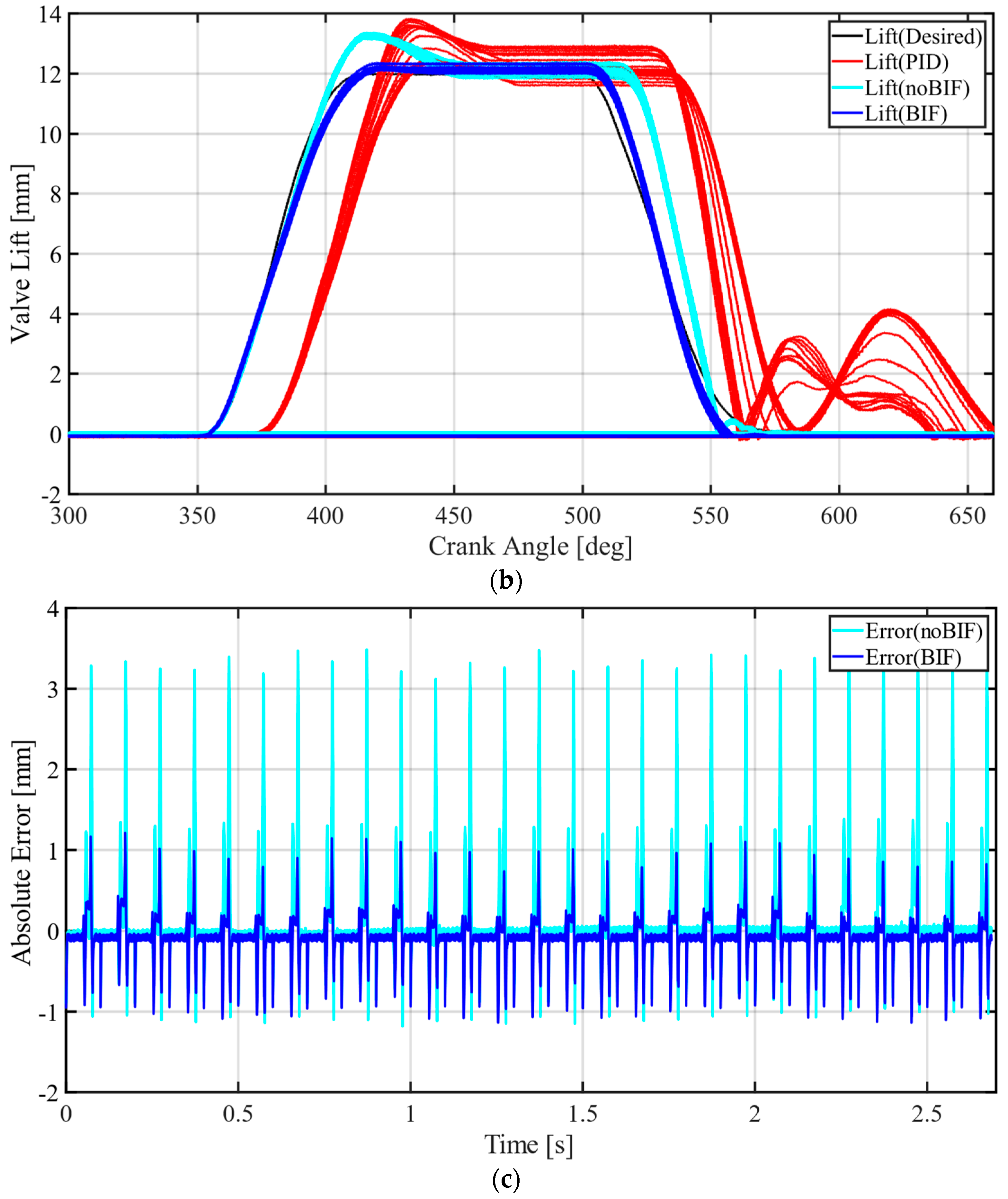
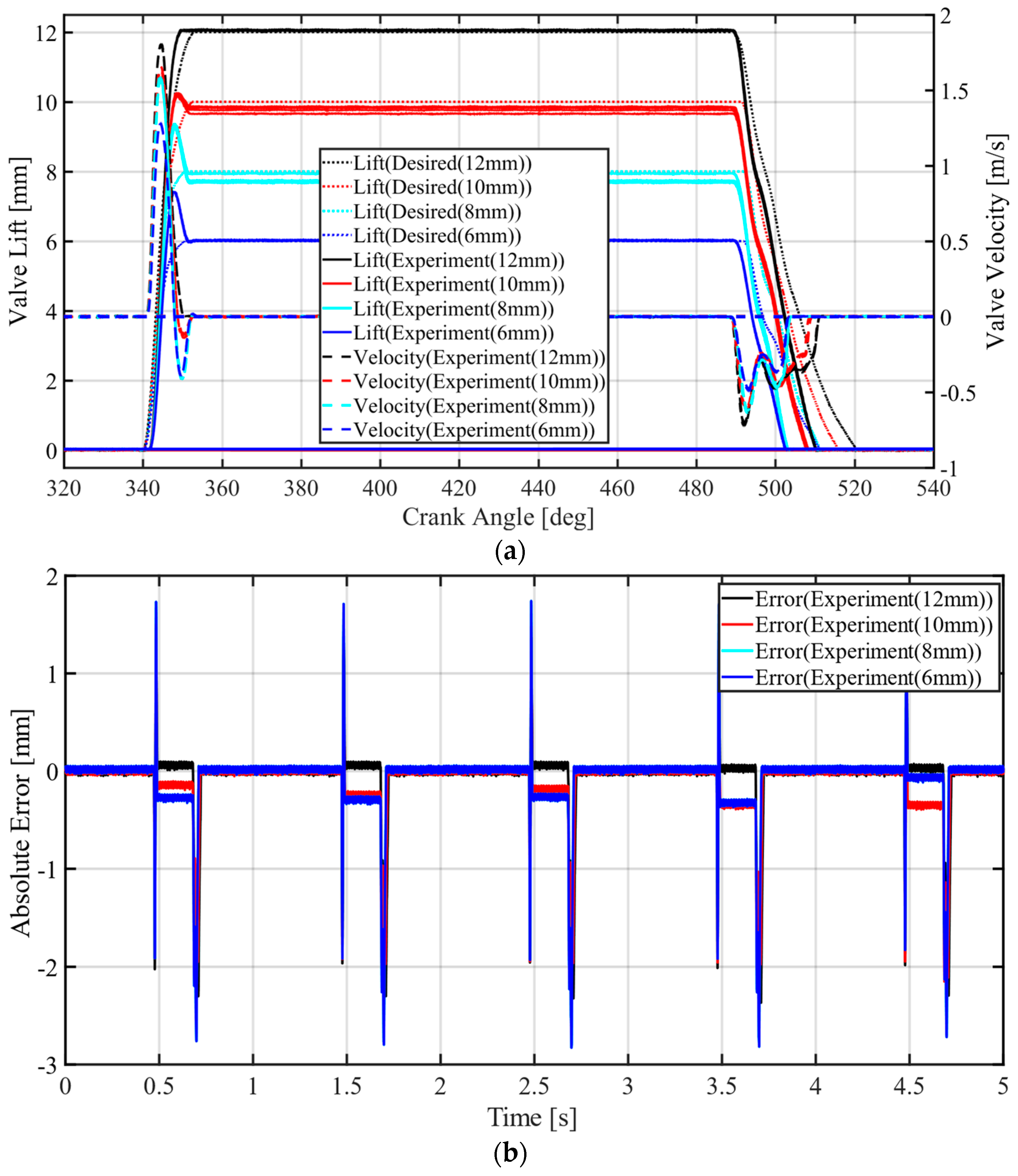
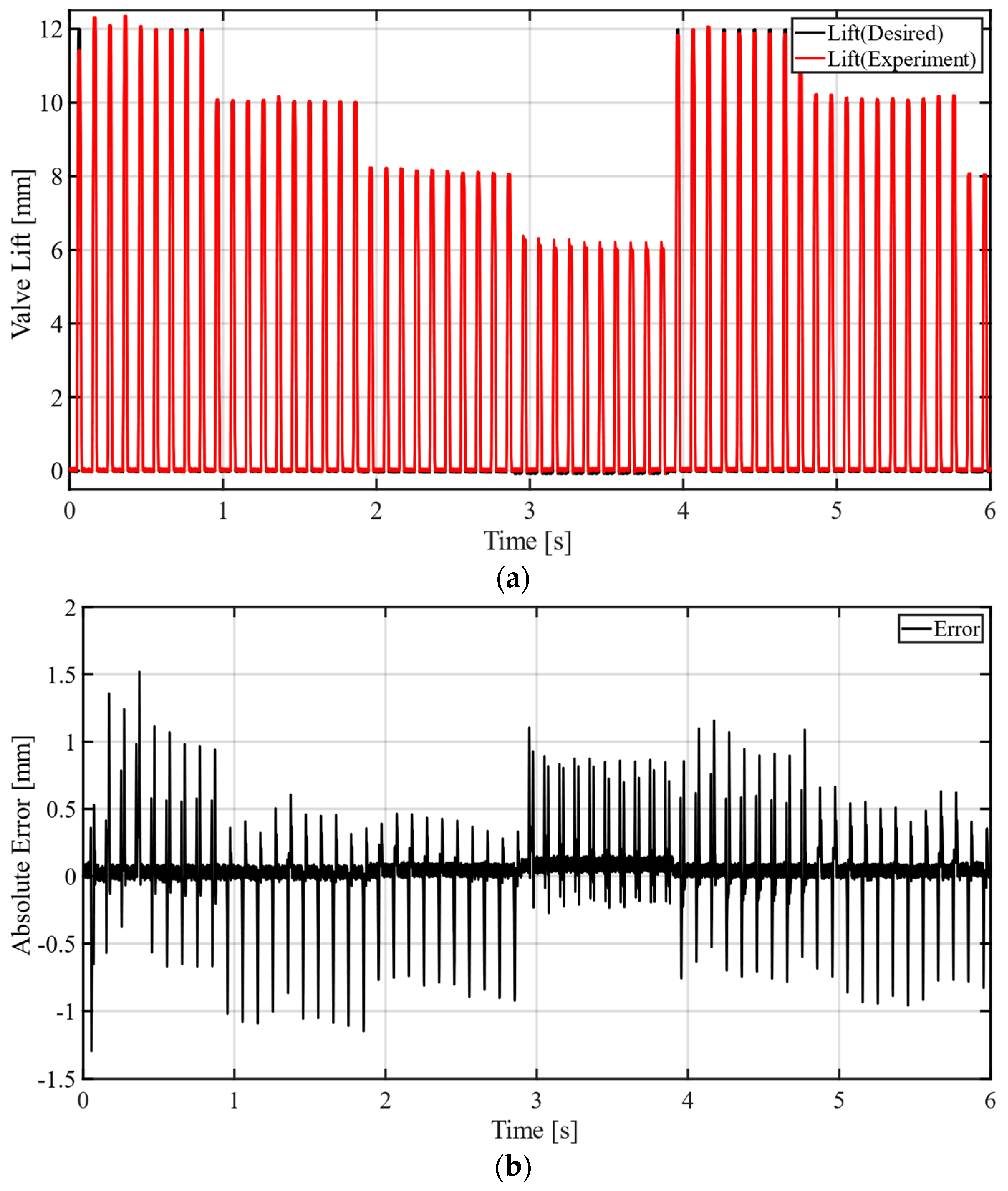
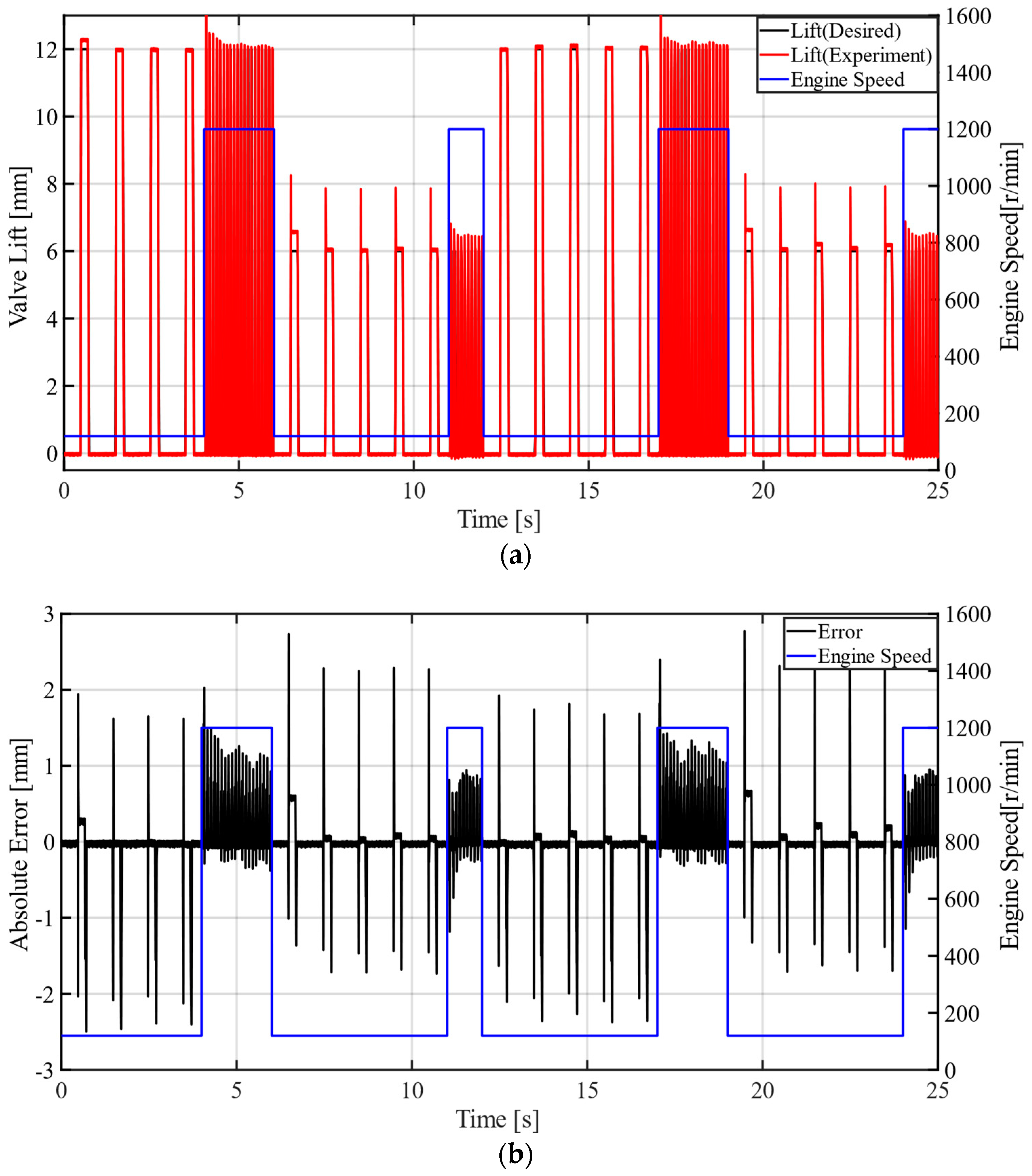
5. Experiment Results and Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| mass of all movement parts | |
| acceleration of valve | |
| pressures of Chamber B | |
| pressures of Chamber C | |
| pressures of Chamber D | |
| effective action areas of Chamber B | |
| effective action areas of Chamber C | |
| effective action areas of Chamber D | |
| friction force of the system | |
| spring force of the engine valve | |
| flow rate into Chamber B | |
| flow rate into Chamber C | |
| flow rate leaked from Chamber C to Chamber B | |
| flow rate leaked from Chamber C to Chamber D | |
| flow rate into Chamber D | |
| elasticity modulus of oil | |
| engine valve velocity | |
| equivalent flow coefficient of oil port in Chamber B | |
| effective flow areas of oil port in Chamber B | |
| equivalent flow coefficient of proportional valve | |
| area gain coefficient of proportional valve | |
| real valve openings of proportional valve | |
| proportional valve displacement | |
| oil supply pressure | |
| equivalent flow coefficient of oil port in Chamber D | |
| effective flow areas of oil port in Chamber D | |
| leakage coefficient leaked from Chamber C to B | |
| leakage coefficient leaked from Chamber C to D | |
| bristle direction coefficient | |
| deformation coefficient of seals | |
| bristle stiffness | |
| damping coefficient of bristle | |
| viscous damping coefficient | |
| bristle displacement | |
| bristle velocity | |
| engine valve velocity | |
| desired valve lift before tuning | |
| desired valve lift after tuning | |
| valve lift error | |
| valve velocity error | |
| error of and | |
| virtual desired valve velocity | |
| virtual control law of | |
| feedforward controller in step 1 | |
| linear feedback to | |
| unmatched uncertainty compensation term in step 1 | |
| feedforward controller in step 2 | |
| linear feedback to | |
| unmatched uncertainty compensation term in step 2 | |
| linear feedback of | |
| integral flow error |
References
- Kock, F.; Ferrari, C. Flatness-Based High Frequency Control of a Hydraulic Actuator. J. Dyn. Syst. Meas. Control 2012, 134, 021003. [Google Scholar] [CrossRef] [Green Version]
- Raghuraman, D.R.S.; Kumar, S.S.; Kalaiarasan, G. Simulation of proportional control of hydraulic actuator using digital hydraulic valves. IOP Conf. Ser. Mater. Sci. Eng. 2017, 263, 62080. [Google Scholar] [CrossRef]
- Yousef, M.A.; Rabie, M.G.; Rateb, R.A. System Identification and Controller Design for a Typical Electro-Hydraulic Servo Motor. In Proceedings of the 2021 International Telecommunications Conference (ITC-Egypt), Alexandria, Egypt, 13–15 July 2021; pp. 1–4. [Google Scholar]
- Abagnale, C.; Aggogeri, F.; Borboni, A.; Strano, S.; Terzo, M. Dead-zone effect on the performance of state estimators for hydraulic actuators. Meccanica 2016, 52, 2189–2199. [Google Scholar] [CrossRef]
- Strano, S.; Terzo, M. Riccati Equation Based Nonlinear Filter: A Case Study for Hydraulic Actuators in the Presence of Dead-Zone. In Advances in Italian Mechanism Science; Springer: Cham, Switzerland, 2017; pp. 145–152. [Google Scholar]
- Lampinen, S.; Koivumäki, J.; Mattila, J.; Niemi, J. Model-Based Control of a Pressure-Compensated Directional Valve with Significant Dead-Zone. In Fluid Power Systems Technology; American Society of Mechanical Engineers: New York, NY, USA, 2019. [Google Scholar]
- Meng, F.; Ren, Y.; Xi, J. Time Delay Characteristics Analysis of Pressure Dynamic Response on Electro-hydraulic Pressure Regulating valve. In Proceedings of the 2021 4th IEEE International Conference on Industrial Cyber-Physical Systems (ICPS), Victoria, BC, Canada, 10–121 May 2021; pp. 761–766. [Google Scholar]
- Sung, H.-J.; Lee, J.-H.; Min, H.-K.; Park, M.-K. Robust hydraulic position control based on uncertainty and disturbance estimation. In Proceedings of the 2017 17th International Conference on Control, Automation and Systems (ICCAS), Ramada Plaza, Korea, 18–21 October 2017; pp. 1132–1136. [Google Scholar]
- Jin, L.; Wang, Q. Accurate model identification of the inertial mass dynamic of hydraulic cylinder with model uncertainty. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 2018, 233, 501–510. [Google Scholar] [CrossRef]
- Nguyen, M.H.; Dao, H.V.; Ahn, K.K. Active Disturbance Rejection Control for Position Tracking of Electro-Hydraulic Servo Systems under Modeling Uncertainty and External Load. Actuators 2021, 10, 20. [Google Scholar] [CrossRef]
- Wen, Y.; Tan, J.; Sang, Y.; Zhou, S. Research on fuzzy symmetrical control of valve controlled asymmetric hydraulic cylinder system. J. Intell. Fuzzy Syst. 2021, 41, 4451–4460. [Google Scholar] [CrossRef]
- Esatoglu, A.; Salamci, M.U. Sliding Mode Control Design for a Two-Stage of Electro-Hydraulic Valve. In Proceedings of the 3rd International Conference on Vision, Image and Signal Processing, Vancouver, BC, Canada, 26–28 August 2019; pp. 1–6. [Google Scholar]
- Lee, I.; Iwan, I.; Choi, S.; Huh, J. Performance Improvement of Electro-Hydraulic Servo Valve Using a Feed-Forward Control and an Input Shaping Filter. In Fluid Applications and Systems; American Society of Mechanical Engineers: New York, NY, USA, 2019. [Google Scholar]
- Kaddissi, C.; Kenne, J.-P.; Saad, M. Indirect Adaptive Control of an Electro-Hydraulic Servo System Based on Nonlinear Backstepping. In Proceedings of the 2006 IEEE International Symposium on Industrial Electronics, Montreal, QC, Canada, 9–13 July 2006; pp. 3147–3153. [Google Scholar]
- Tian, L.; Qian, L.; Chen, L.; Zhang, W. Sliding mode control based on backstepping method for electro-hydraulic single-rod actuator. In Proceedings of the 2015 IEEE International Conference on Information and Automation, Lijiang, China, 8–10 August 2015; pp. 2326–2329. [Google Scholar]
- Nakkarat, P.; Kuntanapreeda, S. Observer-based backstepping force control of an electrohydraulic actuator. Control Eng. Pract. 2009, 17, 895–902. [Google Scholar] [CrossRef]
- Ruderman, M. Full- and reduced-order model of hydraulic cylinder for motion control. In Proceedings of the IECON 2017—43rd Annual Conference of the IEEE Industrial Electronics Society, Beijing, China, 29 October–1 November 2017; pp. 7275–7280. [Google Scholar]
- Wang, C.; Quan, L.; Zhang, S.; Meng, H.; Lan, Y. Reduced-order model based active disturbance rejection control of hydraulic servo system with singular value perturbation theory. ISA Trans. 2017, 67, 455–465. [Google Scholar] [CrossRef] [PubMed]
- Ba, D.X.; Ahn, K.K.; Truong, D.Q.; Park, H.G. Integrated model-based backstepping control for an electro-hydraulic system. Int. J. Precis. Eng. Manuf. 2016, 17, 565–577. [Google Scholar] [CrossRef]
- Swaroop, D.; Gerdes, J.C.; Yip, P.P.; Hedrick, J.K. Dynamic surface control of nonlinear systems. In Proceedings of the 1997 American Control Conference (Cat. No.97CH36041), Albuquerque, NM, USA, 6 June 1997; Volume 3025, pp. 3028–3034. [Google Scholar]
- Pang, H.; Zhang, X.; Yang, J.; Shang, Y. Adaptive backstepping—Based control design for uncertain nonlinear active suspension system with input delay. Int. J. Robust Nonlinear Control 2019, 29, 5781–5800. [Google Scholar] [CrossRef]
- Yao, B.; Bu, F.; Chiu, G.T.C. Non-linear adaptive robust control of electro-hydraulic systems driven by double-rod actuators. Int. J. Control 2001, 74, 761–775. [Google Scholar] [CrossRef]
- Ren, H.-P.; Gong, P.-F.; Li, J. Pneumatic position servo control considering the proportional valve zero point. In Proceedings of the 2017 IEEE International Conference on Mechatronics (ICM), Churchill, Australia, 13–15 February 2017; pp. 166–171. [Google Scholar]
- Wang, C.; Ji, X.; Zhang, Z.; Zhao, B.; Quan, L.; Plummer, A.R. Tracking differentiator based back-stepping control for valve-controlled hydraulic actuator system. ISA Trans. 2022, 119, 208–220. [Google Scholar] [CrossRef]
- Deng, W.; Yao, J. Extended-State-Observer-Based Adaptive Control of Electrohydraulic Servomechanisms Without Velocity Measurement. IEEE/ASME Trans. Mechatron. 2020, 25, 1151–1161. [Google Scholar] [CrossRef]
- Bakhshande, F.; Söffker, D. Proportional-Integral-Observer-Based Backstepping Approach for Position Control of a Hydraulic Differential Cylinder System with Model Uncertainties and Disturbances. J. Dyn. Syst. Meas. Control 2018, 140, 121006. [Google Scholar] [CrossRef]
- Li, H.; Zhu, G.; Huang, Y. Adaptive feedforward control of an electro-hydraulic variable valve actuator for internal combustion engines. In Proceedings of the 2017 IEEE 56th Annual Conference on Decision and Control (CDC), Melbourne, Australia, 12–15 December 2017; pp. 879–884. [Google Scholar]
- Schmidt, L.; Andersen, T.O.; Pedersen, H.C.; Bech, M.M. Robust Position Tracking for Electro-Hydraulic Drives Based on Generalized Feedforward Compensation Approach. Appl. Mech. Mater. 2012, 233, 100–103. [Google Scholar] [CrossRef]
- Ding, X.; Shen, G.; Li, X.; Tang, Y. Delay compensation position tracking control of electro-hydraulic servo systems based on a delay observer. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 2019, 234, 622–633. [Google Scholar] [CrossRef]
- Cao, B.; Nie, X.; Wu, Z.; Xue, C.; Cao, J. Adaptive neural network control for nonstrict-feedback uncertain nonlinear systems with input delay and asymmetric time-varying state constraints. J. Frankl. Inst. 2021, 358, 7073–7095. [Google Scholar] [CrossRef]
- Won, D.; Kim, W.; Tomizuka, M. Nonlinear Control with High-Gain Extended State Observer for Position Tracking of Electro-Hydraulic Systems. IEEE/ASME Trans. Mechatron. 2020, 25, 2610–2621. [Google Scholar] [CrossRef]
- Guo, Q.; Yu, T.; Jiang, D. High-gain observer-based output feedback control of single-rod electro-hydraulic actuator. IET Control Theory Appl. 2015, 9, 2395–2404. [Google Scholar] [CrossRef]
- Du, H.; Shi, J.; Chen, J.; Zhang, Z.; Feng, X. High-gain observer-based integral sliding mode tracking control for heavy vehicle electro-hydraulic servo steering systems. Mechatronics 2021, 74, 102484. [Google Scholar] [CrossRef]
- Won, D.; Kim, W.; Shin, D.; Chung, C.C. High-Gain Disturbance Observer-Based Backstepping Control with Output Tracking Error Constraint for Electro-Hydraulic Systems. IEEE Trans. Control Syst. Technol. 2015, 23, 787–795. [Google Scholar] [CrossRef]
- Xu, G.; Jia, M.; Li, Y.; Chang, Y.; Wang, T. Potential of reactivity controlled compression ignition (RCCI) combustion coupled with variable valve timing (VVT) strategy for meeting Euro 6 emission regulations and high fuel efficiency in a heavy-duty diesel engine. Energy Convers. Manag. 2018, 171, 683–698. [Google Scholar] [CrossRef]
- Sawant, P.; Bari, S. Effects of Variable Intake Valve Timings and Valve Lift on the Performance and Fuel Efficiency of an Internal Combustion Engine; SAE Technical Paper Series; SAE International: Warrendale, PA, USA, 2018. [Google Scholar]
- Lu, Y.; Li, J.; Xiong, L.; Li, B. Simulation and Experimental Study of a Diesel Engine Based on an Electro-Hydraulic FVVA System Optimization. J. Energy Resour. Technol. 2020, 142, 032204. [Google Scholar] [CrossRef]
- Poonia, S.; Singh, A.; Kumar, N.; Singh, J.; Sharma, S. Valve Opening and Closing Event Finalization for Cost Effective Valve Train of Gasoline Engine; SAE Technical Paper Series; SAE International: Warrendale, PA, USA, 2019. [Google Scholar]
- Lu, Y.; Li, J.; He, F.; Miao, L. Global Synchronous Optimization Including Intake Valves and Exhaust Valves Based on MODM Strategy. IEEE Trans. Veh. Technol. 2020, 69, 14858–14868. [Google Scholar] [CrossRef]
- Lu, Y.; Olsen, D.B. Optimization Method and Simulation Study of a Diesel Engine Using Full Variable Valve Motions. J. Eng. Gas Turbines Power 2017, 139, 072804. [Google Scholar] [CrossRef]
- Jingqing, H. From PID technique to active disturbances rejection control technique. Control Eng. China 2002, 9, 13–18. [Google Scholar] [CrossRef]
- Cao, Y.; Fu, Z.; Zhang, M.; Huang, J. Extended-State-Observer-Based Super Twisting Control for Pneumatic Muscle Actuators. Actuators 2021, 10, 35. [Google Scholar] [CrossRef]
- Bin, Y.; Fanping, B.; Reedy, J.; Chiu, G.T.C. Adaptive robust motion control of single-rod hydraulic actuators: Theory and experiments. IEEE/ASME Trans. Mechatron. 2000, 5, 79–91. [Google Scholar] [CrossRef]
- Deng, W.; Yao, J.; Ma, D. Robust adaptive precision motion control of hydraulic actuators with valve dead-zone compensation. ISA Trans. 2017, 70, 269–278. [Google Scholar] [CrossRef]




| Parameters (Units) | Value | Parameters (Units) | Value |
|---|---|---|---|
| 0.17 | 1 × 10−7 | ||
| 1.7 × 109 | 2 × 10−7 | ||
| (m2) | 6.362 × 10−5 | 12.489 | |
| (m2) | 9.0321 × 10−5 | 5 × 10−13 | |
| (m2) | 1.0367 × 10−4 | 5 × 10−13 | |
| (m3) | 1.2 × 10−5 | (N/m) | 400,000 |
| (m3) | 1.3 × 10−4 | (N/m/s) | 300 |
| (m3) | 1.8 × 10−5 | (N/m/s) | 10 |
| 2 × 10−7 |
| Parameters (Units) | Value | Parameters (Units) | Value |
|---|---|---|---|
| 1 | 1 | ||
| 2.55 | −2.5 | ||
| 0.2 |
| Parameters (Units) | Value | Parameters (Units) | Value |
|---|---|---|---|
| 100 | 10 | ||
| 50 | 10 | ||
| 50 | 1 |
| Parameters (Units) | Value | Parameters (Units) | Value |
|---|---|---|---|
| 300 | 20 | ||
| 100 | 20 | ||
| 100 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Lu, Y.; He, F.; Miao, L. High-Frequency Position Servo Control of Hydraulic Actuator with Valve Dynamic Compensation. Actuators 2022, 11, 96. https://doi.org/10.3390/act11030096
Li J, Lu Y, He F, Miao L. High-Frequency Position Servo Control of Hydraulic Actuator with Valve Dynamic Compensation. Actuators. 2022; 11(3):96. https://doi.org/10.3390/act11030096
Chicago/Turabian StyleLi, Jian, Yong Lu, Fengshuo He, and Lixian Miao. 2022. "High-Frequency Position Servo Control of Hydraulic Actuator with Valve Dynamic Compensation" Actuators 11, no. 3: 96. https://doi.org/10.3390/act11030096
APA StyleLi, J., Lu, Y., He, F., & Miao, L. (2022). High-Frequency Position Servo Control of Hydraulic Actuator with Valve Dynamic Compensation. Actuators, 11(3), 96. https://doi.org/10.3390/act11030096






