Two-Phase Stator Vibration Amplitude Compensation of Traveling-Wave Ultrasonic Motor
Abstract
:1. Introduction
2. Theoretical Analysis
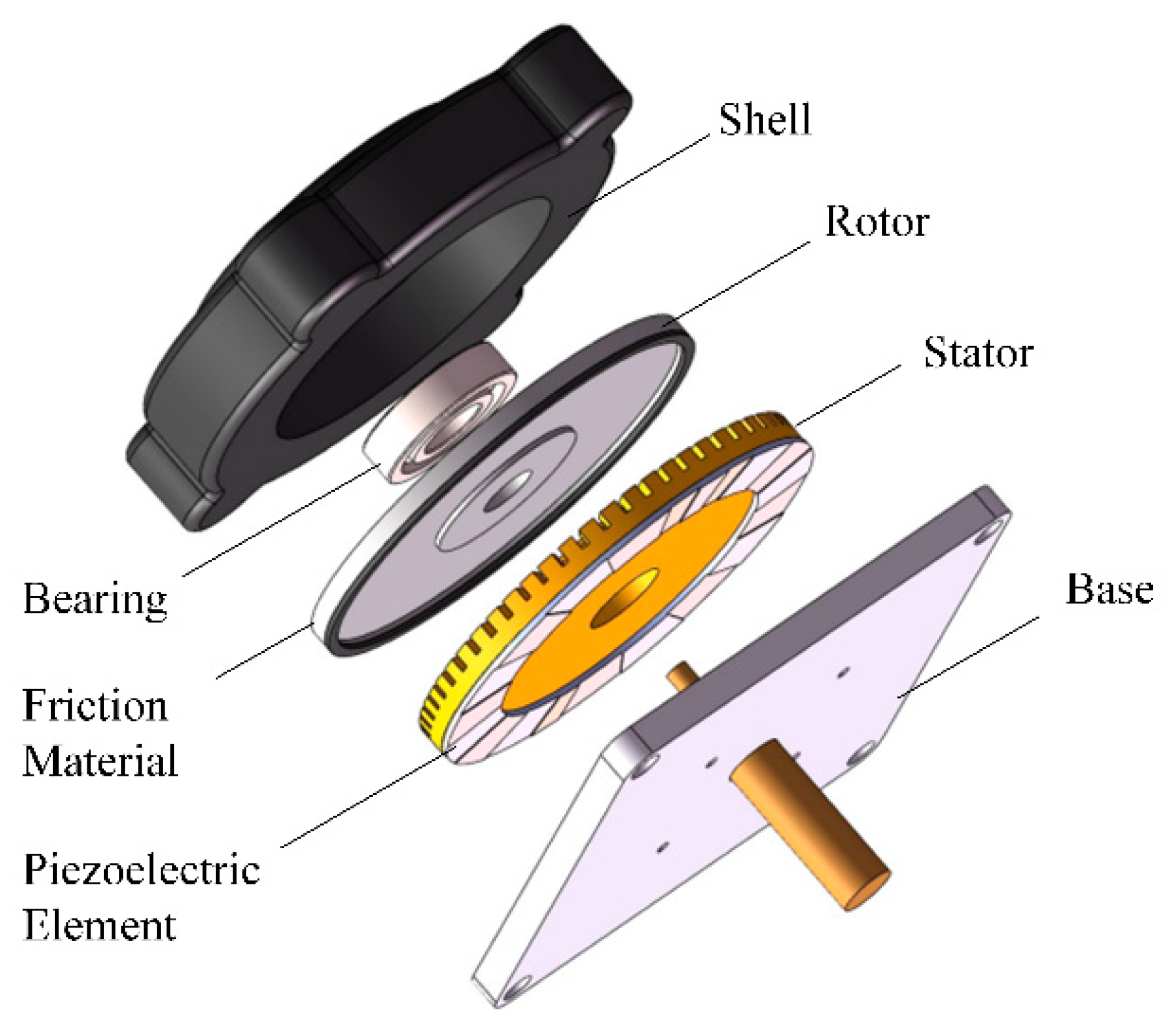
2.1. Structure and Operating Mechanism of TRUSM
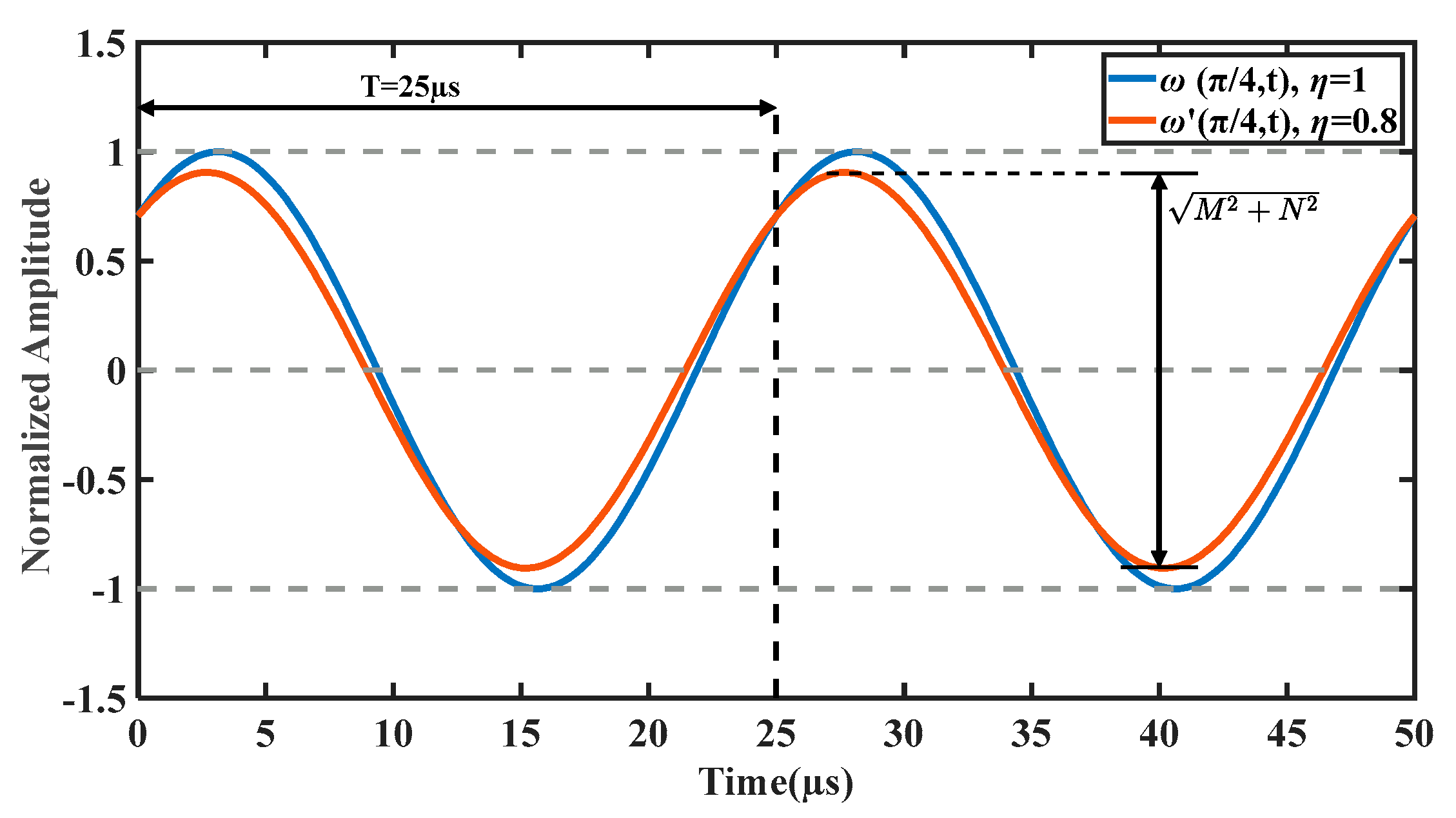
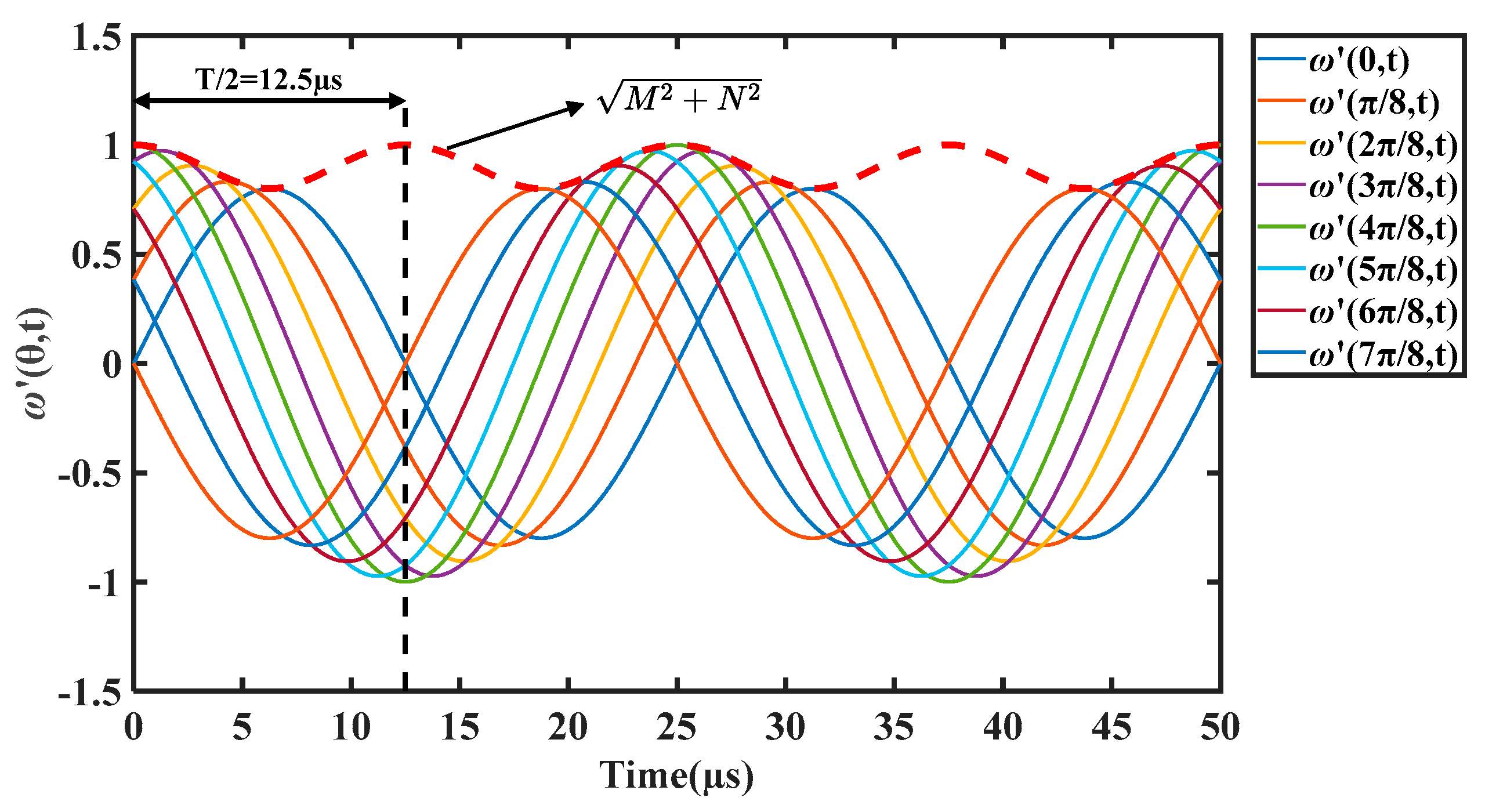
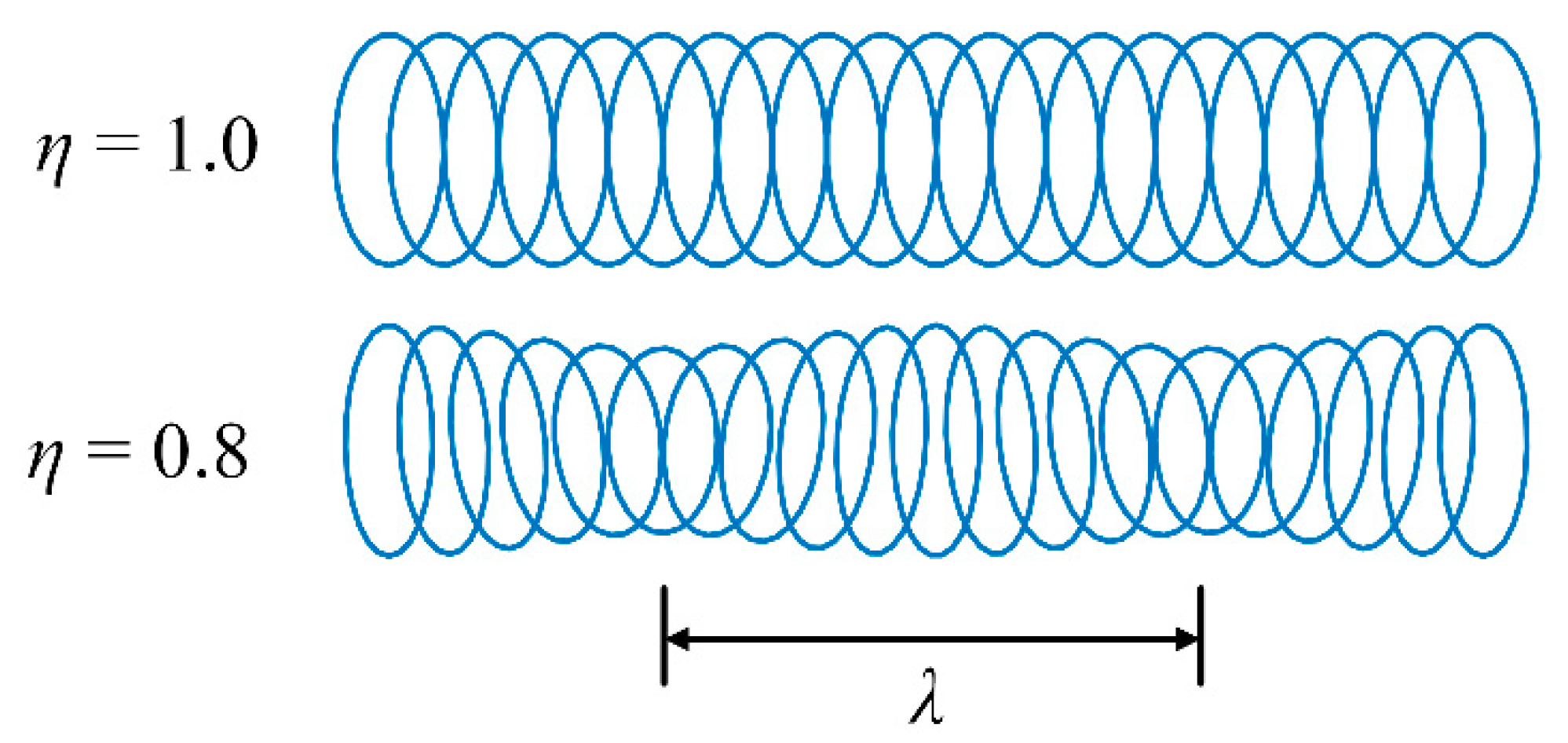
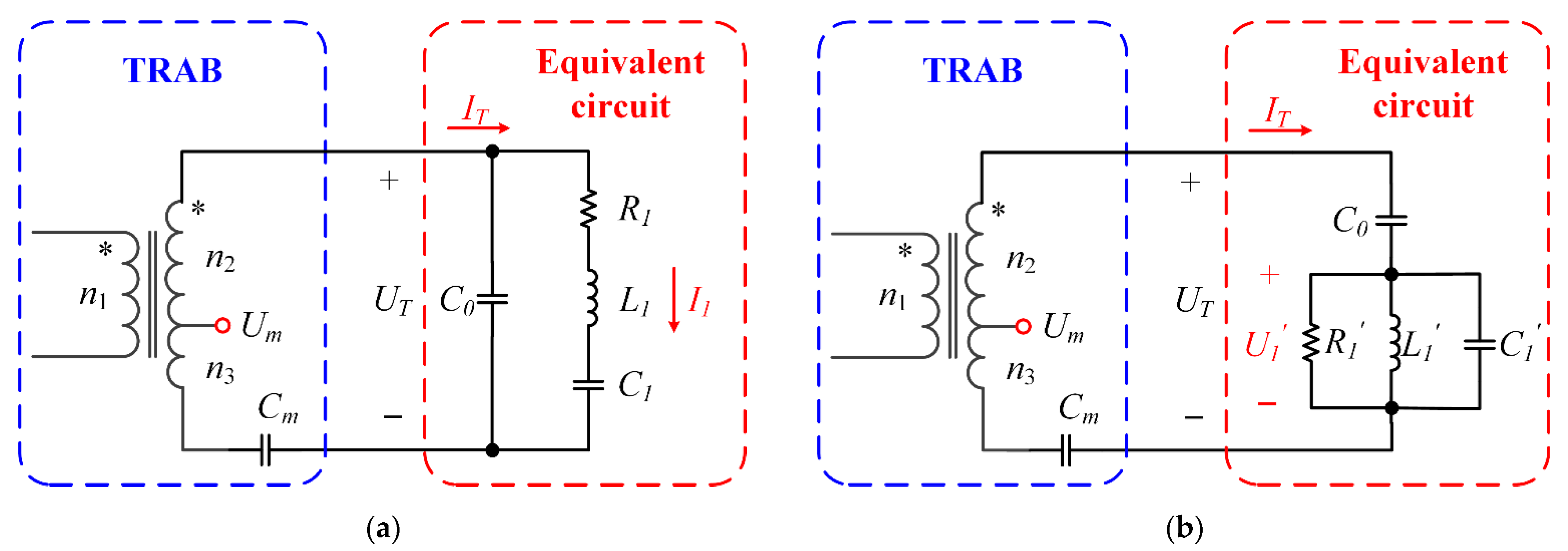
2.2. Influence of Two-Phase Standing Wave Amplitude Difference on Stator’s Surface Particle Motion
3. Implementation of the Proposed Scheme
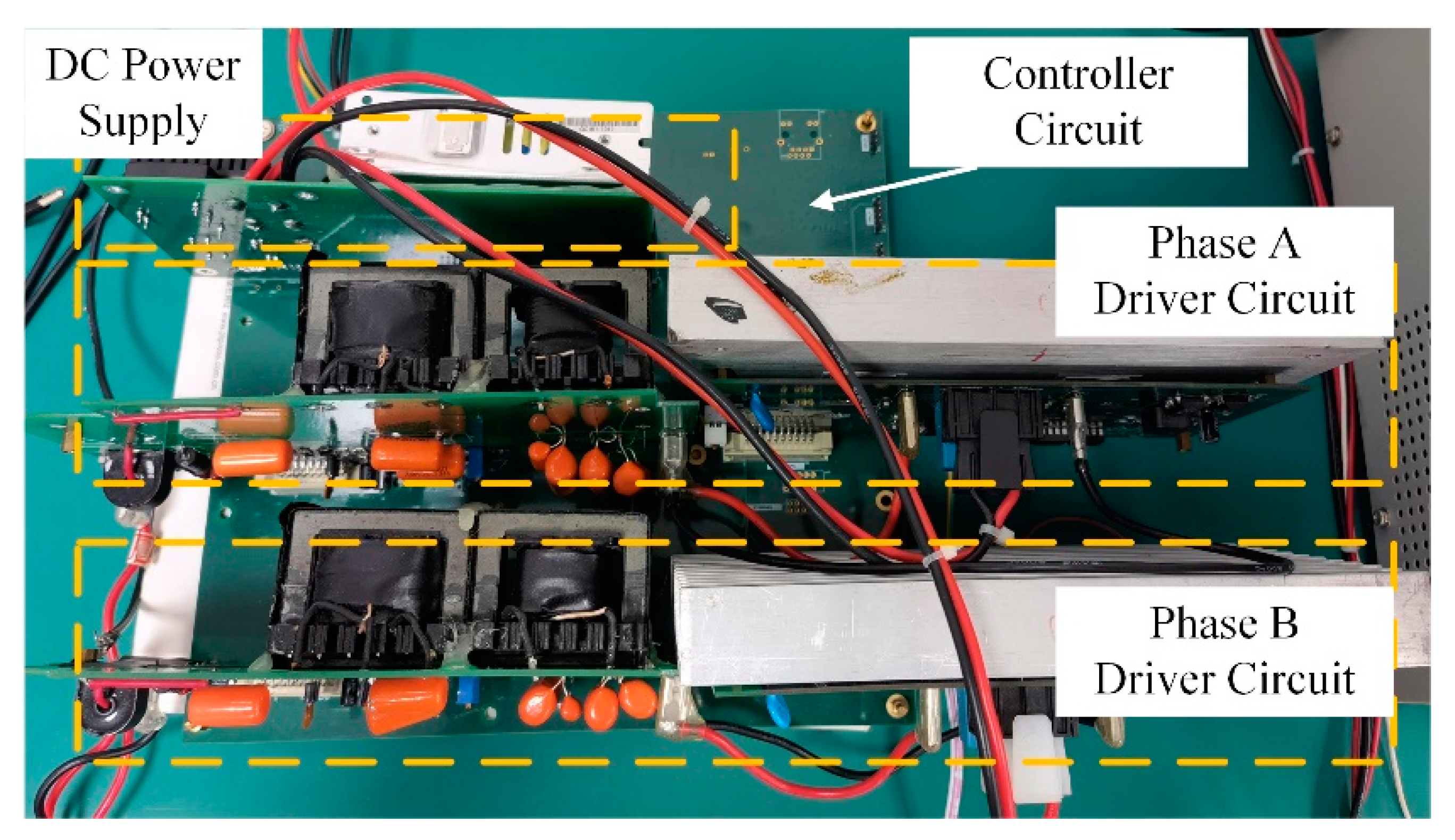
3.1. Hardware Structure
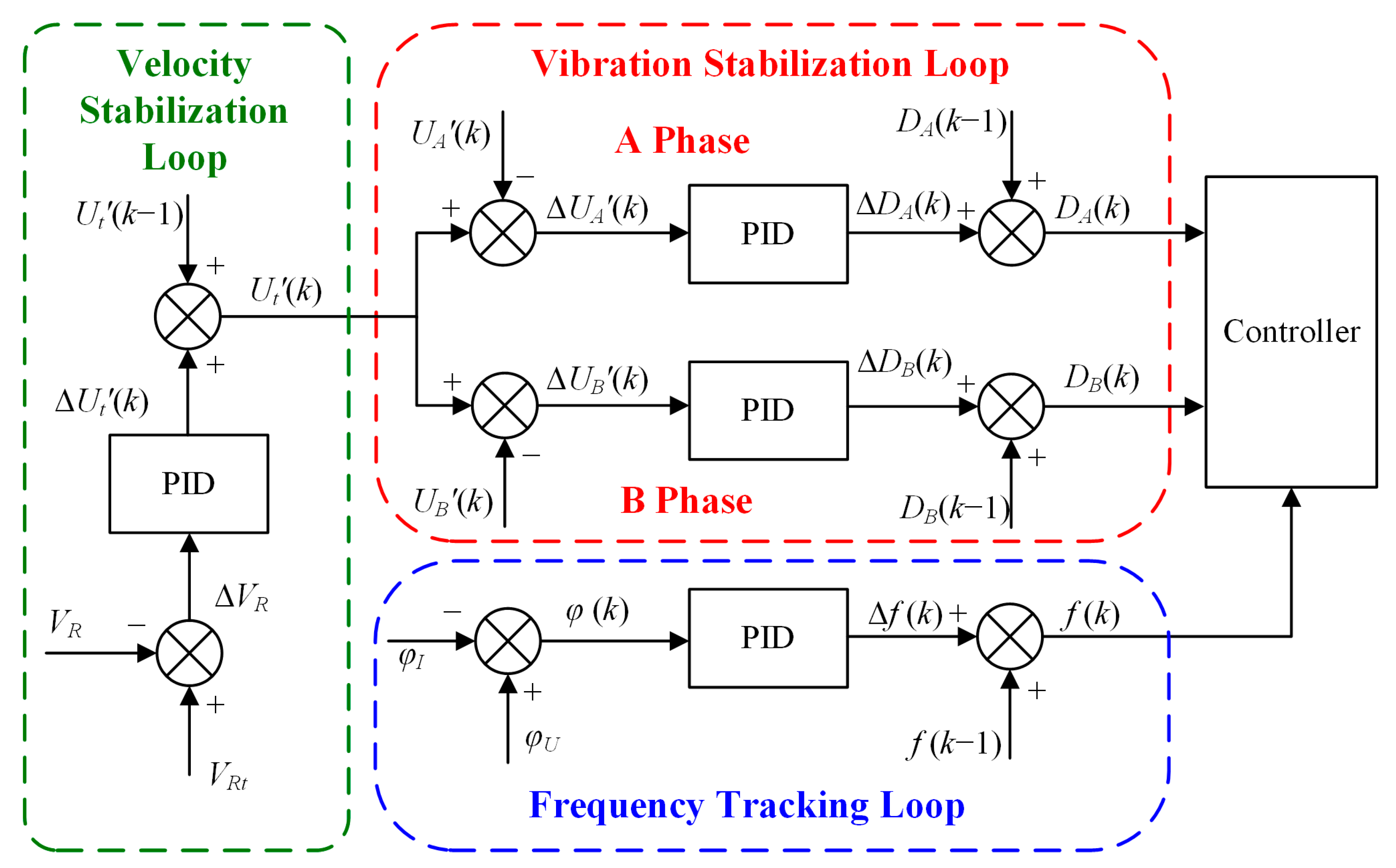
3.2. Control Structure
4. Experimental Results
4.1. Experimental Setup
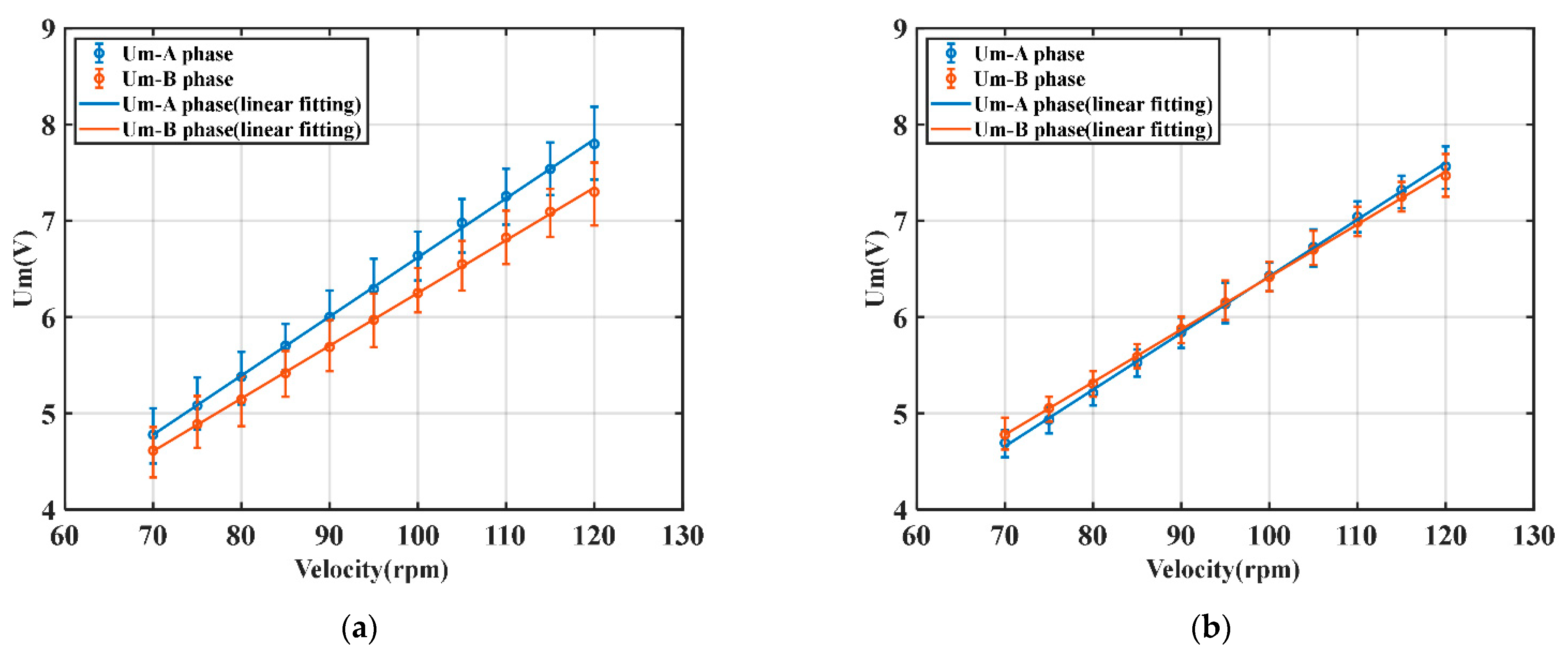
4.2. Verification of the Proposed Scheme
4.3. Stator Vibration Amplitude Analyses
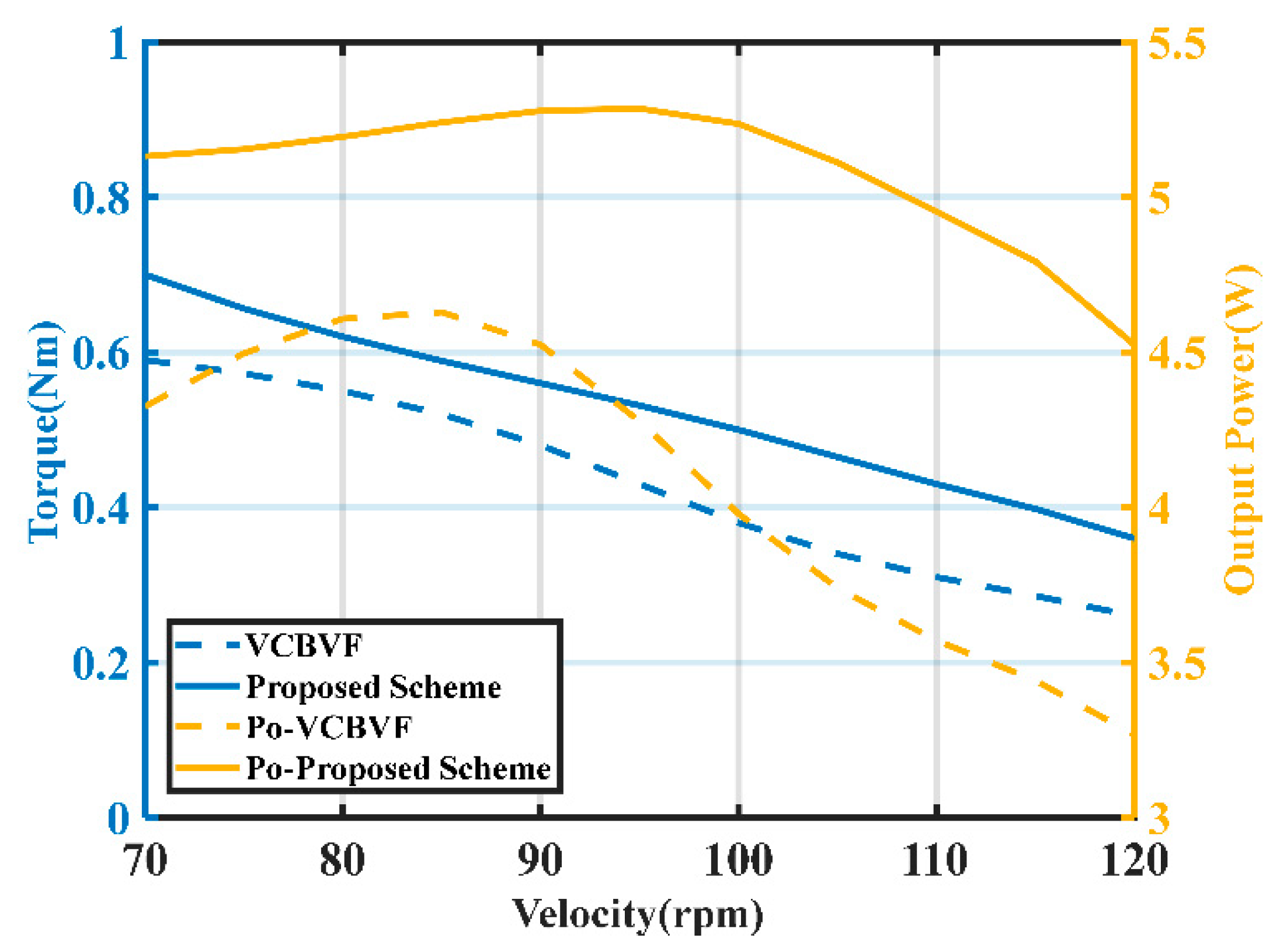
4.4. Output Performance Analyses
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhao, C.S. Ultrasonic Motors: Technologies and Applications; Science Press Beijing: Beijing, China, 2011; pp. 1–494. [Google Scholar]
- Jia, B.T.; Wang, L.; Wang, R.F.; Jin, J.M.; Zhao, Z.H.; Wu, D.W. A novel traveling wave piezoelectric actuated wheeled robot: Design, theoretical analysis, and experimental investigation. Smart Mater. Struct. 2021, 30, 035016. [Google Scholar] [CrossRef]
- Ryndzionek, R.; Sienkiewicz, L. A review of recent advances in the single- and multi-degree-of-freedom ultrasonic piezoelectric motors. Ultrasonics 2021, 116, 106471. [Google Scholar] [CrossRef] [PubMed]
- Feng, Z.; Liang, W.Y.; Ling, J.; Xiao, X.H.; Tan, K.K.; Lee, T.H. Integral terminal sliding-mode-based adaptive integral backstepping control for precision motion of a piezoelectric ultrasonic motor. Mech. Syst. Signal Process. 2020, 144, 106856. [Google Scholar] [CrossRef]
- Tan, K.K.; Liang, W.Y.; Huang, S.; Pham, L.P.; Chen, S.; Gan, C.W.; Lim, H.Y. Precision Control of Piezoelectric Ultrasonic Motor for Myringotomy with Tube Insertion. J. Dyn. Syst. Meas. Control 2015, 137, 064504. [Google Scholar]
- Zhang, X.F.; Zhang, G.B.; Nakamura, K.; Ueha, S. A robot finger joint driven by hybrid multi-DOF piezoelectric ultrasonic motor. Sens. Actuators A Phys. 2011, 169, 206–210. [Google Scholar] [CrossRef]
- Delibas, B.; Koc, B.; Thielager, J.; Stiebel, C. A novel drive and control method for piezoelectric motors in microscopy stages. In Proceedings of the Euspen’s 21st International Conference & Exhibition, Copenhagen, Denmark, 7–10 June 2021. [Google Scholar]
- Tian, X.Q.; Liu, Y.X.; Deng, J.; Wang, L.; Chen, W.S. A review on piezoelectric ultrasonic motors for the past decade: Classification, operating principle, performance, and future work perspectives. Sens. Actuators A Phys. 2020, 306, 111971. [Google Scholar] [CrossRef]
- Liang, W.Y.; Tan, K.K.; Huang, S.N.; Pham, L.P.; Lim, H.Y.; Gan, C.W. Control of a 2-DOF ultrasonic piezomotor stage for grommet insertion. Mechatronics 2013, 23, 1005–1013. [Google Scholar] [CrossRef]
- Zhang, J.-T.; Zhu, H.; Zhao, C.-S. Modal frequency modification of ultrasonic motor stator. Opt. Precis. Eng. 2008, 16, 2406–2413. [Google Scholar]
- Wang, J.; Jin, J.; Zhao, C. Velocity Stability Research of Traveling Wave Type Rotary Ultrasonic Motor. Proc. CSEE 2011, 31, 109–116. [Google Scholar]
- Yang, L.; Ma, C.C.; Ren, W.H.; Zhang, J.J. Beat traveling wave principle and its verification on rotating ultrasonic motor. J. Vib. Control 2021, 27, 2354–2367. [Google Scholar] [CrossRef]
- Sun, H.X.; Jing, K.; Li, G.Q.; Dong, X. An Optimized Operation Method of Two-Phase Coordinated Control for TRUM Based on the Observer of Vibration Mode. Small Spec. Electr. Mach. 2016, 44, 78–81. [Google Scholar]
- Kuhne, M.; Rochin, R.G.; Cos, R.S.; Astorga, G.J.R.; Peer, A. Modeling and Two-Input Sliding Mode Control of Rotary Traveling Wave Ultrasonic Motors. IEEE Trans. Ind. Electron. 2018, 65, 7149–7159. [Google Scholar] [CrossRef]
- Chen, N.; Jiao, X.K.; Tan, R.Y.; Zheng, J.J.; Fan, S.X. A multi-parameter speed control model of traveling wave ultrasonic motor. Int. J. Appl. Electrom. 2020, 64, 457–464. [Google Scholar] [CrossRef]
- Kebbab, F.Z.; Belkhiat, D.E.C.; Jabri, D.; Belkhiat, S. Frequency Speed Control of Rotary Travelling Wave Ultrasonic Motor Using Fuzzy Controller. Eng. Technol. Appl. Sci. 2018, 8, 3276–3281. [Google Scholar] [CrossRef]
- Lu, S.; Shi, J.Z. Nonlinear Hammerstein model of ultrasonic motor for position control using differential evolution algorithm. Ultrasonics 2019, 94, 20–27. [Google Scholar] [CrossRef]
- Fang, Z.W.; Yang, T.Y.; Zhu, Y.F.; Li, S.Y.; Yang, M. Velocity Control of Traveling-Wave Ultrasonic Motors Based on Stator Vibration Amplitude. Sensors 2019, 19, 5326. [Google Scholar] [CrossRef] [PubMed]
- Chen, H.C.; Han, W.; Qiu, J.H. Investigation on the traveling wave and three-dimensional trajectory of motion on the stator of rotational ultrasonic motors. Int. J. Appl. Electrom. 2020, 64, 631–638. [Google Scholar] [CrossRef]
- Mashimo, T.; Terashima, K. Experimental Verification of Elliptical Motion Model in Traveling Wave Ultrasonic Motors. IEEE Trans. Ind. Electron. 2015, 20, 2699–2707. [Google Scholar] [CrossRef]
- Li, S.Y.; Li, D.Y.; Yang, M.; Cao, W.W. Parameters identification and contact analysis of traveling wave ultrasonic motor based on measured force and feedback voltage. Sens. Actuators A Phys. 2018, 284, 201–208. [Google Scholar] [CrossRef]
- Ben Messaoud, W.; Giraud, F.; Lemaire-Semail, B.; Amberg, M.; Bueno, M.A. Amplitude Control of an Ultrasonic Vibration for a Tactile Stimulator. IEEE Trans. Ind. Electron. 2016, 21, 1692–1701. [Google Scholar] [CrossRef]
- Zhu, Y.F.; Yang, T.Y.; Fang, Z.W.; Li, S.Y.; Lu, C.Y.; Yang, M. Contact modeling for control design of traveling wave ultrasonic motors. Sens. Actuators A Phys. 2020, 310, 112037. [Google Scholar] [CrossRef]
- Zhuang, Y.; Ural, S.O.; Rajapurkar, A.; Tuncdemir, S.; Amin, A.; Uchino, K. Derivation of Piezoelectric Losses from Admittance Spectra. Jpn. J. Appl. Phys. 2009, 48, 056509. [Google Scholar] [CrossRef]
- Yang, T.Y.; Zhu, Y.F.; Fang, Z.W.; Wu, H.Y.; Jiang, W.L.; Yang, M. A Driving and Control Scheme of High Power Piezoelectric Systems over a Wide Operating Range. Sensors 2020, 20, 4401. [Google Scholar] [CrossRef]
- Giraud, F.; Lemaire-Semail, B. Causal modeling and identification of a travelling wave ultrasonic motor. Eur. Phys. J.-Appl. Phys. 2003, 21, 151–159. [Google Scholar] [CrossRef]





| Parameters | Values | Parameters | Values |
|---|---|---|---|
| Drive Frequency | 40~45 (kHz) | Rated Velocity | 100 (rpm) |
| Drive Voltage | 130 (Vrms) | Maximum Velocity | 150 (rpm) |
| Rated Torque | 0.5 (Nm) | Rated Output | 5.0 (W) |
| Proposed Scheme | Single-Phase VCBVF Scheme | |||
|---|---|---|---|---|
| Load (Nm) | Velocity Fluctuation (rpm) | Overshoot (%) | Velocity Fluctuation (rpm) | Overshoot (%) |
| 0 | 1.68 | 0.96 | 2.62 | 1.92 |
| 0.1 | 1.68 | 1.92 | 4.32 | 4.88 |
| 0.2 | 1.92 | 1.94 | 5.26 | 5.38 |
| 0.3 | 2.40 | 6.90 | 7.69 | 17.50 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, X.; Yang, M.; Zhu, Y.; Hu, Y. Two-Phase Stator Vibration Amplitude Compensation of Traveling-Wave Ultrasonic Motor. Actuators 2022, 11, 278. https://doi.org/10.3390/act11100278
Gao X, Yang M, Zhu Y, Hu Y. Two-Phase Stator Vibration Amplitude Compensation of Traveling-Wave Ultrasonic Motor. Actuators. 2022; 11(10):278. https://doi.org/10.3390/act11100278
Chicago/Turabian StyleGao, Xiaochen, Ming Yang, Yuanfei Zhu, and Yinghua Hu. 2022. "Two-Phase Stator Vibration Amplitude Compensation of Traveling-Wave Ultrasonic Motor" Actuators 11, no. 10: 278. https://doi.org/10.3390/act11100278
APA StyleGao, X., Yang, M., Zhu, Y., & Hu, Y. (2022). Two-Phase Stator Vibration Amplitude Compensation of Traveling-Wave Ultrasonic Motor. Actuators, 11(10), 278. https://doi.org/10.3390/act11100278






