Modeling of Resistive Forces and Buckling Behavior in Variable Recruitment Fluidic Artificial Muscle Bundles
Abstract
1. Introduction
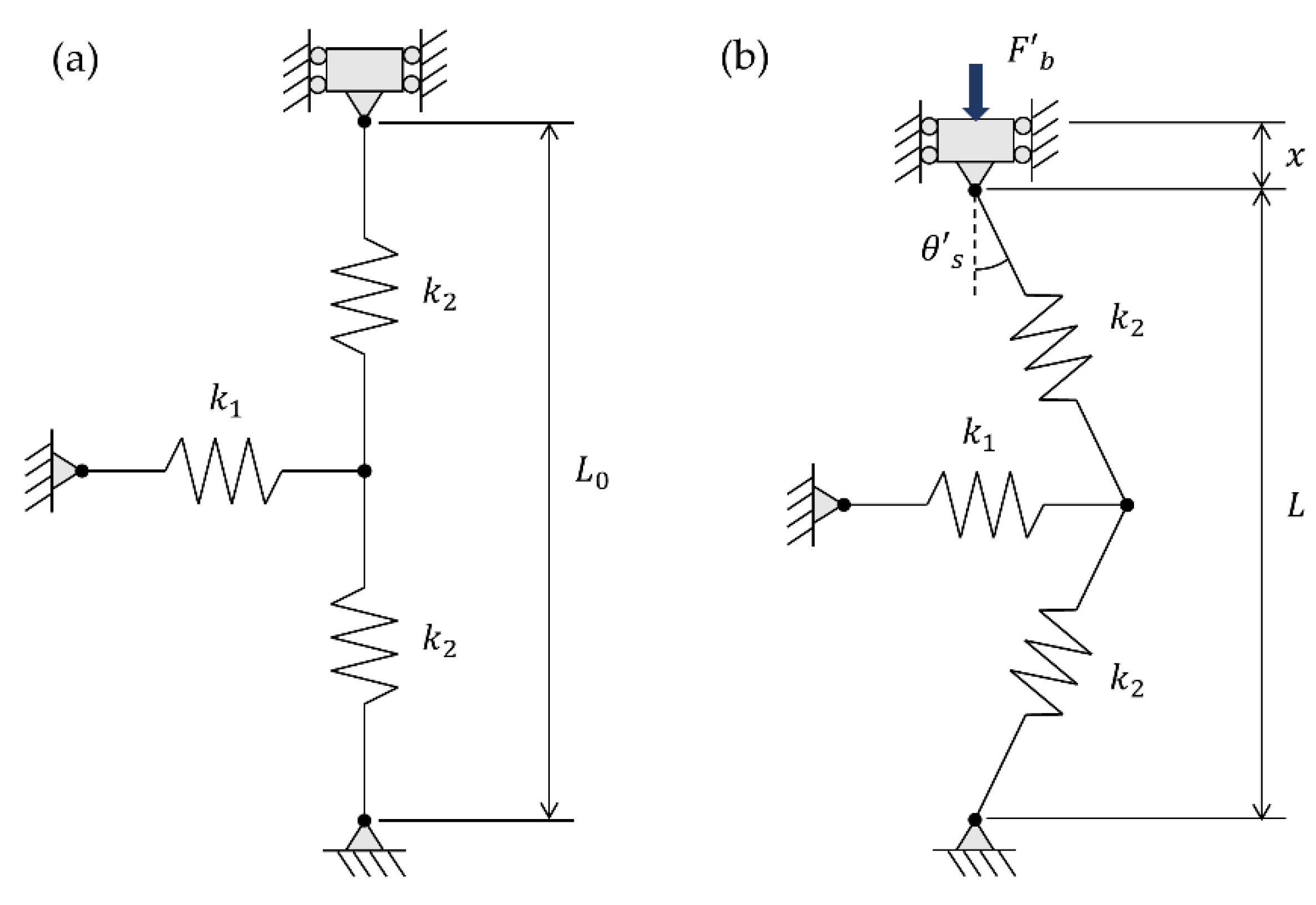
2. Quasi-Static Modeling of Tensile Force Generation
3. Resistive Force Modeling
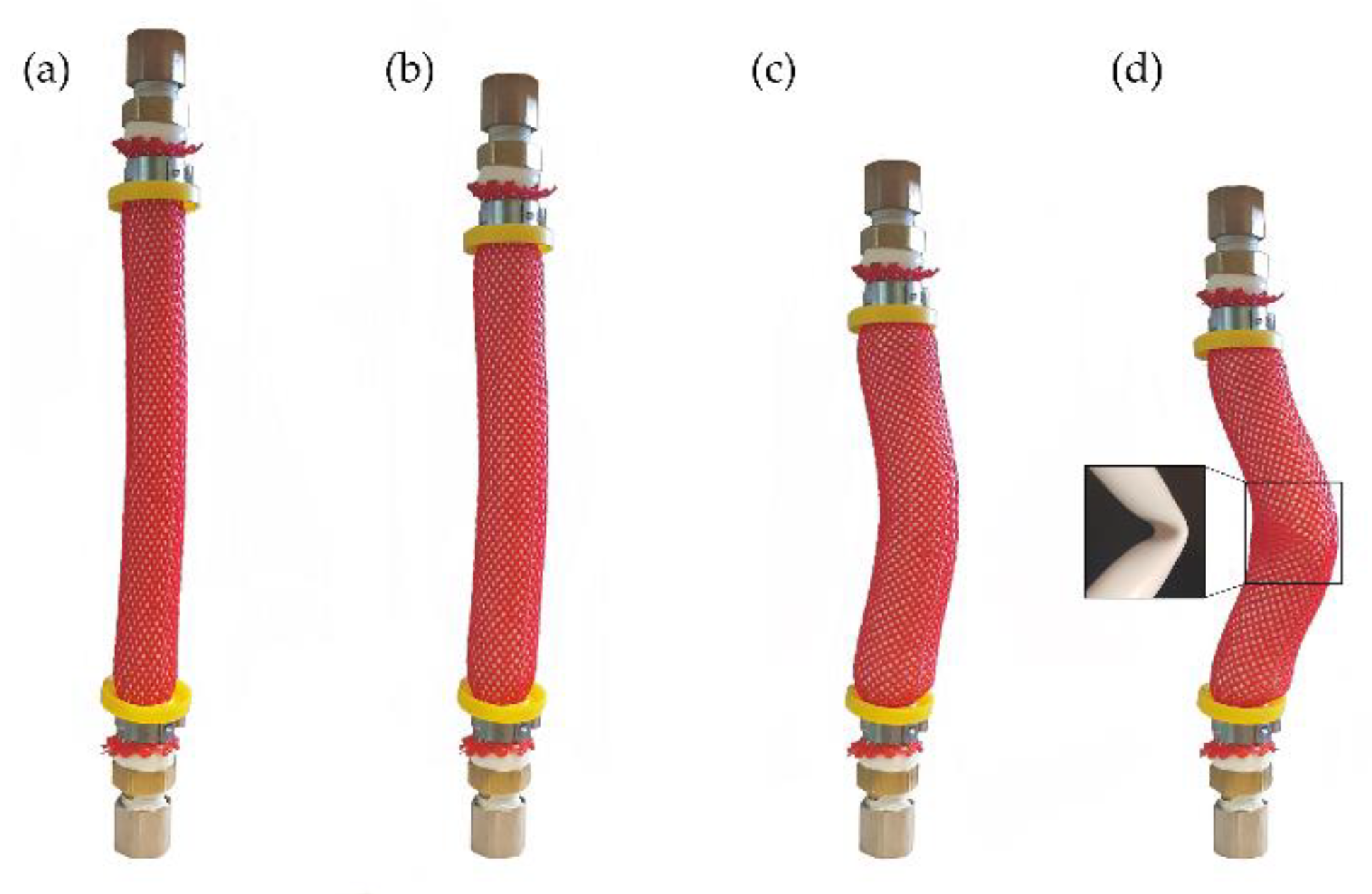
3.1. Experimental Observations of Post-Free Strain FAM Behavior
3.2. Post-Buckling Region
3.3. Post-Collapse Region
3.4. Summary of Resistive Force Piecewise Model
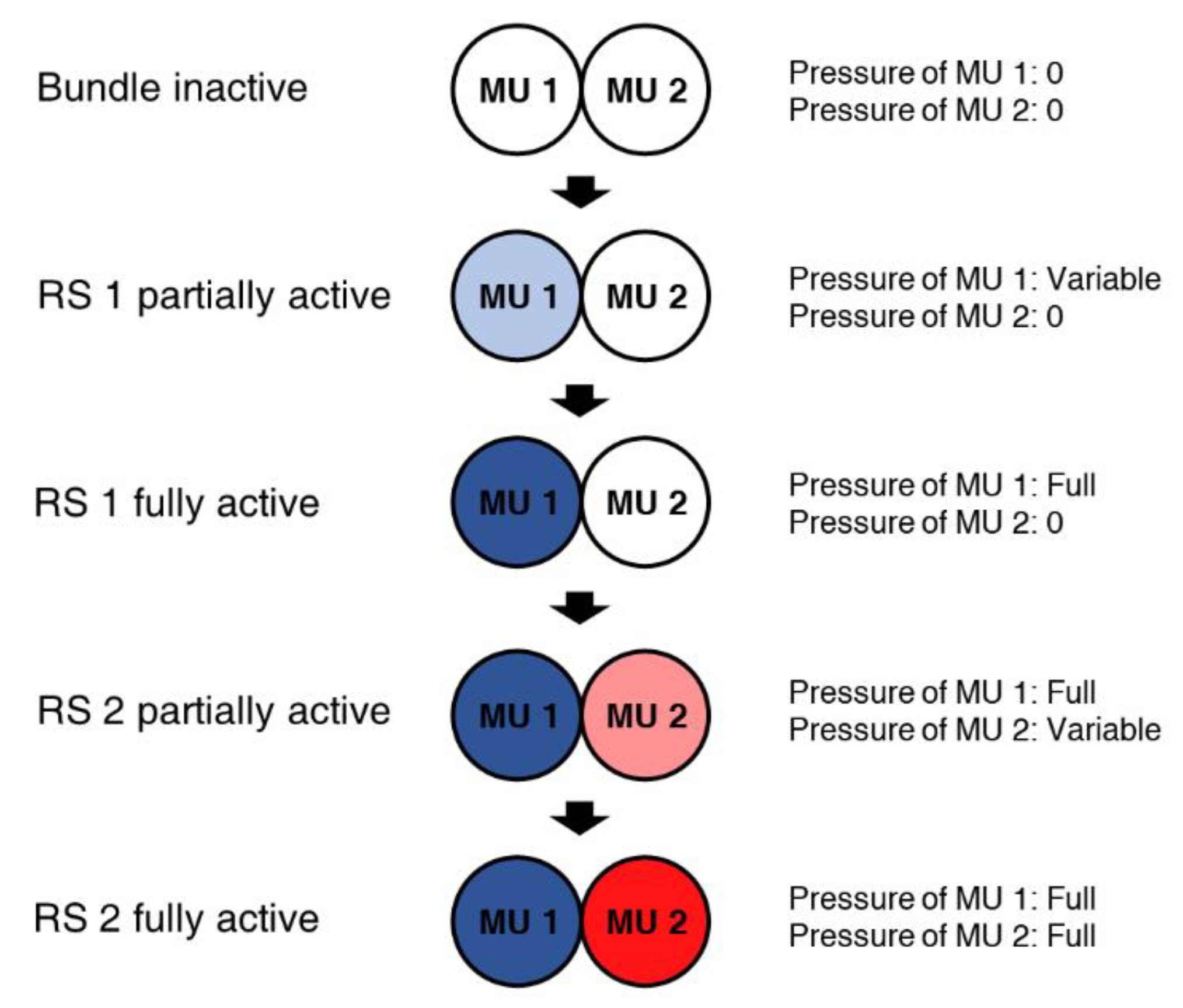
4. Effect of Resistive Force on Overall Performance of a Variable Recruitment Bundle
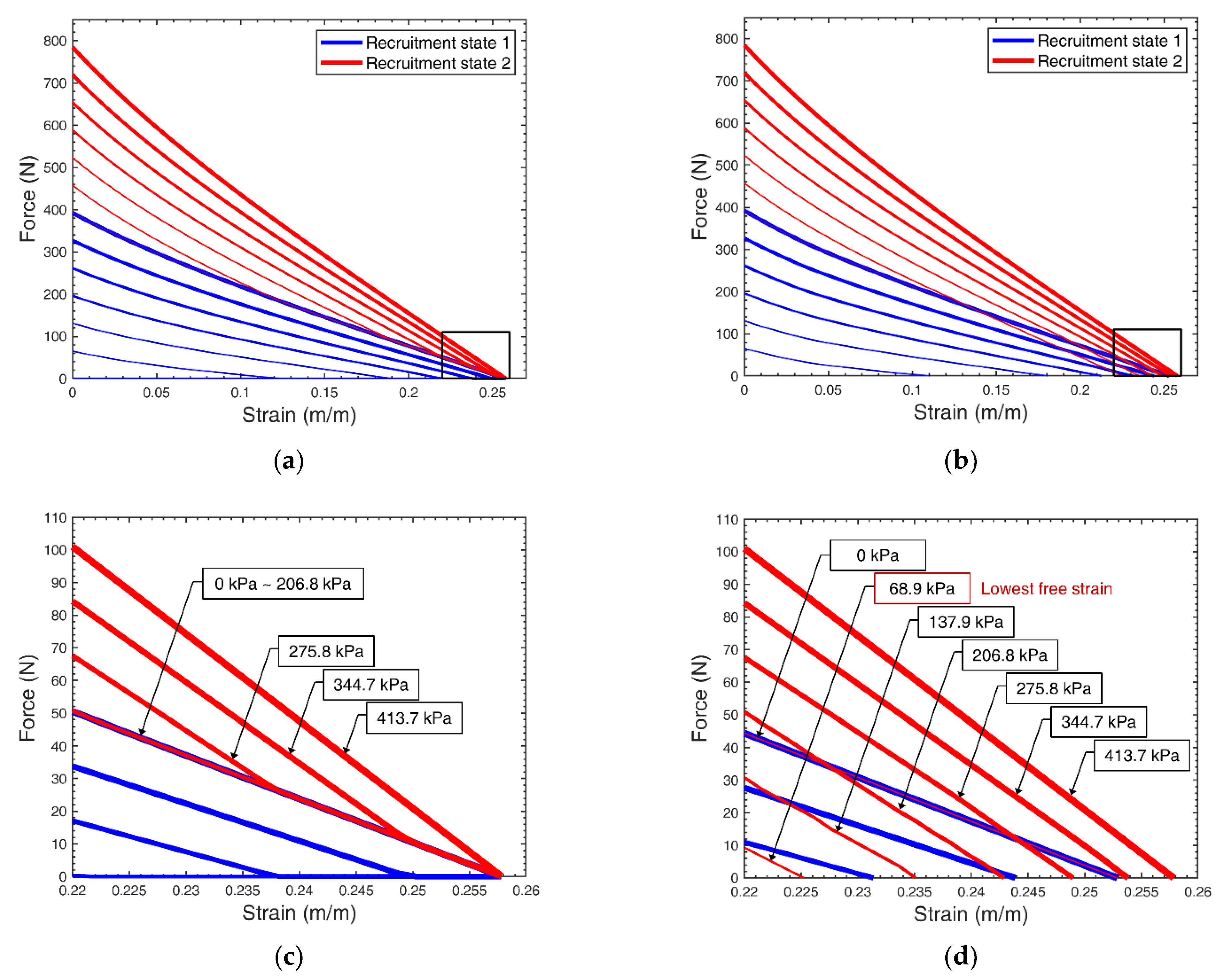
4.1. Quasi-Static Force–Strain Space for Variable Recruitment Bundle with Resistive Forces
4.2. Efficiency Analysis for Isobaric and Isotonic Contraction
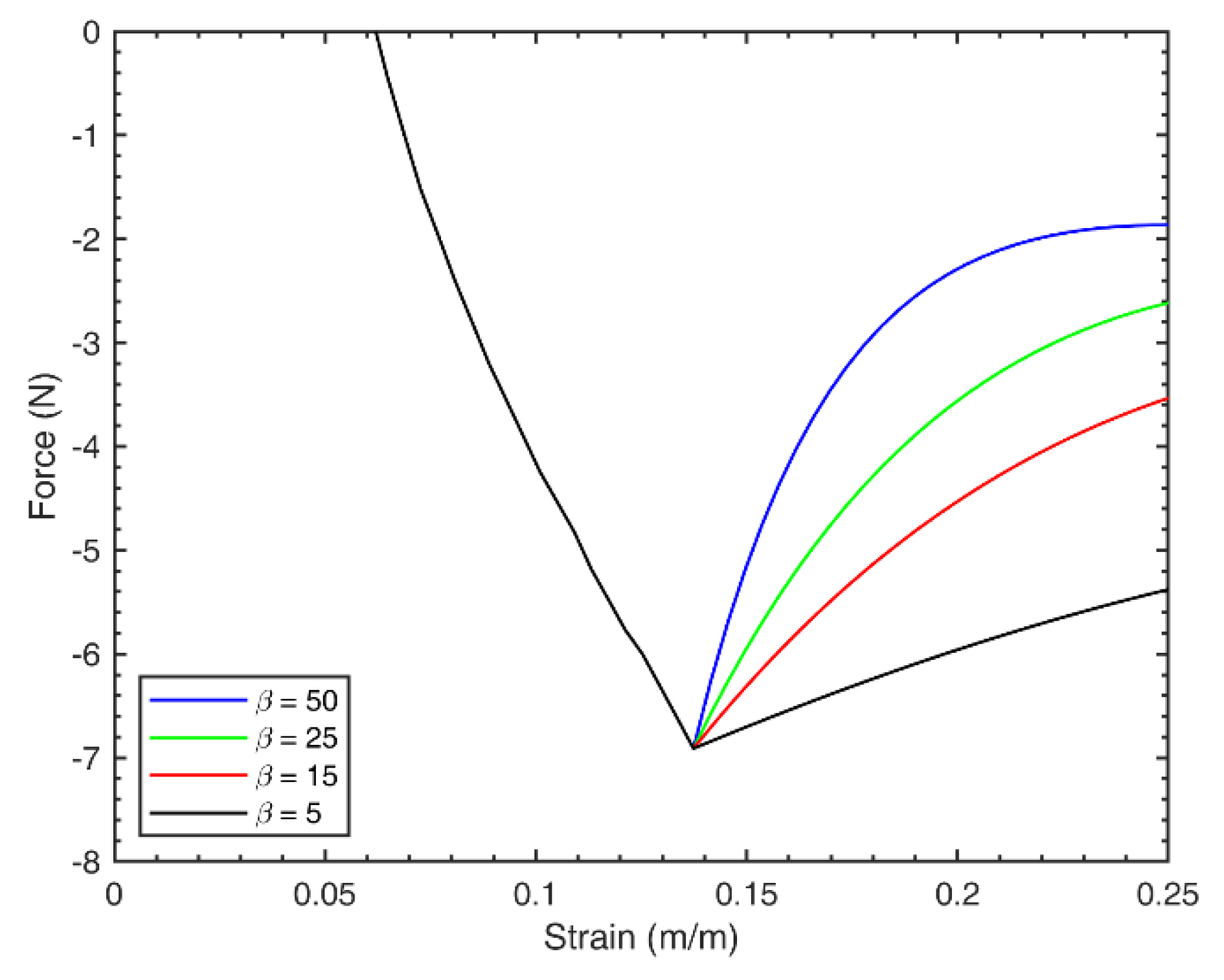
5. Improving the Model Through Empirical Parameter Tuning
5.1. Tensile Force Correction
5.2. Resistive Force Correction
- 1.
- Young’s Modulus,
- 2.
- Collapse moment,
- 3.
- Transition constant,
- 4.
- Torsional spring stiffness,
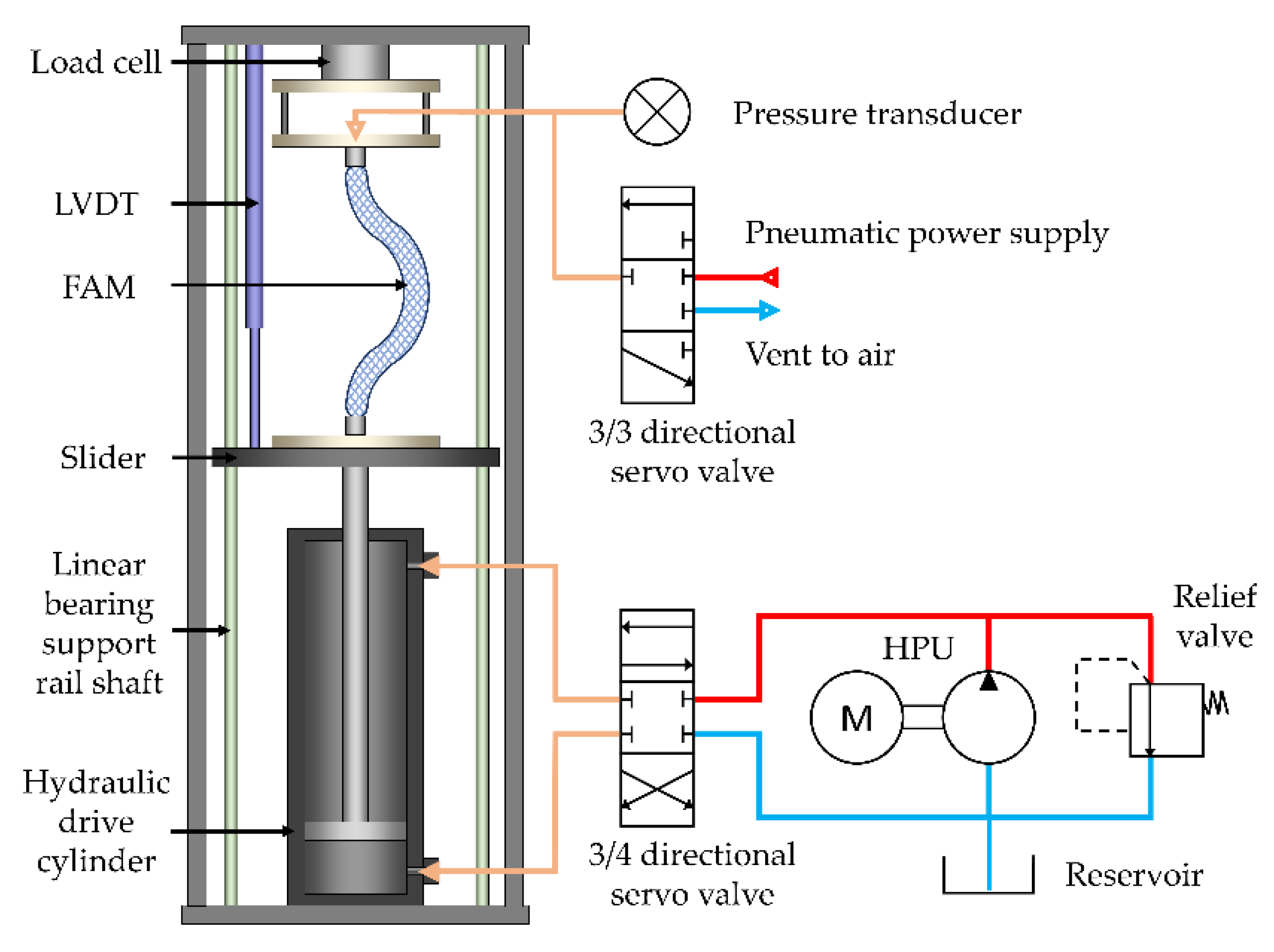
5.3. Experiments to Generate Correction Factors
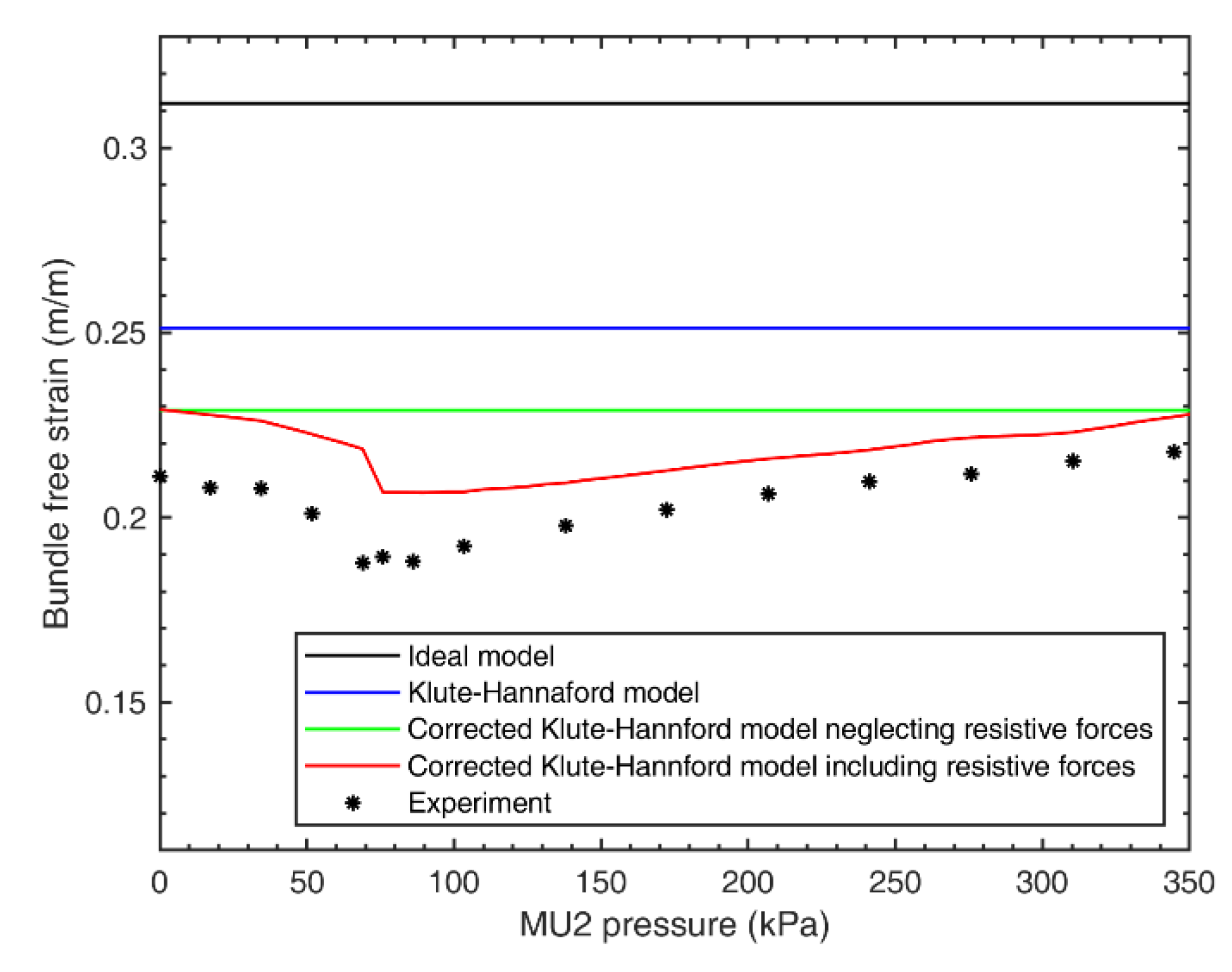
5.4. Results from Empirical Parameter Tuning
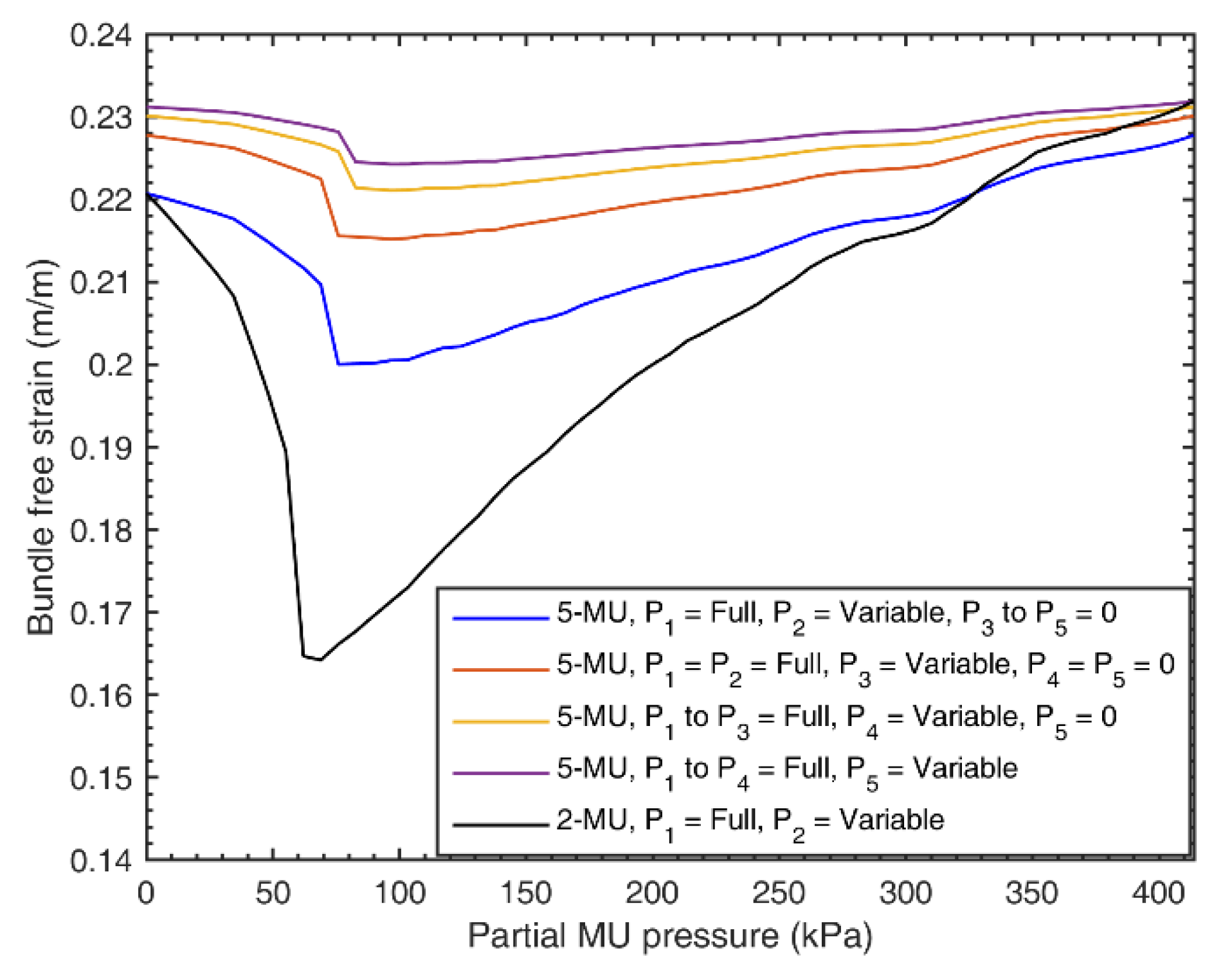
6. Bundle Free Strain Gradient Reversal
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Tondu, B. Modeling of the McKibben artificial muscle: A review. J. Intell. Mater. Syst. Struct. 2012, 23, 225–253. [Google Scholar] [CrossRef]
- Tondu, B.; Ippolito, S.; Guiochet, J.; Daidie, A. A Seven-degrees-of-freedom Robot-arm Driven by Pneumatic Artificial Muscles for Humanoid Robots. Int. J. Robot. Res. 2005, 24, 257–274. [Google Scholar] [CrossRef]
- Gordon, K.E.; Sawicki, G.S.; Ferris, D.P. Mechanical performance of artificial pneumatic muscles to power an ankle–foot orthosis. J. Biomech. 2006, 39, 1832–1841. [Google Scholar] [CrossRef]
- Mori, M.; Suzumori, K.; Wakimoto, S.; Kanda, T.; Takahashi, M.; Hosoya, T.; Takematu, E. Development of power robot hand with shape adaptability using hydraulic McKibben muscles. IEEE Int. Conf. Robot. Autom. 2010, 1162–1168. [Google Scholar] [CrossRef]
- Chapman, E.M.; Jenkins, T.E.; Bryant, M. Modeling and analysis of a meso-hydraulic climbing robot with artificial muscle actuation. Bioinspir. Biomim. 2017, 12, 066010. [Google Scholar] [CrossRef]
- Al-Fahaam, H.; Davis, S.; Nefti-Meziani, S. The design and mathematical modeling of novel extensor bending pneumatic artificial muscles (EBPAMs) for soft exoskeletons. Robot.Auton. Syst. 2018, 99, 63–74. [Google Scholar] [CrossRef]
- Kurumaya, S.; Nabae, H.; Endo, G.; Suzumori, K. Exoskeleton inflatable robotic arm with thin McKibben muscle. IEEE Int. Conf. Soft Robot. 2018, 120–125. [Google Scholar] [CrossRef]
- Ohta, P.; Valle, L.; King, J.; Low, K.; Yi, J.; Atkeson, C.G.; Park, Y.-L. Design of a Lightweight Soft Robotic Arm Using Pneumatic Artificial Muscles and Inflatable Sleeves. Soft Robot. 2018, 5, 204–215. [Google Scholar] [CrossRef] [PubMed]
- Bryant, M.; Meller, M.A.; Garcia, E. Variable Recruitment Fluidic Artificial Muscles: Modeling and Experiments. Smart Mater. Struct. 2014, 23, 74009. [Google Scholar] [CrossRef]
- Jenkins, T.E.; Chapman, E.M.; Bryant, M. Bio-inspired online variable recruitment control of fluidic artificial muscles. Smart Mater. Struct. 2016, 25, 125016. [Google Scholar] [CrossRef]
- Robinson, R.M.; Kothera, C.S.; Wereley, N.M. Variable Recruitment Testing of Pneumatic Artificial Muscles for Robotic Manipulators. IEEE/ASME Trans. Mechatron. 2015, 20, 1642–1652. [Google Scholar] [CrossRef]
- DeLaHunt, S.A.; Pillsbury, T.E.; Wereley, N.M. Variable recruitment in bundles of miniature pneumatic artificial muscles. Bioinspir. Biomim. 2016, 11, 056014. [Google Scholar] [CrossRef]
- Henneman, E.; Somjen, G.; Carpenter, D.O. Excitability and inhibitability of motoneurons of different sizes. J Neurophysiol. 1965, 28, 599–620. [Google Scholar] [CrossRef]
- Meller, M.A.; Bryant, M.; Garcia, E. Reconsidering the McKibben muscle: Energetics, operating fluid, and bladder material. J. Intell. Mater. Syst. Struct. 2014, 25, 2276–2293. [Google Scholar] [CrossRef]
- Chapman, E.M.; Jenkins, T.E.; Bryant, M. Design and analysis of electrohydraulic pressure systems for variable recruitment in fluidic artificial muscles. Smart Mater. Struct. 2018, 27. [Google Scholar] [CrossRef]
- Kim, J.Y.; Mazzoleni, N.; Bryant, M. Investigation of Resistive Forces in Variable Recruitment Fluidic Artificial Muscle Bundles. Conf. Proc. Soc. Exp. Mech. 2021, 8, 305–313. [Google Scholar] [CrossRef]
- Mazzoleni, N.; Jeong, Y.K.; Bryant, M. The effect of resistive forces in variable recruitment fluidic artificial muscle bundles: A configuration study. Proc. SPIE Bioinspir. Biomim. Bioreplication X 2020, 11374, 11374. [Google Scholar] [CrossRef]
- Chou, C.; Hannaford, B. Measurement and Modeling of McKibben Pneumatic Artificial Muscles. IEEE Trans. Robot. Autom. 1996, 12, 90–102. [Google Scholar] [CrossRef]
- Tondu, B.; Lopez, P. Modeling and Control of McKibben Artificial Muscle Robot Actuators. IEEE Control Syst. Mag. 2000, 20, 15–38. [Google Scholar] [CrossRef]
- Klute, G.; Hannaford, B. Accounting for Elastic Energy Storage in McKibben Artificial Muscle Actuators. ASME. J. Dyn. Syst. Meas. Control. 2000, 122, 386–388. [Google Scholar] [CrossRef]
- Kothera, C.S.; Jangid, M.; Sirohi, J.; Wereley, N.M. Experimental Characterization and Static Modeling of McKibben Actuators. ASME. J. Mech. Des. 2009, 131, 091010. [Google Scholar] [CrossRef]
- Ball, E.; Garcia, E. Effects of Bladder Geometry in Pneumatic Artificial Muscles. ASME. J. Med. Devices. 2016, 10, 041001. [Google Scholar] [CrossRef]
- Yu, Z.; Pillsbury, T.; Wang, G.; Wereley, N.M. Hyperelastic analysis of pneumatic artificial muscle with filament-wound sleeve and coated outer layer. Smart. Mater. Struct. 2019, 28, 105019. [Google Scholar] [CrossRef]
- Jones, R.M. Buckling of Bars, Plates, and Shells; Bull Ridge Publishing: Blacksburg, VA, USA, 2006; pp. 49–58. [Google Scholar]
- Simitses, G.J. Buckling and Postbuckling of Imperfect Cylindrical Shells: A Review. Appl. Mech. Rev. 1986, 39, 1517–1524. [Google Scholar] [CrossRef]
- Brazier, L.G. On the Flexure of Thin Cylindrical Shells and Other “Thin” Sections. Proc. R. Soc. A. 1927, 116, 104–114. [Google Scholar]
- Krenk, S. Beams. In Mechanics and Analysis of Beams, Columns and Cables, 2nd ed.; Springer-Verlag: Berlin/Heidelberg, Germany, 2001; pp. 23–43. [Google Scholar]
- Wood, J.D. The flexure of a uniformly pressurized, circular, cylindrical shell. J. Appl. Mech. 1958, 25, 453–458. [Google Scholar]
- Stein, M.; Hedgpeth, J.M. Analysis of Partly Wrinkled Membranes; National Aeronautics and Space Administration: Washington, DC, USA, 1961. [Google Scholar]
- Zender, G.W. The Bending Strength of Pressurized Cylinders. J. Aerosp. Sci. 1961, 29, 362–363. [Google Scholar] [CrossRef]
- Veldman, S.L.; Bergsma, O.K.; Beukers, A. Bending of anisotropic inflated cylindrical beams. Thin-Walled Struct. 2005, 43, 461–475. [Google Scholar] [CrossRef]
- Meller, M.; Chipka, J.; Volkov, A.; Bryant, M.; Garcia, E. Improving actuation efficiency through variable recruitment hydraulic McKibben muscles: Modeling, orderly recruitment control, and experiments. Bioinspir. Biomim. 2016, 11, 065004. [Google Scholar] [CrossRef]
- Kurumaya, S.; Nabae, H.; Endo, G.; Suzumori, K. Design of thin McKibben muscle and multifilament structure. Sens. Actuator A Phys. 2017, 261, 66–74. [Google Scholar] [CrossRef]
- Meller, M.; Kogan, B.; Bryant, M.; Garcia, E. Model-based feedforward and cascade control of hydraulic McKibben muscles. Sens. Actuator A Phys. 2018, 275, 88–98. [Google Scholar] [CrossRef]
- Wielsgosz, C.; Thomas, J.C. Deflections of inflatable fabric panels at high pressure. Thin-Walled Struct. 2002, 40, 523–536. [Google Scholar] [CrossRef]
- Stephans, W.B.; Starnes, J.H.; Almroth, B.O. Collapse of Long Cylindrical Shells under Combined Bending and Pressure Loads. AIAA J. 1975, 13, 20–25. [Google Scholar] [CrossRef]
- Chipka, J.; Meller, M.A.; Volkov, A.; Bryant, M.; Garcia, E. Linear dynamometer testing of hydraulic artificial muscle with variable recruitment. J. Intell. Mater. Syst. Struct. 2017, 28, 2051–2063. [Google Scholar] [CrossRef]
- Diani, J.; Fayolle, B.; Gilormini, P. A review on the Mullins effect. Eur. Polym. J. 2009, 45, 601–612. [Google Scholar] [CrossRef]






| FAM No. | Slenderness Ratio, | Correction Factors | |||
|---|---|---|---|---|---|
| Young’s Modulus | Collapse Moment | Torsional Spring Constant | |||
| 1 | 8 | 1.25 | 1.1 | 100 | |
| 2 | 10 | 0.95 | 0.75 | 100 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, J.Y.; Mazzoleni, N.; Bryant, M. Modeling of Resistive Forces and Buckling Behavior in Variable Recruitment Fluidic Artificial Muscle Bundles. Actuators 2021, 10, 42. https://doi.org/10.3390/act10030042
Kim JY, Mazzoleni N, Bryant M. Modeling of Resistive Forces and Buckling Behavior in Variable Recruitment Fluidic Artificial Muscle Bundles. Actuators. 2021; 10(3):42. https://doi.org/10.3390/act10030042
Chicago/Turabian StyleKim, Jeong Yong, Nicholas Mazzoleni, and Matthew Bryant. 2021. "Modeling of Resistive Forces and Buckling Behavior in Variable Recruitment Fluidic Artificial Muscle Bundles" Actuators 10, no. 3: 42. https://doi.org/10.3390/act10030042
APA StyleKim, J. Y., Mazzoleni, N., & Bryant, M. (2021). Modeling of Resistive Forces and Buckling Behavior in Variable Recruitment Fluidic Artificial Muscle Bundles. Actuators, 10(3), 42. https://doi.org/10.3390/act10030042






