1. Introduction
The main function of the tundish is to act as a steel reservoir between the ladle and the mold, and in the case of multistrand casters, to distribute the liquid into the molds. In addition to being a reservoir of liquid steel, the tundish is more increasingly used as a metallurgical reactor vessel aimed at improving control of steel cleanness, temperature, and composition. Tundish metallurgy was proposed in the early 1980s as a special secondary refining technology and an important link in ensuring excellent steel quality during production from smelting and refining to the formation of slabs or billets [
1]. In the past three decades, many experts and researchers have done a lot of work and published many important papers. A comprehensive review was given in the paper by Sahai [
2] and the book by Sahai and Emi [
3].
The basic physical phenomenon in the tundish involves the flow of molten steel. The metallurgical effect of the tundish is mainly achieved by the reasonable flow of molten steel in the tundish. The flow is intended to deliver the molten metal to the molds evenly and at a designed throughput rate and temperature with minimal contamination by, and maximize flotation of macro inclusions. Hence, the investigation of the flow phenomenon in the tundish is the foundation of tundish metallurgy.
Physical modeling has played a key role in tundish research [
4,
5,
6]. In physical modeling, a low temperature aqueous analog, generally water, is used to represent molten metal in a tundish. In particular, the kinematic viscosity of water is similar to that of molten steel. Water flow in a transparent model tundish can be used to observe melt flow physically taking place in an actual tundish. A full or reduced scale tundish model may be designed based on appropriate similarity criteria in which the flow of molten metal is simulated by the flow of water. As water flow in the model is a realistic representation of the actual tundish melt flow, it may be used to study the melt flow in a tundish.
According to the similarity principle for flow phenomena, the use of a water model to investigate the flow of molten steel in tundish is not only feasible, but also can accurately reflect the values and law of the actual flow of molten steel. However, in the actual hydraulics simulation of a tundish, it is difficult to completely maintain all forces to be equal between the model and the prototype; hence, different situations need to be considered. In a water simulation experiment using a reduced-scale model, it is imperative to use an approximate model method to ensure that the flow in the model is similar to that in the prototype.
Based on flow visualization and image processing technology, a digital image technique has been developed, which combines single point measurement technology (hotline, turbine) and flow visualization technology. Digital image measurement technology can not only achieve the overall structure and transient image field, but also obtain the velocity data of the whole flow field quantitatively. Some researchers [
7,
8,
9] have measured the flow field in the tundish by the particle image velocimetry (PIV), thus that the overall structure and transient image of a planar flow field was obtained quantitatively.
Many attempts have been made to improve melt flow characteristics in existing tundish’s by the installation of various flow control devices, such as weirs, dams, baffles with holes, and turbulence suppressors. The beneficial effects of various flow modification devices have been applied in actual industrial trials as well as physical and mathematical modeling studies [
10,
11]. Optimum placement of flow control devices has been found to result in an increase in the average residence time of fluid as well as an increase in the plug flow volume in the tundish. These flow control devices, properly installed, may create localized mixing in contained regions, which may help in inclusion agglomeration and hence, inclusion removal.
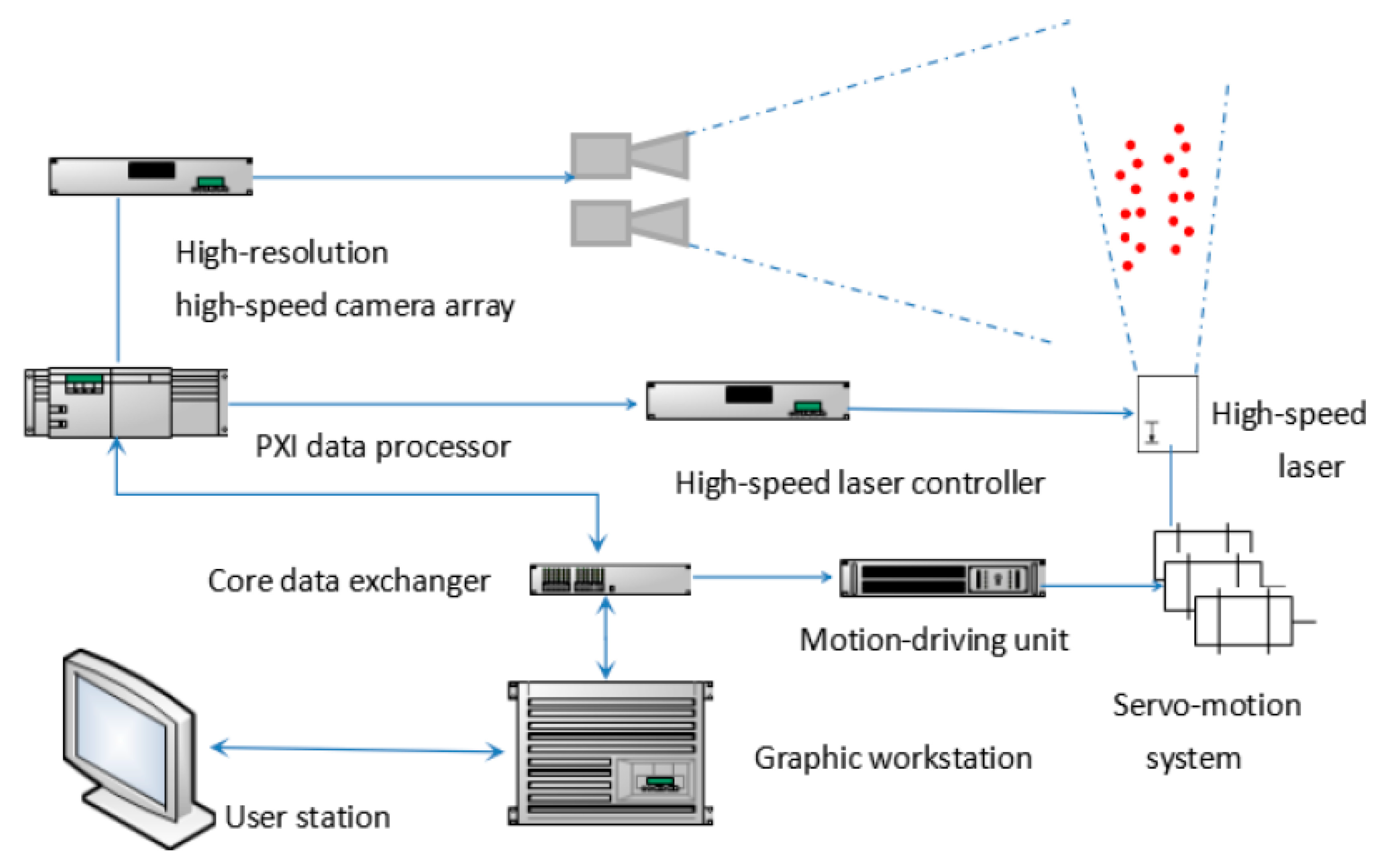
Due to the limited of view of the camera in PIV, developing an approach for measuring a water model with a length close to 5m is an important problem to be solved in this paper. In this study, the PIV flow measurement is carried out for a reduced-scale two-strand tundish model for slab production. The paper analyzes the flow characteristics in a two-strand tundish for continuous slab casting with different flow-control devices. The effect of eddies is discussed.
2. Experimental Object and Scheme
To transfer results of measurements from a model to the original tundish, apart from the geometric similarity, the fluid-dynamic similarity must be considered as well. For an isothermal water model experiment, geometrical similarity, and dynamic similarity between the model and the prototype are required. In order to maintain similarity in depth of liquid, the relationship between the velocities and hence the inlet flow rate of the fluid in the model and in the prototype is to be obtained by following either of Froude (
Fr) similarity or Reynolds (
Re) similarity. For the dynamic similarity, the
Re number and Fr number in the model should be equivalent to those in the prototype. As steel flow in the tundish is gravity-driven, it is understood that flow inside the tundish is Froude criteria dominated. As the flow of liquid steel within the tundish is severely turbulent, the
Re number of the model is in the same self-modelling region as the prototype, the
Re number can meet the requirement naturally. Thus, most reduced scale-modeling studies are done mainly based on Froude similarity criteria [
12].
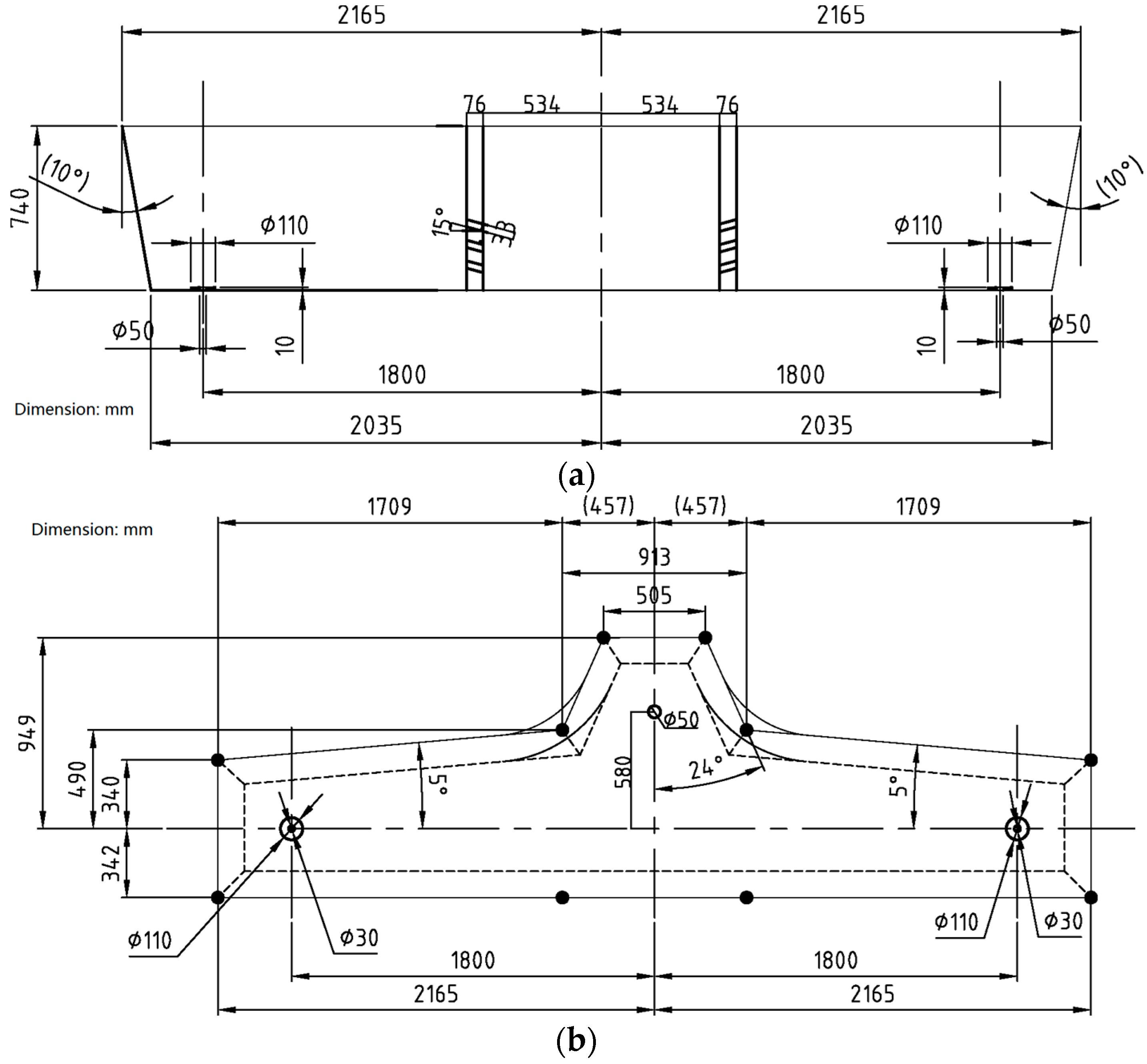
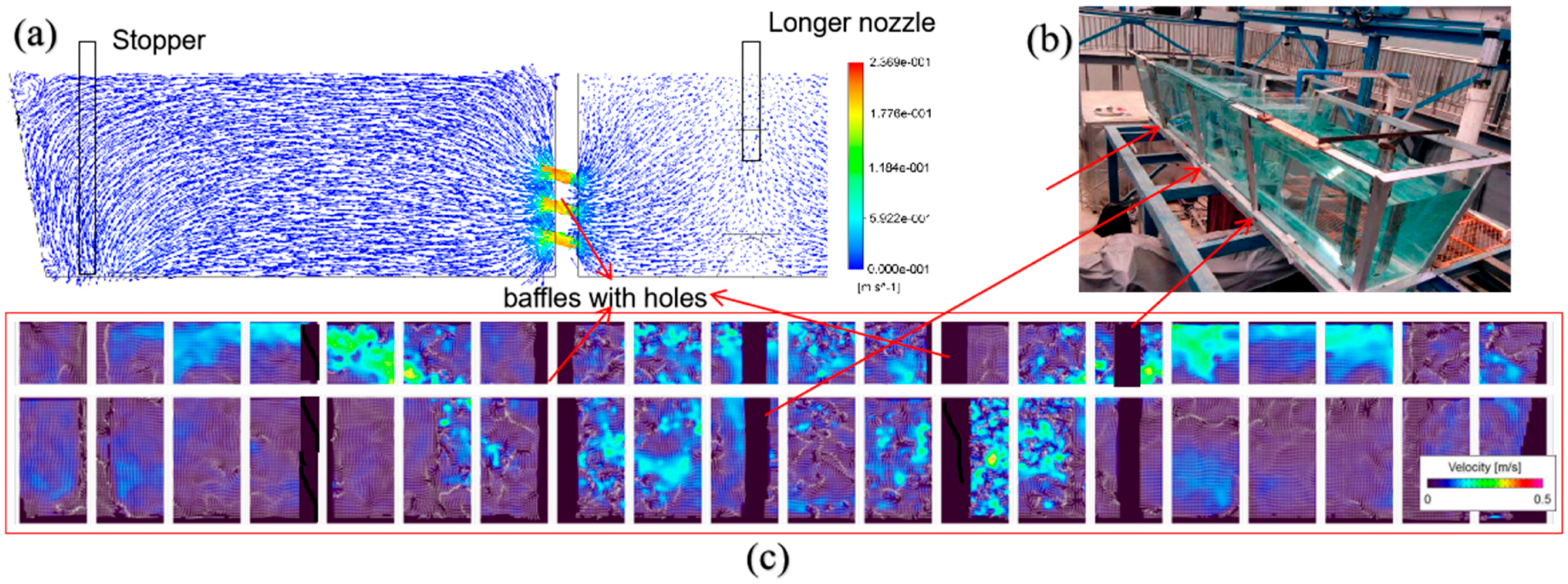
In the experiments, a two-strand tundish of a stainless-steel slab continuous casting is used as the experimental object. The tundish model in the hydraulics experiment comprised clear glass with a 2:3 ratio to the prototype. The flow rate passing the long nozzle in the experiment is determined on the basis of the typical sectional dimension of the cast slab, i.e., 180 mm × 650 mm, and casting speed. The typical casting speed is 1.2 m/min. The long nozzle diameter is 75 mm. The main dimensions of the water model are shown in
Figure 1, all dimensions are in millimeters. The scale factor of 2:3 is defined as the ratio of lengths in the model and prototype systems to ensure geometric similarity.
To satisfy the Froude similarity, the Froude number of the water model should be equal to that of the prototype:
The similarity ratio in velocity can be obtained (model velocity/prototype velocity):
The similarity ratio in the flowrate can be obtained (model flowrate /prototype flowrate):
where
and
represent the velocity of the fluid in the tundish in the prototype and model respectively, m/s;
g is the gravitational acceleration, m/s
2;
is the characteristic length, m;
is the similarity ratio,
and
are the flowrate in the tundish in the prototype and model respectively, m
3/h.
In the water simulation experiment, the quantity of poured steel is controlled by the flowrate at the long nozzle, meanwhile the liquid level is controlled by the level gauge in the tundish model.
Table 1 summarizes the concrete experimental parameters of the tundish prototype and the water model.
Fluid flow characteristics in the tundish with the incorporation of flow control devices is dependent on the optimum location and size. Numerical simulations have been used to optimize current control devices and installation locations. The optimized results are obtained by numerical simulation and must be validated by actual water simulation experiments. Scheme A is an optimization scheme, while the other three are in contrast to it. The installation location and size of these devices is shown in
Figure 1. The flow control devices in the tundish mainly include the baffles with holes and the turbulence suppressor.
Table 2 summarizes the experimental scheme. Experiments are carried out in the presence or absence of the flow control device and different casting speeds. The baffles with holes are placed in the middle of the tundish. The baffles are perpendicular to the walls of the tundish. Each baffle has six circular holes with a diameter of 33 mm and an upward inclination of 15°. To highlight the effect of the flow control device on the flow, the casting speed is doubled for comparing and manifesting the results obtained from the flow test.
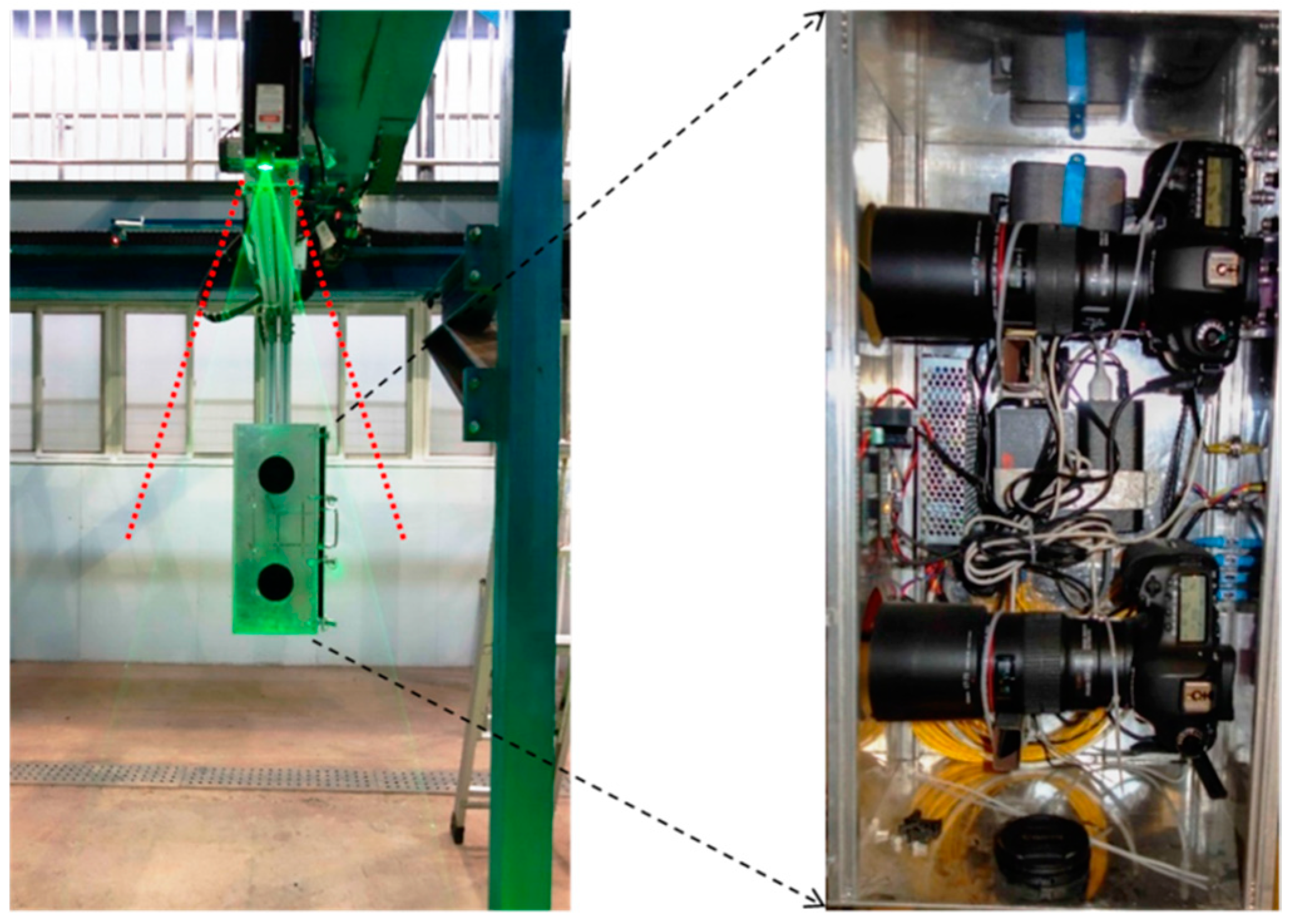
The model tundish is placed on the experimental platform, and water pipelines are connected. After setting the experimental parameters and waiting for 30 min for the flow to become steady, PIV flow measurement starts.
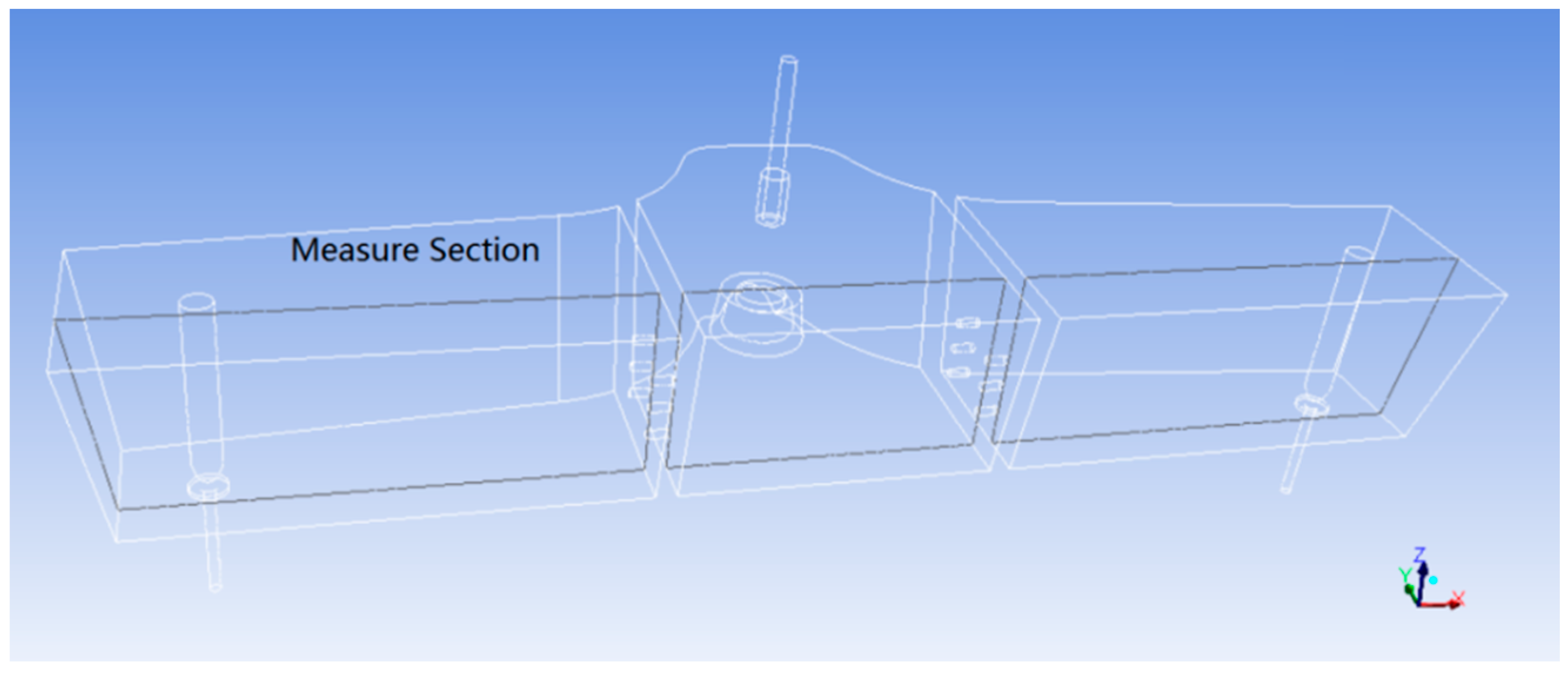
According to the flow path of the molten steel in the tundish, the measuring section is shown in
Figure 2. The measurement section is sequentially scanned with the PIV. The photographs by PIV are processed by PIVview software, then the flow field diagrams from different locations are merged into the full section. PIVview is a compact program package for the evaluation of particle image velocimetry with the PIV-Groups of the German Aerospace Center. The software is be developed in close cooperation with the Institute of Aerodynamics and Flow Technology of the German Aerospace Center in Goettingen, Germany.
4. Experimental Results and Discussion
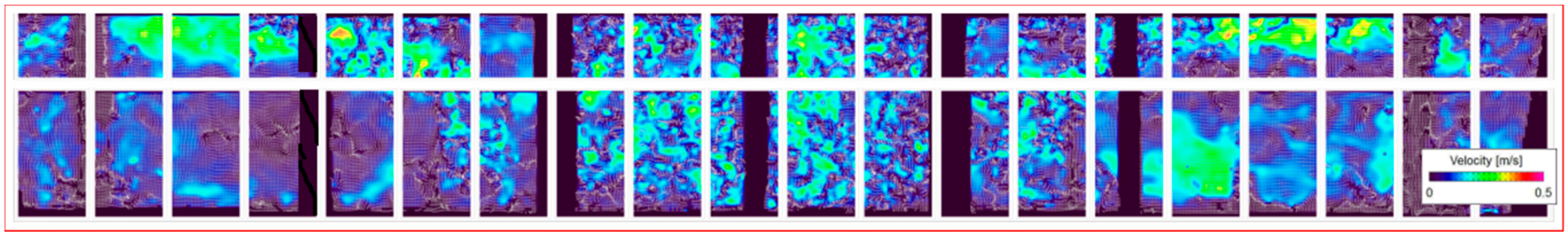
Figure 5 shows the sectional flow field of the tundish with PIV measurements and numerical simulation under schemes A. The position of the measured and simulated results is the same. The simulation shows only half. The position of two baffles with holes is indicated in the diagram. Of the black stripes that appear in the PIV measurement drawing, three are the supporting frames in the process of making the tundish model. The white stripes represent the results of different cameras at different times in
Figure 5c. The results obtained from the different method are shown together.
Figure 5c shows a very detailed velocity field in which the flow is more active in the inlet section. The very marked large circulation is readily apparent from the plot. It can be seen that flow in the tundish is a local pulse, partially rendering a lot of mixing, but the overall flow shows the trend of a certain flow movement.
Figure 5a also shows the flow field for the numerical simulation results obtained by test scheme A. The commercial CFD package FLUENT with ANSYS 15.0 is used to model and solve the tundish fluid flow and it is compared with the available experiment results. The tundish with a long nozzle and exit is modelled. A non-uniform Cartesian mesh is used, with a power-law expansion of cells from the walls outwards. The finest cells are placed next to the jet-impact wall, since that is where maximum resolution is needed for the fast-moving boundary layers. A fine mesh is also used to cover the jet region. In order to test the grid independence of the results, it is necessary to test the sensitivity of the results on the number of mesh points used. A total of about 8,500,000 cells are used in the finest calculation. The mathematical model is based on the assumptions of continuum hypothesis, the standard two equation model,
k-
ε equation is used to model the turbulence. A steady-state incompressible solution is adopted, with the main dependent variables being the pressure, three velocity components and the two turbulent quantities. The SIMPLE (Semi-Implicit Method for Pressure Linked Equations) algorithm is used for the pressure-velocity coupling and QUICK (Quadratic Upstream Interpolation for Convective Kinetics) scheme is used for discretization of momentum, turbulent equations.
As can be observed from the measurement results, the flow complexity in the tundish is far from the simple recirculating flow that is obtained by the Reynolds-averaged Navier-Stokes (RANS) equations, plus an appropriate turbulence model, combining large circulation and small-scale eddies. To gain more knowledge about the transient turbulence process, which cannot be achieved via Reynolds-averaged equations solutions, large-eddy simulations (LES) of the tundish flow field are performed. Alkishriwi et al. [
14,
15] and Jha et al. [
16,
17] have carried out such simulations to investigate the turbulent flow structure and vortex dynamics. They have confirmed that the metallurgical effect of the tundish is mainly accomplished by the flow behavior. LES simulation of the flow field in a tundish is conducted to analyze the flow structure, which determines to a certain extent the steel quality. Many intricate flow details have not been observed by customary RANS approaches.
The motion of the liquid steel is generated by jets into the tundish and continuous casting mold. The flow regime is mostly turbulent, but some turbulence attenuation can occur far from the inlet. The characteristics of the flow in a tundish include jet spreading, jet impingement on the wall, wall jets, and an important decrease of turbulence intensity in the core region of the tundish far from the jet. Compared to the numerical simulation, physical simulation for the flow field provides more details on the evolution of not only velocity but also eddies. Mathematical modeling may provide a much more detailed picture of velocity, turbulence, and temperature fields as a function of location and time. In general, the numerical simulation shows the flow trend, while the water simulation work presents flow details. The two means complement each other and provide a comprehensive understanding of the flow.
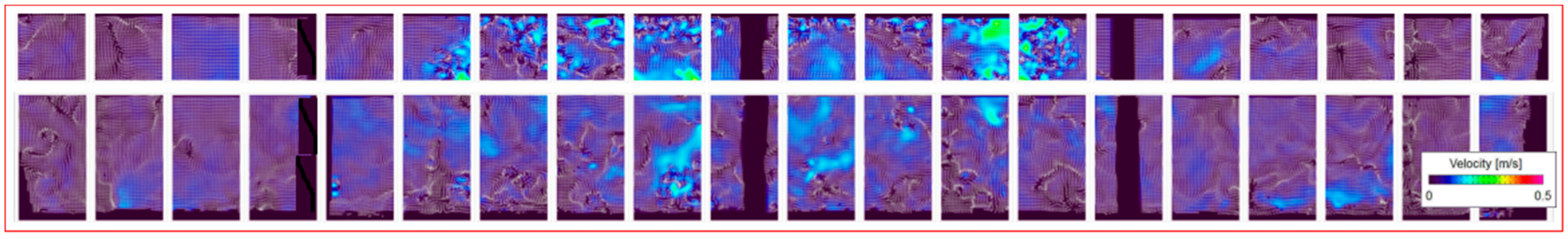
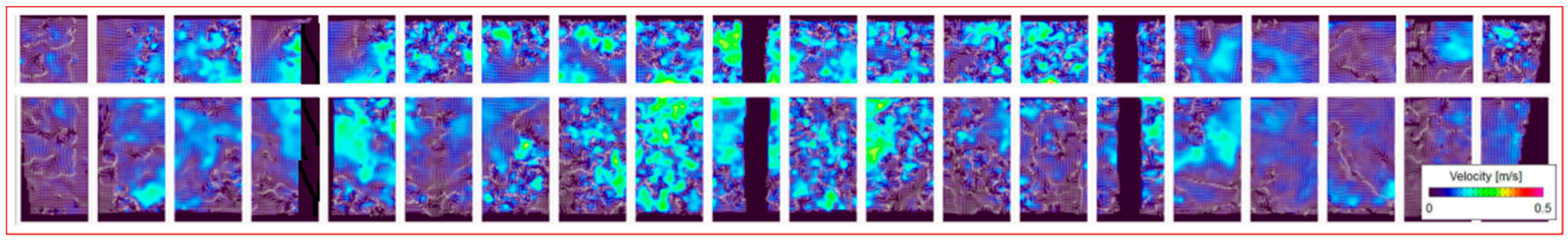
Figure 6,
Figure 7 and
Figure 8 shows the flow for schemes B, C, and D. C and D do not have baffles with holes. These results also show turbulent flow behavior in the tundish. Eddies can be clearly seen by measurement. Moreover, the evolution and interaction between eddies can be observed by the measurement of a large amount of transient data.
As can be seen from the results of the flow field in the tundish, the flow is typically turbulent and consists of eddies in motion. Turbulence exhibits random and orderly characteristics. One of its basic structures includes the eddies having various scales. Statistically, a large number of random small eddies form the background flow field, and large-scale eddies structures with quasi-ordered structures are statistically significant. The large eddies are limited and affected by flow boundary conditions and flow interfaces. The large eddies contain the turbulent kinetic energy, which is associated with the fluctuating velocity components. The small eddies, which are called the micro scale of turbulence, sometimes do not show up due to the limitation of image sampling setting and image resolution in PIVview. According to turbulence theory, the turbulent kinetic energy is being dissipated in the smaller eddies. The small eddies may also play an important role in promoting the coalescence of inclusion particles [
12].
The very important manifestation of turbulent flow is the presence of the eddies. The turbulent fluctuations will bring about a very effective mixing, mass (and also heat and momentum) can be readily transported by the eddies from one part of the fluid to the other [
12]. The metallurgical effect of the tundish needs to be exerted via the transmission effect of these eddies.
The eddy is a flowing form, the vorticity is a physical quantity. The mathematical definition of vorticity is extremely clear. The curl of the flow field is defined as the vorticity:
where
is the velocity, m/s;
is the vorticity, 1/s;
is the nabla operator. From the definition of vorticity, vorticity is caused by the velocity gradient in the flow field. The positions at which the velocity gradients are large exhibit high strain rates according to Newton’s law of viscosity, the flow’s viscosity depends on the strain rate. A causal relationship clearly exists between the vorticity and viscosity of the fluid. For homogeneous incompressible fluids, the vorticity is generated from the fluid-solid interface. Besides moving with the flow, the vorticity diffuses like heat. At the inlet section of the tundish, the impacting jet on the turbulence suppressor generates a large number of eddies. Owing to the fluid viscosity and flow control device, a large number of eddies are generated. For preventing the entrapped slag, the molten steel is required to flow out of the tundish outlet in a stable manner. With respect to the aspects of satisfying the requirements of process and the improvement on the metallurgical effect, the application of either a flow control device or a plug rod or increase in the distance between the inlet and outlet can avoid the interference of the inlet’s strong vortex flow on the outlet.
For a casting speed of 1.2 m·s−1 the theoretical velocity in the outlet section can be calculated to be 0.001 m·s−1 according to the flow rate and sectional area of the tundish. At such low relative average velocity, it is difficult to produce relatively intense heat, mass, and momentum transfer. However, owing to the strong impacting jet action occurring near the long nozzle and the effect of the flow control device, the turbulence occurring in the tundish is clear, and a large number of eddies exist.
By the comparison between the presence and absence of the baffles with holes, the flow control device exerts a fundamental change in the flow within the tundish. In the absence of the flow control device, the turbulent areas concentrate near the long nozzle. According to the analysis related to the reason for the generation of the flow eddies in the tundish, owing to the presence of the flow control device inside the tundish and the number of fluid–solid interfaces increasing, the vorticity generating positions increase, intensifying the momentum exchange of the internal flow and making the overall flow easily uniform.
In the presence of the baffles with holes, the flow is in accordance with the requirement of the designed flow control device, especially in the flow direction and flow velocity. After flow passes through the inlet section, the flow is “thrown up” through the 15° holes to the outlet section surface and flows out from the outlet after sufficient exchange with the surface. Simultaneously, a large circulation is formed in the outlet section. The flow control devices, properly installed, may create localized mixing in contained regions, which may help in inclusion agglomeration and hence, their removal [
18].
By doubling the casting speed, the shape of the flow does not significantly change. The overall velocity of the flow field and the turbulent intensity increase. In the presence of the flow control device, the surface velocity in water simulation can reach 0.3 m·s−1. After similarity conversion, the velocity of molten steel reaches 0.37 m·s−1. Such high speed may destroy the slag layer in the industrial production. Higher surface velocity could cause tundish slag entrainment at the slag/metal boundary due to turbulence arising and also cause refractory erosion near the nozzle or stopper, which requires the use of more expensive refractory practices.
By the millimeter-level spatial resolution of the tundish flow and the measurement and display at the millisecond-level time dimension scale, it is possible to achieve the precise understanding of the flow in the tundish. After combining the reality of the eddy structure and the evolution of eddies, an extremely comprehensive and precise understanding on the flow within the tundish can be achieved. These results provide a good precondition for examining the metallurgical effects of other tundishes on the basis of understanding the flow.