Prediction of Wrinkling of a Beverage Can Subjected to the Redrawing Process by J2 Deformation Theory
Abstract
1. Introduction
2. Classical Plasticity Theory
2.1. J2 Deformation Theory
2.2. J2 Flow Theory
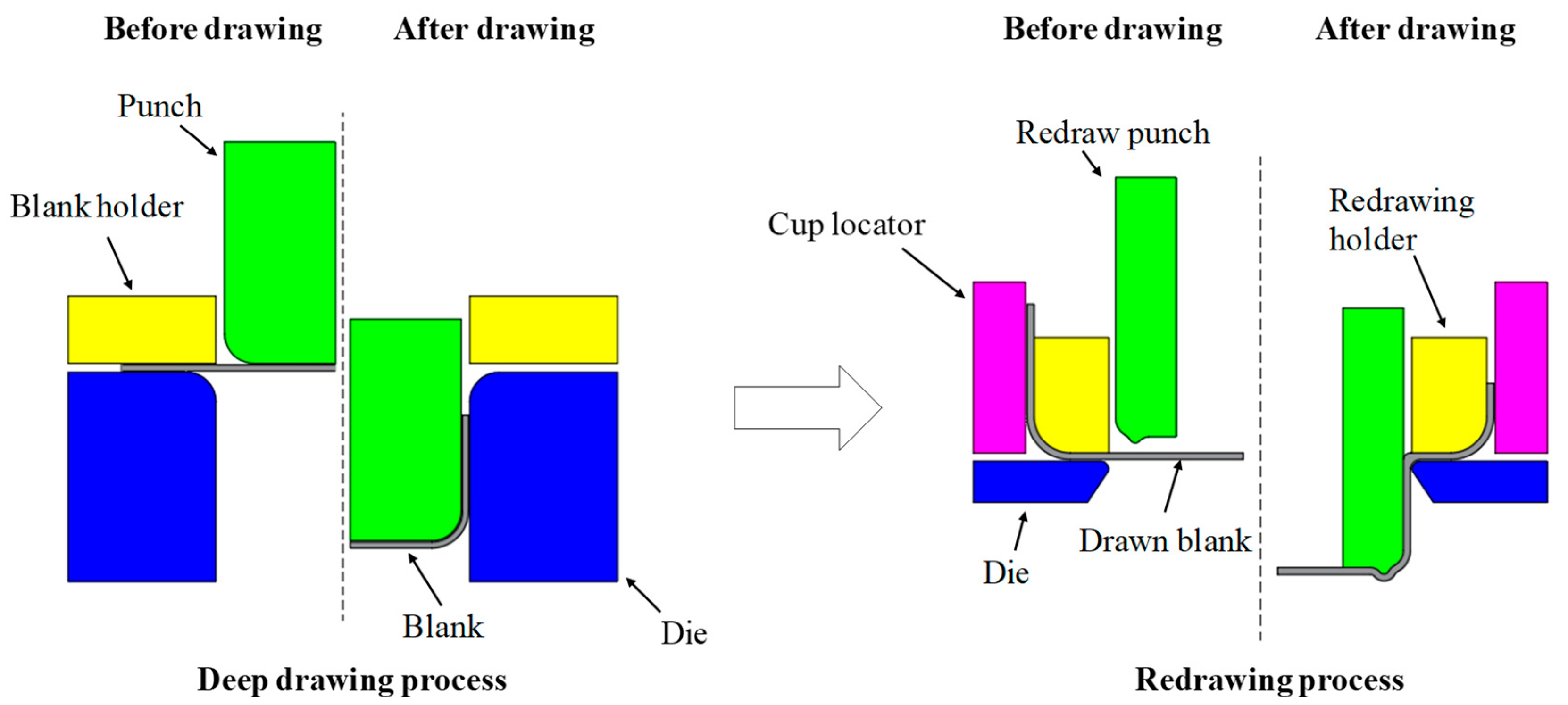
3. Experiment and Finite Element Models
3.1. Uniaxial Tensile Test
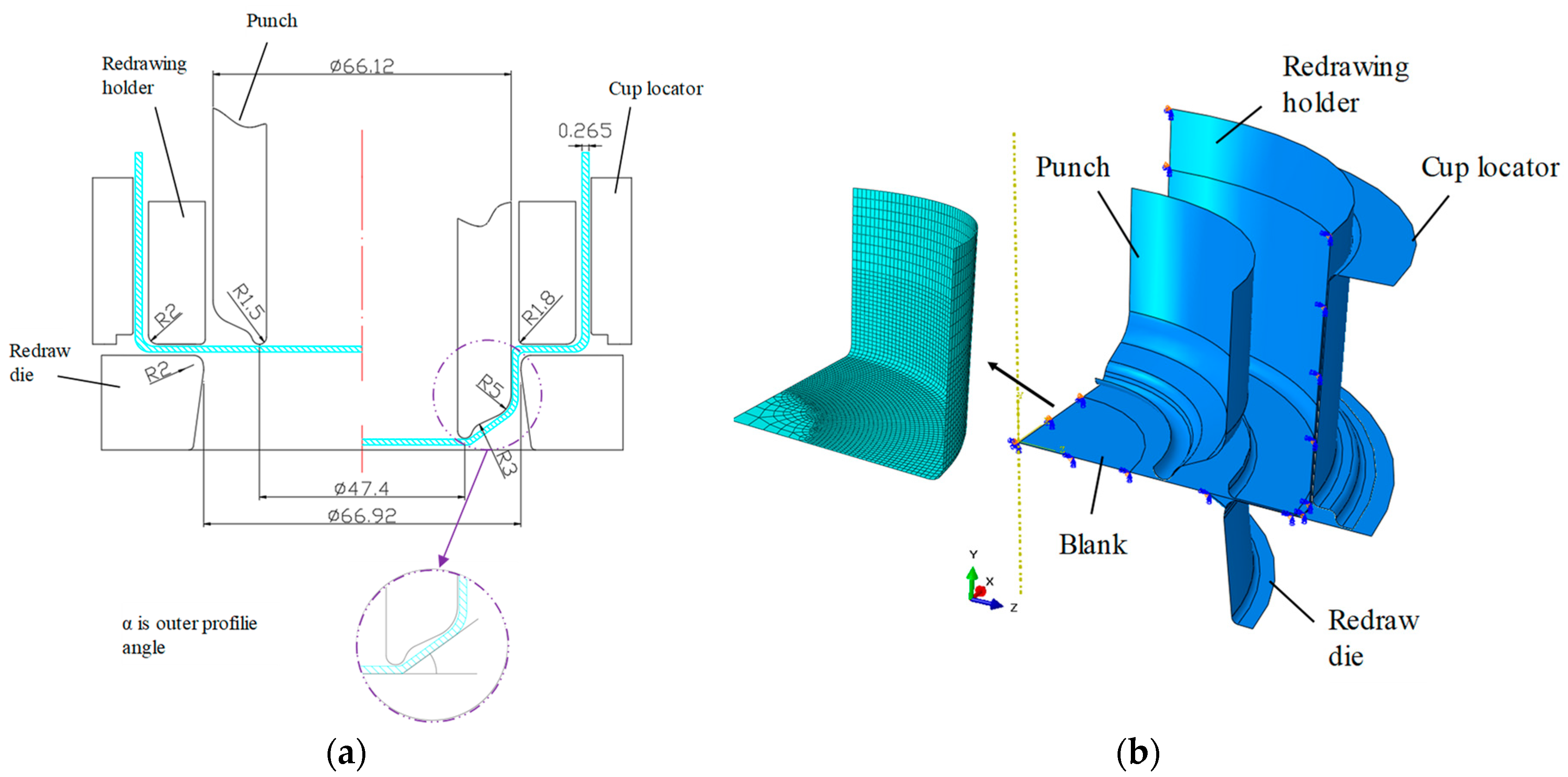
3.2. Geometrical Model
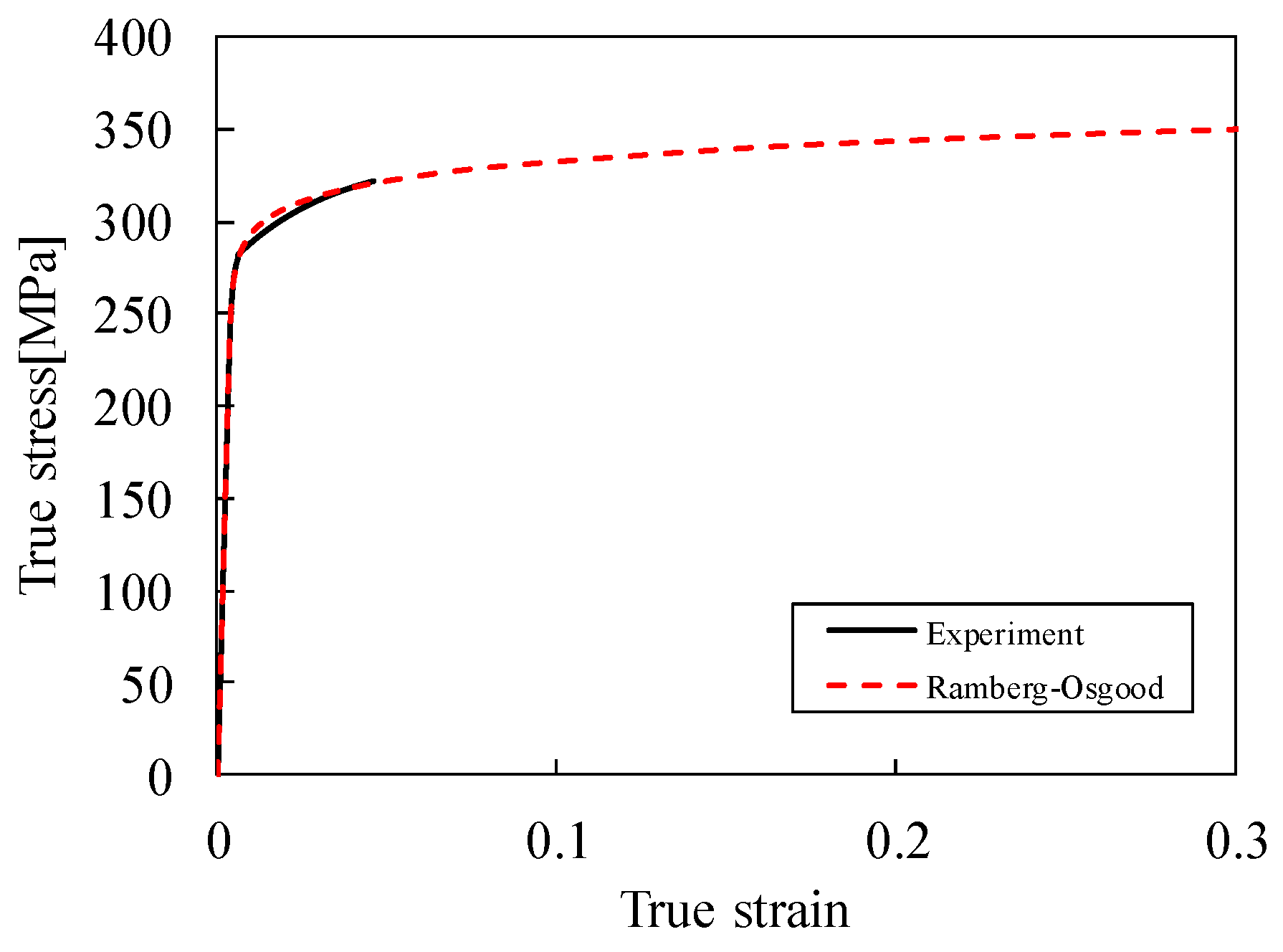
3.3. Material Model
4. Results and Discussions
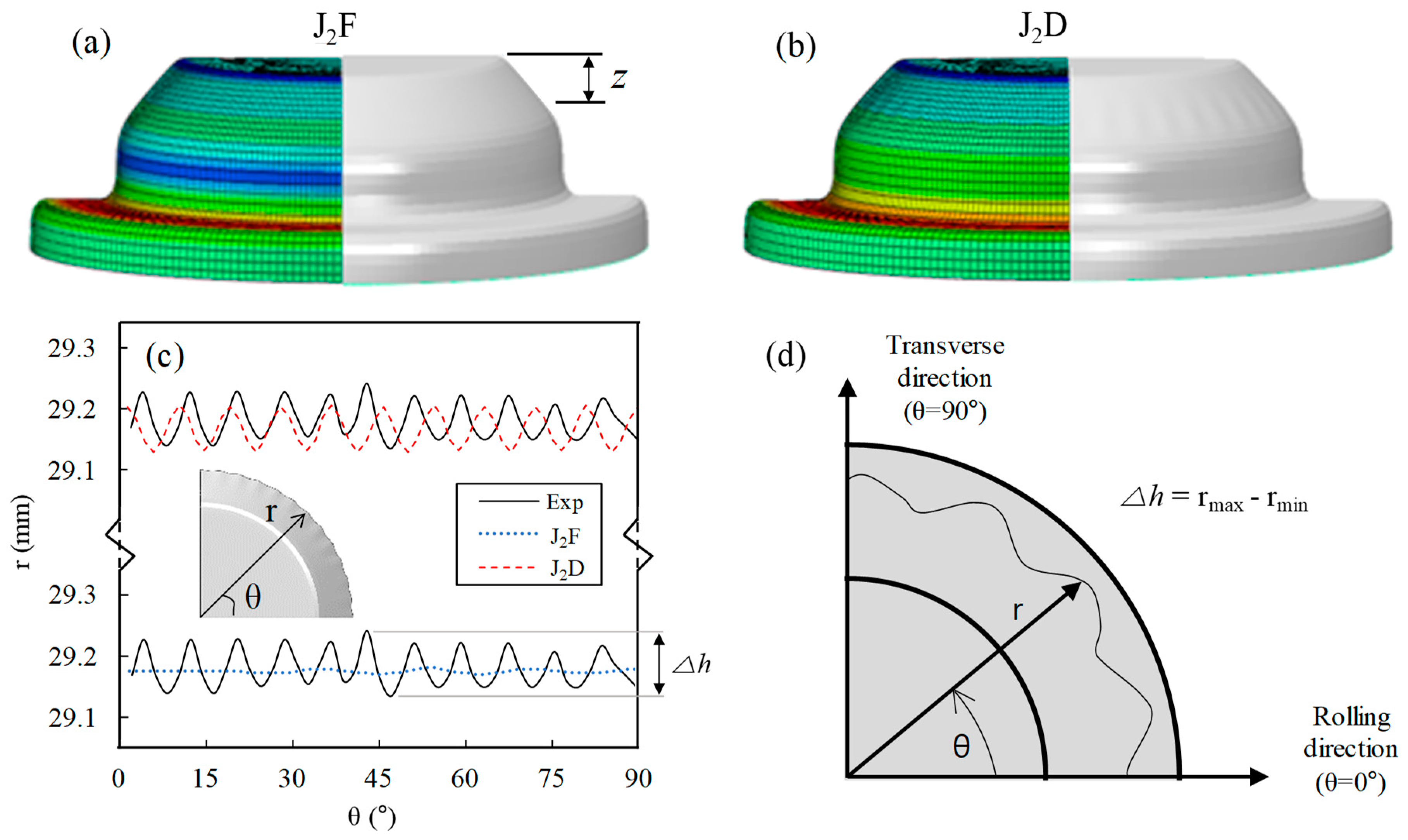
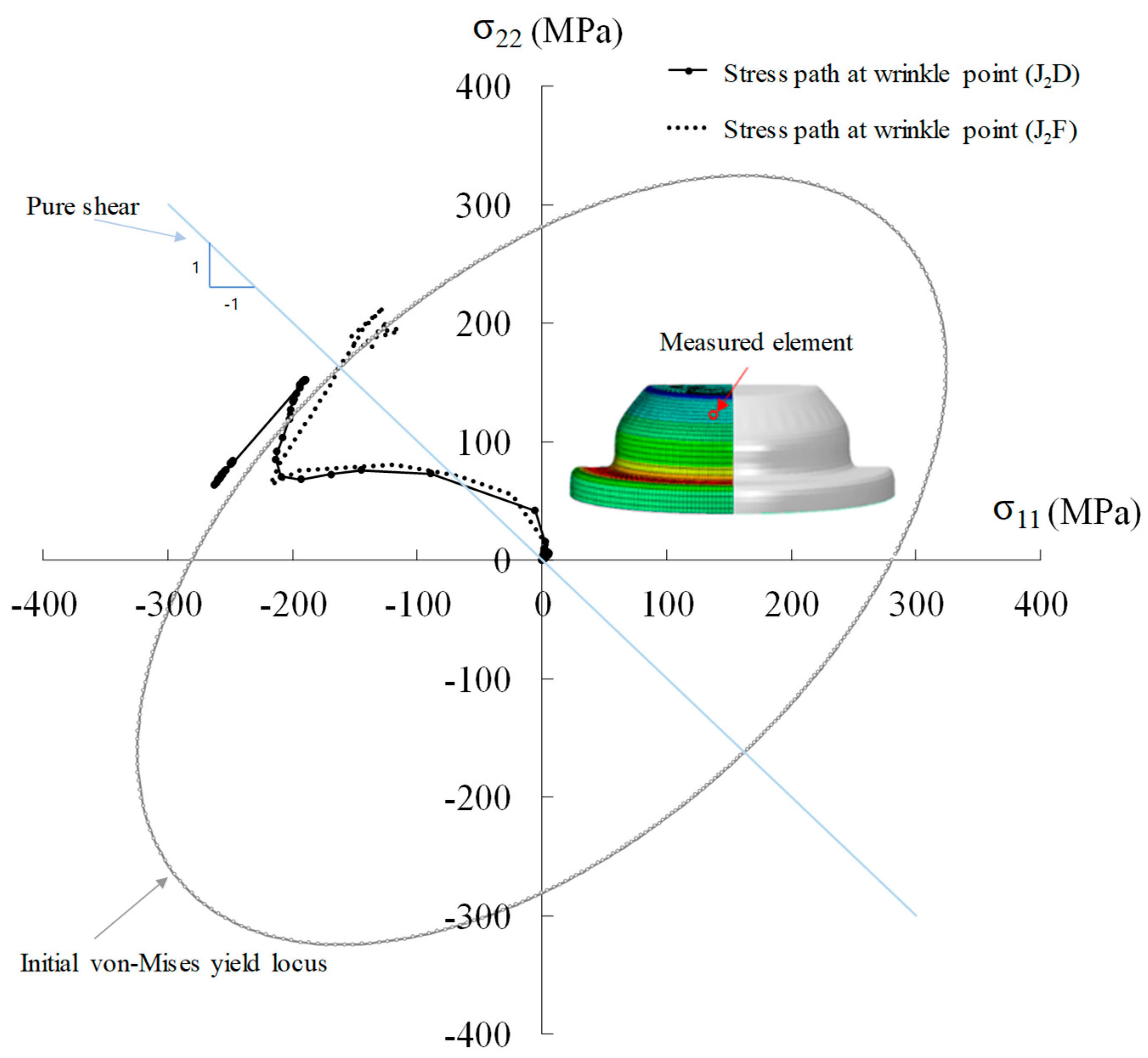
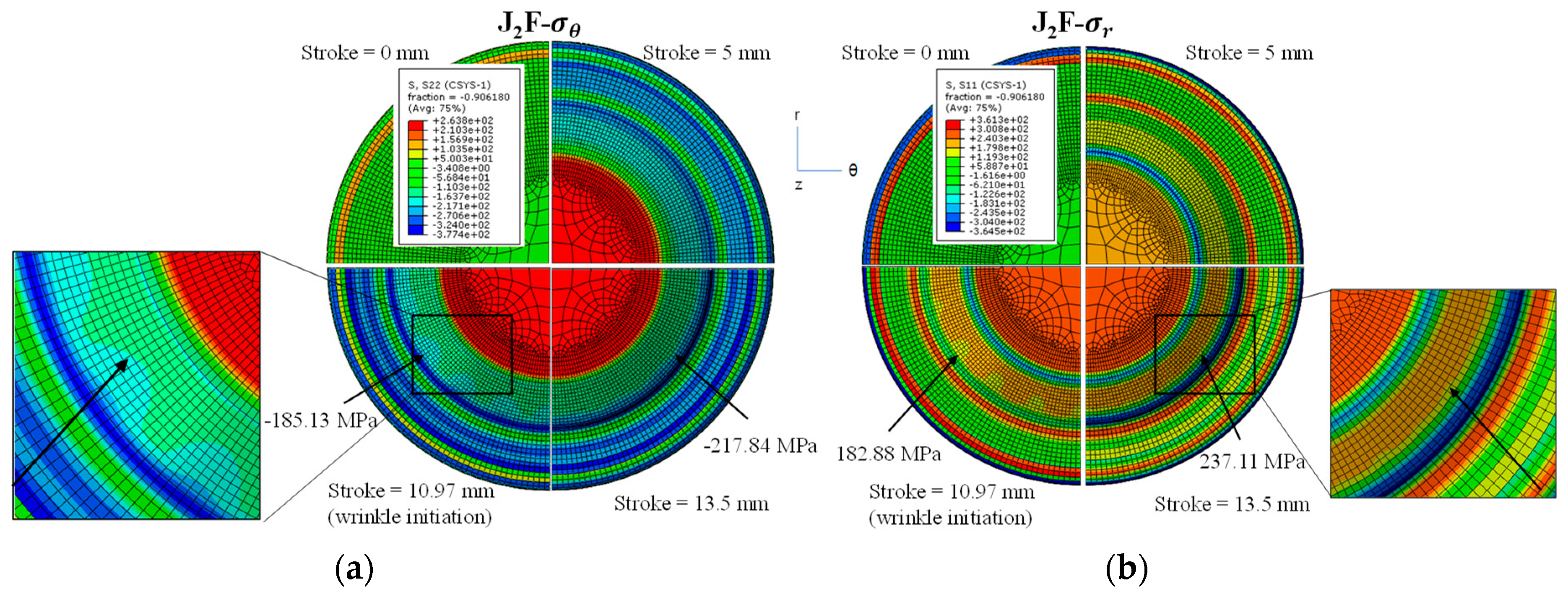
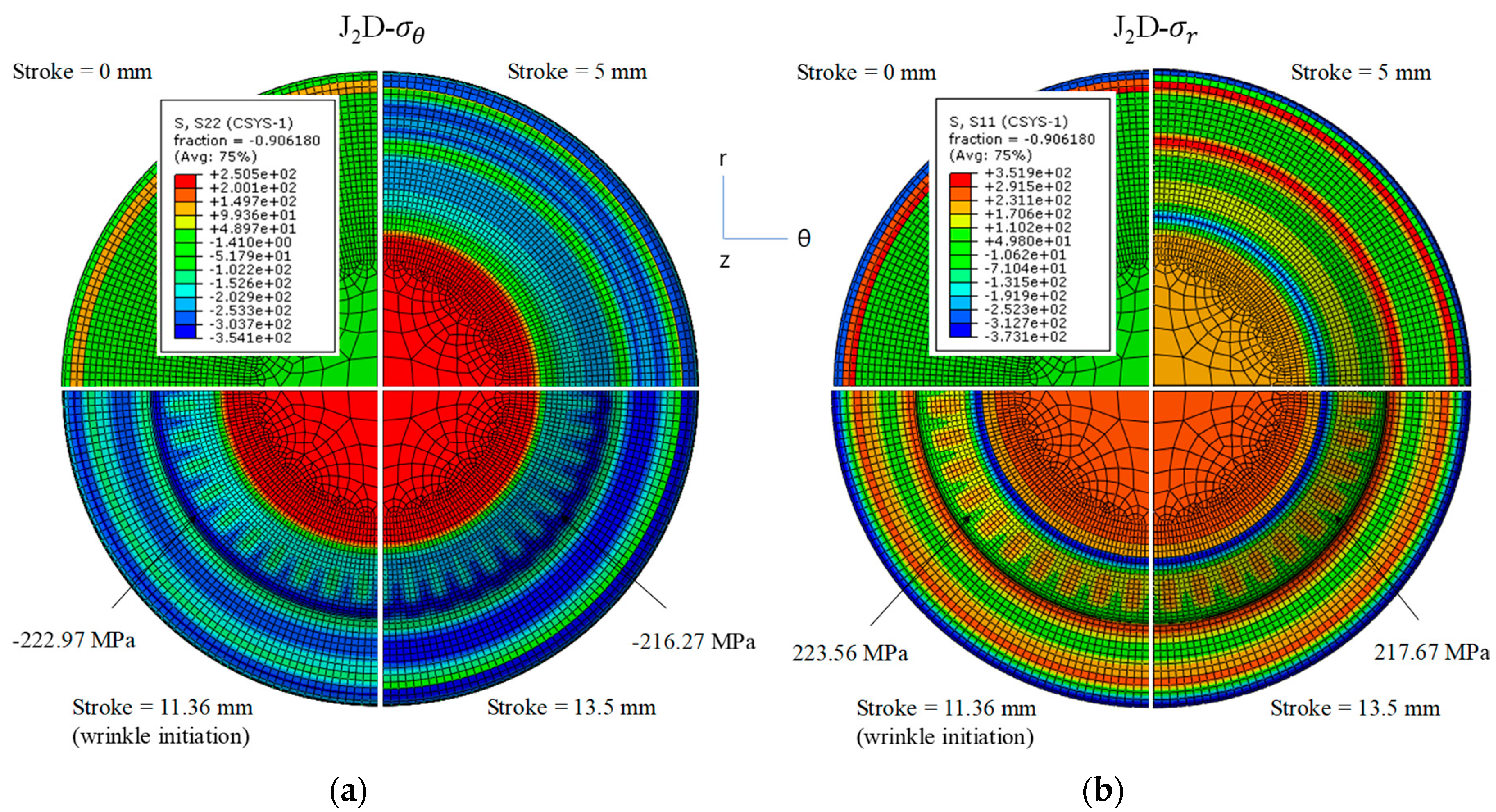
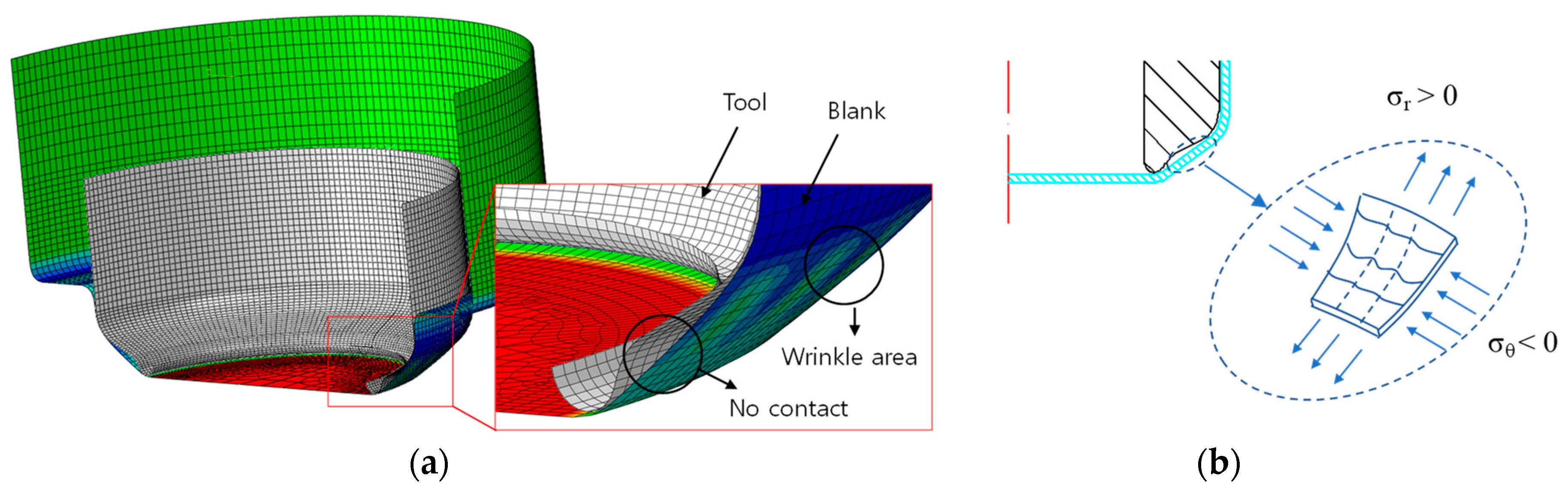
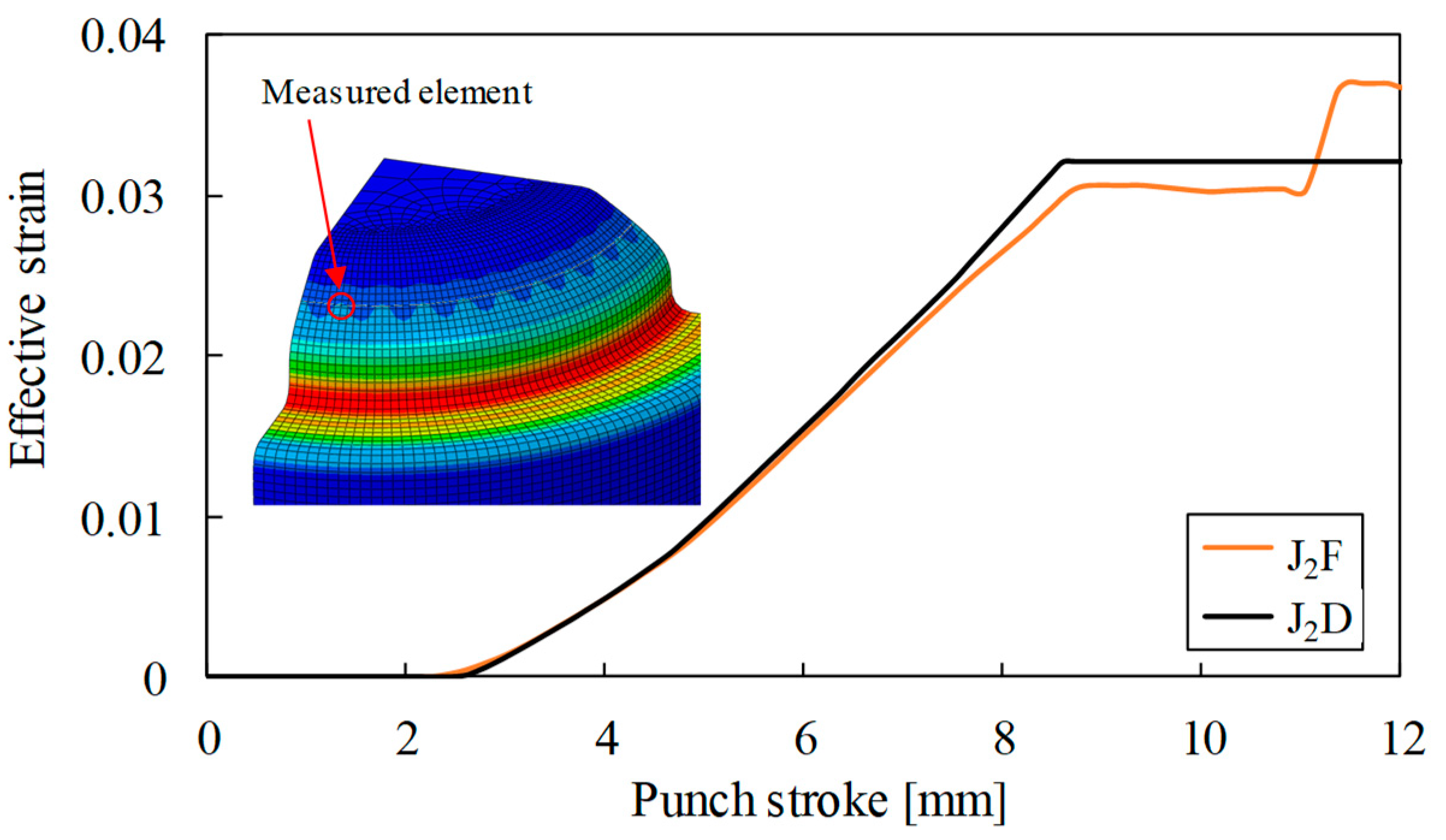
4.1. Wrinkling Prediction
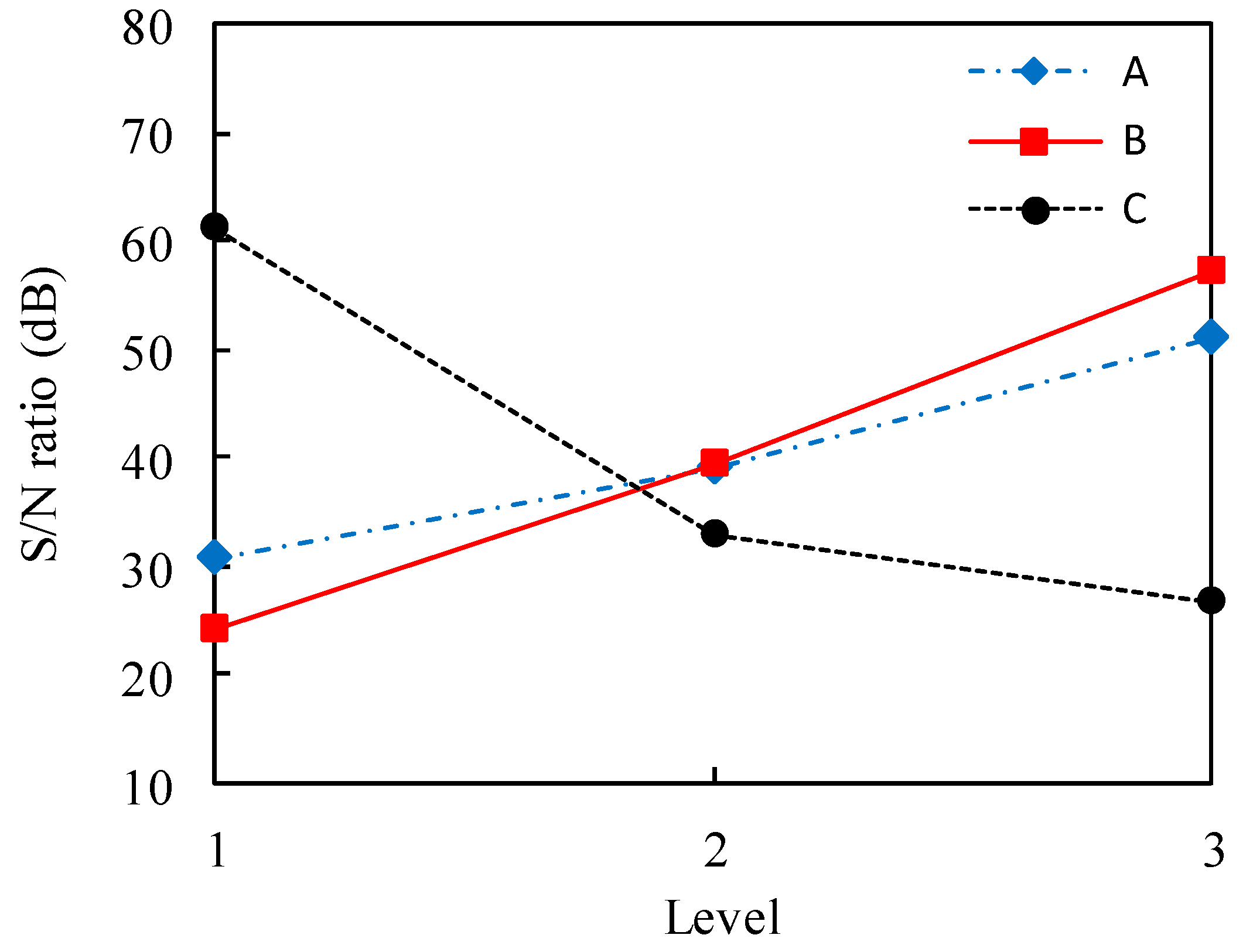
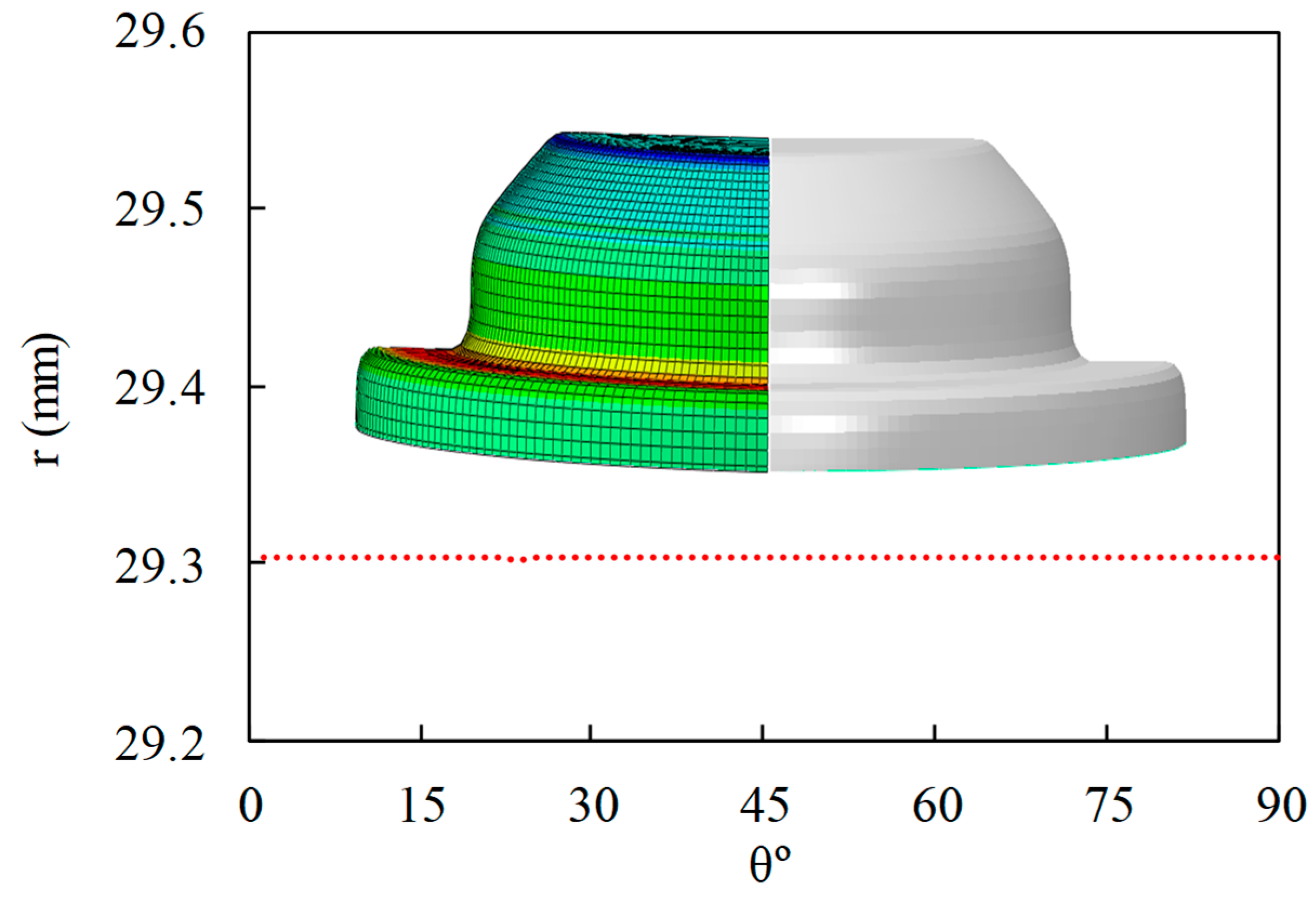
4.2. Wrinkle Optimization
5. Conclusions
- The equivalent plastic strain in the redrawing process for manufacturing beverage cans was as small as <0.04.
- J2F underestimated the amplitude and number of wrinkles.
- The amplitude and the number of wrinkles predicted using J2D was shown to be in agreement with the data measured from the sample.
- The stress paths obtained using J2F and J2D were compared to confirm the difference between them and it was confirmed that the stress path obtained using J2D was in the region dominated by compression.
- To optimize the design parameters, the Taguchi method and ANOVA and ANOM analyses were employed along with FE simulations based on J2D.
- The optimal condition for minimizing wrinkle formation was A3B3C1, which means that the S/N ratio of the friction coefficient μ was optimized in level 3 (μ = 0.07), the blank thickness was optimized in level 3 (t = 0.28), and the outer profile angle was optimized in level 1 (α = 34), respectively.
- A simulation with the optimal condition shows the result without wrinkle.
Author Contributions
Funding
Conflicts of Interest
References
- Moshksar, M.M.; Zamanian, A. Optimization of the tool geometry in the deep drawing of aluminum. J. Mater. Process. Technol. 1997, 72, 363–370. [Google Scholar] [CrossRef]
- Jensen, M.R.; Damborg, F.F.; Nielsen, K.B.; Danckert, J. Optimization of the draw-die design in conventional deep-drawing in order to minimise tool wear. J. Mater. Process. Technol. 1998, 83, 106–114. [Google Scholar] [CrossRef]
- Beritsprecher, T.; Wartzack, S. Neural network based modeling and optimization of deep drawing – extrusion combined process. J. Intell. Manuf. 2014, 25, 77–84. [Google Scholar] [CrossRef]
- Pham, Q.T.; Kim, Y.S. Identification of the plastic deformation characteristics of AL5052-O sheet based on the non-associated flow rule. Met. Mater. Int. 2017, 23, 254–263. [Google Scholar] [CrossRef]
- Pham, Q.T.; Lee, M.G. Characterization of the isotropic-distortional hardening model and its application to commercially pure titanium sheets. Int. J. Mech. Sci. 2019, 160, 90–102. [Google Scholar] [CrossRef]
- Lee, H.S.; Jung, D.W.; Jeong, J.H.; Im, S.Y. Finite element analysis of lateral buckling for beam structures. Computers&Structures 1994, 53, 1357–1371. [Google Scholar] [CrossRef]
- Riks, E. An incremental approach to the solution of snapping and buckling problems. Int. J. Solids Struct. 1978, 15, 529–551. [Google Scholar] [CrossRef]
- Wang, X.; Lee, L.H.N. Postbifurcation behavior of wrinkles in square metal sheets under Yoshida Test. Int. J. Plast. 1993, 9, 1–19. [Google Scholar] [CrossRef]
- Wang, C.T.; Kinzel, G.; Altan, T. Wrinkling criterion for an anisotropic shell with compound curvatures in sheet forming. Int. J. Mech. Sci. 1994, 94, 945–960. [Google Scholar] [CrossRef]
- Tomita, Y.; Shindo, A. Onset and growth of wrinkles in thin square plates subjected to diagonal tension. Int. J. Mech. Sci. 1994, 36, 945–960. [Google Scholar] [CrossRef]
- Gendy, A.S.; Saleeb, A.F. Generalized mixed finite element model for pre- and post-quasistatic buckling response of thin-walled framed structures. Int. J. Numer. Methods Eng. 1994, 37, 297–322. [Google Scholar] [CrossRef]
- Cao, J.; Boyce, M.C. Optimization of Sheet Metal Forming Processes by Instability Analysis. In Proceedings of the 5th International Conference on Numiform, Itchaca, NY, USA, 18–21 June 1995; pp. 675–679. [Google Scholar]
- Cao, J.; Boyce, M.C. Wrinkling behaviour of rectangular plates under lateral constraint. Int. J. Solids Struct. 1997, 34, 153–176. [Google Scholar] [CrossRef]
- Cao, J.; Wang, X. An analytical model for plastic wrinkling under tri-axial loading and its application. Int. J. Mech. Sci. 2000, 42, 617–633. [Google Scholar] [CrossRef]
- Wang, X.; Cao, J. On the prediction of side-wall wrinkling in sheet metal forming processes. Int. J. Mech. Sci. 2000, 42, 2369–2394. [Google Scholar] [CrossRef]
- Makinouchi, A. Sheet metal forming simulation in industry. J. Mater. Process. Technol. 1996, 60, 19–26. [Google Scholar] [CrossRef]
- Nam, J.B.; Han, K.S. Finite Element Analysis of Deep Drawing and Ironing Process in the Steel D&I Canmaking. ISIJ Int. 2000, 40, 1223–1229. [Google Scholar] [CrossRef]
- Rekas, A.; Latos, T.; Budzyn, R.; Kociolek, M.; Styrna, T. Numerical simulations of drawing and redrawing process of forming thin cylindrical element from aluminium series 3XXXX. Key Eng. Mater. 2015, 641, 218–231. [Google Scholar] [CrossRef]
- Kawka, M.; Olejnik, L.; Rosochowski, A.; Sunaga, H.; Makinouchi, A. Simulation of wrinkling in sheet metal forming. J. Mater. Process. Technol. 2001, 109, 283–289. [Google Scholar] [CrossRef]
- Kim, Y.S.; Son, Y.J. Study on wrinkling limit diagram of anisotropic sheet metals. J. Mater. Process. Technol. 2000, 97, 88–94. [Google Scholar] [CrossRef]
- Kim, J.B.; Yoon, J.W.; Yang, D.Y. Investigation into the wrinkling behaviour of thin sheets in the cylindrical cup deep drawing process using bifurcation theory. Int. J. Numer. Method Eng. 2003, 56, 1673–1705. [Google Scholar] [CrossRef]
- Hutchinson, J.W.; Neale, K.W. Sheet necking-II. Time-independent behavior. In Mechanics of Sheet Metal Forming; Springer: Boston, MA, USA, 1978; pp. 127–153. [Google Scholar]
- Hutchinson, J.W.; Neale, K.W. Finite Strain J2 Deformation Theory. In Proceedings of the IUTAM Symposium on Finite Elasticity, Bethlehem, PA, USA, 10–15 August 1980; Springer: Berlin/Heidelberg, Germany, 1982; pp. 237–247. [Google Scholar] [CrossRef]
- Fleck, N.A.; Hutchinson, J.W. A phenomenological theory for strain gradient effects in plasticity. J. Mech. Phys. Solids 1993, 41, 1825–1857. [Google Scholar] [CrossRef]
- Fleck, N.A.; Muller, G.M.; Ashby, M.F.; Hutchinson, J.W. Strain gradient plasticity: Theory and experiment. Acta Metall. Mater. 1994, 42, 475–487. [Google Scholar] [CrossRef]
- Hutchinson, J.W. Generalizing J2 flow theory—Fundamental issues in strain gradient plasticity. Acta Mech. Sin. 2012, 28, 1078–1086. [Google Scholar] [CrossRef]
- Cao, P.; Feng, D.; Zhou, C. A New Method to Achieve Equivalent Plastic Strain Explicit Form of J2 Plastic Isotropic Kinematic Hardening Model and Numerical Verification. Struct. Longev. 2012, 8, 193–206. [Google Scholar] [CrossRef]
- Neal, K.W.; TuGcu, P. A numerical analysis of wrinkle formation tendencies in sheet metals. Int. Numer. Methods Eng. 1990, 30, 1595–1608. [Google Scholar] [CrossRef]
- Dick, R.E.; Yoon, J.W. Wrinkling during Cup Drawing with NUMISHEET2014 Benchmark Test. Steel Res. Int. 2015, 86, 915–921. [Google Scholar] [CrossRef]
- Barlat, F.; Brem, J.C.; Yoon, J.W.; Chung, K.; Dick, R.E.; Lege, D.J.; Pourboghrat, F.; Choi, S.H.; Chu, E. Plane stress yield function for aluminum alloy sheets-part 1: Theory. Int. J. Plast. 2003, 19, 1297–1319. [Google Scholar] [CrossRef]
- Stören, S.; Rice, J.R. Localized necking in thin sheets. J. Mech. Phys. Solids 1975, 23, 421–441. [Google Scholar] [CrossRef]
- Dick, R.E. Improvements to the Beverage can Redraw Process using LSDYNA. In Proceedings of the International LS DYNA Users Conference, Dearborn, MI, USA, 19–21 May 2002. [Google Scholar]
- Ramberg, W.; Osgood, W.R. Description of Stress-Strain Curves by Three Parameters; National Advisory Committee for Aeronautics: Washington, DC, USA, 1943. [Google Scholar]

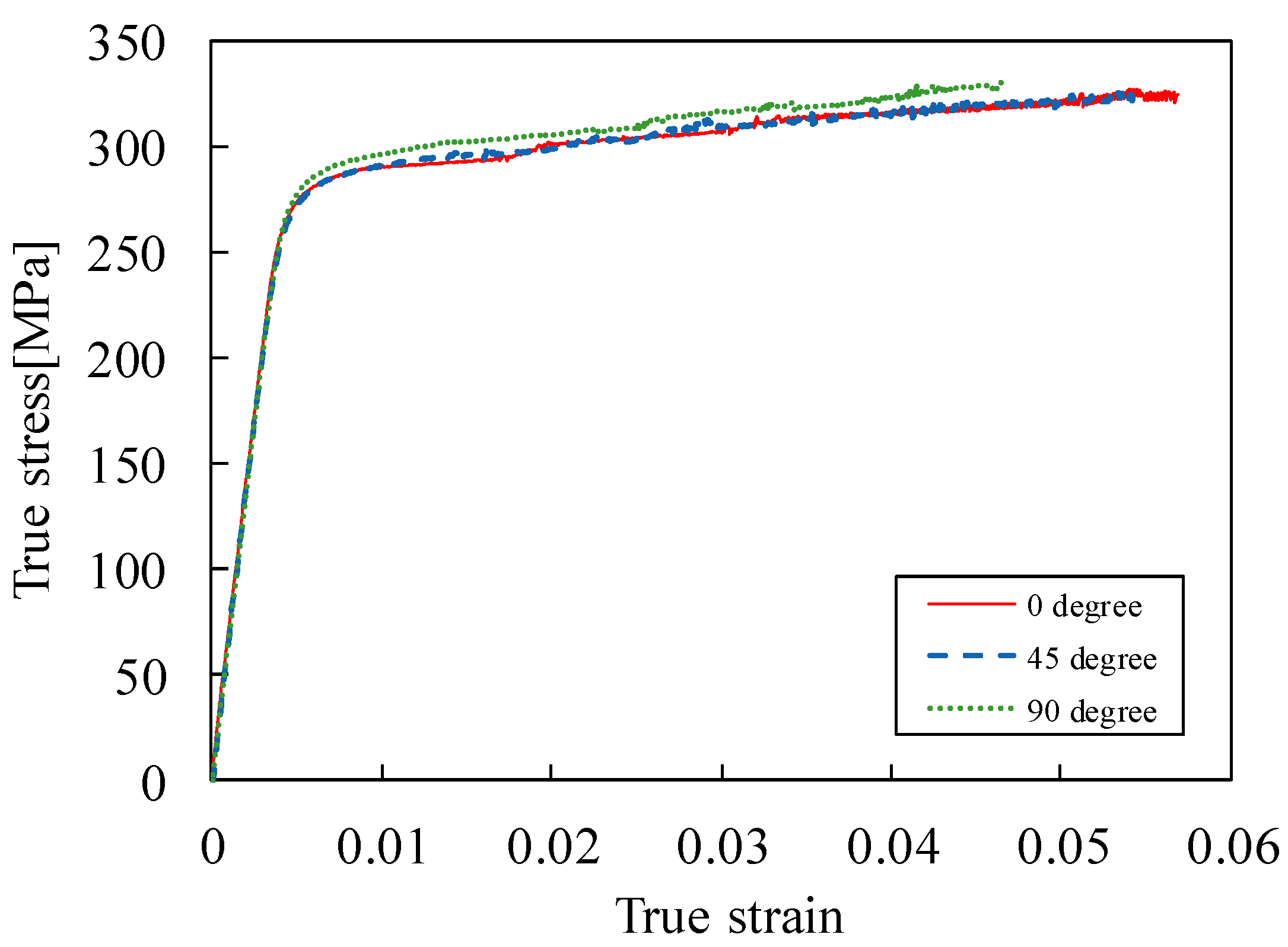
| Test Direction | E (GPa) | υ | UTS (MPa) | YS (MPa) | r-Value |
|---|---|---|---|---|---|
| 0° | 69.73 | 0.33 | 310.5 | 281 | 0.41 |
| 45° | 69.24 | 0.32 | 308.8 | 280 | 0.99 |
| 90° | 69.41 | 0.33 | 316.8 | 286 | 1.02 |
| Factor | Level | ||
|---|---|---|---|
| 1 | 2 | 3 | |
| A—Friction coefficient | 0.03 | 0.05 | 0.07 |
| B—Can thickness (mm) | 0.25 | 0.265 | 0.28 |
| C—Outer profile angle (°) | 34 | 37 | 40 |
| Simulation No. | A | B (mm) | C (°) | ∆h (mm) at z = 3.5 mm | S/N Ratio (dB) |
|---|---|---|---|---|---|
| 1 | 0.03 | 0.25 | 34 | 0.01912 | 34.370 |
| 2 | 0.03 | 0.265 | 37 | 0.07449 | 22.558 |
| 3 | 0.03 | 0.28 | 40 | 0.01818 | 34.808 |
| 4 | 0.05 | 0.25 | 37 | 0.15387 | 16.257 |
| 5 | 0.05 | 0.265 | 40 | 0.02338 | 32.623 |
| 6 | 0.05 | 0.28 | 34 | 0.00014 | 77.077 |
| 7 | 0.07 | 0.25 | 40 | 0.08488 | 21.424 |
| 8 | 0.07 | 0.265 | 34 | 0.00024 | 72.396 |
| 9 | 0.07 | 0.28 | 37 | 0.00103 | 59.743 |
| Factor | by Level (dB) | Degrees of Freedom | SS | Mean Square (%) ** | ||
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | ||||
| A | 30.58 | 38.89 | 51.19 * | 2 | 646.2 | 14.82 |
| B | 24.02 | 39.43 | 57.21* | 2 | 1656.2 | 37.99 |
| C | 61.28* | 32.85 | 26.52 | 2 | 2057.7 | 47.19 |
| Total | - | 6 | 4360.1 | 100 | ||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, J.J.; Nguyen, P.V.; Kim, Y.S. Prediction of Wrinkling of a Beverage Can Subjected to the Redrawing Process by J2 Deformation Theory. Metals 2019, 9, 1168. https://doi.org/10.3390/met9111168
Kim JJ, Nguyen PV, Kim YS. Prediction of Wrinkling of a Beverage Can Subjected to the Redrawing Process by J2 Deformation Theory. Metals. 2019; 9(11):1168. https://doi.org/10.3390/met9111168
Chicago/Turabian StyleKim, Jin Jae, Phu Van Nguyen, and Young Suk Kim. 2019. "Prediction of Wrinkling of a Beverage Can Subjected to the Redrawing Process by J2 Deformation Theory" Metals 9, no. 11: 1168. https://doi.org/10.3390/met9111168
APA StyleKim, J. J., Nguyen, P. V., & Kim, Y. S. (2019). Prediction of Wrinkling of a Beverage Can Subjected to the Redrawing Process by J2 Deformation Theory. Metals, 9(11), 1168. https://doi.org/10.3390/met9111168





