Aspects of Bending High-Borated Austenitic Stainless Steel Sheets for Interim Storage of Spent Nuclear Fuel
Abstract
:1. Introduction
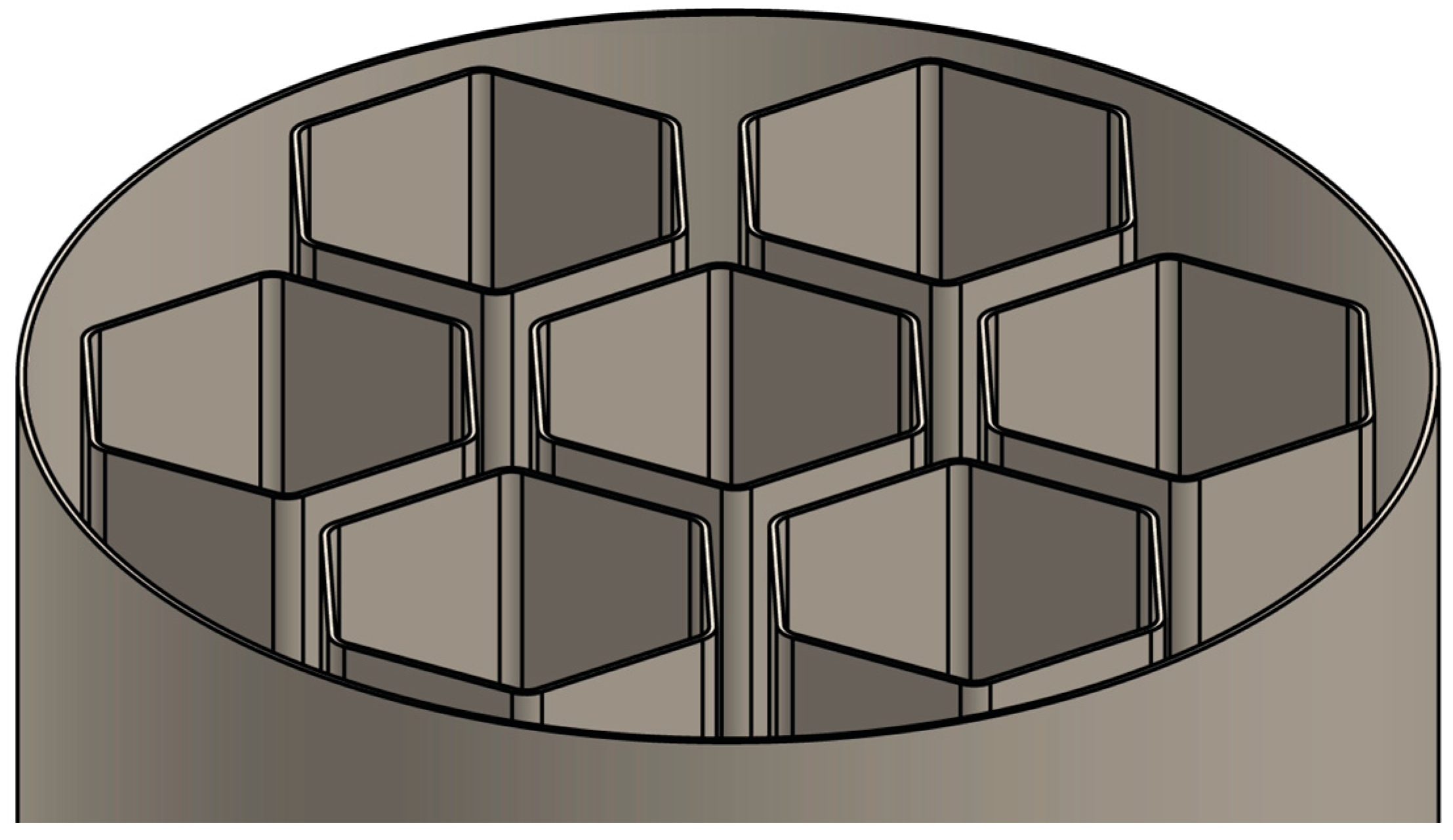
2. Materials and Methods
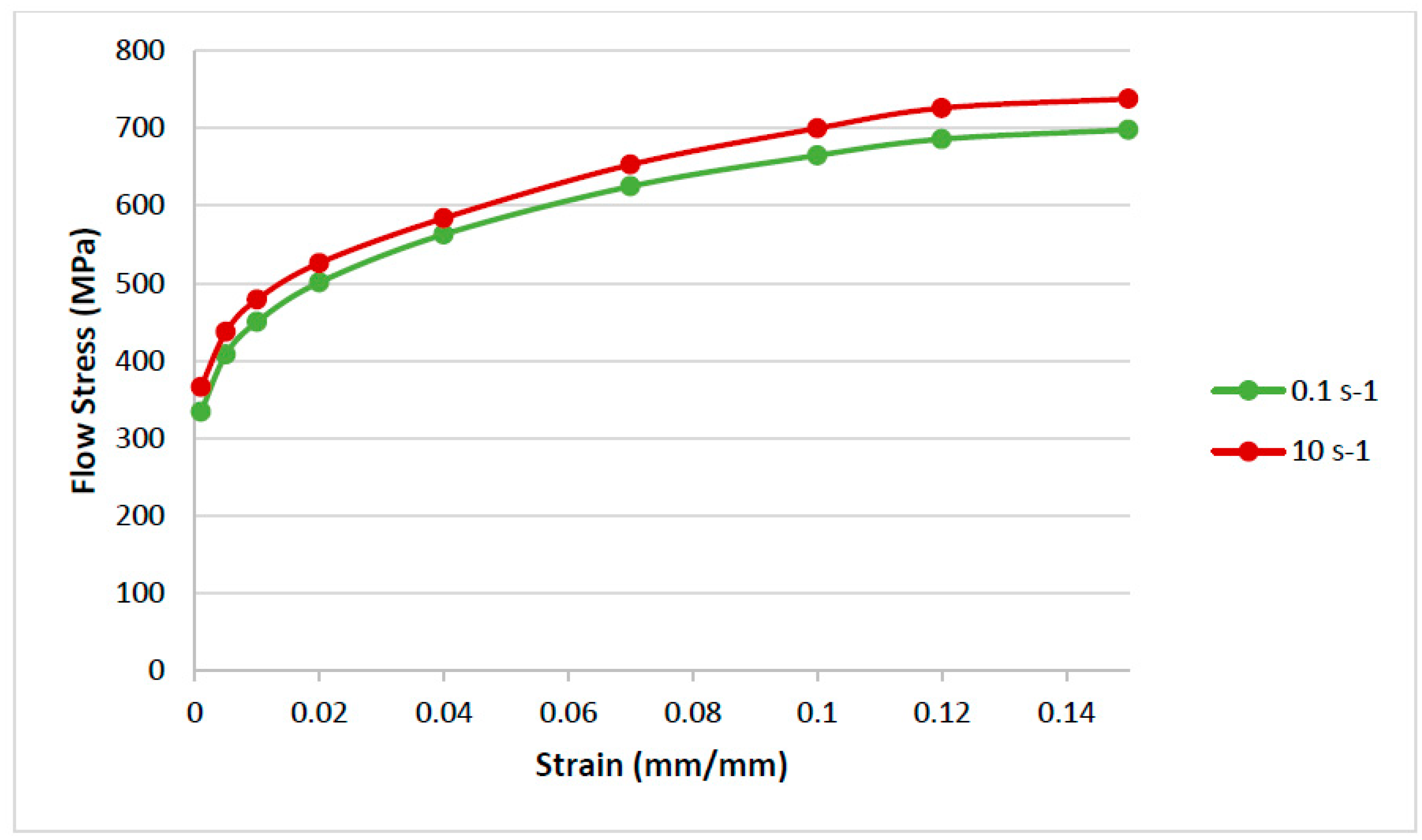
3. Results
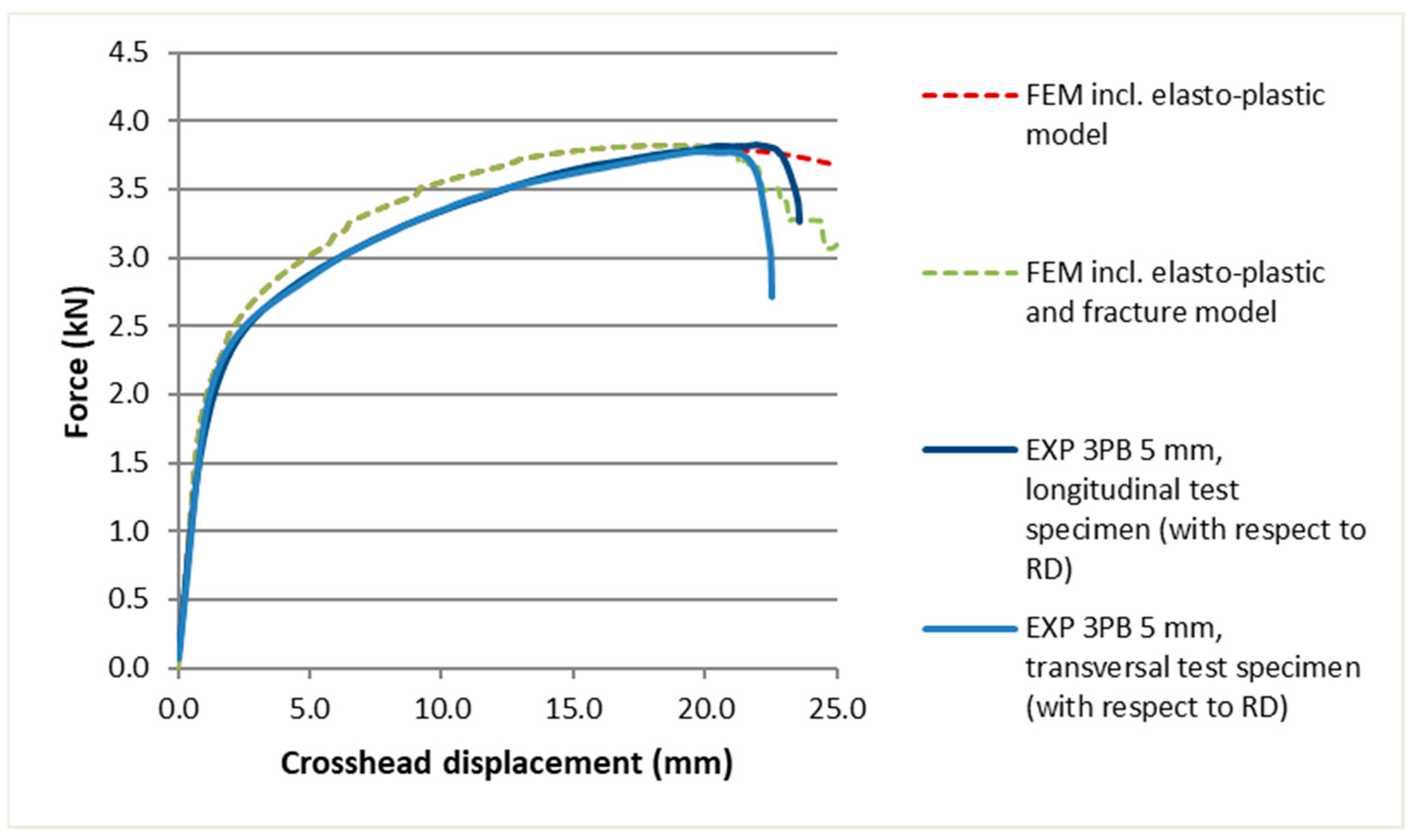
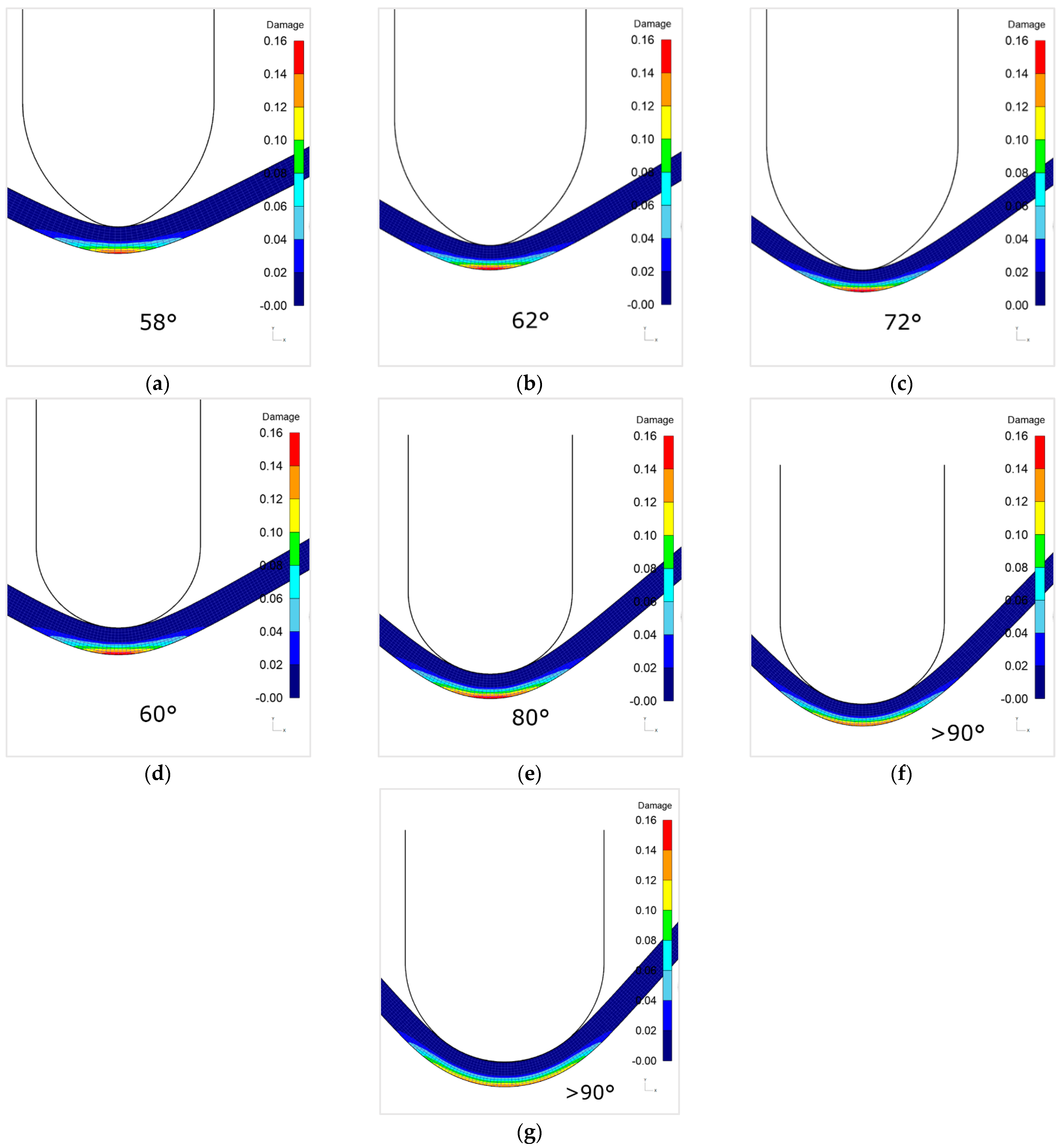
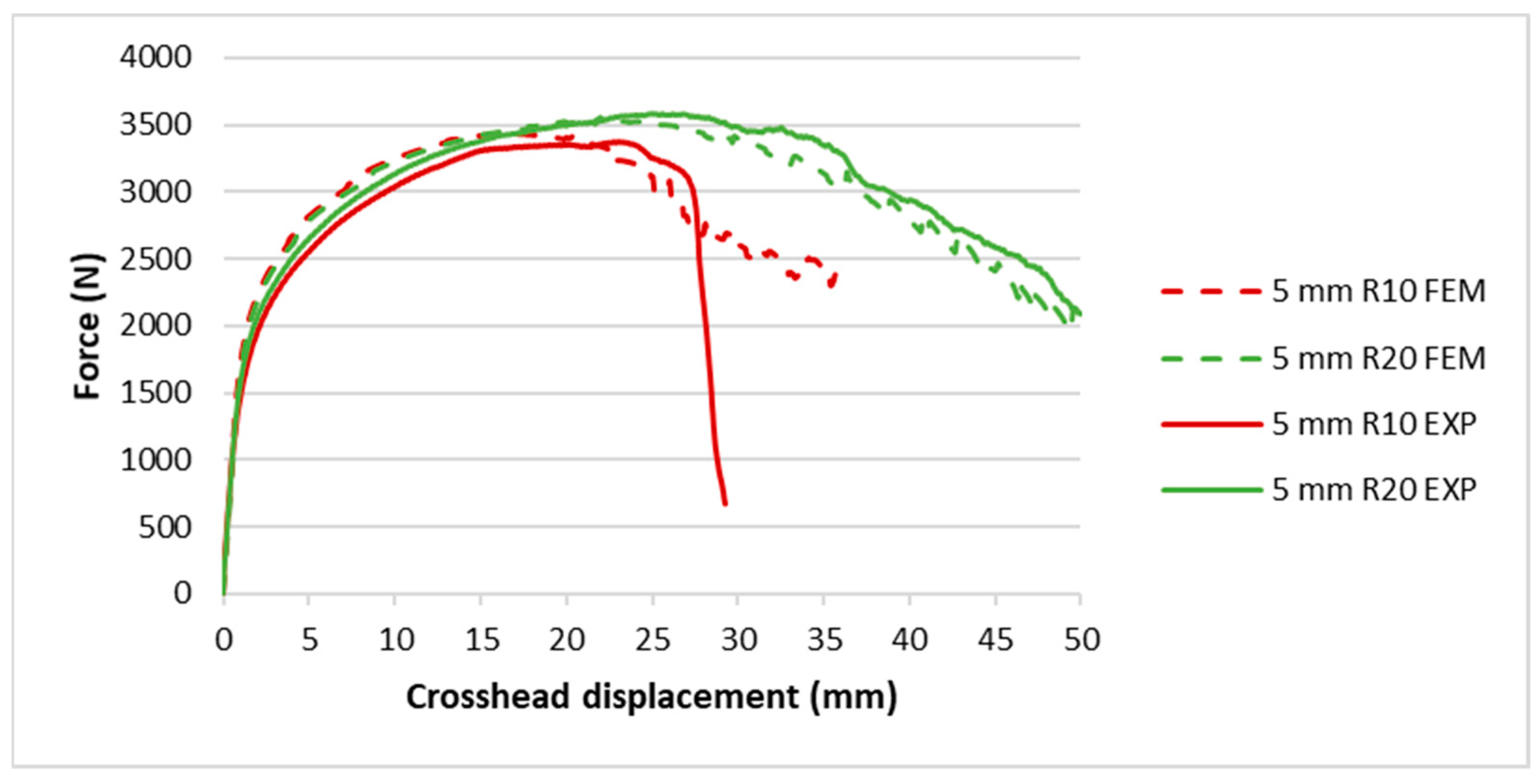
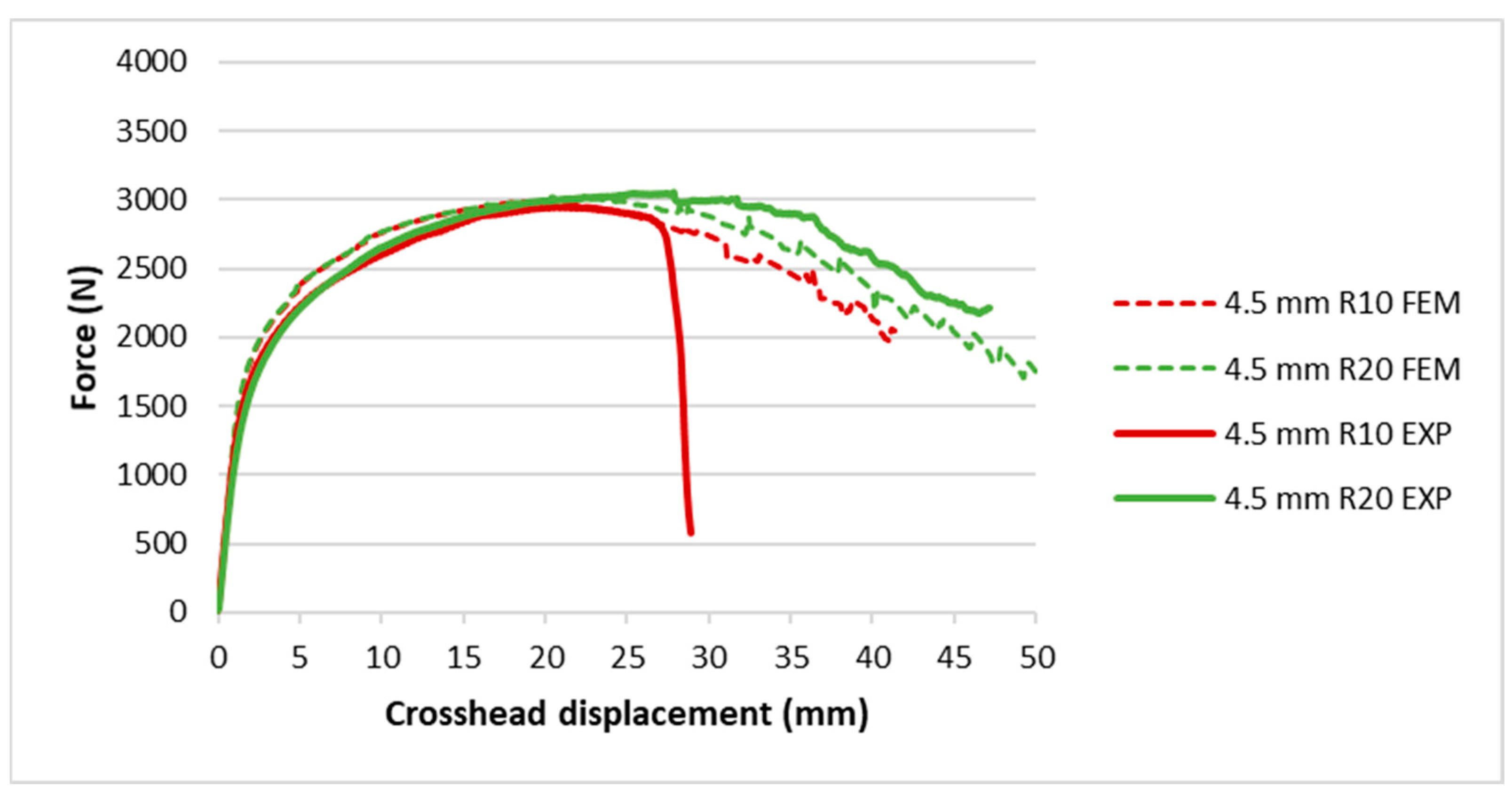
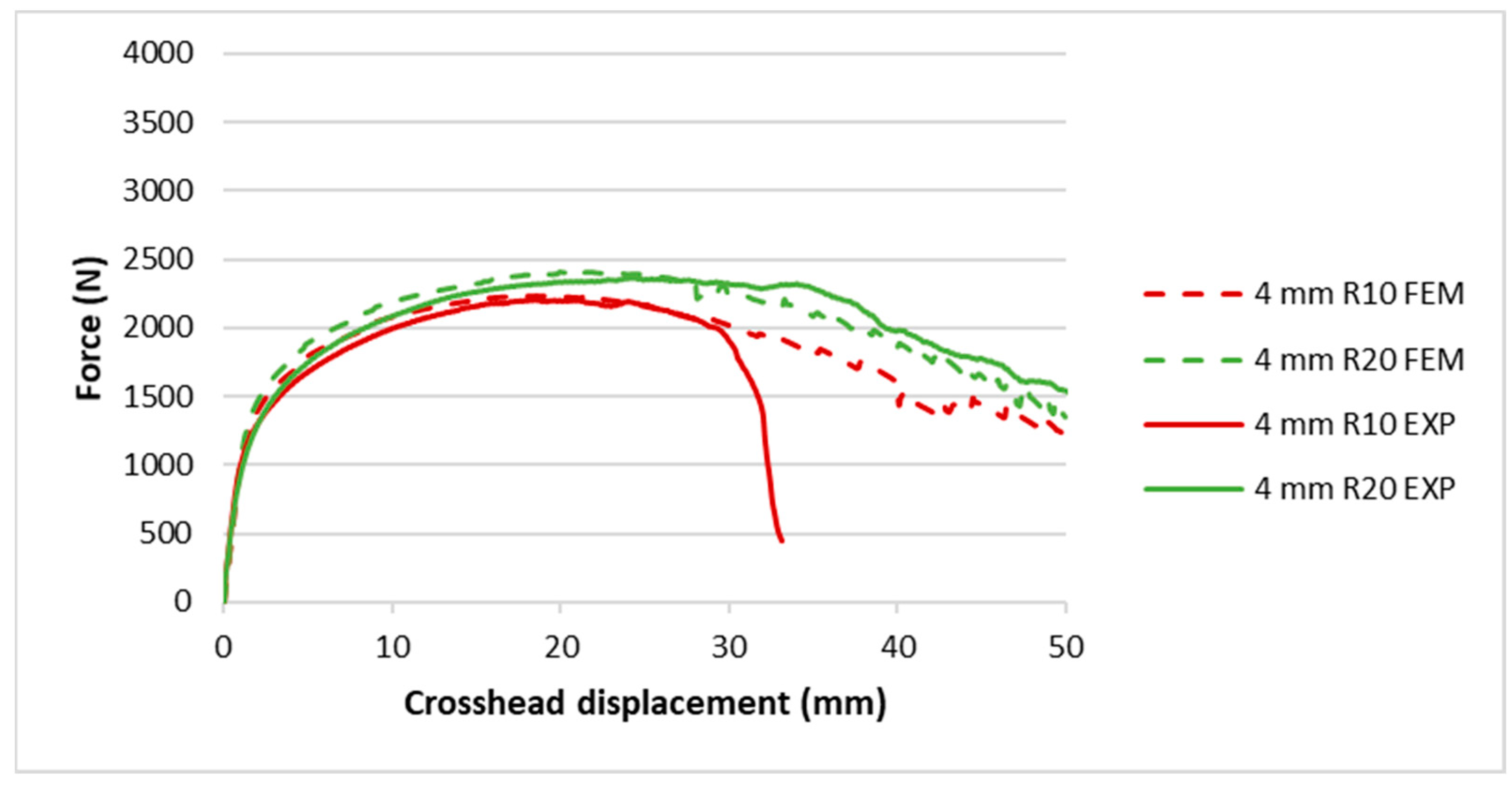
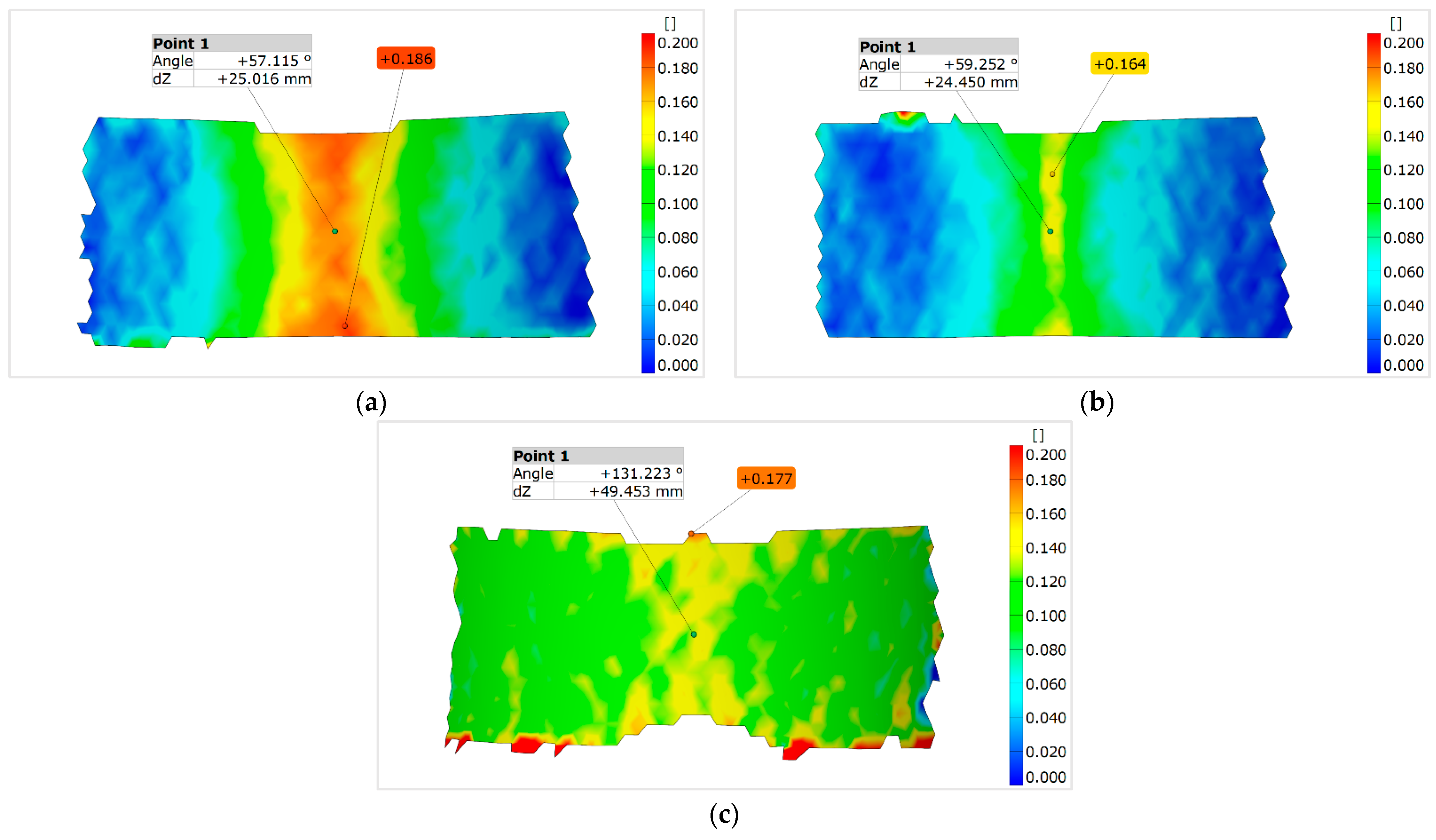
3.1. FEM Simulation and 3PB Experimental Results
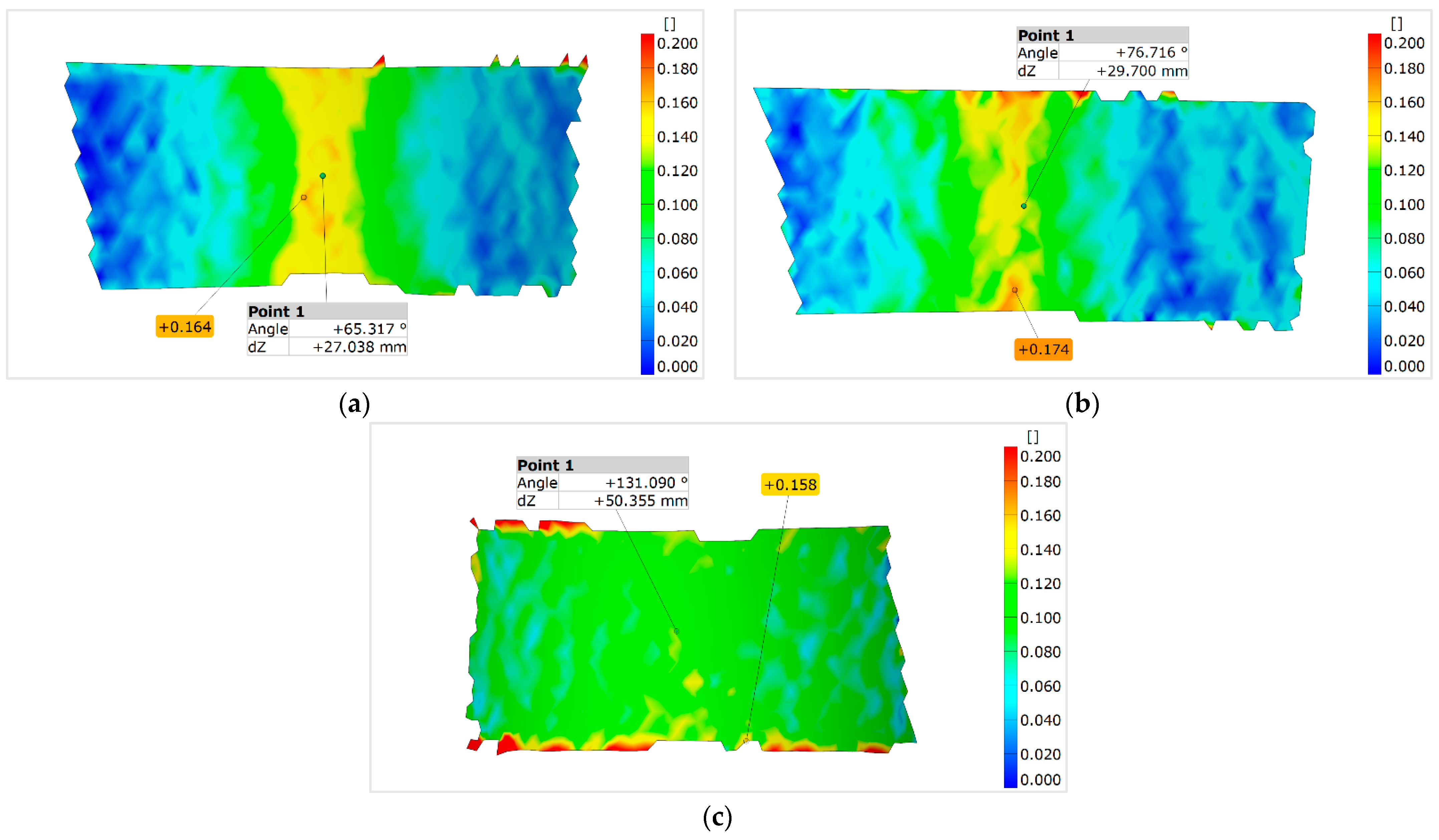
3.2. 3PB Test Variations
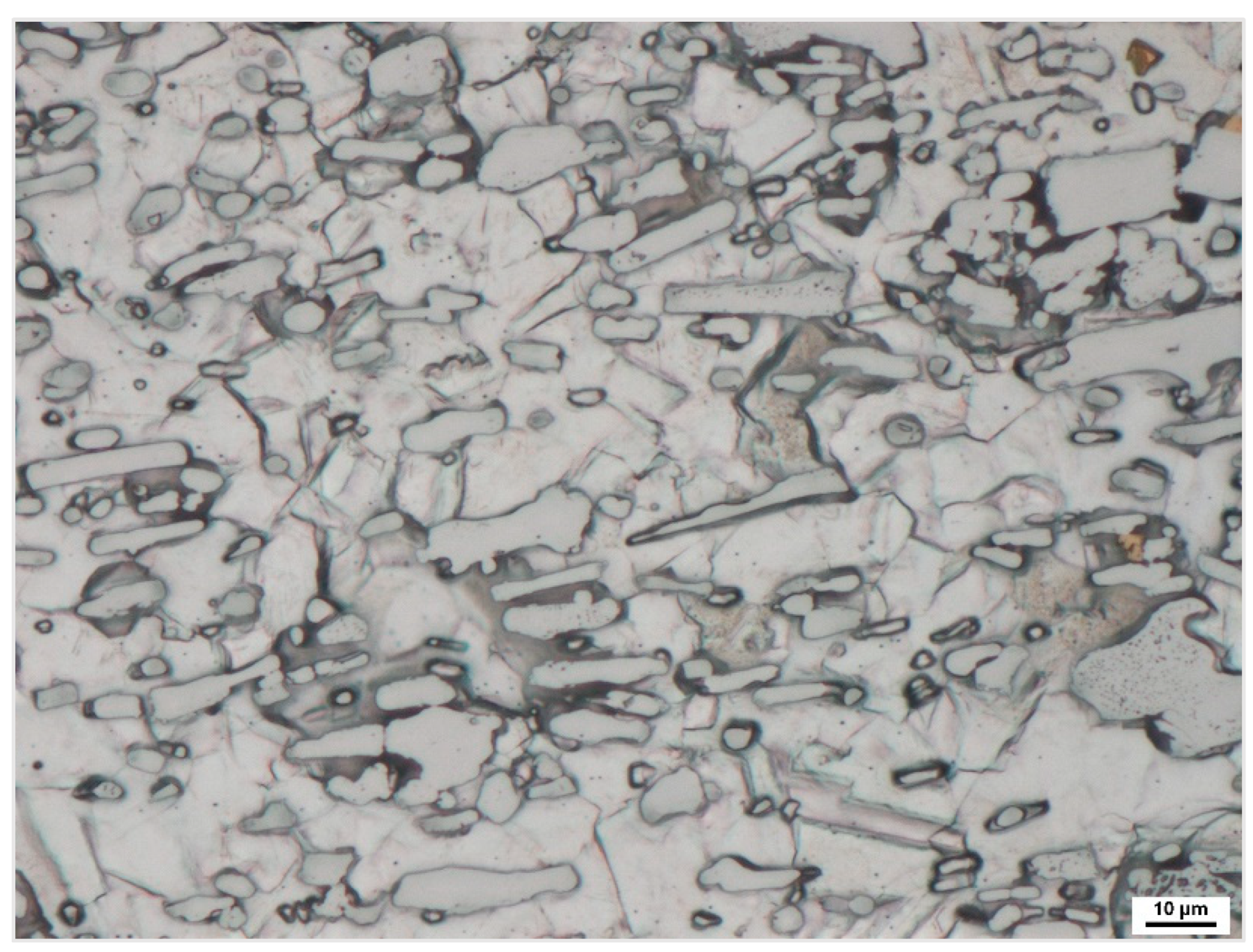
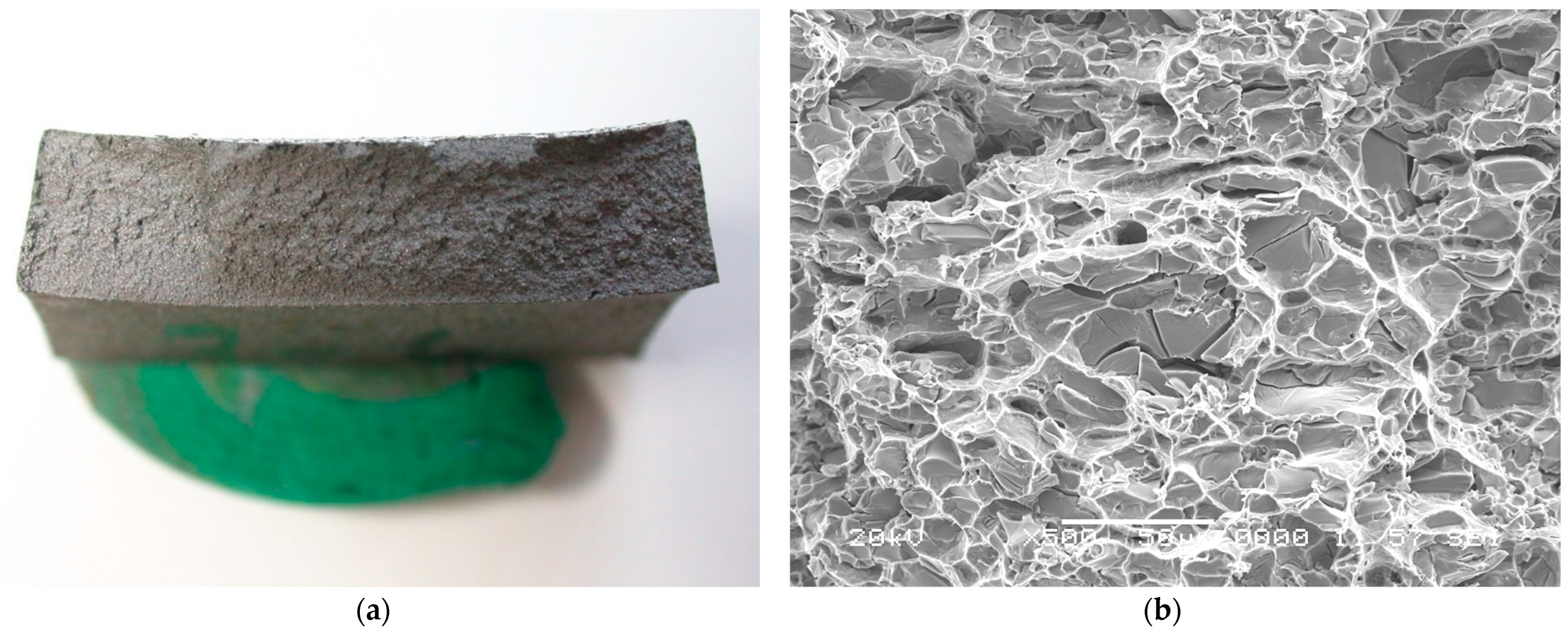
3.3. Fractography
4. Discussion
4.1. Delta Ferrite and Sigma Phase Embrittlement
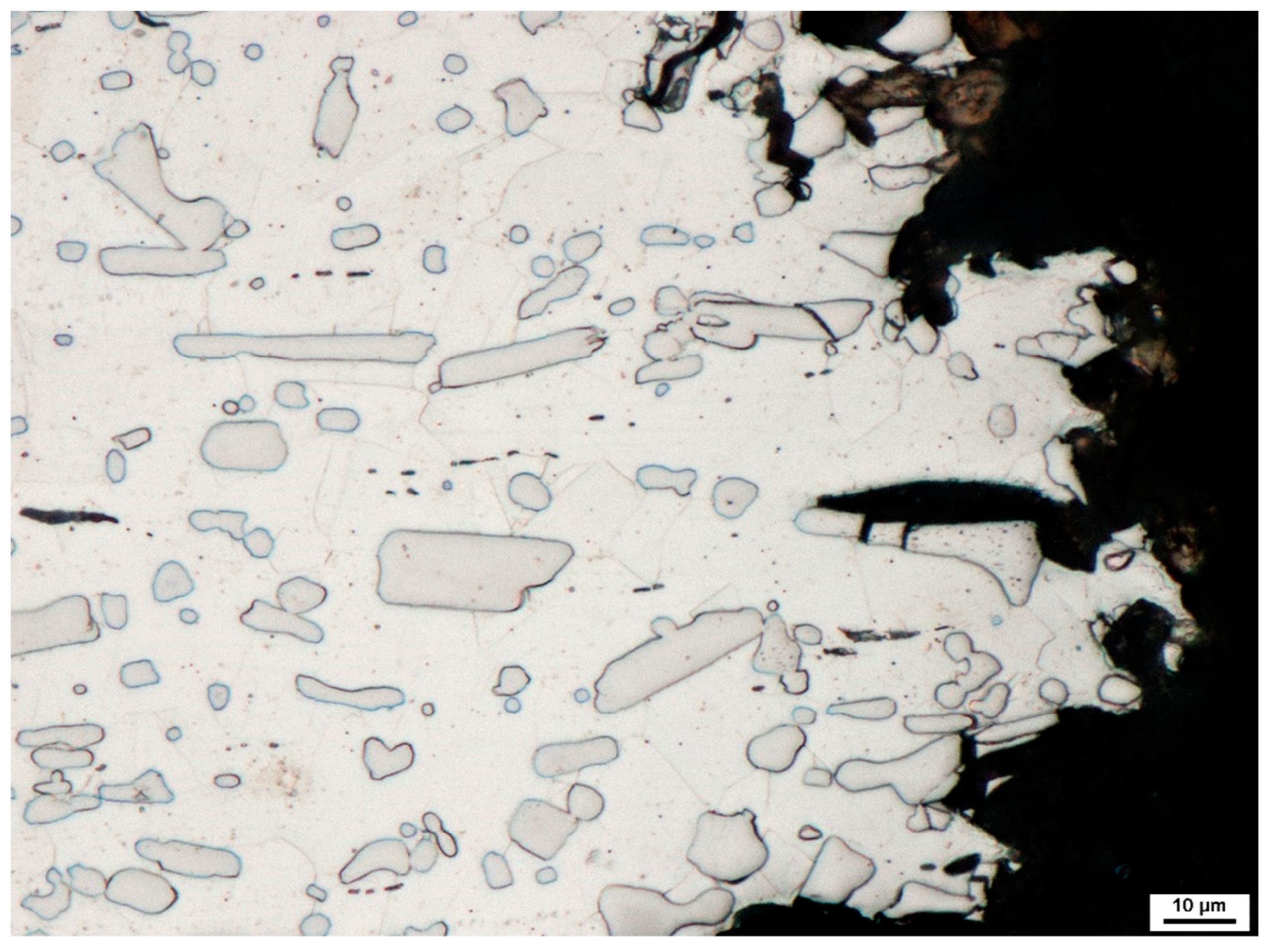
4.2. Grain Size Effect
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- IAEA. IAEA Technical Report No. 218—Storage of Water Reactor Spent Fuel in Water Pools; IAEA: Vienna, Austria, 1982; No. 218. [Google Scholar]
- Committee on the Safety and Security of Commercial Spent Nuclear Fuel Storage, Board on Radioactive Waste Management. Safety and Security of Commercial Spent Nuclear, Illustrate; National Academies Press: Washington, DC, USA, 2006. [Google Scholar]
- Soliman, S.E.; Youchison, D.L.; Baratta, A.J.; Balliett, T.A. Neutron Effects on Borated Stainless Steel. Nucl. Technol. 1991, 96, 346–352. [Google Scholar] [CrossRef]
- Sadawy, M.; El Shazly, R. Nuclear radiation shielding effectiveness and corrosion behavior of some steel alloys for nuclear reactor systems. Def. Technol. 2019, 15, 621–628. [Google Scholar] [CrossRef]
- Stanisz, P.; Oettingen, M.; Cetnar, J. Development of a Trajectory Period Folding Method for Burnup Calculations. Energies 2022, 15, 2245. [Google Scholar] [CrossRef]
- Cetnar, J.; Stanisz, P.; Oettingen, M. Linear Chain Method for Numerical Modelling of Burnup Systems. Energies 2021, 14, 1520. [Google Scholar] [CrossRef]
- Chapter 3—Boron; de Groot, P.A. (Ed.) Elsevier: Amsterdam, The Netherlands, 2009; pp. 227–228. [Google Scholar] [CrossRef]
- Fu, X.; Ji, Z.; Lin, W.; Yu, Y.; Wu, T. The Advancement of Neutron Shielding Materials for the Storage of Spent Nuclear Fuel. Sci. Technol. Nucl. Install. 2021, 2021, 5541047. [Google Scholar] [CrossRef]
- Bastürk, M.; Arztmann, J.; Jerlich, W.; Kardjilov, N.; Lehmann, E.; Zawisky, M. Analysis of neutron attenuation in boron-alloyed stainless steel with neutron radiography and JEN-3 gauge. J. Nucl. Mater. 2005, 341, 189–200. [Google Scholar] [CrossRef]
- Stone, M.; Crow, L.; Fanelli, V.; Niedziela, J. Characterization of shielding materials used in neutron scattering instrumentation. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2019, 946, 162708. [Google Scholar] [CrossRef]
- He, X.; Ahn, T.; Sippel, T. Corrosion of Borated Stainless Steel in Water and Humid Air. NACE Int. Corros. Conf. Ser. 2012, 1, 761–783. [Google Scholar]
- Zhou, X.; Wang, M.; Chen, C.; Zhao, H. Borides Effect on the Workability and Microstructure Evolution of As-Cast Borated Stainless Steel under Hot Compression. Steel Res. Int. 2016, 87, 1648–1656. [Google Scholar] [CrossRef]
- Won, C.-H.; Jang, J.H.; Kim, S.-D.; Moon, J.; Ha, H.-Y.; Kang, J.-Y.; Lee, C.-H.; Lee, T.-H.; Kang, N. Effect of annealing on mechanical properties and microstructure evolution of borated stainless steels. J. Nucl. Mater. 2019, 515, 206––214. [Google Scholar] [CrossRef]
- Li, Y.-W.; Wang, Z.-J.; Fu, D.-G.; Li, G.; Liu, H.-T.; Zhang, X.-M. Fabrication of high borated austenitic stainless steel thick plates with enhanced ductility and toughness using a hot-roll-bonding method. Mater. Sci. Eng. A 2021, 799, 140212. [Google Scholar] [CrossRef]
- He, J.Y.; Soliman, S.E.; Baratta, A.J.; Balliett, T.A. Fracture Mechanism of Borated Stainless Steel. Nucl. Technol. 2000, 130, 218–225. [Google Scholar] [CrossRef]
- Funke, T.; Henig, C. CASTOR® 1000/19: Development and Design of a New Transport and Storage Cask. In Proceedings of the International Youth Nuclear Congress, Interlaken, Switzerland, 20–26 September 2008; pp. 20–26. [Google Scholar]
- Herfurth, K.; Scharf, S. Casting; Viswanathan, S., Apelian, D., Donahue, R.J., Das Gupta, B., Gywn, M., Jorstad, J.L., Monroe, R.W., Sahoo, M., Prucha, T.E., Twarog, D., Eds.; ASM International: Almere, The Netherlands, 2008. [Google Scholar] [CrossRef]
- Kestens, L.; Jonas, J.J. Metalworking: Bulk Forming; Semiatin, S.L., Ed.; ASM International: Almere, The Netherlands, 2005; Volume 14A. [Google Scholar] [CrossRef]
- Xia, Y.-F.; Luo, G.-C.; Wu, D.-S.; Quan, G.-Z.; Zhou, J. The Evaluation of Varying Ductile Fracture Criteria for 3Cr20Ni10W2 Austenitic Heat-Resistant Alloy. Adv. Mech. Eng. 2013, 5, 520127. [Google Scholar] [CrossRef]
- ASM Handbook Committee; Kerlins, V. Modes of Fracture. In Fractography; ASM International: Almere, The Netherlands, 1987; Volume 2, pp. 12–71. [Google Scholar] [CrossRef]
- Kvačkaj, T.; Tiža, J.; Bacsó, J.; Kováčová, A.; Kočiško, R.; Pernis, R.; Fedorčáková, M.; Purcz, P. Cockcroft-Latham Ductile Fracture Criteria for Non Ferrous Materials. Mater. Sci. Forum 2014, 782, 373–378. [Google Scholar] [CrossRef]
- Poláková, I.; Urbánek, M.; Kubina, T.; Dzugan, J. Prediction of fracture in the shearing process using DEFORM and MARC software. IOP Conf. Ser. Mater. Sci. Eng. 2017, 179, 012058. [Google Scholar] [CrossRef]
- Poláková, I.; Zemko, M.; Rund, M.; Džugan, J. Using DEFORM Software for Determination of Parameters for Two Fracture Criteria on DIN 34CRNIMO6. Metals 2020, 10, 445. [Google Scholar] [CrossRef]
- Oliveira, M.; Alves, J.; Chaparro, B.; Menezes, L. Study on the influence of work-hardening modeling in springback prediction. Int. J. Plast. 2007, 23, 516–543. [Google Scholar] [CrossRef]
- Jordan, P.; Maharaj, C. Asset management strategy for HAZ cracking caused by sigma-phase and creep embrittlement in 304H stainless steel piping. Eng. Fail. Anal. 2020, 110, 104452. [Google Scholar] [CrossRef]
- Kuboň, Z.; Stejskalová, Š.; Kander, L. Effect of Sigma Phase on Fracture Behavior of Steels and Weld Joints of Components in Power Industry Working at Supercritical Conditions. In Austenitic Stainless Steels: New Aspects; IntechOpen: Rijeka, Croatia, 2017; pp. 63–92. [Google Scholar] [CrossRef]
- Wang, M.J.; Bao, E.; Wang, Z.X.; Zhang, S.Y.; Chen, C.; Li, Y.M.; Wei, H.Y. Solidification and Boride Characteristics of Boron-Containing Austenitic Stainless Steel. Mater. Sci. Forum 2011, 704–705, 563–568. [Google Scholar] [CrossRef]
- Naghizadeh, M.; Mirzadeh, H. Effects of Grain Size on Mechanical Properties and Work-Hardening Behavior of AISI 304 Austenitic Stainless Steel. Steel Res. Int. 2019, 90, 1900153. [Google Scholar] [CrossRef]




| C | Mn | Si | Cr | Ni | B |
|---|---|---|---|---|---|
| 0.009 | 1.05 | 0.25 | 19.50 | 12.30 | 1.62 |
| ɛ | Rp0.2 | Rm | Ag | A | Z |
|---|---|---|---|---|---|
| s−1 | MPa | MPa | % | % | % |
| 10 | 393 | 649 | 14.0 | 15.0 | 14 |
| 0.1 | 364 | 603 | 12.2 | 15.3 | 14 |
| Thickness | Fracture Initiation D.O.B. (R10) | D.O.B. Springback (R10) | Fracture Initiation D.O.B. (R15) | D.O.B. Springback (R15) | Fracture Initiation D.O.B. (R20) | D.O.B. Springback (R20) |
|---|---|---|---|---|---|---|
| mm | ° | ° | ° | ° | ° | |
| 5.0 | 58 | N.A. | 60 | 54 | >135 | N.A. |
| 4.5 | 62 | 54 | 80 | 75 | >135 | N.A. |
| 4.0 | 72 | 62 | >90 | N.A. | >135 | N.A. |
| Thickness | D.O.B. without Fracture Initiation (R10) | Max. Value of Effective Strain (R10) | D.O.B. without Fracture Initiation (R15) | Max. Value of Effective Strain (R15) | D.O.B. without Fracture Initiation (R20) | Max. Value of Effective Strain (R20) |
|---|---|---|---|---|---|---|
| mm | ° | mm/mm | ° | mm/mm | ° | mm/mm |
| 5.0 | 57 | 0.186 | 59 | 0.164 | >130 | 0.177 |
| 4.5 | 65 | 0.164 | 76 | 0.174 | >130 | 0.157 |
| 4.0 | 69 | 0.170 | 93 | 0.175 | >130 | 0.136 |
| State | Rp0.2 | Rm | Ag | A | Z |
|---|---|---|---|---|---|
| MPa | MPa | % | % | % | |
| As-rolled | 335 | 605 | 11.9 | 12.0 | 14 |
| Solution-treated | 388 | 658 | 14.2 | 16.3 | 14 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Odehnal, J.; Studecký, T.; Čejková, P.; Poláková, I.; Rund, M.; Brunát, V.; Martínek, P.; Francisko, P.; Donik, Č. Aspects of Bending High-Borated Austenitic Stainless Steel Sheets for Interim Storage of Spent Nuclear Fuel. Metals 2023, 13, 348. https://doi.org/10.3390/met13020348
Odehnal J, Studecký T, Čejková P, Poláková I, Rund M, Brunát V, Martínek P, Francisko P, Donik Č. Aspects of Bending High-Borated Austenitic Stainless Steel Sheets for Interim Storage of Spent Nuclear Fuel. Metals. 2023; 13(2):348. https://doi.org/10.3390/met13020348
Chicago/Turabian StyleOdehnal, Josef, Tomáš Studecký, Petra Čejková, Ivana Poláková, Martin Rund, Václav Brunát, Petr Martínek, Pavel Francisko, and Črtomir Donik. 2023. "Aspects of Bending High-Borated Austenitic Stainless Steel Sheets for Interim Storage of Spent Nuclear Fuel" Metals 13, no. 2: 348. https://doi.org/10.3390/met13020348
APA StyleOdehnal, J., Studecký, T., Čejková, P., Poláková, I., Rund, M., Brunát, V., Martínek, P., Francisko, P., & Donik, Č. (2023). Aspects of Bending High-Borated Austenitic Stainless Steel Sheets for Interim Storage of Spent Nuclear Fuel. Metals, 13(2), 348. https://doi.org/10.3390/met13020348







