Solidification of Undercooled Liquid under Supergravity Field by Phase-Field Crystal Approach
Abstract
:1. Introduction
2. Simulation Method
2.1. Phase-Field Crystal Model
2.2. Numerical Implementation
2.3. Model Parameter Settings
3. Result and Discussion
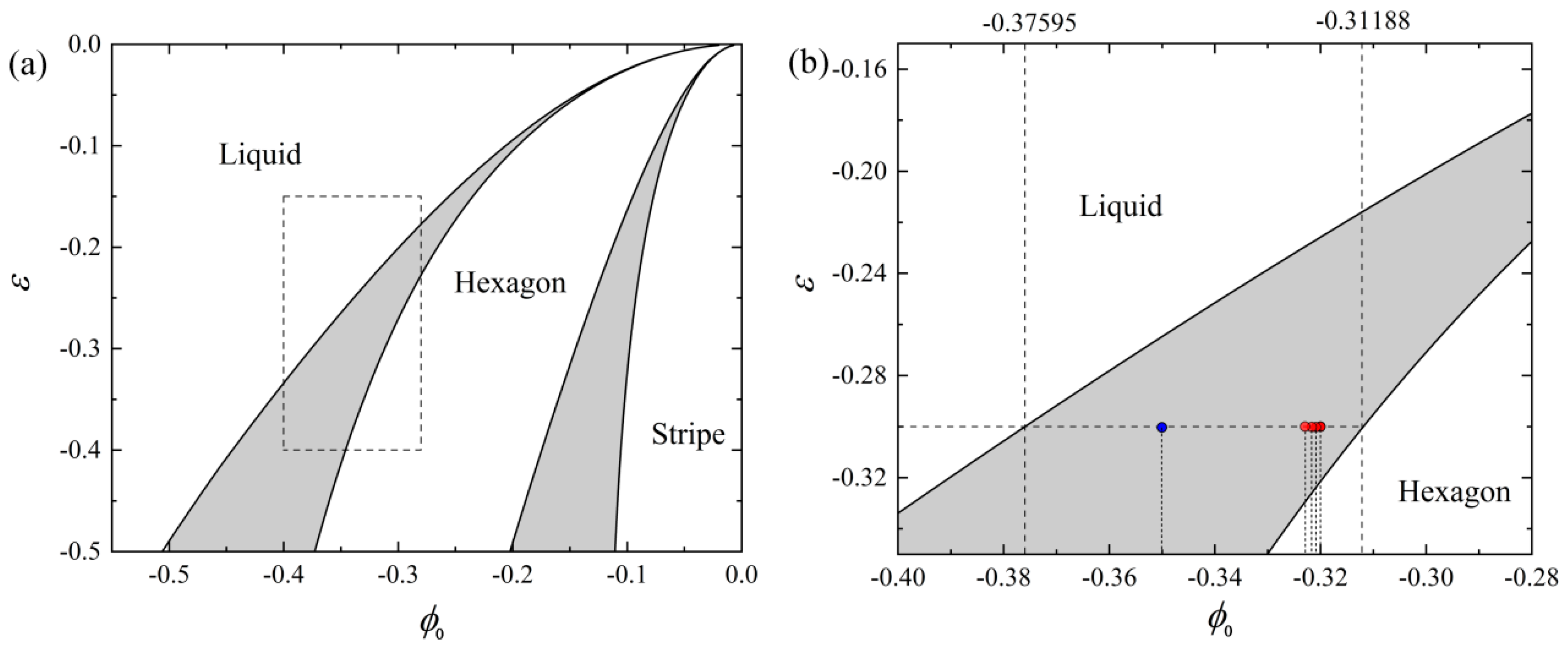
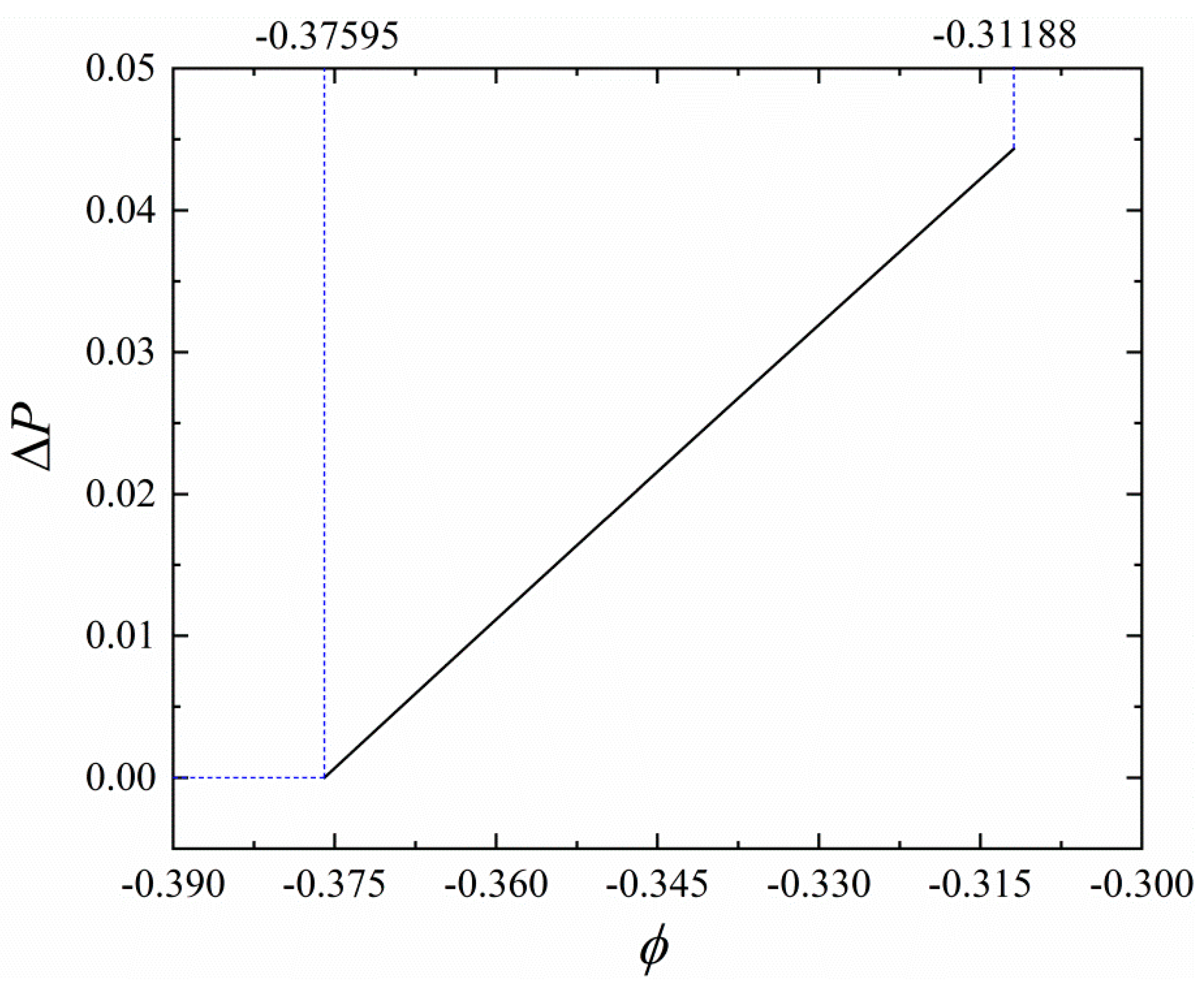
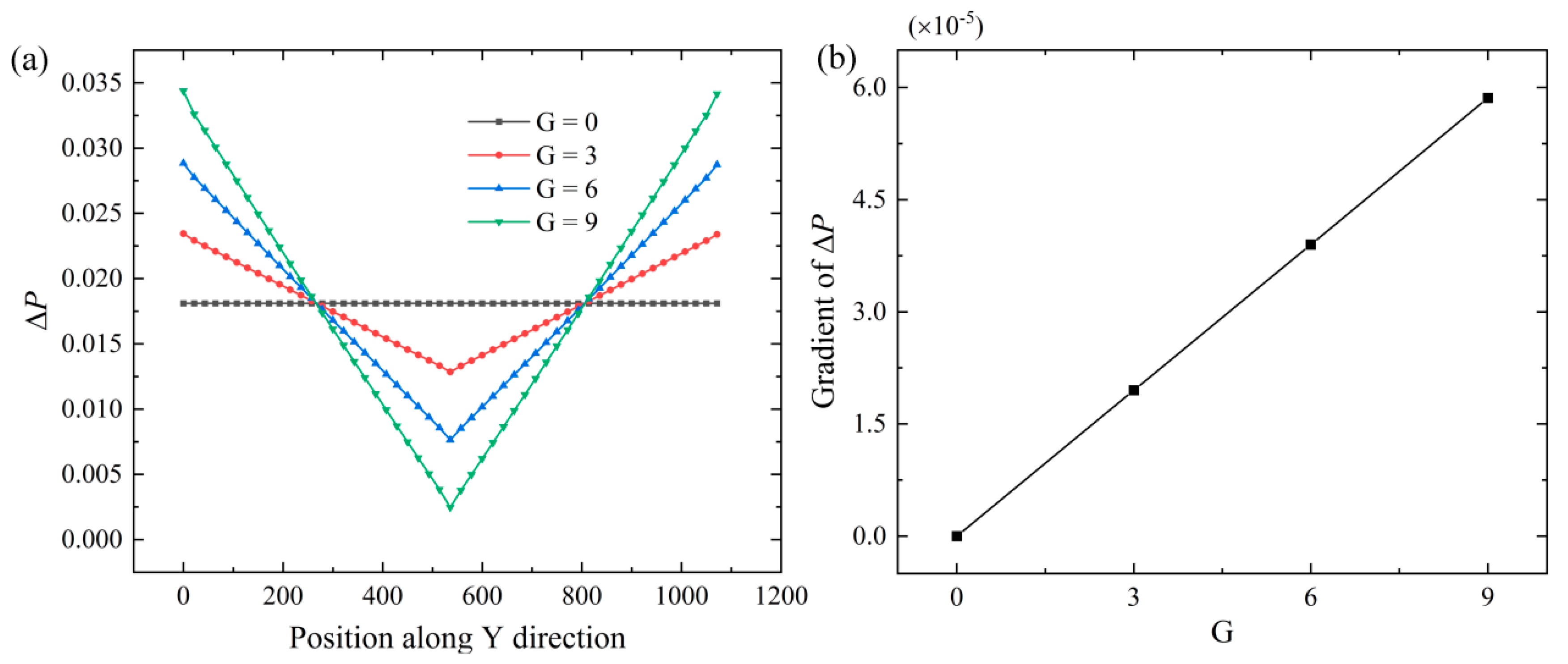
3.1. The Equilibrium Properties of the Undercooled Liquid in the Supergravity Field
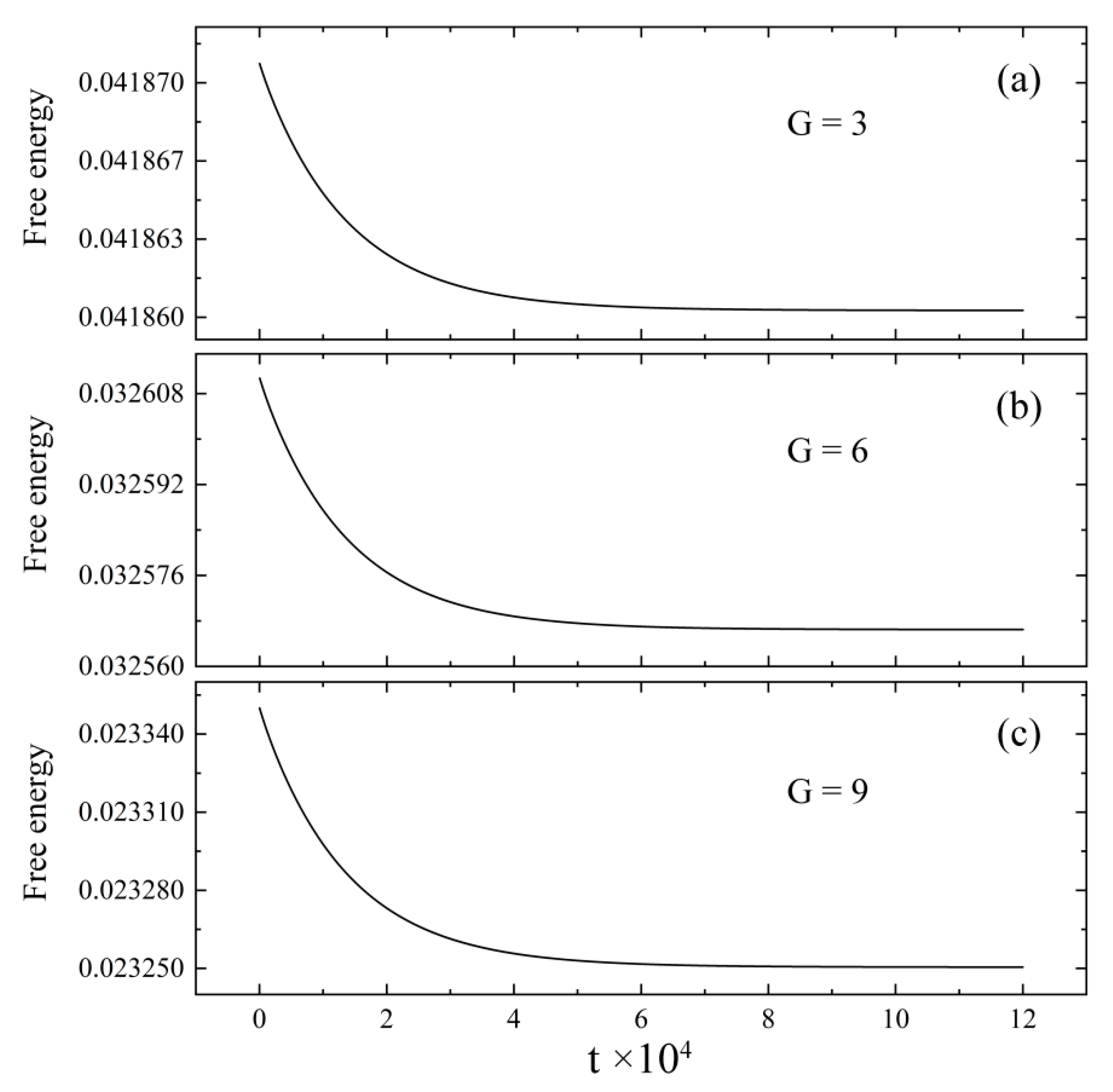
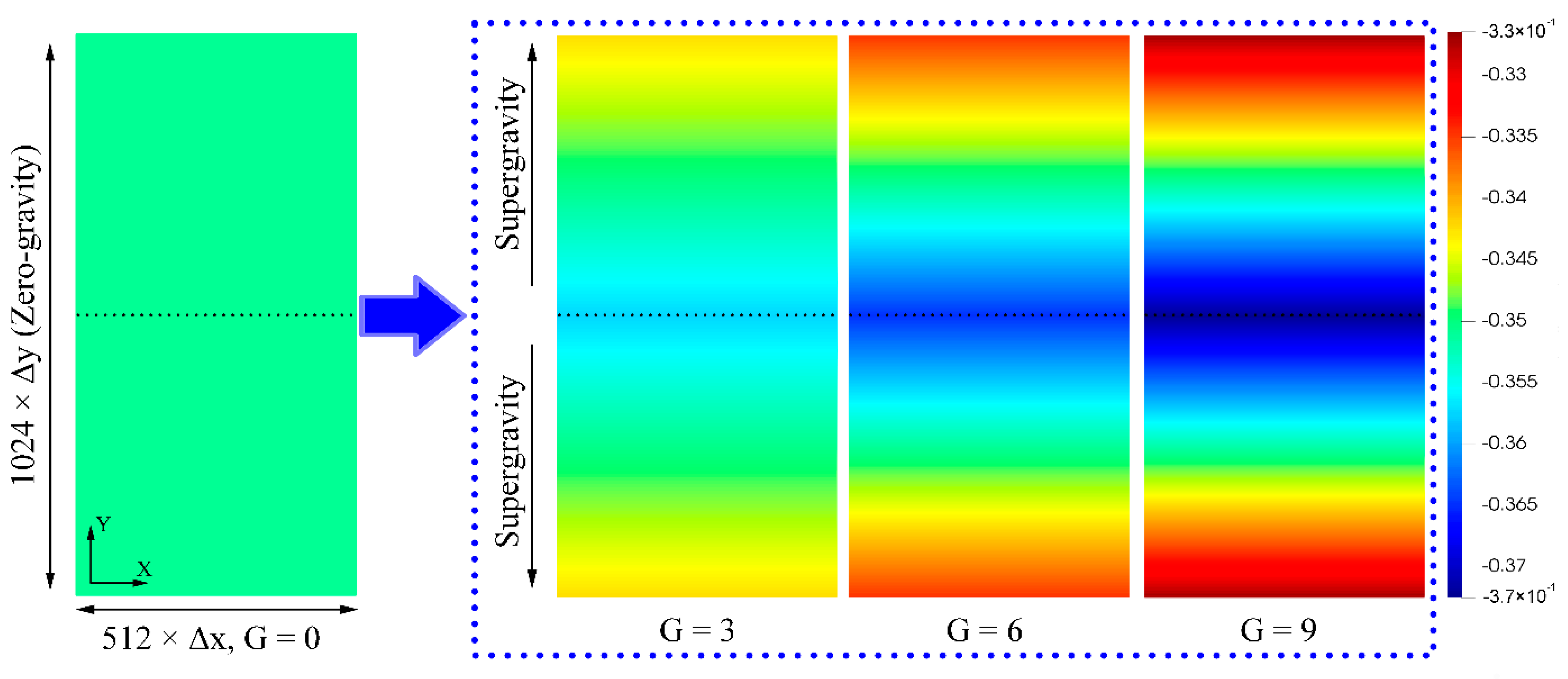
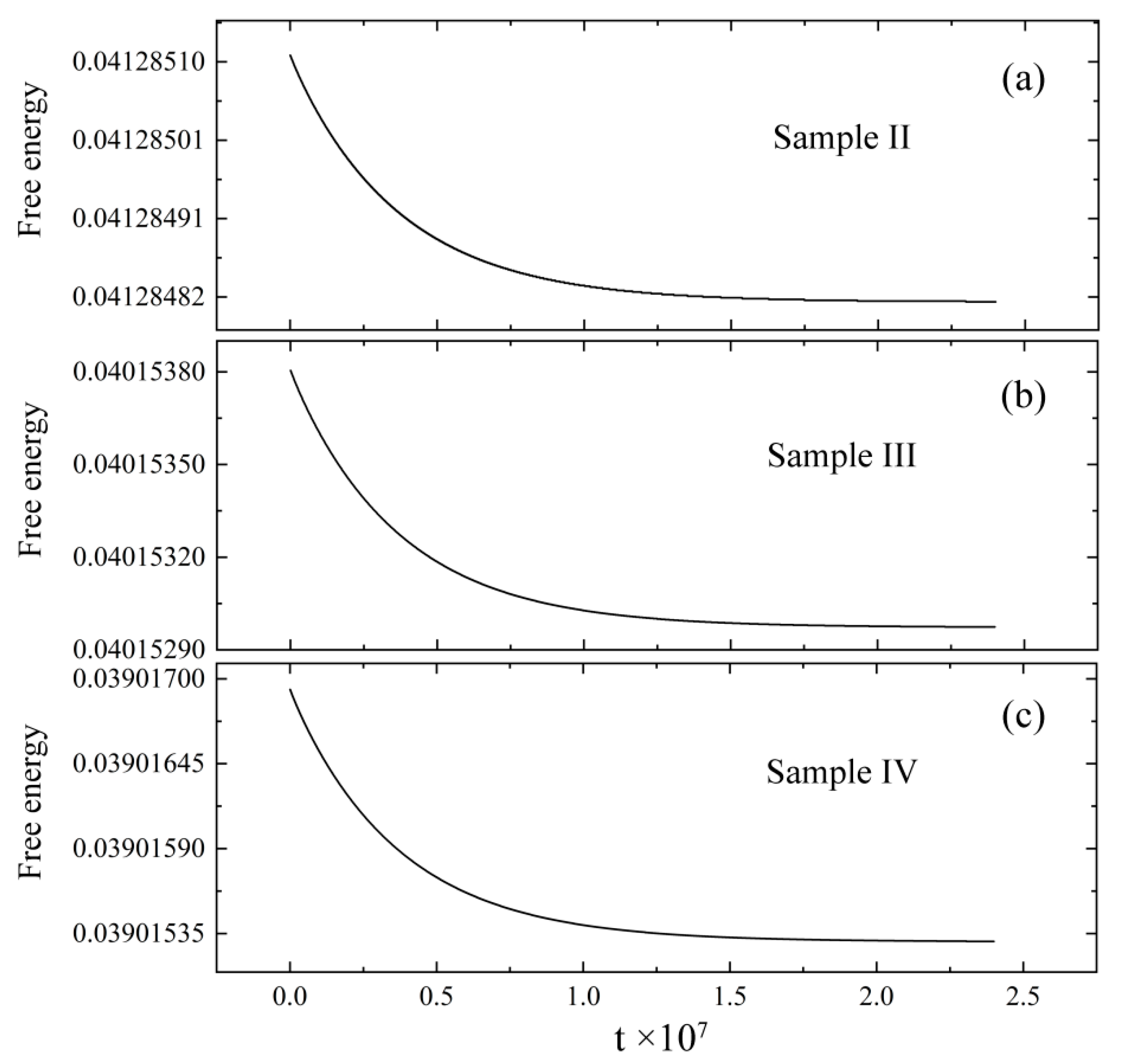
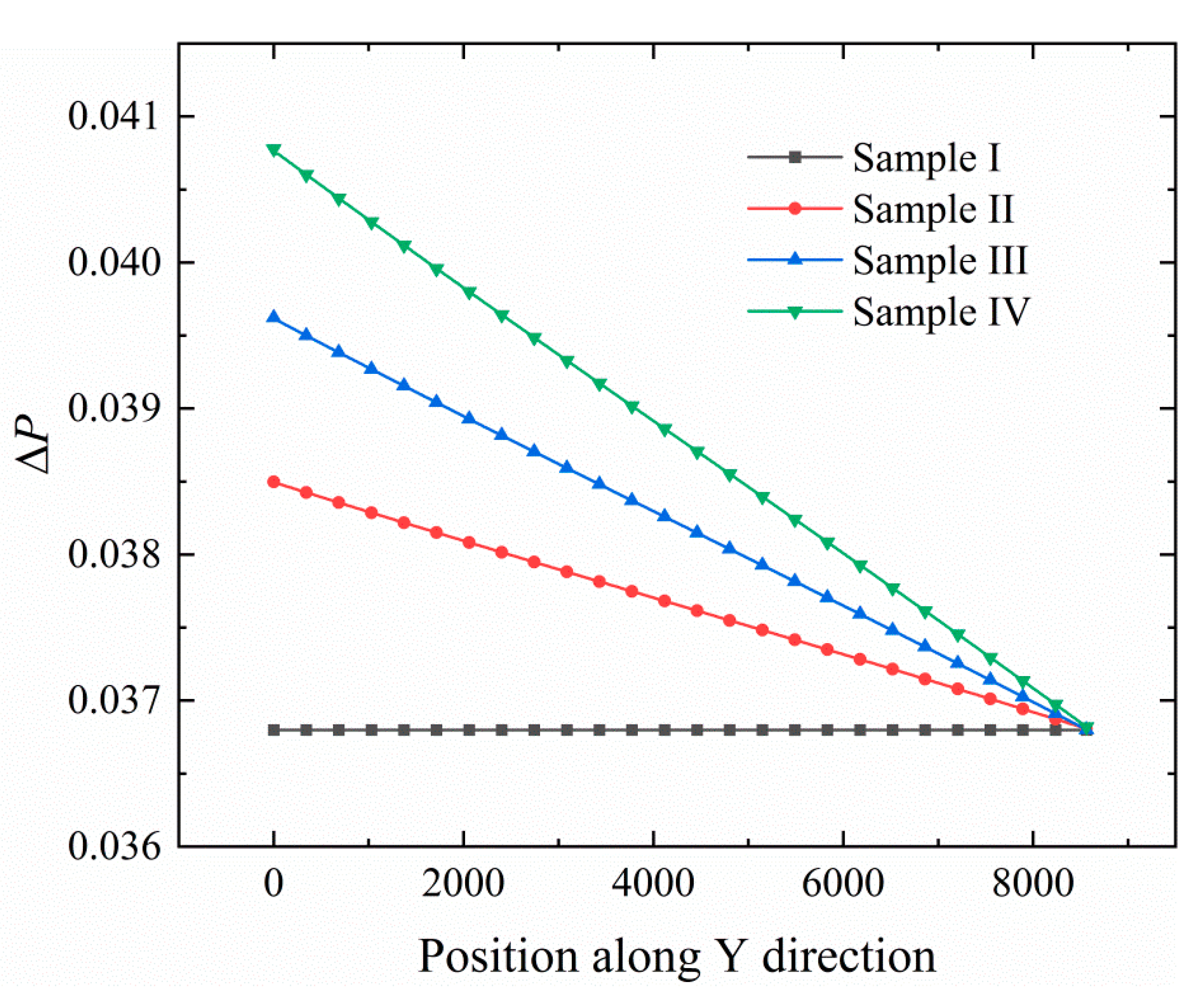
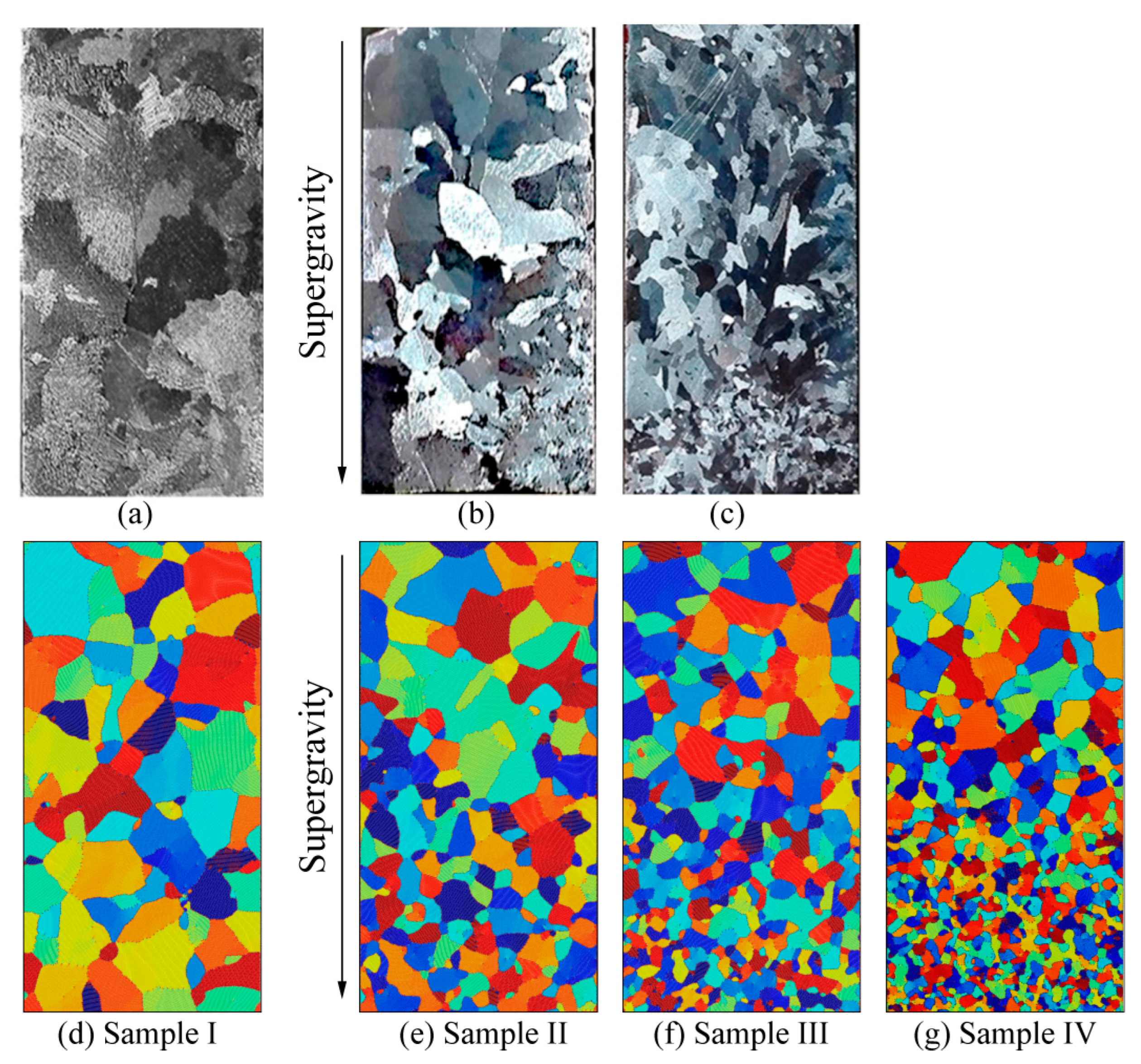
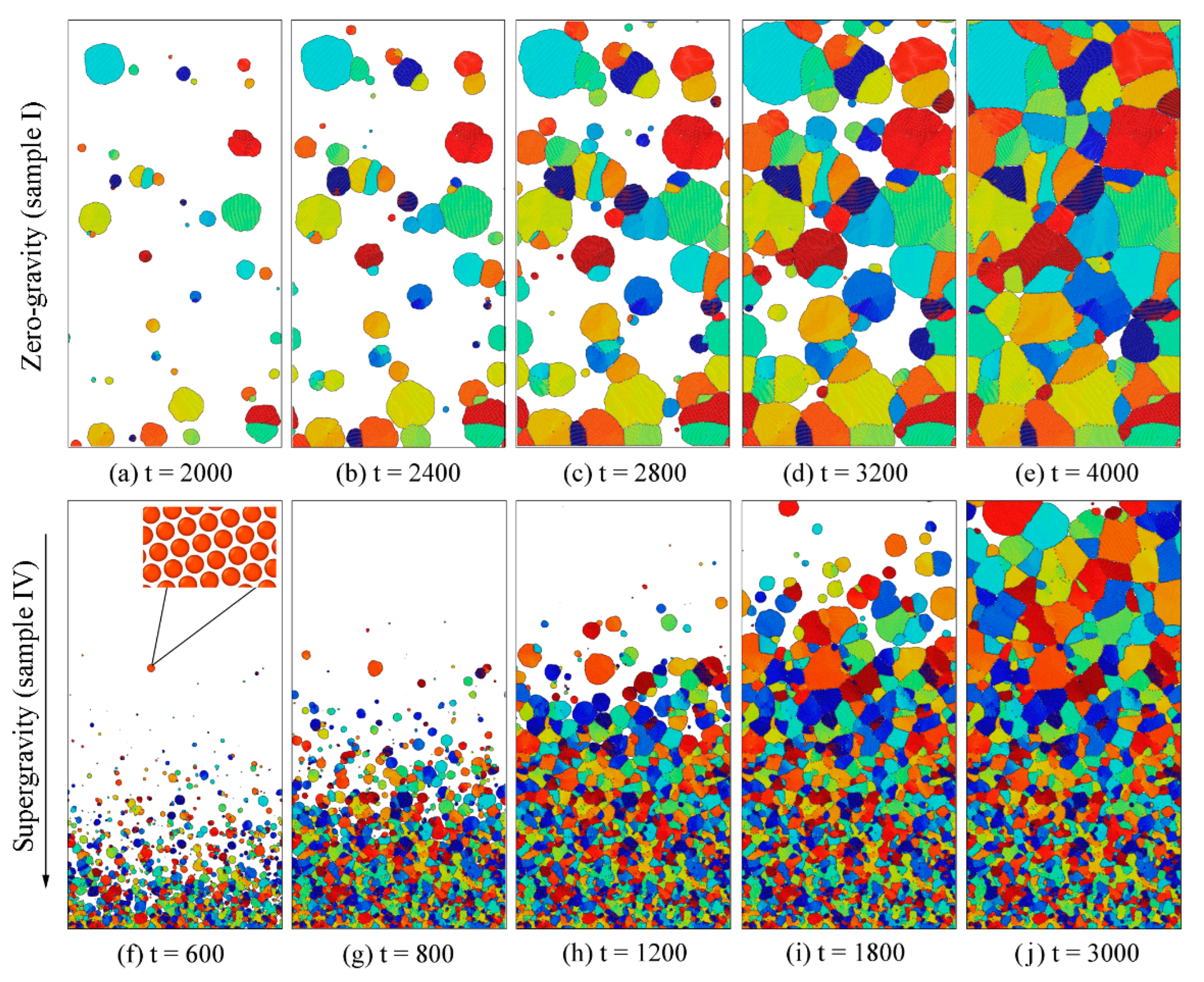
3.2. Sample Preparation for Solidification in a Supergravity Field
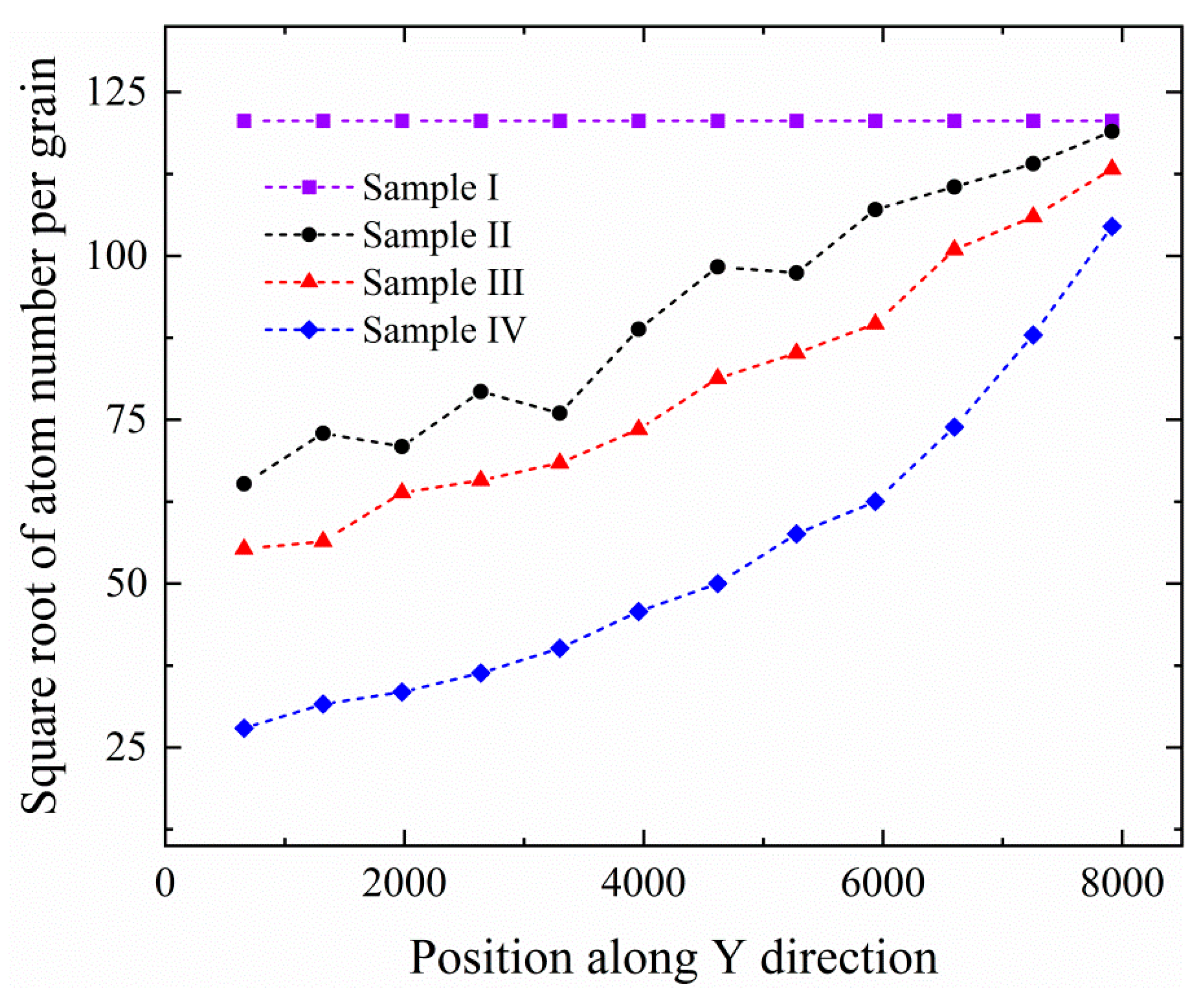
3.3. Effects of the Supergravity Field on the Solidification Microstructures
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Favez, D.; Wagnière, J.D.; Rappaz, M. Au–Fe alloy solidification and solid-state transformations. Acta Mater. 2010, 58, 1016–1025. [Google Scholar] [CrossRef] [Green Version]
- Frazier, W.E. Metal Additive Manufacturing: A Review. J. Mater. Eng. Perform. 2014, 23, 1917–1928. [Google Scholar] [CrossRef]
- Vajd, A.; Samadi, A. Optimization of Centrifugal Casting Parameters to Produce the Functionally Graded Al–15wt%Mg2Si Composites with Higher Tensile Properties. Int. J. Met. 2019, 14, 937–948. [Google Scholar] [CrossRef]
- Li, J.; Ma, J.; Gao, Y.; Zhai, Q. Research on solidification structure refinement of pure aluminum by electric current pulse with parallel electrodes. Mater. Sci. Eng. A 2008, 490, 452–456. [Google Scholar] [CrossRef]
- Li, X.; Gagnoud, A.; Ren, Z.; Fautrelle, Y.; Moreau, R. Investigation of thermoelectric magnetic convection and its effect on solidification structure during directional solidification under a low axial magnetic field. Acta Mater. 2009, 57, 2180–2197. [Google Scholar] [CrossRef]
- Saleh, B.; Jiang, J.; Ma, A.; Song, D.; Yang, D. Effect of Main Parameters on the Mechanical and Wear Behaviour of Functionally Graded Materials by Centrifugal Casting: A Review. Met. Mater. Int. 2019, 25, 1395–1409. [Google Scholar] [CrossRef]
- Li, S.-Y.; Qin, S.-Y.; Xi, X.-J.; Sun, G.-Y.; Yang, W.-S.; Guo, J.; Guo, H.-J. Solidified Structure Refinement of H13 Tool Steel under a Multi-Rotational Speed Super Gravity Field. Metals 2020, 10, 1428. [Google Scholar] [CrossRef]
- Gan, Z.; Wu, H.; Sun, Y.; Su, Y.; Wang, Y.; Wu, C.; Liu, J. Influence of Co contents and super-gravity field on refinement of in-situ ultra-fined fibers in Al-2.5Ni eutectic alloys. J. Alloys Compd. 2020, 822, 153607. [Google Scholar] [CrossRef]
- Nelson, T.; Cai, B.; Warnken, N.; Lee, P.D.; Boller, E.; Magdysyuk, O.V.; Green, N.R. Gravity effect on thermal-solutal convection during solidification revealed by four-dimensional synchrotron imaging with compositional mapping. Scripta Mater. 2020, 180, 29–33. [Google Scholar] [CrossRef]
- Li, R.; Wang, Z.; Guo, Z.; Liaw, P.K.; Zhang, T.; Li, L.; Zhang, Y. Graded microstructures of Al-Li-Mg-Zn-Cu entropic alloys under supergravity. Sci. China Mater. 2018, 62, 736–744. [Google Scholar] [CrossRef] [Green Version]
- Song, G.; Song, B.; Guo, Z.; Yang, Y.; Song, M. Separation of Non-metallic Inclusions from a Fe-Al-O Melt Using a Super-Gravity Field. Metall. Mater. Trans. B 2017, 49, 34–44. [Google Scholar] [CrossRef]
- Gan, Z.; Wu, H.; Sun, Y.; Jiang, P.; Su, Y.; Wu, C.; Liu, J. Super-gravity field assisted homogeneous distribution of sub-micron eutectic Si in Al–Si alloy. J. Alloys Compd. 2020, 817, 152701. [Google Scholar] [CrossRef]
- Yang, Y.; Song, B.; Yang, Z.; Song, G.; Cai, Z.; Guo, Z. The Refining Mechanism of Super Gravity on the Solidification Structure of Al-Cu Alloys. Materials 2016, 9, 1001. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wen, X.; Bao, Q.; Guo, L.; Guo, Z. The introduction of super-gravity into optimization separation of bismuth and zinc from crude bismuth melt. Chem. Eng. Process. 2021, 160, 108266. [Google Scholar] [CrossRef]
- Elder, K.R.; Provatas, N.; Berry, J.; Stefanovic, P.; Grant, M. Phase-field crystal modeling and classical density functional theory of freezing. Phys. Rev. B 2007, 75, 064107. [Google Scholar] [CrossRef] [Green Version]
- Elder, K.R.; Katakowski, M.; Haataja, M.; Grant, M. Modeling elasticity in crystal growth. Phys. Rev. Lett. 2002, 88, 245701. [Google Scholar] [CrossRef] [Green Version]
- Toth, G.I.; Tegze, G.; Pusztai, T.; Toth, G.; Granasy, L. Polymorphism, crystal nucleation and growth in the phase-field crystal model in 2D and 3D. J. Phys. Condens. Matter 2010, 22, 364101. [Google Scholar] [CrossRef]
- Chen, Z.; Wang, Z.; Gu, X.; Chen, Y.; Hao, L.; de Wit, J.; Jin, K. Phase-field crystal simulation facet and branch crystal growth. Appl. Phys. A 2018, 124, 1–8. [Google Scholar] [CrossRef]
- Muhammad, S.; Li, Y.; Yan, Z.; Maqbool, S.; Shi, S.; Muhammad, I. Phase-field crystal modeling of crystal growth patterns with competition of undercooling and atomic density. Phys. Chem. Chem. Phys. 2020, 22, 21858–21871. [Google Scholar] [CrossRef]
- Tang, S.; Backofen, R.; Wang, J.; Zhou, Y.; Voigt, A.; Yu, Y.-M. Three-dimensional phase-field crystal modeling of fcc and bcc dendritic crystal growth. J. Cryst. Growth 2011, 334, 146–152. [Google Scholar] [CrossRef]
- Elder, K.R.; Huang, Z.F.; Provatas, N. Amplitude expansion of the binary phase-field-crystal model. Phys. Rev. E Stat. Nonlin. Soft Matter Phys. 2010, 81, 011602. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gránásy, L.; Tegze, G.; Tóth, G.I.; Pusztai, T. Phase-field crystal modelling of crystal nucleation, heteroepitaxy and patterning. Philos. Mag. 2011, 91, 123–149. [Google Scholar] [CrossRef] [Green Version]
- Toth, G.I.; Pusztai, T.; Tegze, G.; Toth, G.; Granasy, L. Amorphous nucleation precursor in highly nonequilibrium fluids. Phys. Rev. Lett. 2011, 107, 175702. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hubert, J.; Cheng, M.; Emmerich, H. Effect of noise-induced nucleation on grain size distribution studied via the phase-field crystal method. J. Phys. Condens. Matter 2009, 21, 464108. [Google Scholar] [CrossRef]
- Muralidharan, S.; Khodadad, R.; Sullivan, E.; Haataja, M. Multilayer thin film growth on crystalline and quasicrystalline surfaces: A phase-field crystal study. Phys. Rev. B 2012, 85, 245428. [Google Scholar] [CrossRef] [Green Version]
- Wang, K.; Zhang, F.; He, A.; Wang, P. Plasticity and phase transition of crystals under continuous deformations by phase field crystal approach. Int. J. Plast. 2019, 122, 225–243. [Google Scholar] [CrossRef]
- Emmerich, H.; Löwen, H.; Wittkowski, R.; Gruhn, T.; Tóth, G.I.; Tegze, G.; Gránásy, L. Phase-field-crystal models for condensed matter dynamics on atomic length and diffusive time scales: An overview. Adv. Phys. 2012, 61, 665–743. [Google Scholar] [CrossRef] [Green Version]
- Hirouchi, T.; Takaki, T.; Tomita, Y. Development of numerical scheme for phase field crystal deformation simulation. Comput. Mater. Sci. 2009, 44, 1192–1197. [Google Scholar] [CrossRef]
- Li, J.; Ni, B.; Zhang, T.; Gao, H. Phase field crystal modeling of grain boundary structures and growth in polycrystalline graphene. J. Mech. Phys. Solids 2018, 120, 36–48. [Google Scholar] [CrossRef]
- Gao, Y.; Huang, L.; Deng, Q.; Zhou, W.; Luo, Z.; Lin, K. Phase field crystal simulation of dislocation configuration evolution in dynamic recovery in two dimensions. Acta Mater. 2016, 117, 238–251. [Google Scholar] [CrossRef]
- Chan, V.W.L.; Pisutha-Arnond, N.; Thornton, K. Thermodynamic relationships for homogeneous crystalline and liquid phases in the phase-field crystal model. Comput. Mater. Sci. 2017, 135, 205–213. [Google Scholar] [CrossRef]
- Jreidini, P.; Kocher, G.; Provatas, N. Classical nucleation theory in the phase-field crystal model. Phys. Rev. E 2018, 97, 042802. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zimmermann, G.; Hamacher, M.; Sturz, L. Effect of zero, normal and hyper-gravity on columnar dendritic solidification and the columnar-to-equiaxed transition in Neopentylglycol-(D)Camphor alloy. J. Cryst. Growth 2019, 512, 47–60. [Google Scholar] [CrossRef]
- Tegze, G.; Granasy, L.; Toth, G.I.; Podmaniczky, F.; Jaatinen, A.; Ala-Nissila, T.; Pusztai, T. Diffusion-controlled anisotropic growth of stable and metastable crystal polymorphs in the phase-field crystal model. Phys. Rev. Lett. 2009, 103, 035702. [Google Scholar] [CrossRef] [PubMed] [Green Version]
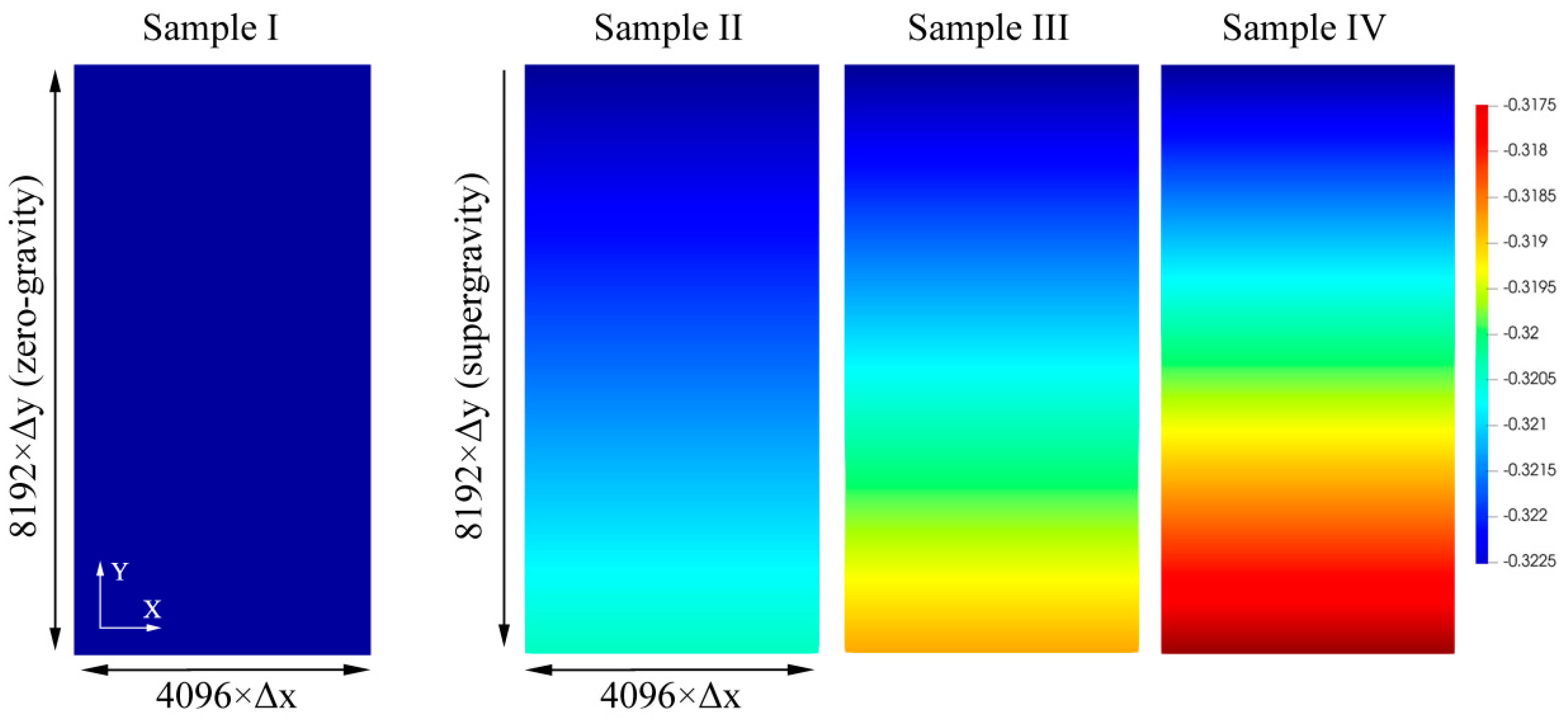


| Sample | ε | G | n | |
|---|---|---|---|---|
| I | −0.3 | −0.3229 | 0 | −7 |
| II | −0.3 | −0.32166 | 3 | −7 |
| III | −0.3 | −0.32085 | 5 | −7 |
| IV | −0.3 | −0.32 | 7 | −7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, N.; Huang, Y.; Wang, K.; Hu, W.; Chen, J.; Deng, H. Solidification of Undercooled Liquid under Supergravity Field by Phase-Field Crystal Approach. Metals 2022, 12, 232. https://doi.org/10.3390/met12020232
Hu N, Huang Y, Wang K, Hu W, Chen J, Deng H. Solidification of Undercooled Liquid under Supergravity Field by Phase-Field Crystal Approach. Metals. 2022; 12(2):232. https://doi.org/10.3390/met12020232
Chicago/Turabian StyleHu, Nengwen, Yongfeng Huang, Kun Wang, Wangyu Hu, Jun Chen, and Huiqiu Deng. 2022. "Solidification of Undercooled Liquid under Supergravity Field by Phase-Field Crystal Approach" Metals 12, no. 2: 232. https://doi.org/10.3390/met12020232
APA StyleHu, N., Huang, Y., Wang, K., Hu, W., Chen, J., & Deng, H. (2022). Solidification of Undercooled Liquid under Supergravity Field by Phase-Field Crystal Approach. Metals, 12(2), 232. https://doi.org/10.3390/met12020232








