Dislocation Dynamics Model to Simulate Motion of Dislocation Loops in Metallic Materials
Abstract
1. Introduction
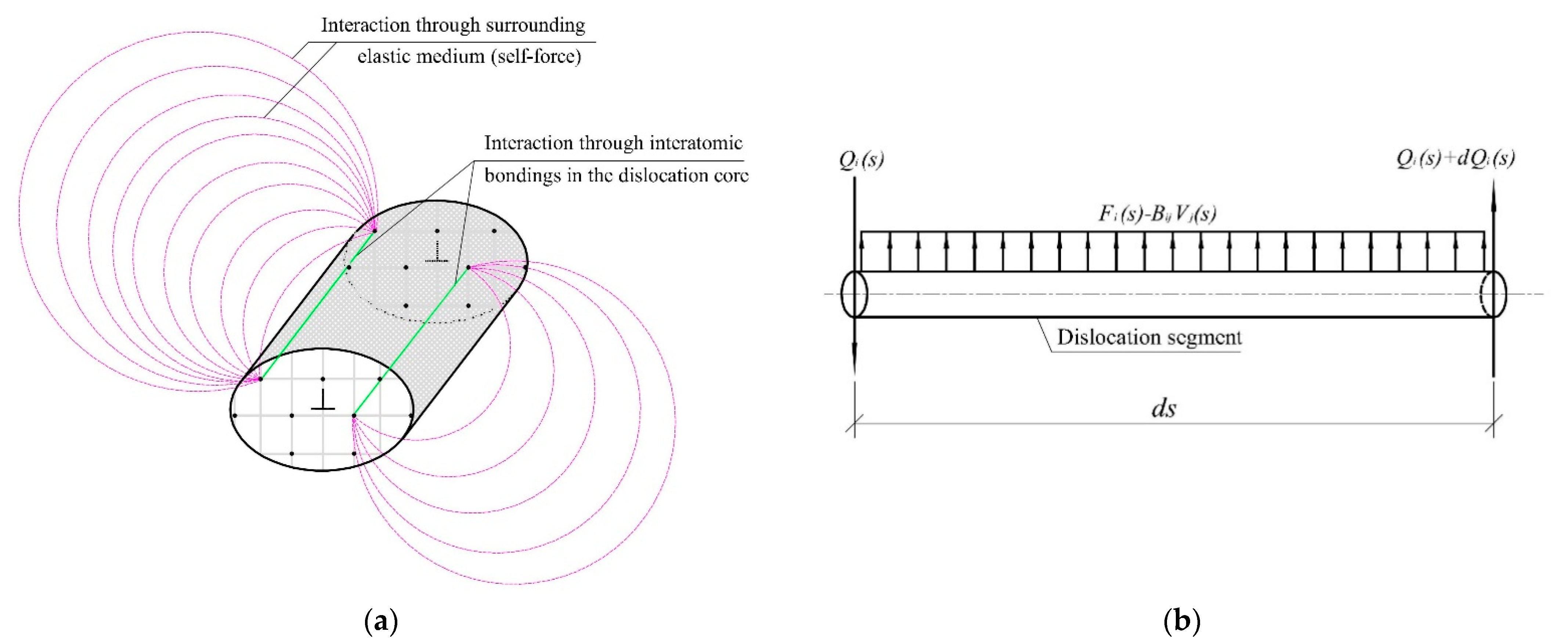
2. Methodology
3. Numerical Implementation
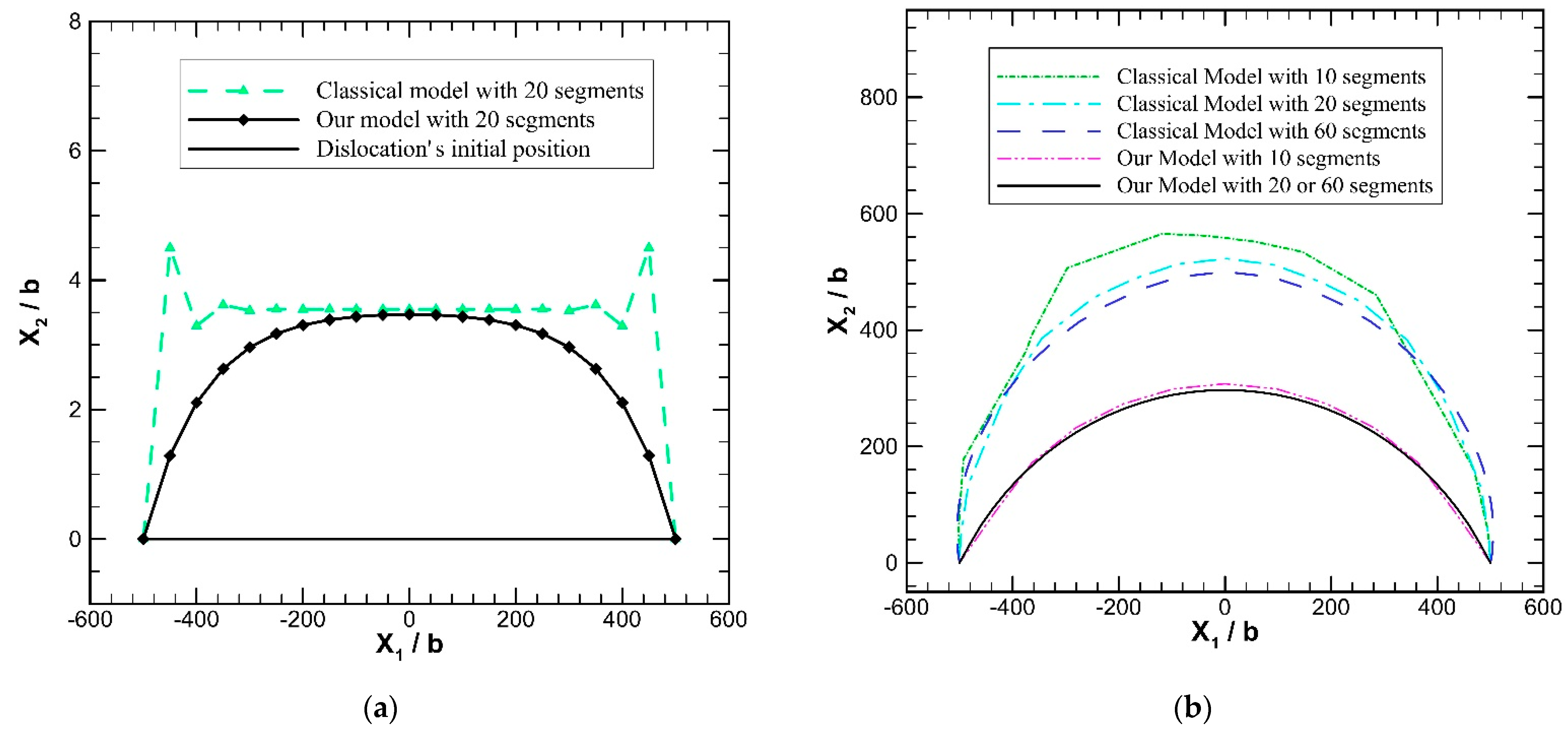
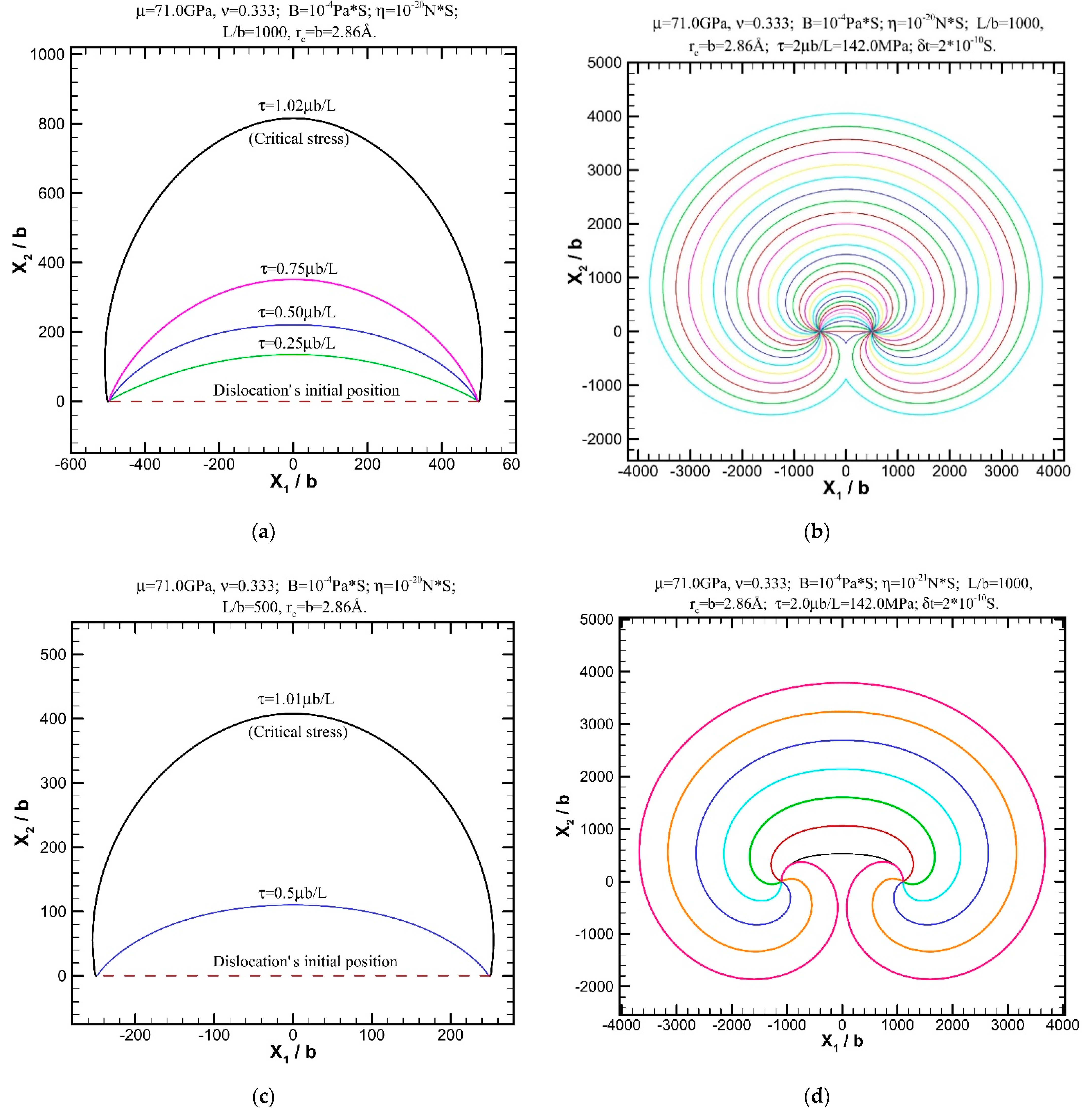
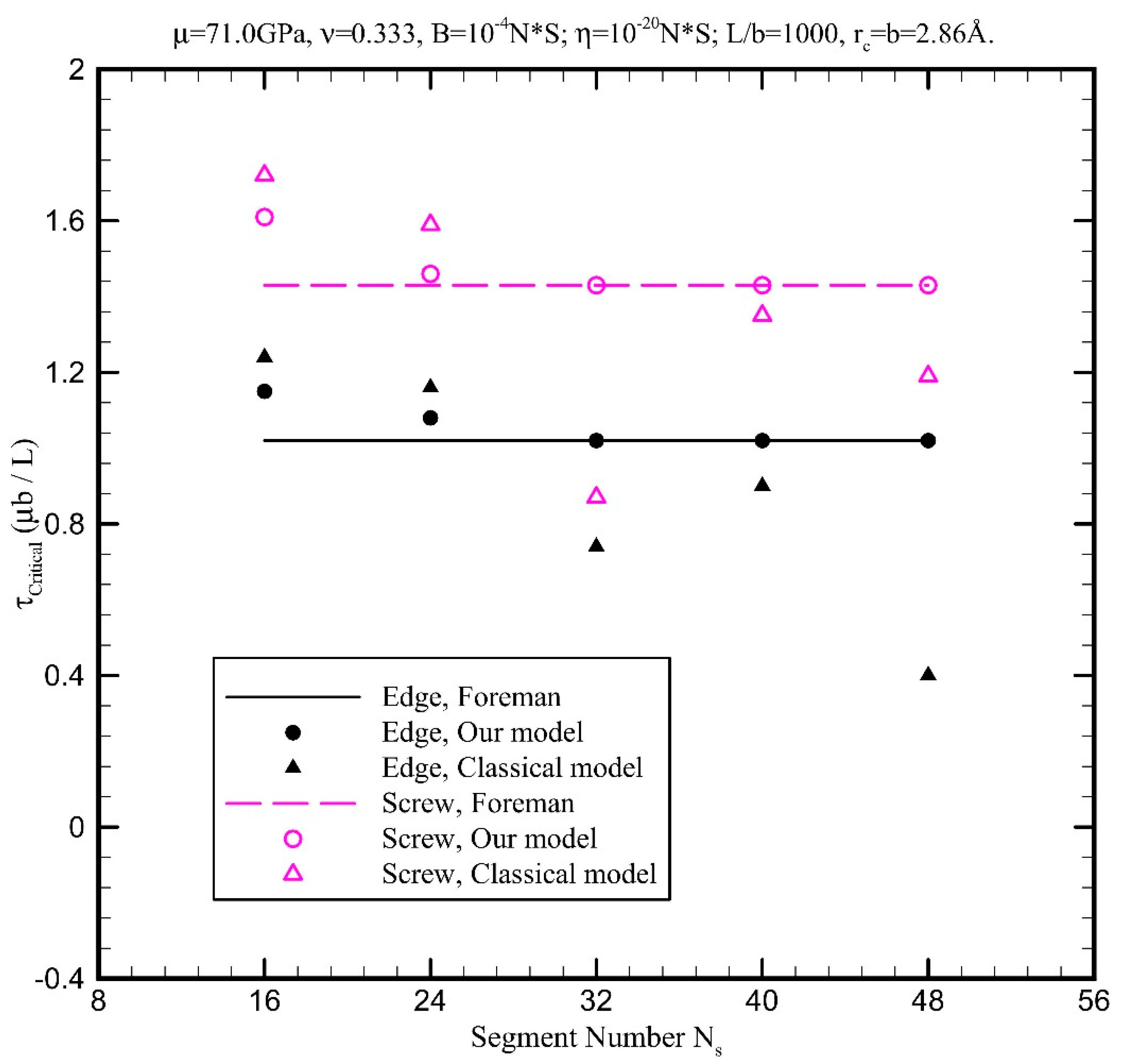
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lepinoux, J.; Kubin, L.P. The dynamic organization of dislocation structures: A simulation. Scr. Metall. 1987, 21, 833–838. [Google Scholar] [CrossRef]
- Amodeo, R.J.; Ghoniem, N.M. Dislocation dynamics I, a proposed methodology for deformation micromechanics. Phys. Rev. B 1990, 41, 6958–6967. [Google Scholar] [CrossRef] [PubMed]
- Amodeo, R.J.; Ghoniem, N.M. Dislocation dynamics I, applications to the formation of persistent slip bands, planar arrays, and dislocation cells. Phys. Rev. B 1990, 41, 6968–6976. [Google Scholar] [CrossRef] [PubMed]
- Van der Giessen, E.; Needleman, A. Discrete dislocation plasticity: A simple planar model. Model. Simul. Mater. Sci. Eng. 1995, 3, 689–735. [Google Scholar] [CrossRef]
- Kubin, L.P.; Canova, G.; Condat, M.; Devincre, B.; Pontikis, V.; Brechet, Y. Dislocation microstructures and plastic flow: A 3D simulation. Solid State Phenom. 1992, 23–24, 455–472. [Google Scholar] [CrossRef]
- Schwarz, K.W.; Tersoff, J. Interaction of threading and misfit dislocations in a strained epitaxial layer. Appl. Phys. Lett. 1996, 69, 1220–1222. [Google Scholar] [CrossRef]
- Schwarz, K.W. Interaction of dislocations on crossed glide planes in a strained epitaxial layer. Phys. Rev. Lett. 1997, 78, 4785–4788. [Google Scholar] [CrossRef]
- Tang, M.; Kubin, L.P.; Canova, G.R. Dislocation mobility and the mechanical response of b.c.c. single crystals: A mesoscopic approach. Acta Mater. 1998, 46, 3221–3235. [Google Scholar] [CrossRef]
- Zbib, H.M.; Rhee, M.; Hirth, J.P. On the plastic deformation and the dynamics of 3D dislocations. Inter. J. Mech. Sci. 1998, 40, 113–127. [Google Scholar] [CrossRef]
- Ghoniem, N.M.; Sun, L.Z. Fast-sum method for the elastic field of three-dimensional dislocation ensembles. Phys. Rev. B 1999, 60, 128–140. [Google Scholar] [CrossRef]
- Ghoniem, N.M.; Tong, S.-H.; Sun, L.Z. Parametric dislocation dynamics: A thermaldynamics-based approach to investigations of mesoscopic plastic deformation. Phys. Rev. B 2000, 61, 913–927. [Google Scholar] [CrossRef]
- Diaz de la Rubia, T.; Zbib, H.M.; Khraishi, T.A.; Wirth, B.D.; Victoria, V.; Caturla, M.J. Multiscale modeling of plastic flow localization in irradiated materials. Nature 2000, 406, 871–874. [Google Scholar] [CrossRef] [PubMed]
- Cai, W.; Bulatov, V.V. Mobility laws in dislocation dynamics simulations. Mater. Sci. Eng. A 2004, 387–389, 277–281. [Google Scholar] [CrossRef]
- Cui, Y.; Ghoniem, N.M. Influence of Size on the Fractal Dimension of Dislocation Microstructure. Metals 2019, 9, 478. [Google Scholar] [CrossRef]
- Gao, S.; Yang, Z.; Grabowski, M.; Rogal, J.; Drautz, R.; Hartmaier, A. Influence of Excess Volumes Induced by Re and W on Dislocation Motion and Creep in Ni-Base Single Crystal Superalloys: A 3D Discrete Dislocation Dynamics Study. Metals 2019, 9, 637. [Google Scholar] [CrossRef]
- Fan, H.; Wang, Q.; El-Awady, J.A.; Raabe, D.; Zaiser, M. Strain rate dependency of dislocation plasticity. Nat. Commun. 2021, 12, 1845. [Google Scholar] [CrossRef]
- Muraishi, S. Internal Stress and Dislocation Interaction of Plate-Shaped Misfitting Precipitates in Aluminum Alloys. Materials 2021, 14, 5811. [Google Scholar] [CrossRef]
- Zheng, H.; Liu, J.; Muraishi, S. Dislocation Topological Evolution and Energy Analysis in Misfit Hardening of Spherical Precipitate by the Parametric Dislocation Dynamics Simulation. Materials 2021, 14, 6368. [Google Scholar] [CrossRef]
- Pachaury, Y.; Po, G.; El-Azab, A. Discrete Dislocation Dynamics for Crystal RVEs Part I: Periodic Network Kinematics. J. Mech. Phys. Solids 2022, 163, 104861. [Google Scholar] [CrossRef]
- Schneider, Y.; Rapp, D.-M.; Yang, Y.; Wasserbach, W.; Schmauder, S. Many-scale Investigations of Deformation Behavior of Polycrystalline Composites: II Micro-Macro Simultaneous FE and Discrete Dislocation Dynamics Simulation. Materials 2022, 15, 2852. [Google Scholar] [CrossRef]
- Gómez-García, D.; Devincre, B.; Kubin, L.P. Dislocation dynamics in confined geometry. J. Comput.-Aided Mater. Des. 1999, 6, 157–164. [Google Scholar] [CrossRef]
- Bulatov, V.V.; Cai, W. Computer Simulations of Dislocations; Oxford University Press: Oxford, UK, 2006. [Google Scholar]
- Yin, H.M.; Sun, L.Z. Magnetoelasticity of chain-structured ferromagnetic composites. Appl. Phys. Lett. 2005, 86, 261901. [Google Scholar] [CrossRef]
- Liu, H.T.; Sun, L.Z.; Ju, J.W. Elastoplastic modeling of progressive interfacial debonding for particle-reinforced metal-matrix composites. Acta Mech. 2006, 181, 1–17. [Google Scholar] [CrossRef]
- Peach, M.; Koehler, J.S. The forces exerted on dislocations and the stress fields produced by them. Phys. Rev. 1950, 80, 436–439. [Google Scholar] [CrossRef]
- Brown, L.M. The self-stress of dislocations and the shape of extended nodes. Phil. Mag. 1964, 10, 441–466. [Google Scholar] [CrossRef]
- Gavazza, S.D.; Barnett, D.M. The self-force on a planar dislocation loop in an anisotropic linear-elastic medium. J. Mech. Phys. Solids 1976, 24, 171–185. [Google Scholar] [CrossRef]
- Finnis, M.W.; Sinclair, J.E. A simple empirical N-body potential for transition metals. Phil. Mag. A 1984, 50, 45–55. [Google Scholar] [CrossRef]
- Telling, R.H.; Ewels, C.P.; El-Barbary, A.A.; Heggie, M.I. Wigner defects bridge the graphite gap. Nat. Mater. 2003, 2, 333–337. [Google Scholar] [CrossRef]
- Foreman, A.J.E. The bowing of a dislocation segment. Phil. Mag. 1967, 15, 1011–1021. [Google Scholar] [CrossRef]
- Devincre, B.; Condat, M. Model validation of a 3D simulation of dislocation dynamics: Discretization and line tension effects. Acta Metall. Mater. 1992, 40, 2629–2637. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tan, X.; Tan, E.; Sun, L. Dislocation Dynamics Model to Simulate Motion of Dislocation Loops in Metallic Materials. Metals 2022, 12, 1804. https://doi.org/10.3390/met12111804
Tan X, Tan E, Sun L. Dislocation Dynamics Model to Simulate Motion of Dislocation Loops in Metallic Materials. Metals. 2022; 12(11):1804. https://doi.org/10.3390/met12111804
Chicago/Turabian StyleTan, Xinze, Enhui Tan, and Lizhi Sun. 2022. "Dislocation Dynamics Model to Simulate Motion of Dislocation Loops in Metallic Materials" Metals 12, no. 11: 1804. https://doi.org/10.3390/met12111804
APA StyleTan, X., Tan, E., & Sun, L. (2022). Dislocation Dynamics Model to Simulate Motion of Dislocation Loops in Metallic Materials. Metals, 12(11), 1804. https://doi.org/10.3390/met12111804







