Stress Rupture Life Prediction Method for Notched Specimens Based on Minimum Average Von Mises Equivalent Stress
Abstract
:1. Introduction
2. Experiment and Results
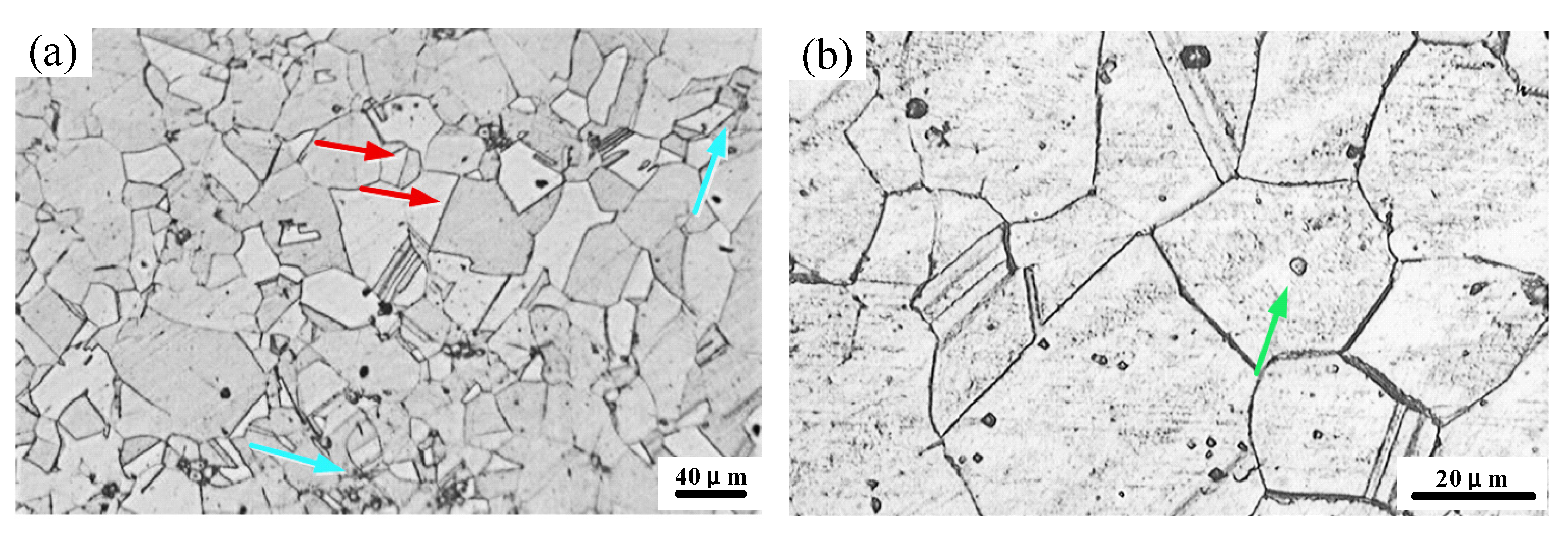
2.1. Composition and Microstructure Characterization
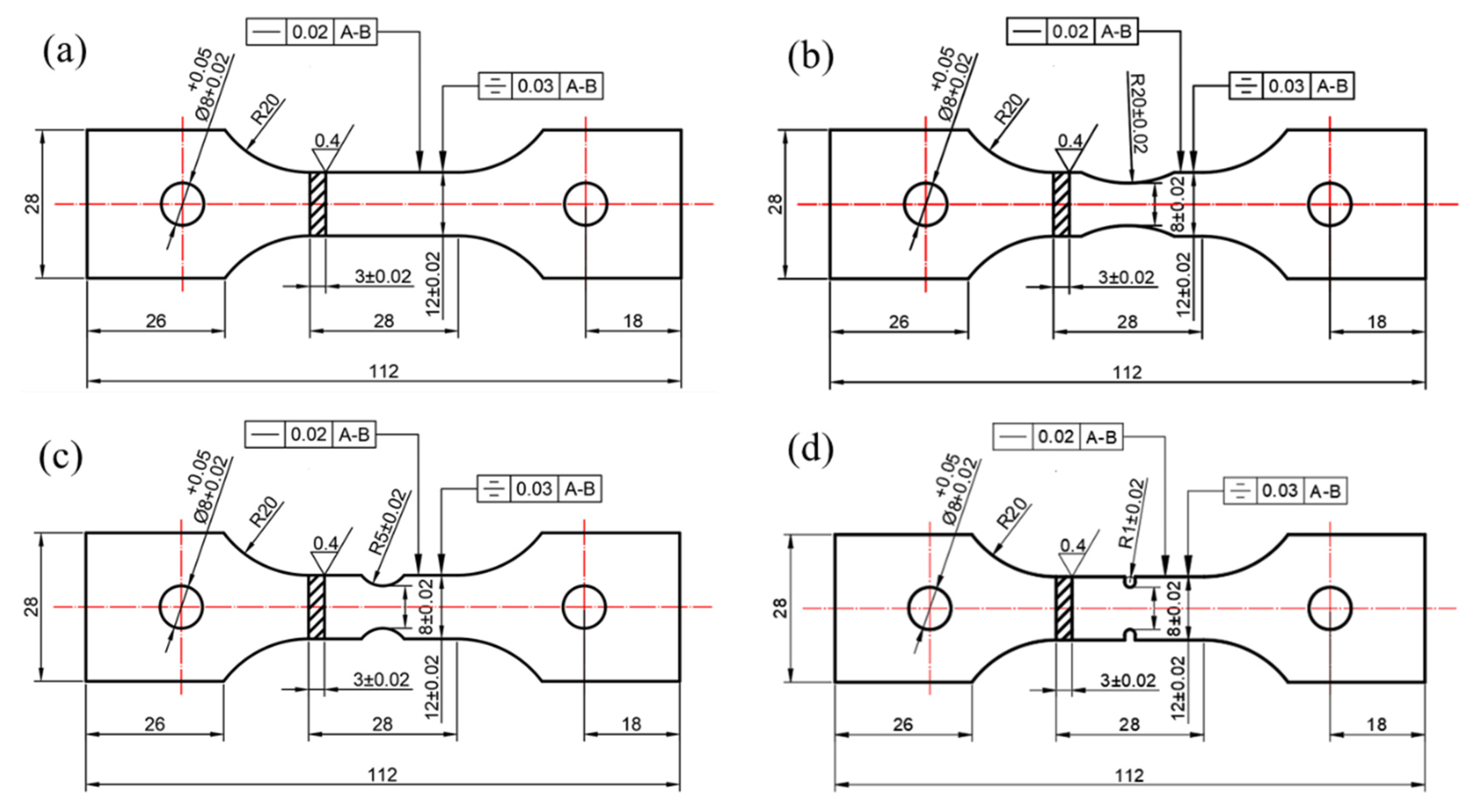
2.2. Specimens and Test
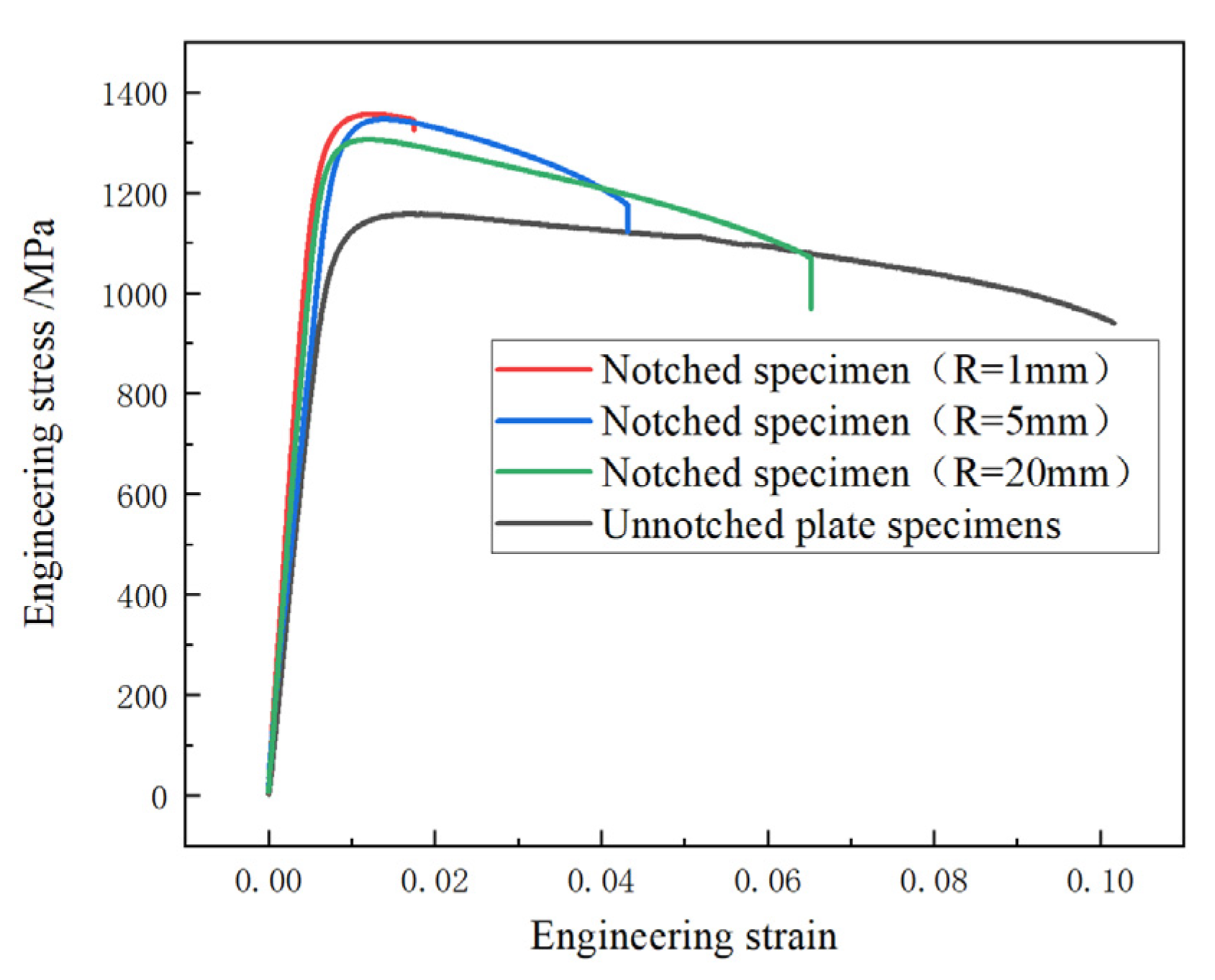
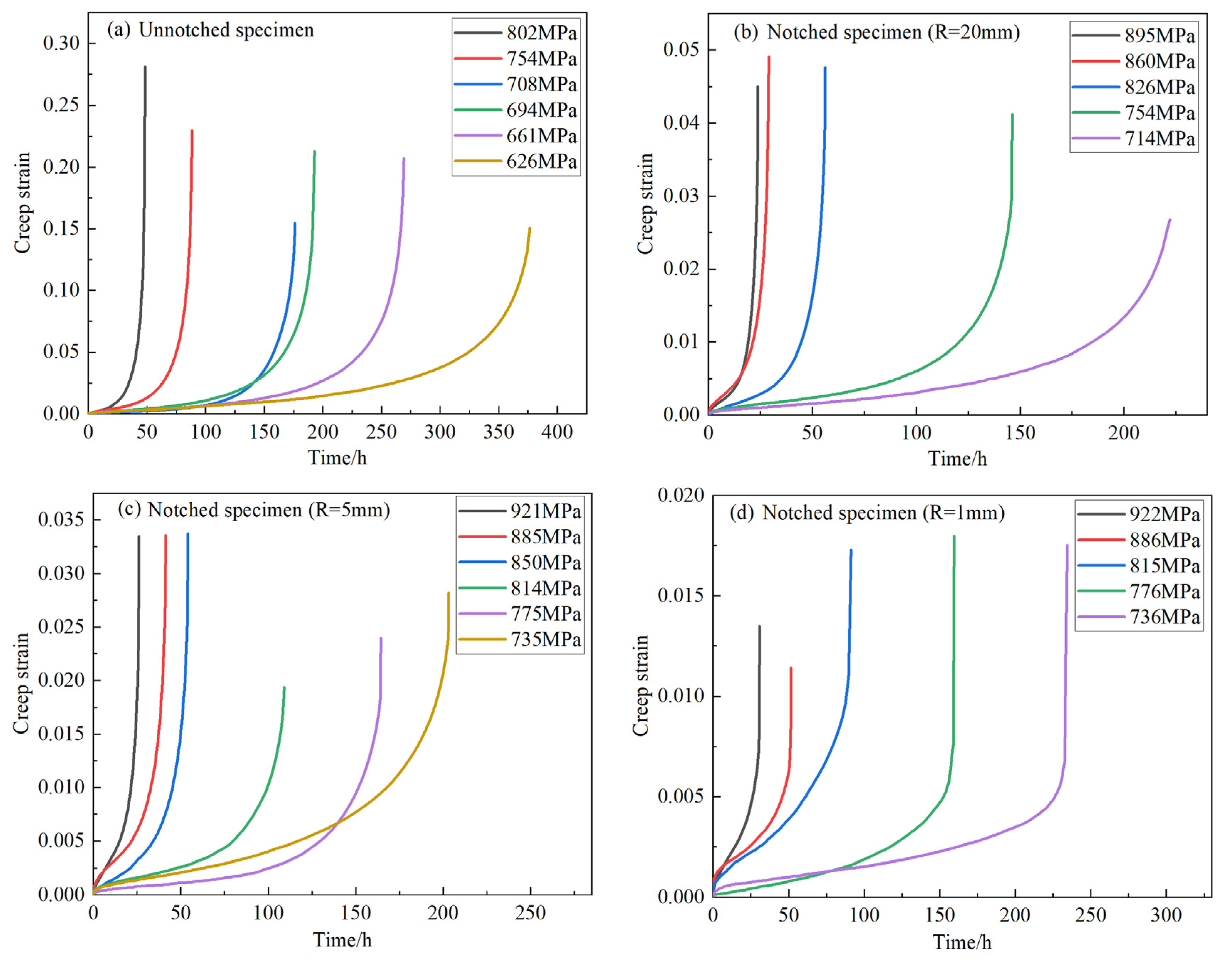
2.3. Experimental Results
3. Life Prediction Method
3.1. Constitutive Model
3.1.1. Multilinear Isotropic Hardening Constitutive Model
3.1.2. Creep Constitutive Model
- 1.
- Norton law
- 2.
- Exponential form
- 3.
- θ Projection approach
- 4.
- Ye model
3.2. Von Mises Equivalent Stress
3.3. Creep Life Equation
- Monkman–Grant equation
- 2.
- Larson–Miller equation
- 3.
- Wilshire equation
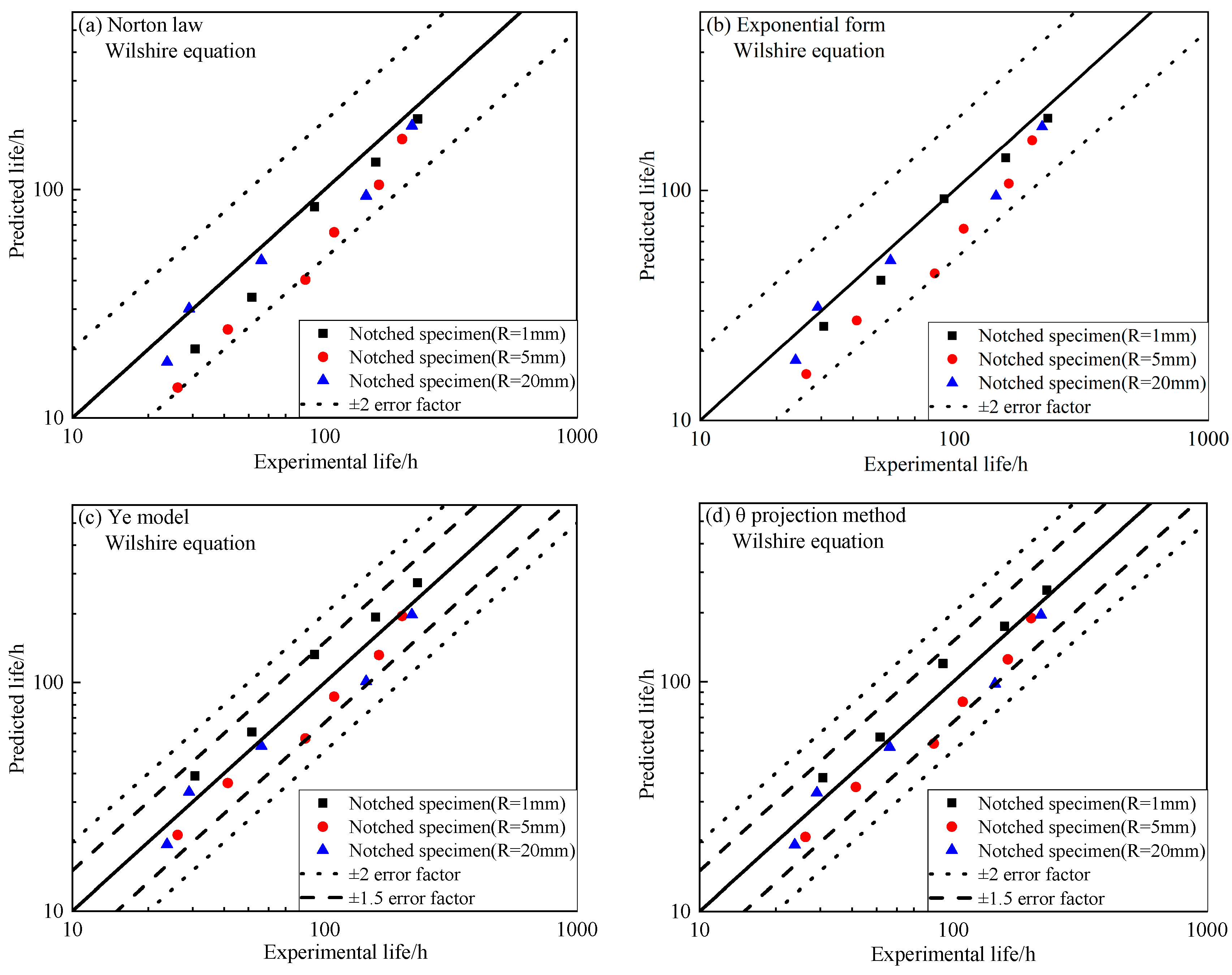
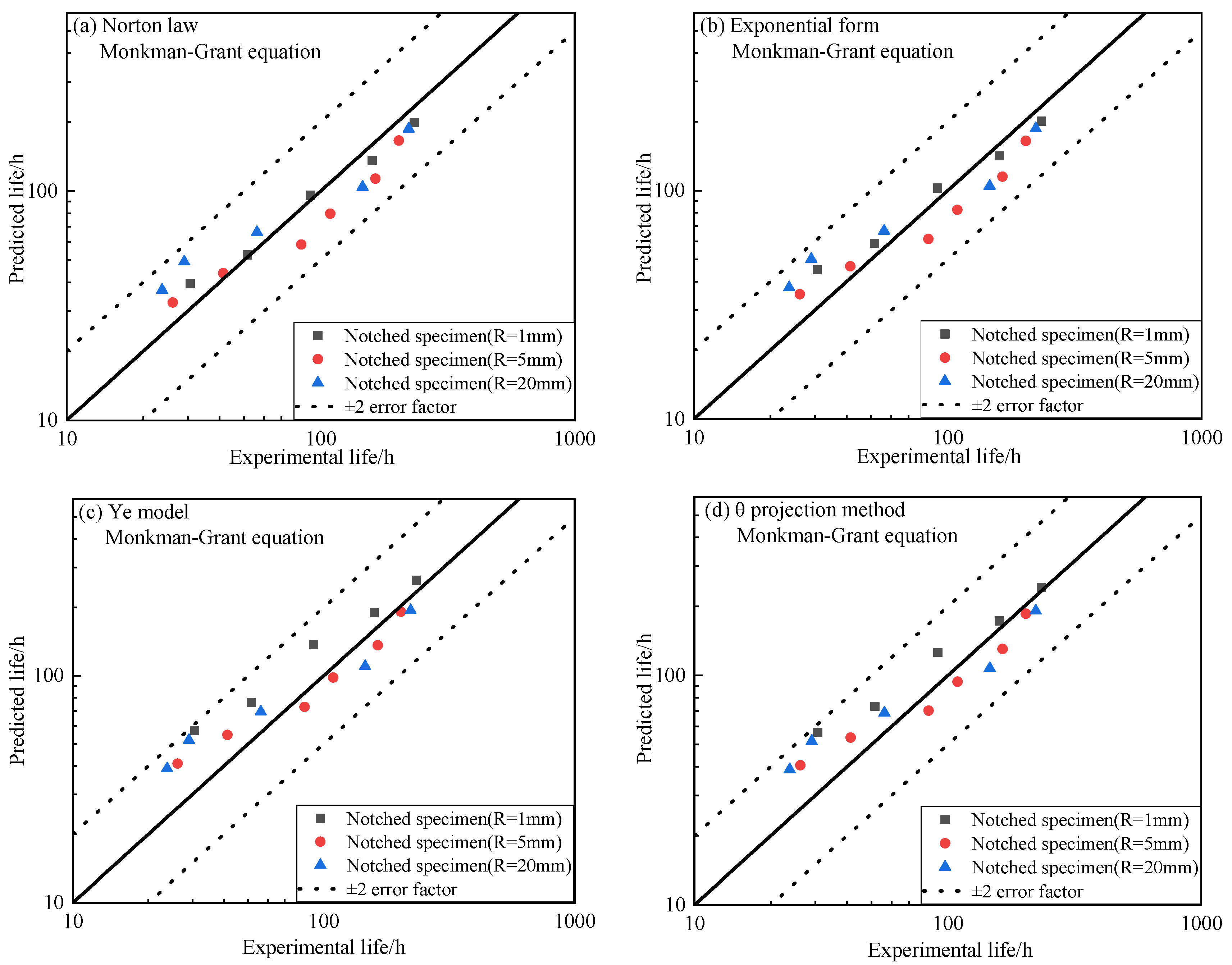
3.4. Analysis of Prediction Results
4. Conclusions
- The change rule of AVES with time is that it first decreases rapidly and then increases slowly, so there is a minimum value of AVES.
- With the increase of notch stress concentration coefficient, the MAVES calculated by the second stage model is larger than that calculated by the whole stage model.
- Whatever the life equation or constitutive model is used, the results of notch life prediction using MAVES as the characteristic stress are within 2 times the dispersion band. If a three-stage creep constitutive model is used, the predicted results are scattered within a factor of 1.5.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Al-Faddagh, K.D.; Fenner, R.T.; Webster, G.A. Steady-state stress distributions in circumferentially notched bars subjected to creep. J. Strain Anal. Eng. Des. 1982, 17, 123–132. [Google Scholar] [CrossRef]
- Hayhurst, D.R.; Webster, G.A. An overview on studies of stress state effects during creep of circumferentially notched bars. In Techniques for Multiaxial Creep Testing; Springer: Berlin/Heidelberg, Germany, 1986; pp. 137–175. [Google Scholar]
- Hyde, T.H.; Xia, L.; Becker, A.A. Prediction of creep failure in aeroengine materials under multi-axial stress states. Int. J. Mech. Sci. 1996, 38, 385–403. [Google Scholar] [CrossRef]
- Eggeler, G.; Wiesner, C. A numerical study of parameters controlling stress redistribution in circular notched specimens during creep. J. Strain Anal. Eng. Des. 1993, 28, 13–22. [Google Scholar] [CrossRef]
- Webster, G.A.; Nikbin, K.M.; Biglari, F. Finite element analysis of notched bar skeletal point stresses and dimension changes due to creep. Fatigue Fract. Eng. Mater. Struct. 2004, 27, 297–303. [Google Scholar] [CrossRef] [Green Version]
- Hayhurst, D.R.; Henderson, J.T. Creep stress redistribution in notched bars. Int. J. Mech. Sci. 1977, 19, 133–146. [Google Scholar] [CrossRef]
- McClintock, F.A. A Criterion for Ductile Fracture by the Growth of Holes. J. Appl. Mech. 1968, 35, 363–371. [Google Scholar] [CrossRef]
- Cocks, A.; Ashby, M.F. Intergranular fracture during power-law creep under multiaxial stresses. Met. Sci. 1980, 14, 395–402. [Google Scholar] [CrossRef]
- Wen, J.F.; Tu, S.T. A multiaxial creep-damage model for creep crack growth considering cavity growth and microcrack interaction. Eng. Fract. Mech. 2014, 123, 197–210. [Google Scholar] [CrossRef]
- Spindler, M.W. The multiaxial and uniaxial creep ductility of Type 304 steel as a function of stress and strain rate. High Temp. Technol. 2004, 21, 47–54. [Google Scholar] [CrossRef]
- Wen, J.F.; Tu, S.T.; Xuan, F.Z.; Zhang, X.W.; Gao, X.L. Effects of stress level and stress state on creep ductility: Evaluation of different models. J. Mater. Sci. Technol. 2016, 32, 695–704. [Google Scholar] [CrossRef]
- Webster, P.S.; Pickard, A.C. The prediction of stress rupture life of notched specimens in the beta-processed titanium alloy Ti5331s. J. Strain Anal. Eng. Des. 1987, 22, 145–153. [Google Scholar] [CrossRef]
- Wang, R.-Z. Creep-fatigue behaviors and life assessments in two nickel-based superalloys. J. Press. Vessel. Technol. 2018, 140, 031405. [Google Scholar] [CrossRef]
- Abdallah, Z.; Gray, V.; Whittaker, M. A critical analysis of the conventionally employed creep lifing methods. Materials 2014, 7, 3371–3398. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kim, W.G.; Yin, S.N.; Kim, Y.W.; Chang, J.H. Creep characterization of a Ni-based Hastelloy-X alloy by using theta projection method. Eng. Fract. Mech. 2008, 75, 4985–4995. [Google Scholar] [CrossRef]
- Evans, R.W.; Scharning, P.J. The θ projection method applied to small strain creep of commercial aluminum alloy. Mater. Sci. Technol. 2001, 17, 487–493. [Google Scholar] [CrossRef]
- Evans, R.W.; Wilshire, B. The role of grain-boundary cavities during tertiary creep. In Creep and Fracture of Engineering Materials and Structures; Wilshire, B., Owen, D.R.J., Eds.; Pineridge Press: Swansea, UK, 1981; pp. 303–314. [Google Scholar]
- Ye, W.; Hu, X.; Song, Y. A new creep model and its application in the evaluation of creep properties of a titanium alloy at 500 °C. J. Mech. Sci. Technol. 2020, 34, 2317–2326. [Google Scholar] [CrossRef]
- Monkman, F.C.; Grant, N.J. An empirical relationship between rupture life and minimum creep rate in creep rupture tests. Proc. ASTM 1956, 56, 91–103. [Google Scholar]
- Dobeš, F.; Milička, K. The relation between minimum creep rate and time to fracture. Met. Sci. 1976, 10, 382–384. [Google Scholar] [CrossRef]
- ACP; AMN; ASLM. Analysis of first order kinetics for tertiary creep in AISI 304 stainless steel. Acta Mater. 1996, 44, 4059–4069. [Google Scholar] [CrossRef]
- Phaniraj, C.; Chou, D.; Hary, B.K.; Rao, K. Relationship between time to reach Monkman–Grant ductility and rupture life. Scripta Mater. 2003, 48, 1313–1318. [Google Scholar] [CrossRef]
- Larson, F.R.; Miller, J. A time-temperature relationship for rupture and creep stresses. Trans. ASME 1952, 74, 765–775. [Google Scholar]
- Evans, M. Method for improving parametric creep rupture life of 2·25Cr–1Mo steel using artificial neural networks. Mater. Sci. Technol. 1999, 15, 647–658. [Google Scholar] [CrossRef]
- Lu, X.; Du, J.; Deng, Q.; Zhuang, J. Stress rupture properties of GH4169 superalloy. J. Mater. Res. Technol. 2014, 3, 107–113. [Google Scholar] [CrossRef] [Green Version]
- Zhao, J.; Li, D.M.; Fang, Y.Y. Application of Manson–Haferd and Larson–Miller Methods in Creep Rupture Property Evaluation of Heat-Resistant Steels. J. Press. Vessel Technol. 2010, 132, 064502. [Google Scholar] [CrossRef]
- Wilshire, B.; Scharning, P.J.; Hurst, R. A new approach to creep data assessment. Mater. Sci. Eng. A 2009, 510, 3–6. [Google Scholar] [CrossRef]
- Holmström, S.; Auerkari, P. Predicting creep rupture from early strain data. Mater. Sci. Eng. A 2009, 510, 25–28. [Google Scholar] [CrossRef]
- Abdallah, Z.; Perkins, K.; Williams, S. Advances in the Wilshire extrapolation technique—Full creep curve representation for the aerospace alloy Titanium 834. Mater. Sci. Eng. A 2012, 550, 176–182. [Google Scholar] [CrossRef]
- Whittaker, M.T.; Harrison, W.J.; Lancaster, R.J.; Williams, S.; Whittaker, M.T. An analysis of modern creep lifing methodologies in the titanium alloy Ti6-4. Mater. Sci. Eng. A 2013, 577, 114–119. [Google Scholar] [CrossRef] [Green Version]
- Jeffs, S.P.; Lancaster, R.J.; Garcia, T.E. Creep lifing methodologies applied to a single crystal superalloy by use of small scale test techniques. Mater. Sci. Eng. A 2015, 636, 529–535. [Google Scholar] [CrossRef] [Green Version]
- Huang, J.; Shi, D.Q.; Yang, X.G. A physically based methodology for predicting anisotropic creep properties of Ni-based superalloys. Rare Met. 2016, 35, 606–614. [Google Scholar] [CrossRef]

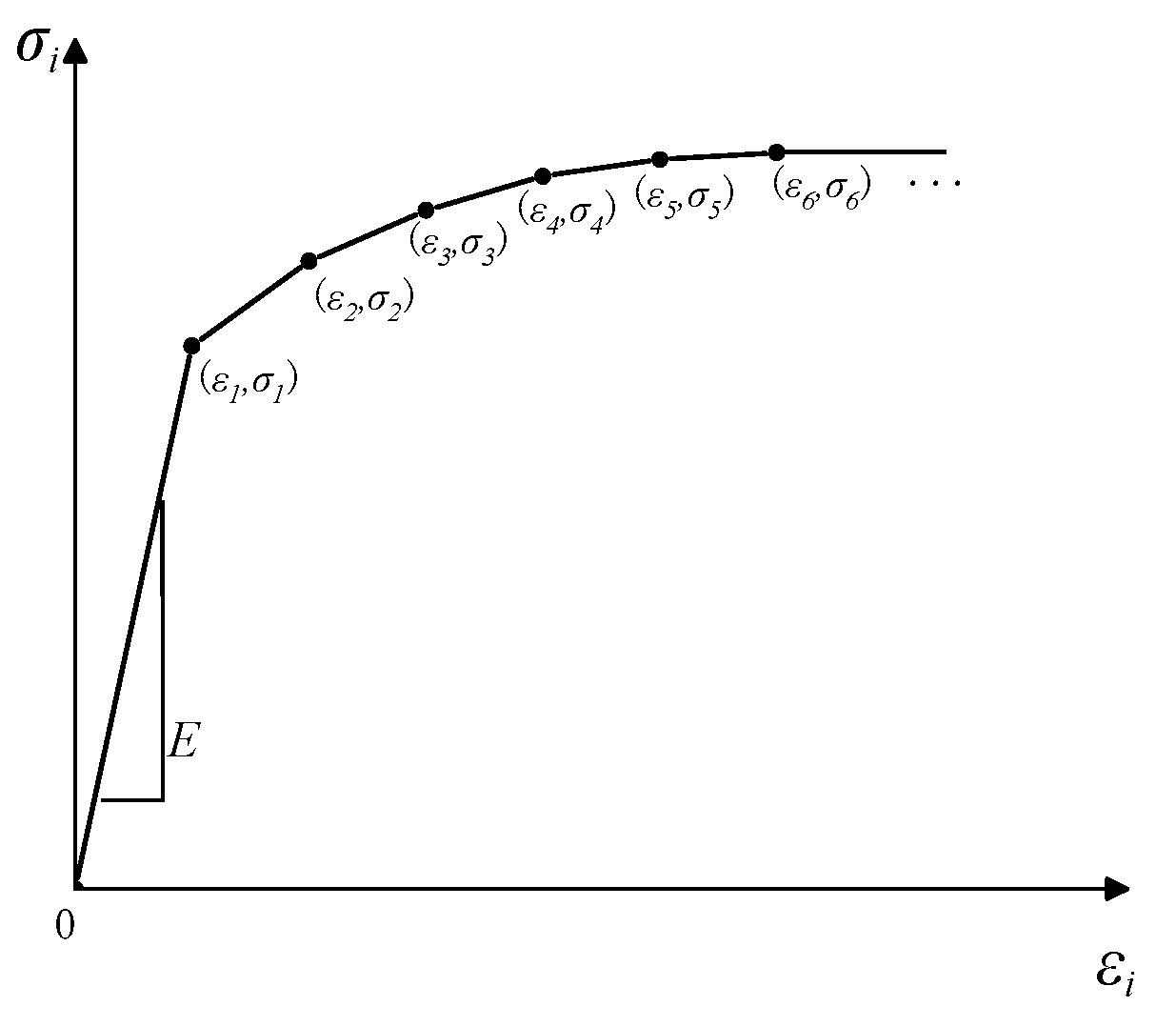

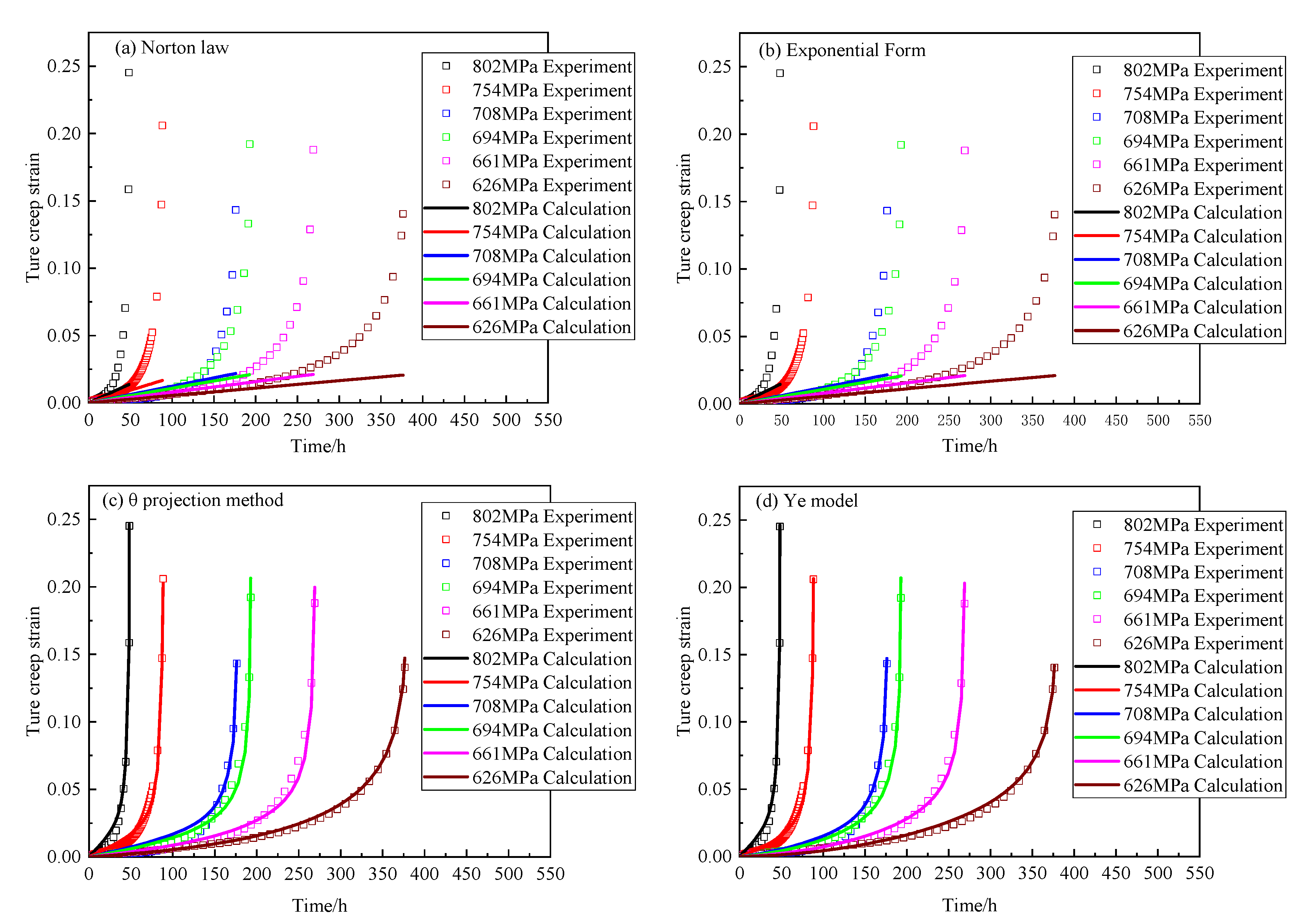
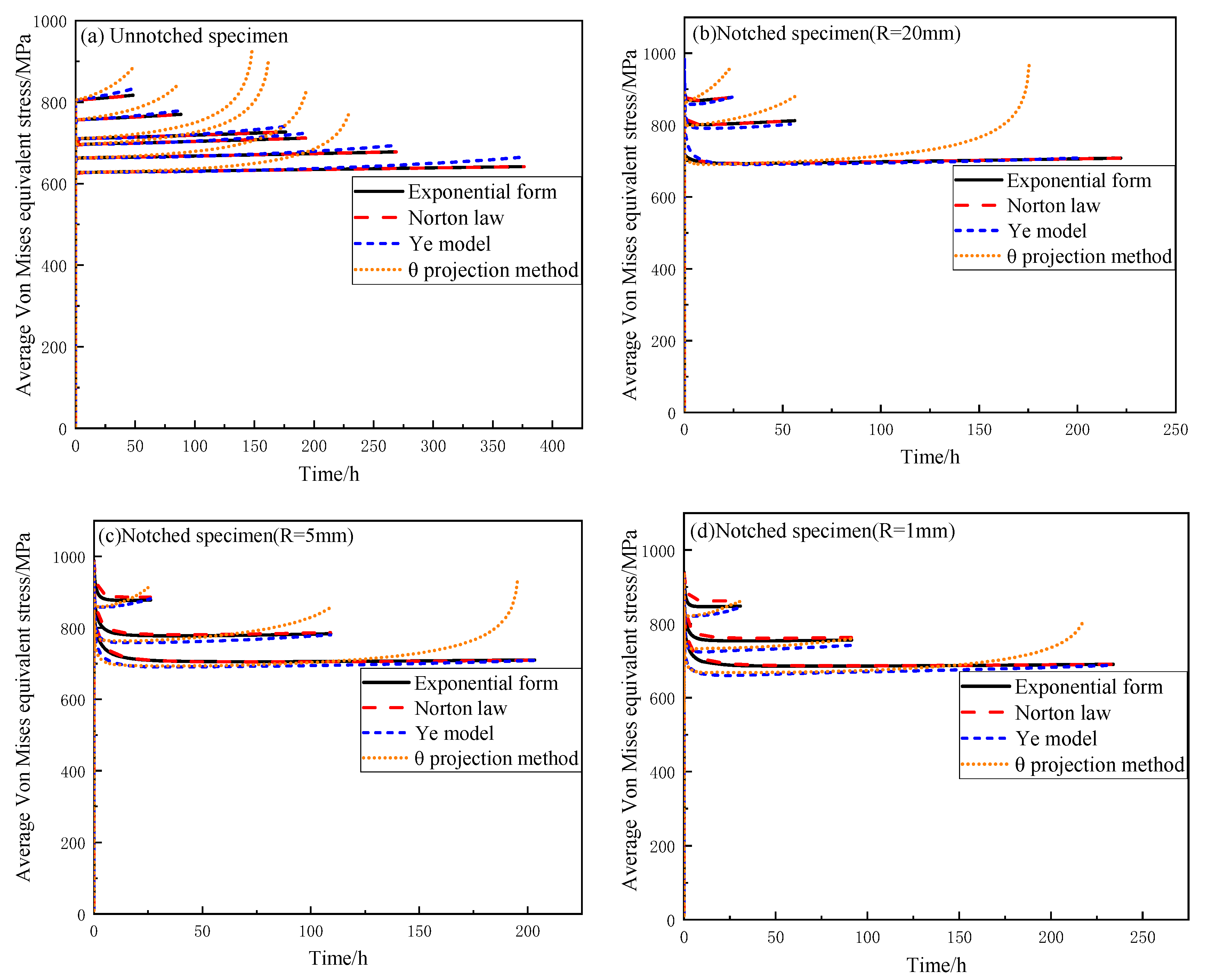
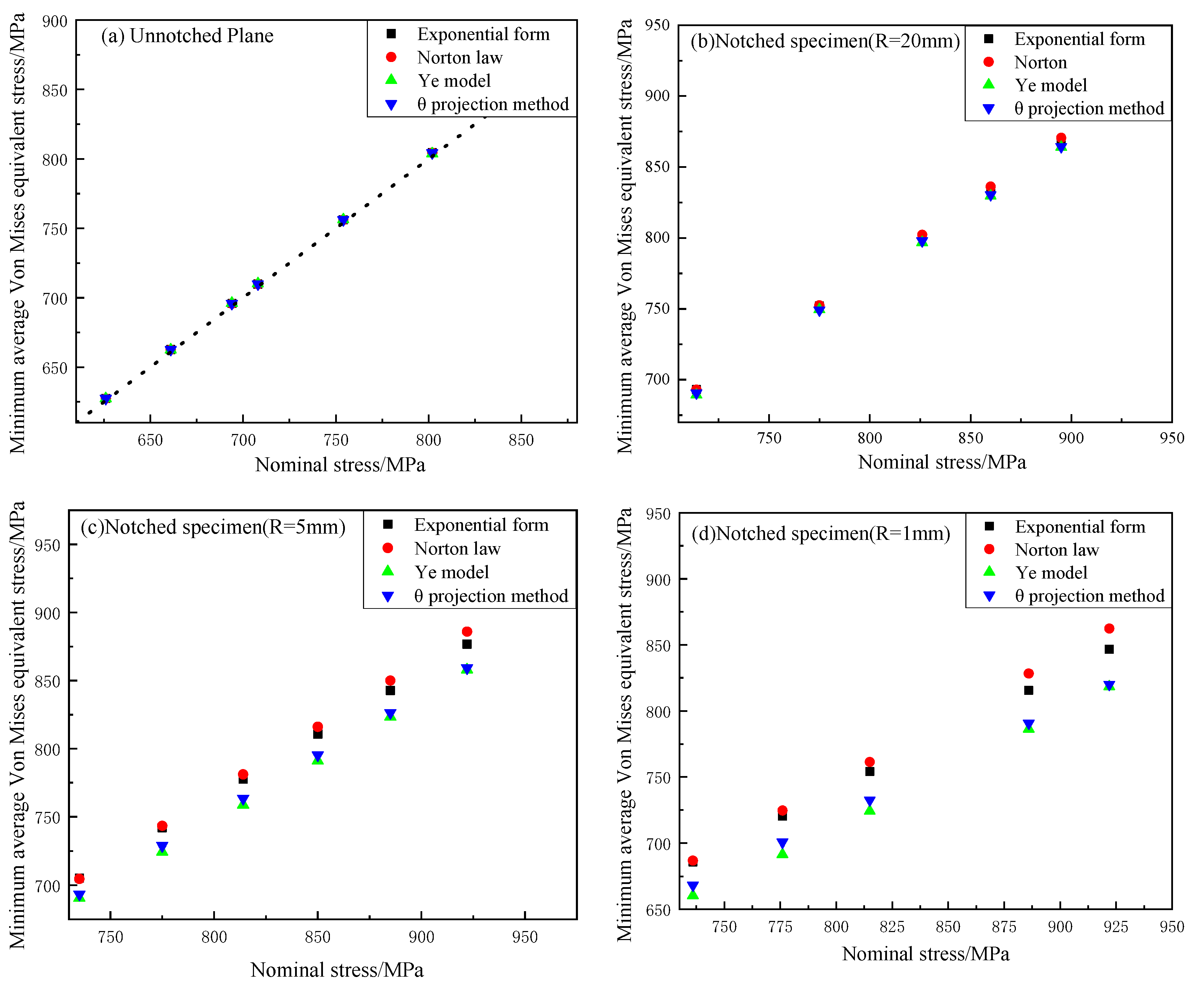


| Element | Cr | Ti | Fe | Mo | Al | Nb |
| Wt% | 17.00~21.00 | 0.75~1.15 | 14.2~24.0 | 2.80~3.30 | 0.30~0.70 | 5.00~5.50 |
| Element | N | C | Mn | Si | P | Ni |
| Wt% | ≤0.01 | 0.015~0.006 | ≤0.35 | ≤0.35 | ≤0.015 | Bal. |
| Norton law | A | n | ||||||
| 1.62 × 10−3 | 6.625 | |||||||
| Exponential form | B | d | ||||||
| 1.41 × 10−7 | 104.68 | |||||||
| θ projection method | b1 | b2 | b3 | b4 | f1 | f2 | f3 | f4 |
| −0.885 | −10.2 | −5.50 | −2.05 | −1.1 × 10−4 | 9.7 × 10−3 | 5.1 × 10−3 | −2.42 × 10−4 | |
| Ye model | c1 | c2 | c3 | c4 | c5 | c6 | c7 | c8 |
| 1.955 | 6.726 | −10.5 | 16.74 | −9.26 | 1.0 × 10−5 | 19.31 | −9.41 |
| Monkman–Grant equation | A″ | n* | ||||
| 1263 | −0.1153 | |||||
| Larson–Miller equation | a1 | a2 | a3 | a4 | CLM | T |
| 2.585 | 11.80 | 137.82 | −5545 | 3.023 | 923.15 | |
| Wilshire equation | σUTS | k1′ | u | |||
| 1150 | 0.1336 | 0.254 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ji, D.; Hu, X.; Zhao, Z.; Jia, X.; Hu, X.; Song, Y. Stress Rupture Life Prediction Method for Notched Specimens Based on Minimum Average Von Mises Equivalent Stress. Metals 2022, 12, 68. https://doi.org/10.3390/met12010068
Ji D, Hu X, Zhao Z, Jia X, Hu X, Song Y. Stress Rupture Life Prediction Method for Notched Specimens Based on Minimum Average Von Mises Equivalent Stress. Metals. 2022; 12(1):68. https://doi.org/10.3390/met12010068
Chicago/Turabian StyleJi, Dawei, Xianming Hu, Zuopeng Zhao, Xu Jia, Xuteng Hu, and Yingdong Song. 2022. "Stress Rupture Life Prediction Method for Notched Specimens Based on Minimum Average Von Mises Equivalent Stress" Metals 12, no. 1: 68. https://doi.org/10.3390/met12010068
APA StyleJi, D., Hu, X., Zhao, Z., Jia, X., Hu, X., & Song, Y. (2022). Stress Rupture Life Prediction Method for Notched Specimens Based on Minimum Average Von Mises Equivalent Stress. Metals, 12(1), 68. https://doi.org/10.3390/met12010068






