Studying the Thermally Activated Processes Operating during Deformation of hcp and bcc Mg–Li Metal-Matrix Composites
Abstract
:1. Introduction
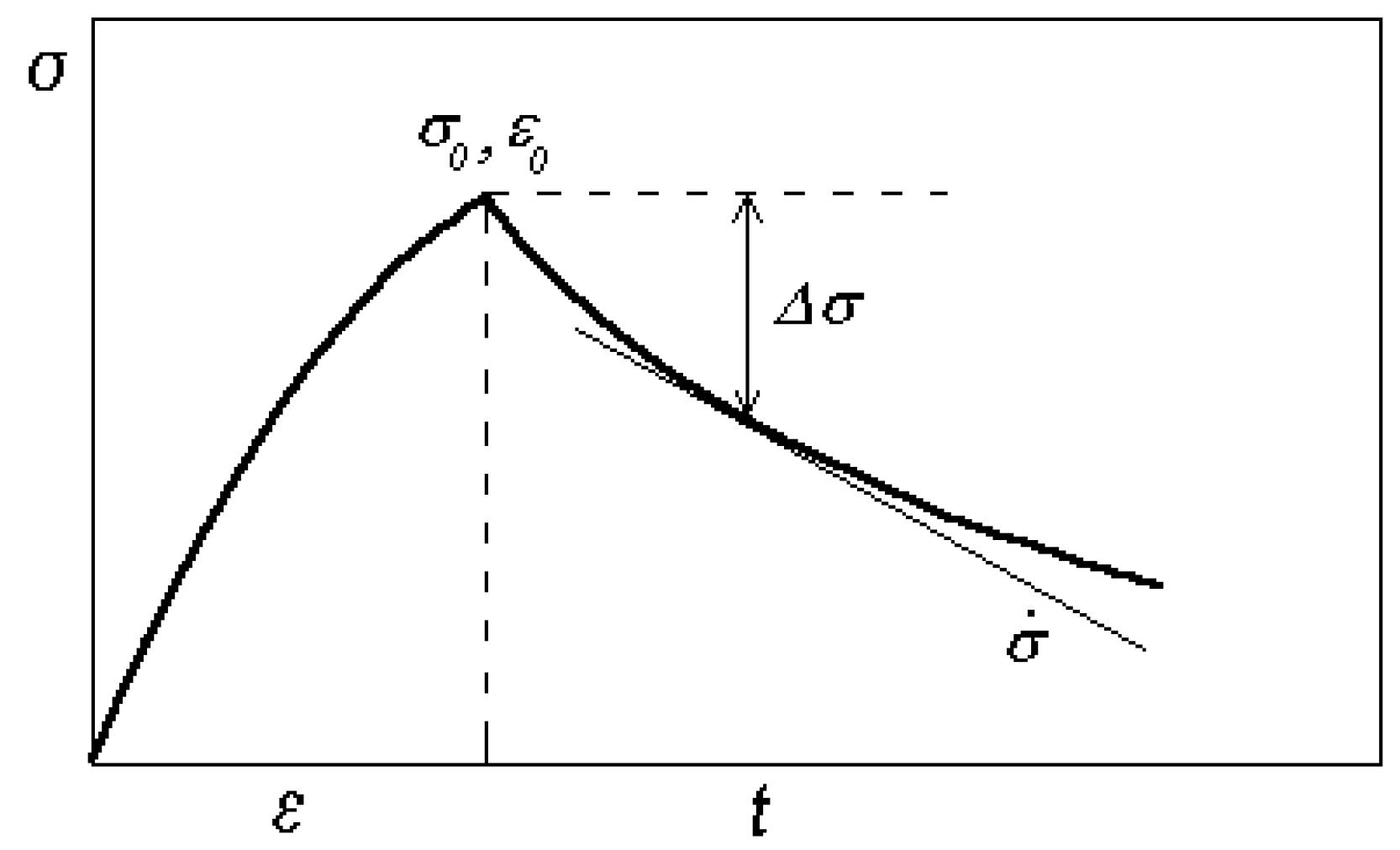
2. Materials and Methods
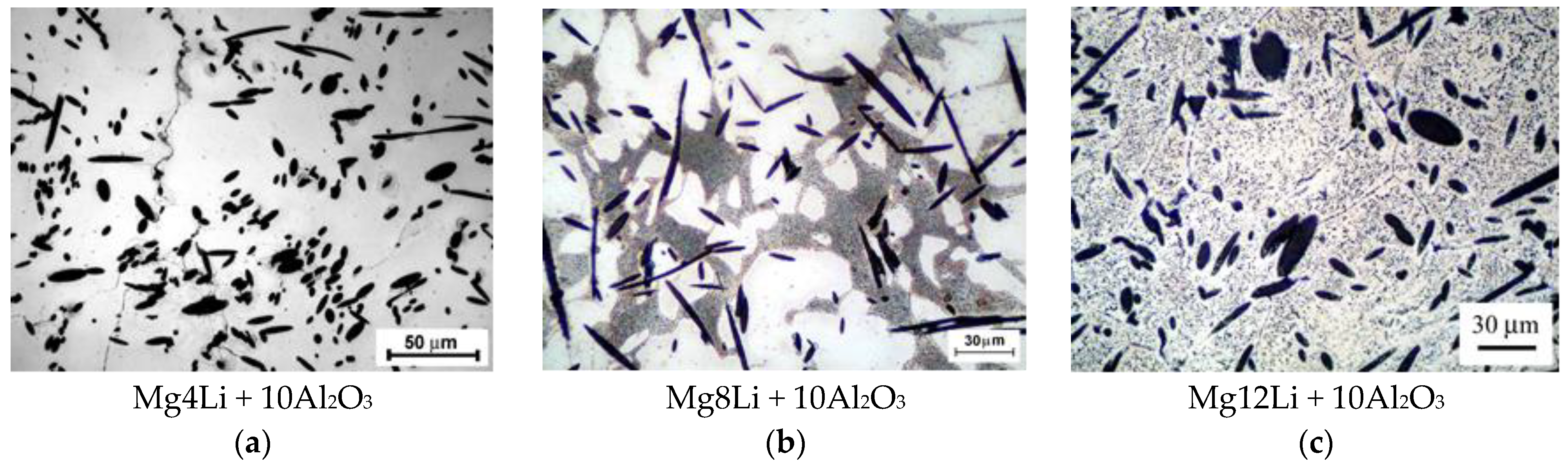
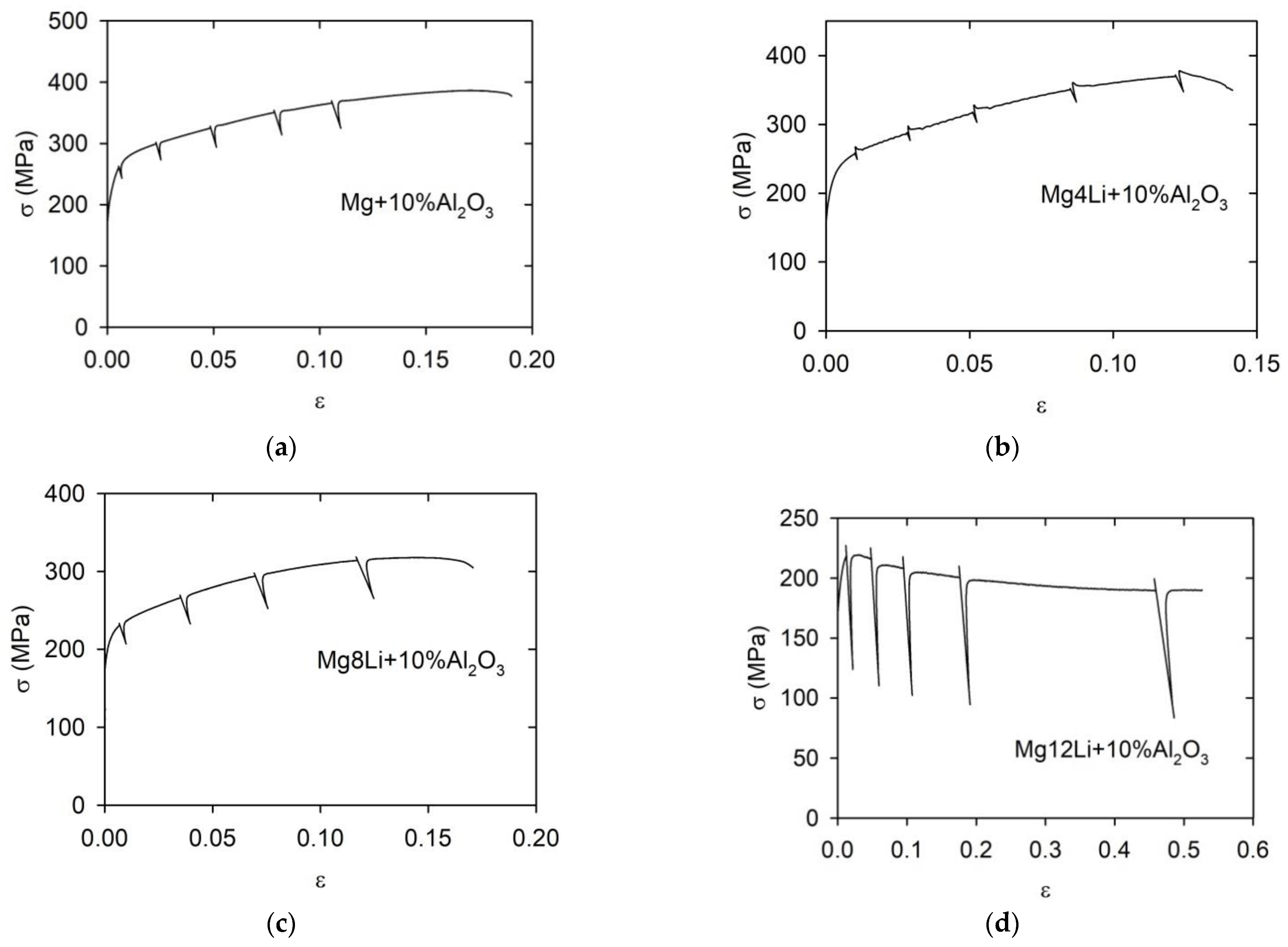
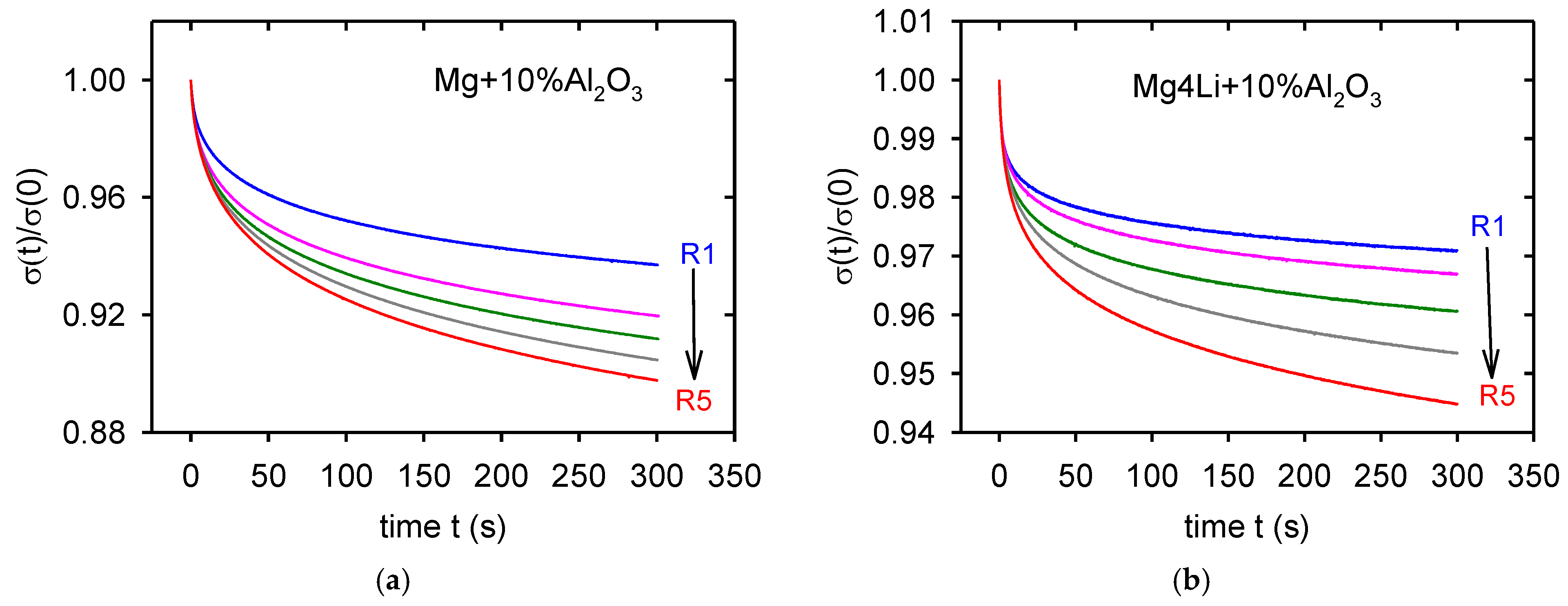
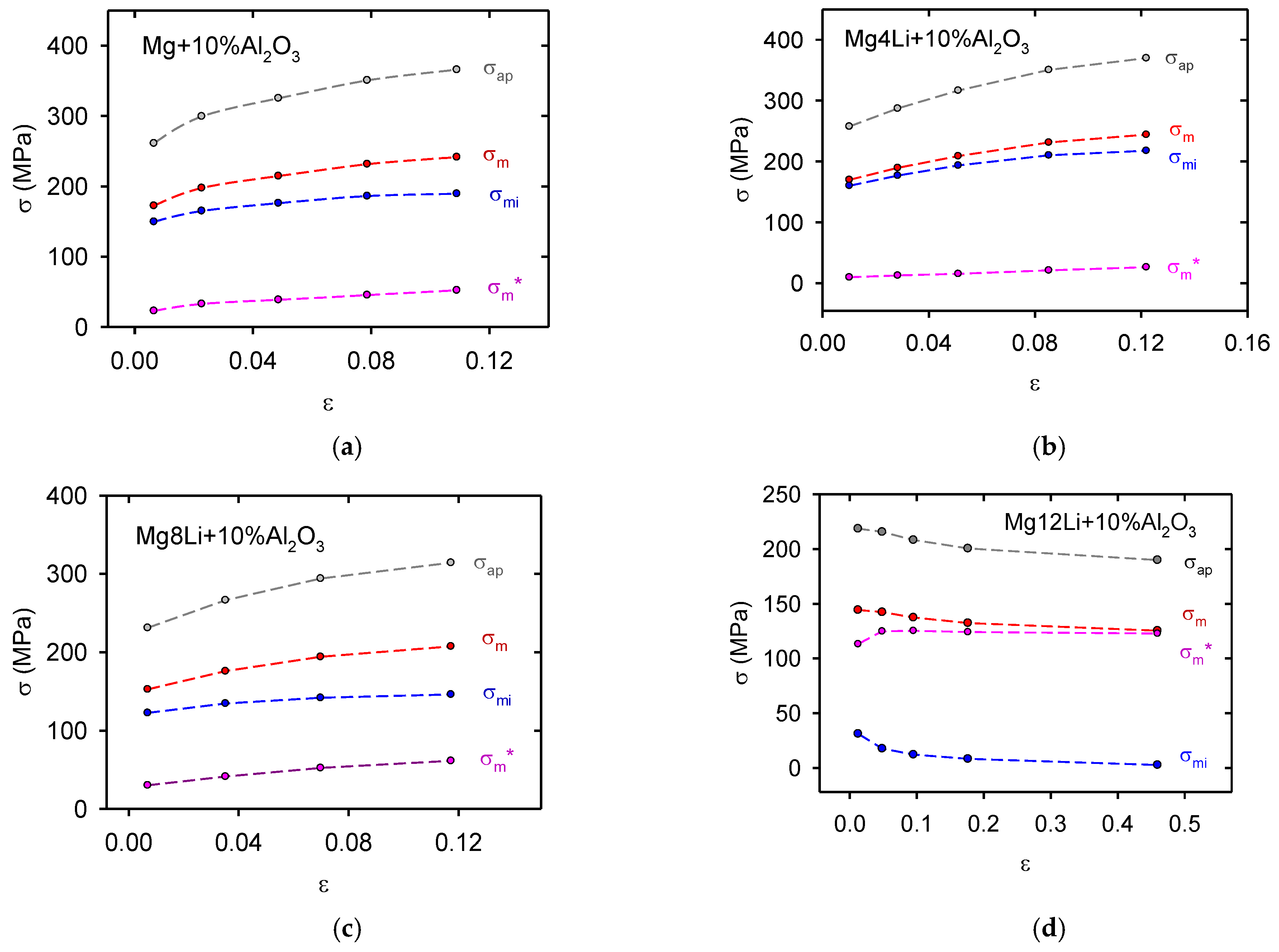
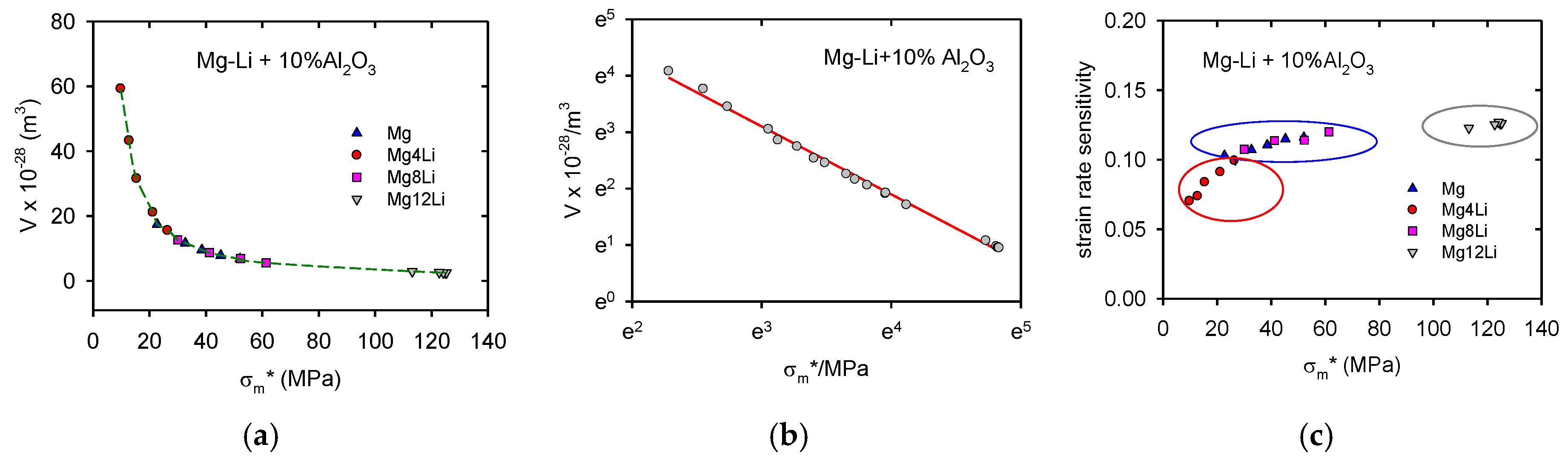
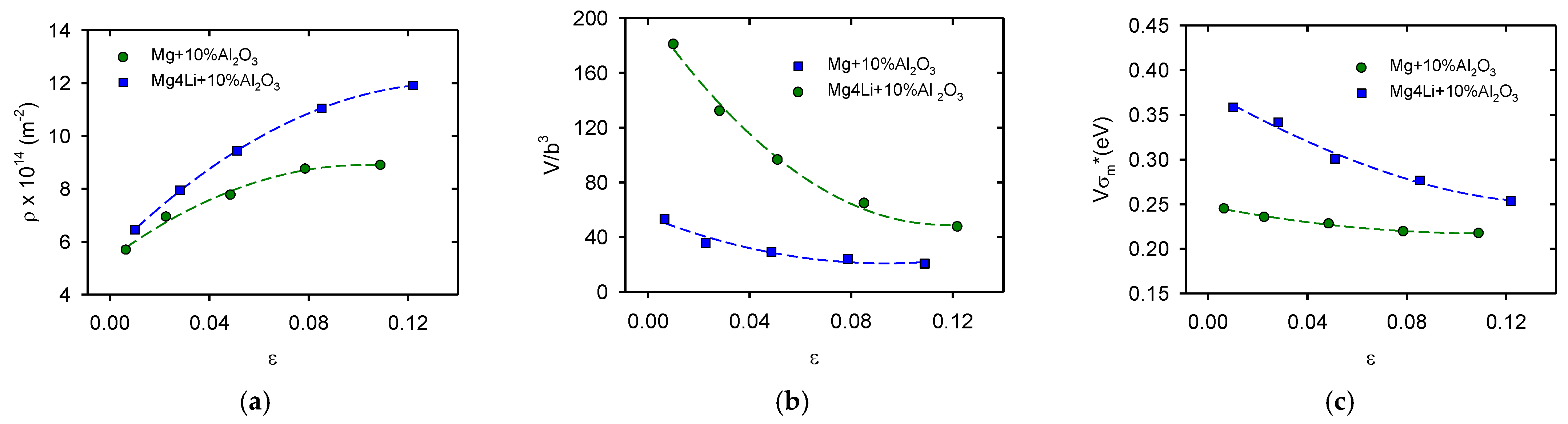
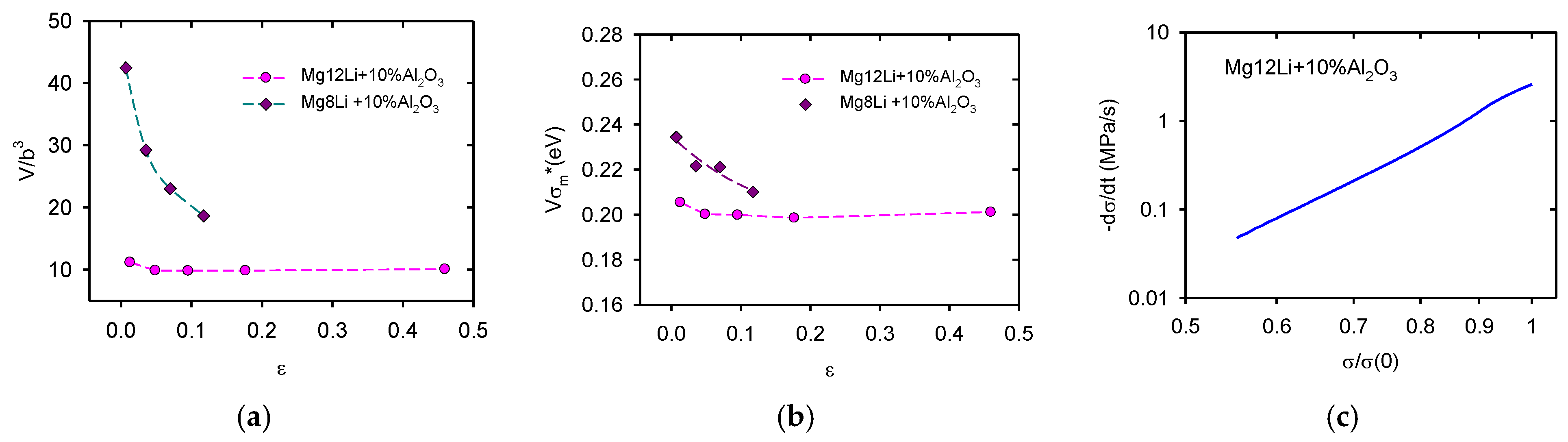
3. Results
4. Discussion
4.1. Mg and Mg4Li Composites (α Phase)
4.2. Mg12Li (β Phase), Mg8Li (α + β) Phase
5. Conclusions
- Components of the matrix stress were estimated.
- While the dislocation density in the Mg and Mg4Li composites increased with strain, in the Mg12Li composite, the dislocation density decreased due to recovery.
- All values of the activation volume vs. effective stress dependence followed one “master curve” independent of the matrix alloy.
- The main thermally activated process in the hcp (Mg, Mg4Li) composites was very probably the dislocation motion in noncompact planes.
- The significant stress decrease during stress-relaxation tests in the Mg12Li composite was observed as a consequence of the massive recovery process. It was a very probable reason for the low value of the internal stress in the bcc matrix.
- The higher stress in the α phase and better ductility of the β phase represent very good issues for many structural applications of the (α + β) alloys.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Feltham, P. Stress Relaxation in Magnesium at Low Temperatures. Phys. Status Sol. 1963, 3, 1340–1346. [Google Scholar] [CrossRef]
- Sargent, G.A. Stress relaxation and thermal activation in niobium. Acta Met. 1965, 13, 663–671. [Google Scholar] [CrossRef]
- Dotsentko, V.I. Stress Relaxation in Crystals. Phys. Status Sol. (B) 1979, 93, 11–43. [Google Scholar] [CrossRef]
- Kubin, L.P. Description de la préplasticité par la théorie des phénomènes thermiquement activés. Philos. Mag. 1974, 30, 705–718. [Google Scholar] [CrossRef]
- Caillard, D.; Martin, J.-L. Thermally Activated Mechanisms in Crystal Plasticity; Pergamon Materials Series; Elsevier: Oxford, UK, 2003; Volume 8. [Google Scholar]
- Hariharan, K.; Jain, J. Stress relaxation test: Issues in modelling and interpretation. Manuf. Lett. 2020, 26, 64–68. [Google Scholar] [CrossRef]
- Lo Picolo, B.; Spätig, P.; Kruml, T.; Martin, J.-L.; Bonneville, J. Characterising thermally activated dislocation mechanisms. Mater. Sci. Eng. A 2001, 309–310, 251–255. [Google Scholar] [CrossRef]
- Bonadé, R.; Spätig, P. The evolution of the mobile dislocation density during successive stress relaxation transients. Mater. Sci. Eng. A 2008, 483–484, 203–206. [Google Scholar] [CrossRef]
- Wu, R.Z.; Qu, Z.K.; Zhang, M.L. Reviews on the influences of alloying elements on the microstructure and mechanical properties of Mg-Li base alloys. Rev. Adv. Mater. Sci. 2010, 24, 35–43. [Google Scholar]
- Zhou, Y.; Chen, Z.; Ji, J.; Sun, Z. Effects of second phases on deformation behavior and dynamic recrystallization of as-cast Mg-4.3Li-4.1Zn-1.4Y alloy during hot compression. J. Alloys Compd. 2019, 770, 540–548. [Google Scholar] [CrossRef]
- Trojanová, Z.; Drozd, Z.; Lukáč, P.; Chmelík, P. Deformation behaviour of Mg–Li alloys at elevated temperatures. Mater. Sci. Eng. A Struct. 2005, 410–411, 148–151. [Google Scholar] [CrossRef]
- Król, M.; Snopiński, P.; Pagáč, M.; Hajnyš, J.; Petrů, J. Hot Deformation Treatment of Grain-Modified Mg–Li Alloy. Materials 2020, 13, 4557. [Google Scholar] [CrossRef]
- Kúdela, S. Magnesium-lithium matrix composites. An overview. Int. J. Mater. Prod. Technol. 2003, 18, 91–115. [Google Scholar] [CrossRef]
- Sun, Y.H.; Wang, R.C.; Peng, C.Q.; Feng, Y.; Yang, M. Recent Progress in Mg-Li matrix composites. Trans. Nonferrous Met. Soc. China 2019, 29, 1–14. [Google Scholar] [CrossRef]
- Trojanová, Z.; Drozd, Z.; Kúdela, S.; Száraz, Z.; Lukáč, P. Strengthening in Mg-Li matrix composites. Compos. Sci. Technol. 2007, 67, 1965–1973. [Google Scholar] [CrossRef]
- Clyne, T.W.; Whithers, P.J. An Introduction to Metal Matrix Composites; Cambridge University Press: Cambridge, UK, 1993. [Google Scholar]
- Aikin, R.M., Jr.; Christodoulou, L. The Role of Equiaxed Particles on the Yield Stress of Composites. Scr. Met. Mater. 1991, 25, 9–14. [Google Scholar] [CrossRef]
- Casati, R.; Vedani, M. Metal matrix composites reinforced by nano particles—A review. Metal 2014, 4, 65–83. [Google Scholar] [CrossRef]
- Li, J.M.C. Dislocation Dynamics in Deformation and Recovery. Can. J. Appl. Phys. 1967, 45, 493–509. [Google Scholar] [CrossRef]
- De Batist, R.; Callens, A. On the analysis of stress relaxation experiments. Phys. Status Sol. (A) 1974, 21, 591–595. [Google Scholar] [CrossRef]
- Kocks, U.F.; Argon, A.S.; Ashby, M.F. Thermodynamics and Kinetics of Slip, Prog. Mater. Sci. 19; Pergamon Press: Oxford, UK; Edingurg, UK; New York, NY, USA; Toronto, ON, Canada, 1975. [Google Scholar]
- Barnett, M.R. Twinning and the ductility of magnesium alloys part I: Tension twins. Mater. Sci. Eng. A 2007, 464, 1–7. [Google Scholar] [CrossRef]
- Barnett, M.R. Twinning and the ductility of magnesium alloys part II. “contraction” twins. Mater. Sci. Eng. A 2007, 464, 8–16. [Google Scholar] [CrossRef]
- Taylor, G.I. The mechanisms of plastic deformation of crystals. Part I.-Theoretical. Proc. R. Soc. Lond. 1934, 145, 362–387. [Google Scholar]
- Lavrentev, F.F.; Pokhil, Y.A. Relation of dislocation density in different slip systems to work-hardening parameters for magnesium crystals. Mater. Sci. Eng. 1975, 18, 261–270. [Google Scholar] [CrossRef]
- Cáceres, C.H.; Lukáč, P. Strain hardening behaviour and the Taylor factor of pure magnesium. Philos. Mag. 2008, 88, 977–989. [Google Scholar] [CrossRef]
- Arsenault, R.J.; Shi, N. Dislocation generation due to differences between the coefficients of thermal expansion. Mater. Sci. Eng. 1986, 81, 175–187. [Google Scholar] [CrossRef]
- Ashby, M.F. The deformation of plastically non-homogeneous materials. Philos. Mag. 1970, 21, 399–424. [Google Scholar] [CrossRef]
- Trojanová, Z.; Lukáč, P.; Dlouhý, A. Hardening and softening in Zr-Sn polycrystals. Mater. Sci. Eng. A 1993, 164, 246–251. [Google Scholar] [CrossRef]
- Trojanová, Z.; Drozd, Z.; Halmešová, K.; Džugan, J.; Škraban, T.; Minárik, P.; Németh, G.; Lukáč, P. Strain Hardening in an AZ31 Alloy Submitted to Rotary Swaging. Materials 2021, 14, 157. [Google Scholar] [CrossRef]
- Trojanová, Z.; Máthis, K.; Lukáč, P.; Németh, G.; Chmelík, F. Internal stress and thermally activated dislocation motion in an AZ63 magnesium alloy. Mater. Chem. Phys. 2011, 130, 1146–1150. [Google Scholar] [CrossRef]
- Couret, A.; Caillard, D. An in situ study of prismatic glide in magnesium–I. The rate controlling mechanism. Acta Met. 1985, 33, 1447–1454. [Google Scholar] [CrossRef]
- Couret, A.; Caillard, D. An in situ study of prismatic glide in magnesium–II. Microscopic activation parameters. Acta Met. 1985, 33, 1455–1462. [Google Scholar] [CrossRef]
- Trojanová, Z.; Száraz, Z.; Lukáč, P.; Drozd, Z.; Džugan, J. Strengthening and Thermally Activated Processes in an AX61/Saffil Metal Matrix Composite. Crystals 2020, 10, 466. [Google Scholar] [CrossRef]
- Amadieh, A.; Mitchell, J.; Dorn, J.E. Lithium alloying and dislocation mechanisms for prismatic slip in magnesium. Trans. Aime 1965, 233, 1130. [Google Scholar]
- Máthis, K.; Nyilas, K.; Axt, A.; Dragomir-Cernatescu, I.; Ungár, T.; Lukáč, P. The evolution of non-basal dislocations as a function of deformation temperature in pure magnesium determined by X-ray diffraction. Acta Mater. 2004, 52, 2889–2894. [Google Scholar] [CrossRef]
- Geng, J.; Nie, J.F. Unloading yield effect in a twin-roll-cast Mg-3Al-1Zn alloy. Scripta Mater. 2015, 100, 78–81. [Google Scholar] [CrossRef]
- Trojanová, Z.; Lukáč, P.; Kainer, K.U.; Gartnerová, V. Dynamic strain ageing in selected magnesium alloys containing rare earth elements. Adv. Eng. Mater. 2005, 7, 1027–1032. [Google Scholar] [CrossRef]
- Lubenets, S.V.; Startsev, V.I.; Fomenko, L.S. Strain ageing in Indium based alloys. Czech. J. Phys. B 1986, 36, 493–497. [Google Scholar] [CrossRef]
- Balík, J.; Lukáč, P. On the kinetics of dynamic strain ageing. Kov. Mater. 1998, 36, 3–9. [Google Scholar]
- Dlouhý, A.; Lukáč, P.; Trojanová, Z. Stress relaxations. Kov. Mater. 1984, 26, 688–694. [Google Scholar]
- Taylor, G. Thermally activated deformation of bcc metals and alloys. Prog. Mater. Sci. 1992, 36, 29–61. [Google Scholar] [CrossRef]
- Siedersleben, M.E.; Taylor, G. Slip systems in b.c.c. Li-Mg alloys. Philos. Mag. A 1989, 60, 631. [Google Scholar] [CrossRef]
- Saka, H.; Taylor, G. Thermal-activation parameters for asymmetric {211} slip in Li-Mg alloy crystals. Philos. Mag. A 1982, 45, 973–982. [Google Scholar] [CrossRef]
- Firth, L.D.; Nowaira, H.N.A.; Scott, W. Lattice parameters of lithium-magnesium and lithium-silver alloys. J. Phys. F Met. Phys. 1974, 4, L200. [Google Scholar] [CrossRef]
- Čadek, J. Creep in Metallic Materials; Academia: Prague, Czech Republic, 1988. [Google Scholar]
- Landau, A.I.; Dotsentko, V.D. Power-like dependence of the effective dislocation velocity on load resulting from the stochastic character of motion through a random array of point obstacles. Phys. Stat. Sol. (A) 1976, 37, 709–718. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Trojanová, Z.; Drozd, Z.; Lukáč, P.; Džugan, J. Studying the Thermally Activated Processes Operating during Deformation of hcp and bcc Mg–Li Metal-Matrix Composites. Metals 2021, 11, 473. https://doi.org/10.3390/met11030473
Trojanová Z, Drozd Z, Lukáč P, Džugan J. Studying the Thermally Activated Processes Operating during Deformation of hcp and bcc Mg–Li Metal-Matrix Composites. Metals. 2021; 11(3):473. https://doi.org/10.3390/met11030473
Chicago/Turabian StyleTrojanová, Zuzanka, Zdeněk Drozd, Pavel Lukáč, and Ján Džugan. 2021. "Studying the Thermally Activated Processes Operating during Deformation of hcp and bcc Mg–Li Metal-Matrix Composites" Metals 11, no. 3: 473. https://doi.org/10.3390/met11030473
APA StyleTrojanová, Z., Drozd, Z., Lukáč, P., & Džugan, J. (2021). Studying the Thermally Activated Processes Operating during Deformation of hcp and bcc Mg–Li Metal-Matrix Composites. Metals, 11(3), 473. https://doi.org/10.3390/met11030473







