Integrated Numerical-Experimental Assessment of the Effect of the AZ31B Anisotropic Behaviour in Extended-Surface Treatments by Laser Shock Processing
Abstract
1. Introduction
2. Materials and Methods
2.1. Material Description
2.2. Methodology
2.2.1. Anisotropic Hardening Formulation based on Hill’s Yield Surface to Model Alternative Loading Paths Presented in LSP
2.2.2. Model Calibration Based on the Anisotropic Stress-Strain Curves of Mg AZ31B Alloy
2.2.3. Experimental Determination of the Spatial Pressure Pulse Profiles
3. Results
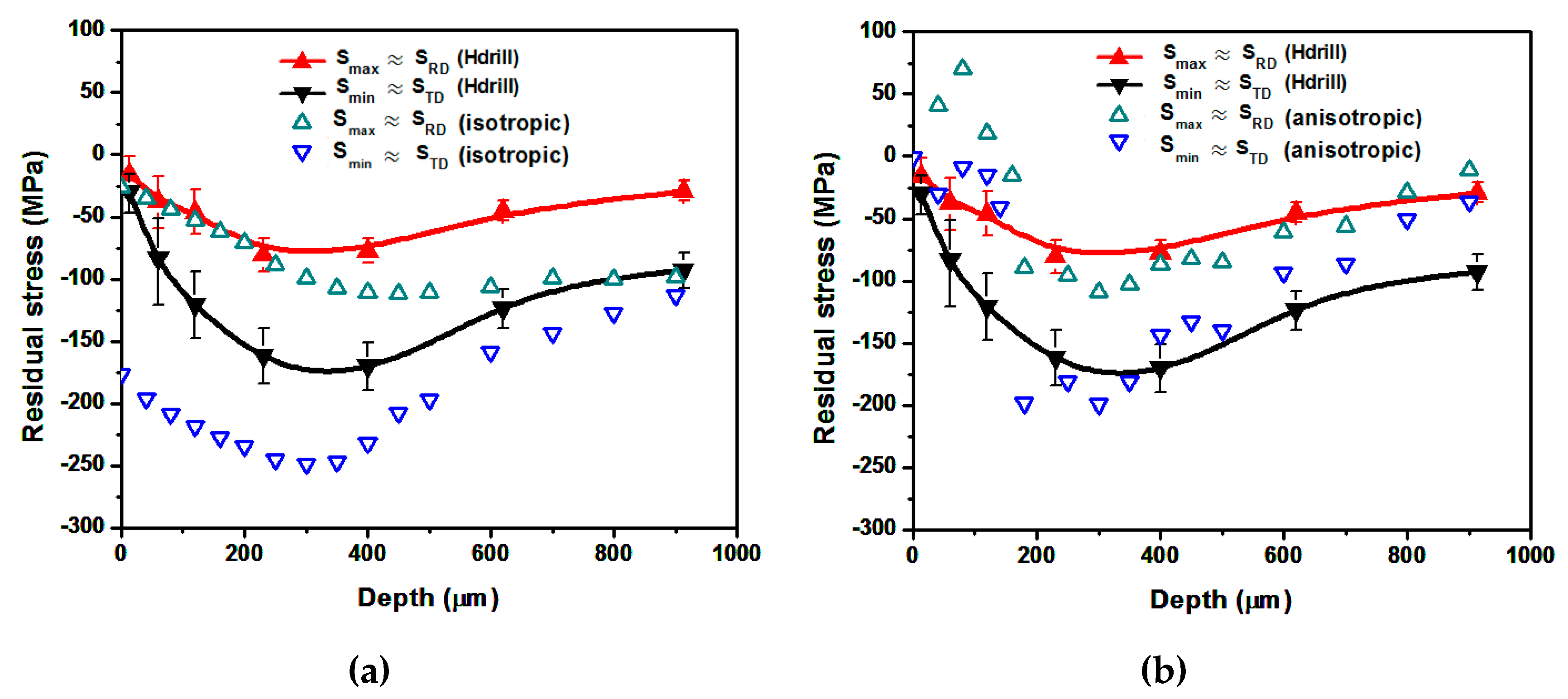
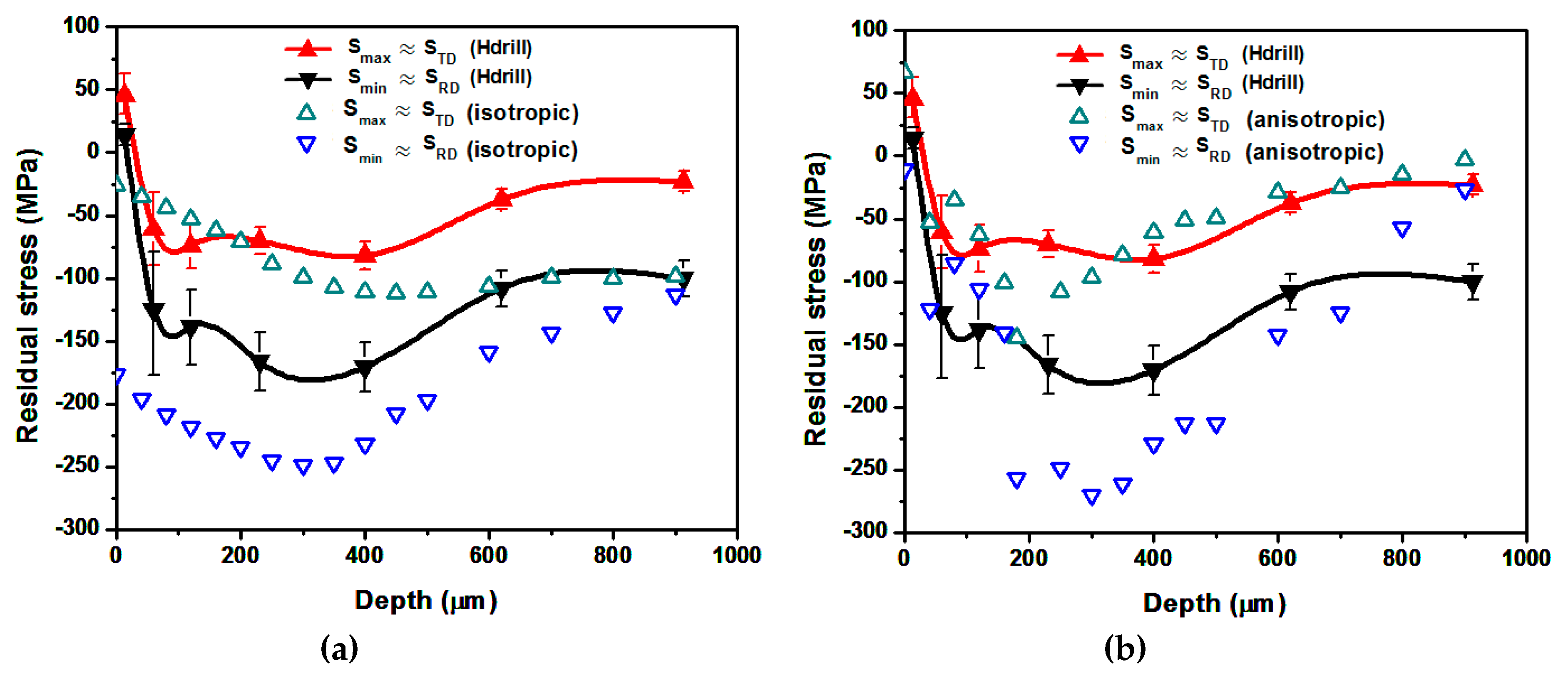
3.1. Experimental Characterization of the Residual Stresses for Different Input Parameters
3.2. Realistic Modelling Results for Extended Surface High-Coverage LSP Treatments
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Alderliesten, R.; Rans, C.; Benedictus, R. The applicability of magnesium based fibre metal laminates in aerospace structures. Compos. Sci. Technol. 2008, 68, 2983–2993. [Google Scholar] [CrossRef]
- Czerwinski, F. Controlling the ignition and flammability of magnesium for aerospace applications. Corros. Sci. 2014, 86, 1–16. [Google Scholar] [CrossRef]
- Staiger, M.P.; Pietak, A.M.; Huadmai, J.; Dias, G. Magnesium and its alloys as orthopedic biomaterials: A review. Biomaterials 2006, 27, 1728–1734. [Google Scholar] [CrossRef] [PubMed]
- Zeng, R.; Dietzel, W.; Witte, F.; Hort, N.; Blawert, C. Progress and challenge for magnesium alloys as biomaterials. Adv. Eng. Mater. 2008, 10, 3–14. [Google Scholar] [CrossRef]
- Witte, F.; Hort, N.; Vogt, C.; Cohen, S.; Kainer, K.U.; Willumeit, R.; Feyerabend, F. Degradable biomaterials based on magnesium corrosion. Curr. Opin. Solid State Mater. Sci. 2008, 12, 63–72. [Google Scholar] [CrossRef]
- Ma, R.; Zhao, Y.; Wang, Y. Grain refinement and mechanical properties improvement of AZ31 Mg alloy sheet obtained by two-stage rolling. Mater. Sci. Eng. 2017, 691, 81–87. [Google Scholar] [CrossRef]
- Pan, F.; Wang, Q.; Jiang, B.; He, J.; Chai, Y.; Xu, J. An effective approach called the composite extrusion to improve the mechanical properties of AZ31 magnesium alloy sheets. Mater. Sci. Eng. 2016, 655, 339–345. [Google Scholar] [CrossRef]
- Kim, S.H.; Bae, S.W.; Lee, S.W.; Moon, B.G.; Kim, H.S.; Kim, Y.M.; Park, S.H. Microstructural evolution and improvement in mechanical properties of extruded AZ31 alloy by combined addition of Ca and Y. Mater. Sci. Eng. 2018, 725, 309–318. [Google Scholar] [CrossRef]
- Cui, L.Y.; Gao, S.D.; Li, P.P.; Zeng, R.C.; Zhang, F.; Li, S.Q.; Han, E.H. Corrosion resistance of a self-healing micro-arc oxidation/polymethyltrimethoxysilane composite coating on magnesium alloy AZ31. Corros. Sci. 2017, 118, 84–95. [Google Scholar] [CrossRef]
- Bagherifard, S.; Hickey, D.J.; Fintová, S.; Pastorek, F.; Fernandez-Pariente, I.; Bandini, M.; Guagliano, M. Effects of nanofeatures induced by severe shot peening (SSP) on mechanical, corrosion and cytocompatibility properties of magnesium alloy AZ31. Acta Biomater. 2018, 66, 93–108. [Google Scholar] [CrossRef]
- Jian, S.Y.; Chu, Y.R.; Lin, C.S. Permanganate conversion coating on AZ31 magnesium alloys with enhanced corrosion resistance. Corros. Sci. 2015, 93, 301–309. [Google Scholar] [CrossRef]
- Mao, B.; Liao, Y.; Li, B. Gradient twinning microstructure generated by laser shock peening in an AZ31B magnesium alloy. Appl. Surf. Sci. 2018, 457, 342–351. [Google Scholar] [CrossRef]
- Ge, M.Z.; Xiang, J.Y. Effect of laser shock peening on microstructure and fatigue crack growth rate of AZ31B magnesium alloy. J. Alloys Compd. 2016, 680, 544–552. [Google Scholar] [CrossRef]
- Zhang, X.; Mao, B.; Siddaiah, A.; Menezes, P.L.; Liao, Y. Direct laser shock surface patterning of an AZ31B magnesium alloy: Microstructure evolution and friction performance. J. Mater. Process. Technol. 2020, 275, 116333. [Google Scholar] [CrossRef]
- Kanel, G.I.; Garkushin, G.V.; Savinykh, A.S.; Razorenov, S.V.; De Resseguier, T.; Proud, W.G.; Tyutin, M.R. Shock response of magnesium single crystals at normal and elevated temperatures. J. Appl. Phys. 2014, 116, 143504. [Google Scholar] [CrossRef]
- De Rességuier, T.; Hemery, S.; Lescoute, E.; Villechaise, P.; Kanel, G.I.; Razorenov, S.V. Spall fracture and twinning in laser shock-loaded single-crystal magnesium. J. Appl. Phys. 2017, 121, 165104. [Google Scholar] [CrossRef]
- Matsuzuki, M.; Horibe, S. Analysis of fatigue damage process in magnesium alloy AZ31. Mater. Sci. Eng. 2009, 504, 169–174. [Google Scholar] [CrossRef]
- Park, S.H.; Hong, S.G.; Yoon, J.; Lee, C.S. Influence of loading direction on the anisotropic fatigue properties of rolled magnesium alloy. Int. J. Fatigue 2016, 87, 210–215. [Google Scholar] [CrossRef]
- Tucker, M.T.; Horstemeyer, M.F.; Gullett, P.M.; El Kadiri, H.; Whittington, W.R. Anisotropic effects on the strain rate dependence of a wrought magnesium alloy. Scr. Mater. 2009, 60, 182–185. [Google Scholar] [CrossRef]
- Ulacia, I.; Dudamell, N.V.; Gálvez, F.; Yi, S.; Pérez-Prado, M.T.; Hurtado, I. Mechanical behavior and microstructural evolution of a Mg AZ31 sheet at dynamic strain rates. Acta Mater. 2010, 58, 2988–2998. [Google Scholar] [CrossRef]
- Jäger, A.; Lukáč, P.; Gärtnerová, V.; Bohlen, J.; Kainer, K.U. Tensile properties of hot rolled AZ31 Mg alloy sheets at elevated temperatures. J. Alloys Compd. 2004, 378, 184–187. [Google Scholar] [CrossRef]
- Chino, Y.; Kimura, K.; Mabuchi, M. Deformation characteristics at room temperature under biaxial tensile stress in textured AZ31 Mg alloy sheets. Acta Mater. 2009, 57, 1476–1485. [Google Scholar] [CrossRef]
- Koh, Y.; Kim, D.; Seok, D.Y.; Bak, J.; Kim, S.W.; Lee, Y.S.; Chung, K. Characterization of mechanical property of magnesium AZ31 alloy sheets for warm temperature forming. Int. J. Mech. Sci. 2015, 93, 204–217. [Google Scholar] [CrossRef]
- Kabirian, F.; Khan, A.S.; Gnäupel-Herlod, T. Visco-plastic modeling of mechanical responses and texture evolution in extruded AZ31 magnesium alloy for various loading conditions. Int. J. Plast. 2015, 68, 1–20. [Google Scholar] [CrossRef]
- Ballard, P.; Fournier, J.; Fabbro, R.; Frelat, J. Residual stresses induced by laser-shocks. J. Phys. IV 1991, 1, C3-487–C3-494. [Google Scholar] [CrossRef]
- Angulo, I.; Cordovilla, F.; García-Beltrán, A.; Smyth, N.S.; Langer, K.; Fitzpatrick, M.E.; Ocaña, J.L. The effect of material cyclic deformation properties on residual stress generation by laser shock processing. Int. J. Mech. Sci. 2019, 156, 370–381. [Google Scholar] [CrossRef]
- Engebretsen, C.C.; Palazotto, A.; Langer, K. Strain Rate Dependent FEM of Laser Shock Induced Residual Stress. In Challenges in Mechanics of Time-Dependent Materials, Volume 2, Proceedings of the 2018 Annual Conference on Experimental and Applied Mechanics, Greenville, SC, USA, 4–7 June 2018; Springer International Publishing: New York, NY, USA, 2019; pp. 109–114. [Google Scholar]
- Langer, K.; Olson, S.; Brockman, R.; Braisted, W.; Spradlin, T.; Fitzpatrick, M.E. High strain-rate material model validation for laser peening simulation. J. Eng. 2015, 13, 150–157. [Google Scholar] [CrossRef]
- Hill, R. A theory of the yielding and plastic flow of anisotropic metals. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1948, 193, 281–297. [Google Scholar] [CrossRef]
- Park, S.H.; Hong, S.G.; Bang, W.; Lee, C.S. Effect of anisotropy on the low-cycle fatigue behavior of rolled AZ31 magnesium alloy. Mater. Sci. Eng. 2010, 527, 417–423. [Google Scholar] [CrossRef]
- Yang, F.; Duan, Q.Q.; Yang, Y.S.; Wu, S.D.; Li, S.X.; Zhang, Z.F. Fatigue properties of rolled magnesium alloy (AZ31) sheet: Influence of specimen orientation. Int. J. Fatigue 2011, 33, 672–682. [Google Scholar] [CrossRef]
- Peyre, P.; Chaieb, I.; Braham, C. FEM calculation of residual stresses induced by laser shock processing in stainless steels. Mater. Sci. Eng. 2007, 15, 205. [Google Scholar] [CrossRef]
- Voce, E. The relationship between stress and strain for homogeneous deformation. J. Inst. Met. 1948, 74, 537–562. [Google Scholar]
- Amarchinta, H.; Granhi, R.; Clauer, A.; Langer, K.; Stargel, D. Simulation of residual stress induced by a laser peening process through inverse optimization of material models. J. Mater. Process. Technol. 2010, 210, 1997–2006. [Google Scholar] [CrossRef]
- Bao, Y.; Wierzbicki, T. On fracture locus in the equivalent strain and stress triaxiality space. Int. J. Mech. Sci. 2004, 46, 81–98. [Google Scholar] [CrossRef]
- Basu, S.; Dogan, E.; Kondori, B.; Karaman, I.; Benzerga, A.A. Towards designing anisotropy for ductility enhancement: A theory-driven investigation in Mg-alloys. Acta Mater. 2017, 131, 349–362. [Google Scholar] [CrossRef]
- Fabbro, R.; Fournier, J.; Ballard, P.; Devaux, D.; Virmont, J. Physical study of laser-produced plasma in confined geometry. J. Appl. Phys. 1990, 68, 775–784. [Google Scholar] [CrossRef]
- MacFarlane, J.J.; Golovkin, I.E.; Woodruff, P.R. Helios-cr–a 1-d radiation-magnetohydrodynamics code with inline atomic kinetics modeling. J. Quant. Spectrosc. Radiat. Transf. 2006, 99, 381–397. [Google Scholar] [CrossRef]
- Morales, M.; Porro, J.A.; Blasco, M.; Molpeceres, C.; Ocana, J.L. Numerical simulation of plasma dynamics in laser shock processing experiments. Appl. Surf. Sci. 2009, 255, 5181–5185. [Google Scholar] [CrossRef]
- Morales, M.; Porro, J.A.; García-Ballesteros, J.J.; Molpeceres, C.; Ocaña, J.L. Effect of plasma confinement on laser shock microforming of thin metal sheets. Appl. Surf. Sci. 2011, 257, 5408–5412. [Google Scholar] [CrossRef]
- Wu, B.; Shin, Y.C. A self-closed thermal model for laser shock peening under the water confinement regime configuration and comparisons to experiments. J. Appl. Phys. 2005, 97, 113517. [Google Scholar] [CrossRef]
- Wu, B.; Shin, Y.C. From incident laser pulse to residual stress: A complete and self-closed model for laser shock peening. J. Manuf. Sci. Eng. 2007, 29, 117–125. [Google Scholar] [CrossRef]
- American Society for Testing and Materials. Standard Test Method for Determining Residual Stresses by the Hole-Drilling Strain-Gage Method; ASTM E837-13a; ASTM International: West Conshohocken, PA, USA, 2013. [Google Scholar] [CrossRef]
- Ocaña, J.L.; Morales, M.; Porro, J.A.; Blasco, M.; Molpeceres, C.; Iordachescu, D.; Rubio-González, C. Induction of engineered residual stresses fields and associate surface properties modification by short pulse laser shock processing. In Materials Science Forum, Proceedings of the International Conference on Processing & Manufacturing of Advanced Materials, Berlin, Germany, 25–29 August 2009; Trans Tech Publications: Zurich, Switzerland, 2010; Volume 638, pp. 2446–2451. [Google Scholar]
- Ocaña, J.L.; Correa, C.; García-Beltrán, A.; Porro, J.A.; Díaz, M.; Ruiz-de-Lara, L.; Peral, D. Laser Shock Processing of thin Al2024-T351 plates for induction of through-thickness compressive residual stresses fields. J. Mater. Process. Technol. 2015, 223, 8–15. [Google Scholar] [CrossRef]
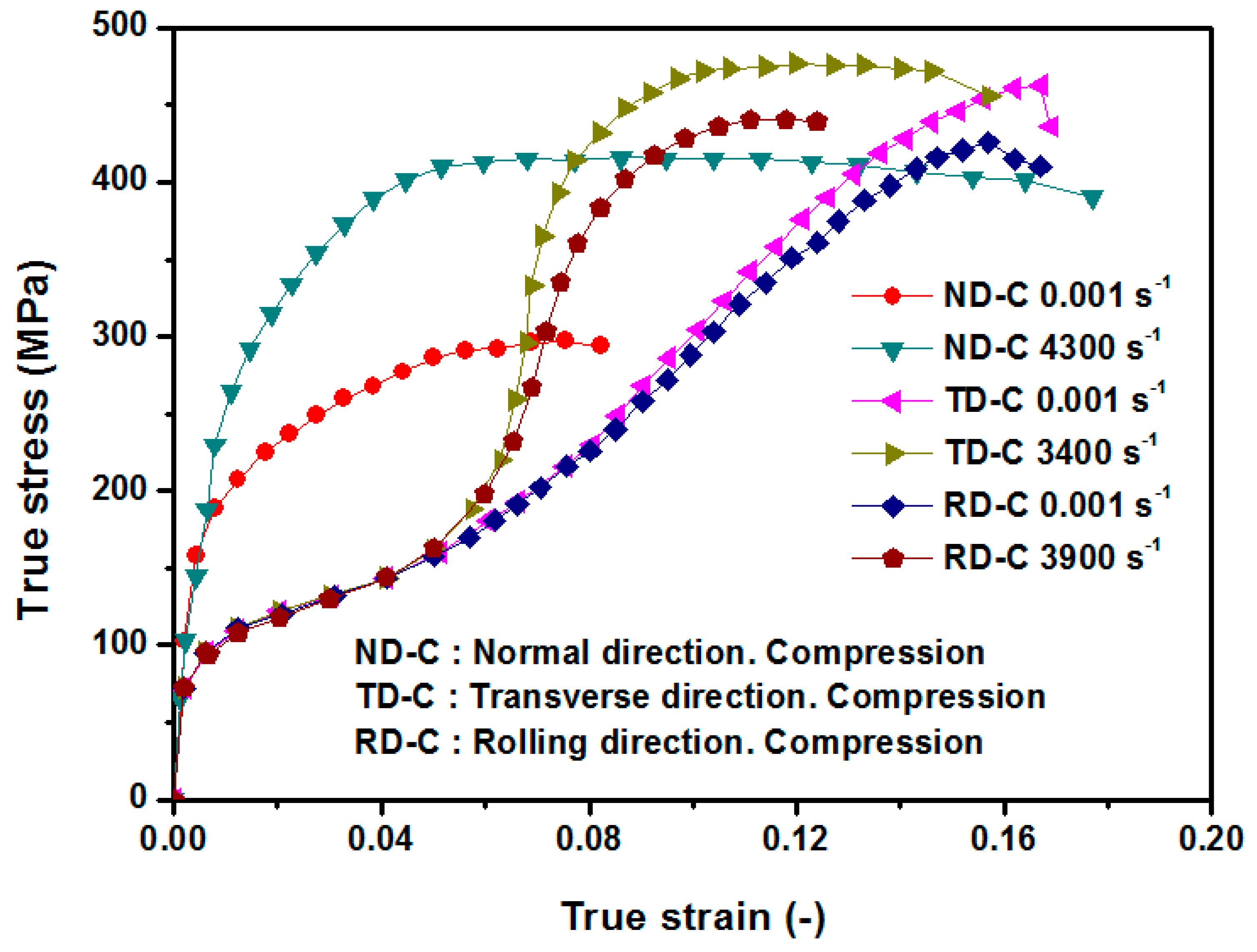
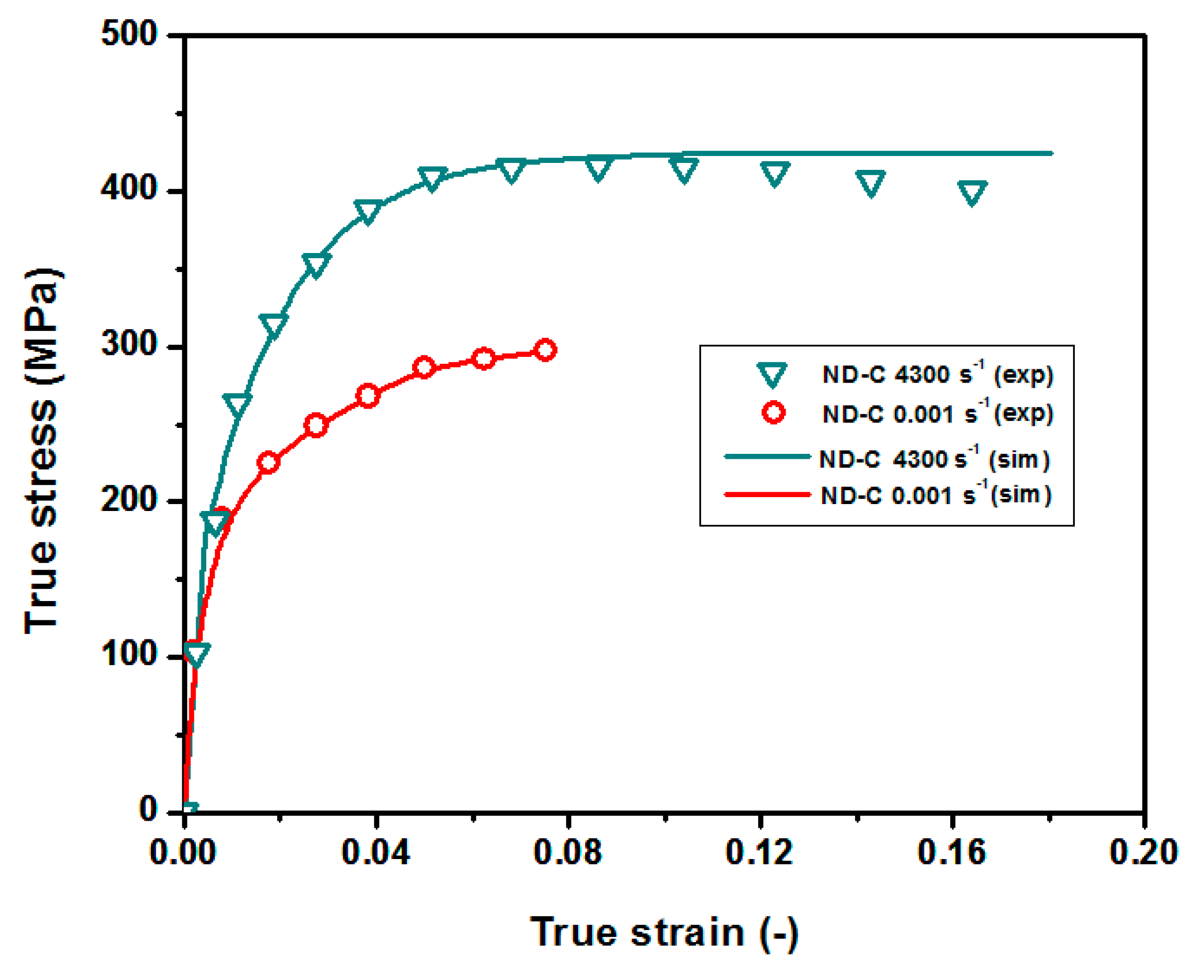
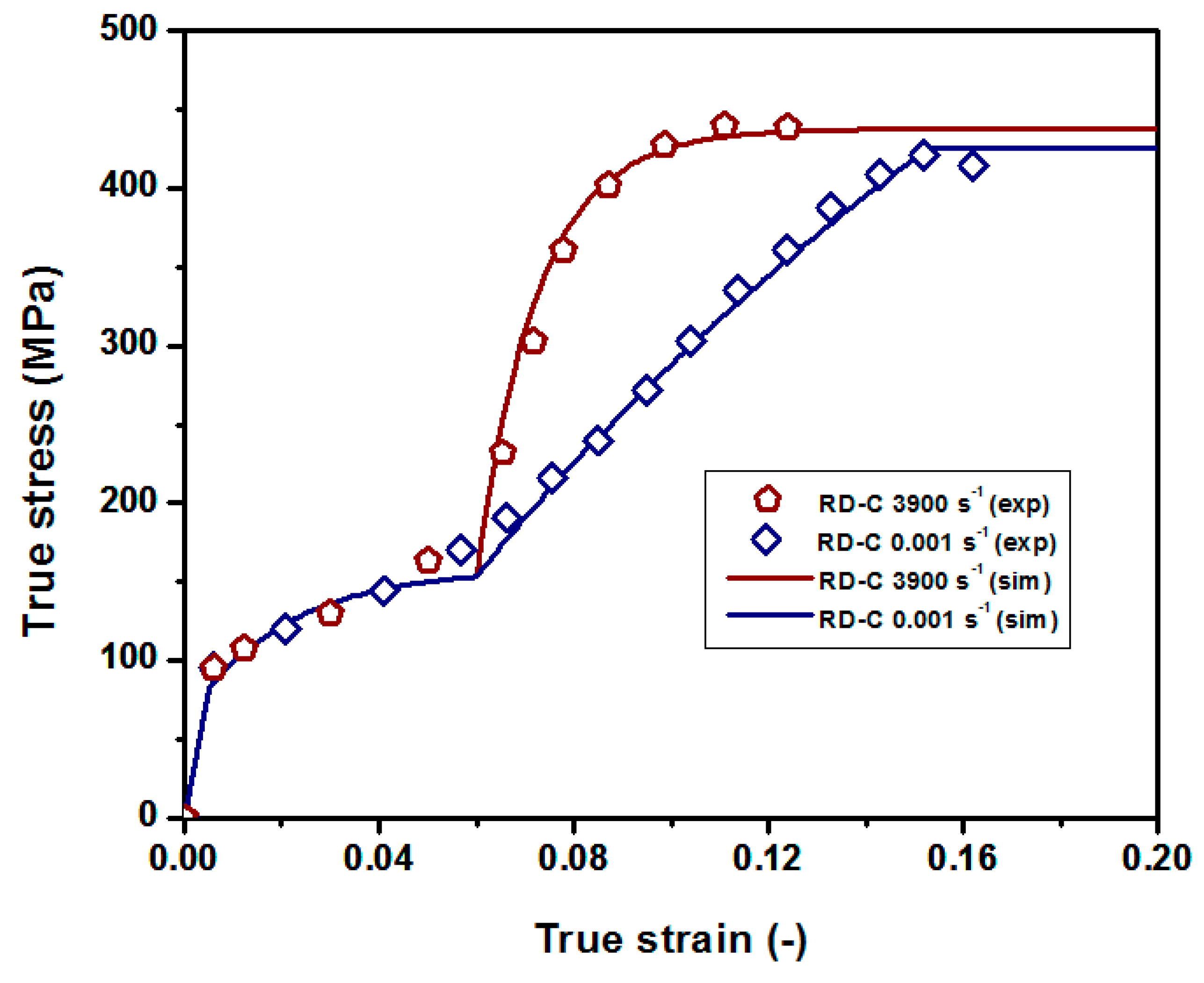
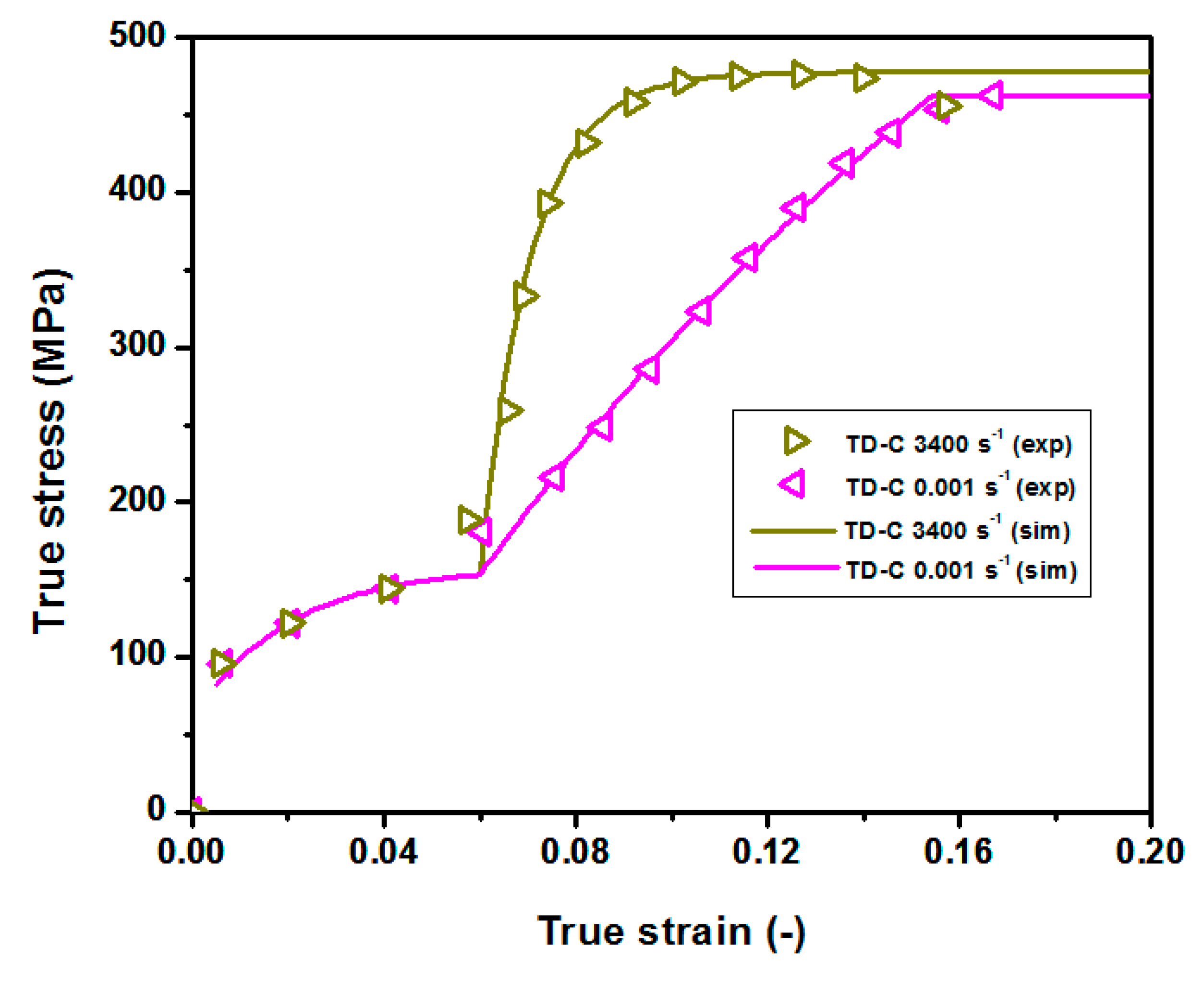
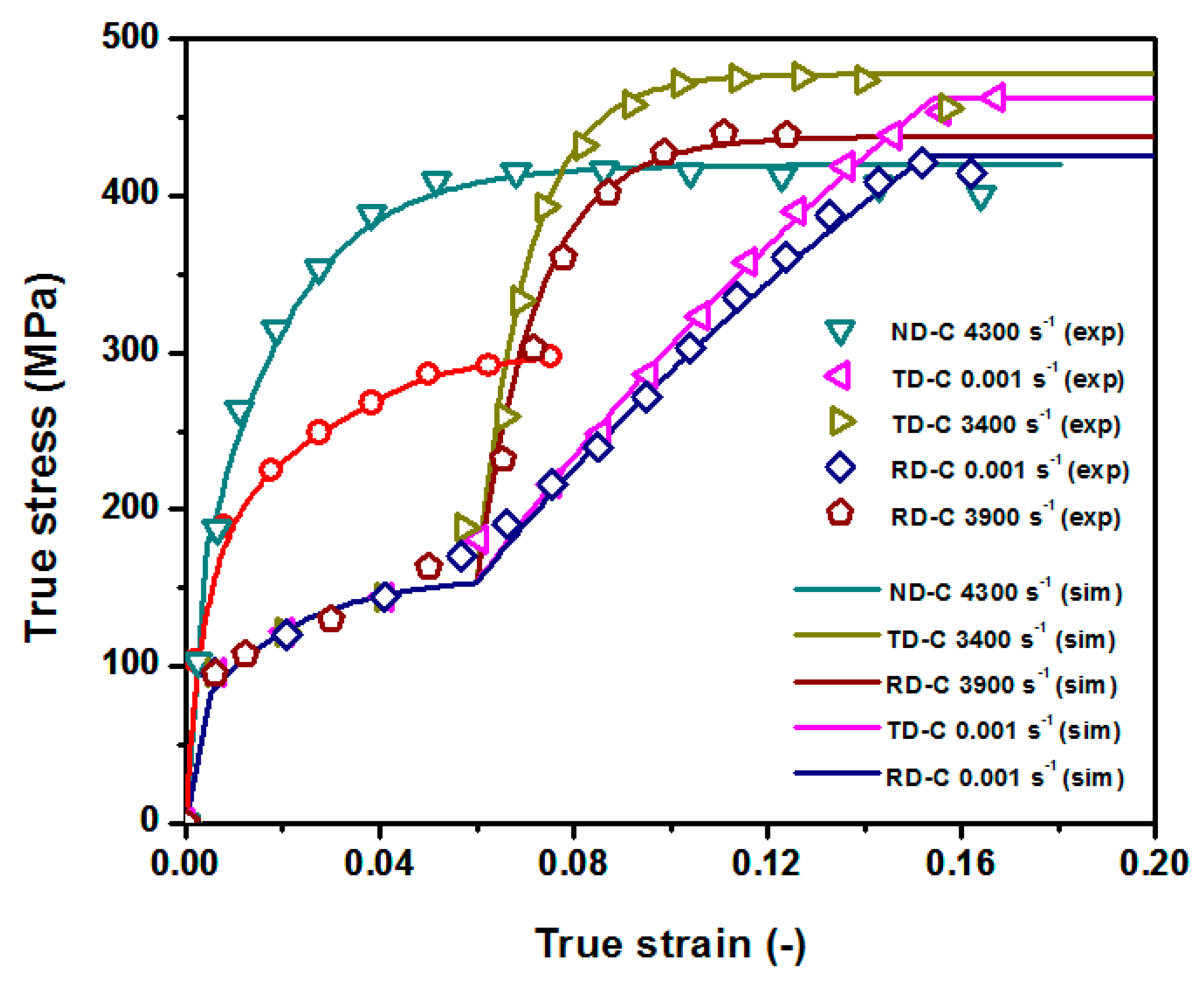

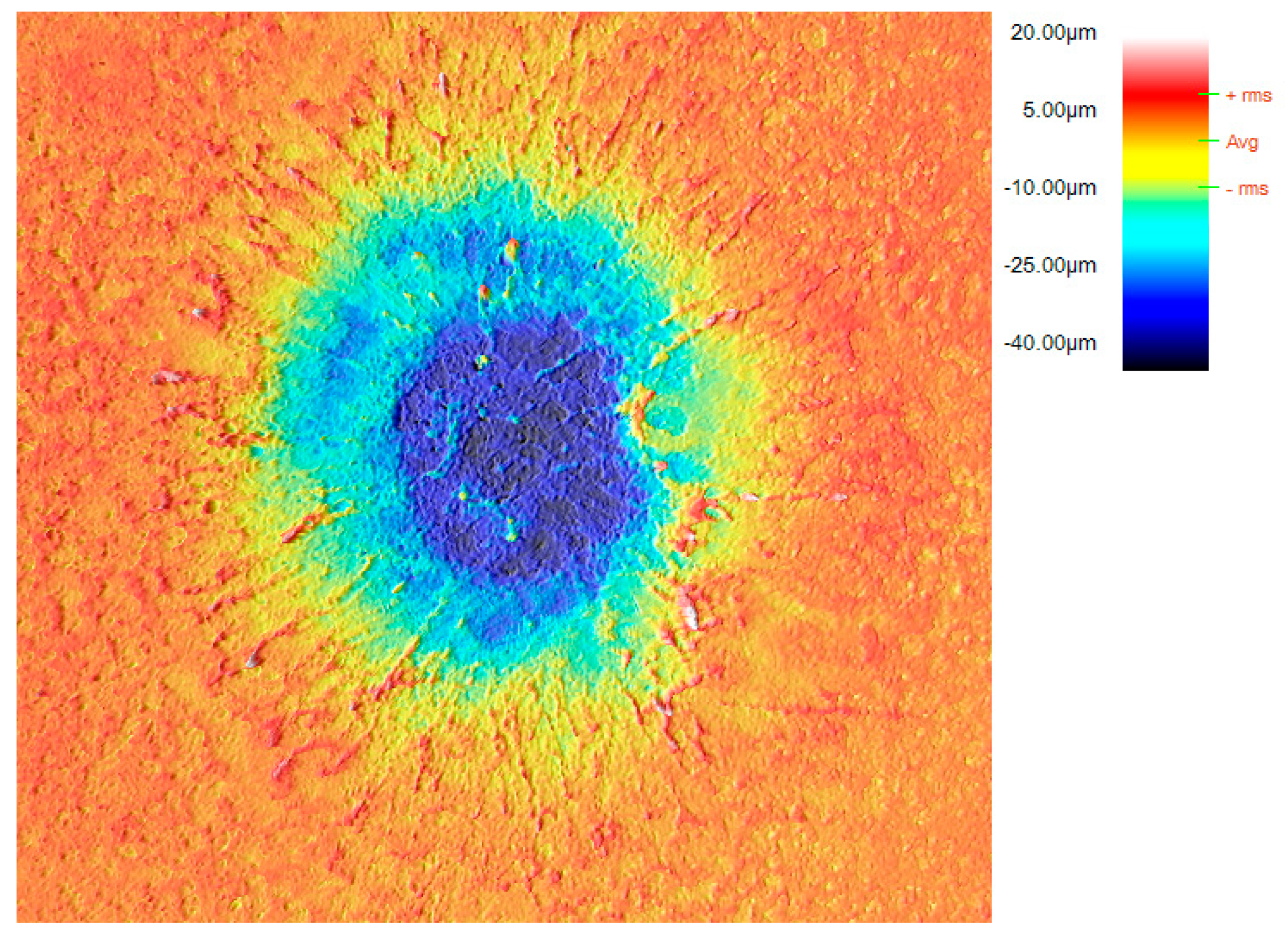
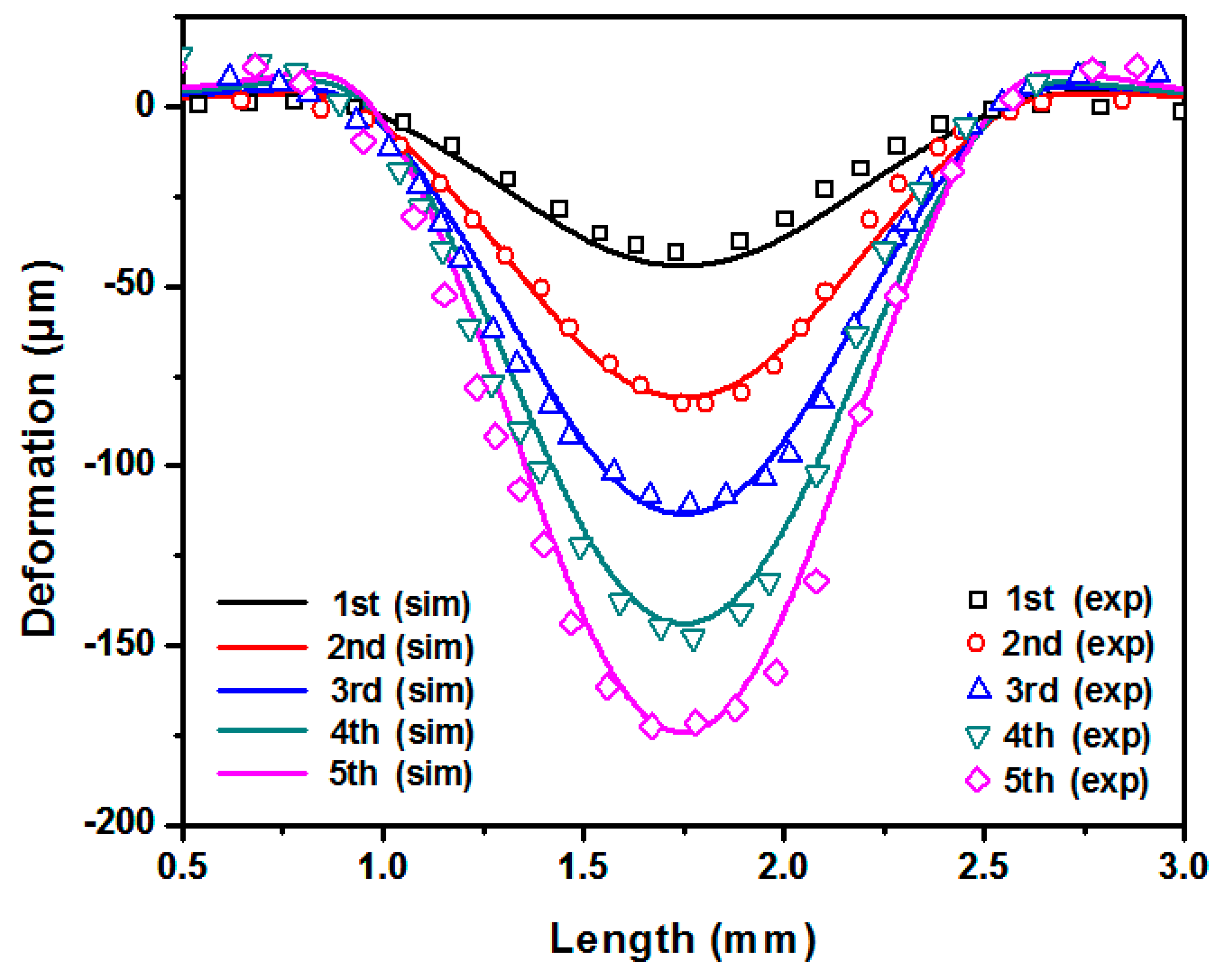
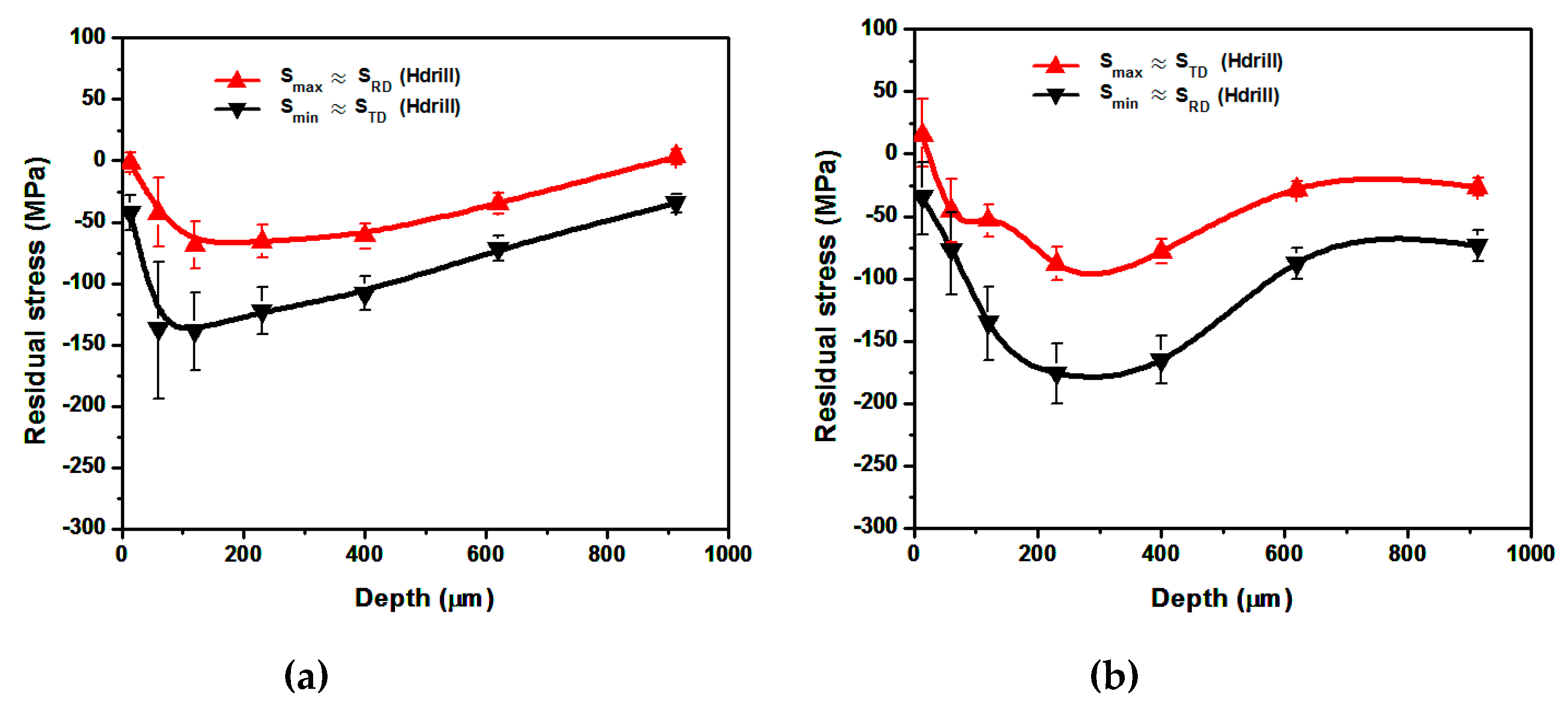
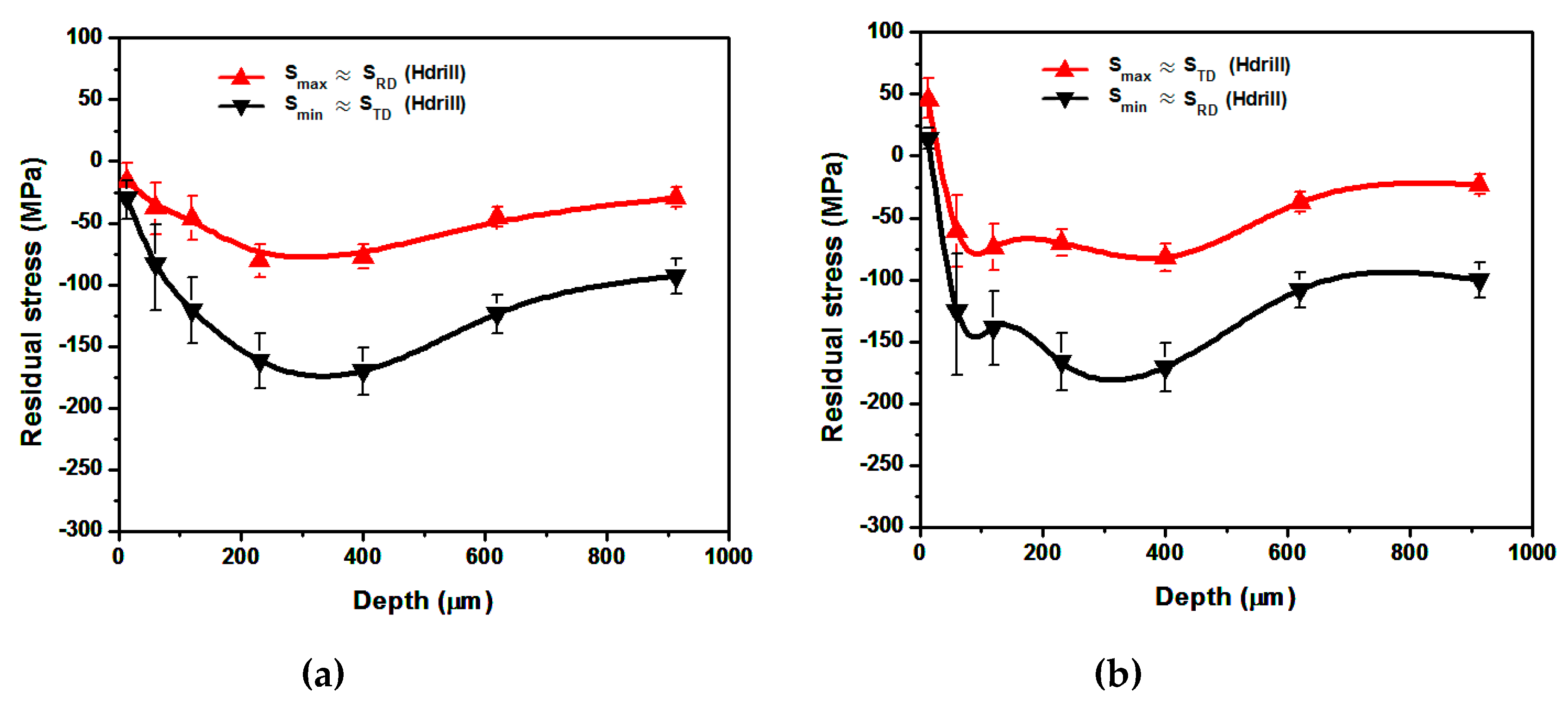
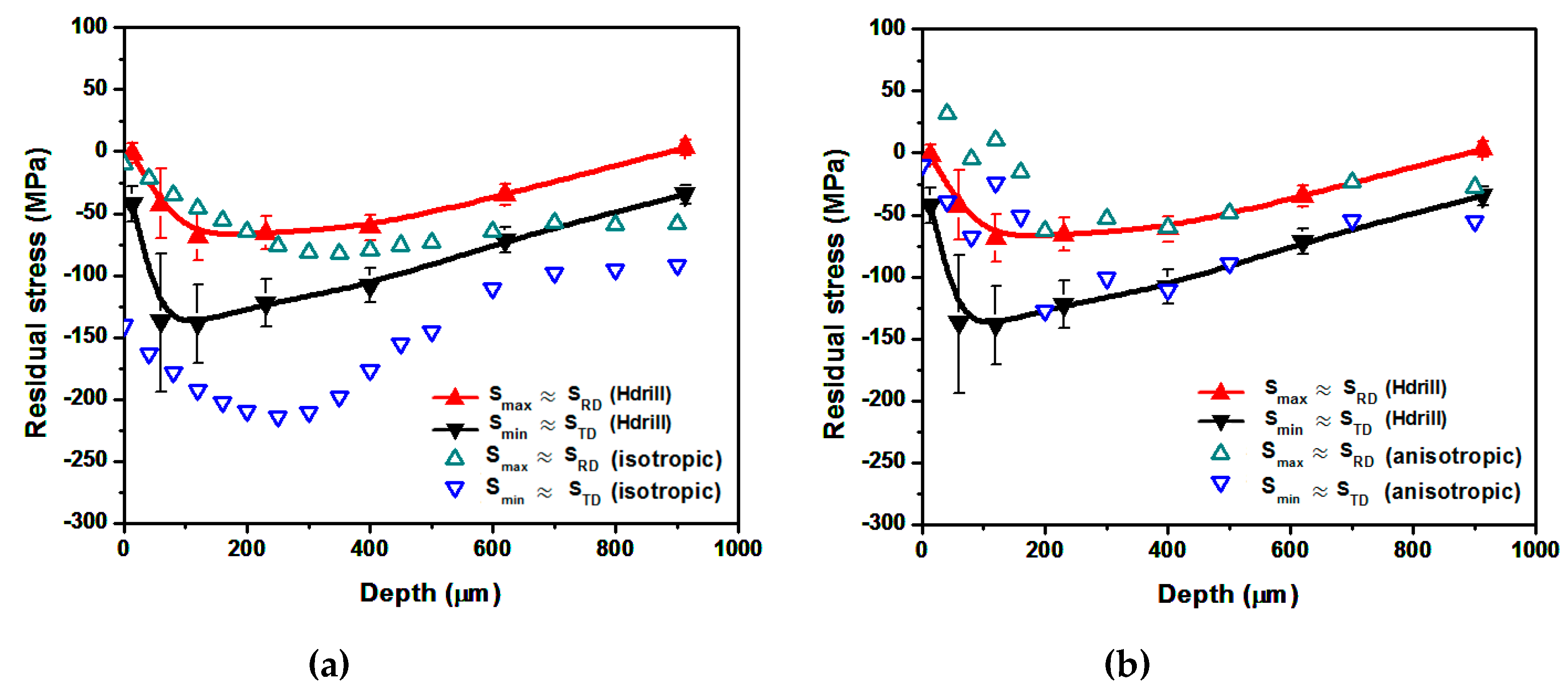
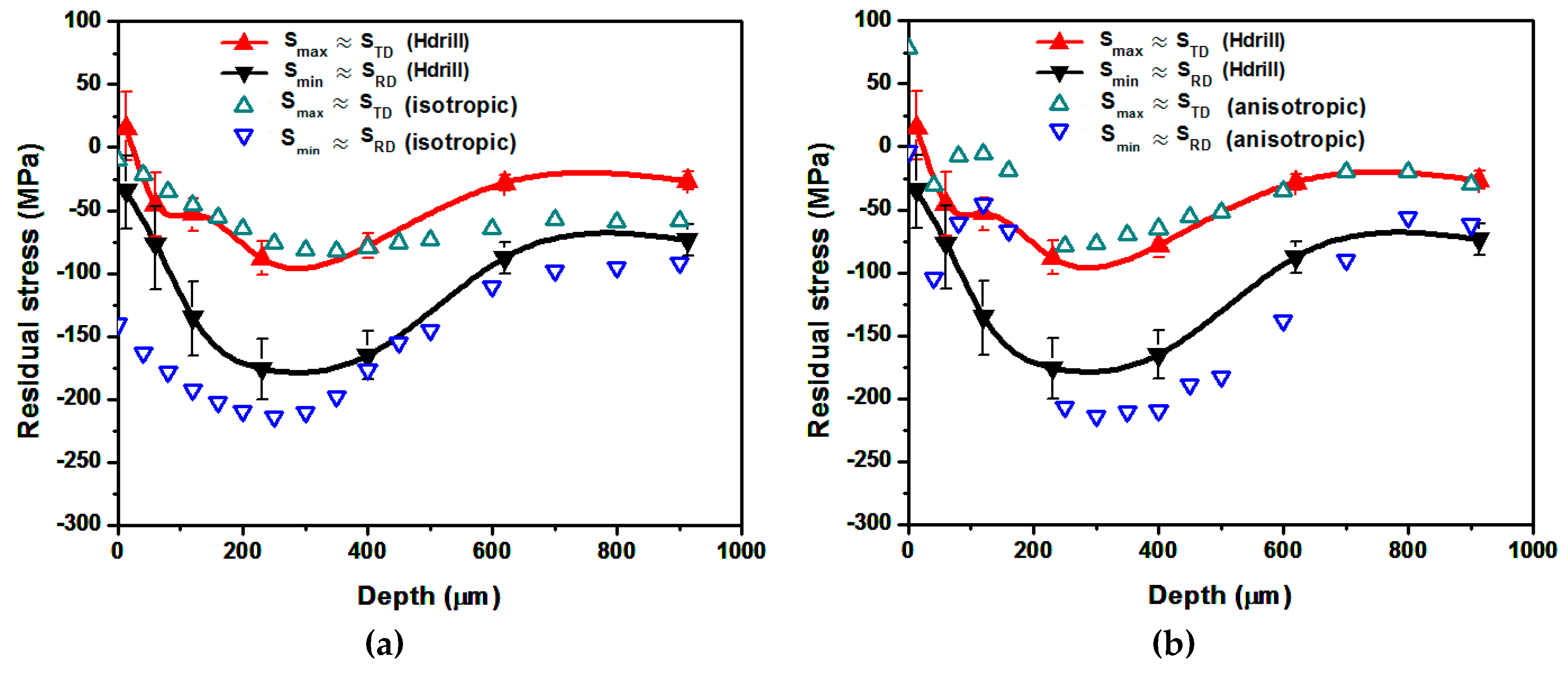
| Parameter | Value |
|---|---|
| (MPa) | 178 |
| (MPa) | 125 |
| (–) | 19 |
| (–) | 18 |
| (s−1) | 0.001 |
| (s−1) | 4300 |
| Parameter | Value |
|---|---|
| (MPa) | 60 |
| (MPa) | 426 |
| (MPa) | 463 |
| (MPa) | 98 |
| (MPa) | 720 |
| (MPa) | 810 |
| (MPa) | 280 |
| (MPa) | 320 |
| (–) | 50 |
| (–) | 5 |
| (–) | 80 |
| (–) | 95 |
| (–) | 0.059 |
| Parameter | Value |
|---|---|
| (–) | 1.9 |
| (mm) | 0.8 |
| (–) | 1.3 |
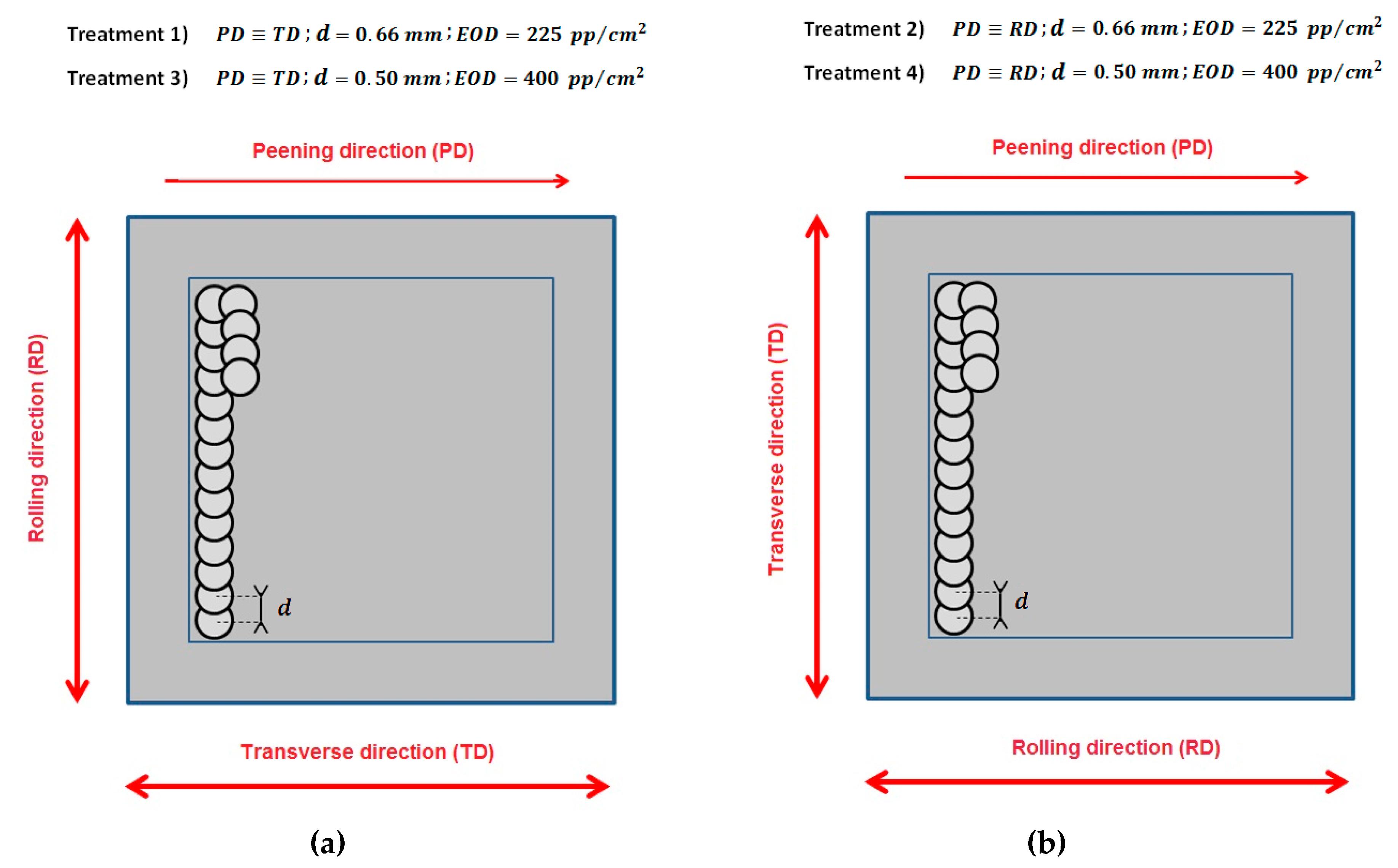
| Feature | (1) | (2) | (3) | (4) |
|---|---|---|---|---|
| (pp/cm2) | 225 | 225 | 400 | 400 |
| Peening direction (–) | TD | RD | TD | RD |
| (µm) | 250 | 250 | 299 | 299 |
| (µm) | 197 | 297 | 297 | 300 |
| (µm) | 100 | 300 | 334 | 315 |
| (MPa) | −215 | −215 | −249 | −249 |
| (MPa) | −130 | −213 | −199 | −268 |
| (MPa) | −136 | −178 | −174 | −181 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Angulo, I.; Cordovilla, F.; García-Beltrán, Á.; Porro, J.A.; Díaz, M.; Ocaña, J.L. Integrated Numerical-Experimental Assessment of the Effect of the AZ31B Anisotropic Behaviour in Extended-Surface Treatments by Laser Shock Processing. Metals 2020, 10, 195. https://doi.org/10.3390/met10020195
Angulo I, Cordovilla F, García-Beltrán Á, Porro JA, Díaz M, Ocaña JL. Integrated Numerical-Experimental Assessment of the Effect of the AZ31B Anisotropic Behaviour in Extended-Surface Treatments by Laser Shock Processing. Metals. 2020; 10(2):195. https://doi.org/10.3390/met10020195
Chicago/Turabian StyleAngulo, Ignacio, Francisco Cordovilla, Ángel García-Beltrán, Juan A. Porro, Marcos Díaz, and José L. Ocaña. 2020. "Integrated Numerical-Experimental Assessment of the Effect of the AZ31B Anisotropic Behaviour in Extended-Surface Treatments by Laser Shock Processing" Metals 10, no. 2: 195. https://doi.org/10.3390/met10020195
APA StyleAngulo, I., Cordovilla, F., García-Beltrán, Á., Porro, J. A., Díaz, M., & Ocaña, J. L. (2020). Integrated Numerical-Experimental Assessment of the Effect of the AZ31B Anisotropic Behaviour in Extended-Surface Treatments by Laser Shock Processing. Metals, 10(2), 195. https://doi.org/10.3390/met10020195







