Using the Isometric Mid-Thigh Pull to Predict Three-Repetition Maximum Squat Values in Female Athletes
Abstract
1. Introduction
2. Materials and Methods
2.1. Experimental Approach to the Problem
2.2. Subjects
2.3. Procedures
2.3.1. Part A and Part B
Part A
Part B
2.3.2. Anthropometry
2.3.3. Isometric Mid-Thigh Pull
2.3.4. 3RM Squat
2.4. Statistical Analysis
2.4.1. Part A
2.4.2. Part B
3. Results
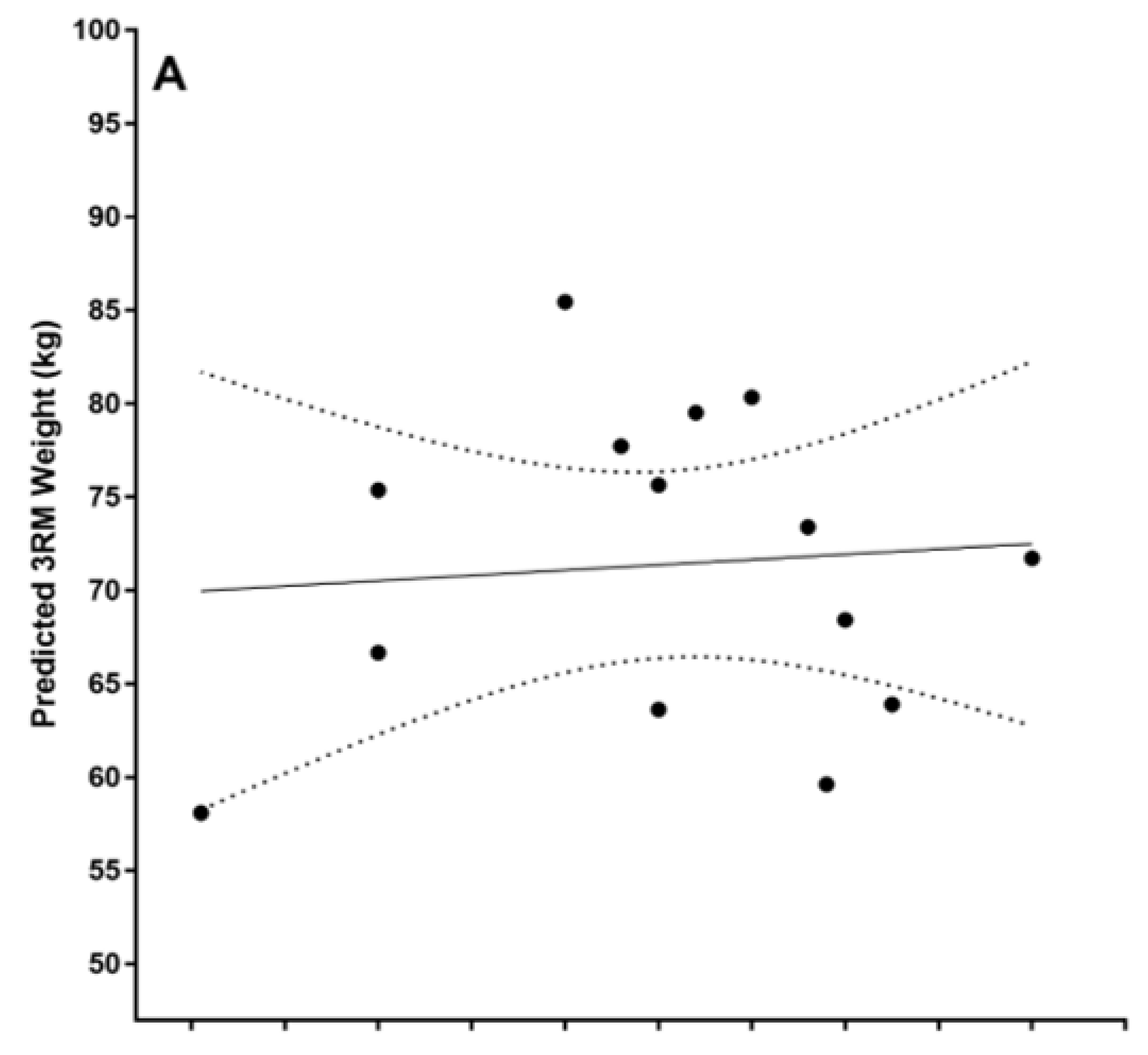
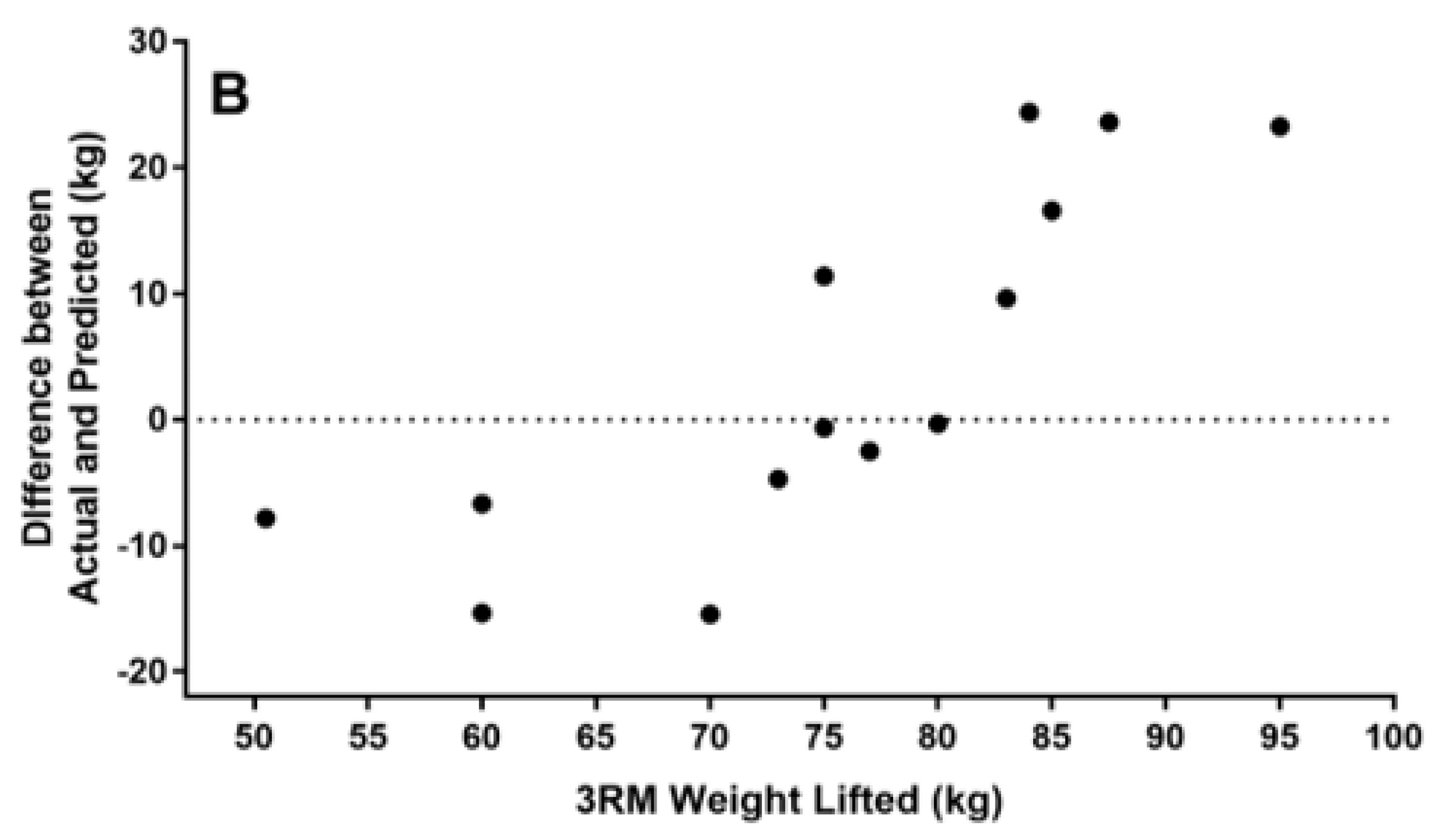
3.1. Part A
3.2. Part B
4. Discussion
5. Practical Application
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Santos, A.C.; Turner, T.J.; Bycura, D.K. Current and Future Trends in Strength and Conditioning for Female Athletes. Int. J. Environ. Res. Public Health 2022, 19, 2687. [Google Scholar] [CrossRef]
- Seitz, L.B.; Reyes, A.; Tran, T.T.; de Villarreal, E.S.; Haff, G.G. Increases in lower-body strength transfer positively to sprint performance: A systematic review with meta-analysis. Sports Med. 2014, 44, 1693–1702. [Google Scholar] [CrossRef]
- Mata, J.D.; Oliver, J.M.; Jagim, A.R.; Jones, M.T. Sex Differences in Strength and Power Support the Use of a Mixed-Model Approach to Resistance Training Programing. Strength Cond. J. 2016, 38, 2–7. [Google Scholar] [CrossRef]
- Dohoney, P.; Chromiak, J.A.; Lemire, D.; Abadie, B.R.; Kovacs, C. Prediction of one repetition maximum (1-RM) strength from a 4-6 RM and a 7-10 RM submaximal strength test in healthy young adult males. J. Exerc. Physiol. 2002, 5, 54–59. [Google Scholar]
- Ferraresi, C.; Baldissera, V.; Perez, S.E.; Junior, E.M.; Bagnato, V.S.; Parizotto, N.A. One-repetition maximum test and isokinetic leg extension and flexion: Correlations and predicted values. Isokinet. Exerc. Sci. 2013, 21, 69–76. [Google Scholar] [CrossRef]
- Newton, R.U.; Dugan, E. Application of Strength Diagnosis. Strength Cond. J. 2002, 24, 50–59. [Google Scholar] [CrossRef]
- Malek, M.H.; Berger, D.E.; Housh, T.J.; Coburn, J.W.; Beck, T.W. Validity of VO2max equations for aerobically trained males and females. Med. Sci. Sports Exerc. 2004, 36, 1427–1432. [Google Scholar] [CrossRef] [PubMed]
- Aagaard, P.; Simonsen, E.B.; Andersen, J.L.; Magnusson, P.; Dyhre-Poulsen, P. Increased rate of force development and neural drive of human skeletal muscle following resistance training. J. Appl. Physiol. 2002, 93, 1318–1326. [Google Scholar] [CrossRef]
- Stauffer, K.A. The Comparison of the Max Jones Quadrathlon with the Vertical Jump and Wingate Cycle Tests as a Method to Assess Anaerobic Power in Female Division I College Basketball Players. Ph.D. Thesis, University of Pittsburgh, Pittsburgh, PA, USA, 2005. [Google Scholar]
- Hunter, S.K.; Angadi, S.S.; Bhargava, A.; Harper, J.; Hirschberg, A.L.; Levine, B.D.; Moreau, K.L.; Nokoff, N.J.; Stachenfeld, N.S.; Bermon, S. The Biological Basis of Sex Differences in Athletic Performance: Consensus Statement for the American College of Sports Medicine. Med. Sci. Sports Exerc. 2023, 55, 2328–2360. [Google Scholar] [CrossRef] [PubMed]
- Ebben, W.P.; Jensen, R.L. Strength training for women: Debunking myths that block opportunity. Physician Sportsmed. 1998, 26, 86–97. [Google Scholar] [CrossRef]
- Comfort, P.; McMahon, J.J.; Lake, J.P.; Ripley, N.J.; Triplett, N.T.; Haff, G.G. Relative strength explains the differences in multi-joint rapid force production between sexes. PLoS ONE 2024, 19, e0296877. [Google Scholar] [CrossRef] [PubMed]
- Judd, H.L.; Yen, S.S. Serum androstenedione and testosterone levels during the menstrual cycle. J. Clin. Endocrinol. Metab. 1973, 36, 475–481. [Google Scholar] [CrossRef]
- Juneja, H.; Verma, S.; Khanna, G. Isometric strength and its relationship to dynamic performance: A systematic review. J. Exerc. Sci. Physiother. 2012, 6, 60. [Google Scholar]
- Mcguigan, M.R.; Newton, M.J.; Winchester, J.B.; Nelson, A.G. Relationship between isometric and dynamic strength in recreationally trained men. J. Strength Cond. Res. 2010, 24, 2570–2573. [Google Scholar] [CrossRef] [PubMed]
- Beckham, G.; Mizuguchi, S.; Carter, C.; Sato, K.; Ramsey, M.; Lamont, H.; Hornsby, G.; Haff, G.; Stone, M. Relationships of isometric mid-thigh pull variables to weightlifting performance. J. Sports Med. Phys. Fit. 2013, 53, 573–581. [Google Scholar]
- Haff, G.G.; Carlock, J.M.; Hartman, M.J.; Kilgore, J.L.; Kawamori, N.; Jackson, J.R.; Morris, R.T.; Sands, W.A.; Stone, M.H. Force-Time Curve Characteristics of Dymanic and Isometric Muscle Contractions of Elite Wome Olympic Weightlifters. J. Strength Cond. Res. 2005, 19, 741–748. [Google Scholar]
- Sha, Z.; Bailey, C.A.; Mclnnis, T.C.; Sato, K.; Stone, M.H. Using Kinestic Isometric Mid-thigh Pull Variable to Predict DI Male Sprinters’ 60 m Performance. In Proceedings of the ISBS-Conference Proceedings Archive, Johnson City, TN, USA, 12–16 July 2014. [Google Scholar]
- Faulkner, J.A.; Brooks, S.V.; Opiteck, J.A. Injury to skeletal muscle fibers during contractions: Conditions of occurrence and prevention. Phys. Ther. 1993, 73, 911–921. [Google Scholar] [CrossRef]
- McKay, A.K.A.; Stellingwerff, T.; Smith, E.S.; Martin, D.T.; Mujika, I.; Goosey-Tolfrey, V.L.; Sheppard, J.; Burke, L.M. Defining Training and Performance Caliber: A Participant Classification Framework. Int. J. Sports Physiol. Perform. 2022, 17, 317–331. [Google Scholar] [CrossRef]
- Willoughby, D.S. The Effects of Mesocycle-Length Weight Training Programs Involving Periodization and Partially Equated Volumes on Upper and Lower Body Strength. J. Strength Cond. Res. 1993, 7, 2–8. [Google Scholar]
- Saiyed, M.Z.; Pais, V.; Shaikh, A.; Shemjaz, A.M.; Pais, S. Relationship of Limb Girth, Segmental Limb Length, Hamstring Flexibility with Vertical Jump in Male Sports Players. Int. J. Curr. Res. Rev. 2015, 7, 72. [Google Scholar]
- Sheppard, J.M.; Chapman, D.; Taylor, K.-L. An evaluation of a strength qualities assessment method for the lower body. J. Aust. Strength Cond. 2011, 19, 4–10. [Google Scholar]
- Sato, K.; Fortenbaugh, D.; Hydock, D.S.; Heise, G.D. Comparison of back squat kinematics between barefoot and shoe conditions. Int. J. Sports Sci. Coach. 2013, 8, 571–578. [Google Scholar] [CrossRef]
- Dos’Santos, T.; Jones, P.A.; Comfort, P.; Thomas, C. Effect of Different Onset Thresholds on Isometric Midthigh Pull Force-Time Variables. J. Strength Cond. Res. 2017, 31, 3463–3473. [Google Scholar] [CrossRef]
- Materko, W.; Santos, E.L. Prediction model of one repetition maximum (1rm) based on anthropometrical characteristics on male and female. Braz. J. Biomotricity 2013, 7, 43–52. [Google Scholar]
- Caven, E.J.G.; Bryan, T.J.E.; Dingley, A.F.; Drury, B.; Garcia-Ramos, A.; Perez-Castilla, A.; Arede, J.; Fernandes, J.F.T. Group versus Individualised Minimum Velocity Thresholds in the Prediction of Maximal Strength in Trained Female Athletes. Int. J. Environ. Res. Public Health 2020, 17, 7811. [Google Scholar] [CrossRef]
- West, D.J.; Owen, N.J.; Jones, M.R.; Bracken, R.M.; Cook, C.J.; Cunningham, D.J.; Shearer, D.A.; Finn, C.V.; Newton, R.U.; Crewther, B.T. Relationships between force–time characteristics of the isometric midthigh pull and dynamic performance in professional rugby league players. J. Strength Cond. Res. 2011, 25, 3070–3075. [Google Scholar] [CrossRef]
- Costello, J.T.; Bieuzen, F.; Bleakley, C.M. Where are all the female participants in Sports and Exercise Medicine research? Eur. J. Sport Sci. 2014, 14, 847–851. [Google Scholar] [CrossRef]
- De Witt, J.K.; English, K.L.; Crowell, J.B.; Kalogera, K.L.; Guilliams, M.E.; Nieschwitz, B.E.; Hanson, A.M.; Ploutz-Snyder, L.L. Isometric Mid-Thigh Pull Reliability and Relationship to Deadlift 1RM. J. Strength Cond. Res. 2018, 32, 528–533. [Google Scholar] [CrossRef] [PubMed]
- Beckham, G.K. The Effect of Various Body Positions on Performance of the Isometric Mid-Thigh Pull. Ph.D. Thesis, East Tennessee State University, Johnson City, TN, USA, 2015. Available online: https://dc.etsu.edu/etd/2544 (accessed on 1 July 2024).
- Kellis, E.; Arambatzi, F.; Papadopoulos, C. Effects of load on ground reaction force and lower limb kinematics during concentric squats. J. Sports Sci. 2005, 23, 1045–1055. [Google Scholar] [CrossRef]
- Comfort, P.; Jones, P.A.; McMahon, J.J.; Newton, R. Effect of knee and trunk angle on kinetic variables during the isometric midthigh pull: Test–retest reliability. Int. J. Sports Physiol. Perform. 2015, 10, 58–63. [Google Scholar] [CrossRef]
- Tan, W.Z.N.; Lum, D. Predicting 1 Repetition Maximum Squat With Peak Force Obtained From Isometric Squat at Multiple Positions. J. Strength Cond. Res. 2024, 38, 1543–1550. [Google Scholar] [CrossRef] [PubMed]
- Ritti-Dias, R.M.; Avelar, A.; Salvador, E.P.; Cyrino, E.S. Influence of previous experience on resistance training on reliability of one-repetition maximum test. J. Strength Cond. Res. 2011, 25, 1418–1422. [Google Scholar] [CrossRef] [PubMed]
- Cadore, E.; Pinto, R.S.; Brentano, M.A.; Silva, R.F.; da Silva, E.M.; Spinelli, R.; Correa, C.S.; Kruel, L.F.M. Prediction of one repetition maximum load by total and lean body mass in trained and untrained men. Med. Sport. 2012, 16, 111–117. [Google Scholar] [CrossRef]
- Hayes, L.D.; Bickerstaff, G.F.; Baker, J.S. Interactions of cortisol, testosterone, and resistance training: Influence of circadian rhythms. Chronobiol. Int. 2010, 27, 675–705. [Google Scholar] [CrossRef]
| Cohort | Age (years) Mean ± SD (Range) | Height (cm) Mean ± SD (Range) | Weight (kg) Mean ± SD (Range) | Seated Height (cm) Mean ± SD (Range) | Arm Span (cm) Mean ± SD (Range) | Biacromial Breadth (cm) Mean ± SD (Range) |
|---|---|---|---|---|---|---|
| Athletics (n = 1) | 19 | 173.4 | 59.40 | 92.1 | 176.0 | 37.9 |
| * Soccer (n = 8) | 16.8 ± 1.8 (16–21) | 165.3 ± 5.7 (155.6–174.9) | 61.6 ± 8.9 (51.2–76.8) | 88.0 ± 3.1 (84.3–93.9) | 163.6 ± 9.1 (149.0–172.0) | 35.9 ± 1.8 (33.4–38.5) |
| Volleyball (n = 5) | 21.2 ± 4.6 (18–28) | 179.2 ± 2.2 (177.5–182.1) | 74.1 ± 5.5 (68.9–81.5) | 95.9 ± 3.3 (92.5–100.2) | 181.4 ± 6.8 (176.0–193.0) | 40.9 ± 1.4 (40.1–43.4) |
| Basketball (n = 13) | 16.6 ± 1.0 (15–18) | 181.4 ± 9.1 (162.4–192.9) | 71.4 ± 7.8 (57.8–82.6) | 94.1 ± 4.0 (86.8–99.8) | 181.7 ± 10.6 (163.0–204.0) | 38.4 ± 1.4 (35.8–41.3) |
| * Netball (n = 7) | 17.0 ± 0.8 (16–18) | 178.1 ± 5.1 (169.4–183.7) | 69.6 ± 12.6 (55.1–90.6) | 93.0 ± 2.1 (91.0–96.3) | 177.4 (169.0–184.0) | 37.4 ± 1.1 (36.3–39.4) |
| All (n = 34) | 17.5 ± 2.5 (15–28) | 176.4 ± 9.2 (155.6–192.9) | 68.8 ± 9.7 (51.2–90.6) | 92.7 ± 4.2 (84.3–100.2) | 176.3 ± 11.2 (149.0–204.0) | 37.9 ± 2.1 (33.4–43.4) |
| Cohort | 3RM (kg) | Peak Force (N) | Force at 50 ms (N) | Force at 100 ms (N) | Force at 150 ms (N) | Force at 200 ms (N) | Force at 250 ms (N) |
|---|---|---|---|---|---|---|---|
| All (n = 34) | 75.5 ± 12 (51–95) | 1977 ± 220 (1616–2330) | 828 ± 207 (628–1333) | 1039 ± 285 (716–1622) | 1257 ± 253 (1088–1767) | 1479 ± 248 (1207–1900) | 1641 ± 217 (1343–1980) |
| Predictors | 3RM Squat | 3RMsquat_sqrt |
|---|---|---|
| Peak force (N) | 0.386 | 0.383 |
| Force at 50 ms (N) | −0.040 | −0.038 |
| Force at 100 ms (N) | −0.128 | −0.132 |
| Force at 150 ms (N) | −0.063 | −0.068 |
| Force at 200 ms (N) | −0.034 | −0.041 |
| Force at 250 ms (N) | 0.045 | 0.037 |
| Height (cm) | 0.294 | 0.304 |
| Weight (kg) | 0.284 | 0.291 |
| Seated height (cm) | 0.260 | 0.271 |
| Leg length (cm) | 0.271 | 0.276 |
| Arm span (cm) | 0.242 | 0.243 |
| Biacromial breadth (cm) | −0.41 | −0.32 |
| Predictor Combination | R | r2 | Adjusted r2 | SEE |
|---|---|---|---|---|
| Peak force (N) Force at 100 ms (N) | 0.508 | 0.258 | 0.210 | 0.706 |
| B | SEβ | β | |
|---|---|---|---|
| Constant | 6.102 | ||
| Peak force (N) | 0.002 | 0.001 | 0.546 |
| Force at 100 ms (N) | −0.001 | 0.000 | −0.372 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pasfield, K.; Ball, N.; Chapman, D.W. Using the Isometric Mid-Thigh Pull to Predict Three-Repetition Maximum Squat Values in Female Athletes. Sports 2024, 12, 230. https://doi.org/10.3390/sports12090230
Pasfield K, Ball N, Chapman DW. Using the Isometric Mid-Thigh Pull to Predict Three-Repetition Maximum Squat Values in Female Athletes. Sports. 2024; 12(9):230. https://doi.org/10.3390/sports12090230
Chicago/Turabian StylePasfield, Keely, Nick Ball, and Dale Wilson Chapman. 2024. "Using the Isometric Mid-Thigh Pull to Predict Three-Repetition Maximum Squat Values in Female Athletes" Sports 12, no. 9: 230. https://doi.org/10.3390/sports12090230
APA StylePasfield, K., Ball, N., & Chapman, D. W. (2024). Using the Isometric Mid-Thigh Pull to Predict Three-Repetition Maximum Squat Values in Female Athletes. Sports, 12(9), 230. https://doi.org/10.3390/sports12090230








