Geometrical Optimization of the EHL Roller Face/Rib Contact for Energy Efficiency in Tapered Roller Bearings
Abstract
1. Introduction
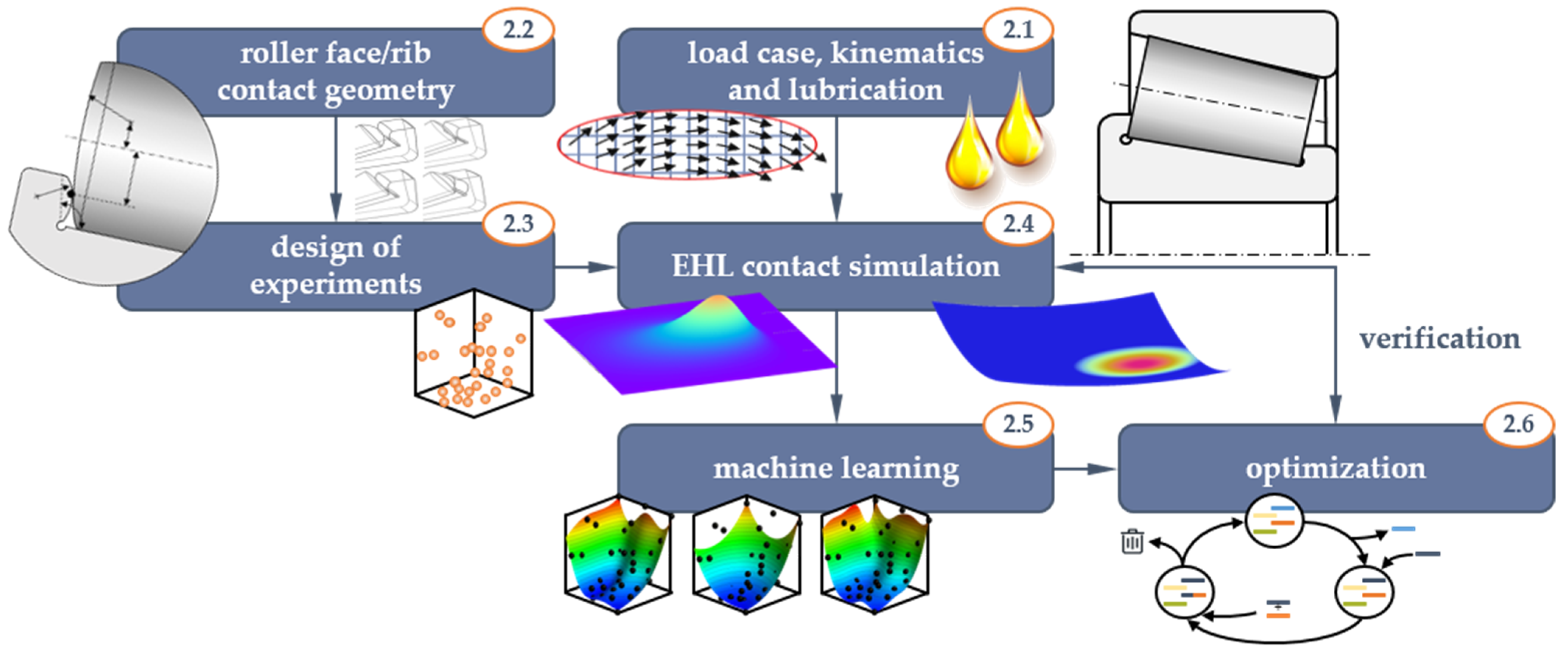
2. Materials and Methods
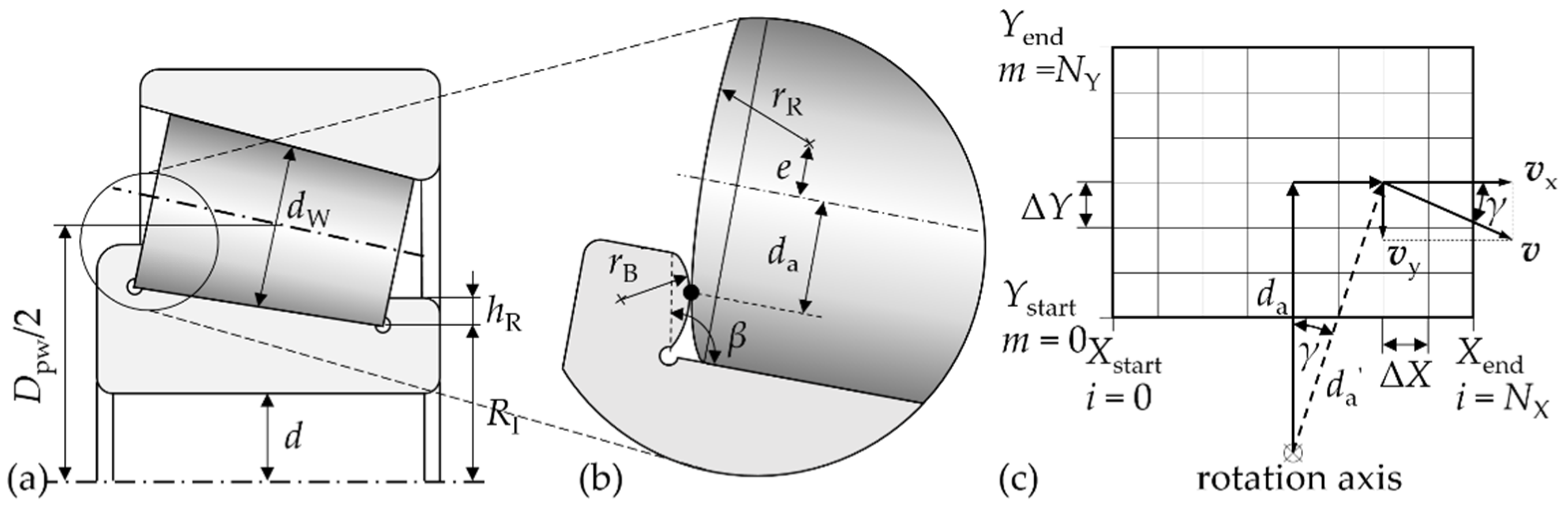
2.1. TRB Load Case, Kinematics and Lubrication
2.2. Roller Face/Rib Contact Geometry
2.3. Design of Experiments
2.4. EHL Contact Simulation
2.5. Machine Learning
2.6. Optimization
3. Results
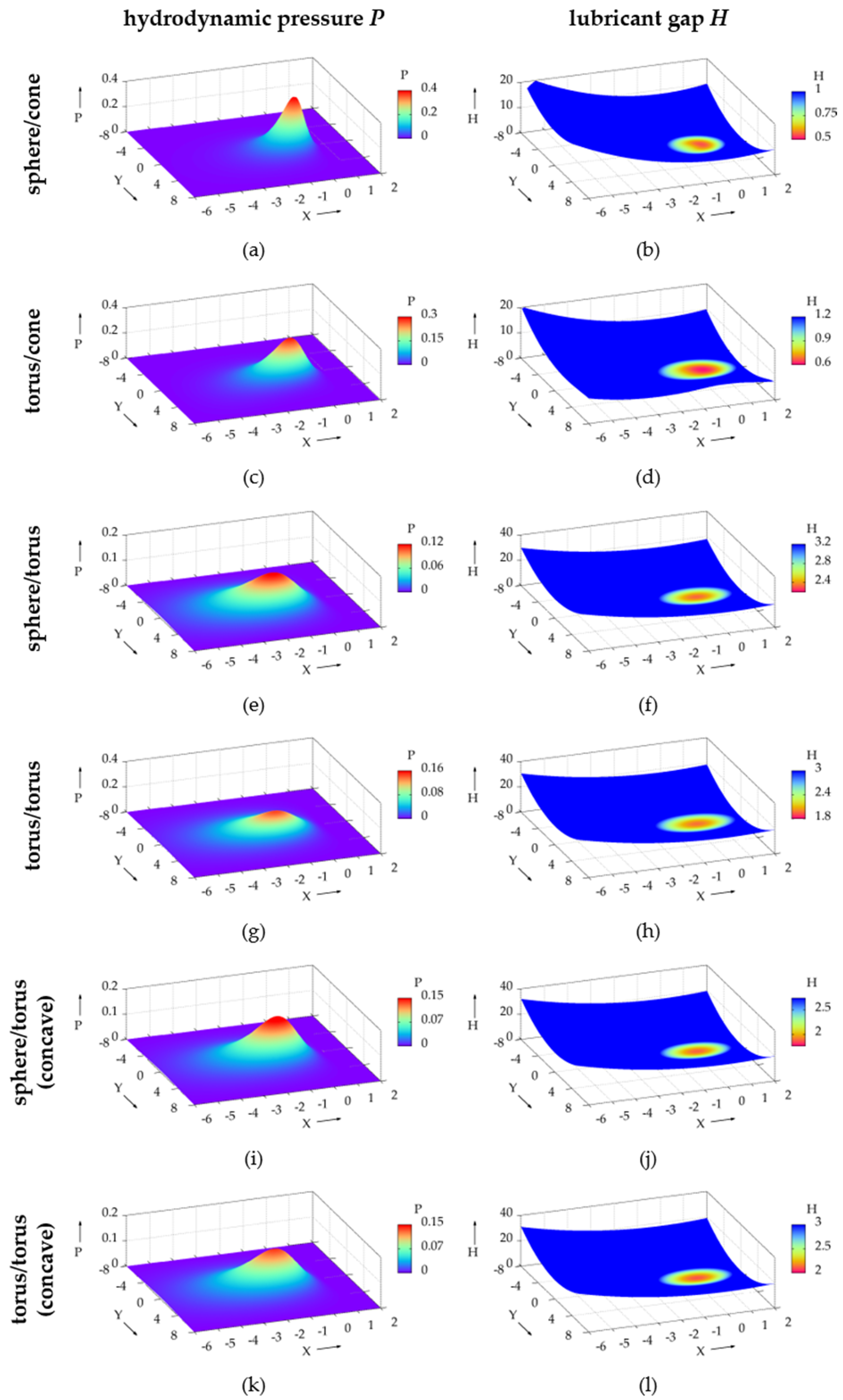
3.1. Pressure, Film Thickness and Friction
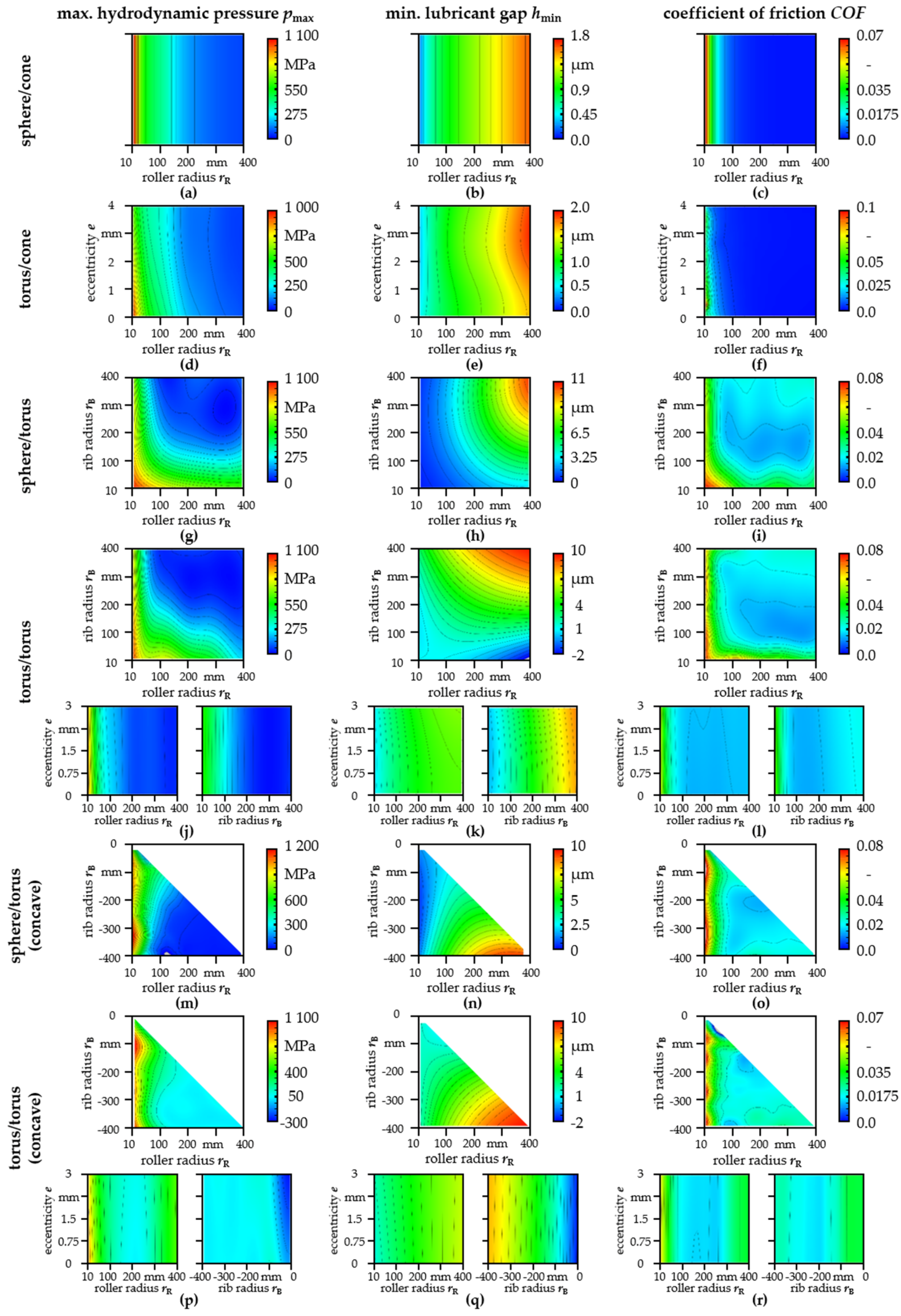
3.2. Influence of the Roller Face/Rib Geometry
3.3. Optimization of the Roller Face/Rib Geometry
3.4. Verification of Optimized Roller Face/Rib Geometry
4. Discussion
4.1. Load Carrying Capracity Versus Friction—Influences of the Geometries
4.2. Applicability and Limitations
4.3. Prediction Capability of the ML Approach
5. Conclusions
- The introduced machine learning approach featured excellent prediction quality and was able to efficiently and effectively support geometry optimization of EHL contacts with respect to the tribological behavior.
- The tribological behavior of the roller end face/rib contact is mainly determined by the basic geometric pairing and the radii; eccentricity is of subordinate role.
- There is a certain trade-off between high load carrying capacity and low frictional losses.
- If the bearing is subjected to rather low axial loads and/or higher velocities, i.e., there is moderate risk of mixed lubrication and wear, but energy losses are to be minimized, spherical or toroidal geometries on the roller end face featuring a large radius paired with a tapered (cone) rib geometry without curvature are favorable.
- If the bearing expects higher demands regarding axial load carrying capacity and/or lower speeds, spherical or toroidal roller on toroidal rib geometries with radii in the center of the analyzed parameter range are advantageous.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| a’ | Hertzian contact width in x-direction |
| b’ | Hertzian contact width in y-direction |
| c’ | Hertzian contact approach in z-direction |
| COD | coefficient of determination |
| COF | coefficient of friction |
| COI | coefficient of importance |
| COP | coefficient of prognosis |
| d | inner ring bore diameter |
| da | contact point distance |
| da’ | distance to rotation axis |
| dW | rolling element diameter |
| Dpw | pitch diameter |
| e | eccentricity |
| E | Young’s modulus |
| F | normal load |
| gi | distance function |
| G | undeformed geometry profile |
| h | lubricant gap |
| hR | rib height |
| H | normalized lubricant gap |
| i | column index |
| m | row index |
| m | slope of the cone |
| nI | inner ring speed |
| nf | number of input faktors |
| nW | rolling element speed |
| normal vector | |
| Ni | number of nodes |
| p | hydrodynamic pressure |
| pR | reference hydrodynamic pressure |
| P | normalized hydrodynamic pressure |
| position vector | |
| r | radius |
| rB | rib radius |
| rR | roller end face radius |
| R | torus radius |
| RI | inner ring raceway radius |
| projection vector | |
| t | distance to projection plane |
| ui | sliding velocity |
| v | tangential sliding velocity |
| vi | sliding velocity |
| x, y, z | cartesian coordinates |
| X, Y, Z | |
| zR | Roelands exponent |
| αp | pressure-viscosity coefficient |
| β | rib angle |
| βV | volume expansion coefficient |
| γ | angle from rotational axis |
| γL | pressure limiting shear stress |
| shear rate | |
| η | fluid viscosity |
| η0 | base viscosity |
| normalized fluid viscosity | |
| ν | Poisson’s ratio |
| ρ | fluid density |
| ρ0 | base density |
| normalized fluid density | |
| τ | shear stress |
| τ0 | equivalent shear stress |
| τL | limiting shear stress |
| Ω | contact domain |
References
- Tong, V.-C.; Hong, S.-W. Characteristics of tapered roller bearing subjected to combined radial and moment loads. Int. J. Precis. Eng. Manuf. Green Technol. 2014, 1, 323–328. [Google Scholar] [CrossRef]
- Dragoni, E. Optimal design of tapered roller bearings for maximum rating life under combined loads. Mech. Ind. 2017, 18, 112. [Google Scholar] [CrossRef]
- Lostado, R.; Escribano García, R.; Fernandez Martinez, R. Optimization of operating conditions for a double-row tapered roller bearing. Int. J. Mech. Mater. Des. 2016, 12, 353–373. [Google Scholar] [CrossRef]
- Dean, S.W.; Cowles, J.H.; Houle, C.A. Roller Profile Development for an Axially Loaded, Single Row Spherical Roller Bearing in an Oscillating Application. J. ASTM Int. 2012, 9, 103891. [Google Scholar] [CrossRef]
- Wang, Z.W.; Meng, L.Q.; Hao, W.S.; Zhang, E. Finite Element Method Analysis and Optimal Design of Roller Convexity of Tapered Roller Bearing. AMR 2010, 139–141, 1079–1083. [Google Scholar] [CrossRef]
- Lostado-Lorza, R.; Escribano-Garcia, R.; Fernandez-Martinez, R.; Illera-cueva, M.; Mac Donald, B.J. Using the finite element method and data mining techniques as an alternative method to determine the maximum load capacity in tapered roller bearings. J. Appl. Log. 2017, 24, 4–14. [Google Scholar] [CrossRef]
- Kalyan, M.; Tiwari, R.; Ahmad, M.S. Multi-objective optimization in geometric design of tapered roller bearings based on fatigue, wear and thermal considerations through genetic algorithms. Sādhanā 2020, 45. [Google Scholar] [CrossRef]
- Tiwari, R.; Sunil, K.K.; Reddy, R.S. An Optimal Design Methodology of Tapered Roller Bearings Using Genetic Algorithms. Int. J. Comput. Methods Eng. Sci. Mech. 2012, 13, 108–127. [Google Scholar] [CrossRef]
- Jurko, J.; Panda, A.; Valíček, J.; Harničárová, M.; Pandová, I. Study on cone roller bearing surface roughness improvement and the effect of surface roughness on tapered roller bearing service life. Int. J. Adv. Manuf. Technol. 2016, 82, 1099–1106. [Google Scholar] [CrossRef]
- Jamison, W.E.; Kauzlarich, J.J.; Mochel, E.V. Geometric Effects on the Rib-Roller Contact in Tapered Roller Bearings. AsLE Trans. 1977, 20, 79–88. [Google Scholar] [CrossRef]
- Korrenn, H. Gleitreibung und Grenzbelastung an den Bordflächen von Kegelrollenlagern Einfluß von Drehzahl, Belastung, Schmierstoff und Gestaltung der Gleitflächen nach Versuch und Berechnung; VDI-Verl.: Düsseldorf, Germany, 1967; ISBN 0341-1648. [Google Scholar]
- Tong, V.-C.; Hong, S.-W. Optimization of partially crowned roller profiles for tapered roller bearings. J. Mech. Sci. Technol. 2017, 31, 641–650. [Google Scholar] [CrossRef]
- Porras-Vazquez, A.; Fillot, N.; Vergne, P.; Philippon, D.; Morales-Espejel, G.E. Influence of spin on film thickness in elastohydrodynamic starved point contacts. Tribol. Int. 2021, 156, 106825. [Google Scholar] [CrossRef]
- Dormois, H.; Fillot, N.; Vergne, P.; Dalmaz, G.; Querry, M.; Ioannides, E.; Morales-Espejel, G.E. First Traction Results of High Spinning Large-Size Circular EHD Contacts from a New Test Rig: Tribogyr. Tribol. Trans. 2009, 52, 171–179. [Google Scholar] [CrossRef]
- Zhang, Z.; Qiu, X.; Hong, Y. EHL Analysis of Rib-Roller End Contact in Tapered Roller Bearings. Tribol. Trans. 1988, 31, 461–467. [Google Scholar] [CrossRef]
- Gadallah, N.; Dalmaz, G. Hydrodynamic Lubrication of the Rib-Roller End Contact of a Tapered Roller Bearing. J. Tribol. Trans. ASME 1984, 106, 265–272. [Google Scholar] [CrossRef]
- Wang, W.; Wong, P.L.; Zhang, Z. Partial EHL analysis of rib-roller end contact in tapered roller bearings. Tribol. Int. 1996, 29, 313–321. [Google Scholar] [CrossRef]
- Patir, N.; Cheng, H.S. An Average Flow Model for Determining Effects of Three-Dimensional Roughness on Partial Hydrodynamic Lubrication. J. Lubr. Technol. 1978, 100, 12–17. [Google Scholar] [CrossRef]
- Greenwood, J.A.; Tripp, J.H. The Contact of Two Nominally Flat Rough Surfaces. Proc. Inst. Mech. Eng. 1970, 185, 625–633. [Google Scholar] [CrossRef]
- Colin, F.; Chevalier, F.; Chaomleffel, J.-P.; Dalmaz, G.; de Mul, J. Starved Elastohydrodynamic Lubrication of the Rib-Roller End Contact in Tapered Roller Bearings: Film Thickness, Traction and Moments. In Tribology for Energy Conservation, Proceedings of the 24th Leeds-Lyon Symposium on Tribology, London, UK, 4–6 September 1997; Elsevier: Amsterdam, The Netherlands, 1998; pp. 253–263. ISBN 9780444500335. [Google Scholar]
- Fujiwara, H.; Tsujimoto, T.; Yamauchi, K. Optimized Radius of Roller Large End Face in Tapered Roller Bearings(Machine Elements, Design and Manufacturing). JSMET 2009, 75, 2319–2326. [Google Scholar] [CrossRef][Green Version]
- Rosenkranz, A.; Marian, M.; Profito, F.J.; Aragon, N.; Shah, R. The Use of Artificial Intelligence in Tribology—A Perspective. Lubricants 2021, 9, 2. [Google Scholar] [CrossRef]
- Marian, M.; Grützmacher, P.; Rosenkranz, A.; Tremmel, S.; Mücklich, F.; Wartzack, S. Designing surface textures for EHL point-contacts—Transient 3D simulations, meta-modeling and experimental validation. Tribol. Int. 2019, 137, 152–163. [Google Scholar] [CrossRef]
- Harris, T.A.; Kotzalas, M.N. Rolling Bearing Analysis; CRC—Taylor & Francis: Boca Raton, FL, USA, 2007; ISBN 9780849381676. [Google Scholar]
- Dowson, D.; Higginson, G.R.; Hopkins, D.W. Elasto-Hydrodynamic Lubrication: International Series on Materials Science and Technology; Elsevier Science: Burlington, MA, USA, 2014; ISBN 9781483181899. [Google Scholar]
- Roelands, C. Correlational Aspects of the Viscosity-Temperature-Pressure Relationship of Lubricating Oils. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 1966. [Google Scholar]
- Ree, F.; Ree, T.; Eyring, H. Relaxation Theory of Transport Problems in Condensed Systems. Ind. Eng. Chem. 1958, 50, 1036–1040. [Google Scholar] [CrossRef]
- Wirsching, S.; Schwarz, S.; Tremmel, S. Use of analytically describable geometries to calculate the contact between rolling element face and rib in bearing simulations. Tribol. Und. Schmier. 2020, 67, 25–33. [Google Scholar] [CrossRef]
- Siebertz, K.; van Bebber, D.; Hochkirchen, T. Statistische Versuchsplanung: Design of Experiments (DoE); Springer: Berlin, Germany, 2010; ISBN 978-3-642-05493-8. [Google Scholar]
- Johnson, M.E.; Moore, L.M.; Ylvisaker, D. Minimax and maximin distance designs. J. Stat. Plan. Inference 1990, 26, 131–148. [Google Scholar] [CrossRef]
- Kalker, J.J. Variational Principles of Contact Elastostatics. IMA J. Appl. Math. 1977, 20, 199–219. [Google Scholar] [CrossRef]
- Polonsky, I.A.; Keer, L.M. A numerical method for solving rough contact problems based on the multi-level multi-summation and conjugate gradient techniques. Wear 1999, 231, 206–219. [Google Scholar] [CrossRef]
- Lubrecht, A.A. The Numerical Solution of the Elastohydrodynamically Lubricated Line- and Point Contact Problem, Using Multigrid Techniques. Ph.D. Thesis, University of Twente, Enschede, The Netherlands, 1987. [Google Scholar]
- Wijnant, Y.H. Contact Dynamics in the Field of Elastohydrodynamic Lubrication. Ph.D. Thesis, University of Twente, Enschede, The Netherlands, 1998. [Google Scholar]
- Venner, C.H.; Lubrecht, A.A. Multilevel Methods in Lubrication, 1st ed.; Elsevier: Amsterdam, The Netherlands, 2000; ISBN 0-444-50503-2. [Google Scholar]
- Venner, C.H. Multilevel Solution of the EHL Line and Point Contact Problems. Ph.D. Thesis, University of Twente, Enschede, The Netherlands, 1991. [Google Scholar]
- Pausch, M. Untersuchung des Einflusses von Definiert Gefertigten Mikrostrukturen auf Schmierfilmbildung und Kontaktpressung in Hoch Belasteten Wälzkontakten; VDI-Verlag: Dusseldorf, Germany, 2013. [Google Scholar]
- Booker, A.J.; Dennis, J.E.; Frank, P.D.; Serafini, D.B.; Torczon, V.; Trosset, M.W. A rigorous framework for optimization of expensive functions by surrogates. Struct. Optim. 1999, 17, 1–13. [Google Scholar] [CrossRef]
- Simpson, T.W.; Poplinski, J.D.; Koch, P.N.; Allen, J.K. Metamodels for Computer-based Engineering Design: Survey and recommendations. EWC 2001, 17, 129–150. [Google Scholar] [CrossRef]
- Most, T.; Will, J. Metamodel of Optimal Prognosis—An automatic approach for variable reduction and optimal meta-model selection. Weimarer Optim. Stochastiktage 2008, 5, 20–21. [Google Scholar] [CrossRef]
- Lancaster, P.; Salkauskas, K. Surfaces Generated by Moving Least Squares Methods. Math. Comput. 1981, 37, 141. [Google Scholar] [CrossRef]
- Martin, J.D.; Simpson, T.W. Use of Kriging Models to Approximate Deterministic Computer Models. AIAA J. 2005, 43, 853–863. [Google Scholar] [CrossRef]
- Most, T.; Will, J. Recent advances in Meta-model of Optimal Prognosis. Proc. Weimarer Optim. Stochastiktage 2010, 7, 21–22. [Google Scholar]
- Nocedal, J.; Wright, S.J. Numerical Optimization; Springer: New York, NY, USA, 2006; ISBN 978-0-387-30303-1. [Google Scholar]
- Box, G.E.P.; Draper, N.R. Response Surfaces, Mixtures, and Ridge Analyses, 2nd ed.; John Wiley & Sons: New York, NY, USA, 2007; ISBN 978-0-470-05357-7. [Google Scholar]
- Eberhart, R.; Kennedy, J. A new optimizer using particle swarm theory. In MHS’95. Proceedings of the Sixth International Symposium on Micro Machine and Human Science, Nagoya, Japan, 4–6 October 1995; IEEE: New York, NY, USA, 1995; pp. 39–43. ISBN 0-7803-2676-8. [Google Scholar]
- Weicker, K. Evolutionäre Algorithmen, 3rd ed.; Springer: Wiesbaden, Germany, 2015; ISBN 978-3-658-09958-9. [Google Scholar]
- Hamrock, B.J.; Schmid, S.R.; Jacobson, B.O. Fundamentals of fluid film lubrication, 2nd ed.; Dekker: New York, NY, USA, 2004; ISBN 0-8247-5371-2. [Google Scholar]
- Vergne, P.; Bair, S. Classical EHL Versus Quantitative EHL: A Perspective Part I—Real Viscosity-Pressure Dependence and the Viscosity-Pressure Coefficient for Predicting Film Thickness. Tribol. Lett. 2014, 54, 1–12. [Google Scholar] [CrossRef]


| Density ρ0 at 40 °C | 867 kg/m3 |
| Kinematic viscosity ν0 at 40 °C | 95 mm2/s |
| Dynamic viscosityη0 at 40 °C | 0.08 Pa·s |
| Pressure-viscosity coefficient αp at 40 °C | 198 MPa−1 |
| Limiting shear stress τL | 11.0 N/mm² |
| Pressure limiting shear stress γL | 4.76 MPa |
| Geometry Pairing (Roller Face/rib) | Roller Face Radius rR | Eccentricity e | Rib Radius rB | Trial Points |
|---|---|---|---|---|
| sphere/cone | 10–400 mm | 0 mm | – | 20 |
| torus/cone | 10–400 mm | 0–3 mm | – | 50 |
| sphere/torus | 10–400 mm | 0 mm | 10–400 mm | 50 |
| torus/torus | 10–400 mm | 0–3 mm | 10–400 mm | 100 |
| sphere/torus concave | 10–400 mm | 0 mm | −400–−10 mm | 50 |
| torus/torus concave | 10–400 mm | 0–3 mm | −400–−10 mm | 100 |
| Criteria | Optimization Goal | Traction Coefficient COF ↓ |
| Min. Fluid Film Height hmin ↑ | ||
| initialization | start population size | 20 |
| min. number of generations | 10 | |
| max. number of generations | 50 | |
| stop after generations of stagnations | 10 | |
| selection | ranking | pareto |
| number of parents | 10 | |
| selection | tournament size 2 | |
| crossover | method (hybrid) | multipoint |
| simulated binary | ||
| crossover probability | 50% | |
| distribution parameter | 2.0 | |
| mutation | type | self-adaptive |
| mutation rate | 70% | |
| standard deviation | 0.05–0.10 |
| Geometry Pairing | Roller Face Radius rR | Eccentricity e | Rib Radius rB | Max. Pressure Pmax | Min. Film Thickness Hmin | Coefficient of Friction COF |
|---|---|---|---|---|---|---|
| sphere/cone | 193 mm | 0 mm | – | 0.406 | 0.553 | 0.00177 |
| torus/cone | 190 mm | 2.5 mm | – | 0.291 | 0.616 | 0.00229 |
| sphere/torus | 211 mm | 0 mm | 234 mm | 0.121 | 2.336 | 0.01567 |
| torus/torus | 206 mm | 0.8 mm | 202 mm | 0.146 | 1.979 | 0.01460 |
| sphere/torus concave | 185 mm | 0 mm | −204 mm | 0.161 | 1.881 | 0.01437 |
| torus/torus concave | 187 mm | 2,2 mm | −219 mm | 0.139 | 2.111 | 0.01528 |
| Geometry Pairing | max. Hydrodynamic Pressure pmax | Min. Lubricant Gap hmin | Coefficient of Friction COF |
|---|---|---|---|
| sphere/cone | 99.5% | 99.8% | 99.0% |
| torus/cone | 98.7% | 98.6% | 99.9% |
| sphere/torus | 94.5% | 98.2% | 90.9% |
| torus/torus | 91.8% | 98.7% | 97.1% |
| sphere/torus concave | 90.8% | 95.0% | 83.9% |
| torus/torus concave | 97.0% | 98.3% | 94.5% |
| Geometry Pairing | Roller Face Radius rR in mm | Eccentricity e in mm | Rib Radius rB in mm | |||
|---|---|---|---|---|---|---|
| (Roller Face/Rib) | min COF | max. hmin | min COF | max. hmin | min COF | max. hmin |
| sphere/cone | 317 | 400 | 0 | 0 | – | – |
| torus/cone | 392 | 393 | 0.1 | 2.9 | – | – |
| sphere/torus | 337 | 370 | 0 | 0 | 159 | 387 |
| torus/torus | 283 | 400 | 0.1 | 2.9 | 137 | 400 |
| sphere/torus concave | 180 | 329 | 0 | 0 | −215 | −393 |
| torus/torus concave | 160 | 364 | 0.7 | 0.3 | −171 | −390 |
| Geometry Pairing | Minimizing COF | Maximizing Lubricant Gap | |||||
|---|---|---|---|---|---|---|---|
| (Roller Face/Rib) | pmax | hmin | COF | pmax | hmin | COF | |
| sphere/cone | 98.5% | 99.8% | 98.2% | 98.2% | 99.7% | 99.8% | |
| torus/cone | 99.5% | 99.8% | 99.6% | 96.4% | 99.0% | 99.2% | |
| sphere/torus | 88.3% | 99.4% | 99.3% | 85.2% | 99.7% | 99.9% | |
| torus/torus | 94.2% | 99.8% | 99.1% | 55.6% | 99.8% | 99.8% | |
| sphere/torus concave | 98.6% | 99.9% | 99.6% | 99.9% | 99.9% | 99.9% | |
| torus/torus concave | 19,7% | 99.9% | 93.2% | 99.9% | 99.9% | 99.8% | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wirsching, S.; Marian, M.; Bartz, M.; Stahl, T.; Wartzack, S. Geometrical Optimization of the EHL Roller Face/Rib Contact for Energy Efficiency in Tapered Roller Bearings. Lubricants 2021, 9, 67. https://doi.org/10.3390/lubricants9070067
Wirsching S, Marian M, Bartz M, Stahl T, Wartzack S. Geometrical Optimization of the EHL Roller Face/Rib Contact for Energy Efficiency in Tapered Roller Bearings. Lubricants. 2021; 9(7):67. https://doi.org/10.3390/lubricants9070067
Chicago/Turabian StyleWirsching, Sven, Max Marian, Marcel Bartz, Thomas Stahl, and Sandro Wartzack. 2021. "Geometrical Optimization of the EHL Roller Face/Rib Contact for Energy Efficiency in Tapered Roller Bearings" Lubricants 9, no. 7: 67. https://doi.org/10.3390/lubricants9070067
APA StyleWirsching, S., Marian, M., Bartz, M., Stahl, T., & Wartzack, S. (2021). Geometrical Optimization of the EHL Roller Face/Rib Contact for Energy Efficiency in Tapered Roller Bearings. Lubricants, 9(7), 67. https://doi.org/10.3390/lubricants9070067






