Thermal Turbulent Flow in Leading Edge Grooved and Conventional Tilting Pad Journal Bearing Segments—A Comparative Study
Abstract
:1. Introduction
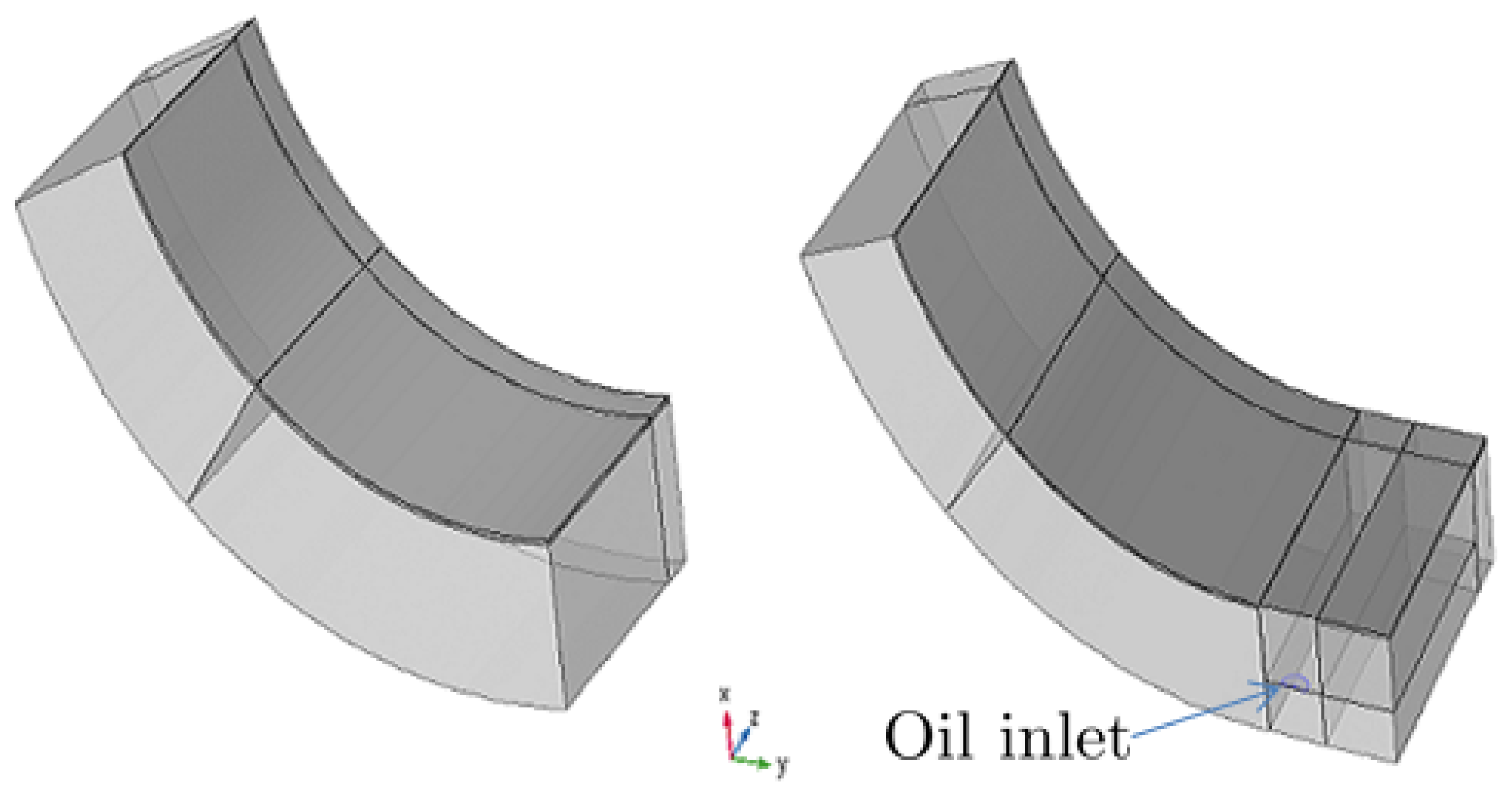
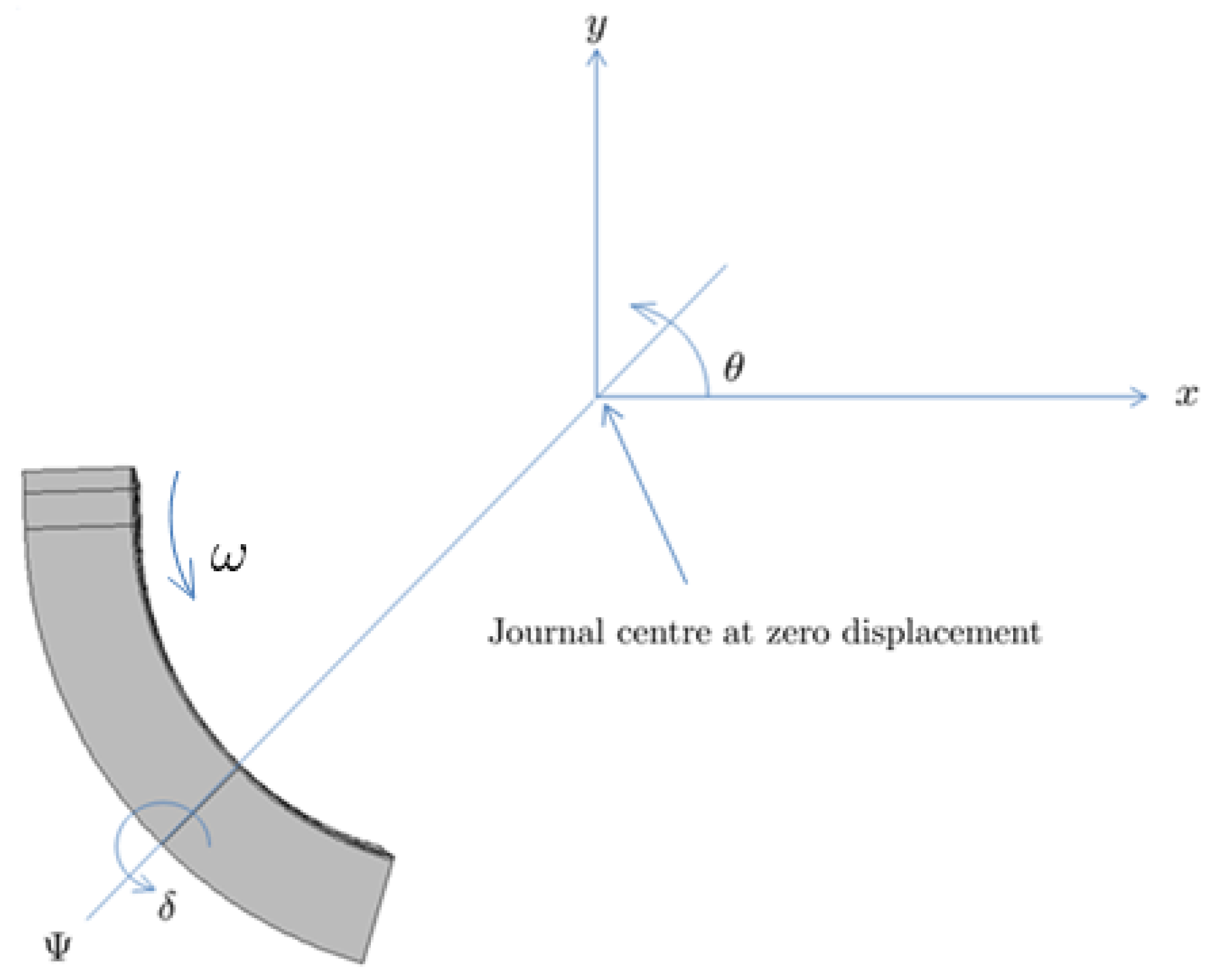
2. Geometry
3. Model
3.1. Fluid Dynamics
3.2. Thermal Equations
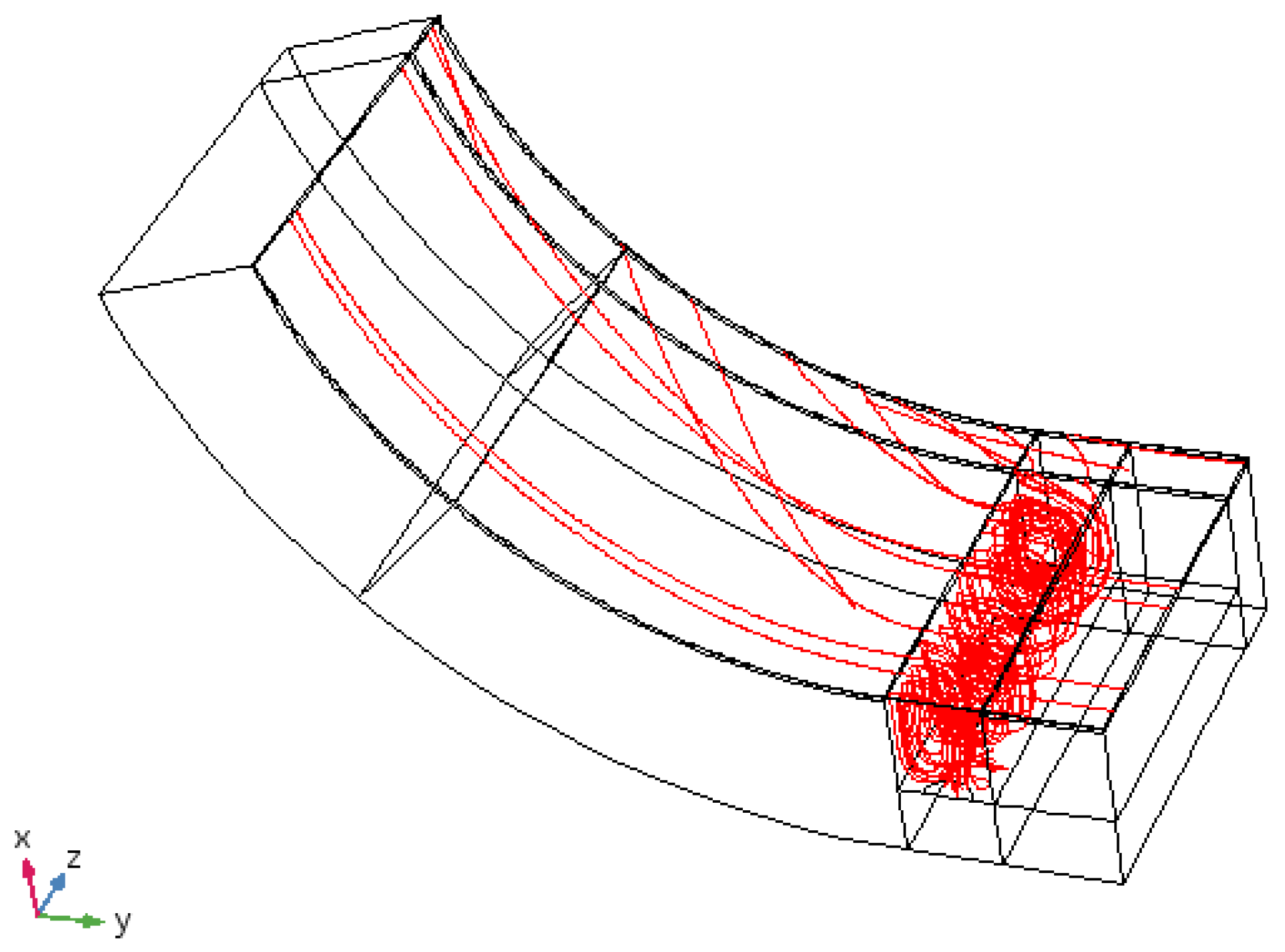
3.3. Dynamic Meshing
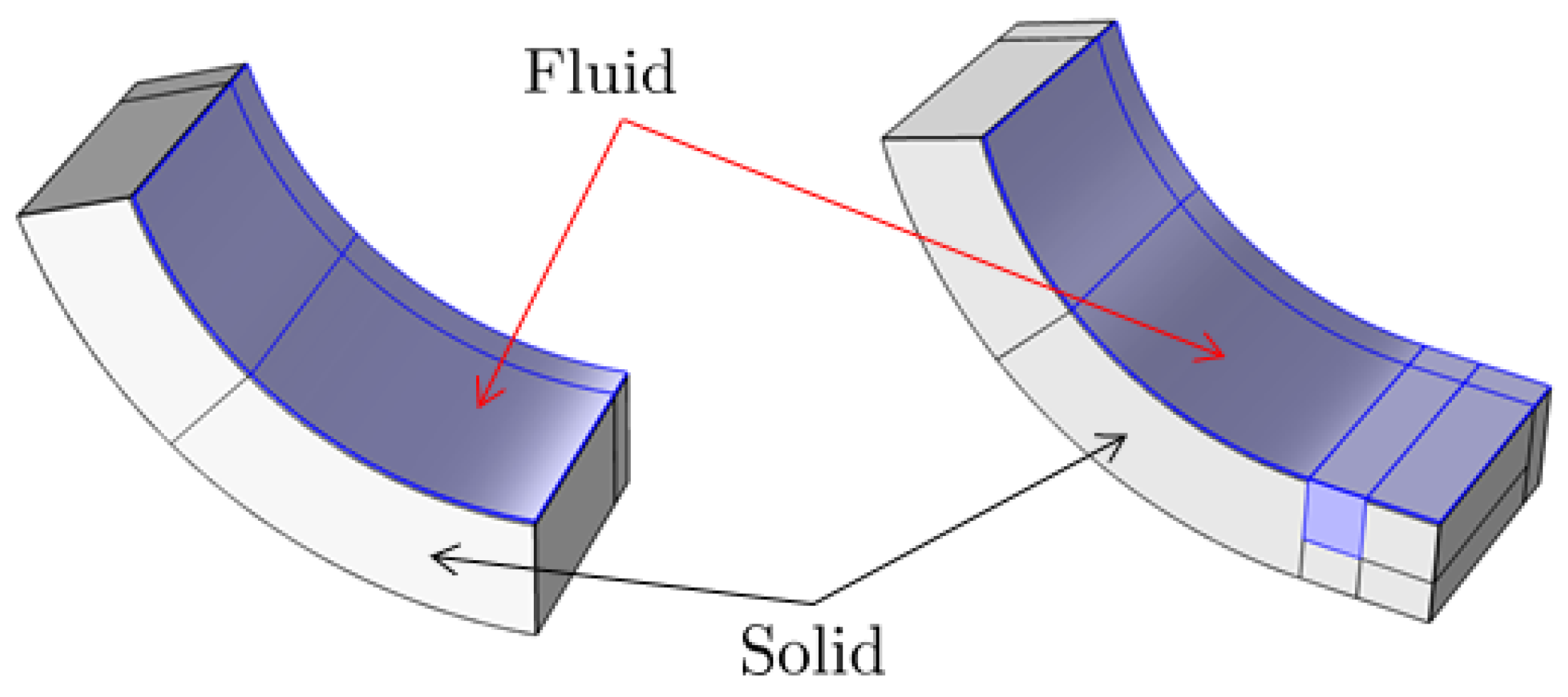
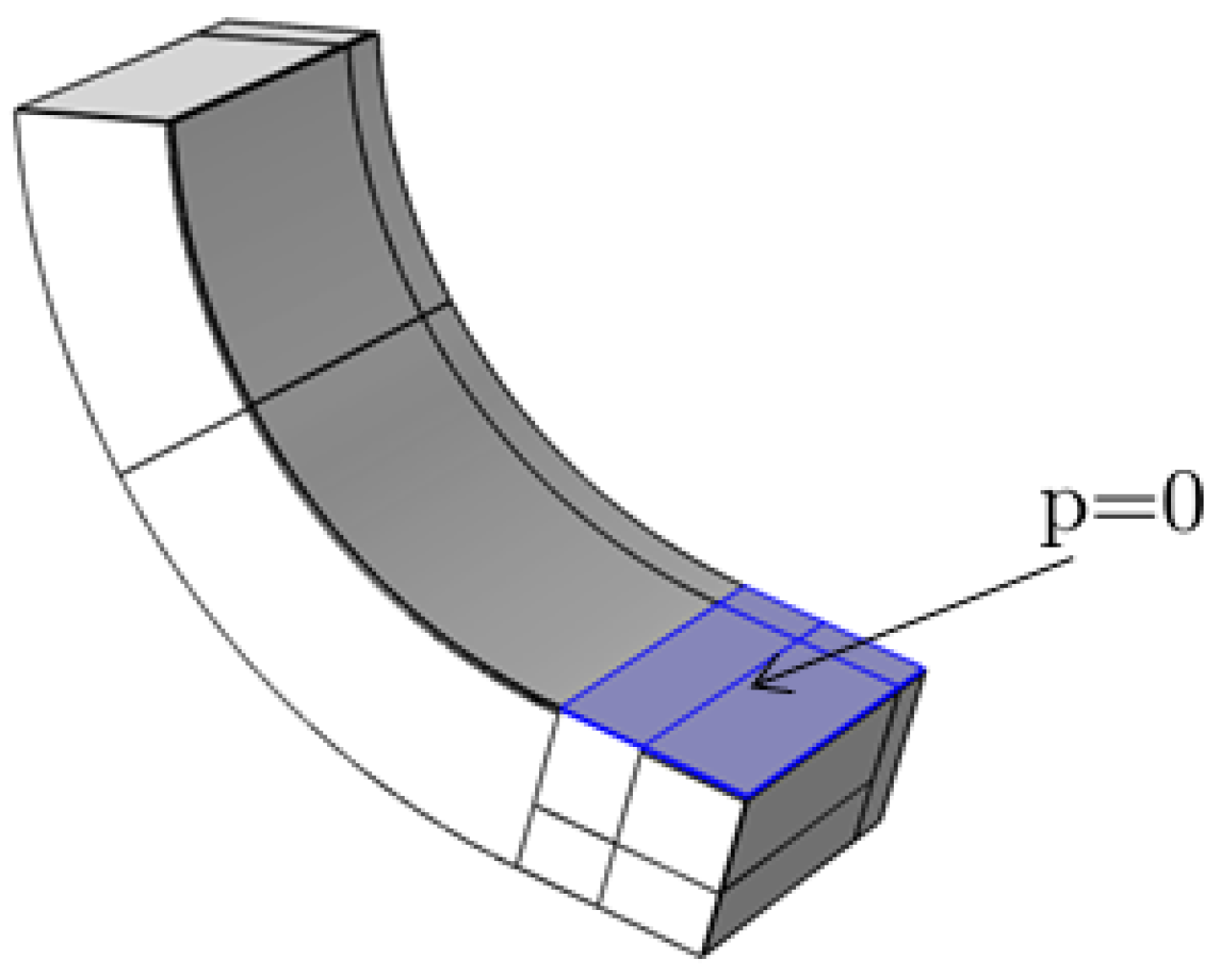
3.4. Boundary Conditions
3.4.1. Fluid Flow
3.4.2. Thermal
3.4.3. Mesh Deformation
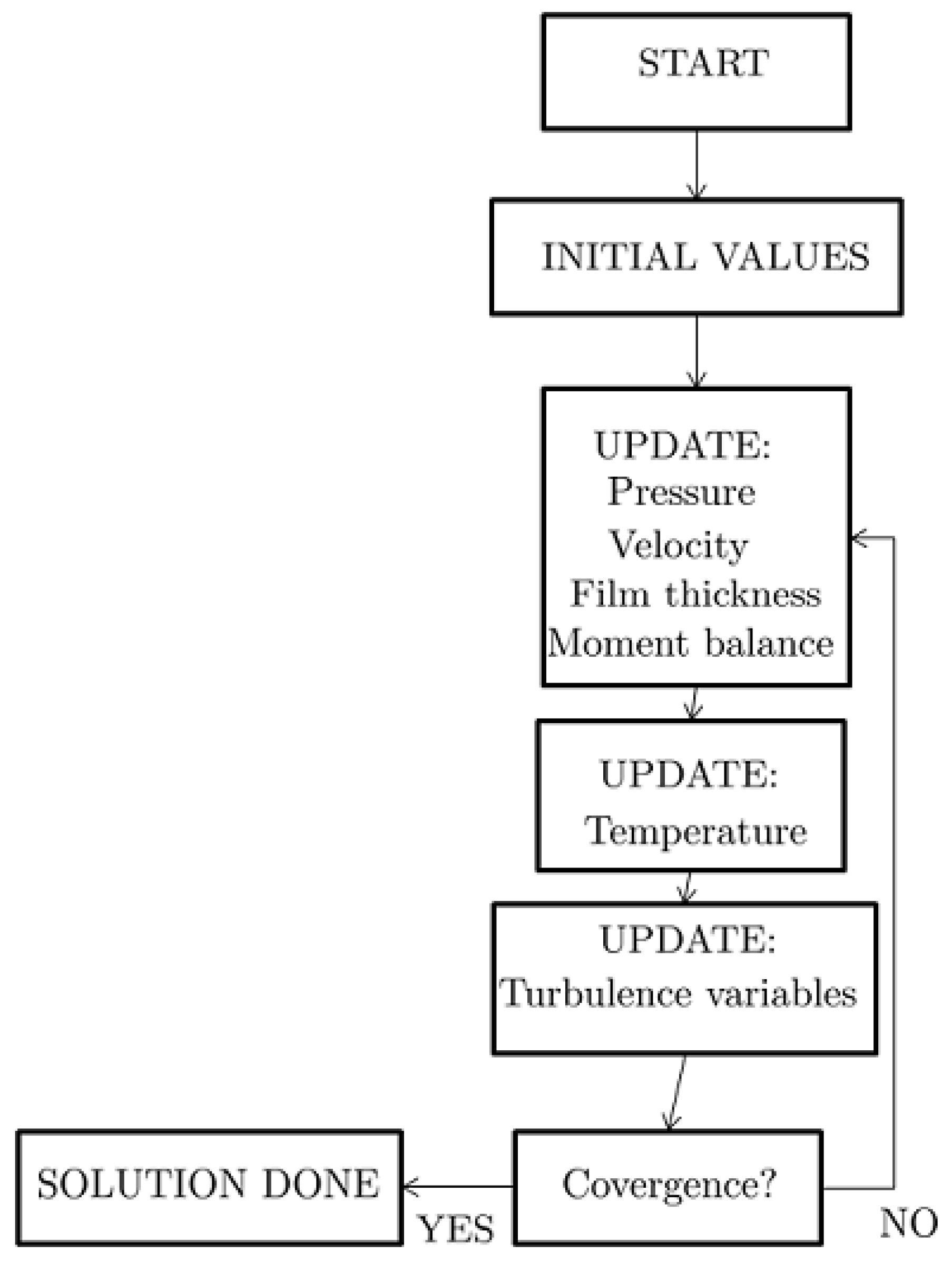
4. Simulation
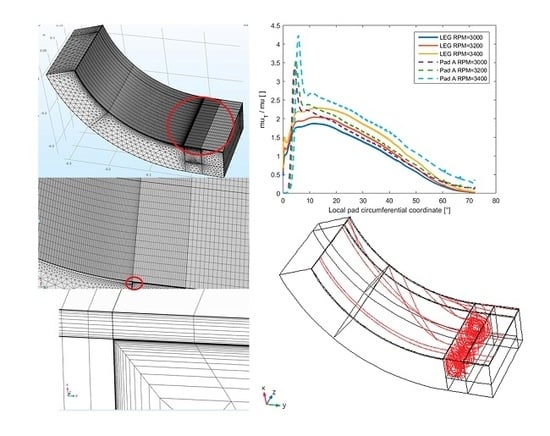
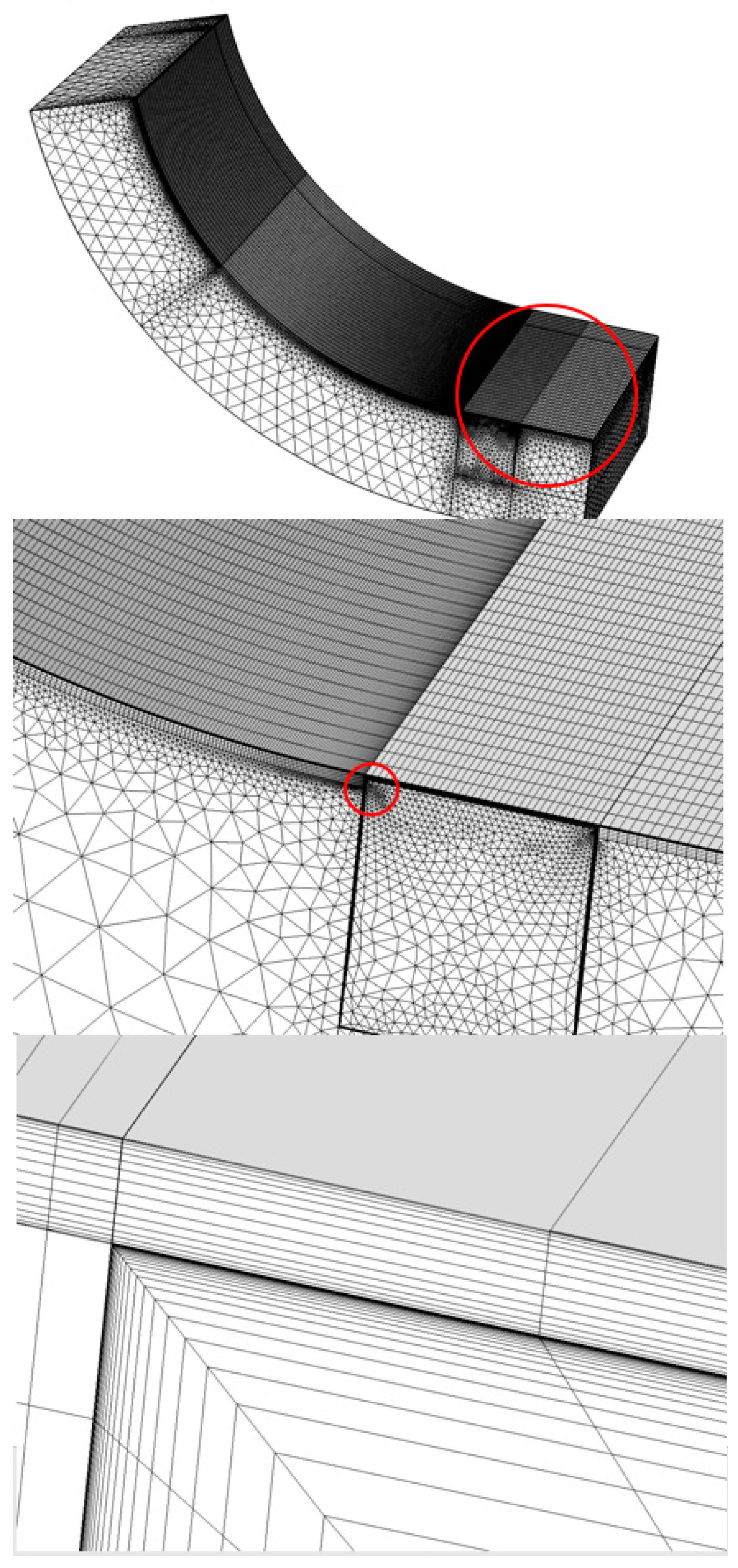
Finite Element Mesh
5. Results and Discussion
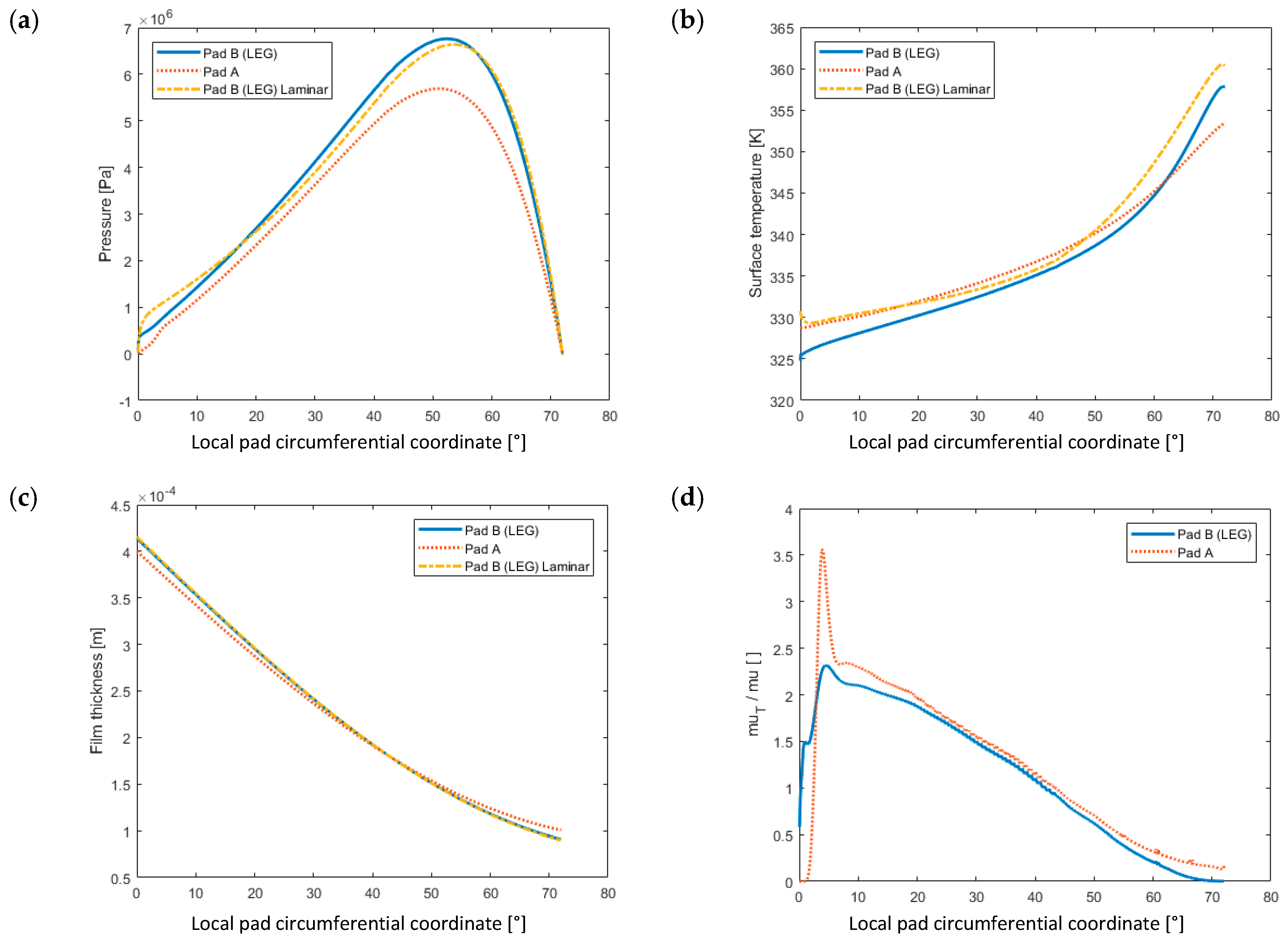
5.1. Pad A vs. Pad B
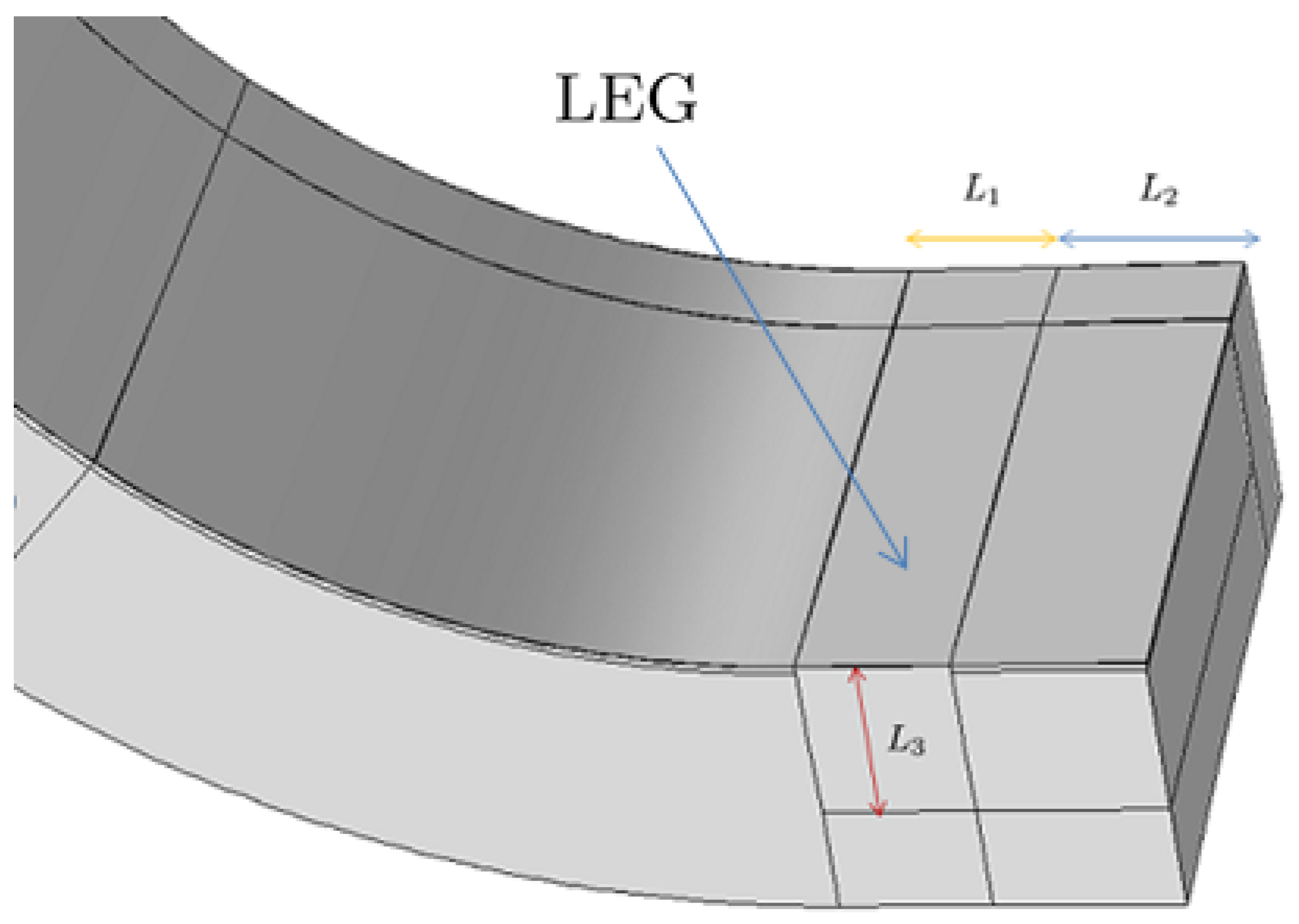
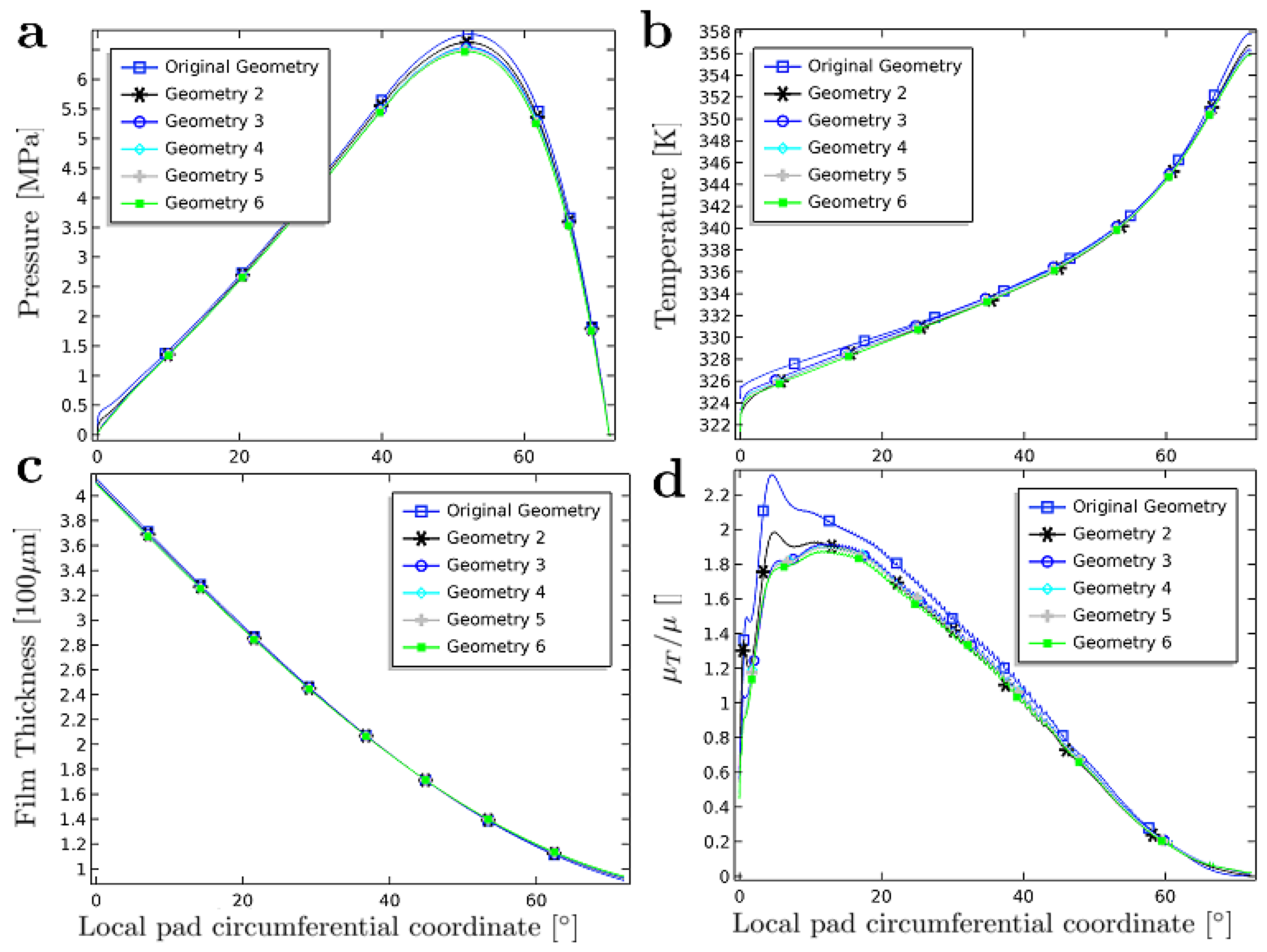
5.2. Modified Pad B—Shrinking the Length of the LEG Portion
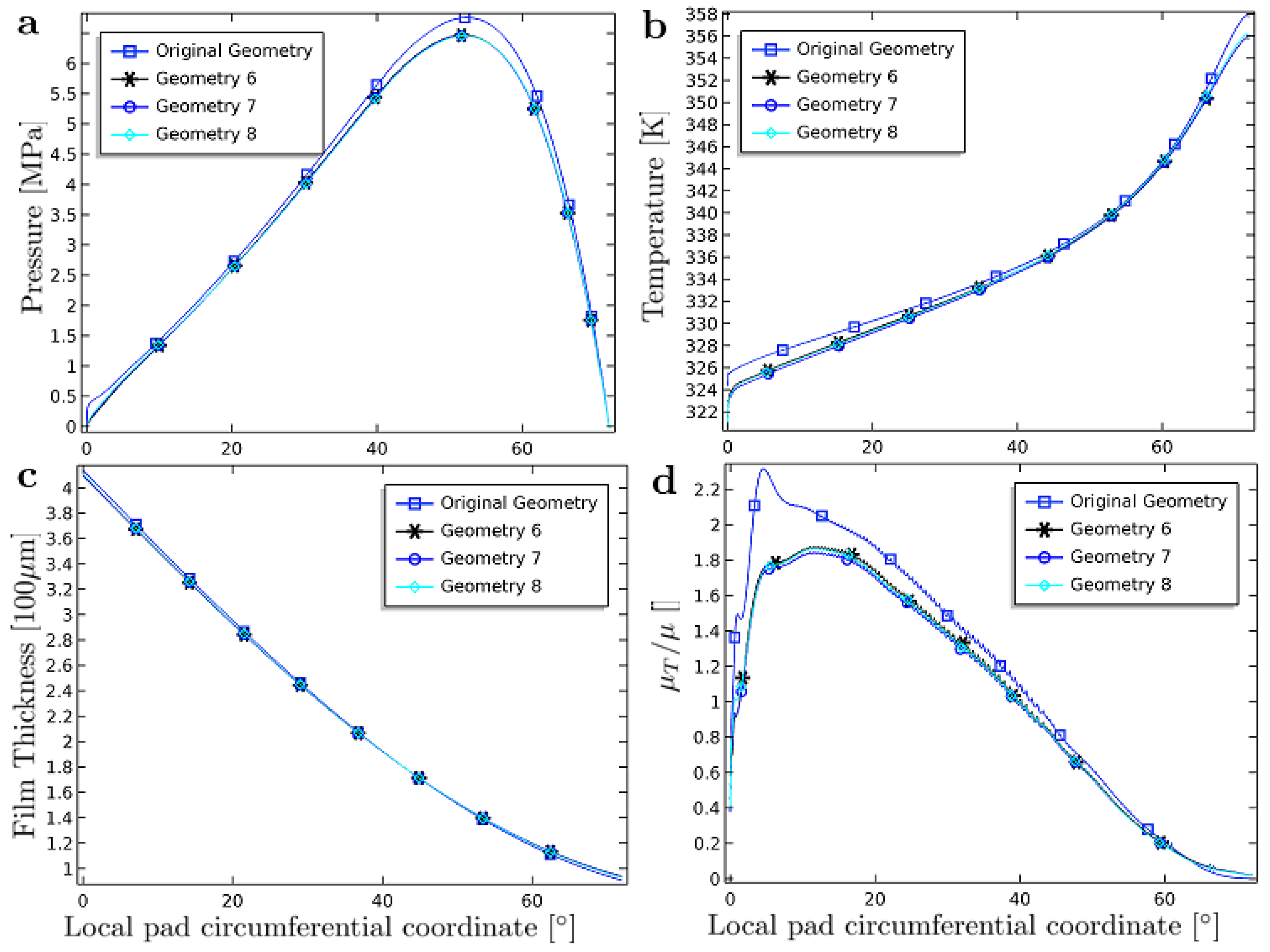
5.3. Modified Pad B—Shrinking the Depth of the LEG
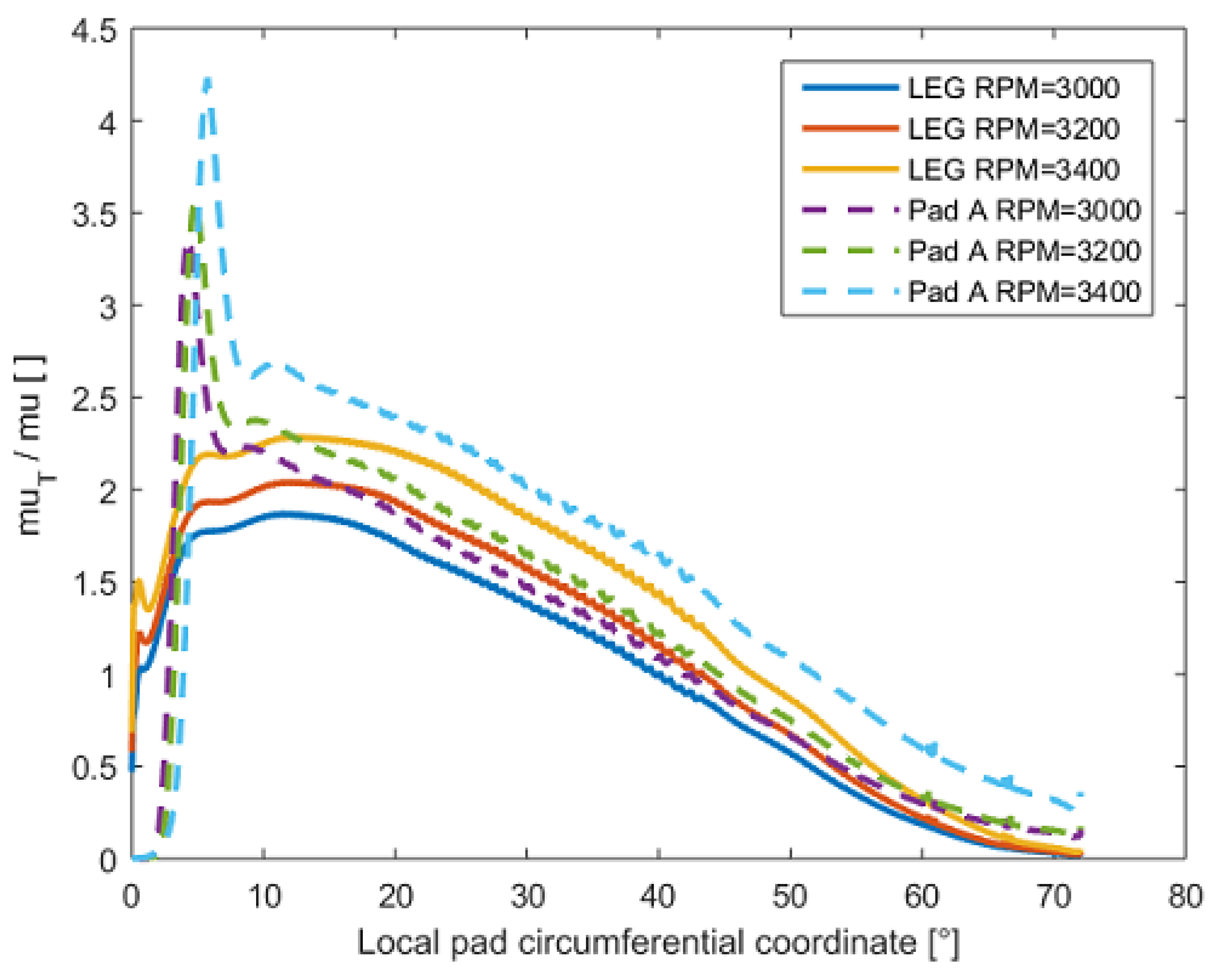
5.4. Modified Pad B vs. Pad A—Increasing the Rotational Speed
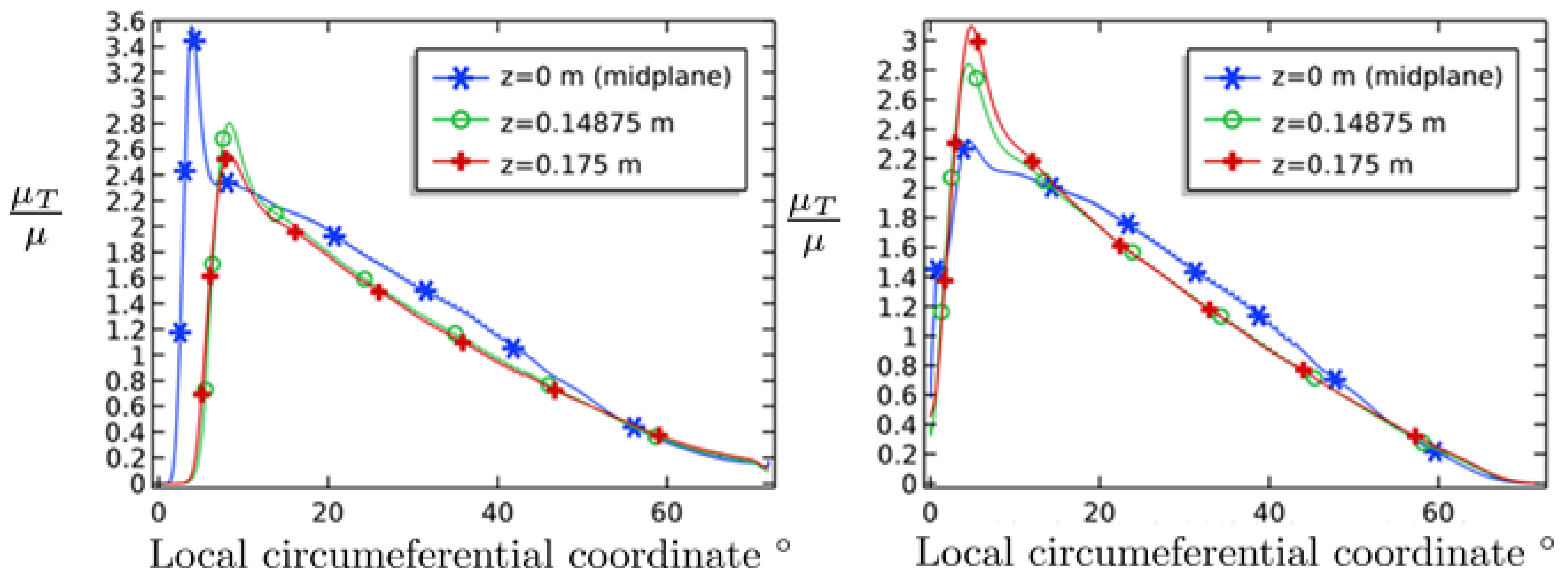
5.5. Turbulence Levels as a Function of Axial Position
6. Conclusions
- The effective temperature boundary condition in Pad A seems to overestimate the inlet temperature compared to Pad B. The difference is quite small however.
- The LEG pad is predicted to have a lower level of turbulence than the conventional pad in the mid plane, the difference becomes even larger as the speed increases. It is however predicted to have a slightly higher value of turbulence when looking at the volume average compared to Pad A. Moreover, the maximum value of turbulence levels is found in the mid plane of Pad A. This makes it so that any conclusion regarding the hypothesis put out by He et al. [7] cannot be stated without a serious amount of skepticism and so this research question cannot be resolved based on the findings of the present study.
- The LEG pad yields a higher maximum pad surface temperature due to the typical transition from turbulent to a laminar flow towards the trailing edge being more prominent than in the no LEG case. This result is further supported by considering Figure 8d which shows that the level of turbulence is indeed somewhat lower for the LEG pad towards the trailing edge.
- The dimensions of the LEG portion has a surprisingly modest influence on the downstream flow characteristics.
- In future similar investigations, the ram pressure should be accounted for in the model for the conventional (no LEG) pad since this might have an notable impact on the down stream flow characteristics.
- As with any attempt to simulate turbulent flow, the findings of the present study must be used with great caution until more refined studies and/or experimental data are available for comparison.
Author Contributions
Funding
Conflicts of Interest
References
- Mikula, A.M.; Gregory, R.S. A comparison of tilting pad thrust bearing lubricant supply methods. J. Lubr. Technol. 1983, 105, 39–45. [Google Scholar] [CrossRef]
- Mikula, A.M. Further test results of the leading-edge-groove (leg) tilting pad thrust bearing. ASME J. Tribol. 1988, 110, 174–180. [Google Scholar] [CrossRef]
- Bang, K.-B.; Kim, J.-H.; Cho, Y.-J. Comparison of power loss and pad temperature for leading edge groove tilting pad journal bearings and conventional tilting pad journal bearings. Tribol. Int. 2010, 43, 1287–1293. [Google Scholar] [CrossRef]
- Harangozo, A.V.; Stolarski, T.A.; Gozdawa, R.J. The effect of different lubrication methods on the performance of a tilting-pad journal bearing. Tribol. Trans. 1991, 34, 529–536. [Google Scholar] [CrossRef]
- Brockwell, K.W.S.A.; Dmochowski, W.; DeCamillo, S.; Mikula, A. Performance evaluation of the leg tilting pad journal bearing. In IMechE Seminar Plain Bearings: Plain Bearings: Energy Efficiency and Design; IMechE: London, UK, 1992; pp. 51–58. [Google Scholar]
- Dmochowski, W.K.S.A.; Brockwell, K.; DeCamillo, S.; Mikula, A. A study of the thermal characteristics of the leading edge groove and conventional tilting pad journal bearings. J. Tribol. 1993, 115, 219–226. [Google Scholar] [CrossRef]
- He, M.; Allaire, P.E.; Barrett, L.; Nicholas, J. Tehd modeling of leading edge groove tilting pad bearings. In Proceedings of the 6th International Conference on Rotor Dynamics (IFTOMM), Sydney, Australia, 30 September–4 October 2002. [Google Scholar]
- Edney, S.L.; Heitland, G.B.; DeCamillo, S.M. Testing, analysis, and cfd modeling of a profiled leading edge groove tilting pad journal bearing. In Proceedings of the ASME 1998 International Gas Turbine and Aeroengine Congress and Exhibition, Stockholm, Sweden, 2–5 June 1998; pp. 2–5. [Google Scholar]
- Varela, A.C.; García, A.B.; Santos, I.F. Modelling of leg tilting pad journal bearings with active lubrication. Tribol. Int. 2017, 107, 250–263. [Google Scholar] [CrossRef]
- Constantinescu, V.N. Analysis of bearings operating in turbulent regime. J. Basic Eng. 1962, 84, 139–151. [Google Scholar] [CrossRef]
- Ng, C.W. Fluid dynamic foundation of turbulent lubrication theory. ASLE Trans. 1964, 7, 311–321. [Google Scholar] [CrossRef]
- Ng, C.W.; Pan, C.H.T. A linearized turbulent lubrication theory. J. Basic Eng. 1965, 87, 675–682. [Google Scholar] [CrossRef]
- Bouard, L.; Fillon, M.; Frene, J. Comparison between three turbulent models: Application to thermohydrodynamic performances of tilting-pad journal bearings. Tribol. Int. 1996, 29, 11–18. [Google Scholar] [CrossRef]
- Bukhari, M.; Jaat, M.; Zaman, I.; Khalid, A. Cfd analysis of thin film lubricated journal bearing. Procedia Eng. 2013, 68, 56–62. [Google Scholar]
- Nadia, B.; Mehala, K.; Abdelkader, Y. Numerical analyses of turbulent flow behavior in plain journal bearing. Ind. Lubr. Tribol. 2016, 68, 76–85. [Google Scholar]
- Armentrout, R.W.; He, M.; Haykin, T.; Reed, A.E. Analysis of turbulence and convective inertia in a water-lubricated tilting-pad journal bearing using conventional and cfd approaches. Tribol. Trans. 2016, 60, 1129–1147. [Google Scholar] [CrossRef]
- Hopf, G.; Schuler, D. Investigations on large turbine bearings working under transitional conditions between laminar and turbulent flow. ASME J. Tribol. 1989, 101, 628–634. [Google Scholar] [CrossRef]
- Gardner, W.W.; Ulschmid, J.G. Turbulence effects in two journal bearing applications. J. Lubr. Technol. 1974, 96, 15–20. [Google Scholar] [CrossRef]
- Monmousseau, P.; Fillon, M. Transient thermoelastohydrodynamic analysis for safe operating conditions of a tilting-pad journal bearing during start-up. Tribol. Int. 2000, 33, 225–231. [Google Scholar] [CrossRef]
- Menter, F.R.; Kuntz, M.; Langtry, R. Ten years of industrial experience with the sst turbulence model. Turbul. Heat Mass Transf. 2003, 4, 625–632. [Google Scholar]
- The Menter Shear Stress Transport Turbulence Model. Available online: http://turbmodels.larc.nasa.gov/sst.html (accessed on 5 December 2017).
- COMSOL 5.3, cfd User’s Guide; COMSOL Inc.: Stockholm, Sweden, 2017.
- Kays, W.M. Turbulent prandtl number: Where are we? J. Heat Transf. 1994, 116, 284–295. [Google Scholar] [CrossRef]
- Comsol Multiphysics Reference Manual, Version 5.3; COMSOL Inc.: Stockholm, Sweden, 2017.
- Suganami, T.; Szeri, A.Z. A thermohydrodynamic analysis of journal bearings. J. Lubr. Technol. 1979, 101, 21–27. [Google Scholar] [CrossRef]
- Knežević, D.; Savić, V. Mathematical Modeling of Changing of Dynamic Viscosity, as a Function of Temperature and Pressure, of Mineral Oils for Hydraulic Systems; Facta Universitatis Series Mechanical Engineering; University of Niš: Beograd, Serbia, 2006. [Google Scholar]
- Taniguchi, S.; Makino, T.; Takeshita, K.; Ichimura, T. A thermohydrodynamic analysis of large tilting-pad journal bearing in laminar and turbulent flow regimes with mixing. J. Tribol. 1990, 112, 542–548. [Google Scholar] [CrossRef]
| Parameter | Value | Description |
|---|---|---|
| 0.25 [m] | Bearing radius | |
| L | 0.350 [m] | Bearing axial length |
| 0.075 [m] | Pad thickness | |
| 0.002 [m] | Babbit thickness | |
| m | 0.25 [] | Pad preload |
| 1.28‰ | Relative clearance | |
| 72 | Pad arc length | |
| p | 0.6 [] | Pivot offset |
| 0 [m] | Journal x-eccentricity | |
| −2 [m] | Journal y-eccentricity | |
| N | 3000 | Journal revolutions per minute |
| 320 [K] | Lubricant injection temperature | |
| 320 [K] | Lubricant leading edge inlet temperature | |
| 323.15 [K] | Convection temperature for the external pad surfaces | |
| 400 | Convection coefficient for the pad external surfaces | |
| 225 | Pivot position in the circumferential coordinate | |
| 0.00175 [] | LEG supply flow | |
| 0.04 [m] | Original length of the LEG | |
| 0.05 [m] | Original length of the pad portion preceding the LEG | |
| 0.0462 [m] | Original depth of the LEG | |
| [m] | Radius of the circular inlet hole in the LEG |
| Case | 2 | 3 | 4 | 5 | 6 |
|---|---|---|---|---|---|
| [m] | 0.03 | 0.025 | 0.025 | 0.025 | 0.025 |
| [m] | 0.04 | 0.035 | 0.025 | 0.02 | 0.015 |
| Case | 7 | 8 |
|---|---|---|
| [m] | 0.0308 | 0.0154 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Croné, P.; Almqvist, A.; Larsson, R. Thermal Turbulent Flow in Leading Edge Grooved and Conventional Tilting Pad Journal Bearing Segments—A Comparative Study. Lubricants 2018, 6, 97. https://doi.org/10.3390/lubricants6040097
Croné P, Almqvist A, Larsson R. Thermal Turbulent Flow in Leading Edge Grooved and Conventional Tilting Pad Journal Bearing Segments—A Comparative Study. Lubricants. 2018; 6(4):97. https://doi.org/10.3390/lubricants6040097
Chicago/Turabian StyleCroné, Philip, Andreas Almqvist, and Roland Larsson. 2018. "Thermal Turbulent Flow in Leading Edge Grooved and Conventional Tilting Pad Journal Bearing Segments—A Comparative Study" Lubricants 6, no. 4: 97. https://doi.org/10.3390/lubricants6040097
APA StyleCroné, P., Almqvist, A., & Larsson, R. (2018). Thermal Turbulent Flow in Leading Edge Grooved and Conventional Tilting Pad Journal Bearing Segments—A Comparative Study. Lubricants, 6(4), 97. https://doi.org/10.3390/lubricants6040097