Cold-Flow Properties of Estolides: The Older (D97 and D2500) versus the Mini-(D5773 and D5949) Methods
Abstract
1. Introduction
2. Materials and Methods
3. Results
3.1. CP and PP Results
3.2. CP Correlations
3.3. PP Correlations
3.3.1. ASTM D5949—D97 Correlations
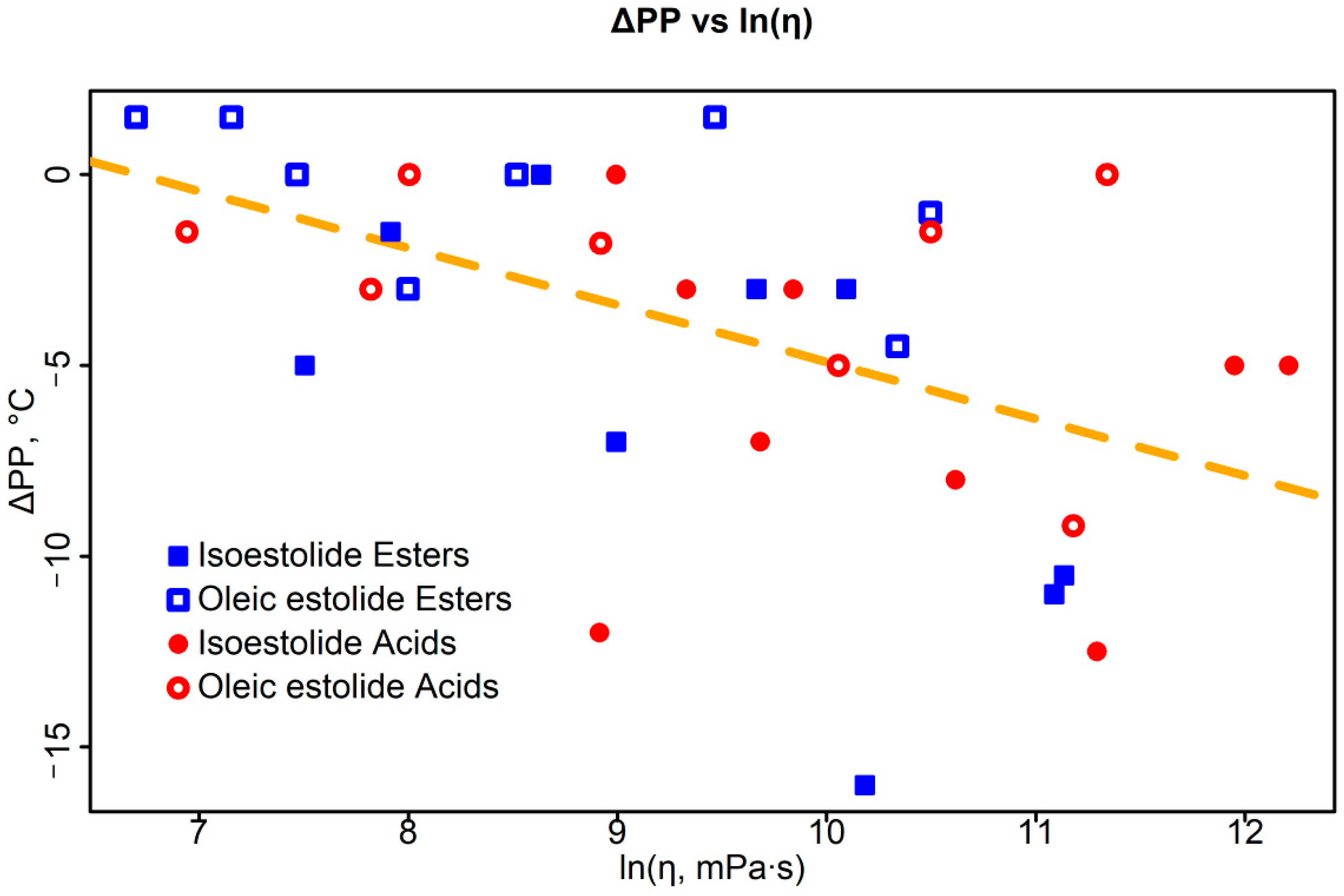
3.3.2. Viscosity–PP Correlations
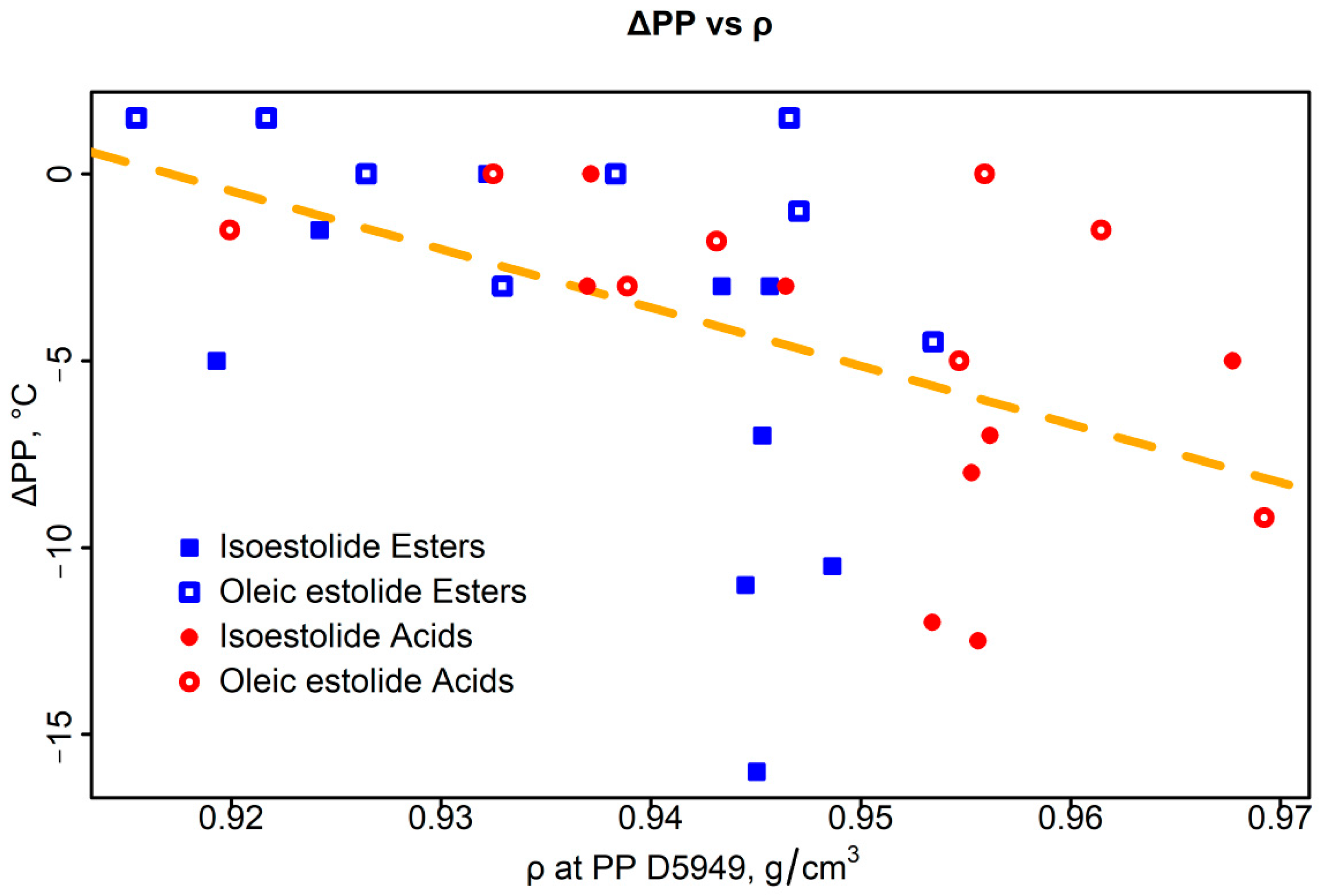
3.3.3. Density and Surface Tension–PP Correlations
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Disclaimer
References
- Ma, S.; Lei, T.; Meng, J.; Liang, X.; Guan, D. Contributions of key countries, enterprises, and refineries to greenhouse gas emissions in global oil refining, 2000–2021. Innovation 2023, 4, 100361. [Google Scholar] [CrossRef] [PubMed]
- Häder, D.P.; Banaszak, A.T.; Villafañe, V.E.; Narvarte, M.A.; González, R.A.; Helbling, E.W. Anthropogenic pollution of aquatic ecosystems: Emerging problems with global implications. Sci. Total Environ. 2020, 713, 136586. [Google Scholar] [CrossRef]
- Chapman, I. The end of Peak Oil? Why this topic is still relevant despite recent denials. Energy Policy 2014, 64, 93–101. [Google Scholar] [CrossRef]
- Nogales-Delgado, S.; Encinar, J.M.; González, J.F. A Review on biolubricants based on vegetable oils through transesterification and the role of catalysts: Current status and future trends. Catalysts 2023, 13, 1299. [Google Scholar] [CrossRef]
- Sanjurjo, C.; Rodríguez, E.; Viesca, J.L.; Battez, A.H. Influence of molecular structure on the physicochemical and tribological properties of biolubricants: A review. Lubricants 2023, 11, 380. [Google Scholar] [CrossRef]
- Narayana Sarma, R.; Vinu, R. Current status and future prospects of biolubricants: Properties and applications. Lubricants 2022, 10, 70. [Google Scholar] [CrossRef]
- Hamnas, A.; Unnikrishnan, G. Bio-lubricants from vegetable oils: Characterization, modifications, applications and challenges—Review. Renew. Sustain. Energy Rev. 2023, 182, 113413. [Google Scholar] [CrossRef]
- Yang, L.; Zhao, X.; Ma, Z.; Ma, S.; Zhou, F. An overview of functional biolubricants. Friction 2023, 11, 23–47. [Google Scholar] [CrossRef]
- Ruhle, T.; Fies, M. Eco Requirements for Lubricant Additives and Base Stocks. In Lubricant Additives: Chemistry and Applications, 3rd ed.; Rudnick, L.R., Ed.; CRC Press: Boca Raton, FL, USA, 2017; pp. 597–619. [Google Scholar] [CrossRef]
- Rudnick, L.R.; Bartz, W.J. Comparison of Synthetic, Mineral Oil, and Bio-based Lubricant Fluids. In Synthetics, Mineral Oils, and Bio-Based Lubricants, 3rd ed.; Rudnick, L.R., Ed.; CRC Press: Boca Raton, FL, USA, 2020; pp. 359–377. [Google Scholar] [CrossRef]
- Schneider, M.P. Plant-oil-based lubricants and hydraulic fluids. J. Sci. Food Agric. 2006, 86, 1769–1780. [Google Scholar] [CrossRef]
- Rabenstein, A.; Koch, T.; Remesch, M.; Brinksmeier, E.; Kuever, J. Microbial degradation of water miscible metal working fluids. Int. Biodeterior. Biodegrad. 2009, 63, 1023–1029. [Google Scholar] [CrossRef]
- Lawate, S.S.; Lal, K.; Huang, C. Vegetable Oils Structure. In Tribology Data Handbook; Richard Booser, E., Ed.; CRC Press: Boca Raton, FL, USA, 1997; pp. 103–116. [Google Scholar]
- Asadauskas, S.; Perez, J.M.; Duda, J.L. Oxidative stability and antiwear properties of high oleic vegetable oils. Lubr. Eng. 1996, 52, 877–882. [Google Scholar]
- Heggs, R. Industrial uses of high-oleic oils. In High Oleic Oils: Development, Properties, and Uses; Flider, F.J., Ed.; AOCS Press: Urbana, IL, USA, 2021; pp. 245–259. [Google Scholar] [CrossRef]
- Winfield, D.D.; Cermak, S.C.; Evangelista, R.L.; Moser, B.R.; McKinney, J.; Pantalone, V. Evaluation of a high oleic soybean oil variety in lubricant and biodiesel applications. J. Am. Oil Chem. Soc. 2023. [Google Scholar] [CrossRef]
- Winfield, D.D.; Dunn, R.O.; Winkler-Moser, J.K.; Cermak, S.C.; Marks, D.M. Characterization, physical properties, and potential industrial applications of high oleic pennycress oil. Ind. Crops Prod. 2024, 210, 118095. [Google Scholar] [CrossRef]
- Karmakar, G.; Ghosh, P.; Kohli, K.; Sharma, B.K.; Erhan, S.Z. Chemicals from vegetable oils, fatty derivatives, and plant biomass. In Innovative Uses of Agricultural Products and Byproducts; Tunick, M.N., Liu, L., Eds.; ACS: Washington, DC, USA, 2020; pp. 1–31. [Google Scholar] [CrossRef]
- Perez, J.M.; Rudnick, L.R.; Erhan, S.Z.; Sharma, B.K.; Kohli, K. Natural Oils as Lubricants. In Synthetics, Mineral Oils, and Bio-Based Lubricants, 3rd ed.; Rudnick, L.R., Ed.; CRC Press: Boca Raton, FL, USA, 2020; p. 392. [Google Scholar] [CrossRef]
- Sharma, B.K.; Karmakar, G.; Erhan, S.Z. Modified Vegetable Oils for Environmentally Friendly Lubricant Applications. In Synthetics, Mineral Oils, and Bio-Based Lubricants, 3rd ed.; Rudnick, L.R., Ed.; CRC Press: Boca Raton, FL, USA, 2020; pp. 399–430. [Google Scholar] [CrossRef]
- Syahir, A.Z.; Zulkifli, N.W.; Masjuki, H.H.; Kalam, M.A.; Alabdulkarem, A.; Gulzar, M.; Khuong, L.S.; Harith, M.H. A review on bio-based lubricants and their applications. J. Clean. Prod. 2017, 168, 997–1016. [Google Scholar] [CrossRef]
- Bantchev, G.B.; Lorenzo-Martin, C.; Ajayi, O.O. Phosphonates from Lipids—Synthesis and Tribological Evaluation. In Conversion of Renewable Biomass into Bioproducts; ACS: Washington, DC, USA, 2021; pp. 139–156. [Google Scholar] [CrossRef]
- Chen, Y.; Biresaw, G.; Cermak, S.C.; Isbell, T.A.; Ngo, H.L.; Chen, L.; Durham, A.L. Fatty acid estolides: A review. J. Am. Oil Chem. Soc. 2020, 97, 231–241. [Google Scholar] [CrossRef]
- Bredsguard, J.W.; Thompson, T.D.; Cermak, S.C.; Isbell, T.A. Estolides: Bioderived synthetic base oils. In Environmentally Friendly and Biobased Lubricants, 1st ed.; Sharma, B.K., Biresaw, G., Eds.; CRC Press: Boca Raton, FL, USA, 2016; pp. 35–49. [Google Scholar] [CrossRef]
- Isbell, T.A.; Lowery, B.A.; Vermillion, K.; Cermak, S.C. Synthesis and characterization of polyethylene glycol diesters from estolides containing epoxides and diols. J. Am. Oil Chem. Soc. 2020, 97, 409–423. [Google Scholar] [CrossRef]
- Biresaw, G.; Chen, Y.; Chen, L.; Ngo, H.; Wagner, K.; Vermillion, K.E.; Cermak, S.C. Iso-oleic estolide products with superior cold flow properties. Ind. Crops Prod. 2022, 182, 114857. [Google Scholar] [CrossRef]
- Hoong, S.S.; Arniza, M.Z.; Mariam, N.M.; Armylisas, A.H.; Ishak, S.A.; Ismail, T.N.; Yeong, S.K. Synthesis of estolide ester and amide from acetylated polyhydroxy estolide for lubricant base oil. Eur. J. Lipid Sci. Technol. 2020, 122, 2000098. [Google Scholar] [CrossRef]
- Joshi, J.R.; Bhanderi, K.K.; Patel, J.V. A review on bio-lubricants from non-edible oils-recent advances, chemical modifications and applications. J. Indian Chem. Soc. 2023, 100, 100849. [Google Scholar] [CrossRef]
- Thakur, R.; Sanap, P.; Gogate, P.; Pratap, A. Ultrasound-assisted synthesis of oleic estolide: Optimization, process intensification and kinetic study. Chem. Eng. Process.-Process Intensif. 2023, 193, 109533. [Google Scholar] [CrossRef]
- Sanap, P.S.; Bhilpawar, O.V.; Patil, S.S.; Mestri, R.S. Synthesis of Polyol Esters of Estolide and Evaluation of Their Tribological Properties. Available SSRN 2024, 4760749. [Google Scholar] [CrossRef]
- Sanap, P.; Sonawane, D.; Patil, S.; Pratap, A. Optimization of oleic-estolide fatty acid synthesis using response surface methodology and artificial neural networks. Ind. Crops Prod. 2022, 188, 115711. [Google Scholar] [CrossRef]
- de Haro, J.C.; del Prado Garrido, M.; Pérez, Á.; Carmona, M.; Rodríguez, J.F. Full conversion of oleic acid to estolides esters, biodiesel and choline carboxylates in three easy steps. J. Clean. Prod. 2018, 184, 579–585. [Google Scholar] [CrossRef]
- Ewing, T.A.; Blaauw, R.; Li, C.; Venkitasubramanian, P.; Hagberg, E.; van Haveren, J. Synthesis and Applications of Fatty Acid Estolides. In Sustainable Green Chemistry in Polymer Research. Volume 1. Biocatalysis and Biobased Materials, 1st ed.; Cheng, H.N., Gross, R.A., Eds.; ACS: Washington, DC, USA, 2023; pp. 145–161. [Google Scholar] [CrossRef]
- Cermak, S.C.; Isbell, T.A. Synthesis of estolides from oleic and saturated fatty acids. J. Am. Oil Chem. Soc. 2001, 78, 557–565. [Google Scholar] [CrossRef]
- USDA BioPreferred Success Stories. Available online: https://www.biopreferred.gov/BioPreferred/faces/pages/SuccessStories.xhtml (accessed on 6 April 2024).
- Kania, D.; Yunus, R.; Omar, R.; Rashid, S.A.; Jan, B.M. A review of biolubricants in drilling fluids: Recent research, performance, and applications. J. Pet. Sci. Eng. 2015, 135, 177–184. [Google Scholar] [CrossRef]
- Stachowiak, G.W.; Batchelor, A.W. Engineering Tribology; Butterworth Heinemann: Woburn, MA, USA, 2001; p. 36. [Google Scholar]
- ASTM D97-17b; Standard Test Method for Pour Point of Petroleum Products. Annual Book of ASTM Standards (Vol. 05.01). ASTM International: West Conshohocken, PA, USA, 2022; pp. 106–112. [CrossRef]
- ASTM D2500-17a; Standard Test Method for Cloud Point of Petroleum Products and Liquid Fuels. Annual Book of ASTM Standards (Vol. 05.01). ASTM International: West Conshohocken, PA, USA, 2022; pp. 996–1001. [CrossRef]
- Ghosh, P.; Das, M. Study of the influence of some polymeric additives as viscosity index improvers and pour point depressants—Synthesis and characterization. J. Pet. Sci. Eng. 2014, 119, 79–84. [Google Scholar] [CrossRef]
- Chan, C.H.; Tang, S.W.; Mohd, N.K.; Lim, W.H.; Yeong, S.K.; Idris, Z. Tribological behavior of biolubricant base stocks and additives. Renew. Sustain. Energy Rev. 2018, 93, 145–157. [Google Scholar] [CrossRef]
- Chiu, C.W.; Schumacher, L.G.; Suppes, G.J. Impact of cold flow improvers on soybean biodiesel blend. Biomass Bioenergy 2004, 27, 485–491. [Google Scholar] [CrossRef]
- ASTM D5949-16; Standard Test Method for Pour Point of Petroleum Products (Automatic Pressure Pulsing Method). Annual Book of ASTM Standards (Vol. 05.021). ASTM International: West Conshohocken, PA, USA, 2022; pp. 1243–1248. [CrossRef]
- ASTM D5773-21; Standard Test Method for Cloud Point of Petroleum Products and Liquid Fuels (Constant Cooling Rate Method). Annual Book of ASTM Standards (Vol. 05.02). ASTM International: West Conshohocken, PA, USA, 2022; pp. 1114–1120. [CrossRef]
- Noel, F. Thermal analysis of lubricating oils. Thermochim. Acta. 1972, 4, 377–392. [Google Scholar] [CrossRef]
- Dunn, R.O. Thermal analysis of alternative diesel fuels from vegetable oils. J. Am. Oil Chem. Soc. 1999, 76, 109–115. [Google Scholar] [CrossRef]
- Leggieri, P.A.; Senra, M.; Soh, L. Cloud point and crystallization in fatty acid ethyl ester biodiesel mixtures with and without additives. Fuel 2018, 222, 243–249. [Google Scholar] [CrossRef]
- Govindapillai, A.; Jayadas, N.H.; Bhasi, M. Analysis of the pour point of coconut oil as a lubricant base stock using differential scanning calorimetry. Lubr. Sci. 2009, 21, 13–26. [Google Scholar] [CrossRef]
- Wang, S.L.; Flamberg, A.; Kikabhai, T. Select the optimum pour point depressant: Feedstocks and products: A special report. Hydrocarb. Process. 1999, 78, 59–62. Available online: https://search.ebscohost.com/login.aspx?direct=true&db=eih&AN=1639926&site=ehost-live (accessed on 5 April 2024).
- Rhee, I.S.; Velez, C.; Von Bernewitz, K. Evaluation of environmentally acceptable hydraulic fluids. In NLGI Spokesman-Including NLGI Annual Meeting-National Lubricating Grease Institute; National Lubricating Grease Institute: Kansas City, MO, USA, 1996; Volume 60, pp. 28–35. [Google Scholar]
- Adhvaryu, A.; Liu, Z.; Erhan, S.Z. Synthesis of novel alkoxylated triacylglycerols and their lubricant base oil properties. Ind. Crops Prod. 2005, 21, 113–119. [Google Scholar] [CrossRef]
- Paiva, F.L.; Marchesini, F.H.; Calado, V.M.; Galliez, A.P. Wax precipitation temperature measurements revisited: The role of the degree of sample confinement. Energy Fuels 2017, 31, 6862–6875. [Google Scholar] [CrossRef]
- Ngo, H.; Latona, R.; Wagner, K.M.; Nuñez, A.; Ashby, R.; Dunn, R.O. Synthesis and low temperature characterization of iso-oleic ester derivatives. Eur. J. Lipid Sci. Technol. 2016, 118, 1915–1925. [Google Scholar] [CrossRef]
- ASTM D7042-11a; Standard Test Method for Dynamic Viscosity and Density of Liquids by Stabinger Viscometer (and the Calculation of Kinematic Viscosity) Annual Book of ASTM Standards (Vol. 05.02). ASTM International: West Conshohocken, PA, USA, 2012; pp. 186–193. [CrossRef]
- Avramov, I.; Milchev, A. Effect of disorder on diffusion and viscosity in condensed systems. J. Non-Cryst. Solids 1988, 104, 253–260. [Google Scholar] [CrossRef]
- Mauro, J.C.; Yue, Y.; Ellison, A.J.; Gupta, P.K.; Allan, D.C. Viscosity of glass-forming liquids. Proc. Natl. Acad. Sci. USA 2009, 106, 19780–19784. [Google Scholar] [CrossRef] [PubMed]
- Nascimento, M.L.; Aparicio, C. Data classification with the Vogel–Fulcher–Tammann–Hesse viscosity equation using correspondence analysis. Phys. B 2007, 398, 71–77. [Google Scholar] [CrossRef]
- Bantchev, G.B.; Cermak, S.C. Correlating viscosity of 2-ethylhexyl oleic estolide esters to their molecular weight. Fuel 2022, 309, 122190. [Google Scholar] [CrossRef]
- Elbro, H.S.; Fredenslund, A.; Rasmussen, P. Group contribution method for the prediction of liquid densities as a function of temperature for solvents, oligomers, and polymers. Ind. Eng. Chem. Res. 1991, 30, 2576–2582. [Google Scholar] [CrossRef]
- van Krevelen, D.W. Properties of Polymers, 3rd ed.; Elsevier: Amsterdam, The Netherlands, 1990; pp. 227–229. [Google Scholar]
- Box, G.E.P.; Hunter, W.G.; Hunter, J.S. Statistics for Experimenters, 2nd ed.; John Wiley & Sins: New York, NY, USA, 1978; pp. 145–150. [Google Scholar]
- Haley, S.; Newcomb, T.; Vickerman, R. Transmissions and Transmission fluids. In Synthetics, Mineral Oils, and Bio-Based Lubricants, 3rd ed.; Rudnick, L.R., Ed.; CRC Press: Boca Raton, FL, USA, 2020; p. 547. [Google Scholar]
- Coutinho, J.A.; Daridon, J.L. The limitations of the cloud point measurement techniques and the influence of the oil composition on its detection. Pet. Sci. Technol. 2005, 23, 1113–1128. [Google Scholar] [CrossRef]
- Dunn, R. Cold flow properties of biodiesel by automatic and manual analysis methods. J. ASTM Int. 2010, 7, 1–5. [Google Scholar] [CrossRef]
- Wyatt, V.T.; Hess, M.A.; Dunn, R.O.; Foglia, T.A.; Haas, M.J.; Marmer, W.N. Fuel Properties and Nitrogen Oxide Emission Levels of Biodiesel Produced from Animal Fats. J. Am. Oil Chem. Soc. 2005, 82, 585–591. [Google Scholar] [CrossRef]
- Chiou, B.S.; El-Mashad, H.M.; Avena-Bustillos, R.J.; Dunn, R.O.; Bechtel, P.J.; McHugh, T.H.; Imam, S.H.; Glenn, G.M.; Orts, W.J.; Zhang, R. Biodiesel from Waste Salmon Oil. Trans. ASABE 2008, 51, 797–802. [Google Scholar] [CrossRef]
- Dunn, R.O. Cold Flow Properties of Soybean Oil Fatty Acid Monoalkyl Ester Admixtures. Energy Fuels 2009, 23, 4082–4091. [Google Scholar] [CrossRef]
- Ocko, B.M.; Wu, X.Z.; Sirota, E.B.; Sinha, S.K.; Gang, O.; Deutsch, M. Surface freezing in chain molecules: Normal alkanes. Phys. Rev. E 1997, 55, 3164. [Google Scholar] [CrossRef]
- Hammami, A.; Ratulowski, J.; Coutinho, J.A. Cloud points: Can we measure or model them? Pet. Sci. Technol. 2003, 21, 345–358. [Google Scholar] [CrossRef]
- Cholakova, D.; Denkov, N. Rotator phases in alkane systems: In bulk, surface layers and micro/nano-confinements. Adv. Colloid Interf. Sci. 2019, 269, 7–42. [Google Scholar] [CrossRef]
- Mortier, R.M.; Fox, M.F.; Orszulik, S.T. (Eds.) Chemistry and Technology of Lubricants, 3rd ed.; Springer: Dordrecht, The Netherlands, 2010. [Google Scholar]
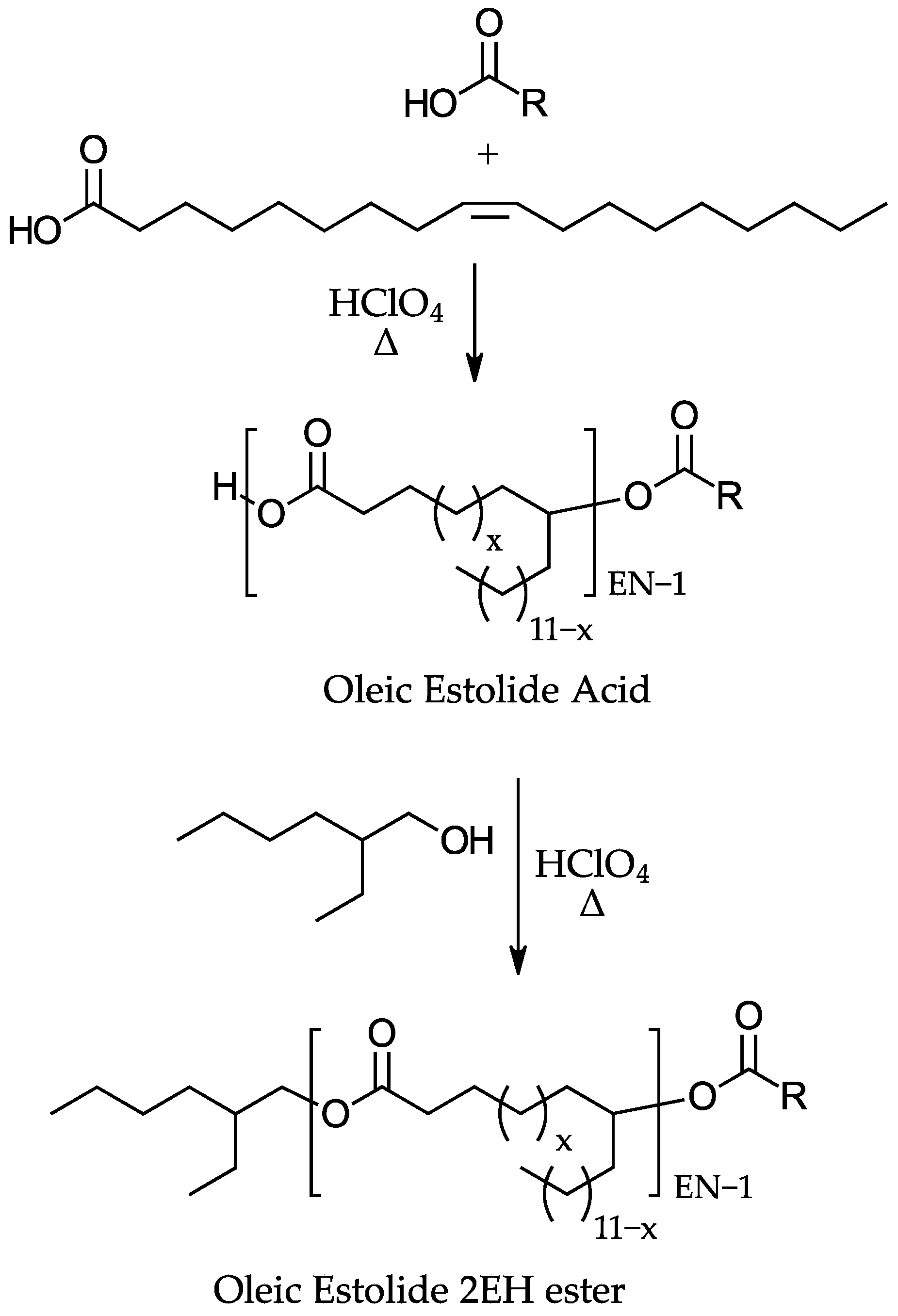
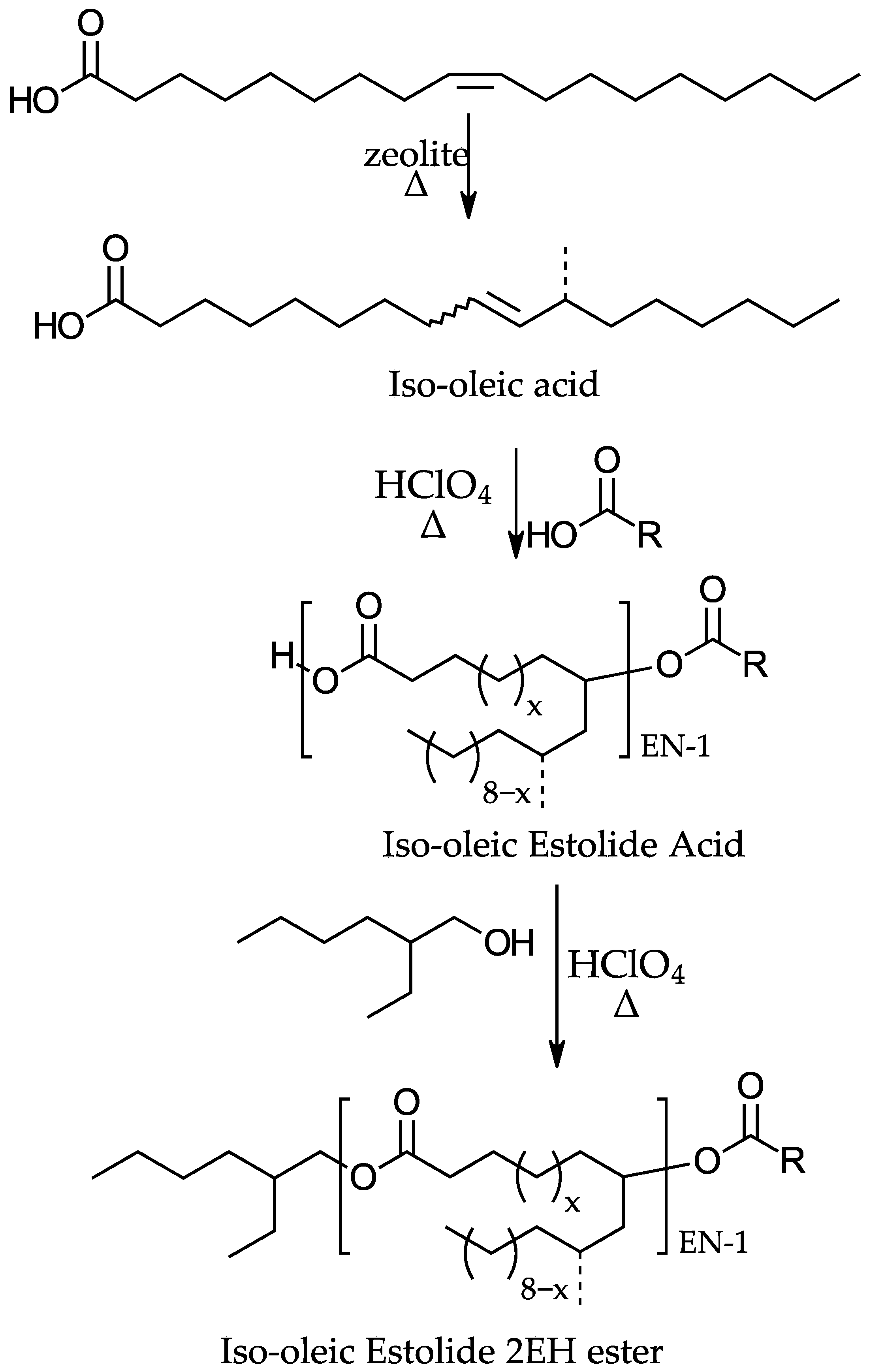





| # | Estolide | CP D5773-21 | PP D5949-16 | ΔCP | ΔPP |
|---|---|---|---|---|---|
| 1 | II | N/A 2 | −27.0 ± 0.0 | N/A | −5.0 |
| 2 | I-C8 | −51.2 ± 2.8 | −33.0 ± 0.0 | 16.4 | −5.0 |
| 3 | I-C10 | N/A | −13.5 ± 2.1 | N/A | −12.5 |
| 4 | I-C12 | −34.5 ± 0.0 | −30.0 ± 0.0 | 2.3 | −8.0 |
| 5 | I-Coco | −18.9 ± 0.7 | −27.0 ± 0.0 | 1.1 | −12.0 |
| 6 | I-C14 | −22.8 ± 0.1 | −21.0 ± 0.0 | 1.8 | −3.0 |
| 7 | I-C16 | −8.9 ± 0.1 | −9.0 ± 0.0 | 0.9 | 0.0 |
| 8 | I-C18 | −0.9 ± 0.2 | 0.0 ± 0.0 | 0.9 | −3.0 |
| 9 | OO | −34.7 ± 0.1 | −30 ± 0.0 | 2.4 | 0.0 |
| 10 | O-C8 | −41.7 ± 0.2 | −37.5 ± 2.1 | 2.0 | −9.2 |
| 11 | O-C10 | −35.4 ± 0.2 | −33.0 ± 0.0 | 1.4 | −1.5 |
| 12 | O-C12 | −31.1 ± 0.1 | −27.0 ± 0.0 | 1.9 | −5.0 |
| 13 | O-Coco | −8.4 ± 0.3 | −9.0 ± 0.0 | 4.1 | −3.0 |
| 14 | O-C14 | −17.9 ± 0.0 | −18.0 ± 0.0 | 1.9 | −1.8 |
| 15 | O-C16 | −8.9 ± 0.1 | −9.0 ± 0.0 | 1.9 | 0.0 |
| 16 | O-C18 | 8.3 ± 0.1 | 7.5 ± 2.1 | 2.0 | −1.5 |
| 17 | II-2EH | −70.1 ± 1.6 | −42.0 ± 0.0 | 28.8 | −11.0 |
| 18 | I-C8-2EH | −33.2 ± 1.1 | −45.0 ± 0.0 | 11.2 | −16.0 |
| 19 | I-C10-2EH | −55.3 ± 0.1 | −49.5 ± 2.1 | 19.6 | −10.5 |
| 20 | I-C12-2EH | −47.4 ± 0.3 | −45.0 ± 0.0 | 22.4 | −3.0 |
| 21 | I-Coco-2EH | −43.2 ± 0.1 | −42.0 ± 0.0 | 20.9 | −3.0 |
| 22 | I-C14-2EH | −32.2 ± 0.2 | −33.0 ± 0.0 | 2.9 | 0.0 |
| 23 | I-C16-2EH | −19.9 ± 0.1 | −22.5 ± 2.1 | 1.6 | −1.5 |
| 24 | I-C18-2EH | −11.1 ± 0.1 | −15.0 ± 0.0 | 1.1 | −5.0 |
| 25 | OO-2EH | −41.2 ± 0.0 | −39.0 ± 0.0 | 3.5 | −1.0 |
| 26 | O-C8-2EH | −47.3 ± 0.0 | −46.5 ± 2.1 | 24.3 | −4.5 |
| 27 | O-C10-2EH | −43.9 ± 0.1 | −43.5 ± 2.1 | 5.6 | 1.5 |
| 28 | O-C12-2EH | −31.5 ± 0.1 | −33.0 ± 0.0 | 2.5 | −3.0 |
| 29 | O-Coco-2EH | −35.0 ± 0.0 | −33.0 ± 0.0 | 22.0 | 0.0 |
| 30 | O-C14-2EH | −26.6 ± 0.1 | −27.0 ± 0.0 | 1.6 | 0.0 |
| 31 | O-C16-2EH | −17.9 ± 0.1 | −19.5 ± 2.1 | 2.9 | 1.5 |
| 32 | O-C18-2EH | −11.5 ± 0.1 | −13.5 ± 2.1 | 2.2 | 1.5 |
| 33 | I-Coco(17) 1 | −34.1 ± 0.3 | −30.0 ± 0.0 | 0.8 | −7.0 |
| 34 | I-Coco-2EH(17) 1 | −43.6 ± 0.1 | −42.0 ± 0.0 | 16.8 | −7.0 |
| # | Estolide | A 1 | B 1 | β 1 | η @D97 2 | η @D5949 2 |
|---|---|---|---|---|---|---|
| 1 | II | −1.3354 | 120.625 | 2.44909 | 3.1 × 105 | 1.5 × 105 |
| 2 | I-C8 | −1.3714 | 120.249 | 2.48942 | 4.2 × 105 | 2.0 × 105 |
| 3 | I-C10 | −1.3685 | 133.360 | 2.46777 | 4.1 × 105 | 8.0 × 104 |
| 4 | I-C12 | −1.3025 | 110.892 | 2.51037 | 1.2 × 105 | 4.1 × 104 |
| 5 | I-Coco | −1.4422 | 93.463 | 2.44228 | 2.9 × 104 | 7.4 × 103 |
| 6 | I-C14 | −1.2788 | 112.005 | 2.49754 | 2.6 × 104 | 1.9 × 104 |
| 7 | I-C16 | −1.3031 | 113.672 | 2.47245 | 8.0 × 103 | 8.0 × 103 |
| 8 | I-C18 | −1.3921 | 126.289 | 2.45442 | 1.5 × 104 | 1.1 × 104 |
| 9 | OO | −1.0688 | 105.020 | 2.40368 | 8.4 × 104 | 4.1 × 103 |
| 10 | O-C8 | −1.3253 | 102.086 | 2.44957 | 2.6 × 105 | 7.2 × 104 |
| 11 | O-C10 | −1.4102 | 99.826 | 2.42697 | 4.3 × 104 | 3.6 × 104 |
| 12 | O-C12 | −1.3390 | 101.374 | 2.42637 | 4.2 × 104 | 2.3 × 104 |
| 13 | O-Coco | −1.3877 | 96.290 | 2.41640 | 3.2 × 103 | 2.5 × 103 |
| 14 | O-C14 | −1.3513 | 99.189 | 2.42102 | 8.9 × 103 | 7.5 × 103 |
| 15 | O-C16 | −1.3590 | 97.412 | 2.41126 | 3.0 × 103 | 3.0 × 103 |
| 16 | O-C18 | −1.1424 | 109.987 | 2.52974 | 1.2 × 103 | 1.0 × 103 |
| 17 | II-2EH | −0.6125 | 124.540 | 2.82254 | 3.7 × 105 | 6.5 × 104 |
| 18 | I-C8-2EH | −0.7917 | 117.352 | 2.87283 | 3.4 × 105 | 2.6 × 104 |
| 19 | I-C10-2EH | −0.7661 | 119.492 | 2.86565 | 4.0 × 105 | 6.9 × 104 |
| 20 | I-C12-2EH | −0.7951 | 115.409 | 2.86214 | 3.7 × 104 | 2.4 × 104 |
| 21 | I-Coco-2EH | −0.7992 | 110.963 | 2.81811 | 2.3 × 104 | 1.6 × 104 |
| 22 | I-C14-2EH | −0.7670 | 115.659 | 2.86472 | 5.6 × 103 | 5.6 × 103 |
| 23 | I-C16-2EH | −0.7489 | 115.710 | 2.82070 | 3.2 × 103 | 2.7 × 103 |
| 24 | I-C18-2EH | −0.7663 | 116.903 | 2.79272 | 2.9 × 103 | 1.8 × 103 |
| 25 | OO-2EH | −0.5535 | 110.547 | 2.70694 | 4.1 × 104 | 3.6 × 104 |
| 26 | O-C8-2EH | −0.7871 | 108.930 | 2.78823 | 5.9 × 104 | 3.1 × 104 |
| 27 | O-C10-2EH | −0.8400 | 106.214 | 2.80580 | 1.1 × 104 | 1.3 × 104 |
| 28 | O-C12-2EH | −0.8592 | 103.273 | 2.80339 | 4.1 × 103 | 3.0 × 103 |
| 29 | O-Coco-2EH | −0.7918 | 106.959 | 2.78660 | 5.0 × 103 | 5.0 × 103 |
| 30 | O-C14-2EH | −0.8385 | 102.842 | 2.79330 | 1.8 × 103 | 1.8 × 103 |
| 31 | O-C16-2EH | −0.7878 | 104.983 | 2.77360 | 1.1 × 103 | 1.3 × 103 |
| 32 | O-C18-2EH | −0.7776 | 104.025 | 2.75936 | 7.2 × 102 | 8.1 × 102 |
| 33 | I-Coco(17) | −1.4220 | 96.128 | 2.42924 | 3.6 × 104 | 1.6 × 104 |
| 34 | I-Coco-2EH(17) | −0.8662 | 101.653 | 2.78441 | 1.9 × 104 | 8.1 × 103 |
| # | b (×104) | c (×107) | t0 | P | MW | 1 | ||
|---|---|---|---|---|---|---|---|---|
| 1 | 1.1187 | 7.97160 | 4.2313 | 62.31 | 1532 | 570 | 0.9547 | 43.5 |
| 2 | 1.1100 | 8.03781 | 4.5781 | 62.31 | 2193 | 826 | 0.9677 | 43.5 |
| 3 | 1.1055 | 7.78824 | 3.8748 | 62.31 | 1958 | 736 | 0.9556 | 41.8 |
| 4 | 1.1243 | 8.29619 | 4.9382 | 63.29 | 1491 | 558 | 0.9553 | 42.5 |
| 5 | 1.1243 | 8.56121 | 5.8704 | 61.05 | 1373 | 513 | 0.9534 | 42.5 |
| 6 | 1.1254 | 8.25076 | 4.7046 | 62.31 | 1512 | 564 | 0.9464 | 41.5 |
| 7 | 1.1255 | 8.19048 | 4.4426 | 62.31 | 1562 | 580 | 0.9371 | 40.4 |
| 8 | 1.1169 | 7.96174 | 3.7581 | 62.31 | 1544 | 572 | 0.9370 | 41.0 |
| 9 | 1.1206 | 8.06205 | 4.1153 | 62.31 | 2207 | 823 | N/A | N/A |
| 10 | 1.1142 | 8.25100 | 4.7752 | 62.31 | 2320 | 874 | 0.9692 | 43.8 |
| 11 | 1.1202 | 8.30260 | 4.8274 | 63.42 | 1876 | 705 | 0.9614 | 42.8 |
| 12 | 1.1214 | 8.27309 | 4.5125 | 62.31 | 1761 | 660 | 0.9547 | 42.2 |
| 13 | 1.1248 | 8.37190 | 4.9172 | 62.31 | 1365 | 510 | 0.9389 | 40.0 |
| 14 | 1.1273 | 8.34030 | 4.4361 | 62.31 | 1631 | 609 | 0.9431 | 40.8 |
| 15 | 1.1313 | 8.37134 | 4.4698 | 61.32 | 1539 | 572 | 0.9325 | 39.7 |
| 16 | 1.1327 | 8.47382 | 2.7545 | 61.32 | 1567 | 580 | 0.9199 | 38.1 |
| 17 | 1.1457 | 8.49367 | 4.9090 | 60.27 | 2242 | 828 | 0.9445 | 42.7 |
| 18 | 1.1528 | 8.81344 | 5.7650 | 62.31 | 1966 | 733 | 0.9450 | 41.4 |
| 19 | 1.1480 | 8.69534 | 5.5415 | 58.33 | 2151 | 800 | 0.9486 | 42.3 |
| 20 | 1.1548 | 8.82528 | 5.6352 | 62.31 | 1864 | 690 | 0.9434 | 42.2 |
| 21 | 1.1493 | 8.80091 | 5.5780 | 62.31 | 1850 | 684 | 0.9457 | 42.8 |
| 22 | 1.1559 | 8.81267 | 5.4968 | 61.32 | 1921 | 709 | 0.9322 | 40.6 |
| 23 | 1.1560 | 8.72107 | 5.2494 | 62.31 | 1777 | 653 | 0.9242 | 40.0 |
| 24 | 1.1546 | 8.63772 | 5.4454 | 62.31 | 1857 | 681 | 0.9193 | 39.5 |
| 25 | 1.1416 | 8.45149 | 4.8747 | 62.31 | 2557 | 947 | 0.9471 | 42.8 |
| 26 | 1.1437 | 8.71584 | 5.5041 | 62.31 | 2708 | 1012 | 0.9535 | 42.4 |
| 27 | 1.1500 | 8.83747 | 5.5966 | 62.31 | 2159 | 803 | 0.9466 | 41.9 |
| 28 | 1.1570 | 8.92684 | 5.6970 | 62.31 | 1841 | 681 | 0.9329 | 40.4 |
| 29 | 1.1493 | 8.75573 | 5.5001 | 62.31 | 2008 | 743 | 0.9383 | 41.3 |
| 30 | 1.1592 | 8.93221 | 5.6568 | 62.31 | 1697 | 625 | 0.9264 | 40.1 |
| 31 | 1.1570 | 8.80172 | 5.2380 | 62.31 | 1882 | 692 | 0.9217 | 39.4 |
| 32 | 1.1591 | 8.80713 | 5.1295 | 62.31 | 1872 | 686 | 0.9155 | 38.8 |
| 33 | 1.1242 | 8.48572 | 5.2812 | 62.31 | 1564 | 585 | 0.9562 | 42.8 |
| 34 | 1.1518 | 9.00052 | 5.9866 | 62.31 | 1839 | 680 | 0.9453 | 42.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bantchev, G.B.; Ngo, H.; Chen, Y.; Winfield, D.D.; Cermak, S.C. Cold-Flow Properties of Estolides: The Older (D97 and D2500) versus the Mini-(D5773 and D5949) Methods. Lubricants 2024, 12, 141. https://doi.org/10.3390/lubricants12050141
Bantchev GB, Ngo H, Chen Y, Winfield DD, Cermak SC. Cold-Flow Properties of Estolides: The Older (D97 and D2500) versus the Mini-(D5773 and D5949) Methods. Lubricants. 2024; 12(5):141. https://doi.org/10.3390/lubricants12050141
Chicago/Turabian StyleBantchev, Grigor B., Helen Ngo, Yunzhi Chen, DeMichael D. Winfield, and Steven C. Cermak. 2024. "Cold-Flow Properties of Estolides: The Older (D97 and D2500) versus the Mini-(D5773 and D5949) Methods" Lubricants 12, no. 5: 141. https://doi.org/10.3390/lubricants12050141
APA StyleBantchev, G. B., Ngo, H., Chen, Y., Winfield, D. D., & Cermak, S. C. (2024). Cold-Flow Properties of Estolides: The Older (D97 and D2500) versus the Mini-(D5773 and D5949) Methods. Lubricants, 12(5), 141. https://doi.org/10.3390/lubricants12050141





