Effects of Structure Parameters on Static Performance of Gas Foil Bearings Based on a New Fully Coupled Elastic–Aerodynamic Model
Abstract
1. Introduction
2. Theoretical Model
2.1. Lubrication Model
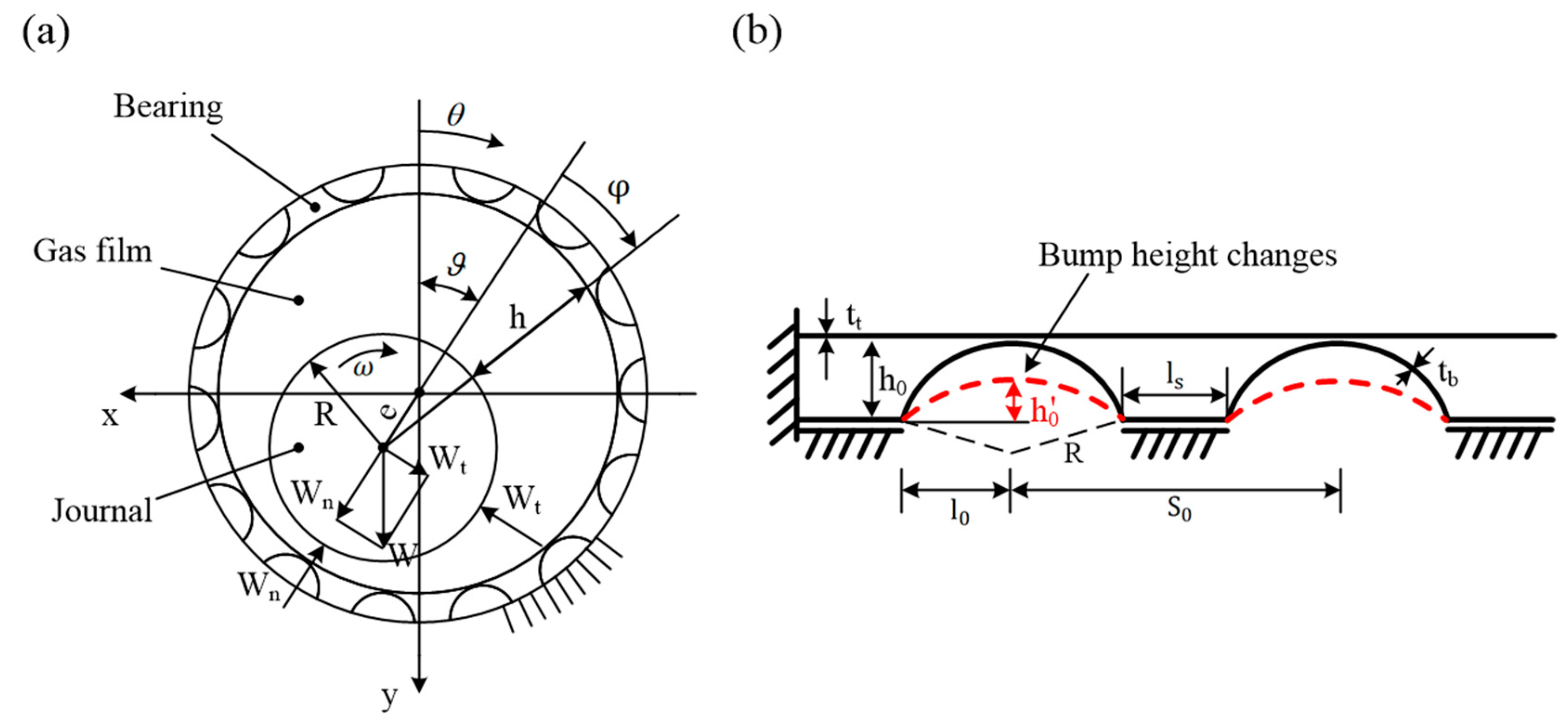
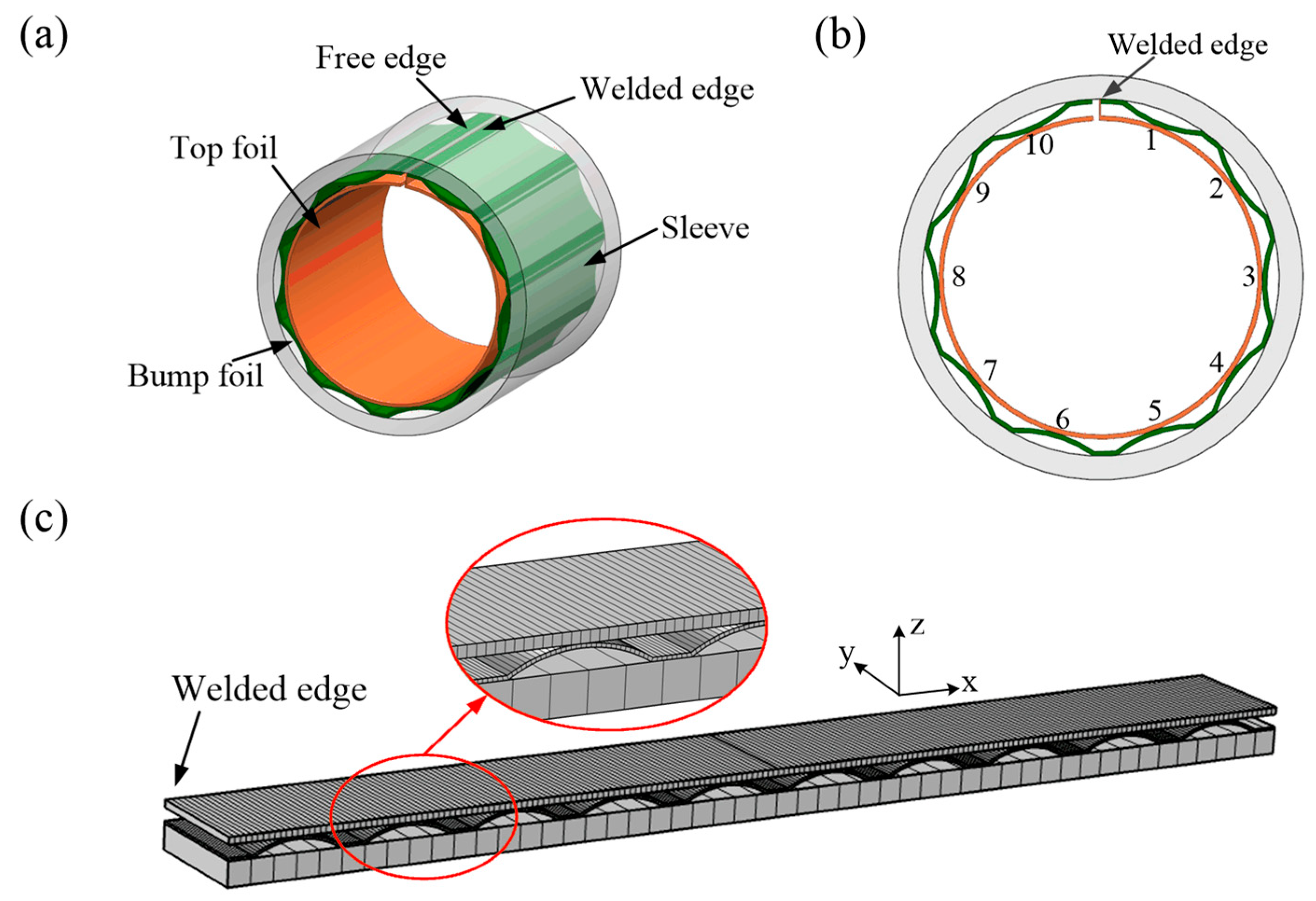
2.2. Contact Model
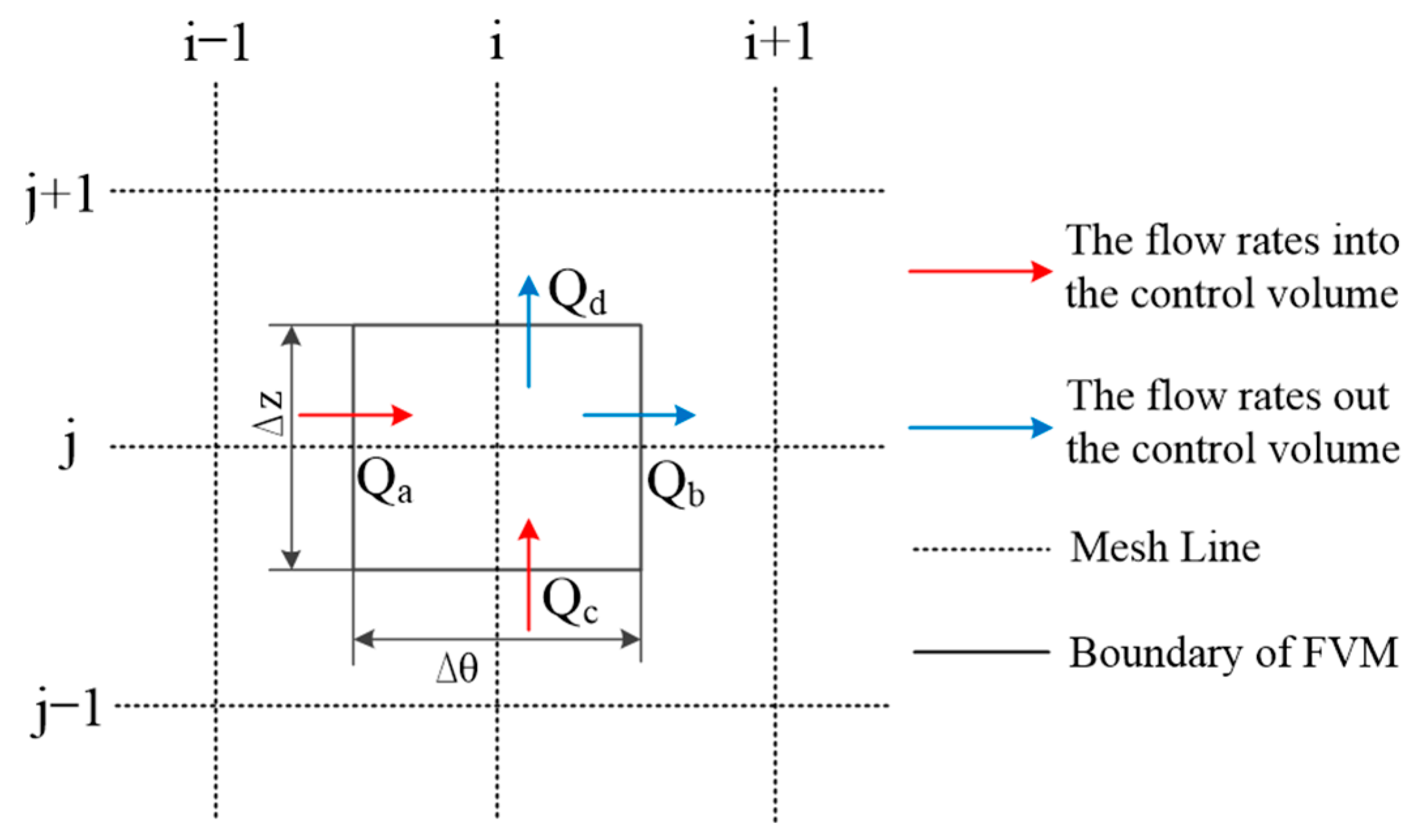
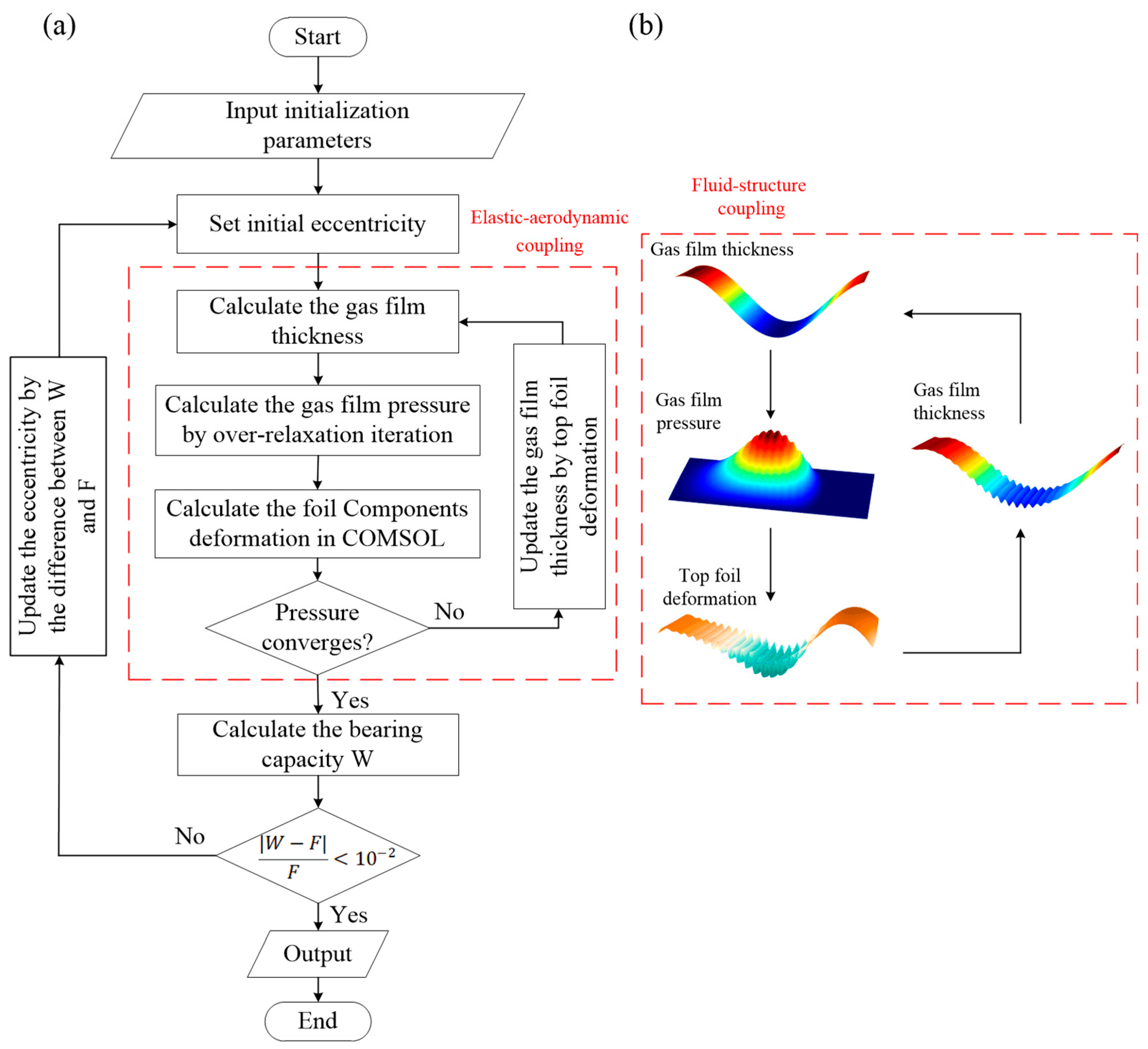
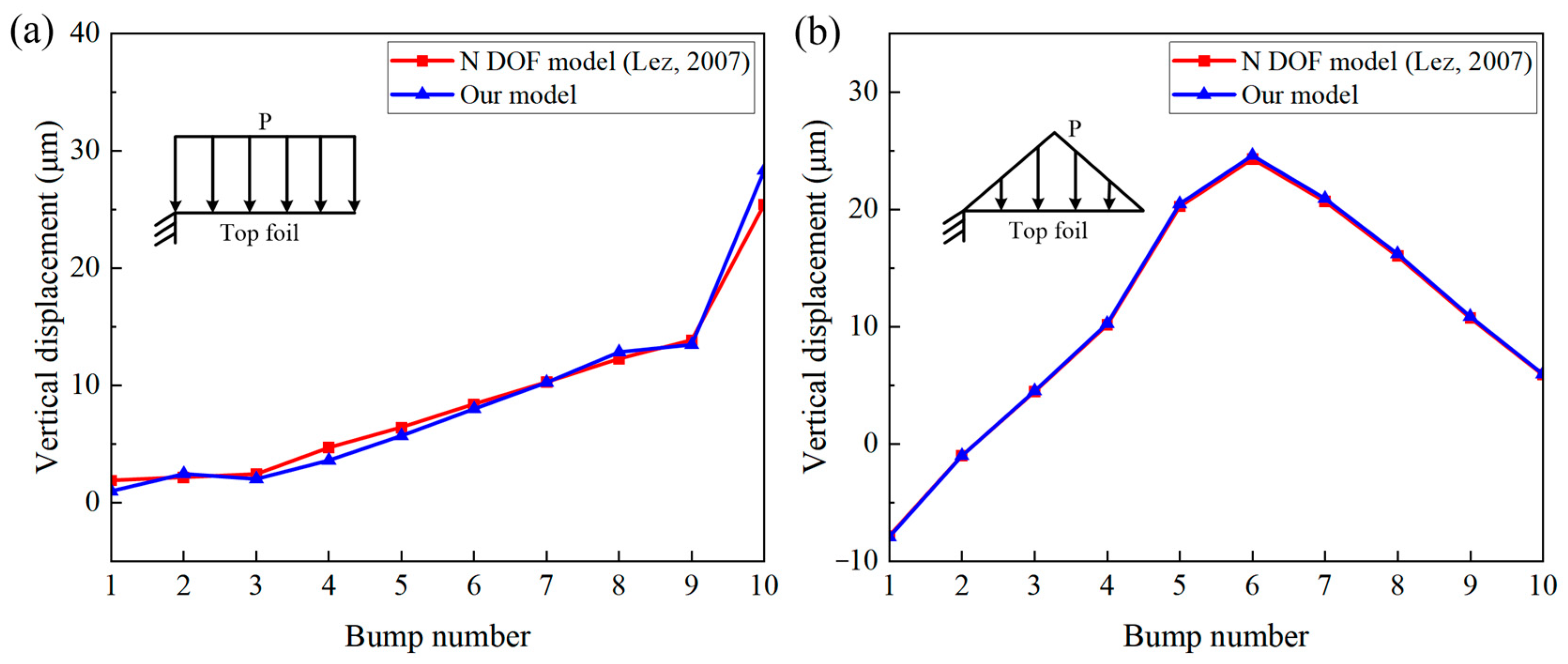
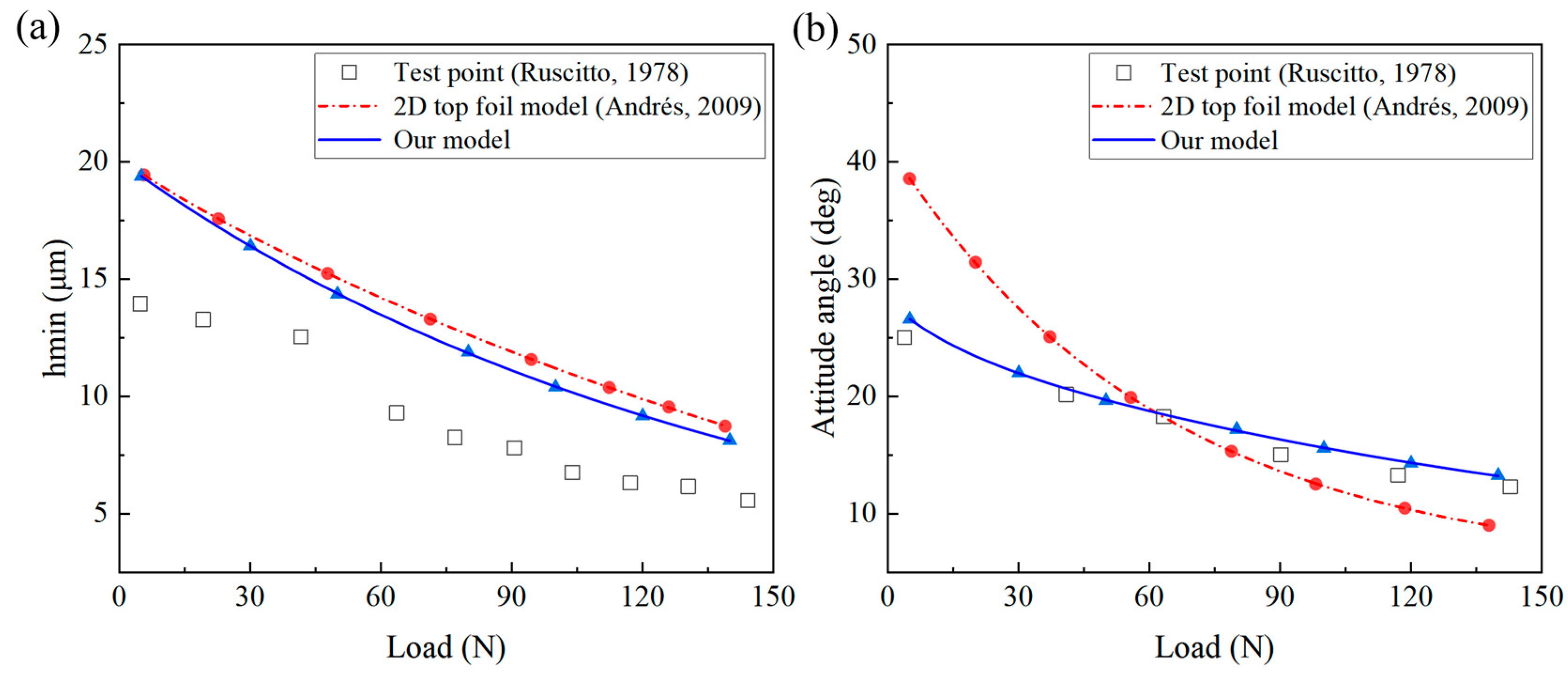
2.3. Numerical Solution
3. Results and Discussion
3.1. Static Stiffness Analysis
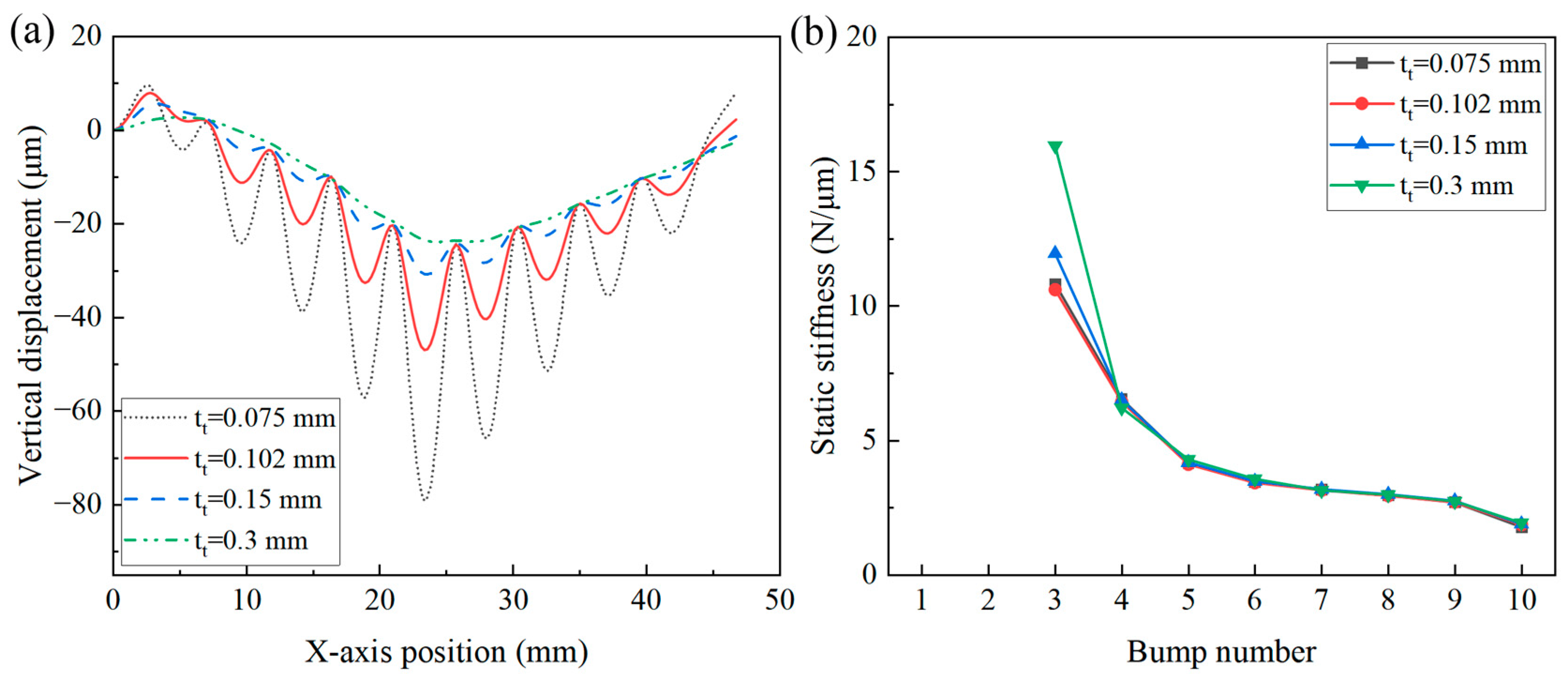
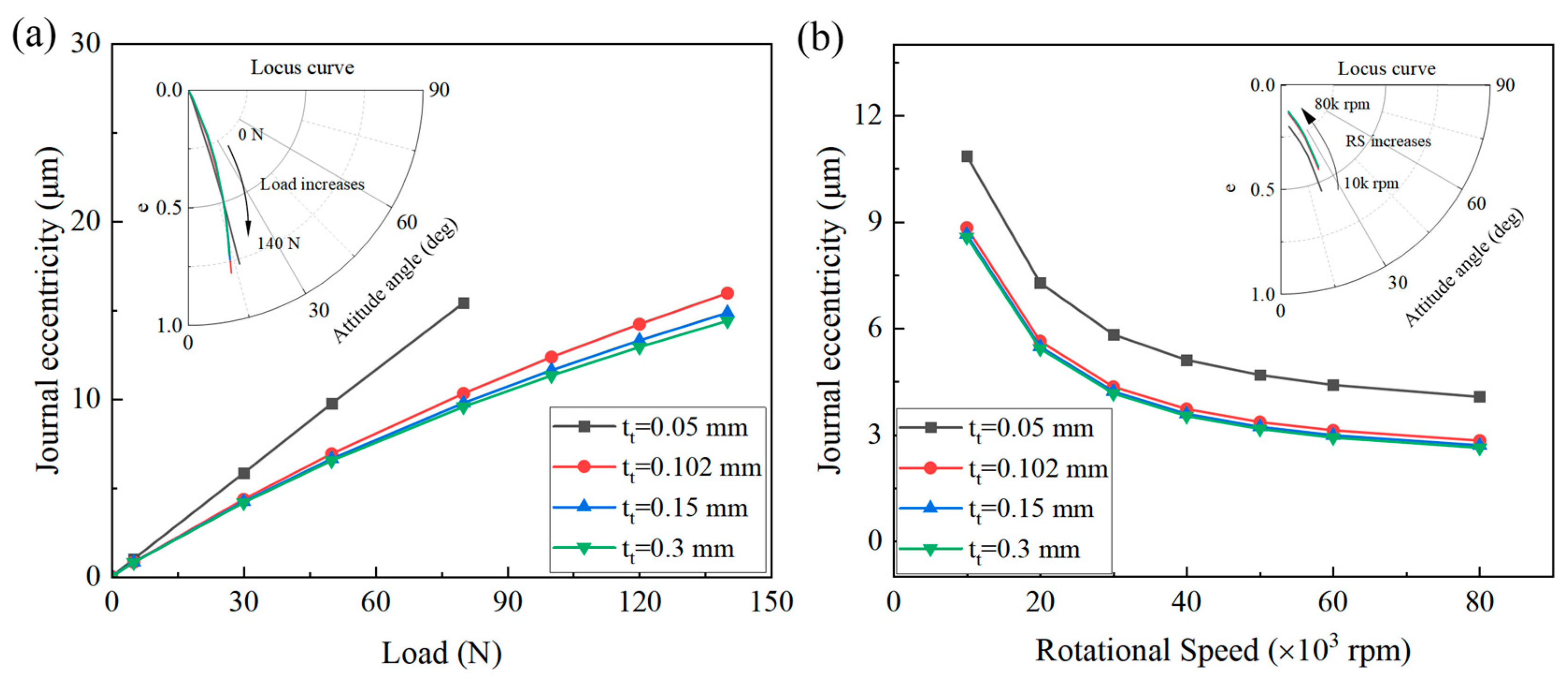
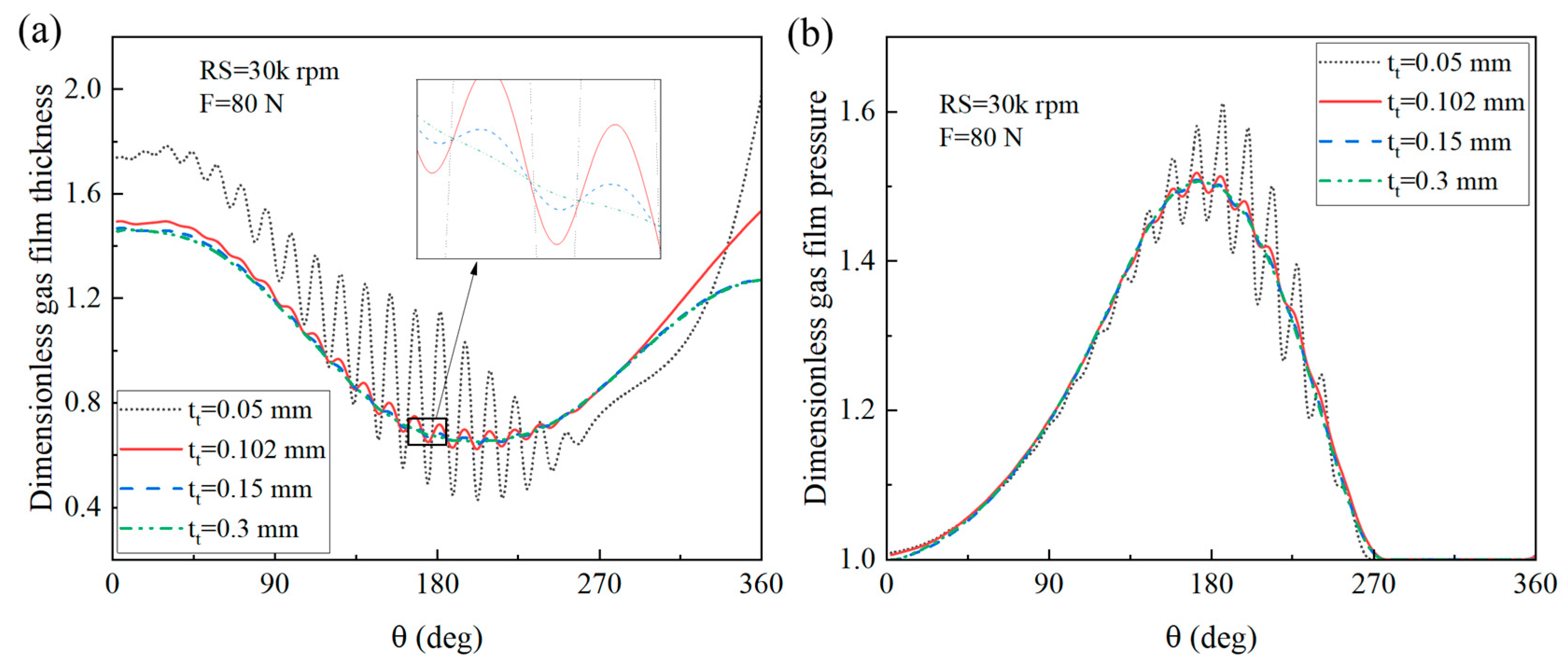
3.1.1. Effect of Top Foil Thickness on Static Stiffness
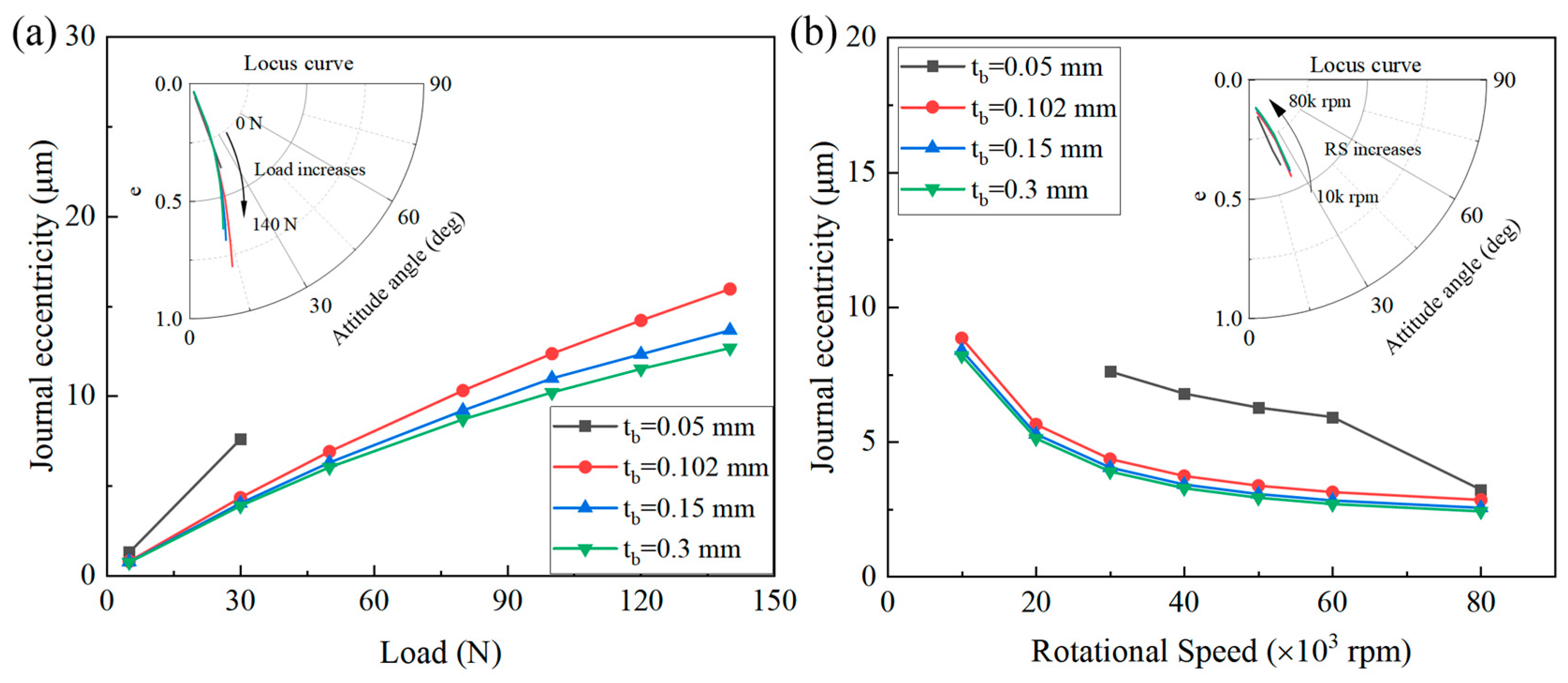
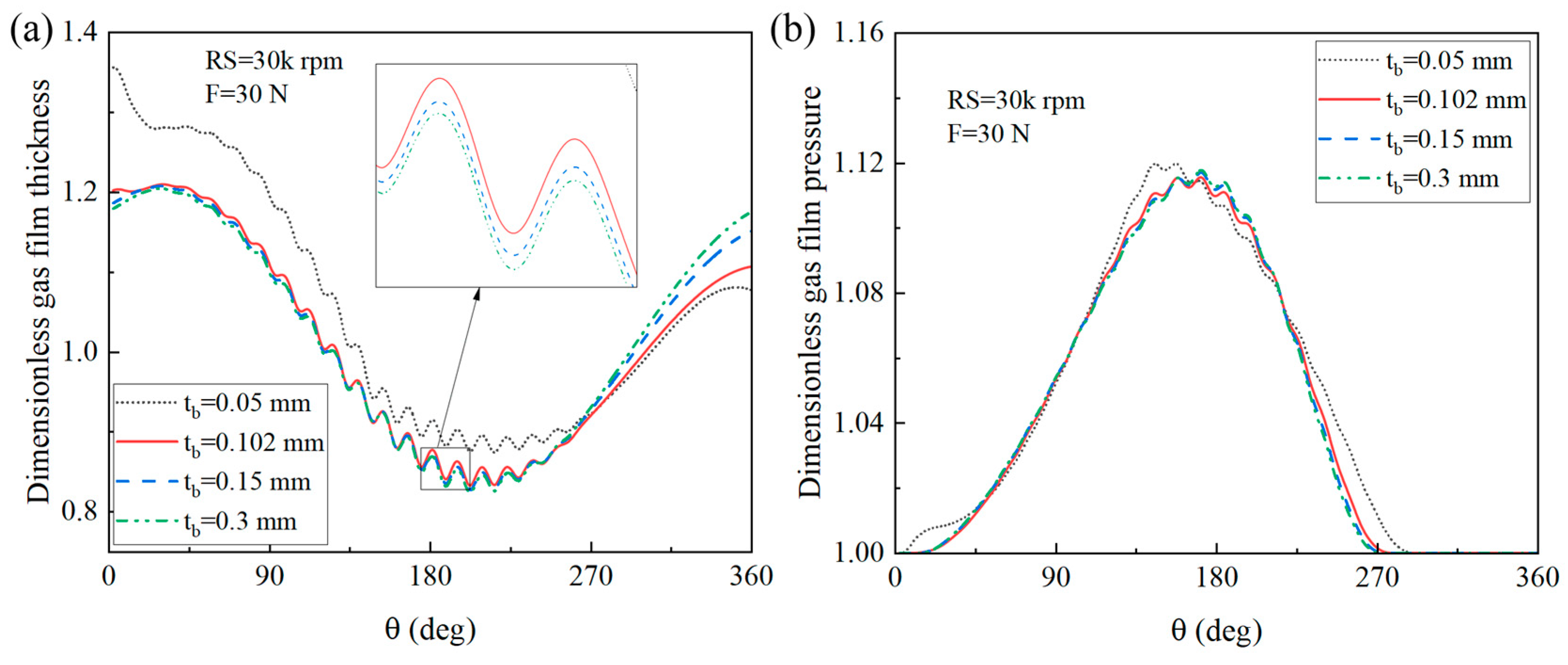
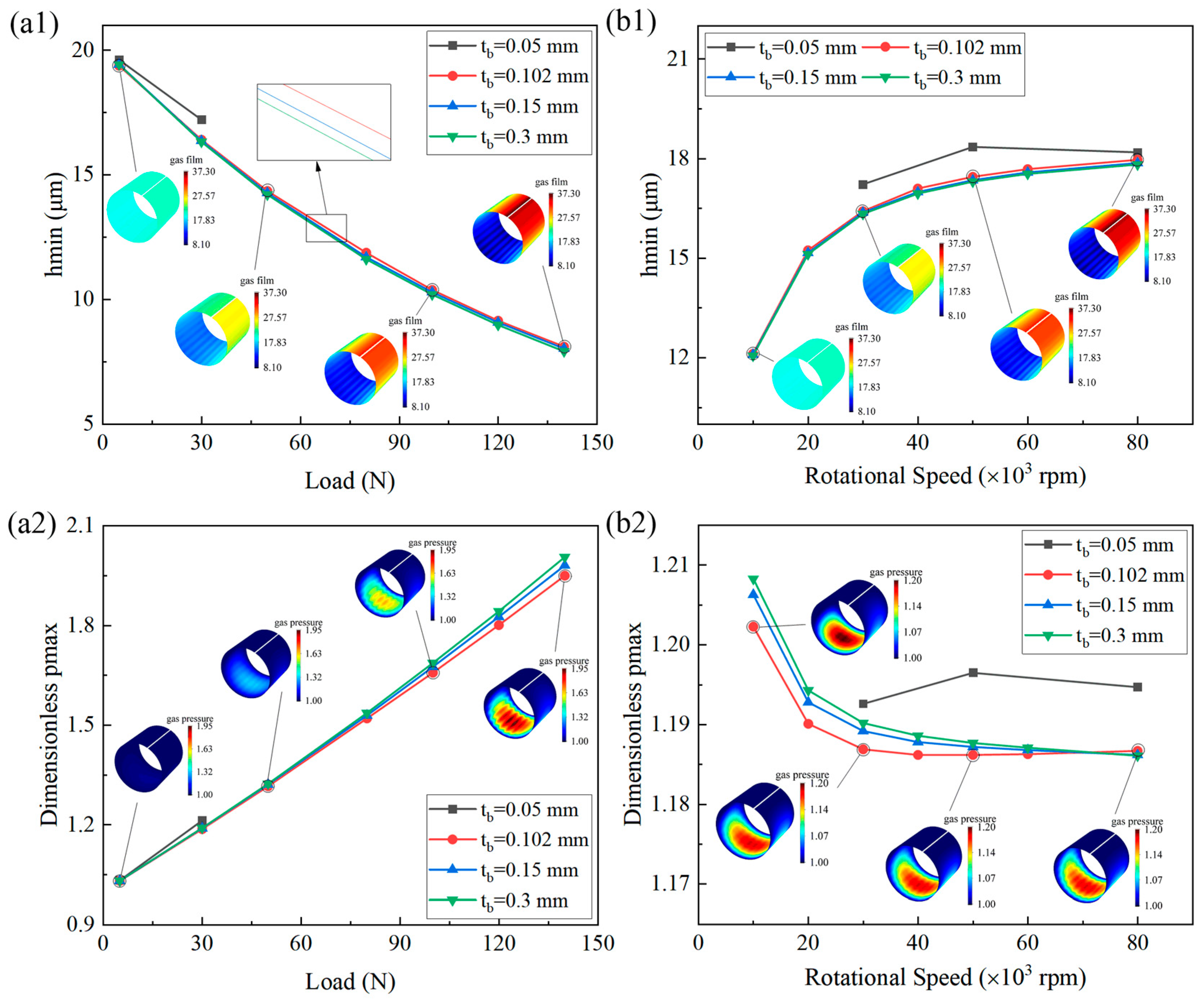
3.1.2. Effect of Bump Foil Thickness on Static Stiffness
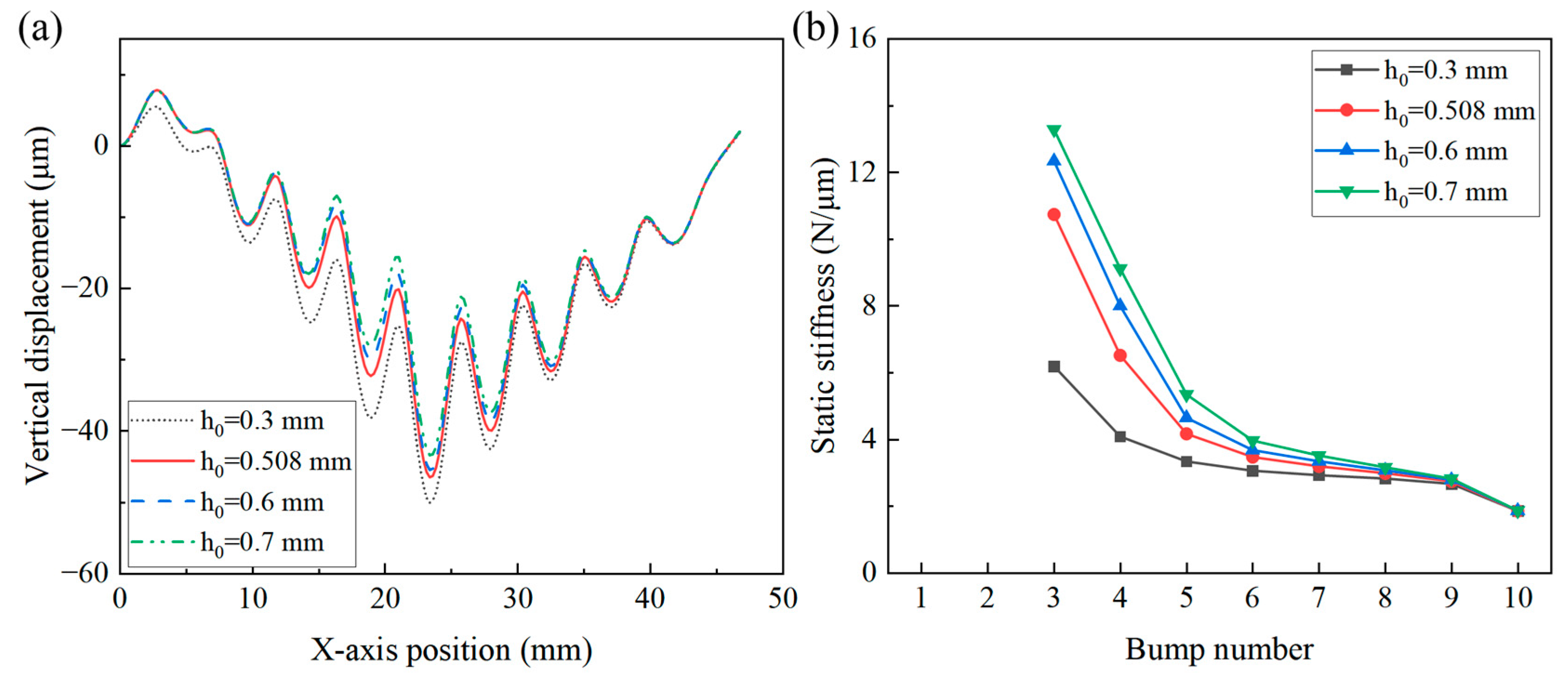
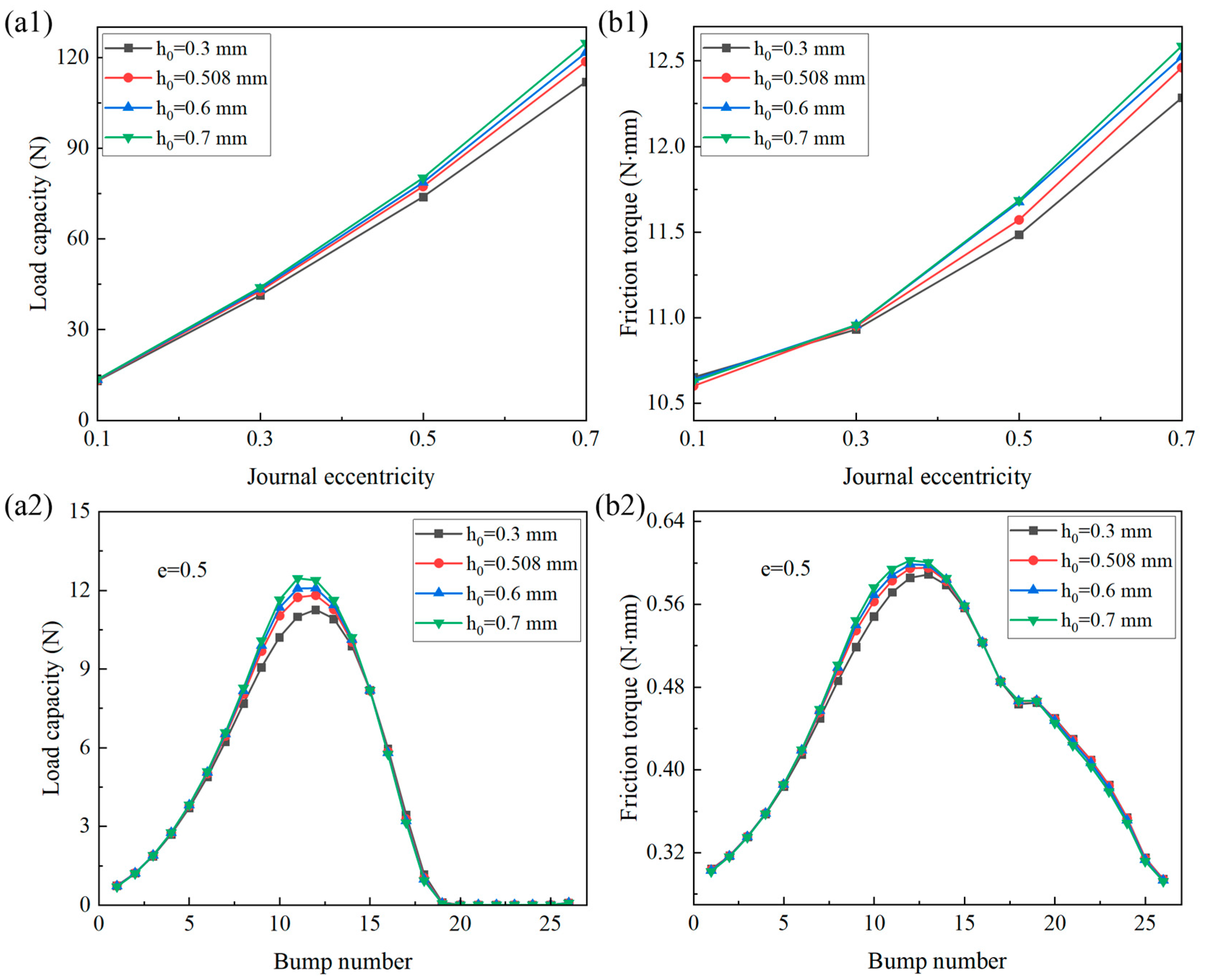
3.1.3. Effect of Bump Height on Static Stiffness
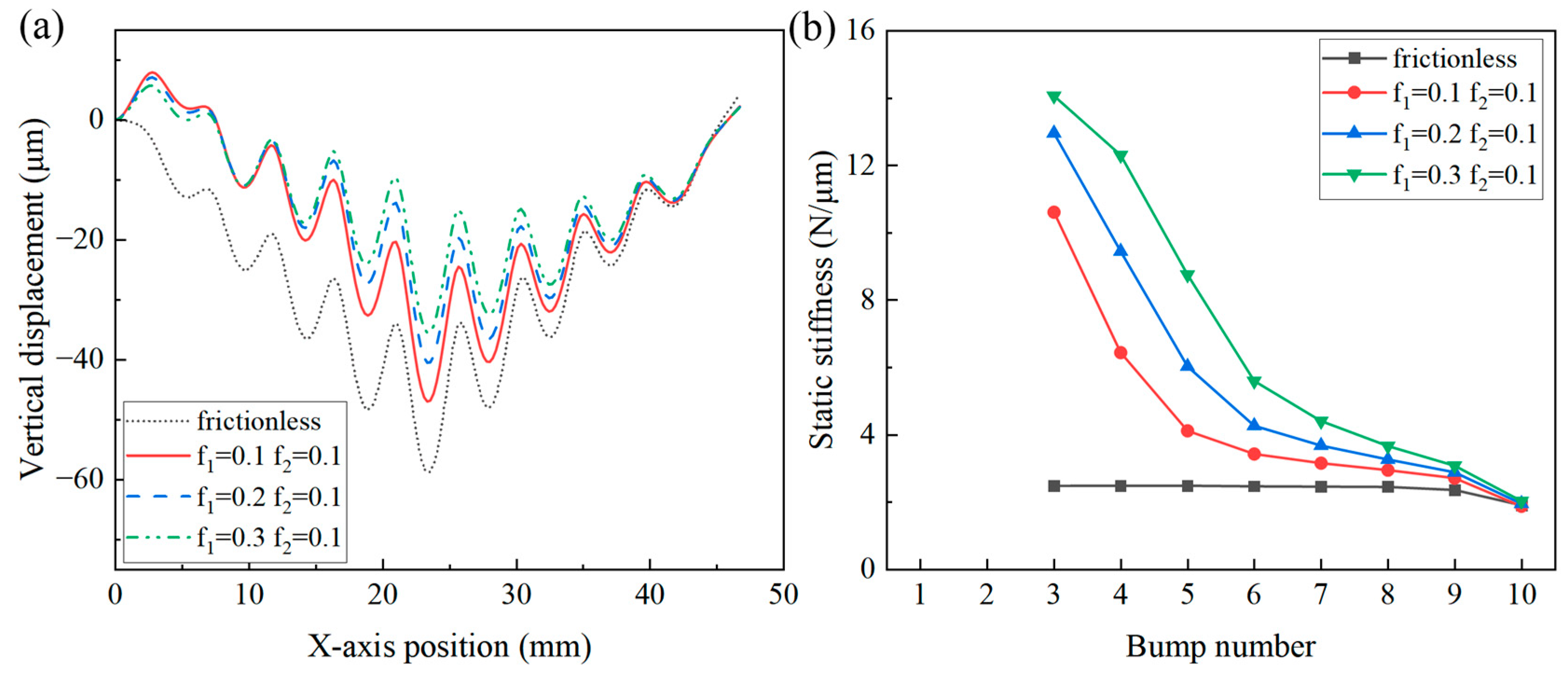
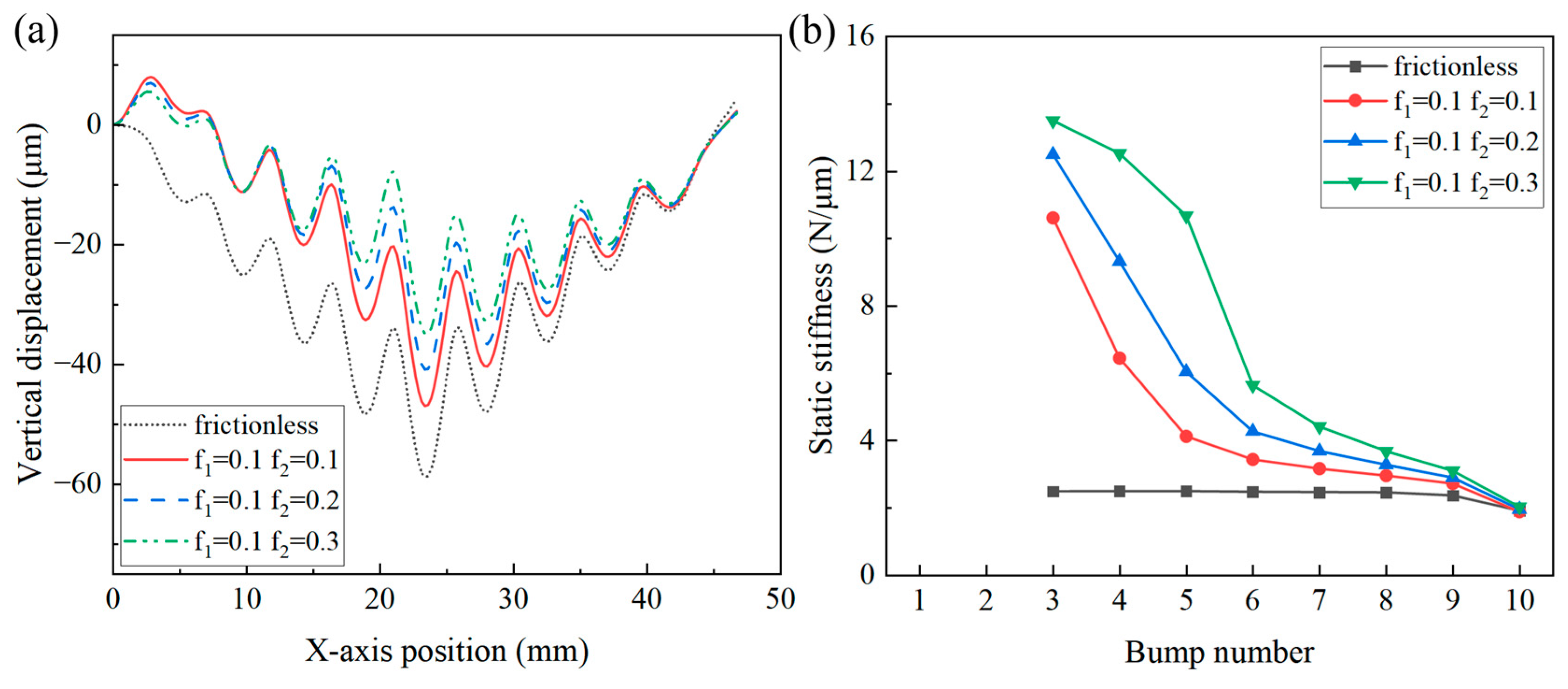
3.1.4. Effect of Friction Coefficient on Static Stiffness
3.2. Static Performance Analysis under Various Eccentricities
3.2.1. Effect of Top Foil Thickness on Static Performance under Various Eccentricities
3.2.2. Effect of Bump Foil Thickness on Static Performance under Various Eccentricities
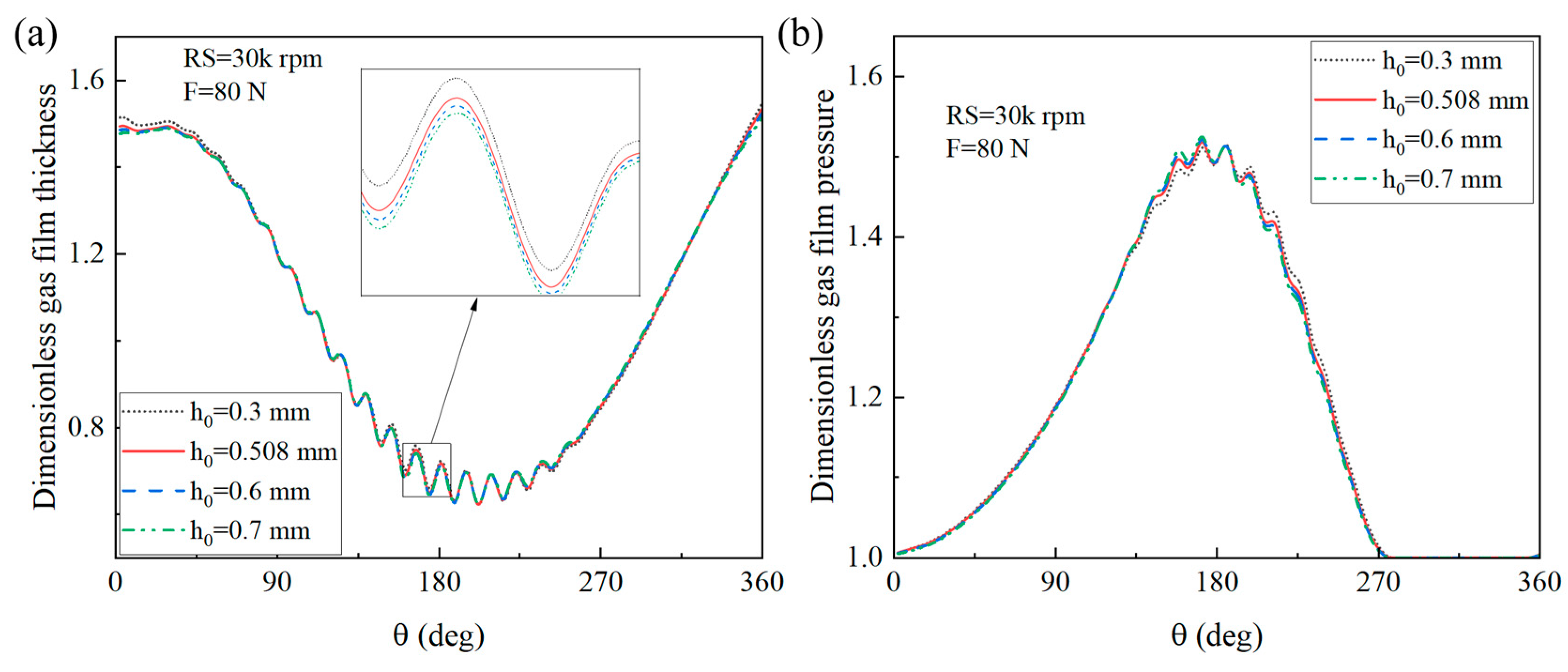
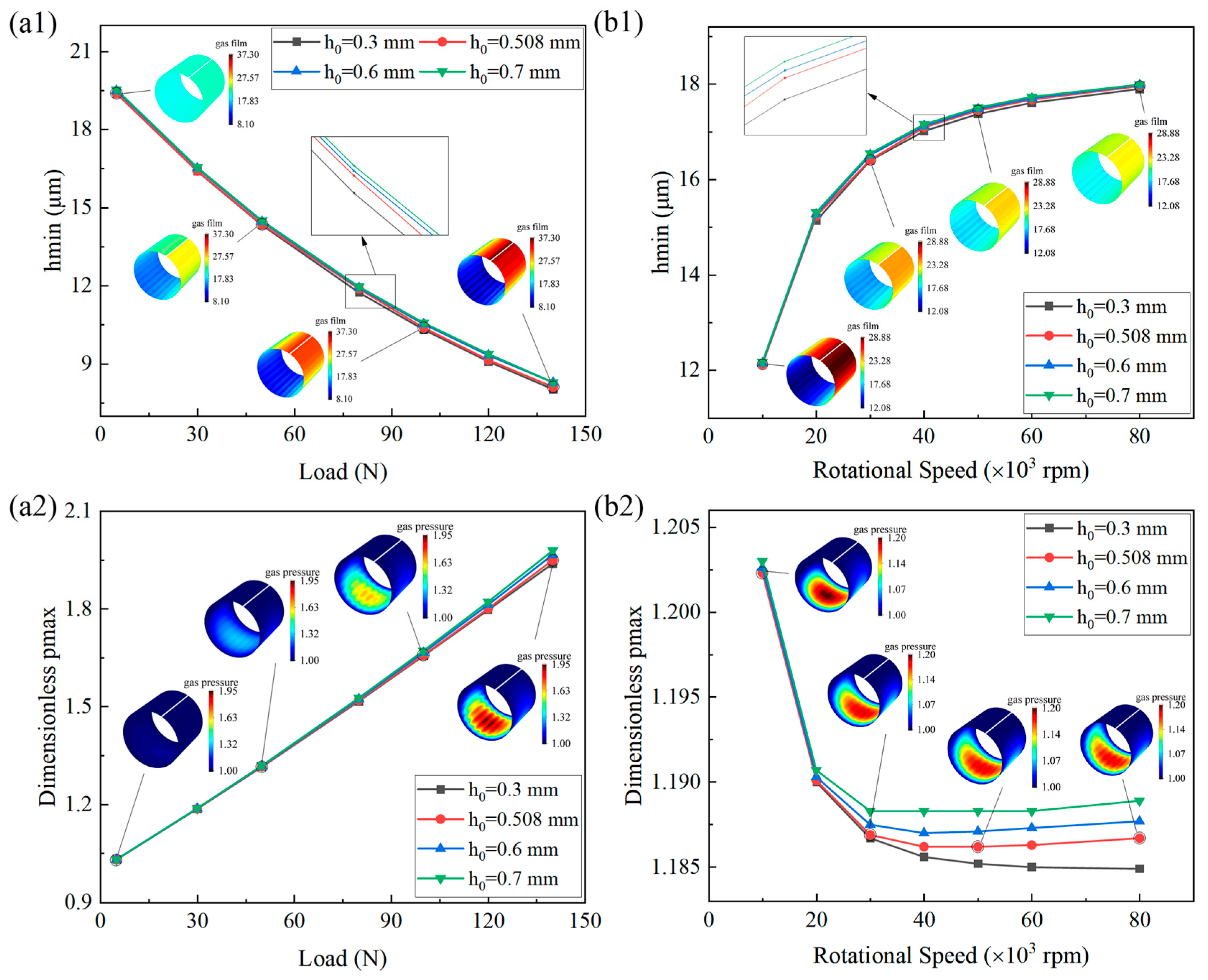
3.2.3. Effect of Bump Height on Static Performance under Various Eccentricities
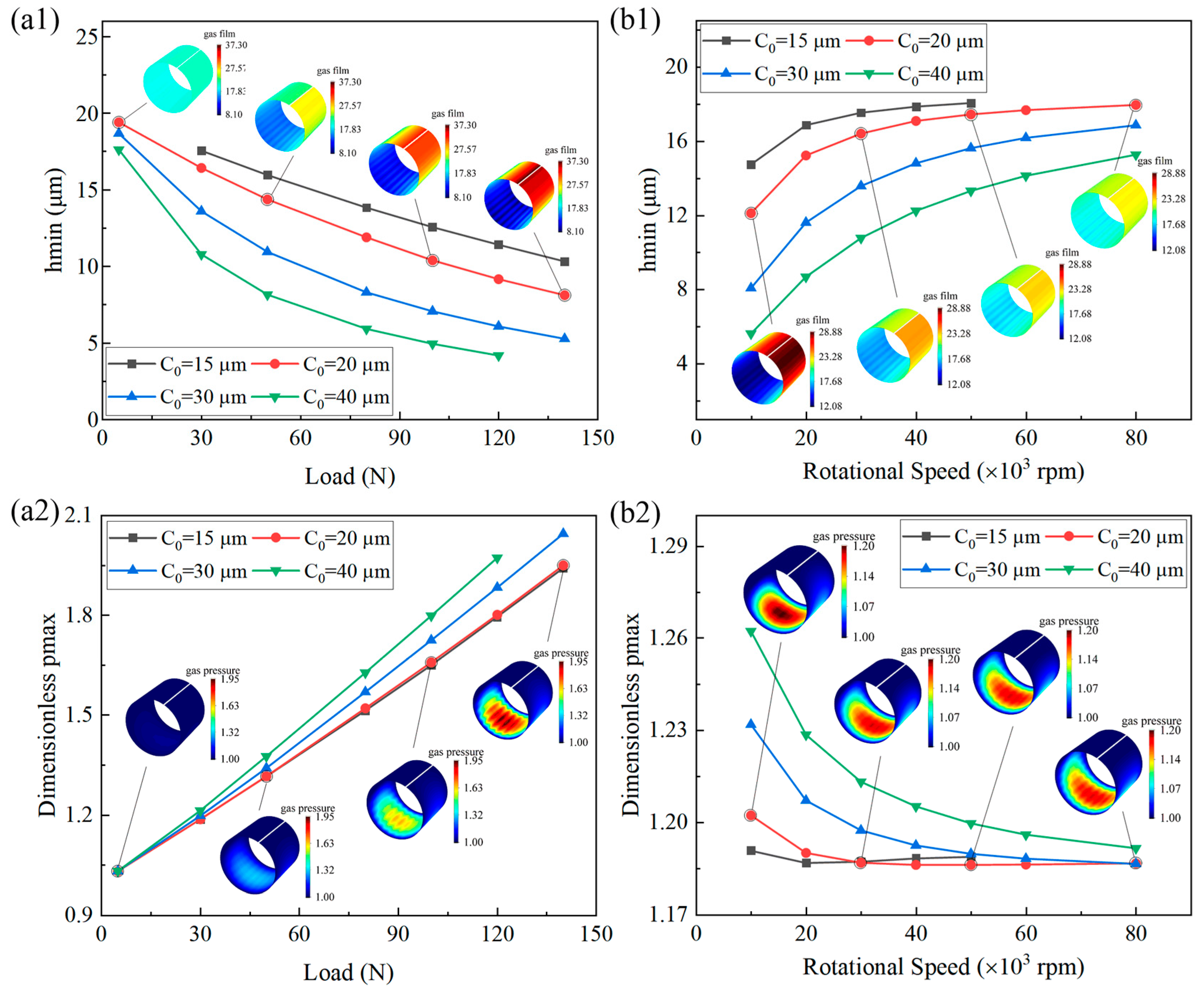
3.2.4. Effect of Radial Clearance on Static Performance under Various Eccentricities
3.3. Static Performance Analysis under Various Loads and Rotational Speeds
3.3.1. Effect of Top Foil Thickness on Static Performance under Various Loads and Rotational Speeds
3.3.2. Effect of Bump Foil Thickness on Static Performance under Various Loads and Rotational Speeds
3.3.3. Effect of Bump Height on Static Performance under Various Loads and Rotational Speeds
3.3.4. Effect of Radial Clearance on Static Performance under Various Loads and Rotational Speeds
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| gas film pressure, | Young’s modulus, | ||
| gas film thickness, | Poisson’s ratio | ||
| , | circumferential and axial positions, | air viscosity, | |
| ambient pressure, | radial component of load capacity, | ||
| radial clearance, | tangential component of load capacity, | ||
| dimensionless gas film pressure, | dimensionless radial component of load capacity, | ||
| dimensionless gas film thickness, | dimensionless tangential component of load capacity, | ||
| bearing radius, | load capacity, | ||
| bearing length, | friction torque, | ||
| top foil thickness, | dimensionless friction torque, | ||
| bump foil thickness, | axis of the coordinate | ||
| bump pitch, | eccentricity of the journal | ||
| half bump length, | rotational speed, | ||
| bump height, | external load, | ||
| attitude angle | top foil deformation | ||
| dimensionless circumferential and axial positions | original position of the material particle | ||
| new position of the material particle | deformation gradient of the material particle | ||
| pure rotation matrix | right extension tensor | ||
| elongation | three orthogonal directional components of | ||
| current length and original length of the material | Green–Lagrange strain tensor | ||
| matrices used in Equation (12) | relative velocity, | ||
| contact force, | friction coefficient | ||
| spring constant and its peak, | friction force, | ||
| flow rates per unit length in directions and | bearing number, | ||
| flow rates through the four faces of the control volume | coefficients used in Equation (18) | ||
| angular velocity of the journal, |
References
- Song, L.; Cheng, K.; Ding, H.; Chen, S. Analysis on discharge coefficients in FEM modeling of hybrid air journal bearings and experimental validation. Tribol. Int. 2018, 119, 549–558. [Google Scholar] [CrossRef]
- Lehn, A.; Mahner, M.; Schweizer, B. Elasto-gasdynamic modeling of air foil thrust bearings with a two-dimensional shell model for top and bump foil. Tribol. Int. 2016, 100, 48–59. [Google Scholar] [CrossRef]
- Pattnayak, M.; Ganai, P.; Pandey, R.; Dutt, J.; Fillon, M. An overview and assessment on aerodynamic journal bearings with important findings and scope for explorations. Tribol. Int. 2022, 174, 107778. [Google Scholar] [CrossRef]
- Samanta, P.; Murmu, N.; Khonsari, M. The evolution of foil bearing technology. Tribol. Int. 2019, 135, 305–323. [Google Scholar] [CrossRef]
- Walowit, J.A.; Anno, J.N.; Hamrock, B.J. Modern developments in lubrication mechanics. J. Lubr. Technol. 1977, 99, 304–305. [Google Scholar] [CrossRef]
- Heshmat, H.; Walowit, J.A.; Pinkus, O. Analysis of gas-lubricated foil journal bearings. J. Lubr. Technol. 1983, 105, 647–655. [Google Scholar] [CrossRef]
- Iordanoff, I. Analysis of an aerodynamic compliant foil thrust bearing: Method for a rapid design. J. Tribol. 1999, 121, 816–822. [Google Scholar] [CrossRef]
- Swanson, E.E. Bump foil damping using a simplified model. J. Tribol. 2006, 128, 542–550. [Google Scholar] [CrossRef][Green Version]
- Le Lez, S.; Arghir, M.; Frene, J. A new bump-type foil bearing structure analytical model. J. Eng. Gas Turbines Power 2007, 129, 1047–1057. [Google Scholar] [CrossRef]
- Gu, Y.; Ren, G.; Zhou, M. A fully coupled elastohydrodynamic model for static performance analysis of gas foil bearings. Tribol. Int. 2020, 147, 106297. [Google Scholar] [CrossRef]
- Gu, Y.; Lan, X.; Ren, G.; Zhou, M. An efficient three-dimensional foil structure model for bump-type gas foil bearings considering friction. Friction 2021, 9, 1450–1463. [Google Scholar] [CrossRef]
- Arghir, M.; Benchekroun, O. A simplified structural model of bump-type foil bearings based on contact mechanics including gaps and friction. Tribol. Int. 2019, 134, 129–144. [Google Scholar] [CrossRef]
- Nielsen, B.B.; Santos, I.F. Transient and steady state behaviour of elasto-aerodynamic air foil bearings, considering bump foil compliance and top foil inertia and flexibility: A numerical investigation. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2017, 231, 1235–1253. [Google Scholar] [CrossRef]
- Gad, A.M.; Kaneko, S. A New Structural Stiffness Model for Bump-Type Foil Bearings: Application to Generation II Gas Lubricated Foil Thrust Bearing. J. Tribol. 2014, 136, 041701. [Google Scholar] [CrossRef]
- Andre´s, L.S.; Kim, T.H. Improvements to the analysis of gas foil bearings: Integration of top foil 1D and 2D structural models. In Proceedings of the Turbo Expo 2007: Power for Land, Sea, and Air, Montreal, QC, Canada, 14–17 May 2007; pp. 779–789. [Google Scholar]
- Lee, Y.-B.; Park, D.-J.; Kim, C.-H.; Kim, S.-J. Operating characteristics of the bump foil journal bearings with top foil bending phenomenon and correlation among bump foils. Tribol. Int. 2008, 41, 221–233. [Google Scholar] [CrossRef]
- Feng, K.; Kaneko, S. Analytical model of bump-type foil bearings using a link-spring structure and a finite-element shell model. J. Tribol. 2010, 132, 021706. [Google Scholar] [CrossRef]
- Carpino, M.; Talmage, G. A fully coupled finite element formulation for elastically supported foil journal bearings. Tribol. Trans. 2003, 46, 560–565. [Google Scholar] [CrossRef]
- Sim, K.; Kim, T.H. Thermohydrodynamic analysis of bump-type gas foil bearings using bump thermal contact and inlet flow mixing models. Tribol. Int. 2012, 48, 137–148. [Google Scholar] [CrossRef]
- Feng, K.; Kaneko, S. A Thermohydrodynamic Sparse Mesh Model of Bump-Type Foil Bearings. J. Eng. Gas Turbines Power 2013, 135, 022501. [Google Scholar] [CrossRef]
- Lee, D.; Kim, D. Thermohydrodynamic Analyses of Bump Air Foil Bearings with Detailed Thermal Model of Foil Structures and Rotor. J. Tribol. 2010, 132, 021704. [Google Scholar] [CrossRef]
- Lehn, A.; Mahner, M.; Schweizer, B. A thermo-elasto-hydrodynamic model for air foil thrust bearings including self-induced convective cooling of the rotor disk and thermal runaway. Tribol. Int. 2018, 119, 281–298. [Google Scholar] [CrossRef]
- Qin, K.; Jacobs, P.A.; Keep, J.A.; Li, D.; Jahn, I.H. A fluid-structure-thermal model for bump-type foil thrust bearings. Tribol. Int. 2018, 121, 481–491. [Google Scholar] [CrossRef]
- Bin Hassan, M.; Bonello, P. A new modal-based approach for modelling the bump foil structure in the simultaneous solution of foil-air bearing rotor dynamic problems. J. Sound Vib. 2017, 396, 255–273. [Google Scholar] [CrossRef]
- Chakraborty, B.; Biswas, N.; Chakraborti, P. An Investigation to Find the Effects on Air Foil Bearing by the Variation of Foil’s Structural Parameters. J. Sci. Ind. Res. 2023, 82, 603–608. [Google Scholar] [CrossRef]
- Zywica, G.; Baginski, P.; Bogulicz, M.; Martowicz, A.; Roemer, J.; Kantor, S. Numerical identification of the dynamic characteristics of a nonlinear foil bearing structure: Effect of the excitation force amplitude and the assembly preload. J. Sound Vib. 2022, 520, 116663. [Google Scholar] [CrossRef]
- Fatu, A.; Arghir, M. Numerical Analysis of the Impact of Manufacturing Errors on the Structural Stiffness of Foil Bearings. J. Eng. Gas Turbines Power 2018, 140, 041506. [Google Scholar] [CrossRef]
- Liu, Z.-S.; Zhang, G.-H.; Xu, H.-J. Performance analysis of rotating externally pressurized air bearings. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2009, 223, 653–663. [Google Scholar] [CrossRef]
- Wang, X.; Xu, Q.; Wang, B.; Zhang, L.; Yang, H.; Peng, Z. Numerical calculation of rotation effects on hybrid air journal bearings. Tribol. Trans. 2017, 60, 195–207. [Google Scholar] [CrossRef]
- Howard, S.A. Preliminary development of characterization methods for compliant air bearings. Tribol. Trans. 1999, 42, 789–794. [Google Scholar] [CrossRef]
- Chiu, C.; Cheng, Y. Influences of operational conditions and geometric parameters on the stiffness of aerostatic journal bearings. Precis. Eng. 2010, 34, 722–734. [Google Scholar] [CrossRef]
- Lee, D.-H.; Kim, Y.-C.; Kim, K.-W. The static performance analysis of foil journal bearings considering three-dimensional shape of the foil structure. J. Tribol. 2008, 130, 031102. [Google Scholar] [CrossRef]
- Kim, D. Parametric studies on static and dynamic performance of air foil bearings with different top foil geometries and bump stiffness distributions. J. Tribol. 2007, 129, 354–364. [Google Scholar] [CrossRef]
- Larsen, J.S.; Hansen, A.J.T.; Santos, I.F. Experimental and theoretical analysis of a rigid rotor supported by air foil bearings. Mech. Ind. 2015, 16, 106. [Google Scholar] [CrossRef]
- Wang, X.; Xu, Q.; Wang, B.; Zhang, L.; Yang, H.; Peng, Z. Effect of surface waviness on the static performance of aerostatic journal bearings. Tribol. Int. 2016, 103, 394–405. [Google Scholar] [CrossRef]
- Dal, A.; Karaçay, T. Effects of angular misalignment on the performance of rotor-bearing systems supported by externally pressurized air bearing. Tribol. Int. 2017, 111, 276–288. [Google Scholar] [CrossRef]
- COMSOL. LiveLink™ for MATLAB® User’s Guide, Part Number: CM020008. Available online: https://doc.comsol.com/5.4/doc/com.comsol.help.llmatlab/LiveLinkForMATLABUsersGuide.pdf (accessed on 30 November 2022).
- Andrés, L.S.; Kim, T.H. Analysis of gas foil bearings integrating FE top foil models. Tribol. Int. 2009, 42, 111–120. [Google Scholar] [CrossRef]
- Ruscitto, D.; McCormick, J.; Gray, S. Hydrodynamic Air Lubricated Compliant Surface Bearing for an Automotive Gas Turbine Engine. 1: Journal Bearing Performance; CONS-9427-1; NASA-CR-135368; Mechanical Technology, Inc.: Latham, NY, USA, 1978. [Google Scholar] [CrossRef]








| Parameters | Values | Units |
|---|---|---|
| / | ||
| Number of Hexahedrons Divided | Degrees of Freedom | Computation Time | Error | |||
|---|---|---|---|---|---|---|
| Sleeve | Bump Foil | Top Foil | ||||
| Mesh 1 | 25 | 152 | 100 | 10,044 | 60 s | 5.02% |
| Mesh 2 | 40 | 242 | 160 | 15,984 | 95 s | 3.74% |
| Mesh 3 | 50 | 382 | 200 | 22,824 | 157 s | 2.90% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ding, P.; Hu, Y.; Wang, X.; Meng, Y. Effects of Structure Parameters on Static Performance of Gas Foil Bearings Based on a New Fully Coupled Elastic–Aerodynamic Model. Lubricants 2024, 12, 348. https://doi.org/10.3390/lubricants12100348
Ding P, Hu Y, Wang X, Meng Y. Effects of Structure Parameters on Static Performance of Gas Foil Bearings Based on a New Fully Coupled Elastic–Aerodynamic Model. Lubricants. 2024; 12(10):348. https://doi.org/10.3390/lubricants12100348
Chicago/Turabian StyleDing, Pengjing, Yang Hu, Xiaojing Wang, and Yonggang Meng. 2024. "Effects of Structure Parameters on Static Performance of Gas Foil Bearings Based on a New Fully Coupled Elastic–Aerodynamic Model" Lubricants 12, no. 10: 348. https://doi.org/10.3390/lubricants12100348
APA StyleDing, P., Hu, Y., Wang, X., & Meng, Y. (2024). Effects of Structure Parameters on Static Performance of Gas Foil Bearings Based on a New Fully Coupled Elastic–Aerodynamic Model. Lubricants, 12(10), 348. https://doi.org/10.3390/lubricants12100348





