A Mesoscopic Simulation Approach Based on Metal Fibre Characterization Data to Evaluate Brake Friction Performance
Abstract
:1. Introduction
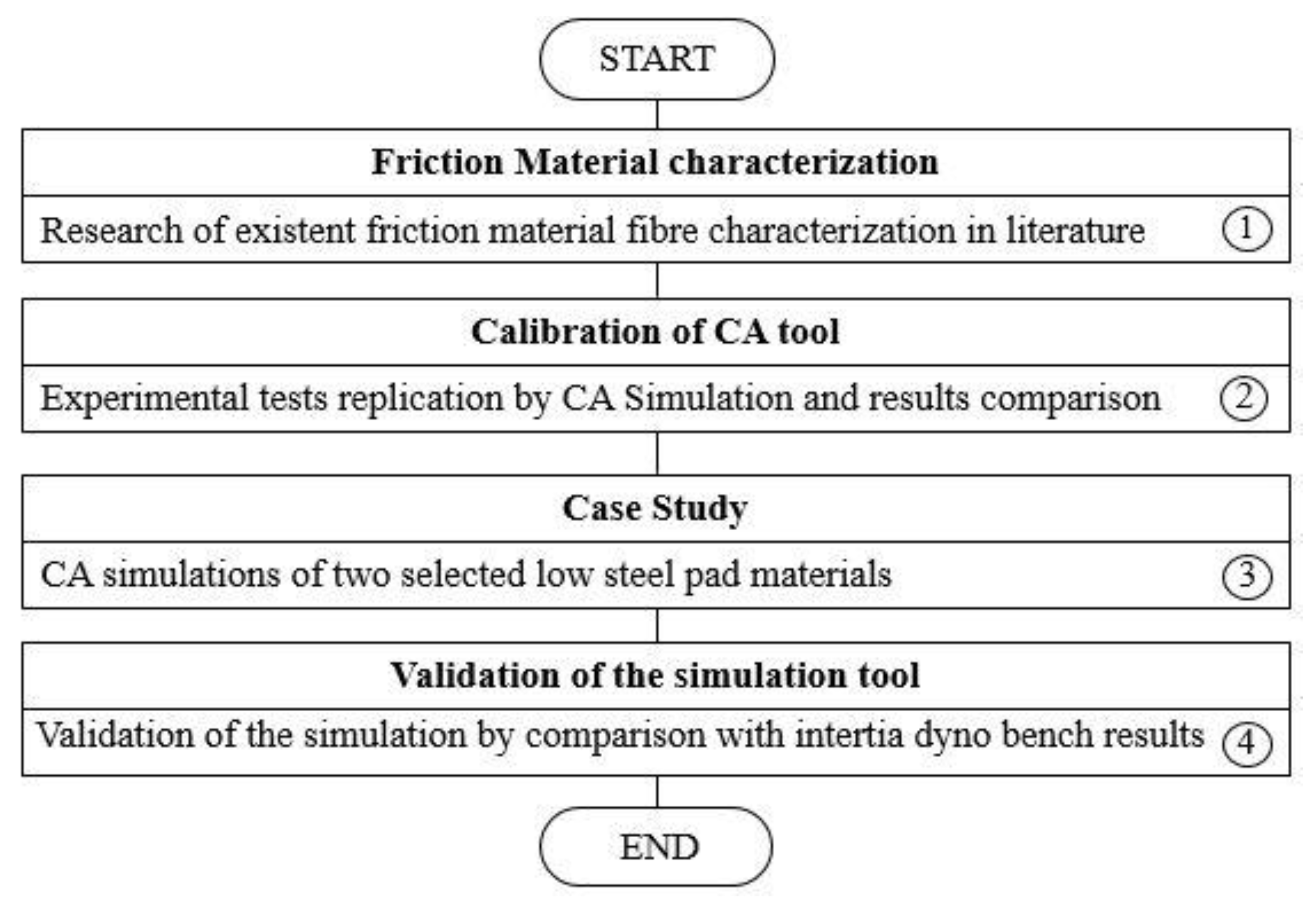
2. Simulation Approach
2.1. Input Friction Maps
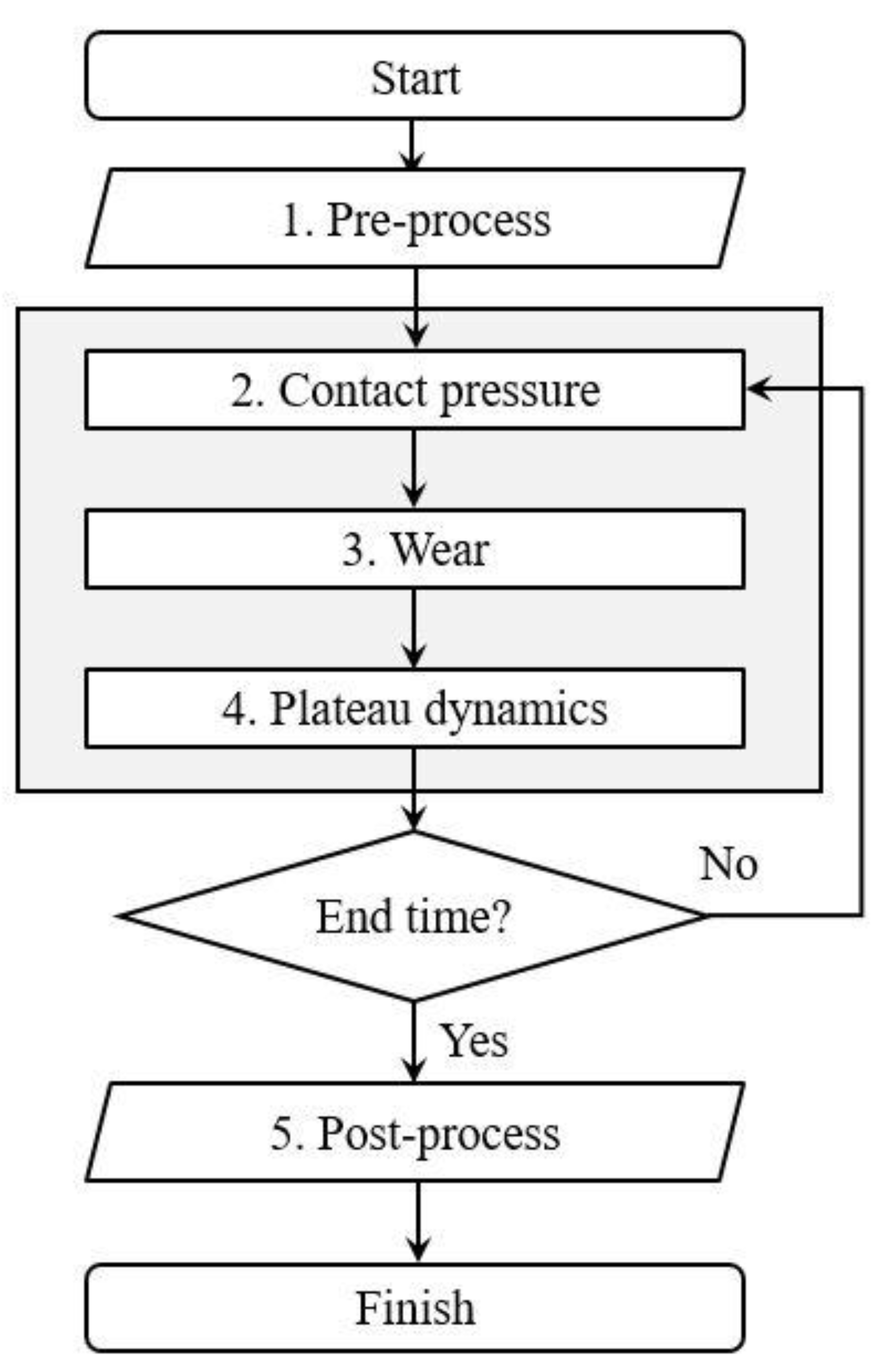
2.2. CA Simulation Tool
2.2.1. Pre-Process
- Disc material and pad fibres: primary plateau status;
- Pad resin: none status;
- Secondary plateaus on the pad and disc: secondary plateau status.
2.2.2. Contact Modelling
2.2.3. Wear Modelling
2.2.4. Plateau Dynamics Modelling
- has one neighbourhood cell having plateau status to east, or
- has one neighbourhood cell having plateau status to east, one to north-east or south- east, or
- has one neighbourhood cell having plateau status to east, one to north-east and one to south-east, and there is a sufficient amount of wear to fill the gap between pad and disc, it reaches the secondary plateau status.
- has no eastern neighbourhood cells
- wears down, it changes to none status
- there is no applied load on it.
- has its height less than the minimum distance between pad and disc
- wears down, it changes to none status.
2.2.5. Post-Process
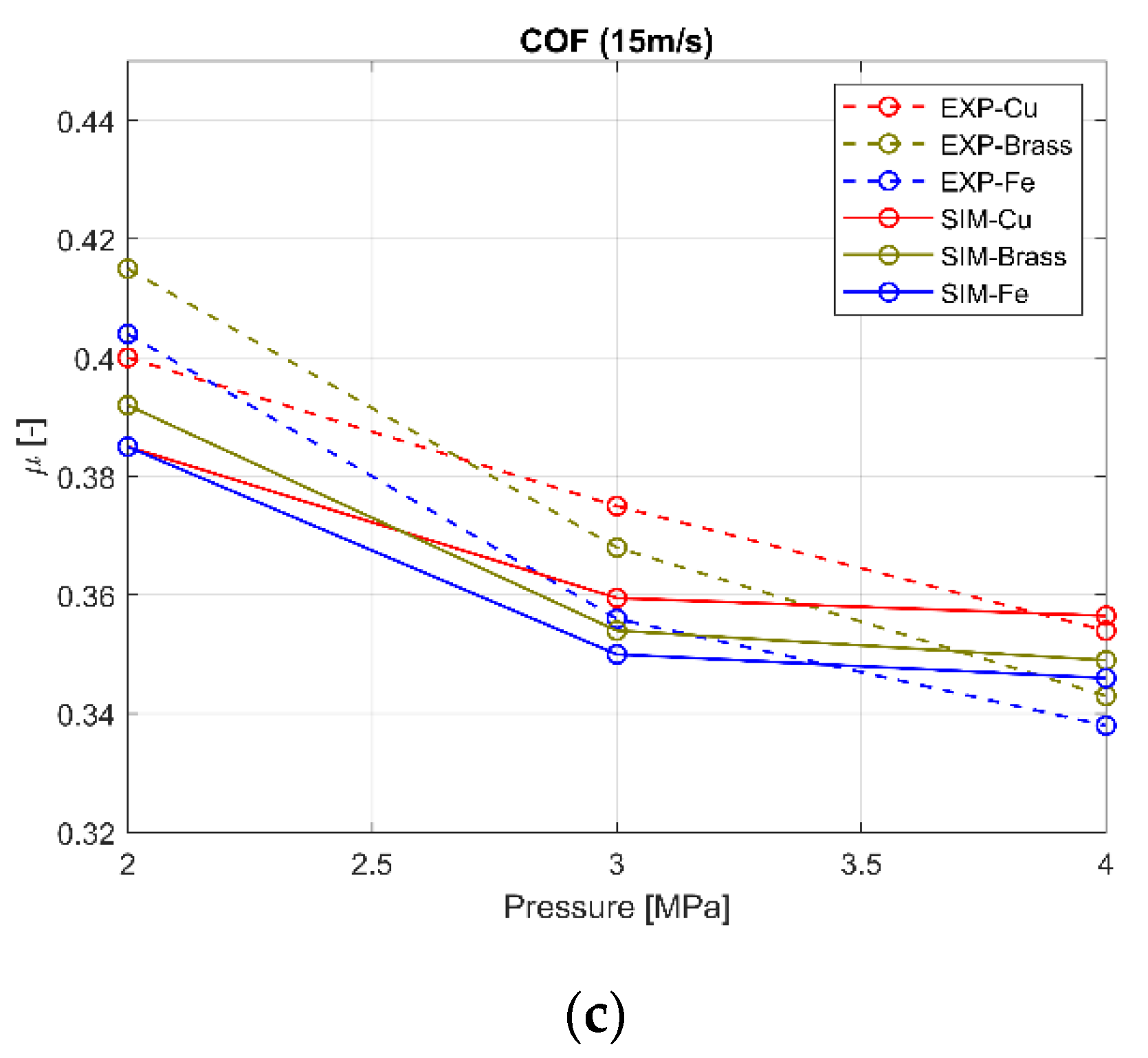
2.3. Calibration of CA Tool
3. Simulation Case Study
3.1. Material and Wear Parameters
3.2. Geometry and Load Parameters
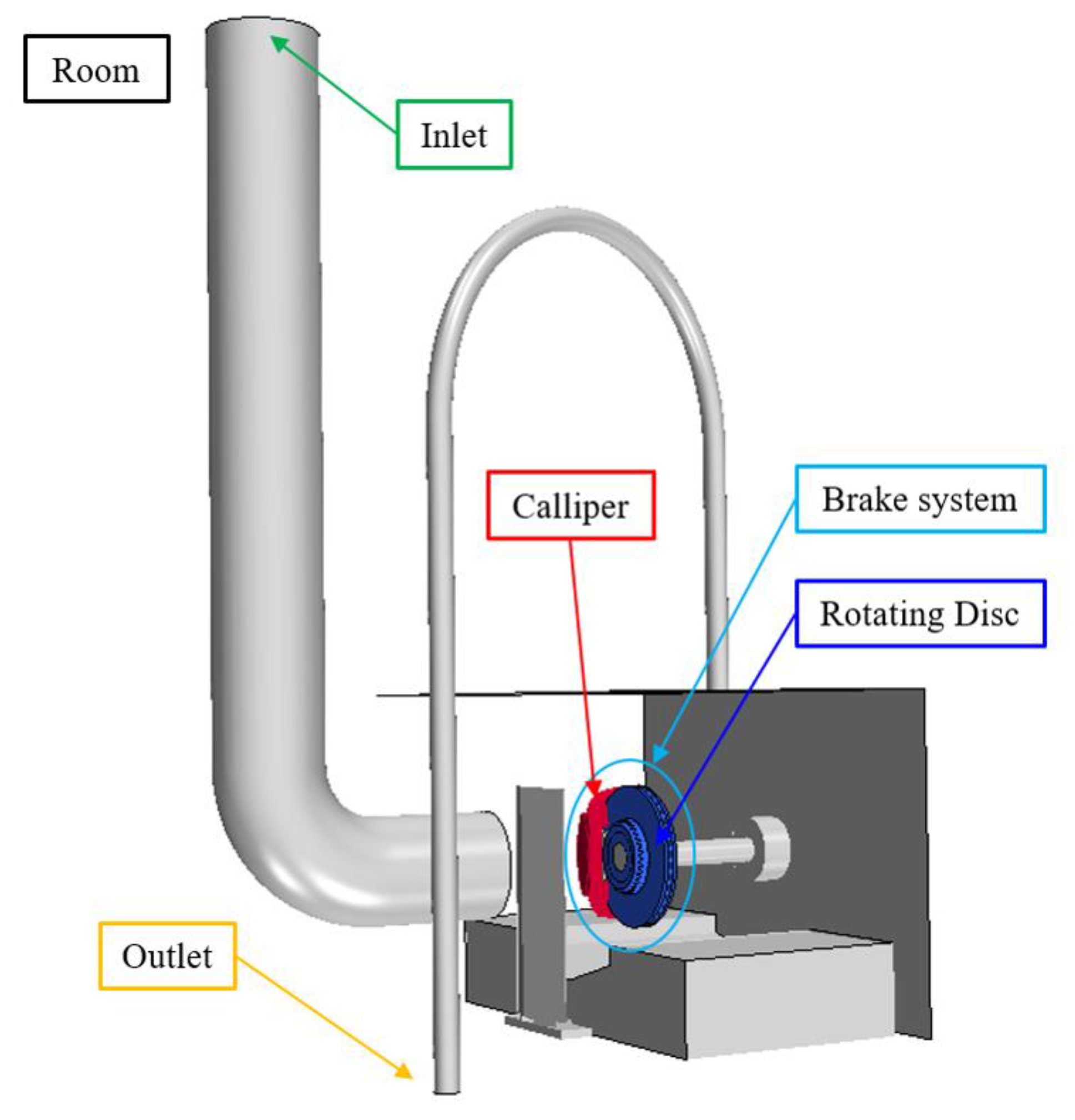
4. Inertia Dyno Bench Tests
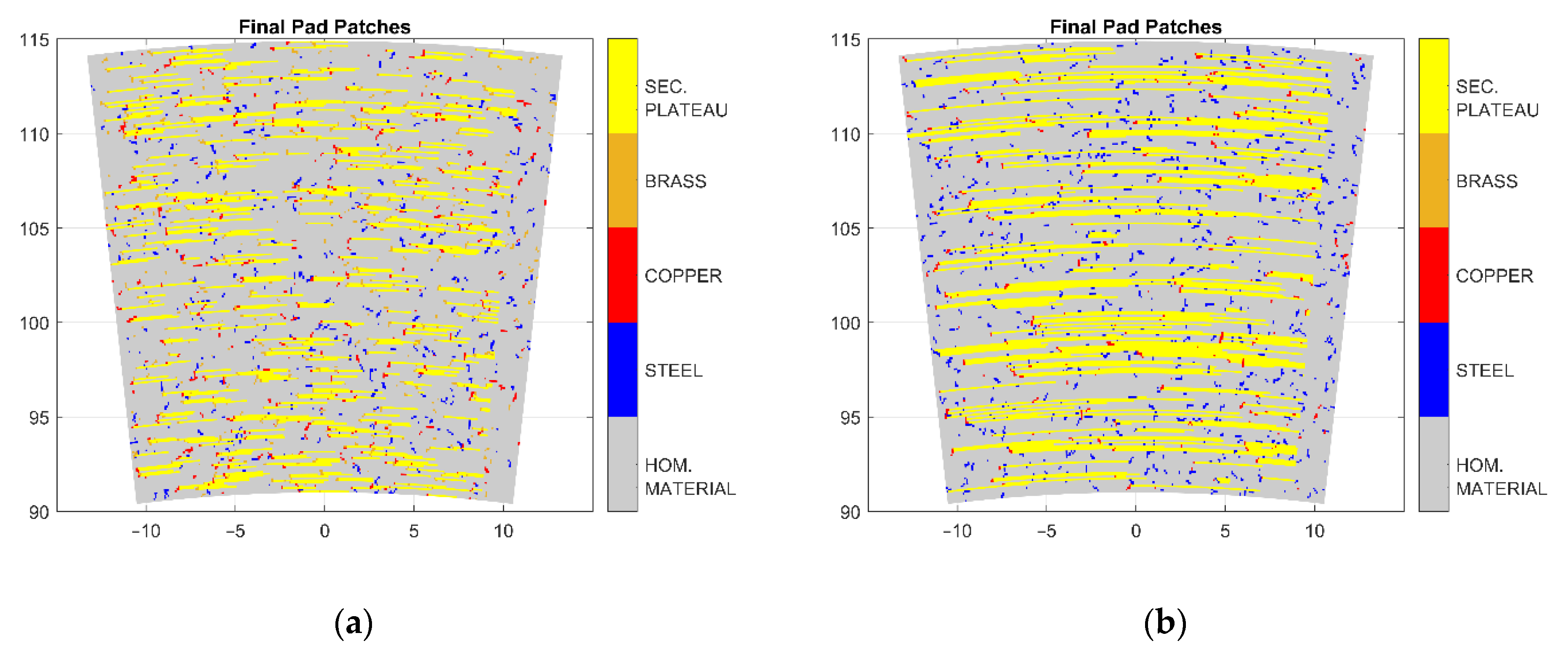
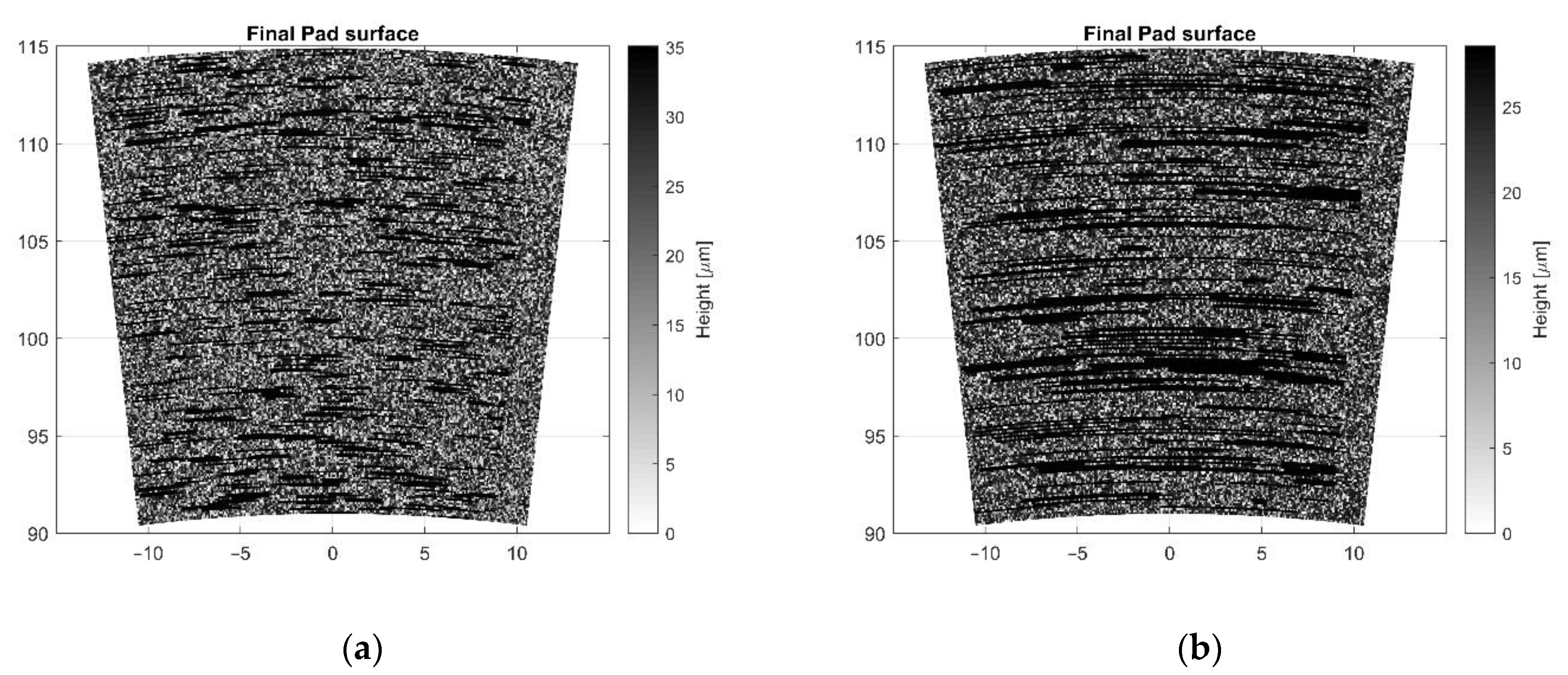
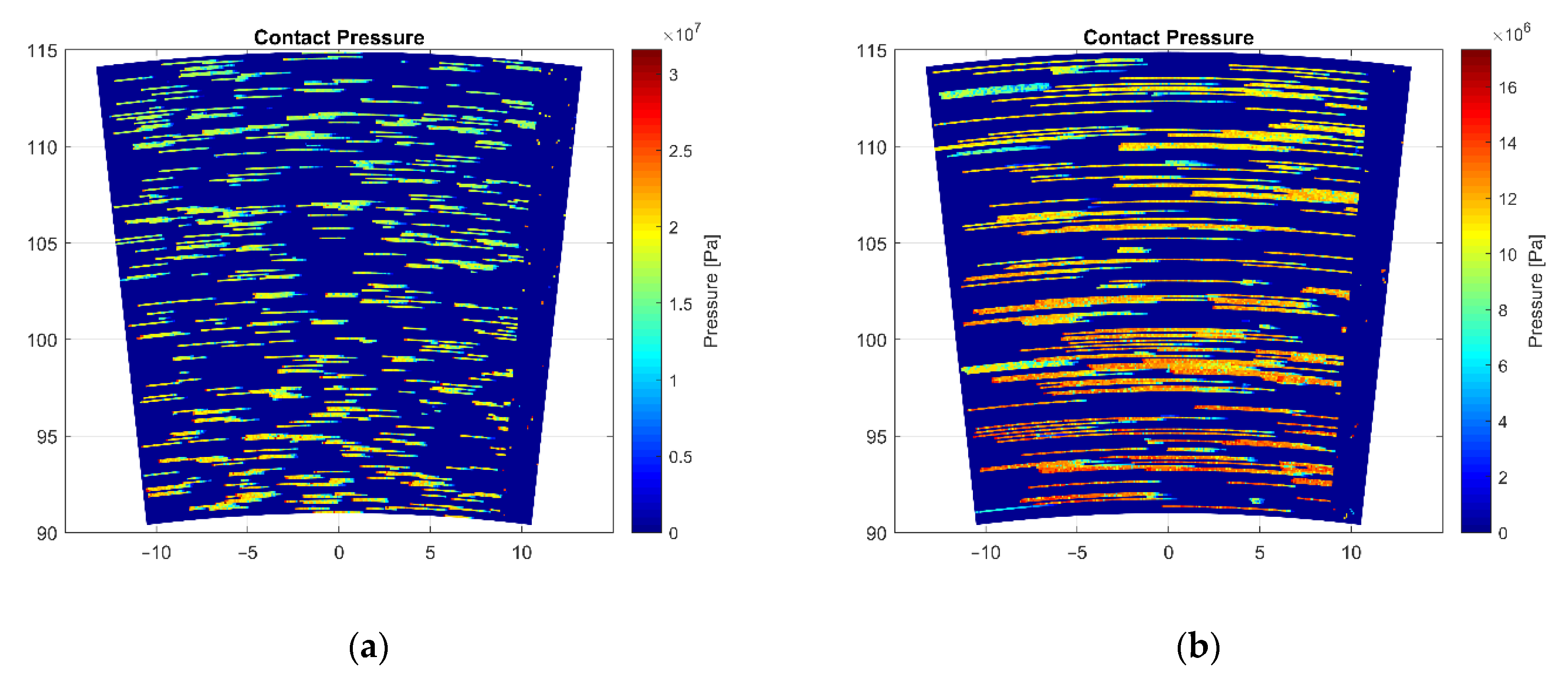
5. Results
6. Discussion
7. Conclusions
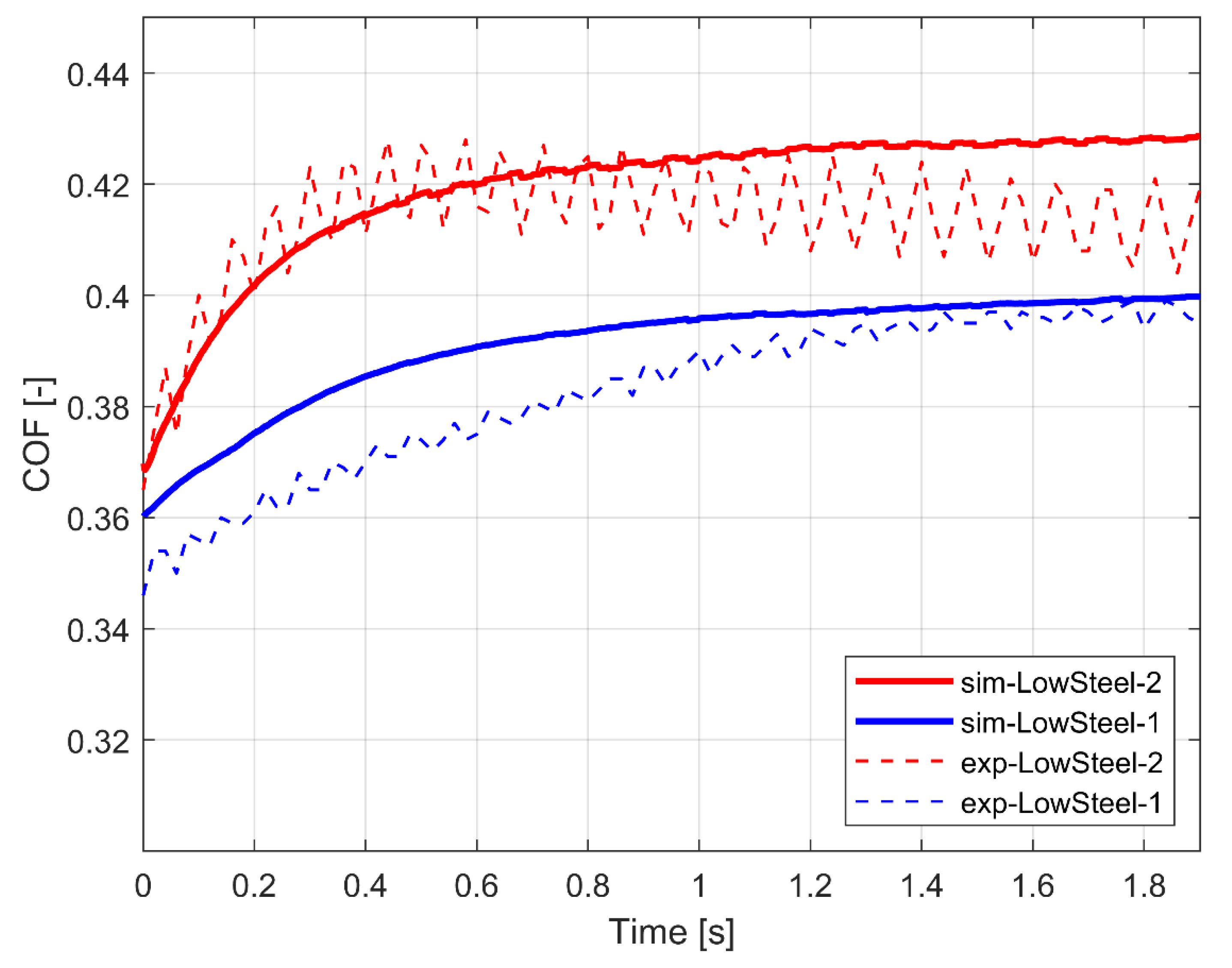
- The simulated COF curve during the entire brake event is similar to the experimentally measured COF curve.
- The mean value of simulated COF curve is similar to the mean value of experimental COF curve.
- There is a difference between the COF values of the two friction materials due to the different amount of primary and secondary contact plateaus as well as different contact pressures. The developed CA approach could simulate the COF difference very well.
- The simulated COF of the friction material having the lowest contact pressure values is higher than the one of the friction material having the highest contact pressure value.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| COF | Coefficient of Friction |
| CA | Cellular Automaton |
| CFD | Computational Fluid Dynamics |
| FEA | Finite Element Analysis |
| LS | Low Steel |
| NAO | Non-Asbestos Organic |
References
- Tirovic, M.; Day, A.J. Disc Brake Interface Pressure Distributions. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 1991, 205, 137–146. [Google Scholar] [CrossRef]
- Garg, B.D.; Cadle, S.H.; Mulawa, P.A.; Groblicki, P.J.; Laroo, C.; Parr, G.A. Brake Wear Particulate Matter Emissions. Environ. Sci. Technol. 2000, 34, 4463–4469. [Google Scholar] [CrossRef]
- Kukutschová, J.; Moravec, P.; Tomášek, V.; Matějka, V.; Smolík, J.; Schwarz, J.; Seidlerová, J.; Šafářová, K.; Filip, P. On airborne nano/micro-sized wear particles released from low-metallic automotive brakes. Environ. Pollut. 2011, 159, 998–1006. [Google Scholar] [CrossRef] [PubMed]
- Sanders, P.G.; Xu, N.; Dalka, T.M.; Maricq, M.M. Airborne Brake Wear Debris: Size Distributions, Composition, and a Comparison of Dynamometer and Vehicle Tests. Environ. Sci. Technol. 2003, 37, 4060–4069. [Google Scholar] [CrossRef]
- Wahlström, J.; Matejka, V.; Lyu, Y.; Söderberg, A. Contact Pressure and Sliding Velocity Maps of the Friction, Wear and Emission from a Low-Metallic/Cast-Iron Disc Brake Contact Pair. Tribol. Ind. 2017, 39, 460–470. [Google Scholar] [CrossRef] [Green Version]
- Perricone, G.; Wahlström, J.; Olofsson, U. Towards a test stand for standardized measurements of the brake emissions. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2016, 230, 1521–1528. [Google Scholar] [CrossRef]
- Mathissen, M.; Grochowicz, J.; Schmidt, C.; Vogt, R.; zum Hagen, F.H.F.; Grabiec, T.; Steven, H.; Grigoratos, T. A novel real-world braking cycle for studying brake wear particle emissions. Wear 2018, 414–415, 219–226. [Google Scholar] [CrossRef]
- Kumar, M.; Bijwe, J. Composite friction materials based on metallic fillers: Sensitivity of µ to operating variables. Tribol. Int. 2011, 44, 106–113. [Google Scholar] [CrossRef]
- Eriksson, M.; Bergman, F.; Jacobson, S. On the nature of tribological contact in automotive brakes. Wear 2002, 252, 26–36. [Google Scholar] [CrossRef]
- Riva, G.; Perricone, G.; Wahlström, J. Simulation of Contact Area and Pressure Dependence of Initial Surface Roughness for Cermet-Coated Discs Used in Disc Brakes. Tribol. Ind. 2019, 41, 1–13. [Google Scholar] [CrossRef]
- Riva, G.; Varriale, F.; Wahlström, J. A finite element analysis (FEA) approach to simulate the coefficient of friction of a brake system starting from material friction characterization. Friction 2020, 9, 1–10. [Google Scholar] [CrossRef]
- Österle, W.; Griepentrog, M.; Gross, T.; Urban, I. Chemical and microstructural changes induced by friction and wear of brakes. Wear 2001, 251, 1469–1476. [Google Scholar] [CrossRef]
- Ostermeyer, G.P. Friction and wear of brake systems. Forsch. Im Ing. 2001, 66, 267–272. [Google Scholar] [CrossRef]
- Hacisalihoglu, Y.; Dalga, Y.B.; Akca, D.; Ergin, E.; Aydin, B. Brake Cooling Modelling & Correlation. In Proceedings of the Eurobrake, Dresden, Germany, 21–23 May 2019. [Google Scholar]
- Thuresson, A. CFD and Design Analysis of Brake Disc. Master’s Thesis, Chalmers University of Technology, Göteborg, Sweden, 2014. [Google Scholar]
- Belhocine, A.; Cho, C.D.; Nouby, M.; Yi, Y.B.; Bakar, A.R.A. Thermal analysis of both ventilated and full disc brake rotors with frictional heat generation. Appl. Comput. Mech. 2014, 8, 5–24. [Google Scholar]
- Afzal, A.; Mujeebu, A. Thermo-Mechanical and Structural Performances of Automobile Disc Brakes: A Review of Numerical and Experimental Studies. Arch. Comput. Methods Eng. 2018, 26, 1489–1513. [Google Scholar] [CrossRef]
- Soderberg, A.; Sellgren, U.; Andersson, S. Using Finite Element Analysis to Predict the Brake Pressure Needed for Effective Rotor Cleaning in Disc Brakes; SAE International: Warrendale, PA, USA, 2008. [Google Scholar]
- Valota, G.; De Luca, S.; Söderberg, A. Using a finite element analysis to simulate the wear in disc brakes during a dyno bench test cycle. In Proceedings of the Eurobrake, Dresden, Germany, 2–4 May 2017. [Google Scholar]
- Wahlström, J.; Söderberg, A.; Olander, L.; Jansson, A.; Olofsson, U. Simulation of airborne wear particles from disc brakes. SAE Tech. Pap. 2010, 268, 763–769. [Google Scholar]
- Wahlström, J. A comparison of measured and simulated friction, wear, and particle emission of disc brakes. Tribol. Int. 2015, 92, 503–511. [Google Scholar] [CrossRef]
- Riva, G.; Valota, G.; Perricone, G.; Wahlström, J. An FEA approach to simulate disc brake wear and airborne particle emissions. Tribol. Int. 2019, 138, 90–98. [Google Scholar] [CrossRef]
- Federici, M.; Perricone, G.; Gialanella, S.; Straffelini, G. Sliding Behaviour of Friction Material Against Cermet Coatings: Pin-on-Disc Study of the Running-in Stage. Tribol. Lett. 2018, 66, 53. [Google Scholar] [CrossRef]
- Müller, M.; Ostermeyer, G. A Cellular Automaton model to describe the three-dimensional friction and wear mechanism of brake systems. Wear 2007, 263, 1175–1188. [Google Scholar] [CrossRef]
- Mueller, M.; Ostermeyer, G. Cellular automata method for macroscopic surface and friction dynamics in brake systems. Tribol. Int. 2007, 40, 942–952. [Google Scholar] [CrossRef]
- Ostermeyer, G.P.; Müller, M. New insights into the tribology of brake systems. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2007, 222, 1167–1200. [Google Scholar] [CrossRef]
- Wahlström, J.; Söderberg, A.; Olofsson, U. A Cellular Automaton Approach to Numerically Simulate the Contact Situation in Disc Brakes. Tribol. Lett. 2011, 42, 253–262. [Google Scholar] [CrossRef]
- Wahlström, J. Towards a cellular automaton to simulate friction, wear, and particle emission of disc brakes. Wear 2014, 313, 75–82. [Google Scholar] [CrossRef]
- Wahlström, J. A Factorial Design to Numerically Study the Effects of Brake Pad Properties on Friction and Wear Emissions. Adv. Tribol. 2016, 2016, 8181260. [Google Scholar] [CrossRef] [Green Version]
- Ostermeyer, G.P.; Merlis, J.H. Modelling the Friction Boundary Layer of an Entire Brake Pad with an Abstract Cellular Automaton. Lubricants 2018, 6, 44. [Google Scholar] [CrossRef] [Green Version]
- Dmitriev, A.I.; Popov, V.L. Numerical modeling of processes of mass transfer in tribological contacts by the method of movable cellular automata. J. Frict. Wear 2009, 30, 12–16. [Google Scholar] [CrossRef]
- Dmitriev, A.; Österle, W.; KloβH. Numerical simulation of typical contact situations of brake friction materials. Tribol. Int. 2008, 41, 1–8. [Google Scholar] [CrossRef]
- Österle, W.; Kloß, H.; Urban, I.; Dmitriev, A. Towards a better understanding of brake friction materials. Wear 2007, 263, 1189–1201. [Google Scholar] [CrossRef]
- Österle, W.; Urban, I. Third body formation on brake pads and rotors. Tribol. Int. 2006, 39, 401–408. [Google Scholar] [CrossRef]
- Riva, G.; Perricone, G.; Wahlström, J. A multi-scale simulation approach to investigate local contact temperatures for commercial Cu-full and Cu-free brake pads. Lubricants 2019, 7, 80. [Google Scholar] [CrossRef] [Green Version]
- Ricciardi, V.; Augsburg, K.; Gramstat, S.; Schreiber, V.; Ivanov, V. Survey on Modelling and Techniques for Friction Estimation in Automotive Brakes. Appl. Sci. 2017, 7, 873. [Google Scholar] [CrossRef]
- Ostermeyer, G. On the dynamics of the friction coefficient. Wear 2003, 254, 852–858. [Google Scholar] [CrossRef]
- Chan, D.; Stachowiak, G.W. Review of automotive brake friction materials. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2004, 218, 953–966. [Google Scholar] [CrossRef]
- EPA. Memorandum of Understanding on Copper Mitigation in Watershed and Waterways. 21 January 2015. Available online: https://www.epa.gov/npdes/copper-free-brake-initiative (accessed on 20 January 2022).
- Chen, H.; Paul, H.-G. Copper-Free Friction Material for Brake Pads. U.S. Patent 20130037360A1, 14 February 2013. [Google Scholar]
- Copper-Free Ceramic Type Friction Material and Preparation Method Thereof. U.S. Patent CN 101948673 B, 26 June 2013.
- Gilardi, R.; Alzati, L.; Thiam, M.; Brunel, J.F.; Desplanques, Y.; Dufrénoy, P.; Sharma, S.; Bijwe, J. Copper substitution and noise reduction in brake pads: Graphite type selection. Materials 2012, 5, 2258–2269. [Google Scholar] [CrossRef] [Green Version]
- Kumar, M.; Bijwe, J. Non-asbestos organic (NAO) friction composites: Role of copper; its shape and amount. Wear 2011, 270, 269–280. [Google Scholar] [CrossRef]
- Lee, P.W.; Filip, P. Friction and wear of Cu-free and Sb-free environmental friendly automotive brake materials. Wear 2013, 302, 1404–1413. [Google Scholar] [CrossRef]
- Schuler, L.J.; Hoang, T.C.; Rand, G.M. Aquatic risk assessment of copper in freshwater and saltwater ecosystems of South Florida. Ecotoxicology 2008, 17, 642–659. [Google Scholar] [CrossRef]
- Yun, R.; Filip, P.; Lu, Y. Performance and evaluation of eco-friendly brake friction materials. Tribol. Int. 2010, 43, 2010–2019. [Google Scholar] [CrossRef]
- Zhang, J.Z. Copper-Free Friction Material Composition for Brake Pads. U.S. Patent WO 2012159286 A1, 21 May 2011. [Google Scholar]
- Menapace, C.; Leonardi, M.; Matějka, V.; Gialanella, S.; Straffelini, G. Dry sliding behavior and friction layer formation in copper-free barite containing friction materials. Wear 2018, 398, 191–200. [Google Scholar] [CrossRef]
- Xiao, X.; Yin, Y.; Bao, J.; Lu, L.; Feng, X. Review on the friction and wear of brake materials. Adv. Mech. Eng. 2016, 8, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Abadi, S.B.K.; Khavandi, A.; Kharazi, Y. The effect of steel & basalt fibers on the friction and wear behavior of composites brake pad non-asbestos. Int. J. Eng. Iran Univ. Sci. Technol. 2007, 4, 87–92. [Google Scholar]
- Abadi, S.B.K.; Khavandi, A.; Kharazi, Y. Effects of Mixing the Steel and Carbon Fibers on the Friction and Wear Properties of a PMC Friction Material. Appl. Compos. Mater. 2009, 17, 151–158. [Google Scholar] [CrossRef]
- Bijwe, J.; Kumar, M.; Gurunath, P.V.; Desplanques, Y.; Degallaix, G. Optimization of brass contents for best combination of tribo-performance and thermal conductivity of non-asbestos organic (NAO) friction composites. Wear 2008, 265, 699–712. [Google Scholar] [CrossRef]
- Söderberg, A.; Björklund, S. Validation of a simplified numerical contact model. Tribol. Int. 2008, 41, 926–933. [Google Scholar] [CrossRef]
- Archard, J.F. Contact and Rubbing of Flat Surfaces. J. Appl. Phys. 1953, 24, 981–988. [Google Scholar] [CrossRef]
- Põdra, P.; Andersson, S. Simulating sliding wear with finite element method. Tribol. Int. 1999, 32, 71–81. [Google Scholar] [CrossRef]
- Eriksson, M.; Lord, J.; Jacobson, S. Wear and contact conditions of brake pads: Dynamical in situ studies of pad on glass. Wear 2001, 249, 272–278. [Google Scholar] [CrossRef]
- Gramstadt, S. Methoden der in-situ Visualisierung der Reibzonendynamik trockenlaufender Reibpaarungen unter Erg¨anzung physikalischer und chemischer Charakterisierungen der Reibpartner. Ph.D. Thesis, Universitatsverlag Ilmenau, Ilmenau, Germany, 2015. [Google Scholar]
- Antanaitis, D.; Sanford, J. The Effect of Racetrack/High Energy Driving on Brake Caliper Performance. In Proceedings of the SAE 2006 Technical Paper Series, Detroit, MI, USA, 3–6 April 2006. [Google Scholar]
- Jang, Y.H. Transient thermoelastic contact problems for an elastic foundation. Int. J. Solids Struct. 2000, 37, 1997–2004. [Google Scholar] [CrossRef]
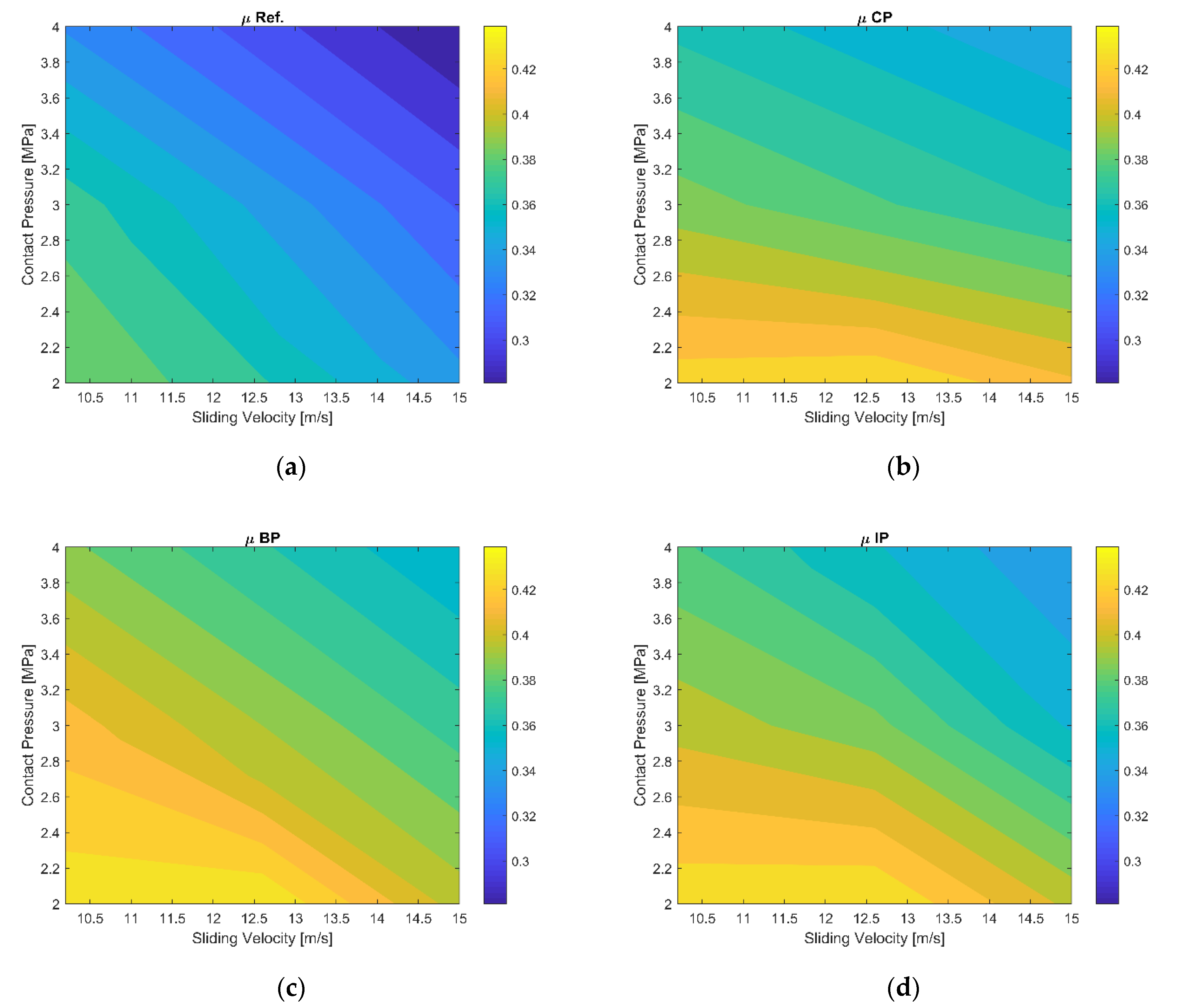


| Samples’ Name | Samples’ Composition |
|---|---|
| (1) Ref. | 10 wt.% barite + 90% of original NAO material composition |
| (2) CP | 10 wt.% copper fibre + 90% of original NAO material composition |
| (3) BP | 10 wt.% brass fibre + 90% of original NAO material composition |
| (4) IP | 10 wt.% iron fibre + 90% of original NAO material composition |
| Sliding Speed | Contact Pressure | Sliding Speed | Contact Pressure | Sliding Speed | Contact Pressure |
|---|---|---|---|---|---|
| 10.2 m/s | 2 MPa | 12.6 m/s | 2 MPa | 12.6 m/s | 2 MPa |
| 3 MPa | 3 MPa | 3 MPa | |||
| 4 MPa | 4 MPa | 4 MPa |
| Fibre (Pressure) | Sliding Velocity 10.2 m/s | Sliding Velocity 12.6 m/s | Sliding Velocity 15 m/s |
|---|---|---|---|
| Brass (2 MPa) | −3.5% | −2.8% | 2.4% |
| Brass (3 MPa) | −6.5% | −2.4% | 3% |
| Brass (4 MPa) | −5.5% | −3.8% | 1.7% |
| Fe (2 MPa) | −3.5% | −3.5% | 1.8% |
| Fe (3 MPa) | −6.4% | −5.1% | 2.5% |
| Fe (4 MPa) | −4.7% | −1.7% | 2.4% |
| Cu (2 MPa) | −3% | −4.5% | 0.8% |
| Cu (3 MPa) | −6.5% | −3.1% | 2.5% |
| Cu (4 MPa) | −3.8% | −4.1% | 0.7% |
| Friction Material Mixture Composition (wt.%) | Max Fibre Length (mm) | Specific Wear Rate (Pa-1) | ||||
|---|---|---|---|---|---|---|
| LS1 | LS2 | LS1 | LS2 | LS1 | LS2 | |
| Steel | 7 | 14.5 | 0.5 | 0.5 | 6.79 × 10−15 | 1.09 × 10−14 |
| Cu | 7 | 4 | 0.5 | 0.5 | 6.79 × 10−15 | 1.09 × 10−14 |
| Brass | 7 | 0 | 0.425 | 0 | 6.79 × 10−15 | 1.09 × 10−14 |
| Aramid | 3 | 1.5 | 1.15 | 1.15 | 6.79 × 10−15 | 1.09 × 10−14 |
| Homogeneous | 76 | 74 | 5 × 10−6 | 5 × 10−6 | ||
| Material | ||||||
| Parameter | Value |
|---|---|
| Radial distance between cells, d_rp | 100 µm |
| Tangential distance between cells, d_fp | 100 µm |
| Depth distance between cells, d_zp | 100 µm |
| Number of cells in r-direction, n_rp | 240 |
| Number of cells in Φ-direction, n_fip | 240 |
| Number of cells in z-direction, n_zp | 2 |
| Number of sub-domains, n_m | 12 |
| Young’s modulus primary plateaus, E1H | 1 × 109 [Pa] |
| Young’s modulus secondary plateaus, E1S | 1× 105 [Pa] |
| Young’s modulus homogeneous material, E2 | 7.8 × 1010 [Pa] |
| Poisson’s ratio primary plateaus, ny1H | 0.28 |
| Poisson’s ratio secondary plateaus, ny1S | 0.28 |
| Poisson’s ratio homogeneous material, ny2 | 0.28 |
| Simulation time, t | 2 s |
| Height of foundation, h | 50 µm |
| Computational time | 5 min |
| Parameter | Value |
|---|---|
| Wheel radius | 361 mm |
| Rotor outer radius | 171 mm |
| Rotor inner radius | 92.2 mm |
| Rotor effective radius | 136 mm |
| Pad surface area | 7507 mm2 |
| Cylinder diameters | 4 × 44 mm |
| COF vs. Time Curve | Mean COF Value [-] |
|---|---|
| Sim-LowSteel-1 | 0.3910 |
| Exp-LowSteel-1 | 0.3824 |
| Sim-LowSteel-2 | 0.4186 |
| Exp-LowSteel-2 | 0.4135 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Varriale, F.; Riva, G.; Wahlström, J.; Lyu, Y. A Mesoscopic Simulation Approach Based on Metal Fibre Characterization Data to Evaluate Brake Friction Performance. Lubricants 2022, 10, 34. https://doi.org/10.3390/lubricants10030034
Varriale F, Riva G, Wahlström J, Lyu Y. A Mesoscopic Simulation Approach Based on Metal Fibre Characterization Data to Evaluate Brake Friction Performance. Lubricants. 2022; 10(3):34. https://doi.org/10.3390/lubricants10030034
Chicago/Turabian StyleVarriale, Francesco, Gabriele Riva, Jens Wahlström, and Yezhe Lyu. 2022. "A Mesoscopic Simulation Approach Based on Metal Fibre Characterization Data to Evaluate Brake Friction Performance" Lubricants 10, no. 3: 34. https://doi.org/10.3390/lubricants10030034
APA StyleVarriale, F., Riva, G., Wahlström, J., & Lyu, Y. (2022). A Mesoscopic Simulation Approach Based on Metal Fibre Characterization Data to Evaluate Brake Friction Performance. Lubricants, 10(3), 34. https://doi.org/10.3390/lubricants10030034







