Dust-Acoustic Rogue Waves in an Electron-Positron-Ion-Dust Plasma Medium
Abstract
1. Introduction
2. Governing Equations
3. Modulational Instability and Rogue Waves
4. Conclusions
- Both modulationally stable (i.e., ) and unstable (i.e., ) DAWs are observed;
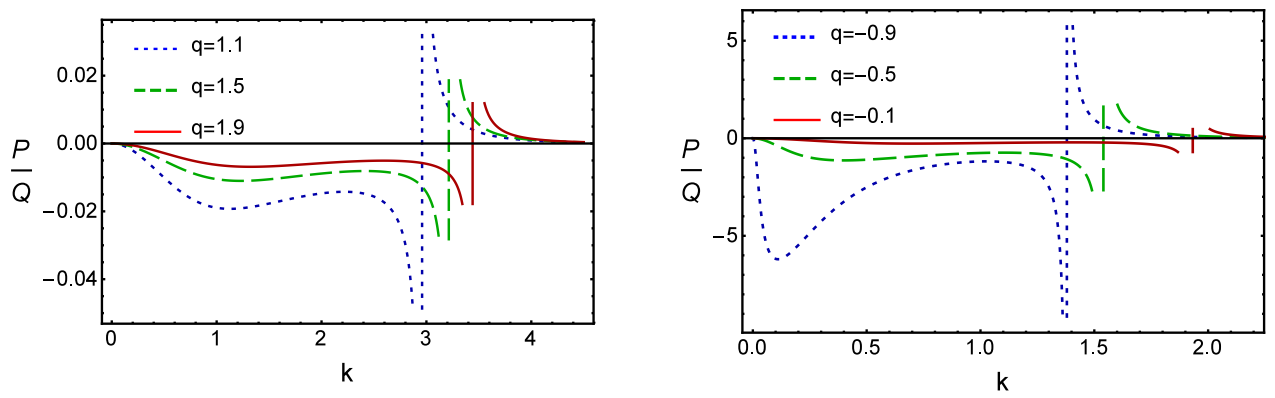
- The direction of the variation of the critical wave number is independent (dependent) of the sign (magnitude) of the q;
- The amplitude of the DA-RWs decreases with increasing population of non-extensive positrons;
- Excess non-extensive ions reduce the height of the DA-RWs.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zurek, W.H. Annihilation radiation from the galactic center-Positrons in dust? Astrophys. J. 1985, 289, 603–608. [Google Scholar] [CrossRef]
- Horányi, M.; Hartquist, T.W.; Havnes, O.; Mendis, D.A.; Morfill, G.E. Dusty plasma effects in saturn’s magnetosphere. Rev. Geophys. 2004, 42, RG4002. [Google Scholar] [CrossRef]
- Krimigis, S.M.; Carbary, J.F.; Keath, E.P.; Armstrong, T.P.; Lanzerotti, L.J.; Gloeckler, G. General characteristics of hot plasma and energetic particles in the Saturnian magnetosphere: Results from the voyager spacecraft. J. Geophys. Res. 1983, 88, 8871–8892. [Google Scholar] [CrossRef]
- Horányi, M.; Morfill, G.E.; Grün, E. Mechanism for the acceleration and ejection of dust grains from Jupiter’s magnetosphere. Nature 1993, 363, 144–146. [Google Scholar] [CrossRef]
- Sturrock, P.A. A model of pulsars. Astrophys. J. 1971, 164, 529–556. [Google Scholar] [CrossRef]
- Ruderman, M.A.; Sutherland, P.G. Theory of pulsars-Polar caps, sparks, and coherent microwave radiation. Astrophys. J. 1975, 196, 51–72. [Google Scholar] [CrossRef]
- Michel, F.C. Theory of pulsar magnetospheres. Rev. Mod. Phys. 1982, 54, 1. [Google Scholar] [CrossRef]
- Shukla, P.K.; Marklund, M. Dust acoustic wave in a strongly magnetized pair-dust plasma. Phys. Scr. 2004, T113, 36. [Google Scholar] [CrossRef]
- Alfvén, H. Cosmic Plasma; D. Reidel Publishing Company: Dordrecht, The Netherlands, 1981. [Google Scholar]
- Spitzer, L., Jr. Physical Processes in the Interstellar Media; John Wiley and Sons: New York, NY, USA, 1978. [Google Scholar]
- Miller, T.J.; Williams, D.A. Dust and Chemistry in Astronomy; Institute of Physics: Bristol, UK, 1993. [Google Scholar]
- Evans, A. The Dusty Universe; John Wiley and Sons: New York, NY, USA, 1994. [Google Scholar]
- Higdon, J.C.; Lingenfelter, R.E.; Rothschild, R.E. The galactic positron annihilation radiation and the propagation of positrons in the interstellar medium. Astrophys. J. 2009, 698, 350. [Google Scholar] [CrossRef][Green Version]
- Shukla, P.K. A note on the formation of large-scale structures in the universe. Phys. Scr. 2008, 77, 068201. [Google Scholar] [CrossRef]
- Ellis, T.A.; Neff, J.S. Numerical simulation of the emission and motion of neutral and charged dust from P/Halley. Icarus 1991, 91, 280–296. [Google Scholar] [CrossRef]
- Mendis, D.A.; Rosenberg, M. Cosmic dusty plasma. Annu. Rev. Astron. Astrophys. 1994, 32, 419–463. [Google Scholar] [CrossRef]
- Horányi, M. Charged dust dynamics in the solar system. Annu. Rev. Astron. Astrophys. 1996, 34, 383–418. [Google Scholar] [CrossRef]
- Murphy, R.J.; Share, G.H.; Skibo, J.G.; Kozlovsky, B. The physics of positron annihilation in the solar atmosphere. Astrophys. J. Suppl. Ser. 2005, 161, 495. [Google Scholar] [CrossRef]
- Chen, F.F. Introduction to Plasma Physics and Controlled Fusion, 3rd ed.; Springer: Cham, Switzerland, 2016. [Google Scholar]
- Shukla, P.K.; Mamun, A.A. Introduction to Dusty Plasma Physics; IOP: London, UK, 2002. [Google Scholar]
- Bandyopadhyay, P.; Prasad, G.; Sen, A.; Kaw, P.K. Experimental study of nonlinear dust acoustic solitary waves in a dusty plasma. Phys. Rev. Lett. 2012, 101, 065006. [Google Scholar] [CrossRef]
- Surko, C.M.; Murphy, T.J. Use of the positron as a plasma particle. Phys. Fluids B 1990, 2, 1372. [Google Scholar] [CrossRef]
- Cho, S.H.; Lee, H.J.; Kim, Y.S. Nonrelativistic electromagnetic surface waves: Dispersion properties in a magnetized dusty electron-positron plasma. Phys. Rev. E 2000, 61, 4357. [Google Scholar] [CrossRef]
- Smirnov, R.D.; Pigarov, A.Y.; Rosenberg, M.; Krasheninnikov, S.I.; Mendis, D.A. Modelling of dynamics and transport of carbon dust particles in tokamaks. Plasma Phys. Control. Fusion 2007, 49, 347. [Google Scholar] [CrossRef]
- Krasheninnikov, S.I.; Pigarov, A.Y.; Smirnov, R.D.; Rosenberg, M.; Tanaka, Y.; Benson, D.J. Recent progress in understanding the behavior of dust in fusion devices. Plasma Phys. Control. Fusion 2008, 50, 124054. [Google Scholar] [CrossRef]
- Nosenko, V.; Zhdanov, S.; Ivlev, A.V.; Morfill, G.; Goree, J.; Piel, A. Heat transport in a two-dimensional complex (dusty) plasma at melting conditions. Phys. Rev. Lett. 2008, 100, 025003. [Google Scholar] [CrossRef]
- Avinash, K.; Merlino, R.L.; Shukla, P.K. Anomalous dust temperature in dusty plasma experiments. Phys. Lett. A 2011, 375, 2854–2857. [Google Scholar] [CrossRef]
- Rosenberg, M.; Mendis, D.A.; Sheehan, D.P. Positively charged dust crystals induced by radiative heating. Phys. Lett. A 1999, 27, 239–242. [Google Scholar] [CrossRef]
- Banerjee, G.; Maitra, S. Arbitrary amplitude dust ion acoustic solitons and double layers in the presence of nonthermal positrons and electrons. Phys. Plasmas 2016, 23, 123701. [Google Scholar] [CrossRef]
- Paul, A.; Bandyopadhyay, A. Dust ion acoustic solitary structures in presence of nonthermal electrons and isothermal positrons. Astrophys. Space Sci. 2016, 361, 172. [Google Scholar] [CrossRef]
- Noman, A.A.; Chowdhury, N.A.; Mannan, A.; Mamun, A.A. Dust-acoustic envelope solitons in super-thermal plasmas. Contrib. Plasma Phys. 2019, 59, e201900023. [Google Scholar] [CrossRef]
- Khondaker, S.; Mannan, A.; Chowdhury, N.A.; Mamun, A.A. Rogue waves in multi-pair plasma medium. Contrib. Plasma Phys. 2019, 59, e201800125. [Google Scholar] [CrossRef]
- Futaana, Y.; Machida, S.; Saito, Y.; Matsuoka, A.; Hayakawa, H. Moon-related nonthermal ions observed by Nozomi: Species, sources, and generation mechanisms. J. Geophys. Res. 2003, 108, 1025. [Google Scholar] [CrossRef]
- Asbridge, J.R.; Bame, S.J.; Strong, I.B. Outward flow of protons from the Earth’s bow shock. J. Geophys. Res. 1968, 73, 5777–5782. [Google Scholar] [CrossRef]
- Hansen, S.H. Cluster temperatures and non-extensive thermo-statistics. New Astron. 2005, 10, 371–377. [Google Scholar] [CrossRef]
- Renyi, A. On a new axiomatic theory of probability. Acta Math. Acad. Sci. Hung. 1955, 6, 285–335. [Google Scholar] [CrossRef]
- Tsallis, C. Possible generalization of Boltzmann-Gibbs statistics. J. Stat. Phys. 1988, 52, 479–487. [Google Scholar] [CrossRef]
- Eslami, P.; Mottaghizadeh, M.; Pakzad, H.R. Nonplanar dust acoustic solitary waves in dusty plasmas with ions and electrons following a q-nonextensive distribution. Phys. Plasmas 2011, 18, 102303. [Google Scholar] [CrossRef]
- Roy, K.; Chatterjee, P.; Kausik, S.S.; Wong, C.S. Shock waves in a dusty plasma having q-nonextensive electron velocity distribution. Astrophys. Space Sci. 2014, 350, 599–605. [Google Scholar] [CrossRef]
- Zaman, D.M.S.; Mannan, A.; Chowdhury, N.A.; Mamun, A.A. Dust-acoustic rogue waves in opposite polarity dusty plasma featuring nonextensive statistics. High Temp. 2020, 58, 789–794. [Google Scholar] [CrossRef]
- Bains, A.S.; Tribeche, M.; Ng, C.S. Dust-acoustic wave modulation in the presence of q-nonextensive electrons and/or ions in dusty plasma. Astrophys. Space Sci. 2013, 343, 621–628. [Google Scholar] [CrossRef]
- Moslem, W.M.; Sabry, R.; El-Labany, S.K.; Shukla, P.K. Dust-acoustic rogue waves in a nonextensive plasma. Phys. Rev. E 2011, 84, 066402. [Google Scholar] [CrossRef]
- Rahman, M.H.; Mannan, A.; Chowdhury, N.A.; Mamun, A.A. Generation of rogue waves in space dusty plasmas. Phys. Plasmas 2018, 25, 102118. [Google Scholar] [CrossRef]
- Esfandyari-Kalejahi, A.; Afsari-Ghazi, M.; Noori, K.; Irani, S. Large amplitude dust-acoustic solitary waves in electron-positron-ion plasma with dust grains. Phys. Plasmas 2012, 19, 082308. [Google Scholar] [CrossRef]
- Jehan, N.; Masood, W.; Mirza, A.M. Planar and nonplanar dust acoustic solitary waves in electron–positron–ion–dust plasmas. Phys. Scr. 2009, 80, 035506. [Google Scholar] [CrossRef]
- Banik, S.; Shikha, R.K.; Noman, A.A.; Chowdhury, N.A.; Mannan, A.; Roy, T.S.; Mamun, A.A. First and second-order dust-ion-acoustic rogue waves in non-thermal plasma. Eur. Phys. J. D 2021, 75, 43. [Google Scholar] [CrossRef]
- Shikha, R.K.; Chowdhury, N.A.; Mannan, A.; Mamun, A.A. Electrostatic dust-acoustic envelope solitons in an electron-depleted plasma. Contrib. Plasma Phys. 2021, 61, e202000117. [Google Scholar] [CrossRef]
- Rajib, T.I.; Tamanna, N.K.; Chowdhury, N.A.; Mannan, A.; Sultana, S.; Mamun, A.A. Dust-ion-acoustic rogue waves in presence of non-extensive non-thermal electrons. Phys. Plasmas 2019, 26, 123701. [Google Scholar] [CrossRef]
- Fedele, R. Envelope Solitons versus Solitons. Phys. Scr. 2002, 65, 502. [Google Scholar] [CrossRef]
- Cousens, S.E.; Sultana, S.; Kourakis, I.; Yaroshenko, V.V.; Verheest, F.; Hellberg, M.A. Nonlinear dust-acoustic solitary waves in strongly coupled dusty plasmas. Phys. Rev. E 2012, 86, 066404. [Google Scholar] [CrossRef]
- Bandyopadhyay, P.; Konopka, U.; Khrapak, S.A.; Morfill, G.E.; Sen, A. Effect of polarization force on the propagation of dust acoustic solitary waves. New J. Phys. 2010, 12, 073002. [Google Scholar] [CrossRef]
- Zadorozhny, A.M. Effects of charged dust on mesospheric electrical structure. Adv. Space Res. 2001, 28, 1059–1064. [Google Scholar] [CrossRef]
- Wang, Y.L.; Guo, X.Y.; Li, Q.S. Nonlinear dust acoustic waves in strongly coupled dusty plasmas with charged dust particles. Commun. Theor. Phys. 2016, 65, 247. [Google Scholar] [CrossRef]
- Akhmediev, N.; Ankiewicz, A.; Soto-Crespo, J.M. Rogue waves and rational solutions of the nonlinear Schrödinger equation. Phys. Rev. E 2009, 80, 026601. [Google Scholar] [CrossRef]
- Ankiewicz, A.; Devine, N.; Akhmediev, N. Are rogue waves robust against perturbations? Phys. Lett. A 2009, 373, 3997–4000. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rahman, M.H.; Chowdhury, N.A.; Mannan, A.; Mamun, A.A. Dust-Acoustic Rogue Waves in an Electron-Positron-Ion-Dust Plasma Medium. Galaxies 2021, 9, 31. https://doi.org/10.3390/galaxies9020031
Rahman MH, Chowdhury NA, Mannan A, Mamun AA. Dust-Acoustic Rogue Waves in an Electron-Positron-Ion-Dust Plasma Medium. Galaxies. 2021; 9(2):31. https://doi.org/10.3390/galaxies9020031
Chicago/Turabian StyleRahman, Md. Habibur, Nure Alam Chowdhury, Abdul Mannan, and A. A. Mamun. 2021. "Dust-Acoustic Rogue Waves in an Electron-Positron-Ion-Dust Plasma Medium" Galaxies 9, no. 2: 31. https://doi.org/10.3390/galaxies9020031
APA StyleRahman, M. H., Chowdhury, N. A., Mannan, A., & Mamun, A. A. (2021). Dust-Acoustic Rogue Waves in an Electron-Positron-Ion-Dust Plasma Medium. Galaxies, 9(2), 31. https://doi.org/10.3390/galaxies9020031






