Testing a Lepto-Hadronic Two-Zone Model with Extreme High-Synchrotron Peaked BL Lacs and Track-like High-Energy Neutrinos
Abstract
1. Introduction
2. The Model
2.1. The Inner Blob
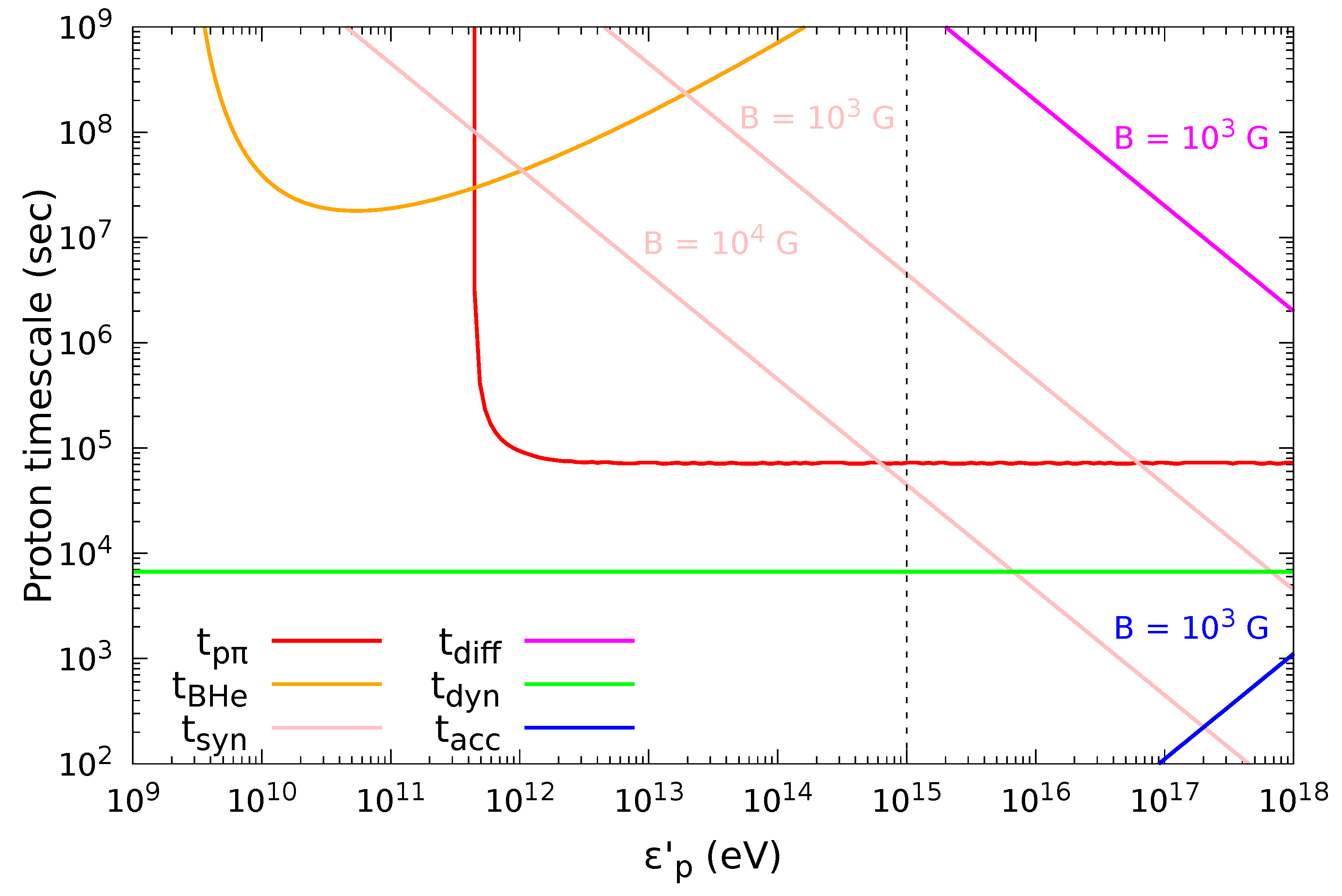
2.1.1. Proton Distribution
2.1.2. Seed Photons by the Pair-Plasma
2.1.3. Photopion Process
High-Energy Neutrinos
Gamma-Rays
Secondary Electrons/Positrons
2.1.4. Photopair Process
2.1.5. Synchrotron Emission of Secondary Pairs
2.1.6. Proton Synchrotron
2.2. The Outer Blob
- (i)
- As the electron population is larger than the proton population (), only leptonic processes are taken into account.
- (ii)
- The blob travels at relativistic speeds, thus we use and as Lorentz and Doppler boost factors, respectively. Our choice agrees with the average value, , reported by [75] who conducted a study with a sample of 217 blazars and utilized a one-zone leptonic model. Even though if take greater values, our model could perform the same fitting, but at the cost of needs smaller emission regions or stronger magnetic field. And and interesting consequence is the reduce of the value for the equipartition parameter .
- (iii)
- Using the assumption that the blob’s variability timescales is with and , we find that the blob’s distance from the SMBH is , this is equivalent to around for a SMBH mass of . This separation is in close proximity to the hypothesized acceleration and collimation zone (e.g., [76,77]) suggesting that may be close to the terminal Lorentz factor of the jet.
- (iv)
- It was possible to determine the magnetic field by looking at the peak of the synchrotron bump, which was around . Now when the timescales of the synchrotron and the adiabatic losses are equivalent, we have the electron energy break, which is written ashence, this blob’s magnetic field strength is
Electron Distribution
3. Cases of Study and Results
3.1. High-Energy Neutrinos Coincident with Extreme High Synchrotron Peaked BL Lacs
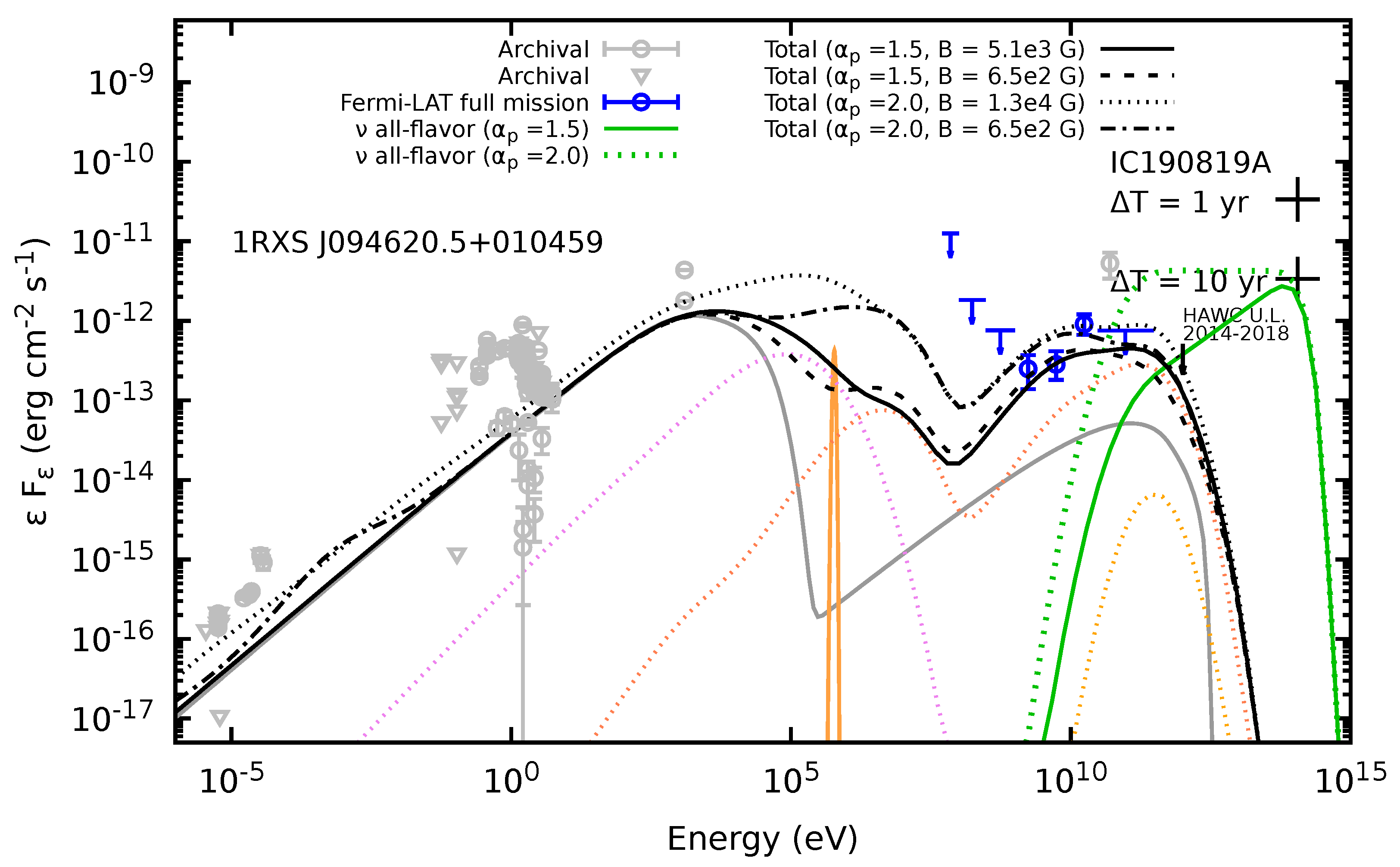
3.1.1. IC190819A and 1RXS J09462.5+010459
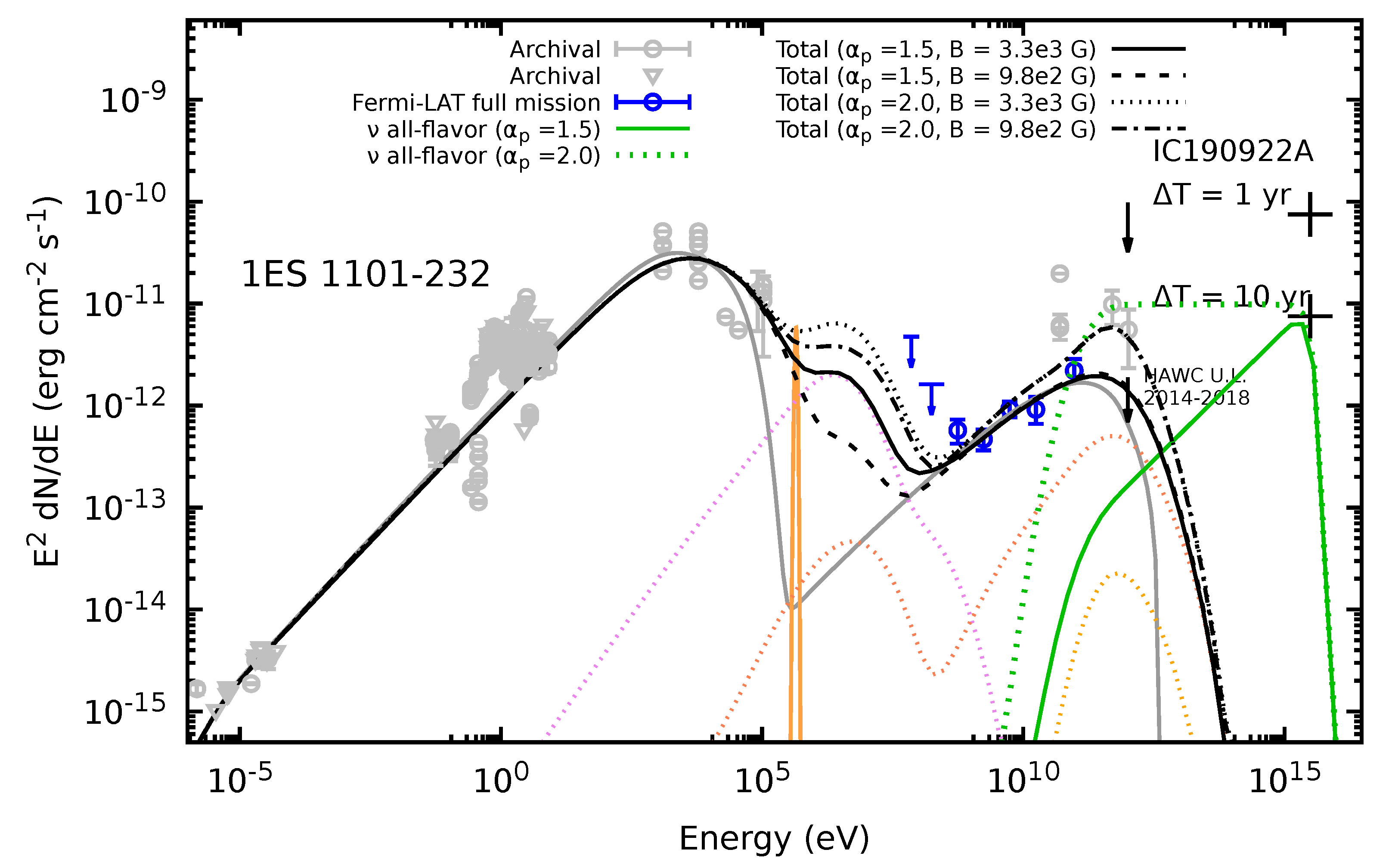
3.1.2. IC190922A and 1ES 1101-232
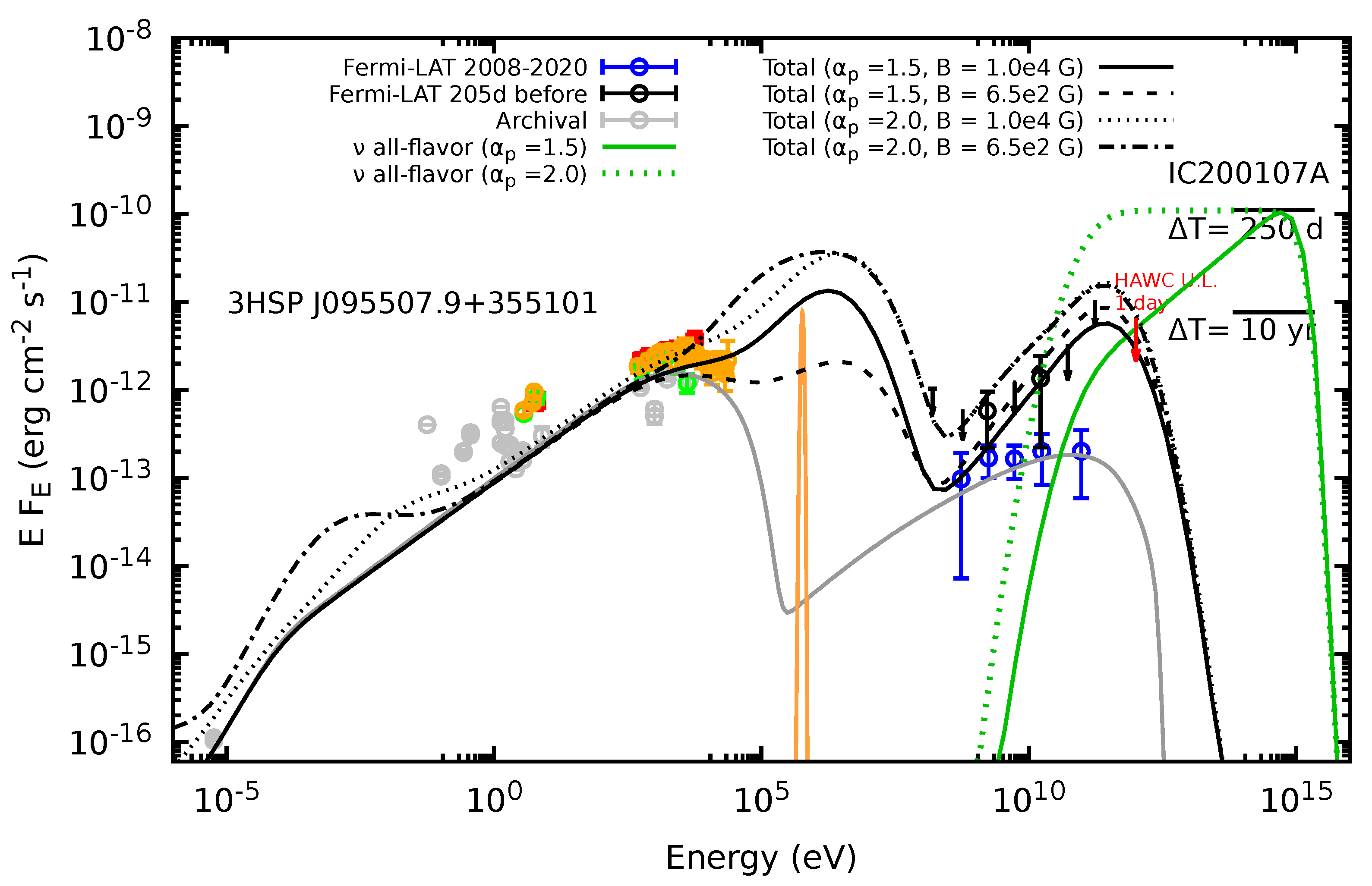
3.1.3. IC200107A and 3HSP J095507.9+355101
3.2. Modeling the SED of EHSP
- Scenario A:
- Scenario B:
3.2.1. 1RXS J09462.5+010459
3.2.2. 1ES 1101-232
3.2.3. 3HSP J095507.9+355101
4. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Blandford, R.D.; Königl, A. Relativistic jets as compact radio sources. Astrophys. J. 1979, 232, 34–48. [Google Scholar] [CrossRef]
- Marcha, M.J.M.; Browne, I.W.A.; Impey, C.D.; Smith, P.S. Optical spectroscopy and polarization of a new sample of optically bright flat radio spectrum sources. Mon. Not. RAS 1996, 281, 425–448. [Google Scholar] [CrossRef]
- Ghisellini, G.; Maraschi, L.; Tavecchio, F. The Fermi blazars’ divide. Mon. Not. RAS 2009, 396, L105–L109. [Google Scholar] [CrossRef]
- Abdo, A.A.; Ackermann, M.; Ajello, M.; Atwood, W.B.; Axelsson, M.; Baldini, L.; Ballet, J.; Barbiellini, G.; Bastieri, D.; Baughman, B.M.; et al. Bright Active Galactic Nuclei Source List from the First Three Months of the Fermi Large Area Telescope All-Sky Survey. Astrophys. J. 2009, 700, 597–622. [Google Scholar] [CrossRef]
- Aartsen, M.G.; et al. [IceCube Collaboration] Neutrino emission from the direction of the blazar TXS 0506+056 prior to the IceCube-170922A alert. Science 2018, 361, 147–151. [Google Scholar] [CrossRef]
- Aartsen, M.G.; et al. [IceCube Collaboration] Multimessenger observations of a flaring blazar coincident with high-energy neutrino IceCube-170922A. Science 2018, 361, eaat1378. [Google Scholar] [CrossRef]
- Wagner, S.J.; Witzel, A. Intraday Variability In Quasars and BL Lac Objects. Annu. Rev. Astron Astrophys. 1995, 33, 163–198. [Google Scholar] [CrossRef]
- Ulrich, M.H.; Maraschi, L.; Urry, C.M. Variability of Active Galactic Nuclei. Annu. Rev. Astron Astrophys. 1997, 35, 445–502. [Google Scholar] [CrossRef]
- Padovani, P.; Giommi, P. The connection between x-ray- and radio-selected BL Lacertae objects. Astrophys. J. 1995, 444, 567–581. [Google Scholar] [CrossRef]
- Ghisellini, G.; Celotti, A.; Costamante, L. Low power BL Lacertae objects and the blazar sequence. Clues on the particle acceleration process. Astron. Astrophys. 2002, 386, 833–842. [Google Scholar] [CrossRef]
- Sambruna, R.M.; Maraschi, L.; Urry, C.M. On the Spectral Energy Distributions of Blazars. Astrophys. J. 1996, 463, 444. [Google Scholar] [CrossRef]
- Abdo, A.A.; Ackermann, M.; Agudo, I.; Ajello, M.; Aller, H.D.; Aller, M.F.; Angelakis, E.; Arkharov, A.A.; Axelsson, M.; Bach, U.; et al. The spectral energy distribution of Fermi bright blazars. Astrophys. J. 2010, 716, 30–70. [Google Scholar] [CrossRef]
- Costamante, L.; Ghisellini, G.; Giommi, P.; Tagliaferri, G.; Celotti, A.; Chiaberge, M.; Fossati, G.; Maraschi, L.; Tavecchio, F.; Treves, A.; et al. Extreme synchrotron BL Lac objects. Stretching the blazar sequence. Astron. Astrophys. 2001, 371, 512–526. [Google Scholar] [CrossRef]
- Tavecchio, F.; Ghisellini, G.; Bonnoli, G.; Foschini, L. Extreme TeV blazars and the intergalactic magnetic field. Mon. Not. RAS 2011, 414, 3566–3576. [Google Scholar] [CrossRef]
- Costamante, L.; Bonnoli, G.; Tavecchio, F.; Ghisellini, G.; Tagliaferri, G.; Khangulyan, D. The NuSTAR view on hard-TeV BL Lacs. Mon. Not. RAS 2018, 477, 4257–4268. [Google Scholar] [CrossRef]
- Ahnen, M.L.; et al. [MAGIC Collaboration] Extreme HBL behavior of Markarian 501 during 2012. Astron. Astrophys. 2018, 620, A181. [Google Scholar] [CrossRef]
- Aharonian, F.A. The time averaged TeV energy spectrum of MKN 501 of the extraordinary 1997 outburst as measured with the stereoscopic Cherenkov telescope system of HEGRA. Astron. Astrophys. 1999, 349, 11–28. [Google Scholar] [CrossRef]
- Archambault, S.; Archer, A.; Beilicke, M.; Benbow, W.; Bird, R.; Biteau, J.; Bouvier, A.; Bugaev, V.; Cardenzana, J.V.; Cerruti, M.; et al. VERITAS Detection of γ-Ray Flaring Activity from the BL Lac Object 1ES 1727+502 during Bright Moonlight Observations. Astrophys. J. 2015, 808, 110. [Google Scholar] [CrossRef]
- Ahnen, T.M.L. MAGIC detection of very high energy γ-ray emission from the low-luminosity blazar 1ES 1741+196. Mon. Not. RAS 2017, 468, 1534–1541. [Google Scholar] [CrossRef]
- Biteau, J.; Prandini, E.; Costamante, L.; Lemoine, M.; Padovani, P.; Pueschel, E.; Resconi, E.; Tavecchio, F.; Taylor, A.; Zech, A. Progress in unveiling extreme particle acceleration in persistent astrophysical jets. Nat. Astron. 2020, 4, 124–131. [Google Scholar] [CrossRef]
- Vraeghe, M.; Aartsen, M.G.; Walck, C.; Abbasi, R.; Waldenmaier, T.; Abdou, Y.; Wallraff, M.; Ackermann, M.; Wasserman, R.; Adams, J.; et al. First Observation of PeV-Energy Neutrinos with IceCube. Phys. Rev. Lett. 2013, 111, 021103. [Google Scholar] [CrossRef]
- Aartsen, M.G.; et al. [IceCube Collaboration] Evidence for High-Energy Extraterrestrial Neutrinos at the IceCube Detector. Science 2013, 342, 1242856. [Google Scholar] [CrossRef]
- Aartsen, M.G.; et al. [IceCube Collaboration] The IceCube Neutrino Observatory—Contributions to ICRC 2017 Part II: Properties of the Atmospheric and Astrophysical Neutrino Flux. arXiv 2017, arXiv:1710.01191. [Google Scholar]
- Aartsen, M.G.; Ackermann, M.; Adams, J.; Aguilar, J.A.; Ahlers, M.; Ahrens, M.; Alispach, C.; Andeen, K.; Anderson, T.; Ansseau, I.; et al. Characteristics of the diffuse astrophysical electron and tau neutrino flux with six years of IceCube high energy cascade data. arXiv 2020, arXiv:2001.09520. [Google Scholar] [CrossRef]
- Abbasi, R.; Ackermann, M.; Adams, J.; Aguilar, J.A.; Ahlers, M.; Ahrens, M.; Alispach, C.; Alves, A.A., Jr.; Amin, N.M.; Andeen, K.; et al. IceCube high-energy starting event sample: Description and flux characterization with 7.5 years of data. Phys. Rev. D 2021, 104, 022002. [Google Scholar] [CrossRef]
- Aartsen, M.G.; Abraham, K.; Ackermann, M.; Adams, J.; Aguilar, J.A.; Ahlers, M.; Ahrens, M.; Altmann, D.; Andeen, K.; Anderson, T.; et al. Observation and Characterization of a Cosmic Muon Neutrino Flux from the Northern Hemisphere Using Six Years of IceCube Data. Astrophys. J. 2016, 833, 3. [Google Scholar] [CrossRef]
- Haack, C.; et al. [IceCube Collaboration] A measurement of the diffuse astrophysical muon neutrino flux using eight years of IceCube data. In Proceedings of the 35th International Cosmic Ray Conference (ICRC2017), Busan, Republic of Korea, 12–20 July 2017; Volume 301, p. 1005. [Google Scholar]
- Stettner, J. Measurement of the diffuse astrophysical muon-neutrino spectrum with ten years of IceCube data. In Proceedings of the 36th International Cosmic Ray Conference (ICRC2019), Madison, WA, USA, 24 July–1 August 2019; Volume 36, p. 1017. [Google Scholar] [CrossRef]
- Abbasi, R.; Ackermann, M.; Adams, J.; Agarwalla, S.K.; Aguilar, J.A.; Ahlers, M.; Alameddine, J.M.; Amin, N.M.; Andeen, K.; Anton, G.; et al. IceCat-1: The IceCube Event Catalog of Alert Tracks. Astrophys. J. Suppl. 2023, 269, 25. [Google Scholar] [CrossRef]
- Abbasi, R.; et al. [IceCube Collaboration] Improved Characterization of the Astrophysical Muon-neutrino Flux with 9.5 Years of IceCube Data. Astrophys. J. 2022, 928, 50. [Google Scholar] [CrossRef]
- Abbasi, R.; et al. [IceCube Collaboration] Evidence for neutrino emission from the nearby active galaxy NGC 1068. Science 2022, 378, 538–543. [Google Scholar] [CrossRef]
- Becker, J.K. High-energy neutrinos in the context of multimessenger astrophysics. Phys. Rep. 2008, 458, 173–246. [Google Scholar] [CrossRef]
- Ahlers, M.; Halzen, F. High-energy cosmic neutrino puzzle: A review. Rep. Prog. Phys. 2015, 78, 126901. [Google Scholar] [CrossRef]
- Gao, S.; Fedynitch, A.; Winter, W.; Pohl, M. Modelling the coincident observation of a high-energy neutrino and a bright blazar flare. Nat. Astron. 2019, 3, 88–92. [Google Scholar] [CrossRef]
- Xue, R.; Liu, R.Y.; Wang, Z.R.; Ding, N.; Wang, X.Y. A Two-zone Blazar Radiation Model for “Orphan” Neutrino Flares. Astrophys. J. 2021, 906, 51. [Google Scholar] [CrossRef]
- Liu, R.Y.; Wang, K.; Xue, R.; Taylor, A.M.; Wang, X.Y.; Li, Z.; Yan, H. Hadronuclear interpretation of a high-energy neutrino event coincident with a blazar flare. Phys. Rev. D 2019, 99, 063008. [Google Scholar] [CrossRef]
- Fraija, N.; Aguilar-Ruiz, E.; Galván-Gámez, A. Electron-positron pair plasma in TXS 0506+056 and the ’neutrino flare’ in 2014–2015. Mon. Not. RAS 2020, 497, 5318–5325. [Google Scholar] [CrossRef]
- Halzen, F.; Kheirandish, A.; Weisgarber, T.; Wakely, S.P. On the Neutrino Flares from the Direction of TXS 0506+056. Astrophys. J. Lett. 2019, 874, L9. [Google Scholar] [CrossRef]
- Keivani, A.; Murase, K.; Petropoulou, M.; Fox, D.B.; Cenko, S.B.; Chaty, S.; Coleiro, A.; DeLaunay, J.J.; Dimitrakoudis, S.; Evans, P.A.; et al. A Multimessenger Picture of the Flaring Blazar TXS 0506+056: Implications for High-energy Neutrino Emission and Cosmic-Ray Acceleration. Astrophys. J. 2018, 864, 84. [Google Scholar] [CrossRef]
- Reimer, A.; Böttcher, M.; Buson, S. Cascading Constraints from Neutrino-emitting Blazars: The Case of TXS 0506+056. Astrophys. J. 2019, 881, 46. [Google Scholar] [CrossRef]
- Rodrigues, X.; Gao, S.; Fedynitch, A.; Palladino, A.; Winter, W. Leptohadronic Blazar Models Applied to the 2014–2015 Flare of TXS 0506+056. Astrophys. J. Lett. 2019, 874, L29. [Google Scholar] [CrossRef]
- Padovani, P.; Petropoulou, M.; Giommi, P.; Resconi, E. A simplified view of blazars: The neutrino background. Mon. Not. RAS 2015, 452, 1877–1887. [Google Scholar] [CrossRef]
- Buson, S.; Tramacere, A.; Pfeiffer, L.; Oswald, L.; de Menezes, R.; Azzollini, A.; Ajello, M. Beginning a Journey Across the Universe: The Discovery of Extragalactic Neutrino Factories. Astrophys. J. Lett. 2022, 933, L43. [Google Scholar] [CrossRef]
- Giommi, P.; Glauch, T.; Padovani, P.; Resconi, E.; Turcati, A.; Chang, Y.L. Dissecting the regions around IceCube high-energy neutrinos: Growing evidence for the blazar connection. arXiv 2020, arXiv:2001.09355. [Google Scholar] [CrossRef]
- Bellenghi, C.; Padovani, P.; Resconi, E.; Giommi, P. Correlating High-energy IceCube Neutrinos with 5BZCAT Blazars and RFC Sources. Astrophys. J. Lett. 2023, 955, L32. [Google Scholar] [CrossRef]
- Abbasi, R.; et al. [IceCube Collaboration] Search for Correlations of High-energy Neutrinos Detected in IceCube with Radio-bright AGN and Gamma-Ray Emission from Blazars. Astrophys. J. 2023, 954, 75. [Google Scholar] [CrossRef]
- Padovani, P.; Resconi, E.; Giommi, P.; Arsioli, B.; Chang, Y.L. Extreme blazars as counterparts of IceCube astrophysical neutrinos. Mon. Not. RAS 2016, 457, 3582–3592. [Google Scholar] [CrossRef]
- Padovani, P.; Resconi, E. Are both BL Lacs and pulsar wind nebulae the astrophysical counterparts of IceCube neutrino events? Mon. Not. RAS 2014, 443, 474–484. [Google Scholar] [CrossRef]
- Petropoulou, M.; Dimitrakoudis, S.; Padovani, P.; Mastichiadis, A.; Resconi, E. Photohadronic origin of γ-ray BL Lac emission: Implications for IceCube neutrinos. Mon. Not. RAS 2015, 448, 2412–2429. [Google Scholar] [CrossRef]
- Giommi, P.; Glauch, T.; Resconi, E. Swift observation of a flaring very extreme blazar in the error region of the high-energy neutrino Ice-Cube 200107A. Astron. Telegr. 2020, 13394, 1. [Google Scholar]
- Petropoulou, M.; Oikonomou, F.; Mastichiadis, A.; Murase, K.; Padovani, P.; Vasilopoulos, G.; Giommi, P. Comprehensive Multimessenger Modeling of the Extreme Blazar 3HSP J095507.9+355101 and Predictions for IceCube. Astrophys. J. 2020, 899, 113. [Google Scholar] [CrossRef]
- Acharyya, A.; et al. [The VERITAS Collaboration] Multiwavelength Observations of the Blazar PKS 0735+178 in Spatial and Temporal Coincidence with an Astrophysical Neutrino Candidate IceCube-211208A. Astrophys. J. 2023, 954, 70. [Google Scholar] [CrossRef]
- Aguilar-Ruiz, E.; Fraija, N.; Galván-Gámez, A.; Benítez, E. A two-zone model as origin of hard TeV spectrum in extreme BL lacs. Mon. Not. RAS 2022, 512, 1557–1566. [Google Scholar] [CrossRef]
- Aguilar-Ruiz, E.; Fraija, N.; Galván-Gámez, A. High-energy neutrino fluxes from hard-TeV BL Lacs. J. High Energy Astrophys. 2023, 38, 1–11. [Google Scholar] [CrossRef]
- Di Gesu, L.; Marshall, H.L.; Ehlert, S.R.; Kim, D.E.; Donnarumma, I.; Tavecchio, F.; Liodakis, I.; Kiehlmann, S.; Agudo, I.; Jorstad, S.G.; et al. Discovery of X-ray polarization angle rotation in the jet from blazar Mrk 421. Nat. Astron. 2023, 7, 1245–1258. [Google Scholar] [CrossRef]
- Liodakis, I.; Marscher, A.P.; Agudo, I.; Berdyugin, A.V.; Bernardos, M.I.; Bonnoli, G.; Borman, G.A.; Casadio, C.; Casanova, V.; Cavazzuti, E.; et al. Polarized blazar X-rays imply particle acceleration in shocks. Nature 2022, 611, 677–681. [Google Scholar] [CrossRef]
- Aghanim, N.; et al. [Planck Collaboration] Planck 2018 results. VI. Cosmological parameters. Astron. Astrophys. 2020, 641, A6. [Google Scholar] [CrossRef]
- Stecker, F.W.; Baring, M.G.; Summerlin, E.J. Blazar γ-rays, Shock Acceleration, and the Extragalactic Background Light. Astrophys. J. Lett. 2007, 667, L29–L32. [Google Scholar] [CrossRef]
- Caprioli, D. Cosmic-ray acceleration in supernova remnants: Non-linear theory revised. J. Cosmol. Astropart. Phys. 2012, 2012, 038. [Google Scholar] [CrossRef]
- Beloborodov, A.M. Electron-positron outflows from gamma-ray emitting accretion discs. Mon. Not. RAS 1999, 305, 181–189. [Google Scholar] [CrossRef]
- Svensson, R. The pair annihilation process in relativistic plasmas. Astrophys. J. 1982, 258, 321–334. [Google Scholar] [CrossRef]
- Sarkar, S.; Chattopadhyay, I. Pair production and annihilation in advective accretion disks around black holes. J. Phys. Conf. Ser. 2020, 1640, 012022. [Google Scholar] [CrossRef]
- Boettcher, M.; Schlickeiser, R. Pair annihilation radiation from relativistic jets in γ-ray blazars. Astron. Astrophys. 1996, 306, 86. [Google Scholar]
- Plotkin, R.M.; Markoff, S.; Trager, S.C.; Anderson, S.F. Dynamical black hole masses of BL Lac objects from the Sloan Digital Sky Survey. Mon. Not. RAS 2011, 413, 805–812. [Google Scholar] [CrossRef][Green Version]
- León-Tavares, J.; Valtaoja, E.; Chavushyan, V.H.; Tornikoski, M.; Añorve, C.; Nieppola, E.; Lähteenmäki, A. The connection between black hole mass and Doppler boosted emission in BL Lacertae type objects. Mon. Not. RAS 2011, 411, 1127–1136. [Google Scholar] [CrossRef]
- Paiano, S.; Falomo, R.; Padovani, P.; Giommi, P.; Gargiulo, A.; Uslenghi, M.; Rossi, A.; Treves, A. The redshift and the host galaxy of the neutrino candidate 4FGL J0955.1+3551 (3HSP J095507.9+355101). Mon. Not. RAS 2020, 495, L108–L111. [Google Scholar] [CrossRef]
- Dermer, C.D.; Menon, G. High Energy Radiation from Black Holes: Gamma Rays, Cosmic Rays, and Neutrinos; Princeton University Press: Princeton, NJ, USA, 2009; p. 568. [Google Scholar]
- Kelner, S.R.; Aharonian, F.A. Energy spectra of gamma rays, electrons, and neutrinos produced at interactions of relativistic protons with low energy radiation. Phys. Rev. D 2008, 78, 034013. [Google Scholar] [CrossRef]
- Stecker, F.W. Effect of Photomeson Production by the Universal Radiation Field on High-Energy Cosmic Rays. Phys. Rev. Lett. 1968, 21, 1016–1018. [Google Scholar] [CrossRef]
- Ahlers, M.; Halzen, F. IceCube: Neutrinos and multimessenger astronomy. Prog. Theor. Exp. Phys. 2017, 2017, 12A105. [Google Scholar] [CrossRef]
- Blumenthal, G.R. Energy Loss of High-Energy Cosmic Rays in Pair-Producing Collisions with Ambient Photons. Phys. Rev. D 1970, 1, 1596–1602. [Google Scholar] [CrossRef]
- Chodorowski, M.J.; Zdziarski, A.A.; Sikora, M. Reaction Rate and Energy-Loss Rate for Photopair Production by Relativistic Nuclei. Astrophys. J. 1992, 400, 181. [Google Scholar] [CrossRef]
- Mastichiadis, A.; Protheroe, R.J.; Kirk, J.G. Spectral and temporal signatures of ultrarelativistic protons in compact sources. I. Effects of Bethe-Heitler pair production. Astron. Astrophys. 2005, 433, 765–776. [Google Scholar] [CrossRef]
- Petropoulou, M.; Mastichiadis, A. Bethe-Heitler emission in BL Lacs: Filling the gap between X-rays and γ-rays. Mon. Not. RAS 2015, 447, 36–48. [Google Scholar] [CrossRef]
- Ghisellini, G.; Tavecchio, F.; Maraschi, L.; Celotti, A.; Sbarrato, T. The power of relativistic jets is larger than the luminosity of their accretion disks. Nature 2014, 515, 376–378. [Google Scholar] [CrossRef] [PubMed]
- Marscher, A.P.; Jorstad, S.G.; D’Arcangelo, F.D.; Smith, P.S.; Williams, G.G.; Larionov, V.M.; Oh, H.; Olmstead, A.R.; Aller, M.F.; Aller, H.D.; et al. The inner jet of an active galactic nucleus as revealed by a radio-to-gamma-ray outburst. Nature 2008, 452, 966–969. [Google Scholar] [CrossRef] [PubMed]
- Walker, R.C.; Hardee, P.E.; Davies, F.B.; Ly, C.; Junor, W. The Structure and Dynamics of the Subparsec Jet in M87 Based on 50 VLBA Observations over 17 Years at 43 GHz. Astrophys. J. 2018, 855, 128. [Google Scholar] [CrossRef]
- Giommi, P.; Padovani, P.; Oikonomou, F.; Glauch, T.; Paiano, S.; Resconi, E. 3HSP J095507.9+355101: A flaring extreme blazar coincident in space and time with IceCube-200107A. Astron. Astrophys. 2020, 640, L4. [Google Scholar] [CrossRef]
- Blaufuss, E.; Kintscher, T.; Lu, L.; Tung, C.F. The Next Generation of IceCube Real-time Neutrino Alerts. In Proceedings of the 36th International Cosmic Ray Conference (ICRC2019), Madison, WA, USA, 24 July–1 August 2019; Volume 36, p. 1021. [Google Scholar]
- Abbasi, R.; et al. [IceCube Collaboration] IceCube-190819A—IceCube Observation of a High-Energy Neutrino Candidate Event; GRB Coordinates Network: Washington, DC, USA, 2019; Volume 25402, p. 1. [Google Scholar]
- Garrappa, S.; et al. [Fermi-LAT Collaboration] Fermi-LAT Gamma-ray Observations of IceCube-190819A; GRB Coordinates Network: Washington, DC, USA, 2019; Volume 25420, p. 1. [Google Scholar]
- Galvan, A.; et al. [HAWC Collaboration] IceCube-190819A—HAWC Follow-Up; GRB Coordinates Network: Washington, DC, USA, 2019; Volume 25411, p. 1. [Google Scholar]
- Molkov, S.; et al. [INTEGRAL Multi-MESSENGER Collaboration] IceCube-190819A: No Counterpart Candidates in INTEGRAL SPI-ACS Prompt Observation; GRB Coordinates Network: Washington, DC, USA, 2019; Volume 25403, p. 1. [Google Scholar]
- Abbasi, R.; et al. [IceCube Collaboration] IceCube-190922A—IceCube Observation of a High-Energy Neutrino Candidate Event; GRB Coordinates Network: Washington, DC, USA, 2019; Volume 25802, p. 1. [Google Scholar]
- Garrappa, S.; et al. [Fermi-LAT Collaboration] Fermi-LAT Gamma-ray Observations of IceCube-190922B; GRB Coordinates Network: Washington, DC, USA, 2019; Volume 25838, p. 1. [Google Scholar]
- Galvan, A.; et al. [HAWC Collaboration] IceCube-190922A—HAWC Follow-Up; GRB Coordinates Network: Washington, DC, USA, 2019; Volume 25805, p. 1. [Google Scholar]
- Onori, F.; et al. [INTEGRAL Multi-MESSENGER Collaboration] IceCube-190922A: No Counterpart Candidates in INTEGRAL SPI-ACS Prompt Observation; GRB Coordinates Network: Washington, DC, USA, 2019; Volume 25803, p. 1. [Google Scholar]
- Abbasi, R.; et al. [IceCube Collaboration] IceCube-200107A: IceCube Observation of a High-Energy Neutrino Candidate Event; GRB Coordinates Network: Washington, DC, USA, 2020; Volume 26655, p. 1. [Google Scholar]
- Ayala, H.; et al. [HAWC Collaboration] IceCube-200107A: No Significant Detection in HAWC; GRB Coordinates Network: Washington, DC, USA, 2020; Volume 26668, p. 1. [Google Scholar]
- Krauss, F.; Gregoire, T.; Fox, D.B.; Kennea, J.; Evans, P. Swift Follow-Up Observations of IceCube-200107A: Identification of X-ray High State for 4FGL J0955.1+3551; GRB Coordinates Network: Washington, DC, USA, 2020; Volume 26691, p. 1. [Google Scholar]
- Paliya, V.S.; Böttcher, M.; Olmo-García, A.; Domínguez, A.; Gil de Paz, A.; Franckowiak, A.; Garrappa, S.; Stein, R. Multifrequency Observations of the Candidate Neutrino-emitting Blazar BZB J0955+3551. Astrophys. J. 2020, 902, 29. [Google Scholar] [CrossRef]
- Finke, J.D.; Dermer, C.D.; Böttcher, M. Synchrotron Self-Compton Analysis of TeV X-ray-Selected BL Lacertae Objects. Astrophys. J. 2008, 686, 181–194. [Google Scholar] [CrossRef]
- Zyla, P.A.; et al. [Particle Data Group] Review of Particle Physics. Prog. Theor. Exp. Phys. 2020, 2020, 083C01. [Google Scholar] [CrossRef]
- Lipari, P.; Lusignoli, M.; Meloni, D. Flavor composition and energy spectrum of astrophysical neutrinos. Phys. Rev. D 2007, 75, 123005. [Google Scholar] [CrossRef]
- Chang, Y.L.; Arsioli, B.; Giommi, P.; Padovani, P.; Brandt, C.H. The 3HSP catalogue of extreme and high-synchrotron peaked blazars. Astron. Astrophys. 2019, 632, A77. [Google Scholar] [CrossRef]
- Liodakis, I.; Petropoulou, M. Proton Synchrotron Gamma-Rays and the Energy Crisis in Blazars. Astrophys. J. Lett. 2020, 893, L20. [Google Scholar] [CrossRef]
- Cerruti, M.; Zech, A.; Boisson, C.; Inoue, S. A hadronic origin for ultra-high-frequency-peaked BL Lac objects. Mon. Not. RAS 2015, 448, 910–927. [Google Scholar] [CrossRef]
- Cao, G.; Wang, J. The Hadronic Origin of the Hard Gamma-ray Spectrum from Blazar 1ES 1101-232. Astrophys. J. 2014, 783, 108. [Google Scholar] [CrossRef]
- Brightman, M.; Bachetti, M.; Earnshaw, H.P.; Fürst, F.; García, J.; Grefenstette, B.; Heida, M.; Kara, E.; Madsen, K.K.; Middleton, M.J.; et al. Breaking the limit: Super-Eddington accretion onto black holes and neutron stars. Bull. AAS 2019, 51, 352. [Google Scholar] [CrossRef]


| HE-Neutrino | R.A. | Dec | Coincident EHSP BL Lac | z | |||||
|---|---|---|---|---|---|---|---|---|---|
| (PeV) | (deg) | (deg) | () | ( Hz) | (Gpc) | ||||
| (1) | (2) | (3) | (4) | (5) | (6) | (7) | (8) | (9) | (10) |
| IC190819A | 0.113 | 29.26 | 1RXS J09462.5+010459 | 0.577 | 3.476 | ||||
| IC190922A | 128.86 | 1ES 1101-232 | 0.186 | 0.879 | |||||
| IC200107A | 0.330 | 20.85 | 3HSP J095507.9+355101 | 5 (≳20 ) | 0.557 | 3.332 |
| Outer Blob | |||
|---|---|---|---|
| 1RXS J09462.5+010459 | 1ES 1101-232 | 3HSP J095507.9+355101 | |
| 5 | 5 | ||
| 10 | 10 | 10 | |
| 1 | 10 | 50 | |
| 2 | 2 | 2 | |
| 1 | 1 | 1 | |
| 4.5 | 6.08 | 1.93 | |
| Inner Blob | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
|
1RXS J09462.5+010459 (Scenario A) |
1ES 1101-232 (Scenario A) |
3HSP J095507.9+355101 (Scenario B) | |||||||||
| 3 | 3 | 3 | |||||||||
| 1 | 1 | 1 | |||||||||
| 10 | 100 | 10 | |||||||||
| 2 | 2 | 2 | |||||||||
| 1 | 1 | 10 | 10 | ||||||||
| 1.3 | 1.9 | ||||||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aguilar-Ruiz, E.; Galván-Gámez, A.; Fraija, N. Testing a Lepto-Hadronic Two-Zone Model with Extreme High-Synchrotron Peaked BL Lacs and Track-like High-Energy Neutrinos. Galaxies 2023, 11, 117. https://doi.org/10.3390/galaxies11060117
Aguilar-Ruiz E, Galván-Gámez A, Fraija N. Testing a Lepto-Hadronic Two-Zone Model with Extreme High-Synchrotron Peaked BL Lacs and Track-like High-Energy Neutrinos. Galaxies. 2023; 11(6):117. https://doi.org/10.3390/galaxies11060117
Chicago/Turabian StyleAguilar-Ruiz, Edilberto, Antonio Galván-Gámez, and Nissim Fraija. 2023. "Testing a Lepto-Hadronic Two-Zone Model with Extreme High-Synchrotron Peaked BL Lacs and Track-like High-Energy Neutrinos" Galaxies 11, no. 6: 117. https://doi.org/10.3390/galaxies11060117
APA StyleAguilar-Ruiz, E., Galván-Gámez, A., & Fraija, N. (2023). Testing a Lepto-Hadronic Two-Zone Model with Extreme High-Synchrotron Peaked BL Lacs and Track-like High-Energy Neutrinos. Galaxies, 11(6), 117. https://doi.org/10.3390/galaxies11060117







