Dark Matter Effects on the Compact Star Properties
Abstract
:1. Introduction
2. Formalism
2.1. Dark Matter Admixed Neutron Star EOS
2.2. Mass and Radius of the NS
2.3. Tidal Love Numbers and Tidal Deformability of the NS
2.4. Shape/Surficial Love Numbers
2.5. Tidal Effects on the Inspiral Phase of BNS
3. Results and Discussions
3.1. Equation of State and Mass-Radius Relations
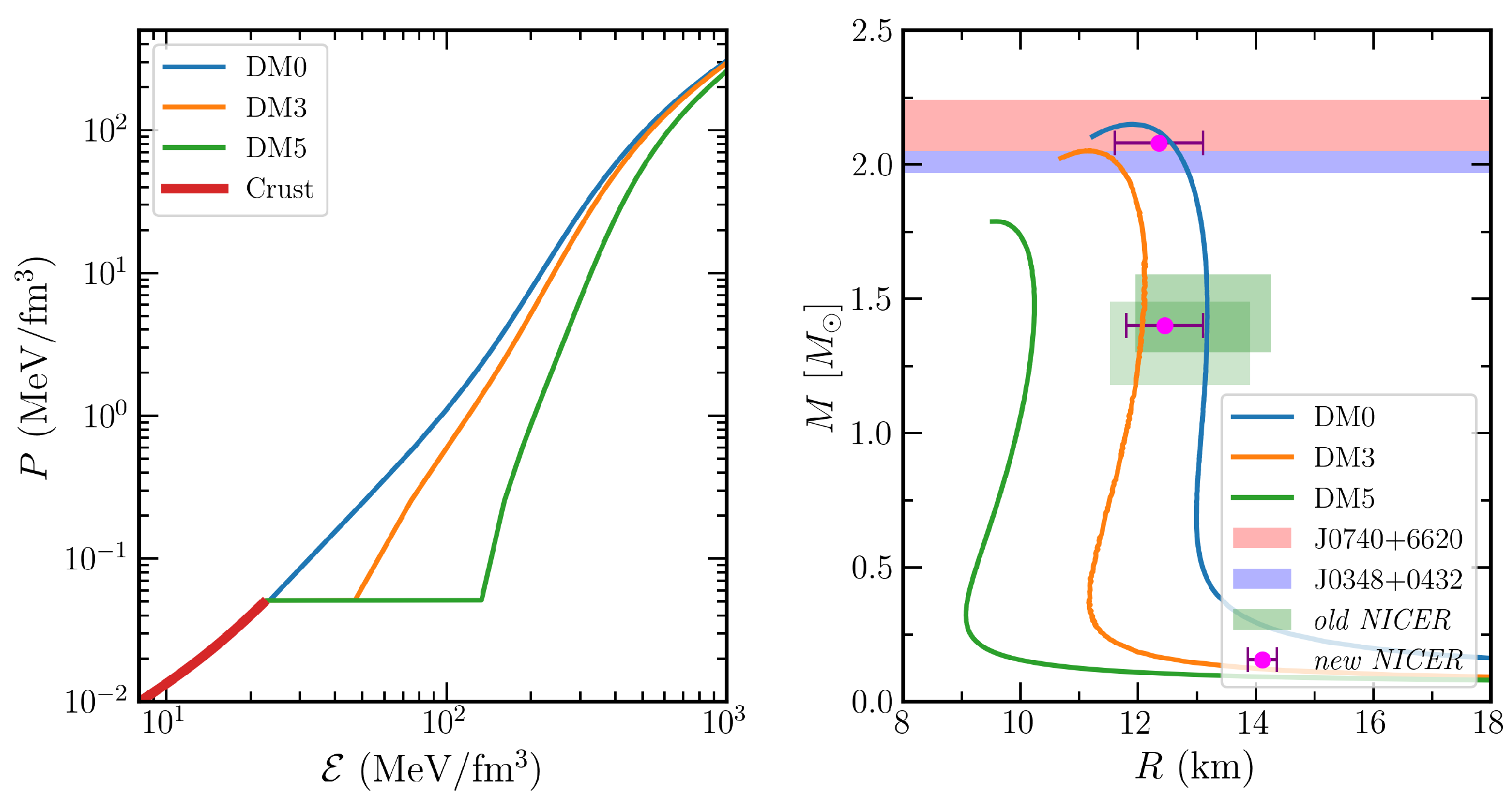
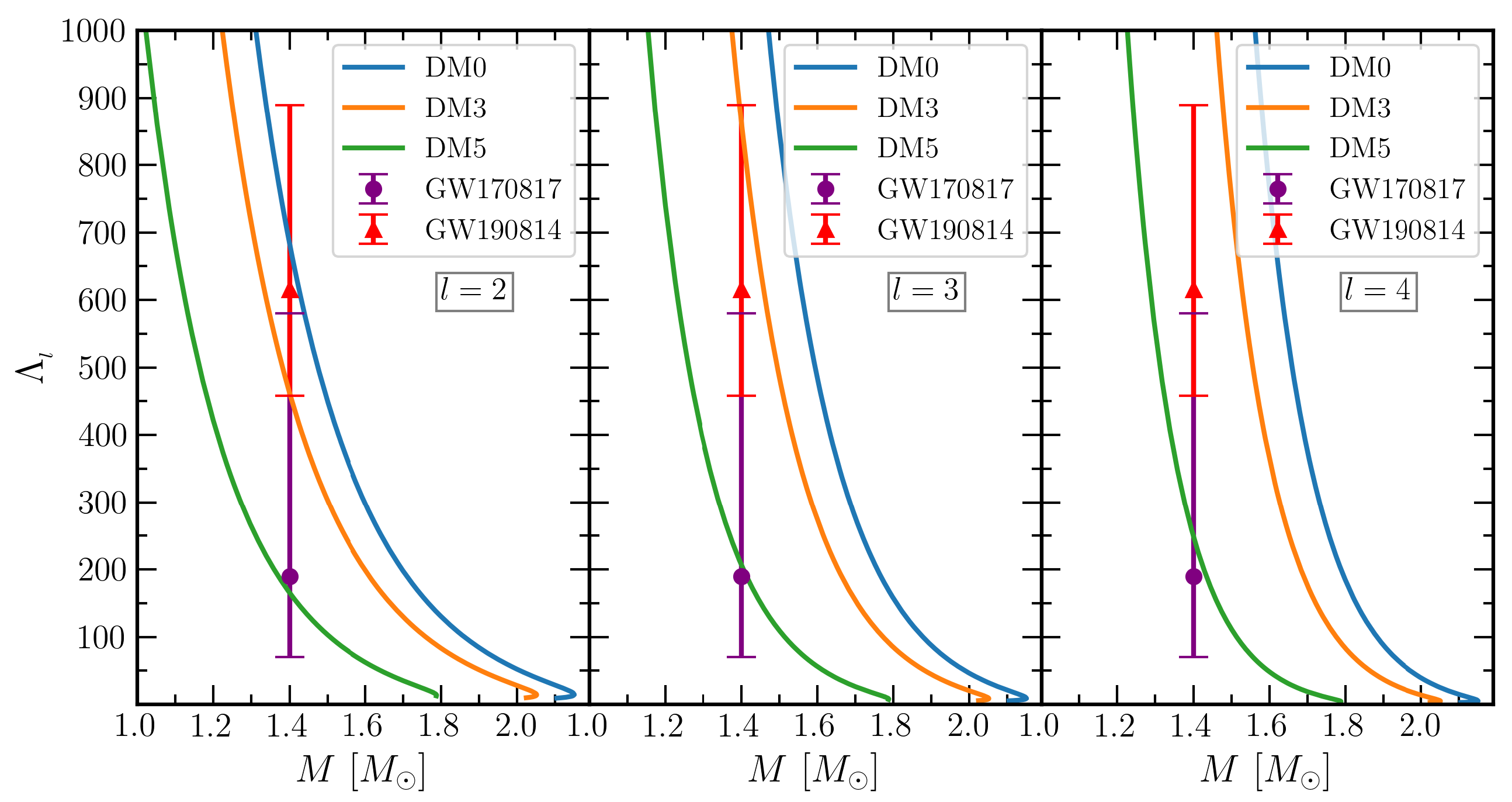
3.2. Electric Love Number and Tidal Deformability
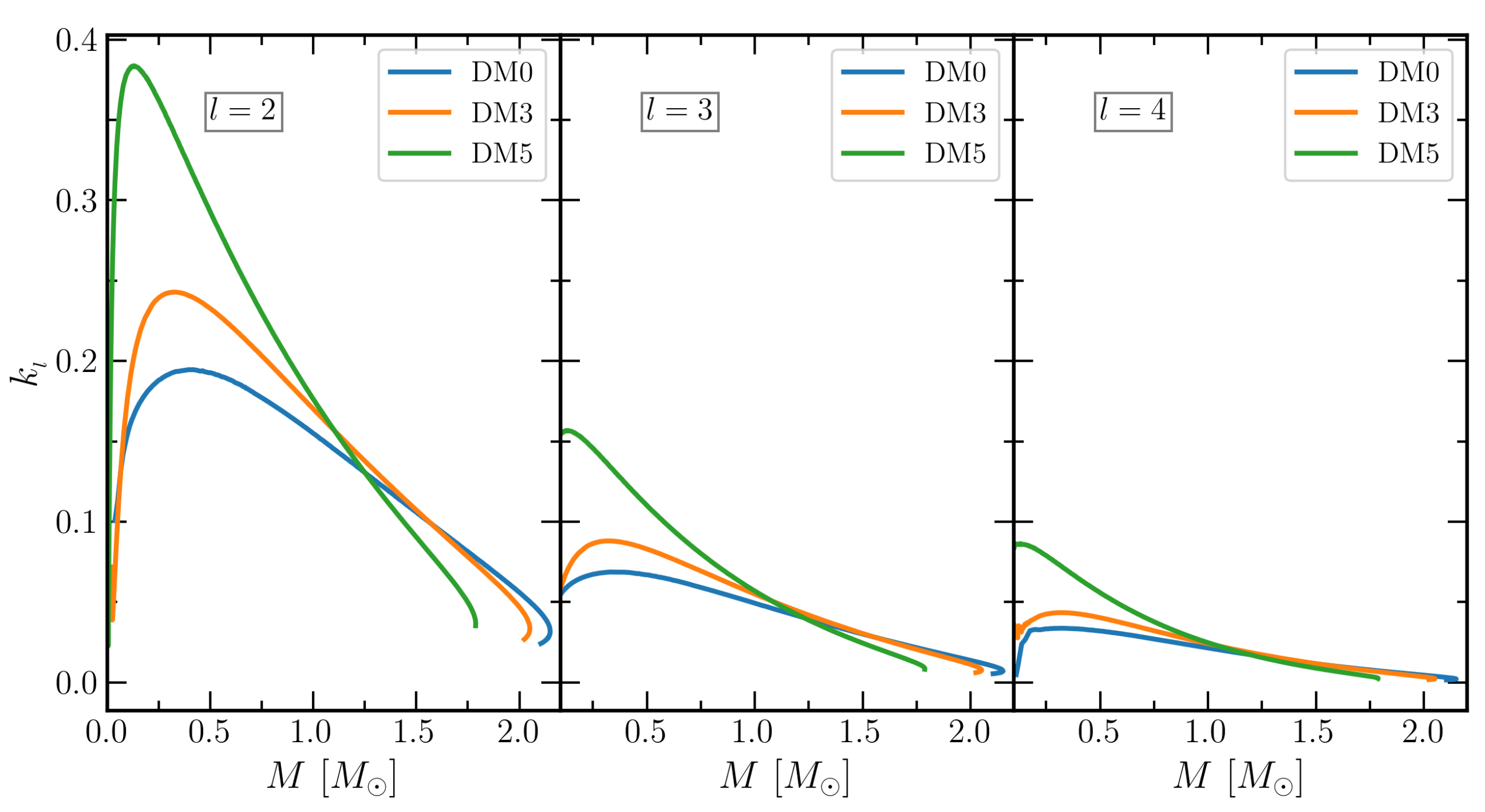
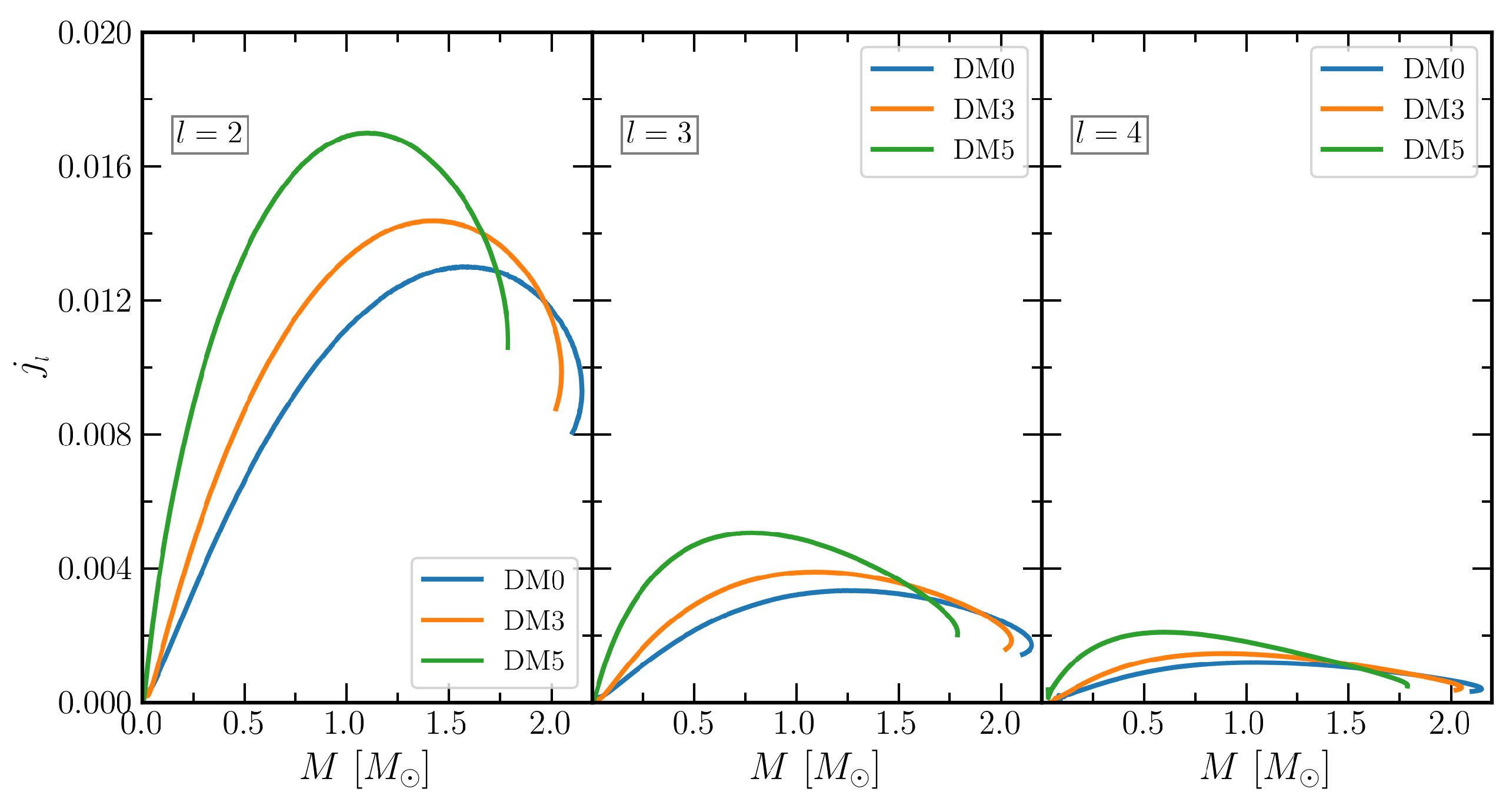
3.3. Magnetic Love Number and Tidal Deformability
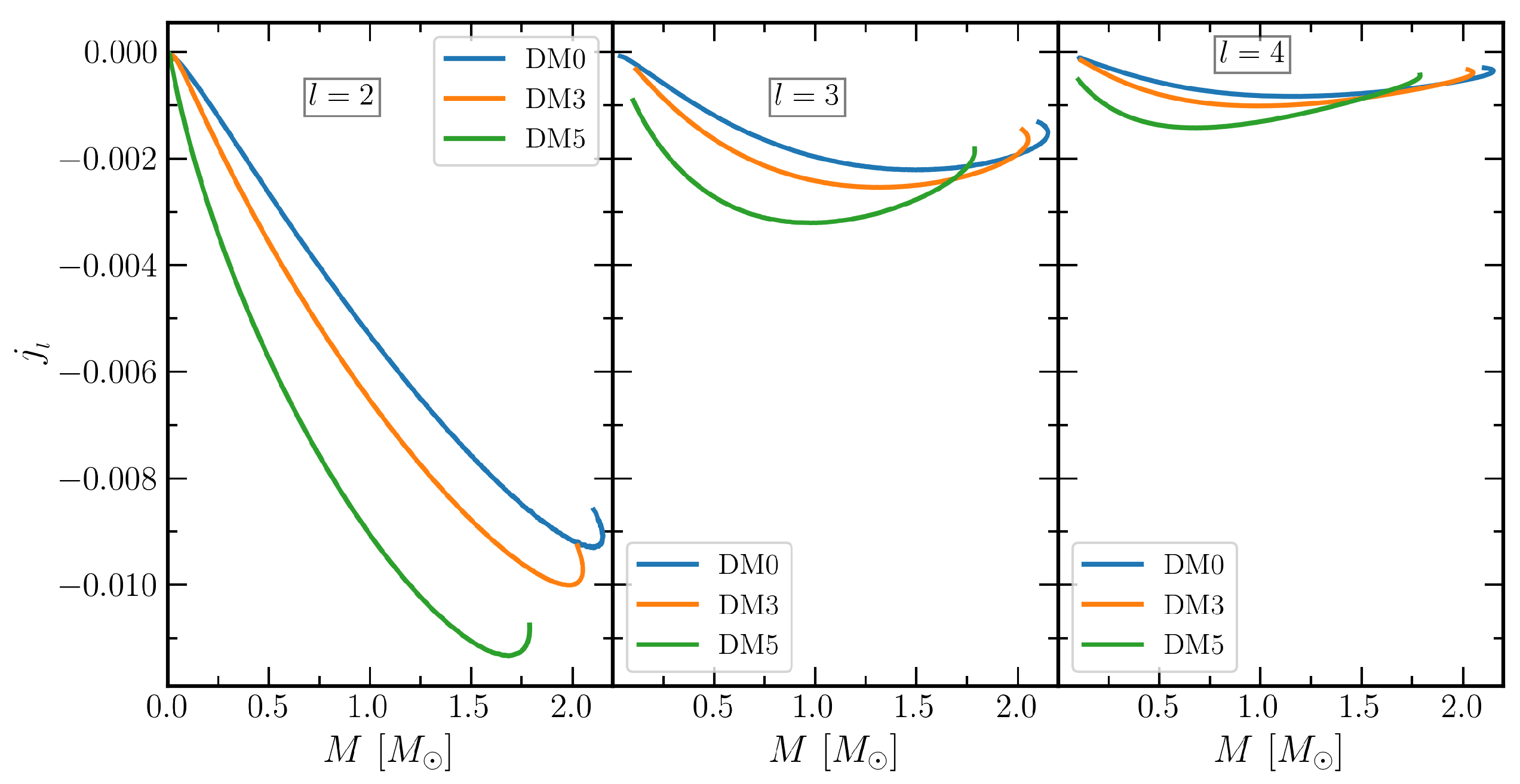
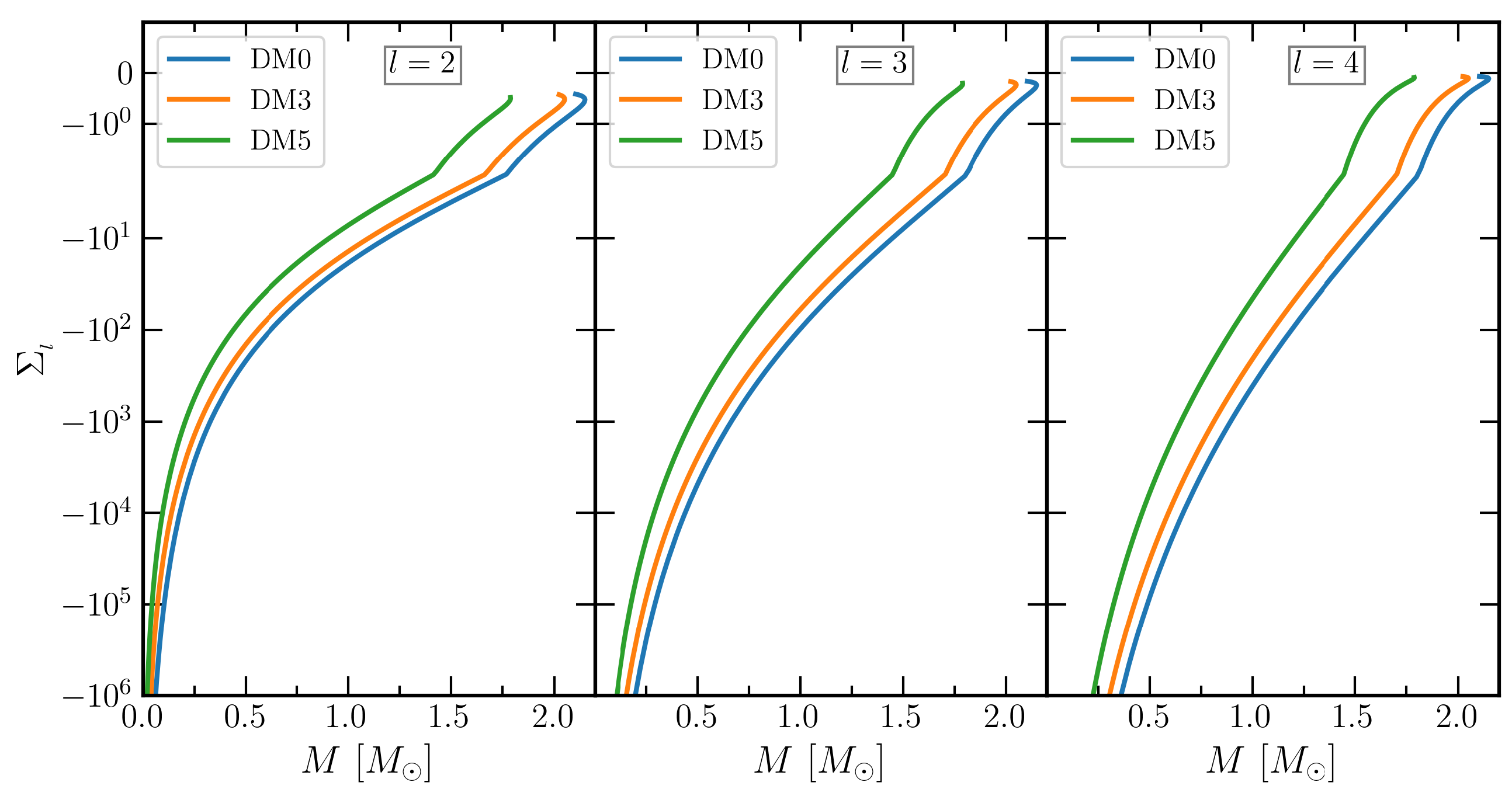
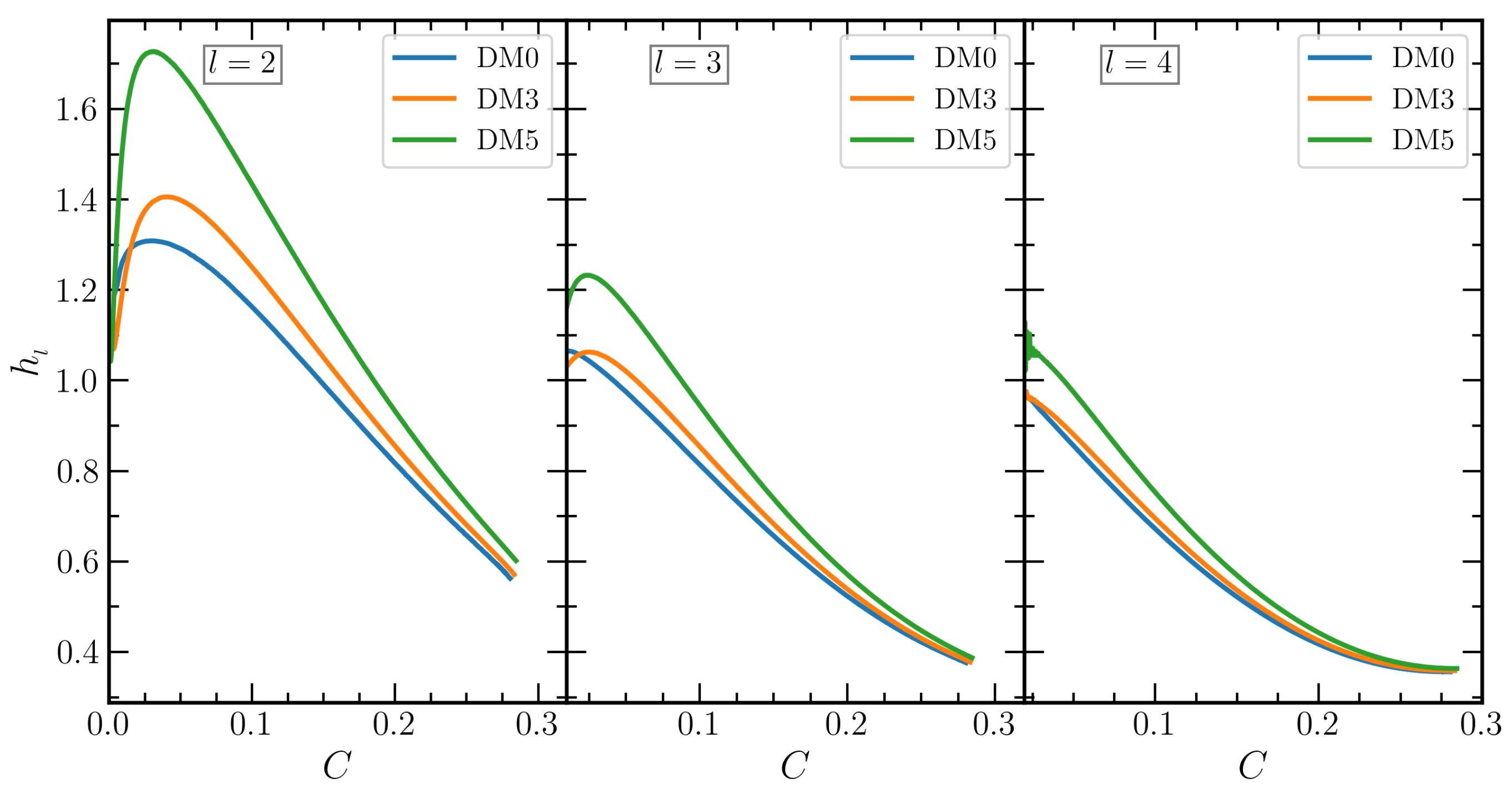
3.4. Surficial Love Numbers
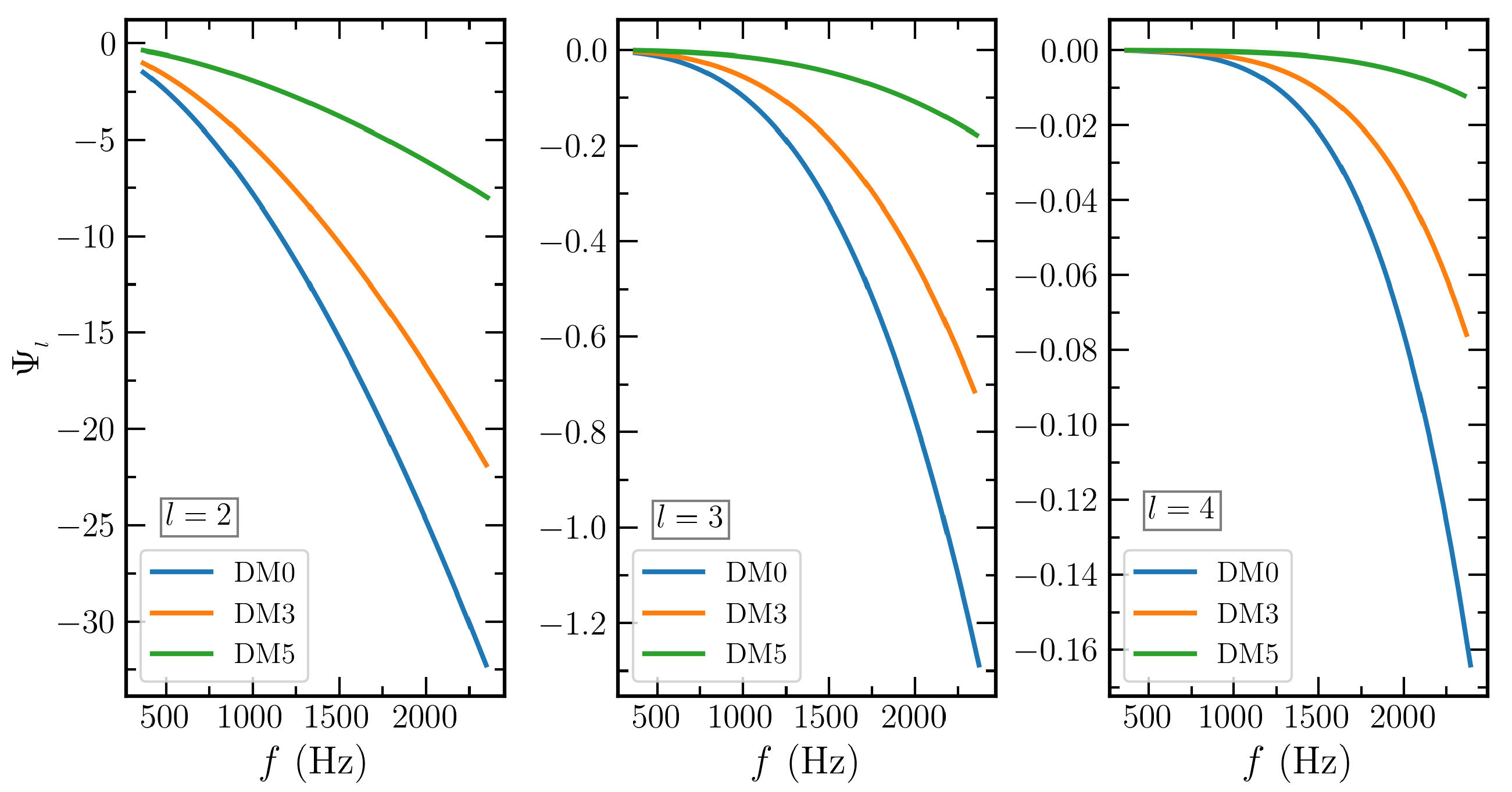
3.5. Tidal Effects on the GW Signal
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. GW170817: Observation of Gravitational Waves from a Binary Neutron Star Inspiral. Phys. Rev. Lett. 2017, 119, 161101. [Google Scholar] [CrossRef] [Green Version]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; et al. GW190425: Observation of a Compact Binary Coalescence with Total Mass ∼ 3.4 M ⊙. Astrophys. J. 2020, 892, L3. [Google Scholar] [CrossRef]
- LIGO. Instrument Science White Paper 2020; LIGO Document T2000407-v3; LIGO: Livingston, LA, USA, 2020. [Google Scholar]
- Accadia, T.; Acernese, F.; Alshourbagy, M.; Amico, P.; Antonucci, F.; Aoudia, S.; Arnaud, N.; Arnault, C.; Arun, K.G.; Astone, P.; et al. Virgo: A laser interferometer to detect gravitational waves. J. Instrum. 2012, 7, P03012. [Google Scholar] [CrossRef]
- Drout, M.R.; Piro, A.L.; Shappee, B.J.; Kilpatrick, C.D.; Simon, J.D.; Contreras, C.; Coulter, D.A.; Foley, R.J.; Siebert, M.R.; Morrell, N.; et al. Light curves of the neutron star merger GW170817/SSS17a: Implications for r-process nucleosynthesis. Science 2017, 358, 1570. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cowperthwaite, P.S.; Berger, E.; Villar, V.A.; Metzger, B.D.; Nicholl, M.; Chornock, R.; Blanchard, P.K.; Fong, W.; Margutti, R.; Soares-Santos, M.; et al. The Electromagnetic Counterpart of the Binary Neutron Star Merger LIGO/Virgo GW170817. II. UV, Optical, and Near-infrared Light Curves and Comparison to Kilonova Models. Astrophys. J. 2017, 848, L17. [Google Scholar] [CrossRef]
- Chornock, R.; Berger, E.; Kasen, D.; Cowperthwaite, P.S.; Nicholl, M.; Villar, V.A.; Alexander, K.D.; Blanchard, P.K.; Eftekhari, T.; Fong, W.; et al. The Electromagnetic Counterpart of the Binary Neutron Star Merger LIGO/Virgo GW170817. IV. Detection of Near-infrared Signatures of r -process Nucleosynthesis with Gemini-South. Astrophys. J. 2017, 848, L19. [Google Scholar] [CrossRef] [Green Version]
- Nicholl, M.; Berger, E.; Kasen, D.; Metzger, B.D.; Elias, J.; Briceno, C.; Alexander, K.D.; Blanchard, P.K.; Chornock, R.; Cowperthwaite, P.S.; et al. The Electromagnetic Counterpart of the Binary Neutron Star Merger LIGO/Virgo GW170817. III. Optical and UV Spectra of a Blue Kilonova from Fast Polar Ejecta. ApJL 2017, 848, L18. [Google Scholar] [CrossRef]
- Margalit, B.; Metzger, B.D. Constraining the Maximum Mass of Neutron Stars from Multi-messenger Observations of GW170817. Astrophys. J. 2017, 850, L19. [Google Scholar] [CrossRef]
- Rezzolla, L.; Most, E.R.; Weih, L.R. Using Gravitational-wave Observations and Quasi-universal Relations to Constrain the Maximum Mass of Neutron Stars. Astrophys. J. 2018, 852, L25. [Google Scholar] [CrossRef]
- Shibata, M.; Zhou, E.; Kiuchi, K.; Fujibayashi, S. Constraint on the maximum mass of neutron stars using GW170817 event. Phys. Rev. D 2019, 100, 023015. [Google Scholar] [CrossRef] [Green Version]
- Demorest, P.B.; Pennucci, T.; Ransom, S.M.; Roberts, M.S.E.; Hessels, J.W.T. A two-solar-mass neutron star measured using Shapiro delay. Nature 2010, 467, 1081–1083. [Google Scholar] [CrossRef]
- Antoniadis, J.; Freire, P.C.C.; Wex, N.; Tauris, T.M.; Lynch, R.S.; van Kerkwijk, M.H.; Kramer, M.; Bassa, C.; Dhillon, V.S.; Driebe, T.; et al. A Massive Pulsar in a Compact Relativistic Binary. Science 2013, 340, 1233232. [Google Scholar] [CrossRef] [Green Version]
- Cromartie, H.T.; Fonseca, E.; Ransom, S.M.; Demorest, P.B.; Arzoumanian, Z.; Blumer, H.; Brook, P.R.; DeCesar, M.E.; Dolch, T.; Ellis, J.A.; et al. Relativistic Shapiro delay measurements of an extremely massive millisecond pulsar. Nat. Astron. 2020, 4, 72–76. [Google Scholar] [CrossRef] [Green Version]
- Miller, M.C.; Lamb, F.K.; Dittmann, A.J.; Bogdanov, S.; Arzoumanian, Z.; Gendreau, K.C.; Guillot, S.; Harding, A.K.; Ho, W.C.G.; Lattimer, J.M.; et al. PSR J0030+0451 Mass and Radius from NICER Data and Implications for the Properties of Neutron Star Matter. APJ 2019, 887, L24. [Google Scholar] [CrossRef] [Green Version]
- Riley, T.E.; Watts, A.L.; Bogdanov, S.; Ray, P.S.; Ludlam, R.M.; Guillot, S.; Arzoumanian, Z.; Baker, C.L.; Bilous, A.V.; Chakrabarty, D.; et al. A NICER View of PSR J0030+0451: Millisecond Pulsar Parameter Estimation. APJ 2018, 887, L21. [Google Scholar] [CrossRef] [Green Version]
- Raaijmakers, G.; Riley, T.E.; Watts, A.L.; Greif, S.K.; Morsink, S.M.; Hebeler, K.; Schwenk, A.; Hinderer, T.; Nissanke, S.; Guillot, S.; et al. A NICER View of PSR J0030+0451: Implications for the Dense Matter Equation of State. Astrophys. J 2019, 887, L22. [Google Scholar] [CrossRef] [Green Version]
- Miller, M.C.; Lamb, F.K.; Dittmann, A.J.; Bogdanov, S.; Arzoumanian, Z.; Gendreau, K.C.; Guillot, S.; Ho, W.C.G.; Lattimer, J.M.; Loewenstein, M.; et al. The Radius of PSR J0740+6620 from NICER and XMM-Newton Data. Astrophys. J. Lett. 2021, 918, L28. [Google Scholar] [CrossRef]
- Hinderer, T. Tidal Love Numbers of Neutron Stars. Astrophys. J. 2008, 677, 1216–1220, Erratum in Astrophys. J. 2009, 697, 964. [Google Scholar] [CrossRef]
- Hinderer, T.; Lackey, B.D.; Lang, R.N.; Read, J.S. Tidal deformability of neutron stars with realistic equations of state and their gravitational wave signatures in binary inspiral. Phys. Rev. D 2010, 81, 123016. [Google Scholar] [CrossRef] [Green Version]
- Agathos, M.; Meidam, J.; Del Pozzo, W.; Li, T.G.F.; Tompitak, M.; Veitch, J.; Vitale, S.; Van Den Broeck, C. Constraining the neutron star equation of state with gravitational wave signals from coalescing binary neutron stars. Phys. Rev. D 2015, 92, 023012. [Google Scholar] [CrossRef] [Green Version]
- Fattoyev, F.J.; Piekarewicz, J.; Horowitz, C.J. Neutron Skins and Neutron Stars in the Multimessenger Era. Phys. Rev. Lett. 2018, 120, 172702. [Google Scholar] [CrossRef] [Green Version]
- Malik, T.; Alam, N.; Fortin, M.; Providência, C.; Agrawal, B.K.; Jha, T.K.; Kumar, B.; Patra, S.K. GW170817: Constraining the nuclear matter equation of state from the neutron star tidal deformability. Phys. Rev. C 2018, 98, 035804. [Google Scholar] [CrossRef]
- Most, E.R.; Weih, L.R.; Rezzolla, L.; Schaffner-Bielich, J. New Constraints on Radii and Tidal Deformabilities of Neutron Stars from GW170817. Phys. Rev. Lett. 2018, 120, 261103. [Google Scholar] [CrossRef] [Green Version]
- Raithel, C.A.; Özel, F.; Psaltis, D. Tidal Deformability from GW170817 as a Direct Probe of the Neutron Star Radius. Astrophys. J. 2018, 857, L23. [Google Scholar] [CrossRef]
- Christian, J.E.; Zacchi, A.; Schaffner-Bielich, J. Signals in the tidal deformability for phase transitions in compact stars with constraints from GW170817. Phys. Rev. D 2019, 99, 023009. [Google Scholar] [CrossRef] [Green Version]
- Han, S.; Steiner, A.W. Tidal deformability with sharp phase transitions in binary neutron stars. Phys. Rev. D 2019, 99, 083014. [Google Scholar] [CrossRef] [Green Version]
- Fattoyev, F.J.; Horowitz, C.J.; Piekarewicz, J.; Reed, B. GW190814: Impact of a 2.6 solar mass neutron star on the nucleonic equations of state. Phys. Rev. C 2020, 102, 065805. [Google Scholar] [CrossRef]
- Andersson, N.; Pnigouras, P. Exploring the effective tidal deformability of neutron stars. Phys. Rev. D 2020, 101, 083001. [Google Scholar] [CrossRef] [Green Version]
- Sabatucci, A.; Benhar, O. Tidal deformation of neutron stars from microscopic models of nuclear dynamics. Phys. Rev. C 2020, 101, 045807. [Google Scholar] [CrossRef]
- Perot, L.; Chamel, N.; Sourie, A. Role of the crust in the tidal deformability of a neutron star within a unified treatment of dense matter. Phys. Rev. C 2020, 101, 015806. [Google Scholar] [CrossRef] [Green Version]
- Perot, L.; Chamel, N. Role of dense matter in tidal deformations of inspiralling neutron stars and in gravitational waveforms with unified equations of state. Phys. Rev. C 2021, 103, 025801. [Google Scholar] [CrossRef]
- Akutsu, T.; Ando, M.; Arai, K.; Arai, Y.; Araki, S.; Araya, A.; Aritomi, N.; Aso, Y.; Bae, S.; Bae, Y.; et al. Overview of KAGRA: Detector design and construction history. arXiv 2020, arXiv:2005.05574. [Google Scholar] [CrossRef]
- Kumar, B.; Biswal, S.K.; Patra, S.K. Tidal deformability of neutron and hyperon stars within relativistic mean field equations of state. Phys. Rev. C 2017, 95, 015801. [Google Scholar] [CrossRef] [Green Version]
- Yagi, K. Multipole Love relations. Phys. Rev. D 2014, 89, 043011. [Google Scholar] [CrossRef] [Green Version]
- Vines, J.; Flanagan, E.E.; Hinderer, T. Post-1-Newtonian tidal effects in the gravitational waveform from binary inspirals. Phys. Rev. D 2011, 83, 084051. [Google Scholar] [CrossRef] [Green Version]
- Ellis, J.; Hütsi, G.; Kannike, K.; Marzola, L.; Raidal, M.; Vaskonen, V. Dark matter effects on neutron star properties. Phys. Rev. D 2018, 97, 123007. [Google Scholar] [CrossRef] [Green Version]
- Das, H.C.; Kumar, A.; Kumar, B.; Biswal, S.K.; Nakatsukasa, T.; Li, A.; Patra, S.K. Effects of dark matter on the nuclear and neutron star matter. MNRAS 2020, 495, 4893–4903. [Google Scholar] [CrossRef]
- Das, H.C.; Kumar, A.; Patra, S.K. Effects of dark matter on the in-spiral properties of the binary neutron stars. MNRAS 2021, 507, 4053–4060. [Google Scholar] [CrossRef]
- Das, H.C.; Kumar, A.; Patra, S.K. Dark matter admixed neutron star as a possible compact component in the GW190814 merger event. Phys. Rev. D 2021, 104, 063028. [Google Scholar] [CrossRef]
- Kouvaris, C.; Tinyakov, P. Constraining asymmetric dark matter through observations of compact stars. Phys. Rev. D 2011, 83, 083512. [Google Scholar] [CrossRef] [Green Version]
- Hooper, D.; Wang, L.T. Direct and indirect detection of neutralino dark matter in selected supersymmetry breaking scenarios. Phys. Rev. D 2004, 69, 035001. [Google Scholar] [CrossRef] [Green Version]
- Han, T.; Liu, Z.; Su, S. Light neutralino dark matter: Direct/indirect detection and collider searches. JHEP 2014, 2014, 93. [Google Scholar] [CrossRef] [Green Version]
- Das, A.; Malik, T.; Nayak, A.C. Confronting nuclear equation of state in the presence of dark matter using GW170817 observation in relativistic mean field theory approach. Phys. Rev. D 2019, 99, 043016. [Google Scholar] [CrossRef] [Green Version]
- Bernal, N.; Heikinheimo, M.; Tenkanen, T.; Tuominen, K.; Vaskonen, V. The dawn of FIMP Dark Matter: A review of models and constraints. IJMP A 2017, 32, 1730023. [Google Scholar] [CrossRef]
- Hall, L.J.; Jedamzik, K.; March-Russell, J.; West, S.M. Freeze-in production of FIMP dark matter. JHEP 2010, 2010, 80. [Google Scholar] [CrossRef] [Green Version]
- Duffy, L.D.; van Bibber, K. Axions as dark matter particles. New J. Phys. 2009, 11, 105008. [Google Scholar] [CrossRef]
- Agnese, R.; Ahmed, Z.; Anderson, A.J.; Arrenberg, S.; Balakishiyeva, D.; Thakur, R.B.; Bauer, D.A.; Billard, J.; Borgland, A.; Brandt, D.; et al. Silicon Detector Dark Matter Results from the Final Exposure of CDMS II. Phys. Rev. Lett. 2013, 111, 251301. [Google Scholar] [CrossRef]
- Akerib, D.S.; Araújo, H.M.; Bai, X.; Bailey, A.J.; Balajthy, J.; Bedikian, S.; Bernard, E.; Bernstein, A.; Bolozdynya, A.; Bradley, A.; et al. First Results from the LUX Dark Matter Experiment at the Sanford Underground Research Facility. Phys. Rev. Lett. 2014, 112, 091303. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Conrad, J. Indirect Detection of WIMP Dark Matter: A compact review. arXiv 2014, arXiv:1411.1925. [Google Scholar]
- Kouvaris, C. WIMP annihilation and cooling of neutron stars. Phys. Rev. D 2008, 77, 023006. [Google Scholar] [CrossRef] [Green Version]
- Bhat, S.A.; Paul, A. Cooling of dark-matter admixed neutron stars with density-dependent equation of state. Eur. Phys. J. 2020, 80, 544. [Google Scholar] [CrossRef]
- De Lavallaz, A.; Fairbairn, M. Neutron stars as dark matter probes. Phys. Rev. D 2010, 81, 123521. [Google Scholar] [CrossRef] [Green Version]
- Ciarcelluti, P.; Sandin, F. Have neutron stars a dark matter core? Phys. Lett. B 2011, 695, 19–21. [Google Scholar] [CrossRef] [Green Version]
- Rafiei Karkevandi, D.; Shakeri, S.; Sagun, V.; Ivanytskyi, O. Bosonic dark matter in neutron stars and its effect on gravitational wave signal. Phys. Rev. D 2022, 105, 023001. [Google Scholar] [CrossRef]
- Das, H.C.; Kumar, A.; Kumar, B.; Biswal, S.K.; Patra, S.K. Impacts of dark matter on the curvature of the neutron star. JCAP 2021, 2021, 7. [Google Scholar] [CrossRef]
- Adhikari, R.X. Gravitational radiation detection with laser interferometry. Rev. Mod. Phys. 2014, 86, 121–151. [Google Scholar] [CrossRef]
- Punturo, M.; Abernathy, M.; Acernese, F.; Allen, B.; Andersson, N.; Arun, K.; Barone, F.; Barr, B.; Barsuglia, M.; Beker, M.; et al. The Einstein Telescope: A third-generation gravitational wave observatory. Class. Quant. Grav. 2010, 27, 194002. [Google Scholar] [CrossRef]
- Kumar, B.; Singh, S.; Agrawal, B.; Patra, S. New parameterization of the effective field theory motivated relativistic mean field model. Nuclear Phys. A 2017, 966, 197–207. [Google Scholar] [CrossRef] [Green Version]
- Kumar, B.; Patra, S.K.; Agrawal, B.K. New relativistic effective interaction for finite nuclei, infinite nuclear matter, and neutron stars. Phys. Rev. C 2018, 97, 045806. [Google Scholar] [CrossRef] [Green Version]
- Kumar, A.; Das, H.C.; Biswal, S.K.; Kumar, B.; Patra, S.K. Warm dense matter and cooling of supernovae remnants. Eur. Phys. J. C 2020, 80, 775. [Google Scholar] [CrossRef]
- Parmar, V.; Das, H.C.; Kumar, A.; Sharma, M.K.; Patra, S.K. Crustal properties of the neutron star within effective relativistic mean-field model. arXiv 2021, arXiv:2111.07278. [Google Scholar]
- Glendenning, N.K. Compact Stars; Springer: New York, NY, USA, 1997. [Google Scholar] [CrossRef]
- Das, H.C.; Kumar, A.; Biswal, S.K.; Patra, S.K. Impacts of dark matter on the f-mode oscillation of hyperon star. Phys. Rev. D 2021, 104, 123006. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Lopes, I. Dark matter effect on realistic equation of state in neutron stars. Phys. Rev. D 2017, 96, 083004. [Google Scholar] [CrossRef] [Green Version]
- Quddus, A.; Panotopoulos, G.; Kumar, B.; Ahmad, S.; Patra, S.K. GW170817 constraints on the properties of a neutron star in the presence of WIMP dark matter. J. Phys. Nucl. Part. Phys. 2020, 47, 095202. [Google Scholar] [CrossRef]
- Cline, J.M.; Scott, P.; Kainulainen, K.; Weniger, C. Update on scalar singlet dark matter. Phys. Rev. D 2013, 88, 055025. [Google Scholar] [CrossRef] [Green Version]
- Aprile, E.; Aalbers, J.; Agostini, F.; Alfonsi, M.; Amaro, F.D.; Anthony, M.; Arazi, L.; Arneodo, F.; Balan, C.; Barrow, P.; et al. Physics reach of the XENON1T dark matter experiment. JCAP 2016, 2016, 27. [Google Scholar] [CrossRef] [Green Version]
- Tan, A.; Xiao, M.; Cui, X.; Chen, X.; Chen, Y.; Fang, D.; Fu, C.; Giboni, K.; Giuliani, F.; Gong, H.; et al. Dark Matter Results from First 98.7 Days of Data from the PandaX-II Experiment. Phys. Rev. Lett. 2016, 117, 121303. [Google Scholar] [CrossRef]
- Meng, Y.; Wang, Z.; Tao, Y.; Abdukerim, A.; Bo, Z.; Chen, W.; Chen, X.; Chen, Y.; Cheng, C.; Cheng, Y.; et al. Dark Matter Search Results from the PandaX-4T Commissioning Run. arXiv 2021, arXiv:2107.13438. [Google Scholar] [CrossRef]
- Akerib, D.S.; Alsum, S.; Araújo, H.M.; Bai, X.; Bailey, A.J.; Balajthy, J.; Beltrame, P.; Bernard, E.P.; Bernstein, A.; Biesiadzinski, T.P.; et al. Results from a Search for Dark Matter in the Complete LUX Exposure. Phys. Rev. Lett. 2017, 118, 021303. [Google Scholar] [CrossRef]
- Djouadi, A.; Lebedev, O.; Mambrini, Y.; Quevillon, J. Implications of LHC searches for Higgs-portal dark matter. Phys. Lett. B 2012, 709, 65–69. [Google Scholar] [CrossRef]
- Czarnecki, A.; Körner, J.G.; Piclum, J.H. Helicity fractions of W bosons from top quark decays at next-to-next-to-leading order in QCD. Phys. Rev. D 2010, 81, 111503. [Google Scholar] [CrossRef] [Green Version]
- Toussaint, D.; Freeman, W. Strange Quark Condensate in the Nucleon in 2+1 Flavor QCD. Phys. Rev. Lett. 2009, 103, 122002. [Google Scholar] [CrossRef] [Green Version]
- Aad, G.; Abbott, B.; Abdallah, J.; Abdinov, O.; Aben, R.; Abolins, M.; AbouZeid, O.S.; Abramowicz, H.; Abreu, H.; Abreu, R.; et al. Constraints on new phenomena via Higgs boson couplings and invisible decays with the ATLAS detector. J. High Energy Phys. 2015, 2015, 206. [Google Scholar] [CrossRef] [Green Version]
- Tolman, R.C. Static Solutions of Einstein’s Field Equations for Spheres of Fluid. Phys. Rev. 1939, 55, 364–373. [Google Scholar] [CrossRef] [Green Version]
- Oppenheimer, J.R.; Volkoff, G.M. On Massive Neutron Cores. Phys. Rev. 1939, 55, 374–381. [Google Scholar] [CrossRef]
- Damour, T.; Nagar, A. Relativistic tidal properties of neutron stars. Phys. Rev. D 2009, 80, 084035. [Google Scholar] [CrossRef] [Green Version]
- Landry, P.; Poisson, E. Relativistic theory of surficial Love numbers. Phys. Rev. D 2014, 89, 124011. [Google Scholar] [CrossRef] [Green Version]
- Damour, T.; Lecian, O.M. Gravitational polarizability of black holes. Phys. Rev. D 2009, 80, 044017. [Google Scholar] [CrossRef] [Green Version]
- Blanchet, L. Gravitational Radiation from Post-Newtonian Sources and Inspiralling Compact Binaries. Living Rev. Relativ. 2006, 9, 4. [Google Scholar] [CrossRef] [Green Version]
- Boyle, M.; Brown, D.A.; Kidder, L.E.; Mroué, A.H.; Pfeiffer, H.P.; Scheel, M.A.; Cook, G.B.; Teukolsky, S.A. High-accuracy comparison of numerical relativity simulations with post-Newtonian expansions. Phys. Rev. D 2007, 76, 124038. [Google Scholar] [CrossRef] [Green Version]
- Blanchet, L.; Faye, G.; Iyer, B.R.; Sinha, S. The third post-Newtonian gravitational wave polarizations and associated spherical harmonic modes for inspiralling compact binaries in quasi-circular orbits. Class. Quantum Gravity 2008, 25, 165003. [Google Scholar] [CrossRef] [Green Version]
- Baiotti, L.; Damour, T.; Giacomazzo, B.; Nagar, A.; Rezzolla, L. Accurate numerical simulations of inspiralling binary neutron stars and their comparison with effective-one-body analytical models. Phys. Rev. D 2011, 84, 024017. [Google Scholar] [CrossRef] [Green Version]
- Hotokezaka, K.; Kyutoku, K.; Shibata, M. Exploring tidal effects of coalescing binary neutron stars in numerical relativity. Phys. Rev. D 2013, 87, 044001. [Google Scholar] [CrossRef] [Green Version]
- Hotokezaka, K.; Kyutoku, K.; Sekiguchi, Y.i.; Shibata, M. Measurability of the tidal deformability by gravitational waves from coalescing binary neutron stars. Phys. Rev. D 2016, 93, 064082. [Google Scholar] [CrossRef] [Green Version]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. GW170817: Measurements of Neutron Star Radii and Equation of State. Phys. Rev. Lett. 2018, 121, 161101. [Google Scholar] [CrossRef] [Green Version]
- Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; Agathos, M.; et al. GW190814: Gravitational Waves from the Coalescence of a 23 Solar Mass Black Hole with a 2.6 Solar Mass Compact Object. Astrophys. J. 2020, 896, L44. [Google Scholar] [CrossRef]
- Huang, K.; Hu, J.; Zhang, Y.; Shen, H. The Possibility of the Secondary Object in GW190814 as a Neutron Star. Astrophys. J. 2020, 904, 39. [Google Scholar] [CrossRef]
- Tan, H.; Noronha-Hostler, J.; Yunes, N. Neutron Star Equation of State in Light of GW190814. Phys. Rev. Lett. 2020, 125, 261104. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, M.; Xia, C.J.; Li, Z.; Biswal, S.K. Constraints on the symmetry energy and its associated parameters from nuclei to neutron stars. Phys. Rev. C 2020, 101, 034303. [Google Scholar] [CrossRef] [Green Version]
- Das, H.C.; Kumar, A.; Kumar, B.; Biswal, S.K.; Patra, S.K. BigApple force and its implications to finite nuclei and astrophysical objects. Int. J. Mod. Phys. E 2021, 30, 2150088. [Google Scholar] [CrossRef]
- Sarin, N.; Lasky, P.D. The evolution of binary neutron star post-merger remnants: A review. Gen. Relativ. Gravit. 2021, 53, 59. [Google Scholar] [CrossRef]
- Pollack, J.; Spergel, D.N.; Steinhardt, P.J. Supermassive Black Holes from ULTRA-Strongly Self-Interacting Dark Matter. Astrophys. J. 2015, 804, 131. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Das, H.C.; Kumar, A.; Kumar, B.; Patra, S.K. Dark Matter Effects on the Compact Star Properties. Galaxies 2022, 10, 14. https://doi.org/10.3390/galaxies10010014
Das HC, Kumar A, Kumar B, Patra SK. Dark Matter Effects on the Compact Star Properties. Galaxies. 2022; 10(1):14. https://doi.org/10.3390/galaxies10010014
Chicago/Turabian StyleDas, H. C., Ankit Kumar, Bharat Kumar, and Suresh Kumar Patra. 2022. "Dark Matter Effects on the Compact Star Properties" Galaxies 10, no. 1: 14. https://doi.org/10.3390/galaxies10010014
APA StyleDas, H. C., Kumar, A., Kumar, B., & Patra, S. K. (2022). Dark Matter Effects on the Compact Star Properties. Galaxies, 10(1), 14. https://doi.org/10.3390/galaxies10010014





