Retinal Vessel Local Tortuosity under a Macula-to-Optic Disc Central-Framing Change
Abstract
1. Introduction
2. Materials and Methods
2.1. Local Tortuosity Indices
2.2. Participants and Equipment
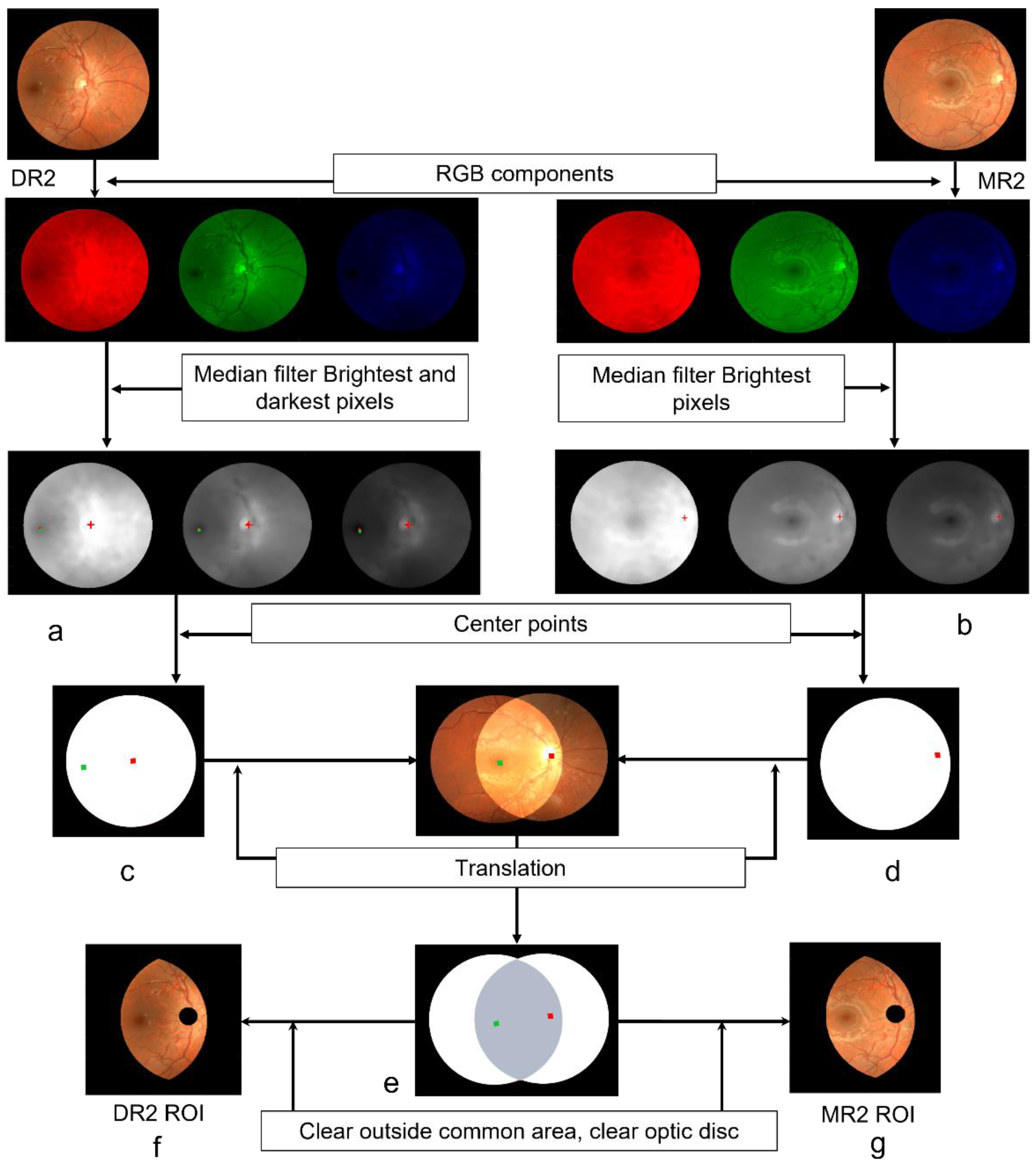
2.3. Region of Interest (ROI)
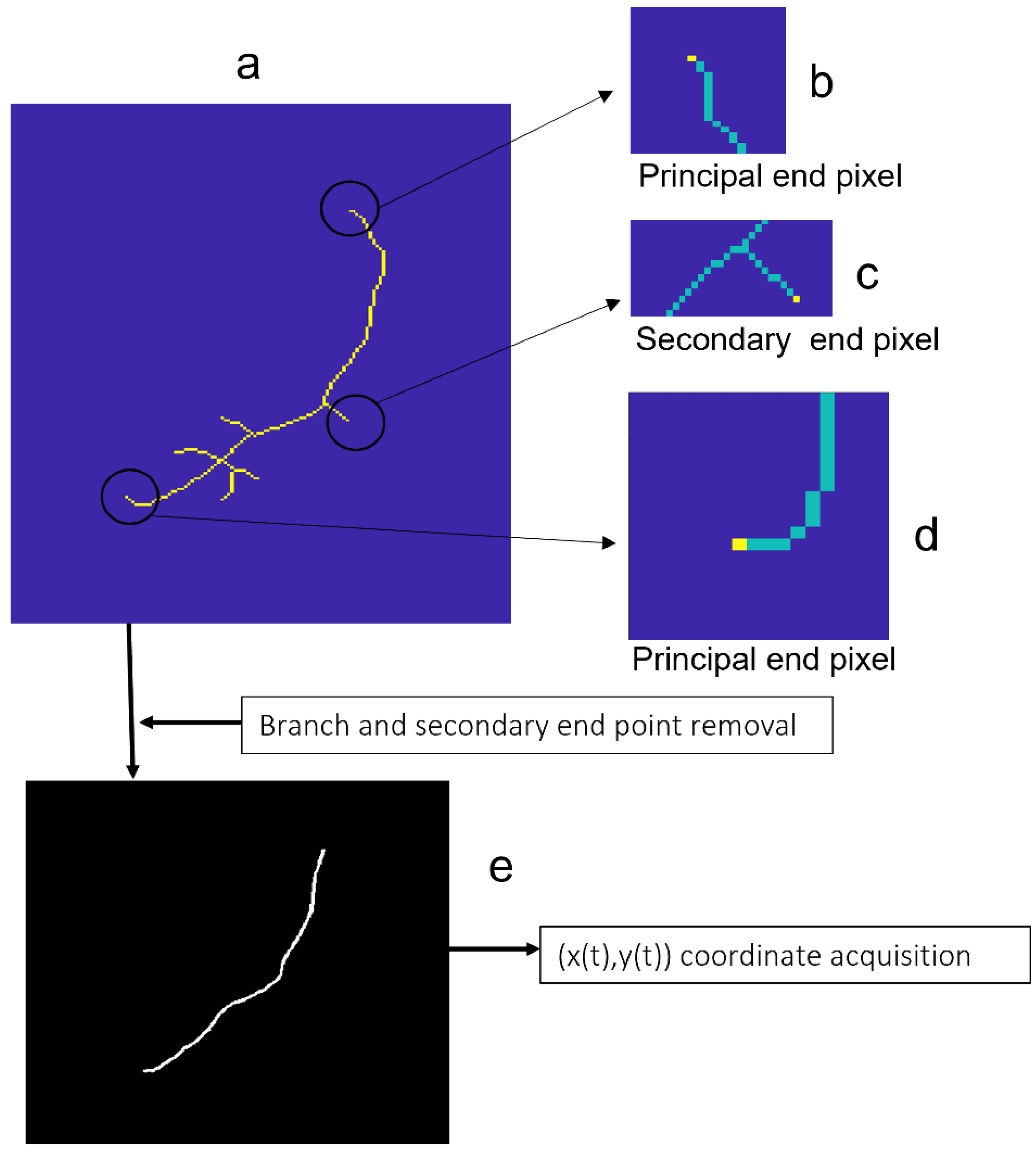
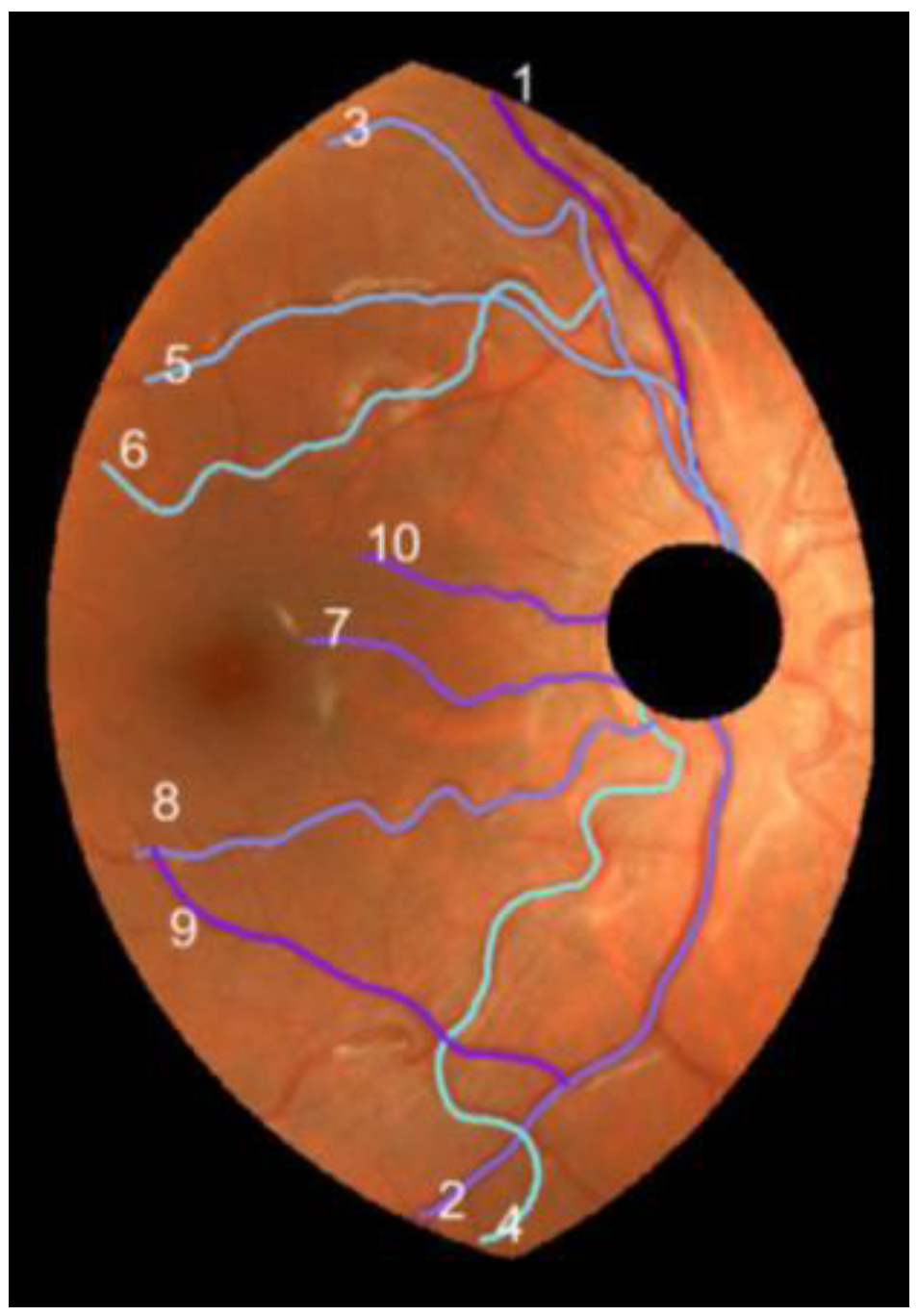
2.4. Vessel Segmentation and Parametrization
2.5. Vessel Curvature
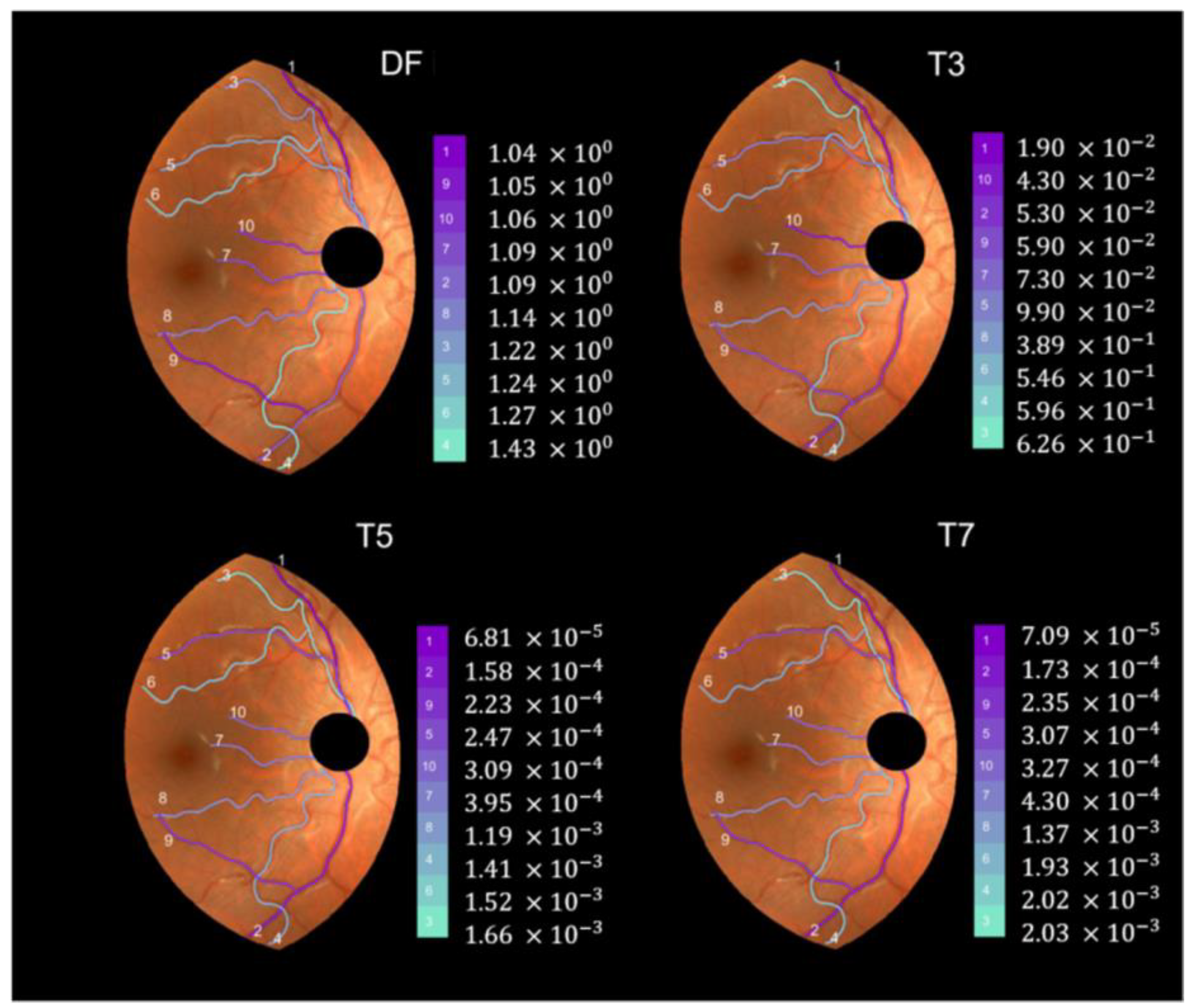
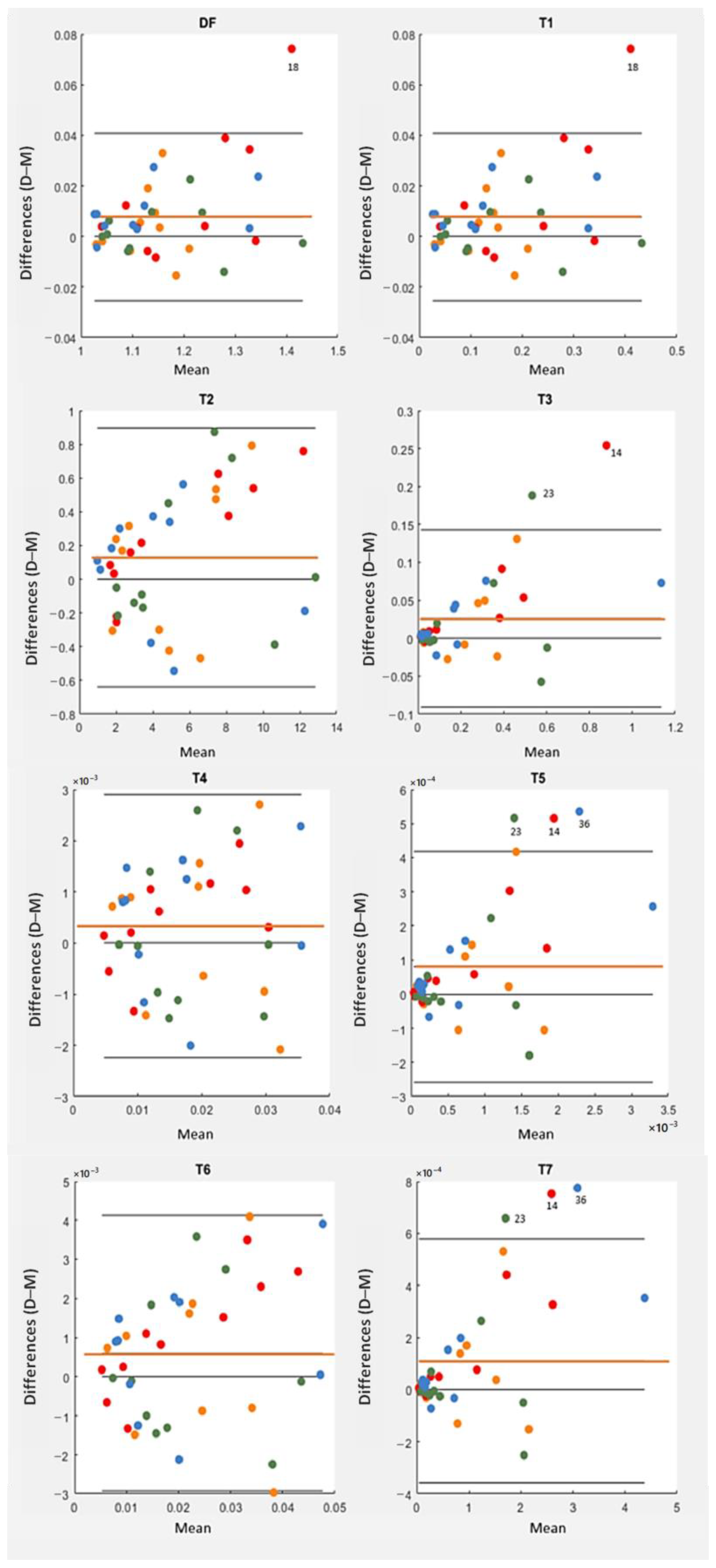
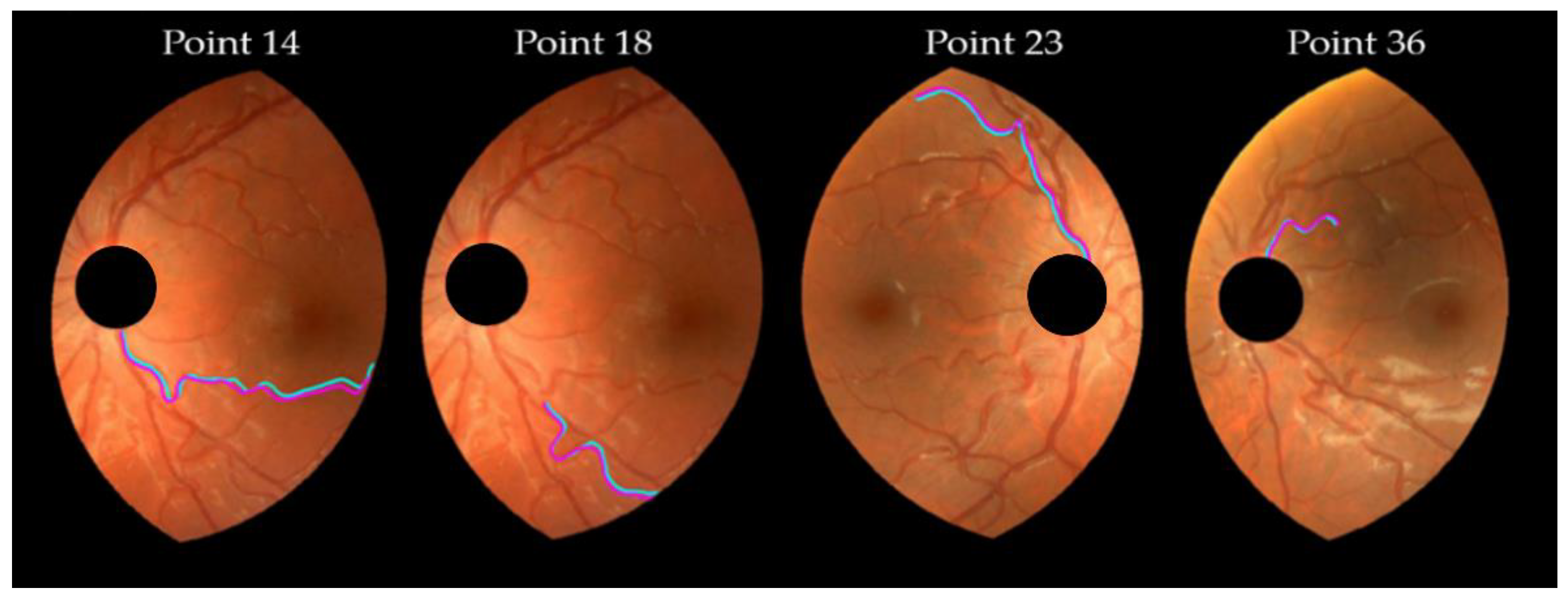
3. Results
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Witt, N.; Wong, T.Y.; Hughes, A.D.; Chaturvedi, N.; Klein, B.E.; Evans, R.; McNamara, M.; Thom, S.A.M.; Klein, R. Abnormalities of retinal microvascular structure and risk of mortality from ischemic heart disease and stroke. Hypertension 2006, 47, 975–981. [Google Scholar] [CrossRef] [PubMed]
- Cheung, C.Y.; Zheng, Y.; Hsu, W.; Lee, M.L.; Bcomp, Q.P.L.; Mitchell, P.; Mmed, J.J.W.; Klein, R.; Wong, T.Y. Retinal vascular tortuosity, blood pressure, and cardiovascular risk factors. Ophthalmology 2011, 118, 812–818. [Google Scholar] [CrossRef] [PubMed]
- Sun, Z.; Yang, D.; Tang, Z.; Ng, D.S.; Cheung, C.Y. Optical coherence tomography angiography in diabetic retinopathy: An updated review. Eye Lond 2021, 35, 149–161. [Google Scholar] [CrossRef] [PubMed]
- Veluchamy, A.; Ballerini, L.; Vitart, V.; Schraut, K.E.; Kirin, M.; Campbell, H.; Joshi, P.K.; Relan, D.; Harris, S.; Brown, E.; et al. Novel Genetic Locus Influencing Retinal Venular Tortuosity Is Also Associated With Risk of Coronary Artery Disease. Arterioscler. Thromb. Vasc. Biol. 2019, 39, 2542–2552. [Google Scholar] [CrossRef] [PubMed]
- van de Kreeke, J.A.; Nguyen, H.T.; Konijnenberg, E.; Tomassen, J.; den Braber, A.; Kate, M.; Sudre, C.H.; Barkhof, F.; Boomsma, D.I.; Tan, H.S.; et al. Retinal and Cerebral Microvasculopathy: Relationships and Their Genetic Contributions. Investig. Ophthalmol. Vis. Sci. 2018, 59, 5025–5031. [Google Scholar] [CrossRef] [PubMed]
- McClintic, B.R.; McClintic, J.I.; Bisognano, J.D.; Block, R.C. The relationship between retinal microvascular abnormalities and coronary heart disease: A review. Am. J. Med. 2010, 123, 374.e1–374.e7. [Google Scholar] [CrossRef] [PubMed]
- Stefánsson, E.; Olafsdottir, O.B.; Einarsdottir, A.B.; Eliasdottir, T.S.; Eysteinsson, T.; Vehmeijer, W.; Vandewalle, E.; Bek, T.; Hardarson, S.H. Retinal Oximetry Discovers Novel Biomarkers in Retinal and Brain Diseases. Invest. Ophthalmol. Vis. Sci. 2017, 58, BIO227–BIO233. [Google Scholar] [CrossRef] [PubMed]
- Lotmar, W.; Freiburghaus, A.; Bracher, D. Measurement of vessel tortuosity on fundus photographs. Albrecht Graefes Arch. Klin. Exp. Ophthalmol. 1979, 211, 49–57. [Google Scholar] [CrossRef] [PubMed]
- Hart, W.E.; Goldbaum, M.; Côté, B.; Kube, P.; Nelson, M.R. Measurement and classification of retinal vascular tortuosity. Int. J. Med. Inform. 1999, 53, 239–252. [Google Scholar] [CrossRef] [PubMed]
- Grisan, E.; Foracchia, M.; Ruggeri, A. A novel method for the automatic grading of retinal vessel tortuosity. IEEE Trans. Med. Imaging 2008, 27, 310–319. [Google Scholar] [CrossRef] [PubMed]
- Trucco, E.; Azegrouz, H.; Dhillon, B. Modeling the tortuosity of retinal vessels: Does caliber play a role? IEEE Trans. Biomed. Eng. 2010, 57, 2239–2247. [Google Scholar] [CrossRef] [PubMed]
- Onkaew, D.; Turior, R.; Uyyanonvara, B.; Akinori, N.; Sinthanayothin, C. Automatic Retinal Vessel Tortuosity Measurement Using Curvature of Improved Chain Code; InECCE: Kuantan, Malaysia, 2011; pp. 183–186. [Google Scholar]
- Kalitzeos, A.A.; Lip, G.Y.; Heitmar, R. Retinal vessel tortuosity measures and their applications. Exp. Eye Res. 2013, 106, 40–46. [Google Scholar] [CrossRef] [PubMed]
- Mapayi, T.; Tapamo, J.R.; Viriri, S.; Adio, A.O. Automatic Retinal Vessel Detection and Tortuosity Measurement. Image Anal. Stereol. 2016, 35, 117–135. [Google Scholar] [CrossRef]
- Ramos, L.; Novo, J.; Rouco, J.; Romeo, S.; Álvarez, M.D.; Ortega, M. Computational assessment of the retinal vascular tortuosity integrating domain-related information. Sci. Rep. 2019, 9, 19940. [Google Scholar] [CrossRef] [PubMed]
- Mok, K.H.; Lee, V.W. Disc-to-macula distance to disc-diameter ratio for optic disc size estimation. J. Glaucoma 2002, 11, 392–395. [Google Scholar] [CrossRef] [PubMed]
- Ralló, M.; Millán, M.S. Connectivity-based segmentation of retinal vessels in eye fundus images. Opt. Pura Apl. 2017, 50, 359–368. [Google Scholar] [CrossRef]
- Lewiner, T.; Gomes, J.D.; Lopes, H.; Craizer, M. Curvature and torsion estimators based on parametric curve fitting. Comp. Grap. 2005, 29, 641–655. [Google Scholar] [CrossRef]
- Ramos, L.; Novo, J.; Rouco, J.; Romeo, S.; Álvarez, M.D.; Ortega, M. Retinal vascular tortuosity assessment: Inter-intra expert analysis and correlation with computational measurements. BMC Med. Res. Methodol. 2018, 18, 144. [Google Scholar] [CrossRef] [PubMed]
- Aslam, T.; Fleck, B.; Patton, N.; Trucco, M.; Azegrouz, H. Digital image analysis of plus disease in retinopathy of prematurity. Acta Ophthalmol. 2009, 87, 368–377. [Google Scholar] [CrossRef] [PubMed]




| Chord length | |
| Arc length | |
| Total curvature | |
| Total squared curvature |
| Right Eye | Left Eye | |||
|---|---|---|---|---|
| Frame Center | Macula | Optic Disc | Macula | Optic Disc |
| Subject 1 | MR1 | DR1 | ML1 | DL1 |
| Subject 2 | MR2 | DR2 | ML2 | DL2 |
| Mean | Standard Deviation | |||
|---|---|---|---|---|
| Index | M | D | M | D |
| DF | 1.1543 | 1.1621 | 0.1101 | 0.1169 |
| T1 | 0.1543 | 0.1621 | 0.1101 | 0.1169 |
| T2 | 4.935 | 5.063 | 3.307 | 3.427 |
| T3 | 0.2085 | 0.2344 | 0.2412 | 0.2756 |
| T4 | 0.01709 | 0.01742 | 0.00915 | 0.00927 |
| T5 | 0.000694 | 0.000774 | 0.000718 | 0.000811 |
| T6 | 0.02046 | 0.02105 | 0.01251 | 0.01292 |
| T7 | 0.000860 | 0.000970 | 0.000952 | 0.001087 |
| Tortuosity Indices | Mean Difference | Standard Deviation | Limits of Agreement | Limits of Agreement | |
|---|---|---|---|---|---|
| Lower | Higher | ||||
| DF | 0.00768 | 0.016570 | −0.02480 | 0.04016 | 0.006 |
| T1 | 0.00768 | 0.016570 | −0.02480 | 0.04016 | 0.006 |
| T2 | 0.12910 | 0.384400 | −0.62430 | 0.88252 | 0.040 |
| T3 | 0.02591 | 0.058390 | −0.08850 | 0.14035 | 0.008 |
| T4 | 0.00033 | 0.001285 | −0.00220 | 0.00285 | 0.112 |
| T5 | 0.00008 | 0.000169 | −0.00025 | 0.00041 | 0.005 |
| T6 | 0.00060 | 0.001764 | −0.00285 | 0.00405 | 0.038 |
| T7 | 0.00011 | 0.000234 | −0.00035 | 0.00057 | 0.005 |
| Vessel | Frame Center | D | L | TK | TSK |
|---|---|---|---|---|---|
| 14 | DR2 | 339.86 | 457.30 | 12.57 | 1.007 |
| MR2 | 340.51 | 446.45 | 11.81 | 0.752 | |
| 18 | DR2 | 187.58 | 271.50 | 8.31 | 0.520 |
| MR2 | 190.69 | 261.84 | 7.93 | 0.466 | |
| 23 | DR2 | 308.30 | 377.31 | 7.79 | 0.627 |
| MR2 | 318.91 | 383.10 | 6.92 | 0.439 | |
| 36 | DR2 | 102.46 | 139.02 | 5.09 | 0.356 |
| MR2 | 103.79 | 138.37 | 4.75 | 0.280 |
| DF | T1 | T2 | T3 | T4 | T5 | T6 | ||
|---|---|---|---|---|---|---|---|---|
| T1 | 1.000 1.000 | |||||||
| T2 | 0.830 0.800 | 0.830 0.800 | ||||||
| T3 | 0.827 0.758 | 0.827 0.758 | 0.975 0.924 | |||||
| T4 | 0.775 0.758 | 0.775 0.758 | 0.869 0.826 | 0.921 0.817 | ||||
| T5 | 0.790 0.760 | 0.790 0.760 | 0.890 0.815 | 0.954 0.919 | 0.983 0.932 | |||
| T6 | 0.814 0.834 | 0.814 0.834 | 0.890 0.846 | 0.938 0.843 | 0.995 0.990 | 0.985 0.943 | ||
| T7 | 0.808 0.791 | 0.808 0.791 | 0.896 0.814 | 0.957 0.922 | 0.982 0.918 | 0.998 0.946 | 0.987 0.941 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ramírez, N.; Ralló, M.; Millan, M.S. Retinal Vessel Local Tortuosity under a Macula-to-Optic Disc Central-Framing Change. Diagnostics 2023, 13, 1030. https://doi.org/10.3390/diagnostics13061030
Ramírez N, Ralló M, Millan MS. Retinal Vessel Local Tortuosity under a Macula-to-Optic Disc Central-Framing Change. Diagnostics. 2023; 13(6):1030. https://doi.org/10.3390/diagnostics13061030
Chicago/Turabian StyleRamírez, Natalia, Miquel Ralló, and Maria S. Millan. 2023. "Retinal Vessel Local Tortuosity under a Macula-to-Optic Disc Central-Framing Change" Diagnostics 13, no. 6: 1030. https://doi.org/10.3390/diagnostics13061030
APA StyleRamírez, N., Ralló, M., & Millan, M. S. (2023). Retinal Vessel Local Tortuosity under a Macula-to-Optic Disc Central-Framing Change. Diagnostics, 13(6), 1030. https://doi.org/10.3390/diagnostics13061030







