Concordance between SIVA, IVAN, and VAMPIRE Software Tools for Semi-Automated Analysis of Retinal Vessel Caliber
Abstract
:1. Introduction
2. Materials and Methods
2.1. Study Population
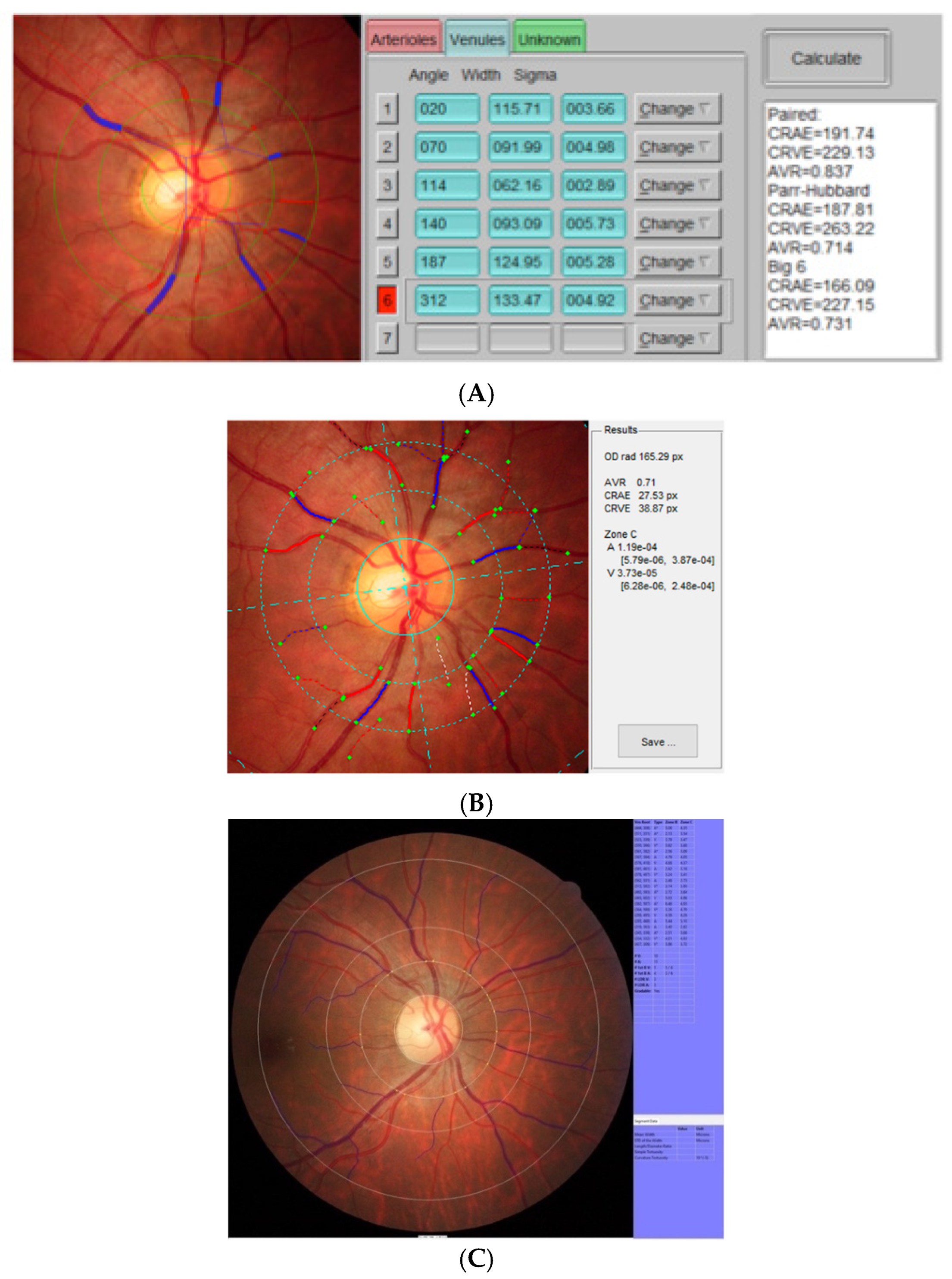
2.2. Retinal Image Analysis
2.3. Pixel-to-Micron Conversion
2.4. Statistical Analysis
2.5. Conversion Algorithm
3. Results
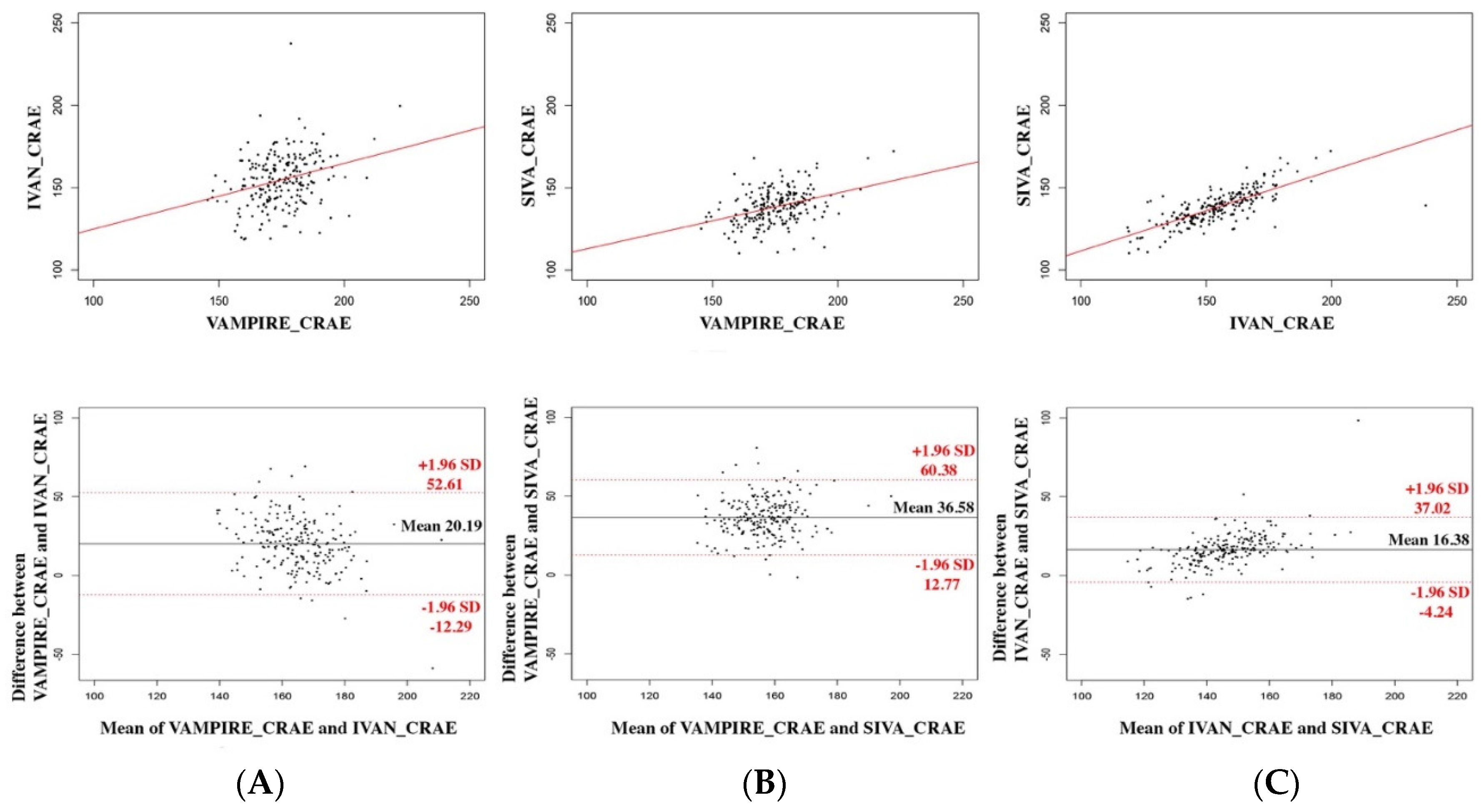
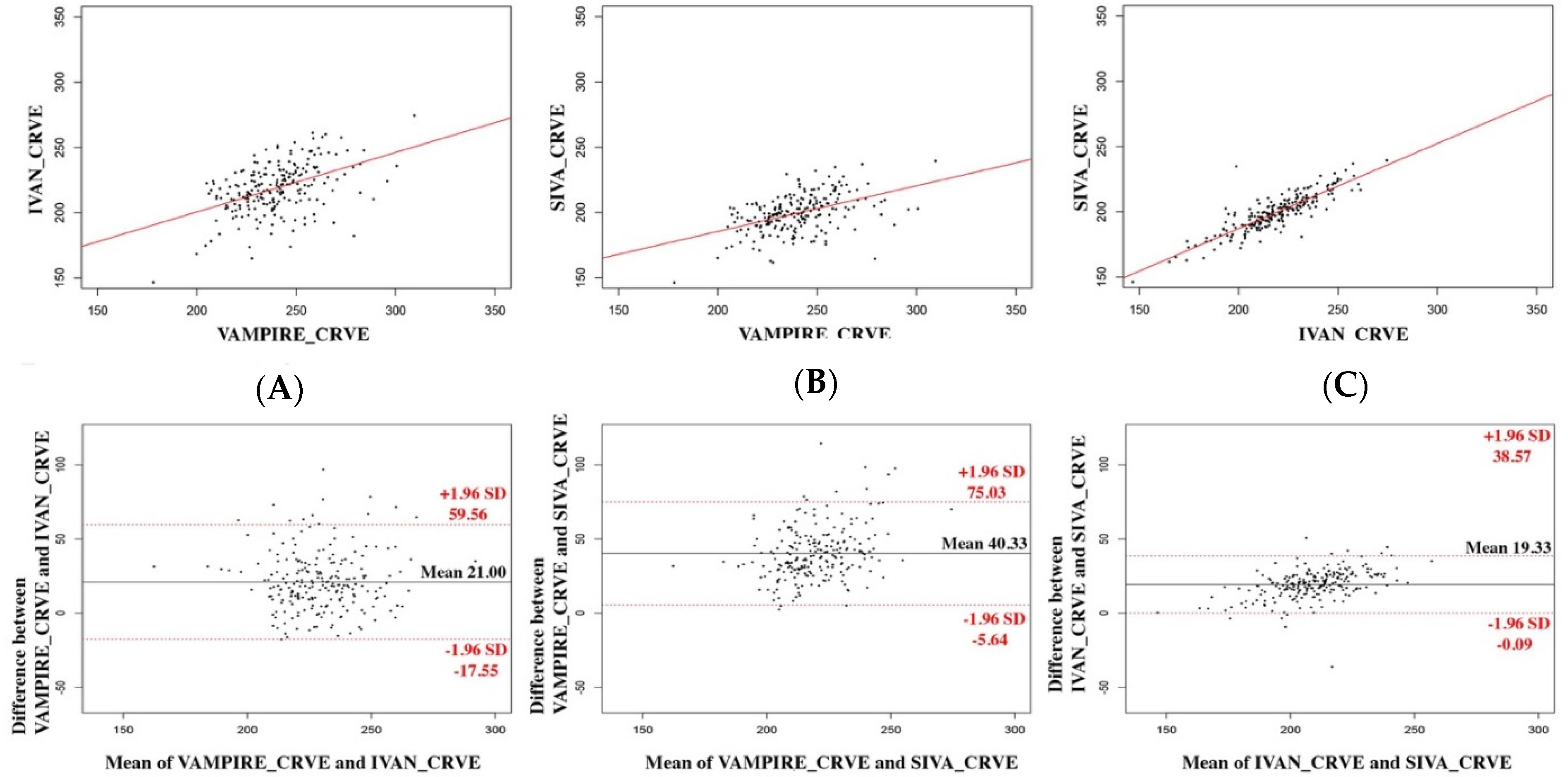
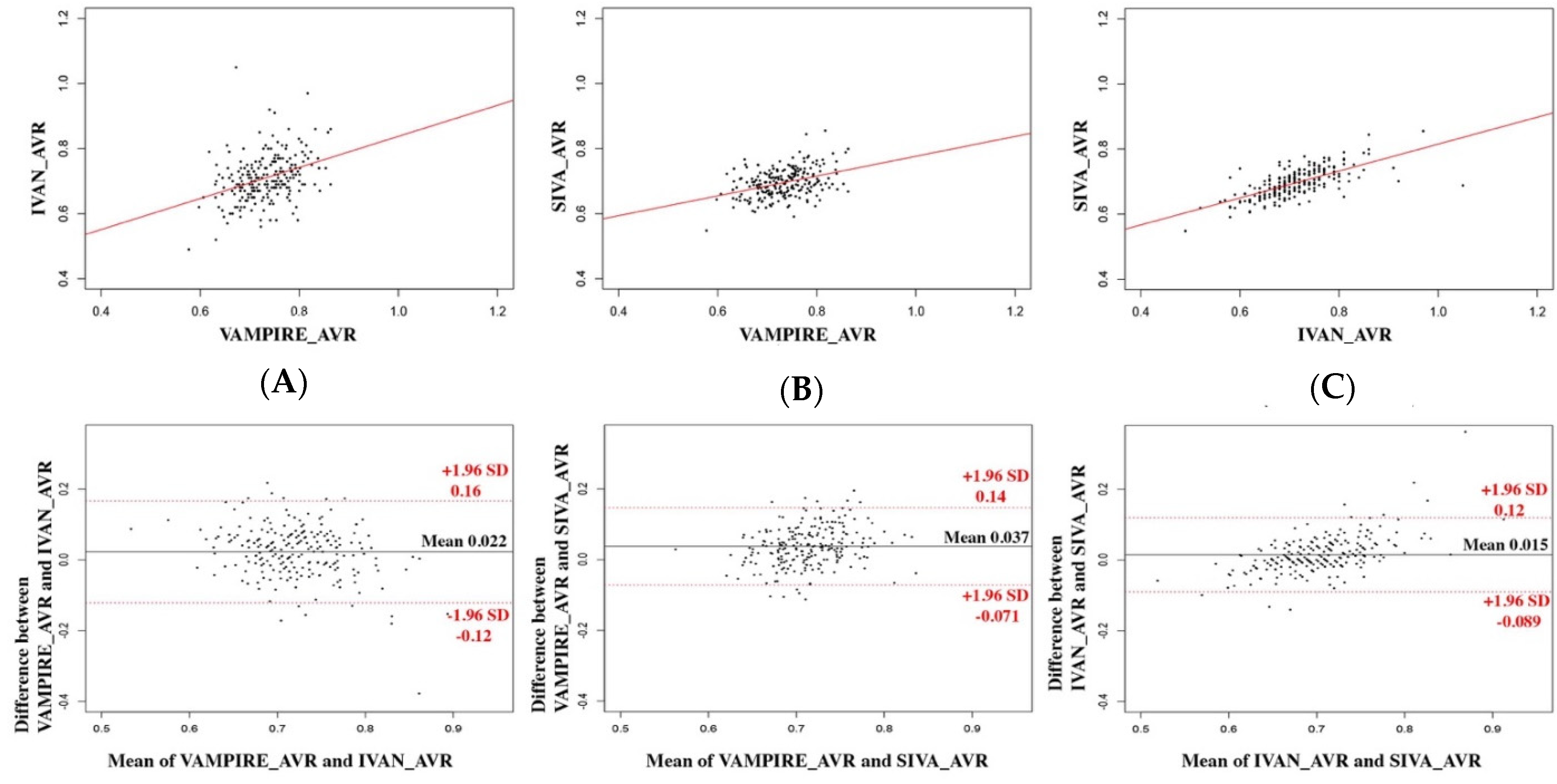
3.1. Between VAMPIRE and IVAN
3.2. Between VAMPIRE and SIVA
3.3. Between IVAN and SIVA
3.4. Between SIVA and IVAN
3.5. Between SIVA and VAMPIRE
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Harjasouliha, A.; Raiji, V.; Garcia Gonzalez, J.M. Review of hypertensive retinopathy. Dis. Mon. 2017, 63, 63–69. [Google Scholar] [CrossRef] [PubMed]
- Yatsuya, H.; Folsom, A.R.; Wong, T.Y.; Klein, R.; Klein, B.E.K.; Sharrett, A.R. Retinal Microvascular Abnormalities and Risk of Lacunar Stroke: Atherosclerosis Risk in Communities Study. Stroke 2010, 41, 1349–1355. [Google Scholar] [CrossRef]
- McGrory, S.; Taylor, A.M.; Kirin, M.; Corley, J.; Pattie, A.; Cox, S.R.; Deary, I.J. Retinal microvascular network geometry and cognitive abilities in community-dwelling older people: The Lothian Birth Cohort 1936 study. Br. J. Ophthalmol. 2017, 101, 993–998. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Seidelmann, S.B.; Claggett, B.; Bravo, P.E.; Gupta, A.; Farhad, H.; Klein, B.E.; Solomon, S.D. Retinal Vessel Calibers in Predicting Long-Term Cardiovascular Outcomes: The Atherosclerosis Risk in Communities Study. Circulation 2016, 134, 1328–1338. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- MacGillivray, T.J.; Trucco, E.; Cameron, J.R.; Dhillon, B.; Houston, J.G.; van Beek, E.J.R. Retinal imaging as a source of biomarkers for diagnosis, characterization and prognosis of chronic illness or long-term conditions. Br. J. Radiol. 2014, 87, 20130832. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cheung, C.Y.; Ikram, M.K.; Klein, R.; Wong, T.Y. The clinical implications of recent studies on the structure and function of the retinal microvasculature in diabetes. Diabetologia 2015, 58, 871–885. [Google Scholar] [CrossRef]
- Cheung, C.Y.; Lui Ikram, M.K.; Chen, C.; Wong, T.Y. Imaging retina to study dementia and stroke. Prog. Retin. Eye Res. 2017, 57, 89–107. [Google Scholar] [CrossRef]
- Ikram, M.K.; Cheung, C.Y.; Lorenzi, M.; Klein, R.; Jones, T.L.; Wong, T.Y.; NIH/JDRF Workshop on Retinal Biomarker for Diabetes Group. Retinal Vascular Caliber as a Biomarker for Diabetes Microvascular Complications. Diabetes Care 2013, 36, 750–759. [Google Scholar] [CrossRef] [Green Version]
- Cheung, C.Y.L.; Ikram, M.K.; Sabanayagam, C.; Wong, T.Y. Retinal Microvasculature as a Model to Study the Manifestations of Hypertension. Hypertension 2012, 60, 1094–1103. [Google Scholar] [CrossRef] [Green Version]
- Wong, T.Y.; Islam, F.M.A.; Klein, R.; Klein, B.E.; Cotch, M.F.; Castro, C.; Shahar, E. Retinal vascular caliber, cardiovascular risk factors, and inflammation: The multi-ethnic study of atherosclerosis (MESA). Investig. Ophthalmol. Vis. Sci. 2006, 47, 2341–2350. [Google Scholar] [CrossRef]
- Klein, R.; Myers, C.E.; Knudtson, M.D.; Lee, K.E.; Gangnon, R.; Wong, T.Y.; Klein, B.E. Relationship of blood pressure and other factors to serial retinal arteriolar diameter measurements over time: The beaver dam eye study. Arch. Ophthalmol. 2012, 130, 1019. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Iwase, A.; Sekine, A.; Suehiro, J.; Tanaka, K.; Kawasaki, Y.; Kawasaki, R.; Araie, M. A New Method of Magnification Correction for Accurately Measuring Retinal Vessel Calibers From Fundus Photographs. Investig. Ophthalmol. Vis. Sci. 2017, 58, 1858–1864. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wei, F.F.; Zhang, Z.Y.; Petit, T.; Cauwenberghs, N.; Gu, Y.M.; Thijs, L.; Staessen, J.A. Retinal microvascular diameter, a hypertension-related trait, in ECG-gated vs. non-gated images analyzed by IVAN and SIVA. Hypertens. Res. 2016, 39, 886–892. [Google Scholar] [CrossRef] [PubMed]
- Dervenis, N.; Coleman, A.L.; Harris, A.; Wilson, M.R.; Yu, F.; Anastasopoulos, E.; Topouzis, F. Factors associated with retinal vessel diameters in an elderly population: The thessaloniki eye study. Investig. Ophthalmol. Vis. Sci. 2019, 60, 2208–2217. [Google Scholar] [CrossRef] [Green Version]
- Cheung, C.; Wong, T.Y. Quantitative and qualitative retinal microvascular characteristics and blood pressure. J. Hypertens. 2011, 29, 1380–1391. [Google Scholar] [CrossRef]
- Cheung, C.Y.L.; Hsu, W.; Lee, M.L.; Wang, J.J.; Mitchell, P.; Lau, Q.P.; Wong, T.Y. A new method to measure peripheral retinal vascular caliber over an extended area. Microcirculation 2010, 17, 495–503. [Google Scholar] [CrossRef]
- Perez-Rovira, A.; MacGillivray, T.; Trucco, E.; Chin, K.S.; Zutis, K.; Lupascu, C.; Dhillon, B. VAMPIRE: Vessel assessment and measurement platform for images of the REtina. Conf. Proc. IEEE Eng. Med. Biol. Soc. 2011, 2011, 3391–3394. [Google Scholar] [CrossRef] [Green Version]
- Trucco, E.; Ballerini, L.; Relan, D.; Giachetti, A.; MacGillivray, T.; Zutis, K.; Dhillon, B. Novel VAMPIRE algorithms for quantitative analysis of the retinal vasculature. In Proceedings of the 2013 ISSNIP Biosignals and Biorobotics Conference: Biosignals and Robotics for Better and Safer Living (BRC), Rio de Janerio, Brazil, 18–20 February 2019; IEEE: Piscataway, NJ, USA, 2013; pp. 1–4. [Google Scholar] [CrossRef]
- Trucco, E.; Giachetti, A.; Ballerini, L.; Relan, D.; Cavinato, A.; MacGillivray, T.J. Morphometric Measurements of the Retinal Vasculature in Fundus Images with VAMPIRE. Biomedical Image Understanding: Methods and Applications; John Wiley & Sons: Hoboken, NJ, USA, 2015. [Google Scholar]
- McGrory, S.; Taylor, A.M.; Pellegrini, E.; Ballerini, L.; Kirin, M.; Doubal, F.N.; MacGillivray, T.J. Towards standardization of quantitative retinal vascular parameters: Comparison of SIVA and VAMPIRE measurements in the lothian birth cohort 1936. Transl. Vis. Sci. Technol. 2018, 7, 12. [Google Scholar] [CrossRef] [Green Version]
- Yip, W.; Tham, Y.C.; Hsu, W.; Lee, M.L.; Klein, R.; Klein, B.; Cheung, C.Y.L. Comparison of common retinal vessel caliber measurement software and a conversion algorithm. Transl. Vis. Sci. Technol. 2016, 5, 11. [Google Scholar] [CrossRef]
- Giachetti, A.; Ballerini, L.; Trucco, E. Accurate and reliable segmentation of the optic disc in digital fundus images. J. Med. Imaging 2014, 1, 024001. [Google Scholar] [CrossRef] [Green Version]
- Relan, D.; MacGillivray, T.; Ballerini, L.; Trucco, E. Automatic retinal vessel classification using a Least Square-Support Vector Machine in VAMPIRE. Conf. Proc. IEEE Eng. Med. Biol. Soc. 2014, 2014, 142–145. [Google Scholar] [CrossRef]
- Pellegrini, E.; Robertson, G.; Trucco, E.; MacGillivray, T.J.; Lupascu, C.; van Hemert, J.; Houston, G. Blood vessel segmentation and width estimation in ultra-wide field scanning laser ophthalmoscopy. Biomed. Opt. Express 2014, 5, 4329. [Google Scholar] [CrossRef] [PubMed]
- Hubbard, L.D.; Brothers, R.J.; King, W.N.; Clegg, L.X.; Klein, R.; Cooper, L.S.; Atherosclerosis Risk in Communities Study Group. Methods for evaluation of retinal microvascular abnormalities associated with hypertension/sclerosis in the Atherosclerosis Risk in Communities Study. Ophthalmology 1999, 106, 2269–2280. [Google Scholar] [CrossRef]
- Knudtson, M.D.; Lee, K.E.; Hubbard, L.D.; Wong, T.Y.; Klein, R.; Klein, B.K. Revised formulas for summarizing retinal vessel diameters. Curr Eye Res. 2003, 27, 143–149. [Google Scholar] [CrossRef]
- Jonas, J.B.; Gusek, G.C.; Naumann, G.O.H. Optic Disc, cup and neurorefinal rim size, configuration ond correlations in normal eyes. Investig. Ophthalmol. Vis. Sci. 1988, 29, 8. [Google Scholar]
- R Core Team. R: A Language and Environment for Statistical Computing; R Foundation for Statistical Computing: Vienna, Austria, 2018. [Google Scholar]
- Koo, T.K.; Li, M.Y. A guideline of selecting and reporting intraclass correlation coefficients for reliability research. J. Chiropr. Med. 2016, 15, 155–163. [Google Scholar] [CrossRef] [Green Version]
- Bland, J.M.; Altman, D.G. Statistical methods for assessing agreement between two methods of clinical measurement. Int. J. Nurs. Stud. 2010, 6, 307–310. [Google Scholar] [CrossRef]
- Bland, J.M.; Altman, D.G. Comparing methods of measurement: Why plotting difference against standard method is misleading. Lancet 1995, 346, 1085–1087. [Google Scholar] [CrossRef] [Green Version]
- Labarère, J.; Bertrand, R.; Fine, M.J. How to derive and validate clinical prediction models for use in intensive care medicine. Intensive Care Med. 2014, 40, 513–527. [Google Scholar] [CrossRef]
- Arnould, L.; Binquet, C.; Guenancia, C.; Alassane, S.; Kawasaki, R.; Daien, V.; Creuzot-Garcher, C. Association between the retinal vascular network with Singapore “I” Vessel Assessment (SIVA) software, cardiovascular history and risk factors in the elderly: The Montrachet study, population-based study. PLoS ONE 2018, 13, e0194694. [Google Scholar] [CrossRef] [Green Version]
- Mautuit, T.; Semecas, R.; Hogg, S.; Daien, V.; Gavard, O.; Chateau, N.; Chiquet, C. Comparing measurements of vascular diameter using adaptative optics imaging and conventional fundus imaging. Diagnostics 2022, 12, 705. [Google Scholar] [CrossRef] [PubMed]
- Pauli, T.W.; Gangaputra, S.; Hubbard, L.D.; Thayer, D.W.; Chandler, C.S.; Peng, Q.; Danis, R.P. Effect of image compression and resolution on retinal vascular caliber. Investig. Ophthalmol. Vis. Sci. 2012, 53, 5117. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mookiah, M.R.K.; McGrory, S.; Hogg, S.; Price, J.; Forster, R.; MacGillivray, T.J.; Trucco, E. Towards Standardization of Retinal Vascular Measurements: On the Effect of Image Centering. In Computational Pathology and Ophthalmic Medical Image Analysis; Stoyanov, D., Taylor, Z., Ciompi, F., Xu, Y., Martel, A., Maier-Hein, L., Rajpoot, N., van der Laak, J., Veta, M., McKenna, S., et al., Eds.; Lecture Notes in Computer Science; Springer International Publishing: Berlin, Germany, 2018; Volume 11039, pp. 294–302. [Google Scholar] [CrossRef] [Green Version]
- Cheung, C.Y.; Xu, D.; Cheng, C.Y.; Sabanayagam, C.; Tham, Y.C.; Yu, M.; Wong, T.Y. A deep-learning system for the assessment of cardiovascular disease risk via the measurement of retinal-vessel calibre. Nat. Biomed. Eng. 2021, 5, 498–508. [Google Scholar] [CrossRef] [PubMed]
- Cree, M.J.; Jelinek, H.F. The Effect of JPEG Compression on Automated Detection of Microaneurysms in Retinal Images; Niel, K.S., Fofi, D., Eds.; SPIE: Munich, Germany, 2008; p. 68130M. [Google Scholar] [CrossRef]
- Conrath, J.; Erginay, A.; Giorgi, R.; Lecleire-Collet, A.; Vicaut, E.; Klein, J.C.; Massin, P. Evaluation of the effect of JPEG and JPEG2000 image compression on the detection of diabetic retinopathy. Eye 2007, 21, 487–493. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Eikelboom, R.H.; Yogesan, K.; Barry, C.J.; Constable, I.J.; Tay–Kearney, M.L.; Jitskaia, L.; House, P.H. Methods and limits of digital image compression of retinal images for telemedicine. Investig. Ophthalmol. Vis. Sci. 2000, 41, 9. [Google Scholar]
| Characteristics | Participants (n = 133) Mean (SD)/n (%) |
|---|---|
| Age, years | 47.9 (22.9) |
| Sex ratio male/female | 0.6 |
| Male | 50 (37.6) |
| Female | 83 (62.4) |
| Axial length, mm | 23.7 (0.9) |
| Intra-ocular pressure, mmHg | 14.3 (2.8) |
| Systolic blood pressure, mmHg | 125.9 (12.5) |
| Diastolic blood pressure, mmHg | 75.7 (9.7) |
| Heart rate, bpm | 73.1 (10.7) |
| Body mass index, kg/m2 | 22.5 (7.1) |
| Retinal Vessel Parameter | Mean (±SD) | ||
|---|---|---|---|
| VAMPIRE | IVAN | SIVA | |
| CRAE (µm) | 174.9 (±11.3) | 154.7 (±15.7) | 138.3 (±10.2) |
| CRVE (µm) | 239.7 (±19.1) | 218.7 (±18.8) | 199.4 (±14.2) |
| AVR | 0.73 (±0.054) | 0.71 (±0.072) | 0.69 (0.04) |
| VAMPIRE and IVAN | Mean Difference | 95%LOA | p Value * | r | ICC | IC 95 | p Value † |
|---|---|---|---|---|---|---|---|
| CRAE (µm) | 20.2 | (−12.2 to 52.6) | <0.001 | 0.29 | 0.27 | (0.15–0.39) | <0.001 |
| CRVE (µm) | 21.0 | (−17.5 to 59.5) | <0.001 | 0.46 | 0.46 | (0.35–0.56) | 0.82 |
| AVR | 0.023 | (−0.12 to 0.16) | <0.001 | 0.36 | 0.35 | (0.23–0.46) | <0.001 |
| VAMPIRE and SIVA | |||||||
| CRAE (µm) | 36.6 | (12.8 to 60.4) | <0.001 | 0.38 | 0.37 | (0.25–0.48) | 0.09 |
| CRVE (µm) | 40.3 | (5.6 to 75.0) | <0.001 | 0.47 | 0.45 | (0.33–0.55) | <0.001 |
| AVR | 0.037 | (−0.071 to 0.15) | <0.001 | 0.38 | 0.37 | (0.25–0.48) | <0.001 |
| IVAN and SIVA | |||||||
| CRAE (µm) | 16.4 | (−4.25 to 37.0) | <0.001 | 0.75 | 0.69 | (0.61–0.75) | <0.001 |
| CRVE (µm) | 19.3 | (0.09 to 38.6) | <0.001 | 0.86 | 0.83 | (0.78–0.87) | <0.001 |
| AVR | 0.015 | (−0.09 to 0.11) | <0.001 | 0.68 | 0.61 | (0.52–0.68) | <0.001 |
| Between SIVA Measurements and VAMPIRE-Derived SIVA Approximate | |||
| RMSE (µm) | 95% CI | ||
| CRAE | 7.27 | (6.48–8.15) | |
| CRVE | 9.69 | (8.71–10.79) | |
| Between SIVA Measurements and IVAN-Derived SIVA Approximate | |||
| RMSE (µm) | 95% CI | ||
| CRAE | 4.88 | (4.35–5.60) | |
| CRVE | 5.19 | (4.66–6.03) | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mautuit, T.; Cunnac, P.; Cheung, C.Y.; Wong, T.Y.; Hogg, S.; Trucco, E.; Daien, V.; MacGillivray, T.J.; Labarère, J.; Chiquet, C. Concordance between SIVA, IVAN, and VAMPIRE Software Tools for Semi-Automated Analysis of Retinal Vessel Caliber. Diagnostics 2022, 12, 1317. https://doi.org/10.3390/diagnostics12061317
Mautuit T, Cunnac P, Cheung CY, Wong TY, Hogg S, Trucco E, Daien V, MacGillivray TJ, Labarère J, Chiquet C. Concordance between SIVA, IVAN, and VAMPIRE Software Tools for Semi-Automated Analysis of Retinal Vessel Caliber. Diagnostics. 2022; 12(6):1317. https://doi.org/10.3390/diagnostics12061317
Chicago/Turabian StyleMautuit, Thibaud, Pierre Cunnac, Carol Y. Cheung, Tien Y. Wong, Stephen Hogg, Emanuele Trucco, Vincent Daien, Thomas J. MacGillivray, José Labarère, and Christophe Chiquet. 2022. "Concordance between SIVA, IVAN, and VAMPIRE Software Tools for Semi-Automated Analysis of Retinal Vessel Caliber" Diagnostics 12, no. 6: 1317. https://doi.org/10.3390/diagnostics12061317
APA StyleMautuit, T., Cunnac, P., Cheung, C. Y., Wong, T. Y., Hogg, S., Trucco, E., Daien, V., MacGillivray, T. J., Labarère, J., & Chiquet, C. (2022). Concordance between SIVA, IVAN, and VAMPIRE Software Tools for Semi-Automated Analysis of Retinal Vessel Caliber. Diagnostics, 12(6), 1317. https://doi.org/10.3390/diagnostics12061317







