Effects of Setting Errors (Insert Run-Outs) on Surface Roughness in Face Milling When Using Circular Inserts
Abstract
1. Introduction
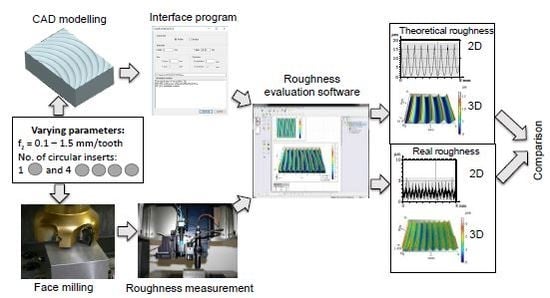
2. Materials and Methods
2.1. Investigation Method
2.2. Experimental Conditions
2.3. Determination of Roughness Values by CAD Modelling
2.4. Examination of Roughness of the Machined (Milled) Surface
3. Results
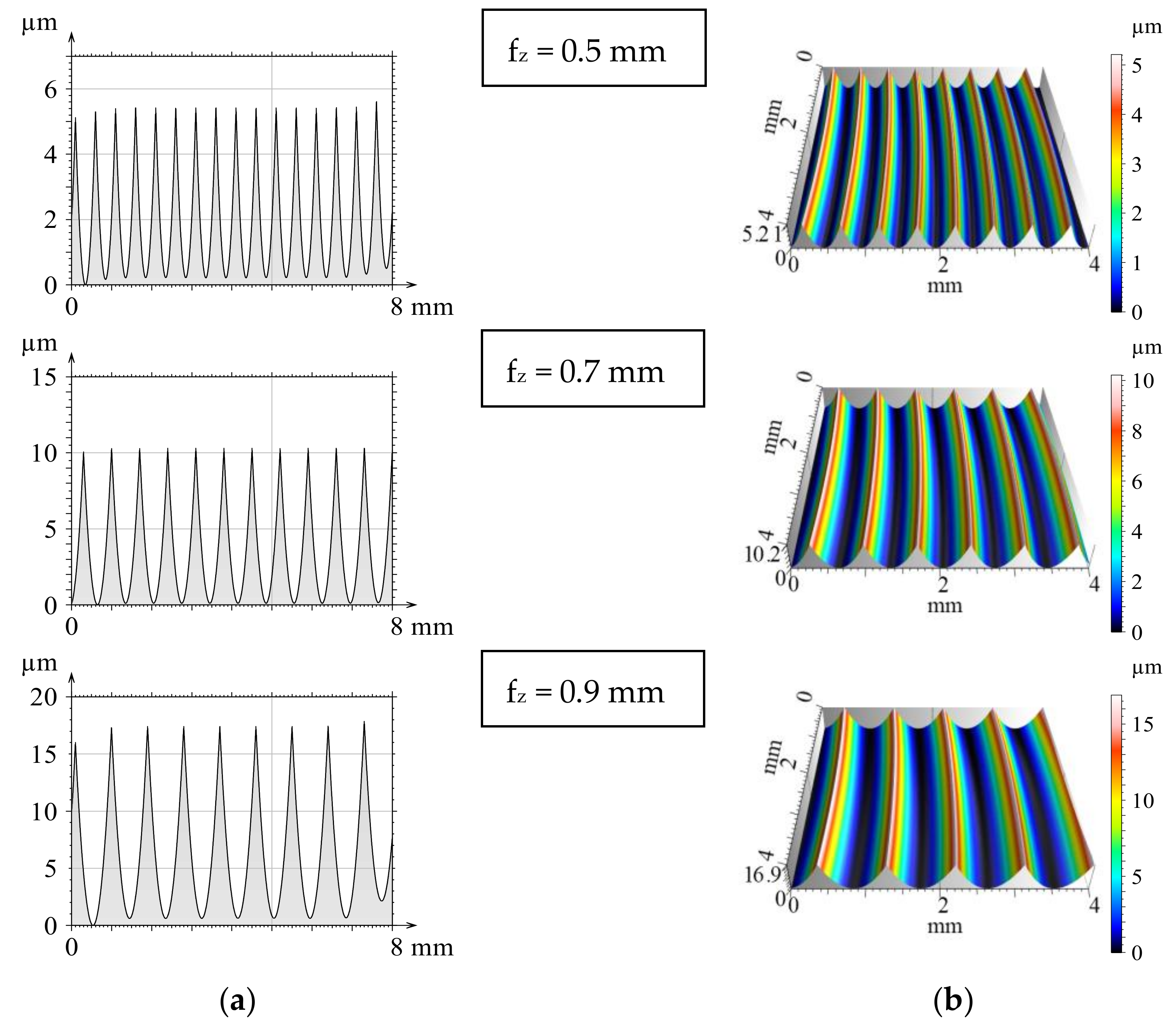
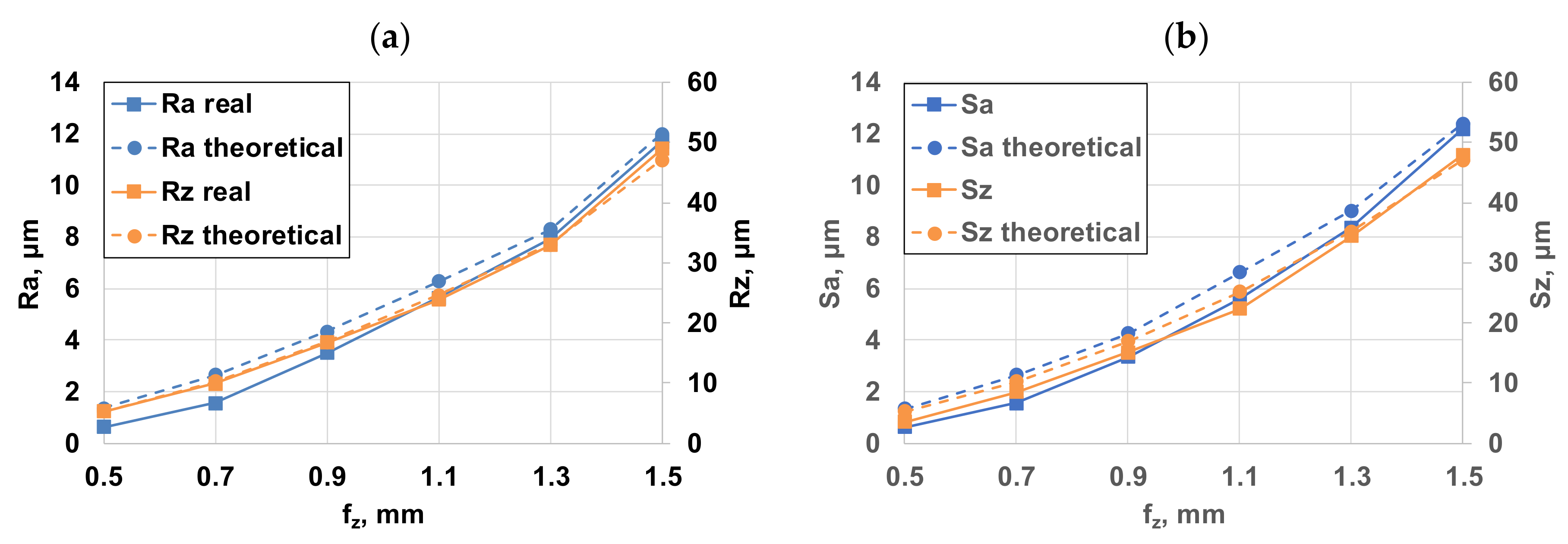
3.1. Roughness of the Modelled (Theoretical) Surface
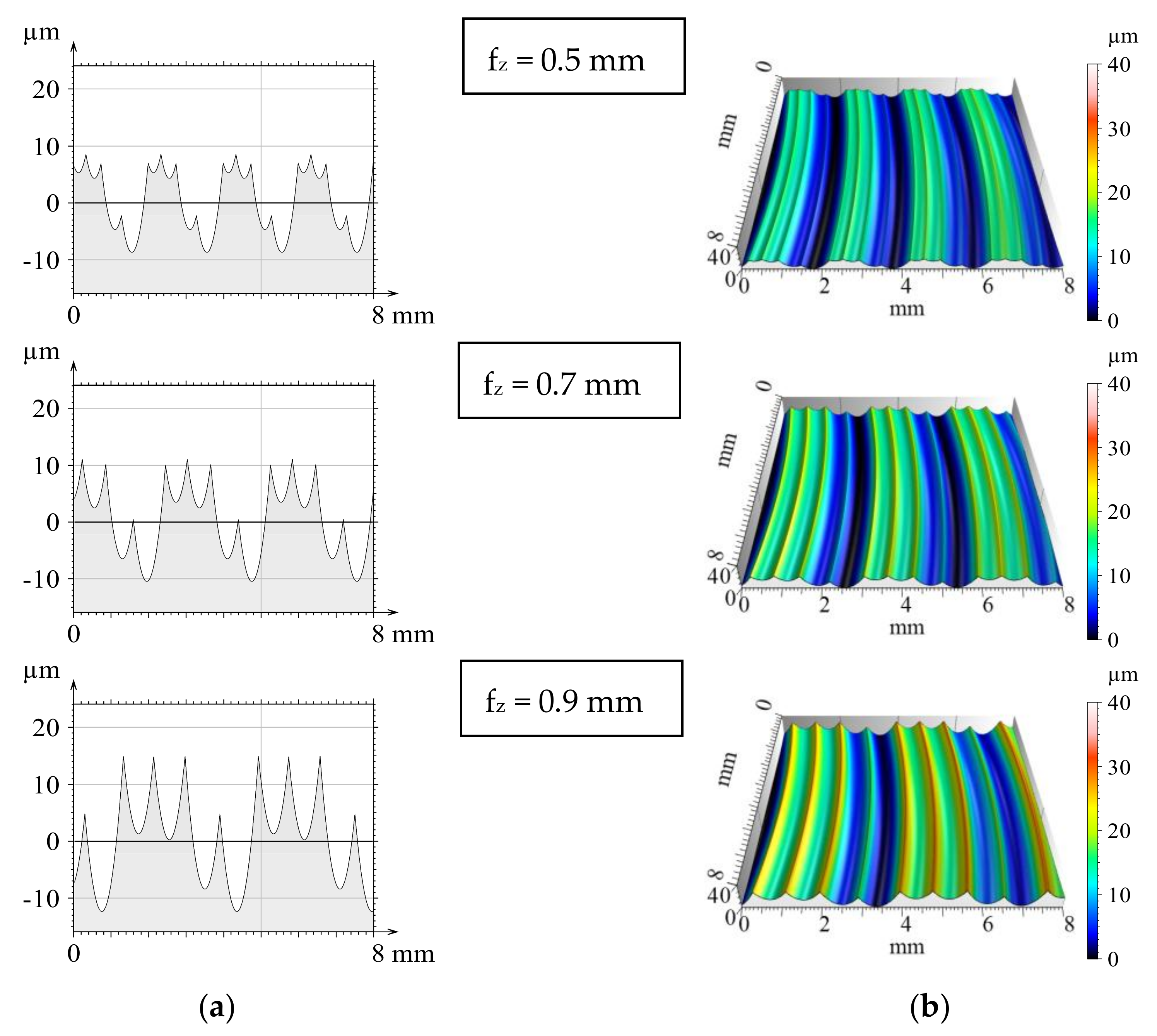
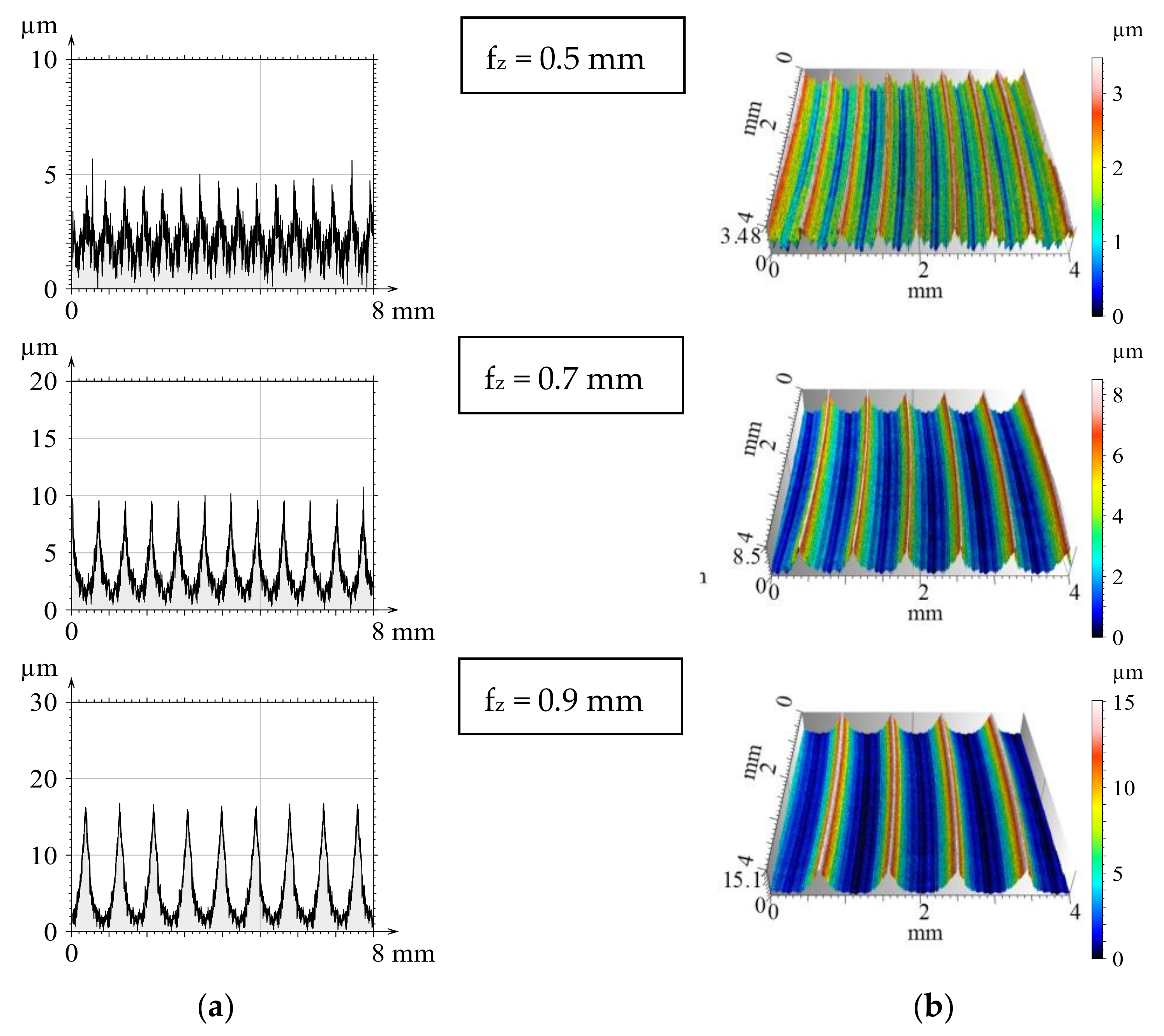
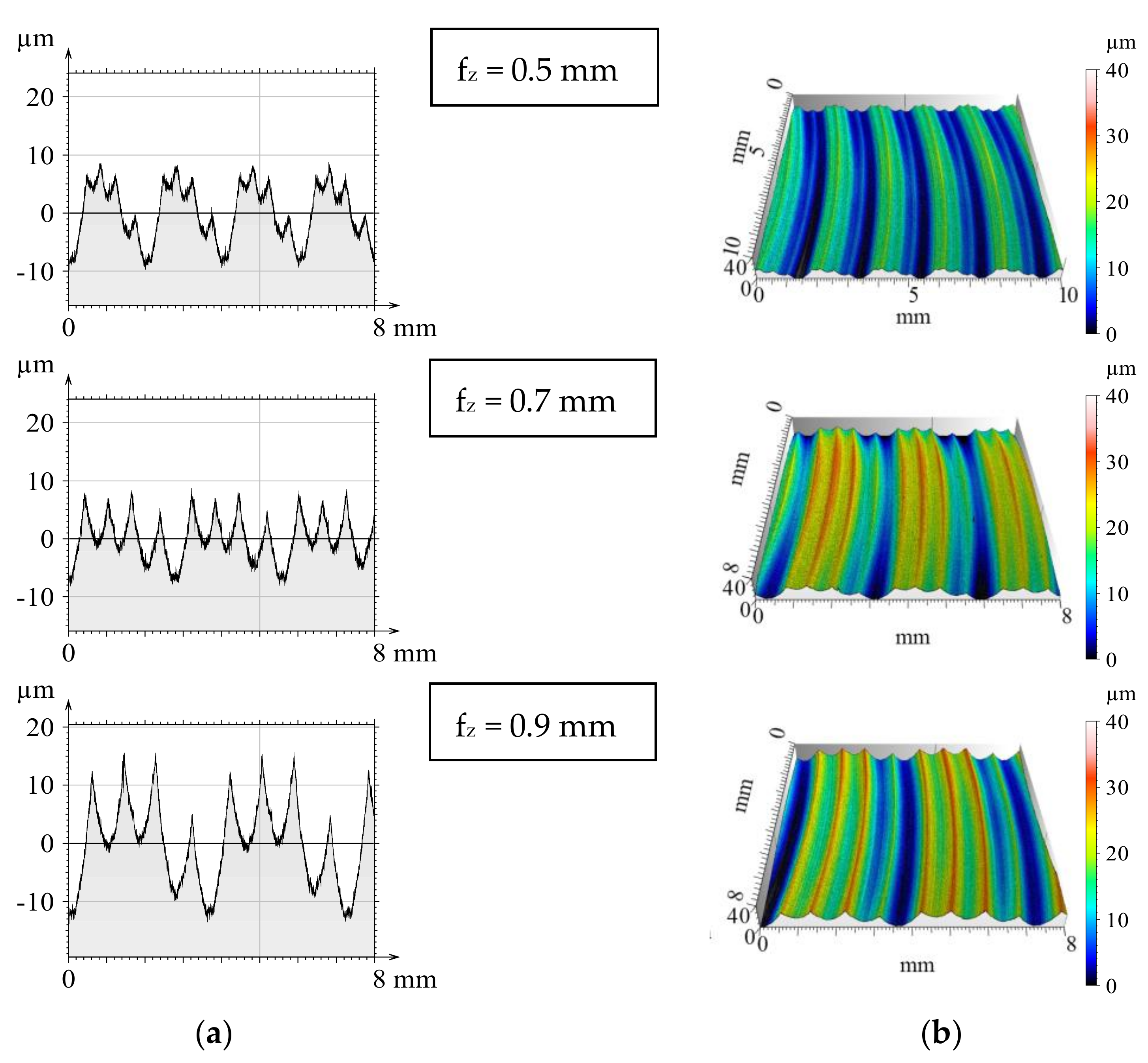
3.2. Roughness of Milled Surfaces
4. Discussion
5. Summary and Outlook
Acknowledgments
Author Contributions
Conflicts of Interest
References
- He, C.L.; Zong, W.J.; Zhang, J.J. Influencing factors and theoretical modelling methods of surface roughness in turning process: State-of-the-art. Int. J. Mach. Tools Manuf. 2018. [Google Scholar] [CrossRef]
- Tomov, M.; Kuzinovski, M.; Cichosz, P. Modelling and prediction of surface roughness profile in longitudinal turning. J. Manuf. Process. 2016, 24, 231–255. [Google Scholar] [CrossRef]
- Grzenda, M.; Bustillo, A. The evolutionary development of roughness prediction models. Appl. Soft Comput. 2013, 13, 2913–2922. [Google Scholar] [CrossRef]
- Colak, O.; Kurbanoglu, C.; Kayacan, M.C. Milling surface roughness prediction using evolutionary programming methods. Mater. Des. 2007, 28, 657–666. [Google Scholar] [CrossRef]
- El-Sonbaty, I.A.; Khashaba, U.A.; Selmy, A.I.; Ali, A.I. Prediction of surface roughness profiles for milled surfaces using an artificial neural network and fractal geometry approach. J. Mater. Process. Technol. 2008, 200, 271–278. [Google Scholar] [CrossRef]
- Tseng, T.-L.; Konada, U.; Kwon, Y. A novel approach to predict surface roughness in machining operations using fuzzy set theory. J. Comput. Des. Eng. 2016, 3, 1–13. [Google Scholar] [CrossRef]
- Rawangwong, S.; Chatthong, J.; Boonchouytan, W.; Burapa, R. Influence of Cutting Parameters in Face Milling Semi-Solid AA 7075 Using Carbide Tool Affected the Surface Roughness and Tool Wear. Energy Procedia 2014, 5, 448–457. [Google Scholar] [CrossRef]
- Zeroudi, N.; Fontaine, M. Prediction of machined surface geometry based on analytical modelling of ball-end milling. Procedia CIRP 2012, 1, 108–113. [Google Scholar] [CrossRef][Green Version]
- Miko, E.; Nowakowski, L. Analysis and Verification of Surface Roughness Constitution Model after Machining Process. Procedia Eng. 2012, 39, 395–404. [Google Scholar] [CrossRef]
- Ozcelik, B.; Bayramoglu, M. The statistical modelling of surface roughness in high-speed flat end milling. Int. J. Mach. Tools Manuf. 2006, 46, 1395–1402. [Google Scholar] [CrossRef]
- Karkalos, N.E.; Galanis, N.I.; Markopoulos, A.P. Surface roughness prediction for the milling of Ti–6Al–4V ELI alloy with the use of statistical and soft computing techniques. Measurement 2016, 90, 25–35. [Google Scholar] [CrossRef]
- Mikó, B.; Beno, J.; Mankova, I. Experimental Verification of Cusp Heights when 3D Milling Rounded Surfaces. Acta Polytech. Hung. 2012, 9, 101–116. [Google Scholar]
- Shyha, I.; Gariani, S.; El-Sayed, M.A.; Huo, D. Analysis of Microstructure and Chip Formation When Machining Ti-6Al-4V. Metals 2018, 8, 185. [Google Scholar] [CrossRef]
- Kilickap, E.; Yardimeden, A.; Celik, Y.H. Mathematical Modelling and Optimization of Cutting Force, Tool Wear and Surface Roughness by Using Artificial Neural Network and Response Surface Methodology in Milling of Ti-6242S. Appl. Sci. 2017, 7, 1064. [Google Scholar] [CrossRef]
- Selaimia, A.-A.; Yallese, M.A.; Bensouilah, H.; Meddour, I.; Khattabi, R.; Mabrouki, T. Modelling and optimization in dry face milling of X2CrNi18-9 austenitic stainless steel using RMS and desirability approach. Measurement 2017, 107, 53–67. [Google Scholar] [CrossRef]
- Nguyen, H.-T.; Hsu, Q.-C. Surface Roughness Analysis in the Hard Milling of JIS SKD61 Alloy Steel. Appl. Sci. 2016, 6, 172. [Google Scholar] [CrossRef]
- Baek, D.K.; Ko, T.J.; Kim, H.S. Optimization of feedrate in a face milling operation using a surface roughness model. Int. J. Mach. Tools Manuf. 2001, 41, 451–462. [Google Scholar] [CrossRef]
- Schmitz, T.L.; Couey, J.; Marsh, E.; Mauntler, N.; Hughes, D. Runout effects in milling: Surface finish, surface location error and stability. Int. J. Mach. Tools Manuf. 2007, 47, 841–851. [Google Scholar] [CrossRef]
- Franco, P.; Estrems, M.; Faura, F. Influence of radial and axial runouts on surface roughness in face milling with round insert cutting tools. Int. J. Mach. Tools Manuf. 2004, 44, 1555–1565. [Google Scholar] [CrossRef]
- Felho, C. Investigation of Surface Roughness in Machining by Single and Multi-Point Tools. Ph.D. Thesis, Otto von Guericke University, Magdeburg, Germany, 2014. [Google Scholar]
- Felho, C.; Karpuschewski, B.; Kundrak, J. Surface roughness modelling in face milling. Procedia CIRP 2015, 31, 136–141. [Google Scholar] [CrossRef]
- International Organization for Standardization (ISO). Geometrical Product Specifications (GPS)—Surface Texture: Profile Method—Terms, Definitions and Surface Texture Parameters; ISO 4287; ISO: Geneva, Switzerland, 1997. [Google Scholar]
- International Organization for Standardization (ISO). Geometrical Product Specifications (GPS)—Surface Texture: Profile Method—Rules and Procedures for the Assessment of Surface Texture; ISO 4288; ISO: Geneva, Switzerland, 1998. [Google Scholar]
- International Organization for Standardization (ISO). Geometrical Product Specifications (GPS)—Surface Texture: Areal—Part 2: Terms, Definitions and Surface Texture Parameters; ISO 25178-2; ISO: Geneva, Switzerland, 2012. [Google Scholar]
- Beno, J.; Mankova, I.; Vrabel, M.; Karpuschewski, B.; Emmer, T.; Schmidt, K. Operation Safety and Performance of Milling Cutters with Shank Style Holders of Tool Inserts. Procedia Eng. 2012, 48, 15–23. [Google Scholar] [CrossRef][Green Version]


| C (%) | Si (%) | Mn (%) | P (%) | S (%) | Cr (%) | Ni (%) | Mo (%) |
|---|---|---|---|---|---|---|---|
| 0.430 | 0.170 | 0.740 | 0.009 | 0.032 | 0.070 | 0.080 | 0.013 |
| Characteristic | One Insert | Four Inserts |
|---|---|---|
| fz (mm/tooth) | 0.5; 0.7; 0.9; 1.1; 1.3; 1.5 | 0.1; 0.3; 0.5; 0.7; 0.9 |
| n (1/min) | 950 | |
| vc (m/min) | 203 | |
| ap (mm) | 0.9 | |
| ae (mm) | 50 mm | |
| Milling head | Sandvik Coromant Coromill R200 milling head (R200-068Q27-12L) | |
| Milling insert | Circular insert, RCKT1204M0-PM 4230 iC = 12 mm | |
| Specimens | C45, 50 × 50 × 100 mm3 | |
| Machine | Perfect Jet MCV-M8 CNC vertical machining centre | |
| Theoretical Roughness 1 Insert | Measured Data 1 Insert | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| fz (mm) | Ra μm | Sa μm | Rq μm | Sq μm | Rz μm | Sz μm | Ra μm | Sa μm | Rq μm | Sq μm | Rz μm | Sz μm |
| 0.5 | 1.34 | 1.33 | 1.56 | 1.55 | 5.2 | 5.21 | 0.62 | 0.62 | 0.79 | 0.785 | 5.21 | 3.48 |
| 0.7 | 2.64 | 2.63 | 3.07 | 3.05 | 10.2 | 10.2 | 1.56 | 1.56 | 1.97 | 1.96 | 9.94 | 8.5 |
| 0.9 | 4.33 | 4.26 | 5.01 | 4.96 | 16.8 | 16.9 | 3.5 | 3.35 | 4.21 | 4.12 | 16.7 | 15.1 |
| 1.1 | 6.27 | 6.62 | 7.29 | 7.62 | 24.6 | 25.1 | 5.65 | 5.61 | 6.49 | 6.51 | 23.9 | 22.3 |
| 1.3 | 8.29 | 9.03 | 9.69 | 10.5 | 33 | 35.2 | 7.91 | 8.36 | 9.21 | 9.69 | 32.9 | 34.5 |
| 1.5 | 12 | 12.4 | 14 | 14.3 | 47.1 | 47.1 | 11.7 | 12.2 | 13.7 | 14.1 | 48.9 | 48 |
| Theoretical Roughness 4 Inserts | Measured Data 4 Inserts | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| fz (mm) | Ra μm | Sa μm | Rq μm | Sq μm | Rz μm | Sz μm | Ra μm | Sa μm | Rq μm | Sq μm | Rz μm | Sz μm |
| 0.1 | 0.856 | 0.851 | 0.994 | 0.989 | 3.33 | 3.3 | 1.05 | 1.14 | 1.35 | 1.41 | 6.41 | 6.99 |
| 0.3 | 4.04 | 4.26 | 4.62 | 4.85 | 14 | 14.7 | 3.66 | 3.79 | 4.26 | 4.42 | 16.5 | 16.4 |
| 0.5 | 5.11 | 5.04 | 5.52 | 5.47 | 17.2 | 18 | 4.57 | 4.78 | 5.09 | 5.3 | 19.2 | 19.1 |
| 0.7 | 5.47 | 5.32 | 6.13 | 6 | 21.5 | 21.4 | 5 | 5.75 | 5.74 | 6.81 | 23.3 | 29.5 |
| 0.9 | 5.97 | 6.12 | 7.27 | 7.4 | 27.3 | 29 | 5.83 | 6.26 | 7.08 | 7.49 | 30.6 | 30.4 |
| Theoretical | Measured | |||||||
|---|---|---|---|---|---|---|---|---|
| fz (mm) | Ra | Rz | Sa | Sz | Ra | Rz | Sa | Sz |
| 0.5 | 3.81 | 3.31 | 3.79 | 3.45 | 7.37 | 3.69 | 7.71 | 5.49 |
| 0.7 | 2.07 | 2.11 | 2.02 | 2.10 | 3.21 | 2.34 | 3.69 | 3.47 |
| 0.9 | 1.38 | 1.63 | 1.44 | 1.72 | 1.67 | 1.83 | 1.87 | 2.01 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Felhő, C.; Kundrák, J. Effects of Setting Errors (Insert Run-Outs) on Surface Roughness in Face Milling When Using Circular Inserts. Machines 2018, 6, 14. https://doi.org/10.3390/machines6020014
Felhő C, Kundrák J. Effects of Setting Errors (Insert Run-Outs) on Surface Roughness in Face Milling When Using Circular Inserts. Machines. 2018; 6(2):14. https://doi.org/10.3390/machines6020014
Chicago/Turabian StyleFelhő, Csaba, and János Kundrák. 2018. "Effects of Setting Errors (Insert Run-Outs) on Surface Roughness in Face Milling When Using Circular Inserts" Machines 6, no. 2: 14. https://doi.org/10.3390/machines6020014
APA StyleFelhő, C., & Kundrák, J. (2018). Effects of Setting Errors (Insert Run-Outs) on Surface Roughness in Face Milling When Using Circular Inserts. Machines, 6(2), 14. https://doi.org/10.3390/machines6020014