Dynamic Simulation of the Harvester Boom Cylinder
Abstract
:1. Introduction
2. The Method of Complete Dynamic Calculation
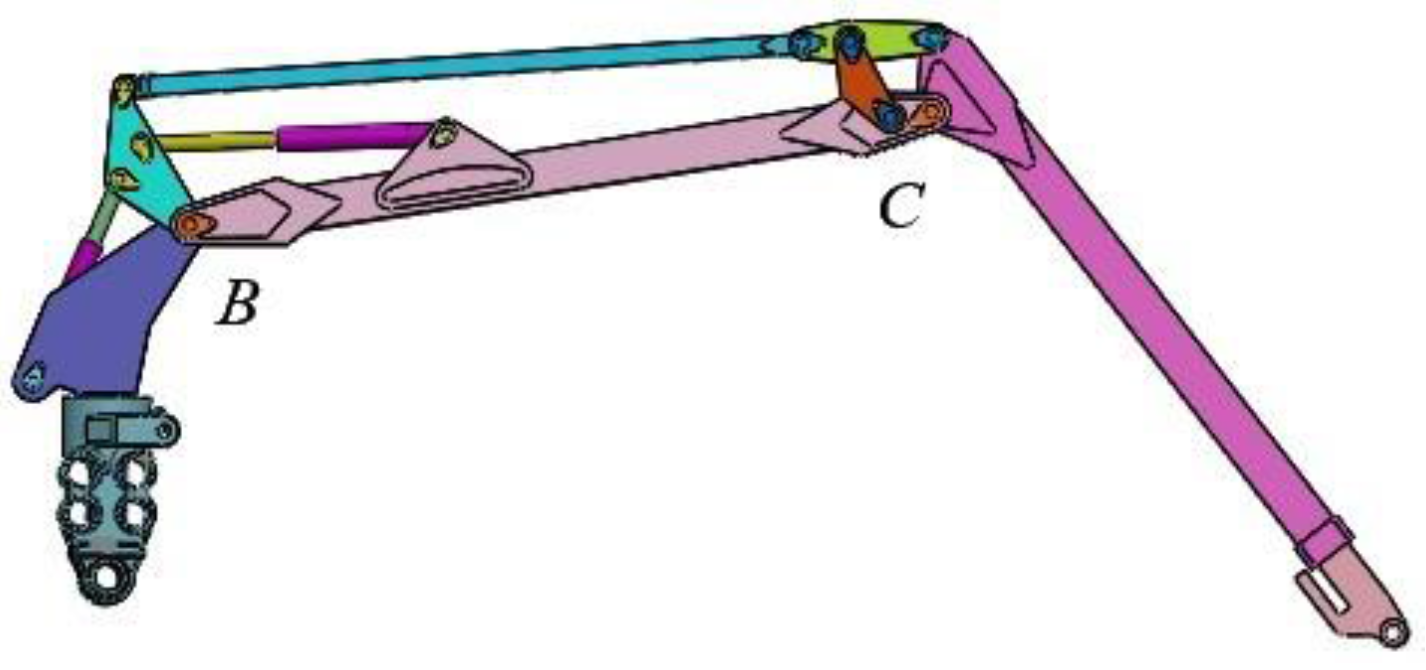
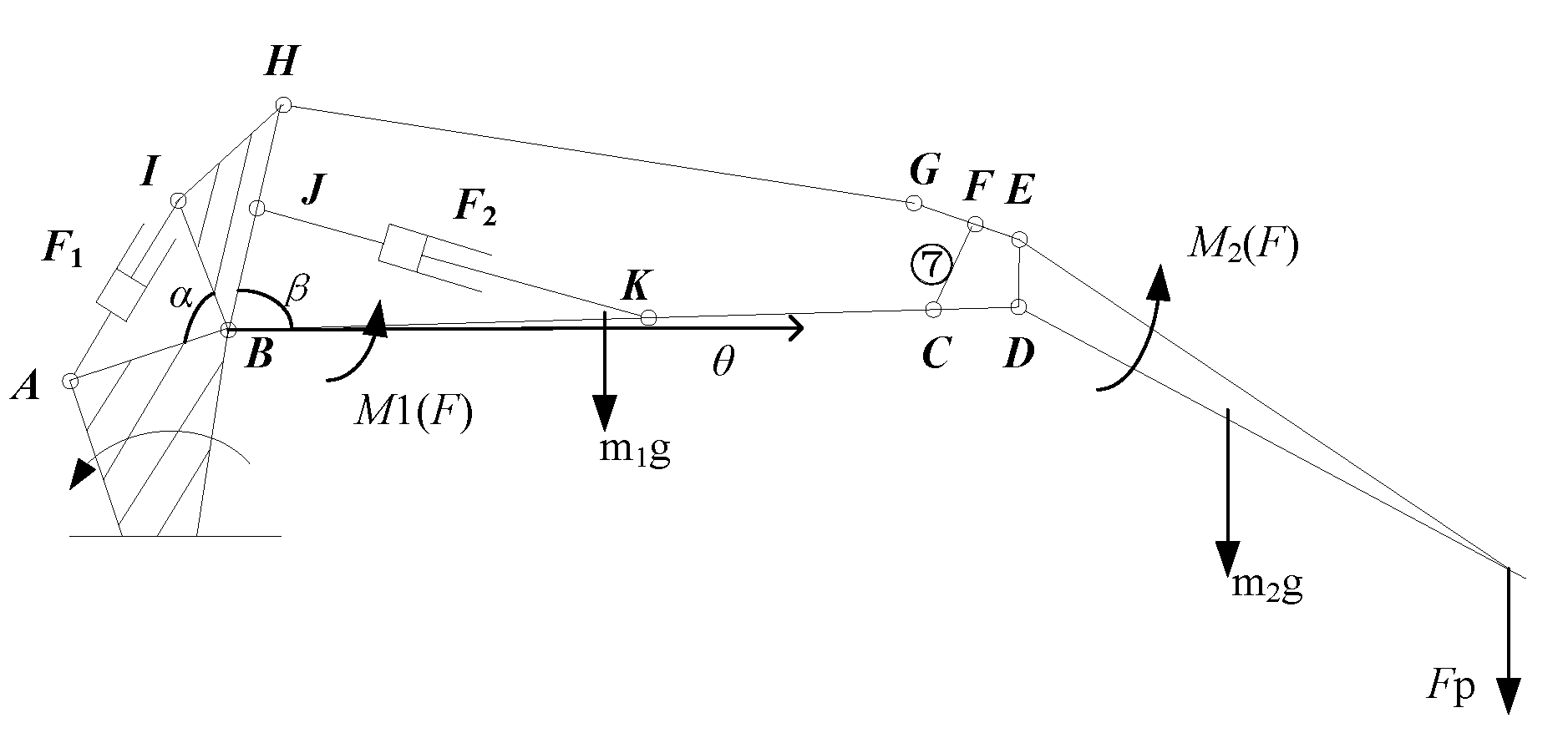
2.1. Calculation of the Main Cylinder
2.1.1. Force Analysis
2.1.2. Column Stability Calculation
2.2. Calculation of the Second Cylinder
2.2.1. Force Analysis
2.2.2. Column Stability Calculation
3. Dynamic Simulation of the Cylinder
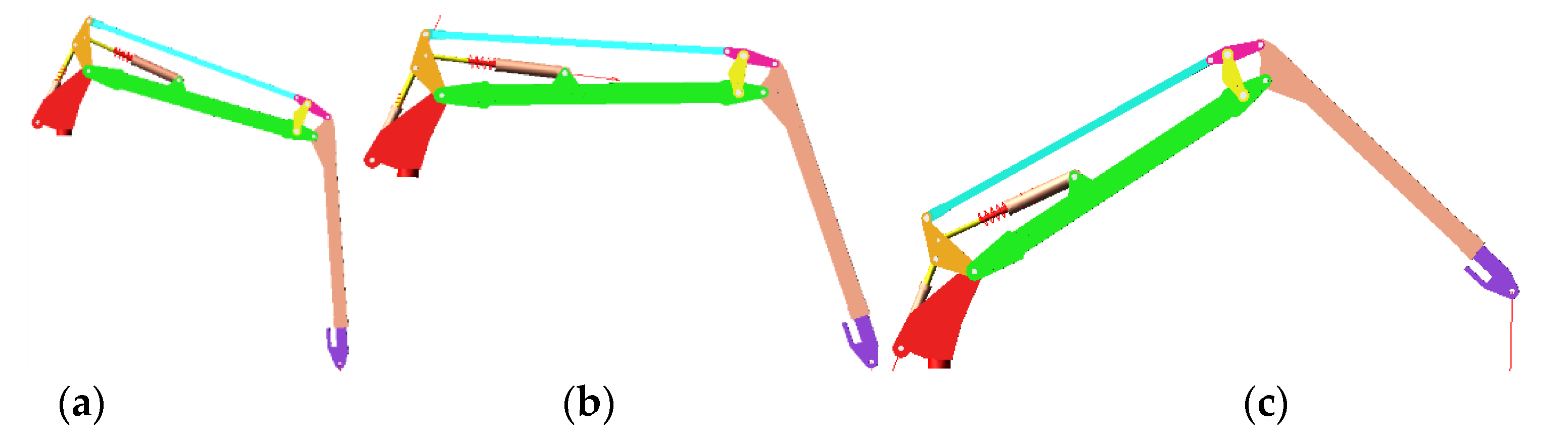
3.1. The Preprocessor of the Boom’s Virtual Prototyping
3.2. The Boom’s Movement Law
3.3. Cylinder Drive
3.4. Secondary Cylinder Drive
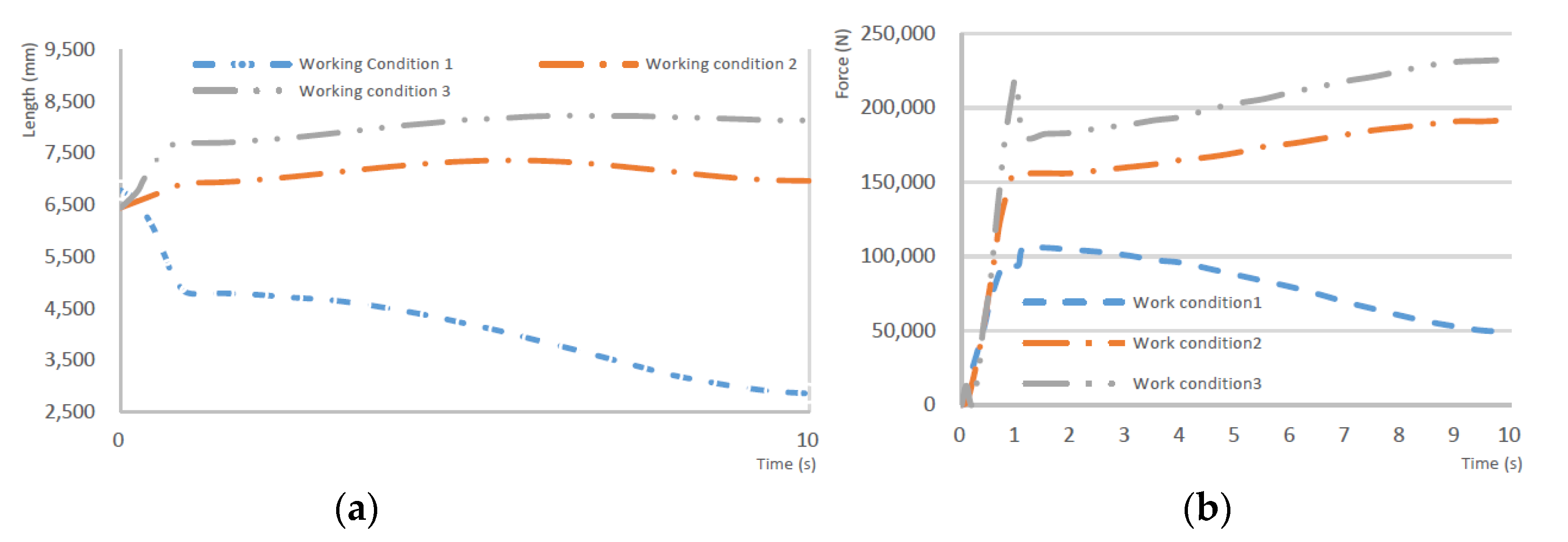
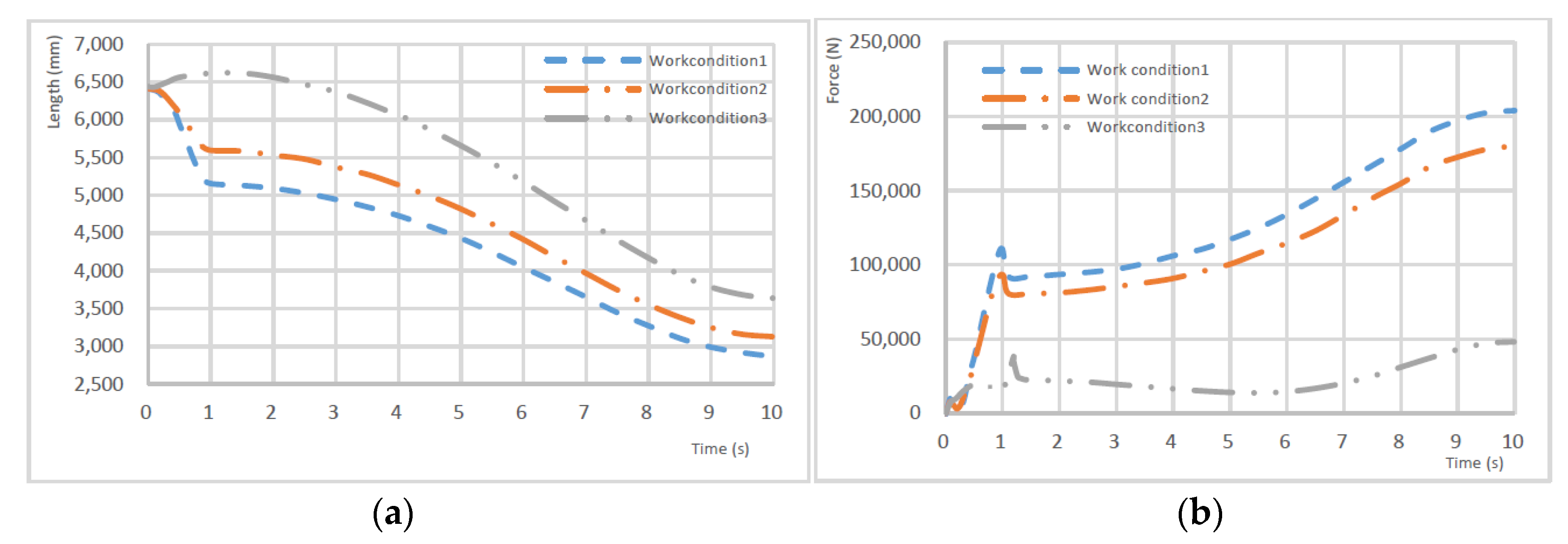
4. Results Analysis
5. Conclusions
Author Contributions
Conflicts of Interest
References
- Pijuan, J.; Comellas, M.; Nogués, M.; Roca, J.; Potau, X. Active bogies and chassis levelling for a vehicle operating in rough terrain. J. Terramech. 2012, 49, 161–171. [Google Scholar] [CrossRef]
- Shen, R.; Liu, J.; Wang, D. Stroke length of hydraulic cylinder and moving trail of harvester boom. J. Beijing For. Univ. 2010, 32, 157–160. [Google Scholar]
- Grebner, D.L.; Bettinger, P.; Siry, J.P. Chapter 12—Forest Harvesting Systems. In Introduction to Forestry and Natural Resources; Academic Press: San Diego, CA, USA, 2013; pp. 287–302. [Google Scholar]
- Sun, G.; Liu, J. Dynamic responses of hydraulic crane during luffing motion. Mech. Mach. Theory 2006, 41, 1273–1288. [Google Scholar] [CrossRef]
- Sun, G.; Kleeberger, M.; Liu, J. Complete dynamic calculation of lattice mobile crane during hoisting motion. Mech. Mach. Theory 2005, 40, 447–466. [Google Scholar] [CrossRef]
- Chin, C.; Nayfeh, A.H.; Abdel-Rahman, E. Nonlinear dynamics of a boom crane. J. Vib. Control 2001, 7, 199–220. [Google Scholar] [CrossRef]
- Hong, K.-S.; Ngo, Q.H. Dynamics of the container crane on a mobile harbor. Ocean Eng. 2012, 53, 16–24. [Google Scholar] [CrossRef]
- Blackburn, D.; Lawrence, J.; Danielson, J.; Singhose, W.; Kamoi, T.; Taura, A. Radial-motion assisted command shapers for nonlinear tower crane rotational slewing. Control Eng. Pract. 2010, 18, 523–531. [Google Scholar] [CrossRef]
- Hannan, M.A.; Bai, W. Analysis of nonlinear dynamics of fully submerged payload hanging from offshore crane vessel. Ocean Eng. 2016, 128, 132–146. [Google Scholar] [CrossRef]
- Sawodny, O.; Schneider, K.; Aschemann, H.; Kumpel, J.; Tarin, C. Aktives Lastpendeldampfungssystem fur Liebherrauslegerdrehkrane; Herausgegeben als Begleitband zur gleichnamigen Fachtagung: Dresden, Germany, 2001; pp. 1–17. [Google Scholar]
- Araya, H.; Kakuzen, M.; Kinugawa, H.; Arai, T. Level luffing control system for crawler cranes. Automat. Construct. 2004, 13, 689–697. [Google Scholar] [CrossRef]
- Kilicaslan, S.; Balkan, T.; Ider, S.K. Tipping load of mobile cranes with flexible booms. J. Sound Vib. 1999, 223, 645–657. [Google Scholar] [CrossRef]
- Shen, R.; Liu, J. Operation trajectory of harvester manipulator. J. Fujian Agric. For. Univ. (Nat. Sci. Ed.) 2009, 38, 431–435. [Google Scholar]
- Shen, R. Research on Forestry Felling & Cultivation Machine Executive Mechanism and Load-Sensing Hydraulic System; Being Forestry University: Being, China, 2010; pp. 34–38. [Google Scholar]
- Zhao, Z. Kinematics Analysis and Jacobian Matrix Solution for the Manipulator of a Logging Harvester. For. Mach. Woodwork. Equip. 2010, 38, 45–47. [Google Scholar]
- Ge, A.; Tao, P.; Li, Q. Kinematic Simulation and Analysis of the Harvester Arm System Based on ADAMS. For. Eng. 2012, 32, 33–37. [Google Scholar]
- Jiang, F.-M.; Guo, W.-D.; Zhang, P.; Zhao, Y.B. Design and Research of Robot Arm for Transfering Pipette Based on Virtual Prototype Technology. Mach. Des. Manuf. 2013, 25, 94–96. [Google Scholar]

| Part i | Part j | Constraint Relationship |
|---|---|---|
| Base | Ground | Fixed |
| Base | Main cylinder | Revolute |
| Base | Boom | Revolute |
| Cylinder | Piston rod | Translational |
| Main boom | Second boom | Revolute |
| Jib | Telescopic boom | Translational |
| Pusher | Puller | Revolute |
| Max Working Range (mm) | Max Force of Cylinder (N) | Average Force of Cylinder (N) | |
|---|---|---|---|
| Work condition 1 | 4798 | 1.06 × 105 | 0.78 × 105 |
| Work condition 2 | 6923 | 1.92 × 105 | 1.63 × 105 |
| Work condition 3 | 8235 | 2.33 × 105 | 1.95 × 105 |
| Work condition 4 | 5148 | 2.05 × 105 | 1.27 × 105 |
| Work condition 5 | 5592 | 1.8 × 105 | 1.10 × 105 |
| Work condition 6 | 6630 | 0.48 × 105 | 0.23 × 105 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shen, R.; Zhang, X.; Zhou, C. Dynamic Simulation of the Harvester Boom Cylinder. Machines 2017, 5, 13. https://doi.org/10.3390/machines5020013
Shen R, Zhang X, Zhou C. Dynamic Simulation of the Harvester Boom Cylinder. Machines. 2017; 5(2):13. https://doi.org/10.3390/machines5020013
Chicago/Turabian StyleShen, Rongfeng, Xiaozhen Zhang, and Chengjun Zhou. 2017. "Dynamic Simulation of the Harvester Boom Cylinder" Machines 5, no. 2: 13. https://doi.org/10.3390/machines5020013
APA StyleShen, R., Zhang, X., & Zhou, C. (2017). Dynamic Simulation of the Harvester Boom Cylinder. Machines, 5(2), 13. https://doi.org/10.3390/machines5020013





