An Exergoeconomic Evaluation of an Innovative Polygeneration System Using a Solar-Driven Rankine Cycle Integrated with the Al-Qayyara Gas Turbine Power Plant and the Absorption Refrigeration Cycle
Abstract
1. Introduction
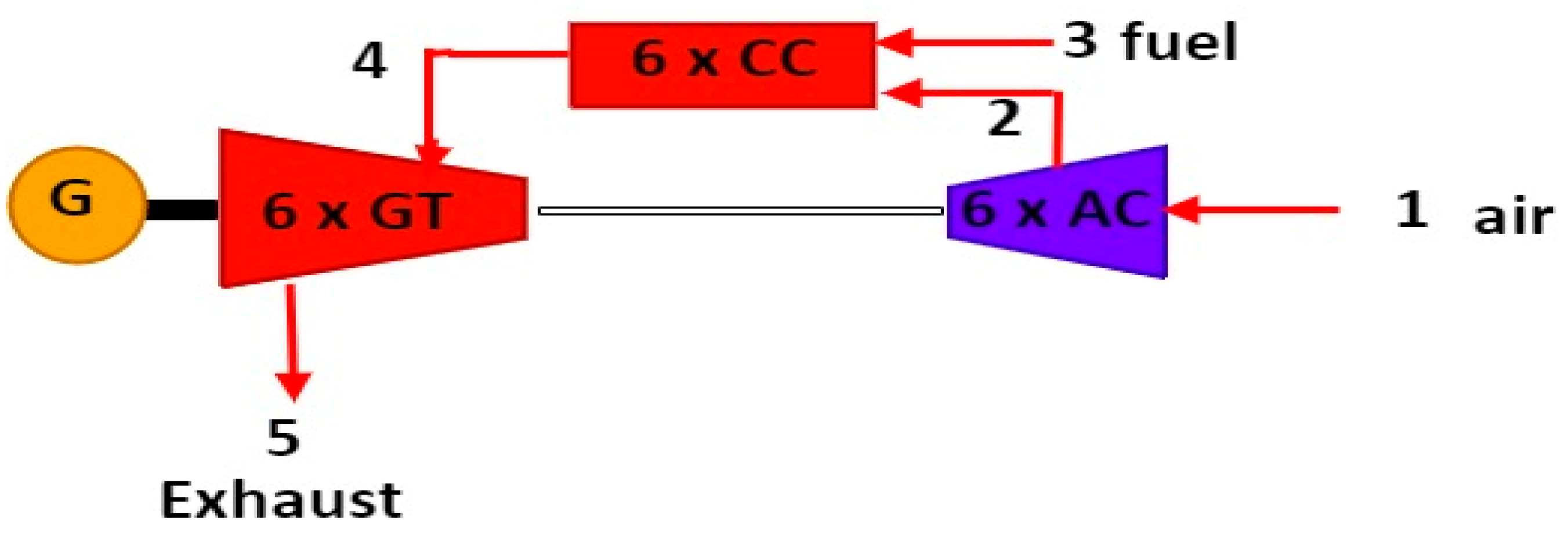
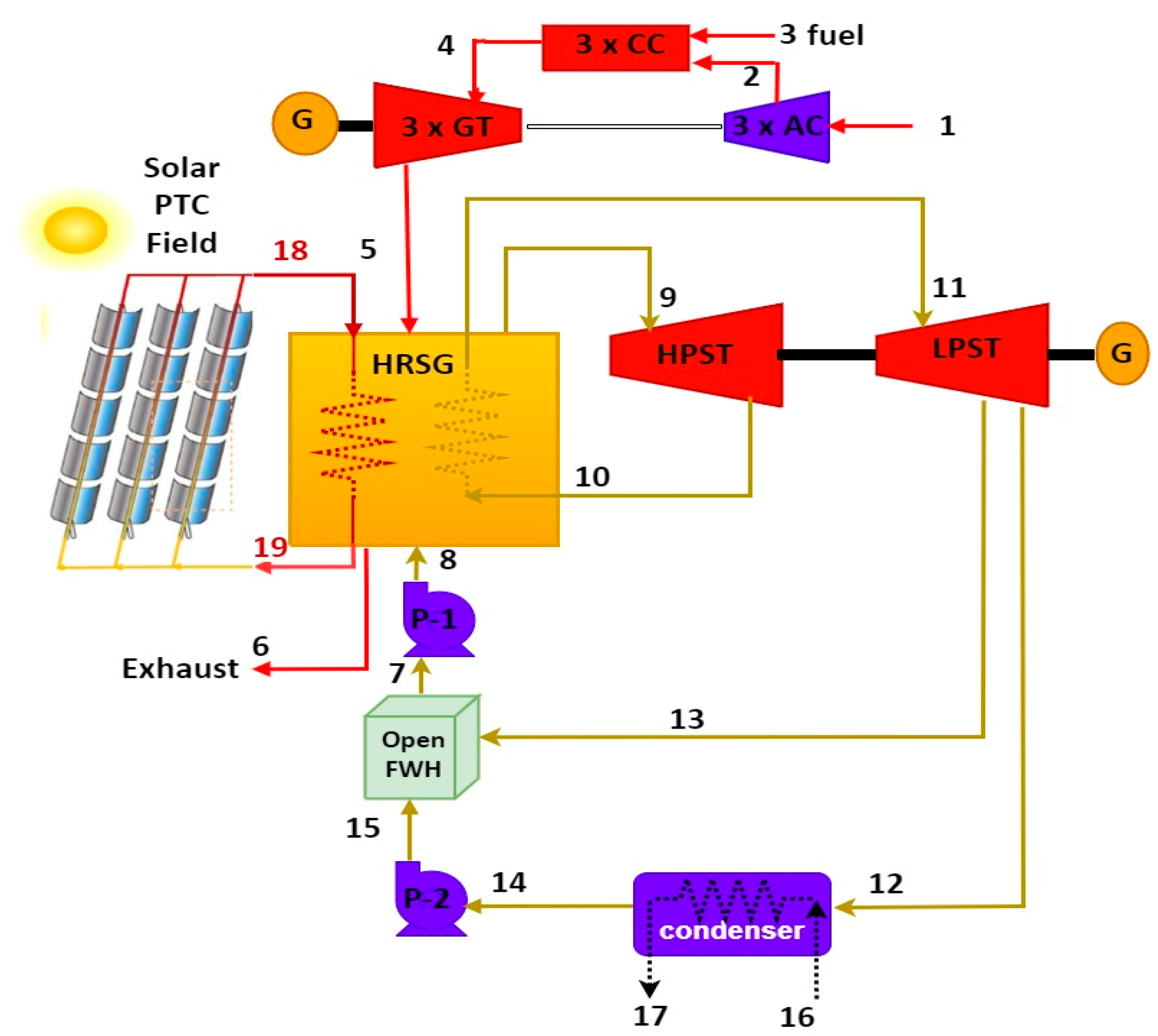
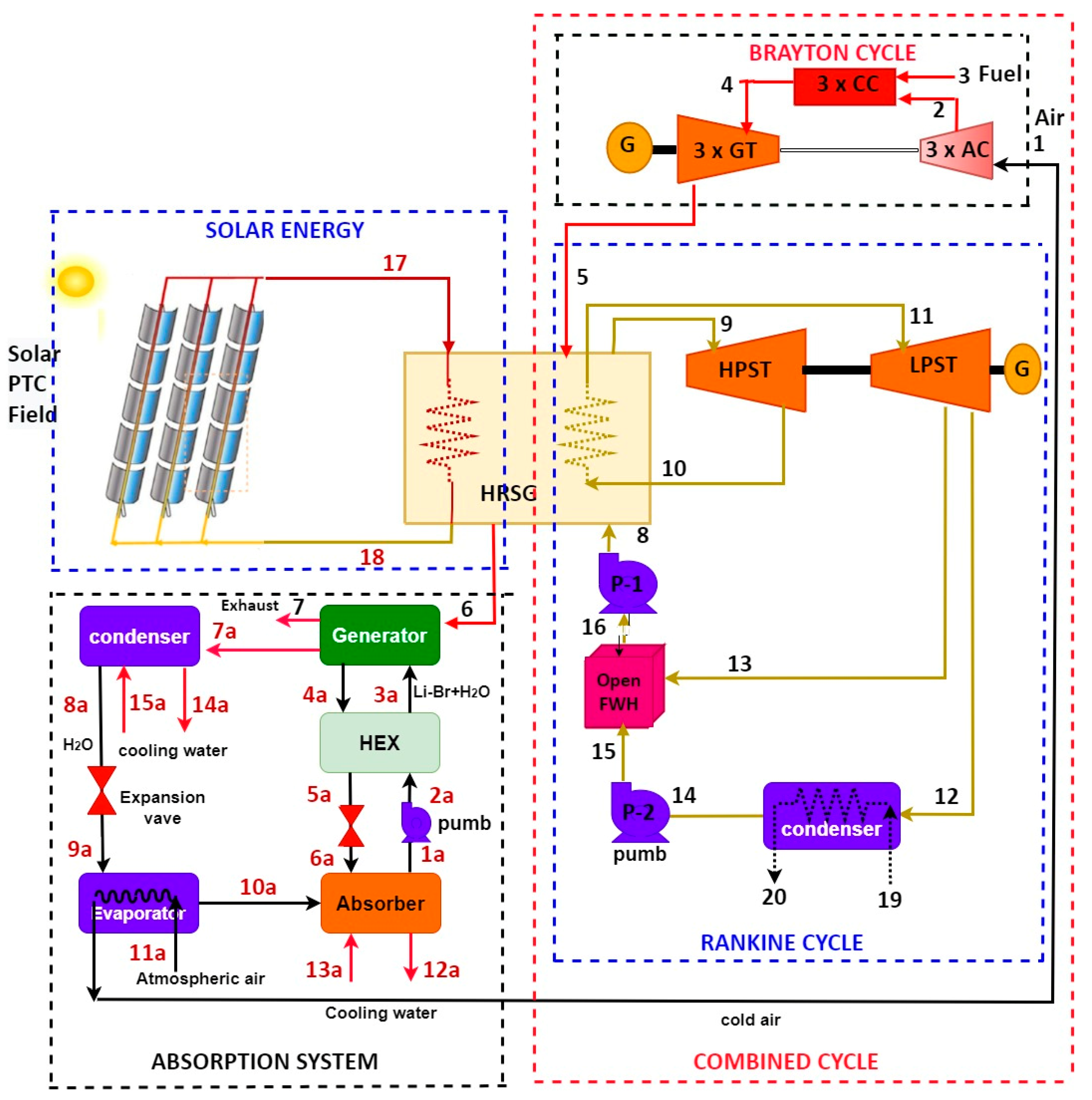
2. System Description
3. Thermodynamics Model
- The system operates in a steady-state condition.
- Air is classified as an ideal gas.
- The pressure drop, heat loss, and friction effect of the pipe network and heat exchangers are all negligible.
- There are no changes in kinetic and potential energy, and the energies remain at zero.
- Compressors, turbines, and pumps are mathematically represented using adiabatic models that include a certain isentropic efficiency.
3.1. PTC System Model
3.2. Thermodynamic Analysis
3.3. Investment Cost of the Main Equipment
4. Results and Discussion
4.1. Base Case
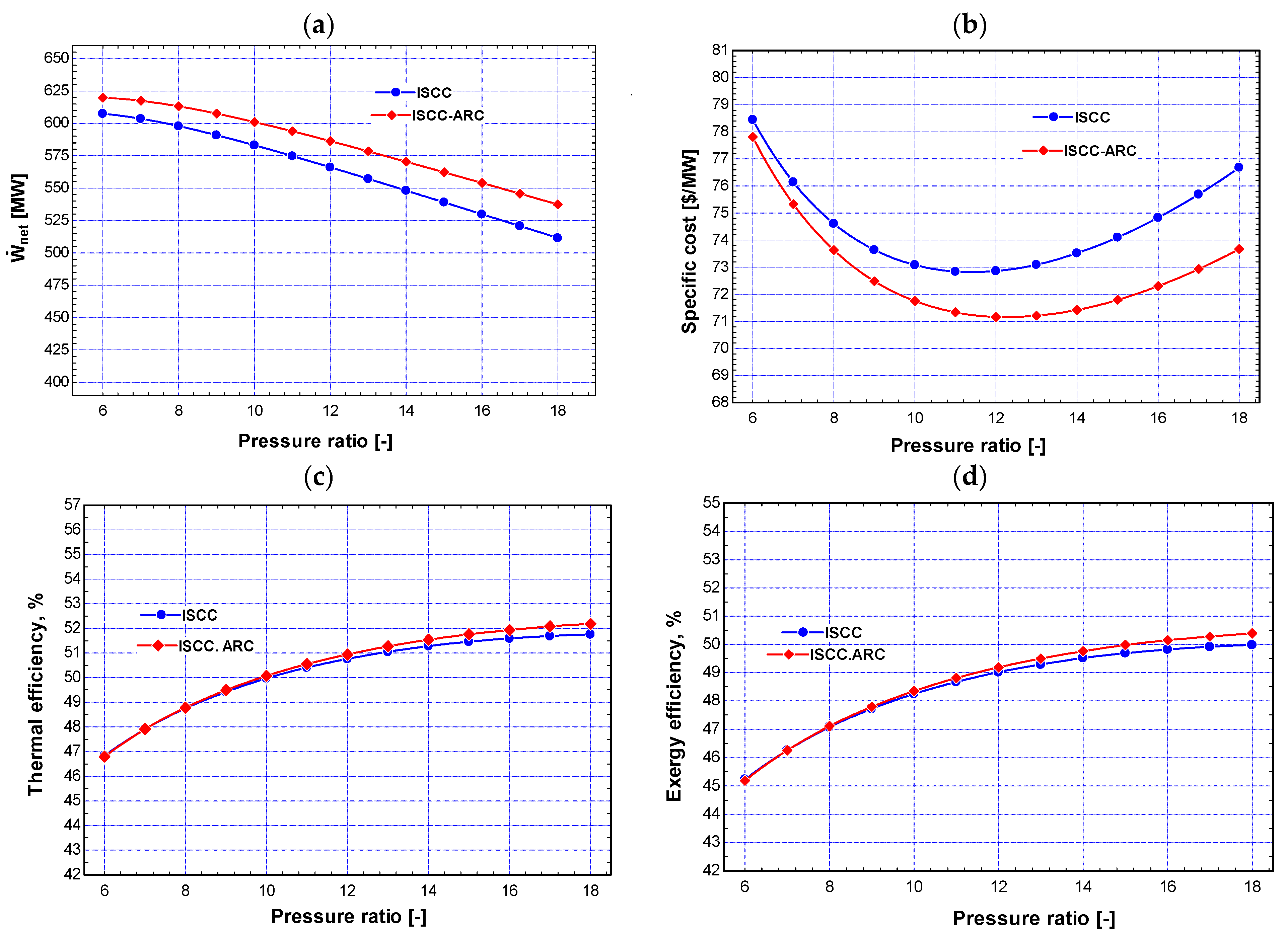
4.2. Results of the Parametric Studies
4.3. Validation Study Results
5. Conclusions and Recommendations
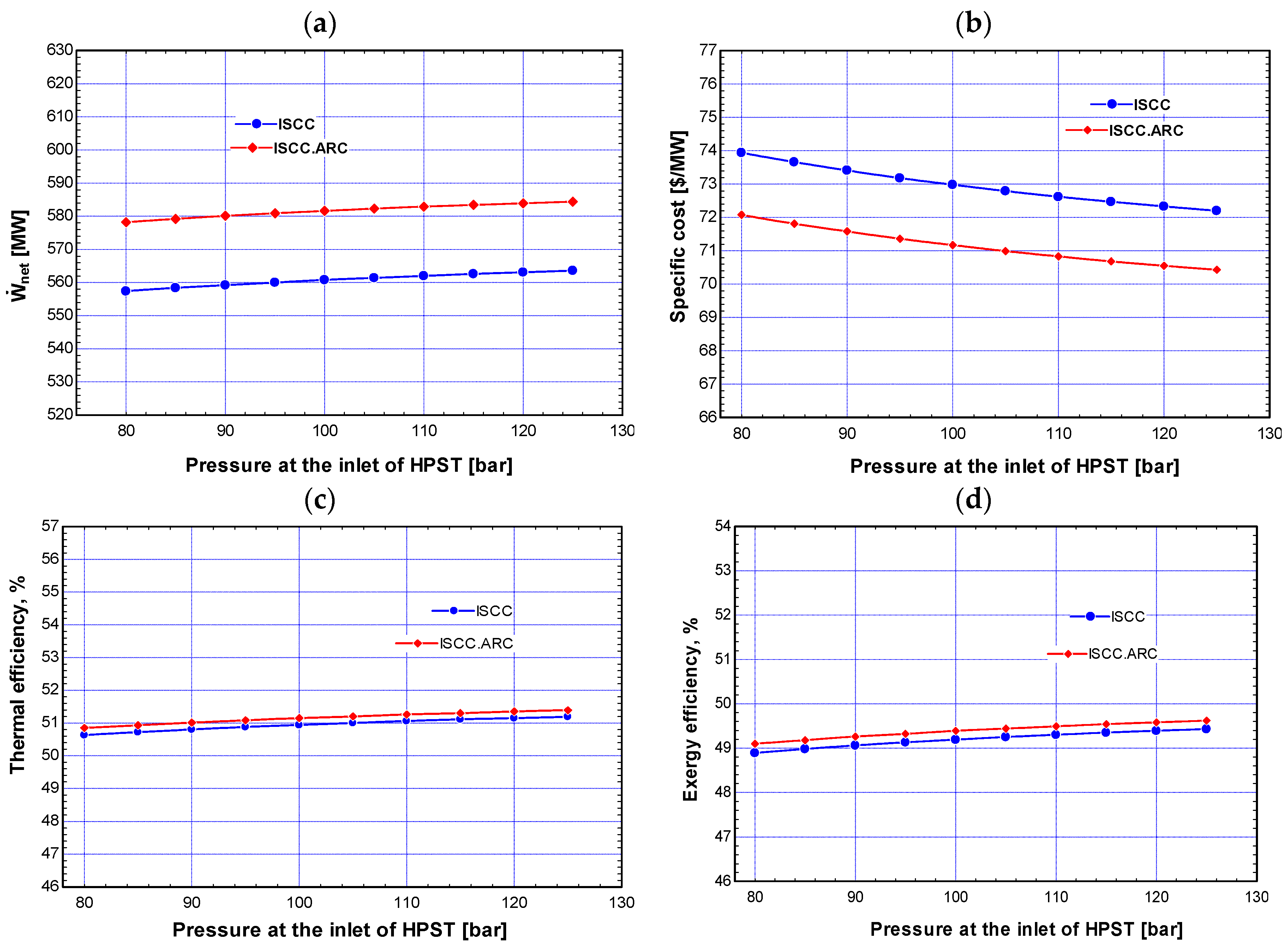
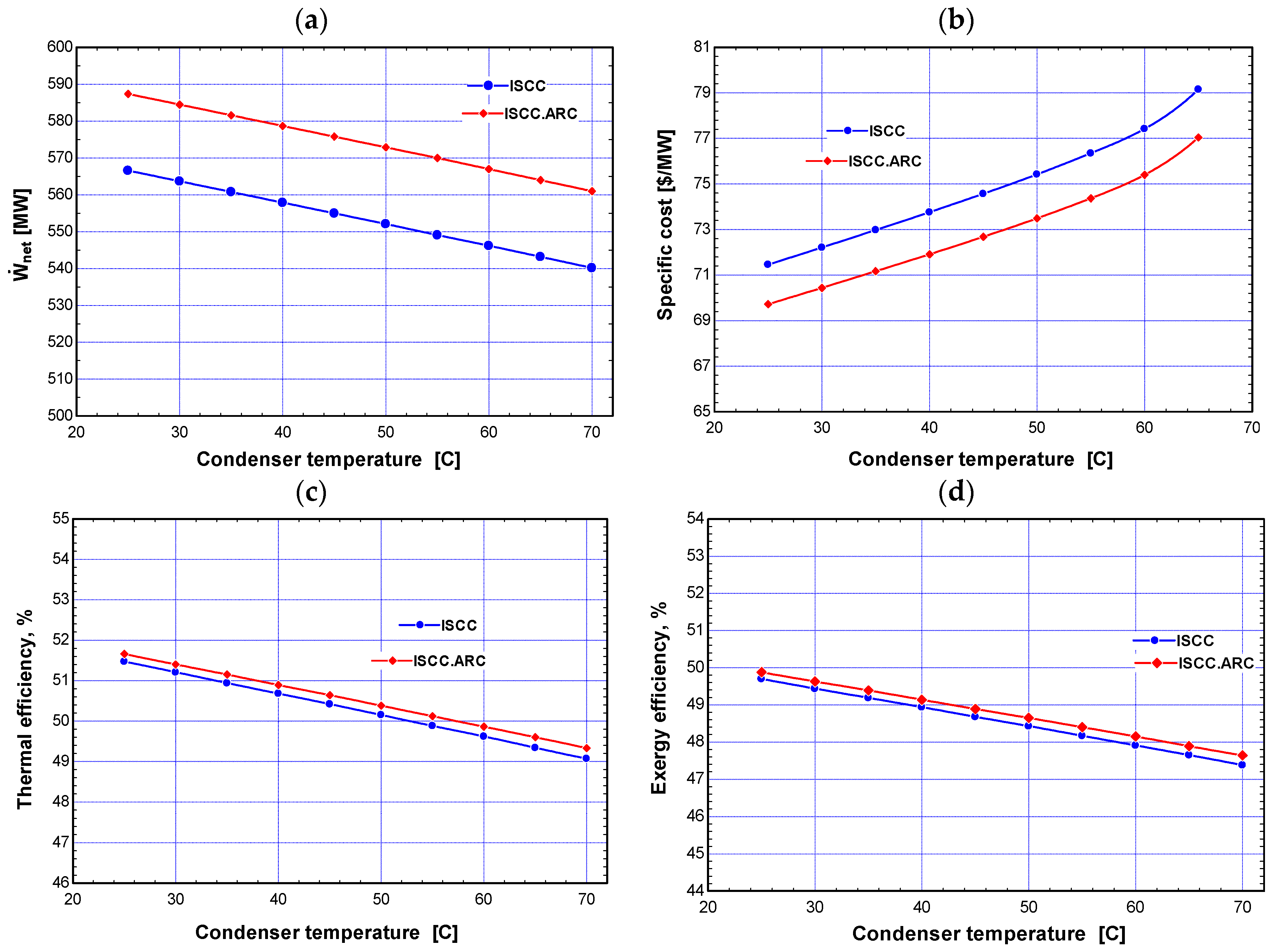
- Adding the absorption refrigeration cycle improves the , , and of the ISCC-ARC system, the performance of which is far better than that of the ISCC system at keeping the temperature at the inlet of the air compressor at 10 °C, enhancing the , , and of the ISCC-ARC system. In contrast, the , , and of the ISCC system reduce with the increase in the ambient temperature. The work input to the compressor decreases from 315.2 MW to 271.5 MW due to maintaining the temperature of the intake air for the AC at 10 °C.
- The ISCC produces 547.4 MW, while the ISCC-ARC produces 581.6 MW. Adding an ARC to the system increases the produced output by 34.2 MW.
- The thermal and exergy efficiencies and ) are, respectively, 50.89% and 49.14% for the ISCC system, whereas they increase to 51.15% and 46.4%, respectively, for the ISCC-ARC system.
- The highest exergy destruction of the elements in both systems is related to the combustion chambers because of their chemical reaction. The for each element of the ISCC-ARC system is lower than that for the ISCC, except for the CC because the increase in the flow rate of fuel in the ISCC-ARC system causes an elevation in the for the CC.
- A high steam turbine inlet pressure positively impacts cycle performance because the efficiencies and of both systems increase as the PHPST.in increases.
- The gas turbine inlet temperature increases the thermal energy for the exhaust gases at the inlet of the GT, which leads to an increase in the and efficiencies of both cycles.
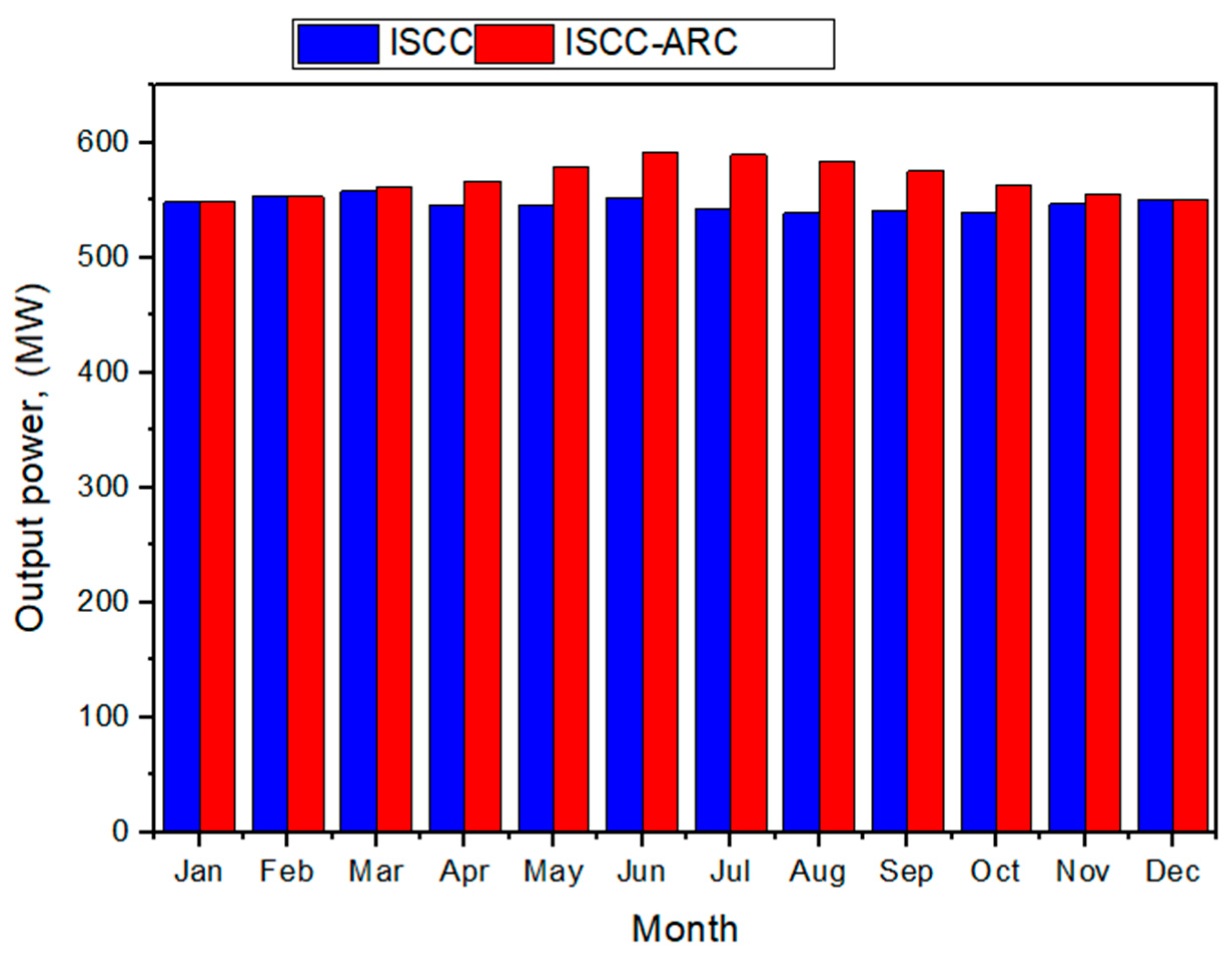
- The overall specific costs for the ISCC-ARC system range from 69.09 $/MWh in June to 79.05 $/MWh in December. The overall specific costs of the ISCC also fluctuate during the year, from 72.56 $/MWh in June to 78.73 $/MWh in December.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Area of the solar field (m2) | |
| Cost rate ($/h) | |
| DNI | Direct normal irradiance of the sun |
| Exergy rate (kJ) | |
| Exergy destruct | |
| Heat loss exergy | |
| Exergy of power | |
| Mass flow rate (kg/s) | |
| h | Specific enthalpy (kJ·kg−1) |
| i | Interest rate |
| N | Number of operating hours |
| Lower heating value of fuel | |
| Heat transfer rate (kW) | |
| T | Temperature |
| Tcond | Condenser temperature |
| DNI sun temperature | |
| Power (kW) | |
| Entire cost rate | |
| Greek Symbols | |
| Energy efficiency | |
| Energy performance | |
| Exergy efficiency | |
| Maintenance factor | |
| Exergy efficiency | |
| Subscripts | |
| D | Destruction |
| e | Exit |
| i | Inlet |
| f | Fuel |
| p | Product |
| q | Related to heat |
| w | Related to work |
| tot | Total |
| Therminol VP-1 | |
| Abbreviations | |
| AC | Air compressor |
| ARC | Absorption refrigeration cycle |
| BC | Brayton cycle |
| CC | Combustion chamber |
| CCPP | Combined cycle power plant |
| Con | Condenser |
| CRF | Capital Recovery Factor |
| CSP | Concentrating Solar Power |
| EES | Engineering Equation Solver |
| GE | General Electric |
| GT | Gas turbine |
| GTIT | Gas turbine inlet temperature |
| HRSG | Heat Recovery Steam Generation |
| HPST | High-pressure steam turbine |
| ISCC | Integrated solar combined cycle |
| ISCC-ARC | Integrated solar combined cycle with an absorption refrigeration cycle |
| LiBr | Lithium bromide |
| LPST | Low-pressure steam turbine |
| ORC | Organic Rankine cycle |
| Pr | Pressure ratio |
| PTC | Parabolic trough collector |
References
- Ibraheem, W.E.; Abdulraheem, A.A. Design of 5MW PV Power Plant in Iraq in Al-Sharqiyah Diyala Substation. In Proceedings of the 2021 Fourth International Conference on Electrical, Computer and Communication Technologies (ICECCT), Erode, India, 15–17 September 2021; pp. 1–7. [Google Scholar]
- Dawood, T.A.; Raphael, R.; Barwari, I.; Akroot, A. Solar Energy and Factors Affecting the Efficiency and Performance of Panels in Erbil/Kurdistan. Int. J. Heat Technol. 2023, 41, 304–312. [Google Scholar] [CrossRef]
- Khudhur, J.; Akroot, A.; Al-samari, A. Experimental Investigation of Direct Solar Photovoltaics that Drives Absorption Refrigeration System. J. Adv. Res. Fluid Mech. Therm. Sci. 2023, 1, 116–135. [Google Scholar] [CrossRef]
- Abed, F.M.; Al-Douri, Y.; Al-Shahery, G.M. Review on the energy and renewable energy status in Iraq: The outlooks. Renew. Sustain. Energy Rev. 2014, 39, 816–827. [Google Scholar] [CrossRef]
- Abusaibaa, G.Y.; Al-Aasam, A.B.; Al-Waeli, A.H.A.; Al-Fatlawi, A.W.A.; Sopian, K. Performance analysis of solar absorption cooling systems in Iraq. Int. J. Renew. Energy Res. 2020, 10, 223–230. [Google Scholar] [CrossRef]
- U.S. Energy Information Administration (EIA). International Energy Outlook 2016. Available online: http://www.eia.gov/forecasts/ieo/ (accessed on 1 October 2023).
- Haseeb, Q.S.; Yunus, S.M.; Shoshan, A.A.A.; Aziz, A.I. A study of the optimal form and orientation for more energy efficiency to mass model mult-istorey buildings of Kirkuk city, Iraq. Alex. Eng. J. 2023, 71, 731–741. [Google Scholar] [CrossRef]
- Saeed, I.M.; Ramli, A.T.; Saleh, M.A. Assessment of sustainability in energy of Iraq, and achievable opportunities in the long run. Renew. Sustain. Energy Rev. 2016, 58, 1207–1215. [Google Scholar] [CrossRef]
- Hassan, Q.; Hafedh, S.A.; Hasan, A.; Jaszczur, M. Evaluation of energy generation in Iraqi territory by solar photovoltaic power plants with a capacity of 20 MW. Energy Harvest. Syst. 2022, 9, 97–111. [Google Scholar] [CrossRef]
- Kazem, H.A.; Chaichan, M.T. Status and future prospects of renewable energy in Iraq. Renew. Sustain. Energy Rev. 2012, 16, 6007–6012. [Google Scholar] [CrossRef]
- Talal, W.; Akroot, A. Exergoeconomic Analysis of an Integrated Solar Combined Cycle in the Al-Qayara Power Plant in Iraq. Processes 2023, 11, 656. [Google Scholar] [CrossRef]
- Faisal, S.H.; Naeem, N.K.; Jassim, A.A. Energy and exergy study of Shatt Al-Basra gas turbine power plant. J. Phys. Conf. Ser. 2021, 1773, 012020. [Google Scholar] [CrossRef]
- Salah, S.A.; Abbas, E.F.; Ali, O.M.; Alwan, N.T.; Yaqoob, S.J.; Alayi, R. Evaluation of the gas turbine unit in the Kirkuk gas power plant to analyse the energy and exergy using ChemCad simulation. Int. J. Low-Carbon Technol. 2022, 17, 603–610. [Google Scholar] [CrossRef]
- Ahmed, A.H.; Ahmed, A.M.; Hamid, Q.Y. Exergy and energy analysis of 150 MW gas turbine unit: A case study. J. Adv. Res. Fluid Mech. Therm. Sci. 2020, 67, 186–192. [Google Scholar]
- Kareem, A.F.; Akroot, A.; Wahhab, H.A.A.; Talal, W.; Ghazal, R.M.; Alfaris, A. Exergo–Economic and Parametric Analysis of Waste Heat Recovery from Taji Gas Turbines Power Plant Using Rankine Cycle and Organic Rankine Cycle. Sustainability 2023, 15, 9376. [Google Scholar] [CrossRef]
- Elberry, M.; Elsayed, A.; Teamah, M.; Abdel-Rahman, A.; Elsafty, A. Performance improvement of power plants using absorption cooling system. Alex. Eng. J. 2018, 57, 2679–2686. [Google Scholar] [CrossRef]
- Santos, A.P.P.; Andrade, C.R.; Zaparoli, E. Comparison of different gas turbine inlet air cooling methods, World Academy of Science. Eng. Technol. 2012, 6, 1–6. [Google Scholar]
- Kwon, H.M.; Kim, T.S.; Sohn, J.L.; Kang, D.W. Performance improvement of gas turbine combined cycle power plant by dual cooling of the inlet air and turbine coolant using an absorption chiller. Energy 2018, 163, 1050–1061. [Google Scholar] [CrossRef]
- Javanfam, F.; Ghiasirad, H.; Khoshbakhti Saray, R. Efficiency Improvement and Cost Analysis of a New Combined Absorption Cooling and Power System; Springer International Publishing: New York, NY, USA, 2022. [Google Scholar] [CrossRef]
- Sa, A.D.; Zubaidy, S.A. Gas turbine performance at varying ambient temperature. Appl. Therm. Eng. 2011, 31, 2735–2739. [Google Scholar] [CrossRef]
- Oko, C.O.C.; Njoku, I. Performance analysis of an integrated gas-, steam- and organic fluid-cycle thermal power plant. Energy 2017, 122, 431–443. [Google Scholar] [CrossRef]
- Shukla, A.K.; Singh, O. Effect of Compressor Inlet Temperature & Relative Humidity on Gas Turbine Cycle Performance. Eng. Environ. Sci. 2014, 5, 664–671. [Google Scholar]
- Karaalı, R. Exergy analysis of a combined power and cooling cycle. Acta Phys. Pol. A 2016, 130, 209–213. [Google Scholar] [CrossRef]
- Settino, J.; Ferraro, V.; Morrone, P. Energy analysis of novel hybrid solar and natural gas combined cycle plants. Appl. Therm. Eng. 2023, 230, 120673. [Google Scholar] [CrossRef]
- Roshanzadeh, B.; Asadi, A.; Mohan, G. Technical and Economic Feasibility Analysis of Solar Inlet Air Cooling Systems for Combined Cycle Power Plants. Energies 2023, 16, 5352. [Google Scholar] [CrossRef]
- Nami, H.; Mahmoudi, S.M.A.; Nemati, A. Exergy, economic and environmental impact assessment and optimization of a novel cogeneration system including a gas turbine, a supercritical CO2 and an organic Rankine cycle (GT-HRSG/SCO2). Appl. Therm. Eng. 2017, 110, 1315–1330. [Google Scholar] [CrossRef]
- Bakhshmand, S.K.; Saray, R.K.; Bahlouli, K.; Eftekhari, H.; Ebrahimi, A. Exergoeconomic analysis and optimization of a triple-pressure combined cycle plant using evolutionary algorithm. Energy 2015, 93, 555–567. [Google Scholar] [CrossRef]
- Akroot, A.; Namli, L. Performance assessment of an electrolyte-supported and anode-supported planar solid oxide fuel cells hybrid system. J. Ther. Eng. 2021, 7, 1921–1935. [Google Scholar] [CrossRef]
- Nourpour, M.; Manesh, M.K. Evaluation of novel integrated combined cycle based on gas turbine-SOFC-geothermal-steam and organic Rankine cycles for gas turbo compressor station. Energy Convers. Manag. 2022, 252, 115050. [Google Scholar] [CrossRef]
- Köse, A.; Koç, Y.; Yağlı, H. Energy, exergy, economy and environmental (4E) analysis and optimization of single, dual and triple configurations of the power systems: Rankine Cycle/Kalina Cycle, driven by a gas turbine. Energy Convers. Manag. 2021, 227, 113604. [Google Scholar] [CrossRef]
- Mohammadkhani, F.; Shokati, N.; Mahmoudi, S.; Yari, M.; Rosen, M. Exergoeconomic assessment and parametric study of a Gas Turbine-Modular Helium Reactor combined with two Organic Rankine Cycles. Energy 2014, 65, 533–543. [Google Scholar] [CrossRef]
- Cavalcanti, E.J.C. Exergoeconomic and exergoenvironmental analyses of an integrated solar combined cycle system. Renew. Sustain. Energy Rev. 2017, 67, 507–519. [Google Scholar] [CrossRef]
- Li, Z.; Liu, L.; Jing, Y. Exergoeconomic analysis of solar absorption-subcooled compression hybrid cooling system. Energy Convers. Manag. 2017, 144, 205–216. [Google Scholar] [CrossRef]
- Xie, N.; Xiao, Z.; Du, W.; Deng, C.; Liu, Z.; Yang, S. Thermodynamic and exergoeconomic analysis of a proton exchange membrane fuel cell/absorption chiller CCHP system based on biomass gasification. Energy 2023, 262, 125595. [Google Scholar] [CrossRef]
- Musharavati, F.; Khanmohammadi, S.; Pakseresht, A. A novel multi-generation energy system based on geothermal energy source: Thermo-economic evaluation and optimization. Energy Convers. Manag. 2021, 230, 113829. [Google Scholar] [CrossRef]
- Sachdeva, J.; Singh, O. Thermodynamic analysis of solar powered triple combined Brayton, Rankine and organic Rankine cycle for carbon free power. Renew. Energy 2019, 139, 765–780. [Google Scholar] [CrossRef]
- Lugand, P.; Parietti, C. Combined Cycle Plants with Frame 9F Gas Turbines; American Society of Mechanical Engineers: New York, NY, USA, 1990; Volume 4, pp. 1–8. [Google Scholar] [CrossRef]
- Moran, M.J.; Shapiro, H.N.; Boettner, D.D. Fundamentals of Engineering Thermodynamics; Wiley: Hoboken, NJ, USA, 2020. [Google Scholar] [CrossRef]


| Component | Energy Balances | Exergy Balances |
|---|---|---|
| Compressor | ||
| Combustion chamber | ||
| Gas turbine | ||
| HRSG | ||
| HPST | ||
| LPST | ||
| Condenser | ||
| Pump 1 | ||
| Pump 2 | ||
| OFWH | ||
| PTC | ||
| Generator | ||
| Absorber | ||
| HEX | ||
| Pump 3 | ||
| Evaporator | ||
| EV 1 | ||
| EV2 |
| Component | Exergetic Cost Rate Balance Equation | Auxiliary Equation |
|---|---|---|
| AC | ||
| CC | , | |
| GT | ||
| HRSG | ||
| HPST | ||
| LPST | = | |
| Condenser 1 | ||
| Pump 1 | ||
| Pump 2 | ||
| OFWH | ||
| PTC | ||
| Generator | ||
| Pump 3 | ||
| SHEX | ||
| EV1 | ||
| Absorber | , | |
| Evaporator | , | |
| EV2 | ||
| Condenser 2 | , |
| Part | Purchased Equation |
|---|---|
| AC | |
| CC | |
| GT | |
| HRSG | |
| HPST | |
| LPST | |
| Pump 1 | |
| Pump 2 | |
| OFWH | |
| PTC | |
| Generator | 17,500 |
| Absorber | 16,000 |
| SHEX | 309.14 |
| Pump 3 | 17,585 |
| Evaporator | 16,000 |
| Ev1 | |
| Ev2 |
| Factor | Significance | |
|---|---|---|
| Number of (GT) units | 3 | |
| Brayton cycle | Ratio of compression | 12.4 |
| Mass flowrate (kg/s) | (420 × 3) | |
| Gas inlet temperature (°C) | 1090 | |
| Atmosphere temperature (°C) | 25 | |
| Low-heat-value fuel (kJ.kg−1) | 50,050 | |
| (%) | 85 | |
| (%) | 87 | |
| (%) | 99.51 | |
| Rankine cycle | High-pressure steam turbine (bar) | 100 |
| Low-pressure steam turbine (bar) | 20 | |
| Condensate temperature (°C) | 36 | |
| (%) | 87 | |
| (%) | 82 | |
| Effectiveness for HRSG (%) | 72 | |
| ARS | Generator temperature (°C) | 88 |
| Condenser temperature (°C) | 39 | |
| Absorber outlet temperature (°C) | 48 | |
| SHE effectiveness (%) | 53 | |
| Evaporator temperature (°C) | 5 | |
| Evaporator inlet air temperature (°C) | 50 | |
| Evaporator outlet air temperature (°C) | 10 | |
| Condenser inlet water temperature (°C) | 25 | |
| Condenser outlet water temperature (°C) | 35 | |
| Absorber inlet water temperature (°C) | 25 | |
| Absorber outlet water temperature (°C) | 35 | |
| LiBr Solution | Solution strength (%) | 53 |
| Solar Area | Latitude (degrees) | 35.36° N |
| Longitude (degrees) | 43.17° E | |
| Place | Mosul/Iraq | |
| Solar area (m2) | 510,130 | |
| Outlet temperature (°C) | 395 | |
| Inlet temperature (°C) | 295 | |
| Heat transfer fluid | Therminol VP-1 |
| State | Mass (kg/s) | Pressure (kPa) | Temperature (K) | Enthalpy (kJ/kg) | Entropy (KJ/kg. K) | Exergy (MW) |
|---|---|---|---|---|---|---|
| 1 | 418 | 101.3 | 283 | 261.3 | 5.679 | 0 |
| 2 | 418 | 1277 | 632.7 | 621.8 | 5.774 | 138.8 |
| 3 | 7.573 | 101.3 | 288 | −4672 | 11.53 | 392.6 |
| 4 | 425.6 | 1213 | 1360 | 235.4 | 8.036 | 406.8 |
| 5 | 425.6 | 104.5 | 822.9 | −419.2 | 8.144 | 114.4 |
| 6 | 425.6 | 101.3 | 402.9 | −885.1 | 7.365 | 15.04 |
| 7 | 665.4 | 371.5 | 101.3 | −922.3 | 7.322 | 6.135 |
| 8 | 150.4 | 121.6 | 373.1 | 548.8 | 1.65 | 16.76 |
| 9 | 150.4 | 10,133 | 406.4 | 566.8 | 1.659 | 19.07 |
| 10 | 150.4 | 9829 | 794.9 | 3433 | 6.681 | 296.7 |
| 11 | 150.4 | 2007 | 581.8 | 3044 | 6.802 | 232.8 |
| 12 | 150.4 | 1946 | 774.9 | 3473 | 7.452 | 268.2 |
| 13 | 135.4 | 31 | 352.5 | 2645 | 7.81 | 114.8 |
| 14 | 15.04 | 121.6 | 463.4 | 2855 | 7.702 | 16.4 |
| 15 | 135.4 | 31 | 343 | 292.4 | 0.9533 | 8.504 |
| 16 | 135.4 | 121.6 | 343 | 292.6 | 0.9534 | 8.517 |
| 17 | 520.5 | 1000 | 665 | 780.6 | 1.675 | 146.5 |
| 18 | 520.5 | 1000 | 566 | 539.3 | 1.283 | 81.68 |
| 19 | 7038 | 101 | 283 | 41.39 | 0.1489 | 0 |
| 20 | 7038 | 101 | 295 | 91.66 | 0.3228 | 7.301 |
| 1a | 85.21 | 310 | 0.8634 | 81.92 | 0.238 | 3.291 |
| 2a | 85.21 | 310.2 | 6.944 | 81.93 | 0.238 | 3.291 |
| 3a | 85.21 | 342.9 | 6.944 | 151.5 | 0.451 | 3.807 |
| 4a | 72.84 | 361 | 6.944 | 219.6 | 0.4816 | 7.554 |
| 5a | 72.84 | 333 | 6.944 | 167.2 | 0.3305 | 7.019 |
| 6a | 72.84 | 321.6 | 0.8634 | 167.2 | 0.3306 | 7.017 |
| 7a | 12.37 | 361 | 6.944 | 2656 | 7.506 | 3.291 |
| 8a | 12.37 | 312 | 6.944 | 162.7 | 0.557 | 0.01654 |
| 9a | 12.37 | 278 | 0.8634 | 162.7 | 0.586 | −0.09024 |
| 10a | 12.37 | 278 | 0.8634 | 2510 | 9.029 | −2.179 |
| 11a | 650 | 323 | 298 | 323.6 | 5.777 | 0.9202 |
| 12a | 1845 | 298 | 308 | 104.3 | 0.3651 | 0.2987 |
| 13a | 1845 | 308 | 298 | 146.1 | 0.5031 | 1.33 |
| 14a | 2890 | 298 | 308 | 104.3 | 0.3651 | 0.4681 |
| 15a | 2890 | 308 | 298 | 146.1 | 0.5031 | 2.084 |
| Output Quantity | ISCC | ISCC-ARC |
|---|---|---|
| Power supplied to ACs (MW) | 486.98 | 451.984 |
| Power output of GTs (MW) | 836.63 | 835.832 |
| Power output of HPST (MW) | 56.144 | 56.136 |
| Power output of LPST (MW) | 143.544 | 143.516 |
| Power supplied to P1 (kW) | 1881 | 1881 |
| Power supplied to P2 (kW) | 18.96 | 18.96 |
| Power supplied to P3 (kW) | - | 0.08 |
| Total work net of the system (MW) | 547.4 | 581.6 |
| Overall energy efficiency (%) | 50.89 | 51.15 |
| Overall exergy efficiency (%) | 49.14 | 49.4 |
| Part | (MW) | (MW) | (MW) | (%) | Ψ (%) |
|---|---|---|---|---|---|
| AC | 525 | 416.5 | 35.42 | 5.071 | 92.16 |
| CC | 1594 | 1220 | 373.8 | 53.51 | 76.55 |
| GT | 877.1 | 835.8 | 41.25 | 5.905 | 95.3 |
| HRSG | 363 | 305.2 | 57.83 | 8.278 | 84.07 |
| HPST | 61.35 | 56.14 | 5.209 | 0.7457 | 91.51 |
| LPST | 163.6 | 143.5 | 20.06 | 2.871 | 87.74 |
| Cond 1 | 71.56 | 6.828 | 64.74 | 9.267 | 9.541 |
| OFWH | 22.34 | 12.08 | 10.27 | 1.47 | 54.05 |
| Pump 1 | 1.881 | 1.581 | 0.3001 | 0.04296 | 84.04 |
| Pump 2 | 0.01896 | 0.01529 | 0.003669 | 0.0005253 | 80.64 |
| PTC | 139.4 | 64.79 | 74.64 | 10.68 | 46.47 |
| Generator | 9.926 | 1.727 | 8.199 | 1.181 | 17.4 |
| Condenser | 0.8035 | 0.3105 | 0.493 | 0.07105 | 38.64 |
| Absorber | 1.449 | 0.4865 | 0.9625 | 0.13873 | 33.58 |
| HEX | 0.1311 | 0.1266 | 0.004481 | 0.00064587 | 96.58 |
| Pump 3 | 0.00008 | 0.00008 | 0.00000029 | 0.000000041 | 100 |
| Evaporator | 0.5125 | 0.167 | 0.3456 | 0.0498134 | 32.58 |
| Ev1 | 0.004058 | 0.02214 | 0.262 | 0.0377636 | 84.51 |
| Ev2 | 1.722 | 1.722 | 0.0005273 | 0.000076 | 99.97 |
| Component | ($/GJ) | ($/GJ) | ($/h) | ($/h) | ($/h) | (%) |
|---|---|---|---|---|---|---|
| AC | 21.41 | 22.5 | 210.7 | 1107.4 | 1318 | 84.01 |
| CC | 14.94 | 19.51 | 1551 | 5.04 | 1556.04 | 0.03263 |
| GT | 19.51 | 20.73 | 223.6 | 781.2 | 1005 | 77.76 |
| HRSG | 18.32 | 20.44 | 294.4 | 506.9 | 801.3 | 63.26 |
| HPST | 21.86 | 24.87 | 31.63 | 197.1 | 228.73 | 86.17 |
| LPST | 21.17 | 24.87 | 118 | 380.16 | 489.16 | 76.32 |
| Cond 1 | 21.17 | 222 | 380.7 | 3.98 | 384.7 | 1.036 |
| OFWH | 21.18 | 41.83 | 60.41 | 116.892 | 177.3 | 65.92 |
| Pump 1 | 24.87 | 31.63 | 2.073 | 11.64 | 13.71 | 84.88 |
| Pump 2 | 24.87 | 38.92 | 0.02534 | 0.445 | 0.473 | 94.61 |
| PTC | 0 | 12.87 | 0 | 1000.44 | 1000.44 | 100 |
| Generator | 0.008237 | 0.04758 | 0.2431 | 5.152 | 5.395 | 95.49 |
| Absorber | 0.01908 | 0.05698 | 0.06612 | 0.9432 | 1.009 | 93.45 |
| HEX | 0.04582 | 0.0475 | 0.00074 | 0.1 | 0.10074 | 99.24 |
| Pump 3 | 24.93 | 0.01575 | 0 | 0.009 | 0.009 | 100 |
| Evaporator | 2.178 | 153.7 | 2.71 | 1.2456 | 3.956 | 31.49 |
| Ev1 | 276.6 | 50.76 | 0.00006 | 0.0054 | 0.00547 | 98.9 |
| Ev2 | 0.04582 | 0.04583 | 0.000087 | 0.03 | 0.03 | 99.73 |
| Total System | 2875.56 | 4118.763 | 6944.323 | 58.9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Talal, W.; Akroot, A. An Exergoeconomic Evaluation of an Innovative Polygeneration System Using a Solar-Driven Rankine Cycle Integrated with the Al-Qayyara Gas Turbine Power Plant and the Absorption Refrigeration Cycle. Machines 2024, 12, 133. https://doi.org/10.3390/machines12020133
Talal W, Akroot A. An Exergoeconomic Evaluation of an Innovative Polygeneration System Using a Solar-Driven Rankine Cycle Integrated with the Al-Qayyara Gas Turbine Power Plant and the Absorption Refrigeration Cycle. Machines. 2024; 12(2):133. https://doi.org/10.3390/machines12020133
Chicago/Turabian StyleTalal, Wadah, and Abdulrazzak Akroot. 2024. "An Exergoeconomic Evaluation of an Innovative Polygeneration System Using a Solar-Driven Rankine Cycle Integrated with the Al-Qayyara Gas Turbine Power Plant and the Absorption Refrigeration Cycle" Machines 12, no. 2: 133. https://doi.org/10.3390/machines12020133
APA StyleTalal, W., & Akroot, A. (2024). An Exergoeconomic Evaluation of an Innovative Polygeneration System Using a Solar-Driven Rankine Cycle Integrated with the Al-Qayyara Gas Turbine Power Plant and the Absorption Refrigeration Cycle. Machines, 12(2), 133. https://doi.org/10.3390/machines12020133







