Simulation and Experimental Study of Ultrasonic Vibratory Grinding of Internal Splines
Abstract
1. Introduction
2. Theoretical Model of Ultrasonic Vibratory Grinding
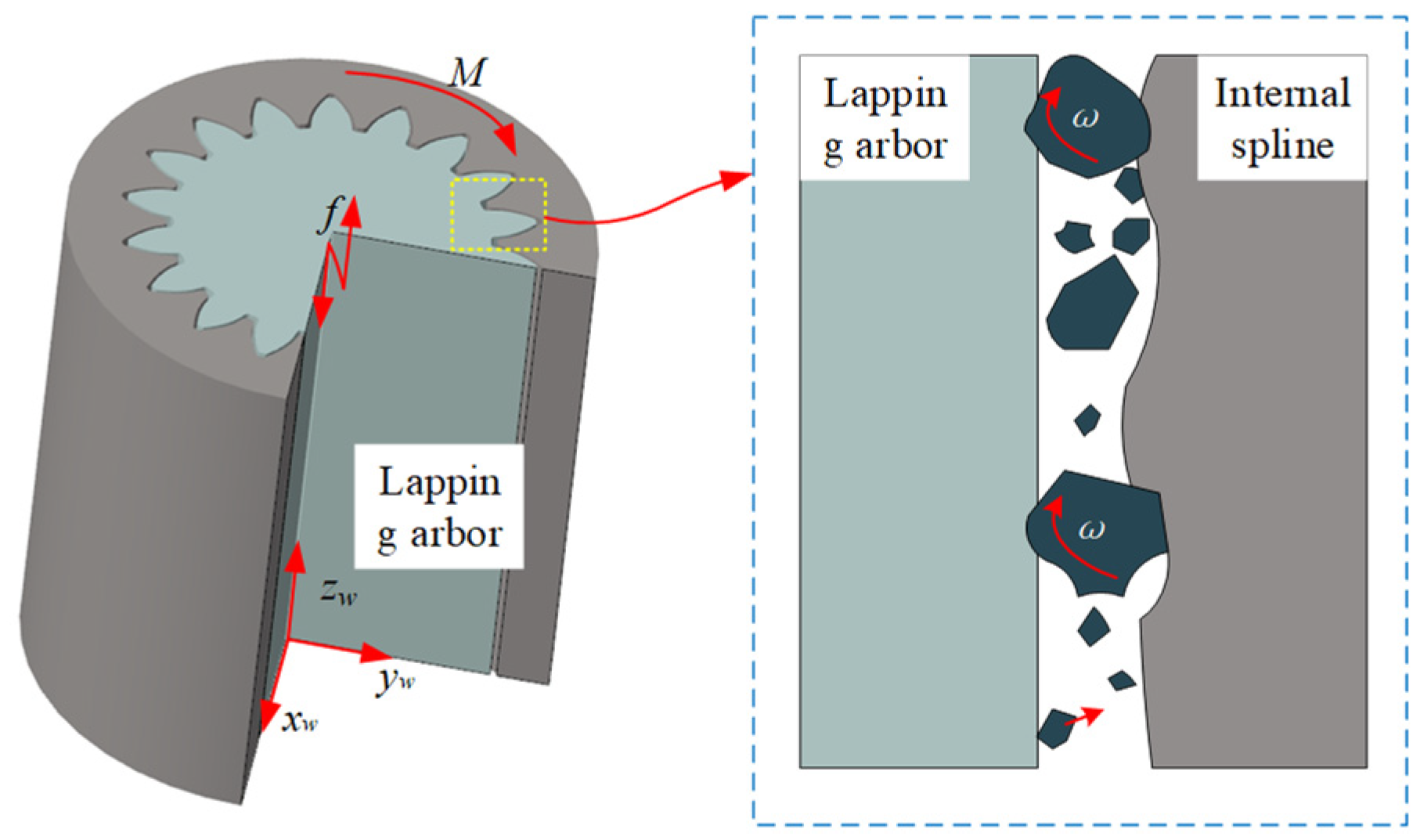
2.1. Effective Abrasive Particles in the Grinding Process
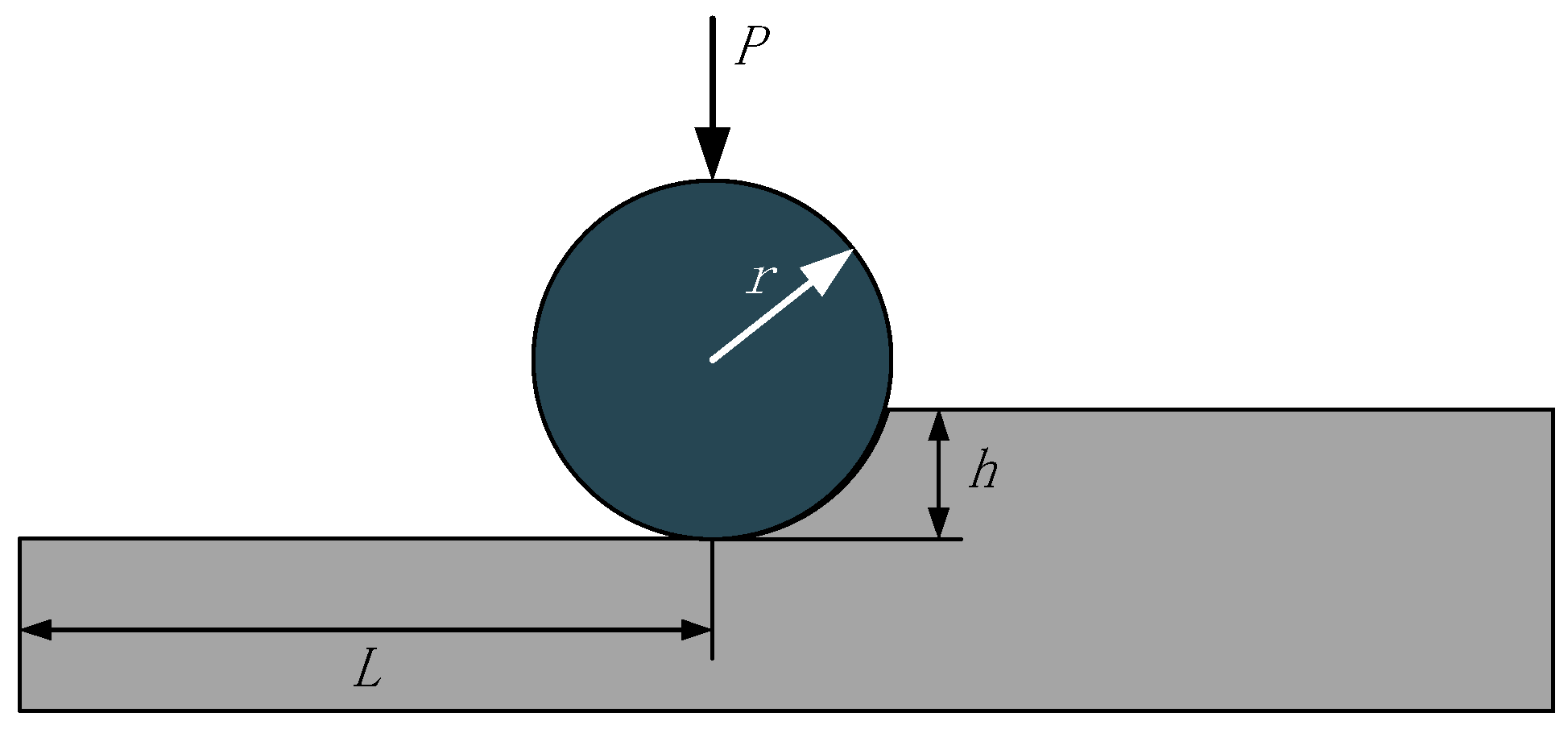
2.2. Material Removal Rate of Individual Abrasive Grains
3. Simulation and Analysis of Single-Grain Ultrasonic Vibratory Grinding
3.1. Ultrasonic Vibration Simulation Model of Internal Spline
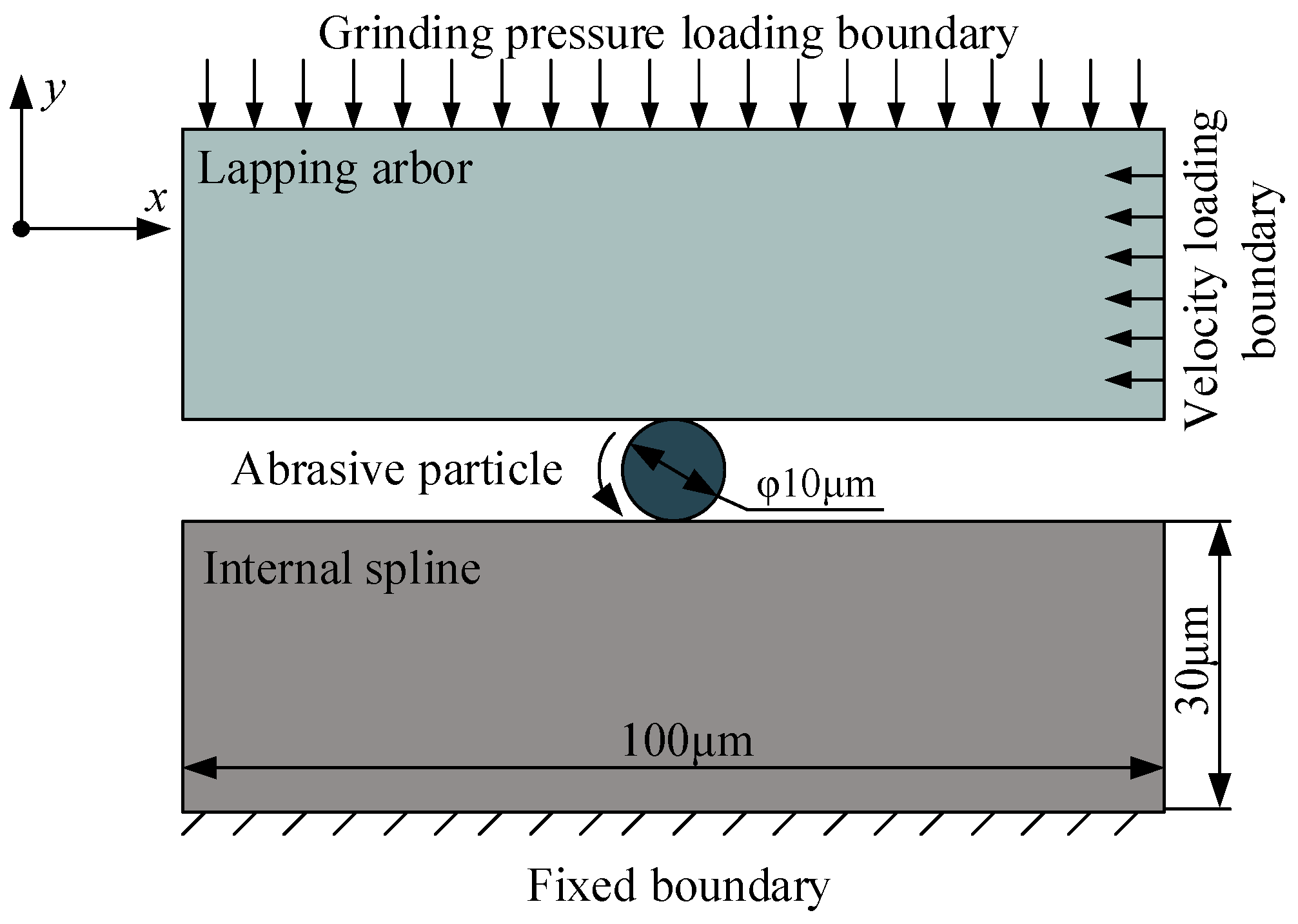
3.1.1. Two-Dimensional Model
3.1.2. Material Constitutive Parameters
3.2. Effect of Grinding Pressure
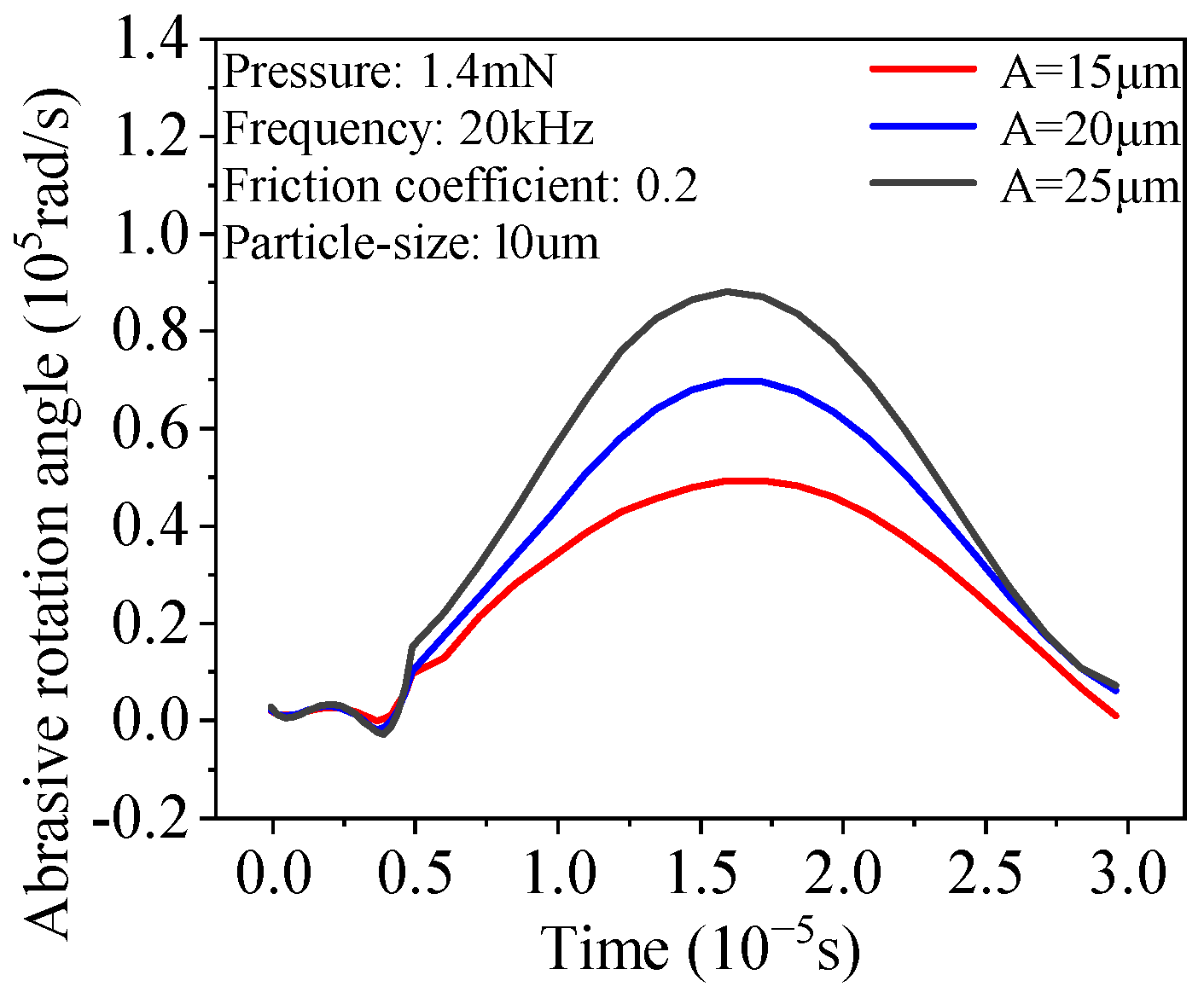
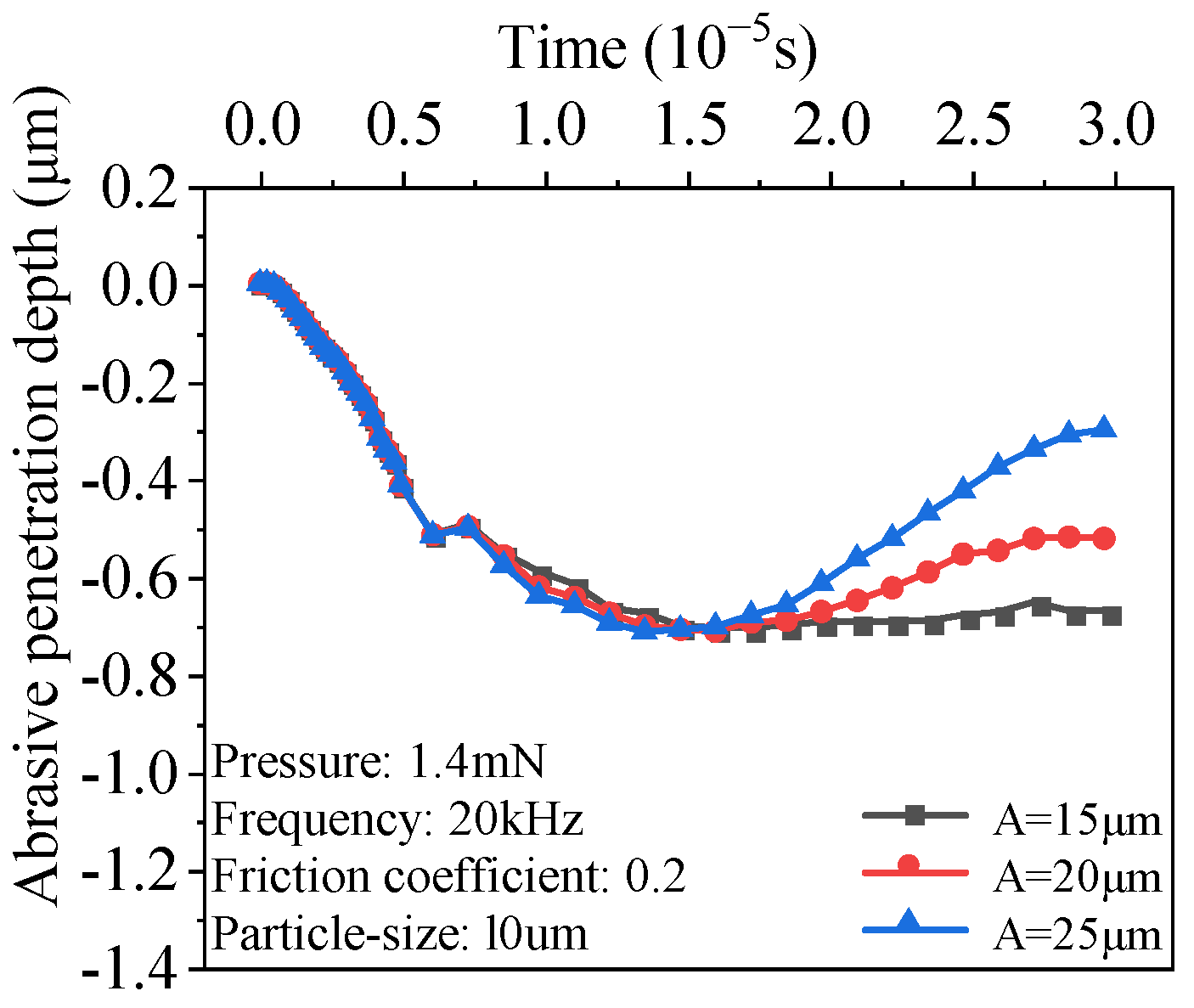
3.3. Effect of Ultrasonic Vibration Amplitude
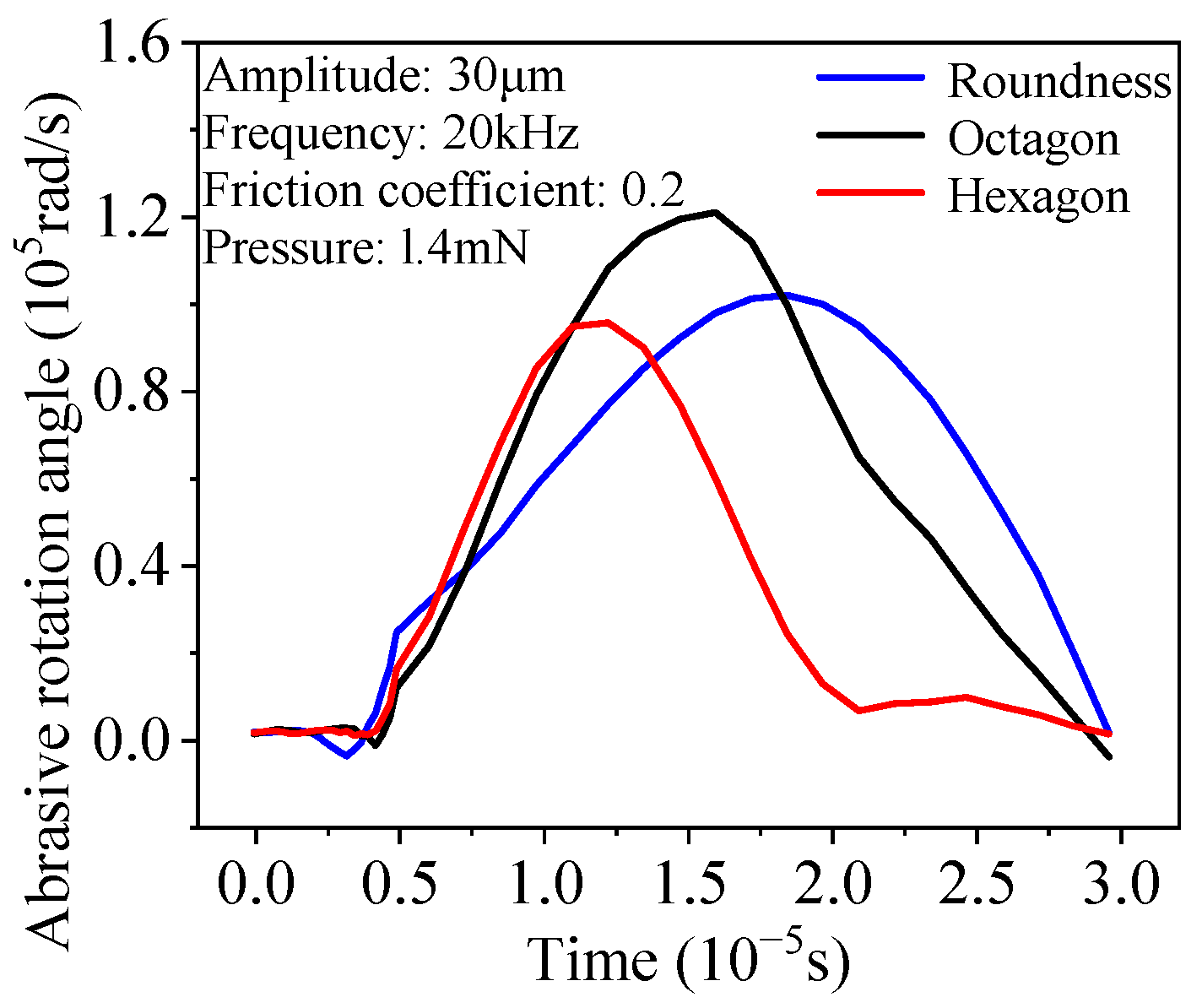
3.4. Influence of Abrasive Grain Shape
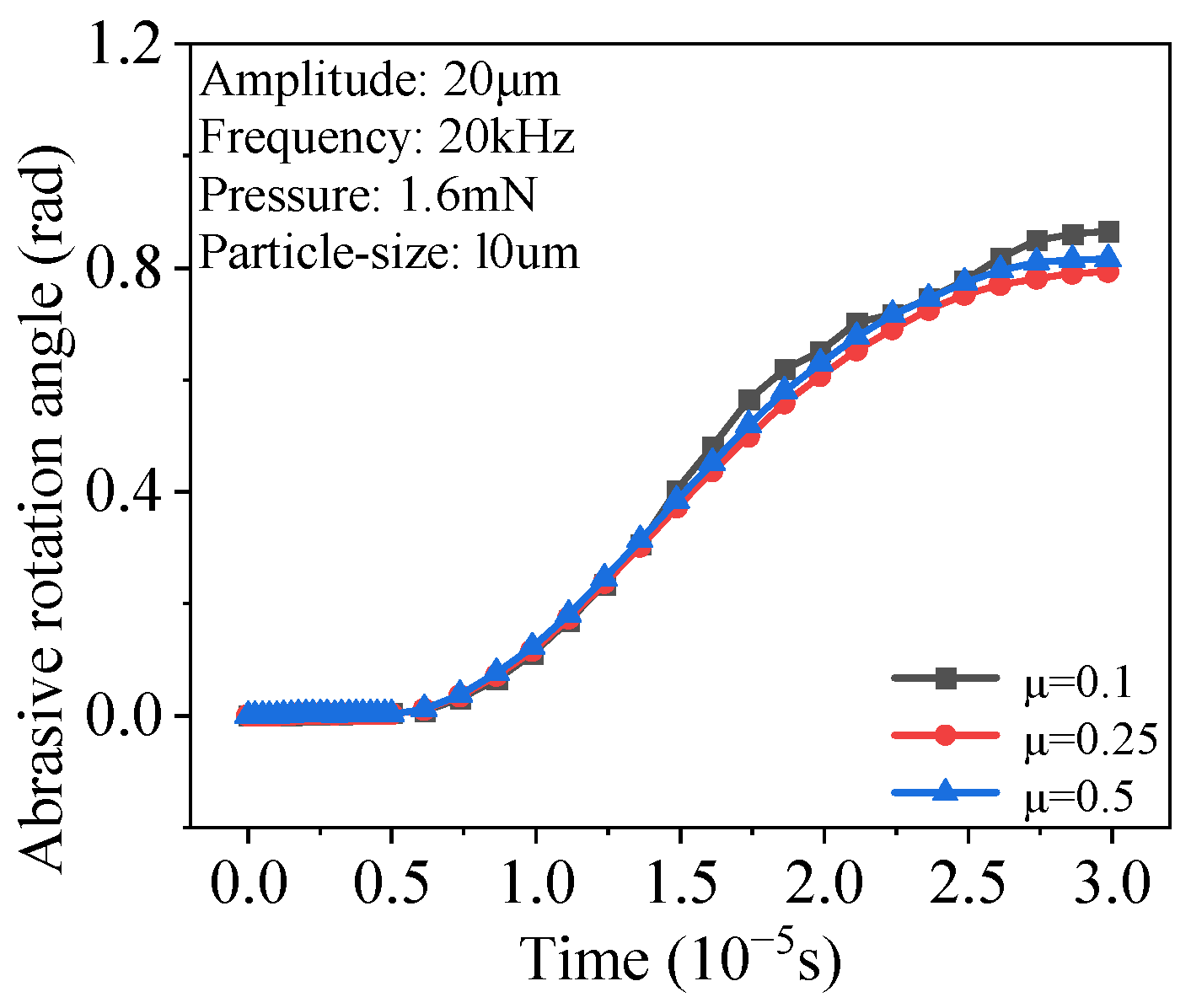
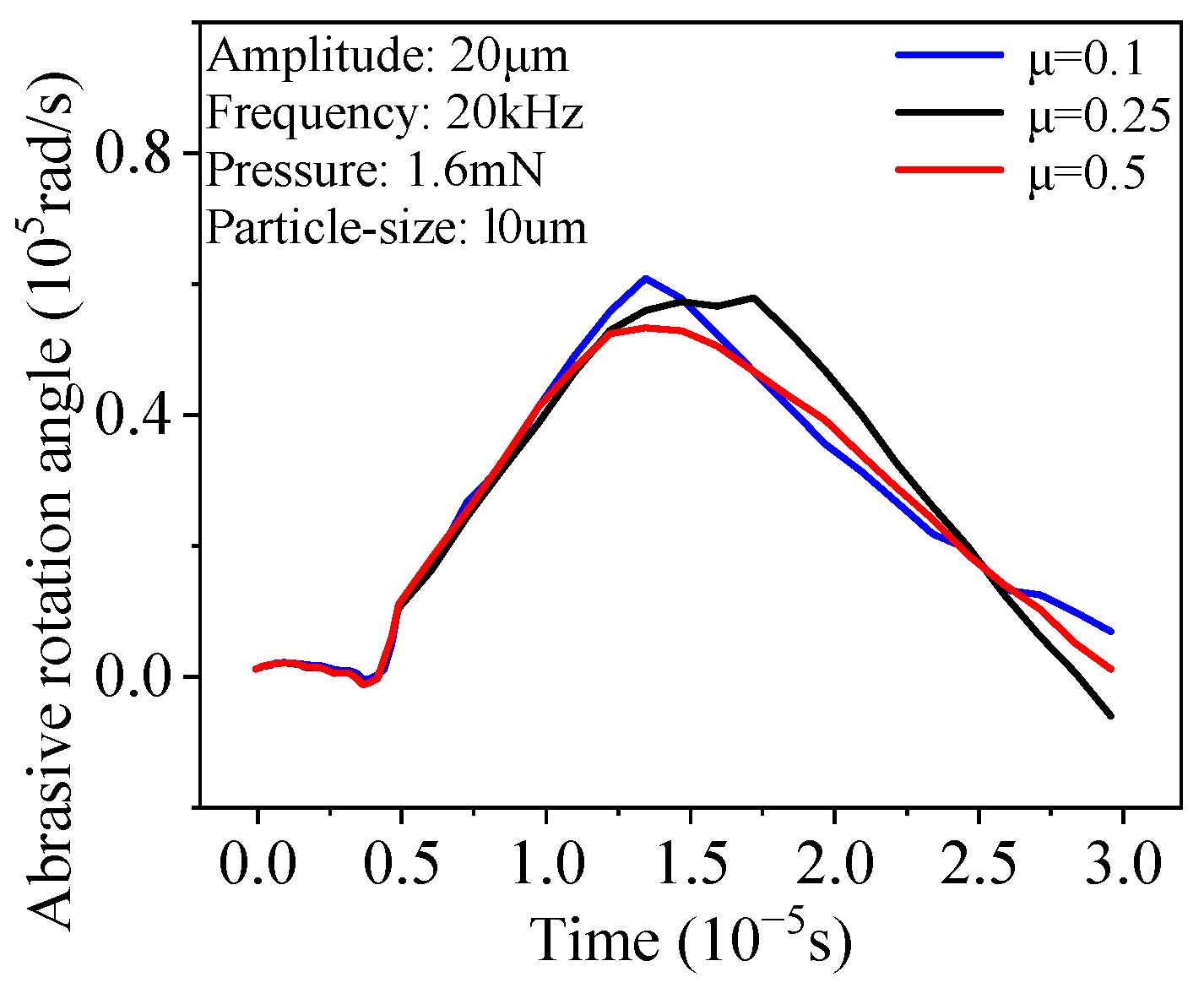
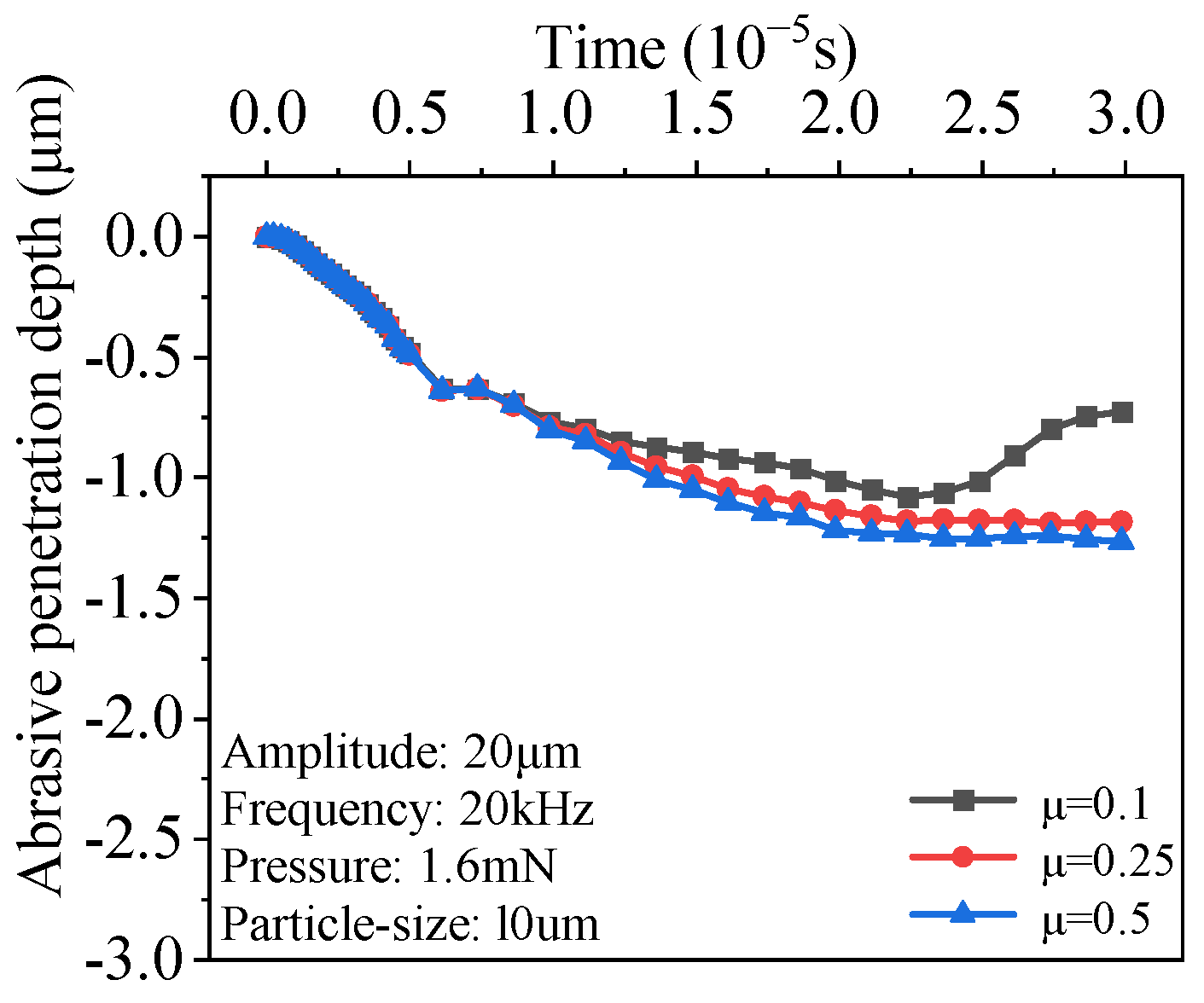
3.5. Effect of Coefficient of Friction
4. Experimental Setup and Discussion of Results
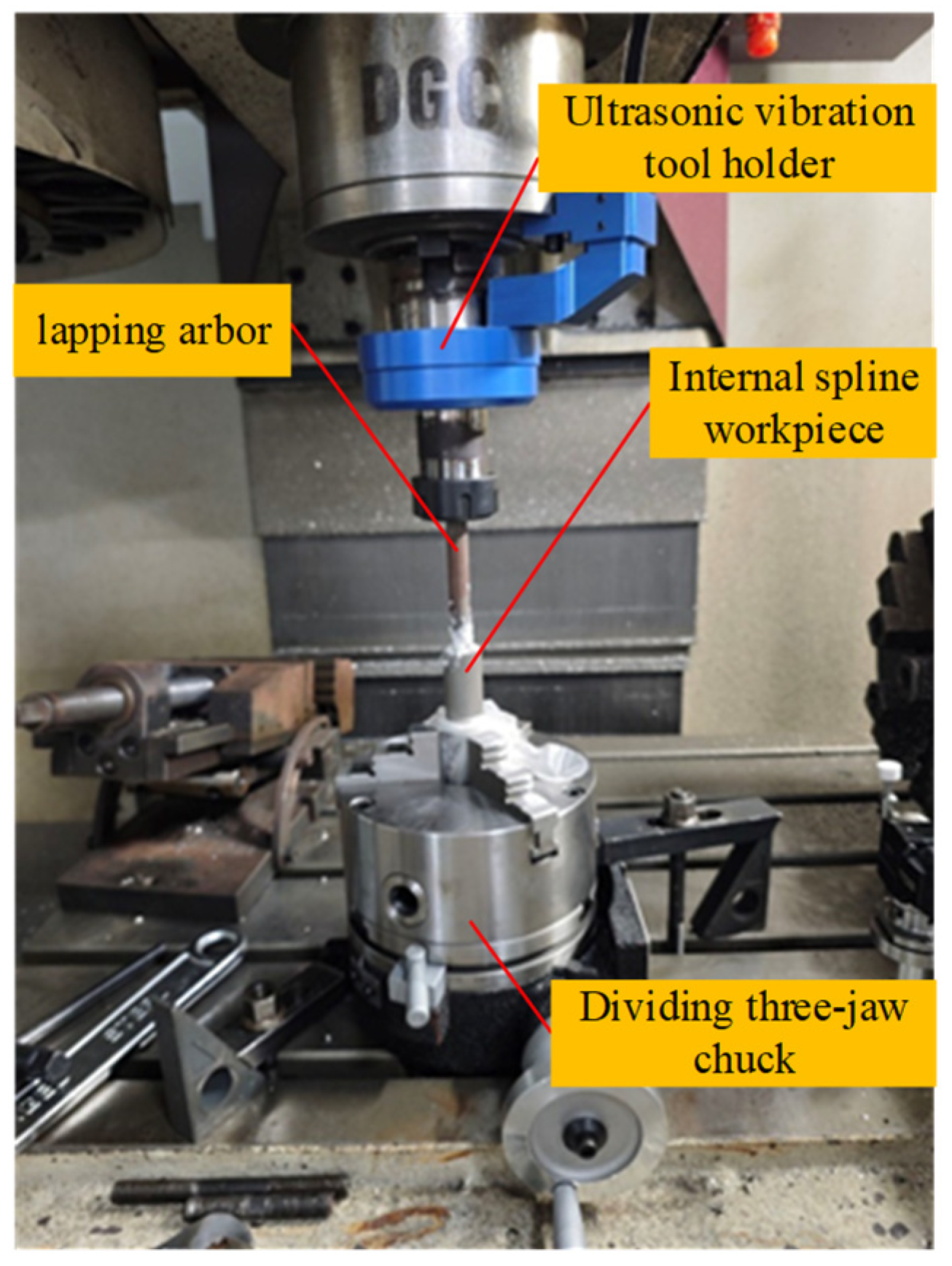
4.1. Experimental Planning
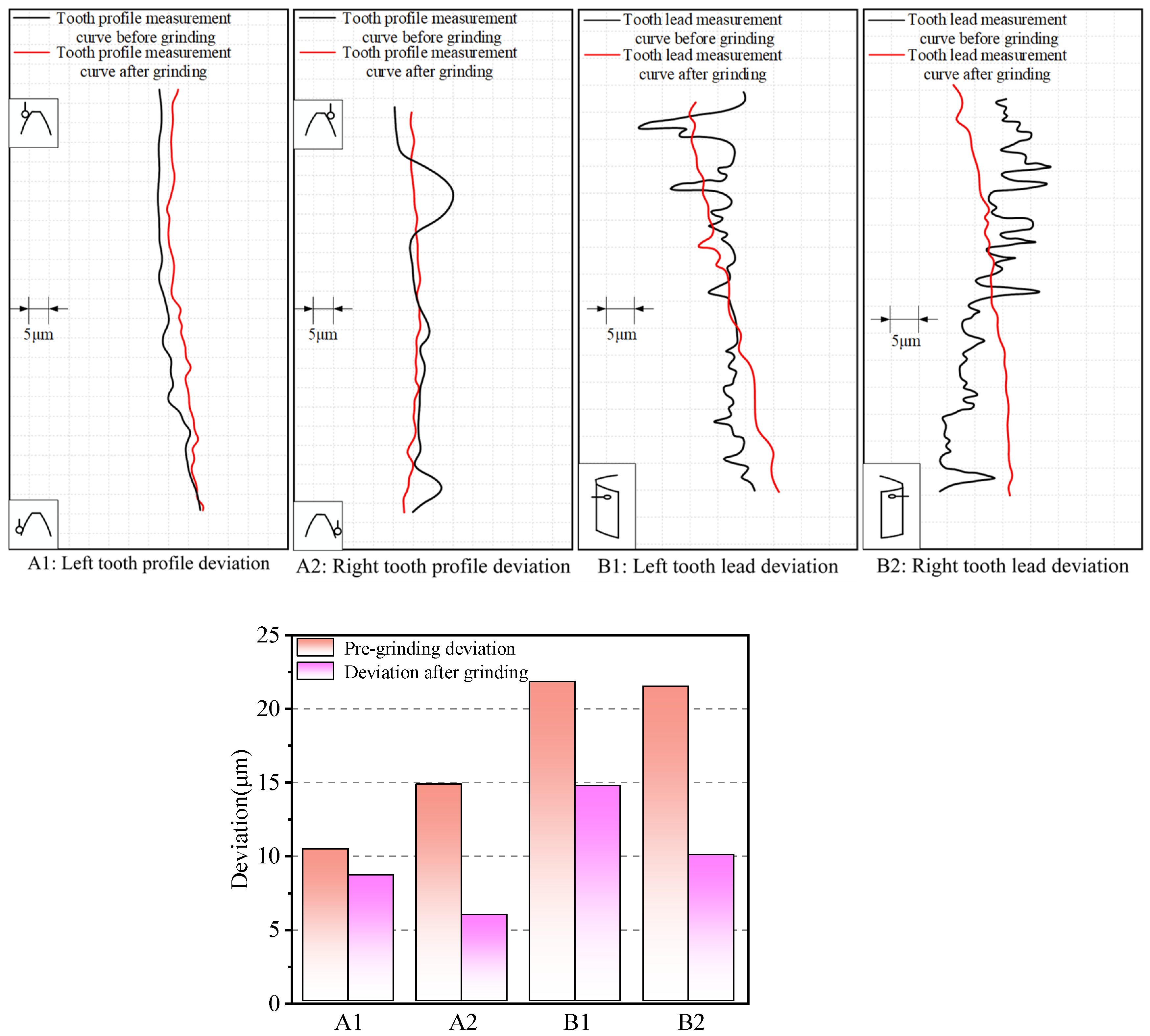
4.2. Analysis of Internal Spline Tooth Lead and Tooth Lead Tolerance Results
5. Conclusions
- (1)
- The established abrasive material removal simulation model was used to study the surface removal of internal spline materials during abrasive grinding under different grinding parameters by three-body grinding, which has certain guiding significance for actual machining.
- (2)
- The grinding pressure has the greatest influence among the simulation parameters. The greater the pressure, the faster the material removal on the internal spline surface and the higher the efficiency of the grinding process. The second most influential factor is the shape of the abrasive particles. In actual processing, the use of more angular abrasive particles is helpful to improve the grinding and shaping efficiency of internal splines.
- (3)
- In the experiment, by measuring the tooth profile deviation and tooth direction deviation after ultrasonic vibration grinding, the tooth profile deviation was reduced by 41.9%, and the tooth direction deviation was reduced by 44.1%, proving that ultrasonic vibration grinding can make the internal spline meet the requirements after heat treatment.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kim, J.-R.; Yoon, S.-H.; Jung, Y.-C.; Suh, C.-H.; Kwon, T.-H. A Study on the Thermal Deformation Simulation of Spur Gear According to the Heat Zones in Heat Treatment Process. J. Korean Soc. Manuf. Process Eng. 2020, 19, 60–66. [Google Scholar] [CrossRef]
- Li, X.; Ju, D.; Cao, J.; Wang, S.; Chen, Y.; He, F.; Li, H. Effect of Transformation Plasticity on Gear Distortion and Residual Stresses in Carburizing Quenching Simulation. Coatings 2021, 11, 1224. [Google Scholar] [CrossRef]
- Yang, J.; Zhang, Z.; Wang, S.; Wu, J. Tooth Surface Deviation Analysis for Continuous Generation Grinding of Internal Gears. Appl. Sci. 2024, 14, 6551. [Google Scholar] [CrossRef]
- Li, G.; Bie, W.; Zhao, B.; Chen, F.; Zhao, C.; Zhang, Y. Ultrasonic Assisted Machining of Gears with Enhanced Fatigue Resistance: A Comprehensive Review. Adv. Mech. Eng. 2022, 14, 168781322210828. [Google Scholar] [CrossRef]
- Yanase, Y.; Komori, M.; Ochi, M. Grinding of Internal Gears by Setting a Large Crossed-Axes Angle Using a Barrel-Shaped Grinding Wheel. Precis. Eng. 2018, 52, 384–391. [Google Scholar] [CrossRef]
- Li, H.; Chen, T.; Li, H.; Zhang, Y. Influence of Ultrasonic Vibration on Machining Quality of down/up Grinding in Ultrasonic Vibration Assisted Grinding of Silicon Carbide. Mach. Sci. Technol. 2023, 27, 1–19. [Google Scholar] [CrossRef]
- Maroju, N.K.; Kundhojalla, S.M.; Kesherwani, A.K.; Pasam, V.K. Finite Element Modeling of Multi-Grit Stochastic Grinding Tool for Vibration-Assisted Surface Grinding of Ti6Al4V. J. Mater. Eng. Perform. 2024. ahead of print. [Google Scholar] [CrossRef]
- Yang, Z.; Zhu, L.; Zhang, G.; Ni, C.; Lin, B. Review of Ultrasonic Vibration-Assisted Machining in Advanced Materials. Int. J. Mach. Tools Manuf. 2020, 156, 103594. [Google Scholar] [CrossRef]
- Kurniawan, R.; Kiswanto, G.; Ko, T.J. Surface Roughness of Two-Frequency Elliptical Vibration Texturing (TFEVT) Method for Micro-Dimple Pattern Process. Int. J. Mach. Tools Manuf. 2017, 116, 77–95. [Google Scholar] [CrossRef]
- Lotfi, M.; Amini, S.; Aghaei, M. 3D Analysis of Surface Topography in Vibratory Turning. Int. J. Adv. Manuf. Technol. 2018, 95, 197–204. [Google Scholar] [CrossRef]
- Wei, B.Y.; Deng, X.Z.; Fang, Z.D. Study on Ultrasonic-Assisted Lapping of Gears. Int. J. Mach. Tools Manuf. 2007, 47, 2051–2056. [Google Scholar] [CrossRef]
- Lv, M.; Ma, L.; Liang, G.X.; Zhang, Y. Research on Design and Manufacture of Ultrasonic-Vibration-Based Gear-Honing Device. Key Eng. Mater. 2007, 359–360, 436–440. [Google Scholar] [CrossRef]
- Mulik, R.S.; Pandey, P.M. Mechanism of Surface Finishing in Ultrasonic-Assisted Magnetic Abrasive Finishing Process. Mater. Manuf. Process. 2010, 25, 1418–1427. [Google Scholar] [CrossRef]
- Mulik, R.S.; Pandey, P.M. Ultrasonic Assisted Magnetic Abrasive Finishing of Hardened AISI 52100 Steel Using Unbonded SiC Abrasives. Int. J. Refract. Met. Hard Mater. 2011, 29, 68–77. [Google Scholar] [CrossRef]
- Mulik, R.S.; Pandey, P.M. Experimental Investigations and Modeling of Finishing Force and Torque in Ultrasonic Assisted Magnetic Abrasive Finishing. J. Manuf. Sci. Eng. 2012, 134, 051008. [Google Scholar] [CrossRef]
- Wang, Y.; Lin, B.; Wang, S.; Cao, X. Study on the System Matching of Ultrasonic Vibration Assisted Grinding for Hard and Brittle Materials Processing. Int. J. Mach. Tools Manuf. 2014, 77, 66–73. [Google Scholar] [CrossRef]
- Venkatesh, G.; Sharma, A.K.; Kumar, P. On Ultrasonic Assisted Abrasive Flow Finishing of Bevel Gears. Int. J. Mach. Tools Manuf. 2015, 89, 29–38. [Google Scholar] [CrossRef]
- Singh, H.; Jain, P.K. Study on Ultrasonic-Assisted Electrochemical Honing of Bevel Gears. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2018, 232, 705–712. [Google Scholar] [CrossRef]
- Bie, W.; Zhao, B.; Zhao, C.; Yin, L.; Guo, X. System Design and Experimental Research on the Tangential Ultrasonic Vibration-Assisted Grinding Gear. Int. J. Adv. Manuf. Technol. 2021, 116, 597–610. [Google Scholar] [CrossRef]
- Zhu, D.-R.; Yang, J.-J.; Deng, X.-Z.; Jiang, C.; Li, J.-B. Influence of Gear Parameters on Dynamic Characteristics of an Ultrasonic Vibration System. Trans. Can. Soc. Mech. Eng. 2018, 42, 252–267. [Google Scholar] [CrossRef]
- Han, G.; Zhao, J.; Wang, X. Research on Unbounded Abrasive Polishing Process with Assisted Ultrasonic Vibration of Workpiece. Int. J. Adv. Manuf. Technol. 2017, 88, 209–218. [Google Scholar] [CrossRef]
- Song, T.; Zhu, X.; Ye, L.; Zhao, J. Experimental Study on the Influence of Micro-Abrasive and Micro-Jet Impact on the Natural Frequency of Materials under Ultrasonic Cavitation. Machines 2022, 10, 891. [Google Scholar] [CrossRef]
- Yu, T.; Yang, X.; An, J.; Yu, X.; Zhao, J. Material Removal Mechanism of Two-Dimensional Ultrasonic Vibration Assisted Polishing Inconel718 Nickel-Based Alloy. Int. J. Adv. Manuf. Technol. 2018, 96, 657–667. [Google Scholar] [CrossRef]
- Pei, L.G.; Shi, G.F.; Chen, J.Z.; Yao, D.; Yang, Y.M.; Li, J.Y. Simulation and Experiment of Ultrasonic-Assisted Grinding Process for Natural Diamond. Diam. Abras. Eng. 2023, 43, 720–726. [Google Scholar] [CrossRef]
- Huang, H.L.; Li, S.H.; Wu, Y.H.; Sun, J.; Wang, P.F.; Zhao, Z.C. Surface Quality of Double-Sided Grinding Si3N4 Cylindrical Rollers. Diam. Abras. Eng. 2023, 43, 371–378. [Google Scholar] [CrossRef]
- Liu, S.; Zheng, K.; Li, H.; Cao, Z.; Zhao, S. Multi-Objective Optimization of Process Parameters in Longitudinal-Torsional Ultrasonic Vibration Face Grinding CFRP. Machines 2023, 11, 935. [Google Scholar] [CrossRef]
- Xie, G.; Yu, X.; Gao, Z.; Xue, W.; Zheng, L. The Modified Johnson-Cook Strain-Stress Constitutive Model According to the Deformation Behaviors of a Ni-W-Co-C Alloy. J. Mater. Res. Technol. 2022, 20, 1020–1027. [Google Scholar] [CrossRef]
- Ji, S.; Liu, L.; Zhao, J.; Sun, C. Finite Element Analysis and Simulation about Microgrinding of SiC. J. Nanomater. 2015, 2015, 575398. [Google Scholar] [CrossRef]
- Miller, L.; Zhou, K.; Tang, J.; Frame, L.D.; Hebert, R.J.; Narayan, L.R.; Alpay, S.P.; Merkouriou, A.; Kim, J. Thermomechanical Finite Element Simulation and Correlation Analysis for Orthogonal Cutting of Normalized AISI 9310 Steels. Int. J. Adv. Manuf. Technol. 2021, 114, 3337–3356. [Google Scholar] [CrossRef]
- Zhang, X.N. The Influence of Low Temperature Impact Toughness and the Fracture Behavior of Ferritic Ductile Iron. Ph.D. Thesis, Shenyang University of Technology, Shenyang, China, 2015. [Google Scholar]


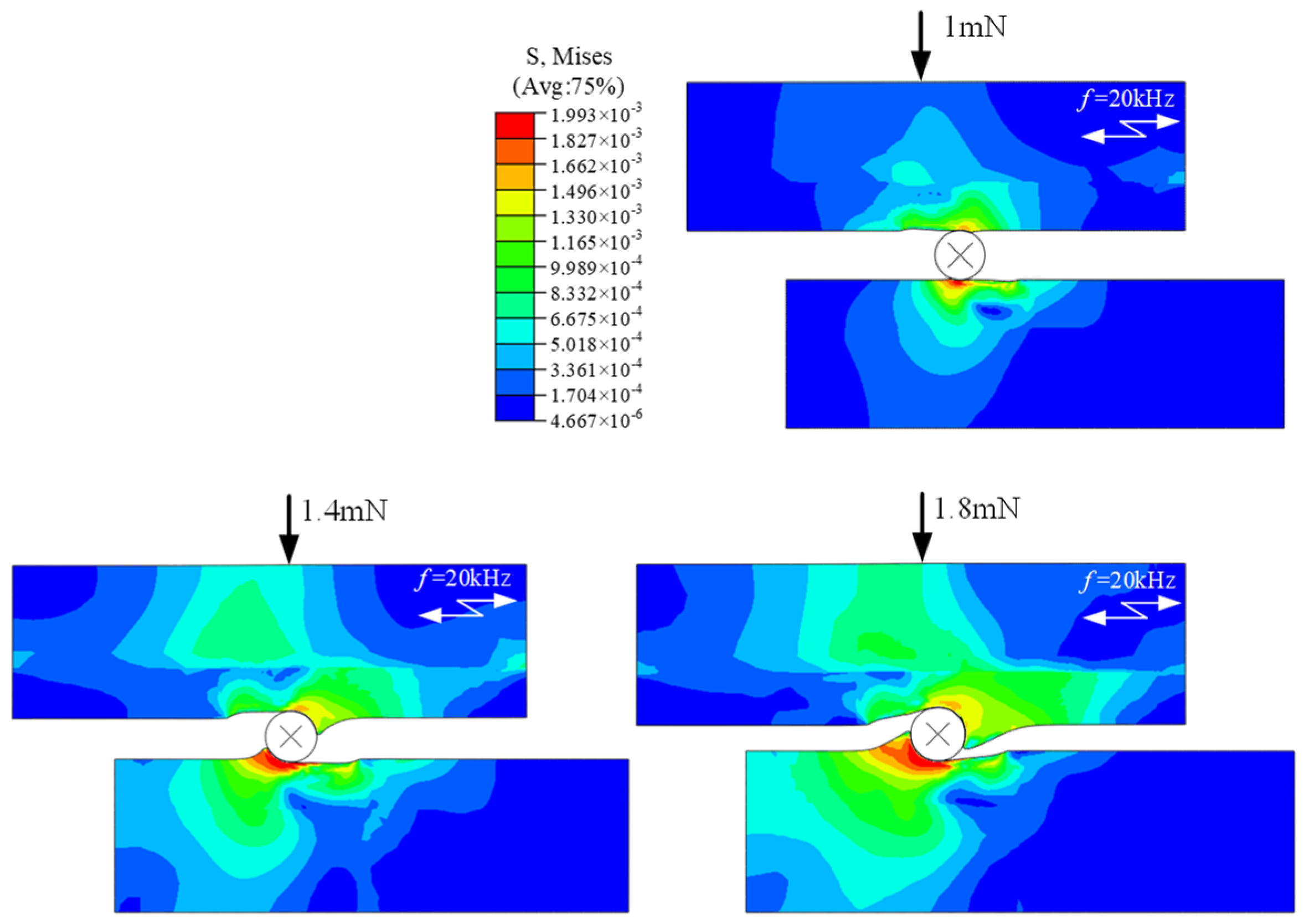
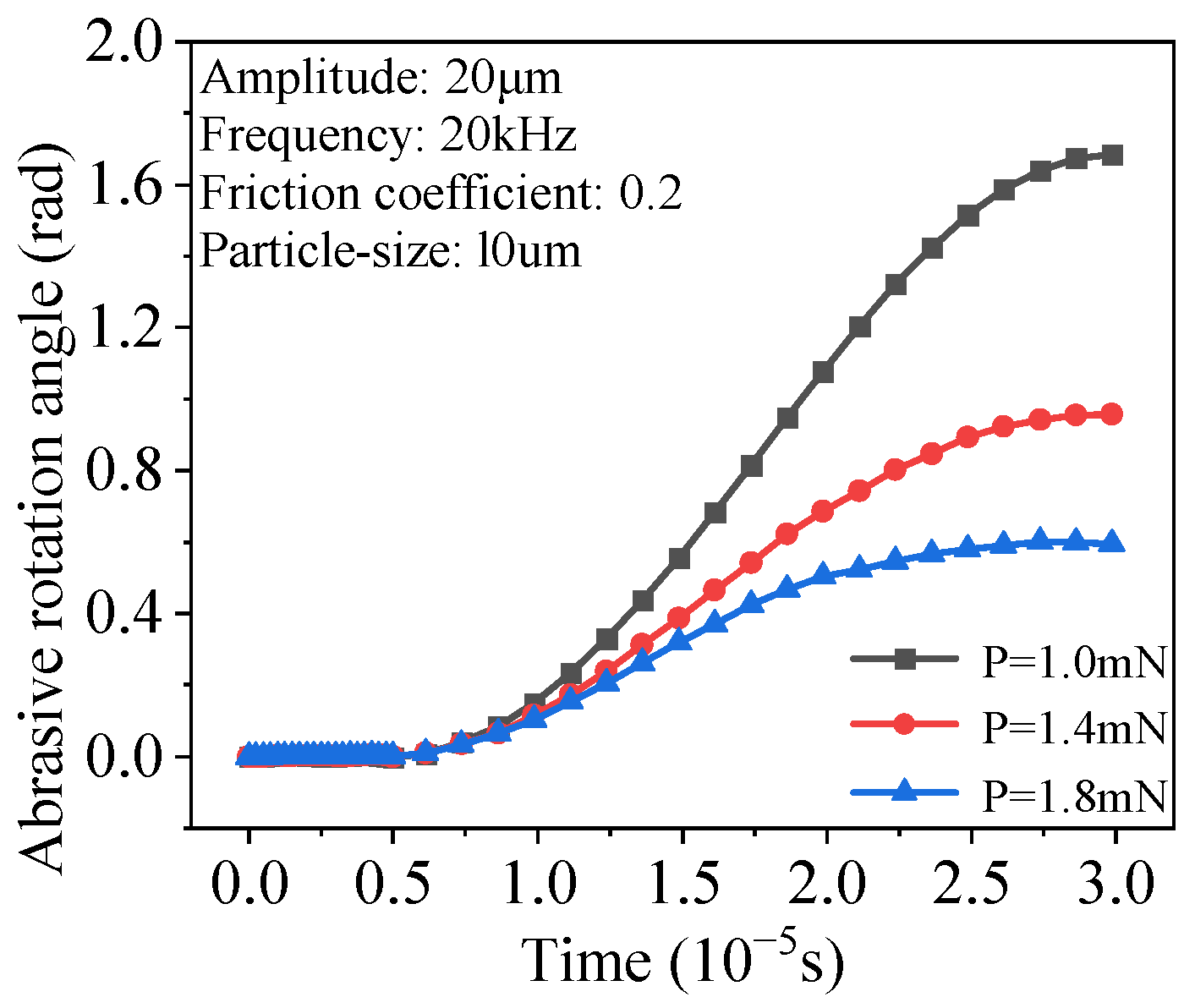
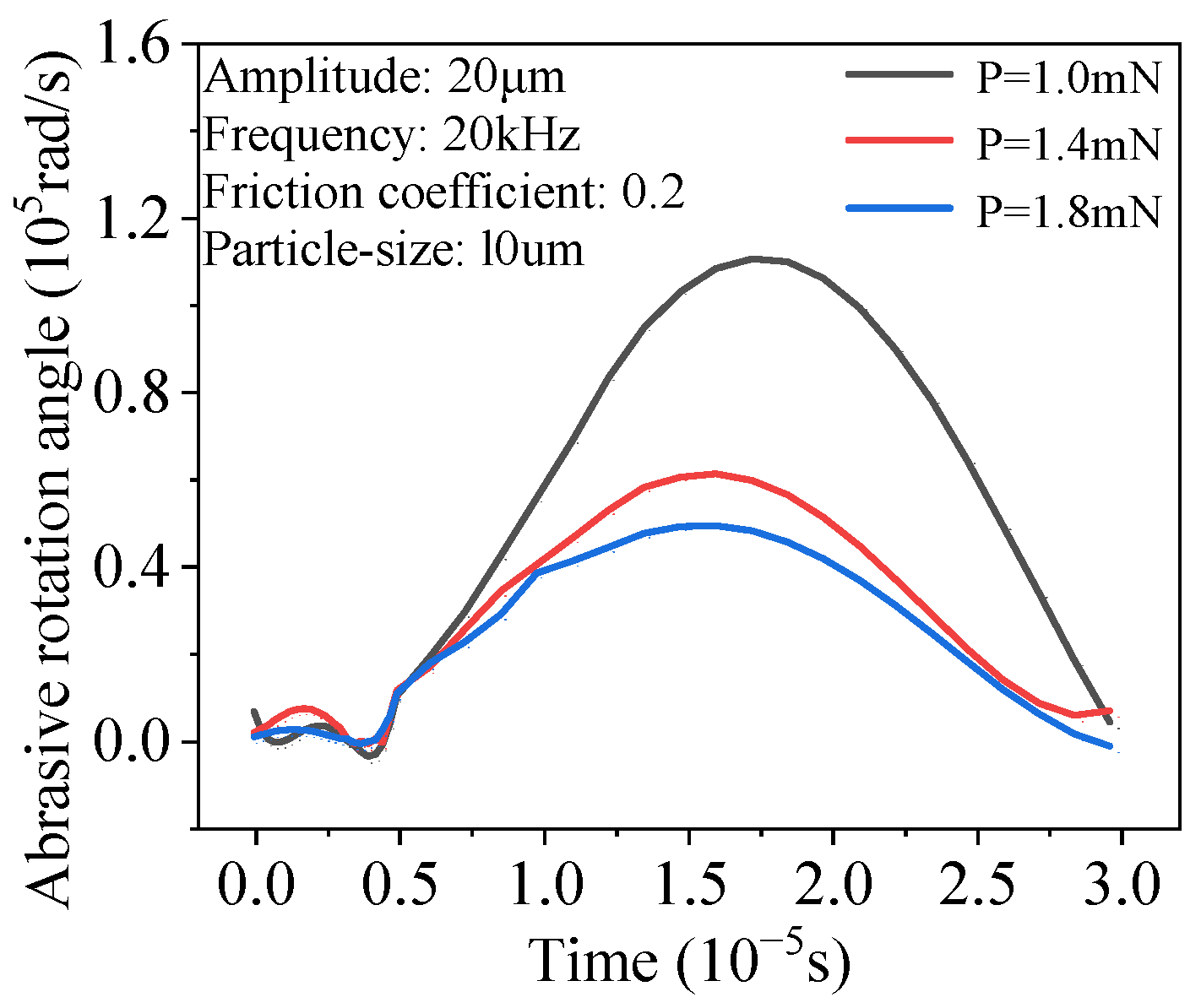
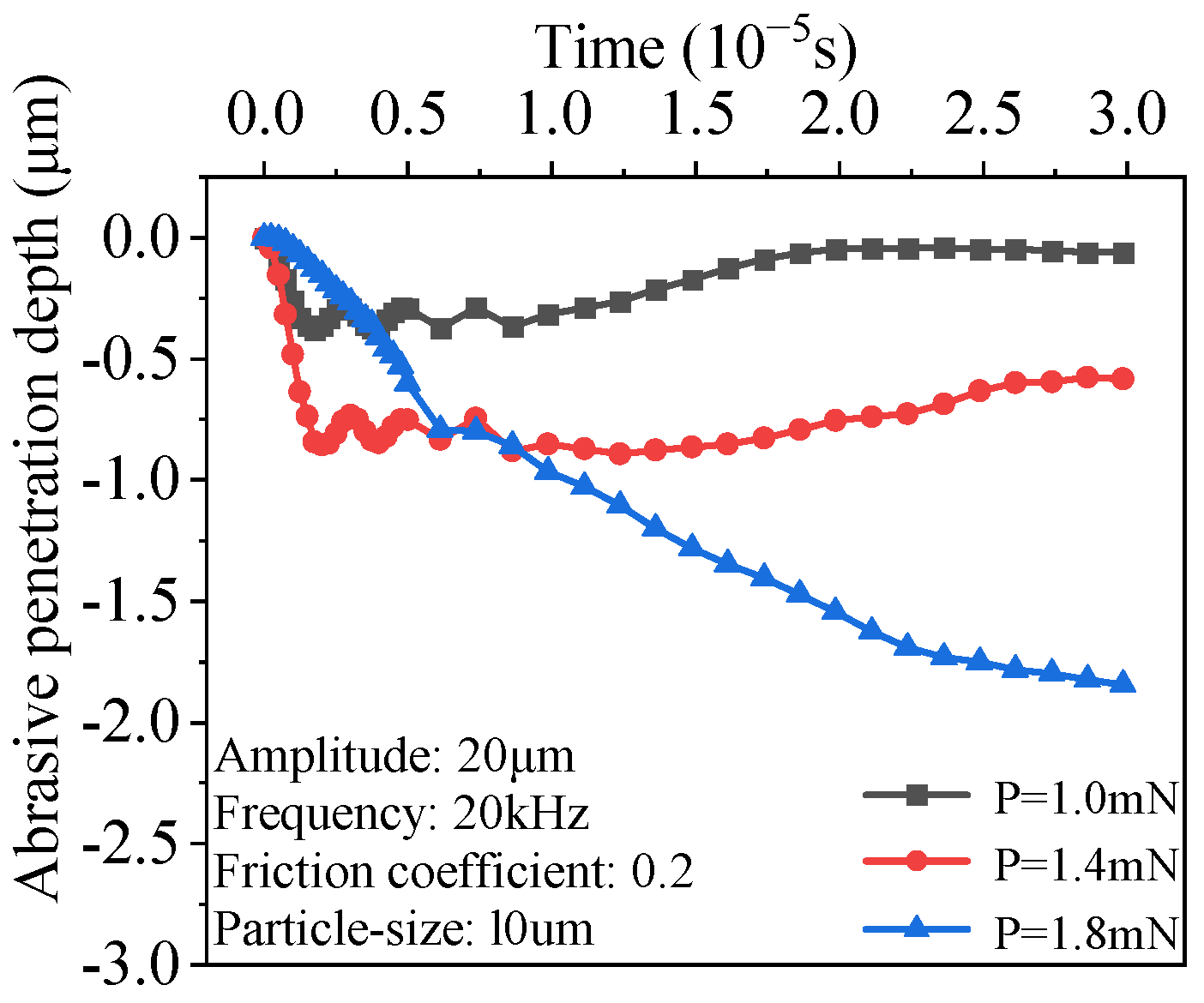



| Materials | Densities kg/m3 | Modulus of Elasticity E/MPa | Poisson’s Ratio |
|---|---|---|---|
| Abrasive particle (SiC) [28] | 3210 | 450,000 | 0.2 |
| Lapping arbor (QT400) [29] | 7010 | 161,000 | 0.274 |
| Internal spline (9310) [30] | 7800 | 210,000 | 0.3 |
| Materials | A/MPa | B/MPa | C | m | n |
|---|---|---|---|---|---|
| 9310 | 1000 | 800 | 0.014 | 1.03 | 0.25 |
| QT400 | 656 | 2286.5 | 0.025 | 0.3 | 0.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, Z.; Zhou, S.; Liu, Q.; Zhang, L.; Shen, B.; Han, J. Simulation and Experimental Study of Ultrasonic Vibratory Grinding of Internal Splines. Machines 2024, 12, 732. https://doi.org/10.3390/machines12100732
Zhao Z, Zhou S, Liu Q, Zhang L, Shen B, Han J. Simulation and Experimental Study of Ultrasonic Vibratory Grinding of Internal Splines. Machines. 2024; 12(10):732. https://doi.org/10.3390/machines12100732
Chicago/Turabian StyleZhao, Zemin, Shuangshuang Zhou, Qiang Liu, Long Zhang, Bin Shen, and Jiaming Han. 2024. "Simulation and Experimental Study of Ultrasonic Vibratory Grinding of Internal Splines" Machines 12, no. 10: 732. https://doi.org/10.3390/machines12100732
APA StyleZhao, Z., Zhou, S., Liu, Q., Zhang, L., Shen, B., & Han, J. (2024). Simulation and Experimental Study of Ultrasonic Vibratory Grinding of Internal Splines. Machines, 12(10), 732. https://doi.org/10.3390/machines12100732





