Optimization of the Synchronous Pressurization Process for the Elimination of Double-Layer Oxide Film Defects
Abstract
1. Introduction
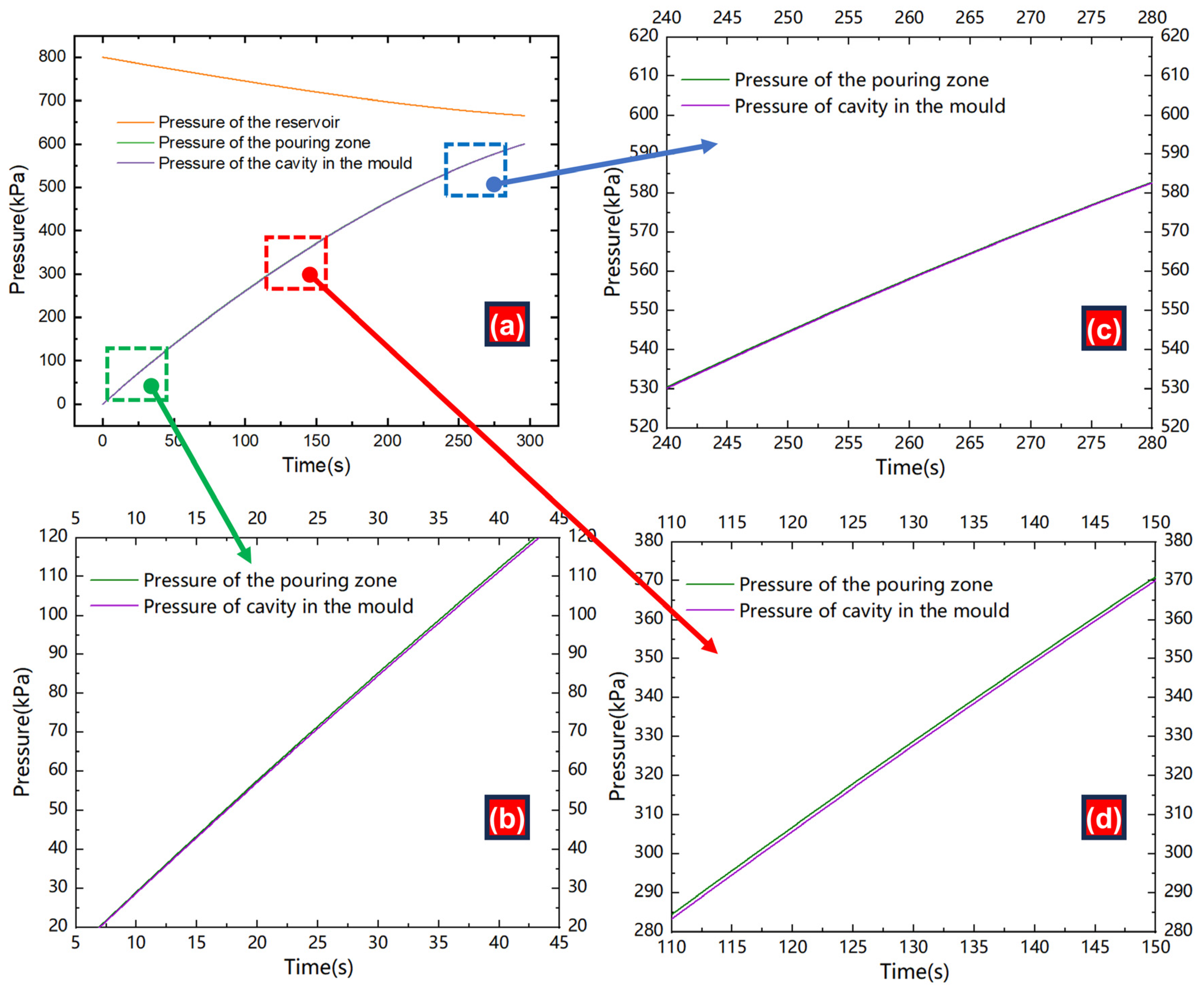
- In the synchronous pressurization stage, the control system connects the pouring zone and the melting zone and pressurizes them to 600 kPa, as shown in Figure 1d;
- In the stage of filling the riser with melt, the control system keeps the pressure of the melting zone unchanged and makes the melt rise in the transfer tube by reducing the pressure of the pouring zone, as shown in Figure 1e;
- In the stage of filling the cavity with melt, the control system continuously reduces the pressure of the pouring zone and makes the melt continue to rise to fill the cavity, as shown in Figure 1f,g;
- In the pressurization and pressure holding stage, a layer of solid metal is formed between the melt and the mold through the first pressurization and a short time of pressure holding, and then between the second pressurization and a long time of pressure holding, as shown in Figure 1h. This process can improve the surface quality of the casting while improving the melt feeding pressure.
2. Experimental Methods
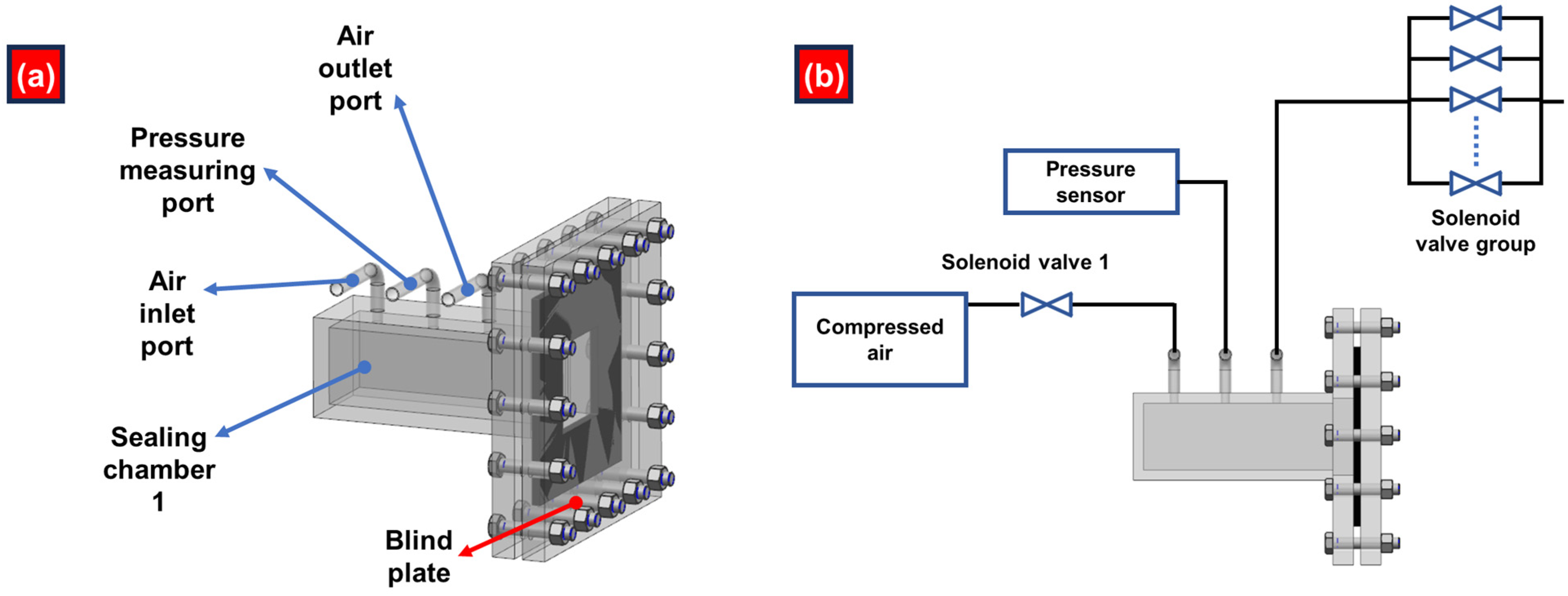
2.1. Sand Permeability Test Device and Method
- The solenoid valve 1 is open, the solenoid valve 2 is closed, the control pressure sensor value is 120 kPa, and the differential pressure sensor value is 0 kPa;
- The solenoid valve 1 is closed, the solenoid valve 2 is open; when the differential pressure sensor increases from 0 kPa to the peak value, start to record the real-time data of the differential pressure sensor, record the value every 0.5s, until the differential pressure sensor data drops to 0 kPa.
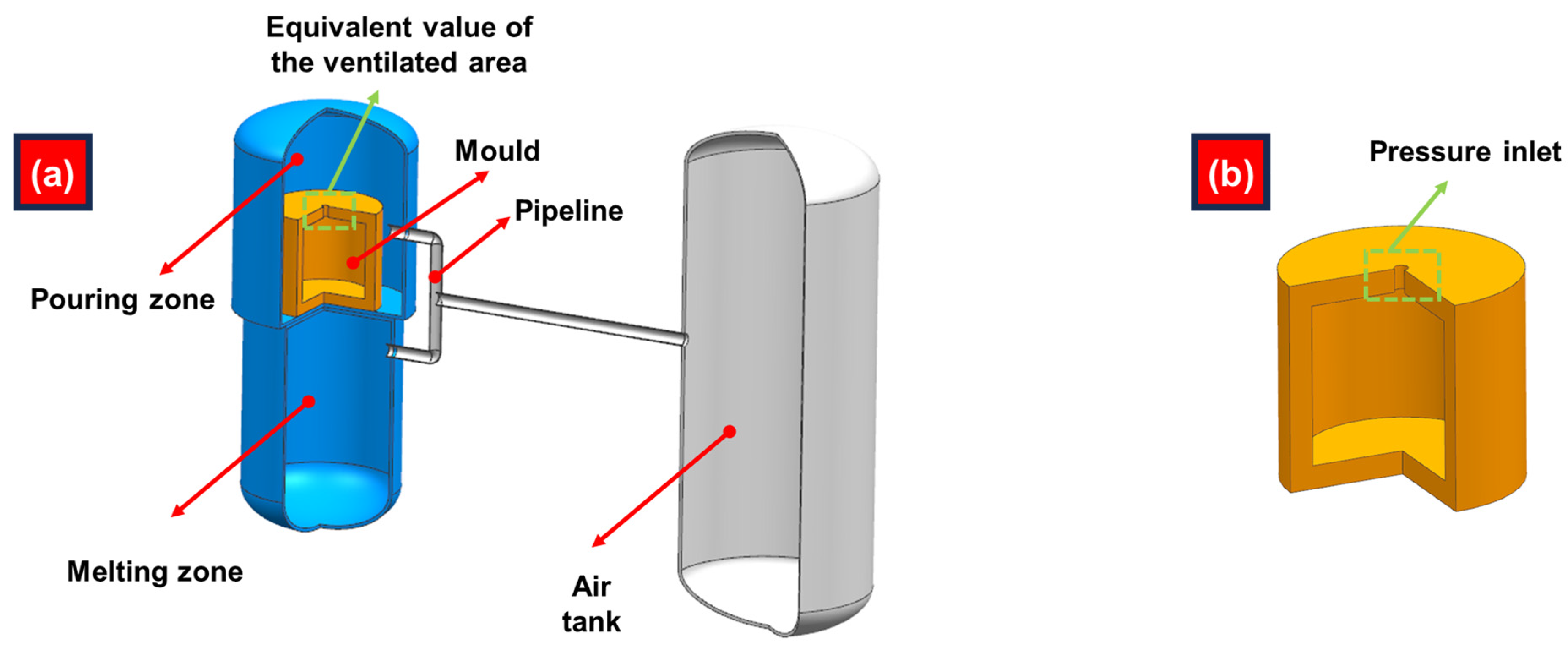
2.2. Device and Method for Equivalent Measurement of Sand Permeability and Effective Pore Area
- Raise the pressure in sealed chamber 1 to 100 kPa;
- The air permeability equation of the sand mold with the corresponding thickness is simulated and input into the control system as the target value, and the control solenoid valve group tracks the corresponding equation to exhaust the gas until the pressure is 0 kPa;
- Record the opening and closing date of the solenoid valve group and calculate the overall effective cross-sectional area; the air permeability of the corresponding thickness of the sand mold is equivalent to the effective pore area.
2.3. Preparation of Sand Mold
2.4. Mathematical Model of the Simulation Process
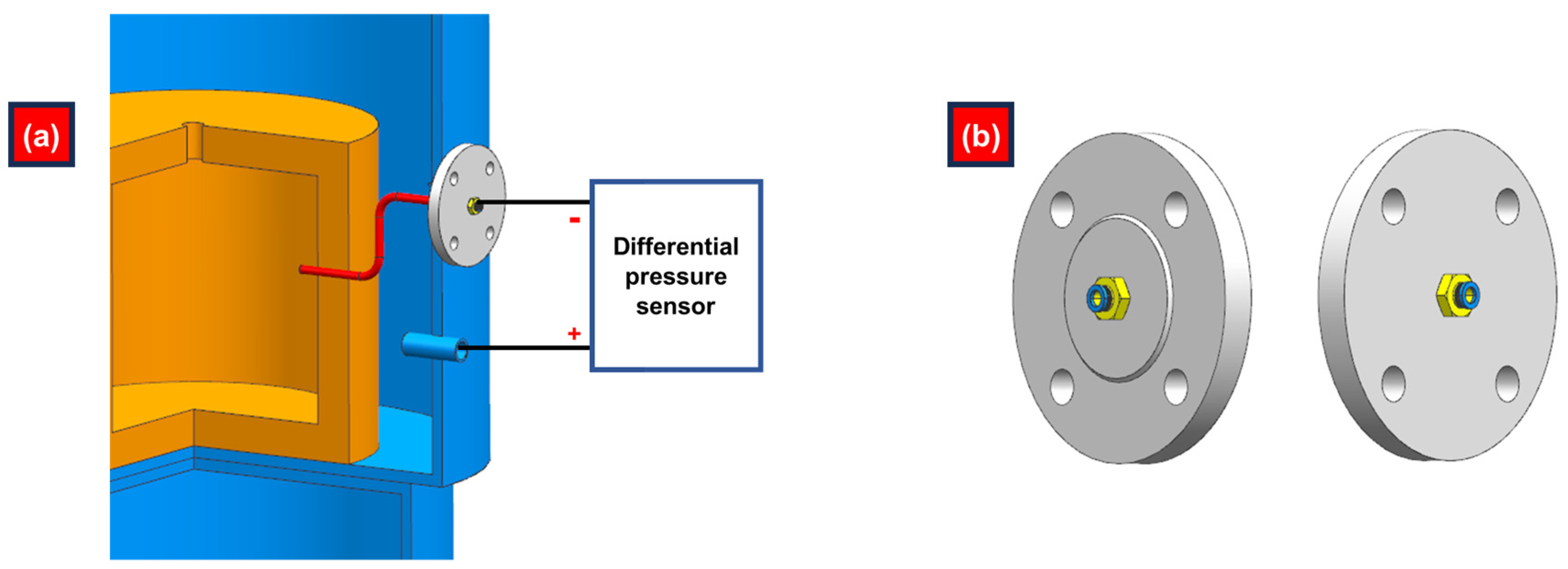
2.5. Method for Measuring the Pressure Difference between the Pouring Zone and the Mold
2.6. Physical Property Parameters of Magnesium Alloys Used in Experiments
3. Results and Discussion
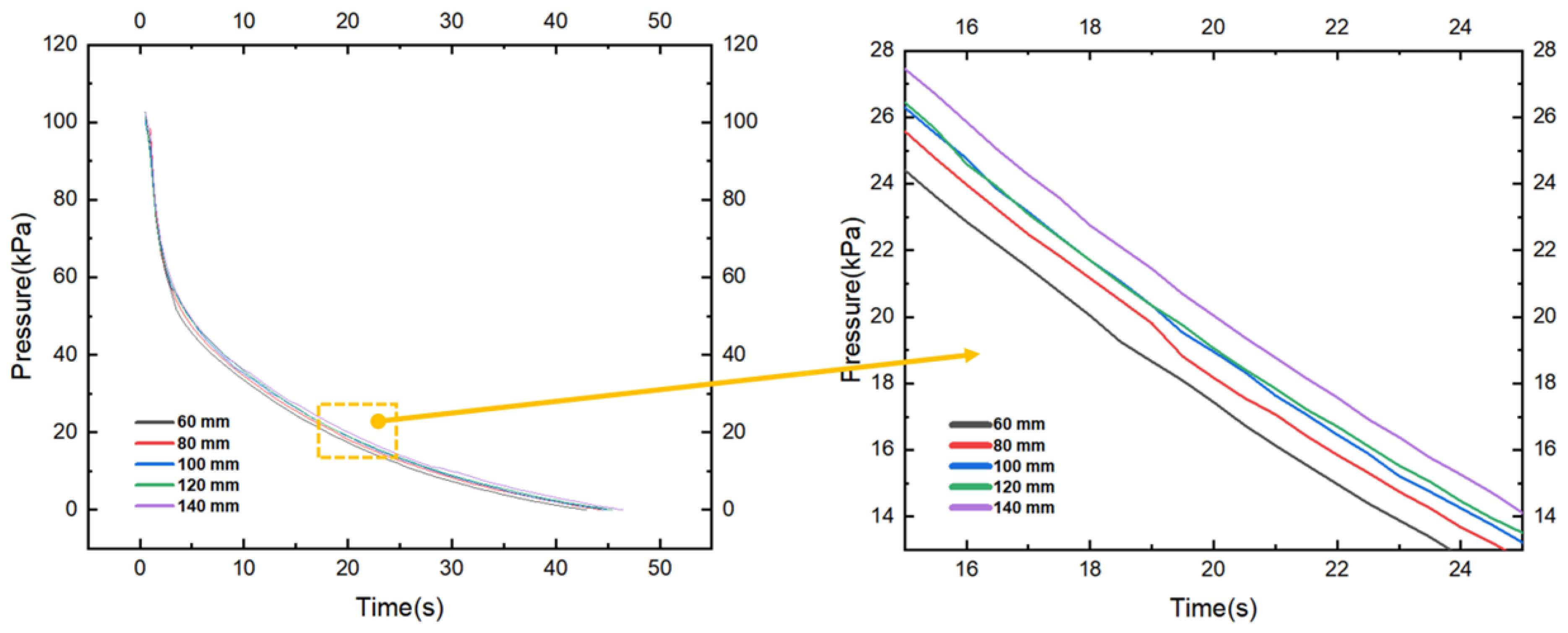
3.1. Permeability of Sand Mold
3.2. Equivalent Permeability of Sand Mold to Effective Pore Diameter
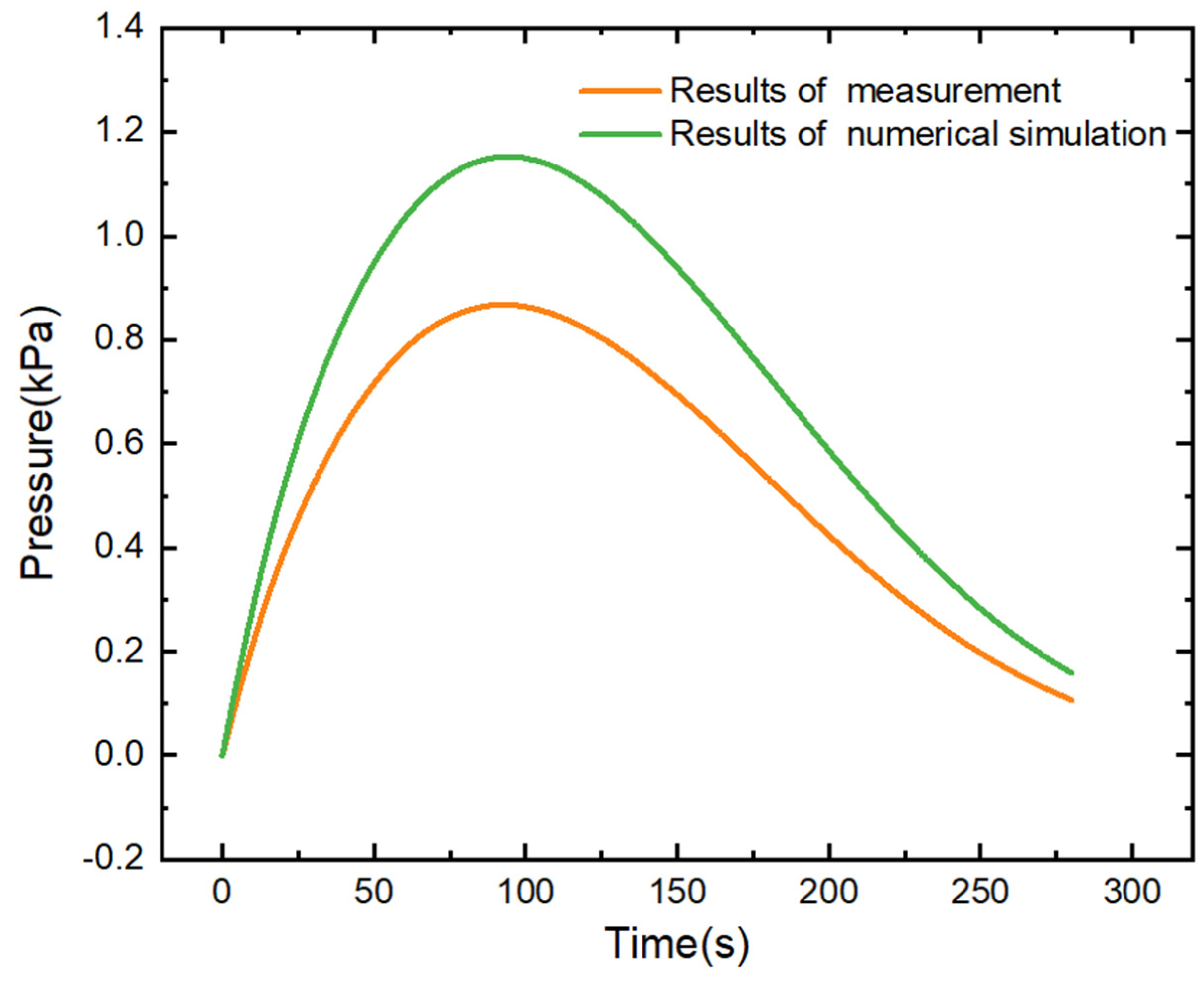
3.3. Influence of the Pressurization Process on Melt Surface Fluctuation during Synchronous Pressurization
4. Conclusions
- The numerical simulation results show that the metal liquid rises first and then falls back in the process of synchronous pressurization under the condition of considering the permeability of the sand mold;
- With the process curve of synchronous pressurization designed by exponential function. The metal liquid rises slowly in the process of synchronous pressurization until the end. The falling behavior of the liquid metal is eliminated;
- The optimized synchronous pressurization process reduces the height of the metal liquid in the riser tube. Taking magnesium alloy as an example, the height is reduced from 51.6 mm to 4.62 mm, which is about 90% lower.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Luo, A.A.; Sachdev, A.K.; Apelian, D. Alloy development and process innovations for light metals casting. J. Mater. Process. Technol. 2022, 306, 117606. [Google Scholar] [CrossRef]
- Ruff, G.; Prucha, T.E.; Barry, J.; Patterson, D. Pressure Counter Pressure Casting (PCPC) for Automotive Aluminum Structural Components. In SAE World Congress; SAE International: Warrendale, PA, USA, 2002. [Google Scholar]
- Bonollo, F.; Urban, J.; Bonatto, B.; Botter, M. Gravity and low pressure die casting of aluminium alloys: A technical and economical benchmark. In Proceedings of the Die Casting Conference of the Australian Die Casting Association, Columbus, OH, USA, 18–21 April 2006. [Google Scholar]
- Sun, J.Y.; Le, Q.C.; Fu, L.; Bai, J.; Tretter, J.; Herbold, K.; Huo, H.W. Gas entrainment behavior of aluminum alloy engine crankcases during the low-pressure-die-casting process. J. Mater. Process. Technol. 2019, 266, 274–282. [Google Scholar] [CrossRef]
- Katzarov, I.; Arsov, Y.; Stoyanov, P.; Zeuner, T.; Buehrig-Polaczek, A.; Sahm, P. Porosity formation in axi-symmetric castings produced by counter-pressure casting method. Int. J. Heat Mass Transf. 2001, 44, 111–119. [Google Scholar] [CrossRef]
- Mi, G.; Xin, P.; Zeng, S. Effect of solidification condition on secondary dendrite arm spacing of the A357 alloy under counter-pressure casting. J. Wuhan Univ. Technol.-Mater. Sci. Ed. 2009, 24, 119–122. [Google Scholar] [CrossRef]
- Yan, Q.; Yu, H.; Xu, Z.; Xiong, B.; Cai, C. Effect of holding pressure on the microstructure of vacuum counter-pressure casting aluminum alloy. J. Alloys Compd. 2010, 501, 352–357. [Google Scholar] [CrossRef]
- Zhensong, S.J.M.R. Development State and Prospect of Counter-pressure Casting Technology. Mater. Rev. 2004. [Google Scholar]
- Yan, Q.S.; Yu, H.; Lu, G.; Xiong, B.W.; Zou, X. Effect of crystallization pressure on secondary dendrite arm spacing of vacuum counter-pressure casting aluminum alloy. Sch. Aeronaut. Manuf. Eng. Nanchang Hangkong Univ. 2014, 24, 1194–1199. [Google Scholar]
- Runyoro, J.; Boutorabi, S.M.A.; Campbell, J. Critical gate velocities for film-forming casting alloys: A basis for process specification. Chukung 1992, 23, 67–77. [Google Scholar]
- Griffiths, W.D.; Lai, N.W. Double Oxide Film Defects in Cast Magnesium Alloy. Met. Mater. Trans. A 2007, 38, 190–196. [Google Scholar] [CrossRef]
- Zhao, X.Y.; Ning, Z.L.; Cao, F.Y.; Liu, S.G.; Huang, Y.J.; Liu, J.S.; Sun, J.F. Effect of Double Oxide Film Defects on Mechanical Properties of As-Cast C95800 Alloy. Acta Metall. Sin.-Engl. Lett. 2017, 30, 541–549. [Google Scholar] [CrossRef]
- Fox, S.; Campbell, J. Visualisation of oxide film defects during solidification of aluminium alloys. Scr. Mater. 2000, 43, 881–886. [Google Scholar] [CrossRef]
- Mi, J.; Harding, R.A.; Wickins, M.; Campbell, J. Entrained oxide films in TiAl castings. Intermetallics 2003, 11, 377–385. [Google Scholar] [CrossRef]
- Cais, J.; Weiss, V.; Svobodová, J. Microstructure and Mechanical Properties of Products of Alloy AL+3%Mg Made by Low-Pressure Casting. Metallurgist 2016, 60, 99–102. [Google Scholar] [CrossRef]
- Jiang, W.; Fan, Z.; Liao, D.; Dong, X.; Zhao, Z. A new shell casting process based on expendable pattern with vacuum and low-pressure casting for aluminum and magnesium alloys. Int. J. Adv. Manuf. Technol. 2010, 51, 25–34. [Google Scholar] [CrossRef]
- Bahreinian, F.; Boutorabi, S.M.A.; Campbell, J. Critical gate velocity for magnesium casting alloy (ZK51A). Int. J. Cast. Met. Res. 2006, 19, 45–51. [Google Scholar] [CrossRef]
- Fu, P.; Luo, A.A.; Jiang, H.; Peng, L.; Yu, Y.; Zhai, C.; Sachdev, A. Low-pressure die casting of magnesium alloy AM50: Response to process parameters. J. Mech. Work. Technol. 2008, 205, 224–234. [Google Scholar] [CrossRef]
- Zhang, Z.; Liu, J.; Chen, J.; Wen, F.; Jia, R.; Ma, Q. Optimization of residual stress in low-pressure casting of ZL205A alloys. J. Manuf. Process. 2023, 99, 338–350. [Google Scholar] [CrossRef]
- Kuo, J.H.; Hsu, F.L.; Hwang, W.S. Development of an interactive simulation system for the determination of the pressure-time relationship during the filling in a low pressure casting process. Sci. Technol. Adv. Mater. 2001, 2, 131. [Google Scholar] [CrossRef]
- Liu, B.L.; Kang, J.W.; Yang, X.; Zhang, B.; Bian, Y. Effects of hollow sand mold on the microstructure and mechanical properties of a low pressure aluminum alloy casting. J. Mater. Res. Technol.-JMRT 2024, 28, 4488–4497. [Google Scholar] [CrossRef]
- Bi, J.; Dong, G.J.; Zhang, L.; Ma, S.; Zhang, D.; Wang, J.; Li, W.; Xu, Z. Process Optimization of A356 Aluminum Alloy Wheel Hub Fabricated by Low-Pressure Die Casting with Simulation and Experimental Coupling Methods. J. Mater. Res. Technol. 2023, 24, 3118–3132. [Google Scholar]
- Duan, Z.Q.; Chen, W.P.; Pei, X.L.; Hou, H.; Zhao, Y.H. A multimodal data-driven design of low pressure die casting gating system for aluminum alloy cabin. J. Mater. Res. Technol.-JMRT 2023, 27, 2723–2736. [Google Scholar] [CrossRef]
- Tominaga, Y.; Stathopoulos, T. Numerical simulation of dispersion around an isolated cubic building: Comparison of various types of k-ε models. Atmosp. Environ. 2009, 43, 3200–3210. [Google Scholar] [CrossRef]
- Campbell, J. Casting, Complete Casting Handbook; Elsevier: Oxford, UK, 2011. [Google Scholar]



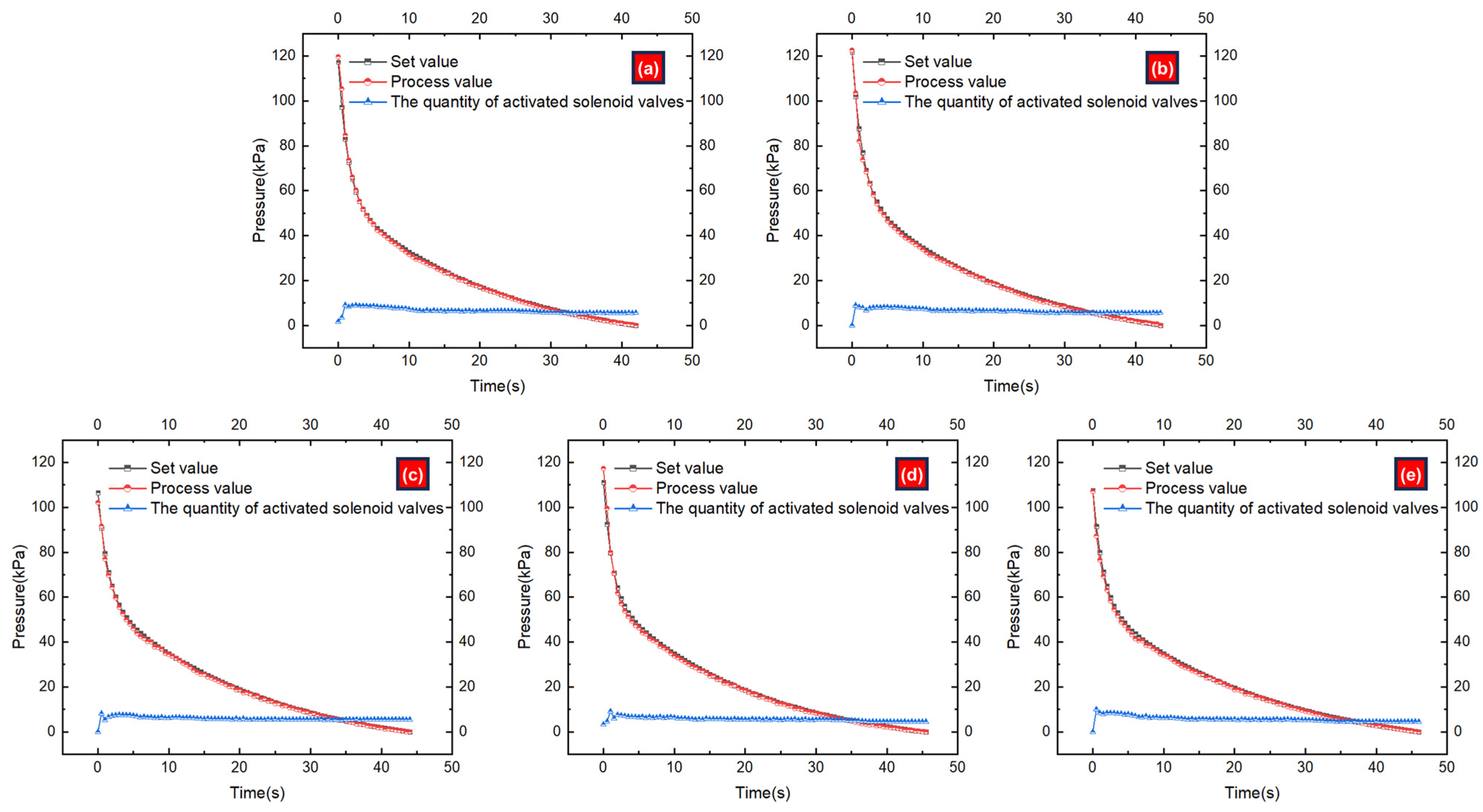


| Effective permeable section size (mm) | 100 × 100 |
| Sand mold thickness (mm) | 160 140 120 100 80 |
| Zones | Effective Volume (m3) | Initial Pressure (kPa) |
|---|---|---|
| Pouring zone | 8 | 0 |
| Melting zone | 4.6 | 0 |
| Mold | 0.2 | 0 |
| Air storage tank | 36 | 800 |
| Equivalent ventilation area | 0.0027 | - |
| Zn | Nd | Zr | Cu | Ni |
|---|---|---|---|---|
| 0.20 | 2.80 | 0.40 | 0.1 | 0.01 |
| Temperature (K) | Density (g/cm3) | Liquid Viscosity (mPa·s) | Surface Tension (mN/m) |
|---|---|---|---|
| 1033.15 | 1.59902 | 1.08833 | 521.1553 |
| 1028.15 | 1.60033 | 1.09972 | 522.904 |
| 1023.15 | 1.60165 | 1.11133 | 524.6526 |
| 1018.15 | 1.60296 | 1.12319 | 526.4012 |
| 1013.15 | 1.60427 | 1.13529 | 528.1499 |
| 1008.15 | 1.60557 | 1.14764 | 529.8985 |
| 1003.15 | 1.60688 | 1.16026 | 531.6471 |
| 998.15 | 1.60818 | 1.17314 | 533.3958 |
| 993.15 | 1.60947 | 1.18629 | 535.1444 |
| 988.15 | 1.61077 | 1.19973 | 536.893 |
| 983.15 | 1.61206 | 1.21346 | 538.6416 |
| 978.15 | 1.61334 | 1.22749 | 540.3903 |
| 973.15 | 1.61463 | 1.24182 | 542.1389 |
| 968.15 | 1.61591 | 1.25648 | 543.8875 |
| 963.15 | 1.61719 | 1.27146 | 545.6361 |
| Thickness of Sand Mold (mm) | A1 | t1 | A2 | t2 | y0 |
|---|---|---|---|---|---|
| 80 | 69.88 ± 1.57 | 1.36 ± 0.05 | 67.75 ± 0.59 | 22.28 ± 0.89 | −10.15 ± 0.99 |
| 100 | 66.72 ± 2.15 | 1.45 ± 0.08 | 69.30 ± 0.81 | 22.29 ± 1.22 | −9.52 ± 1.34 |
| 120 | 62.54 ± 1.30 | 1.40 ± 0.05 | 71.01 ± 0.48 | 22.89 ± 0.70 | −10.26 ± 0.79 |
| 140 | 64.10 ± 0.82 | 1.21 ± 0.03 | 69.75 ± 0.24 | 20.78 ± 0.31 | −7.73 ± 0.35 |
| 160 | 65.44 ± 1.36 | 1.46 ± 0.06 | 68.87 ± 0.52 | 24.04 ± 0.83 | −9.92 ± 0.87 |
| Thickness of Sand Mold (mm) | Average of the Open Area of the Solenoid Valve (mm2) |
|---|---|
| 80 | 15.57 |
| 100 | 14.78 |
| 120 | 14.13 |
| 140 | 13.68 |
| 160 | 13.32 |
| Parameters | Value |
|---|---|
| a | 0.1 |
| b | 1.53 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiu, Z.; Zhang, C.; Jin, Z.; Cao, G.; Zhang, L.; Jiang, S.; Cao, F.; Shen, H.; Zhao, X.; Song, H.; et al. Optimization of the Synchronous Pressurization Process for the Elimination of Double-Layer Oxide Film Defects. Machines 2024, 12, 702. https://doi.org/10.3390/machines12100702
Qiu Z, Zhang C, Jin Z, Cao G, Zhang L, Jiang S, Cao F, Shen H, Zhao X, Song H, et al. Optimization of the Synchronous Pressurization Process for the Elimination of Double-Layer Oxide Film Defects. Machines. 2024; 12(10):702. https://doi.org/10.3390/machines12100702
Chicago/Turabian StyleQiu, Ziao, Chaojun Zhang, Zhishuai Jin, Guanyu Cao, Lunyong Zhang, Sida Jiang, Fuyang Cao, Hongxian Shen, Xinyi Zhao, Heqian Song, and et al. 2024. "Optimization of the Synchronous Pressurization Process for the Elimination of Double-Layer Oxide Film Defects" Machines 12, no. 10: 702. https://doi.org/10.3390/machines12100702
APA StyleQiu, Z., Zhang, C., Jin, Z., Cao, G., Zhang, L., Jiang, S., Cao, F., Shen, H., Zhao, X., Song, H., & Sun, J. (2024). Optimization of the Synchronous Pressurization Process for the Elimination of Double-Layer Oxide Film Defects. Machines, 12(10), 702. https://doi.org/10.3390/machines12100702






