Correction of Shape Error at Cut-In and Cut-Out Points in Abrasive Waterjet Cutting of Carbon Fiber Reinforced Polymer (CFRP)
Abstract
:1. Introduction
2. Materials and Methods
2.1. Design of Experiments
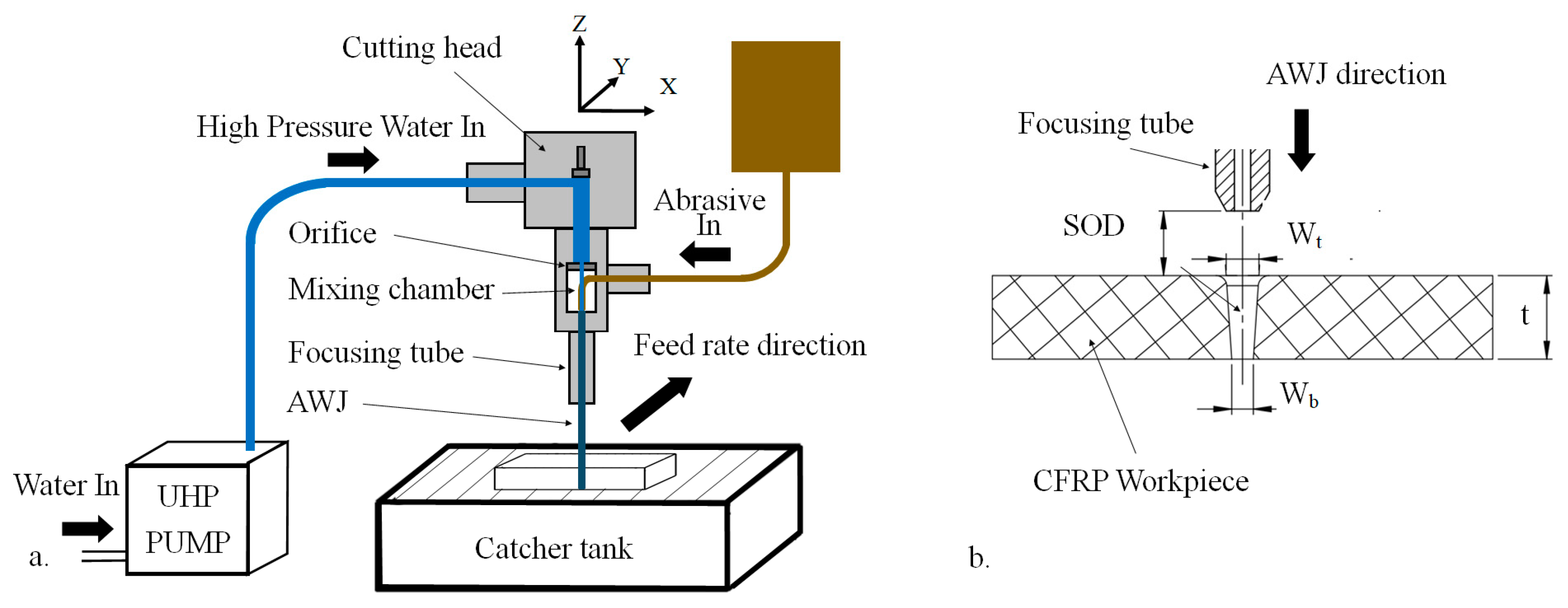
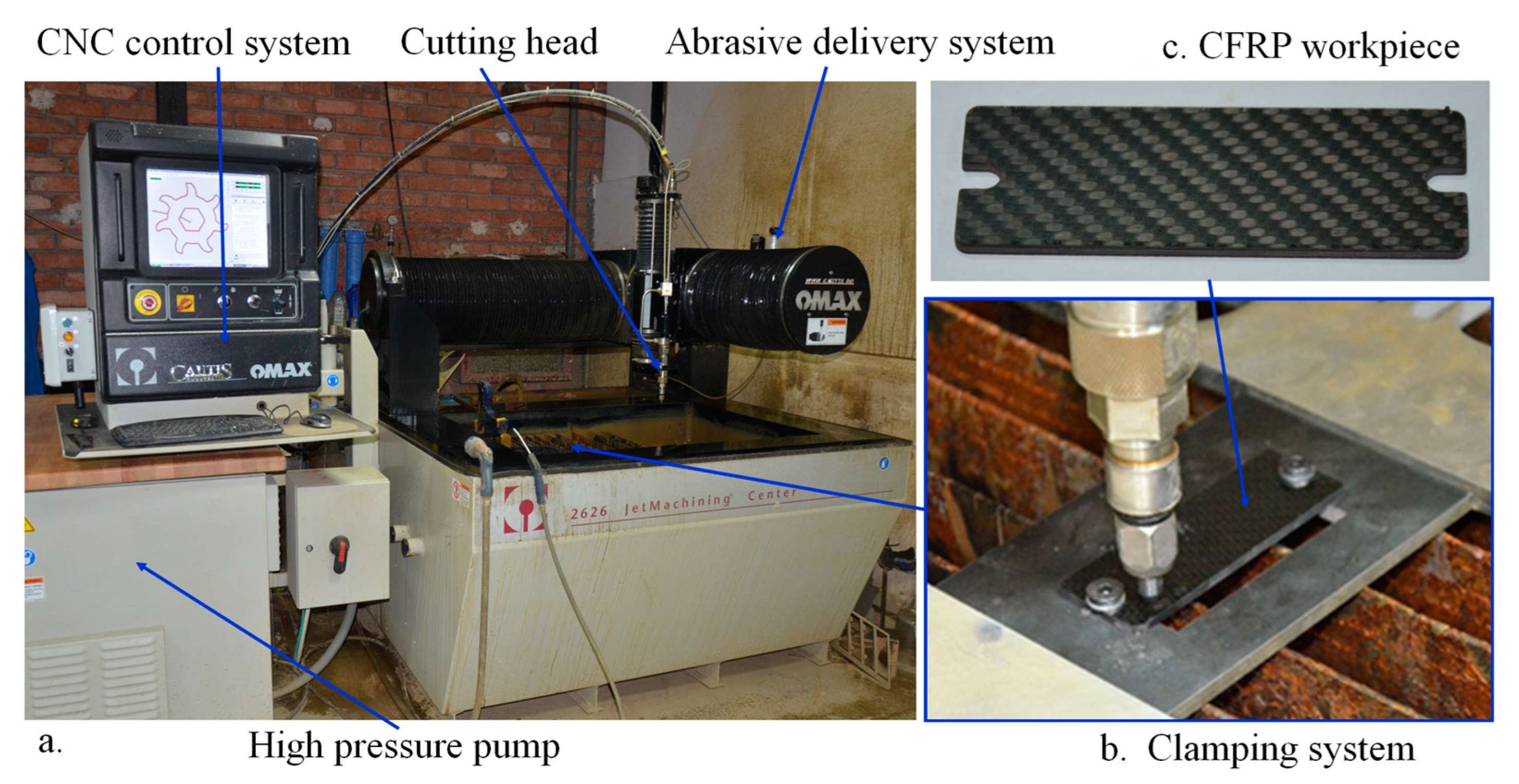
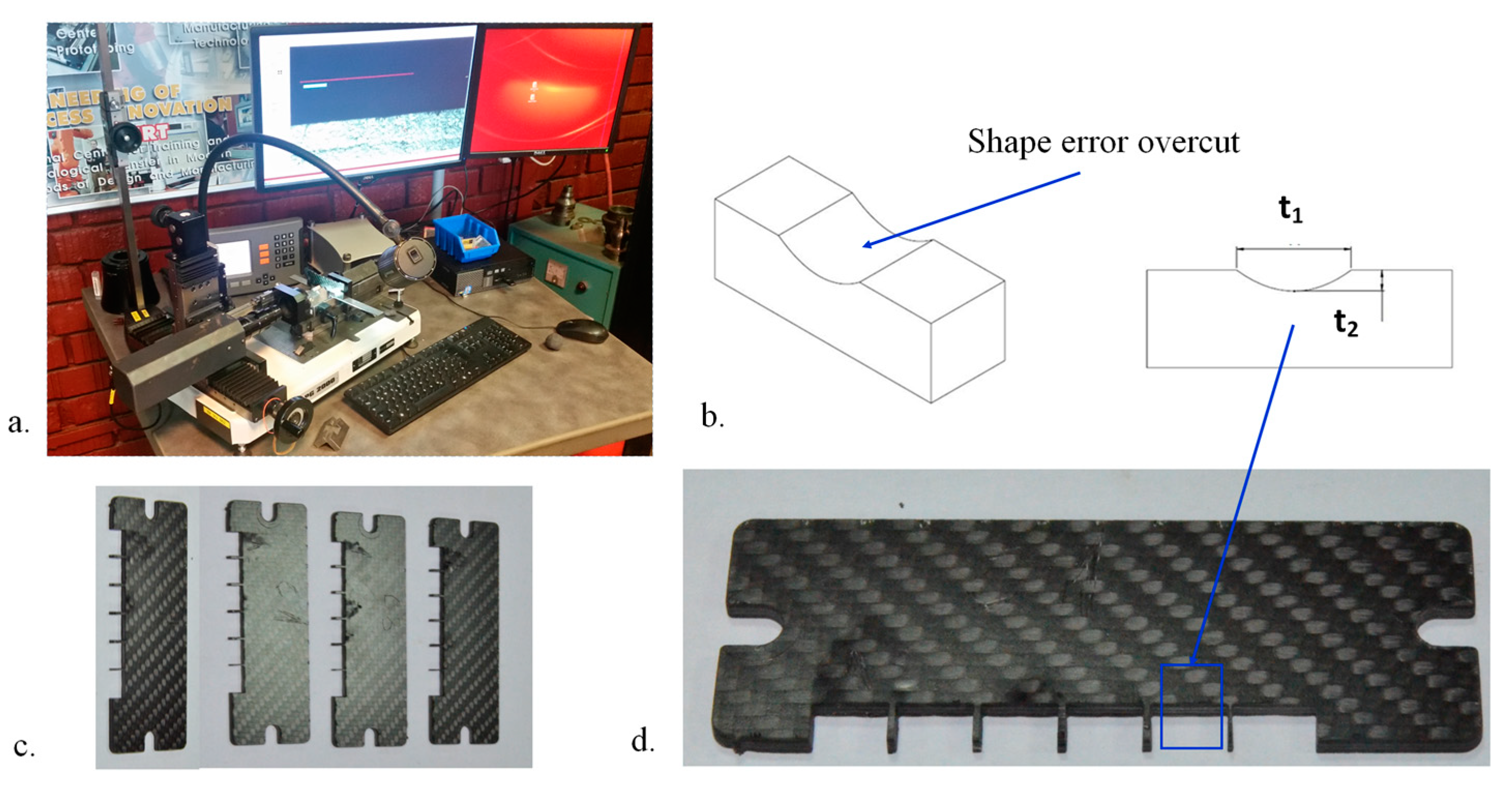
2.2. Experimental Setup
2.3. Materials
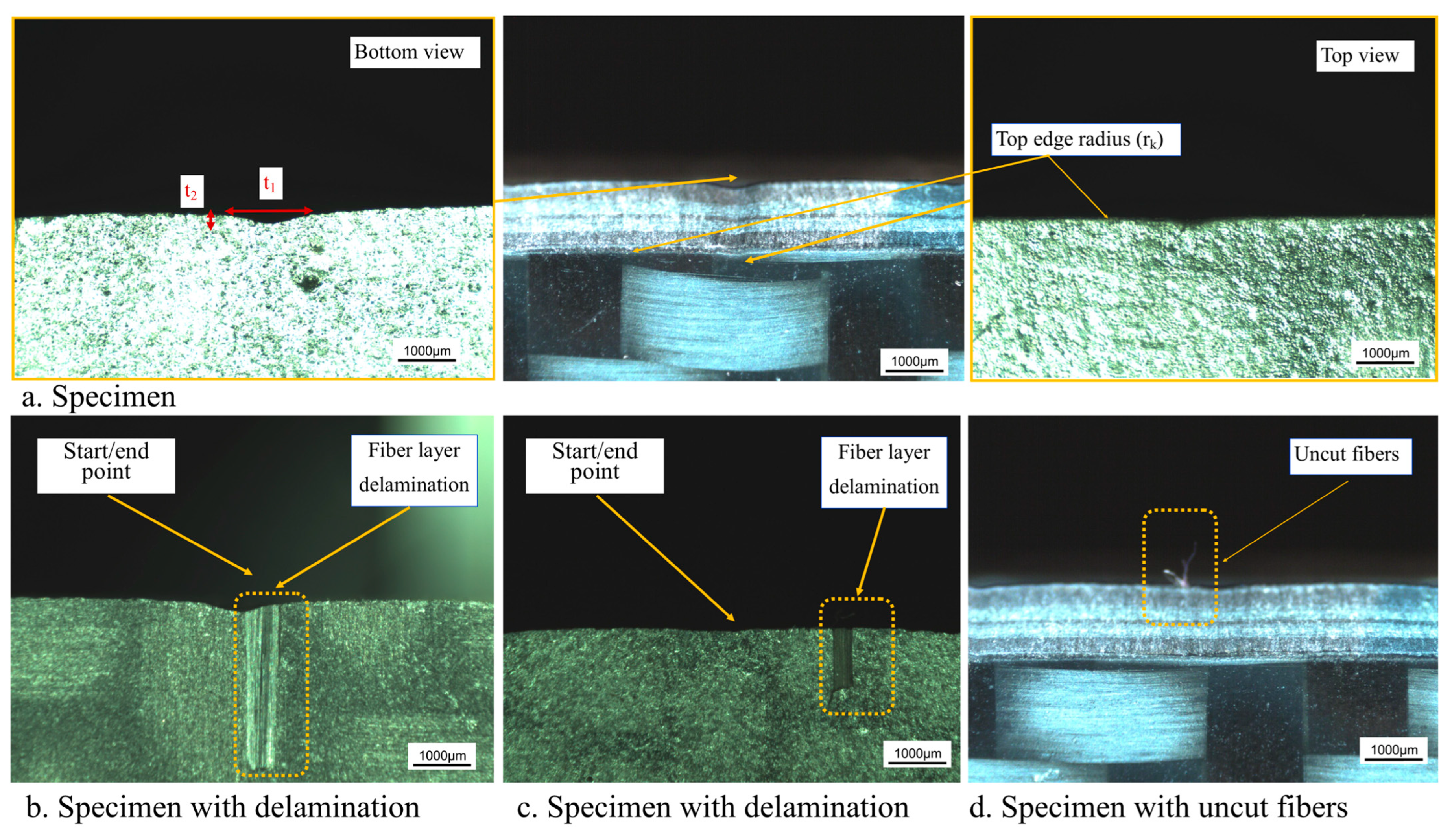
2.4. Experimental Results
3. Results and Discussions
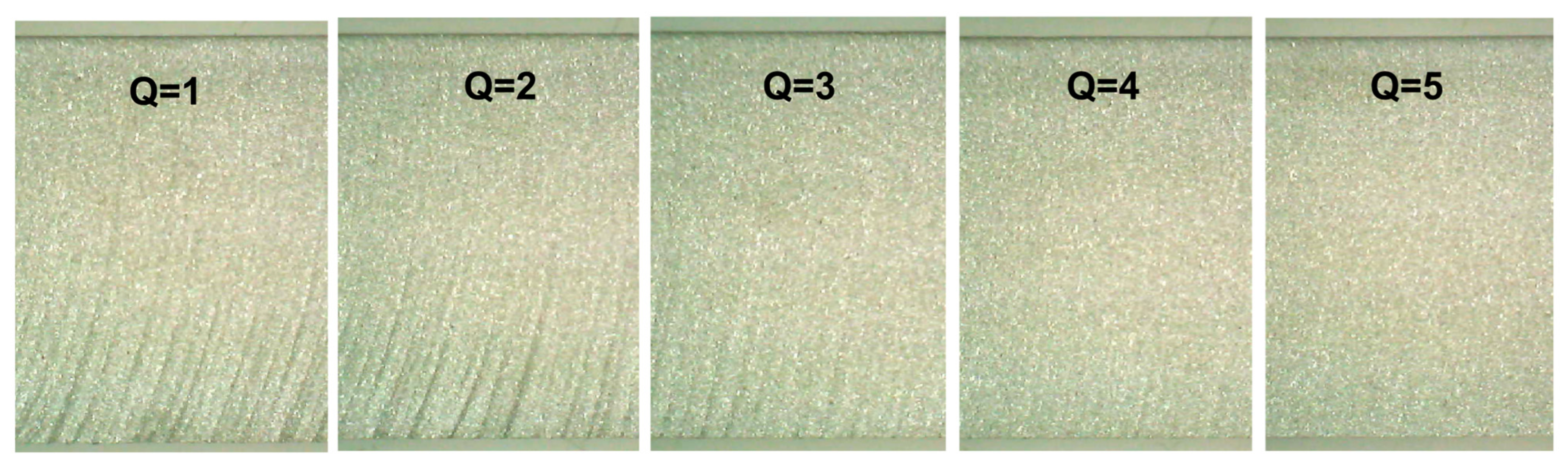
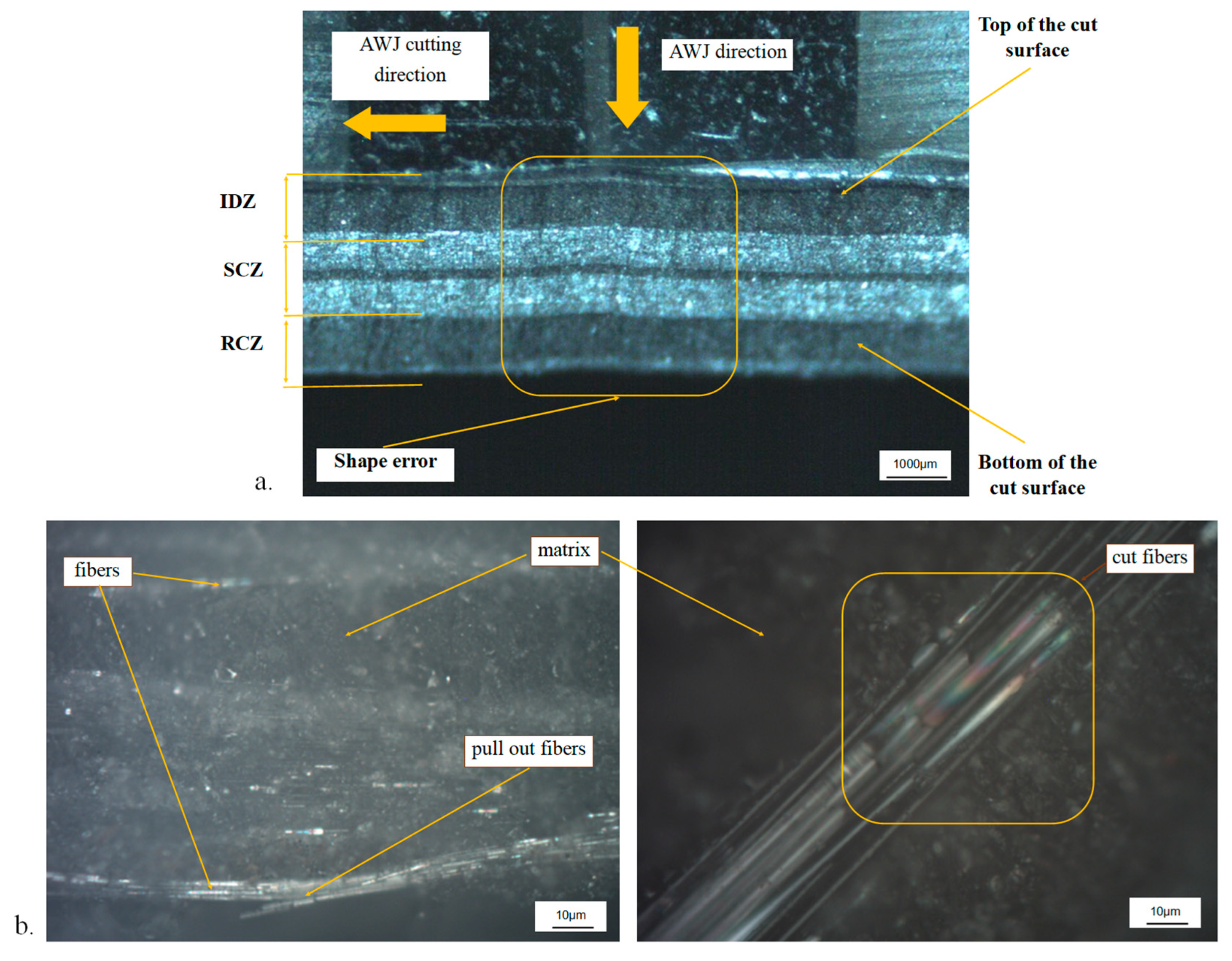
3.1. Morphology of the Cut Surfaces
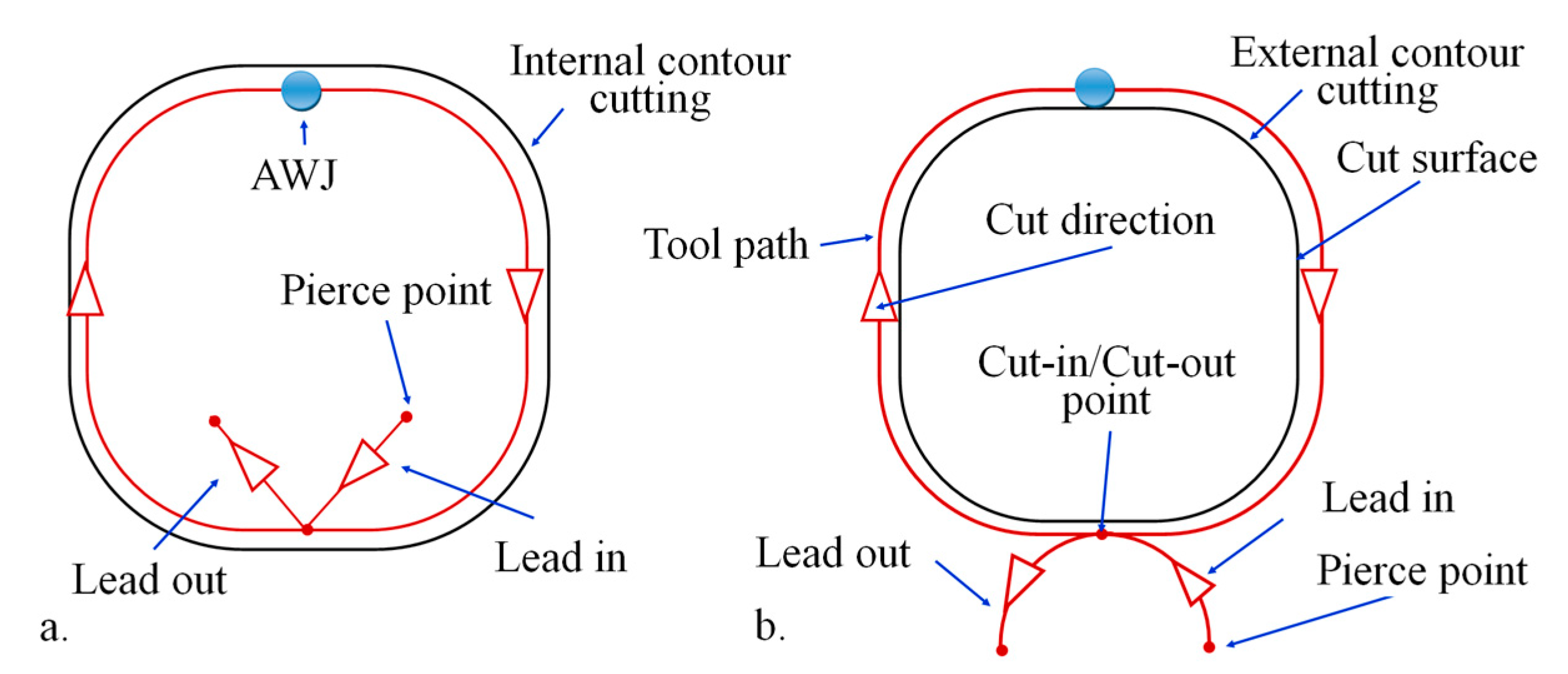
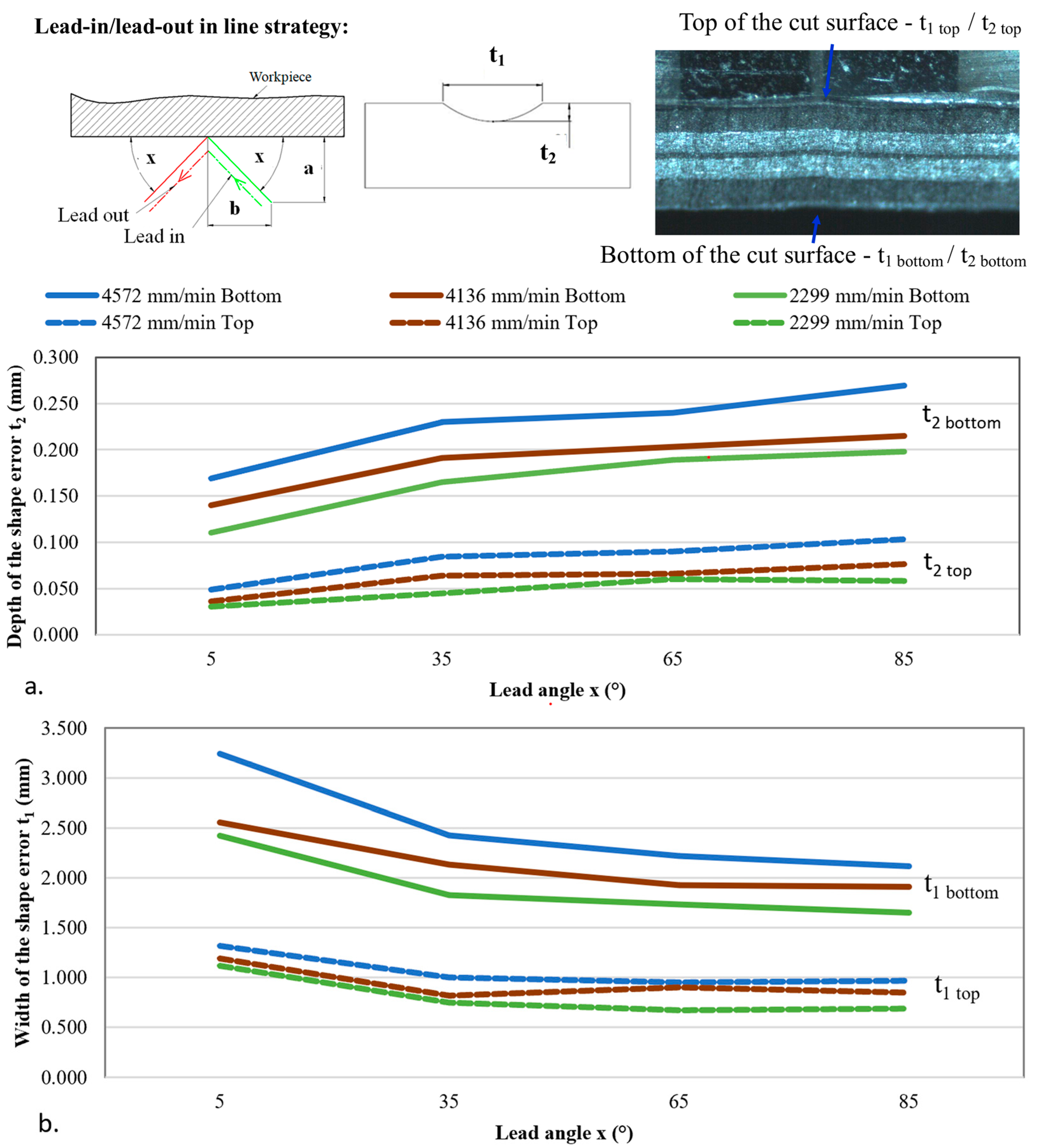
3.2. Investigation on the Shape Error Generated by Using the Lead-In/Lead-Out in Line Strategy
3.3. Investigation on the Shape Error Generated by Using the Lead-In/Lead-Out in Arc Strategy
3.4. Discussions and Mathematical Modelling
3.5. Shape Error Correction Method
- The lead-in/lead-out in arc strategy should be chosen, as it facilitates smooth AWJ movement and helps to maintain a constant feed rate. It is recommended to increase the lead arc radius to a value greater than 3 mm.
- To minimize the depth of the shape error, it is essential to find the optimal combination of process parameters. One key parameter is the feed rate or traverse speed. It is recommended to decrease the feed rate, as this helps decrease the depth of the shape error. The feed rate can be determined using the Zeng model, which takes into account the key parameters of the AWJC process (Equation (1)). In this application, the finishing cutting quality Q5 is recommended.
- The model that was formulated (Equation (3)) can be employed to estimate the depth of the shape error.
3.6. Method Validation
4. Conclusions
- The shape error observed during the cutting process is caused by the decrease in the feed rate at the cut-in and cut-out points. This is attributed to the machine deceleration during lead-in and lead-out movements, resulting in the removal of extra material from the workpiece.
- Although the shape error could be significantly reduced, it was not completely eliminated. In the case of lead-in/lead-out in arc strategy, the minimum dimensions of the shape error were found to be 0.049 mm in depth and 1.736 mm in width. Conversely, for lead-in/lead-out in line strategy, the minimum dimensions were 0.11 mm in depth and 3.240 mm in width. Based on the criterion of minimizing the depth of the shape error, the lead-in/lead-out in arc strategy was found to be more suitable for CFRP cutting.
- A shape error correction method was proposed, providing a set of recommendations for selecting the appropriate lead-in/out strategy and a suitable combination of process parameters. This approach offers several benefits, including minimizing the shape error (up to 80%), improving overall cutting accuracy (up to ±0.05 mm), and reducing the machining time.
- The proposed method has been successfully validated and implemented in industrial applications, demonstrating its effectiveness in improving the accuracy of CFRP cutting and reducing the machining time. This research contributes to the advancement of AWJC technology and provides valuable insights for practical applications in the manufacturing industry.
- A mathematical model was formulated to estimate the depth of the shape error, considering the main process parameters of AWJC. This model provides a predictive tool with over 85% accuracy, enabling the estimation of the shape error depth and optimization of the cutting process.
- Future investigations could explore new composite materials (reinforced with fiberglass, kevlar, wood, metal), optimize the jet trajectory by involving multi-axis AWJC machines, or design new geometries for lead-in/lead-out tool paths, such as spline curves.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ding, J.; Cheng, L.; Chen, X.; Chen, C.; Liu, K. A Review on Ultra-High Cycle Fatigue of CFRP. Compos. Struct. 2021, 256, 113058. [Google Scholar] [CrossRef]
- Al-Lami, A.; Hilmer, P.; Sinapius, M. Eco-Efficiency Assessment of Manufacturing Carbon Fiber Reinforced Polymers (CFRP) in Aerospace Industry. Aerosp. Sci. Technol. 2018, 79, 669–678. [Google Scholar] [CrossRef]
- Poór, D.I.; Geier, N.; Pereszlai, C.; Xu, J. A Critical Review of the Drilling of CFRP Composites: Burr Formation, Characterisation and Challenges. Compos. Part B Eng. 2021, 223, 109155. [Google Scholar] [CrossRef]
- Chua, C.Y.X.; Liu, H.-C.; Di Trani, N.; Susnjar, A.; Ho, J.; Scorrano, G.; Rhudy, J.; Sizovs, A.; Lolli, G.; Hernandez, N.; et al. Carbon Fiber Reinforced Polymers for Implantable Medical Devices. Biomaterials 2021, 271, 120719. [Google Scholar] [CrossRef]
- Guo, F.; Xiao, Q.; Xiao, S.; Wang, Z. Assembly Technology for Aeronautical CFRP Structures under the Collaborative Constrains of Geometric Shape, Physical Performance and Service Stability. Compos. Struct. 2023, 318, 117071. [Google Scholar] [CrossRef]
- Popan, I.A.; Balc, N.; Popan, A.I. Avoiding Carbon Fibre Reinforced Polymer Delamination during Abrasive Water Jet Piercing: A New Piercing Method. Int. J. Adv. Manuf. Technol. 2022, 119, 1139–1152. [Google Scholar] [CrossRef]
- Chen, M.; Zhang, S.; Zeng, J.; Chen, B.; Xue, J.; Ji, L. Correcting Shape Error on External Corners Caused by the Cut-in/Cut-out Process in Abrasive Water Jet Cutting. Int. J. Adv. Manuf. Technol. 2019, 103, 849–859. [Google Scholar] [CrossRef]
- Hutyrová, Z.; Ščučka, J.; Hloch, S.; Hlaváček, P.; Zeleňák, M. Turning of Wood Plastic Composites by Water Jet and Abrasive Water Jet. Int. J. Adv. Manuf. Technol. 2015, 84, 1615–1623. [Google Scholar] [CrossRef]
- Dekster, L.; Karkalos, N.E.; Karmiris-Obratański, P.; Markopoulos, A.P. Evaluation of the Machinability of Ti-6Al-4V Titanium Alloy by AWJM Using a Multipass Strategy. Appl. Sci. 2023, 13, 3774. [Google Scholar] [CrossRef]
- Hardy, M.C.; Herbert, C.R.J.; Kwong, J.; Li, W.; Axinte, D.A.; Sharman, A.R.C.; Encinas-Oropesa, A.; Withers, P.J. Characterising the Integrity of Machined Surfaces in a Powder Nickel Alloy Used in Aircraft Engines. Procedia CIRP 2014, 13, 411–416. [Google Scholar] [CrossRef]
- Nag, A.; Srivastava, M.; Petrů, J.; Váňová, P.; Srivastava, A.K.; Hloch, S. Comparison of Continuous and Pulsating Water Jet during Piercing of Ductile Material. Materials 2023, 16, 3558. [Google Scholar] [CrossRef] [PubMed]
- Srinivasu, D.S.; Axinte, D.A. Surface Integrity Analysis of Plain Waterjet Milled Advanced Engineering Composite Materials. Procedia CIRP 2014, 13, 371–376. [Google Scholar] [CrossRef] [Green Version]
- Zou, X.; Fu, L.; Wu, L.; Zuo, W. Research on Multiphase Flow and Nozzle Wear in a High-Pressure Abrasive Water Jet Cutting Head. Machines 2023, 11, 614. [Google Scholar] [CrossRef]
- Basarman, A.-P.; Lobonțiu, M. Kerf Variation Analysing for Abrasive Water Jet Cutting of a Steel Square Part. MATEC Web Conf. 2017, 112, 03002. [Google Scholar] [CrossRef]
- Dhanawade, A.; Kumar, S. Experimental Study of Delamination and Kerf Geometry of Carbon Epoxy Composite Machined by Abrasive Water Jet. J. Compos. Mater. 2017, 51, 3373–3390. [Google Scholar] [CrossRef]
- Popan, I.A.; Contiu, G.; Campbell, I. Investigation on Standoff Distance Influence on Kerf Characteristics in Abrasive Water Jet Cutting of Composite Materials. MATEC Web Conf. 2017, 137, 01009. [Google Scholar] [CrossRef] [Green Version]
- Szatkiewicz, T.; Perec, A.; Radomska-Zalas, A.; Banaszek, K.; Balasz, B. Preliminary Studies into Cutting of a Novel Two Component 3D-Printed Stainless Steel–Polymer Composite Material by Abrasive Water Jet. Materials 2023, 16, 1170. [Google Scholar] [CrossRef]
- Bañon, F.; Sambruno, A.; Batista, M.; Simonet, B.; Salguero, J. Study of the Surface Quality of Carbon Fiber–Reinforced Thermoplastic Matrix Composite (CFRTP) Machined by Abrasive Water Jet (AWJM). Int. J. Adv. Manuf. Technol. 2020, 107, 3299–3313. [Google Scholar] [CrossRef]
- Kim, G.; Denos, B.R.; Sterkenburg, R. Influence of Different Piercing Methods of Abrasive Waterjet on Delamination of Fiber Reinforced Composite Laminate. Compos. Struct. 2020, 240, 112065. [Google Scholar] [CrossRef]
- Shanmugam, D.K.; Nguyen, T.; Wang, J. A Study of Delamination on Graphite/Epoxy Composites in Abrasive Waterjet Machining. Compos. Part A Appl. Sci. Manuf. 2008, 39, 923–929. [Google Scholar] [CrossRef]
- Lissek, F.; Haeger, A.; Knoblauch, V.; Hloch, S.; Pude, F.; Kaufeld, M. Acoustic Emission for Interlaminar Toughness Testing of CFRP: Evaluation of the Crack Growth Due to Burst Analysis. Compos. Part B Eng. 2018, 136, 55–62. [Google Scholar] [CrossRef]
- Popan, I.A.; Bocanet, V.; Balc, N.; Popan, A.I. Investigation on Feed Rate Influence on Surface Quality in Abrasive Water Jet Cutting of Composite Materials, Monitoring Acoustic Emissions. In Advances in Manufacturing Engineering and Materials; Lecture Notes in Mechanical, Engineering; Hloch, S., Klichová, D., Krolczyk, G.M., Chattopadhyaya, S., Ruppenthalová, L., Eds.; Springer International Publishing: Cham, Switzerland, 2019; pp. 105–113. ISBN 978-3-319-99352-2. [Google Scholar]
- Yamamoto, G.; Onodera, M.; Koizumi, K.; Watanabe, J.; Okuda, H.; Tanaka, F.; Okabe, T. Considering the Stress Concentration of Fiber Surfaces in the Prediction of the Tensile Strength of Unidirectional Carbon Fiber-Reinforced Plastic Composites. Compos. Part A Appl. Sci. Manuf. 2019, 121, 499–509. [Google Scholar] [CrossRef]
- Perec, A.; Fajdek-Bieda, A.; Pude, F.; Radomska-Zalas, A. Process optimization by applying the response surface methodology (RSM) to the abrasive suspension water jet cutting of phenolic composites. Facta Univ. 2022, 20, 1–16. [Google Scholar] [CrossRef]
- Mohamed, H. Trimming of CFRP Aircraft Components. In Proceedings of the WJTA-IMCA Conference and Expo, Houston, TX, USA, 9–11 September 2013. [Google Scholar]
- Chen, M.; Zhang, S.; Zeng, J.; Chen, B. Correcting Shape Error Located in Cut-in/Cut-out Region in Abrasive Water Jet Cutting Process. Int. J. Adv. Manuf. Technol. 2019, 102, 1165–1178. [Google Scholar] [CrossRef]
- Omax “Precision Practices: Lead-Ins and Lead-Outs”. 2023. Available online: https://www.Omax.Com/En/Us/Media-Center/Tips/Precision-Practices-Lead-Ins-and-Lead-Outs (accessed on 1 June 2023).
- WARDJet Reducing Lead-in/Lead-out Witness Marks. 2023. Available online: https://my.wardjet.com/Waterjet/University/Precision-Quality (accessed on 1 June 2023).
- Hlaváč, L.M. Revised Model of Abrasive Water Jet Cutting for Industrial Use. Materials 2021, 14, 4032. [Google Scholar] [CrossRef] [PubMed]
- Kumar, S.A.; Rajesh, R.; Pugazhendhi, S. A Review of Stress Concentration Studies on Fibre Composite Panels with Holes/Cutouts. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2020, 234, 1461–1472. [Google Scholar] [CrossRef]
- Di Franco, G.; Zuccarello, B. Analysis and Optimization of Hybrid Double Lap Aluminum-GFRP Joints. Compos. Struct. 2014, 116, 682–693. [Google Scholar] [CrossRef] [Green Version]
- Kant, T.; Swaminathan, K. Estimation of Transverse/Interlaminar Stresses in Laminated Composites—A Selective Review and Survey of Current Developments. Compos. Struct. 2000, 49, 65–75. [Google Scholar] [CrossRef]
- Mohamed Makki, M.; Chokri, B. Experimental, Analytical, and Finite Element Study of Stress Concentration Factors for Composite Materials. J. Compos. Mater. 2017, 51, 1583–1594. [Google Scholar] [CrossRef]
- Ryo, N.; Mitsuhiro, O.; Daisuke, F. Effects of Stress Concentration on the Mechanical Properties of Carbon Fiber Reinforced Plastic. Int. J. Smart Mater. Mechatron. 2015, 2, 136–139. [Google Scholar]
- Montgomery, D.C. Design and Analysis of Experiments, 10th ed.; Wiley: Hoboken, NJ, USA, 2020; ISBN 978-1-119-49247-4. [Google Scholar]
- Rammohan, S.; Kumaran, S.T.; Uthayakumar, M.; Korniejenko, K.; Nykiel, M.; Velayutham, A. Prediction of Abrasive Waterjet Machining Parameters of Military-Grade Armor Steel by Semi-Empirical and Regression Models. Materials 2022, 15, 4368. [Google Scholar] [CrossRef] [PubMed]
- Jankovic, P.; Igic, T.; Nikodijevic, D. Process Parameters Effect on Material Removal Mechanism and Cut Quality of Abrasive Water Jet Machining. Theor. Appl. Mech. 2013, 40, 277–291. [Google Scholar] [CrossRef] [Green Version]
- Zeng, J.; Kim, T.J. Development of an Abrasive Waterjet Kerf Cutting Model for Brittle Materials. In Jet Cutting Technology; Springer: Berlin/Heidelberg, Germany, 1992; pp. 483–501. [Google Scholar]
- Valleyknife. Available online: http://www.valleyknife.com/waterjet_services.html (accessed on 1 June 2023).
- ECOTECH—CFRP Manufacturer. Available online: https://www.r-g.de/en/index.html (accessed on 26 May 2023).
- Nag, A.; Ščučka, J.; Hlavacek, P.; Klichová, D.; Srivastava, A.K.; Hloch, S.; Dixit, A.R.; Foldyna, J.; Zelenak, M. Hybrid Aluminium Matrix Composite AWJ Turning Using Olivine and Barton Garnet. Int. J. Adv. Manuf. Technol. 2018, 94, 2293–2300. [Google Scholar] [CrossRef]
- Thongkaew, K.; Wang, J.; Li, W. An Investigation of the Hole Machining Processes on Woven Carbon-Fiber Reinforced Polymers (CFRPs) Using Abrasive Waterjets. Mach. Sci. Technol. 2019, 23, 19–38. [Google Scholar] [CrossRef]
- Mardi, K.B.; Dixit, A.R.; Pramanik, A.; Hvizdos, P.; Mallick, A.; Nag, A.; Hloch, S. Surface Topography Analysis of Mg-Based Composites with Different Nanoparticle Contents Disintegrated Using Abrasive Water Jet. Materials 2021, 14, 5471. [Google Scholar] [CrossRef]
- Yang, M.; Yang, J.; Zhu, L.; Yu, X. A Novel Curvature Circle Iterative Algorithm for Contour Error Control of Multi-Axis CNC Machine Tools. Precis. Eng. 2020, 65, 23–31. [Google Scholar] [CrossRef]
- Mohankumar, V.; Kanthababu, M.; Velayudham, A. Abrasive Waterjet Cutting of Boron Carbide Particles Reinforced Al 6063 MMCs—A Semi Empirical Modeling Approach in the Prediction of Kerf Angle. Measurement 2021, 181, 109492. [Google Scholar] [CrossRef]




| Lead-In and Lead-Out Strategy: | Parameters | Value |
|---|---|---|
| Line | Lead angle (x) | 5, 35, 65, 85° |
| Feed rate (V) | 2299, 4136, 4572 mm/min | |
| Arc | Radius of the arc (r) | 1, 3, 5, 7 mm |
| Feed rate (V) | 2299, 4136, 4572 mm/min |
| Parameters | Value |
|---|---|
| Feed rate (V) | 4572, 4136, 2299 mm/min |
| Waterjet pressure (P) | 350 MPa |
| Standoff distance (SOD) | 1 mm |
| Cutting depth (t) | 3 mm |
| Abrasive mass flow (ma) | 0.45 kg/min |
| Focusing tube diameter | 0.76 mm |
| Water nozzle diameter | 0.35 mm |
| Feed Rate | Shape Error | Lead-In/Lead-Out in Line | Lead-In/Lead-Out in Arc | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Lead Angle x (°) | Radius of the Arc r (mm) | ||||||||||
| Position Dimensions | 5 | 35 | 65 | 85 | 1 | 3 | 5 | 7 | |||
| Q1 separation quality 4572 mm/min | Top | t2 (mm) | Average | 0.049 | 0.084 | 0.090 | 0.103 | 0.057 | 0.056 | 0.046 | 0.047 |
| St.dev. | 0.0014 | 0.0109 | 0.009 | 0.0083 | 0.0018 | 0.0151 | 0.0023 | 0.0051 | |||
| t1 (mm) | Average | 1.320 | 1.003 | 0.950 | 0.970 | 1.230 | 0.755 | 0.799 | 0.749 | ||
| St.dev. | 0.0065 | 0.0108 | 0.0076 | 0.0066 | 0.0175 | 0.0101 | 0.0095 | 0.013 | |||
| Bottom | t2 (mm) | Average | 0.169 | 0.230 | 0.240 | 0.269 | 0.091 | 0.066 | 0.059 | 0.057 | |
| St.dev. | 0.0077 | 0.0112 | 0.0056 | 0.0082 | 0.0103 | 0.0037 | 0.0122 | 0.0071 | |||
| t1 (mm) | Average | 3.240 | 2.430 | 2.218 | 2.118 | 3.120 | 2.999 | 2.180 | 2.080 | ||
| St.dev. | 0.0066 | 0.0123 | 0.013 | 0.0032 | 0.0067 | 0.0035 | 0.0047 | 0.0052 | |||
| Q3 medium quality 4136 mm/min | Top | t2 (mm) | Average | 0.036 | 0.064 | 0.066 | 0.076 | 0.050 | 0.042 | 0.043 | 0.040 |
| St.dev. | 0.0066 | 0.0029 | 0.0049 | 0.0103 | 0.0007 | 0.0022 | 0.0078 | 0.0031 | |||
| t1 (mm) | Average | 1.191 | 0.821 | 0.900 | 0.850 | 0.860 | 0.699 | 0.500 | 0.560 | ||
| St.dev. | 0.0044 | 0.007 | 0.0131 | 0.0116 | 0.0043 | 0.0034 | 0.0142 | 0.0072 | |||
| Bottom | t2 (mm) | Average | 0.140 | 0.191 | 0.203 | 0.215 | 0.089 | 0.060 | 0.053 | 0.055 | |
| St.dev. | 0.0079 | 0.003 | 0.0083 | 0.0062 | 0.0026 | 0.0062 | 0.0051 | 0.0038 | |||
| t1 (mm) | Average | 2.560 | 2.133 | 1.927 | 1.910 | 2.857 | 2.500 | 1.990 | 1.890 | ||
| St.dev. | 0.0156 | 0.0056 | 0.0026 | 0.0046 | 0.0091 | 0.0053 | 0.0064 | 0.0074 | |||
| Q5 finishing quality 2299 mm/min | Top | t2 (mm) | Average | 0.030 | 0.045 | 0.060 | 0.058 | 0.042 | 0.037 | 0.036 | 0.038 |
| St.dev. | 0.0101 | 0.0093 | 0.0098 | 0.0051 | 0.0042 | 0.0047 | 0.0071 | 0.0049 | |||
| t1 (mm) | Average | 1.120 | 0.751 | 0.670 | 0.690 | 0.710 | 0.458 | 0.430 | 0.400 | ||
| St.dev. | 0.0082 | 0.0045 | 0.0024 | 0.0091 | 0.0033 | 0.0037 | 0.007 | 0.0032 | |||
| Bottom | t2 (mm) | Average | 0.110 | 0.165 | 0.189 | 0.198 | 0.086 | 0.059 | 0.051 | 0.049 | |
| St.dev. | 0.0177 | 0.0104 | 0.0087 | 0.0038 | 0.0051 | 0.0082 | 0.0021 | 0.0032 | |||
| t1 (mm) | Average | 2.423 | 1.830 | 1.736 | 1.650 | 2.810 | 1.985 | 1.836 | 1.736 | ||
| St.dev. | 0.0066 | 0.0155 | 0.0058 | 0.0039 | 0.0015 | 0.0061 | 0.0092 | 0.0083 | |||
| Multiple R | R Square | Adjusted R Square | Standard Error | ||
|---|---|---|---|---|---|
| 0.9529 | 0.9081 | 0.7868 | 0.0212 | ||
| ANOVA | df | SS | Mean Square | F | Significance F |
| Regression | 2 | 0.04022 | 0.02012 | 44.5108 | 0.00046 |
| Residual | 9 | 0.00406 | 0.00045 | ||
| Total | 11 | 0.04432 | |||
| Coefficients | Standard Error | t Stat | p-Value | ||
| Intercept | 0.0748 | 0.00952 | 7.84962 | 5.00465 × 10−5 | |
| Lead radius (r) | −0.0056 | 0.00121 | −4.60739 | 0.00173 | |
| Feed rate (V) | 2.95 × 10−6 | 2.71438 × 10−6 | 1.08727 | 0.30858 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Popan, I.A.; Cosma, C.; Popan, A.I.; Panc, N.; Filip, D.; Balc, N. Correction of Shape Error at Cut-In and Cut-Out Points in Abrasive Waterjet Cutting of Carbon Fiber Reinforced Polymer (CFRP). Machines 2023, 11, 800. https://doi.org/10.3390/machines11080800
Popan IA, Cosma C, Popan AI, Panc N, Filip D, Balc N. Correction of Shape Error at Cut-In and Cut-Out Points in Abrasive Waterjet Cutting of Carbon Fiber Reinforced Polymer (CFRP). Machines. 2023; 11(8):800. https://doi.org/10.3390/machines11080800
Chicago/Turabian StylePopan, Ioan Alexandru, Cosmin Cosma, Alina Ioana Popan, Nicolae Panc, Daniel Filip, and Nicolae Balc. 2023. "Correction of Shape Error at Cut-In and Cut-Out Points in Abrasive Waterjet Cutting of Carbon Fiber Reinforced Polymer (CFRP)" Machines 11, no. 8: 800. https://doi.org/10.3390/machines11080800
APA StylePopan, I. A., Cosma, C., Popan, A. I., Panc, N., Filip, D., & Balc, N. (2023). Correction of Shape Error at Cut-In and Cut-Out Points in Abrasive Waterjet Cutting of Carbon Fiber Reinforced Polymer (CFRP). Machines, 11(8), 800. https://doi.org/10.3390/machines11080800








