Research on the Influence of Charging Oil Conditions on the Axial Force of Hydraulic Torque Converter
Abstract
1. Introduction
2. Numerical Methods
2.1. CFD Model and Boundary Settings
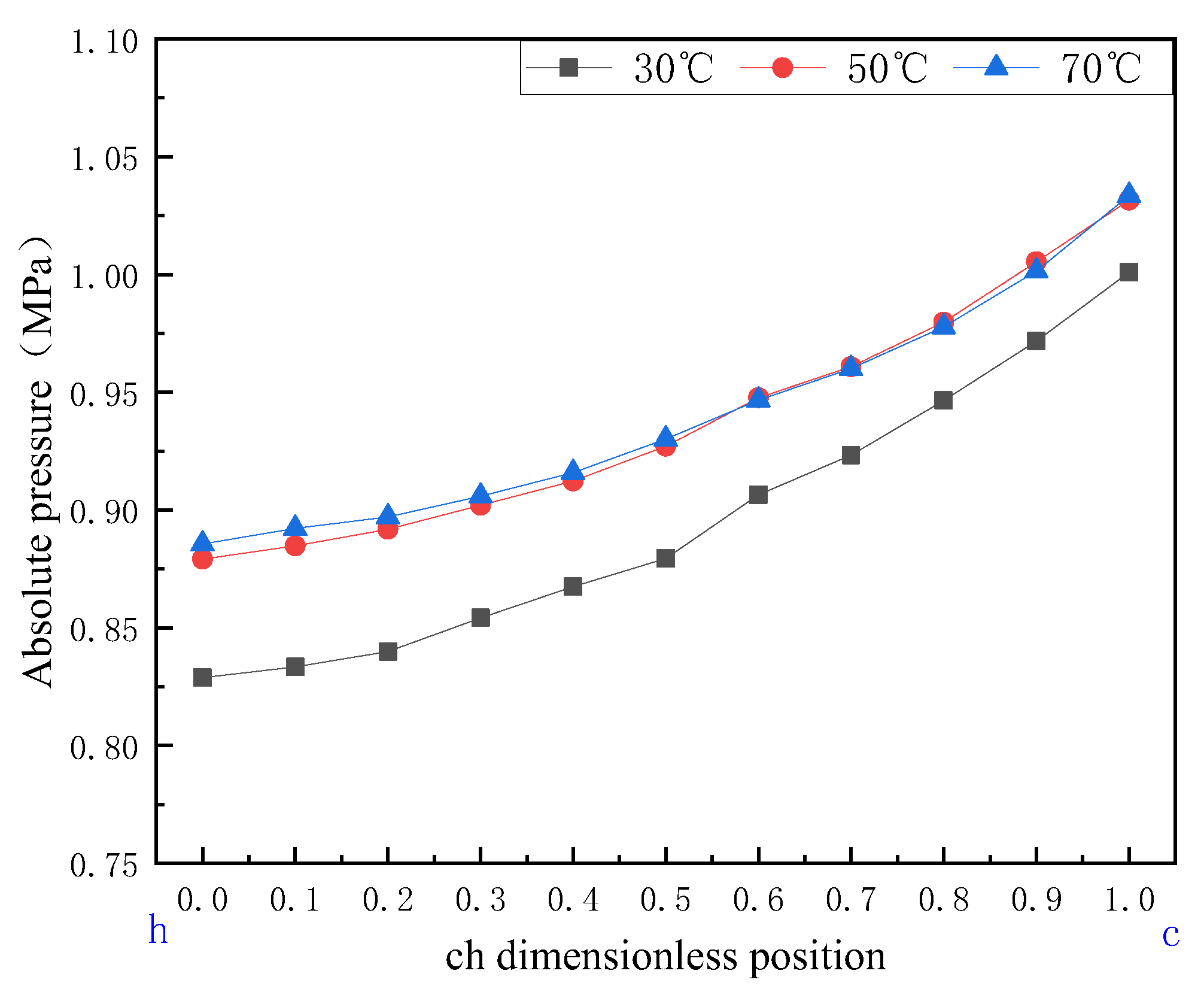
2.2. Mesh Independence Analysis and Model Accuracy Verification
3. The Axial Force Testing System of the Hydraulic Torque Converter
3.1. The Testing Method for Axial Forces
3.2. Hydraulic Torque Converter Axial Force Test Bench
4. The Influence Mechanism of Charging Oil on Axial Force
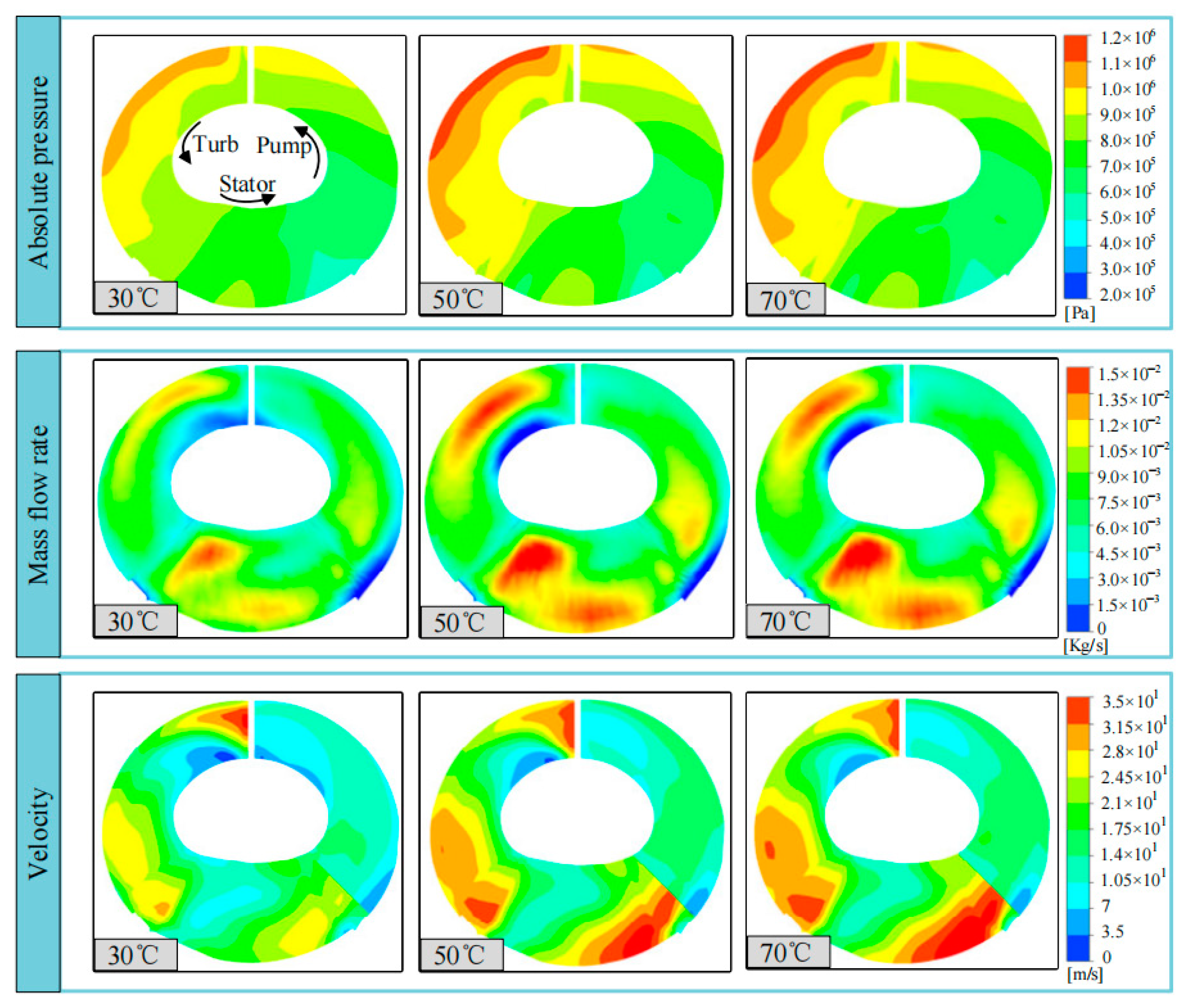
4.1. Axial Force at Different Temperatures
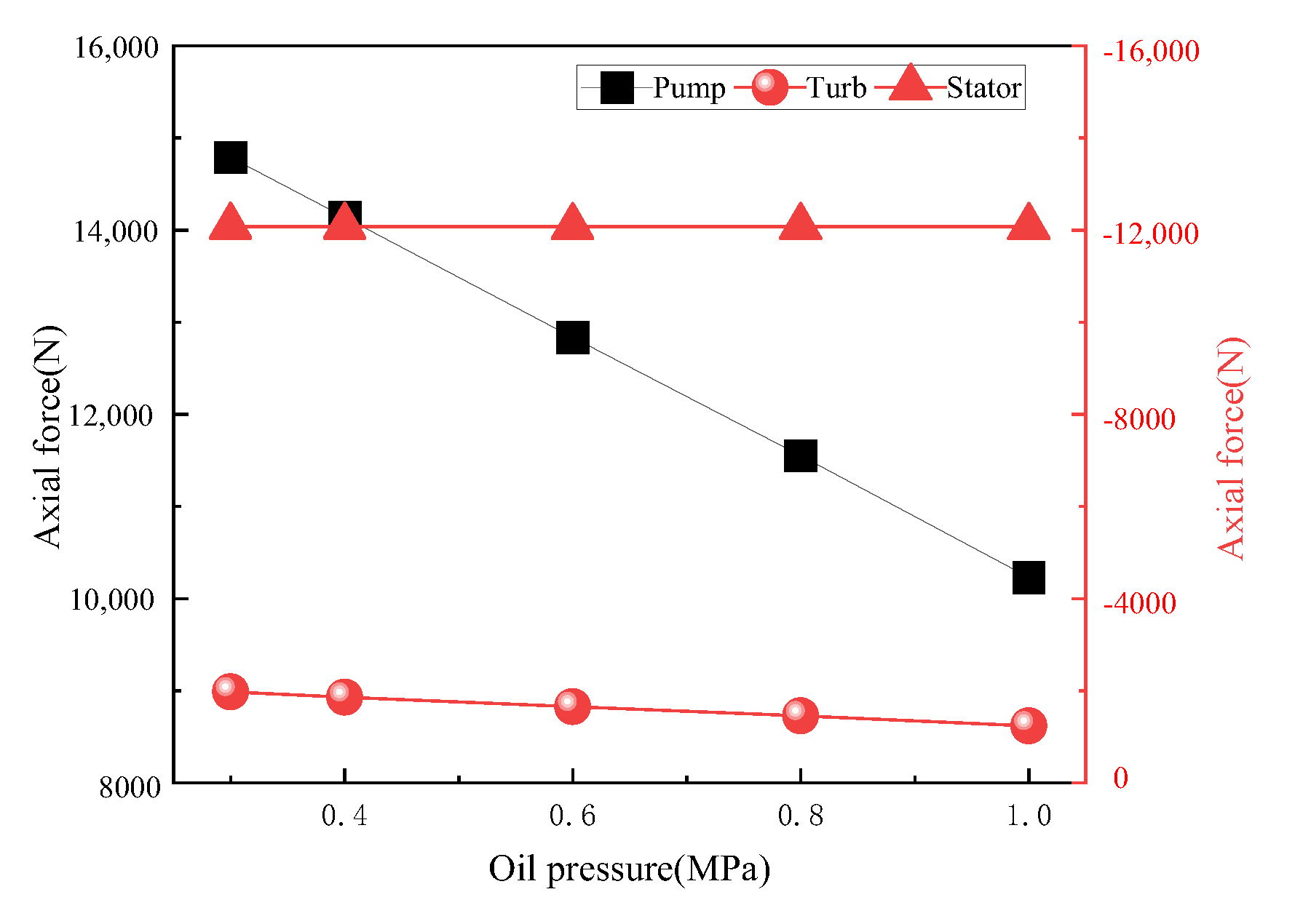
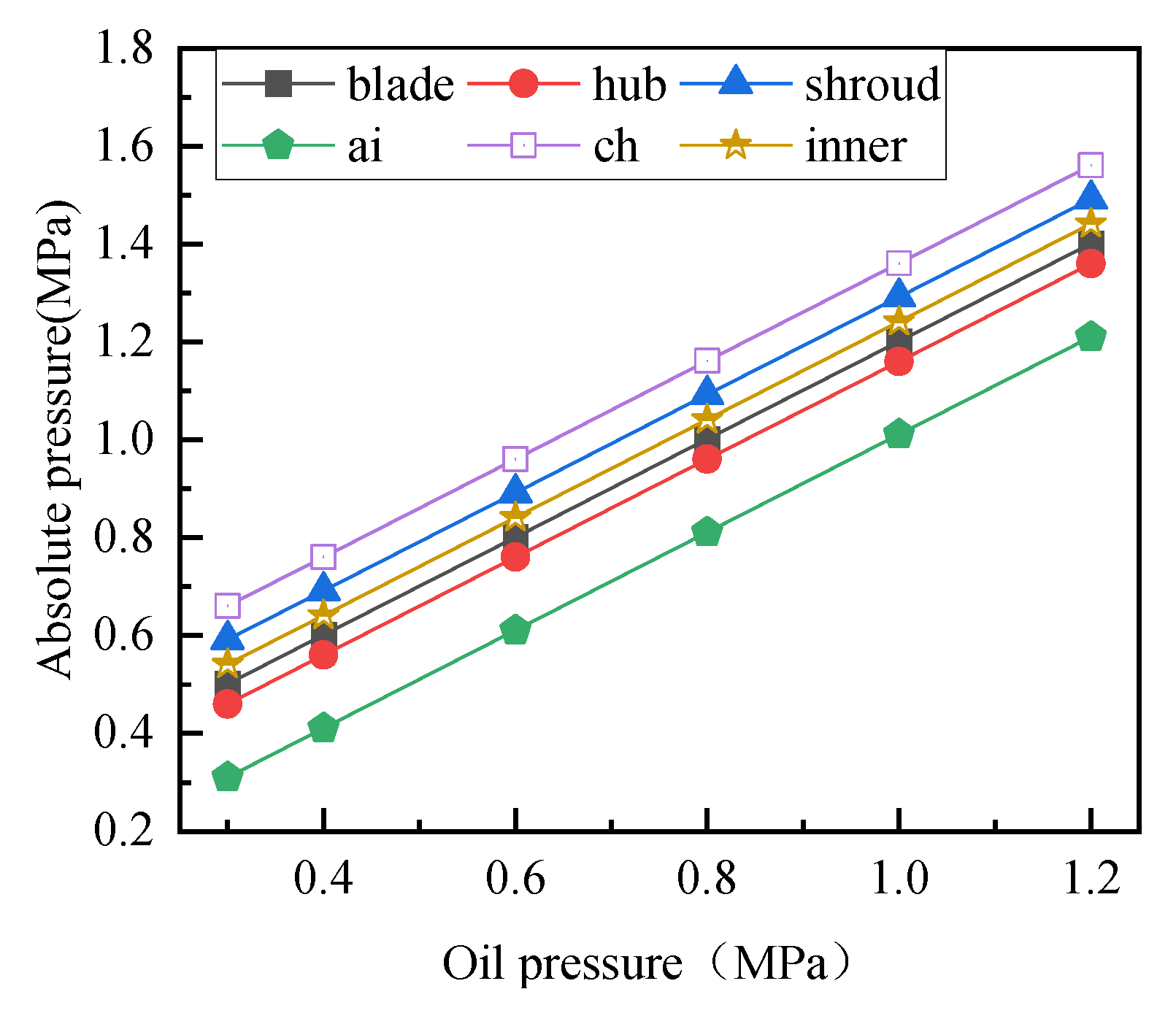
4.2. Axial Force under Different Pressure of Charging Oil
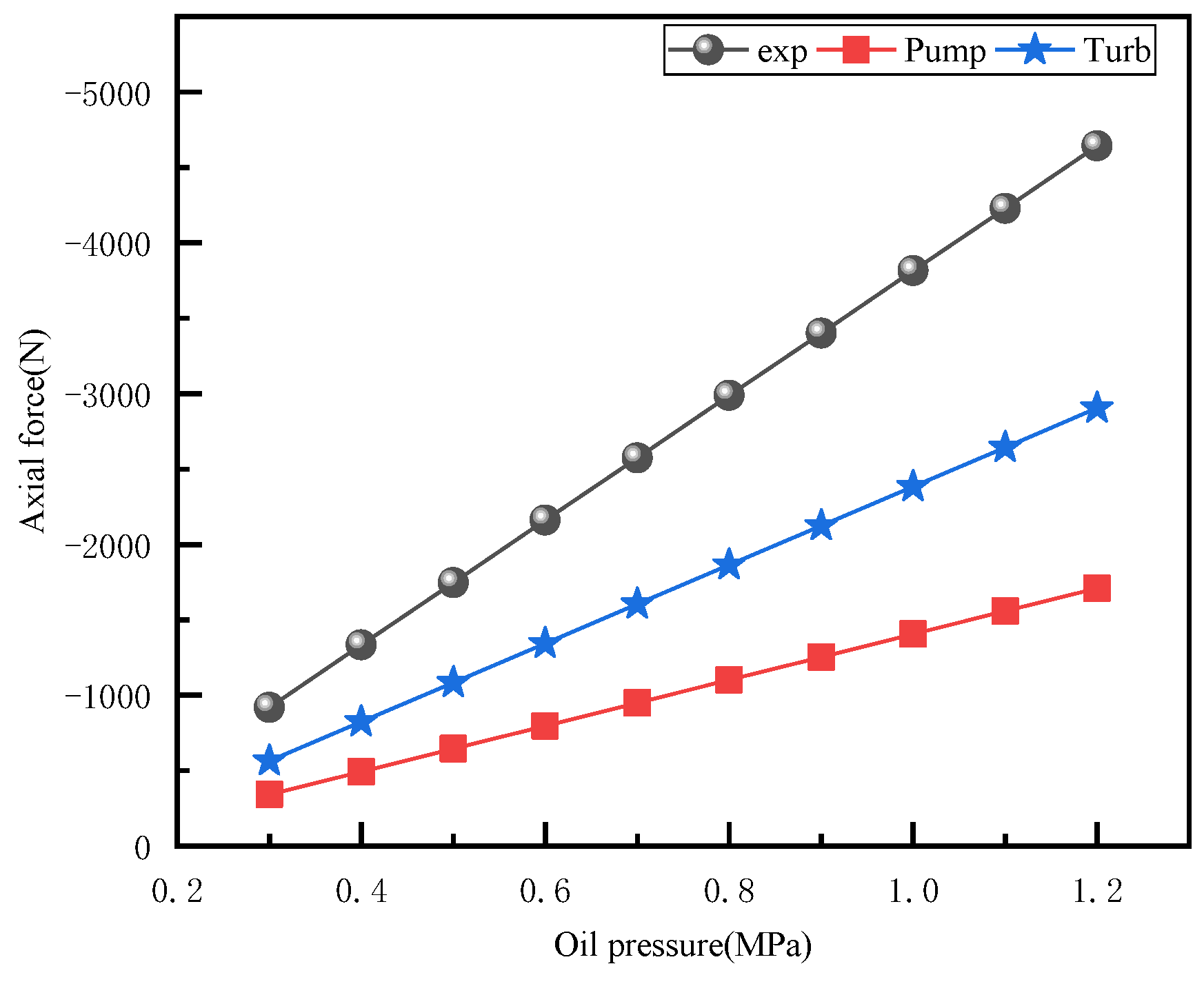
4.3. Test Verification
5. Conclusions
- (1)
- A CFD numerical calculation model of the axial force of the torque converter is proposed, which consists of the flow field of the torque converter and its leakage region. The experiments demonstrate that the results from the simulations are within 5% error. The CFD model is able to accurately simulate the axial force of the torque converter under various operating conditions, which is of significant importance for the precise calculation of the axial force in hydraulic torque converters.
- (2)
- A novel experimental method for testing axial forces in the hydraulic torque converter is proposed. The axial forces on the impellers within a closed-coupled wheelset are measured accurately through the arrangement of different sensors and circlip in the hydraulic torque converter.
- (3)
- Research indicates that the axial forces on the pump and turbine are in the same direction and act on the turbine-side bearing under certain conditions, resulting in stress concentration in the bearing and reduced life. Thus, it is important to pay particular attention to the shaft bearing characteristics and its suppression on the turbine side during the design of the torque converter.
- (4)
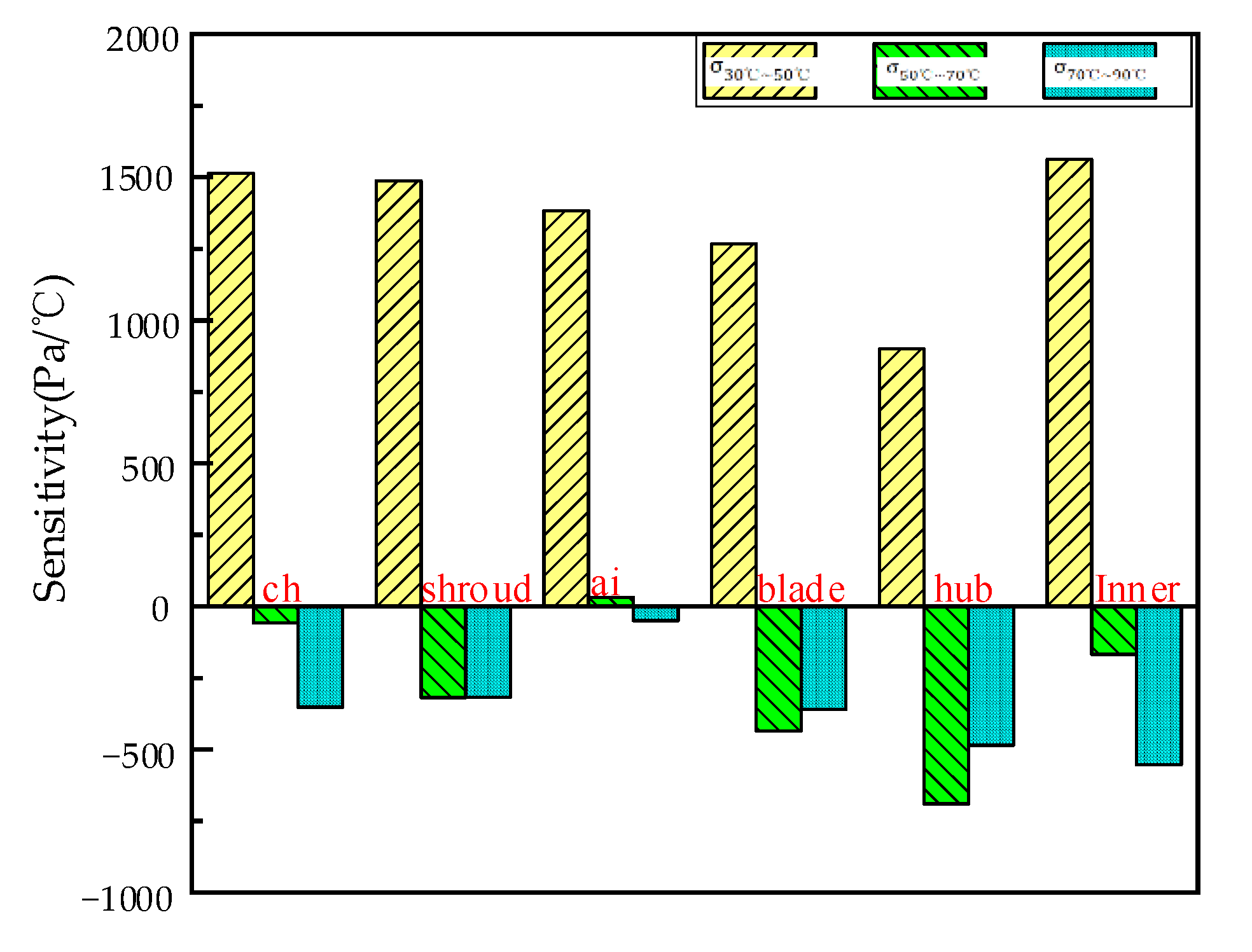
- The axial force of the torque converter is significantly influenced by the temperature of the charging oil. The increase in temperature causes a reduction in the axial force of the pump and turbine, while it is unchanged in the stator. In addition, the variation in temperature directly affects the viscosity of oil, leading to significant changes in the axial force. Consequently, the use of a lower viscosity fluid is more effective in reducing and suppressing the axial force of the torque converter.
- (5)
- The charging pressure of the torque converter affects its axial force, especially in the pump. The results indicate that the axial forces of the pump wheel and turbine gradually decrease as the pressure increases, while the axial force of the stator keeps constant.
- (6)
- Research shows that the axial unbalanced area is an important parameter for predicting axial force variation, which determines the direction and magnitude of the axial force change of the hydraulic torque converter. A formula of axial force is proposed that enables fast prediction of turbine axial forces under various conditions.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| CC | =TP × D−5 × ωP−2, Capacity constant [kg/rad2/m3] |
| D | Torque converter torus diameter [m] |
| K | =TT/TP, Torque ratio |
| NP | Rotating speed of pump [rpm] |
| NS | Rotating speed of stator [rpm] |
| NT | Rotating speed of turbine [rpm] |
| Fi | Impeller axial force [N], i represents different impellers |
| Fij | Axial force[N], where i represents different impellers and j represents different positions |
| Si | Axial imbalance area [m2], i represents different impellers |
References
- Yan, Q.; Wei, W.; Liu, C.; Liu, S.; Li, J.; Liu, B. Torque Converter Modern Design Theory and Methodology. Chin. Hydraul. Pneum. 2015, 2015, 1–8. [Google Scholar]
- Liu, Y.; Liu, C.; He, D.; Pa, Y. Three-dimensional Flow Numerical Calculation of Axial Load in a Torque Converter. Mach. Des. Res. 2011, 27, 42–44. [Google Scholar]
- Amer, W.S.; Farag, A.M.; Abady, I.M. Asymptotic analysis and numerical solutions for the rigid body containing a viscous liquid in cavity in the presence of gyrostatic moment. Arch. Appl. Mech. 2021, 91, 3889–3902. [Google Scholar] [CrossRef]
- Chen, Y. Theoretical Analysis and Experimental Research on Axial Force of Hydraulic Torque Converter. Ph.D. Thesis, Jilin University, Changchun, China, 1987. [Google Scholar]
- Yan, Q.; Tan, L.; Wei, W.; Song, Z. Thrust Load with Different Balance Holes Pattern of Turine Hub in a Hydrodynamic Torque Converter. Trans. Beijing Inst. Technol. 2019, 39, 7–13. [Google Scholar]
- Liu, C.; Ma, W.; Xu, R. Calculation and Analysis of Axial Force in Hydrodynamic Torque Converter based on CFD. J. Jilin Univ. (Eng. Technol. Ed.) 2009, 39, 1181–1185. [Google Scholar]
- Yan, Q.; Li, J.; Wei, W. Research on Effect of Working Oil Temperature for Hydraulic Torque Converter Performance Using CFD and Test. J. Mech. Eng. 2014, 50, 118–125. [Google Scholar] [CrossRef]
- By, R.R.; Lakshminarayana, B. Measurement and analysis of static pressure field in a torque converter turbine. J. Fluids Eng.-Trans. Asme 1995, 117, 473–478. [Google Scholar] [CrossRef]
- By, R.R.; Lakshminarayana, B. Static Pressure Measurement in a Torque Converter Stator. SAE911934, SAE Book SP879. SAE Trans. 1991, 100, 1756–1764. [Google Scholar]
- Yan, Q.; Liu, C.; Wei, W. Numerical simulation of the flow field of a flat torque converter. J. Beijing Inst. Technol. 2012, 21, 309–314. [Google Scholar]
- Sun, B.; Ma, W.; Li, Y.; Lü, Y. Axial Force Caculation Method of Hydrodynamic Coupling. Trans. Chin. Soc. Agric. Mach. 2014, 2, 40–46. [Google Scholar]
- Cai, W.; Ma, W.; Liu, C.; Yuan, Z. PIV Test and Analysis on Pump Internal Flow of Torque Converter. J. Jiangsu Univ. (Nat. Sci. Ed.) 2014, 3, 274–278. [Google Scholar]
- Chai, B.; Wang, Y.; Ma, W.; Wang, W. Test and analysis of internal flow field in turbine of hydrodynamic torque converter based on particle image velocimetry. Trans. Chin. Soc. Agric. Eng. 2015, 12, 92–98. [Google Scholar]
- Chai, B.; Liu, C.; Wang, W. Extraction of internal flow velocity in pump of hydrodynamic torque converter based on Hough transform. J. Drain. Irrig. Mach. Eng. 2014, 4, 283–289. [Google Scholar]
- Wu, G.Q.; Chen, J.; Zhu, W.J. Performance Analysis and Improvement of Flat Torque Converters Using DOE Method. Chin. J. Mech. Eng. 2018, 31, 1–9. [Google Scholar] [CrossRef]
- Chen, M.; Guo, X.; Tan, G.; Pei, X.; Zhang, W. Effects of blade lean angle on a hydraulic retarder. Adv. Mech. Eng. 2016, 8, 1–9. [Google Scholar] [CrossRef]
- Li, W.; Wang, A.; Li, X.; Zhang, Q. Performance optimization of the design space of torque converter’s blade angle under the condition of driving cycle. Harbin Gongcheng Daxue Xuebao/J. Harbin Eng. Univ. 2017, 38, 1781. [Google Scholar]
- Pan, X.; Xinyuan, C.; Hongjun, S.; Jiping, Z.; Lin, W.; Huichao, G. Effect of the Blade Shaped by Joukowsky Airfoil Transformation on the Characteristics of the Torque Converter. Proc. Inst. Mech. Eng. 2021, 235, 3314–3321. [Google Scholar] [CrossRef]
- Pan, X.; Xinyuan, C.; Jianghong, D.; Liangcai, Z.; Feng, Z. Application of slotted blade in the improvement of turbomachinery performance. AIP Adv. 2021, 11, 045017. [Google Scholar] [CrossRef]
- Kunisaki, Y.; Murata, S.; Tanaka, Y. Internal Flow Structure of Torque Converter Analyzed with Dynamic Mode Decomposition under Steady Driving Condition. Int. J. Automot. Eng. 2020, 11, 108. [Google Scholar] [CrossRef]
- Hempy, A.A. Transient Maneuvers and Pressure Analysis on an Automotive Torque Converter. Master’s Thesis, Michigan Technological University, Houghton, MI, USA, 2021. [Google Scholar]
- Rivera, E.D.J.; Woodland, M.; Robinette, D.; Blough, J.; Anderson, C.; Frait, S.; Devendran, R. Torque and Pressure CFD Correlation of a Torque Converter. SAE Int. J. Passeng. Cars Mech. Syst. 2019, 12, 157–168. [Google Scholar]
- De Jesus Rivera, E. Pressure Measurements Inside Multiple Cavities of a Torque Converter and CFD Correlation. Ph.D. Thesis, Michigan Technological University, Houghton, MI, USA, 2018. [Google Scholar]
- Huang, J.; Wu, G.; Wang, L. Analysis and optimization of axial force in torque converter. J. Jiamusi Univ. (Nat. Sci. Ed.) 2012, 30, 6–9. [Google Scholar]
- Gu, X. CFD Calculation and Investigation on Axial Force in a Torque Converter. Master’s Thesis, Harbin Institute of Technology, Harbin, China, 2015. [Google Scholar]
- Zhang, H. Research on Axial Force of High Speed Ratio Adjustable Hydraulic Torque Converter. Master’s Thesis, Harbin Institute of Technology, Harbin, China, 2018. [Google Scholar]
- Guo, M.; Liu, C.; Zhang, J.; Liu, S.; Ke, Z.; Yan, Q.; Khoo, B.C. Parametric analysis of the effects of blade exit angle on the cavitation characteristics in a hydraulic torque converter. Phys. Fluids 2022, 34, 053309. [Google Scholar] [CrossRef]
- Menter, F.R.; Kuntz, M.; Langtry, R. Ten years of industrial experience with the SST turbulence model. Heat Mass Transf. 2003, 4, 625–632. [Google Scholar]
- Zhang, J.; Li, J.Y.; Wang, T.; Qian, Z.S. Influence of nozzle structure on the flow field of the prestage of nozzle flapper servo valve. Int. J. Hydromechatronics 2023, 6, 59–75. [Google Scholar] [CrossRef]
- Guo, Y.; Chen, K.; Li, Y.; Chen, S.; Yuan, H.; Wang, Y.; Li, D. Analysis of conjugate heat transfer characteristics of nozzle in middle-high speed marine diesel engine. Int. J. Hydromechatronics 2022, 5, 124–135. [Google Scholar] [CrossRef]

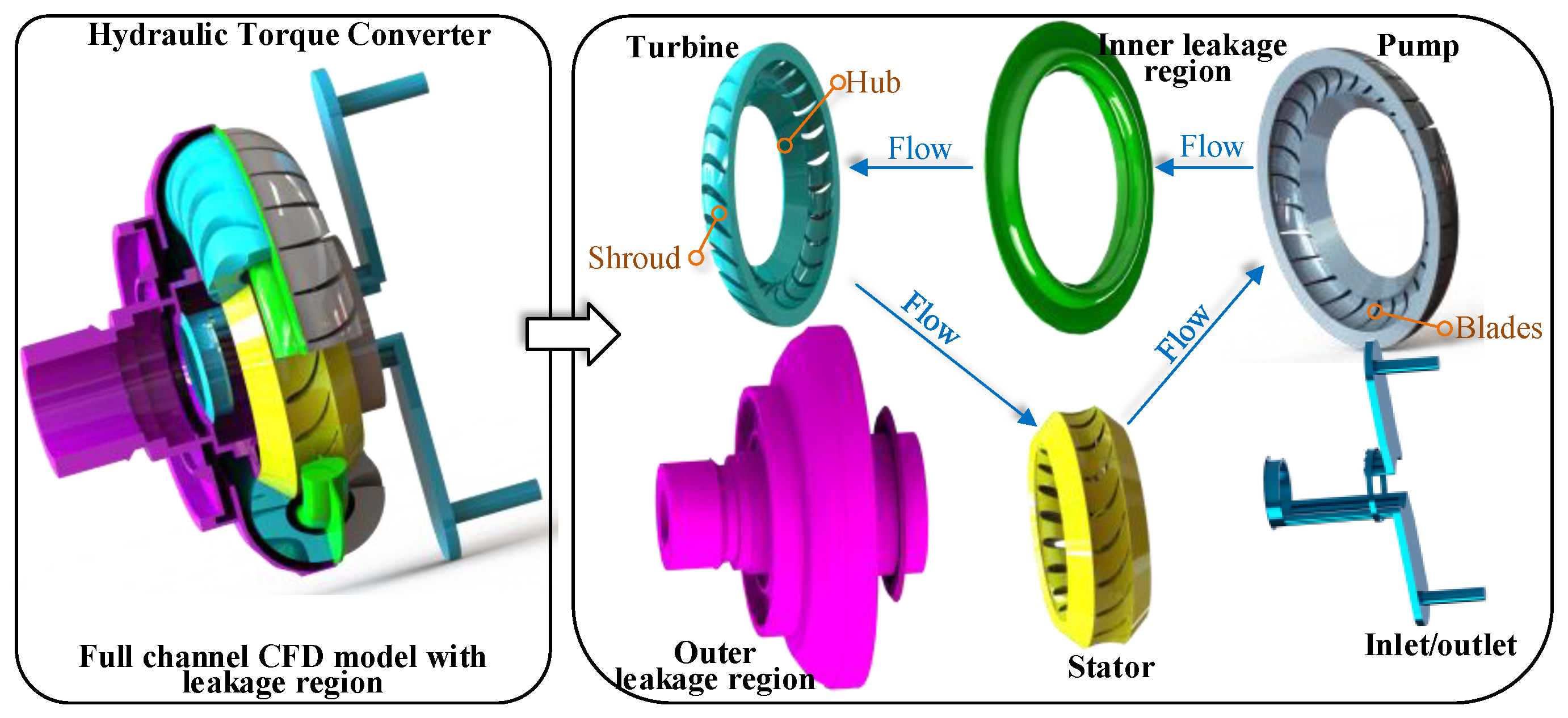
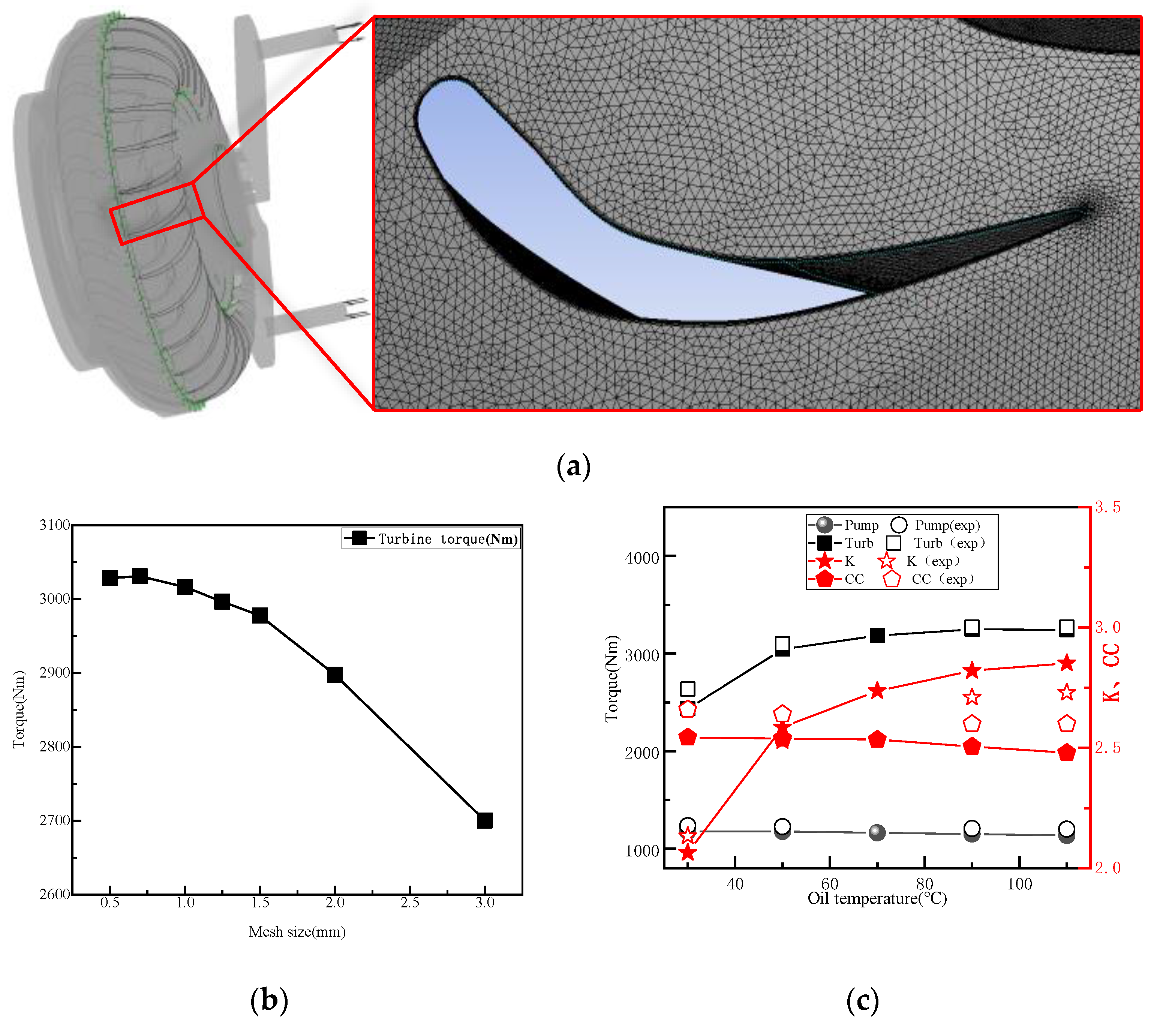


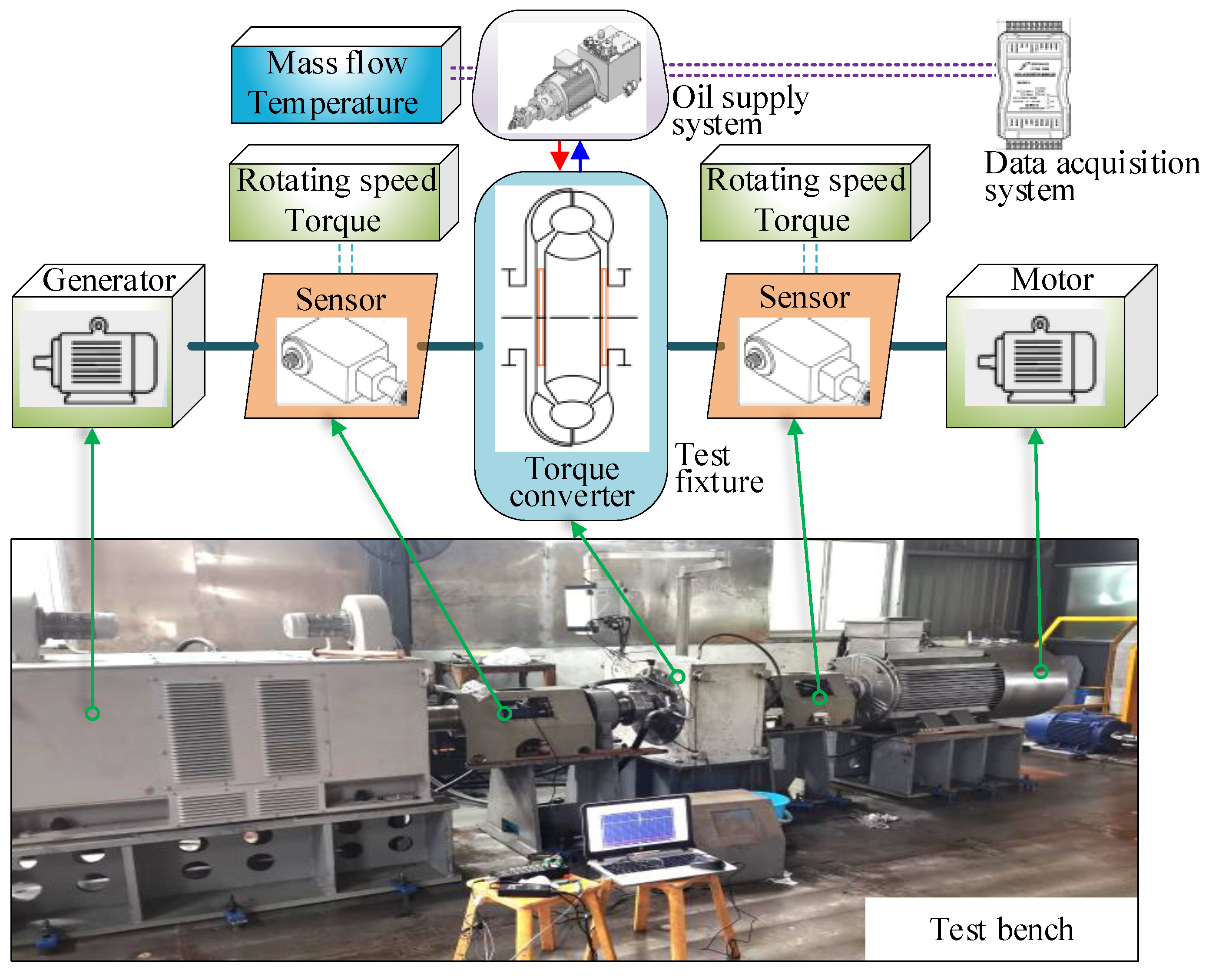

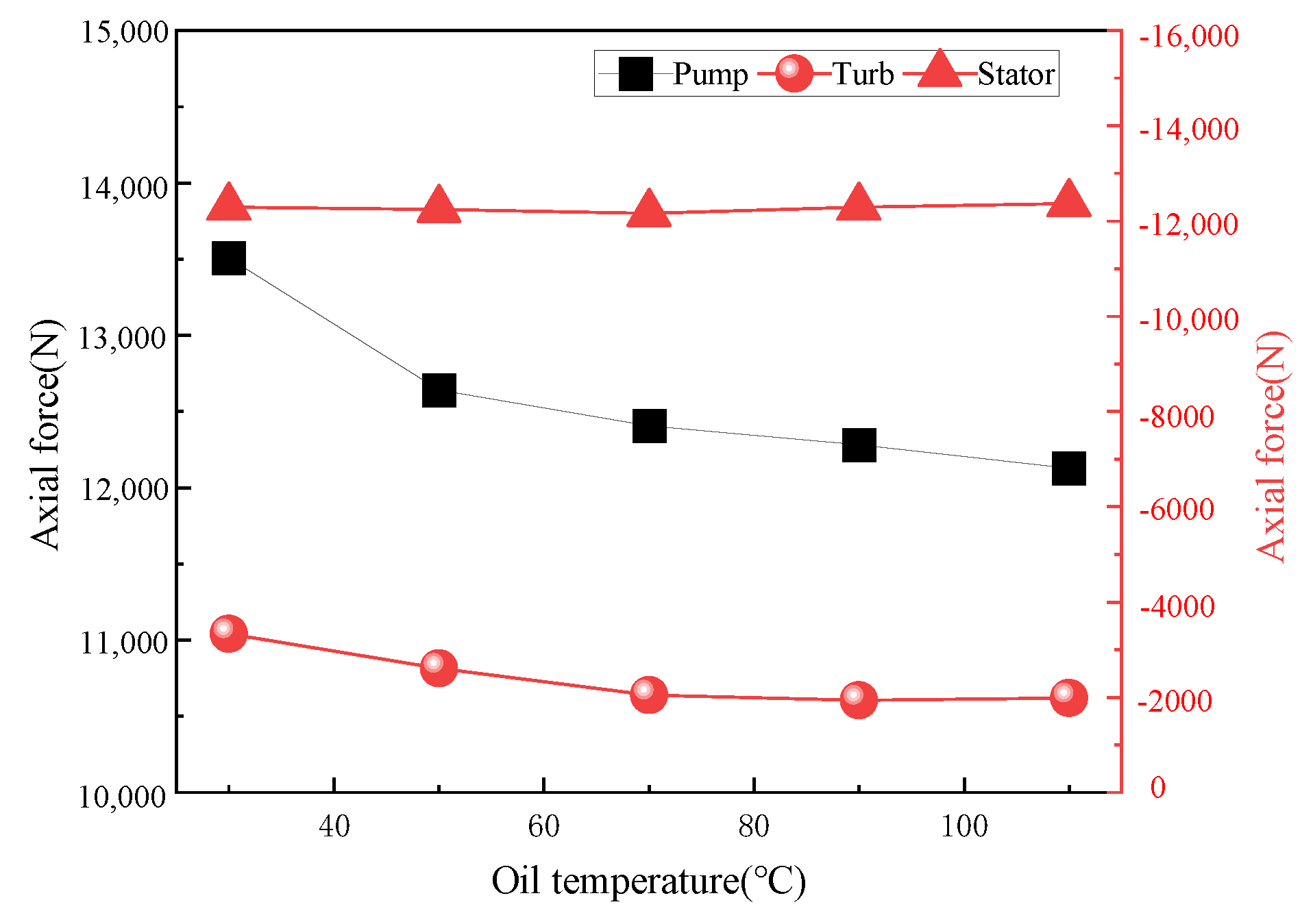
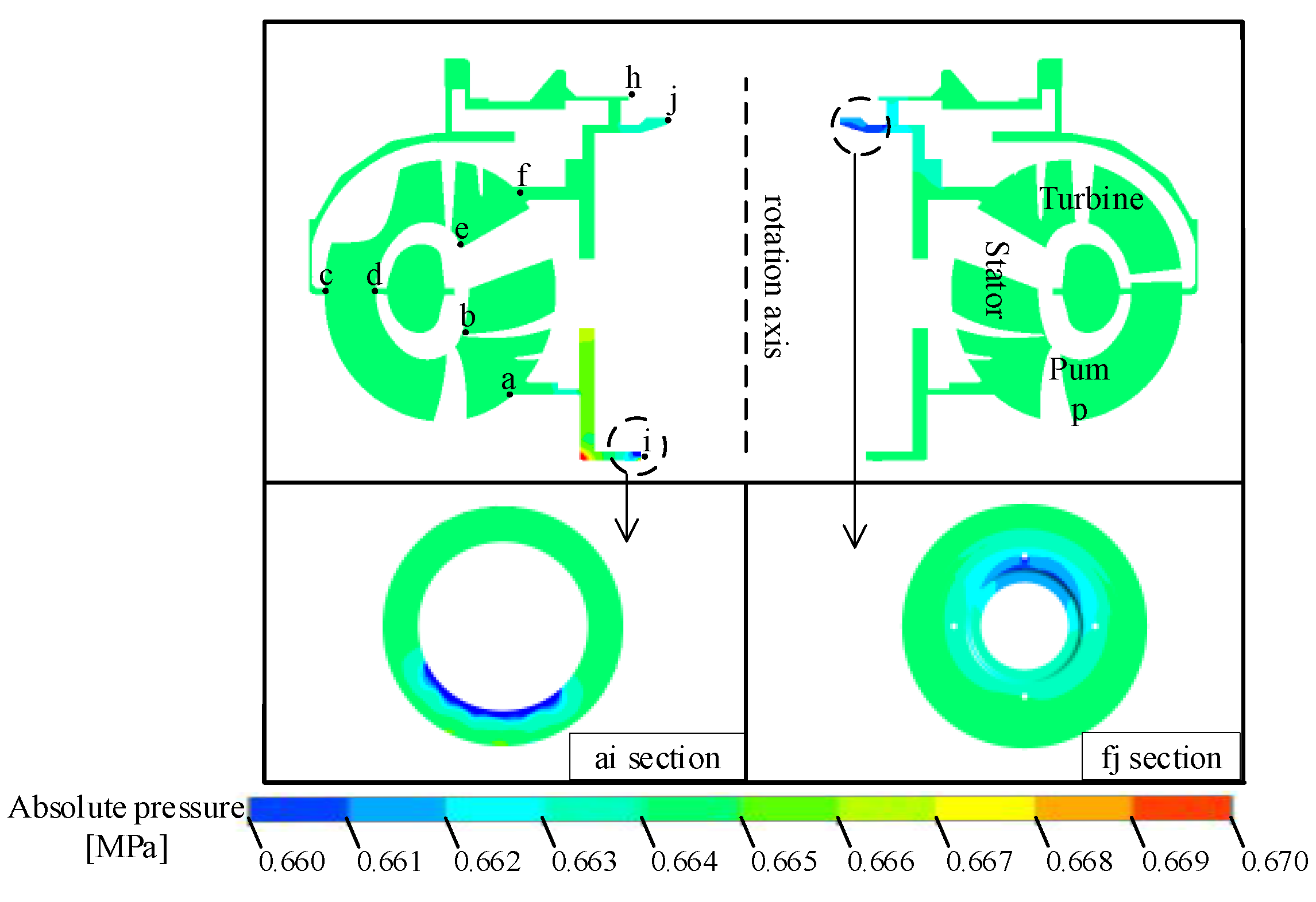



| Analysis Step | Axial Force Simulation of the Torque Converter |
|---|---|
| Analysis type | Steady-state |
| Advection scheme | High resolution scheme |
| Interface model | Frozen rotor |
| Time step | Auto timescale |
| Step number | 400 |
| Convergence targe | RMS 1 × 10−5 |
| Turbulence model | SST (Shear Stress Transfer) |
| Pump status | 1800 rpm |
| Speed ratio | 0 |
| Stator status | Stationary |
| Boundary details | No-slip and smooth wall |
| Inlet conditions | Static pressure |
| Outlet conditions | Mass flow rate |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, C.; Jin, C.; Guo, M.; Yan, Q.; Wei, W. Research on the Influence of Charging Oil Conditions on the Axial Force of Hydraulic Torque Converter. Machines 2023, 11, 730. https://doi.org/10.3390/machines11070730
Liu C, Jin C, Guo M, Yan Q, Wei W. Research on the Influence of Charging Oil Conditions on the Axial Force of Hydraulic Torque Converter. Machines. 2023; 11(7):730. https://doi.org/10.3390/machines11070730
Chicago/Turabian StyleLiu, Cheng, Chen Jin, Meng Guo, Qingdong Yan, and Wei Wei. 2023. "Research on the Influence of Charging Oil Conditions on the Axial Force of Hydraulic Torque Converter" Machines 11, no. 7: 730. https://doi.org/10.3390/machines11070730
APA StyleLiu, C., Jin, C., Guo, M., Yan, Q., & Wei, W. (2023). Research on the Influence of Charging Oil Conditions on the Axial Force of Hydraulic Torque Converter. Machines, 11(7), 730. https://doi.org/10.3390/machines11070730







