Development of a Digital Twin for a Hydraulic, Active Seat Suspension System
Abstract
1. Introduction
2. Prototype of an Agricultural Machine Seat Suspension
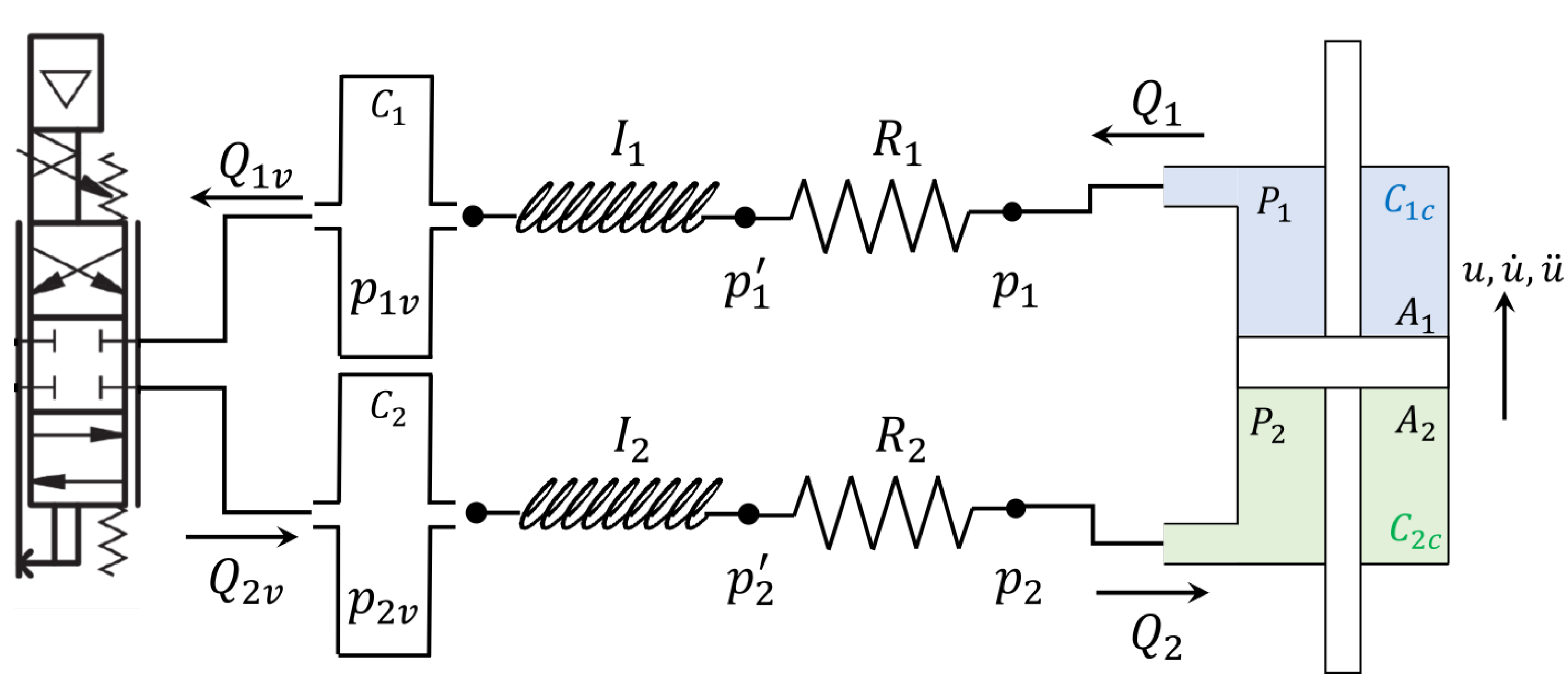
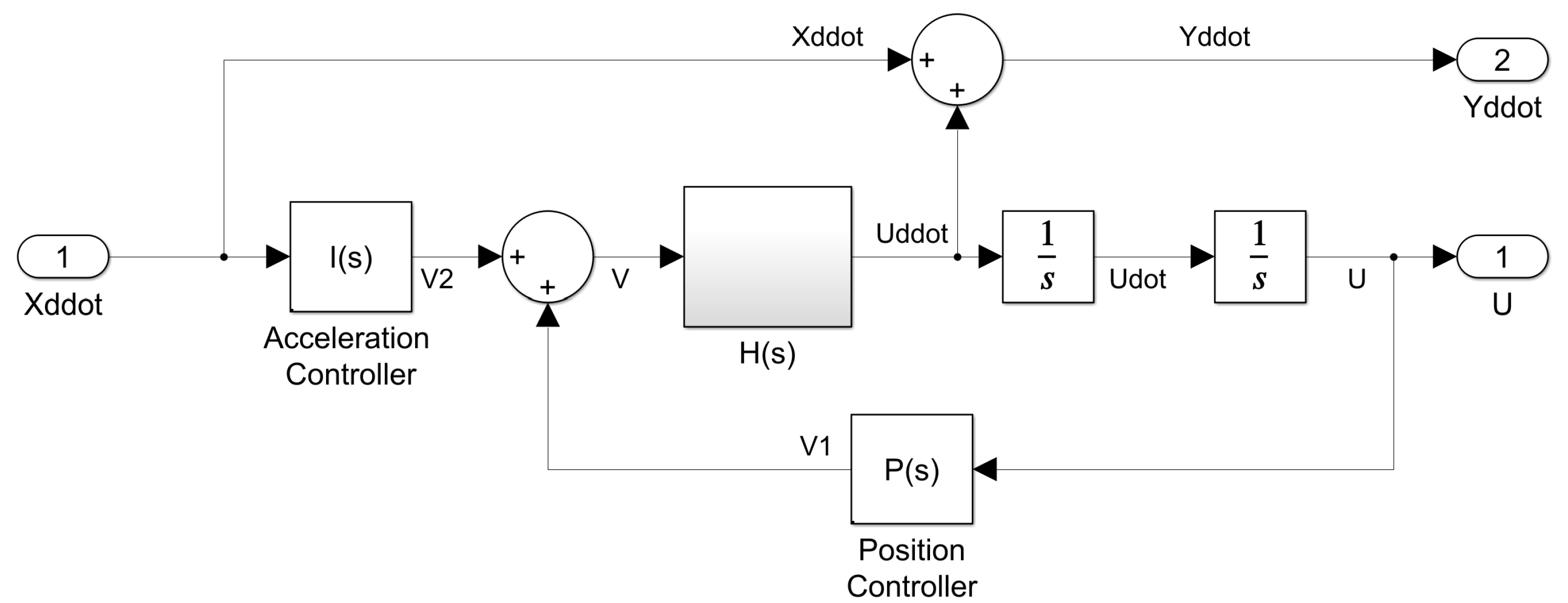
3. Hydraulic Active Suspension System Modeling
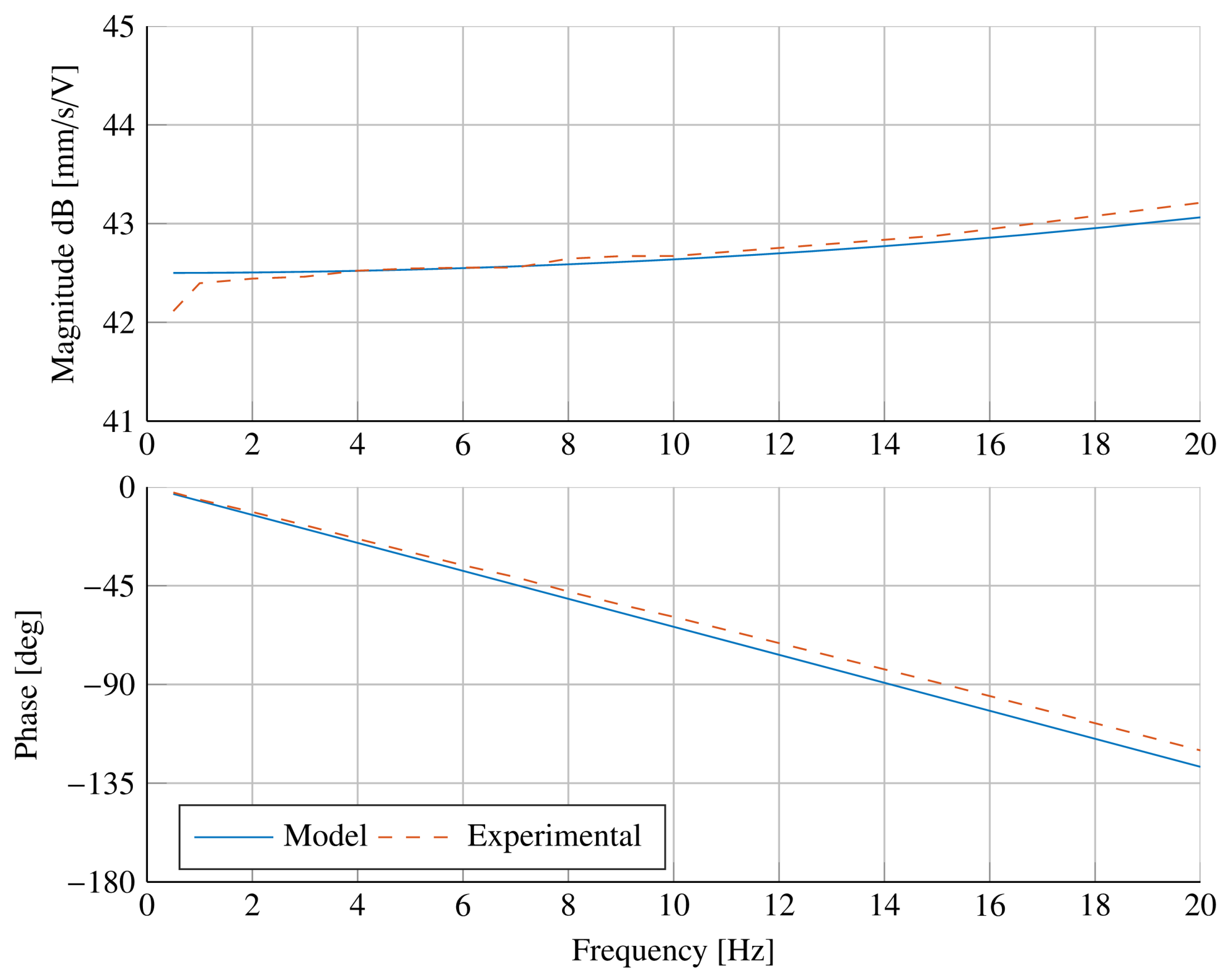
4. Experimental Validation of the Actuation System Model
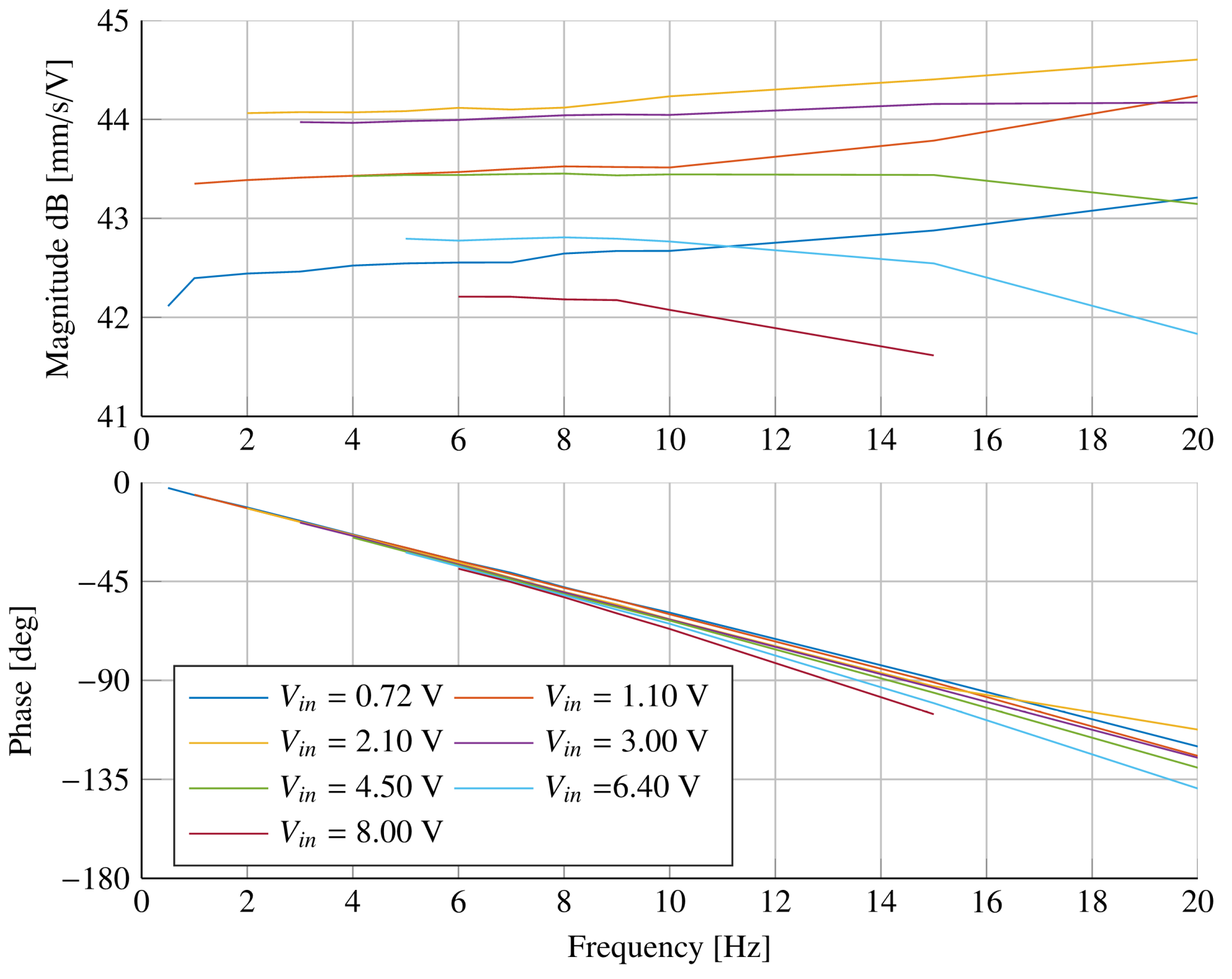
4.1. Test
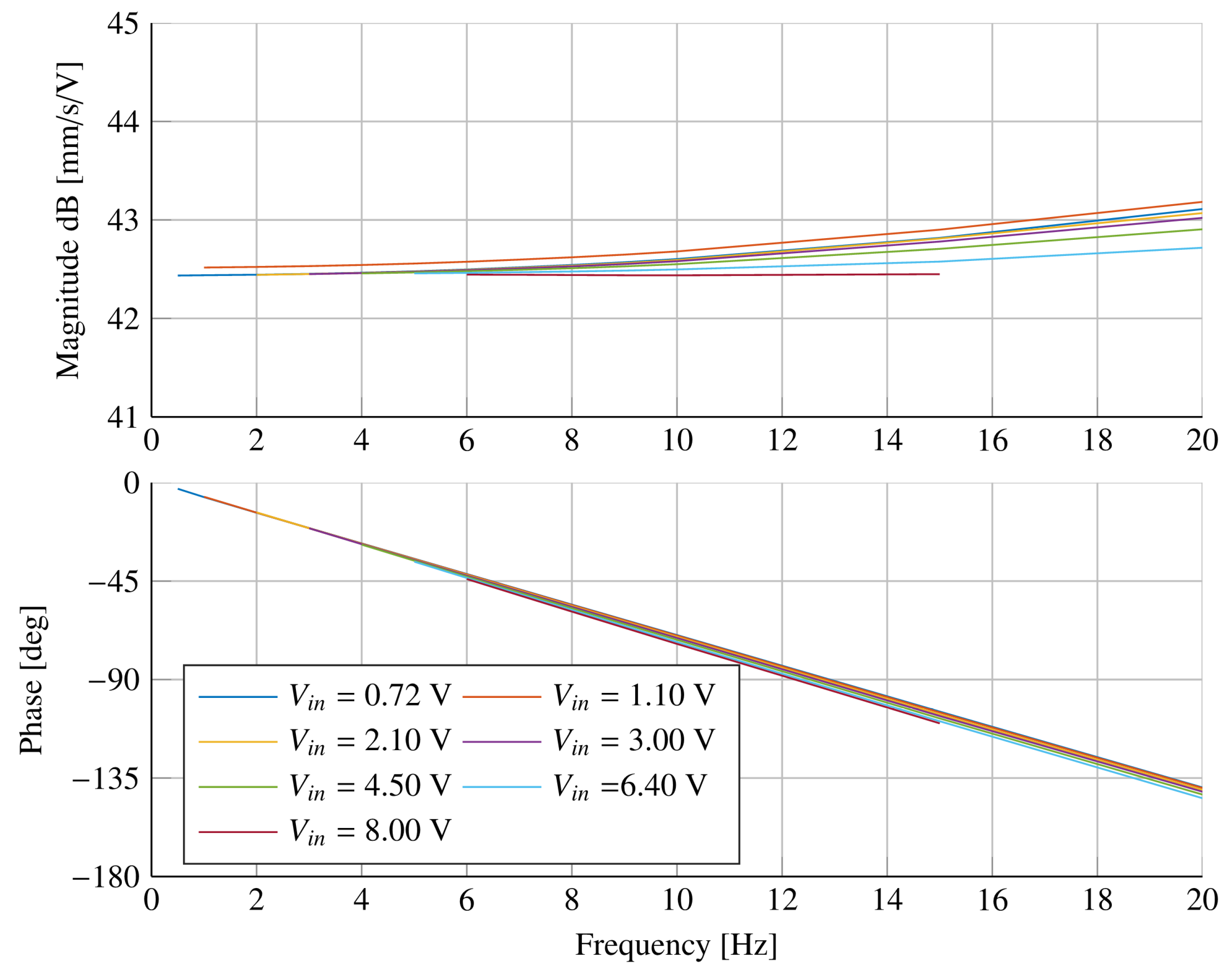
4.2. Model Adjustment
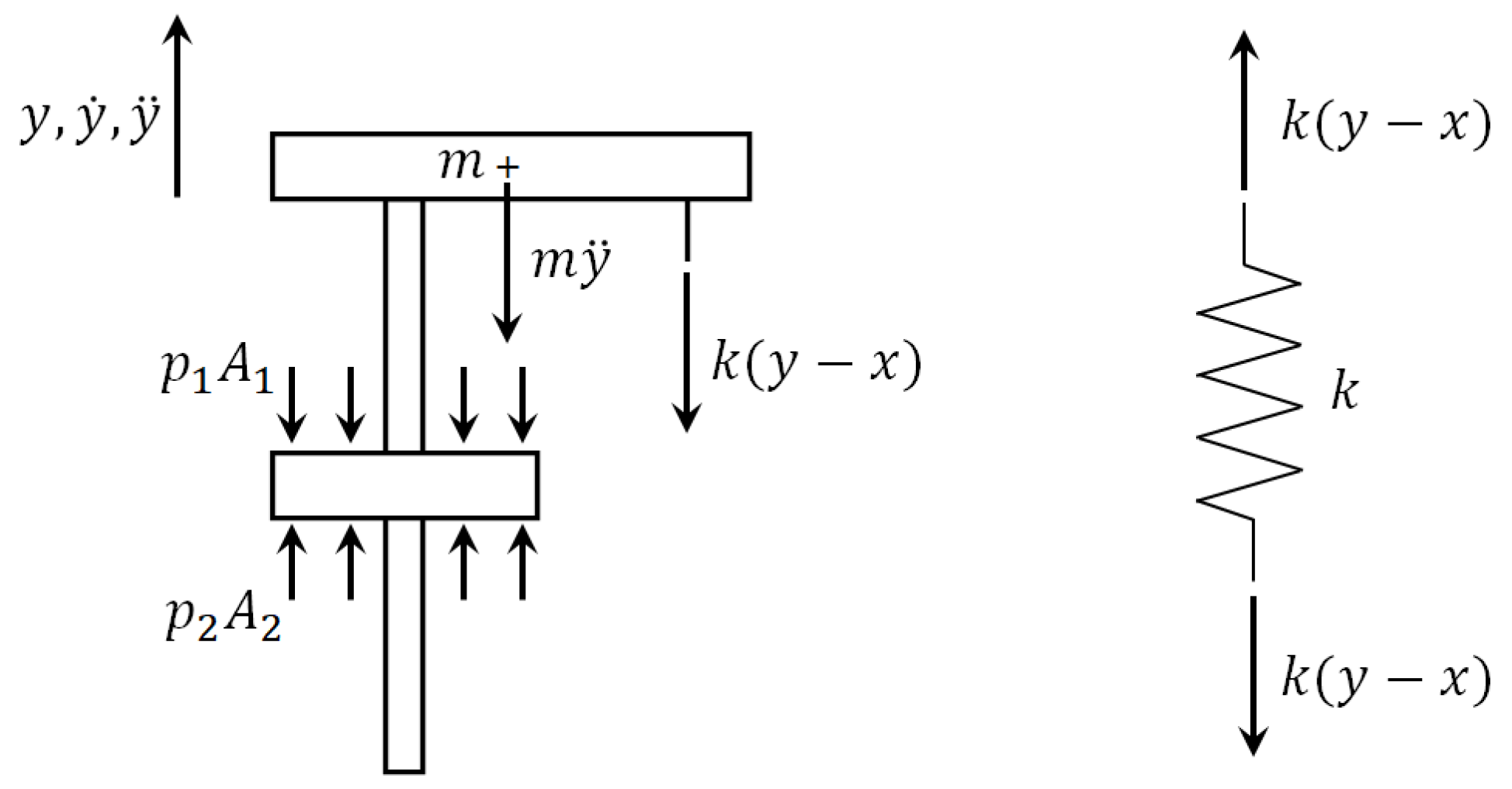
5. Simplified Model
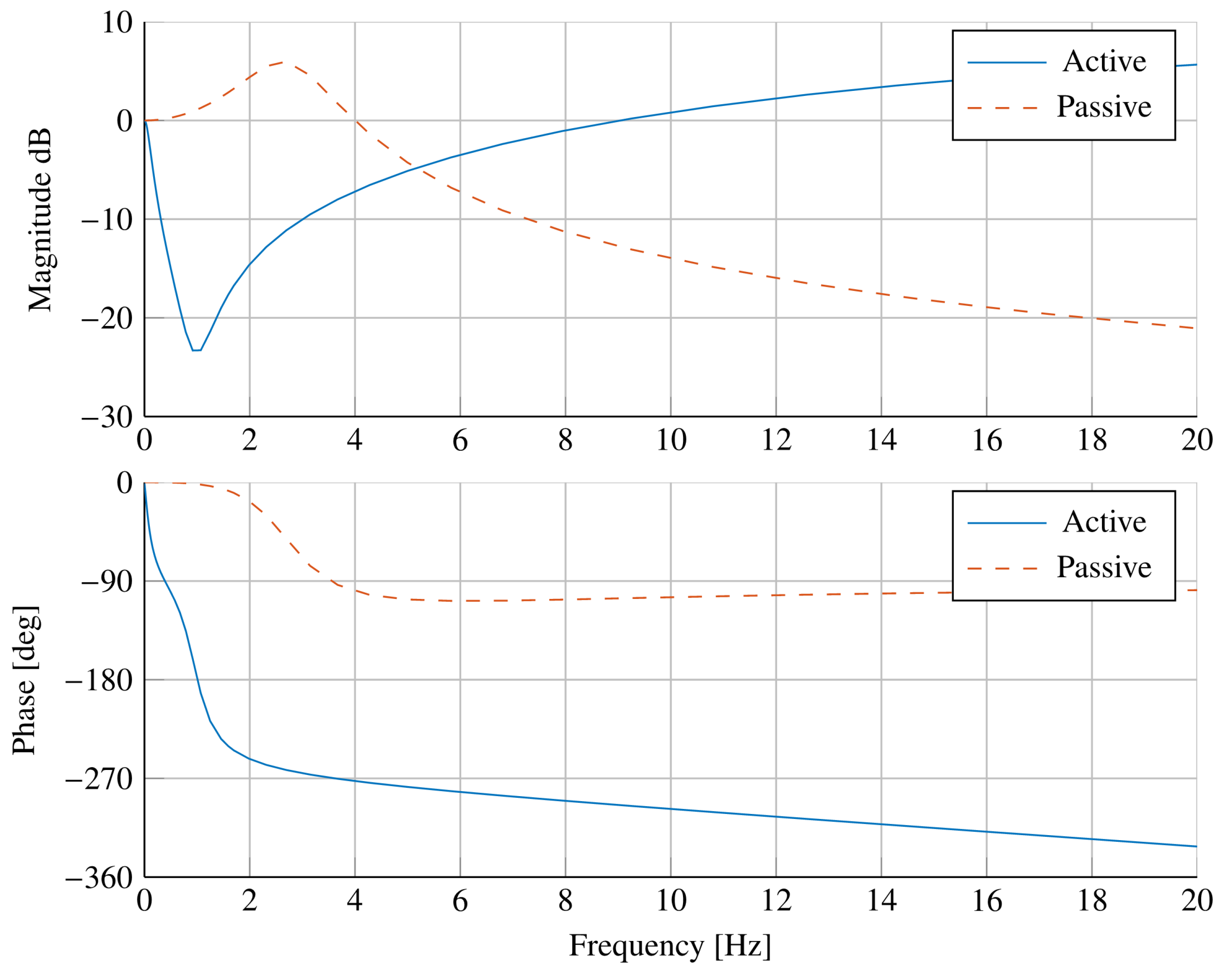
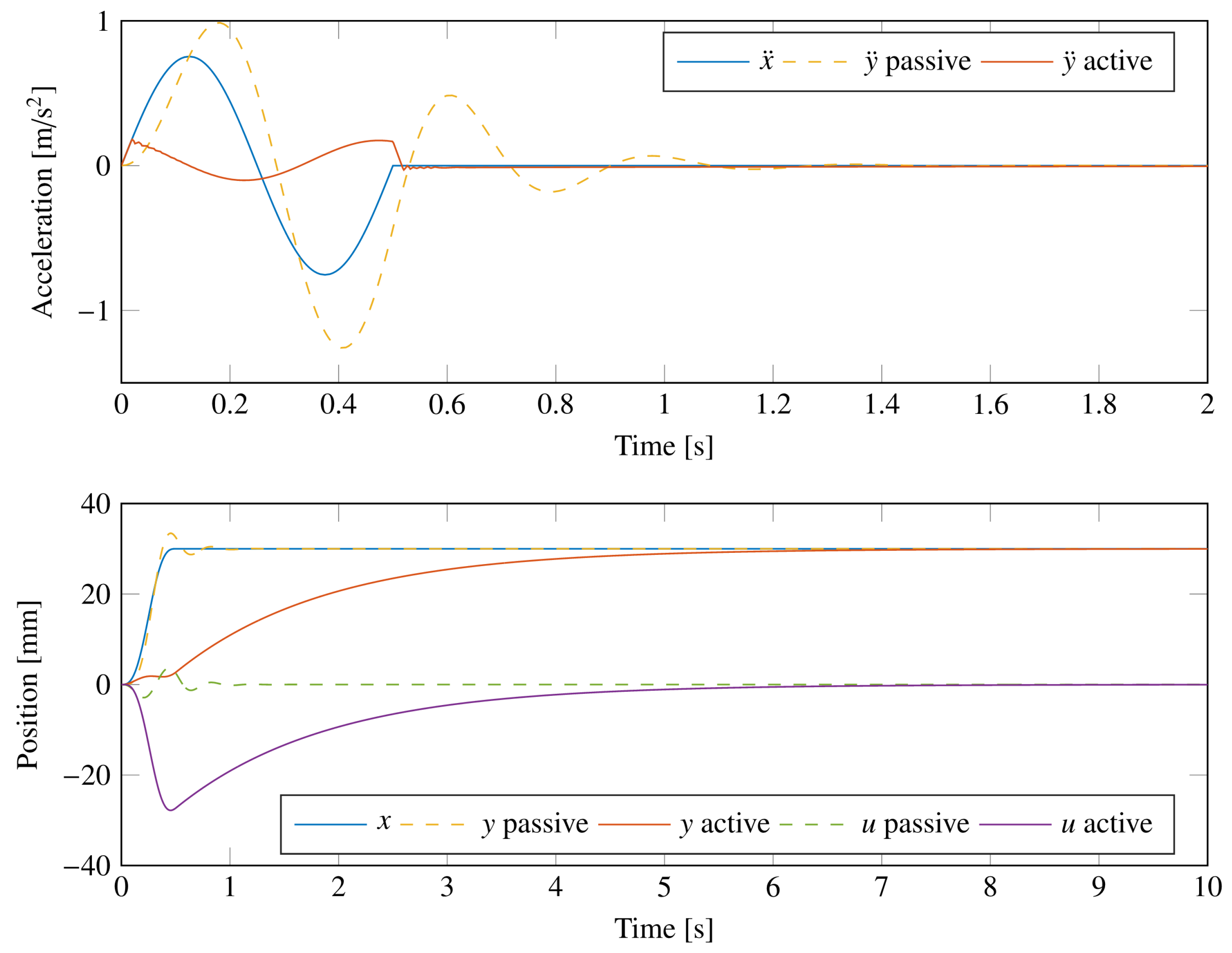
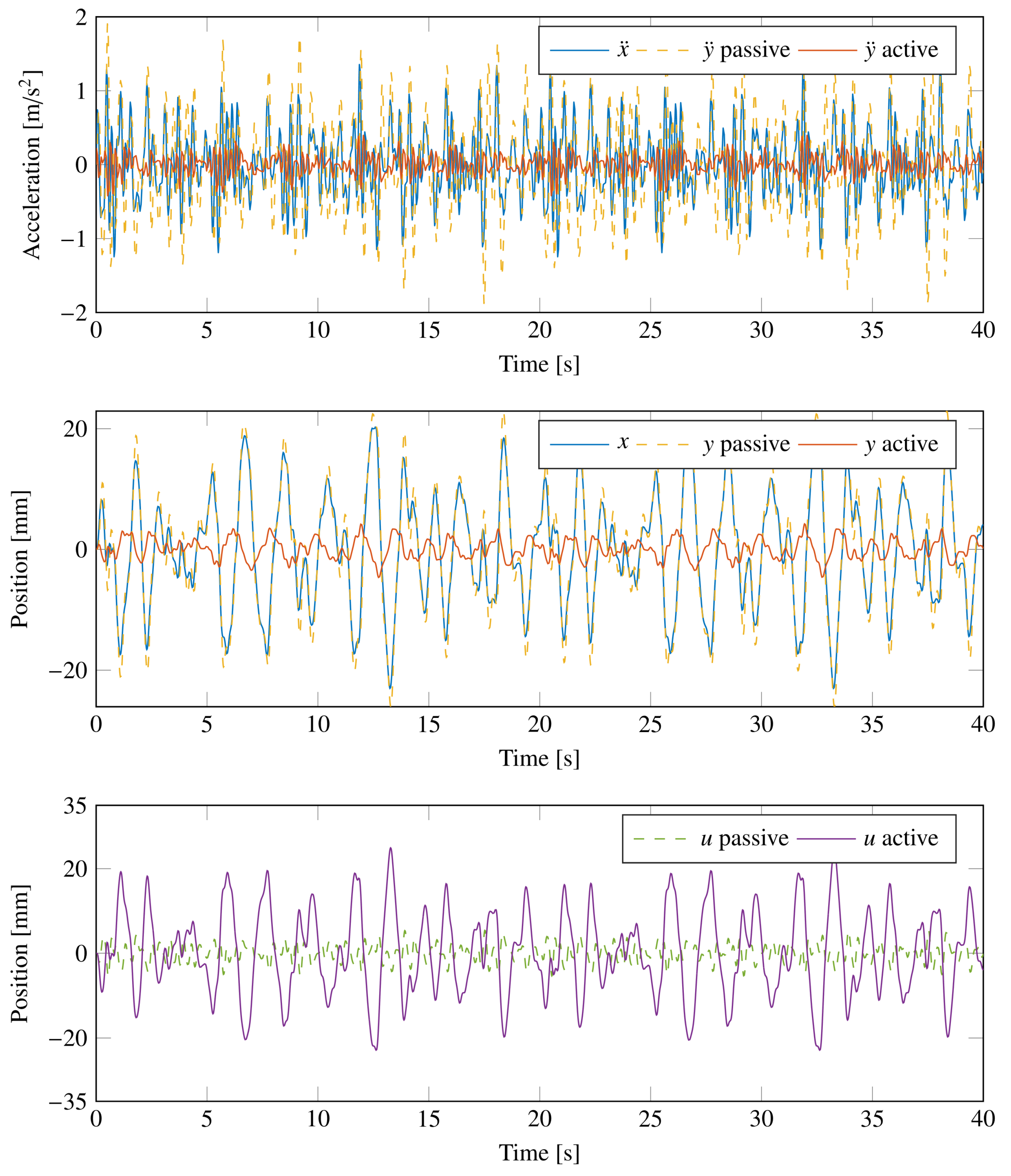
6. Control of the Active Suspension System
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zeng, X.; Kociolek, A.M.; Khan, M.I.; Milosavljevic, S.; Bath, B.; Trask, C. Whole body vibration exposure patterns in Canadian prairie farmers. Ergonomics 2017, 60, 1064–1073. [Google Scholar] [CrossRef] [PubMed]
- Stayner, R. Whole-Body Vibration and Shock: A Literature Review: Extension of a Study of Overtravel and Seat Suspensions; University of Southampton, Institute of Sound and Vibration Research: Southampton, UK, 2001. [Google Scholar]
- Kim, J.; Dennerlein, J.; Johnson, P. The effect of a multi-axis suspension on whole body vibration exposures and physical stress in the neck and low back in agricultural tractor applications. Appl. Ergon. 2018, 68, 80–89. [Google Scholar] [CrossRef] [PubMed]
- Boshuizen, H.C.; Bongers, P.M.; Hulshof, C.T. Self-reported back pain in tractor drivers exposed to whole-body vibration. Int. Arch. Occup. Environ. Health 1990, 62, 109–115. [Google Scholar] [CrossRef]
- Bovenzi, M.; Betta, A. Low-back disorders in agricultural tractor drivers exposed to whole-body vibration and postural stress. Appl. Ergon. 1994, 25, 231–241. [Google Scholar] [CrossRef]
- Essien, S.K.; Trask, C.; Khan, M.; Boden, C.; Bath, B. Association between whole-body vibration and low-back disorders in farmers: A scoping review. J. Agromed. 2018, 23, 105–120. [Google Scholar] [CrossRef] [PubMed]
- Heidarian, A.; Wang, X. Review on Seat Suspension System Technology Development. Appl. Sci. 2019, 9, 2834. [Google Scholar] [CrossRef]
- Al-Ashmori, M.; Wang, X. A Systematic Literature Review of Various Control Techniques for Active Seat Suspension Systems. Appl. Sci. 2020, 10, 1148. [Google Scholar] [CrossRef]
- Zhao, Y.; Wang, X. A Review of Low-Frequency Active Vibration Control of Seat Suspension Systems. Appl. Sci. 2019, 9, 3326. [Google Scholar] [CrossRef]
- ISO 2631-1; Mechanical Vibration and Shock—Evaluation of Human Exposure to Whole-Body Vibration General Requirements. International Organization for Standardization: Geneva, Switzerland, 1997.
- Lines, J. Ride vibration of agricultural tractors: Transfer functions between the ground and the tractor body. J. Agric. Eng. Res. 1987, 37, 81–91. [Google Scholar] [CrossRef]
- Lewis, C.; Griffin, M. Evaluating the vibration isolation of soft seat cushions using an active anthropodynamic dummy. J. Sound Vib. 2002, 253, 295–311. [Google Scholar] [CrossRef]
- Commission Delegated Regulation (EU) No 1322/2014 of 19 September 2014 Supplementing and Amending Regulation (EU) No 167/2013 of the European Parliament and of the Council with Regard to Vehicle Construction and General Requirements for the Approval of Agricultural and Forestry Vehicles. 2018. Available online: http://data.europa.eu/eli/reg_del/2014/1322/oj (accessed on 26 June 2018).
- Zehsaz, M.; Sadeghi, M.H.; Ettefagh, M.M.; Shams, F. Tractor cabin’s passive suspension parameters optimization via experimental and numerical methods. J. Terramech. 2011, 48, 439–450. [Google Scholar] [CrossRef]
- Liao, X.; Zhang, N.; Du, X.; Zhang, W. Theoretical Modeling and Vibration Isolation Performance Analysis of a Seat Suspension System Based on a Negative Stiffness Structure. Appl. Sci. 2021, 11, 6928. [Google Scholar] [CrossRef]
- Hostens, I.; Deprez, K.; Ramon, H. An improved design of air suspension for seats of mobile agricultural machines. J. Sound Vib. 2004, 276, 141–156. [Google Scholar] [CrossRef]
- Soliman, A.; Kaldas, M. Semi-active suspension systems from research to mass-market—A review. J. Low Freq. Noise Vib. Act. Control 2021, 40, 1005–1023. [Google Scholar] [CrossRef]
- Nieto, A.; Morales, A.; Trapero, J.; Chicharro, J.; Pintado, P. An adaptive pneumatic suspension based on the estimation of the excitation frequency. J. Sound Vib. 2011, 330, 1891–1903. [Google Scholar] [CrossRef]
- Stein, G.; Ballo, I. Active Vibration Control System for the Driver’s Seat for Off-Road Vehicles. Veh. Syst. Dyn. 1991, 20, 57–78. [Google Scholar] [CrossRef]
- Talib, M.H.A.; Mat Darns, I.Z. Self-tuning PID controller for active suspension system with hydraulic actuator. In Proceedings of the 2013 IEEE Symposium on Computers & Informatics (ISCI), Langkawi, Malaysia, 7–9 April 2013; pp. 86–91. [Google Scholar] [CrossRef]
- Quaini, D.; Sazgetdinov, K.; Ivanov, V.; Ferrara, A. Optimization Based Sliding Mode Control in Active Suspensions: Design and Hardware-in-the-Loop Assessment. In Proceedings of the 2020 European Control Conference (ECC), Virtual Event, 12–15 May 2020; pp. 1607–1612. [Google Scholar] [CrossRef]
- East, W.; Turcotte, J.; Plante, J.S.; Julio, G. Experimental assessment of a linear actuator driven by magnetorheological clutches for automotive active suspensions. J. Intell. Mater. Syst. Struct. 2021, 32, 955–970. [Google Scholar] [CrossRef] [PubMed]
- Brunetti, J.; D’Ambrogio, W.; Fregolent, A. Analysis of the vibrations of operators’ seats in agricultural machinery using dynamic substructuring. Appl. Sci. 2021, 11, 4749. [Google Scholar] [CrossRef]
- Antonelli, M.G.; Brunetti, J.; D’ambrogio, W.; Fregolent, A.; Latini, F. Experimental identification of a pneumatic valve-cylinder system for attitude control. In Theoretical and Applied Mechanics—AIMETA 2022; Di Paola, M., Fratini, L., Micari, F., Pirrotta, A., Eds.; Materials Research Proceedings; MRF: Millersville, PA, USA, 2023; Volume 26, pp. 177–182. [Google Scholar] [CrossRef]
- Chen, P.C.; Huang, A.C. Adaptive Multiple-surface Sliding Control of Hydraulic Active Suspension Systems Based on the Function Approximation Technique. J. Vib. Control 2005, 11, 685–706. [Google Scholar] [CrossRef]
- Liu, Y.J.; Zeng, Q.; Liu, L.; Tong, S. An Adaptive Neural Network Controller for Active Suspension Systems with Hydraulic Actuator. IEEE Trans. Syst. Man Cybern. Syst. 2020, 50, 5351–5360. [Google Scholar] [CrossRef]
- Bolton, W. Basic System Models, System Models. In Mechatronics—Electronics Control Systems in Mechanical and Electrical Engineering; Pearson: Harlow, UK, 2015; pp. 413–448. [Google Scholar]





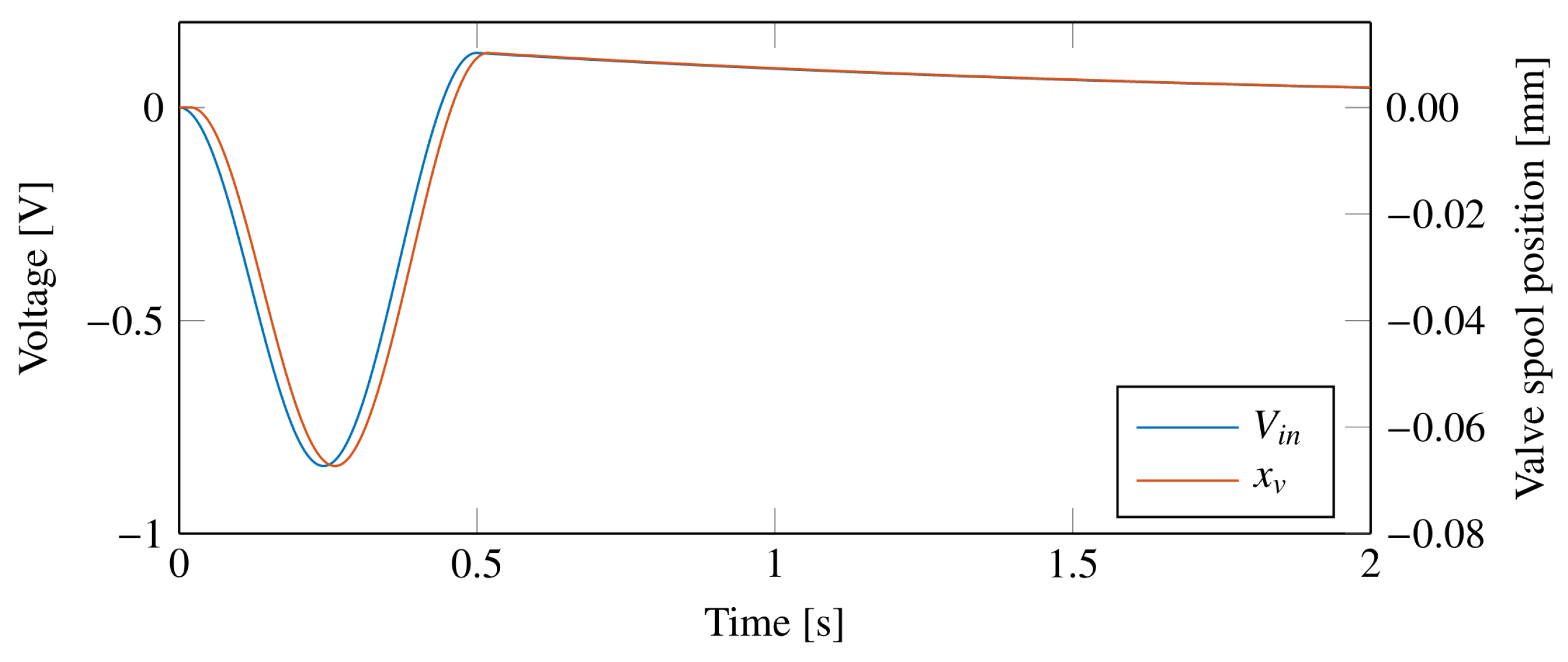

| Amplitude [V] | 0.72 | 1.10 | 2.10 | 3.00 | 4.50 | 6.40 | 8.00 | |
|---|---|---|---|---|---|---|---|---|
| Frequency [Hz] | ||||||||
| 0.5 | X | |||||||
| 1.0 | X | X | ||||||
| 2.0 | X | X | X | |||||
| 3.0 | X | X | X | X | ||||
| 4.0 | X | X | X | X | X | |||
| 5.0 | X | X | X | X | X | X | ||
| 6.0 | X | X | X | X | X | X | X | |
| 7.0 | X | X | X | X | X | X | X | |
| 8.0 | X | X | X | X | X | X | X | |
| 9.0 | X | X | X | X | X | X | X | |
| 10.0 | X | X | X | X | X | X | X | |
| 15.0 | X | X | X | X | X | X | X | |
| 20.0 | X | X | X | X | X | X | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Antonelli, M.G.; Brunetti, J.; D’Ambrogio, W.; Fregolent, A.; Nataletti, P. Development of a Digital Twin for a Hydraulic, Active Seat Suspension System. Machines 2023, 11, 708. https://doi.org/10.3390/machines11070708
Antonelli MG, Brunetti J, D’Ambrogio W, Fregolent A, Nataletti P. Development of a Digital Twin for a Hydraulic, Active Seat Suspension System. Machines. 2023; 11(7):708. https://doi.org/10.3390/machines11070708
Chicago/Turabian StyleAntonelli, Michele Gabrio, Jacopo Brunetti, Walter D’Ambrogio, Annalisa Fregolent, and Pietro Nataletti. 2023. "Development of a Digital Twin for a Hydraulic, Active Seat Suspension System" Machines 11, no. 7: 708. https://doi.org/10.3390/machines11070708
APA StyleAntonelli, M. G., Brunetti, J., D’Ambrogio, W., Fregolent, A., & Nataletti, P. (2023). Development of a Digital Twin for a Hydraulic, Active Seat Suspension System. Machines, 11(7), 708. https://doi.org/10.3390/machines11070708







