Effect of Metal Coating on Displacement of the Silicon Electrothermal V-Shaped Actuator
Abstract
1. Introduction
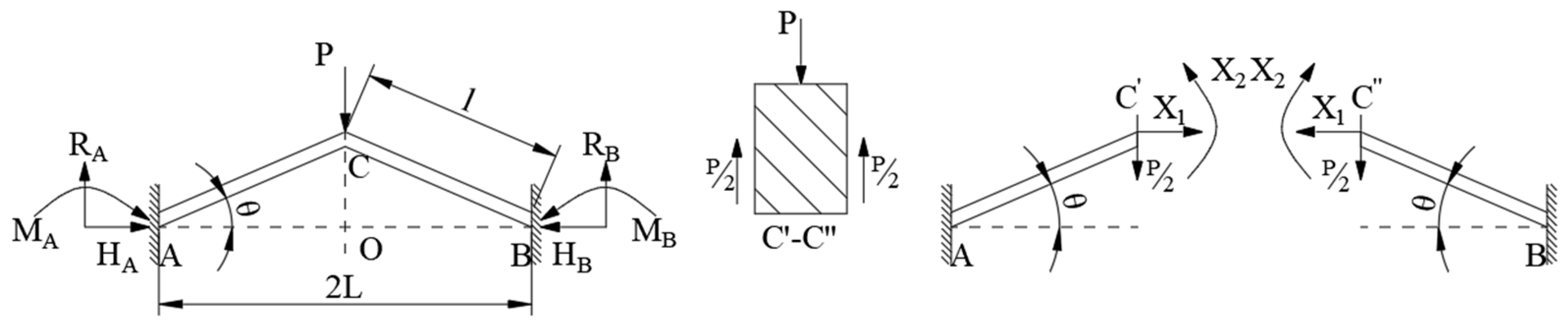
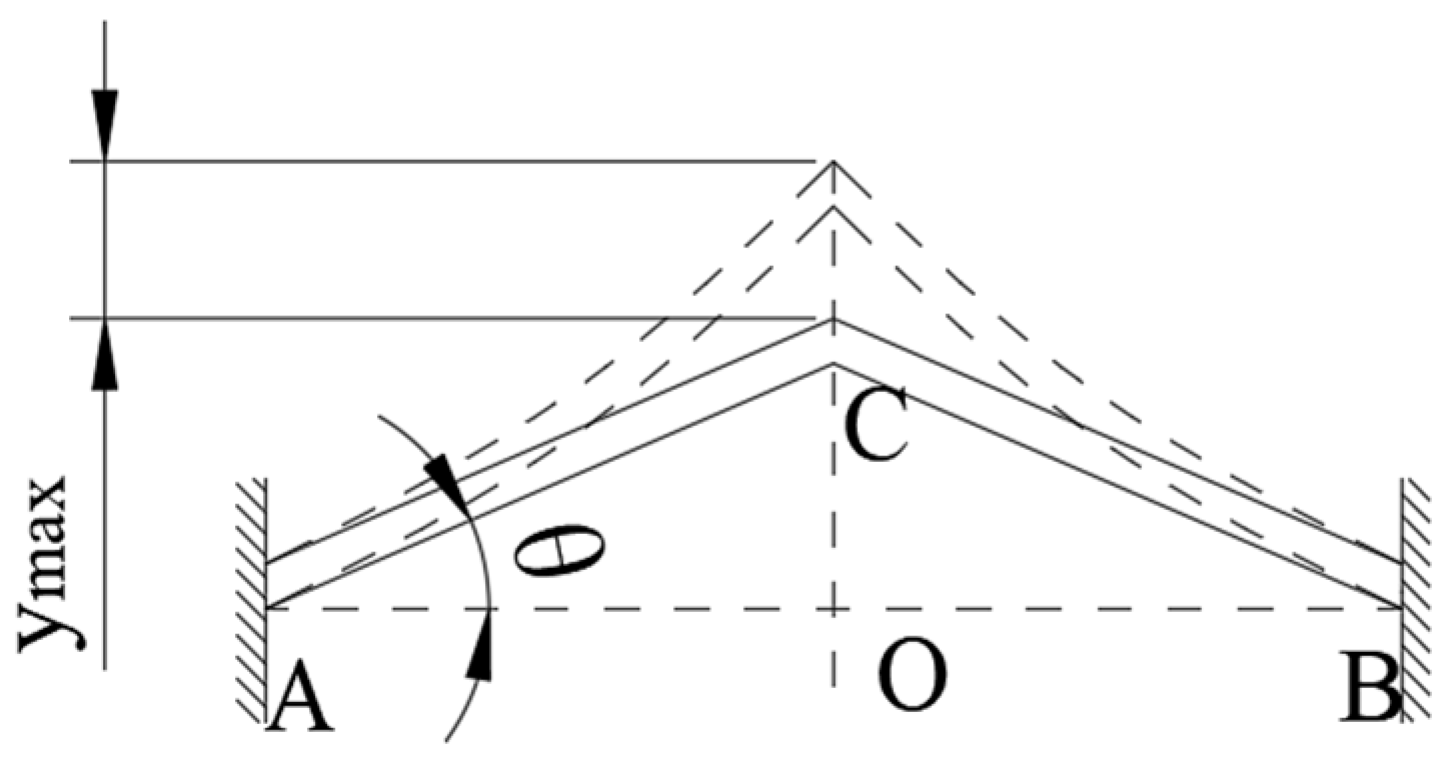
2. Theoretical Analysis
- In , the bending moment at section can be calculated.
- 2.
- In , the bending moment at section can be calculated.
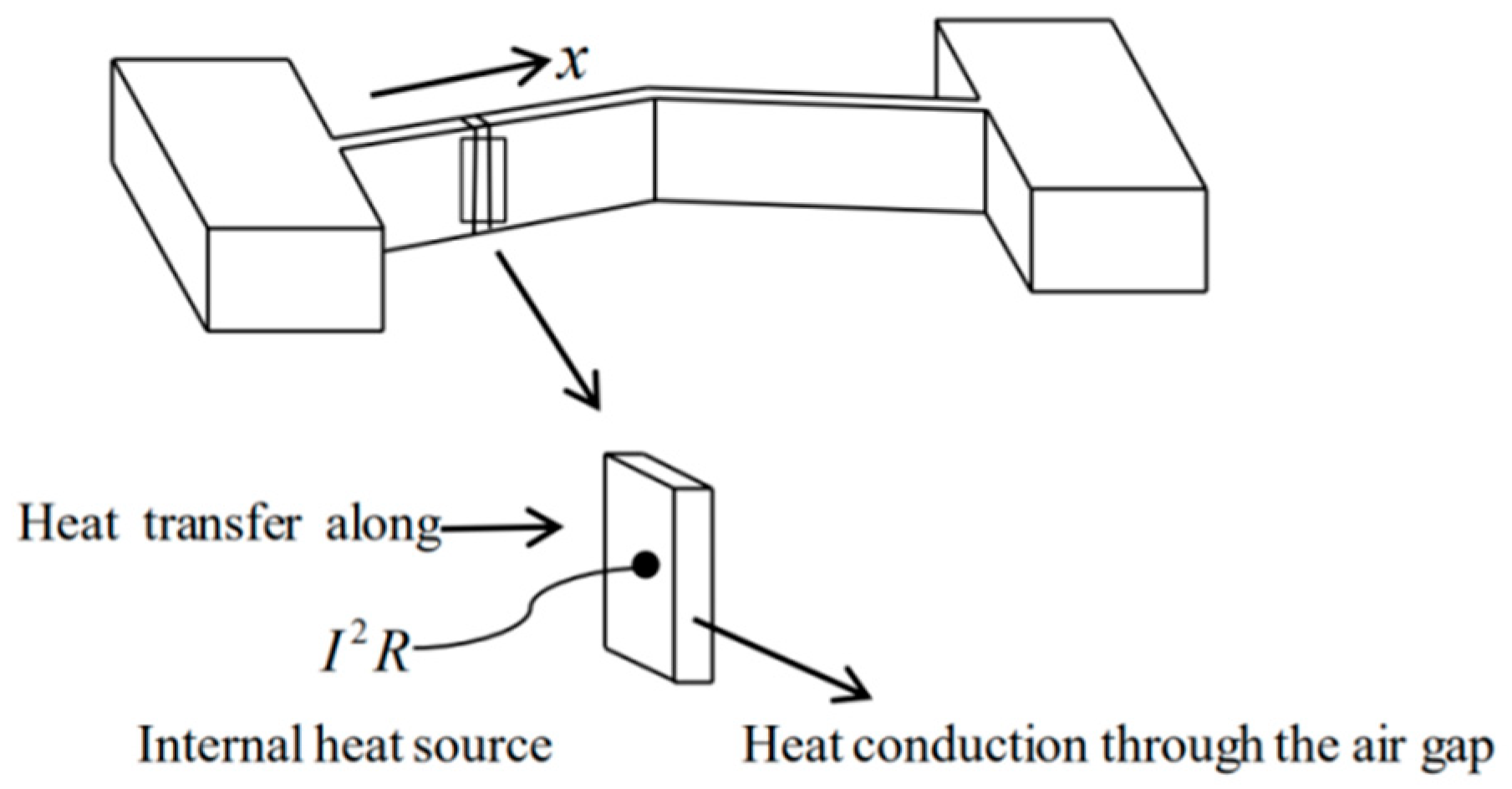
2.1. Silicon EVA Heat Transfer Model without Metal Coating
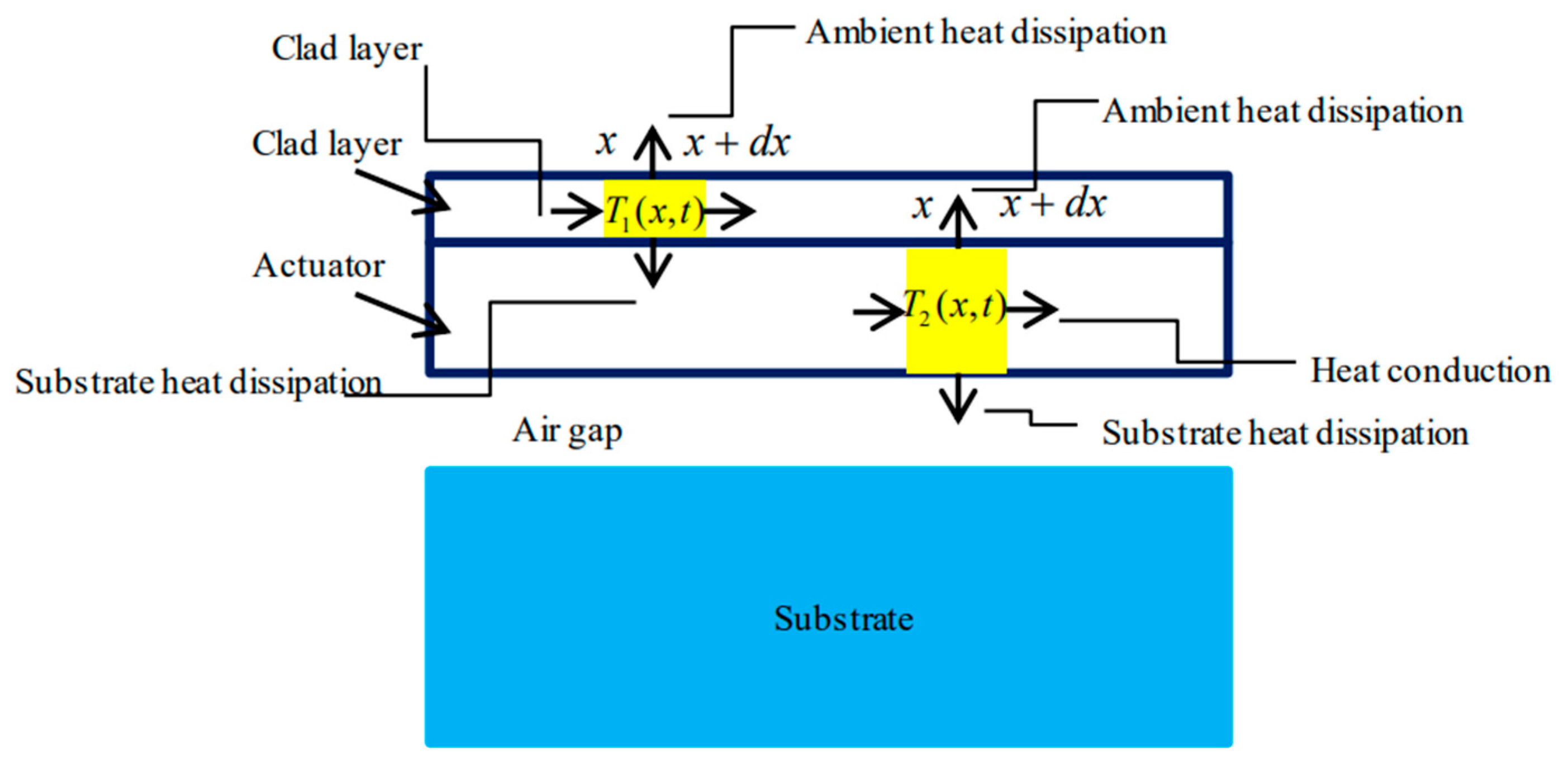
2.2. Heat Transfer Model of Silicon EVA with Metal Coating
3. Results and Discussion
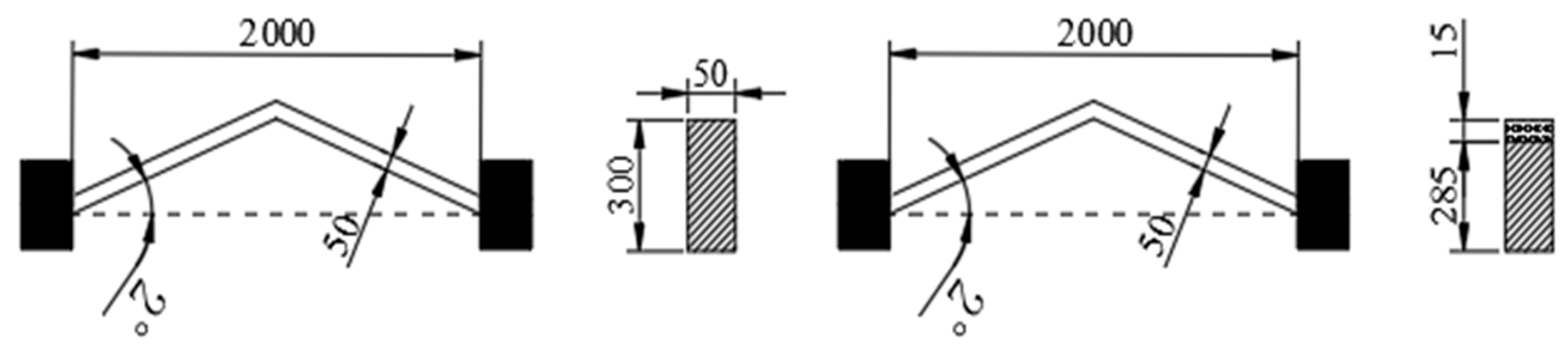
3.1. Simulation Parameter Setting
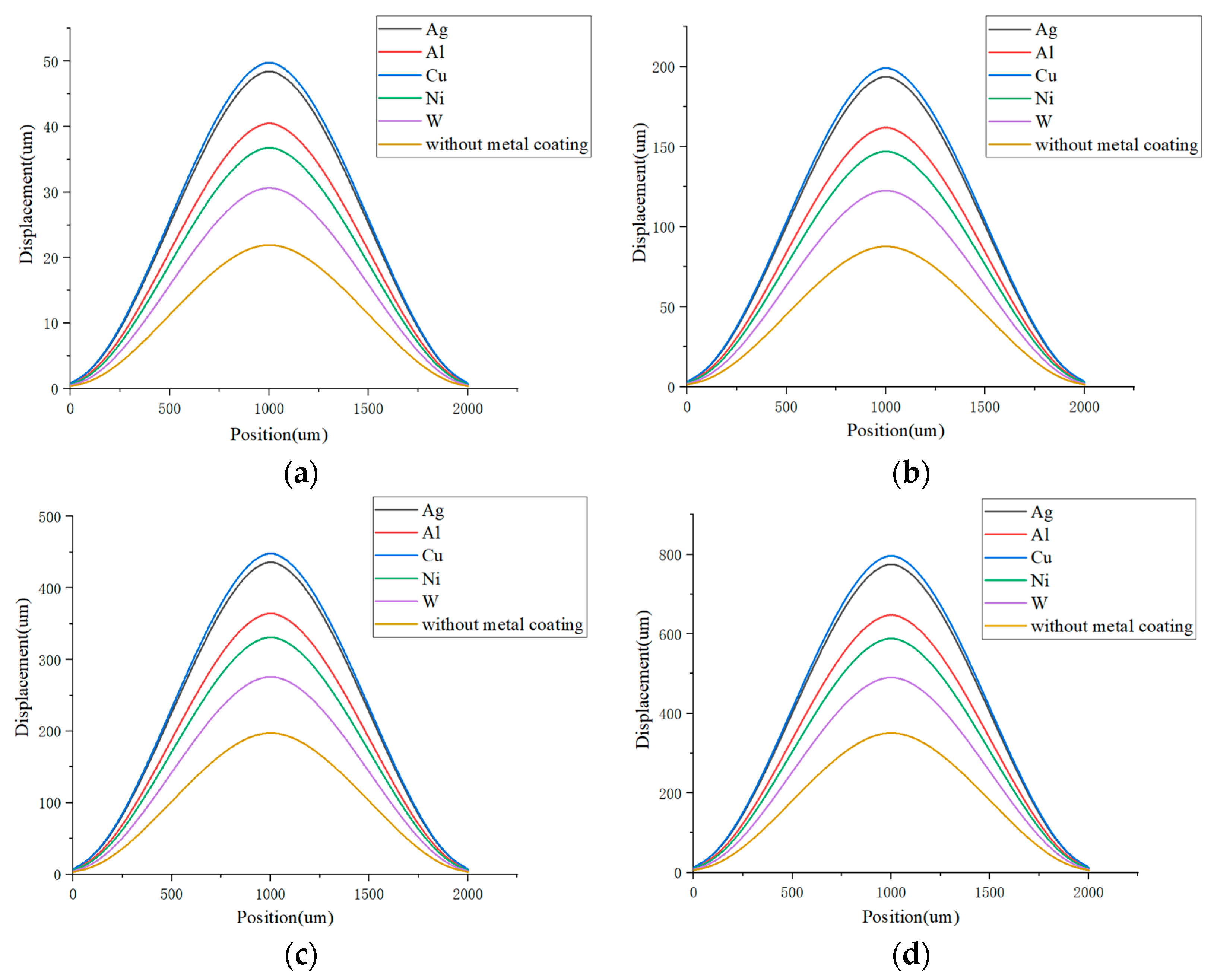
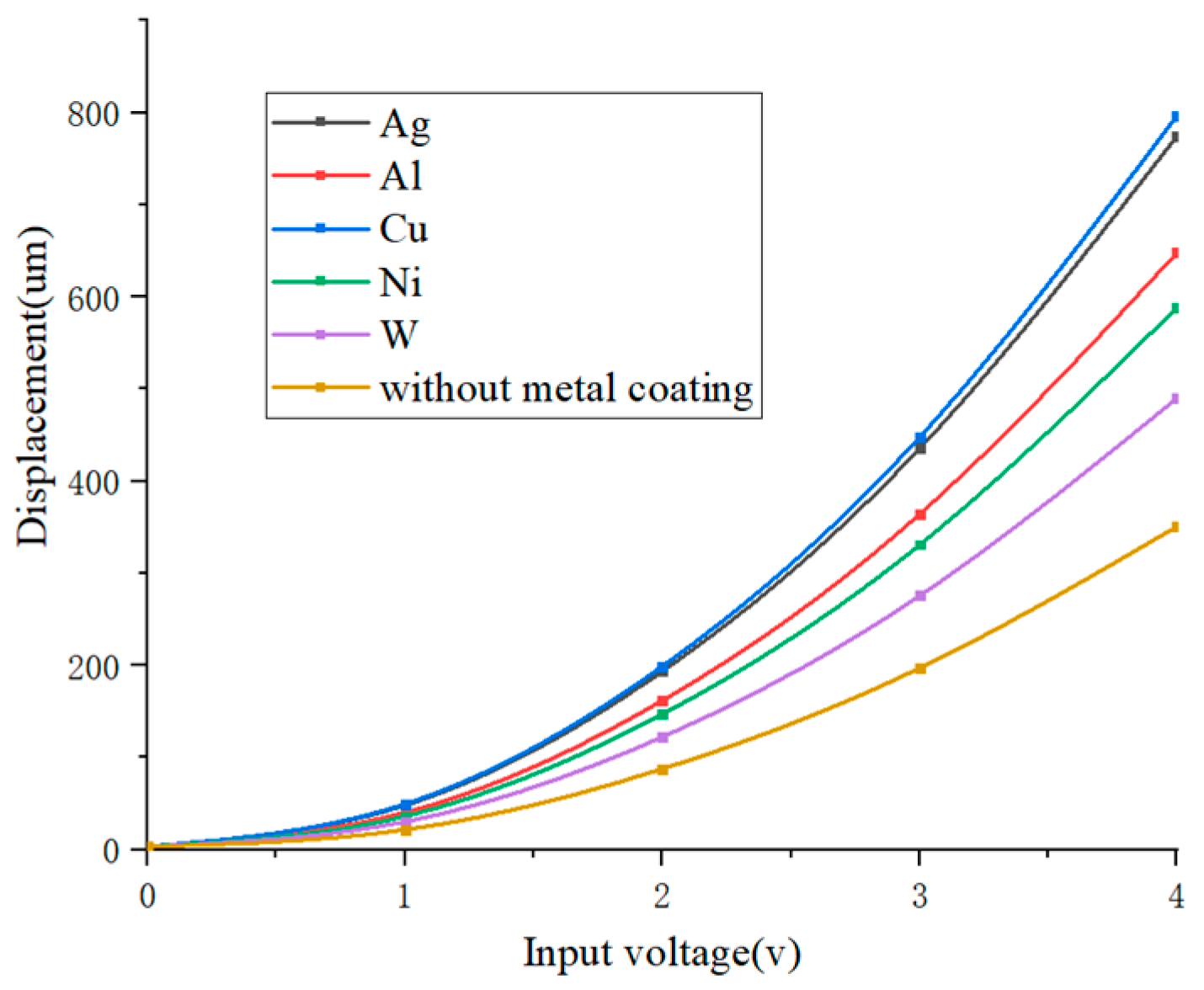
3.2. Calculation and Simulation Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Afrang, S.; Nematkhah, N. A new MEMS based variable capacitor using electrostatic vertical comb drive actuator and auxiliary cantilever beams. Microsyst. Technol. 2019, 25, 3317–3327. [Google Scholar] [CrossRef]
- Gupta, S.; Pahwa, T.; Narwal, R.; Prasad, B.; Kumar, D. Optimiz ing the performance of MEMS electrostatic comb drive actuator with different flexure springs. In Proceedings of the 2012 COMSOL Conference, Bangalore, India, 1–2 November 2012. [Google Scholar]
- Takanami, S.; Kitagawa, W.; Takeshita, T. Design for improvement of torque-thrust characteristic in simultaneous drive in two-degree-of-freedom electromagnetic actuator. In Proceedings of the 2016 19th International Conference on Electrical Machines and Systems (ICEMS), Chiba, Japan, 13–16 November 2016; pp. 1–6. [Google Scholar]
- Moussa, R.; Grossard, M.; Boukallel, M.; Hubert, A.; Chaillet, N. Modeling and control of a piezoelectric microactuator with pro prioceptive sensing capabilities. Measurement 2014, 24, 590–604. [Google Scholar]
- Sarkar, N.; Ellis, M.; Skidmore, G.D. Electrothermal properties and modeling of polysilicon microthermal actuators. J. Microelectromech. Syst. 2003, 12, 513–523. [Google Scholar]
- Maloney, J.M.; Schreiber, D.S.; Devoe, D.L. Large-force electrothermal linear micromotors. J. Micromech. Microeng. 2004, 14, 226–234. [Google Scholar] [CrossRef]
- Espinosa, H.D.; Zhu, Y.; Moldovan, N. Design and operation of a MEMS-based material testing system for nanomechanical characterization. J. Microelectromech. Syst. 2007, 16, 1219–1231. [Google Scholar] [CrossRef]
- Andersen, K.N.; Carlson, K.; Petersen, D.H.; Molhave, K.; Eichhorn, V.; Fatikow, S.; Bøggild, P. Electrothermal microgrippers for pick-and-place operations. Microelectron. Eng. 2008, 85, 1128–1130. [Google Scholar] [CrossRef]
- Hu, T.; Zhao, Y.; Li, X.; Zhao, Y.; Bai, Y. Integration design of MEMS electro-thermal safety-and-arming devices. Microsyst. Technol. 2017, 23, 953–958. [Google Scholar] [CrossRef]
- Wang, Z.; Shen, X.; Chen, X. Design, modeling, and characteriza tion of a MEMS electrothermal microgripper. Microsyst. Technol. 2015, 21, 2307–2314. [Google Scholar] [CrossRef]
- Zhang, Z.; Yu, Y.; Liu, X.; Zhang, X. A Comparison model of V- and Z-shaped electrothermal microactuators. In Proceedings of the 2015 IEEE International Conference on Mechatronics and Automation, Beijing, China, 2–5 August 2015. [Google Scholar]
- Shivhare, P.; Uma, G.; Umapathy, M. Design enhancement of a chevron electrothermally actuated microgripper for improved gripping performance. Microsyst. Technol. 2016, 22, 2623–2631. [Google Scholar] [CrossRef]
- Chu, J.K.; Zhang, R.; Chen, Z.P. A novel SU-8 electrothermal microgripper based on the type synthesis of the kinematic chain method and the stiffness matrix method. J. Micromech. Microeng. 2011, 21, 054030. [Google Scholar] [CrossRef]
- Zhang, Z.; Yu, Y.; Zhang, X. Experimental Study on the Life and Nonlinear Actuation Behaviors of V-shaped SU-8 Electrothermal Microactuators. In Proceedings of the 2018 IEEE 14th International Conference on Control and Automation (ICCA), Anchorage, AK, USA, 12–15 June 2018; pp. 1052–1057. [Google Scholar]
- Tsai, L.N.; Shen, G.R.; Cheng, Y.T.; Hsu, W. Performance improvement of an electrothermal microactuator fabricated using Ni-diamond nanocomposite. J. Microelectromech. Syst. 2006, 15, 149–158. [Google Scholar] [CrossRef]
- Ochiai, K.; Osada, T.; Muro, H. Study on MEMS Thermal Microactuators with Pedestal-Type Beam Shape and Au Electrocoating. IEE J. Trans. SM 2012, 133, 100–104. [Google Scholar]
- Nguyen, D.T.; Hoang, K.T.; Pham, P.H. Larger displacement of silicon electrothermal V-shaped actuator using surface sputtering process. Microsyst. Technol. 2021, 27, 1985–1991. [Google Scholar] [CrossRef]
- Hussein, H.; Younis, M.I.; Fariborzi, H. Task feasibility of V shape electrothermal actuators. Eng. Res. Exp. 2020, 2, 035035. [Google Scholar] [CrossRef]
- Fan, Q.; Miao, J.; Tian, M. Low-voltage Driven Flexible Double-layer Electrothermal Actuator for Smart Human-machine Interactions. Sens. Actuators Phys. 2020, 315, 112352. [Google Scholar] [CrossRef]
- Zhang, D.; Cai, A.; Zhao, Y.; Hu, T. Macro Modeling of V-Shaped Electro-Thermal MEMS Actuator with Human Error Factor. Micromachines 2021, 12, 622. [Google Scholar] [CrossRef] [PubMed]
- Alcheikh, N.; Ouakad, H.M.; Younis, M.I. Dynamics of V-Shaped Electrothermal MEMS-Based Resonators. J. Microelectromech. Syst. 2020, 29, 1372–1381. [Google Scholar] [CrossRef]
- Zhang, X. Research on New Electrothermal Microdrives. Ph.D. Thesis, Shanghai Jiaotong University, Shanghai, China, 2010. [Google Scholar]
- Liu, H.W. Mechanics of Materials; Machinery Industry Press: Beijing, China, 1993; pp. 56–60. [Google Scholar]
- Kolahdoozan, M.; Rouhani Esfahani, A.; Hassani, M. Experimental and numerical investigation of the arms displacement in a new electrothermal MEMS actuator. J. Adv. Des. Manuf. Technol. 2017, 10, 71–81. [Google Scholar]
- Chen, R.S.; Kung, C.; Lee, G.B. Analysis of the optimal dimension on the electrothermal microactuator. J. Micromech. Microeng. 2002, 12, 291. [Google Scholar] [CrossRef]
- Liu, C. Foundations of MEMS; Prentice Hall: Hoboken, NJ, USA, 2011; Chapter 6; pp. 101–102. [Google Scholar]




| Si | Al | Cu | Ni | Ag | W | |
|---|---|---|---|---|---|---|
| Heat capacity | 678 | 904 | 384 | 445 | 235 | 132 |
| Density | 2320 | 2700 | 8960 | 8900 | 10,500 | 19,350 |
| Thermal conductivity | 34 | 237 | 401 | 90.7 | 429 | 174 |
| Young’s modulus | 160 | 70 | 120 | 219 | 83 | 411 |
| Poisson’s ratio | 0.22 | 0.35 | 0.34 | 0.31 | 0.37 | 0.28 |
| Resistivity | 230 × 10−6 | 2.8 × 10−8 | 1.7 × 10−8 | 6.8 × 10−8 | 1.58 × 10−8 | 4.9 × 10−8 |
| Relative Permittivity | 4.5 | 1 | 1 | 1 | 1 | 1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dai, F.; Liu, S.; Hao, Y.; Liu, F. Effect of Metal Coating on Displacement of the Silicon Electrothermal V-Shaped Actuator. Machines 2023, 11, 687. https://doi.org/10.3390/machines11070687
Dai F, Liu S, Hao Y, Liu F. Effect of Metal Coating on Displacement of the Silicon Electrothermal V-Shaped Actuator. Machines. 2023; 11(7):687. https://doi.org/10.3390/machines11070687
Chicago/Turabian StyleDai, Fengqi, Shuangjie Liu, Yongping Hao, and Fengli Liu. 2023. "Effect of Metal Coating on Displacement of the Silicon Electrothermal V-Shaped Actuator" Machines 11, no. 7: 687. https://doi.org/10.3390/machines11070687
APA StyleDai, F., Liu, S., Hao, Y., & Liu, F. (2023). Effect of Metal Coating on Displacement of the Silicon Electrothermal V-Shaped Actuator. Machines, 11(7), 687. https://doi.org/10.3390/machines11070687





