Durability Effects of Gas Nozzle Shape on Marine Two-Stroke Dual-Fuel Engines Using Numerical Analysis
Abstract
1. Introduction
2. Materials and Boundary Condition
2.1. Nozzle Modeling
2.2. Nozzle Materials
2.3. Spray Simulation
2.3.1. Boundary Conditions and Initial Conditions
2.3.2. Model Validation
2.4. Thermal-Structure Simulation
Boundary Conditions and Initial Conditions
2.5. Mesh Generation
3. Results and Discussion
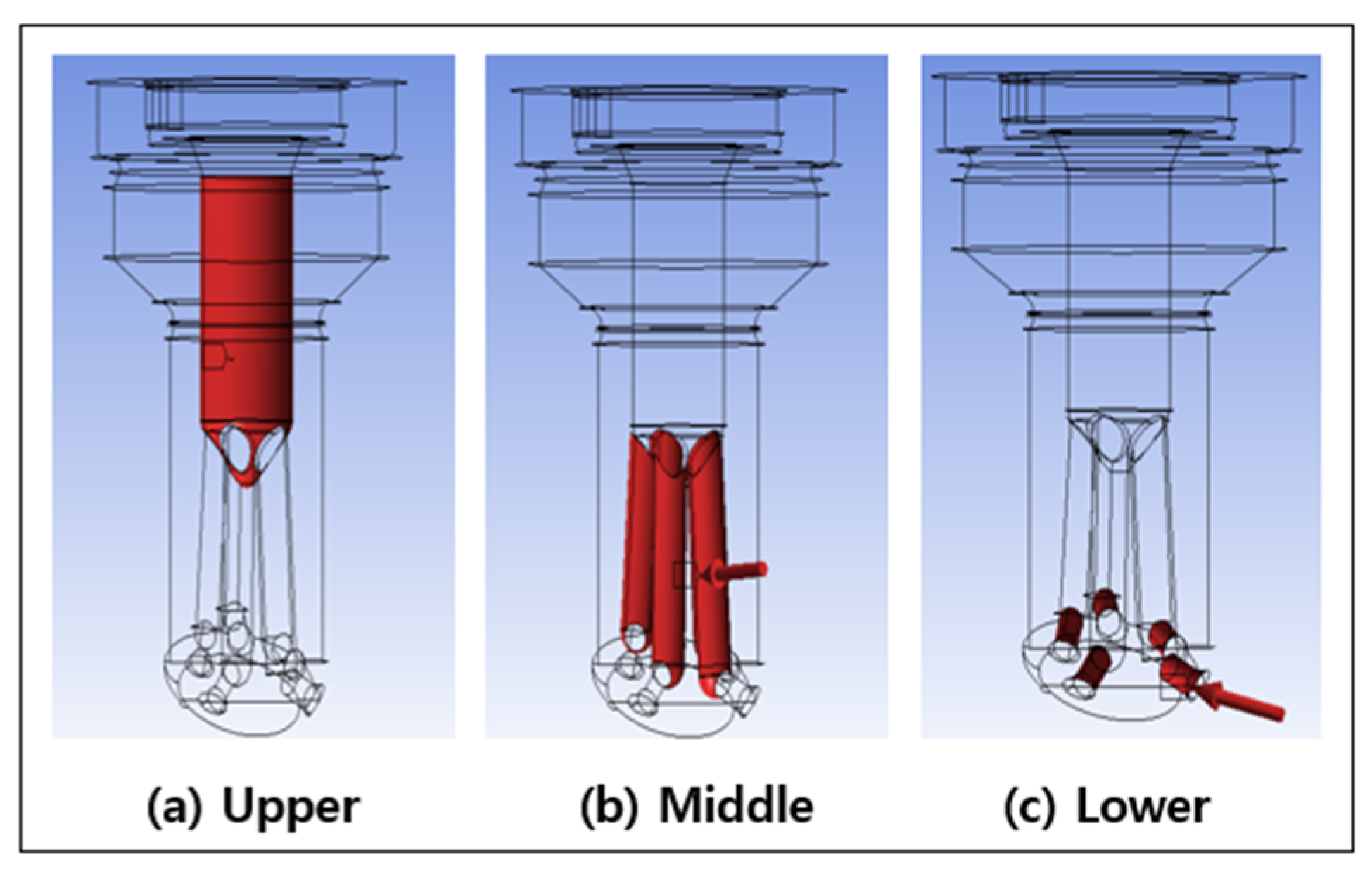
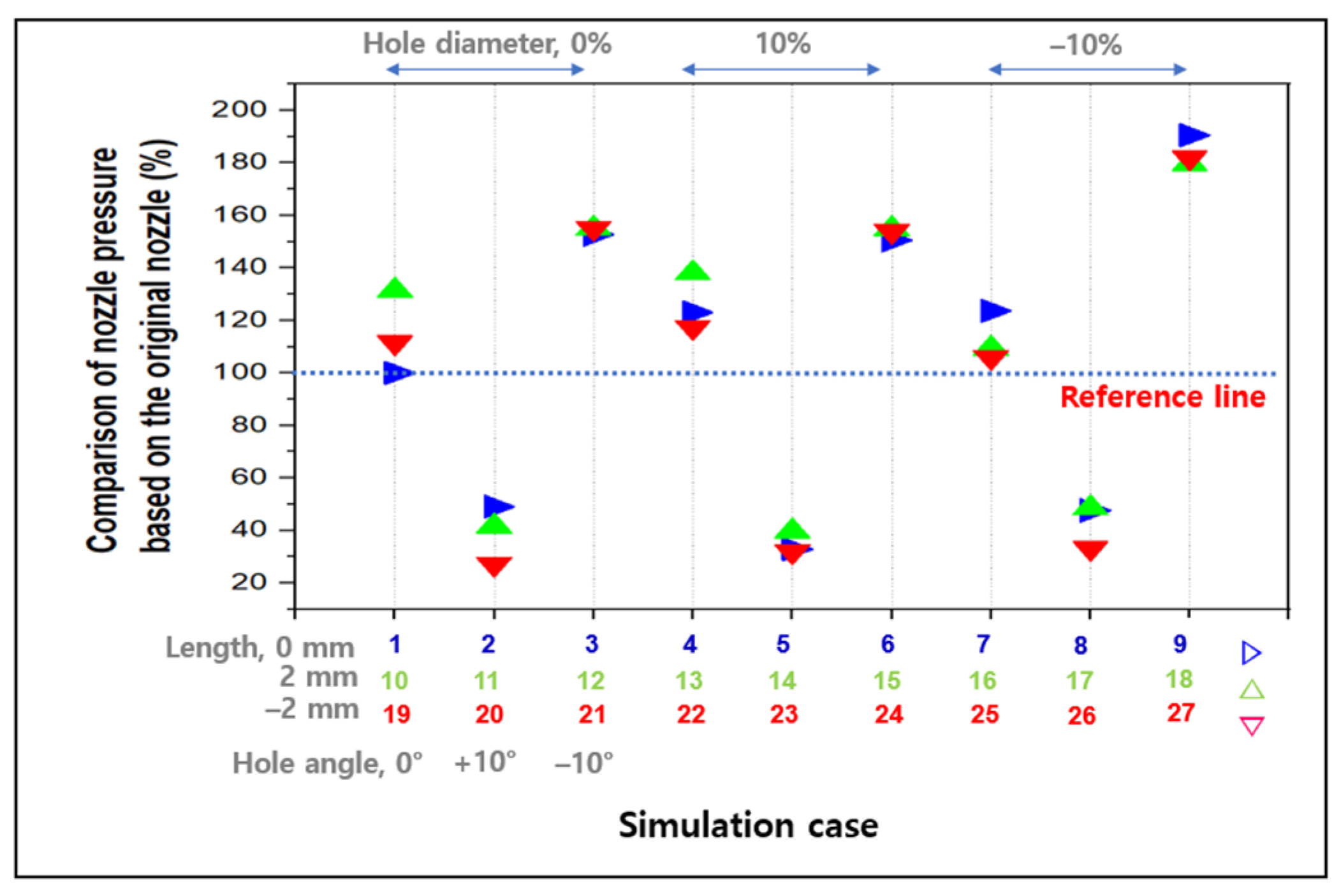
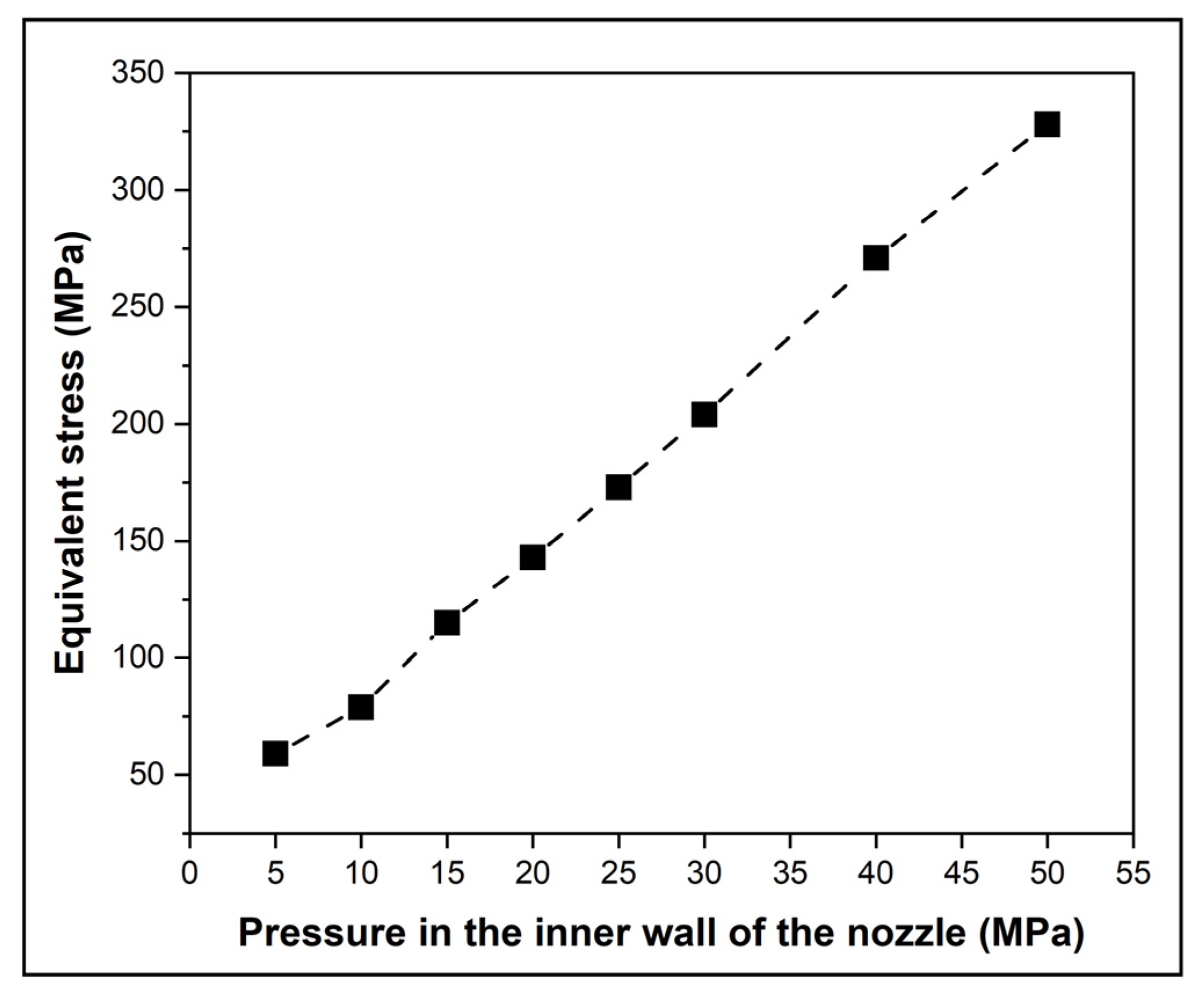
3.1. Pressure in the Inner Wall of the Nozzle
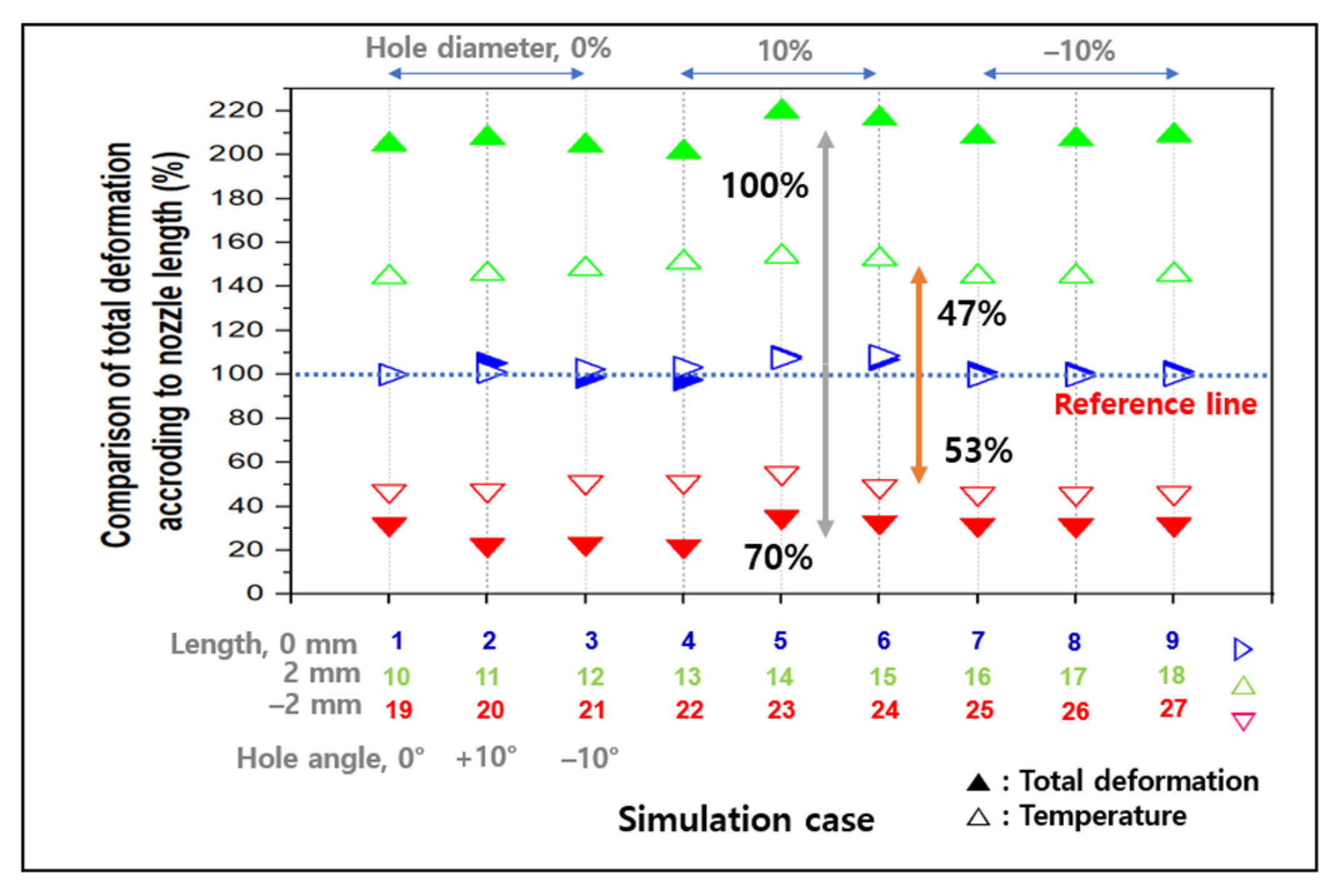
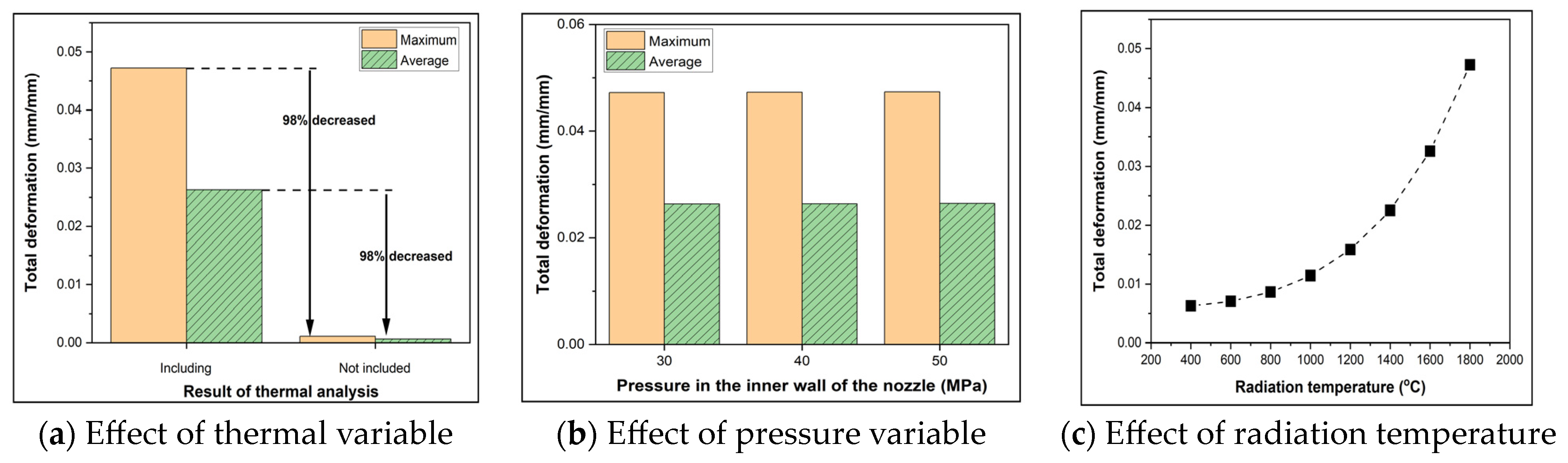
3.2. Total Deformation
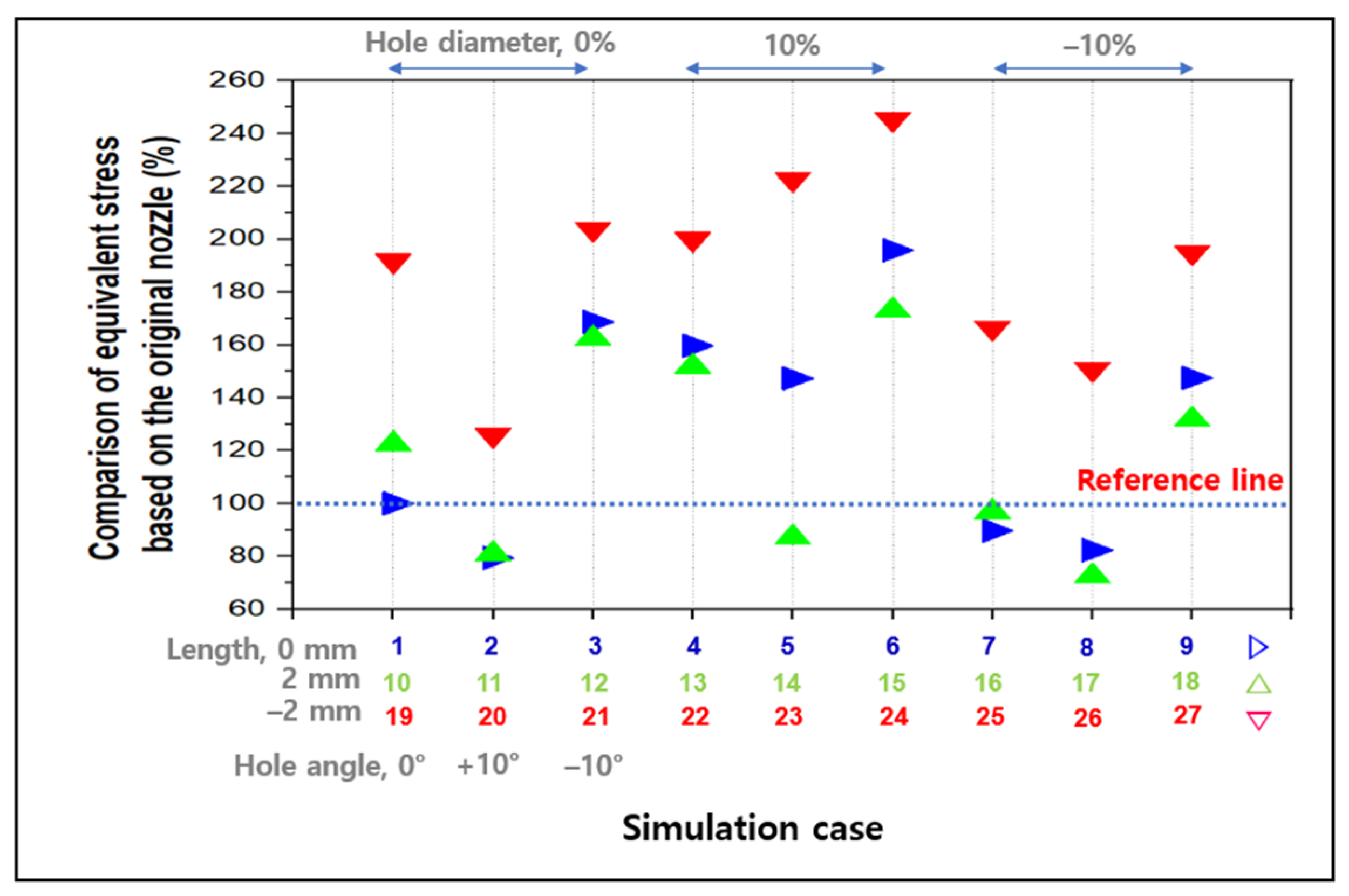
3.3. Equivalent Stress
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| BOG | boil-off gas |
| CFD | computational fluid dynamics |
| DF | dual fuel |
| DME | dimethyl ether |
| EGE | exhaust gas emission |
| IP | injection position |
| LNG | liquefied natural gas |
| UHC | unburned hydrocarbons |
Appendix A
| Case | (a) Upper Part of Nozzle Inside (MPa) | (b) Middle Part of Nozzle Inside (MPa) | (c) Lower Part of Nozzle Inside (MPa) | |||
|---|---|---|---|---|---|---|
| 1 | 3.055 | - | 2.742 | - | 1.102 | - |
| 2 | 3.040 | 100% | 2.734 | 100% | 0.540 | 49% |
| 3 | 3.037 | 99% | 2.812 | 103% | 1.682 | 153% |
| 4 | 3.044 | 100% | 2.763 | 101% | 1.356 | 123% |
| 5 | 3.081 | 101% | 2.702 | 99% | 0.362 | 33% |
| 6 | 3.044 | 100% | 2.826 | 103% | 1.658 | 151% |
| 7 | 3.031 | 99% | 2.761 | 101% | 1.362 | 124% |
| 8 | 3.030 | 99% | 2.742 | 100% | 0.525 | 48% |
| 9 | 3.028 | 99% | 2.788 | 102% | 2.099 | 191% |
| 10 | 3.025 | 99% | 2.749 | 100% | 1.447 | 131% |
| 11 | 3.032 | 99% | 2.726 | 99% | 0.458 | 42% |
| 12 | 3.021 | 99% | 2.841 | 104% | 1.707 | 155% |
| 13 | 3.042 | 100% | 2.874 | 105% | 1.521 | 138% |
| 14 | 3.048 | 100% | 2.694 | 98% | 0.437 | 40% |
| 15 | 3.025 | 99% | 2.823 | 103% | 1.706 | 155% |
| 16 | 3.028 | 99% | 2.762 | 101% | 1.204 | 109% |
| 17 | 3.022 | 99% | 2.737 | 100% | 0.534 | 48% |
| 18 | 3.019 | 99% | 2.823 | 103% | 1.977 | 179% |
| 19 | 3.063 | 100% | 2.759 | 101% | 1.230 | 112% |
| 20 | 3.061 | 100% | 2.733 | 100% | 0.300 | 27% |
| 21 | 3.046 | 100% | 2.836 | 103% | 1.710 | 155% |
| 22 | 3.072 | 101% | 2.756 | 100% | 1.294 | 117% |
| 23 | 3.068 | 100% | 2.702 | 99% | 0.353 | 32% |
| 24 | 3.044 | 100% | 2.827 | 103% | 1.697 | 154% |
| 25 | 3.039 | 99% | 2.774 | 101% | 1.169 | 106% |
| 26 | 3.046 | 100% | 2.734 | 100% | 0.368 | 33% |
| 27 | 3.037 | 99% | 2.830 | 103% | 2.006 | 182% |
References
- Bilgili, L. Life cycle comparison of marine fuels for IMO 2020 Sulphur Cap. Sci. Total Environ. 2021, 774, 145719. [Google Scholar] [CrossRef]
- Shu, J.; Fu, J.; Liu, J.; Ma, Y.; Wang, S.; Deng, B.; Zeng, D. Effects of injector spray angle on combustion and emissions characteristics of a natural gas (NG)-diesel dual fuel engine based on CFD coupled with reduced chemical kinetic model. Appl. Energy 2019, 233, 182–195. [Google Scholar] [CrossRef]
- Deng, J.; Wang, X.; Wei, Z.; Wang, L.; Wang, C.; Chen, Z. A review of NOx and SOx emission reduction technologies for marine diesel engines and the potential evaluation of liquefied natural gas fuelled vessels. Sci Total Environ. 2021, 766, 144319. [Google Scholar] [CrossRef]
- Sharafian, A.; Blomerus, P.; Mérida, W. Natural gas as a ship fuel: Assessment of greenhouse gas and air pollutant reduction potential. Energy Policy 2019, 131, 332–346. [Google Scholar] [CrossRef]
- Yusuf, A.A.; Inambao, F.L.; Ampah, J.D. Evaluation of biodiesel on speciated PM2.5, organic compound, ultrafine particle and gaseous emissions from a low-speed EPA Tier II marine diesel engine coupled with DPF, DEP and SCR filter at various loads. Energy 2022, 239, 121837. [Google Scholar] [CrossRef]
- Yusuf, A.A.; Yusuf, D.A.; Jie, Z.; Bello, T.Y.; Tambaya, M.; Abdullahi, B.; Muhammed-Dabo, I.A.; Yahuza, I.; Dandakouta, H. Influence of waste oil-biodiesel on toxic pollutants from marine engine coupled with emission reduction measures at various loads. Atmos. Pollut. Res. 2022, 13, 101258. [Google Scholar] [CrossRef]
- Chu Van, T.; Ramirez, J.; Rainey, T.; Ristovski, Z.; Brown, R.J. Global impacts of recent IMO regulations on marine fuel oil refining processes and ship emissions. Transp. Res. Part D Transp. Environ. 2019, 70, 123–134. [Google Scholar] [CrossRef]
- Andreadis, P.; Zobanakis, A.; Chryssakis, C.; Kaiktsis, L. Effects of the Fuel Injection Parameters on the Performance and Emissions Formation in a Large-Bore Marine Diesel Engine. Int. J. Engine Res. 2010, 12, 14–29. [Google Scholar] [CrossRef]
- Hayashi, T.; Suzuki, M.; Ikemoto, M. Effects of internal flow in a diesel nozzle on spray combustion. Int. J. Engine Res. 2013, 14, 646–654. [Google Scholar] [CrossRef]
- Korkmaz, M.; Ritter, D.; Jochim, B.; Beeckmann, J.; Abel, D.; Pitsch, H. Effects of injection strategy on performance and emissions metrics in a diesel/methane dual-fuel single-cylinder compression ignition engine. Int. J. Engine Res. 2019, 20, 1059–1072. [Google Scholar] [CrossRef]
- Maghbouli, A.; Yang, W.; An, H.; Li, J.; Shafee, S. Effects of Injection Strategies and Fuel Injector Configuration on Combustion and Emission Characteristics of a D.I. Diesel Engine Fueled by Bio-Diesel. Renew. Energy 2015, 76, 687–698. [Google Scholar] [CrossRef]
- Mobasheri, R.; Peng, Z. A Computational Investigation into the Effects of Included Spray Angle on Heavy-Duty Diesel Engine Operating Parameters. In Proceedings of the SAE 2012 International Powertrains, Fuels & Lubricants Meeting, Malmo, Sweden, 18–20 September 2012; SAE International: Warrendale, PA, USA, 2012. [Google Scholar]
- Yoon, S.H.; Cha, J.P.; Lee, C.S. An investigation of the effects of spray angle and injection strategy on dimethyl ether (DME) combustion and exhaust emission characteristics in a common-rail diesel engine. Fuel Process. Technol. 2010, 91, 1364–1372. [Google Scholar] [CrossRef]
- Park, S.W.; Reitz, R.D. Optimization of fuel/air mixture formation for stoichiometric diesel combustion using a 2-spray-angle group-hole nozzle. Fuel 2009, 88, 843–852. [Google Scholar] [CrossRef]
- Dolak, J.; Reitz, R.D. Optimization of the piston geometry of a diesel engine using a two-spray-angle nozzle. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2011, 225, 406–421. [Google Scholar] [CrossRef]
- Taghavifar, H.; Mardani, A.; Mohebbi, A. Exhaust emissions prognostication for DI diesel group-hole injectors using a supervised artificial neural network approach. Fuel 2014, 125, 81–89. [Google Scholar] [CrossRef]
- Siewert, R.M. Spray Angle and Rail Pressure Study for Low NOx Diesel Combustion. In Proceedings of the SAE World Congress & Exhibition 2007, Detroit, MI, USA, 16–19 April 2007; SAE International: Warrendale, PA, USA, 2007. [Google Scholar]
- Kitasei, T.; Yamada, J.; Shoji, T.; Shiino, S.; Mori, K. Influence of the Different Fuel Spray Wall Impingement Angles on Smoke Emission in a DI-Diesel Engine; SAE International: Warrendale, PA, USA, 2008. [Google Scholar]
- Kim, H.; Reitz, R.; Kong, S.-C. Modeling Combustion and Emissions of HSDI Diesel Engines Using Injectors with Different Included Spray Angles. SAE Technical paper: Warrendale, PA, USA, 2006. [Google Scholar]
- Fang, T.; Coverdill, R.E.; Chia-fon, F.L.; White, R.A. Effects of injection angles on combustion processes using multiple injection strategies in an HSDI diesel engine. Fuel 2008, 87, 3232–3239. [Google Scholar] [CrossRef]
- Lim, J.; Min, K. The Effects of Spray Angle and Piston Bowl Shape on Diesel Engine Soot Emissions Using 3-D CFD Simulation; SAE International: Warrendale, PA, USA, 2005. [Google Scholar]
- Di Iorio, S.; Magno, A.; Mancaruso, E.; Vaglieco, B. Diesel/methane dual fuel strategy to improve environmental performance of energy power systems. Int. J. Heat Technol. 2016, 34, 581–588. [Google Scholar] [CrossRef]
- Marashi, J.; Yakushina, E.; Xirouchakis, P.; Zante, R.; Foster, J. An evaluation of H13 tool steel deformation in hot forging conditions. J. Mater. Process. Technol. 2017, 246, 276–284. [Google Scholar] [CrossRef]
- Tridello, A. VHCF Response of Two AISI H13 Steels: Effect of Manufacturing Process and Size-Effect. Metals 2019, 9, 133. [Google Scholar] [CrossRef]
- Son, J.; Shin, G.; Lee, K.; Choi, C.-H.; Shim, D. High-Temperature Properties of Hot-Work Tool Steel (AISI H13) Deposited via Direct Energy Deposition, in Forming the Future; Springer: Berlin/Heidelberg, Germany, 2021; pp. 1665–1676. [Google Scholar]
- Sadafi, M.; Jahn, I.; Hooman, K. Nozzle arrangement effect on cooling performance of saline water spray cooling. Appl. Therm. Eng. 2016, 105, 1061–1066. [Google Scholar] [CrossRef]
- Ishak, M.; Ismail, F.; Mat, S.C.; Abdullah, M.; Aziz, M.A.; Idroas, M. Numerical analysis of nozzle flow and spray characteristics from different nozzles using diesel and biofuel blends. Energies 2019, 12, 281. [Google Scholar] [CrossRef]
- Wang, F.; Wu, J.; Liu, Z. Surface Tensions of Mixtures of Diesel Oil or Gasoline and Dimethoxymethane, Dimethyl Carbonate, or Ethanol. Energy Fuels 2006, 20, 2471–2474. [Google Scholar] [CrossRef]
- Esteban, B.; Riba, J.-R.; Baquero, G.; Puig, R.; Rius, A. Characterization of the surface tension of vegetable oils to be used as fuel in diesel engines. Fuel 2012, 102, 231–238. [Google Scholar] [CrossRef]
- Meng, F.-Q.; He, B.-J.; Zhu, J.; Zhao, D.-X.; Darko, A.; Zhao, Z.-Q. Sensitivity analysis of wind pressure coefficients on CAARC standard tall buildings in CFD simulations. J. Build. Eng. 2018, 16, 146–158. [Google Scholar] [CrossRef]
- Montazeri, H.; Blocken, B. CFD simulation of wind-induced pressure coefficients on buildings with and without balconies: Validation and sensitivity analysis. Build. Environ. 2013, 60, 137–149. [Google Scholar] [CrossRef]
- Thompson, M.K.; Thompson, J.M. ANSYS Mechanical APDL for Finite Element Analysis; Butterworth-Heinemann: Oxford, UK, 2017. [Google Scholar]
- Han, M.-S.; Cho, J.-U. Structural Analysis of Engine Mounting Bracket. J. Manuf. Eng. Technol. 2012, 21, 525–531. [Google Scholar]
- Rong, Z.; Yi, J.; Li, F.; Liu, Y.; Eckert, J. Thermal stress analysis and structural optimization of ladle nozzle based on finite element simulation. Mater. Res. Express 2022, 9, 045601. [Google Scholar] [CrossRef]
- Zhang, F.; Ma, G.; Tan, Y. The nozzle structure design and analysis for continuous carbon fiber composite 3D printing. In Proceedings of the 2017 7th International Conference on Advanced Design and Manufacturing Engineering (ICADME 2017), Shenzhen, China, 10–11 May 2017; Atlantis Press: Amsterdam, The Netherlands, 2017. [Google Scholar]
- Gwak, C.-Y.; Shin, B.-S.; Go, J.-S.; Kim, M.-J.; Yoo, C.-J.; Yun, D.-H. A Study on the Simulation Analysis of Nozzle Length and Inner Spiral Structure of a Waterjet. Korean Soc. Manuf. Process Eng. 2017, 16, 118–123. [Google Scholar] [CrossRef]
- Nygård, A.; Altimira, M.; Semlitsch, B.; Wittberg, L.P.; Fuchs, L. Analysis of Vortical Structures in Intermittent Jets. In Proceedings of the 5th International Conference on Jets, Wakes and Separated Flows (ICJWSF2015); Springer International Publishing: Cham, Switzerland, 2016; pp. 3–10. [Google Scholar]
- Oh, J.-S.; Lee, I.-S.; Yoon, H.-K.; Sung, H.-K. Thermal and Structural Analyses of Semi-metallic Gasket Joined with Graphite Seal for Ship Engine Piping Flange. J. Ocean Eng. Technol. 2017, 31, 352–356. [Google Scholar] [CrossRef]
- Zhou, H.; Zhou, H.; Zhao, Z.; Li, K.; Yin, J. Numerical Simulation and Verification of Laser-Polishing Free Surface of S136D Die Steel. Metals 2021, 11, 400. [Google Scholar] [CrossRef]
- Wei, M.X.; Wang, S.; Wang, L.; Cui, X.; Chen, K. Effect of tempering conditions on wear resistance in various wear mechanisms of H13 steel. Tribol. Int. 2011, 44, 898–905. [Google Scholar] [CrossRef]
- Wu, Y.-T.; Shin, Y.; Sues, R.; Cesare, M. Safety-factor based approach for probability-based design optimization. In Proceedings of the 19th AIAA Applied Aerodynamics Conference, Anaheim, CA, USA, 11–14 June 2001. [Google Scholar]
- Drucker, D.; Greenberg, H.; Prager, W. The safety factor of an elastic-plastic body in plane strain. J. Appl. Mech. 1951, 18, 371–378. [Google Scholar] [CrossRef]
- Freudenthal, A.M. The safety of structures. Trans. Am. Soc. Civ. Eng. 1947, 112, 125–159. [Google Scholar] [CrossRef]
- Choi, H.-G.; Hong, S.-Y.; Song, J.-H.; Jang, W.-S.; Choi, W.-S. A Study on Vortex-Induced Vibration Characteristics of Hydrofoils considering High-order Modes. J. Korean Soc. Mar. Environ. Saf. 2022, 28, 377–384. [Google Scholar] [CrossRef]
- Jia, W.; Zhang, Y.; Li, C.; Luo, P.; Song, X.; Wang, Y.; Hu, X. Experimental and numerical simulation of erosion-corrosion of 90° steel elbow in shale gas pipeline. J. Nat. Gas Sci. Eng. 2021, 89, 103871. [Google Scholar] [CrossRef]
- Wang, H.; Yu, Y.; Yu, J.; Xu, W.; Li, X.; Yu, S. Numerical simulation of the erosion of pipe bends considering fluid-induced stress and surface scar evolution. Wear 2019, 440, 203043. [Google Scholar] [CrossRef]
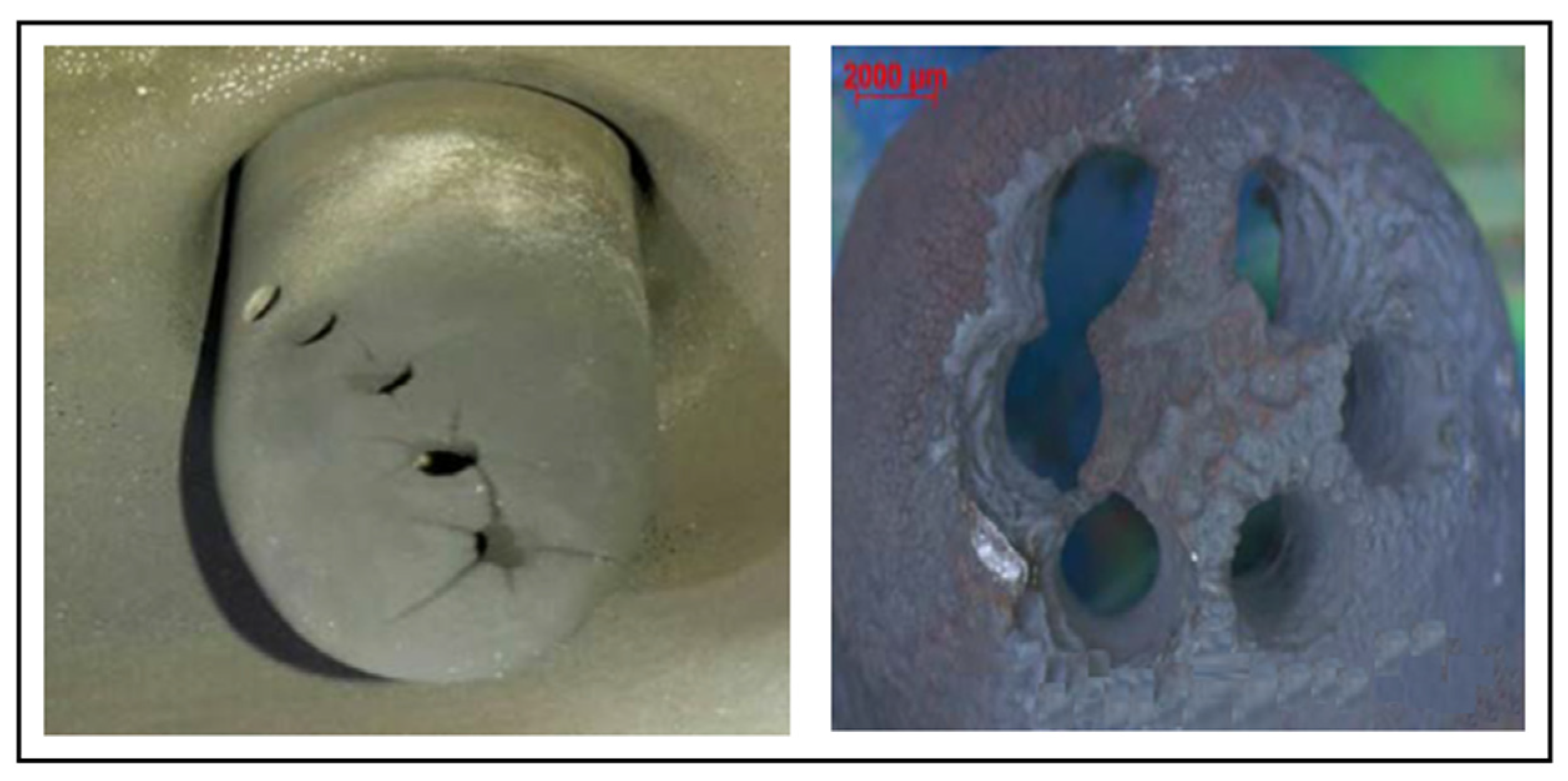
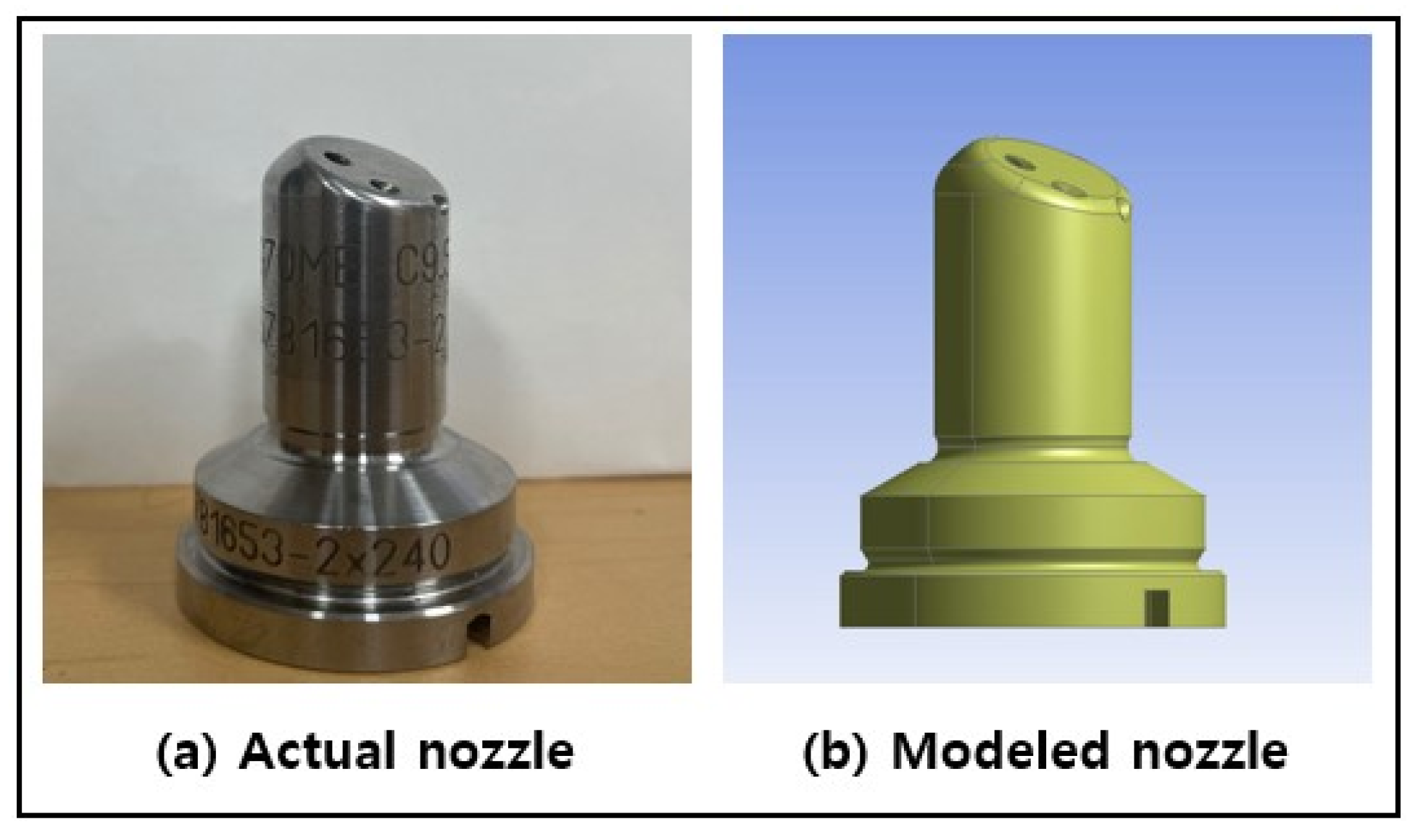


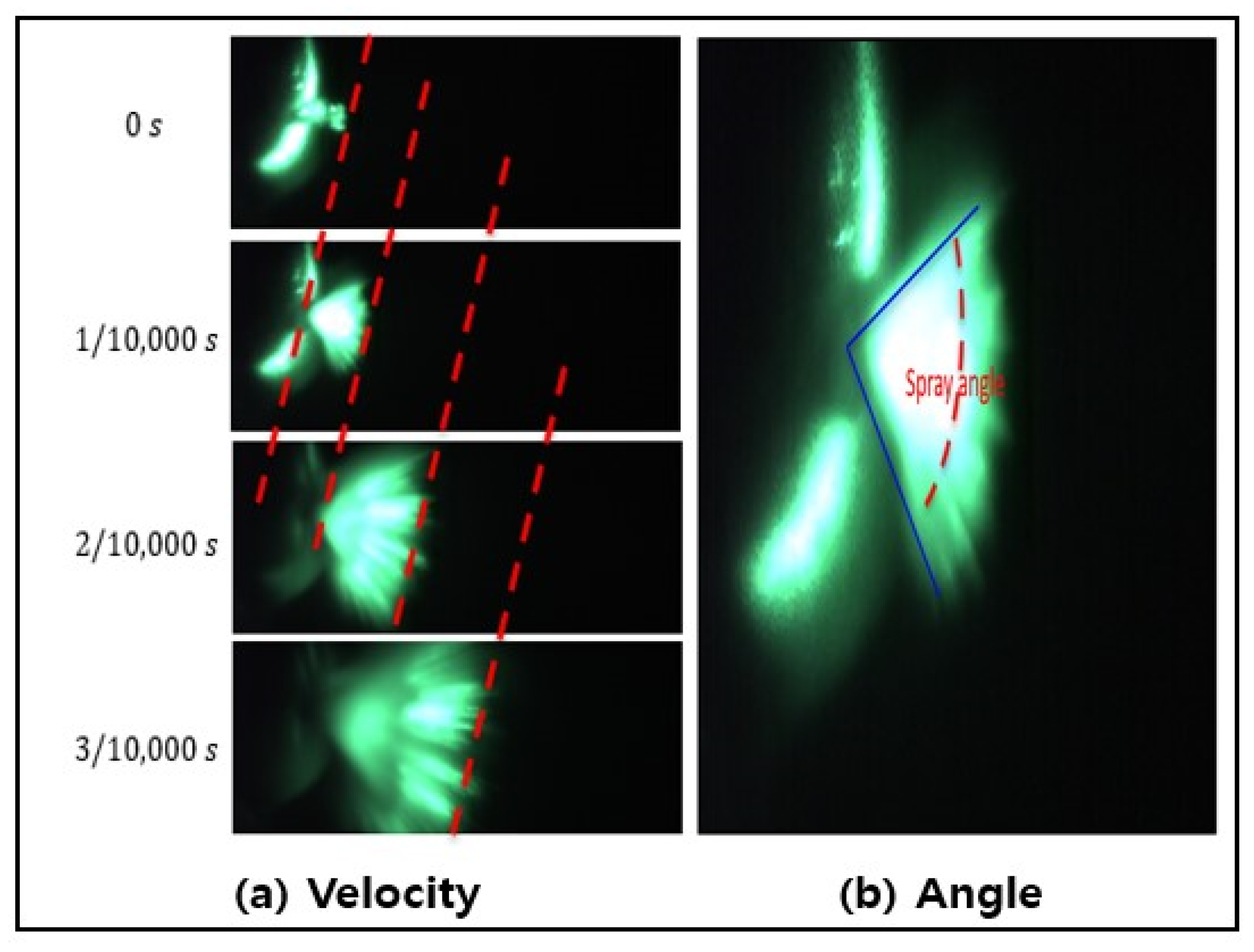

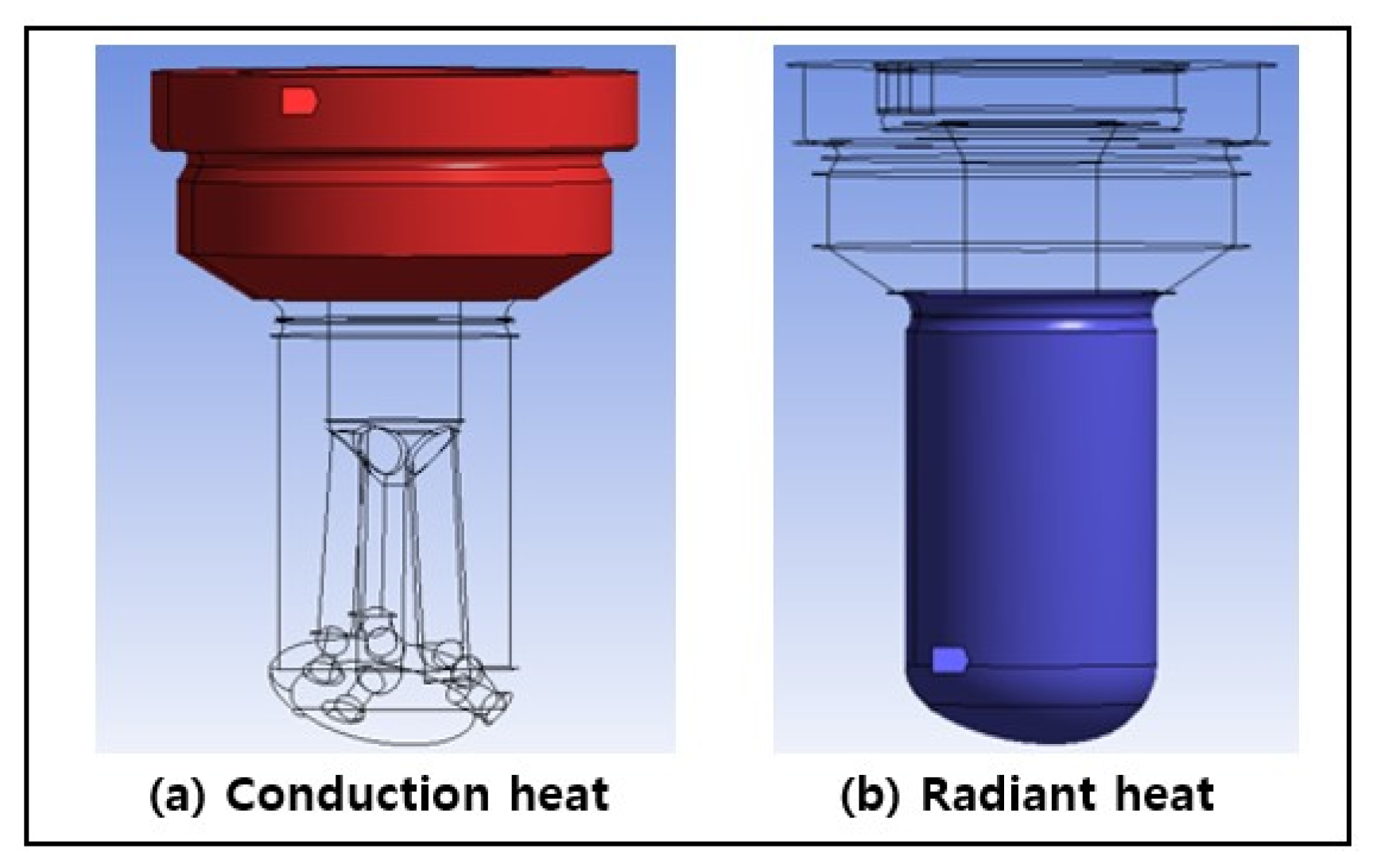

| Number of Nozzle Hole | Coordinate from Nozzle Center Line (mm) | Angle (Degree) | |||
|---|---|---|---|---|---|
| X | Y | Z | Vertical | Horizon | |
| No.1 hole | 0.5034 | −1.2584 | −2.5981 | 116 | 82.3 |
| No.2 hole | −0.5193 | −1.2647 | −2.9224 | 112 | 100.3 |
| No.3 hole | −1.0620 | −0.4718 | −3.3349 | 111 | 117.3 |
| No.4 hole | 0.4621 | 0.2860 | −3.3318 | 125 | 43.3 |
| No.5 hole | 0.9479 | −0.3783 | −2.8961 | 126 | 64.3 |
| Species | Composition | Species | Composition |
|---|---|---|---|
| C | 0.35–0.42 | S | 4.8–5.5 |
| Si | 0.8–1.2 | Cr | 4.75–5.5 |
| Mn | 0.25–0.5 | Mo | 1.1–1.75 |
| P | 0.03 | V | 0.8–1.15 |
| Property | Unit | Contents | |||
|---|---|---|---|---|---|
| Density | g/cm3 | 20 °C | 400 °C | 1110 °C | |
| 7.8 | 7.7 | 7.6 | |||
| Coefficient of thermal expansion | μm/m·°C | 25–95 °C | 25–205 °C | 25–540 °C | |
| 11 | 11.5 | 12.4 | |||
| Specific heat capacity | J/g·°C | 0.460 | |||
| Thermal conductivity | W/m·K | 215 °C | 350 °C | 475 °C | 605 °C |
| 24.3 | 24.3 | 24.4 | 24.7 | ||
| Tensile strength, ultimate | MPa | 1990 | |||
| Tensile strength, yield | MPa | 1650 | |||
| Modulus of elasticity | GPa | 210 | |||
| Poisson’s ratio | - | 0.30 | |||
| Item | Contents | Remark |
|---|---|---|
| Multiphases | Liquid diesel | Inlet pressure: 300 bar |
| Air | Outlet pressure: 0 bar | |
| Surface tension coefficients (n/m) | 0.026 [28,29] | |
| Gravitational acceleration (m/s2) | −9.81 | |
| Viscous model | Standard k-ε | |
| Turbulent kinetic energy | Second order upwind | |
| Turbulent dissipation rate | Second order upwind |
| Element Quality | Aspect Ratio | Skewness | Orthogonal Quality | Node | Element | |
|---|---|---|---|---|---|---|
| Average | 0.8341 | 9.4522 | 0.8250 | 0.1777 | 107,311 | 553,830 |
| Min | 0.83322 | 8.0712 | 0.79892 | 0.11384 | 99,688 | 513,908 |
| Max | 0.83472 | 10.763 | 0.88616 | 0.20892 | 113,560 | 585,245 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, J.-S.; Choi, J.-H. Durability Effects of Gas Nozzle Shape on Marine Two-Stroke Dual-Fuel Engines Using Numerical Analysis. Machines 2023, 11, 456. https://doi.org/10.3390/machines11040456
Kim J-S, Choi J-H. Durability Effects of Gas Nozzle Shape on Marine Two-Stroke Dual-Fuel Engines Using Numerical Analysis. Machines. 2023; 11(4):456. https://doi.org/10.3390/machines11040456
Chicago/Turabian StyleKim, Jun-Soo, and Jae-Hyuk Choi. 2023. "Durability Effects of Gas Nozzle Shape on Marine Two-Stroke Dual-Fuel Engines Using Numerical Analysis" Machines 11, no. 4: 456. https://doi.org/10.3390/machines11040456
APA StyleKim, J.-S., & Choi, J.-H. (2023). Durability Effects of Gas Nozzle Shape on Marine Two-Stroke Dual-Fuel Engines Using Numerical Analysis. Machines, 11(4), 456. https://doi.org/10.3390/machines11040456






