Author Contributions
Conceptualization, M.E.A. and A.Z.; methodology, M.E.A.; software, M.E.A. and A.Z.; validation, M.E.A., B.B. and N.V.; formal analysis, M.E.A. and B.B.; investigation, M.E.A., B.B. and N.V.; resources, B.B. and N.V.; original draft preparation, M.E.A., A.Z. and B.B.; review and editing, M.E.A., A.Z. and B.B.; visualization, M.E.A., B.B. and N.V.; supervision, B.B. and N.V.; project administration, B.B.; funding acquisition, B.B. and N.V. All authors have read and agreed to the published version of the manuscript.
Figure 1.
Comparison of the magnetic properties of HIPERCO
® 50/50A [
22].
Figure 1.
Comparison of the magnetic properties of HIPERCO
® 50/50A [
22].
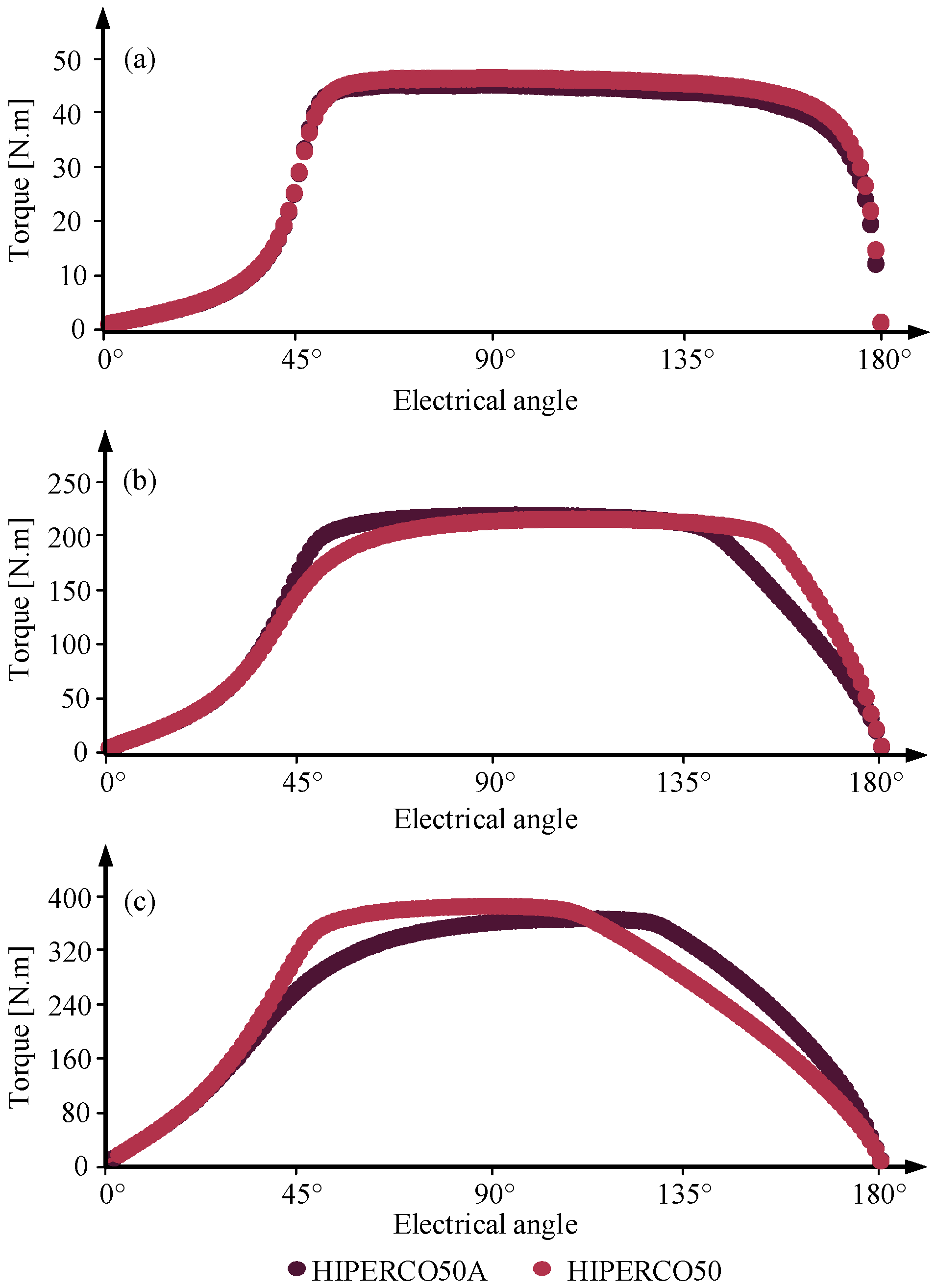
Figure 2.
Comparison of static torque production with HIPERCO® 50 and HIPERCO® 50A for the dimensional constraints and current density levels of LSA SRM: (a) J = 5 A/mm2, (b) J = 15 A/mm2, and (c) J = 25 A/mm2.
Figure 2.
Comparison of static torque production with HIPERCO® 50 and HIPERCO® 50A for the dimensional constraints and current density levels of LSA SRM: (a) J = 5 A/mm2, (b) J = 15 A/mm2, and (c) J = 25 A/mm2.
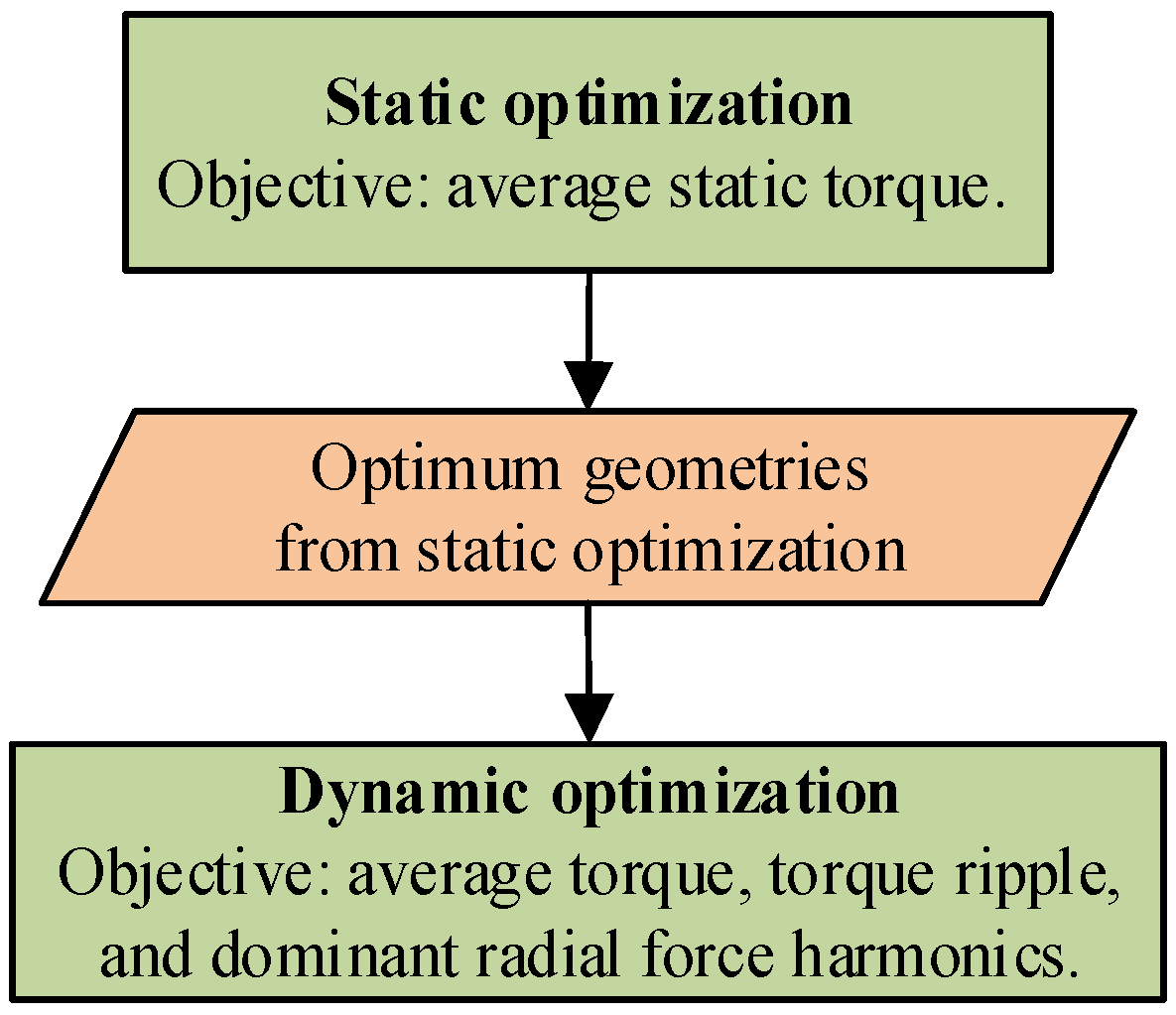
Figure 3.
Proposed multi-objective optimization framework [
23].
Figure 3.
Proposed multi-objective optimization framework [
23].
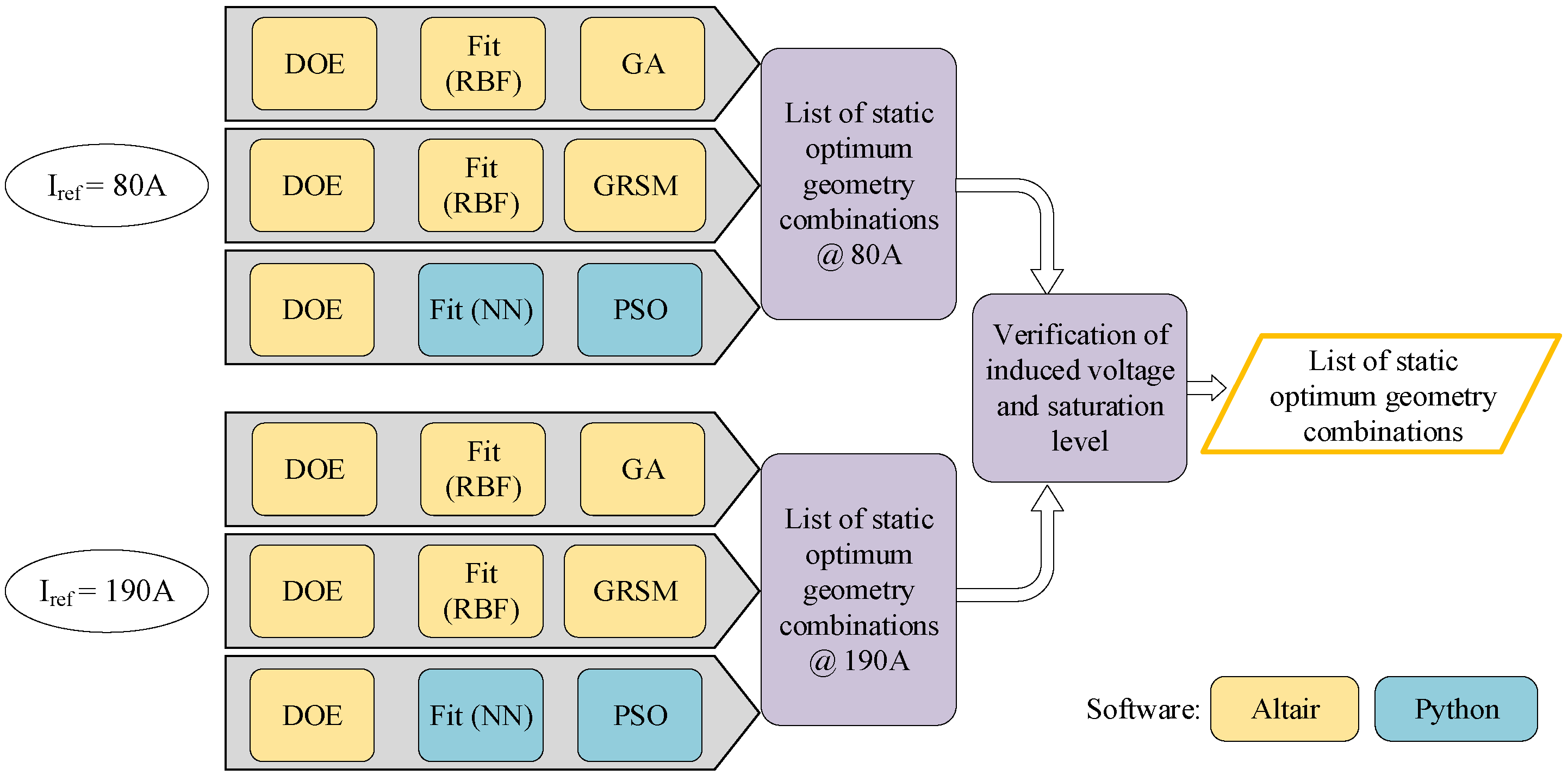
Figure 4.
Block diagram of the static design: fit models and global optimizations used for two different current ratings [
23].
Figure 4.
Block diagram of the static design: fit models and global optimizations used for two different current ratings [
23].
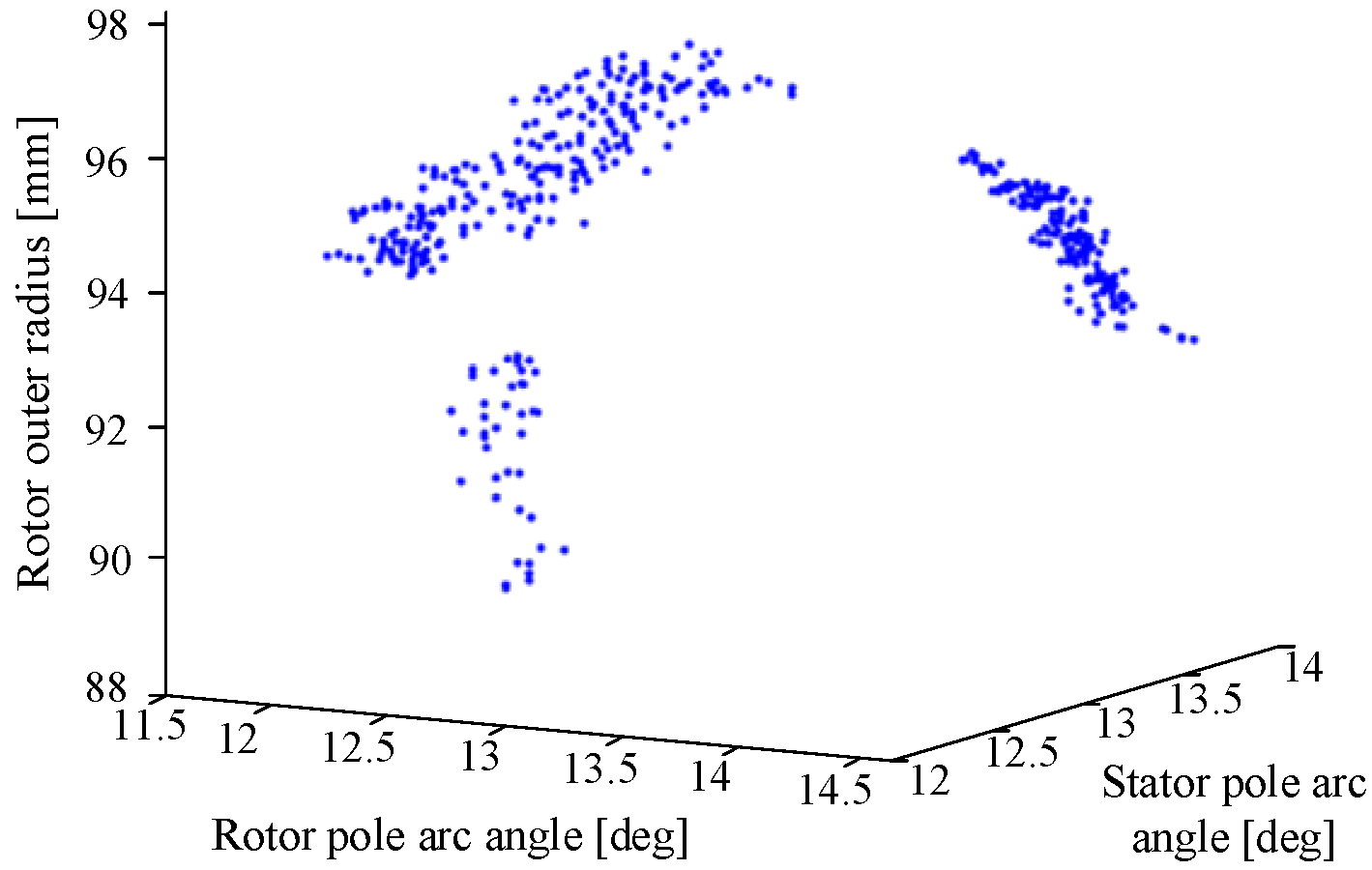
Figure 5.
Illustration of static design results with respect to the three geometry parameters with highest correlation to average torque.
Figure 5.
Illustration of static design results with respect to the three geometry parameters with highest correlation to average torque.
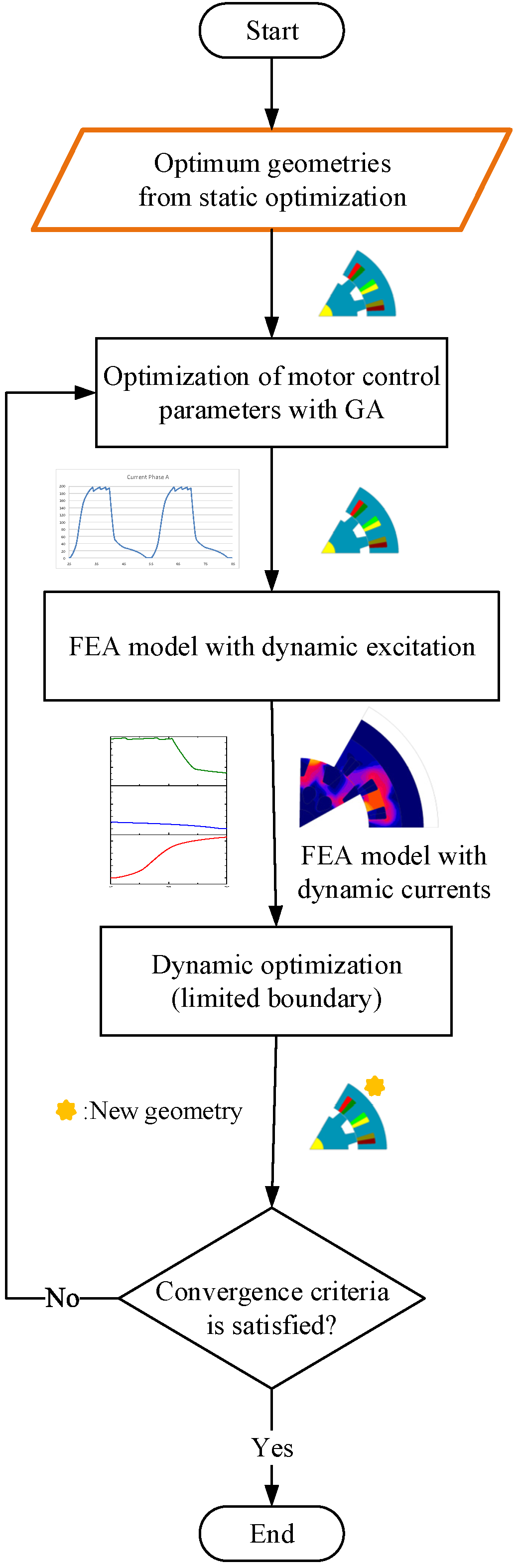
Figure 6.
Block diagram of the dynamic optimization stage [
23].
Figure 6.
Block diagram of the dynamic optimization stage [
23].
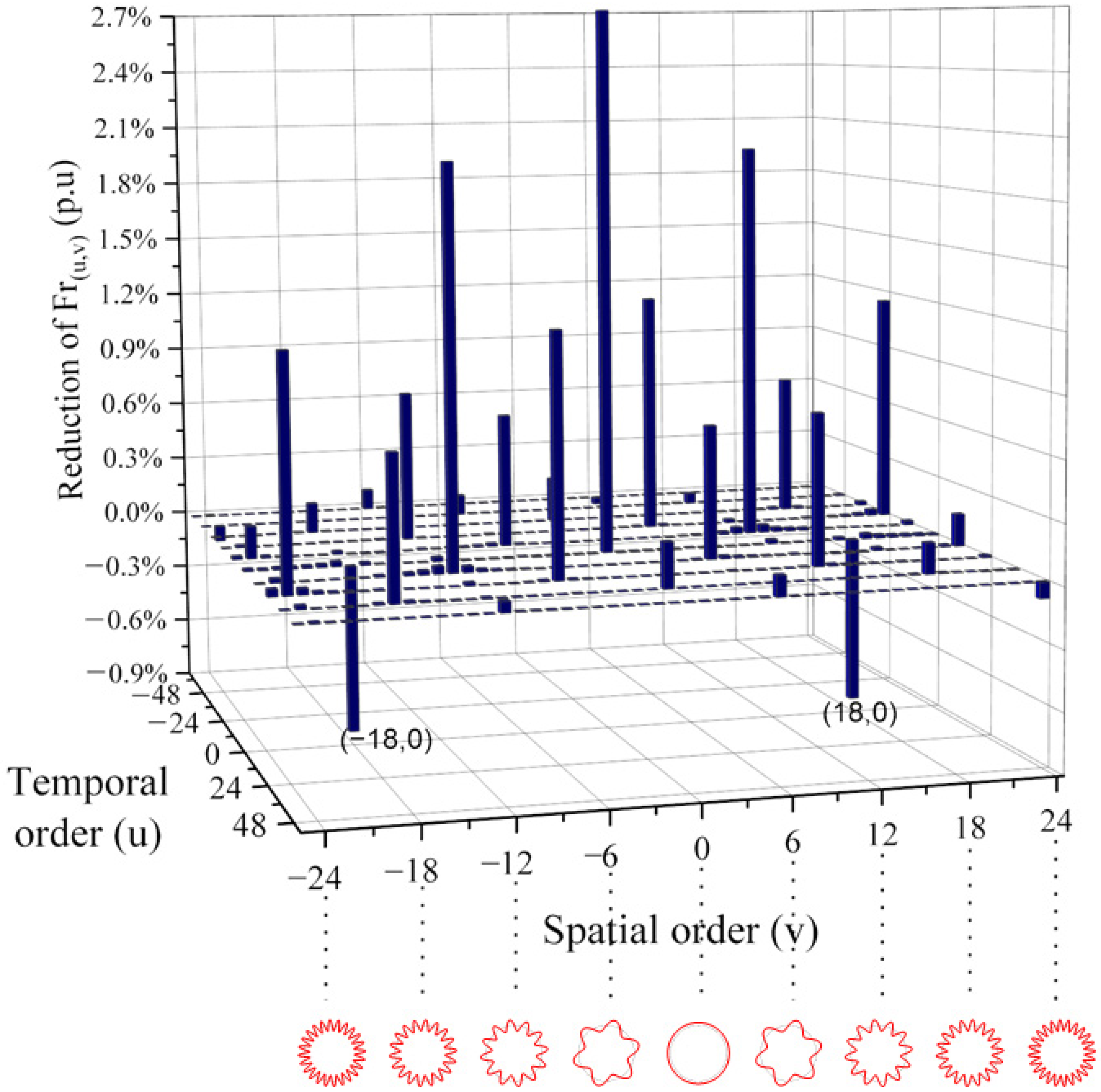
Figure 7.
The change in radial force after five iterations of dynamic optimization.
Figure 7.
The change in radial force after five iterations of dynamic optimization.
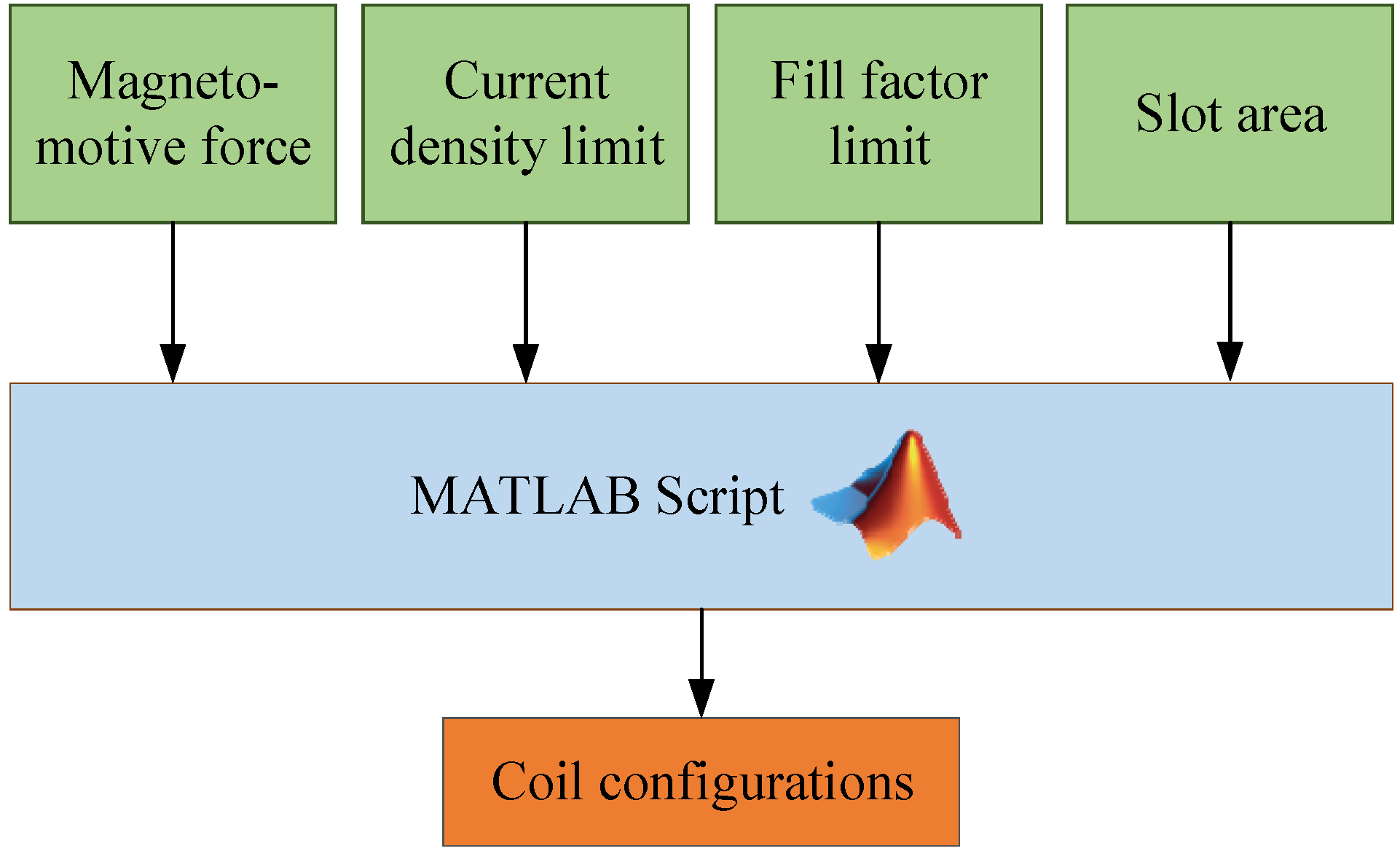
Figure 8.
Block diagram showing the operation of the script to determine the coil parameters.
Figure 8.
Block diagram showing the operation of the script to determine the coil parameters.
Figure 9.
Conversion of stranded coil region to coil with individual wires.
Figure 9.
Conversion of stranded coil region to coil with individual wires.
Figure 10.
Comparison of AC copper losses for two different coil options.
Figure 10.
Comparison of AC copper losses for two different coil options.
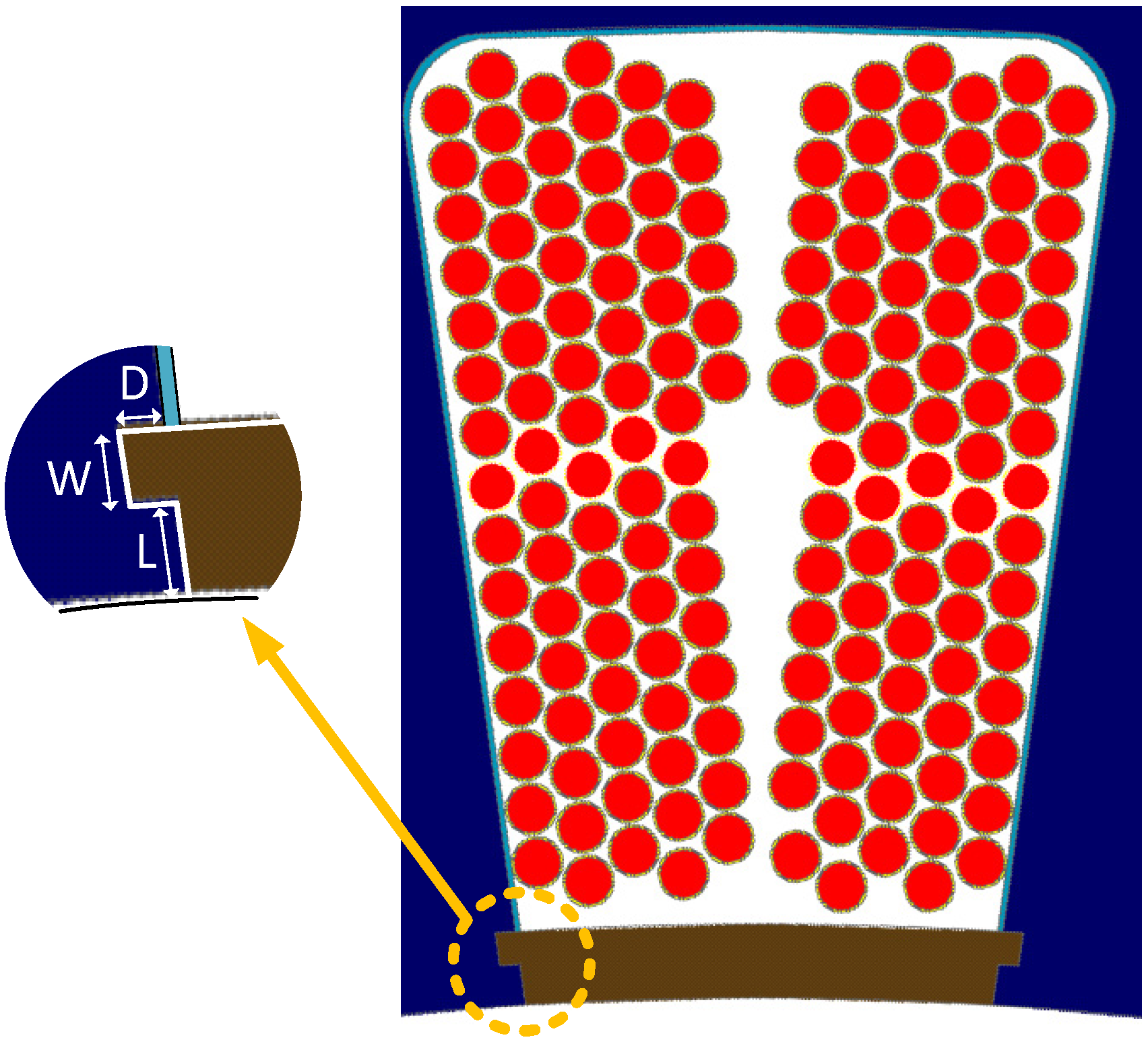
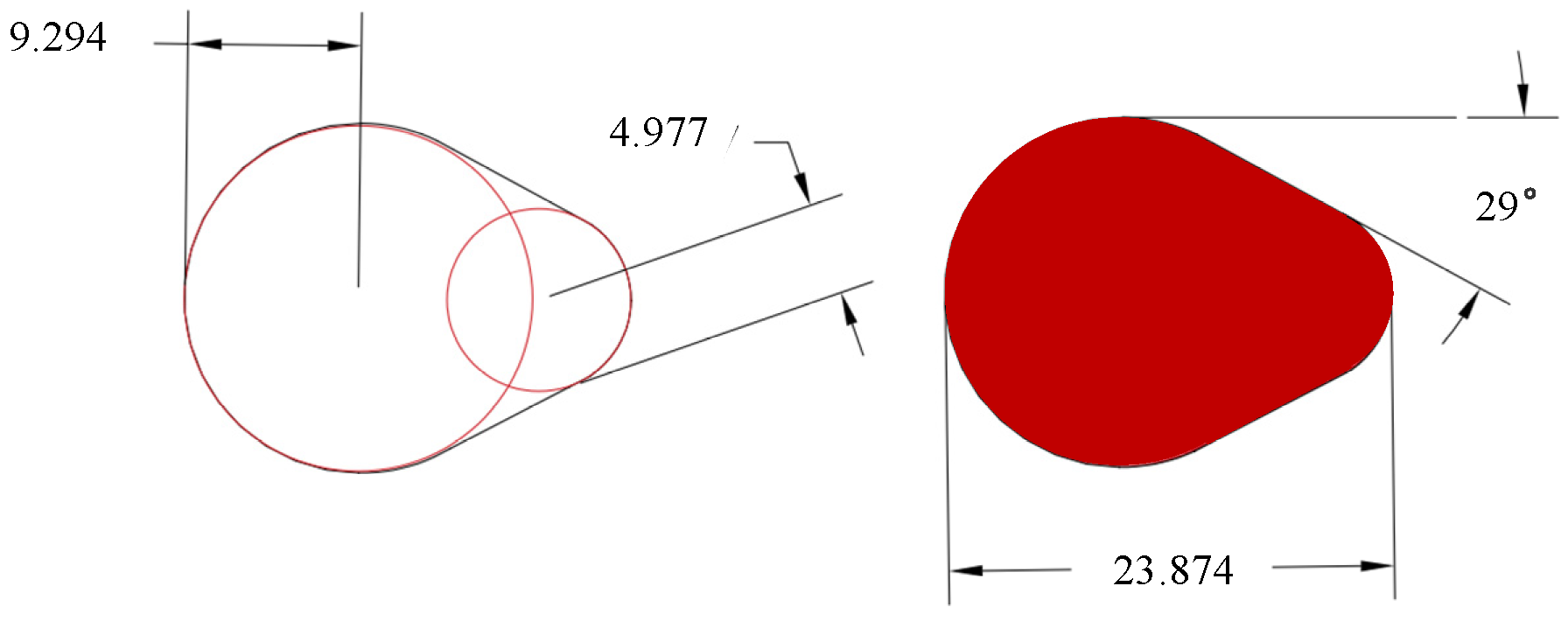
Figure 11.
Coil retention with grooves and slot wedge, and the geometry parameters of the slot wedge.
Figure 11.
Coil retention with grooves and slot wedge, and the geometry parameters of the slot wedge.
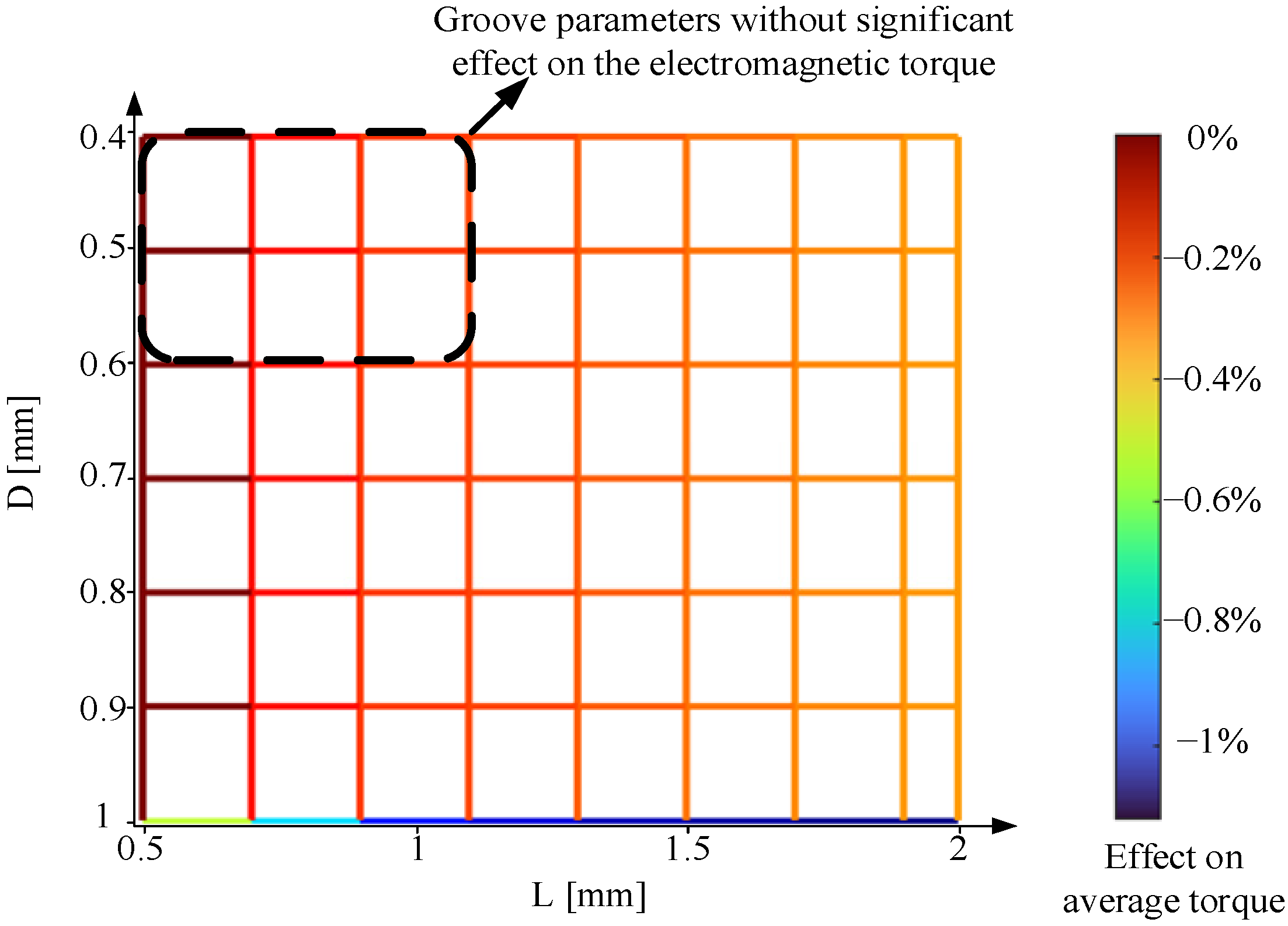
Figure 12.
Effect of groove dimensions on torque production with 0.8 mm groove width.
Figure 12.
Effect of groove dimensions on torque production with 0.8 mm groove width.
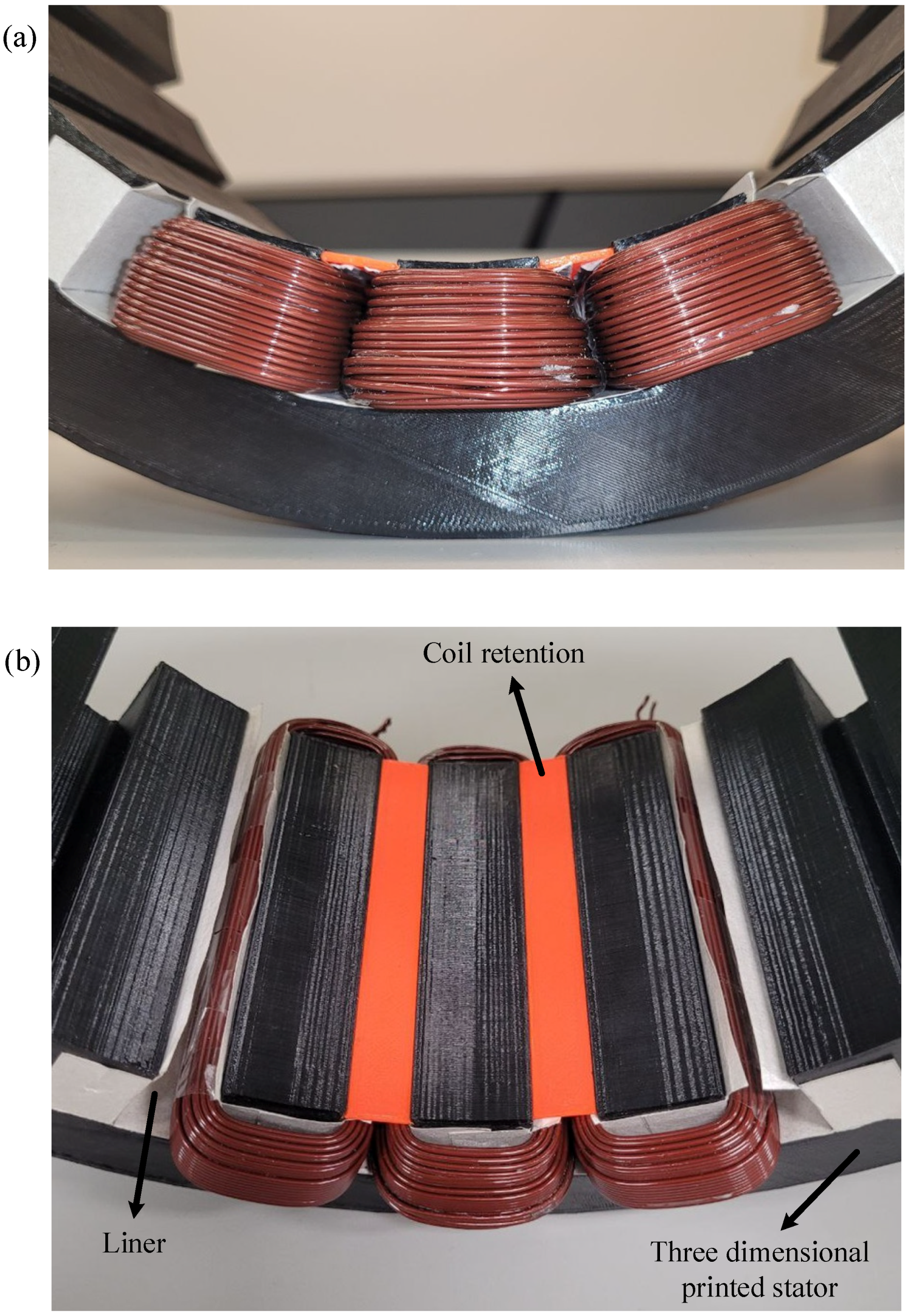
Figure 13.
Experimental verification of coil fit, fill factor, and slot retention with 3D printed stator core for optimized dimensions: (a) front view and (b) top view.
Figure 13.
Experimental verification of coil fit, fill factor, and slot retention with 3D printed stator core for optimized dimensions: (a) front view and (b) top view.
Figure 14.
Rotor cutout dimension and general geometry (dimensions are in mm).
Figure 14.
Rotor cutout dimension and general geometry (dimensions are in mm).
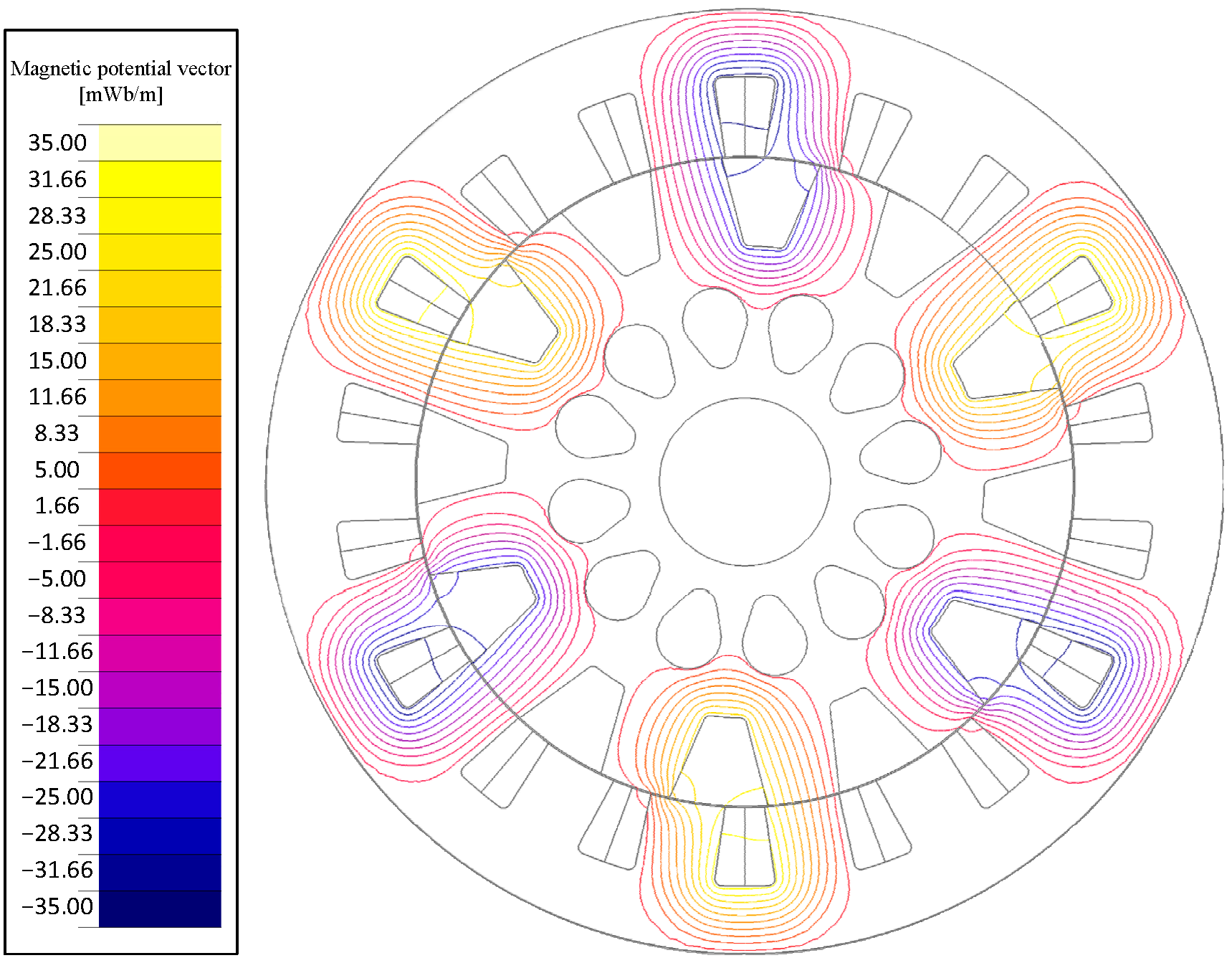
Figure 15.
Magnetic potential vector in the dynamic model of 18/12 SRM.
Figure 15.
Magnetic potential vector in the dynamic model of 18/12 SRM.
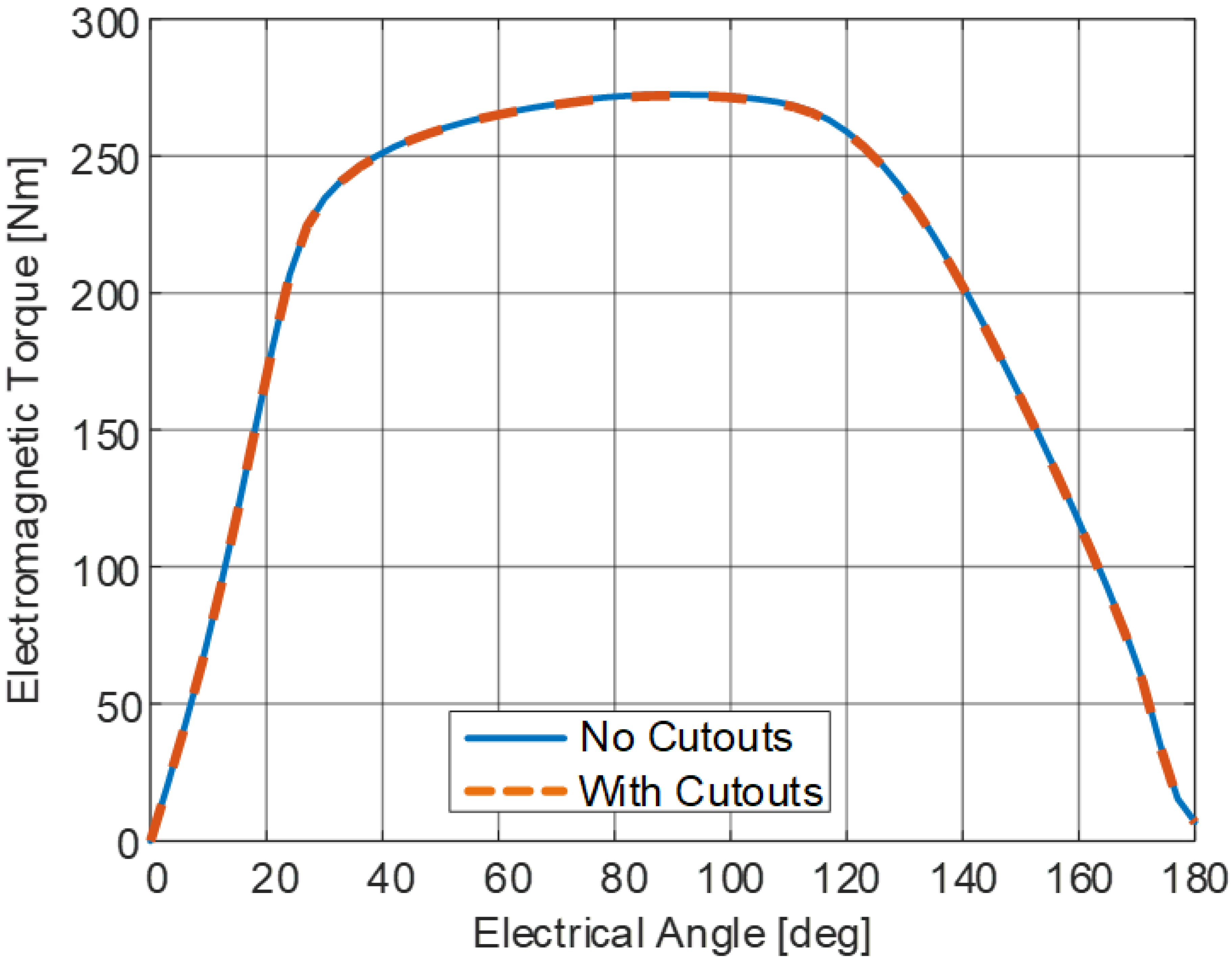
Figure 16.
Static torque for half of an electrical cycle with 195 A current reference before and after rotor cutouts.
Figure 16.
Static torque for half of an electrical cycle with 195 A current reference before and after rotor cutouts.
Figure 17.
Magnetic flux density distribution in the dynamic model of 18/12 LSA SRM.
Figure 17.
Magnetic flux density distribution in the dynamic model of 18/12 LSA SRM.
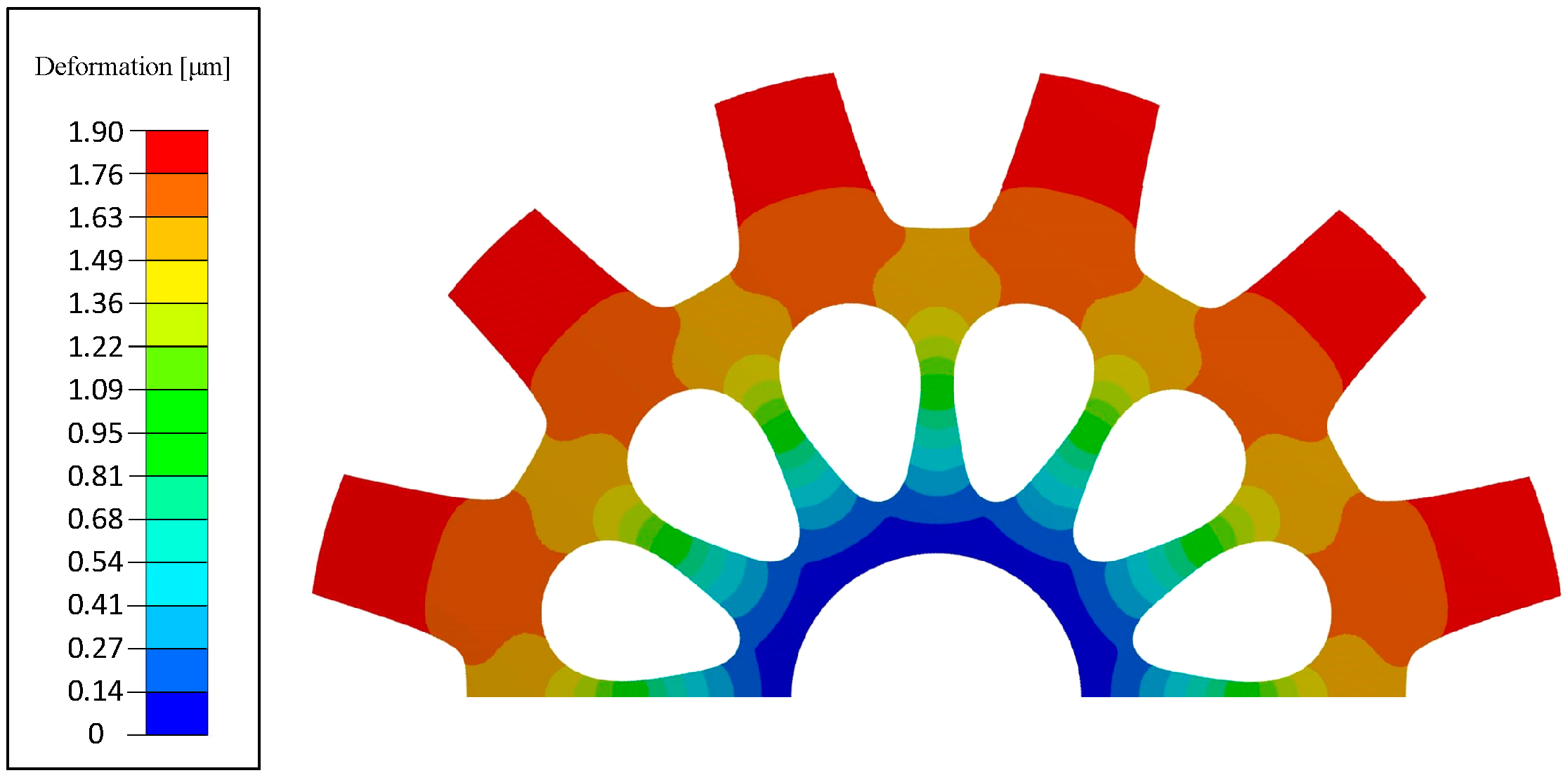
Figure 18.
Total deformation analysis with rotor cutouts at 4500 rpm.
Figure 18.
Total deformation analysis with rotor cutouts at 4500 rpm.
Figure 19.
Von-Mises Stress analysis with rotor cutouts at 4500 rpm.
Figure 19.
Von-Mises Stress analysis with rotor cutouts at 4500 rpm.
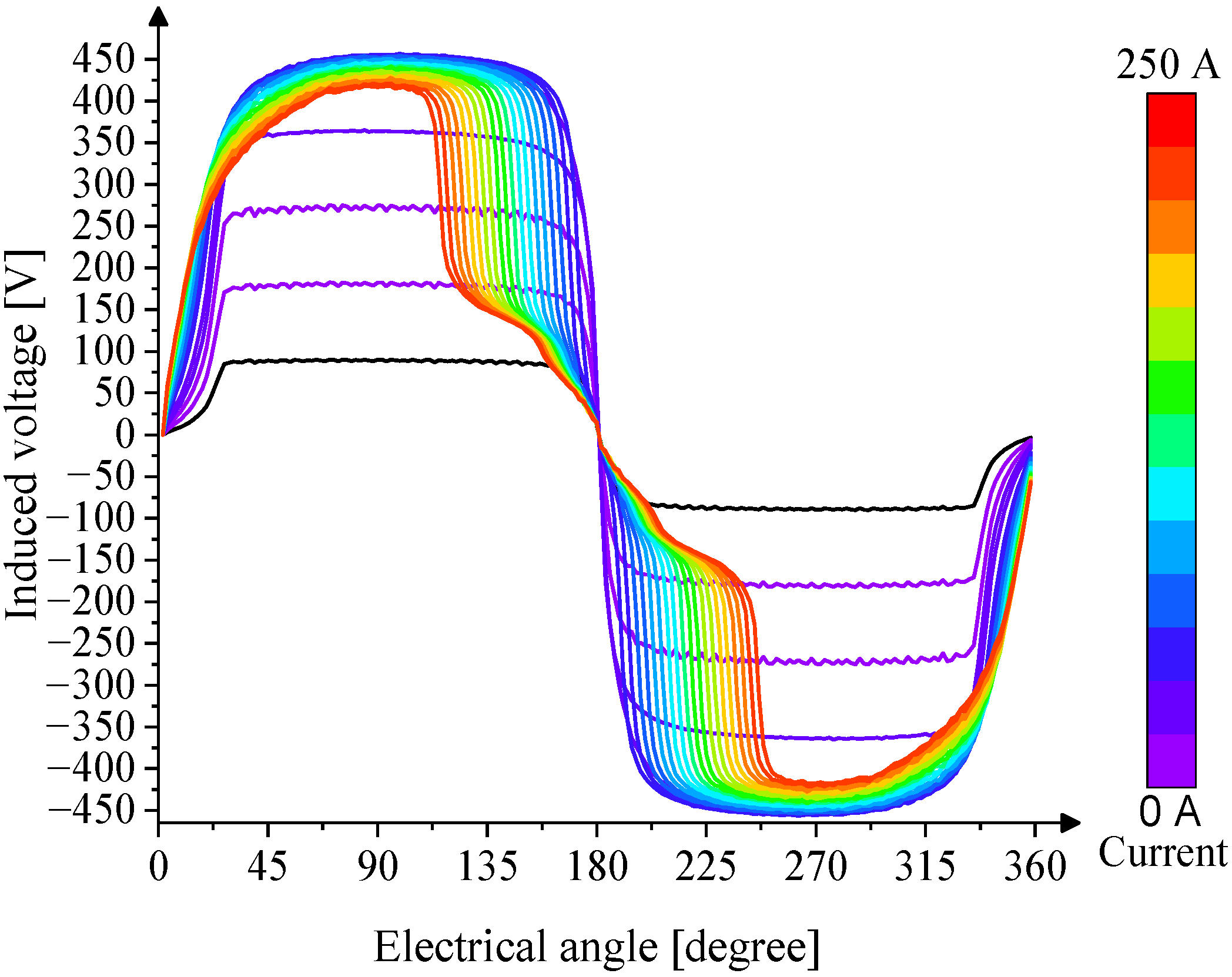
Figure 20.
Static voltage waveform at 2600 rpm for different current references.
Figure 20.
Static voltage waveform at 2600 rpm for different current references.
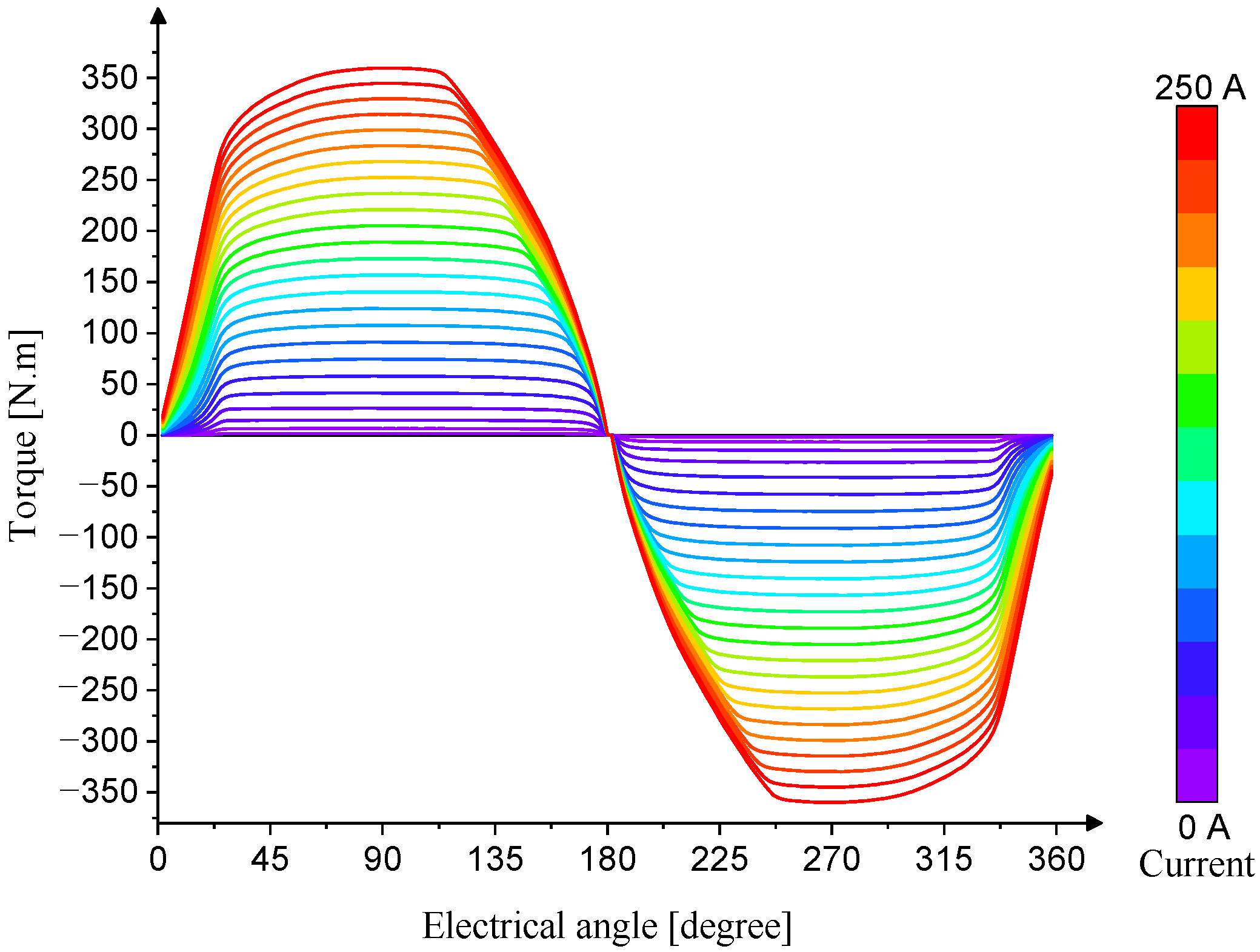
Figure 21.
Static electromagnetic torque for different current references.
Figure 21.
Static electromagnetic torque for different current references.
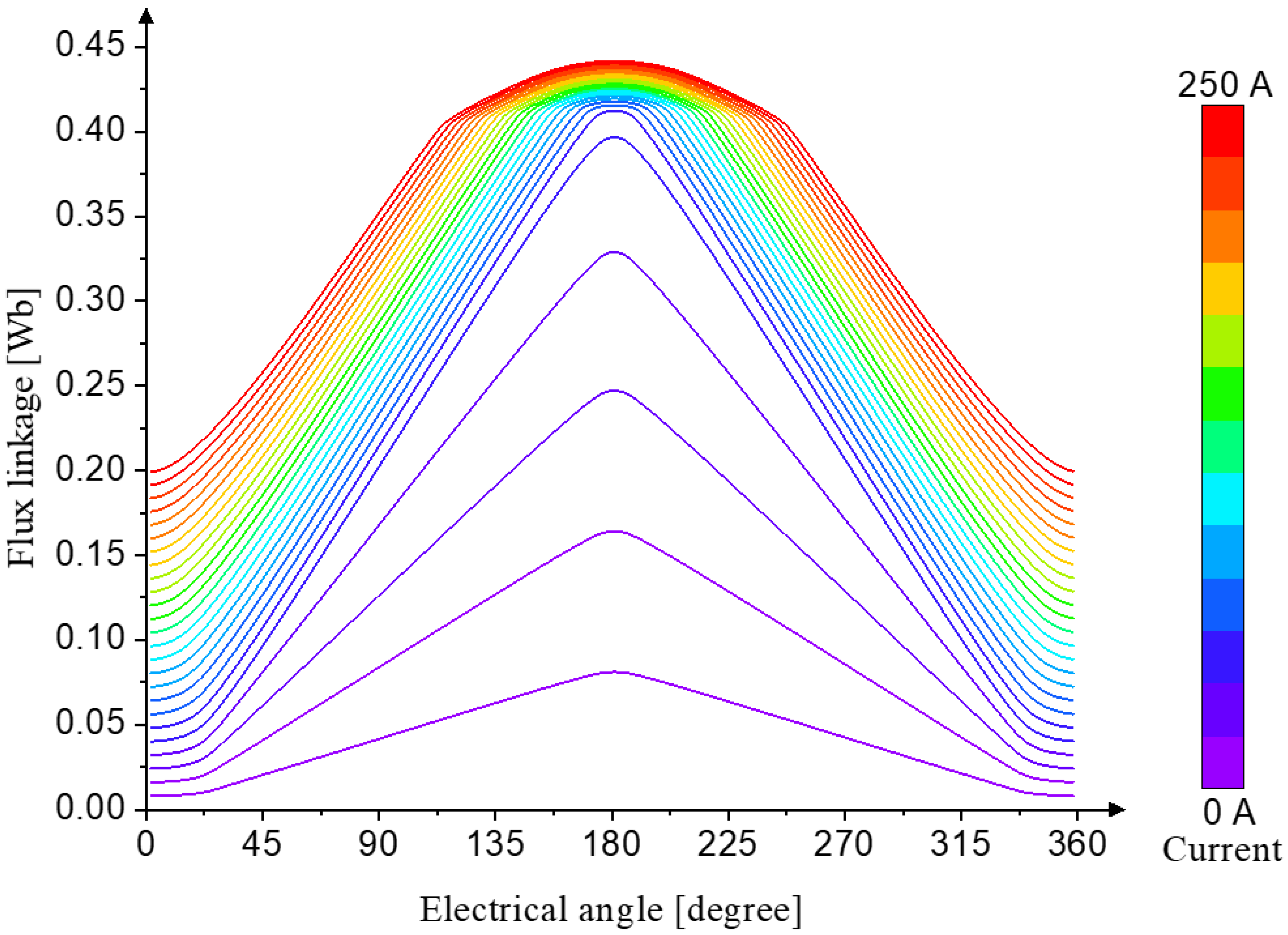
Figure 22.
Phase flux linkage for different current references.
Figure 22.
Phase flux linkage for different current references.
Figure 23.
Dynamic phase current waveforms of LSA SRM at 2600 rpm.
Figure 23.
Dynamic phase current waveforms of LSA SRM at 2600 rpm.
Figure 24.
Comparison of torque production with three-dimensional and two-dimensional FEA at 2600 RPM with conduction angle −47.9° and 125°, and reference current of 200 A.
Figure 24.
Comparison of torque production with three-dimensional and two-dimensional FEA at 2600 RPM with conduction angle −47.9° and 125°, and reference current of 200 A.
Figure 25.
Torque speed characteristics of the designed motor with optimized turn-on and turn-off angles.
Figure 25.
Torque speed characteristics of the designed motor with optimized turn-on and turn-off angles.
Figure 26.
Steady-state thermal FEA result for LSA SRM at the base speed and maximum continuous power.
Figure 26.
Steady-state thermal FEA result for LSA SRM at the base speed and maximum continuous power.
Figure 27.
Transient thermal performance of the LSA SRM calculated in MotorCAD.
Figure 27.
Transient thermal performance of the LSA SRM calculated in MotorCAD.
Table 1.
Specification of SP70d PMSM [
19].
Table 1.
Specification of SP70d PMSM [
19].
| Parameter | Value |
|---|
| Continuous power | 70 kW |
| Continues speed | 2600 rpm |
| Continuous torque @ 2600 rpm | 260 Nm |
| DC-link voltage | 450 V |
| Mass | 26 kg |
| Cooling system | Water/Glycol |
| Max efficiency | 95% |
| Estimated motor diameter | ~300 mm |
| Estimated stack length | 50 to 100 mm |
| Shaft diameter | 50 mm |
Table 2.
The improvements of the motor performance after five iterations in the dynamic design process for the selected design.
Table 2.
The improvements of the motor performance after five iterations in the dynamic design process for the selected design.
| Parameter | Initial Iteration | Final Iteration |
|---|
| Stator pole arc angle, βs | 13.25° | 13.153° |
| Rotor pole arc angle, βr | 12.65° | 12.74° |
| Turn on angle | −54.65° | −47.9° |
| Turn off angle | 122.93° | 125° |
| Average torque | 258.56 N.m | 267.8 N.m |
| Torque ripple (%) | 18.04 | 19.48 |
| Radial force objective | 245.45 | 241.52 |
Table 3.
Coil possibilities for the design.
Table 3.
Coil possibilities for the design.
| Winding Pattern | Number of Turns per Coil | Magnetic Wire American WIRE Gauge (AWG) | Number of Strands | Copper Fill Factor [%] | Current Density (@120 A) [A/mm2] | Phase Resistance (@25 °C) [mΩ] |
|---|
| 6 coils in series | 14 | 9 | 1 | 53.36 | 18.10 | 53.31 |
| 12 | 2 | 53.23 | 18.14 | 53.43 |
| 14 | 3 | 50.25 | 19.21 | 56.64 |
| 15 | 4 | 53.17 | 18.16 | 53.57 |
| 16 | 5 | 52.61 | 18.35 | 54.05 |
| 2-parallel, 3-series | 28 | 12 | 1 | 53.23 | 18.14 | 53.43 |
| 15 | 2 | 53.17 | 18.16 | 53.57 |
| 17 | 3 | 50.20 | 19.23 | 56.80 |
| 3-parallel, 2-series | 42 | 14 | 1 | 50.25 | 19.21 | 56.64 |
| 17 | 2 | 50.20 | 19.23 | 56.80 |
| 6 coils in parallel * | 83 | 17 | 1 | 49.60 | 19.23 | 56.12 |
| 20 | 2 | 49.50 | 19.27 | 56.25 |
Table 4.
Comparison of different coil options.
Table 4.
Comparison of different coil options.
| Parameter | Option 1 | Option 2 |
|---|
| Pattern | 6 P | 6 P |
| American wire gauge (AWG) | 17 | 20 |
| Number of turns | 83 | 83 |
| Number of strands | 1 | 2 |
| Wire fill factor | 0.59 | 0.59 |
| Current density (120 A) | 19.23 A/mm2 | 19.27 A/mm2 |
| Phase resistance @ 25 °C | 56.12 mΩ | 56.25 mΩ |
| Phase resistance with end turn @ 200 °C | 111.2254 mΩ | 111.4831 |
Table 5.
18/12 SRM Final Geometry and Coil Configuration.
Table 5.
18/12 SRM Final Geometry and Coil Configuration.
| Parameter | Value |
|---|
| Number of stator poles | 18 |
| Airgap length | 0.4 mm |
| Stator outside diameter | 280 mm |
| Stator pole arc angle | 13.15° |
| Stack length | 100 mm |
| Wire gauge | 17 AWG |
| Current density (RMS) | 19.23 A/mm2 |
| Phase resistance @ 200 °C | 111.22 mΩ |
| Number of rotor poles | 12 |
| Shaft diameter | 50 mm |
| Rotor pole arc angle | 12.74° |
| Wire fill factor | 59% |
| Number of turns | 83 |
| Number of strands | 1 |
| Phase winding configuration | 6 Parallel |
Table 6.
Modified Coef. and Exp. of Bertotti Method.
Table 6.
Modified Coef. and Exp. of Bertotti Method.
| Exponents | Value | Coefficients | Value |
|---|
| α1 | 1.61939 | k1 | 54.56280 |
| α2 | 2.113459 | k2 | 0.0350734 |
| α3 | 1.386721 | k3 | 6.527015 |
Table 7.
Calculated Losses and Efficiency for Nominal Current Reference and Conduction Angles of −47.9° To 125°.
Table 7.
Calculated Losses and Efficiency for Nominal Current Reference and Conduction Angles of −47.9° To 125°.
| Parameter | Value |
|---|
| Average core loss in the rotor | 287.65 W |
| Average core loss in the stator | 337.52 W |
| Copper loss (including AC copper loss) | 3307.75 W |
| Efficiency | 94.8% |
Table 8.
Thermal Conductivity Characteristics for Materials.
Table 8.
Thermal Conductivity Characteristics for Materials.
| Application | Material | Thermal Cond. [W/m∙K] | Max Temp. [°C] |
|---|
| Stator/Rotor Laminations | HIPERCO® 50A [22] | 29.83 | 704 * |
| Conductors | Copper | 394.0 | 1080 |
| Magnetic Wire | Polyimide [28,29] | 0.26 | 240 |
| Impregnation Material | Lord CoolTherm® EP-2000 [30] | 1.9 | 204 |
| Coil retention | DuraForm® PA plastic [31] | 0.7 | 180 |
| Liner | Nomex 818 [32] | More than 0.1 | 250 |
| Cooling jacket | Aluminum | 247 | 660 |
| Air | Air | 0.024 | -- |
| Moving air | Air | 0.24 | -- |
Table 9.
Heat Transfer Coefficients Guideline.
Table 9.
Heat Transfer Coefficients Guideline.
| Cooling Type | Heat Transfer Coefficient Applied [W/(m2∙K)] |
|---|
| Air flow by salient rotor poles | 150 |
| Liquid cooled | 6000 |