Combined Thermal and Wear Analysis of Linear Rolling Guide Subjected to Rigid–Flexible Coupling Motion Stage
Abstract
1. Introduction
2. Theoretical Analysis
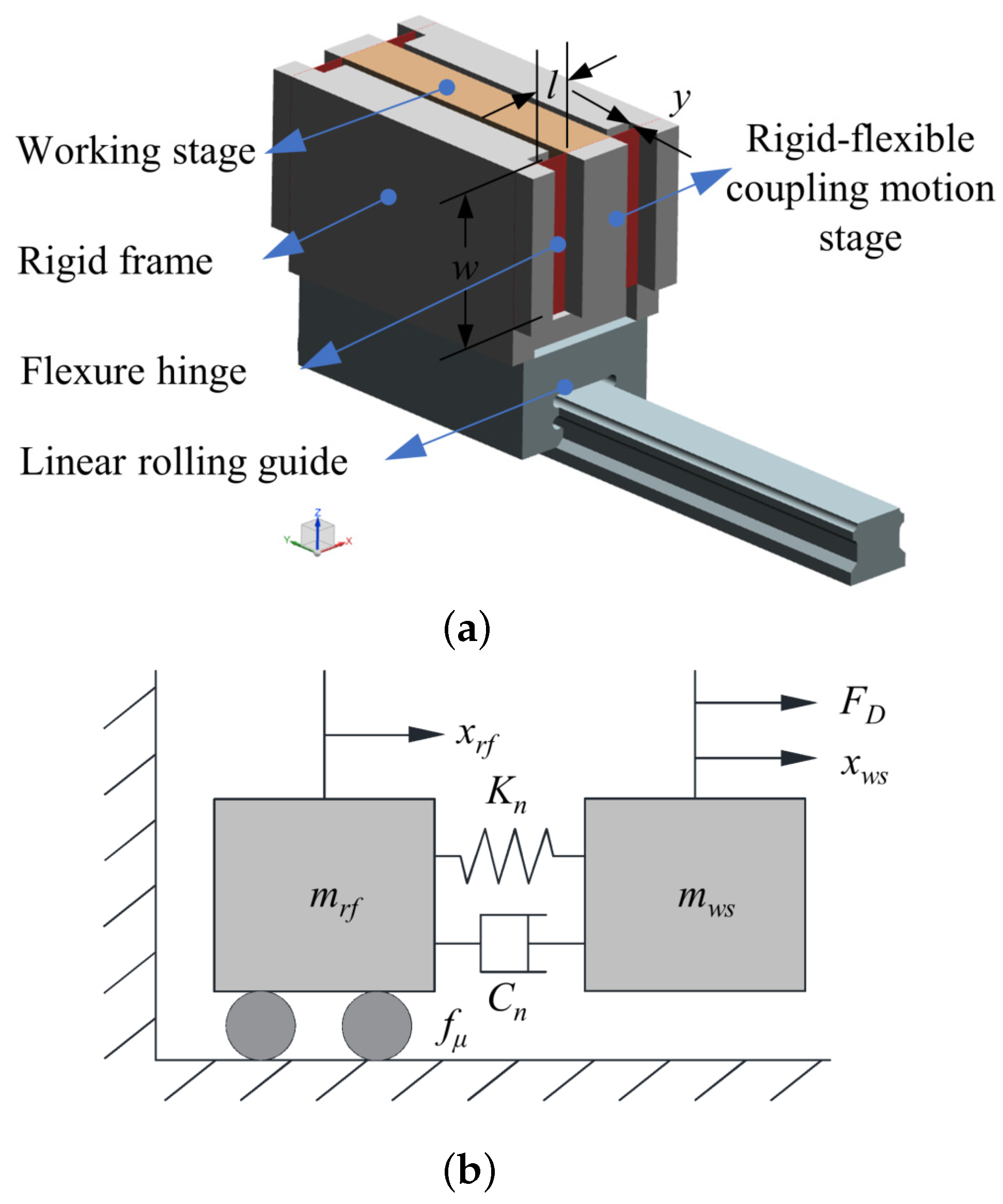
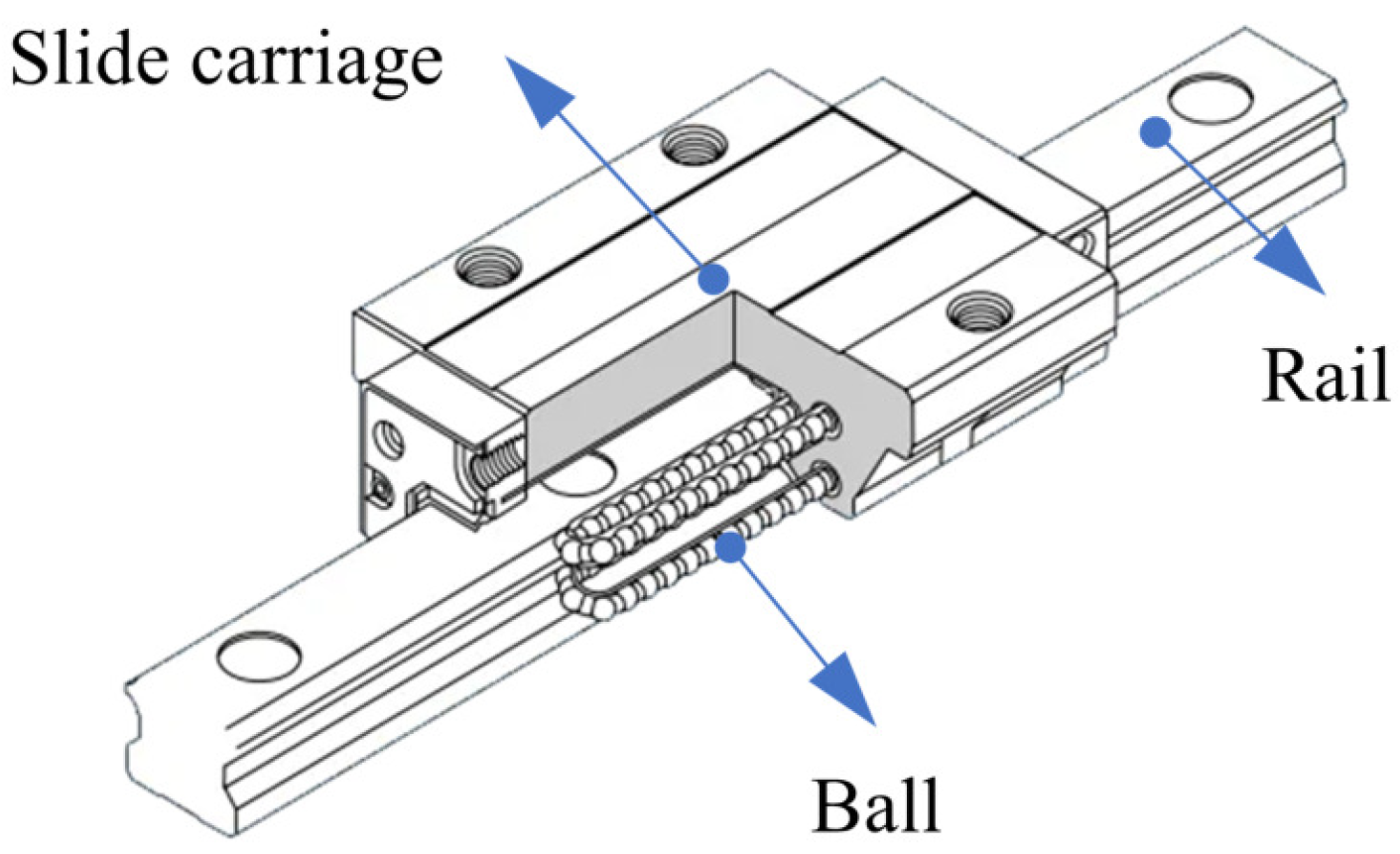
2.1. RFCMS Model
- When the driving force was less than the maximum static friction force (f), the rigid frame was static due to the friction dead-zone, and the flexure hinge utilized the elastic deformation of the material to introduce the displacement from the driving force. In the positioning phase, only the working stage was used for the micro/nano-displacement to achieve precision positioning.
- When the driving force was greater than the maximum static friction force (f), the driving working stage affected the flexure hinge, utilizing the elastic deformation of the material to initiate displacement, and the rigid frame overcame the friction dead-zone and achieved long stroke displacement. In the positioning phase, the rigid frame halted due to friction, the flexure hinge continued to deform, and the working stage completed the final positioning.
2.2. Frictional Heat Generation Model
2.2.1. Basic Modes and Laws of Heat Transfer
2.2.2. Heat Conduction Differential Equation of Temperature Field
2.2.3. Thermal Boundary Conditions of LRG
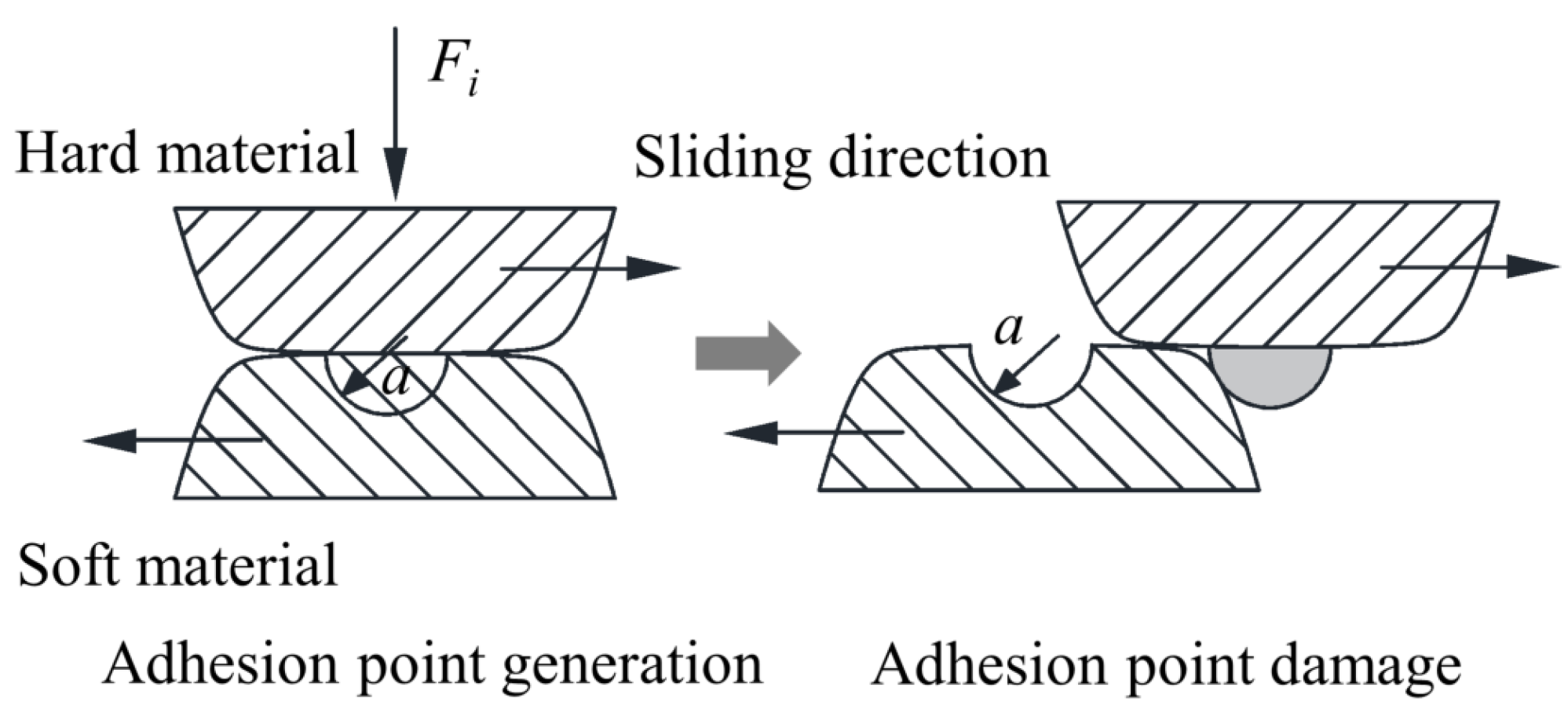
2.3. Wear Prediction Model of LRG
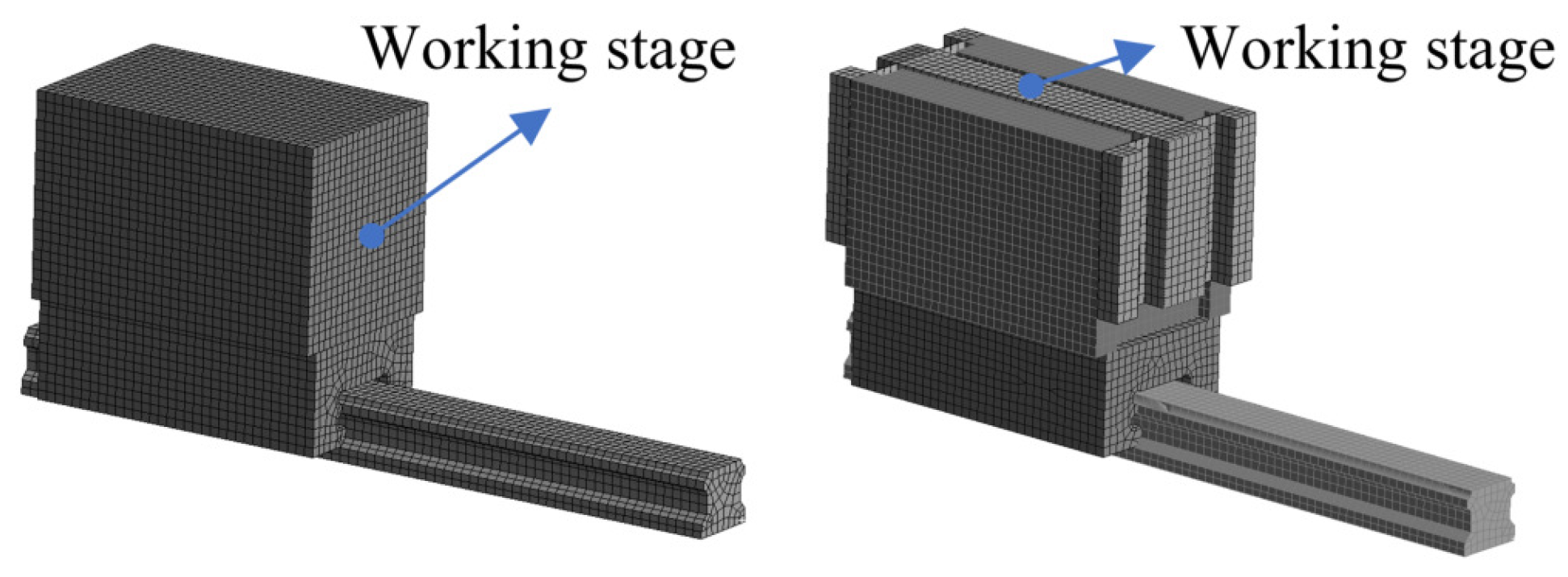
3. Simulations
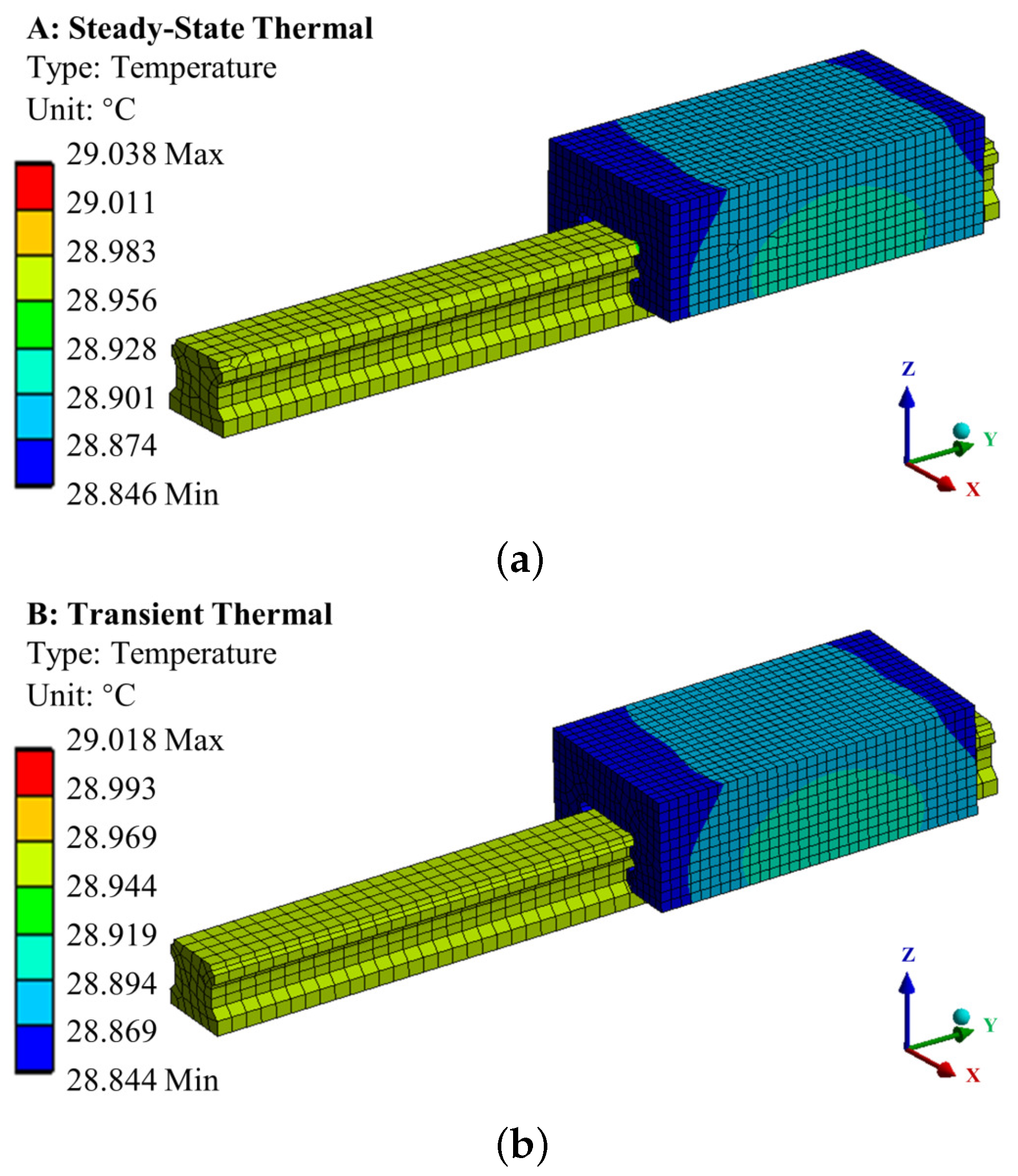
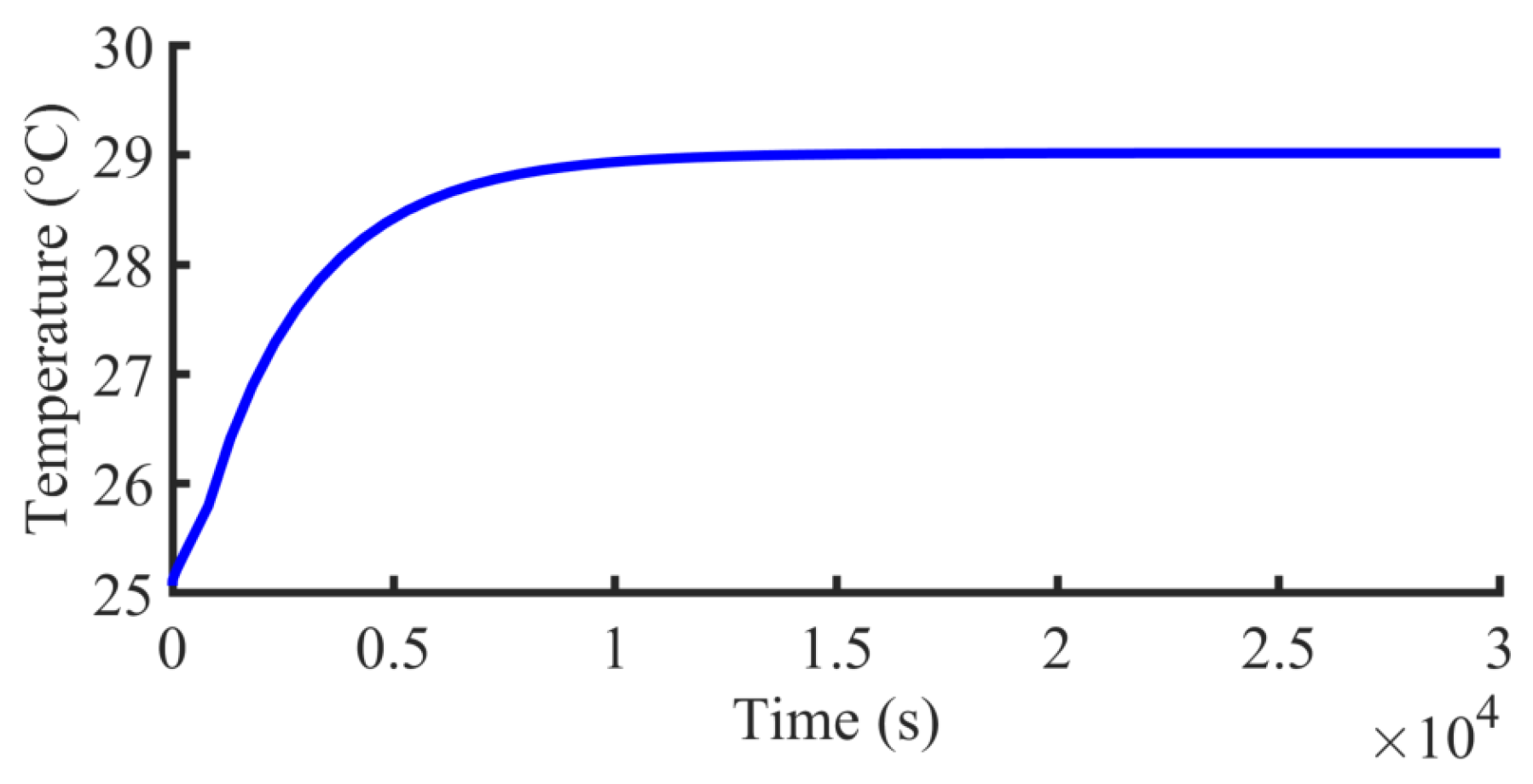
3.1. Steady-State and Transient Thermal Analysis
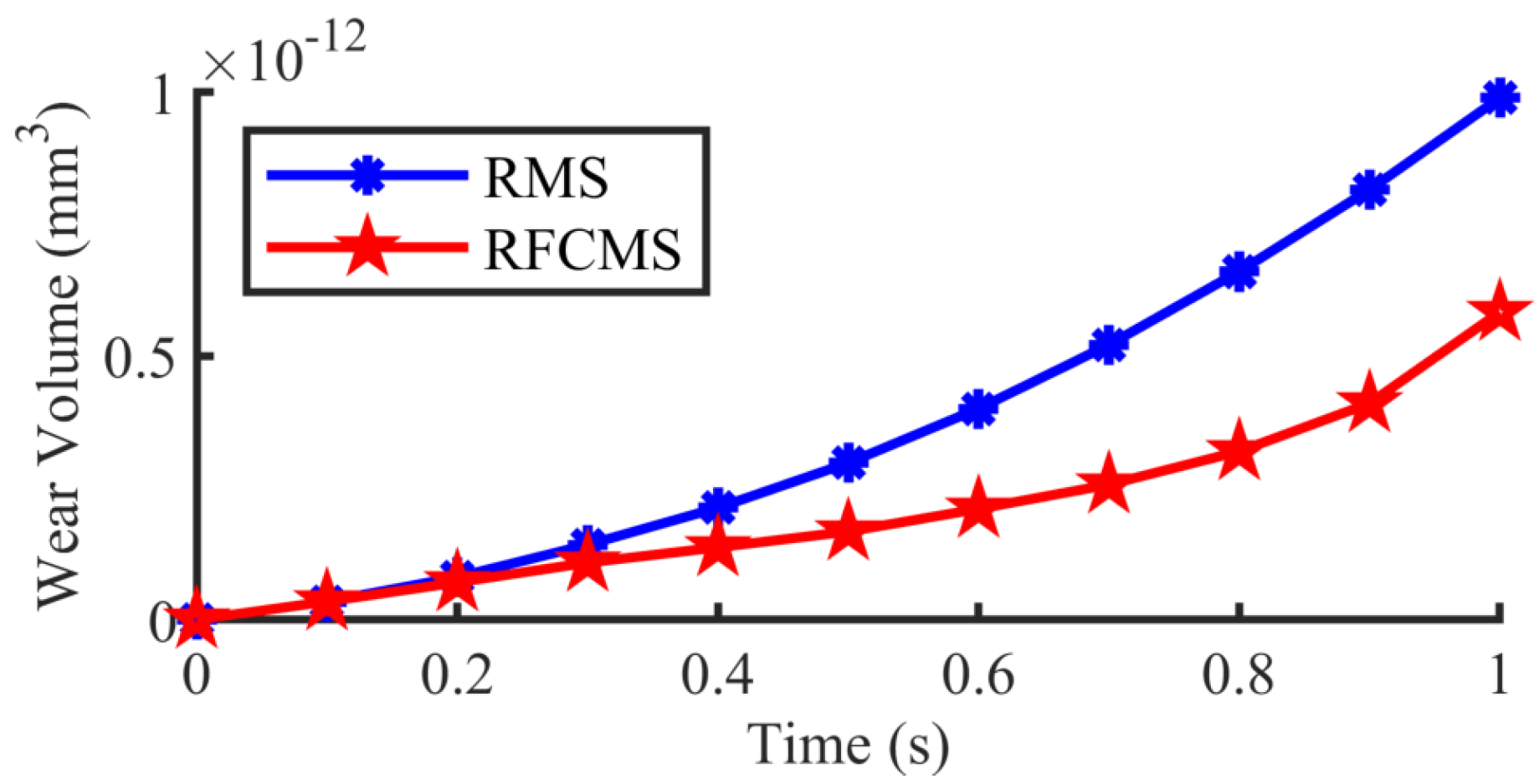
3.2. Verification of Wear Prediction Model
4. Experiment
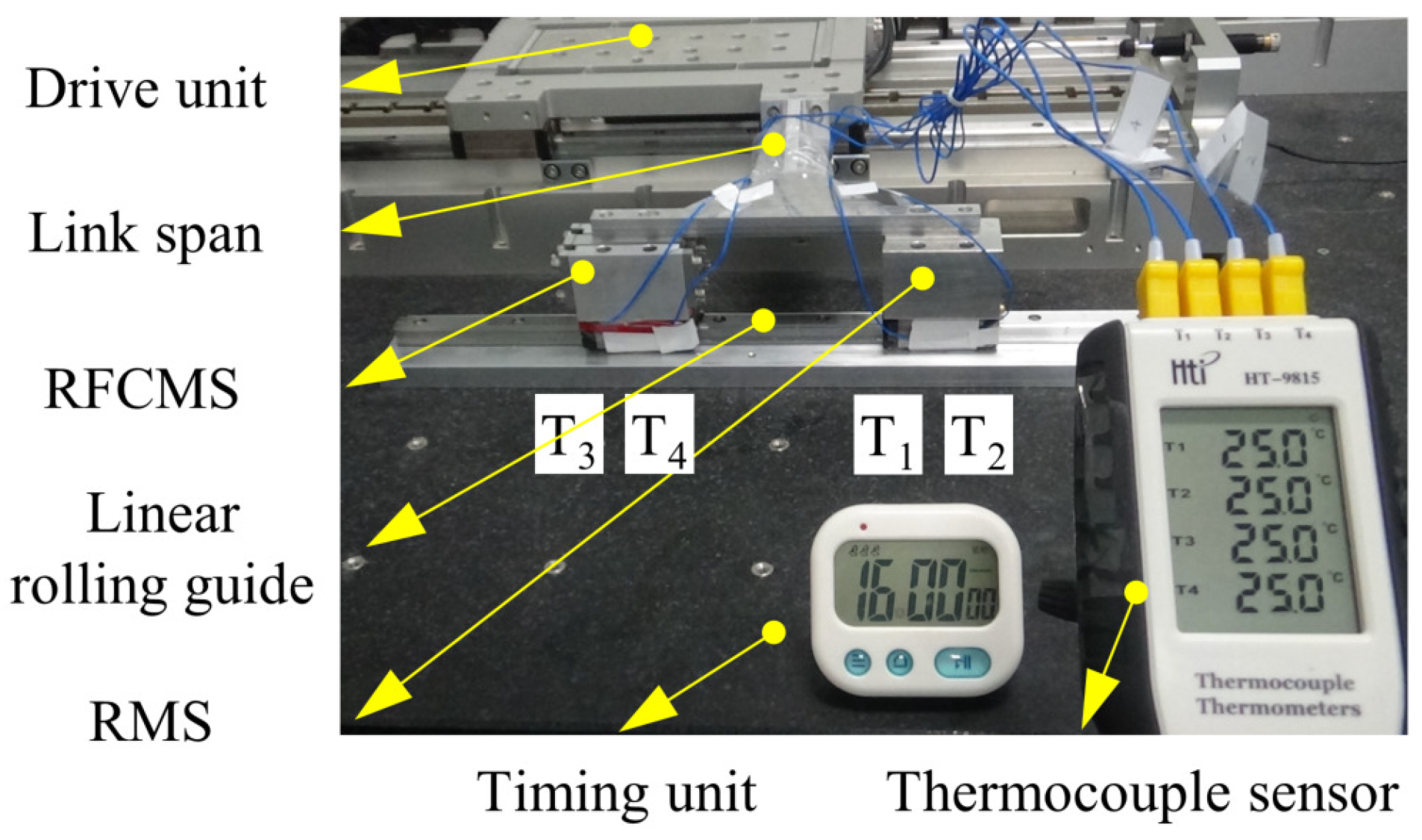
4.1. Description of Apparatus
4.2. Comparative Experiment
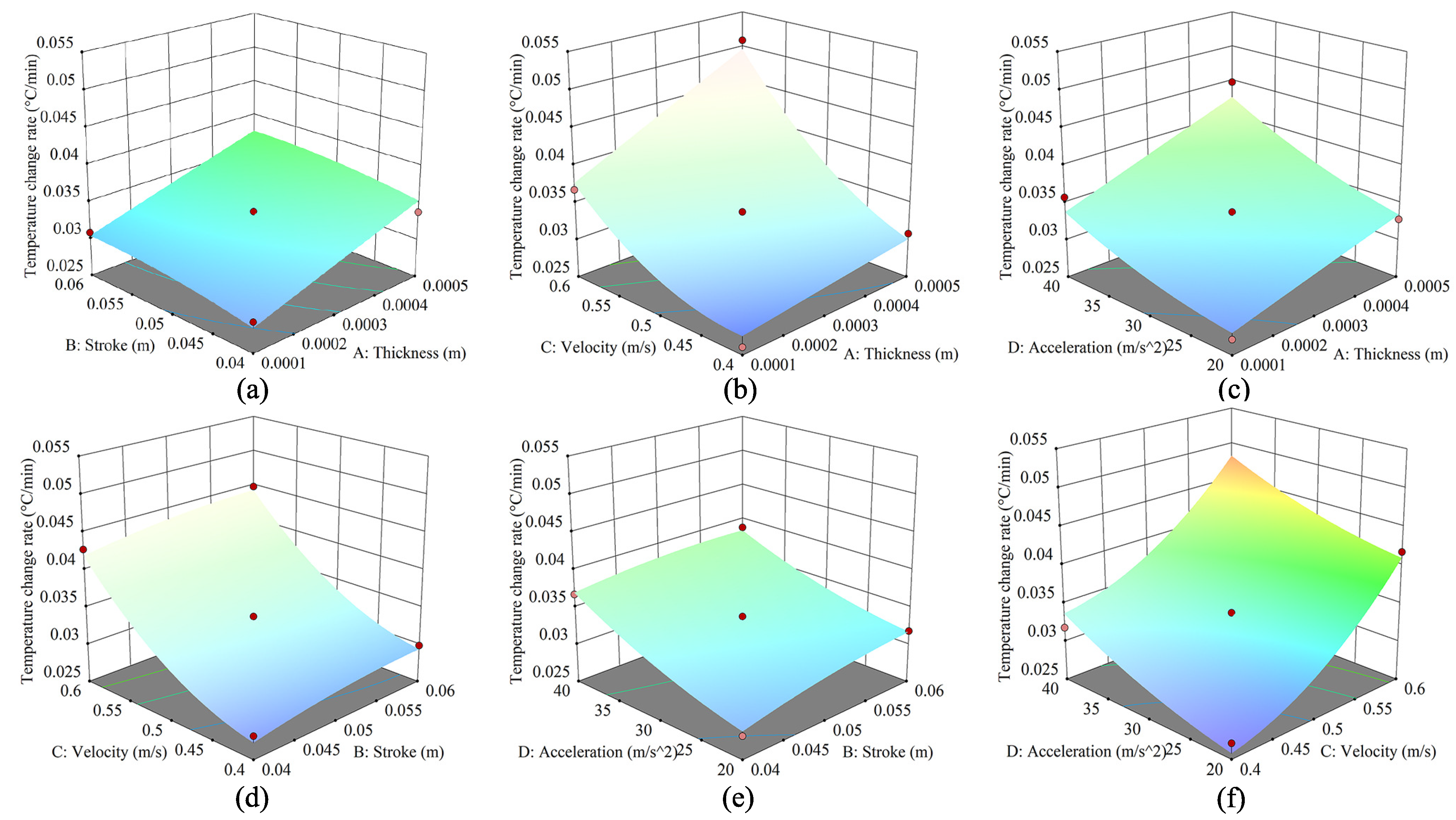
4.3. Response Surface Methodology
5. Conclusions
- (1)
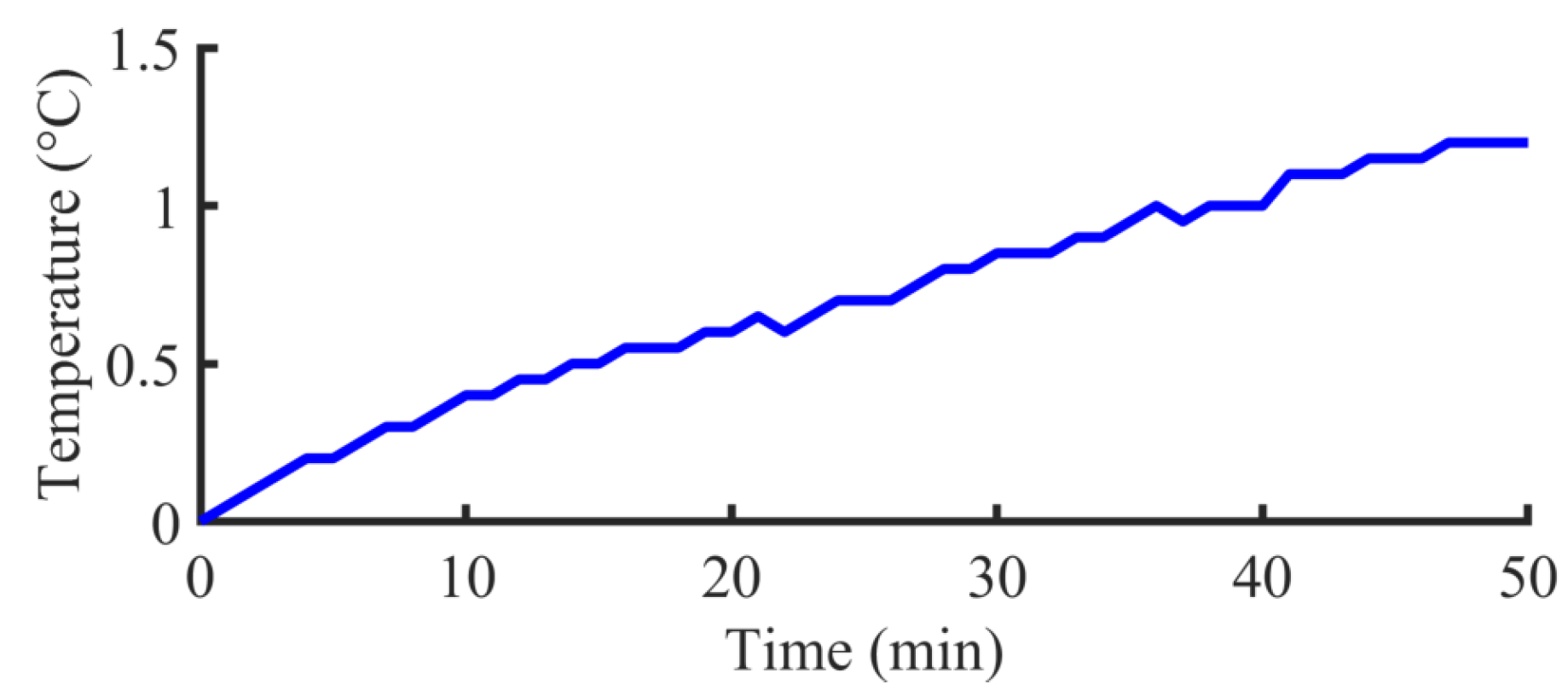
- The differential equation of heat conduction and the thermal boundary conditions of the LRG were developed, and the temperature increase in the LRG due to friction was predicted through steady-state and transient thermal simulations. The LRG reaches a thermal equilibrium state within 5 h, during which the temperature increased rapidly within in the first 1.5 h. Therefore, to avoid the thermal errors caused by increasing temperatures, it was necessary to pre-heat the LRG before its operation.
- (2)
- The wear between the RFCMS and RMS simulation was compared. The wear volume of the RFCMS was small per unit of time at only 58.93% of that found in the RMS. This provided a simulated basis for the reduction in the LRG wear via an RFCMS.
- (3)
- An evaluation method for LRG wear in an RFCMS was proposed. By comparing the temperature increases in friction heat between an RFCMS and an RMS, while under the same motion condition, it was concluded that the TCR of the RMS was higher than in an RFCMS, which verified that the elastic deformation of the flexible hinge of the RFCMS reduced the wear of the LRG. Therefore, an RFCMS could reduce the operating temperature of the LRG and, therefore, reduce the wear on the contact surfaces.
- (4)
- Based on the response surface methodology, the influences of different flexure hinge thicknesses, strokes, velocities, and accelerations on the TCR of the LRG were obtained, and the ARE of the TCR was reported, which could predict the TCR of the LRG under different operating parameters. Through this experiment, the error between the optimized value and the experimental value was only 4.35%. This proved the accuracy of the optimization method.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| LRG Linear rolling guide | |||
| RFCMS Rigid–flexible coupling motion stage | |||
| TCR Temperature change rate | |||
| FEM Finite element model | |||
| RMS Rigid motion stage | |||
| ARE Approximate regression equation | |||
| ANOVA Analysis of variance | |||
| Nomenclature | |||
| m | mass/kg | Dimensionless numbers | |
| x | displacement/m | Nu | Nusselt number |
| K | stiffness/N·m | Re | Reynolds number |
| C | damping/N·s·m | Pr | Prandtl number |
| E | Young’s modulus/GPa | Greek letters | |
| w | width/m | friction coefficient | |
| y | thickness/m | thermal conductivity/W·m·K | |
| l | length/m | density/kg·m | |
| deflection/m | internal heat source intensity/W·m | ||
| f | friction/N | air velocity/ m·s | |
| n | number of flexure hinges | air kinematic viscosity/m·s | |
| q | heat flux/W·m | air dynamic viscosity/N·s·m | |
| t | temperature/°C | contact angle/° | |
| h | heat transfer coefficient/W·m·K | temperature change rate/°C·min | |
| T | temperature field/°C | Subscripts | |
| c | specific heat capacity/J·kg·K | rf | rigid frame |
| Q | frictional heat generation/J·s | ws | work stage |
| F | load/N | t | total |
| v | velocity/m·s | e | surface |
| a | acceleration/m·s | ∞ | ambient |
| J | thermal equivalent/J·cal | s | static |
| V | volume/m | k | dynamic |
| L | feature size/m | wear | wear |
| K | adhesive wear coefficient | b | ball |
| L | sliding distance/m | r | raceway |
| H | Brinell hardness | 0 | initial |
| R | ball radius/m | 1 | end |
| O | form factor | i | normal |
| N | total number of balls | z | on slide carriage |
| u | time/min | D | motor |
| / | maximum/static friction | ||
References
- Lin, R.; Li, Y.; Zhang, Y.; Wang, T.; Wang, Z.; Song, Z.; Dou, Z.; Qian, J. Design of A flexure-based mixed-kinematic XY high-precision positioning platform with large range. Mech. Mach. Theory 2019, 142, 103609. [Google Scholar] [CrossRef]
- Zhang, L.; Gao, J.; Chen, X. A Rapid Dynamic Positioning Method for Settling Time Reduction Through a Macro–Micro Composite Stage With High Positioning Accuracy. IEEE Trans. Ind. Electron. 2018, 65, 4849–4860. [Google Scholar] [CrossRef]
- He, G.; Sun, G.; Zhang, H.; Huang, C.; Zhang, D. Hierarchical Error Model to Estimate Motion Error of Linear Motion Bearing Table. Int. J. Adv. Manuf. Technol. 2017, 93, 5–8. [Google Scholar] [CrossRef]
- Chen, C.; Wang, J.; Huang, G.; Hsu, M.; Chen, T. High-Precision Rapid Prototyping Technology for Manufacturing Linear Guides. Int. J. Adv. Manuf. Technol. 2017, 92, 9–12. [Google Scholar] [CrossRef]
- Li, X.; Li, Z.; Jin, S.; Zhang, J.; Ding, S.; Niu, Z. A Novel Error Equivalence Model on the Kinematic Error of the Linear Axis of High-End Machine Tool. Int. J. Adv. Manuf. Technol. 2022, 118, 7–8. [Google Scholar] [CrossRef]
- Hu, P.; Lei, Y.; Ou, Y. Analysis of Motion Errors of Linear Guide Pair Based on Parallel Mechanism. Machines 2021, 9, 33. [Google Scholar] [CrossRef]
- Dong, X.; Okwudire, C.E. An Experimental Investigation of the Effects of the Compliant Joint Method on Feedback Compensation of Pre-Sliding/Pre-Rolling Friction. Precis. Eng. 2018, 54, 81–90. [Google Scholar] [CrossRef]
- Wang, X.; Feng, H.; Zhou, H.; Zhou, C.; Chen, Z. Modeling of Friction Forces for Linear Motion Ball Guides Considering Variation of the Friction Parameter with Operating Condition. Tribol. Trans. 2022, 65, 592–609. [Google Scholar] [CrossRef]
- Tao, W.; Zhong, Y.; Feng, H.; Wang, Y. Model for Wear Prediction of Roller Linear Guides. Wear 2013, 305, 260–266. [Google Scholar] [CrossRef]
- Weng, L.; Gao, W.; Zhang, D.; Huang, T.; Liu, T.; Li, W.; Zheng, Y.; Shi, K.; Chang, W. Analytical Modelling Method for Thermal Balancing Design of Machine Tool Structural Components. Int. J. Mach. Tools Manuf. 2021, 164, 103715. [Google Scholar] [CrossRef]
- Wang, X.; Feng, H.; Zhou, C.; Ye, K. A Thermal Model for Real-Time Temperature Forecast of Rolling Linear Guide Considering Loading Working Conditions. Int. J. Adv. Manuf. Technol. 2020, 109, 2249–2271. [Google Scholar] [CrossRef]
- Zou, H.; Wang, B. Investigation of the Contact Stiffness Variation of Linear Rolling Guides Due to the Effects of Friction and Wear during Operation. Tribol. Int. 2015, 92, 472–484. [Google Scholar] [CrossRef]
- Chen, M.; Huang, W.; Chen, Y. Design of the aerostatic linear guideway with a passive disk-spring compensator for PCB drilling machine. Tribol. Int. 2010, 43, 395–403. [Google Scholar] [CrossRef]
- Lee, S.; Yoo, J.; Yang, M. Effect of Thermal Deformation on Machine Tool Slide Guide Motion. Tribol. Int. 2003, 36, 41–47. [Google Scholar] [CrossRef]
- Ji, J.; Hong, R.; Sun, F.; Huang, X. Thermal characteristic analysis of Z-axis guideway based on thermal contact resistance. Adv. Mech. Eng. 2018, 10, 168781401880532. [Google Scholar] [CrossRef]
- Tong, V.; Khim, G.; Hong, S. Effects of Carriage Flexibility on Friction Force in Linear Ball Guides. J. Tribol. 2021, 143, 121202. [Google Scholar] [CrossRef]
- Xu, S.; Cheng, D.; Zhang, S. Analysis of friction fluctuations mechanism of a preloaded roller linear motion guide based on a new 5-DOF dynamic stiffness model. Measurement 2022, 190, 110768. [Google Scholar] [CrossRef]
- Cheng, D.; Xu, F.; Xu, S.; Kim, S. Investigation of the Frictional Behavior in a Roller Linear Motion Guide under Mixed EHL. Tribol. Trans. 2020, 63, 528–542. [Google Scholar] [CrossRef]
- Oh, K.; Khim, G.; Park, C.; Chung, S. Explicit Modeling and Investigation of Friction Forces in Linear Motion Ball Guides. Tribol. Int. 2019, 129, 16–28. [Google Scholar] [CrossRef]
- Yun, W.S.; Kim, S.K.; Cho, D.W. Thermal error analysis for a CNC lathe feed drive system. Int. J. Mach. Tools Manuf. 1999, 39, 1087–1101. [Google Scholar] [CrossRef]
- Su, L.Y.; Huang, R.R.; Peng, H.; Yang, Z.J. Optimal Design for Parameters of Structure and Controller for Rigid-Flexible Coupling Motion Stage. In Proceedings of the 2020 IEEE 9th Data Driven Control and Learning Systems Conference (DDCLS), Liuzhou, China, 20–22 November 2020; pp. 1188–1192. [Google Scholar]
- Peng, H.; Yang, Z.; Xue, W.; Huang, R.; Huang, Y. The Design and Control of a Rigid-Flexible Coupling Positioning Stage for Enhanced Settling Performance. J. Dyn. Syst. Meas. Control 2021, 143, 111003. [Google Scholar] [CrossRef]
- Yang, Z.; Bai, Y.; Chen, X.; Wang, M.; Gao, J.; Yang, H. A New Design Method of Dynamic Characteristics Adjustable Micro Motion Stage Based on Tension Stiffening. J. Mech. Eng. 2015, 51, 153. (In Chinese) [Google Scholar] [CrossRef]
- Jang, S.; Khim, G.; Park, C. Estimation of friction heat in a linear motion bearing using Box–Behnken design. Int. J. Adv. Manuf. Technol. 2017, 89, 2021–2029. [Google Scholar] [CrossRef]
- Cheng, D.; Park, J.; Suh, J.; Kim, S.; Park, C. Effect of Frictional Heat Generation on the Temperature Distribution in Roller Linear Motion Rail Surface. J. Mech. Sci. Technol. 2017, 31, 1477–1487. [Google Scholar] [CrossRef]
- Chow, J.; Zhong, Z.; Lin, W.; Khoo, L. A Study of Thermal Deformation in the Carriage of a Permanent Magnet Direct Drive Linear Motor Stage. Appl. Therm. Eng. 2012, 48, 89–96. [Google Scholar] [CrossRef]
- Archard, J. Contact and Rubbing of Flat Surfaces. J. Appl. Phys. 1953, 24, 981–988. [Google Scholar] [CrossRef]
- Kragelski, N.B. Calculation Principle of Friction and Wear; China Machine Press: Beijing, China, 1982. [Google Scholar]
- Rabinowicz, E. Wear Coefficients Metals; Wear Control Handbook; The American Society of Mechanical Engineering: New York, NY, USA, 1980. [Google Scholar]
- Wang, J.; Zhang, G.; Fan, H.; Fan, Z.; Huang, Y. Effect of Off-Sized Balls on Contact Stiffness and Stress and Analysis of the Wear Prediction Model of Linear Rolling Guideways. Adv. Mech. Eng. 2021, 13, 168781402110344. [Google Scholar] [CrossRef]
- Zhang, J.; Peng, Y.; Liu, H.; Liu, Y. Analysis of Wear Mechanism and Influence Factors of Drum Segment of Hot Rolling Coiler. Chin. J. Mech. Eng. 2013, 26, 351–355. [Google Scholar] [CrossRef]
- Takabi, J.; Khonsari, M. Experimental Testing and Thermal Analysis of Ball Bearings. Tribol. Int. 2013, 60, 93–103. [Google Scholar] [CrossRef]

| Type | Parameter |
|---|---|
| Initial pre-load, F/(N) | 171 (low pre-load) |
| Ball radius, R/(mm) | 1.3497 |
| Raceway radius, R/(mm) | 1.4307 |
| Form factor, O | 0.52 |
| Total number of balls, N | 18 |
| Contact angle, /(°) | 45 |
| Type | Material | Density (kg/m) | Young’s Modulus (GPa) | Poisson’s Ratio | Thermal Conductivity W/(m·K) | Specific Heat Capacity J/(kg·K) |
|---|---|---|---|---|---|---|
| Rail/slide carriage | GCr15 | 7830 | 219 | 0.3 | 44 | 460 |
| Ball | 40Cr | 7870 | 211 | 0.277 | 44 | 460 |
| Stage | 6061-T6 Al | 2700 | 69 | 0.33 | 166.9 | 896 |
| Flexure Hinge | 65Mn | 7820 | 211 | 0.288 | 48 | 450 |
| Levels | |||
|---|---|---|---|
| Variables | −1 | 0 | 1 |
| Thickness (m) | 0.0001 | 0.0003 | 0.0005 |
| Stroke (m) | 0.04 | 0.05 | 0.06 |
| Velocity (m/s) | 0.4 | 0.5 | 0.6 |
| Acceleration (m/s) | 20 | 30 | 40 |
| Order | A (m) | B (m) | C (m/s) | D (m/s) | (°C/min) |
|---|---|---|---|---|---|
| 1 | 0.0003 | 0.04 | 0.4 | 30 | 0.028 |
| 2 | 0.0005 | 0.04 | 0.5 | 30 | 0.034 |
| 3 | 0.0003 | 0.05 | 0.6 | 40 | 0.046 |
| 4 | 0.0003 | 0.06 | 0.4 | 30 | 0.030 |
| 5 | 0.0003 | 0.05 | 0.4 | 40 | 0.032 |
| 6 | 0.0003 | 0.05 | 0.5 | 30 | 0.034 |
| 7 | 0.0005 | 0.05 | 0.6 | 30 | 0.051 |
| 8 | 0.0003 | 0.04 | 0.5 | 40 | 0.037 |
| 9 | 0.0003 | 0.04 | 0.6 | 30 | 0.043 |
| 10 | 0.0001 | 0.05 | 0.5 | 20 | 0.027 |
| 11 | 0.0001 | 0.05 | 0.4 | 30 | 0.026 |
| 12 | 0.0005 | 0.05 | 0.5 | 40 | 0.045 |
| 13 | 0.0001 | 0.04 | 0.5 | 30 | 0.029 |
| 14 | 0.0005 | 0.06 | 0.5 | 30 | 0.036 |
| 15 | 0.0003 | 0.06 | 0.5 | 40 | 0.039 |
| 16 | 0.0003 | 0.05 | 0.6 | 20 | 0.042 |
| 17 | 0.0003 | 0.05 | 0.5 | 30 | 0.033 |
| 18 | 0.0001 | 0.05 | 0.6 | 30 | 0.037 |
| 19 | 0.0005 | 0.05 | 0.4 | 30 | 0.031 |
| 20 | 0.0003 | 0.06 | 0.6 | 30 | 0.045 |
| 21 | 0.0003 | 0.05 | 0.5 | 30 | 0.034 |
| 22 | 0.0003 | 0.04 | 0.5 | 20 | 0.028 |
| 23 | 0.0005 | 0.05 | 0.5 | 20 | 0.033 |
| 24 | 0.0001 | 0.06 | 0.5 | 30 | 0.031 |
| 25 | 0.0003 | 0.06 | 0.5 | 20 | 0.032 |
| 26 | 0.0001 | 0.05 | 0.5 | 40 | 0.036 |
| 27 | 0.0003 | 0.05 | 0.4 | 20 | 0.027 |
| Source | Sum of Squares | df | Mean Square | F-Value | p-Value |
|---|---|---|---|---|---|
| Model | 1.11 × 10 | 14 | 7.92 × 10 | 26.03 | <0.0001 |
| A | 1.61 × 10 | 1 | 1.61 × 10 | 53.04 | <0.0001 |
| B | 1.63 × 10 | 1 | 1.63 × 10 | 5.37 | 0.0390 |
| C | 6.75 × 10 | 1 | 6.75 × 10 | 221.92 | <0.0001 |
| D | 1.76 × 10 | 1 | 1.76 × 10 | 57.97 | <0.0001 |
| AB | 0.00 | 1 | 0.00 | 0.00 | 1.0000 |
| AC | 2.03 × 10 | 1 | 2.03 × 10 | 6.66 | 0.0241 |
| AD | 2.25 × 10 | 1 | 2.25 × 10 | 0.74 | 0.4066 |
| BC | 0.00 | 1 | 0.00 | 0.00 | 1.0000 |
| BD | 1.00 × 10 | 1 | 1.00 × 10 | 0.33 | 0.5770 |
| CD | 2.50 × 10 | 1 | 2.50 × 10 | 0.082 | 0.7792 |
| A | 9.26 × 10 | 1 | 9.26 × 10 | 3.04 × 10 | 0.9569 |
| B | 1.57 × 10 | 1 | 1.57 × 10 | 0.51 | 0.4869 |
| C | 3.91 × 10 | 1 | 3.91 × 10 | 12.86 | 0.0037 |
| D | 4.90 × 10 | 1 | 4.90 × 10 | 1.61 | 0.2285 |
| Residual | 3.65 × 10 | 12 | 3.04 × 10 | ||
| Lack of Fit | 3.58 × 10 | 10 | 3.58 × 10 | 10.75 | 0.0880 |
| Pure Error | 6.67 × 10 | 2 | 3.33 × 10 | ||
| Cor Total | 1.15 × 10 | 26 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Su, L.; Zhang, C.; Yang, Z. Combined Thermal and Wear Analysis of Linear Rolling Guide Subjected to Rigid–Flexible Coupling Motion Stage. Machines 2023, 11, 358. https://doi.org/10.3390/machines11030358
Su L, Zhang C, Yang Z. Combined Thermal and Wear Analysis of Linear Rolling Guide Subjected to Rigid–Flexible Coupling Motion Stage. Machines. 2023; 11(3):358. https://doi.org/10.3390/machines11030358
Chicago/Turabian StyleSu, Liyun, Chunheng Zhang, and Zhijun Yang. 2023. "Combined Thermal and Wear Analysis of Linear Rolling Guide Subjected to Rigid–Flexible Coupling Motion Stage" Machines 11, no. 3: 358. https://doi.org/10.3390/machines11030358
APA StyleSu, L., Zhang, C., & Yang, Z. (2023). Combined Thermal and Wear Analysis of Linear Rolling Guide Subjected to Rigid–Flexible Coupling Motion Stage. Machines, 11(3), 358. https://doi.org/10.3390/machines11030358





