Analysis of the Magneto-Thermal Bidirectional Coupling Strength of Macro and Micro Integration for Self-Starting Permanent Magnet Hysteresis Synchronous Motors
Abstract
:1. Introduction
2. Temperature Stability Calculation of AlNiCo
3. Structure and Strength Analysis of Permanent Magnet Hysteresis Column Rotor
4. Magneto-Thermal Bidirectional Coupling Strength of Rotor
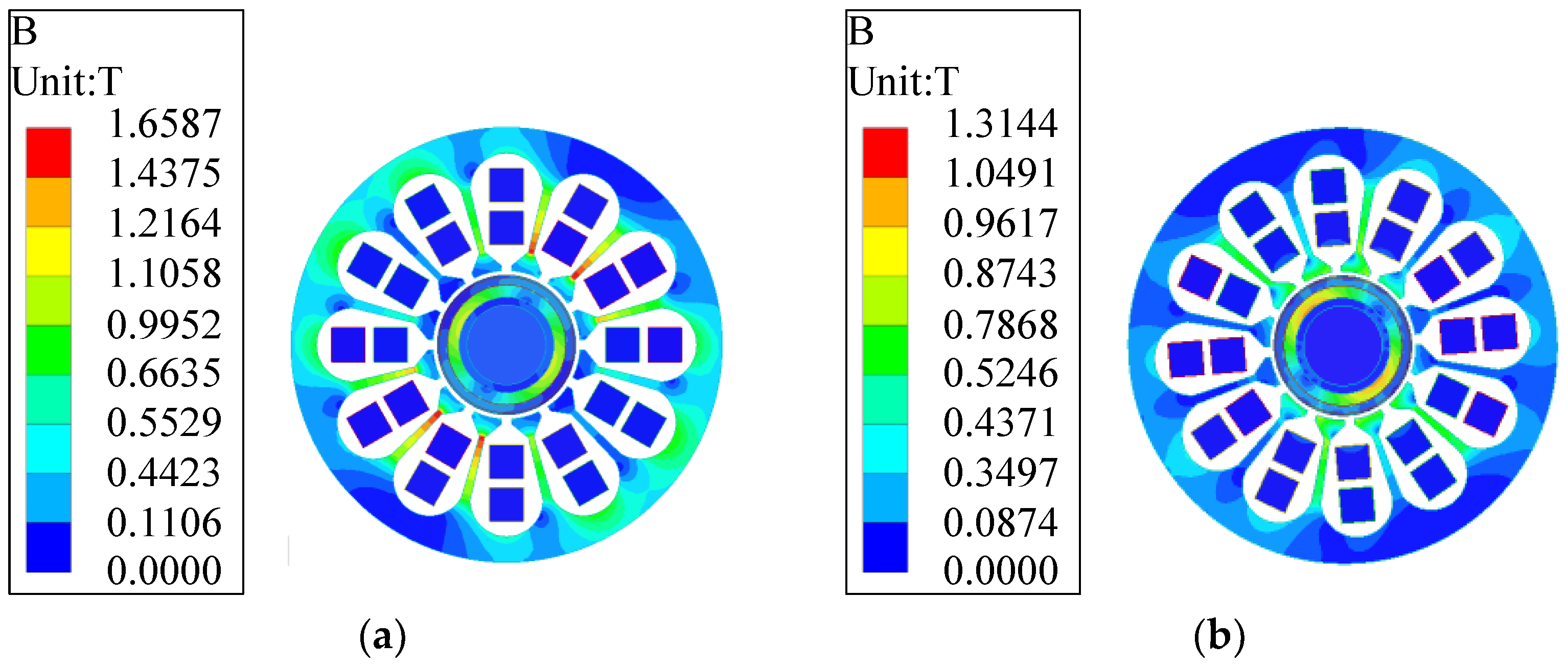
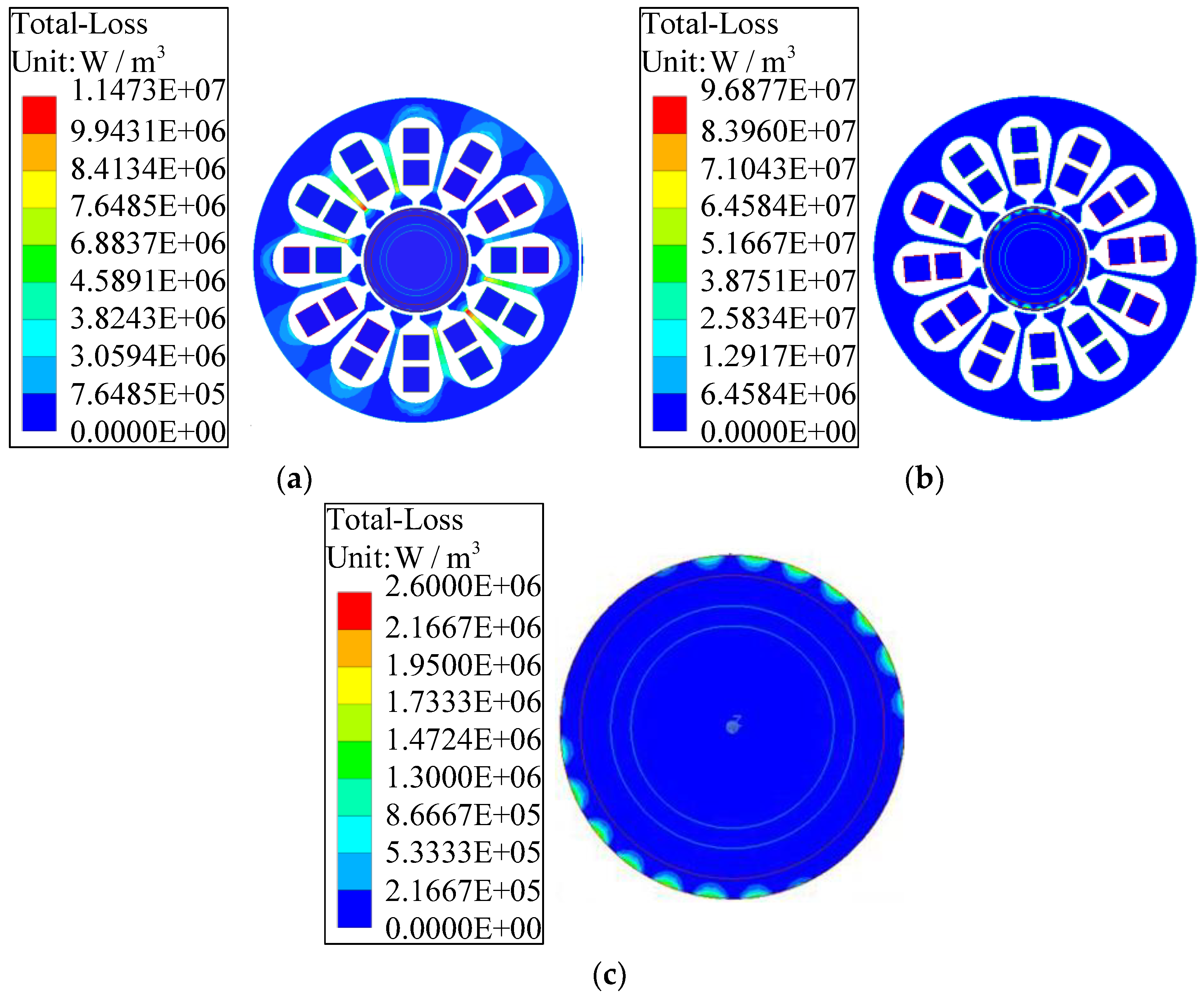
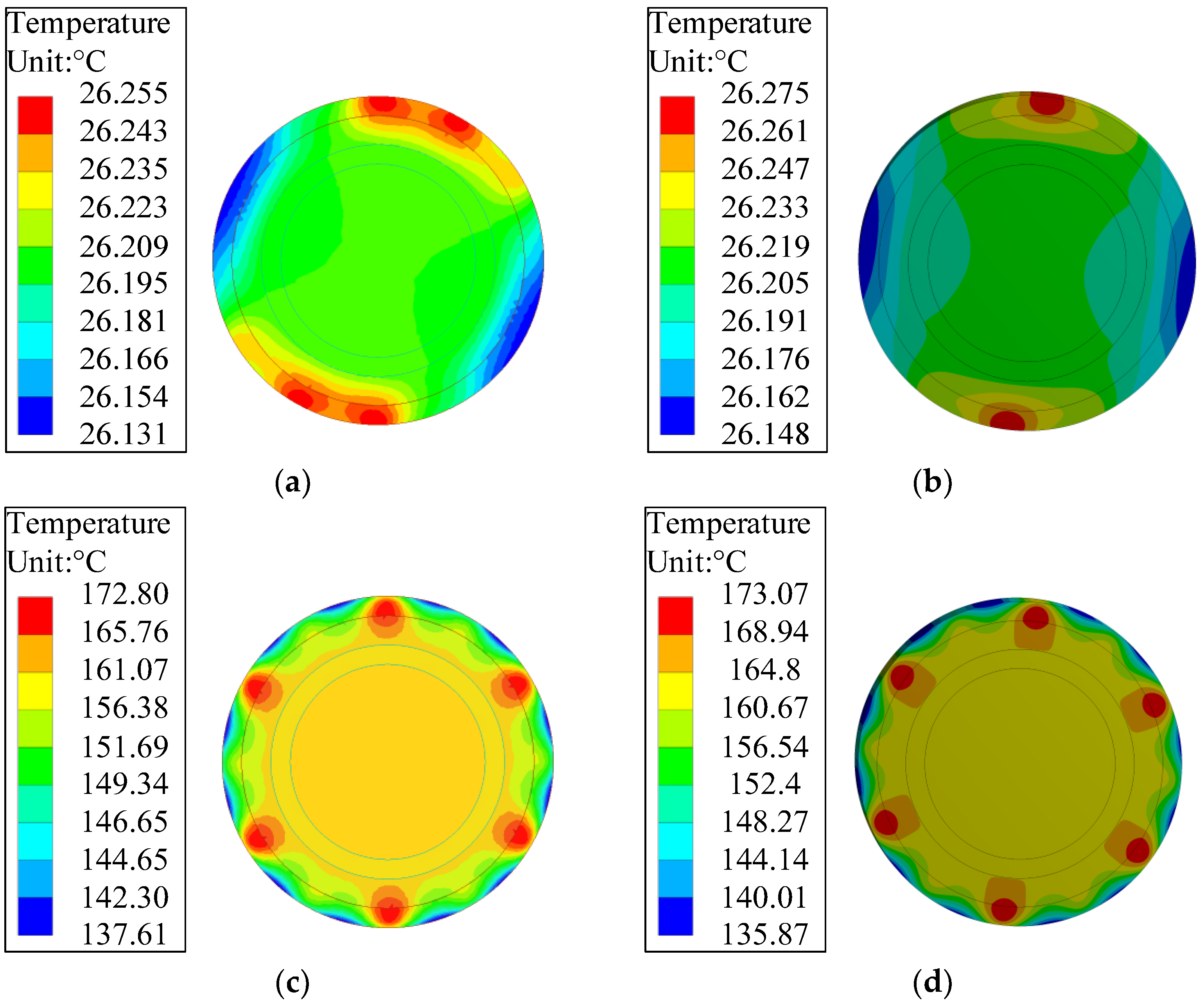
4.1. Loss Analysis of Magneto-Thermal Bidirectional Coupling
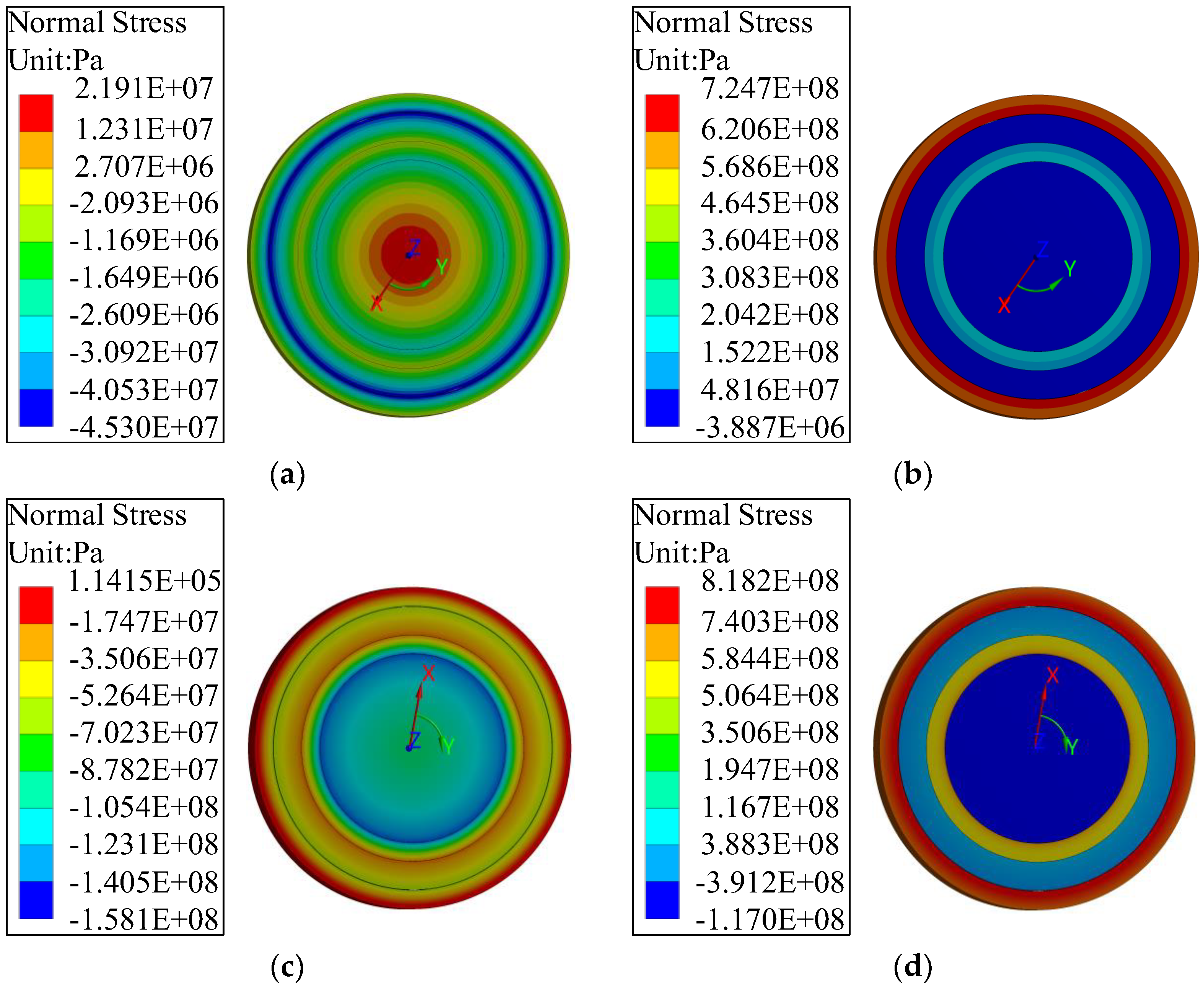
4.2. Rotor Strength under No-Load and Load
5. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhao, W.; Yang, Z.; Liu, Y.; Wang, X. Analysis of a Novel Surface-Mounted Permanent Magnet Motor with Hybrid Magnets for Low Cost and Low Torque Pulsation. IEEE Trans. Magn. 2021, 57, 1–4. [Google Scholar] [CrossRef]
- Gerada, D.; Mebarki, A.; Brown, N.L.; Gerada, C.; Cavagnino, A.; Boglietti, A. High-Speed Electrical Machines: Technologies, Trends, and Developments. IEEE Trans. Ind. Electron. 2013, 61, 2946–2959. [Google Scholar] [CrossRef]
- Cheng, W.; Li, W.; Xiao, L.; Li, M.; Tian, Y.; Sun, Y.; Yu, L. Transient line starting analysis of the ultra-high speed PMSM. AIP Adv. 2017, 7, 903–910. [Google Scholar] [CrossRef] [PubMed]
- Zhang, F.; Du, G.; Wang, T.; Liu, G.; Cao, W. Rotor Retaining Sleeve Design for a 1.12-MW High-Speed PM Machine. IEEE Trans. Ind. Appl. 2015, 51, 3675–3685. [Google Scholar] [CrossRef]
- Zhao, L.; Ham, C.; Zheng, L.; Wu, T.; Sundaram, K.; Kapat, J.; Chow, L. A highly efficient 200000 RPM permanent magnet motor system. IEEE Trans. Magn. 2007, 43, 2528–2530. [Google Scholar] [CrossRef]
- Rahman, M.A.; Chiba, A.; Fukao, T. Super high speed electrical machines-Summary. In Proceedings of the IEEE Power Engineering Society General Meeting, Denver, CO, USA, 6–10 June 2004; pp. 1272–1275. [Google Scholar]
- Liu, Z.H.; Nie, J.; Wei, H.L.; Chen, L.; Li, X.H.; Lv, M.Y. Switched PI Control Based MRAS for Sensorless Control of PMSM Drives UsingFuzzy-Logic-Controller. IEEE Open J. Power Electron. 2022, 3, 368–381. [Google Scholar] [CrossRef]
- Nair, S.V.; Hatua, K.; Prasad, N.D.; Reddy, D.K. A Quick I-f Starting of PMSM Drive with Pole Slipping Prevention and ReducedSpeed Oscillations. IEEE Trans. Ind. Electron. 2021, 68, 6650–6660. [Google Scholar] [CrossRef]
- Rabbi, S.F.; Rahman, M.A. Analysis of Starting and Synchronization Process for Line Start IPM Motors. In Proceedings of the 2012 7th International Conference on Electrical and Computer Engineering, Dhaka, Bangladesh, 20–22 December 2012; IEEE: Piscataway, NJ, USA, 2012; pp. 311–314. [Google Scholar]
- Xu, F.; Chen, A.; Yang, S.; Cao, J.; Liu, X.; Li, L. AC Loss Prediction in BSCCO-Tape Armature Winding Design of a Synchronous Motor. IEEE Trans. Appl. Supercond. 2010, 20, 1005–1008. [Google Scholar]
- Yamazaki, K.; Kokubu, S. Induction Motor Analysis by Considering Hysteresis Loops in Stator and Rotor. IEEE Trans. Magn. 2021, 57, 1–4. [Google Scholar] [CrossRef]
- Behniafar, A.; Darabi, A. Analytical modeling of disc-type permanent magnet hysteresis motor in steady-state operational conditions. Int. J. Comput. Math. Electr. Electron. Eng. 2017, 36, 991–1007. [Google Scholar] [CrossRef]
- Qian, J.H.; Rahman, M.A. Analysis of field oriented control for permanent magnet hysteresis synchronous motors. IEEE Trans. Ind. Appl. 2002, 29, 1156–1163. [Google Scholar] [CrossRef]
- Rehman, S.U.; Huang, Q.; Sagar, R.U.R.; Jiang, Q.; Yang, M.; Zhong, Z. Interaction mechanism, magnetic properties and microstructure of Ce-Fe-B/Alnico spark plasma sintered magnets. J. Magn. Magn. Mater. 2021, 537, 168237. [Google Scholar] [CrossRef]
- Gallicchio, G.; Palmieri, M.; Di Nardo, M.; Cupertino, F. Fast Torque Computation of Hysteresis Motors and Clutches Using Magneto-static Finite Element Simulation. Energies 2019, 12, 3311–3327. [Google Scholar] [CrossRef]
- Jagiela, M.; Garbiec, T.; Kowol, M. Design of High-Speed Hybrid Hysteresis Motor Rotor Using Finite Element Model and Decision Process. IEEE Trans. Magn. 2014, 50, 861–864. [Google Scholar] [CrossRef]
- Pyrhonen, J.; Ruoho, S.; Nerg, J. Hysteresis losses in Sintered NdFeB permanent magnets in rotating electrical machines. IEEE Trans. Ind. Electron. 2015, 62, 857–865. [Google Scholar] [CrossRef]
- Mohapatra, J.; Xing, M.; Elkins, J.; Liu, J.P. Hard and semi-hard magnetic materials based on cobalt and cobalt alloys. J. Alloys Compd. 2020, 824, 153874. [Google Scholar] [CrossRef]
- Duan, N.; Xu, W.; Feng, H.; Wang, S.; Guo, Z.; Li, Y.; Wang, S.; Zhu, J. A Scalar Hysteresis Model of Ferromagnetic Materials Based on the Elemental Operators. IEEE Trans. Magn. 2018, 54, 1–4. [Google Scholar] [CrossRef]
- Ma, H.J.; Zhang, J.T.; Li, G.H.; Zhang, W.X.; Wang, W.M. Effect of Zr on the thermal stability and magnetic properties of Fe78Si9B13, glassyalloy. J. Alloys Compd. 2010, 501, 227–232. [Google Scholar] [CrossRef]
- Wang, J.; Wang, F.; Bao, W.; Guan, E. Rotor Design and Strength Analysis of High Speed Permanent magnet Motor. Proc. CSEE 2005, 25, 140–145. (In Chinese) [Google Scholar]
- Zhang, F.; Du, G.; Wang, T.; Huang, N. Strength Analysis of Rotor Protection Measures for High Speed Permanent magnet Motor. Proc. CSEE 2013, 33, 195–202. (In Chinese) [Google Scholar]
- Cheng, W.; Deng, Z.; Xiao, L.; Zhong, B.; Zhang, B. Strength analytical solution to ultra-high-speed permanent magnet rotor considering temperature gradient and segmental permanent magnet effect. Adv. Mech. Eng. 2019, 11, 1–4. [Google Scholar] [CrossRef]
- Chen, L.; Zhu, C.; Qiao, X. Strength Analysis of High Speed Split SPMSM Rotor fixed with Carbon Fiber. Electr. Mach. Control 2019, 23, 93–103. (In Chinese) [Google Scholar]
- Chen, L.; Zhu, C.; Jiang, K. Rotor Strength Analysis of High Speed Surface Mount Permanent Magnet Synchronous Motor with interelectrode Filling Block. J. Zhejiang Univ. Eng. Ed. 2015, 9, 1738–1748. (In Chinese) [Google Scholar]
- Mo, L.; Zhu, X.; Zhang, T.; Quan, L.; Wang, Y.; Huang, J. Temperature Rise Calculation of a Flux-Switching Permanent-Magnet Double-Rotor Machine Using Electromagnetic-Thermal Coupling Analysis. IEEE Trans. Magn. 2017, 54, 1–4. [Google Scholar] [CrossRef]
- Huang, Z.; Fang, J.; Liu, X.; Han, B. Loss Calculation and Thermal Analysis of Rotors Supported by Active Magnetic Bearings for High-Speed Permanent-Magnet Electrical Machines. IEEE Trans. Ind. Electron. 2015, 63, 1. [Google Scholar] [CrossRef]
- Hanappier, N.; Charkaluk, E.; Triantafyllidis, N. A coupled electromagnetic–thermomechanical approach for the modeling of a electric motors. J. Mech. Phys. Solids 2021, 149, 2567–2569. [Google Scholar] [CrossRef]
- Preis, K.; Biro, O.; Dyczijedlinger, R. Application of FEM to coupled electric, thermal and mechanical problems. IEEE Trans. Magn. 1994, 30, 3316–3319. [Google Scholar] [CrossRef]
- Maroufian, S.S.; Pillay, P. Design and Analysis of a Novel PM-Assisted Synchronous Reluctance Machine Topology with AlNiCo Magnets. IEEE Trans. Ind. Appl. 2019, 55, 4733–4742. [Google Scholar] [CrossRef]
- Rehman, S.U.; Wei, C.; Huang, Q.; Jiang, Q.; Haq, A.U.; Wang, J.; Zhong, Z. Tailoring the microstructure, magnetic properties and interaction mechanisms of Alnico-Ta alloys by magnetic field treatment. J. Alloys Compd. 2021, 857, 157586. [Google Scholar] [CrossRef]
- Zhang, S.-D.; Wang, S.; Chen, S.-Y.; Yu, X.; Sun, J.-B. Structure and magnetic properties of alnico 8 ribbons. Phys. B Condens. Matter 2020, 597, 412423. [Google Scholar] [CrossRef]
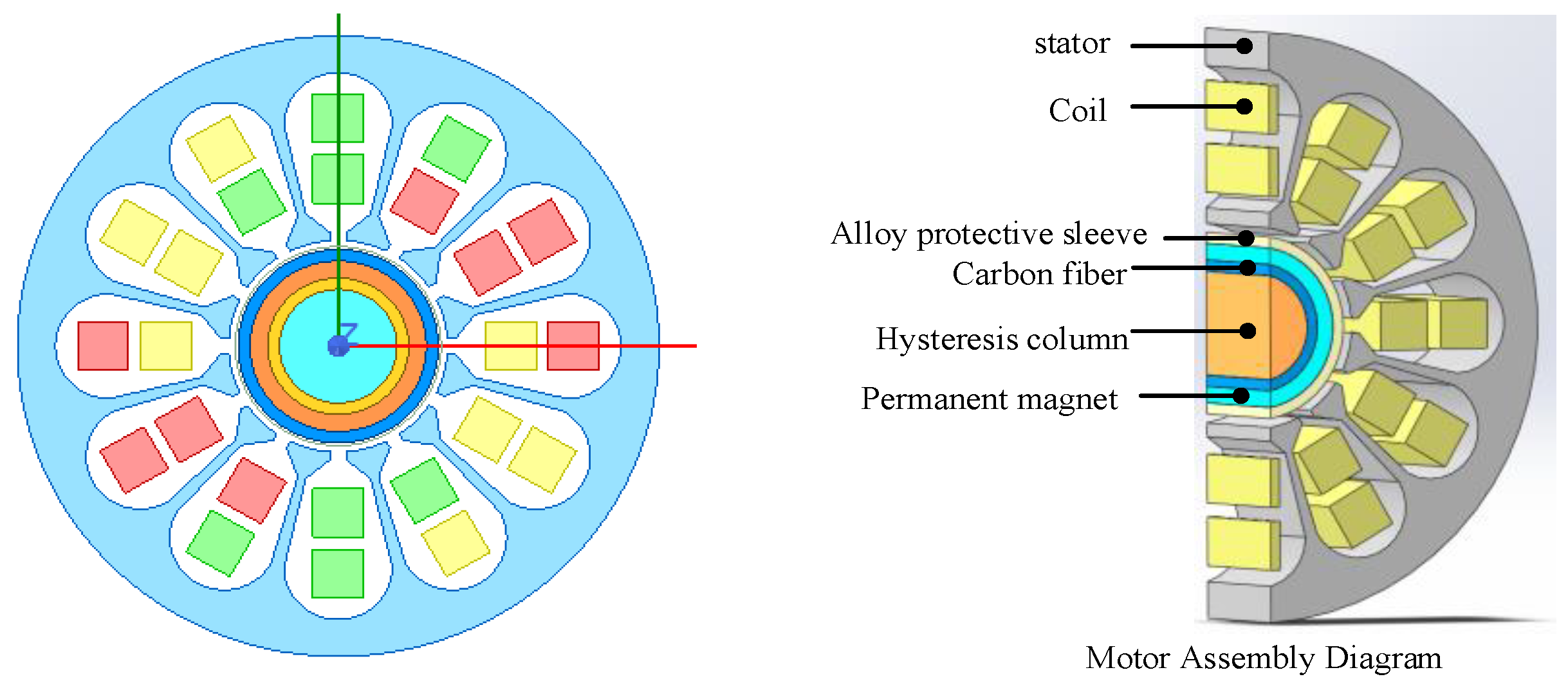
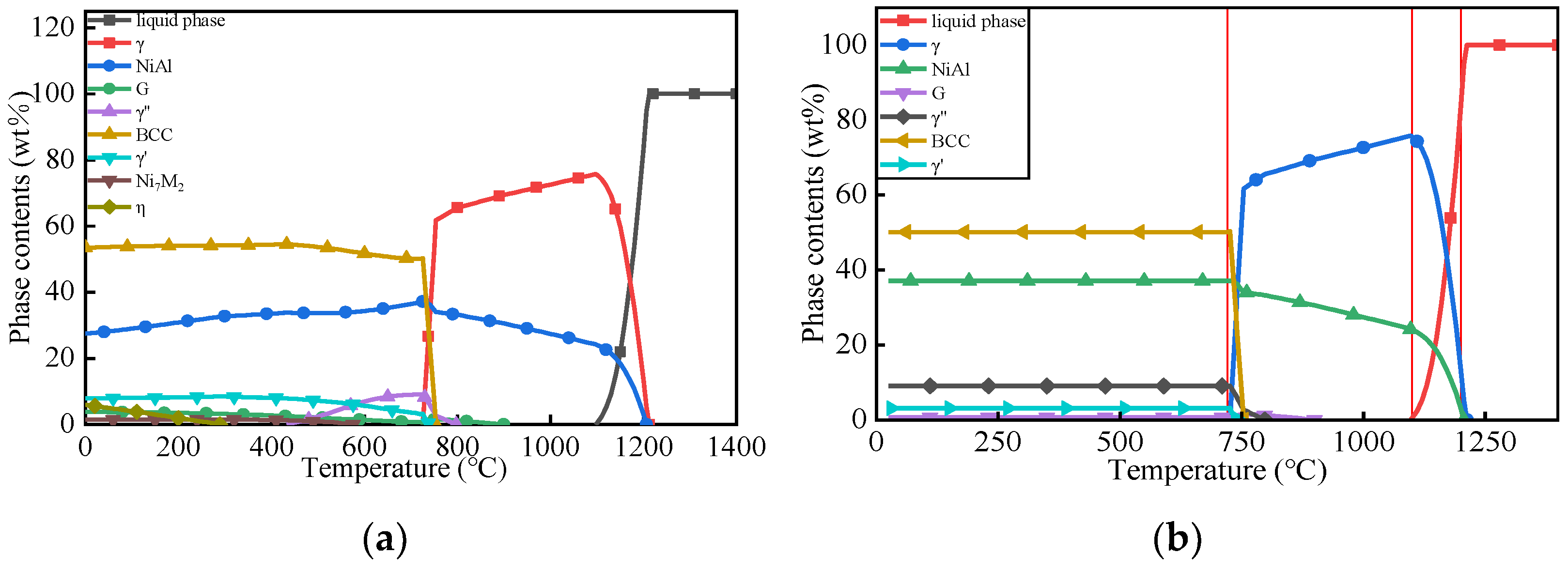

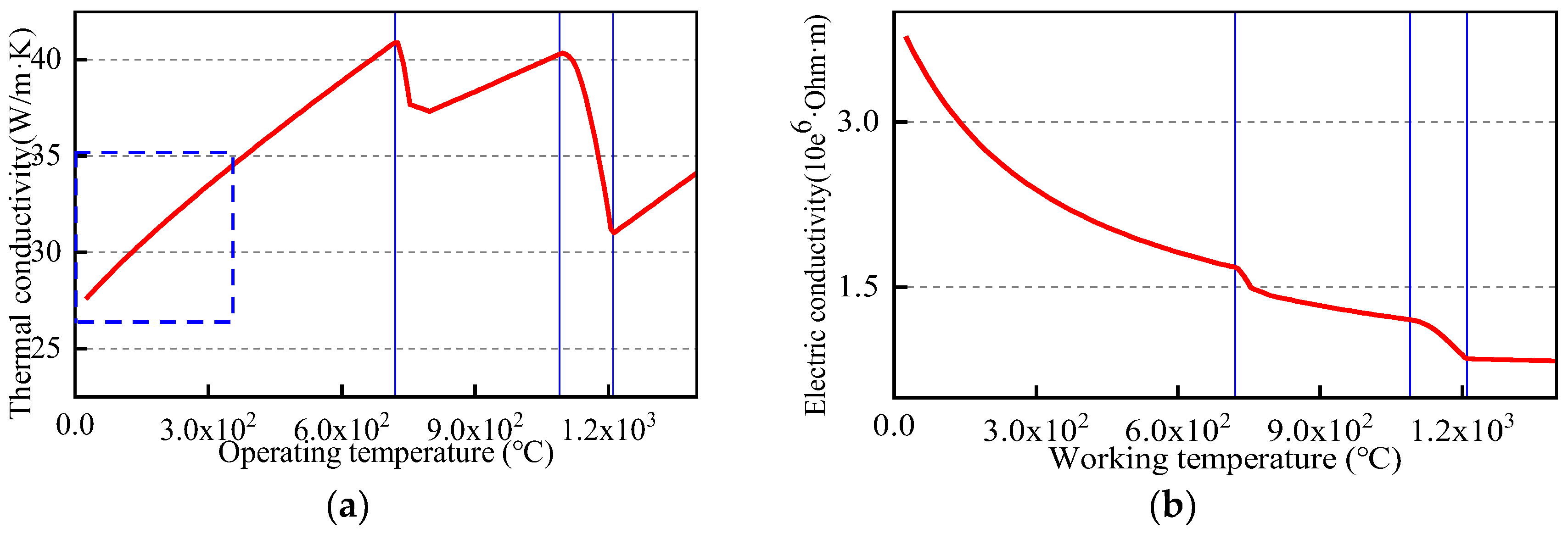
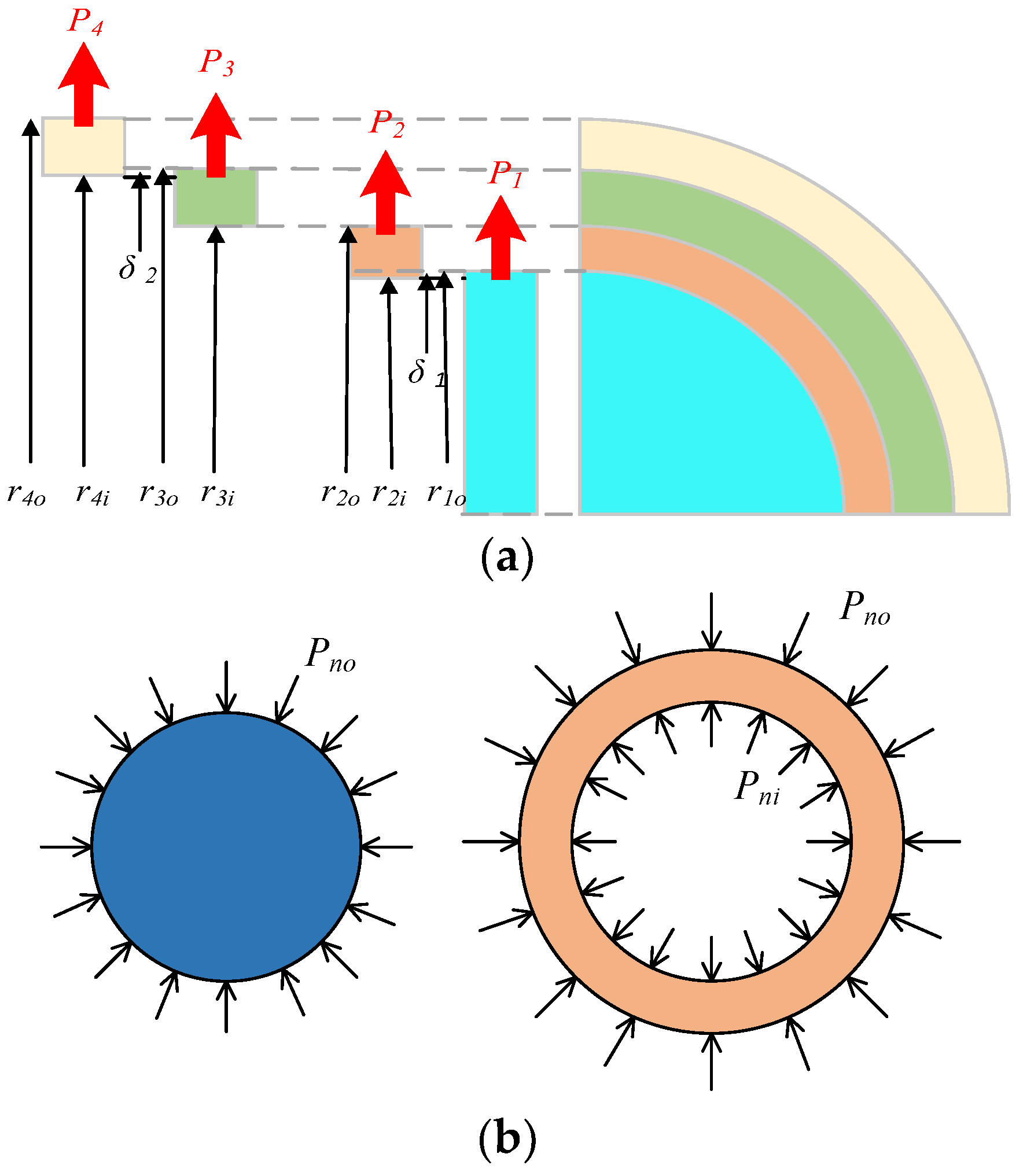


| Component | Al | Ni | Co | Cu | Ti | Si | Nb | Zr | Fe |
|---|---|---|---|---|---|---|---|---|---|
| AlNiCo8/wt% | 7 | 14 | 35 | 4 | 5 | 0 | 0 | 0 | 35 |
| Improved AlNiCo /wt% | 7.5 | 17.45 | 25.5 | 3.8 | 4.5 | 0.5 | 0.8 | 0.45 | 39.5 |
| contact pressure (unit: N) | |
| r | radius (unit: mm) |
| n | the subscript numbers |
| E | modulus of elasticity (unit: Pa) |
| radial elastic modulus (unit: Pa) | |
| tangential elastic modulus (unit: Pa) | |
| ΔT | temperature rise of each part (unit: °C) |
| inside diameter (unit: mm) | |
| outside diameter (unit: mm) | |
| undetermined coefficients | |
| displacement (unit: mm) |
| α | thermal expansion coefficient (unit: ) |
| radial thermal expansion coefficient(unit: ) | |
| tangential thermal expansion coefficient (unit: ) | |
| strain | |
| radial strain | |
| tangential strain | |
| stress (unit: Pa) | |
| ω | angular velocity (unit: rad/s) |
| tangential stress (unit: Pa) | |
| radial stress (unit: Pa) | |
| Poisson’s ratio | |
| radial Poisson’s ratio | |
| tangential Poisson’s ratio | |
| material density (kg/m3) | |
| suitable quantity (unit: mm) |
| Hysteresis Column | Carbon Fiber | Permanent Magnet | Protective Sleeve | |
|---|---|---|---|---|
| 1.6 × 1011 | 2.7 × 1010 | 1.08 × 1011 | 2.06 × 1011 | |
| 1.6 × 1011 | 4.56 × 1011 | 1.08 × 1011 | 2.06 × 1011 | |
| 7500 | 1750 | 8300 | 8190 | |
| 11 × 10−6 | 22.5 × 10−6 | 9 × 10−6 | 11.8 × 10−6 | |
| 11 × 10−6 | 0.02 × 10−6 | 9 × 10−6 | 11.8 × 10−6 | |
| 0.24 | 0.15 | 0.24 | 0.28 | |
| 0.24 | 0.018 | 0.24 | 0.28 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiao, L.; Zheng, S.; Dou, J.; Cheng, W. Analysis of the Magneto-Thermal Bidirectional Coupling Strength of Macro and Micro Integration for Self-Starting Permanent Magnet Hysteresis Synchronous Motors. Machines 2023, 11, 931. https://doi.org/10.3390/machines11100931
Xiao L, Zheng S, Dou J, Cheng W. Analysis of the Magneto-Thermal Bidirectional Coupling Strength of Macro and Micro Integration for Self-Starting Permanent Magnet Hysteresis Synchronous Motors. Machines. 2023; 11(10):931. https://doi.org/10.3390/machines11100931
Chicago/Turabian StyleXiao, Ling, Shandong Zheng, Jingwei Dou, and Wenjie Cheng. 2023. "Analysis of the Magneto-Thermal Bidirectional Coupling Strength of Macro and Micro Integration for Self-Starting Permanent Magnet Hysteresis Synchronous Motors" Machines 11, no. 10: 931. https://doi.org/10.3390/machines11100931
APA StyleXiao, L., Zheng, S., Dou, J., & Cheng, W. (2023). Analysis of the Magneto-Thermal Bidirectional Coupling Strength of Macro and Micro Integration for Self-Starting Permanent Magnet Hysteresis Synchronous Motors. Machines, 11(10), 931. https://doi.org/10.3390/machines11100931





