Effects of the Magnetic Model of Interior Permanent Magnet Machine on MTPA, Flux Weakening and MTPV Evaluation
Abstract
1. Introduction
2. Operating Strategies in the Linear Model
- R: phase resistance;
- : voltage vector;
- : current vector;
- : mechanical speed;
- : pole pairs number;
- : flux linkage vector;
- : flux linkage due to PM;
- : inductance matrix.
- Pure sinusoidal currents and air gap magnetic field distribution;
- No cross-coupling between the equivalent magnetic circuit and the d-q axes. The two axes are magnetically decoupled because they are at 90 electrical degrees;
- No saturation effects, i.e., no variation of the inductances versus currents.
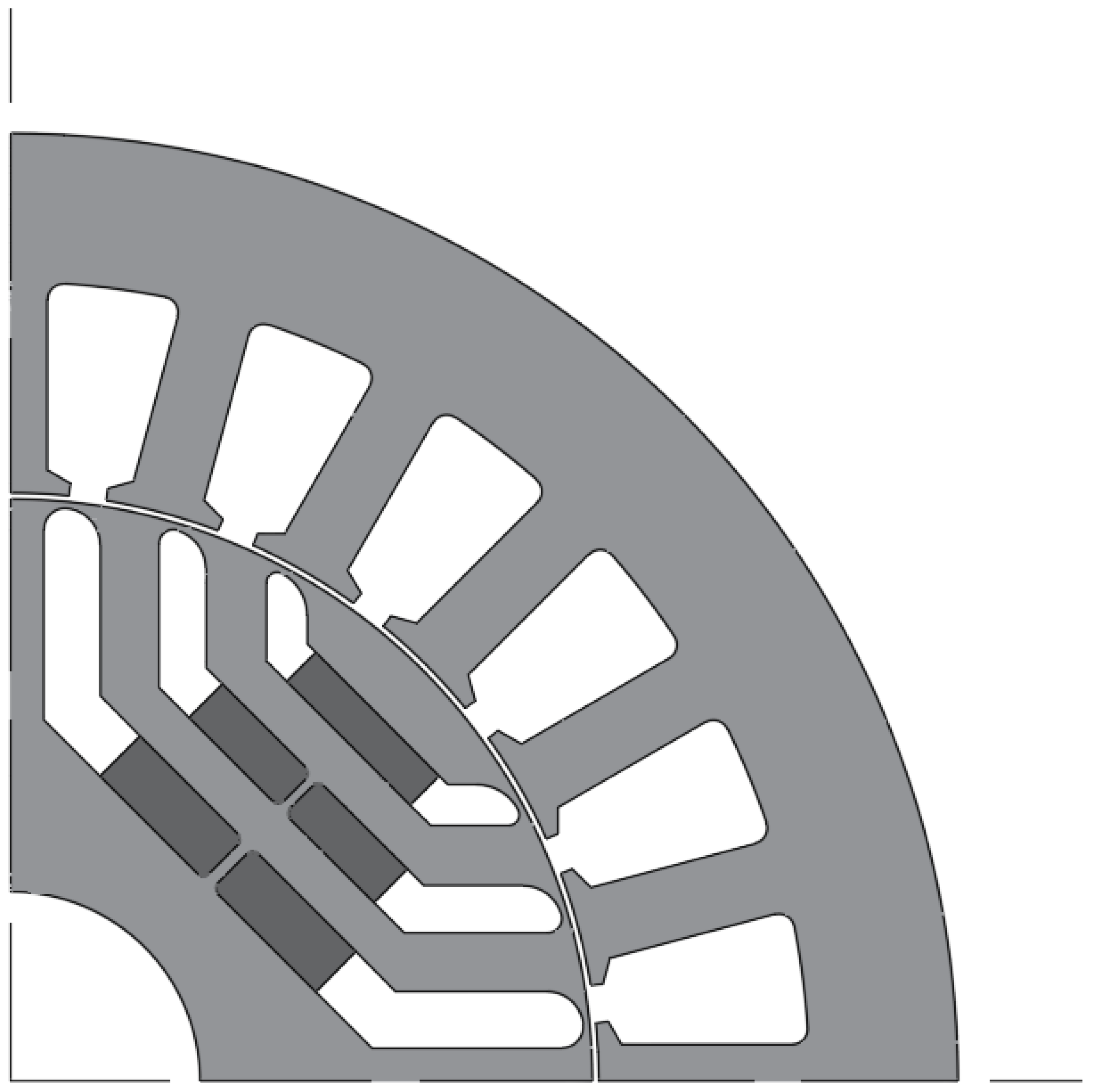
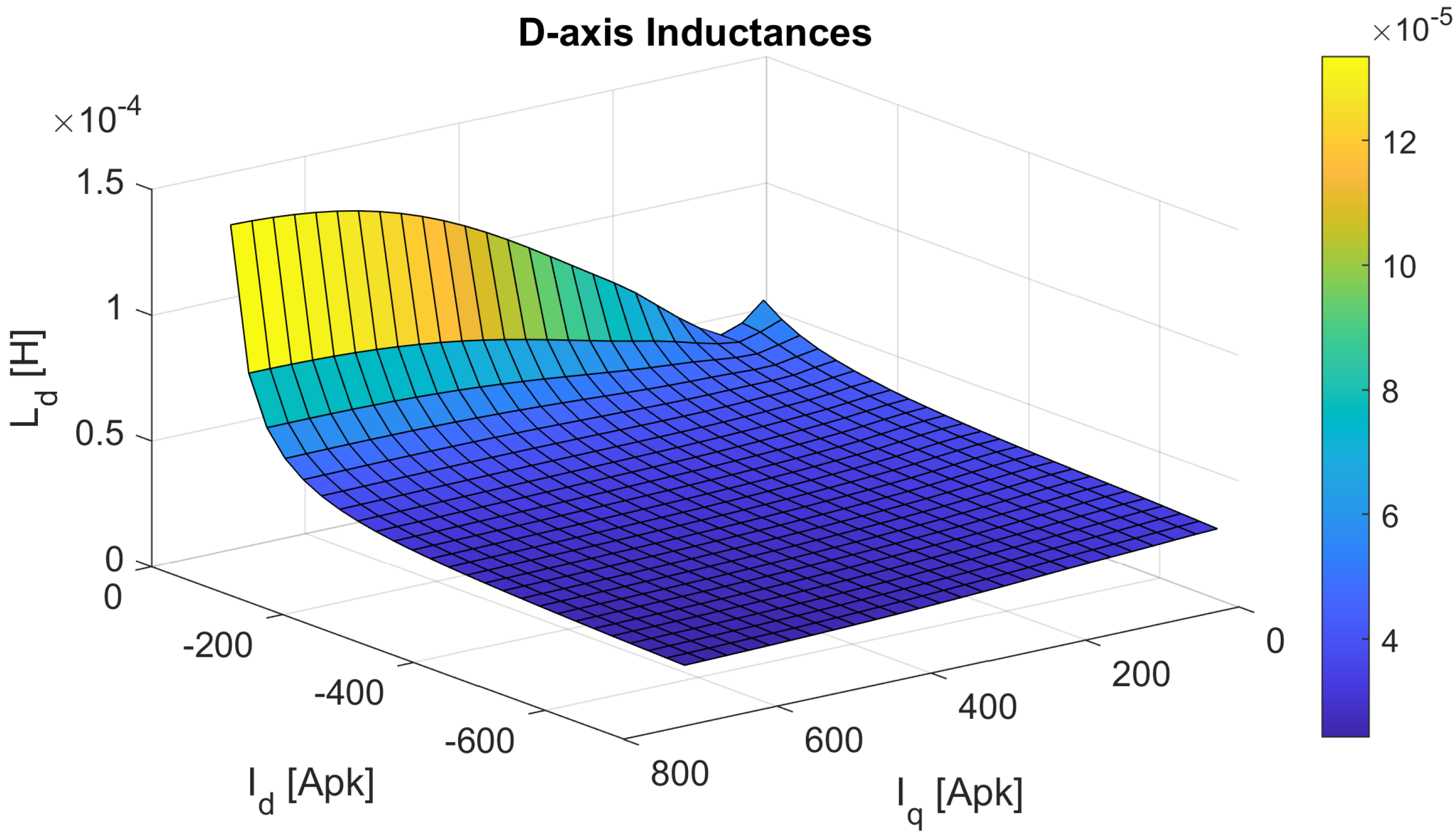
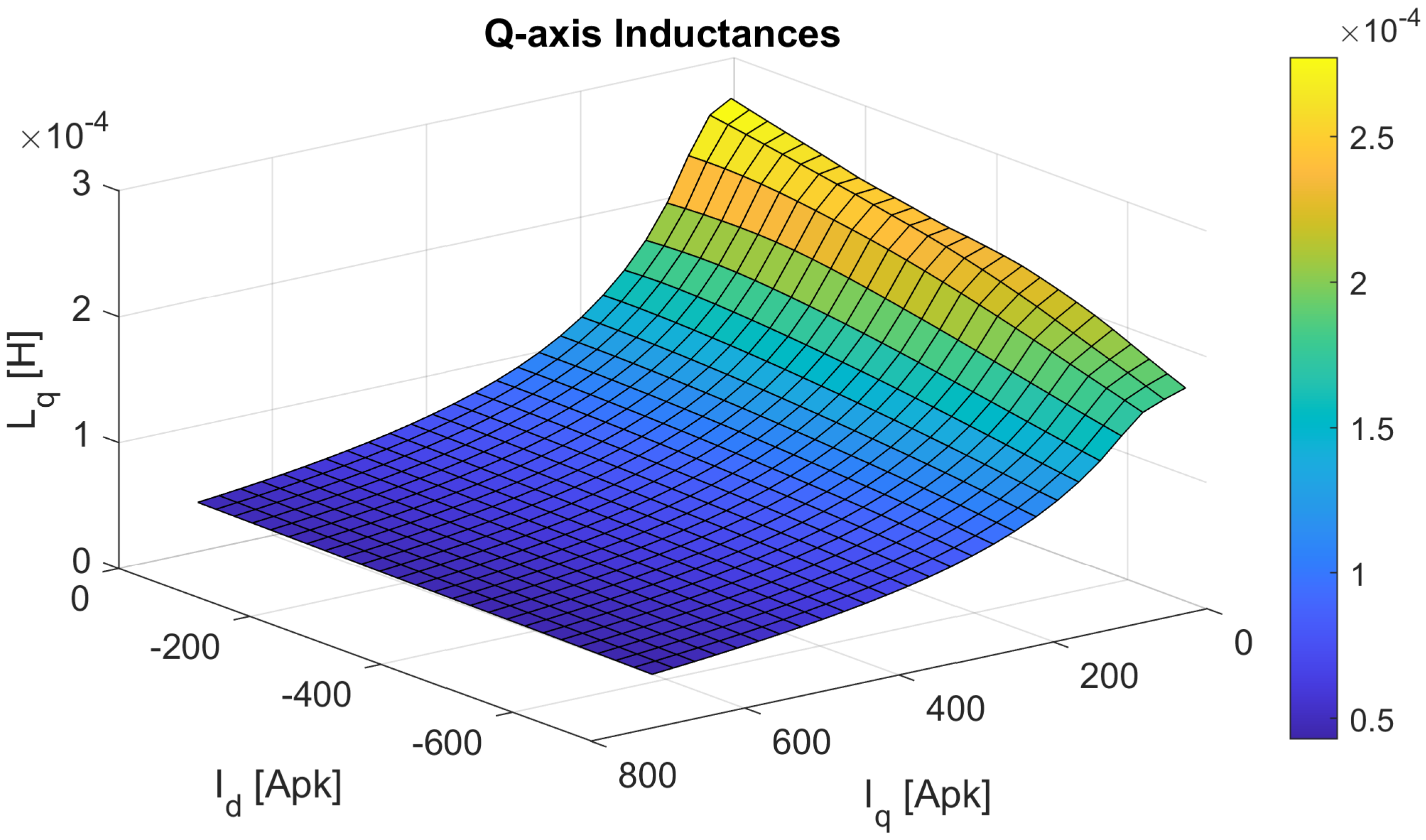
3. Finite Element Analysis for Magnetic Model Mapping
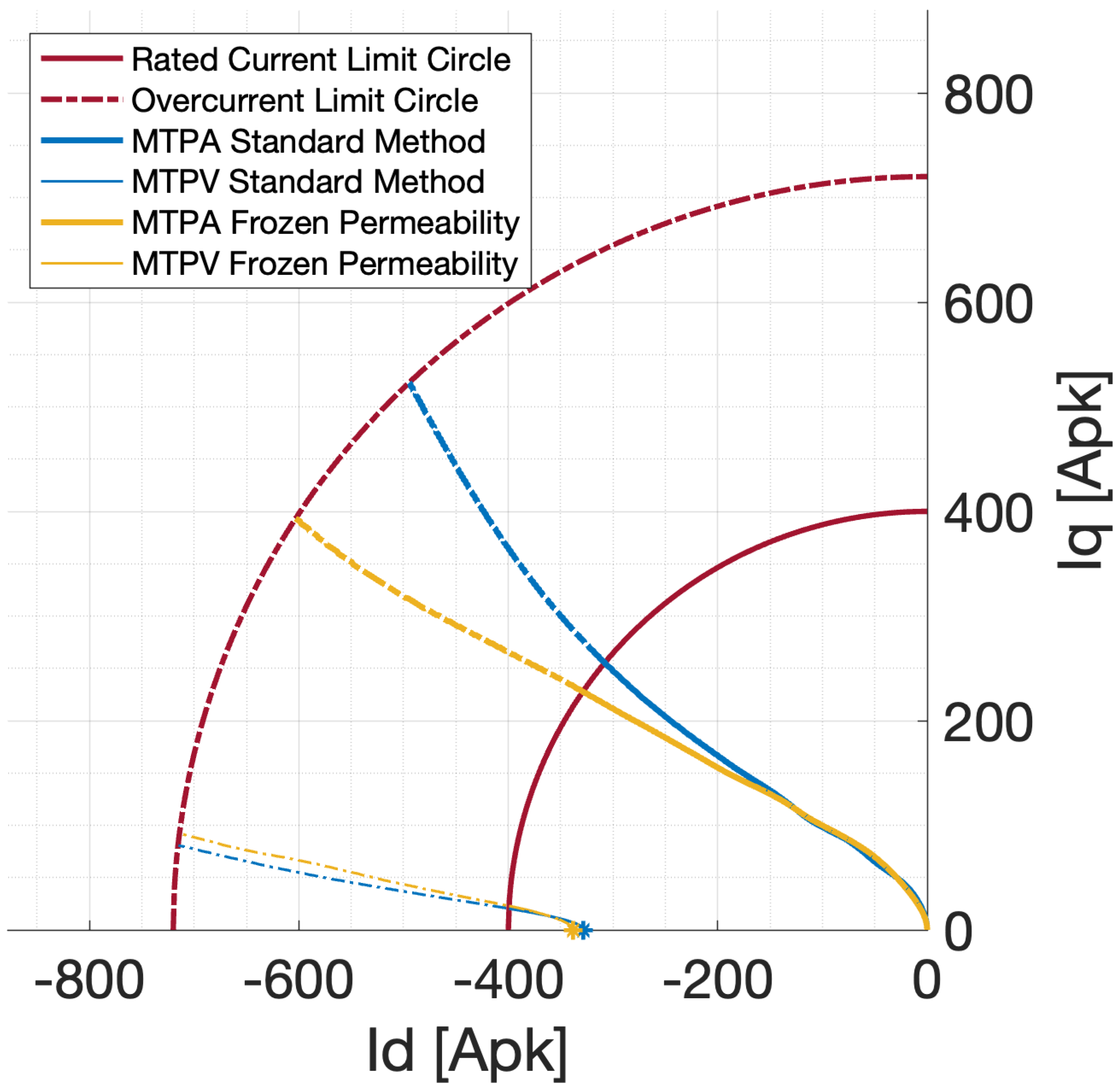
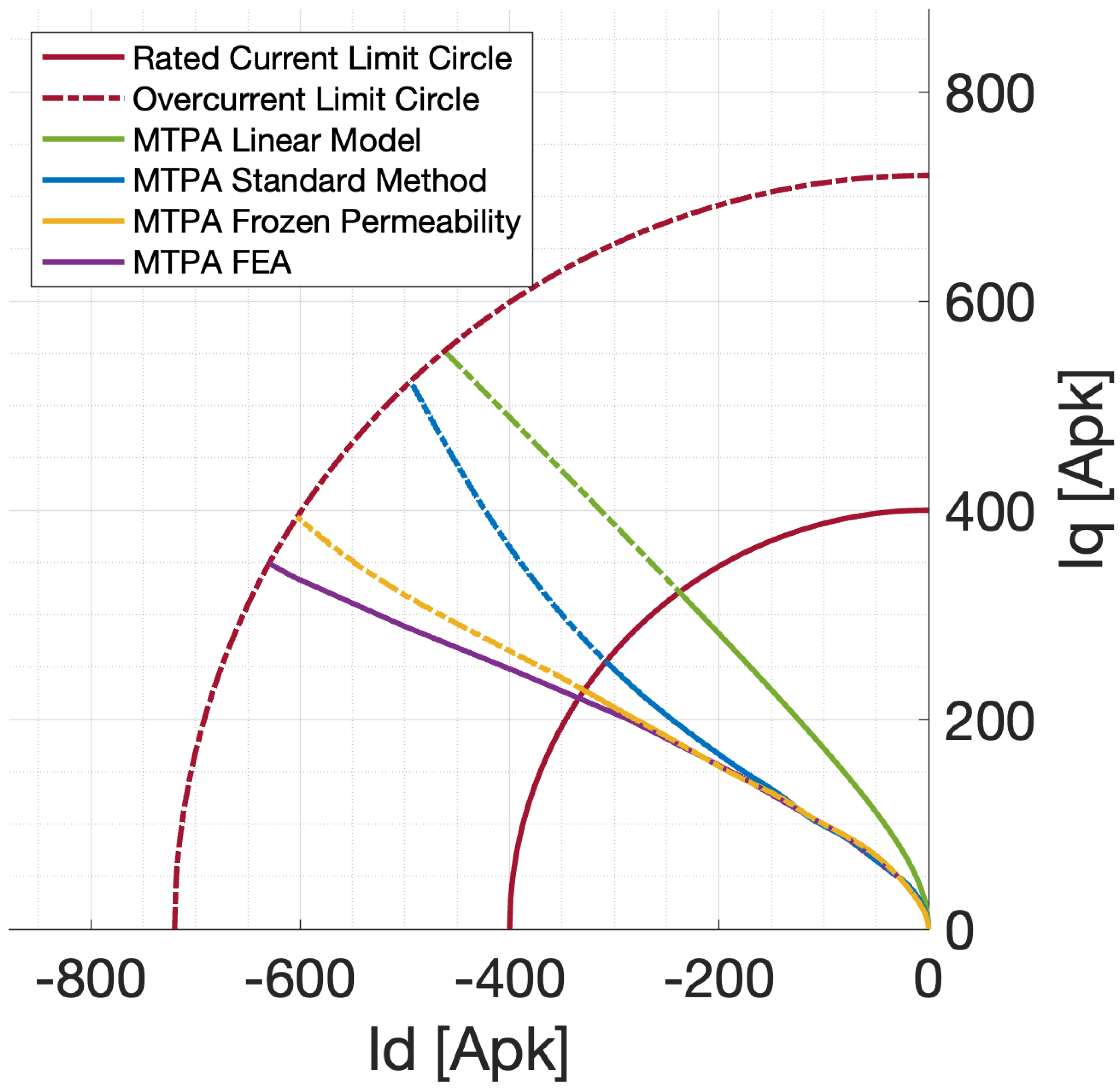
3.1. Standard Method
3.2. Frozen Permeability Method
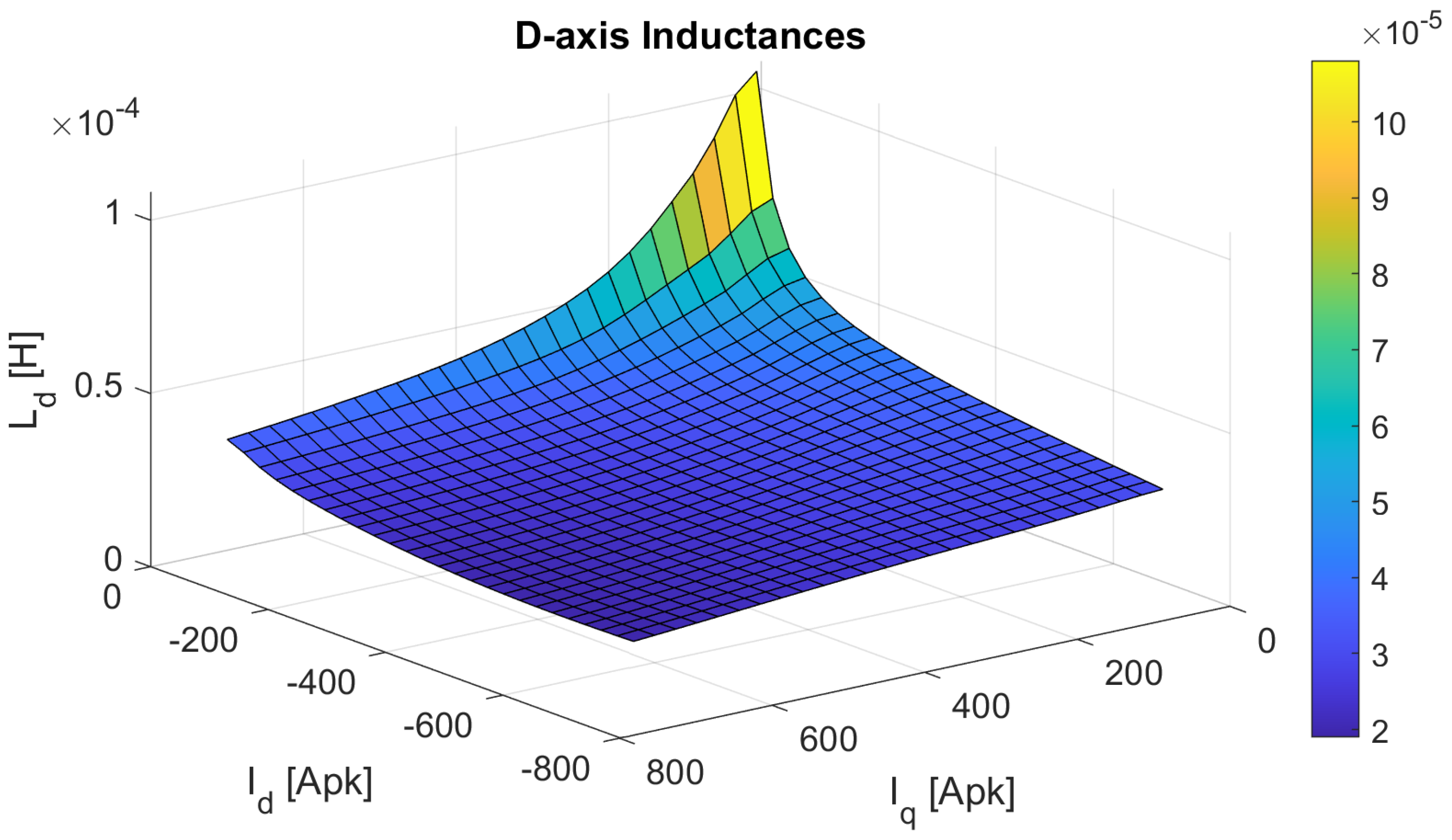
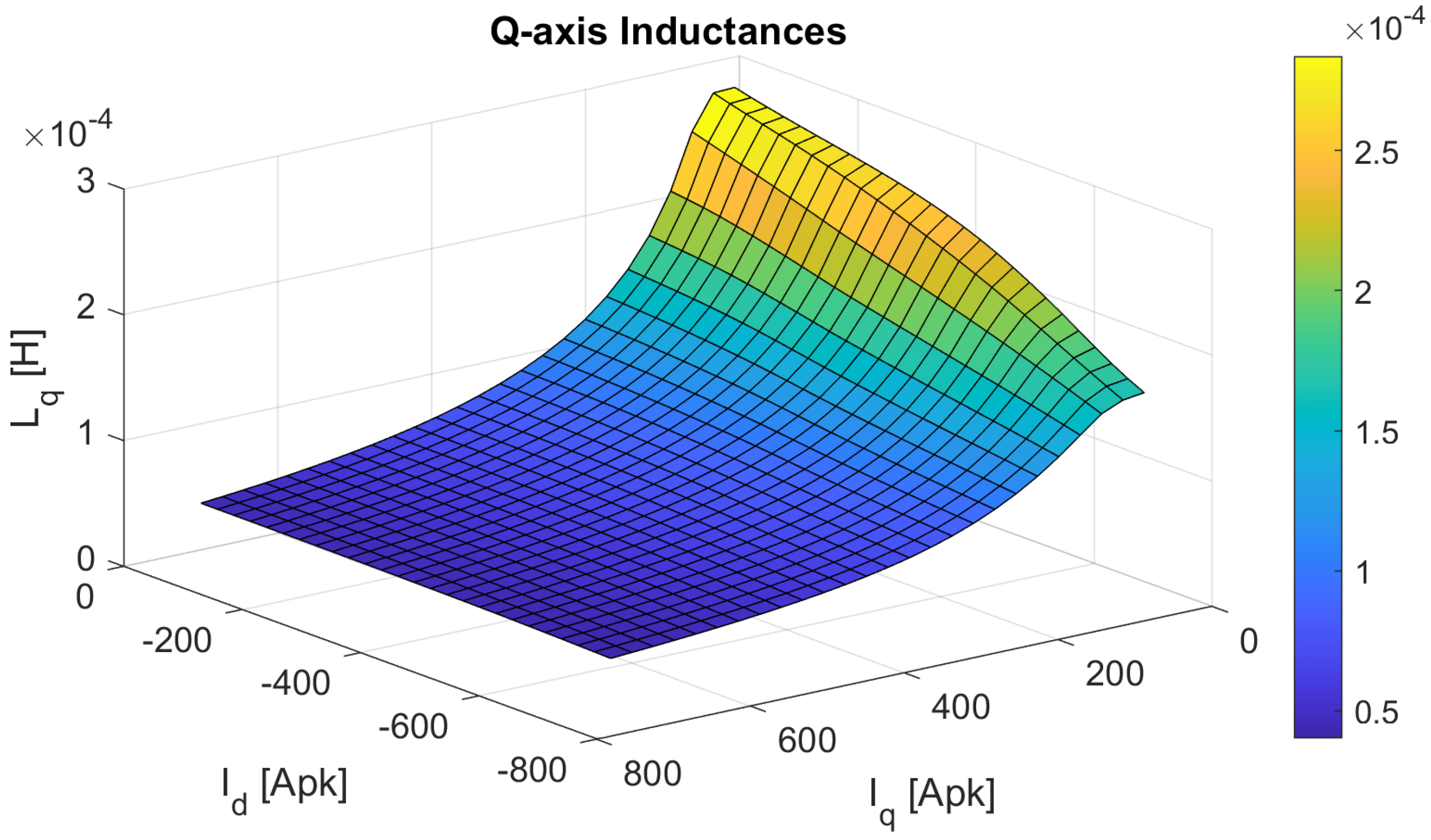
4. Nonlinear d-q Model Computation
- Define a starting value of , considering a known point belonging to the desired control curve;
- Re-compute , with the current values of , and the inductances map;
- Use the new value of , computed in the previous bullet for the next iteration.
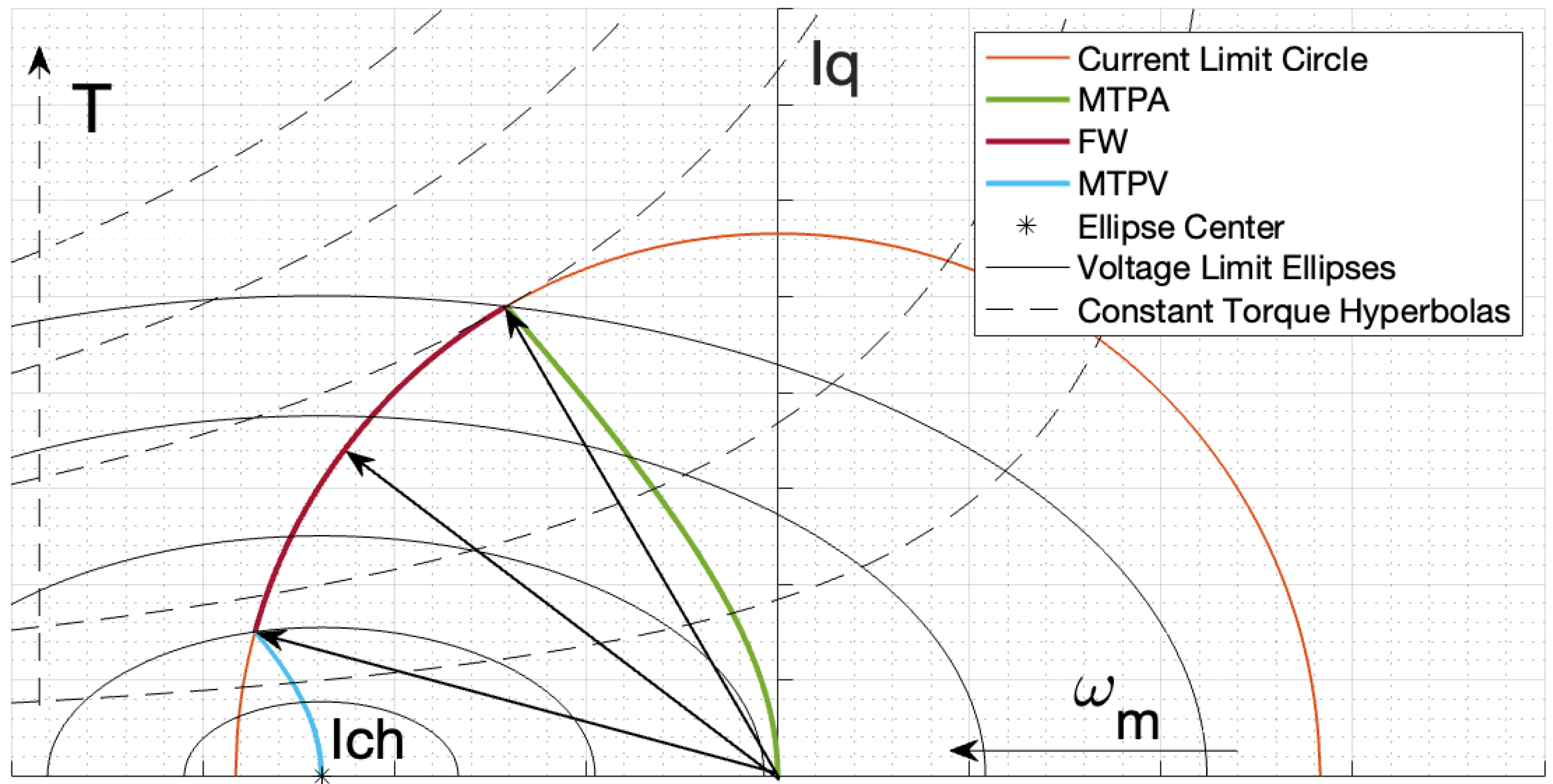
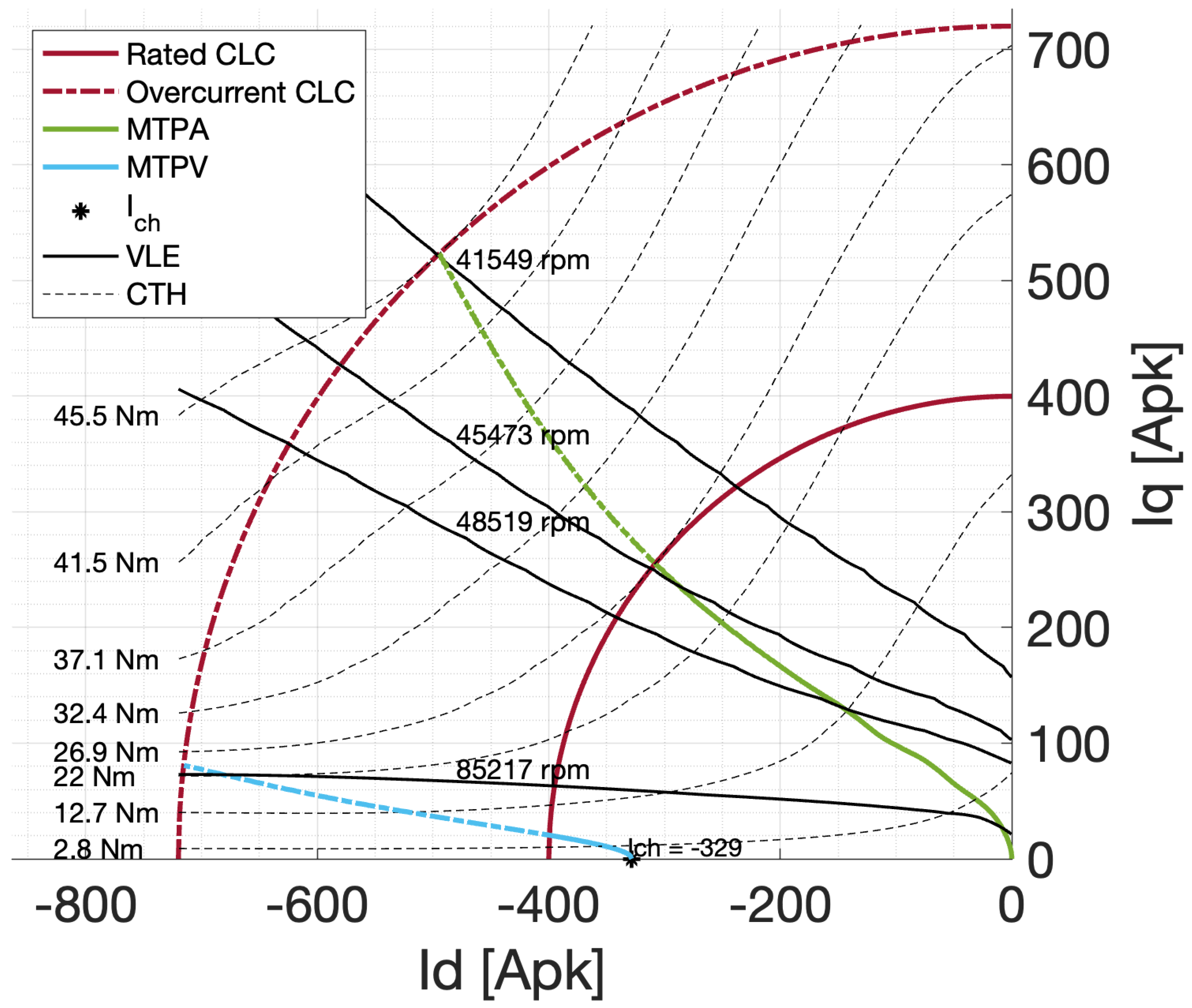
4.1. Maximum Torque Per Ampere (MTPA)
- A space vector of current amplitude is defined ranging from 0 to current limit value;
- For each value of , a vector of current phase is defined ranging from 90° to 180°;
- Each couple of the matrix identifies an operating point in the second quadrant of the d-q plane;
- For each couple, and are interpolated from inductances maps at disposal. Torque is computed by Equation (4) and stored in a matrix;
- For each value of , the maximum torque is computed comparing the matrix elements corresponding to each value of . Also the corresponding values of , , and are computed and stored.
4.2. Flux Weakening (FW)
- The last element of the base speed vector of MTPA is selected as the starting element for the FW speed ();
- The limit speed in FW () is computed according to Equation (15);
- The FW speed vector ranges from to ;
- Starting values of and are computed in the end point of MTPA trajectory: and ;
- For each element of the speed vector, the following parameters are computed and stored:
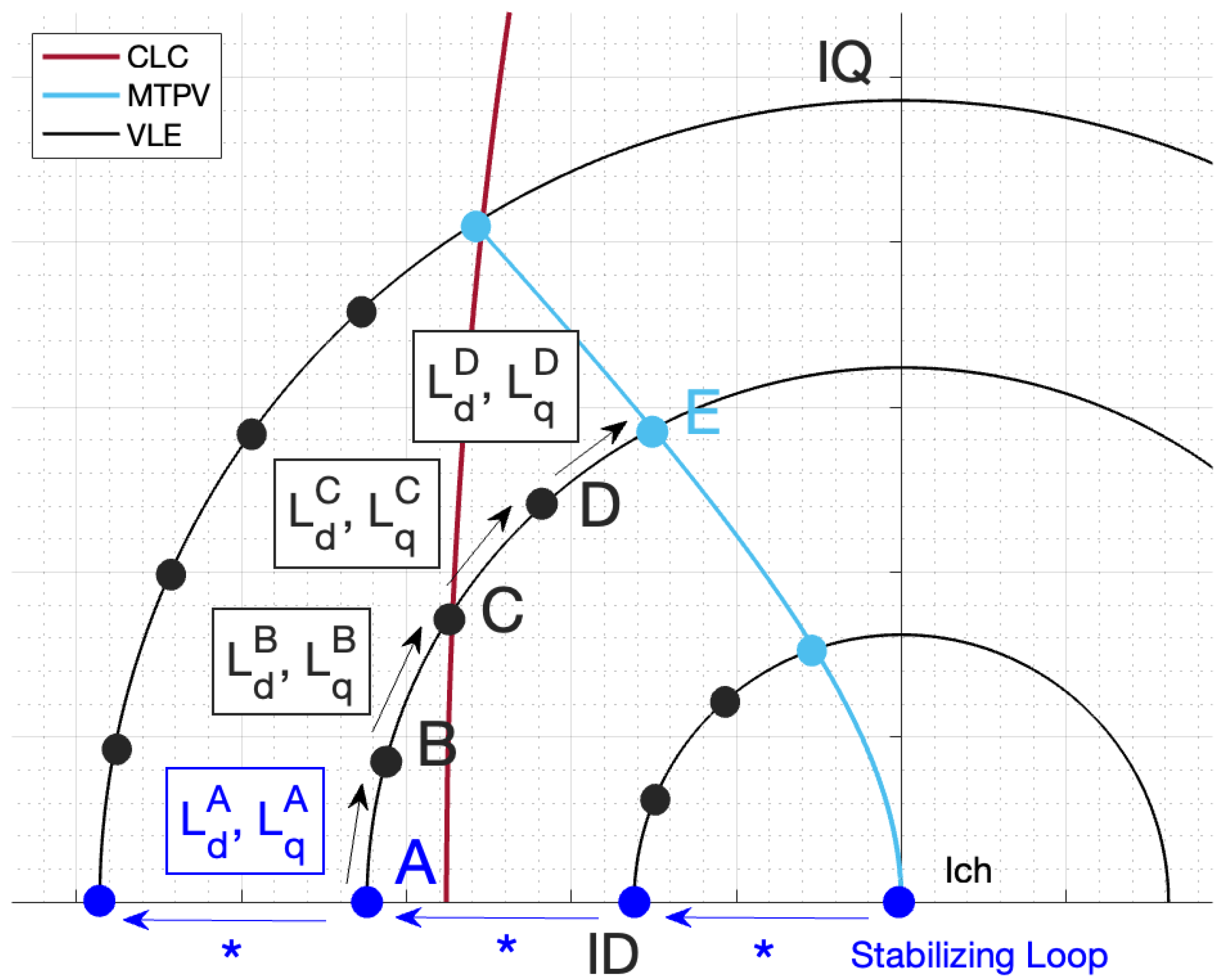
4.3. Maximum Torque Per Volt (MTPV)
- Starting values of and are computed in the starting point with the stabilizing loop described in Section 4;
- For each element of the speed vector with , the and values computed at the previous iteration are taken as starting values and the stabilizing loop is performed. After their values are fixed with high accuracy, the following parameters are computed:
4.4. Voltage Limit Ellipses
- The corresponding on the MTPA trajectory is identified;
- The starting values of and are interpolated from the inductance map;
- The corresponding speed value is computed by Equation (7);
- A vector ranging from the identified to the current limit is defined. For each value of this vector:
- -
- is evaluated with the and of the previous step by Equation (7);
- -
- The new and with the current values of and are interpolated from the maps for the next iteration.
4.5. Constant Torque Hyperbolas
- The corresponding on the MTPA trajectory is identified;
- The starting values of and are interpolated from the inductance map;
- The corresponding torque value is computed by Equation (4);
- A vector ranging from the identified to the current limit is defined. For each value of this vector:
- -
- is evaluated with the and of the previous step by Equation (4);
- -
- The new and with the current values of and are interpolated from the map for the next iteration.
5. Results and Comparisons
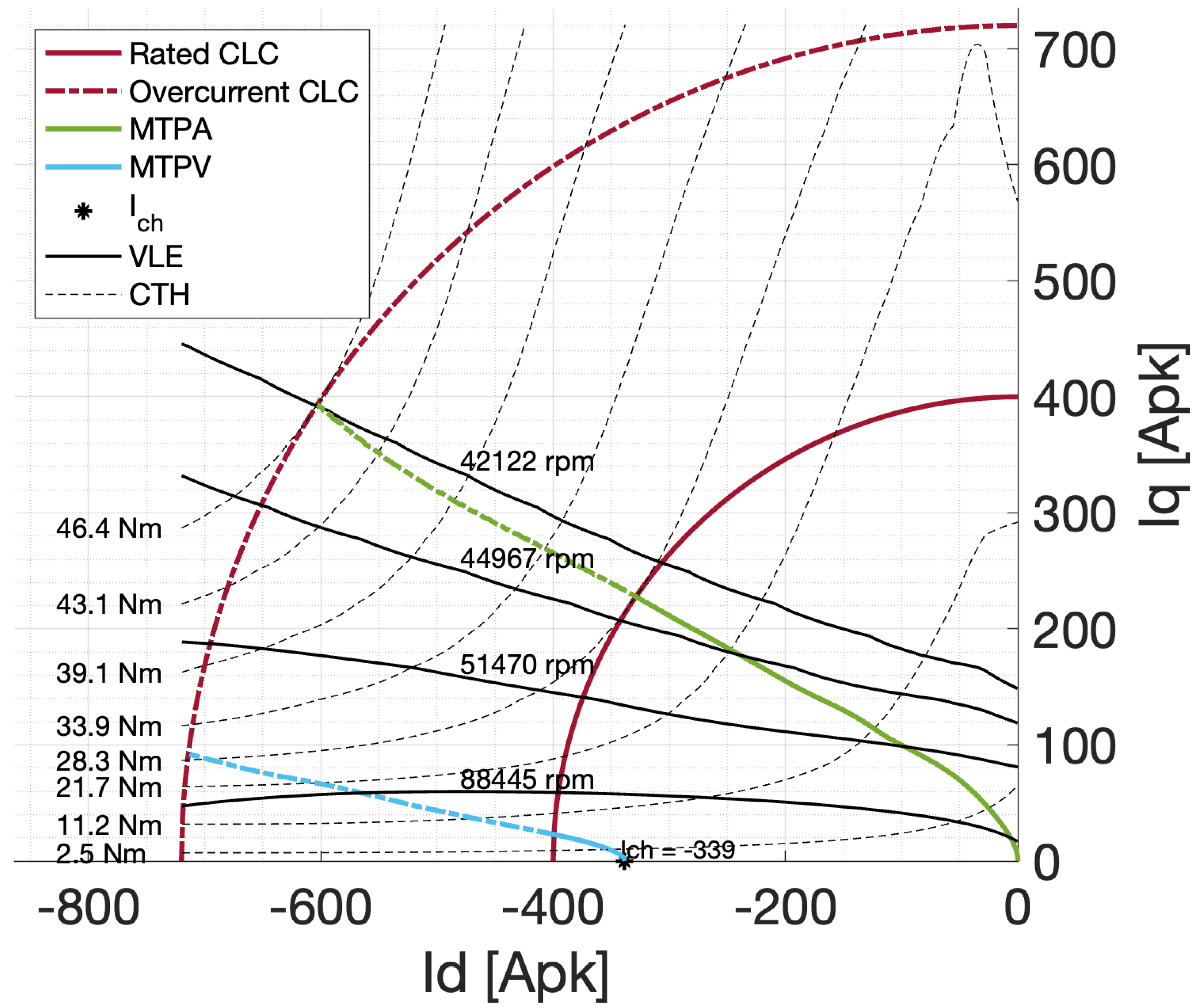
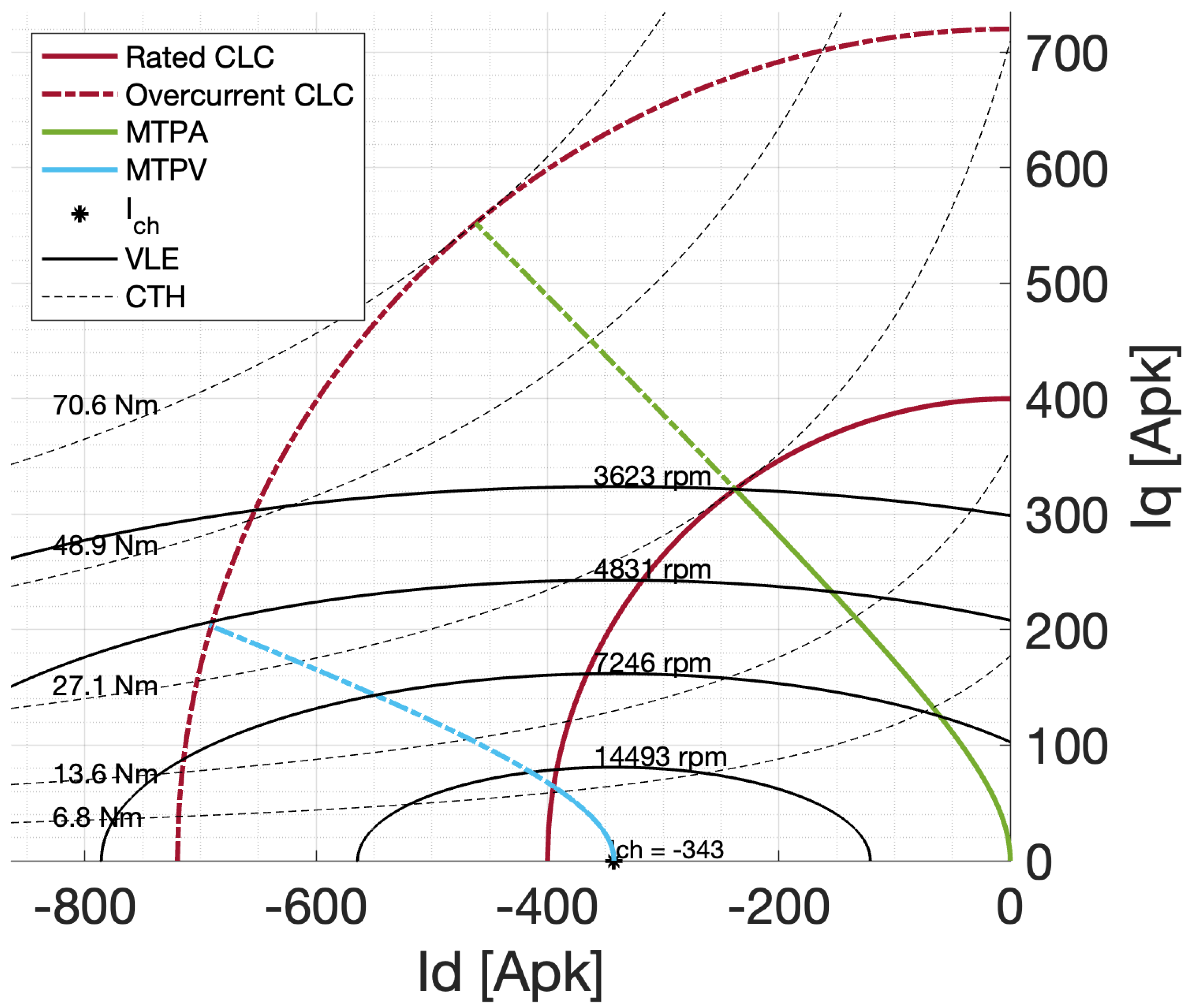
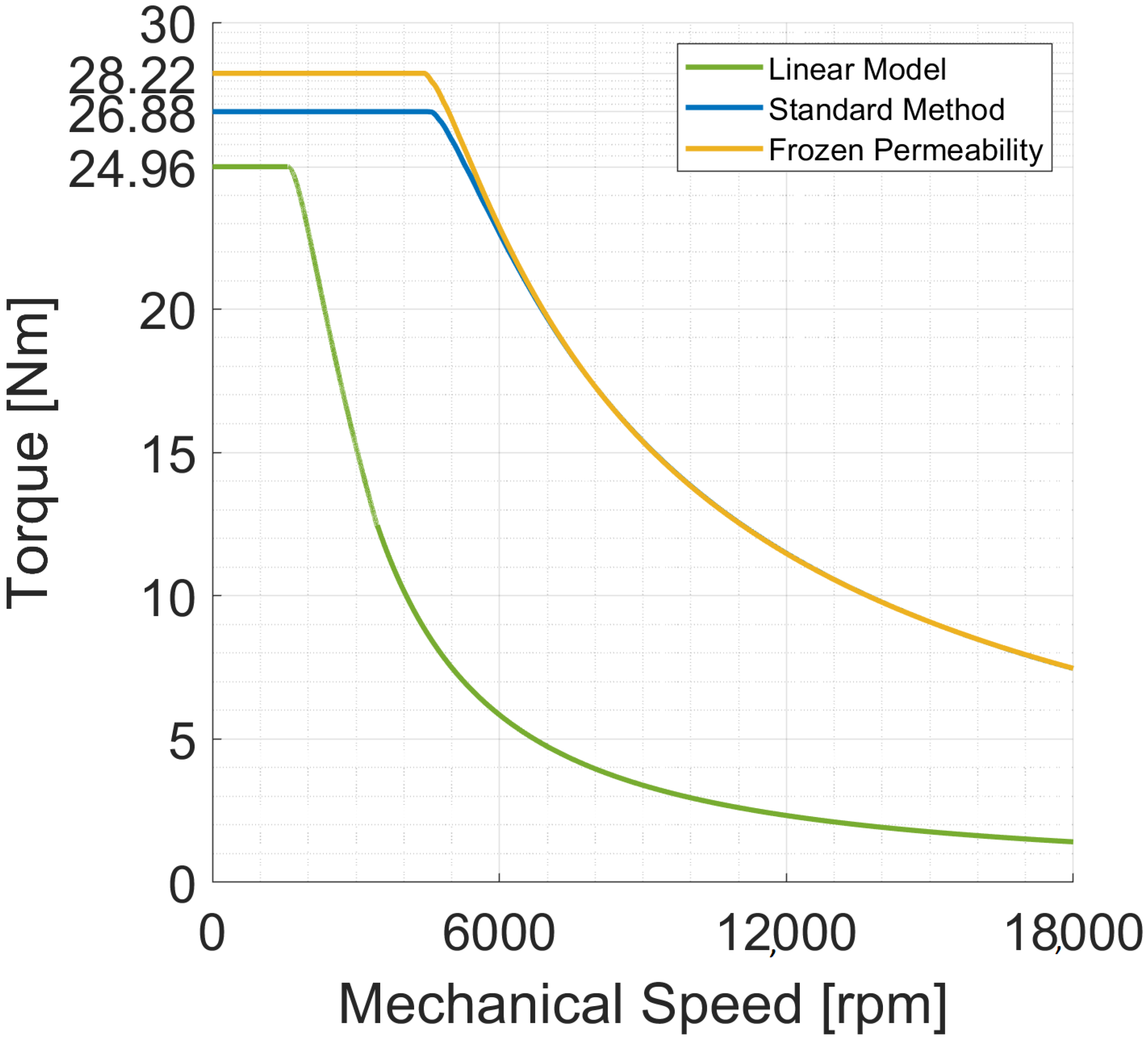
5.1. Generated Control Trajectories
5.2. Comparison of the Two Different Nonlinear Methods
- A range of current density is defined;
- A range of current phase angle is defined;
- For each couple of matrix, five different rotor positions are defined (6 mechanical degrees apart one from the other in order to cover 360 electrical degrees);
- The solution of the magnetic model is found for all the different rotor positions and torque is evaluated by the Maxwell’s stress tensor;
- The mean value of the torque on all position is computed;
6. Conclusions and Future Development
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yang, Z.; Shang, F.; Brown, I.P.; Krishnamurthy, M. Comparative Study of Interior Permanent Magnet, Induction, and Switched Reluctance Motor Drives for EV and HEV Applications. IEEE Trans. Transp. Electrif. 2015, 1, 245–254. [Google Scholar] [CrossRef]
- Pellegrino, G.; Vagati, A.; Guglielmi, P.; Boazzo, B. Performance Comparison Between Surface-Mounted and Interior PM Motor Drives for Electric Vehicle Application. IEEE Trans. Ind. Electron. 2012, 59, 803–811. [Google Scholar] [CrossRef]
- Grunditz, E.A.; Thiringer, T. Performance Analysis of Current BEVs Based on a Comprehensive Review of Specifications. IEEE Trans. Transp. Electrif. 2016, 2, 270–289. [Google Scholar] [CrossRef]
- Bilgin, B.; Magne, P.; Malysz, P.; Yang, Y.; Pantelic, V.; Preindl, M.; Korobkine, A.; Jiang, W.; Lawford, M.; Emadi, A. Making the Case for Electrified Transportation. IEEE Trans. Transp. Electrif. 2015, 1, 4–17. [Google Scholar] [CrossRef]
- Sarlioglu, B.; Morris, C.T. More Electric Aircraft: Review, Challenges, and Opportunities for Commercial Transport Aircraft. IEEE Trans. Transp. Electrif. 2015, 1, 54–64. [Google Scholar] [CrossRef]
- Sulligoi, G.; Vicenzutti, A.; Menis, R. All-Electric Ship Design: From Electrical Propulsion to Integrated Electrical and Electronic Power Systems. IEEE Trans. Transp. Electrif. 2016, 2, 507–521. [Google Scholar] [CrossRef]
- Jahns, T.M.; Kliman, G.B.; Neumann, T.W. Interior Permanent-Magnet Synchronous Motors for Adjustable-Speed Drives. IEEE Trans. Ind. Appl. 1986, IA-22, 738–747. [Google Scholar] [CrossRef]
- Morimoto, S.; Takeda, Y.; Hirasa, T.; Taniguchi, K. Expansion of operating limits for permanent magnet motor by current vector control considering inverter capacity. IEEE Trans. Ind. Appl. 1990, 26, 866–871. [Google Scholar] [CrossRef]
- Bianchi, N.; Jahns, T.M. Design, Analysis, and Control of Interior PM Synchronous Machines. In Proceedings of the IEEE Industry Applications Society, Seattle, WA, USA, 3–7 October 2004; Chapter 8. p. 1. [Google Scholar]
- Bianchi, N. Electrical Machine Analysis Using Finite Elements, 1st ed.; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar] [CrossRef]
- Stumberger, B.; Stumberger, G.; Dolinar, D.; Hamler, A.; Trlep, M. Evaluation of saturation and cross-magnetization effects in interior permanent-magnet synchronous motor. IEEE Trans. Ind. Appl. 2003, 39, 1264–1271. [Google Scholar] [CrossRef]
- Guglielmi, P.; Pastorelli, M.; Vagati, A. Cross-Saturation Effects in IPM Motors and Related Impact on Sensorless Control. IEEE Trans. Ind. Appl. 2006, 42, 1516–1522. [Google Scholar] [CrossRef]
- Miller, T.; Popescu, M.; Cossar, C.; McGilp, M. Performance estimation of interior permanent-magnet brushless motors using the voltage-driven flux-MMF diagram. IEEE Trans. Magn. 2006, 42, 1867–1872. [Google Scholar] [CrossRef]
- Bojoi, R.; Cavagnino, A.; Cossale, M.; Vaschetto, S. Methodology for the IPM motor magnetic model computation based on finite element analysis. In Proceedings of the IECON 2014—40th Annual Conference of the IEEE Industrial Electronics Society, Dallas, TX, USA, 29 October–1 November 2014; pp. 722–728. [Google Scholar] [CrossRef]
- Li, S.; Han, D.; Sarlioglu, B. Modeling of Interior Permanent Magnet Machine Considering Saturation, Cross Coupling, Spatial Harmonics, and Temperature Effects. IEEE Trans. Transp. Electrif. 2017, 3, 682–693. [Google Scholar] [CrossRef]
- Chen, X.; Wang, J.; Griffo, A. A High-Fidelity and Computationally Efficient Electrothermally Coupled Model for Interior Permanent-Magnet Machines in Electric Vehicle Traction Applications. IEEE Trans. Transp. Electrif. 2015, 1, 336–347. [Google Scholar] [CrossRef]
- Chen, X.; Wang, J.; Sen, B.; Lazari, P.; Sun, T. A High-Fidelity and Computationally Efficient Model for Interior Permanent-Magnet Machines Considering the Magnetic Saturation, Spatial Harmonics, and Iron Loss Effect. IEEE Trans. Ind. Electron. 2015, 62, 4044–4055. [Google Scholar] [CrossRef]
- Li, Z.; Li, H. MTPA control of PMSM system considering saturation and cross-coupling. In Proceedings of the 2012 15th International Conference on Electrical Machines and Systems (ICEMS), Sapporo, Japan, 21–24 October 2012; pp. 1–5. [Google Scholar]
- Li, S.; Sarlioglu, B.; Jurkovic, S.; Patel, N.; Savagian, P. Analysis of temperature effects on performance of interior permanent magnet machines. In Proceedings of the 2016 IEEE Energy Conversion Congress and Exposition (ECCE), Milwaukee, WI, USA, 18–22 September 2016; pp. 1–8. [Google Scholar] [CrossRef]
- Consoli, A.; Scarcella, G.; Scelba, G.; Sindoni, S.; Testa, A. Steady-State and Transient Analysis of Maximum Torque per Ampere Control for IPMSMs. In Proceedings of the 2008 IEEE Industry Applications Society Annual Meeting, Edmonton, AB, Canada, 5–9 October 2008; pp. 1–8. [Google Scholar] [CrossRef]
- Mademlis, C.; Agelidis, V. On considering magnetic saturation with maximum torque to current control in interior permanent magnet synchronous motor drives. In Proceedings of the 2002 IEEE Power Engineering Society Winter Meeting, Conference Proceedings (Cat. No.02CH37309). New York, NY, USA, 27–31 January 2002; Volume 2, p. 1234. [Google Scholar] [CrossRef]
- Armando, E.; Guglielmi, P.; Pellegrino, G.; Pastorelli, M.; Vagati, A. Accurate Modeling and Performance Analysis of IPM-PMASR Motors. IEEE Trans. Ind. Appl. 2009, 45, 123–130. [Google Scholar] [CrossRef]
- Hadžiselimović, M.; Štumberger, G.; Štumberger, B.; Zagradišnik, I. Magnetically nonlinear dynamic model of synchronous motor with permanent magnets. J. Magn. Magn. Mater. 2007, 316, e257–e260. [Google Scholar] [CrossRef]
- Bianchi, N.; Bolognani, S. Magnetic models of saturated interior permanent magnet motors based on finite element analysis. In Proceedings of the Conference Record of 1998 IEEE Industry Applications Conference, Thirty-Third IAS Annual Meeting (Cat. No.98CH36242). St. Louis, MO, USA, 12–15 October 1998; Volume 1, pp. 27–34. [Google Scholar] [CrossRef]
- Lopez-Torres, C.; Bacco, G.; Bianchi, N.; Espinosa, A.G.; Romeral, L. A Parallel Analytical Computation of Synchronous Reluctance Machine. In Proceedings of the 2018 XIII International Conference on Electrical Machines (ICEM), Alexandroupoli, Greece, 3–6 September 2018; pp. 25–31. [Google Scholar] [CrossRef]
- Hoang, K.D.; Aorith, H.K.A. Online Control of IPMSM Drives for Traction Applications Considering Machine Parameter and Inverter Nonlinearities. IEEE Trans. Transp. Electrif. 2015, 1, 312–325. [Google Scholar] [CrossRef]
- Yan, Y.; Zhu, J.; Lu, H.; Guo, Y.; Wang, S. Study of A PMSM Model Incorporating Structural and Saturation Saliencies. In Proceedings of the 2005 International Conference on Power Electronics and Drives Systems, Kuala Lumpur, Malaysia, 28 November–1 December 2005; Volume 1, pp. 575–580. [Google Scholar] [CrossRef]
- Yan, Y.; Zhu, J.; Guo, Y.; Lu, H. Modeling and Simulation of Direct Torque Controlled PMSM Drive System Incorporating Structural and Saturation Saliencies. In Proceedings of the Conference Record of the 2006 IEEE Industry Applications Conference Forty-First IAS Annual Meeting, Tampa, FL, USA, 8–12 October 2006; Volume 1, pp. 76–83. [Google Scholar] [CrossRef]
- Liu, Z.; Feng, G.; Han, Y. Extended-Kalman-Filter-Based Magnet Flux Linkage and Inductance Estimation for PMSM Considering Magnetic Saturation. In Proceedings of the 2021 36th Youth Academic Annual Conference of Chinese Association of Automation (YAC), Nanchang, China, 28–30 May 2021; pp. 430–435. [Google Scholar] [CrossRef]
- Park, G.; Kim, G.; Gu, B.G. Sensorless PMSM Drive Inductance Estimation Based on a Data-Driven Approach. Electronics 2021, 10, 791. [Google Scholar] [CrossRef]
- Wu, H.; Zhao, W.; Zhu, G.; Li, M. Optimal Design and Control of a Spoke-Type IPM Motor with Asymmetric Flux Barriers to Improve Torque Density. Symmetry 2022, 14, 1788. [Google Scholar] [CrossRef]
- Dorrell, D.G. On the Use of the Frozen Permeability Method for Torque Separation in Synchronous Machines Invited Oral Poster. In Proceedings of the 2016 International Conference of Asian Union of Magnetics Societies (ICAUMS), Tainan, Taiwan, 1–5 August 2016; pp. 1–4. [Google Scholar] [CrossRef]
- Chen, J.; Li, J.; Qu, R.; Ge, M. Magnet-Frozen-Permeability FEA and DC-Biased Measurement for Machine Inductance: Application on a Variable-Flux PM Machine. IEEE Trans. Ind. Electron. 2018, 65, 4599–4607. [Google Scholar] [CrossRef]
- Neumann, J.; Hénaux, C.; Fadel, M.; Prieto, D.; Fournier, E.; Yamdeu, M.T. Improved dq model and analytical parameters determination of a Permanent Magnet Assisted Synchronous Reluctance Motor (PMa-SynRM) under saturation using Frozen Permeability Method. In Proceedings of the 2020 International Conference on Electrical Machines (ICEM), Gothenburg, Sweden, 23–26 August 2020; Volume 1, pp. 481–487. [Google Scholar] [CrossRef]
- Shuto, D.; Takahashi, Y.; Fujiwara, K. Frozen Permeability Method for Magnetic Field Analysis of Permanent Magnet Motors Considering Hysteretic Property. IEEE Trans. Magn. 2019, 55, 1–4. [Google Scholar] [CrossRef]
- Meeker, D. Finite Element Method Magnetic. User’s Manual. 2018. Available online: https://www.femm.info/wiki/HomePage (accessed on 16 May 2020).


| Parameter | Symbol | Value | Unit |
|---|---|---|---|
| n° of Pole Pairs | 2 | - | |
| n° of Stator Slots | Q | 24 | - |
| n° of Conductors per Slot | 2 | - | |
| Stack Length | 100 | mm | |
| Phase Resistance | R | 0.0032 | |
| Flux Linkage of PM | 0.0128 | Wb | |
| Rated Current | 400 | A | |
| Overload Current | 720 | A | |
| DC BUS Voltage | 48 | V | |
| Maximum Speed | 30 | krpm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bianchini, C.; Bisceglie, G.; Torreggiani, A.; Davoli, M.; Macrelli, E.; Bellini, A.; Frigieri, M. Effects of the Magnetic Model of Interior Permanent Magnet Machine on MTPA, Flux Weakening and MTPV Evaluation. Machines 2023, 11, 77. https://doi.org/10.3390/machines11010077
Bianchini C, Bisceglie G, Torreggiani A, Davoli M, Macrelli E, Bellini A, Frigieri M. Effects of the Magnetic Model of Interior Permanent Magnet Machine on MTPA, Flux Weakening and MTPV Evaluation. Machines. 2023; 11(1):77. https://doi.org/10.3390/machines11010077
Chicago/Turabian StyleBianchini, Claudio, Giorgio Bisceglie, Ambra Torreggiani, Matteo Davoli, Elena Macrelli, Alberto Bellini, and Matteo Frigieri. 2023. "Effects of the Magnetic Model of Interior Permanent Magnet Machine on MTPA, Flux Weakening and MTPV Evaluation" Machines 11, no. 1: 77. https://doi.org/10.3390/machines11010077
APA StyleBianchini, C., Bisceglie, G., Torreggiani, A., Davoli, M., Macrelli, E., Bellini, A., & Frigieri, M. (2023). Effects of the Magnetic Model of Interior Permanent Magnet Machine on MTPA, Flux Weakening and MTPV Evaluation. Machines, 11(1), 77. https://doi.org/10.3390/machines11010077








