Research on Pneumatic–liquid On-Line Automatic Balance Technology for High-End Turbine Units
Abstract
:1. Introduction
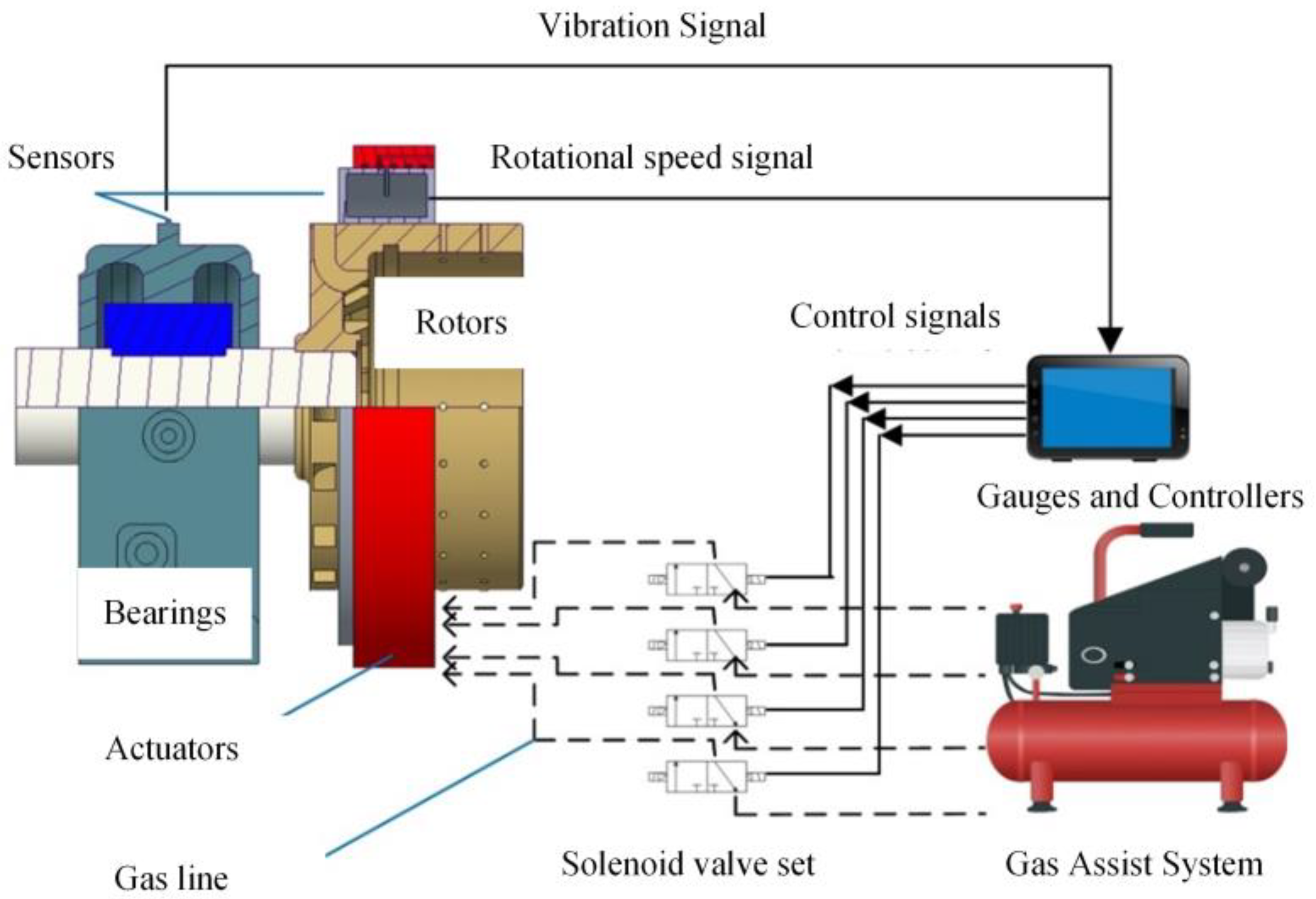
2. Composition and Working Principle of Pneumatic–liquid On-Line Automatic Balance System
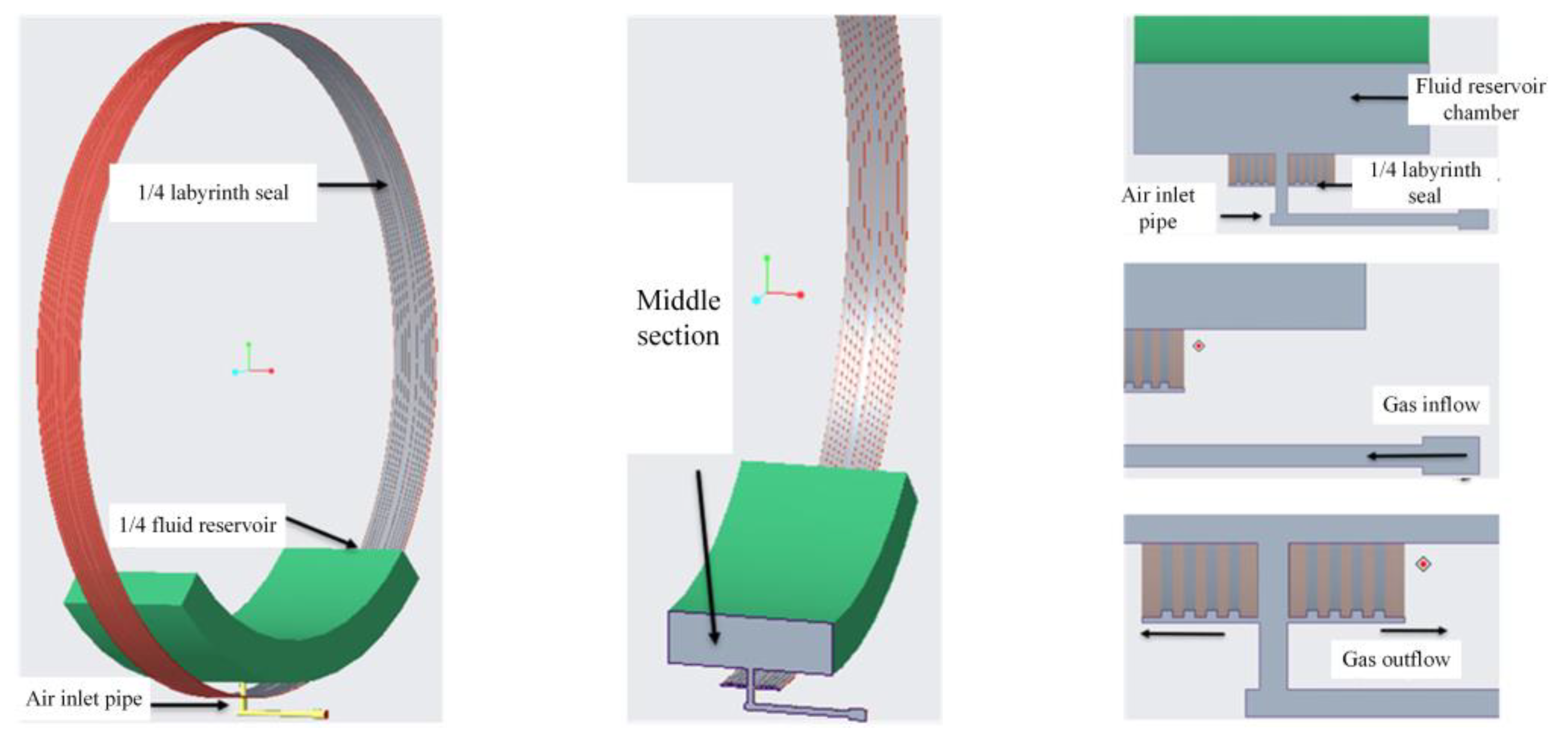
2.1. Composition of Pneumatic–liquid On-Line Automatic Balance System
2.2. Working Principle of Pneumatic–liquid On-Line Automatic Balance System
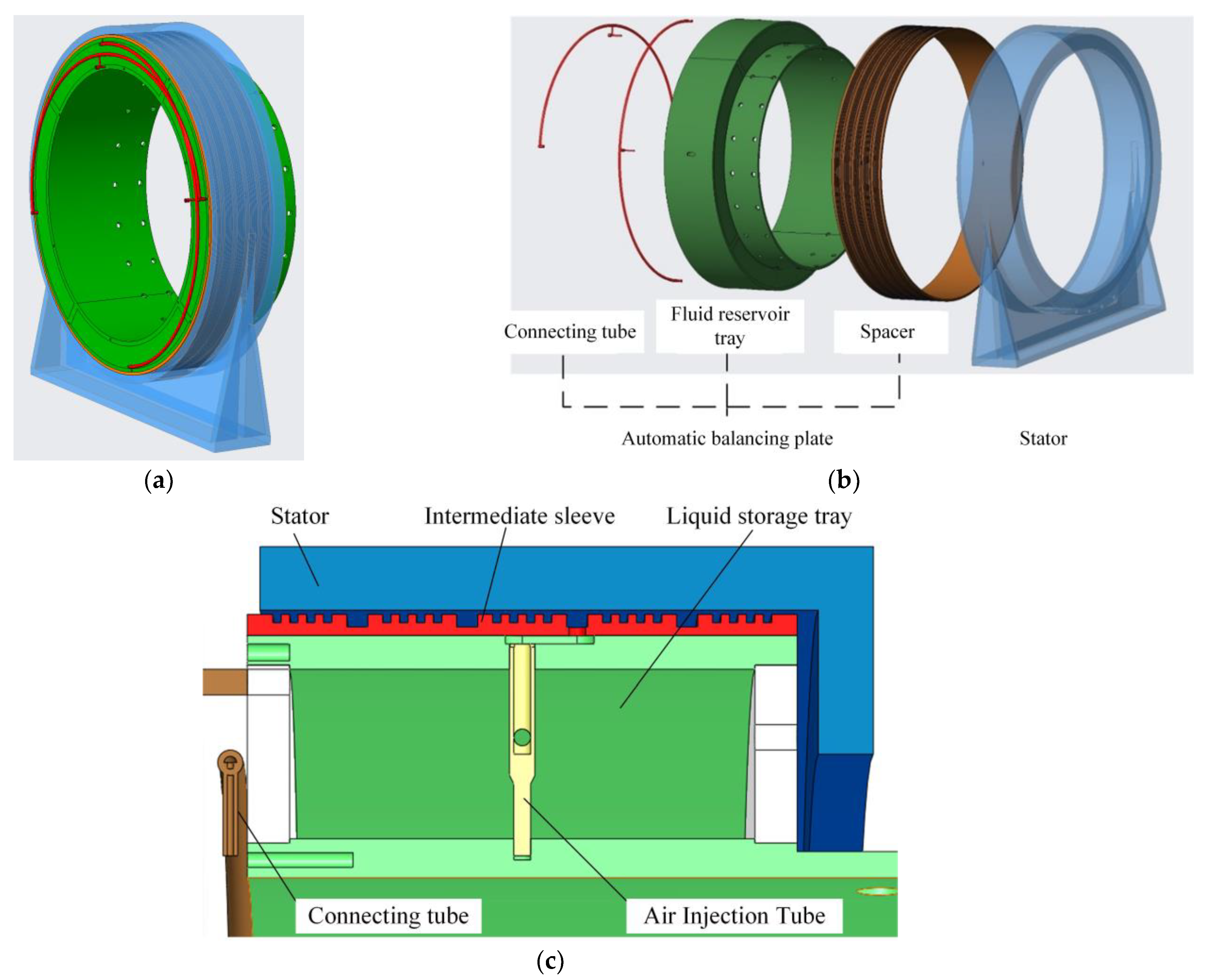
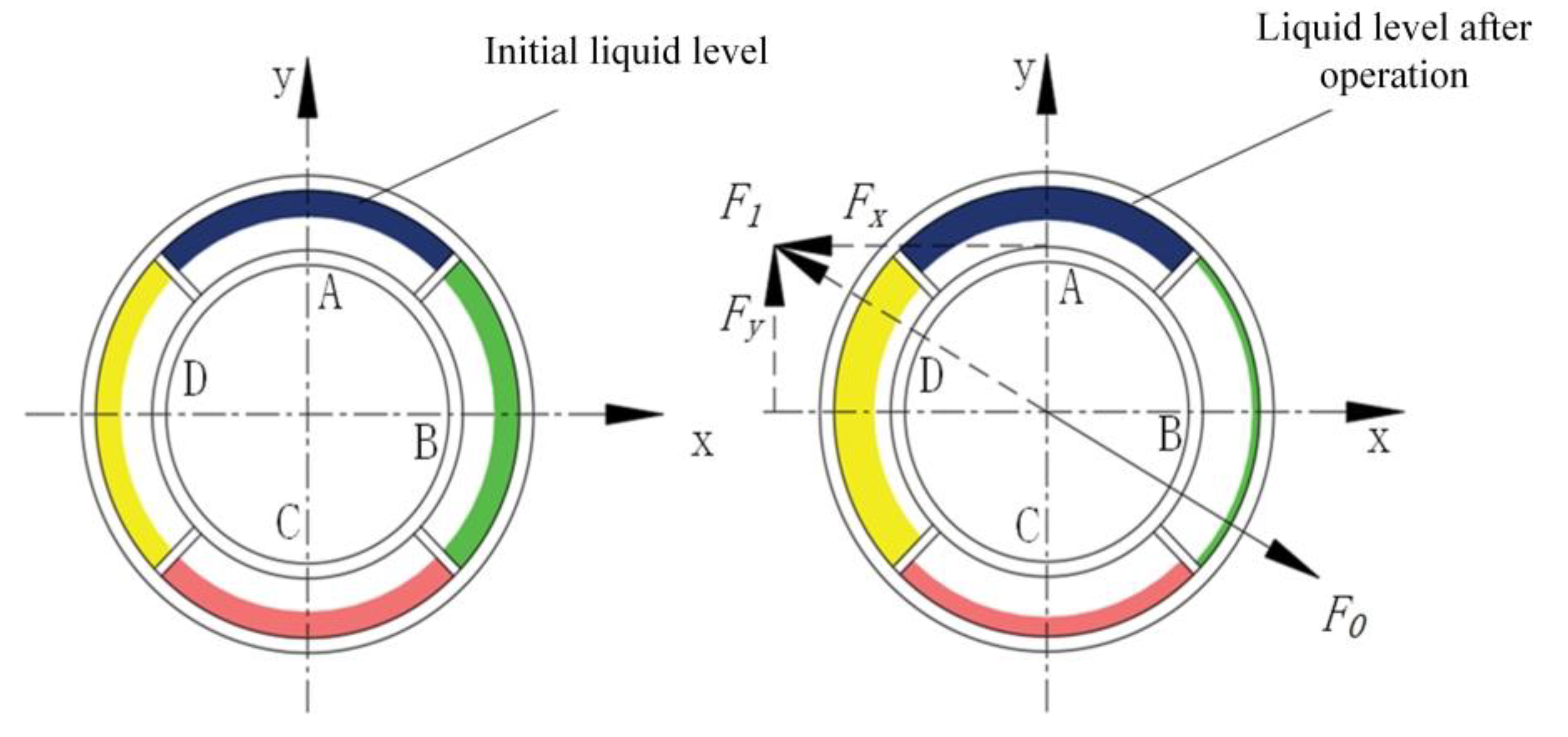
2.2.1. Actuator Balancing Mechanism
2.2.2. Actuator Balance Performance
3. Actuator Performance Simulation Study
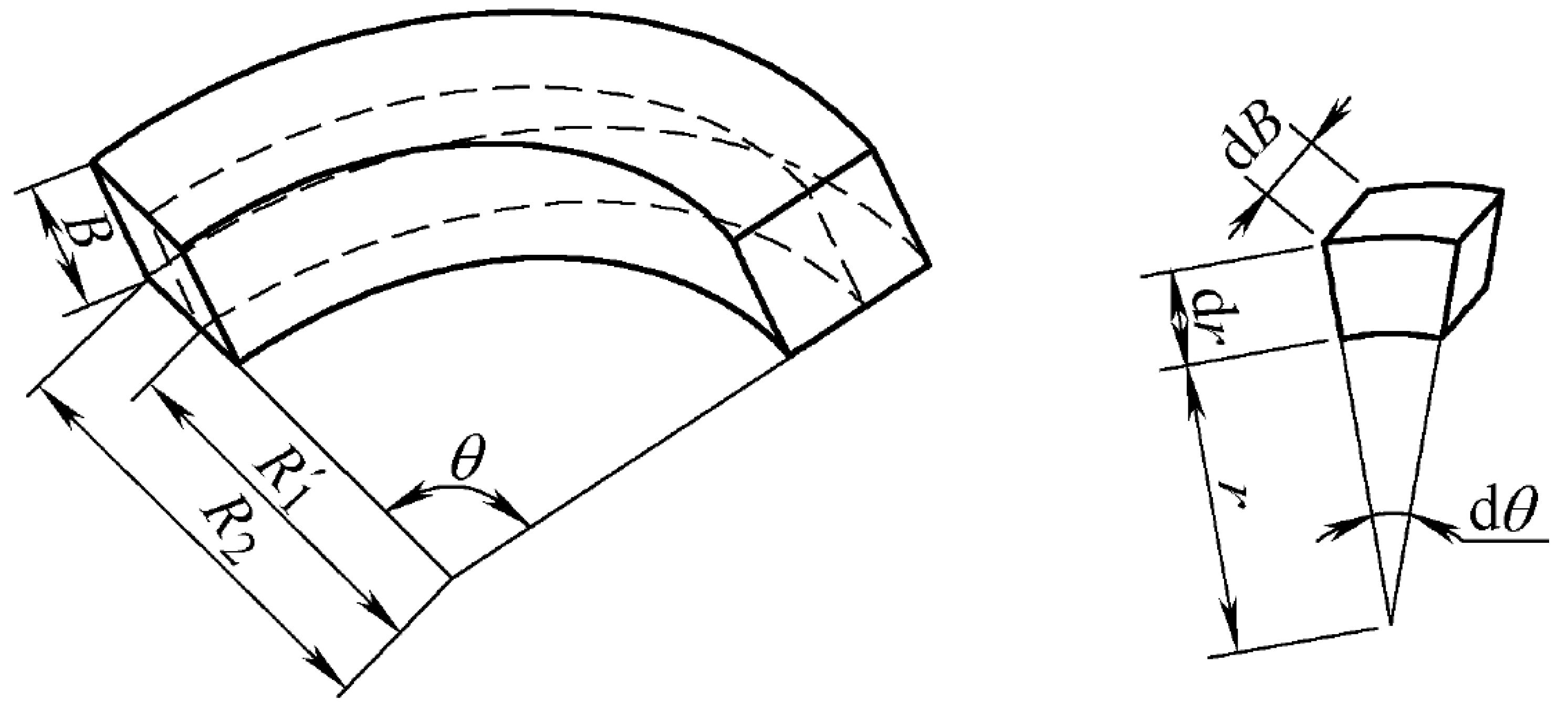
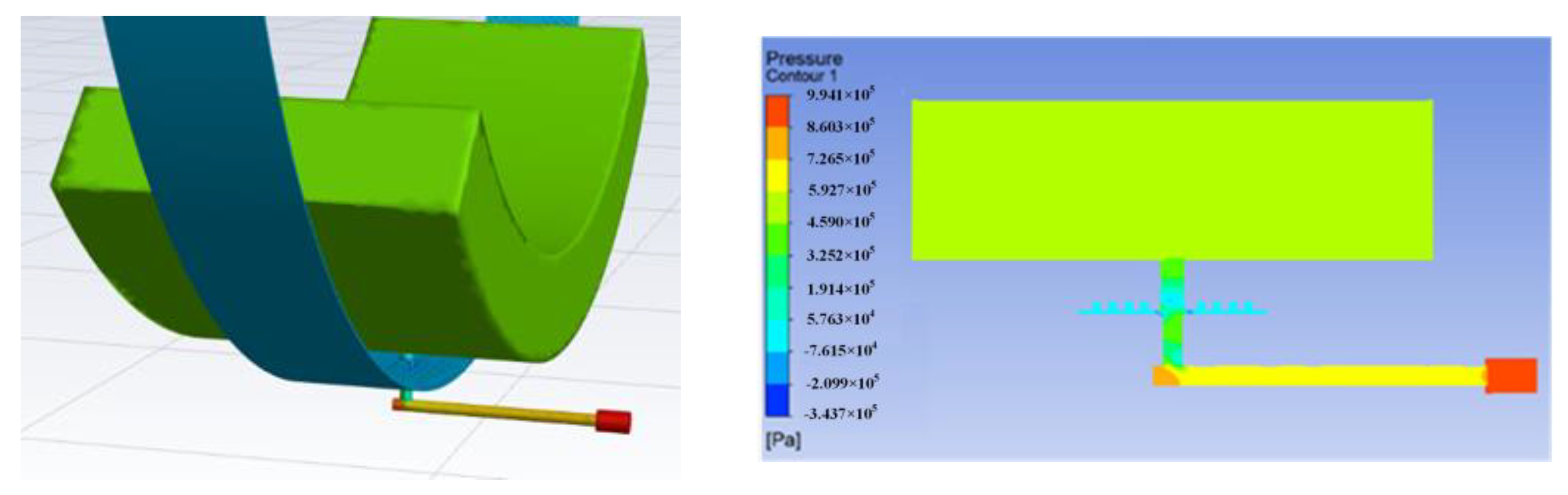
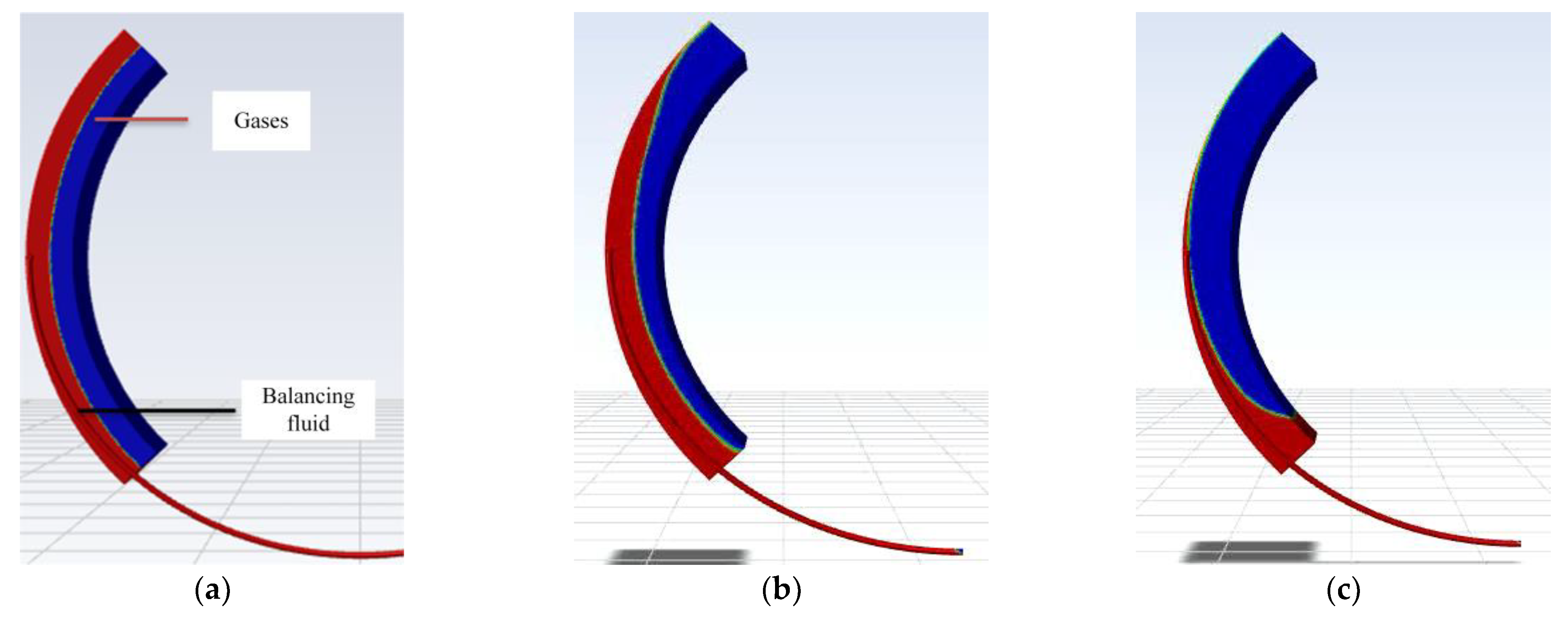
3.1. Gas Drive Force Transfer Simulation Analysis
- (1)
- Extracting the gas flow field inside the actuator, the pressure distribution nephogram when the external gas enters the reservoir chamber was obtained by Fluent analysis. When the stator inlet pipe diameter is initially designed to 5 mm, the internal pressure of the reservoir cavity can reach 0.47 MPa and the transmission efficiency of the driving force is 47%; and
- (2)
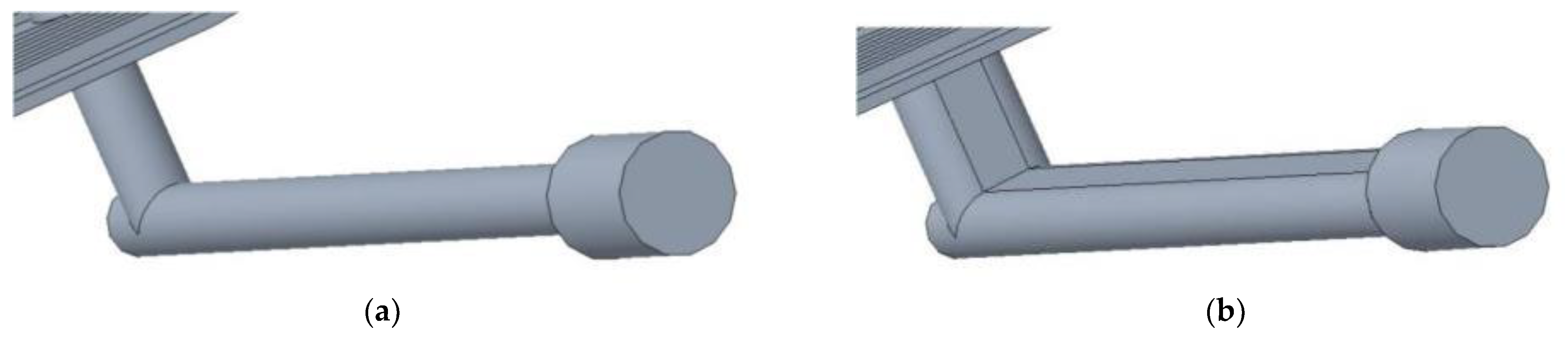
- The correspondence between the gas driving force and the cross-sectional area and equivalent diameter of the gas pipe was obtained, and it was obvious that the elliptical pipe cross-section is conducive to increasing the transmission efficiency of the gas driving force, which provides reference for the subsequent structural design of the stator inlet pipe part of the actuator. Furthermore, the intake pipe cross-sectional area can be considered to be appropriately increased to optimize the actuator intake effect.
3.2. Equilibrium Fluid Transfer Simulation Analysis
- (1)
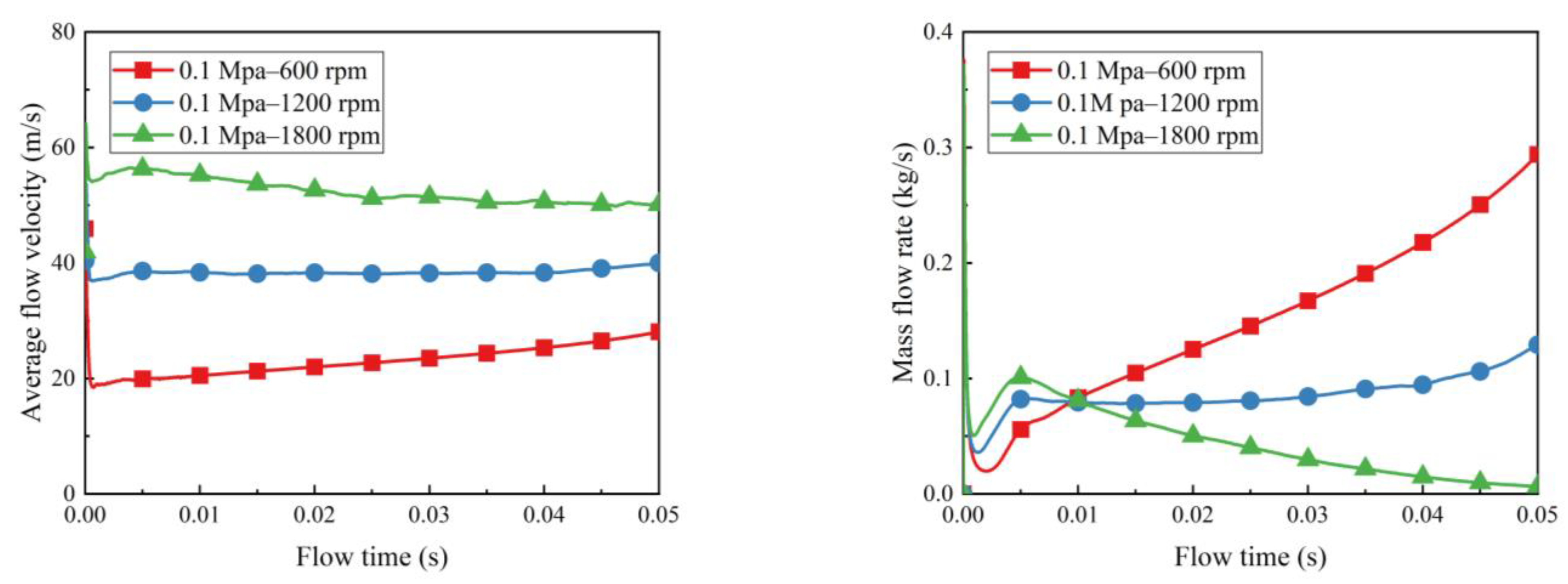
- The inlet pressure was set to 0.1 Mpa, the outlet radius was unchanged, and the speed of the reference system was changed to 600, 1200, and 1800 rpm for three working conditions, respectively. The results after simulation calculation are shown in Figure 13.
- (2)
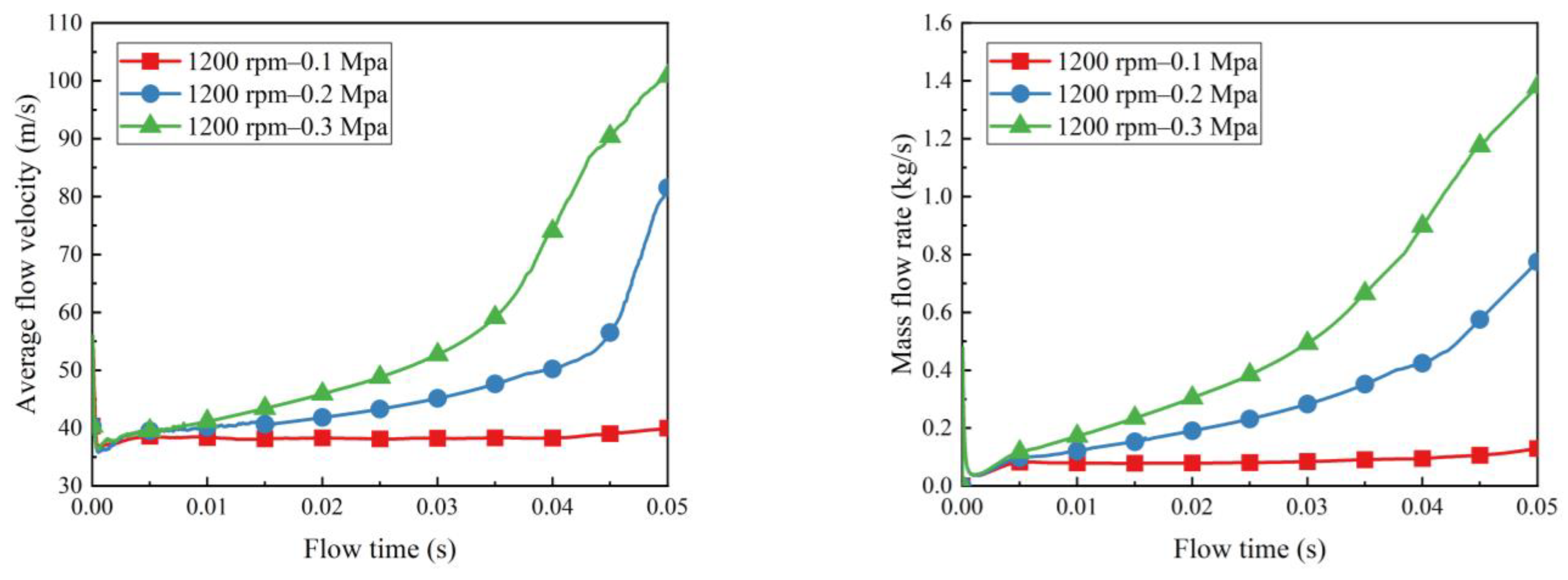
- The speed of the reference system was 1200 rpm, the outlet radius was 3 mm, and the pressure inlet was set for three conditions, which were 0.1, 0.2, and 0.3 Mpa, and plots of the average flow velocity and mass flux at the exit surface with time were obtained. The results are shown in Figure 14.
- (3)
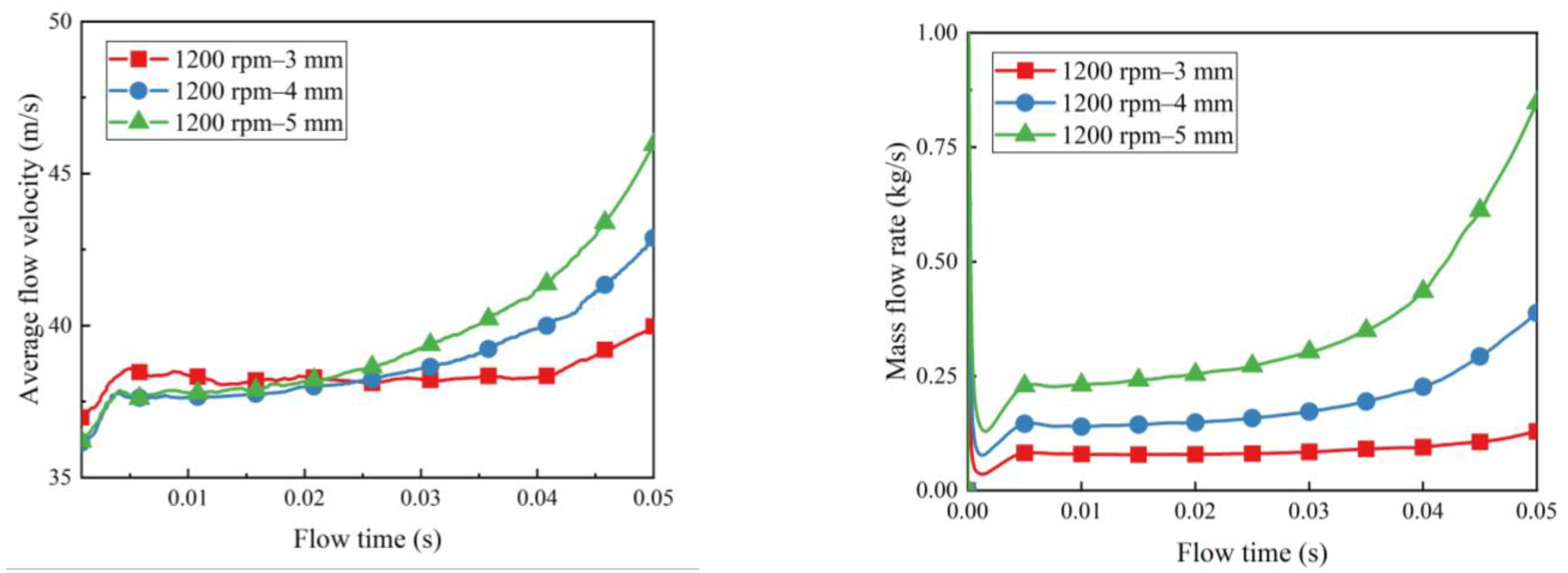
- Assuming the inlet and outlet pressure was 0.1 Mpa and the speed of the reference system was 1200 rpm, the outlet radius was set to 3, 4 and 5 mm, respectively. After transient analysis of two-phase flow, average flow velocity and mass flux plots at the various outlet radius were obtained. The simulation results are shown in Figure 15.
- (1)
- At three different speed operating conditions, the trend of the rate of balancing liquid transfer with time is stable, which proves that the equilibrium device can complete the balance process stably at all three speeds. The flow direction of the two-phase flow is controlled by both gas pressure and rotational speed. The flow direction of the two-phase flow is controlled by both gas pressure and rotational speed. When the rotational speed increases, the rate of flow decreases and the balance fluid is more likely to be affected and remain in the chamber, slowing down the response to failure;
- (2)
- The average flow velocity and mass rate of flow increase with increasing inlet pressure. When the inlet pressure is low, the transfer speed is stable and the control accuracy is high; when the inlet pressure increases, the speed changes faster and the control accuracy decreases. Therefore, when controlling the balance fluid transfer, it is important to choose the appropriate pressure for a stable balance process; and
- (3)
- As the cross-sectional diameter of the connecting tube increases, there is a significant increase in average flow velocity and mass flux. In terms of flux, the larger the exit radius, the more significant the increase. However, due to the structural limitations of the actuator reservoir chamber itself, an excessively large radius of the connecting tube will cause the high-pressure gas phase to react more easily on it, and the gas entering the connecting tube is not conducive to the full transfer of liquid, so a reasonable size must be designed considering a combination of factors in future structural optimization.
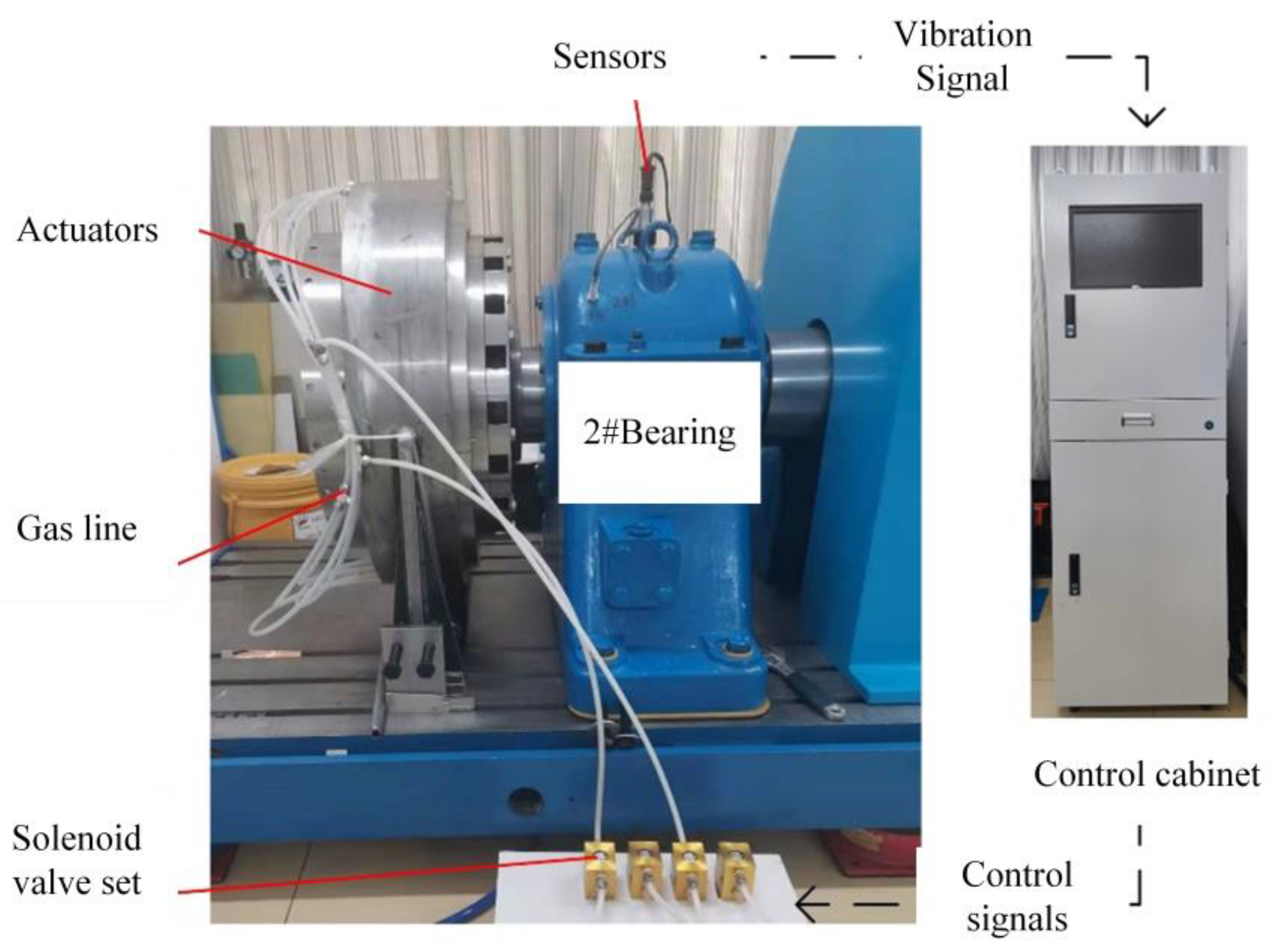
4. Experimental Study of Pneumatic–liquid On-Line Automatic Balance System
4.1. Pneumatic–liquid On-Line Automatic Balance System
4.2. Actuator Performance Testing
4.2.1. Actuator Static Performance Test
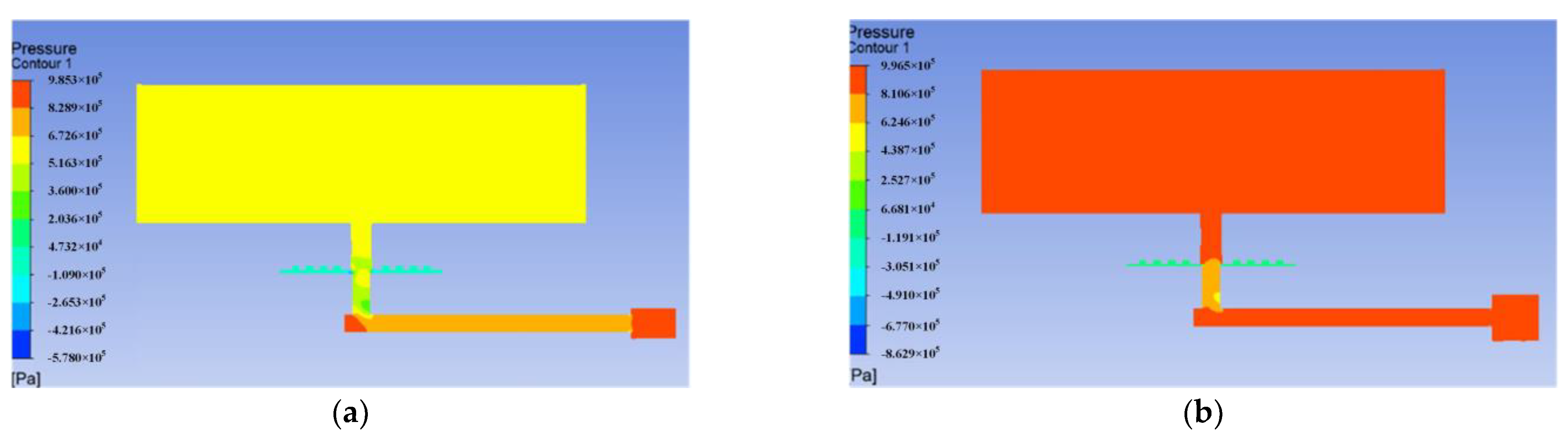
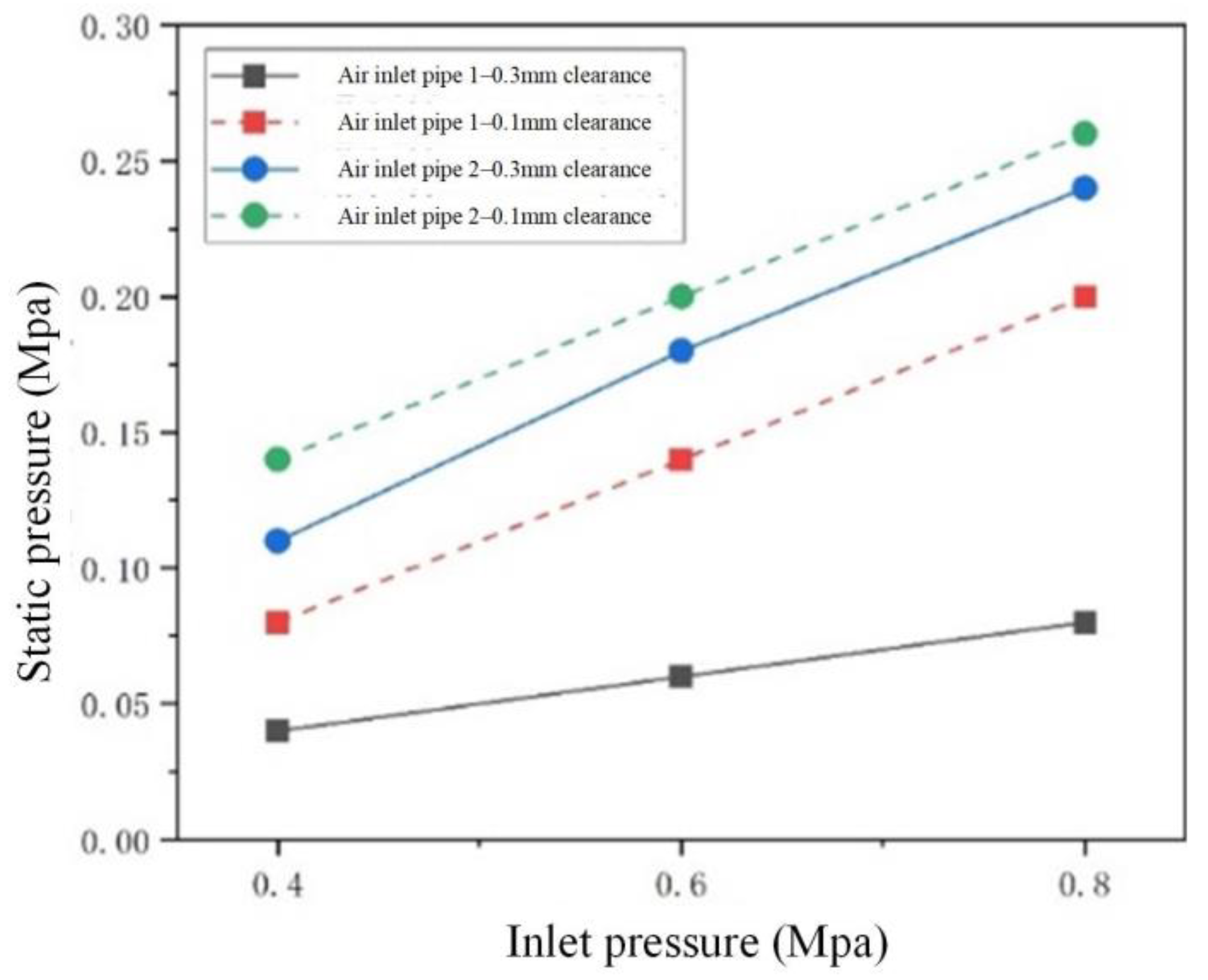
- (1)
- By simulation, the intake tube cross-sectional area is tripled and the intake efficiency is doubled in dynamic time. In the actual static test, under the same sealing gap, the air intake efficiency using intake tube 2 is nearly twice that of intake tube 1, which is comparable to the simulation results; and
- (2)
- The actual obtained static pressure, which can be considered as the minimum of the dynamic gas driving force. The actual assembly gap is about 0.3 mm, and the preliminary test ensures that the pressure in the cavity reaches 0.1 MPa or more, so it is more reasonable to choose the air inlet pipe 2.
4.2.2. Actuator Dynamic Performance Test
4.3. Automatic Balancing Test
5. Summary
- (1)
- The current status of research on dynamic balancing of large rotating equipment and the development direction of structural optimization design of liquid type automatic balancing actuator were summarized. For the application of automatic balancing of large rotating equipment, a PLOABS system was proposed, whose composition and working principle were introduced, and the theoretical analysis of balancing capacity and balancing accuracy was carried out;
- (2)
- The actuator internal gas flow field model was established, which was analyzed to obtain the actuator internal flow field characteristics, and the model stator inlet pipe parameters were adjusted to obtain higher and more stable gas driving force. The actuator internal gas–liquid two-phase flow field model was established, which was analyzed to obtain the equilibrium liquid transfer characteristics under different working conditions, by adjusting the reference system speed, pressure inlet, outlet radius, providing reference for the subsequent actuator optimization design; and
- (3)
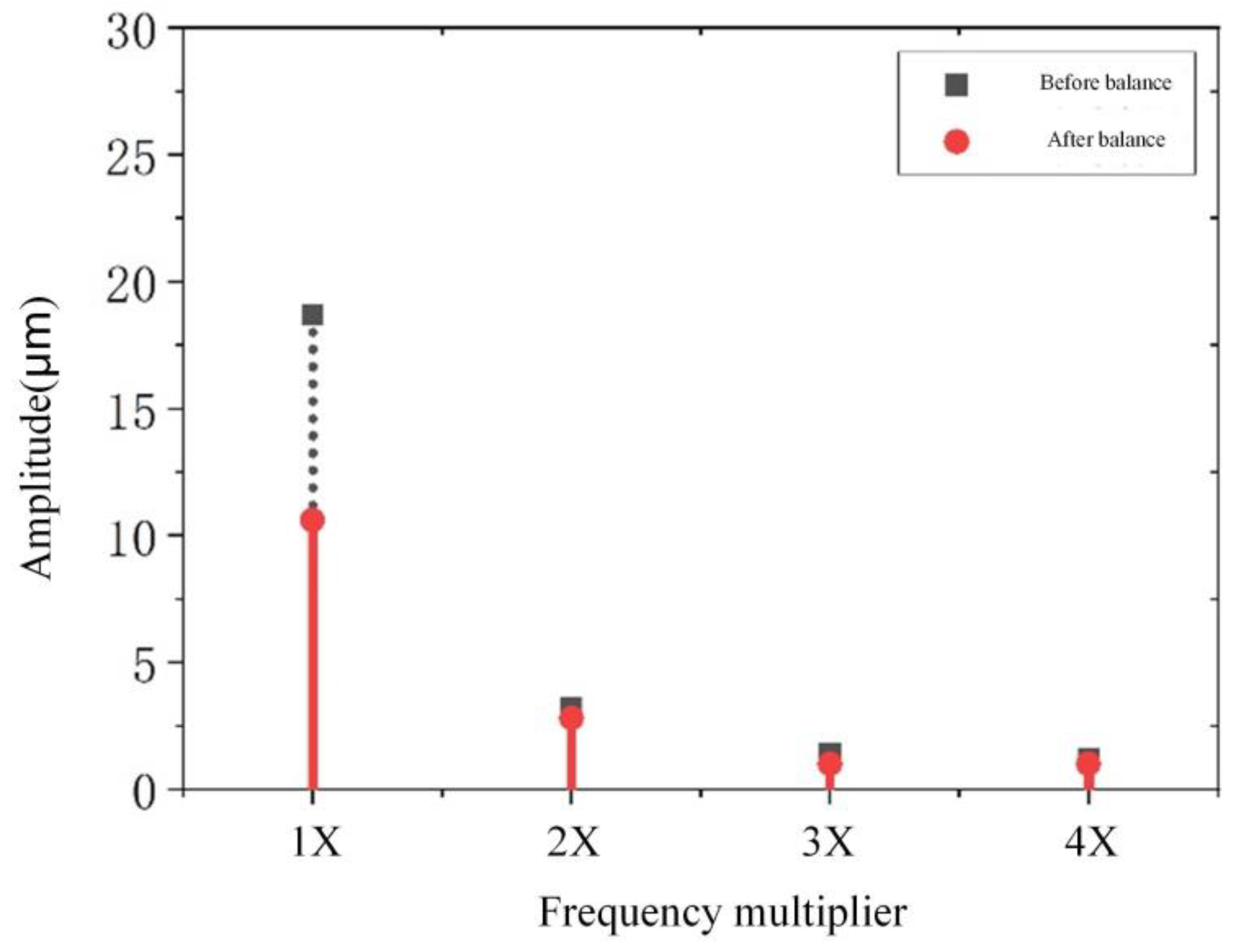
- Based on a large rotating equipment simulation test bench, the PLOABS system was built to test the static and dynamic performance of the actuator, in order to make the actuator meet the action requirements. Finally, the automatic balancing test was performed, and the system reduced the measured rotor amplitude from 18.3 to 10.6 um, which verified the feasibility and effectiveness of the improved automatic balancing system, and provided theoretical support and experimental verification for the subsequent structural innovation and engineering application of the actuator.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tong, Z.T.; Yang, X.M.; Shang, P.X.; Ma, J.Y.; Zhang, Y.; Zhang, C. Effect of Inlet Condition on the Performance Curve of a 10 MW Supercritical Carbon Dioxide Centrifugal Compressor. Machines 2022, 10, 359. [Google Scholar] [CrossRef]
- Tuerxun, W.; Xu, C.; Haderbieke, M.; Guo, L.; Cheng, Z.M. A Wind Turbine Fault Classification Model Using Broad Learning System Optimized by Improved Pelican Optimization Algorithm. Machines 2022, 10, 407. [Google Scholar] [CrossRef]
- Jalayer, M.; Kaboli, A.; Orsenigo, C.; Vercellis, C. Fault Detection and Diagnosis with Imbalanced and Noisy Data: A Hybrid Framework for Rotating Machinery. Machines 2022, 10, 237. [Google Scholar] [CrossRef]
- Jin, X.; Liu, Y. Numerical and Experimental Analysis for the Dynamics of Flawed-Machining Rod-Disk Rotor with Inner Misalignment. Machines 2022, 10, 355. [Google Scholar] [CrossRef]
- Ranjan, G.; Tiwari, R. Application of active magnetic bearings for in situ flexible rotor residual balancing using a novel generalized influence coefficient method. Inverse Probl. Sci. Eng. 2019, 27, 943–968. [Google Scholar] [CrossRef]
- Xu, X.; Fan, P.P. Rigid Rotor Dynamic Balancing by Two-plane Correction with the Influence Coefficient Method. In Applied Mechanics and Materials; Trans Tech Publications Ltd.: Wallerau, Switzeland, 2013; Volume 365, pp. 211–215. [Google Scholar]
- Quartarone, C.; Beqari, J.; Detoni, J.G.; Cometti, F.; Emelli, E. Balancing of Turbomolecular Pumps: Modal Balancing Approach and Experimental Results. In Proceedings of the 10th International Conference on Rotor Dynamics—IFToMM; Springer: Cham, Switzereland, 2019; Volume 4, pp. 119–128. [Google Scholar]
- Bin, G.F.; He, L.D.; Gao, J.J.; Li, L.B. High-speed dynamic balancing method for low pressure rotor of a large steam turbine based on modal shape analysis. J. Sound Vib. 2013, 32, 87–92. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, M.; Yao, H.Z.; Gou, Y.J.; Wang, X.Y. A modal-based balancing method for a high-speed rotor without trial weights. Mech. Sci. 2021, 12, 85–96. [Google Scholar] [CrossRef]
- Liu, S. A modified low-speed balancing method for flexible rotors based on holospectrum. Mech. Syst. Signal Process. 2007, 21, 348–364. [Google Scholar] [CrossRef]
- Hao, C.Z.; Du, J.R.; Liang, H.R. Imbalanced Fault Diagnosis of Rolling Bearing Using Data Synthesis Based on Multi-Resolution Fusion Generative Adversarial Networks. Machines 2022, 10, 295. [Google Scholar] [CrossRef]
- Fan, H.W.; Jing, M.Q.; Wang, R.C.; Liu, H.; Zhi, J.J. New electromagnetic ring balancer for active imbalance compensation of rotating machinery. J. Sound Vib. 2014, 333, 3837–3858. [Google Scholar] [CrossRef]
- Zachwieja, J.; Jarzyna, T. Dynamic Balancing of a Horizontal Rotor with a Cracked Shaft. In Scientific Session on Applied Mechanics X; AIP Publishing: Melville, NY, USA, 2019. [Google Scholar]
- Su, N.Q.; Li, X.; Zhang, Q.H. Fault Diagnosis of Rotating Machinery Based on Wavelet Domain Denoising and Metric Distance. IEEE Access 2019, 7, 73262–73270. [Google Scholar] [CrossRef]
- Li, K.X.; Peng, C.; Deng, Z.Q.; Huang, W.; Zhang, Z.M. Field dynamic balancing for active magnetic bearings supporting rigid rotor shaft based on extended state observer. Mech. Syst. Signal Process. 2021, 158, 107801. [Google Scholar] [CrossRef]
- Pan, X.; Lu, J.Q.; Huo, J.J.; Gao, J.J.; Wu, H.Q. A Review on Self-Recovery Regulation (SR) Technique for Unbalance Vibration of High-End Equipment. Chin. J. Mech. Eng. 2020, 33, 89. [Google Scholar] [CrossRef]
- Shin, K.K.; Ni, J. Adaptive control of active balancing systems for speed-varying rotors using feedforward gain adaptation technique. J. Dyn. Syst. Meas. Control.-Trans. Asme 2001, 123, 346–352. [Google Scholar] [CrossRef]
- Pan, X.; He, X.T.; Wu, H.Q.; Ju, C.L.; Jiang, Z.N.; Gao, J.J. Optimal Design of Novel Electromagnetic-Ring Active Balancing Actuator with Radial Excitation. Chin. J. Mech. Eng. 2021, 34, 9. [Google Scholar] [CrossRef]
- Pan, X.; Xie, Z.; Lu, J.; Wu, H.Q.; Gao, J.J.; Jiang, Z.N. Novel Liquid Transfer Active Balancing System for Hollow Rotors of High-Speed Rotating Machinery. Appl. Sci. 2019, 9, 833. [Google Scholar] [CrossRef]
- Pan, X.; Gao, J.J. Research on the principle and method of pneumatic liquid and electromagnetic slip ring automatic balancing for high-end Machine Tools. J. Mech. Eng. 2017, 53, 183. [Google Scholar]
- Rumin, R.; Bergander, M.; Cieslik, J.; Kulpa, M. Extended Active Vibration Control for Wind Turbines. In Proceedings of the 2017 XIIIth International Conference on Perspective Technologies and Methods in Mems Design (MEMSTECH), Lviv, Ukraine, 20–23 April 2017; pp. 38–40. [Google Scholar]
- Yun, X.L.; Mei, X.S.; Jiang, G.D.; Hu, Z.B.; Zhang, Z.H. Investigation on a No Trial Weight Spray Online Dynamic Balancer. Shock Vib. 2018, 2018, 7021215. [Google Scholar] [CrossRef]
- Pan, X.; Wu, H.Q.; Gao, J.J. Pneumatic Liquid Transfer Type Active Balance System for Shaft Mounting. CN106090120B, 7 September 2018. [Google Scholar]
- Pan, X.; Wu, H.Q.; Gao, J.J. Control strategy and experiment research on liquid-transfer active balancing device by pneumatic means for grinding machines. J. Vib. Shock 2015, 34, 1–5. [Google Scholar] [CrossRef]





| Cross-Sectional Shape |  |  | 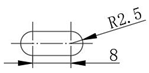 |
|---|---|---|---|
| Equivalent diameter/mm | 5 | 6.69 | 7.52 |
| Cross-sectional area/mm2 | 19.635 | 39.635 | 59.635 |
| Gas drive/MPa | 0.47 | 0.598 | 0.891 |
| Intake Pressure/MPa | 0.4 | 0.6 | 0.8 | |
|---|---|---|---|---|
| Air inlet pipe-1 | 0.1 mm clearance | 0.08 | 0.14 | 0.20 |
| 0.3 mm clearance | 0.04 | 0.06 | 0.08 | |
| Air inlet pipe-2 | 0.1 mm clearance | 0.14 | 0.20 | 0.26 |
| 0.3 mm clearance | 0.11 | 0.18 | 0.24 | |
| Amplitude/μm | No Test Weight | After Fluid Injection | First Air Injection | Second Gas Injection | Third Gas Injection |
|---|---|---|---|---|---|
| 1 × 45° | 15.157 | 22.102 | 19.46 | 15.13 | 15.44 |
| 1 × 135° | 11.185 | 11.425 | 11.15 | 11.38 | 10.77 |
| 2 × 45° | 16.851 | 44.86 | 31.59 | 25.15 | 13.38 |
| 2 × 135° | 5.609 | 16.514 | 7.9 | 8.58 | 9.46 |
| Measurement Points | Initial Vibration/μm | Residual Vibration after Balancing/μm | Range of Discount/100% |
|---|---|---|---|
| 1# Bearing | 14.9 | 14.6 | 2.01 |
| 2# Bearing | 18.3 | 10.6 | 42.08 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pan, X.; Ge, D.; Li, W.; Huo, J. Research on Pneumatic–liquid On-Line Automatic Balance Technology for High-End Turbine Units. Machines 2022, 10, 744. https://doi.org/10.3390/machines10090744
Pan X, Ge D, Li W, Huo J. Research on Pneumatic–liquid On-Line Automatic Balance Technology for High-End Turbine Units. Machines. 2022; 10(9):744. https://doi.org/10.3390/machines10090744
Chicago/Turabian StylePan, Xin, Dehong Ge, Wei Li, and Jiaji Huo. 2022. "Research on Pneumatic–liquid On-Line Automatic Balance Technology for High-End Turbine Units" Machines 10, no. 9: 744. https://doi.org/10.3390/machines10090744
APA StylePan, X., Ge, D., Li, W., & Huo, J. (2022). Research on Pneumatic–liquid On-Line Automatic Balance Technology for High-End Turbine Units. Machines, 10(9), 744. https://doi.org/10.3390/machines10090744






