Analysis of Load Inhomogeneity of Two-Tooth Difference Swing-Rod Movable Teeth Transmission System under External Excitation
Abstract
:1. Introduction
2. Equivalent Calculation Model
3. Dynamic Load Calculation of the Transmission System
3.1. Relative Displacement and Differential Equation of Motion of the Transmission System
3.2. Calculation of Dynamic Load and Load Proportional Coefficient
4. Analysis of the Influence of External Excitation on System Load Inhomogeneity
4.1. Influence of Load Torque on System Load Inhomogeneity
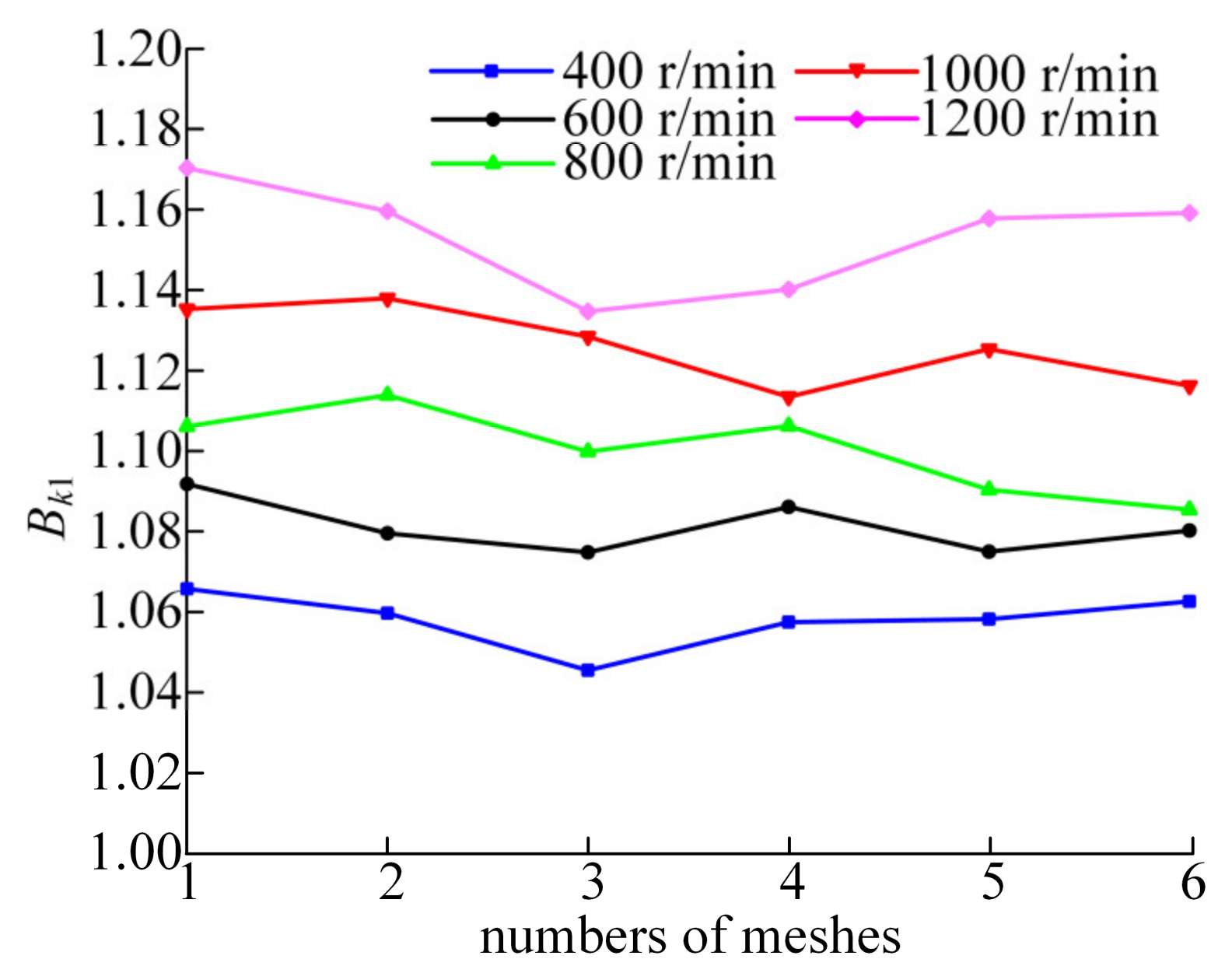
4.2. Influence of Input Speed on System Load Inhomogeneity
5. Dynamic Load Simulation Analysis
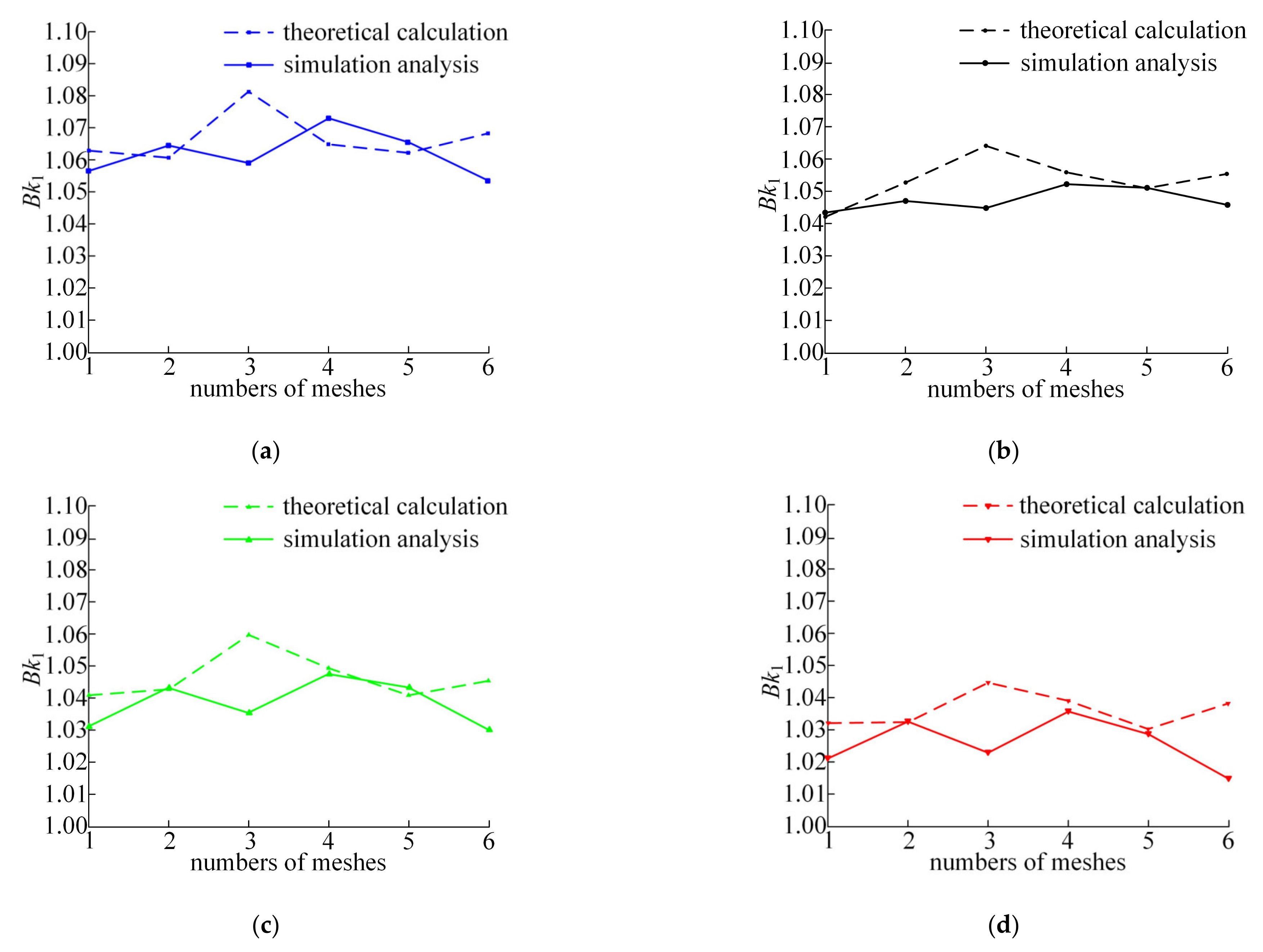
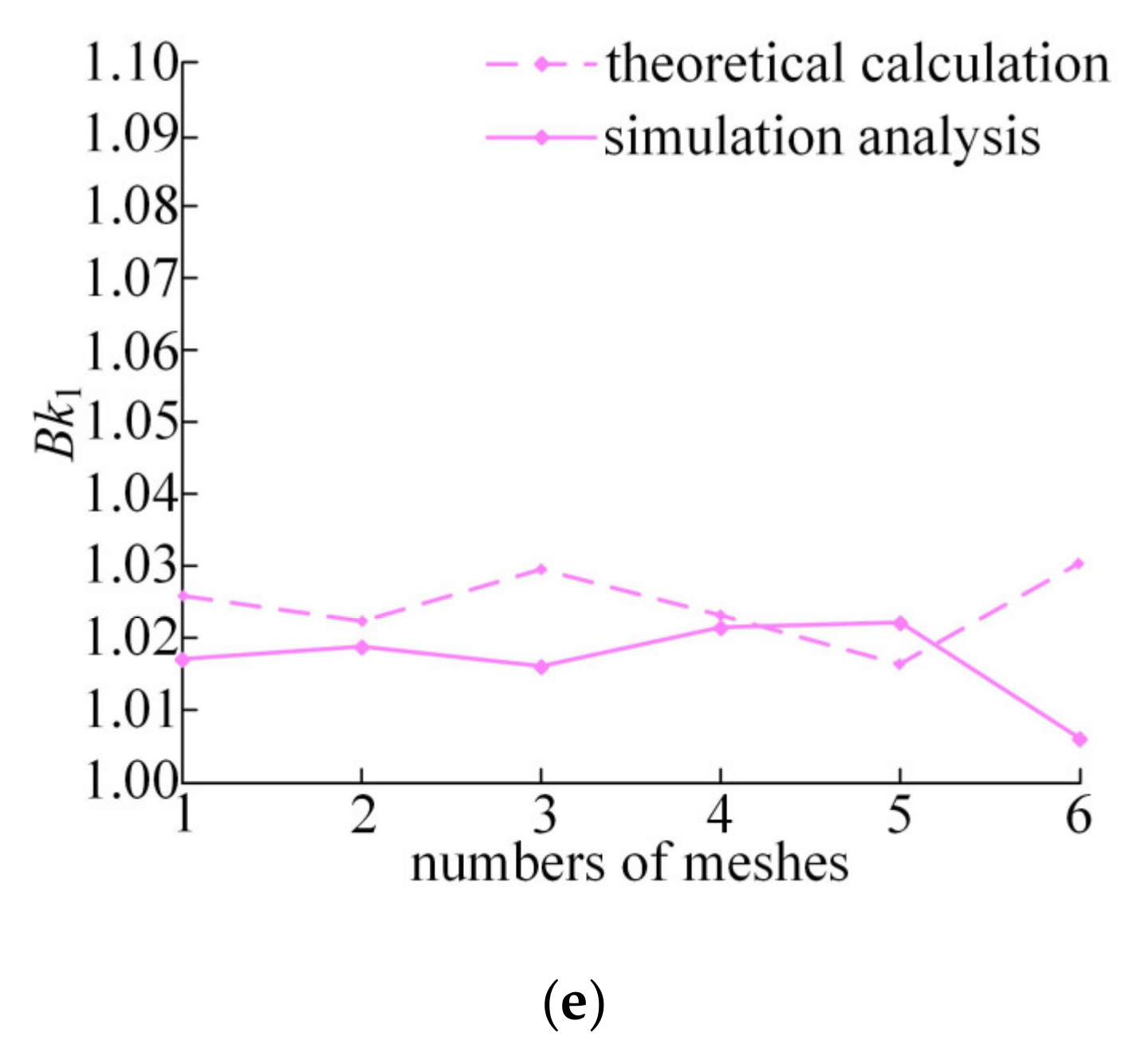
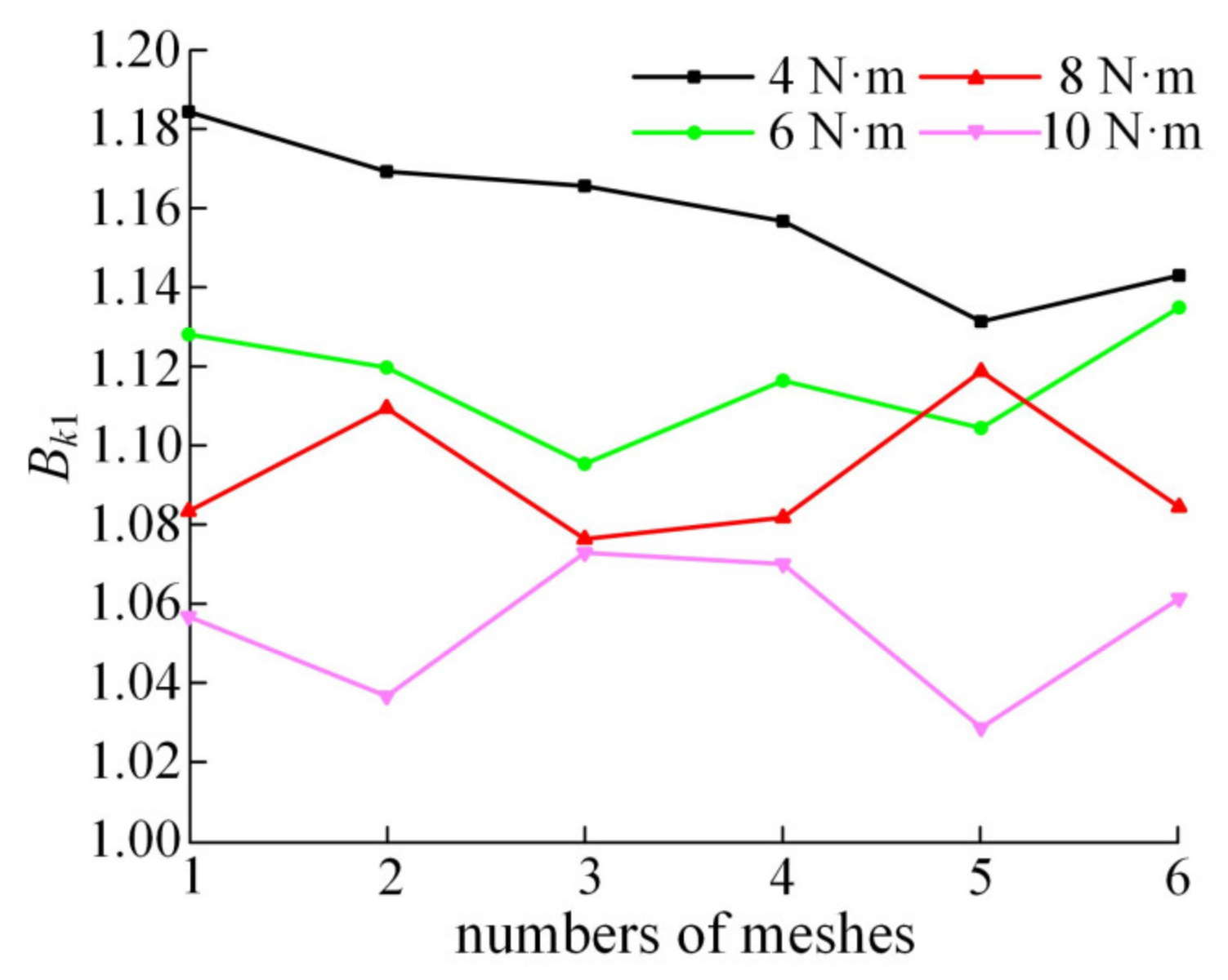
5.1. Simulation Analysis of Load Torque
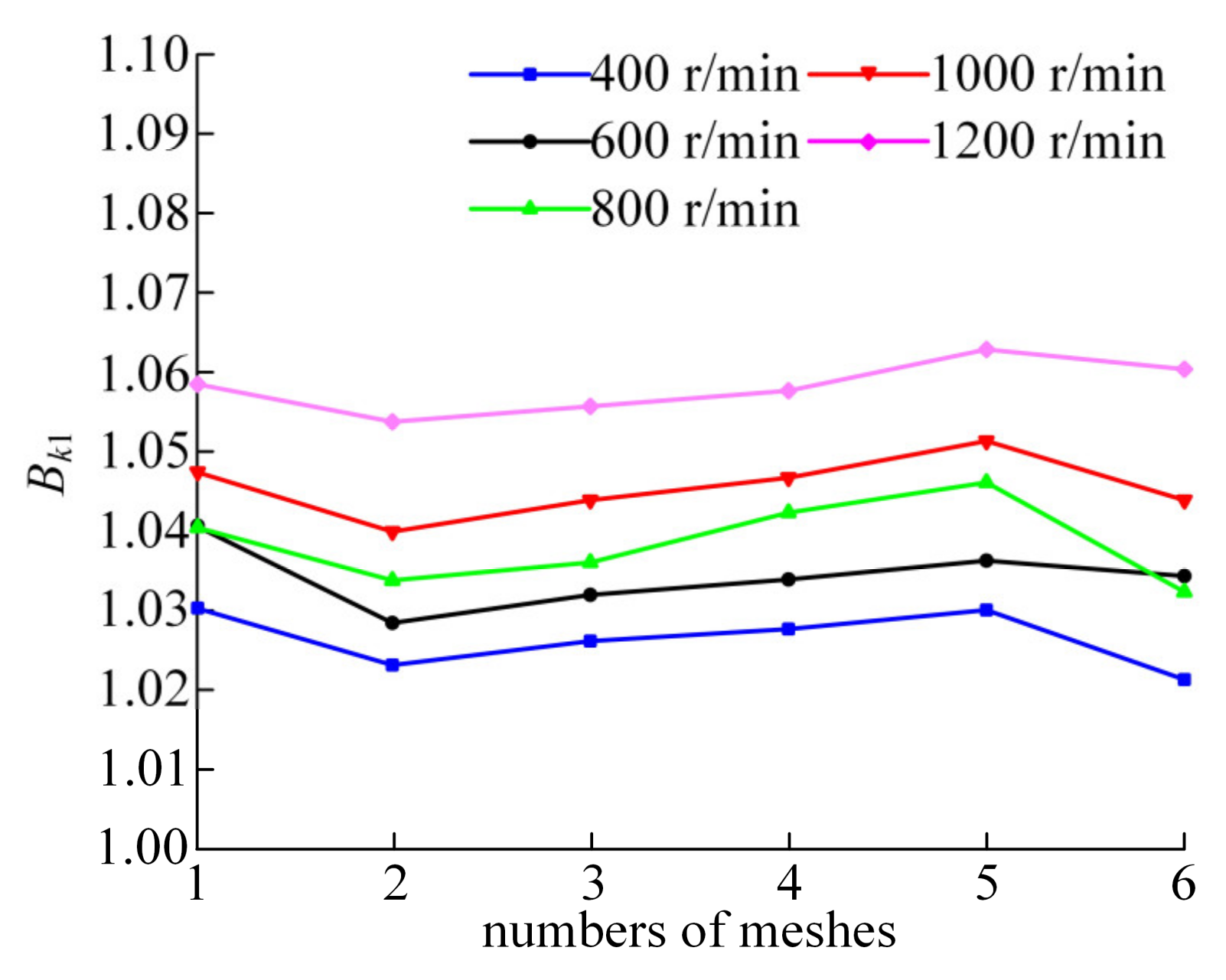
5.2. Simulation Analysis of Input Speed
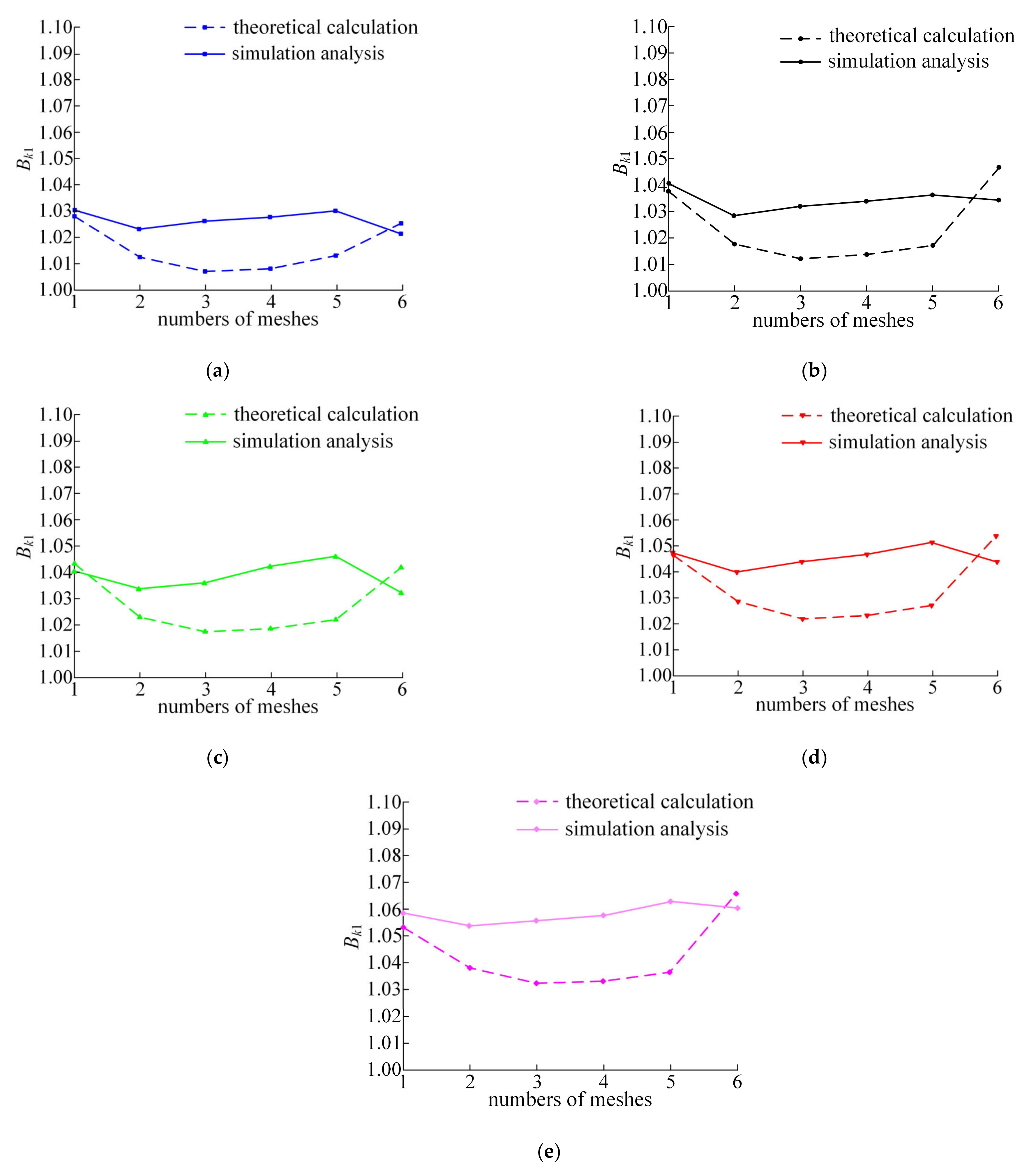
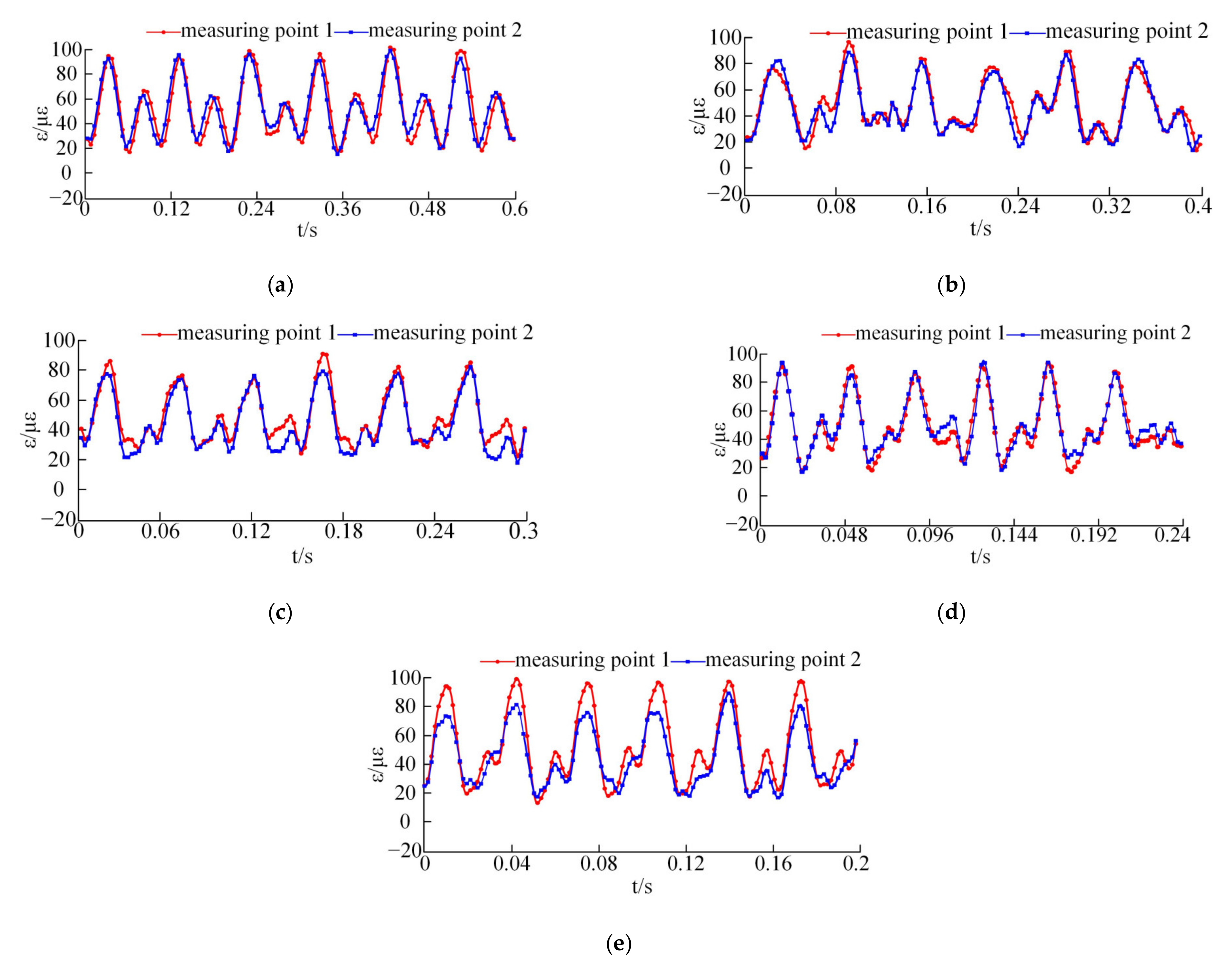
6. Experimental Test and Analysis
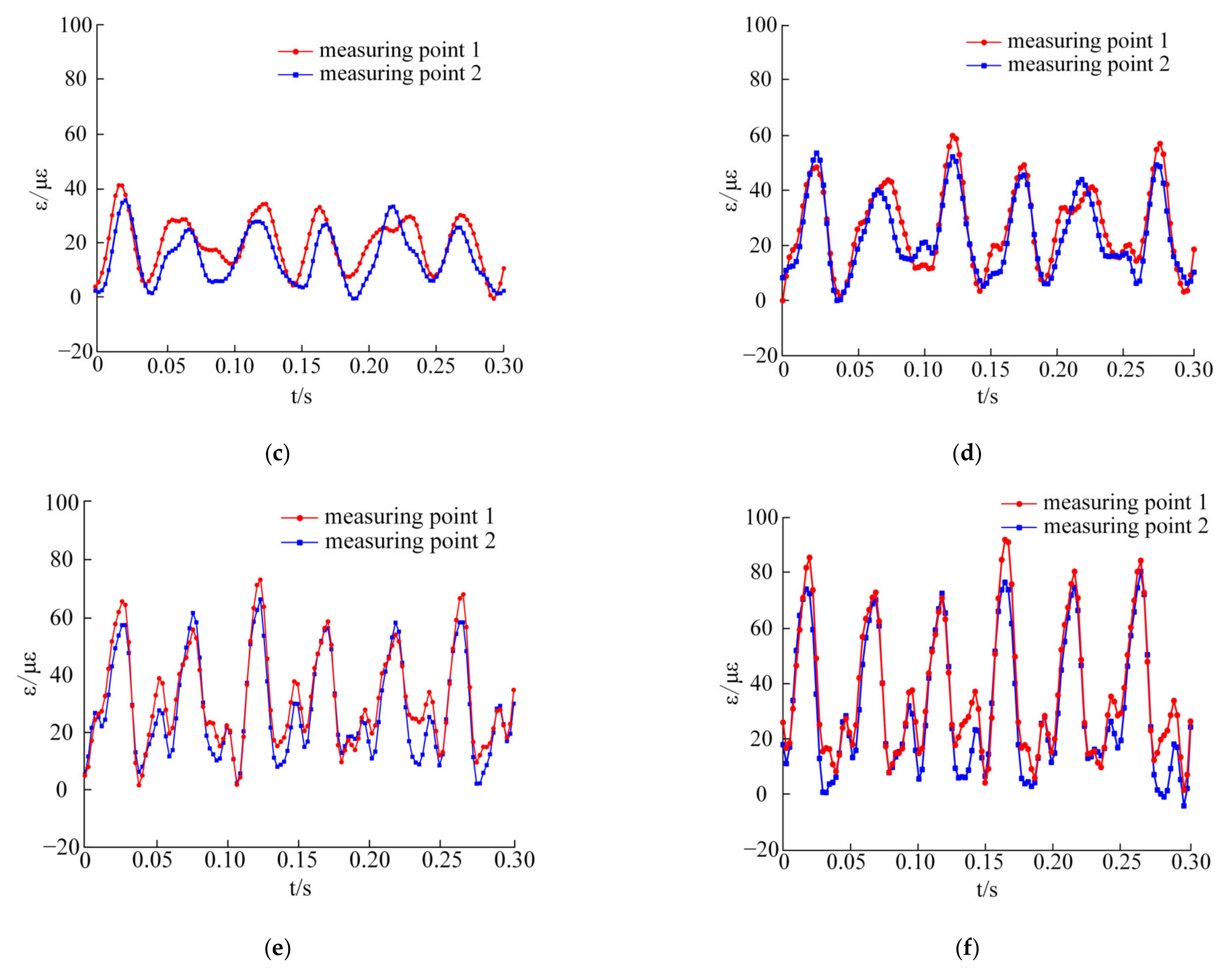
6.1. Influence of Load on Dynamic Load of Meshing Pairs
6.2. Influence of Rotational Speed on Dynamic Load of Meshing Pairs
7. Conclusions
- (1)
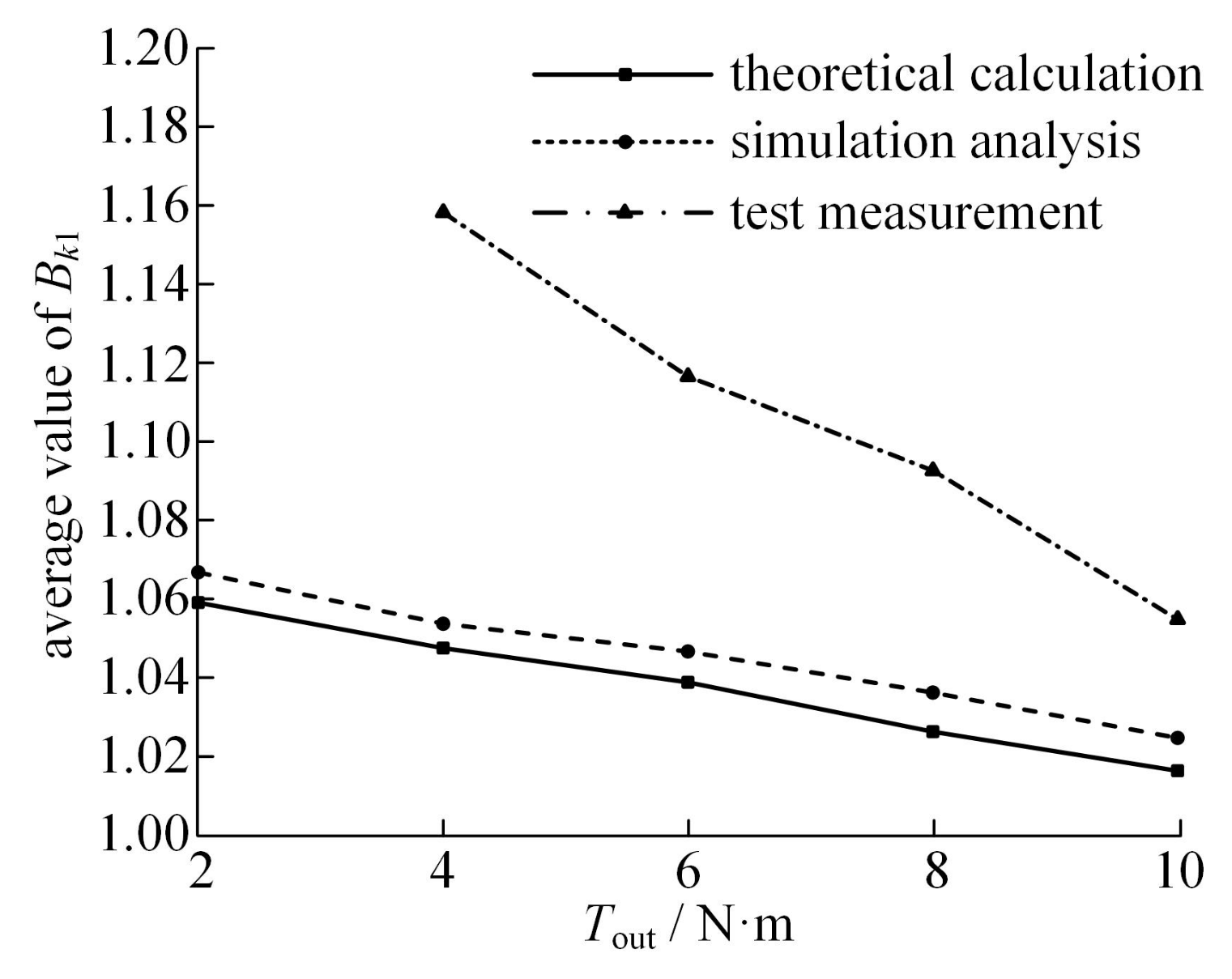
- Under different working conditions, the difference rate between the average value of the load proportional coefficients measured by experiment and that obtained by theoretical calculation and simulation analysis is within 10.7%. In addition, the general variation trend of the average value of the load proportional coefficients obtained from theoretical calculation, simulation analysis, and test measurement are consistent, which verifies the correctness of the theoretical model, the virtual prototype model, and the feasibility of the test method.
- (2)
- The deformation of components can ameliorate the uneven load distribution caused by errors to a certain extent. The increase of the load torque increases the strain amplitude of the meshing pair, thereby reducing the interference of the errors on the uniform distribution of the load, which makes the load distribution more uniform with the increase in load.
- (3)
- The change in input speed has little effect on the maximum value of the strain amplitude. However, the increase in input speed leads to more obvious load difference between the two movable teeth with center symmetry, which makes the system load non-uniformity more serious. This shows that the load uniformity of the two-tooth difference swing-rod movable teeth transmission system is better when working under low speed conditions.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| Notation | Unit | Description |
| kp | N/m | the support stiffness of the part p; p = h, g, k |
| cp | N·s/m | the support damping of the part p; p = h, g, k |
| kpt | N/m | the torsional stiffness of the part p; p = h, g, k |
| cpt | N·s/m | the torsional damping of the part p; p = h, g, k |
| kip | N/m | the meshing stiffness between the ith movable tooth and the part p; p = h, g, k |
| cip | N·s/m | the meshing damping between the ith movable tooth and the part p; p = h, g, k |
| xp | m | the transverse displacement of the part p; p = h, g, k |
| yp | m | the longitudinal displacement of the part p; p = h, g, k |
| up | m | The torsional displacement of the part p; p = h, g, k |
| xi | m | the transverse displacement of the ith movable tooth |
| yi | m | the longitudinal displacement of the ith movable tooth |
| ui | m | the torsional displacement of the ith movable tooth |
| bh | m | The support clearance of the wave generator |
| bih | m | half of the meshing gap between the ith movable tooth and the wave generator |
| bik | m | half of the meshing gap between the ith movable tooth and the ring gear |
| M | the meshing point of the ith movable tooth and the ring gear | |
| N | the meshing point of the ith movable tooth and the wave generator | |
| rad | the angle between the normal line at the meshing point N relative to the fixed horizontal axis XK | |
| rad | the angle between the normal line at the meshing point M relative to the fixed horizontal axis XK | |
| rad | the angle between the horizontal axis XK and the line connecting the coordinate origin O and the movable tooth center O2 | |
| rad | the angle between the length direction of the swing rod and the line connecting the coordinate origin O and the swing rod rotation center O1 | |
| rad | the angle between the length direction of the swing rod and the line connecting the movable tooth center O2 and the coordinate origin O | |
| Δih | m | the relative displacement of the wave generator to the ith movable tooth in the normal direction of their meshing point N |
| Δik | m | the relative displacement of the ring gear to the ith movable tooth in the normal direction of their meshing point M |
| Δig | m | the relative displacement of the separator to the ith movable tooth in the length direction of the swing rod |
| eih | m | the equivalent error of the ith movable tooth meshing with the wave generator |
| eik | m | the equivalent error of the ith movable tooth meshing with the ring gear |
| mp | kg | the mass of the part p; p = h, g, k |
| Ip | kg·m2 | the moment of inertia of the part p; p = h, g, k |
| rp | m | the equivalent radius of the part p; p = h, g, k |
| Tin | N·m | the input torque of the wave generator |
| Tout | N·m | the output torque of the separator |
| mi | kg | the mass of the ith movable tooth |
| Ii | kg·m2 | the moment of inertia of the ith movable tooth |
| ri | m | the equivalent radius of the ith movable tooth |
| Fki | N | the dynamic load between the ith movable tooth and the ring gear |
| the load proportional coefficient when the ith movable tooth meshes with the ring gear | ||
| Bki | the load proportional coefficient of the ith movable tooth with the ring gear in the meshing period | |
| r/min | the input speed of the wave generator | |
| r/min | the output speed of the separator |
References
- Wang, Y.F.; Zhang, Q.P. Study on Virtual Prototype Modeling of Swing Movable Teeth Transmission. Appl. Mech. Mater. 2014, 607, 325–328. [Google Scholar] [CrossRef]
- Qu, J.F. Mechanism Innovation Principle; Science Press: Beijing, China, 2001; pp. 1–20. [Google Scholar]
- Qin, B.; Yin, H.; Wang, Z.; Zhang, J.Q.; Li, Z.J.; Wang, J.G. Application of EMPE and KP-KELM in Fault Diagnosis of Planetary Gearbox. J. Mech. Transm. 2019, 43, 146–151. [Google Scholar]
- Dong, X.R.; Li, J.F.; Wang, X.H.; Liu, Z.F. Structural and Tooth Profile Analysis on Cam Profile Compound Teeth Transmission. China Mech. Eng. 2006, 16, 1661–1665. [Google Scholar]
- Wei, R.; Jin, H.R.; Yi, Y.L. Research on the transmission error of swing-rod movable teeth transmission system. Mech. Ind. 2020, 21, 409. [Google Scholar] [CrossRef]
- Liang, S.M.; Zhang, J.F.; Xu, L.J. Study on elasto-dynamic model of swing movable teeth transmission system. J. Mech. Eng. 2002, 38, 142–146. [Google Scholar] [CrossRef]
- Yang, R.G.; An, Z.J.; Duan, L.Y. Analysis of Free Vibration of Cycloid Ball Planetary Transmission. China Mech. Eng. 2016, 27, 1883–1891. [Google Scholar]
- Yi, Y.L.; Gao, Y.F.; He, L.; Jin, H.R. Thermoelastic stress-strain analysis of the meshing pairs of a two-tooth difference swing-rod movable toothed drive. Strength Mater. 2019, 51, 633–645. [Google Scholar] [CrossRef]
- Xu, L.Z.; Wang, W.P. Quasi-static analysis of forces and stress for a novel two-step movable tooth drive. Mech. Des. Struct. Mach. 2018, 46, 285–295. [Google Scholar] [CrossRef]
- Dong, J.X.; Wang, Q.B.; Tang, J.Y.; Hu, Z.H.; Li, X.Q. Dynamic characteristics and load-sharing performance of concentric face gear split-torque transmission systems with time-varying mesh stiffness, flexible supports and deformable shafts. Meccanica 2021, 56, 2893–2918. [Google Scholar] [CrossRef]
- Dong, H.; Zhang, H.Q.; Zhao, X.L.; Duan, L.L. Study on the load-sharing characteristics of face-gear four-branching split-torque transmission system. Adv. Mech. Eng. 2021, 13, 1–15. [Google Scholar]
- Mo, S.; Yue, Z.X.; Feng, Z.Y.; Gao, H.J. Analytical investigation on load sharing characteristics for face gear split flow system. J. Huazhong Univ. Sci. Technol. 2020, 48, 23–28. [Google Scholar] [CrossRef]
- Hu, S.Y.; Fang, Z.D.; Xu, Y.Q.; Guan, Y.B.; Shen, R. Meshing impact analysis of planetary transmission system considering the influence of multiple errors and its effect on the load sharing and dynamic load factor characteristics of the system. J. Multi-Body Dyn. 2021, 235, 57–74. [Google Scholar] [CrossRef]
- Xu, X.Y.; Yang, W.; Diao, P.; Tao, Y.C. Research of the Dynamic Load Sharing of Heavy Load Planetary Gear System with Multi-floating Component. J. Mech. Transm. 2016, 40, 6–11. [Google Scholar]
- Zhang, H.B.; Wu, S.J.; Peng, Z.M. A nonlinear dynamic model for analysis of the combined influences of nonlinear internal excitations on the load sharing behavior of a compound planetary gear set. J. Mech. Eng. Sci. 2016, 230, 1048–1068. [Google Scholar] [CrossRef]
- Zhang, H.B.; Shen, X.F. A dynamic tooth wear prediction model for reflecting “two-sides” coupling relation between tooth wear accumulation and load sharing behavior in compound planetary gear set. J. Mech. Eng. Sci. 2020, 234, 1746–1763. [Google Scholar] [CrossRef]
- Bodas, A.; Kahraman, A. Influence of Carrier and Gear Manufacturing Errors on the Static Load Sharing Behavior of Planetary Gear Sets. JSME Int. J. Ser. C 2004, 47, 908–915. [Google Scholar] [CrossRef] [Green Version]
- Sanchez-Espiga, J.; Fernandez-del-Rincon, A.; Iglesias, M.; Viadero, F. Planetary gear transmissions load sharing measurement from tooth root strains: Numerical evaluation of mesh phasing influence. Mech. Mach. Theory 2021, 163, 104370. [Google Scholar] [CrossRef]
- Sun, W.; Li, X.; Wei, J.; Zhang, A.Q.; Ding, X.; Hu, X.L. A study on load-sharing structure of multi-stage planetary transmission system. J. Mech. Sci. Technol. 2015, 29, 1501–1511. [Google Scholar] [CrossRef]
- Wang, Y.F.; Yang, B. Study on Dynamic Simulation of Swing Movable Teeth Reducer. Appl. Mech. Mater. 2014, 496–500, 749–753. [Google Scholar] [CrossRef]
- Yi, Y.L.; Guo, H.; Wei, R.; Jin, H.R. Transmission error analysis of swing-rod movable teeth drive based on functionary line increment means. Manuf. Technol. Mach. Tool 2018, 8, 64–70. [Google Scholar]
- Yi, Y.L.; Dou, L.R.; Guo, H.; Jin, H.R. Coupling Stiffness of Double Outer Generator Swing Rod Movable Teeth Transmission. China Mech. Eng. 2018, 29, 644–649. [Google Scholar]
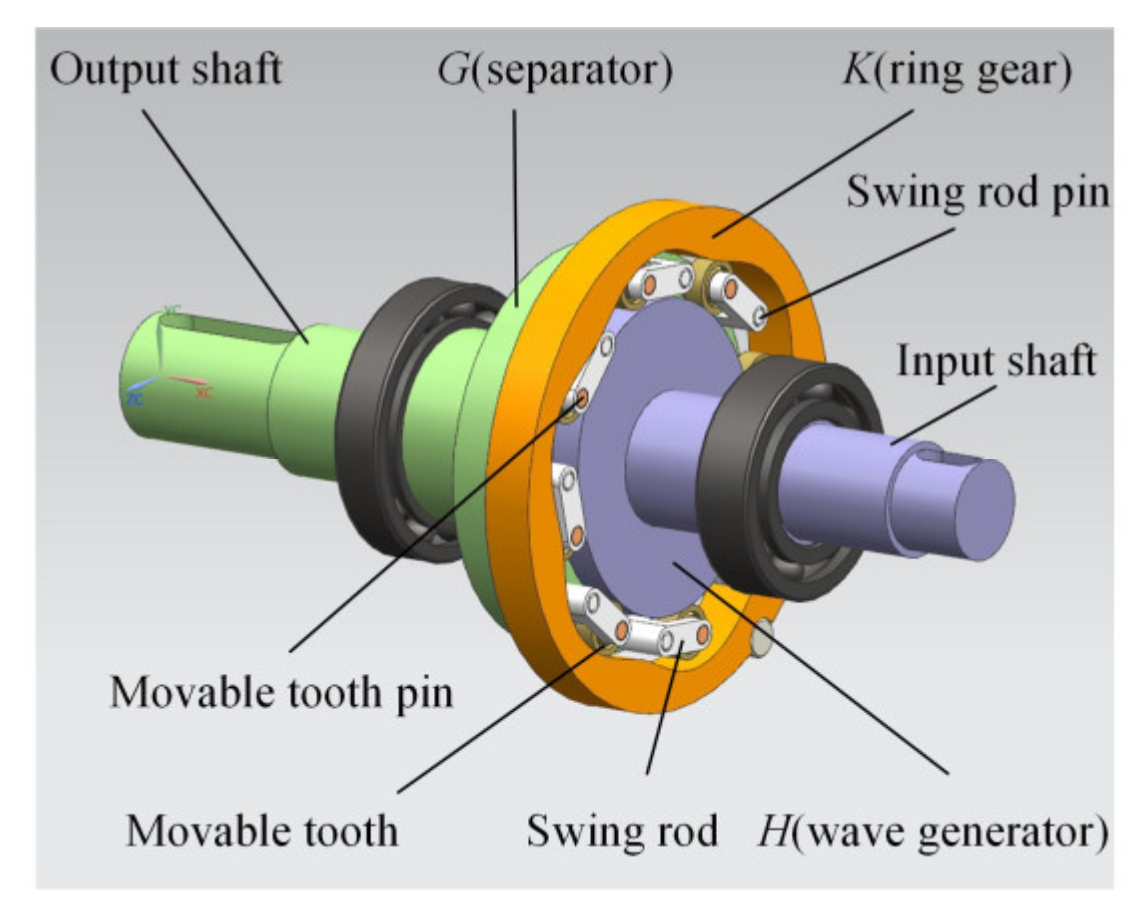
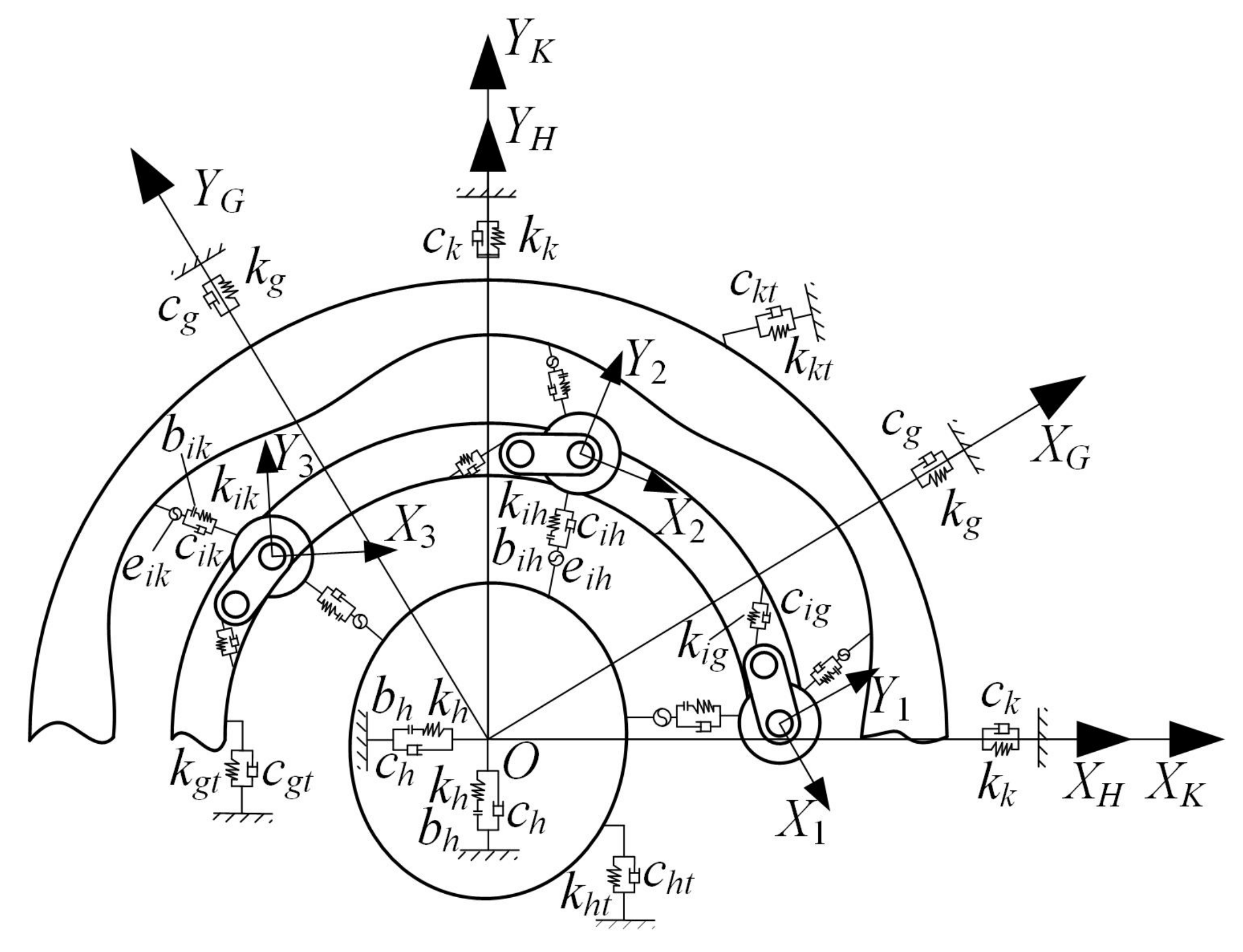

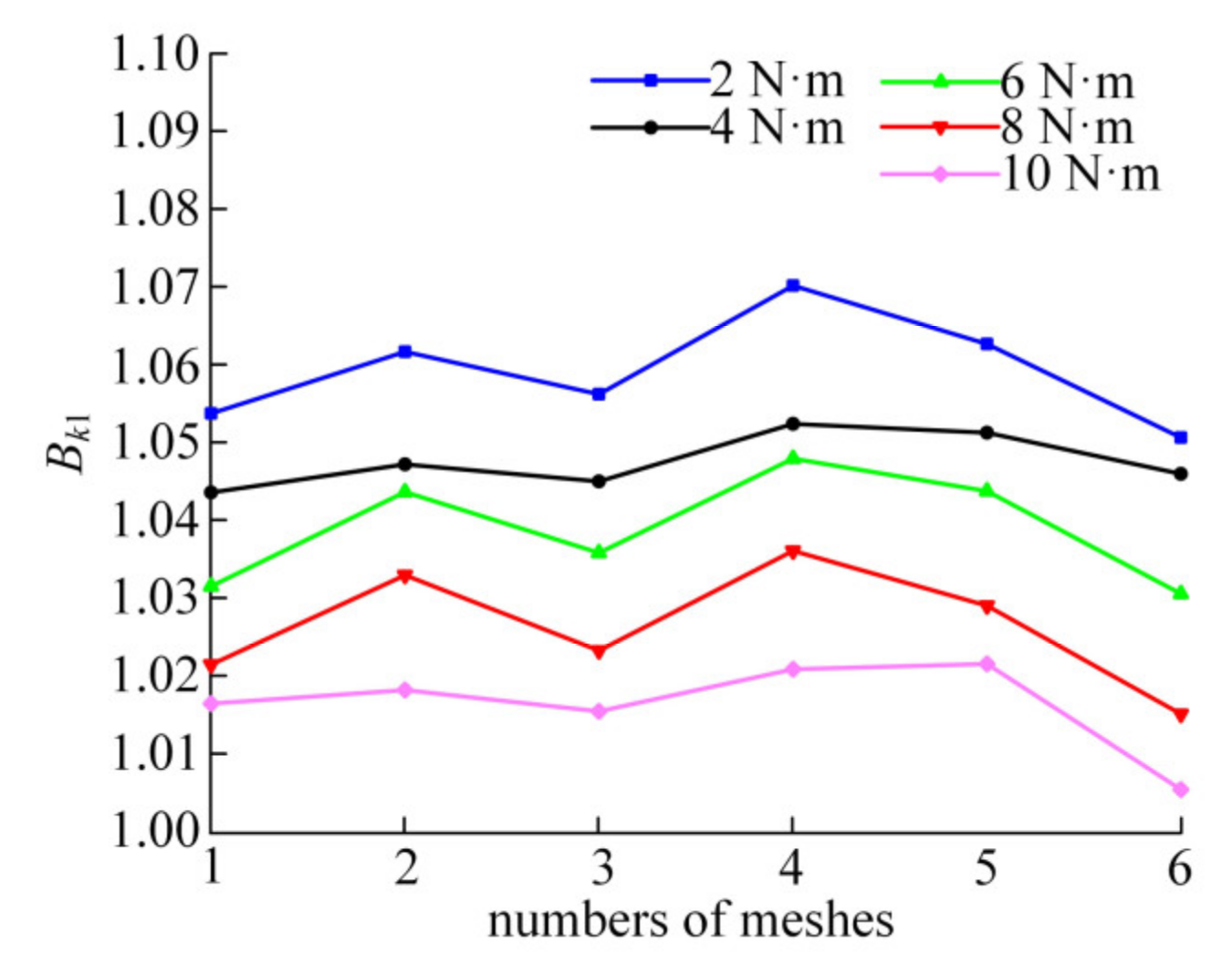
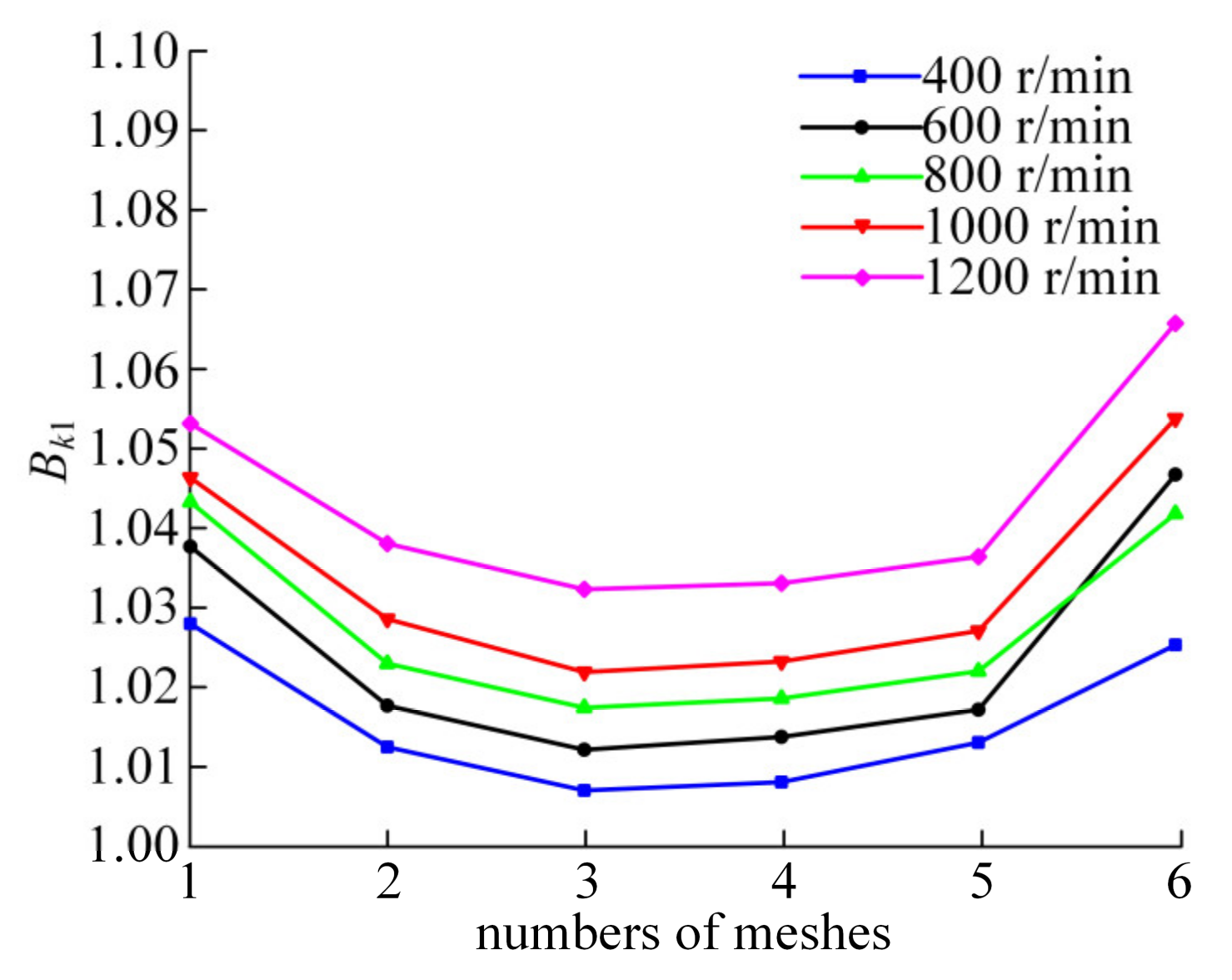



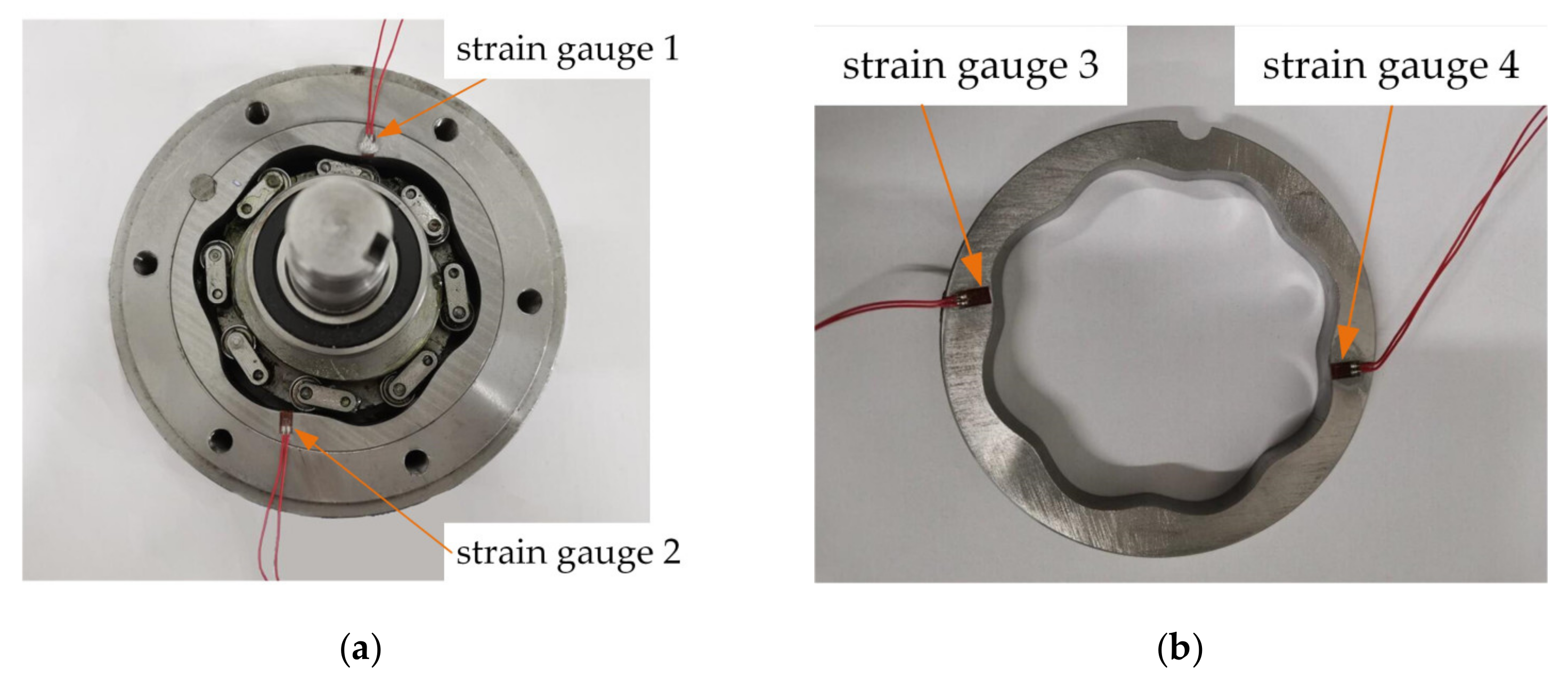
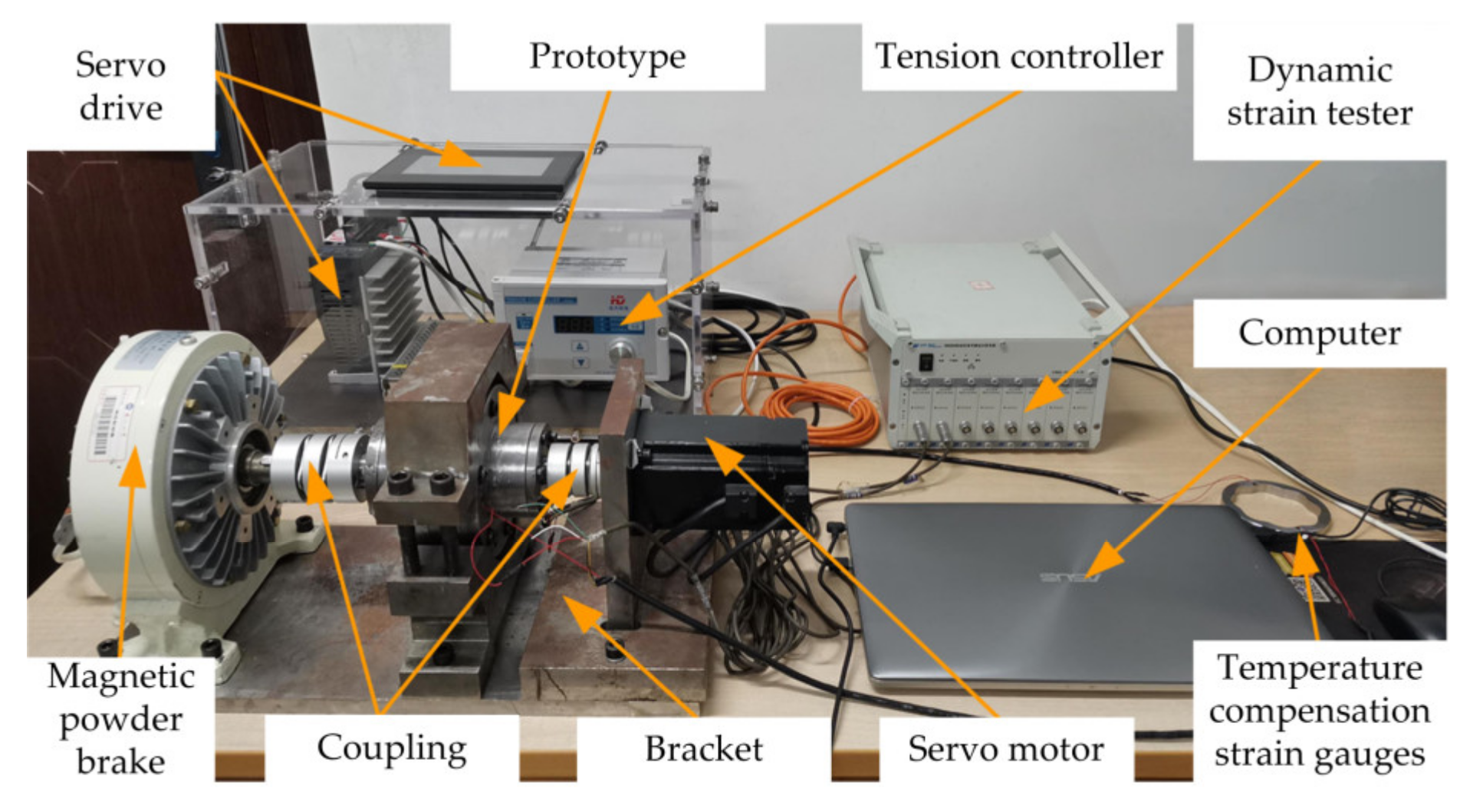


| Name of Component | Number of Teeth | Quality (kg) | Moment of Inertia (kg·m2) |
|---|---|---|---|
| H (wave generator) | 2 | 0.4258 | 8.525 × 10−5 |
| K (ring gear) | 6 | 0.1832 | 3.868 × 10−4 |
| G (separator) | 0.9871 | 4.420 × 10−4 | |
| movable tooth | 8 | 0.0103 | 1.193 × 10−5 |
| Parts | Reference | Constraint Type |
|---|---|---|
| ring gear | the earth | fixed pair |
| wave generator | the earth | revolute pair |
| separator | the earth | revolute pair |
| swing rod | separator | revolute pair |
| movable tooth | swing rod | fixed pair |
| movable tooth | wave generator | contact pair |
| movable tooth | ring gear | contact pair |
| Load | Theoretical Calculation Value | Simulation Value | Error (%) |
|---|---|---|---|
| 2 N∙m | 1.0592 | 1.0669 | 0.7 |
| 4 N∙m | 1.0477 | 1.0538 | 0.6 |
| 6 N∙m | 1.039 | 1.0468 | 0.8 |
| 8 N∙m | 1.0265 | 1.0364 | 1.0 |
| 10 N∙m | 1.0166 | 1.0249 | 0.8 |
| Input Speed | Theoretical Calculation Value | Simulation Value | Error (%) |
|---|---|---|---|
| 400 r/min | 1.0159 | 1.027 | 1.1 |
| 600 r/min | 1.0244 | 1.0348 | 1.0 |
| 800 r/min | 1.0279 | 1.0389 | 1.1 |
| 1000 r/min | 1.0337 | 1.046 | 1.2 |
| 1200 r/min | 1.0433 | 1.0585 | 1.5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, R.; Yi, Y.; Wu, M.; Chen, M.; Jin, H. Analysis of Load Inhomogeneity of Two-Tooth Difference Swing-Rod Movable Teeth Transmission System under External Excitation. Machines 2022, 10, 502. https://doi.org/10.3390/machines10070502
Wei R, Yi Y, Wu M, Chen M, Jin H. Analysis of Load Inhomogeneity of Two-Tooth Difference Swing-Rod Movable Teeth Transmission System under External Excitation. Machines. 2022; 10(7):502. https://doi.org/10.3390/machines10070502
Chicago/Turabian StyleWei, Rui, Yali Yi, Menglei Wu, Meiyu Chen, and Herong Jin. 2022. "Analysis of Load Inhomogeneity of Two-Tooth Difference Swing-Rod Movable Teeth Transmission System under External Excitation" Machines 10, no. 7: 502. https://doi.org/10.3390/machines10070502
APA StyleWei, R., Yi, Y., Wu, M., Chen, M., & Jin, H. (2022). Analysis of Load Inhomogeneity of Two-Tooth Difference Swing-Rod Movable Teeth Transmission System under External Excitation. Machines, 10(7), 502. https://doi.org/10.3390/machines10070502






