Fractal Model of Contact Thermal Stiffness
Abstract
1. Introduction
2. Materials and Methods
2.1. Fractal Characterization of Surface Morphology
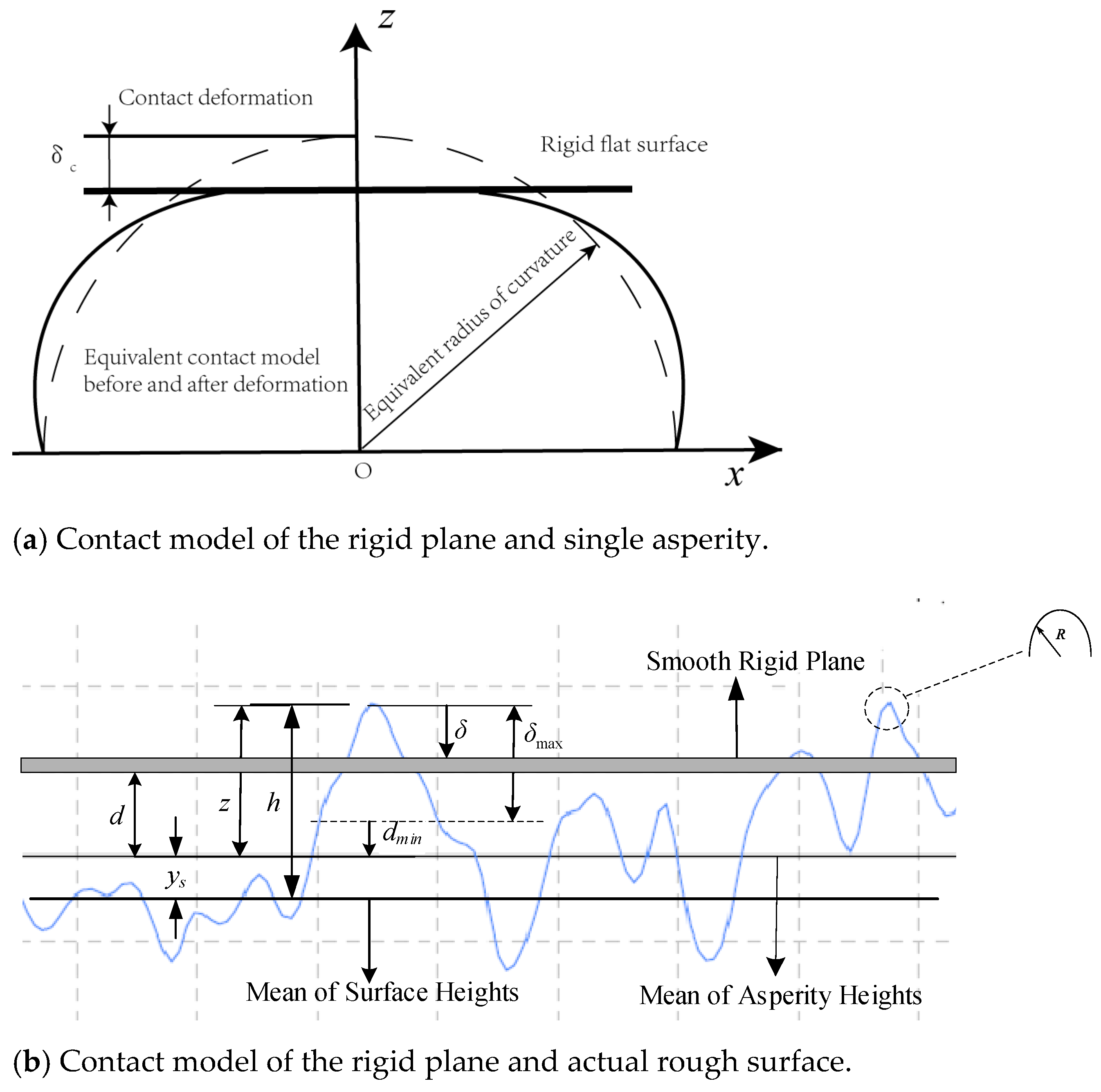
2.2. Fractal Model of Contact Mechanics of Asperity
2.3. Thermal–Mechanical Coupling Contact Interface
2.3.1. Thermal Contact Resistance of Rough Surface Interface
2.3.2. Composite Stiffness
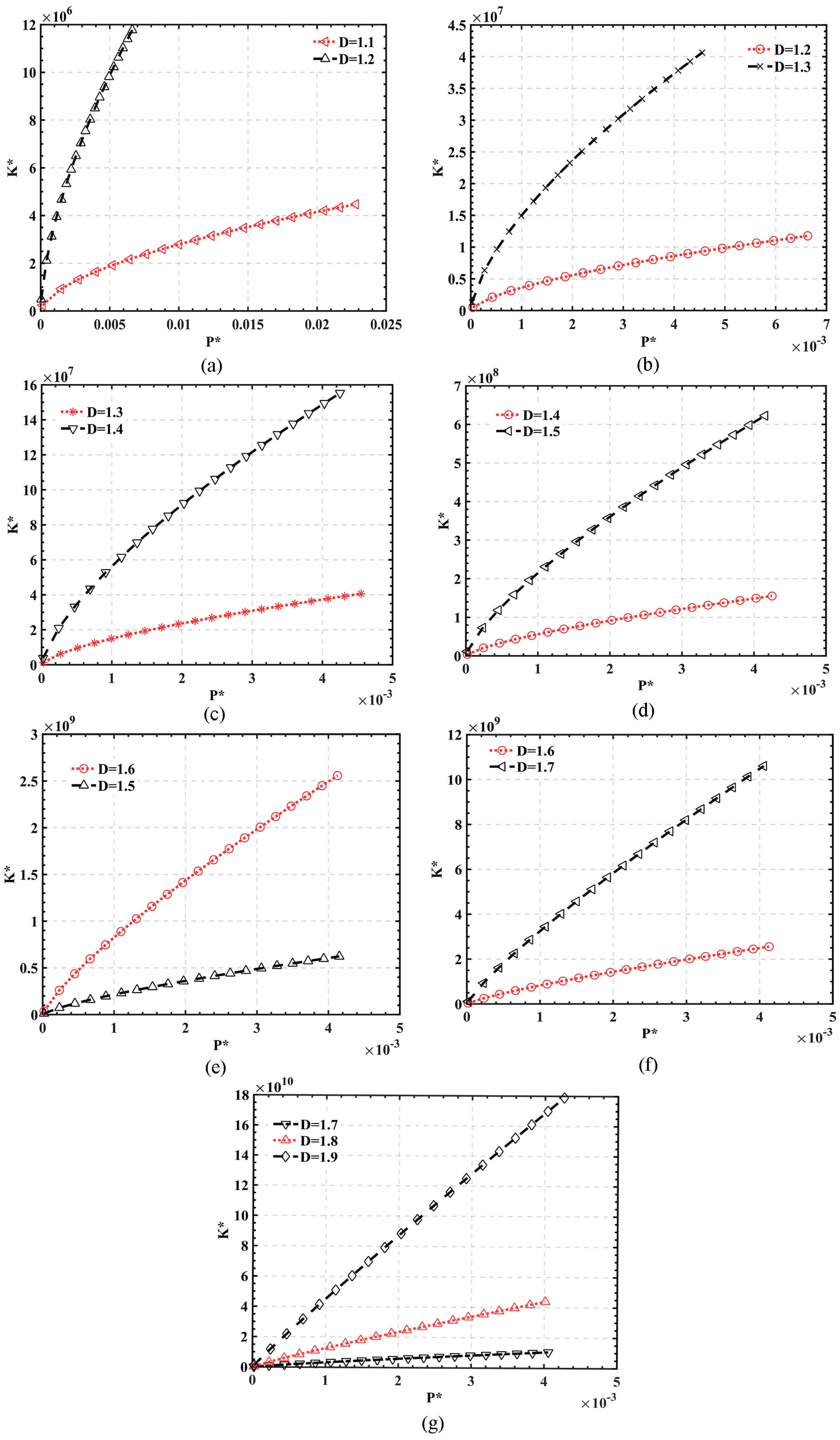
3. Results and Discussion
Numerical Simulation
4. Conclusions
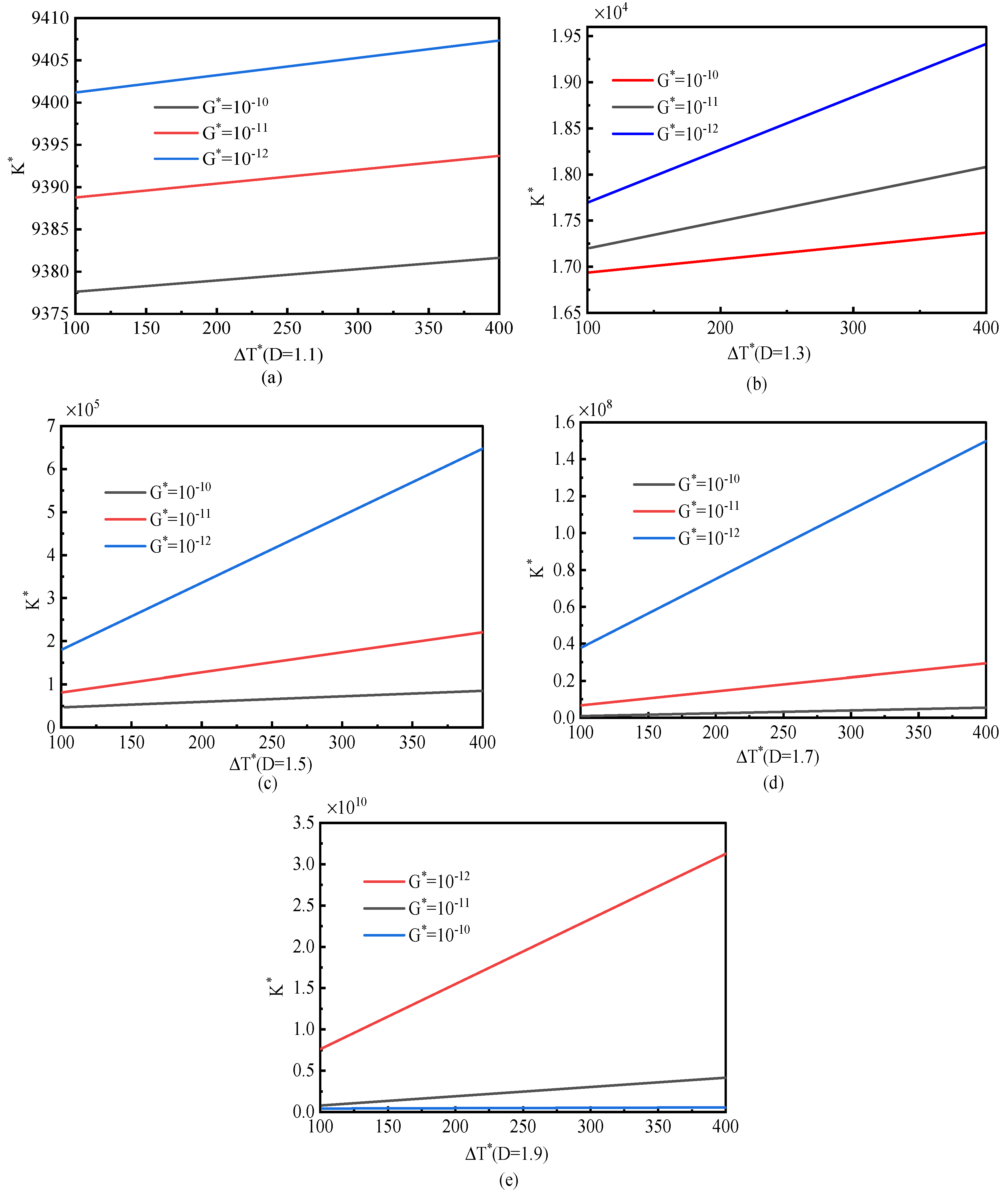
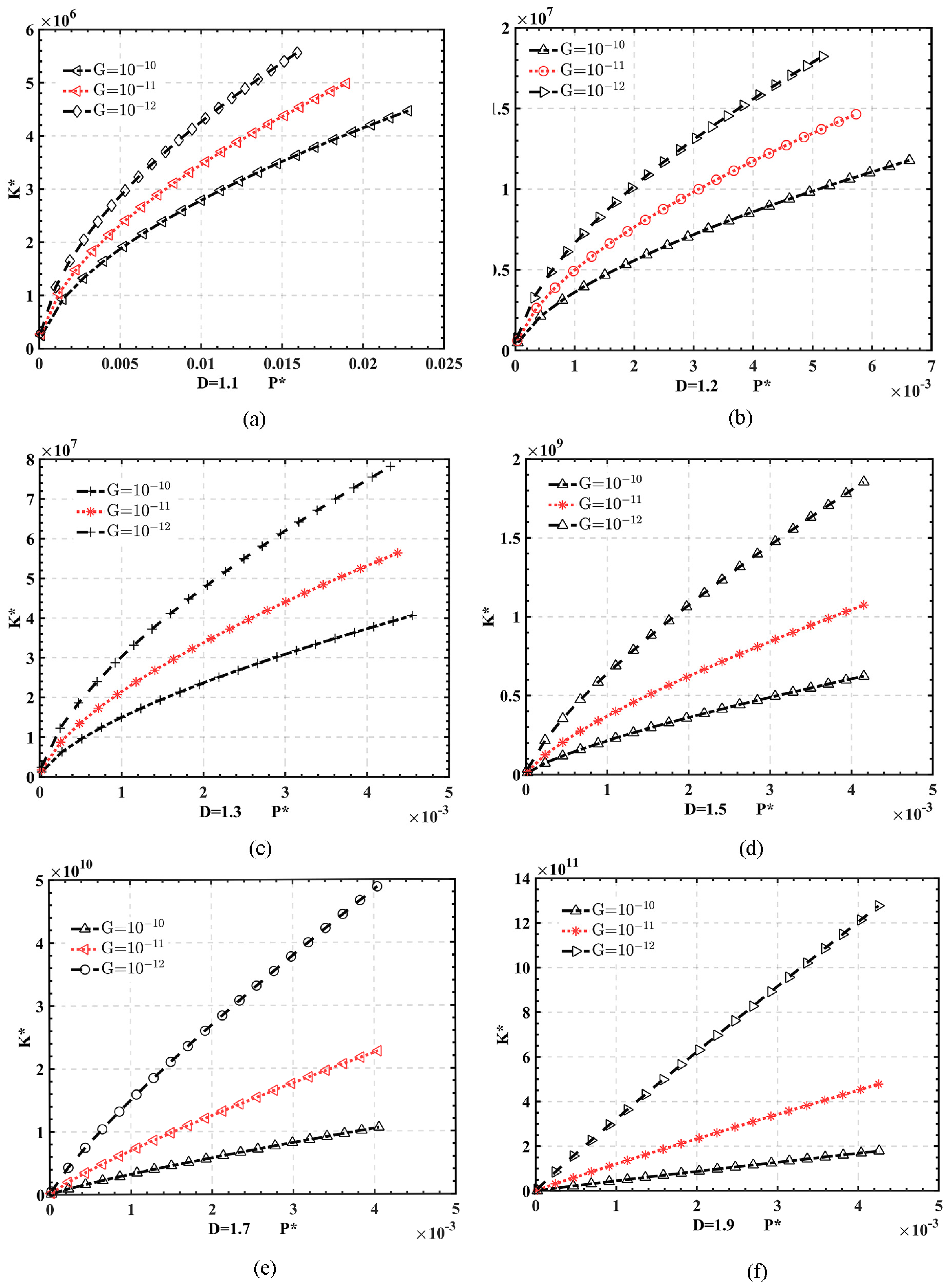
- The change in interface stiffness under the thermal effect increases with temperature, and the linear relationship between the temperature and interface stiffness is apparent. Therefore, a reasonable cooling method must be adopted in the GMT system in the operation process so that the system can work in a reasonable temperature range.
- When , the interface nonlinearity is pronounced. For the same change temperature, K* decreases with the increase in G within a specific range, and this change becomes particularly significant at .
- The proposed interface thermal stiffness contact model can provide theoretical guidance for the study and optimal design of nonlinear dynamic characteristics of ultrasonic vibration systems (e.g., rotating giant magnetostrictive transducers) or other thermal products from the point of view of the contact interface.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| random phase | |
| n | spatial frequency index |
| M | number of overlapping uplifts on the structural surface |
| G | fractal roughness coefficient determined by frequency |
| L | sample length |
| area distribution density of the contact point | |
| N | number of asperities larger than contact area a |
| real contact area of joint surface | |
| peak-to-valley amplitude of the z(x) function | |
| R | curvature radius of the asperity |
| elastic critical deformation | |
| K | the hardness coefficient |
| Poisson’s ratio | |
| critical contact area of asperity | |
| elastic contact load | |
| contact mechanics of elastic–plastic deformation zone 1 | |
| contact mechanics of elastic–plastic deformation zone 2 | |
| contact mechanics of complete plastic deformation | |
| thermal shrinkage resistance at contact point of a single asperity | |
| contact thermal conductance of asperity | |
| contact thermal conductivity of real area of joint surface | |
| normal load caused by thermal stress | |
| normal force of a single asperity containing thermal stress in elastic deformation stage | |
| total contact force containing thermoelastic stress | |
| total contact stiffness of whole joint surface containing thermal stiffness | |
| nominal contact area |
References
- Ma, K.; Wang, J.; Zhang, J.; Feng, P.; Yu, D.; Ahmad, S. A highly temperature-stable and complete-resonance rotary giant magnetostrictive ultrasonic system. Int. J. Mech. Sci. 2022, 214, 106927. [Google Scholar] [CrossRef]
- Zhou, H.; Zhang, J.; Feng, P.; Yu, D.; Wu, Z. An amplitude prediction model for a giant magnetostrictive ultrasonic transducer. Ultrasonics 2020, 108, 106017. [Google Scholar] [CrossRef] [PubMed]
- Zhan, Y.-S.; Lin, C.-H. A Constitutive Model of Coupled Magneto-thermo-mechanical Hysteresis Behavior for Giant Magnetostrictive Materials. Mech. Mater. 2020, 148, 103477. [Google Scholar] [CrossRef]
- Wang, T.-Z.; Zhou, Y.-H. Nonlinear dynamic model with multi-fields coupling effects for giant magnetostrictive actuators. Int. J. Solids Struct. 2013, 50, 2970–2979. [Google Scholar] [CrossRef]
- Xiao, Y.; Gou, X.F.; Zhang, D.G. A one-dimension nonlinear hysteretic constitutive model with elasto-thermo-magnetic coupling for giant magnetostrictive materials. J. Magn. Magn. Mater. 2017, 441, 642–649. [Google Scholar] [CrossRef]
- Zhan, Y.-S.; Lin, C.-H. Micromechanics-based constitutive modeling of magnetostrictive 1–3 and 0–3 composites. Compos. Struct. 2021, 260. [Google Scholar] [CrossRef]
- Li, P.; Liu, Q.; Zhou, X.; Xu, G.; Li, W.; Wang, Q.; Yang, M. Effect of Terfenol-D rod structure on vibration performance of giant magnetostrictive ultrasonic transducer. J. Vib. Control 2020, 27, 573–581. [Google Scholar] [CrossRef]
- Chen, Y.; Zhang, X.; Wen, S.; Lan, G. Fractal Model for Normal Contact Damping of Joint Surface Considering Elastoplastic Phase. J. Mech. Eng. 2019, 55, 58–68. [Google Scholar]
- Kogut, L.; Etsion, I. Elastic-Plastic Contact Analysis of a Sphere and a Rigid Flat. J. Appl. Mech. 2002, 69, 657–662. [Google Scholar] [CrossRef]
- Kogut, L.; Etsion, I. A Finite Element Based Elastic-Plastic Model for the Contact of Rough Surfaces. Tribol. Trans. 2003, 46, 383–390. [Google Scholar] [CrossRef]
- Brake, M.R. An analytical elastic-perfectly plastic contact model. Int. J. Solids Struct. 2012, 49, 3129–3141. [Google Scholar] [CrossRef]
- Xu, C.; Wang, D. An Improved Analytical Model for Normal Elastic-Plastic Contact of Rough Surfaces. J. Xi’an Jiaotong Univ. 2014, 48, 115–121. [Google Scholar]
- Jiang, S.; Zheng, Y.; Zhu, H. A Contact Stiffness Model of Machined Plane Joint Based on Fractal Theory. J. Tribol.-Trans. Asme 2010, 132, 011401. [Google Scholar] [CrossRef]
- Raffa, M.L.; Lebon, F.; Vairo, G. Normal and tangential stiffnesses of rough surfaces in contact via an imperfect interface model. Int. J. Solids Struct. 2016, 87, 245–253. [Google Scholar] [CrossRef]
- Liu, W.; Yang, J.; Xi, N.; Shen, J.; Li, L. A study of normal dynamic parameter models of joint interfaces based on fractal theory. J. Adv. Mech. Des. Syst. Manuf. 2015, 9, JAMDSM0070. [Google Scholar] [CrossRef]
- Liou, J.L.; Tsai, C.M.; Lin, J.-F. A microcontact model developed for sphere- and cylinder-based fractal bodies in contact with a rigid flat surface. Wear 2010, 268, 431–442. [Google Scholar] [CrossRef]
- Liou, J.L.; Lin, J.F. A modified fractal microcontact model developed for asperity heights with variable morphology parameters. Wear 2010, 268, 133–144. [Google Scholar] [CrossRef]
- Wang, Z.; Liu, X. Model for Elastic-Plastic Contact between Rough Surfaces. J. Tribol. 2018, 140, 051402. [Google Scholar] [CrossRef]
- He, L.G.; Zuo, Z.X.; Xiang, J.H. Normal Contact Stiffness Fractal Model Considering Asperity Elastic-Plastic Transitional Deformation Mechanism of Joints. J. Shanghai Jiaotong Univ. 2015, 49, 116–121. [Google Scholar]
- Li, L.; Liang, X.; Xing, Y.Z.; Yan, D.; Wang, G. Measurement of Real Contact Area for Rough Metal Surfaces and the Distinction of Contribution From Elasticity and Plasticity. J. Tribol. 2021, 143, 071501. [Google Scholar] [CrossRef]
- Kogut, L.; Komvopoulos, K. Electrical contact resistance theory for conductive rough surfaces separated by a thin insulating film. J. Appl. Phys. 2004, 95, 576–585. [Google Scholar] [CrossRef]
- Wang, S.; Komvopoulos, K. Closure to “Discussion of ‘A Fractal Theory of the Interfacial Temperature Distribution in the Slow Sliding Regime: Part I—Elastic Contact and Heat Transfer Analysis’” (1994, ASME J. Tribol., 116, p. 822). J. Tribol. 1994, 116, 822–823. [Google Scholar] [CrossRef][Green Version]
- Shi, X.; Wu, A.; Jin, C.; Qu, S. Thermomechanical modeling and transient analysis of sliding contacts between an elastic–plastic asperity and a rigid isothermal flat. Tribol. Int. 2015, 81, 53–60. [Google Scholar] [CrossRef]
- Song, W.; Li, L.; Ovcharenko, A.; Talke, F.E. Thermo-mechanical contact between a rigid sphere and an elastic–plastic sphere. Tribol. Int. 2016, 95, 132–138. [Google Scholar] [CrossRef]
- Horovistiz, A.; Laranjeira, S.; Davim, J.P. 3-D reconstruction by extended depth-of-field in tribological analysis: Fractal approach of sliding surface in Polyamide66 with glass fiber reinforcement. Polym. Test. 2019, 73, 178–185. [Google Scholar] [CrossRef]
- Yan, W.; Komvopoulos, K. Contact analysis of elastic-plastic fractal surfaces. J. Appl. Phys. 1998, 84, 3617–3624. [Google Scholar] [CrossRef]
- Majumdar, A.; Bhushan, B. Fractal Model of Elastic-Plastic Contact Between Rough Surfaces. J. Tribol. 1991, 113, 1–11. [Google Scholar] [CrossRef]
- Cooper, M.G.; Mikic, B.B.; Yovanovich, M.M.; Transfer, M. Thermal Contact Conductance. Int. J. Heat Mass Transf. 1969, 12, 279–300. [Google Scholar] [CrossRef]
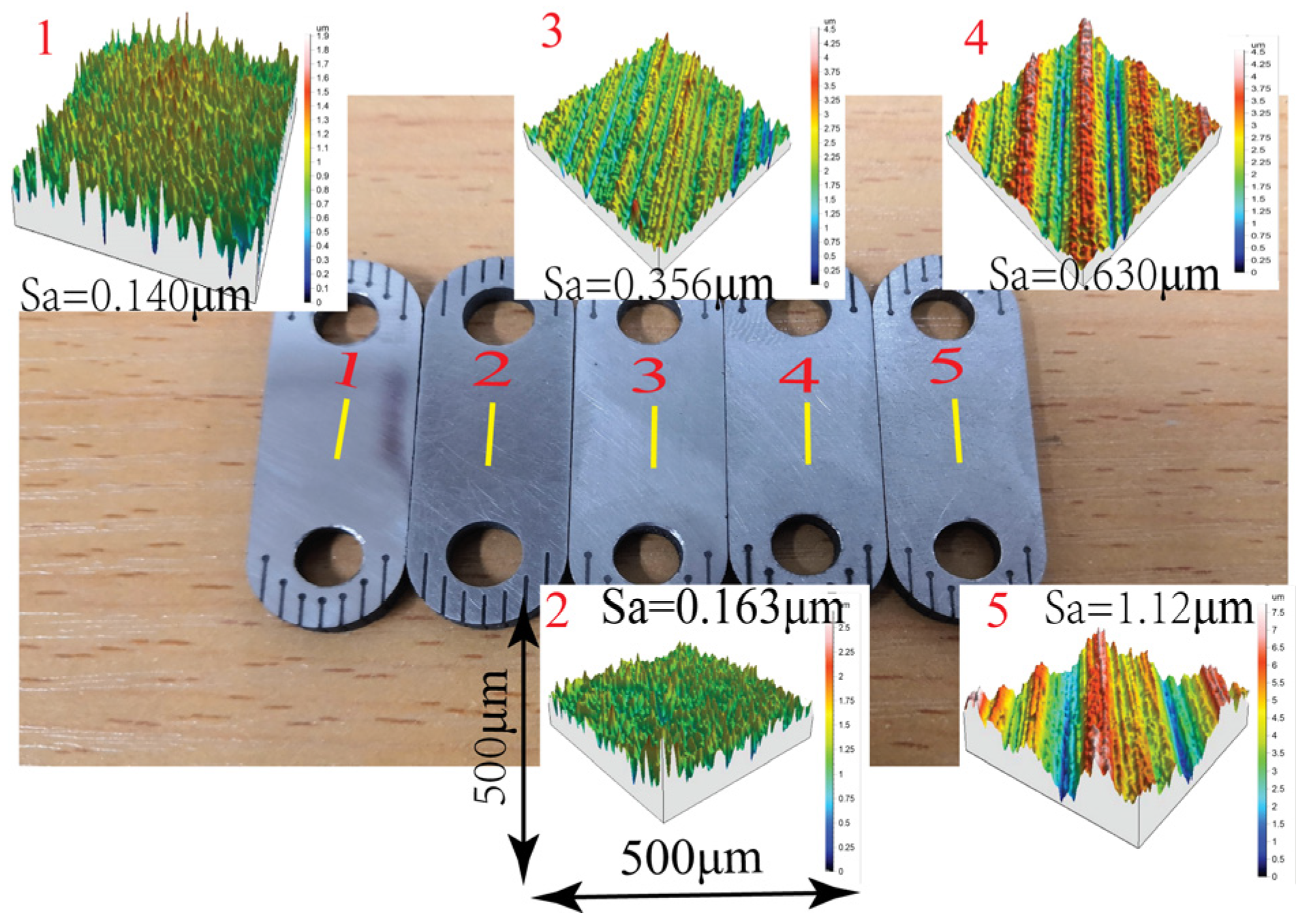

| Experimental Times | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
|---|---|---|---|---|---|---|---|---|
| D | 1.4007 | 1.4061 | 1.4021 | 1.4613 | 1.4026 | 1.4525 | 1.4533 | 1.4060 |
| G (10−10 mm) | 6.5340 | 8.6558 | 9.2133 | 24.005 | 25.682 | 8.9967 | 41.003 | 31.017 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Y.; Li, P.; Sun, J.; Shang, M.; Shao, L. Fractal Model of Contact Thermal Stiffness. Machines 2022, 10, 464. https://doi.org/10.3390/machines10060464
Chen Y, Li P, Sun J, Shang M, Shao L. Fractal Model of Contact Thermal Stiffness. Machines. 2022; 10(6):464. https://doi.org/10.3390/machines10060464
Chicago/Turabian StyleChen, Yunshuai, Pengyang Li, Jian Sun, Miao Shang, and Limin Shao. 2022. "Fractal Model of Contact Thermal Stiffness" Machines 10, no. 6: 464. https://doi.org/10.3390/machines10060464
APA StyleChen, Y., Li, P., Sun, J., Shang, M., & Shao, L. (2022). Fractal Model of Contact Thermal Stiffness. Machines, 10(6), 464. https://doi.org/10.3390/machines10060464






