Orthogonal Experimental Design Based Nozzle Geometry Optimization for the Underwater Abrasive Water Jet
Abstract
1. Introduction
2. Nozzle Geometry and CFD Simulation
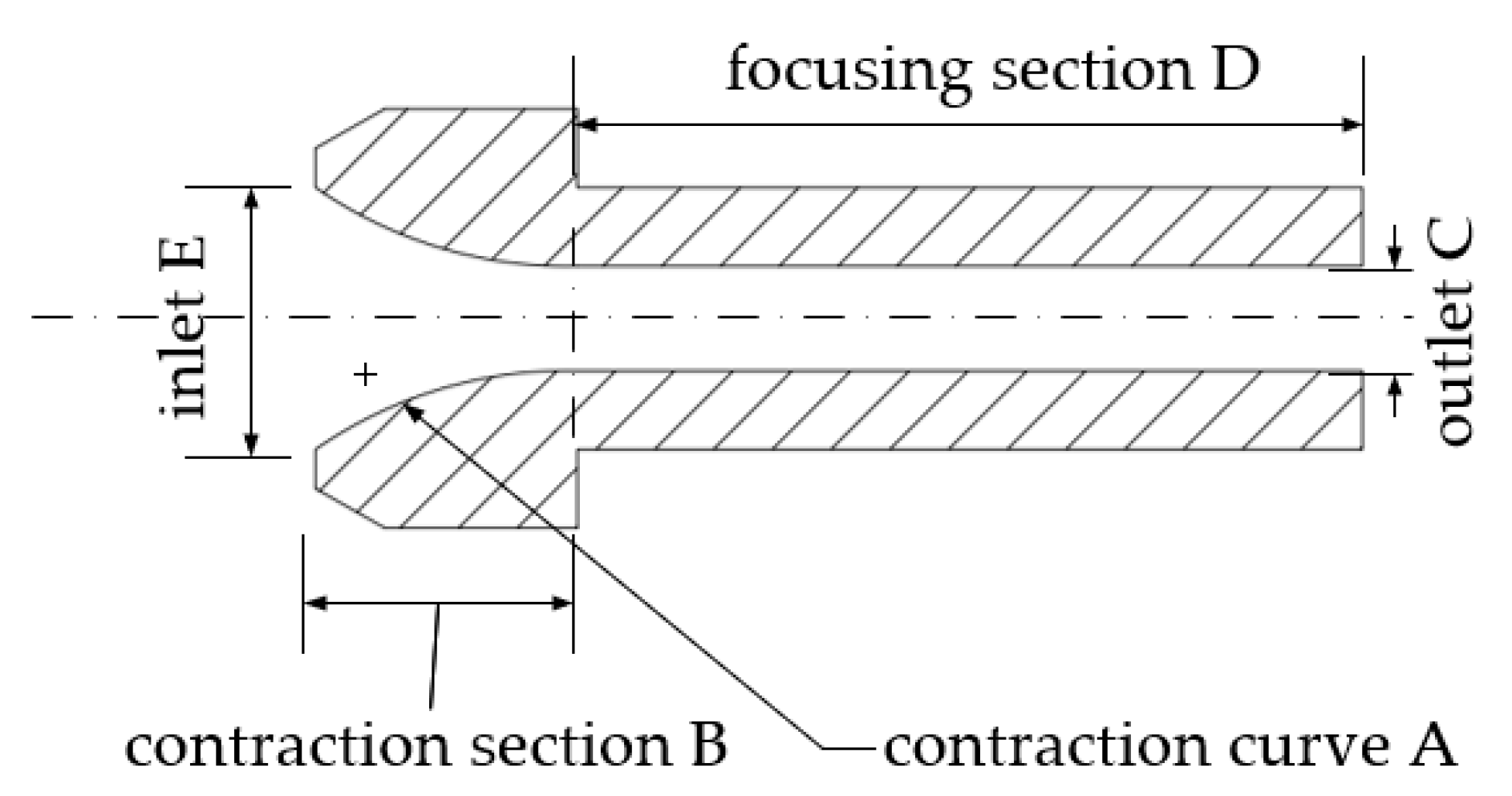
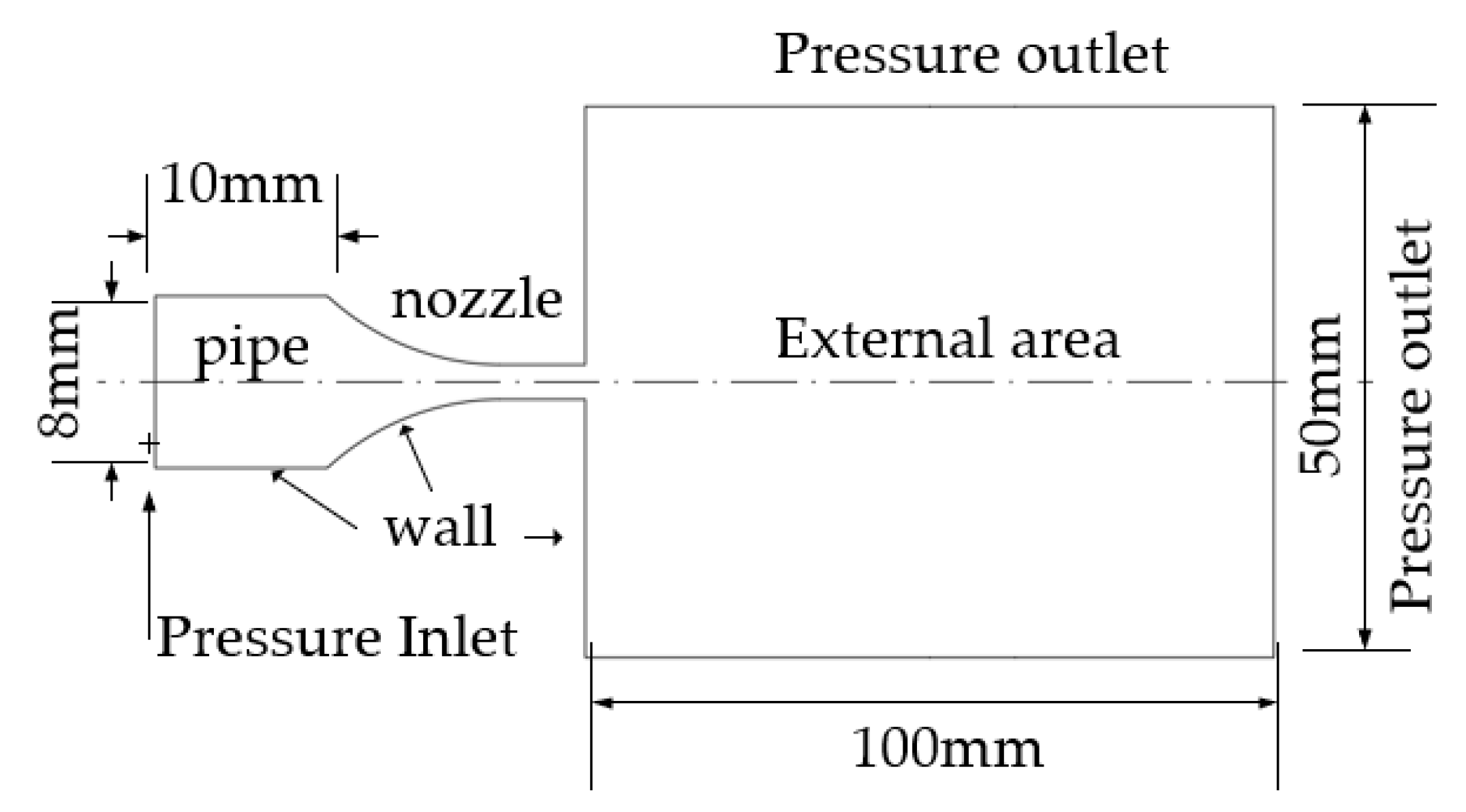
2.1. Geometry Model
- (1)
- Water is of a continuous phase and incompressible.
- (2)
- The abrasive particles are rigid and spherical, with equal diameters, and there is no mass exchange between the particles and the water.
- (3)
- There is no heat exchange between the particles and the water in the flow, and the temperature remains constant.
2.2. Turbulence Model
2.3. Discrete Phase Model
2.4. Erosion Model
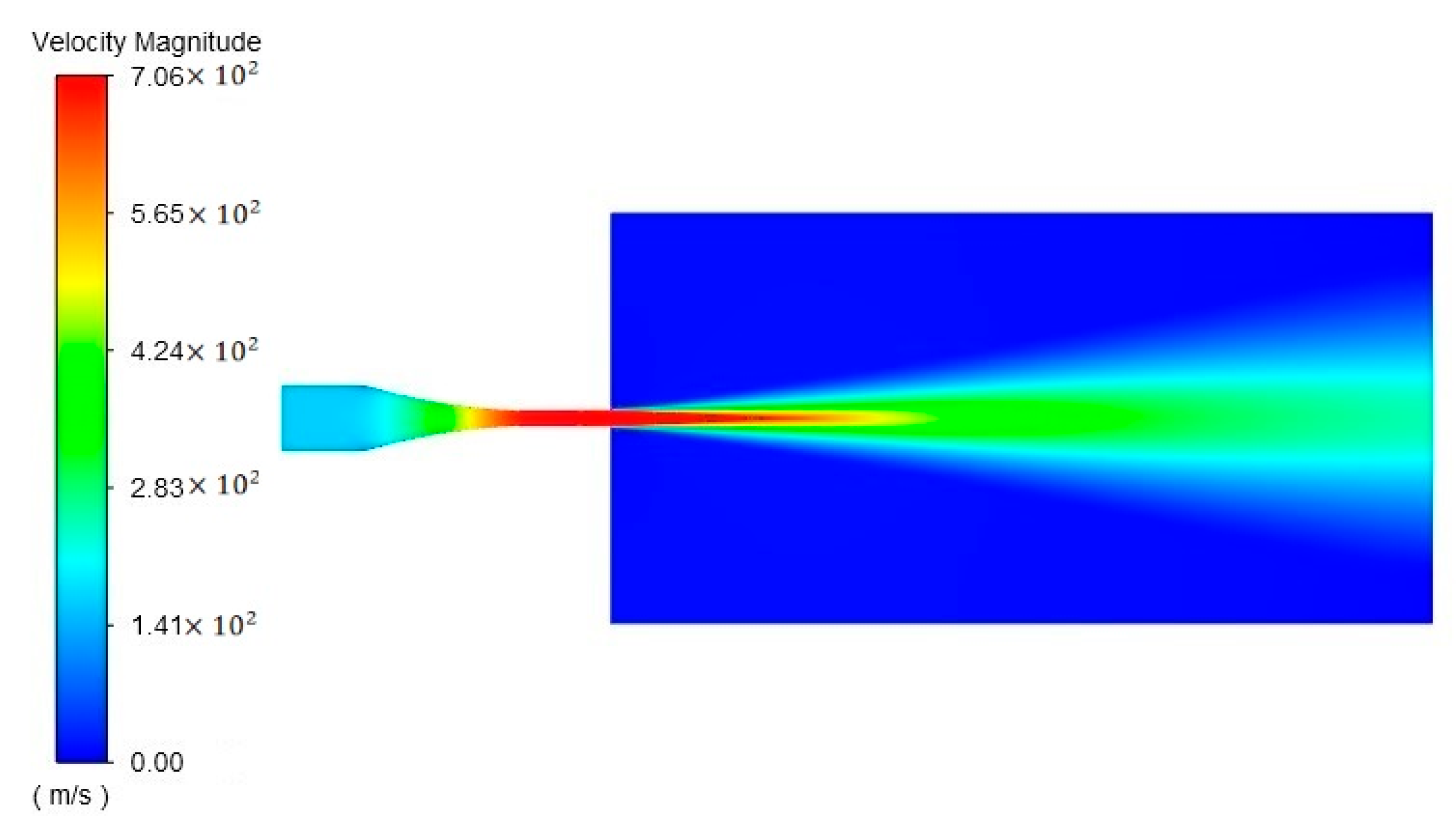
2.5. Mesh and Independency
3. Optimization of Nozzle Geometry
3.1. Geometry Parameters
3.2. Effects of the Nozzle Geometric Parameters
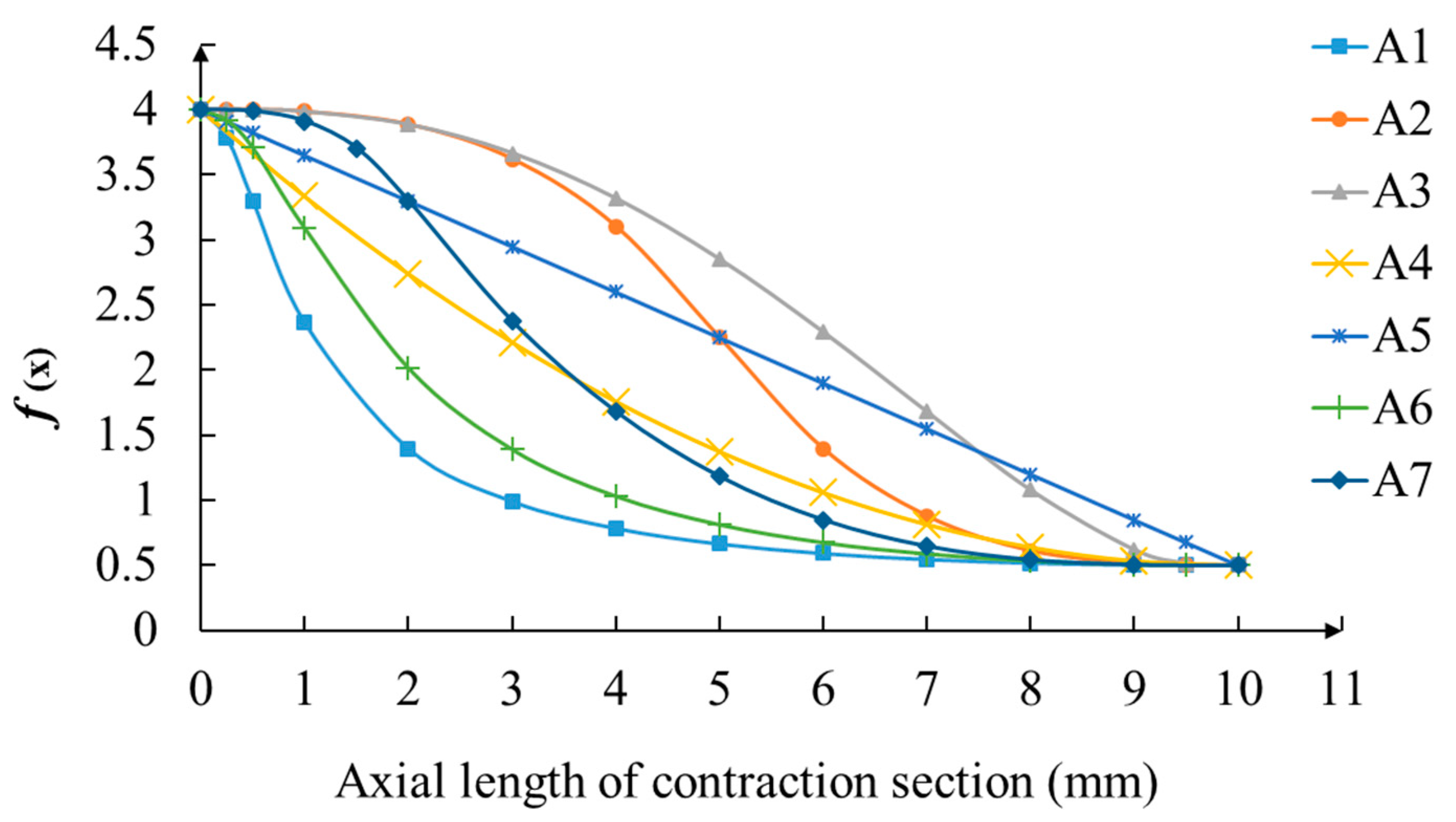
3.2.1. Effect of Contraction Curves
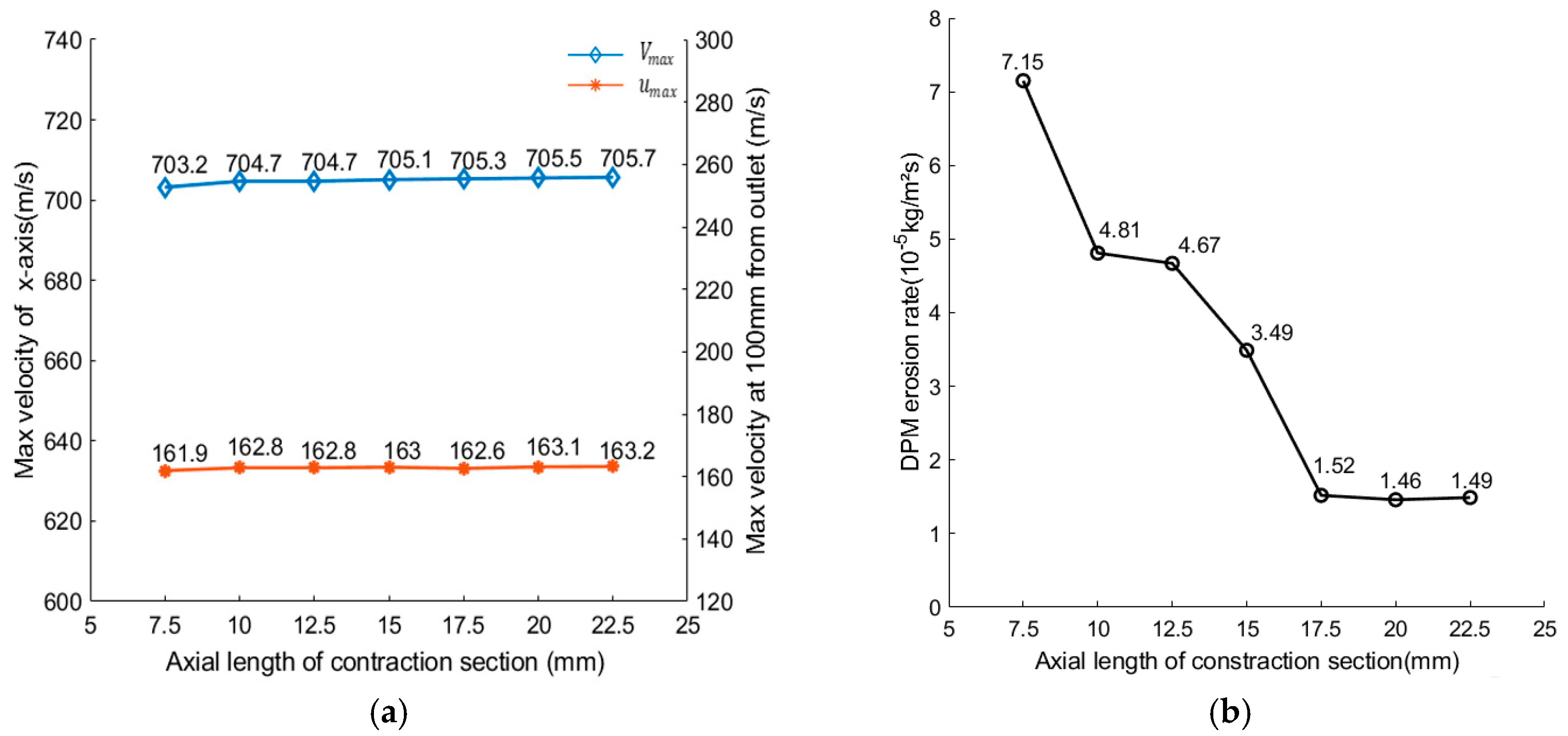
3.2.2. Effect of Axial Length of Contraction Section
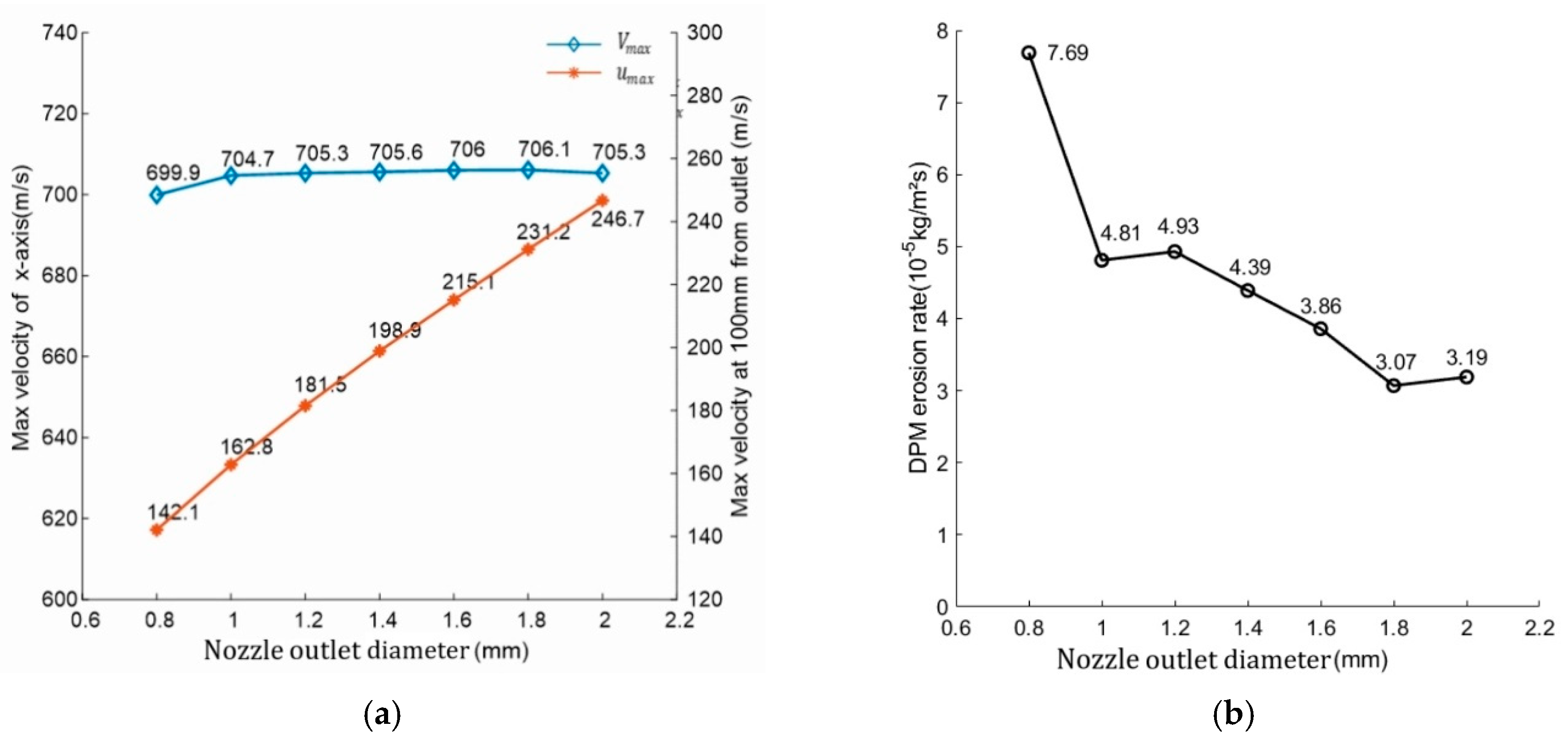
3.2.3. Effect of Outlet Diameter
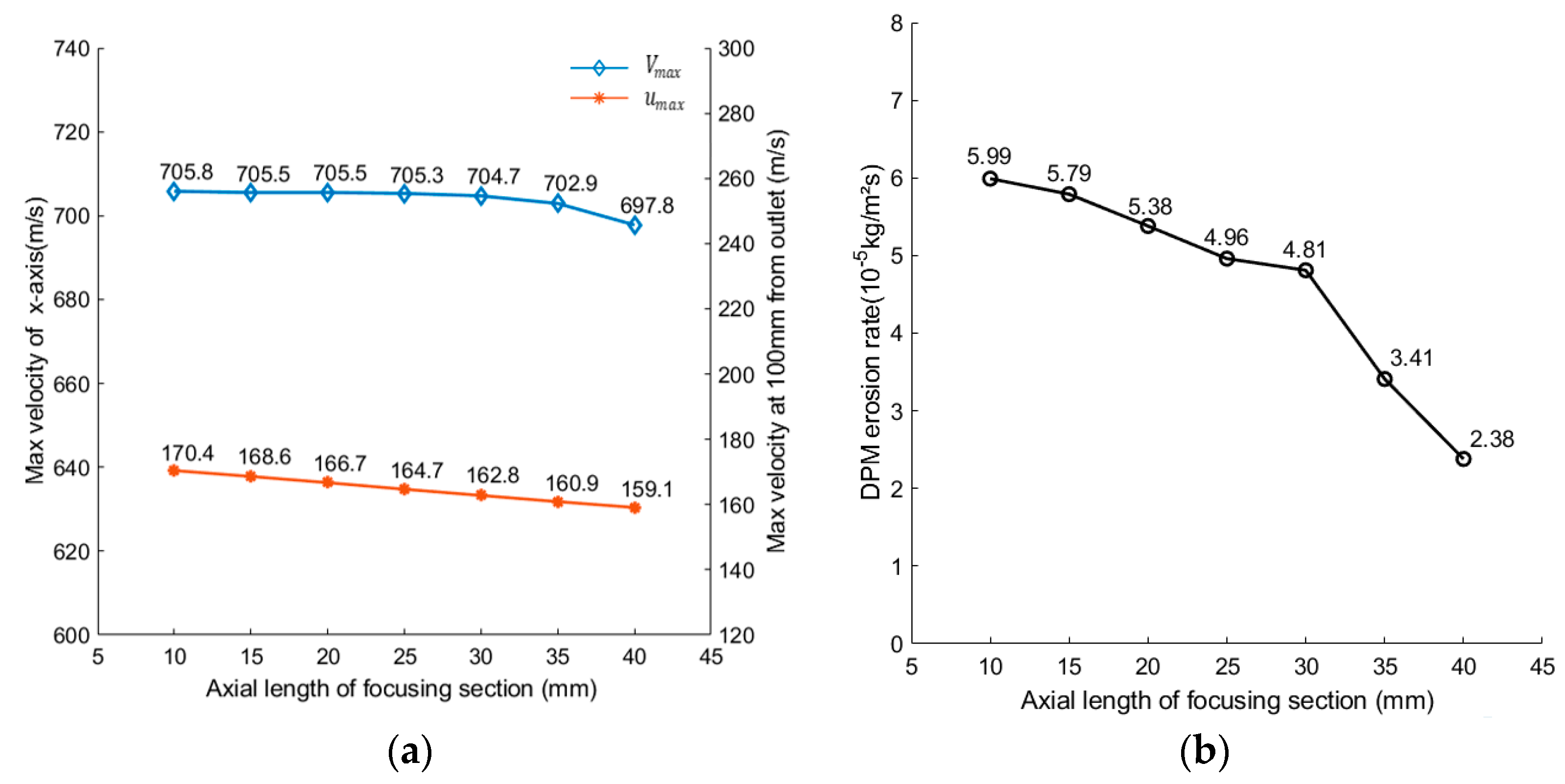
3.2.4. Effect of Axial Length of Focusing Section
3.3. Nozzle Geometry Optimization
3.3.1. Orthogonal Experimental Design Method
3.3.2. Simulation and Discussion
4. Conclusions
- (1)
- The contraction section curve, axial length of contraction section and axial length of focusing section mainly affect the nozzle service life, while nozzle-outlet diameter mainly affects nozzle cutting capacity.
- (2)
- The effect significances of the structural parameters, from high to low, are: outlet diameter > axial length of contraction section > axial length of focusing section > contraction curve.
- (3)
- According to the optimal performance index of the nozzle, the optimal nozzle structure parameters are a contraction curve of (parabolic), an axial length of contraction section of 20 mm, an outlet diameter of 2 mm, and an axial length of focusing section of 10 mm.
- (4)
- With the optimal parameters, the nozzle performance excellence index is = 1.441, which is the optimization objective and 44.1% higher than the baseline of the conical nozzle; the maximum velocity at a distance of 100 mm is improved by 56% and the maximum erosion rate is reduced by 72% compared to those of the conical nozzle.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Llanto, J.M.; Tolouei-Rad, M.; Aamir, M. Recent Progress Trend on Abrasive Waterjet Cutting of Metallic Materials: A Review. Appl. Sci. 2021, 11, 3344. [Google Scholar] [CrossRef]
- Liu, X.C.; Liang, Z.W.; Wen, G.L.; Yuan, X.F. Waterjet machining and research developments: A review. Int. J. Adv. Manuf. Technol. 2019, 102, 1257–1335. [Google Scholar] [CrossRef]
- Haldar, B.; Ghara, T.; Ansari, R.; Das, S.; Saha, P. Abrasive jet system and its various applications in abrasive jet machining, erosion testing, shot-peening, and fast cleaning. Mater. Today Proc. 2018, 5 Pt 2, 13061–13068. [Google Scholar] [CrossRef]
- Cao, L.P.; Liu, S.; Huang, Y.S.; Liu, Q.; Li, Z.H. Study of high-pressure waterjet characteristics based on CFD simulation. Appl. Mech. Mater. 2012, 224, 307–311. [Google Scholar] [CrossRef]
- Yu, Y.; Sun, T.X.; Gao, H.; Wang, X.P. Experimental investigation into the effect of abrasive process parameters on the cutting performance for abrasive waterjet technology: A case study. Int. J. Adv. Manuf. Technol. 2020, 107, 2757–2765. [Google Scholar] [CrossRef]
- Khan, A.A.; Munajat, N.B.; Tajudin, H.B. A Study on Abrasive Water Jet Machining of Aluminum with Garnet Abrasives. J. Appl. Sci. 2005, 5, 1650–1654. [Google Scholar] [CrossRef]
- Liao, W.T.; Deng, X.Y. Study on Flow Field Characteristics of Nozzle Water Jet in Hydraulic cutting. IOP Conf. Ser. Earth Environ. Sci. 2017, 81, 012167. [Google Scholar] [CrossRef]
- Khan, A.A.; Haque, M.M. Performance of different abrasive materials during abrasive water jet machining of glass. J. Mater. Process. Technol. 2007, 191, 404–407. [Google Scholar] [CrossRef]
- Chen, X.C.; Deng, S.S.; Hua, W.X. Experiment and simulation research on abrasive water jet nozzle wear behavior and anti-wear structural improvement. J. Braz. Soc. Mech. Sci. Eng. 2017, 39, 2023–2033. [Google Scholar] [CrossRef]
- Pi, V.N.; Tuan, N.Q. A study on nozzle wear modeling in Abrasive Waterjet Cutting. Adv. Mater. Res. 2009, 78, 345–350. [Google Scholar] [CrossRef]
- Du, M.M.; Wang, H.J.; Guo, Y.J.; Ke, Y.L. Numerical research on multi-particle movements and nozzle wear involved in abrasive waterjet machining. Int. J. Adv. Manuf. Technol. 2021, 117, 2845–2858. [Google Scholar] [CrossRef]
- Ding, M.W.; Fu, C.J.; Yin, S.B. Optimization on the Structure of Ceramic Nozzles by FLUENT Simulation. Appl. Mech. Mater. 2013, 397–400, 213–217. [Google Scholar] [CrossRef]
- Liu, Y.L.; Zhu, H.Q.; Huang, S.G. Effect of structural parameters of high-pressure water jet nozzles on flow field features. Int. J. Heat Technol. 2017, 4, 707–712. [Google Scholar] [CrossRef]
- Deepak, D.; Jodel, A.Q.; Midhun, A.M.; Shiva, P.U. Numerical analysis of the effect of nozzle geometry on flow parameters in abrasive water jet machines. Sci. Technol 2017, 2, 497–506. [Google Scholar]
- Wen, J.W.; Qi, Z.W.; Behbahani, S.S.; Pei, X.J.; Iseley, T. Research on the structures and hydraulic performances of the typical direct jet nozzles for water jet technology. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 570. [Google Scholar] [CrossRef]
- Zhang, Y.X.; Wang, L.F.; Yao, F.; Wang, H.; Men, X.H.; Ye, B.B. Experimental Study on the Influence of Nozzle Diameter on abrasive jet Cutting Performance. Adv. Mater. Res. 2011, 337, 466–469. [Google Scholar] [CrossRef]
- Nanduri, M.; Taggart, D.G.; Kim, T.J. The effects of system and geometric parameters on abrasive water jet nozzle wear. Int. J. Mach. Tools Manuf. 2002, 42, 615–623. [Google Scholar] [CrossRef]
- Guan, J.F.; Deng, S.S.; Jiao, G.W.; Chen, M.; Hua, W.X. Numerical Simulation Study of Influence of Nozzle Entrance Diameter on Jet Performance of Pre-mixed Abrasive Water Jet. Appl. Mech. Mechatron. Intell. Syst. 2015, 2016, 57–62. [Google Scholar]
- Syazwani, H.; Mebrahitom, G.; Azmir, A. A review on nozzle wear in abrasive water jet machining application. IOP Conf. Ser. Mater. Sci. Eng. 2016, 114, 12020–12027. [Google Scholar] [CrossRef]
- Liu, H.; Wang, J.; Kelson, N.; Brown, R.J. A study of abrasive waterjet characteristics by CFD simulation. J. Mater. Process. Technol. 2004, 153–154, 488–493. [Google Scholar] [CrossRef]
- Long, X.P.; Ruan, X.P.; Liu, Q.; Xue, S.X.; Wu, Z.Q. Numerical investigation on the internal flow and the particle movement in the abrasive waterjet nozzle. Powder Technol. 2017, 314, 635–640. [Google Scholar] [CrossRef]
- Toapanta-Ramos, L.F.; Zapata-Cautillo, J.A.; Cholango-Gvailanes, A.I.; Qutiaquez, W.; Nieto-Londono, C.; Zapata-Benabithe, Z. Numerical and comparative study of the turbulence effect on elbows and bends for sanitary water distribution. Rev. Fac. De Ing. 2019, 28, 101–118. [Google Scholar] [CrossRef]
- Kamarudin, N.H.; Prasada Rao, A.K.; Azhari, A. CFD Based Erosion Modelling of Abrasive Waterjet Nozzle using Discrete Phase Method. IOP Conf. Ser. Mater. Sci. Eng. 2016, 114, 12016–12023. [Google Scholar] [CrossRef]
- Pătîrnac, I.; Ripeanu, R.; Laudacescu, G.E. Abrasive flow modelling through active parts water jet machine using CFD simulation. IOP Conf. Ser. Mater. Sci. Eng. 2020, 724, 12001–12006. [Google Scholar] [CrossRef]
- Soyama, H. Effect of nozzle geometry on a standard cavitation erosion test using a cavitating jet. Wear 2013, 297, 895–902. [Google Scholar] [CrossRef]
- Chen, J.; Guo, L.W.; Hu, Y.W.; Chen, Y. Internal structure of a jet nozzle for coalbed methane mining based on airfoil curves. Shock Vib. 2018, 2018, 3840834. [Google Scholar] [CrossRef]
- Deepak, D.; Anjaiah, D.; Vasudeve, K.K.; Sharma, N.Y. CFD simulation of flow in an abrasive water suspension jet: The effect of inlet operating pressure and volume fraction on skin friction and exit kinetic energy. Adv. Mech. Eng. 2011, 2192, 2004–2008. [Google Scholar] [CrossRef]
- Li, S.L.; Wu, G.G.; Wang, P.F.; Cui, Y.; Tian, C.; Han, H. A mathematical model for predicting the sauter mean diameter of Liquid-Medium ultrasonic atomizing nozzle based on orthogonal design. Appl. Sci. 2021, 11, 11628. [Google Scholar] [CrossRef]
- Shen, C.M.; Lin, B.Q.; Meng, F.W. Structure optimization and application of conical convergence high-pressure jet nozzle. Adv. Mater. Res. 2011, 228–229, 1001–1006. [Google Scholar] [CrossRef]

| Grid Size | Number of Grids | (m/s) | ||
|---|---|---|---|---|
| 0.2 mm | 162257 | 704.5 | 163.0 | 4.98 |
| 0.3 mm | 84229 | 704.7 | 162.8 | 4.81 |
| 0.4 mm | 56012 | 704.8 | 162.7 | 4.43 |
| 0.5 mm | 41417 | 704.8 | 162.6 | 4.83 |
| Average | 704.7 | 162.8 | 4.77 |
| Curves | |||||||
|---|---|---|---|---|---|---|---|
| Types | Wiedosinki | Bicubic | Quintuplet | Quadratic | Conical | Shift Wiedosinki | Shift Bicubic |
| Geometric Parameter | Values | Initial Value |
|---|---|---|
| (mm) | 7.5 10 12.5 15 17.5 20 22.5 | 10 |
| (mm) | 0.8 1 1.2 1.4 1.6 1.8 2 | 1 |
| (mm) | 10 15 20 25 30 35 40 | 30 |
| Level (j) | ||||
|---|---|---|---|---|
| Contraction Curve | Axial Length of Contraction Section (mm) | Outlet Diameter (mm) | Axial Length of Focusing Section (mm) | |
| 1 | 7.5 | 0.8 | 10 | |
| 2 | 10 | 1 | 15 | |
| 3 | 12.5 | 1.2 | 20 | |
| 4 | 15 | 1.4 | 25 | |
| 5 | 17.5 | 1.6 | 30 | |
| 6 | 20 | 1.8 | 35 | |
| 7 | 22.5 | 2 | 40 |
| Factor | |||||||
|---|---|---|---|---|---|---|---|
| Number (i) | 1 | 2 | 3 | 4 | |||
| 1 | 7.5 | 0.8 | 10 | 147.7 | 1.78 | 1.097 | |
| 2 | 10 | 1.2 | 25 | 182.7 | 2.91 | 1.147 | |
| 3 | 12.5 | 1.6 | 40 | 211.5 | 3.63 | 1.201 | |
| 4 | 15 | 2 | 20 | 248.7 | 2.85 | 1.352 | |
| 5 | 17.5 | 1 | 35 | 158 | 1.28 | 1.156 | |
| 6 | 20 | 1.4 | 15 | 202.1 | 1.49 | 1.280 | |
| 7 | 22.5 | 1.8 | 30 | 229.3 | 1.99 | 1.336 | |
| 8 | 7.5 | 2 | 35 | 245.6 | 5.91 | 1.211 | |
| 9 | 10 | 1 | 15 | 168.7 | 2.05 | 1.147 | |
| 10 | 12.5 | 1.4 | 30 | 198.3 | 3.45 | 1.169 | |
| 11 | 15 | 1.8 | 10 | 237.3 | 2.35 | 1.342 | |
| 12 | 17.5 | 0.8 | 25 | 141.9 | 0.87 | 1.130 | |
| 13 | 20 | 1.2 | 40 | 177.5 | 1.57 | 1.200 | |
| 14 | 22.5 | 1.6 | 20 | 222.6 | 2.37 | 1.296 | |
| 15 | 7.5 | 1.8 | 25 | 233.2 | 5.84 | 1.175 | |
| 16 | 10 | 0.8 | 40 | 135.4 | 1.92 | 1.052 | |
| 17 | 12.5 | 1.2 | 20 | 190.9 | 1.84 | 1.226 | |
| 18 | 15 | 1.6 | 35 | 214.1 | 1.87 | 1.296 | |
| 19 | 17.5 | 2 | 15 | 236 | 1.82 | 1.366 | |
| 20 | 20 | 1 | 30 | 162.9 | 0.61 | 1.209 | |
| 21 | 22.5 | 1.4 | 10 | 205.7 | 0.93 | 1.322 | |
| 22 | 7.5 | 1.6 | 15 | 220.6 | 3.02 | 1.258 | |
| 23 | 10 | 2 | 30 | 246.8 | 2.84 | 1.347 | |
| 24 | 12.5 | 1 | 10 | 169.9 | 0.82 | 1.219 | |
| 25 | 15 | 1.4 | 25 | 200.3 | 0.96 | 1.304 | |
| 26 | 17.5 | 1.8 | 40 | 227.8 | 1.71 | 1.347 | |
| 27 | 20 | 0.8 | 20 | 144.1 | 0.33 | 1.168 | |
| 28 | 22.5 | 1.2 | 35 | 179.1 | 0.38 | 1.273 | |
| 29 | 7.5 | 1.4 | 40 | 194.8 | 6.99 | 1.015 | |
| 30 | 10 | 1.8 | 20 | 234.1 | 3.29 | 1.286 | |
| 31 | 12.5 | 0.8 | 35 | 138.5 | 4.89 | 0.922 | |
| 32 | 15 | 1.2 | 15 | 187.2 | 1.92 | 1.211 | |
| 33 | 17.5 | 1.6 | 30 | 215.6 | 1.32 | 1.331 | |
| 34 | 20 | 2 | 10 | 253 | 1.42 | 1.439 | |
| 35 | 22.5 | 1 | 25 | 164.9 | 1.47 | 1.167 | |
| 36 | 7.5 | 1.2 | 30 | 181.5 | 3.93 | 1.095 | |
| 37 | 10 | 1.6 | 10 | 221.7 | 2.44 | 1.290 | |
| 38 | 12.5 | 2 | 25 | 247.8 | 3.42 | 1.322 | |
| 39 | 15 | 1 | 40 | 158 | 1.11 | 1.165 | |
| 40 | 17.5 | 1.4 | 20 | 201.1 | 2.54 | 1.222 | |
| 41 | 20 | 1.8 | 35 | 228.5 | 2.13 | 1.327 | |
| 42 | 22.5 | 0.8 | 15 | 145.9 | 0.67 | 1.154 | |
| 43 | 7.5 | 1 | 20 | 166.7 | 1.89 | 1.149 | |
| 44 | 10 | 1.4 | 35 | 197.1 | 3.13 | 1.180 | |
| 45 | 12.5 | 1.8 | 15 | 235.5 | 3.55 | 1.279 | |
| 46 | 15 | 0.8 | 30 | 138.8 | 0.71 | 1.129 | |
| 47 | 17.5 | 1.2 | 10 | 186.9 | 1.38 | 1.239 | |
| 48 | 20 | 1.6 | 25 | 215.8 | 1.57 | 1.317 | |
| 49 | 22.5 | 2 | 40 | 243.1 | 1.74 | 1.392 |
| Level (j) | ||||
|---|---|---|---|---|
| 1.224 | 1.143 | 1.093 | 1.278 | |
| 1.213 | 1.207 | 1.173 | 1.242 | |
| 1.235 | 1.191 | 1.199 | 1.243 | |
| 1.274 | 1.257 | 1.213 | 1.223 | |
| 1.195 | 1.256 | 1.284 | 1.231 | |
| 1.225 | 1.278 | 1.299 | 1.195 | |
| 1.241 | 1.277 | 1.347 | 1.196 | |
| 0.060 | 0.134 | 0.244 | 0.083 | |
| Superior level | 4 | 6 | 7 | 1 |
| Factor priority | 4 | 2 | 1 | 3 |
| Source of Variance | Deviation Sum of Squares | Degree of Freedom | Variance | Significant Level | |
|---|---|---|---|---|---|
| 0.025 | 6 | 0.004 | 3.153 | * | |
| 0.108 | 6 | 0.018 | 13.869 | *** | |
| 0.313 | 6 | 0.052 | 40.018 | **** | |
| 0.036 | 6 | 0.006 | 4.574 | ** | |
| Error | 0.031 | 24 | 0.001 | ||
| Sum | 48 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, X.; Wu, Y.; Jia, P.; Liu, H.; Yun, F.; Li, Z.; Wang, L. Orthogonal Experimental Design Based Nozzle Geometry Optimization for the Underwater Abrasive Water Jet. Machines 2022, 10, 1243. https://doi.org/10.3390/machines10121243
Wang X, Wu Y, Jia P, Liu H, Yun F, Li Z, Wang L. Orthogonal Experimental Design Based Nozzle Geometry Optimization for the Underwater Abrasive Water Jet. Machines. 2022; 10(12):1243. https://doi.org/10.3390/machines10121243
Chicago/Turabian StyleWang, Xiangyu, Yongtao Wu, Peng Jia, Huadong Liu, Feihong Yun, Zhibo Li, and Liquan Wang. 2022. "Orthogonal Experimental Design Based Nozzle Geometry Optimization for the Underwater Abrasive Water Jet" Machines 10, no. 12: 1243. https://doi.org/10.3390/machines10121243
APA StyleWang, X., Wu, Y., Jia, P., Liu, H., Yun, F., Li, Z., & Wang, L. (2022). Orthogonal Experimental Design Based Nozzle Geometry Optimization for the Underwater Abrasive Water Jet. Machines, 10(12), 1243. https://doi.org/10.3390/machines10121243







