A Non-Intrusive Stochastic Isogeometric Analysis of Functionally Graded Plates with Material Uncertainty
Abstract
:1. Introduction
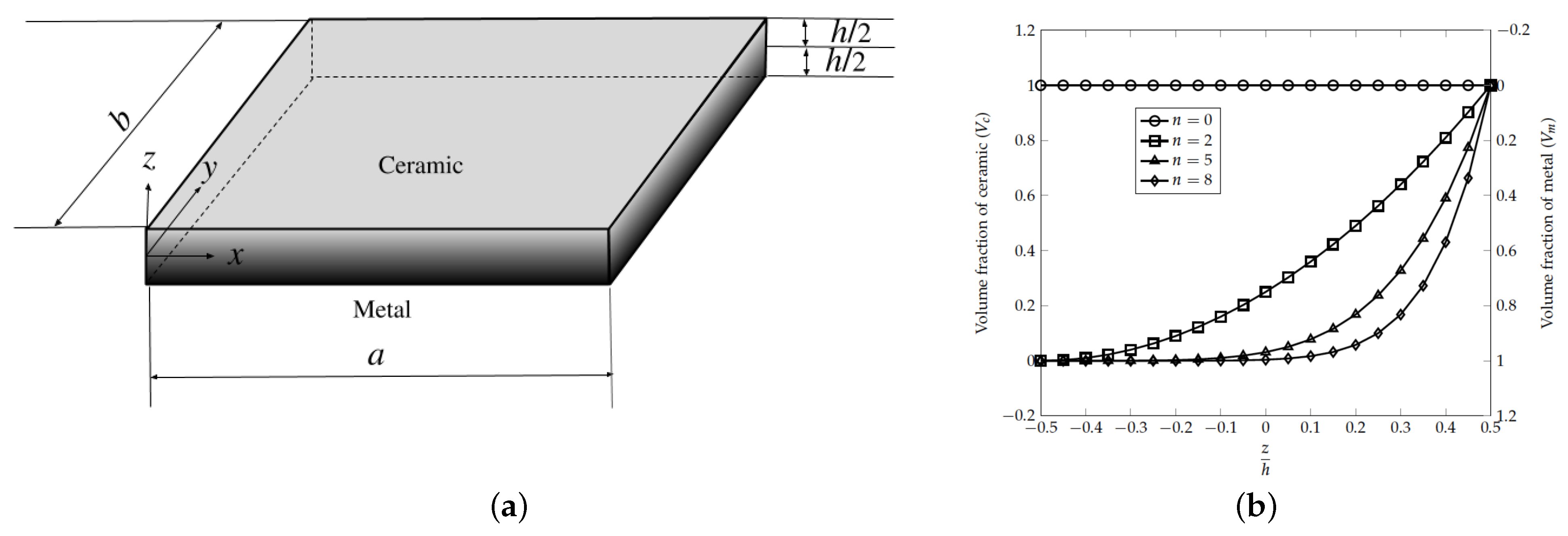
2. Analysis of Functionally Graded Panels
2.1. Effective Modulus and Poisson’s Ratio
2.2. First Order Shear Deformation Theory
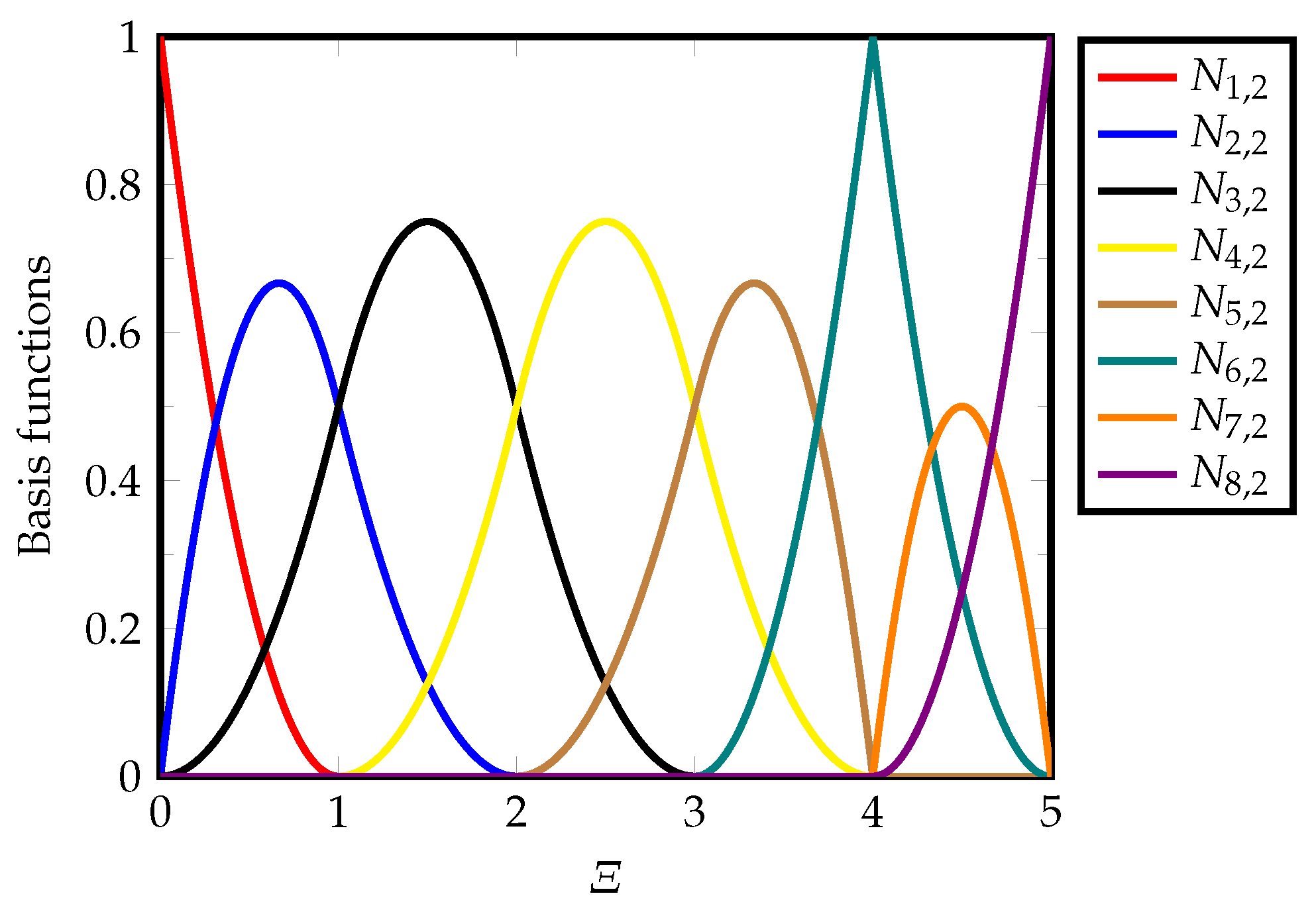
3. Overview of Isogeometric Analysis
4. Non-Intrusive Spectral Projection
5. Numerical Examples
5.1. Validation
5.2. Static Bending
- Case A: is random,
- Case B: and is random.
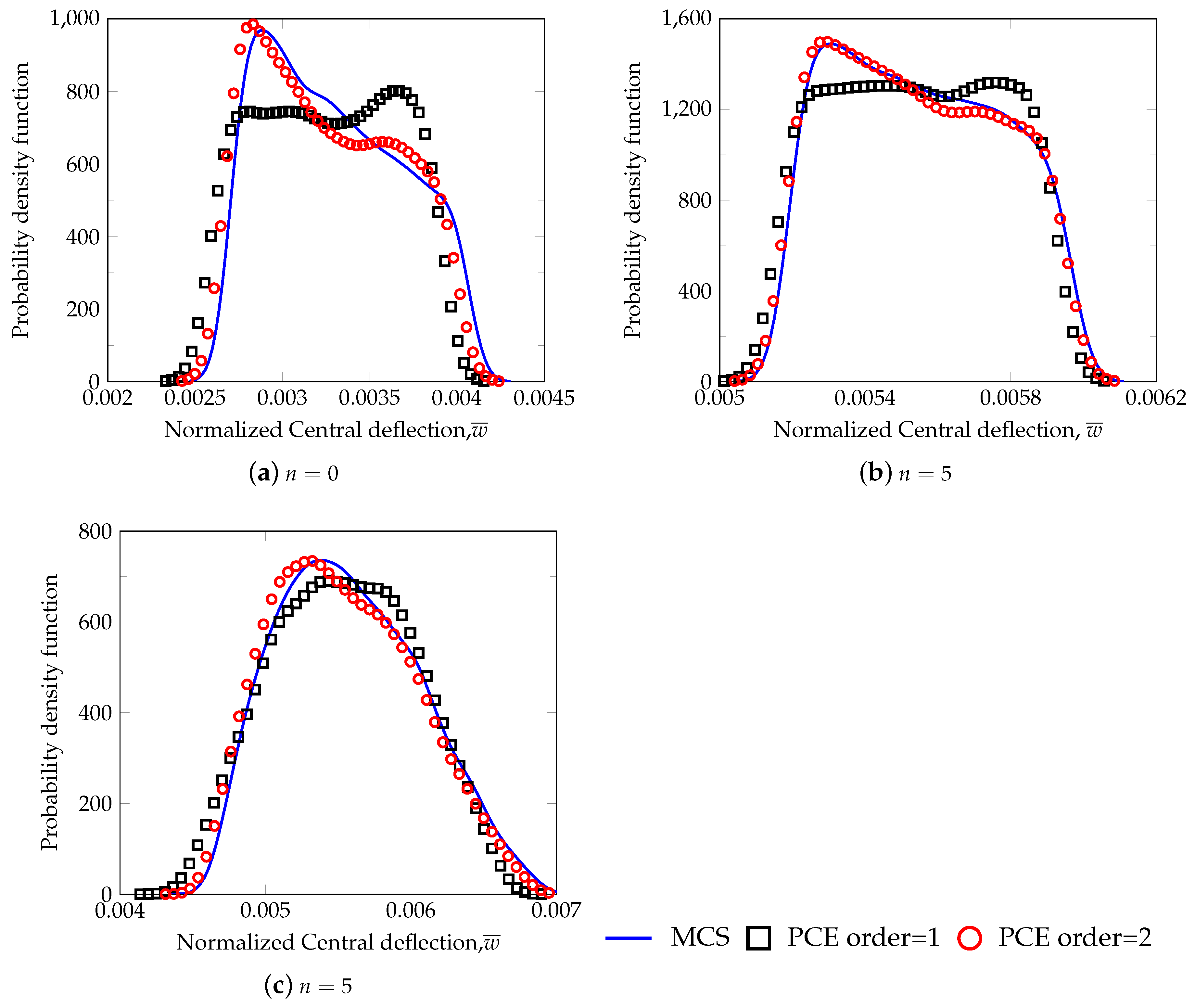
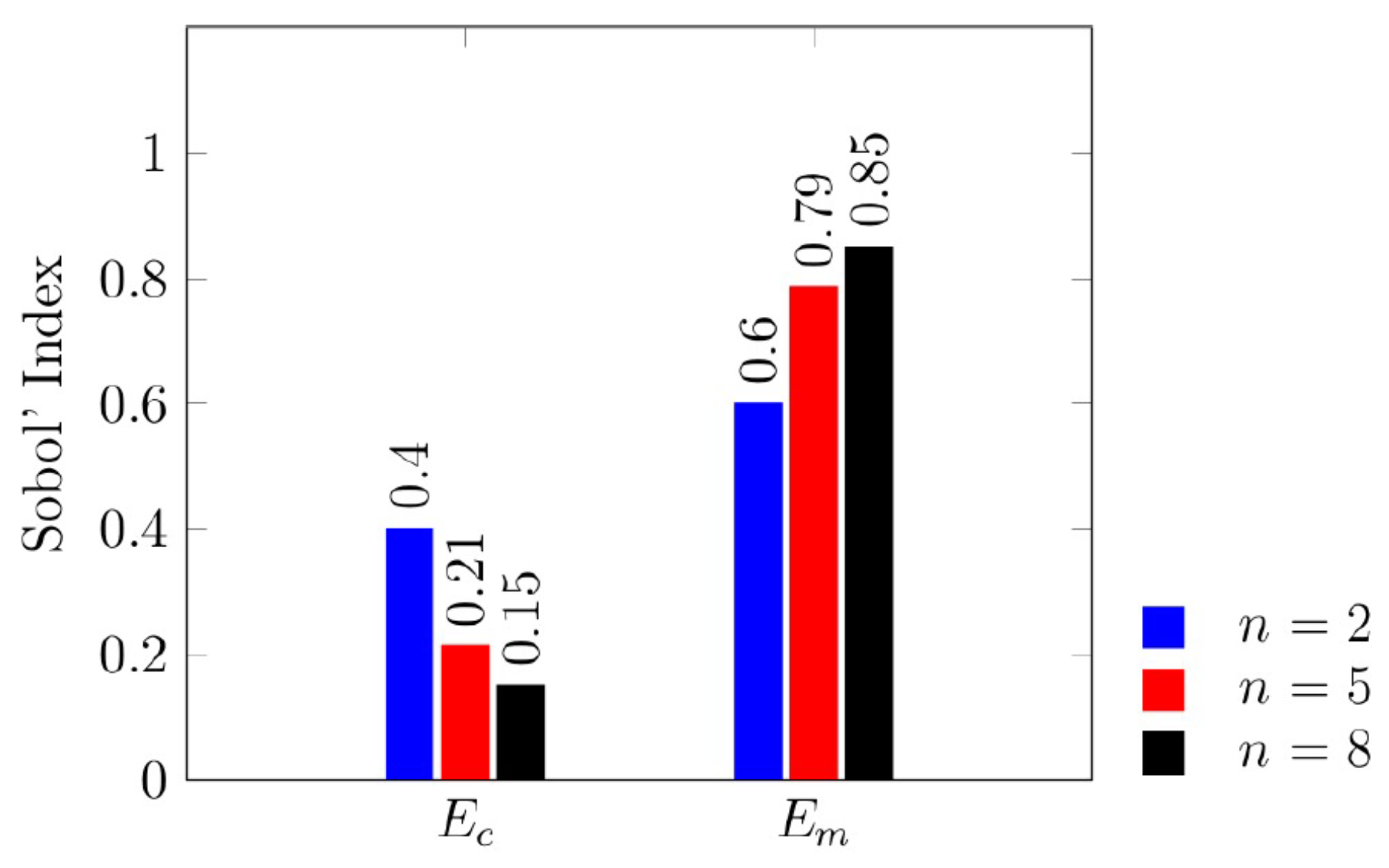
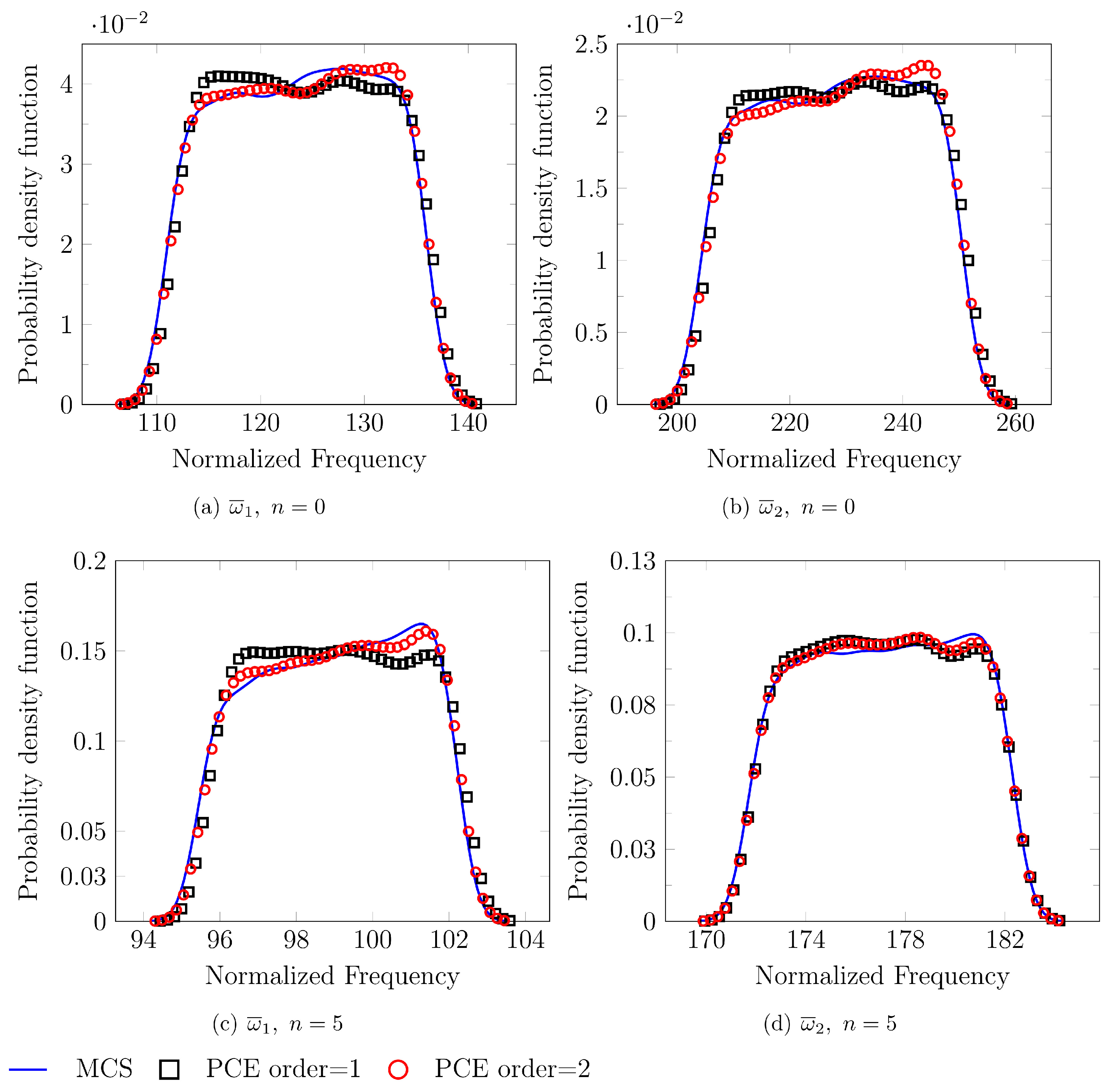
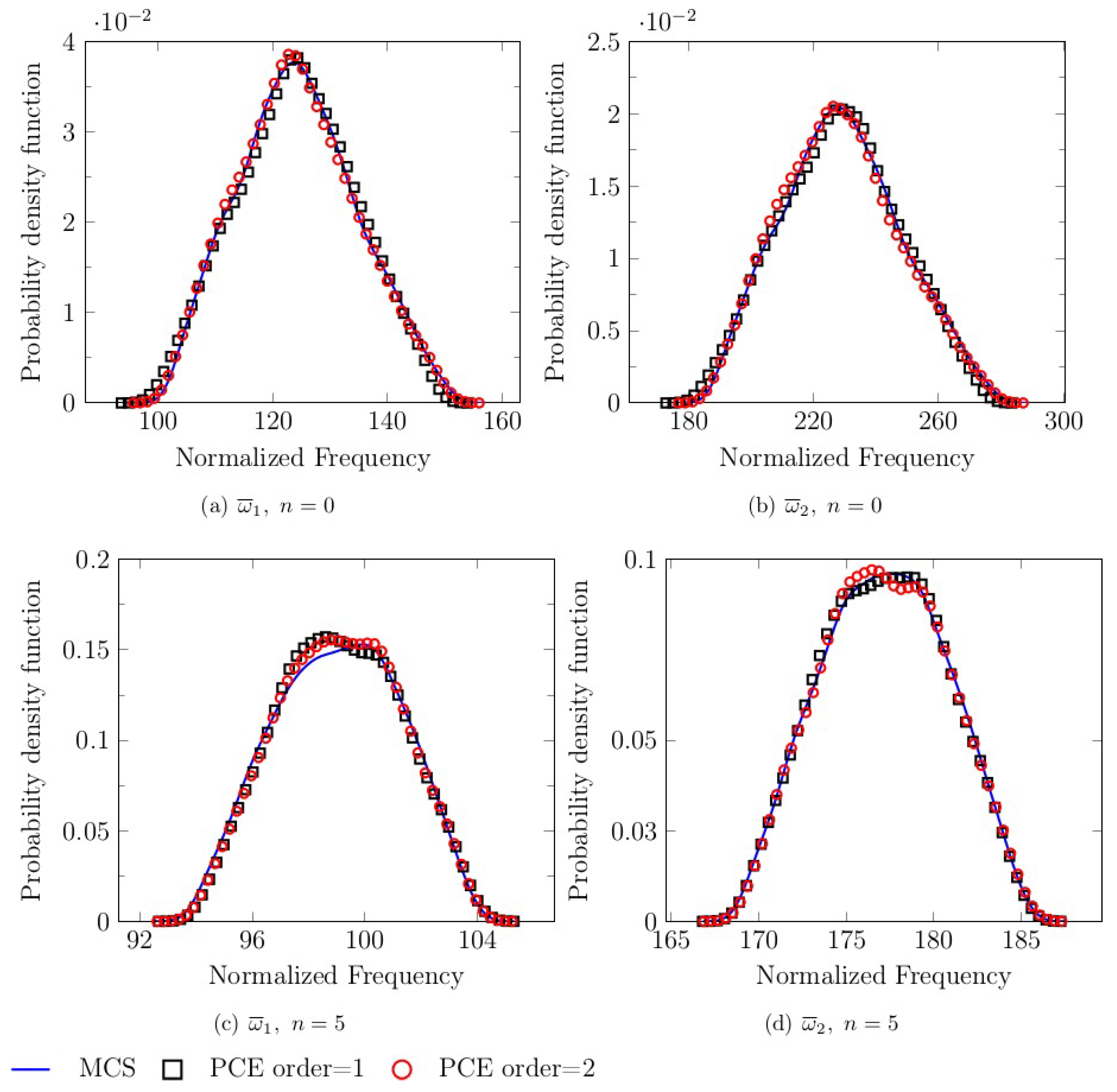
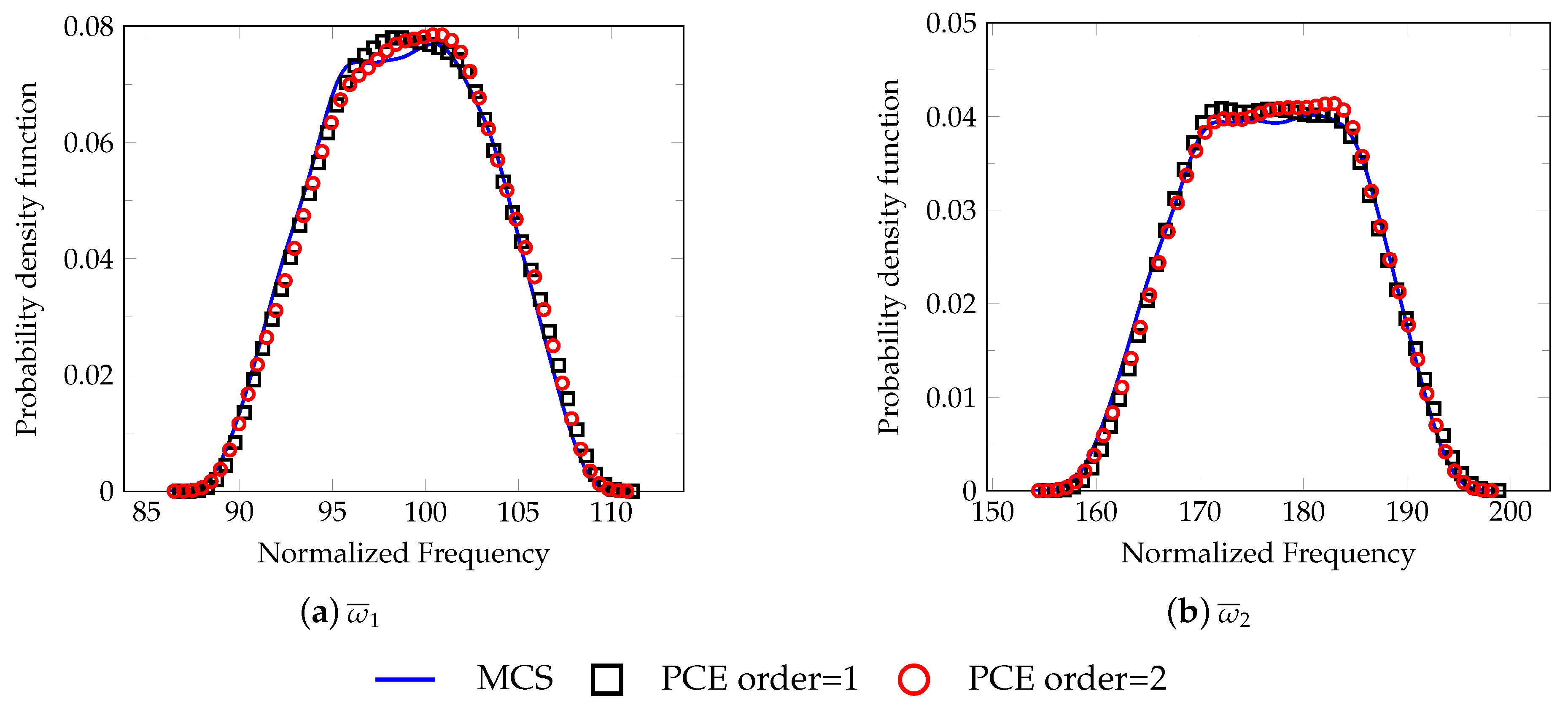
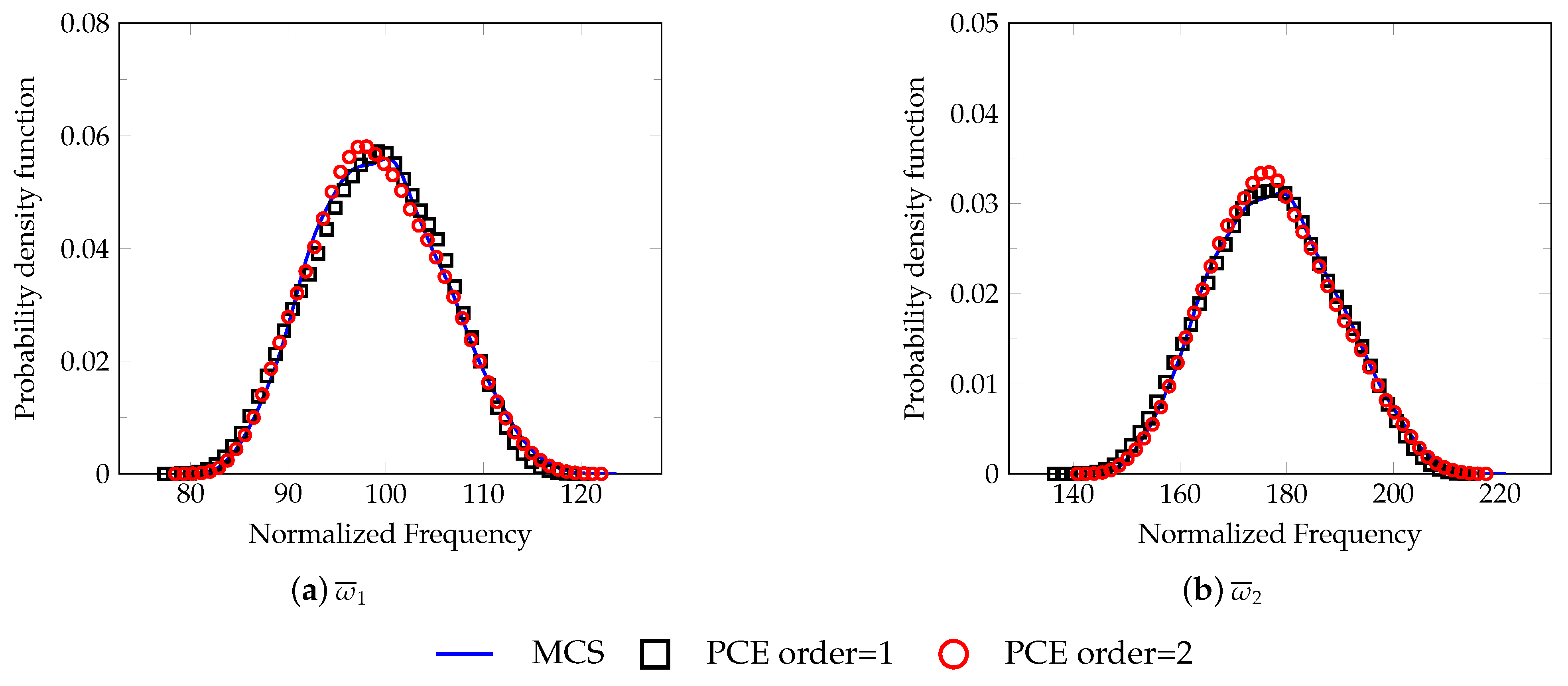
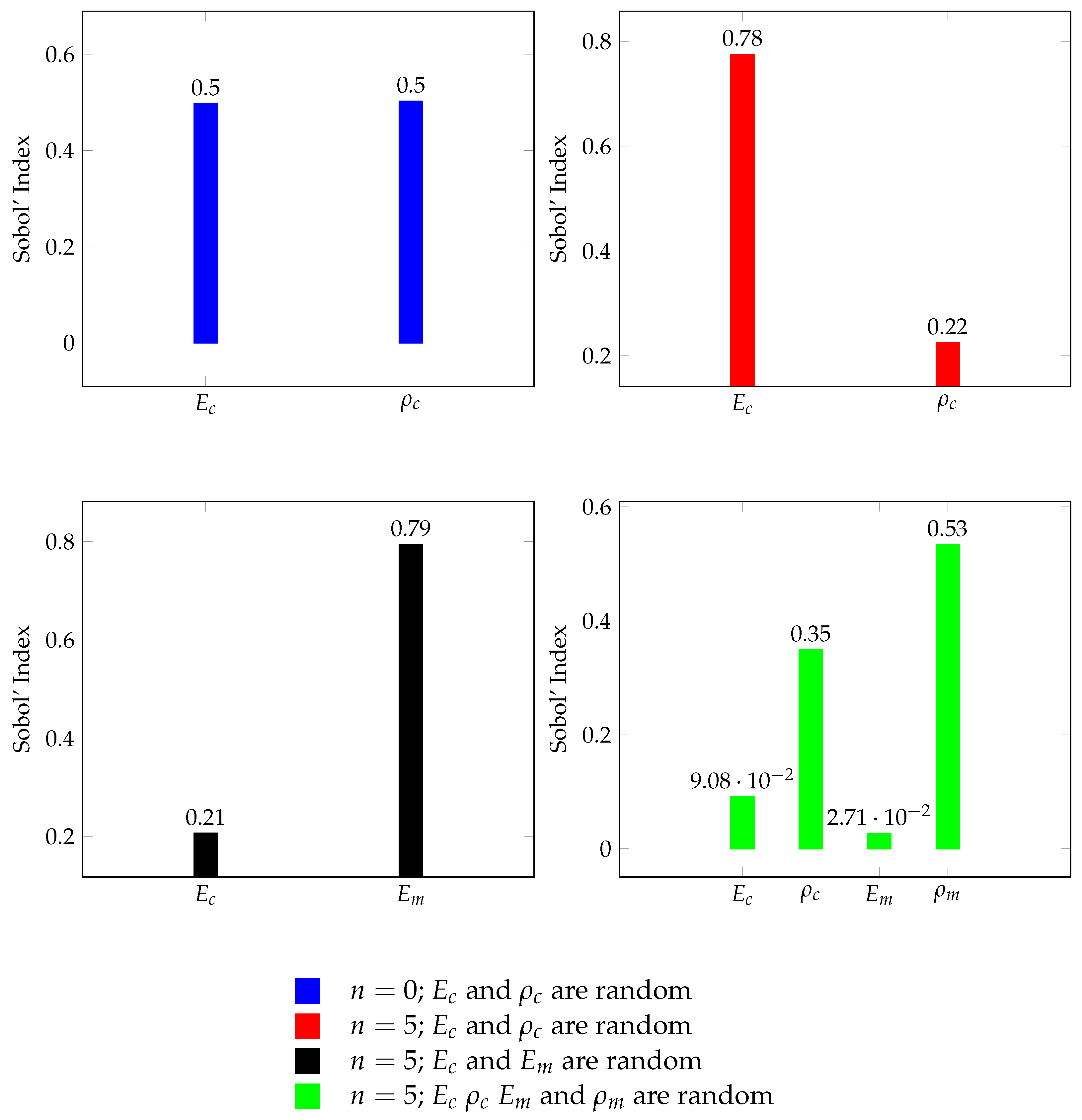
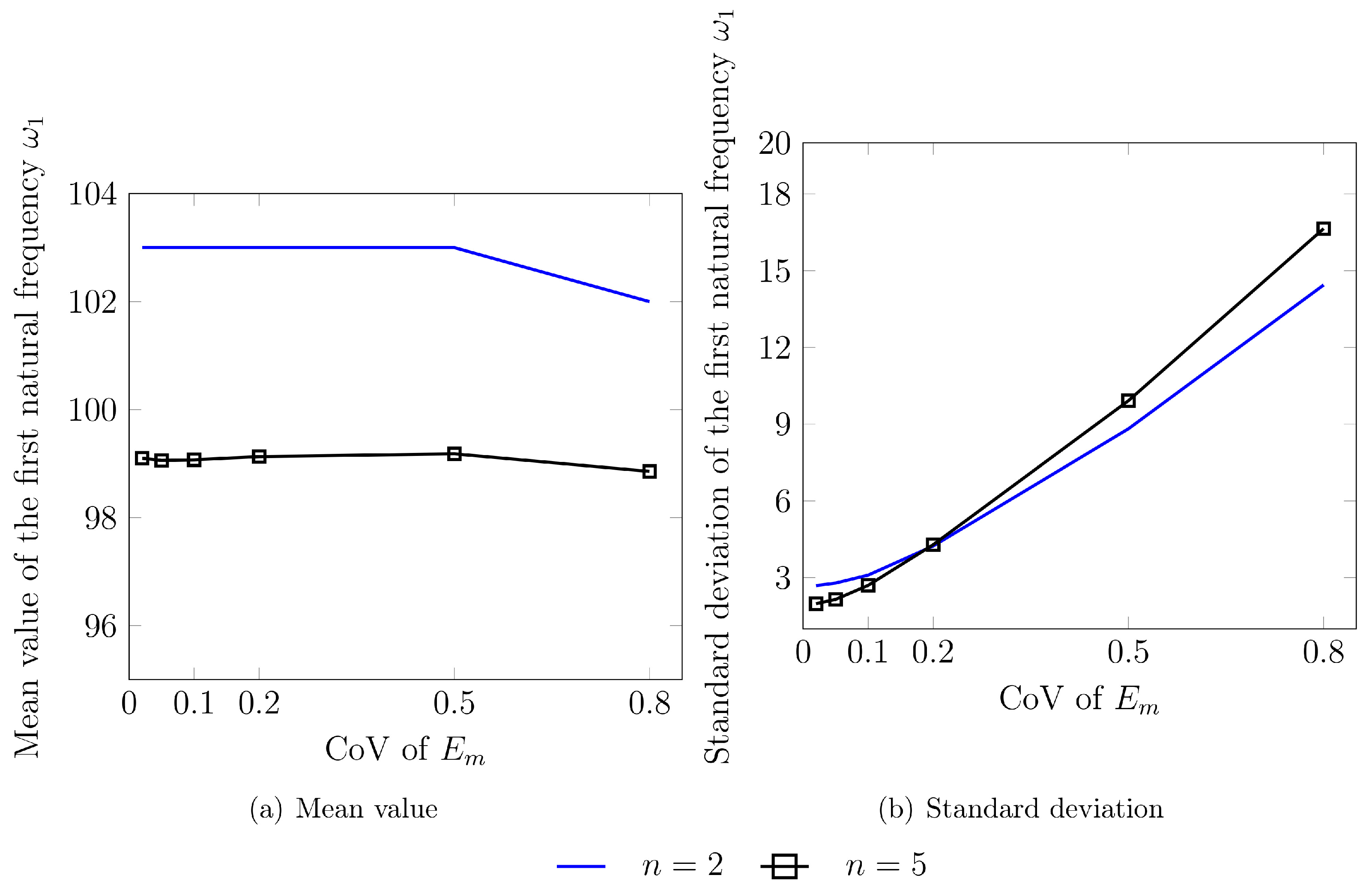
5.3. Free Vibration Analysis
- Case A: is random.
- Case B: and is random.
- Case C: and is random.
- Case D: and is random.
6. Conclusions
- It can handle a wider range of CoV, unlike the perturbation based methods.
- Does not require to modify the existing code like the intrusive based approaches.
Author Contributions
Funding
Conflicts of Interest
References
- Balaganesan, G.; Khan, V.C. Energy absorption of repaired composite laminates subjected to impact loading. Compos. Part B Eng. 2016, 98, 39–48. [Google Scholar] [CrossRef]
- Budarapu, P.; Kumar, S.; Prusty, B.G.; Paggi, M. Stress transfer through the interphase in curved-fiber pullout tests of nanocomposites. Compos. Part B Eng. 2019, 165, 417–434. [Google Scholar] [CrossRef]
- Budarapu, P.; Thakur, S.; Kumar, S.; Paggi, M. Micromechanics of engineered interphases in nacre-like composite structures. Mech. Adv. Mater. Struct. 2020, 1–16. [Google Scholar] [CrossRef]
- Jagtap, K.; Lal, A.; Singh, B. Stochastic nonlinear free vibration analysis of elastically supported functionally graded materials plate with system randomness in thermal environment. Compos. Struct. 2011, 93, 3185–3199. [Google Scholar] [CrossRef]
- Kitipornchai, S.; Yang, J.; Liew, K. Random vibration of the functionally graded laminates in thermal environments. Comput. Methods Appl. Mech. Eng. 2006, 195, 1075–1095. [Google Scholar] [CrossRef]
- Liu, W.K.; Belytschko, T.; Mani, A. Random field finite elements. Int. J. Num. Methods Eng. 1986, 23, 1831–1845. [Google Scholar] [CrossRef]
- Takada, T. Weighted integral method in stochastic finite element analysis. Probab. Eng. Mech. 1990, 5, 146–156. [Google Scholar] [CrossRef]
- Ghanem, R.G.; Spanos, P.D. Spectral Stochastic Finite Element Formulation for Reliability Analysis. J. Eng. Mech. 1991, 117, 2351–2372. [Google Scholar] [CrossRef]
- Kleiber, M.; Hien, T.D. The Stochastic Finite Element Method: Basic Perturbation Technique and Computer Implementation; Applied Stochastic Models and Data Analysis; Wiley: Chichester, UK, 1992; p. 297. [Google Scholar]
- Sudret, B.; Kiureghian, A.D. Stochastic Finite Element Methods and Reliability: A State-of-the-art Report; Report No. UCB/SEMM-2000/08; Department of Civil and Environmental Engineering, University of California: Berkeley, CA, USA, 2000. [Google Scholar]
- Chiba, R.; Sugano, Y. Stochastic analysis of a thermoelastic problem in functionally graded plates with uncertain material properties. Arch. Appl. Mech. 2007, 78, 749. [Google Scholar] [CrossRef]
- Shaker, A.; Abdelrahman, W.; Tawfik, M.; Sadek, E. Stochastic Finite element analysis of the free vibration of functionally graded material plates. Comput. Mech. 2008, 41, 707–714. [Google Scholar] [CrossRef]
- Li, K.; Wu, D.; Gao, W. Spectral stochastic isogeometric analysis for static response of FGM plate with material uncertainty. Thin-Walled Struct. 2018, 132, 504–521. [Google Scholar] [CrossRef]
- Kumar, R.; Mukhopadhyay, T.; Pandey, K.; Dey, S. Stochastic buckling analysis of sandwich plates: The importance of higher order modes. Int. J. Mech. Sci. 2019, 152, 630–643. [Google Scholar] [CrossRef]
- Thanh, C.L.; Tran, L.V.; Bui, T.Q.; Nguyen, H.X.; Abdel-Wahab, M. Isogeometric analysis for size-dependent nonlinear thermal stability of porous FG microplates. Compos. Struct. 2019, 221, 110838. [Google Scholar] [CrossRef]
- Wu, F.; Gao, Q.; Xu, X.M.; Zhong, W.X. A Modified Computational Scheme for the Stochastic Perturbation Finite Element Method. Latin Am. J. Solids Struct. 2015, 12, 2480–2505. [Google Scholar] [CrossRef] [Green Version]
- Kumaraian, M.L.; Rebbagondla, J.; Mathew, T.V.; Natarajan, S. Stochastic vibration analysis of functionally graded material paltes with material randomness using cell based smoothed discrete shear gap method. Int. J. Struct. Stabil. Dyn. 2019, 19, 1950037-1–1950037-20. [Google Scholar] [CrossRef]
- Minh, P.P.; Duc, N.D. The effect of cracks on the stability of the functionally graded plates with variable-thickness using HSDT and phase-field theory. Compos. Part B Eng. 2019, 175, 107086. [Google Scholar] [CrossRef]
- Sepahvand, K.; Marburg, S.; Hardtke, H.J. Stochastic free vibration of orthotropic plates using generalized polynomial chaos expansion. J. Sound Vibr. 2012, 331, 167–179. [Google Scholar] [CrossRef]
- Karsh, P.; Mukhopadhyay, T.; Chakraborty, S.; Naskar, S.; Dey, S. A hybrid stochastic sensitivity analysis for low-frequency vibration and low-velocity impact of functionally graded plates. Compos. Part B Eng. 2019, 176, 107221. [Google Scholar] [CrossRef]
- Tran, L.V.; Phung-Van, P.; Lee, J.; Wahab, M.A.; Nguyen-Xuan, H. Isogeometric analysis for nonlinear thermomechanical stability of functionally graded plates. Compos. Struct. 2016, 140, 655–667. [Google Scholar] [CrossRef] [Green Version]
- Lieu, Q.X.; Lee, J. Modeling and optimization of functionally graded plates under thermo-mechanical load using isogeometric analysis and adaptive hybrid evolutionary firefly algorithm. Compos. Struct. 2017, 179, 89–106. [Google Scholar] [CrossRef]
- Sundararajan, N.; Prakash, T.; Ganapathi, M. Nonlinear free flexural vibrations of functionally graded rectangular and skew plates under thermal environments. Finite Elem. Anal. Des. 2005, 42, 152–168. [Google Scholar] [CrossRef] [Green Version]
- Rajasekaran, S.; Murray, D. Incremental finite element matrices. ASCE J. Struct. Div. 1973, 99, 2423–2438. [Google Scholar]
- Cottrell, J.A.; Hughes, T.J.; Bazilevs, Y. Isogeometric Analysis: Toward Integration of CAD and FEA; John Wiley: Hoboken, NJ, USA, 2009. [Google Scholar]
- Nguyen, V.P.; Simpson, R.N.; Bordas, S.; Rabczuk, T. An introduction to Isogeometric Analysis with Matlab implementation: FEM and XFEM formulations. arXiv 2012, arXiv:1205.2129. [Google Scholar]
- Kikuchi, F.; Ishii, K. An improved 4-node quadrilateral plate bending element of the Reissne-Mindlin type. Comput. Mech. 1999, 23, 240–249. [Google Scholar] [CrossRef]
- Valizadeh, N.; Natarajan, S.; Gonzalez-Estrada, O.A.; Rabczuk, T.; Bui, T.Q.; Bordas, S.P. NURBS-based finite element analysis of functionally graded plates: Static bending, vibration, buckling and flutter. Compos. Struct. 2013, 99, 309–326. [Google Scholar] [CrossRef] [Green Version]
- Wiener, N. The homogeneous chaos. Am. J. Math. 1938, 60, 897–936. [Google Scholar] [CrossRef]
- Nguyen-Xuan, H.; Tran, L.V.; Thai, H.; Nguyen-Thoi, T. Analysis of functionally graded plates by an efficient finite element method with node-based strain smoothing. Thin Walled Struct. 2012, 54, 1–18. [Google Scholar] [CrossRef]
- Matsunaga, H. Free vibration and stability of functionally graded plates according to a 2D higher-order deformation theory. Compos. Struct. 2008, 82, 499–512. [Google Scholar] [CrossRef]
- Lee, Y.; Zhao, X.; Liew, K. Thermo-elastic analysis of functionally graded plates using the element free kp−Ritz method. Smart Mater. Struct. 2009, 18, 035007. [Google Scholar] [CrossRef]
- Zhao, X.; Lee, Y.; Liew, K. Free vibration analysis of functionally graded plates using the element free kp−Ritz method. J. Sound Vibr. 2009, 319, 918–939. [Google Scholar] [CrossRef]
- Nguyen-Xuan, H.; Tran, L.V.; Nguyen-Thoi, T.; Vu-Do, H. Analysis of functionally graded plates using an edge-based smoothed finite element method. Compos. Struct. 2011, 93, 3019–3039. [Google Scholar] [CrossRef]
| Distribution Types | Polynomials | Support | |
|---|---|---|---|
| Continuous | Beta | Jacobi | |
| Exponential | Laguerre | ||
| Gamma | General Laguerre | ||
| Normal | Hermite | ||
| Uniform | Legendre | ||
| Discrete | Poisson | Charlier | |
| Binomial | Krawtchouk | ||
| Negative Binomial | Meixner | ||
| Hypergeometric | Hahn |
| Random Input Variable | Symbol | Mean Value | CoV | |
|---|---|---|---|---|
| ZrO | Young’s modulus | 151 GPa | 0.2 | |
| Density | 3000 kg/m | 0.2 | ||
| Al | Young’s modulus | 70 GPa | 0.2 | |
| Density | 2770 kg/m | 0.2 |
| Method | Number of | Gradient Index, n | |||
|---|---|---|---|---|---|
| Control Points | 0 | 0.5 | 1 | 2 | |
| Quadratic | 4 | 0.162098 | 0.218914 | 0.256018 | 0.293806 |
| 8 | 0.171617 | 0.232392 | 0.271879 | 0.311459 | |
| 16 | 0.171649 | 0.232439 | 0.271935 | 0.311520 | |
| 24 | 0.171651 | 0.232441 | 0.271938 | 0.311523 | |
| ES-DSG3 [30] | 0.1700 | 0.2296 | 0.2680 | 0.3066 | |
| MITC4 [30] | 0.1715 | 0.2317 | 0.2704 | 0.3093 | |
| Ritz [32] | 0.1722 | 0.2403 | 0.2811 | 0.3221 | |
| Method | Control Points | Gradient Index, n | ||||
|---|---|---|---|---|---|---|
| 0 | 0.5 | 1 | 4 | 10 | ||
| IGA-Quadratic | 8 | 0.21128 | 0.18051 | 0.16309 | 0.13962 | 0.13231 |
| 16 | 0.21121 | 0.18045 | 0.16303 | 0.13957 | 0.13227 | |
| 24 | 0.21121 | 0.18044 | 0.16303 | 0.13957 | 0.13227 | |
| MITC4 (16×16) [34] | 0.21182 | 0.18082 | 0.16323 | 0.13968 | 0.13251 | |
| HSDT [31] | 0.21210 | 0.18190 | 0.16400 | 0.13830 | 0.13060 | |
| Ritz [33] | 0.20550 | 0.17570 | 0.15870 | 0.13560 | 0.12840 | |
| Central | Gradient | Random | Mean | Standard Deviation | ||
|---|---|---|---|---|---|---|
| Deflection | Index, n | Variables | MCS | PCE | MCS | PCE |
| 0 | ||||||
| 5 | ||||||
| Frequency | Gradient | Random | Mean | Standard Deviation | ||
|---|---|---|---|---|---|---|
| Index, n | Variables | MCS | PCE | MCS | PCE | |
| 0 | 123.72 | 123.97 | 7.20 | 7.19 | ||
| 124.42 | 124.02 | 1.95 | 1.95 | |||
| 5 | 99.03 | 99.02 | 2.22 | 2.20 | ||
| 99.03 | 99.02 | 2.22 | 2.20 | |||
| 98.80 | 99.03 | 4.28 | 4.24 | |||
| 99.27 | 99.05 | 6.53 | 6.44 | |||
| 0 | 227.89 | 228.59 | 13.27 | 13.34 | ||
| 229.17 | 228.39 | 18.93 | 18.80 | |||
| 5 | 177.15 | 177.14 | 3.05 | 3.02 | ||
| 177.16 | 177.14 | 3.56 | 3.53 | |||
| 176.85 | 177.25 | 7.85 | 7.86 | |||
| 177.69 | 177.04 | 11.84 | 11.80 | |||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dsouza, S.M.; Varghese, T.M.; Budarapu, P.R.; Natarajan, S. A Non-Intrusive Stochastic Isogeometric Analysis of Functionally Graded Plates with Material Uncertainty. Axioms 2020, 9, 92. https://doi.org/10.3390/axioms9030092
Dsouza SM, Varghese TM, Budarapu PR, Natarajan S. A Non-Intrusive Stochastic Isogeometric Analysis of Functionally Graded Plates with Material Uncertainty. Axioms. 2020; 9(3):92. https://doi.org/10.3390/axioms9030092
Chicago/Turabian StyleDsouza, Shaima M., Tittu Mathew Varghese, P. R. Budarapu, and S. Natarajan. 2020. "A Non-Intrusive Stochastic Isogeometric Analysis of Functionally Graded Plates with Material Uncertainty" Axioms 9, no. 3: 92. https://doi.org/10.3390/axioms9030092
APA StyleDsouza, S. M., Varghese, T. M., Budarapu, P. R., & Natarajan, S. (2020). A Non-Intrusive Stochastic Isogeometric Analysis of Functionally Graded Plates with Material Uncertainty. Axioms, 9(3), 92. https://doi.org/10.3390/axioms9030092






