Odd Cycles in Conditionally Faulty Enhanced Hypercube Networks

Abstract

The n-dimensional enhanced hypercube $Q_{n,k}(1\le k\le n-1)$ is a well-known variation of hypercube networks. Its structure can be obtained from the hypercube by adding $2^{n-1}$ complementary edges. We denote a network G to be a conditionally faulty model if every fault-free vertex of G connects at least two fault-free edges. Let $F_v$ and $F_e$ be the set of faulty vertices and faulty edges in $Q_{n,k}(1\le k\le n-1)$, respectively. In this paper, for the conditionally faulty $Q_{n,k}$ with $\left|F_v\right|+\left|F_e\right|\le 2n-5$, where $n(\ge 3)$ and k have different parity, I prove that $Q_{n,k}-F_v-F_e$ contains a fault-free cycle with every odd length l, where $n-k+4\le l\le 2^n-2\left|F_v\right|-1$.

1. Introduction

Interconnection networks are significant for designing massive parallel or distributed systems, and they have attracted more and more research interest [1]. Hypercubes, as a kind of interconnection network topology, have many positive characteristics, such as regularity, a recursive structure, symmetry, and a logarithmic diameter [1]. Numerous variants of the hypercube have been proposed in the literature [2,3,4,5,6,7,8]. One variant that has attracted a great deal of research interest is the enhanced hypercube $Q_{n,k}(1\le k\le n-1)$ [9], since it possesses many properties superior to the standard hypercube [9,10,11,12,13,14,15]. Specifically, when $k=1$, we call the folded hypercube $F{Q}_n$ [16,17,18,19] in the case where $Q_{n,1}=F{Q}_n$.

A variety of network topologies have been investigated and constructed by researchers. However, failures may occur when a network is put to use. Latifi [1] introduced the conditionally faulty model. A network is a g-conditionally faulty model if each vertex of the network is incident to at least g fault-free neighbors, where $g\ge 1$. Many fault-tolerant embeddings in conditionally faulty networks are being investigated. We will use $F_v^{\prime }$ and $F_e^{\prime }$ to denote the set of faulty vertices and faulty edges in $Q_n$. Hsieh and Kuo [3] proved that a fault-free cycle of length $2^n-2\left|F_v^{\prime }\right|$ can be embedded in $Q_n$ when $\left|F_e^{\prime }\right|\le n-2$ and $\left|F_v^{\prime }\right|+\left|F_e^{\prime }\right|\le 2n-4$. Hsieh and Chang [4] showed that $Q_n(n\ge 3)$ contains a fault-free cycle of length at least $2^n-2\left|F_v^{\prime }\right|$ with $\left|F_e^{\prime }\right|\le 2n-5$, and $\left|F_v^{\prime }\right|+\left|F_e^{\prime }\right|\le 2n-4$, in which each node is incident to at least two critical edges. We define $F{F}_v$ and $F{F}_e$ as the set of faulty vertices and faulty edges in $F{Q}_n$. Kuo [17] studied $F{Q}_n$, indicating that when $\left|F{F}_v\right|+\left|F{F}_e\right|\le n-1$, $F{Q}_n-F{F}_e-F{F}_v$ has a fault-free even cycle with length from 4 to $2^n-2\left|F{F}_v\right|$, where $n\ge 3$, while also having a fault-free odd cycle with length from $n+1$ to $2^n-2\left|F{F}_v\right|-1$, where $n(\ge 4)$ is even. Kuo and Hsieh [19] studied the conditionally faulty $F{Q}_n$ with $\left|F{F}_e\right|\le 2n-3$, and proved that $F{Q}_n-F{F}_e$ has a fault-free even cycle with length from 4 to $2^n$ and also has a fault-free odd cycle with length from $n+1$ to $2^n-1$ when $n(\ge 2)$ is even. Kuo et al. [18] proved that under the condition that each vertex connects at least four fault-free neighbors, for $n\ge 3$, $F{Q}_n-F{F}_v$ has a fault-free even cycle with length from 4 to $2^n-2\left|F{F}_v\right|$, where $\left|F{F}_v\right|\le 2n-5$; for even $n\ge 4$, it has a fault-free odd cycle with length from $n+1$ to $2^n-2\left|F{F}_v\right|-1$, where $\left|F{F}_v\right|\le 2n-5$. We represent with $\{f_1,f_2\}$ the set of a pair of adjacent faulty vertices in $F{Q}_n$. Kuo et al. [20] showed that in $F{Q}_n-\{f_1,f_2\}$, every fault-free edge lies on an even cycle with length from 4 to $2^n-4$ for $n\ge 3$; it also lies on an odd cycle with length from $n+1$ to $2^n-3$ for even $n\ge 4$. We will use $F_v$ and $F_e$ to represent the set of faulty vertices and faulty edges in $Q_{n,k}(1\le k\le n-1)$. Liu et al. [13] considered $Q_{n,k}(1\le k\le n-1)$ with $\left|F_e\right|\le 2n-3$ under the conditionally faulty model and proved that $Q_{n,k}-F_e$ contains a fault-free cycle of every even length from 4 to $2^n$ when $n(\ge 3)$ and k have the same parity; also, $Q_{n,k}-F_e$ contains a fault-free cycle of every odd length from $n-k+2$ to $2^n-1$ when $n(\ge 2)$ and k have different parity. Liu et al. [14] also considered $Q_{n,k}(1\le k\le n-1)$ with $\left|F_v\right|+\left|F_e\right|\le 2n-4$, $\left|F_e\right|\le n-1$, and $n\ge 3$, showing that there is a cycle with length $2^n-2\left|F_v\right|$ in $Q_{n,k}-F_e-F_v$. Due to the above motivation, in this paper, I completely improve [19] and partially improve [11] odd cycle embedding and consider the cycle-embedding properties in the conditionally faulty enhanced hypercube. For $n(\ge 3)$ and k having different parity, if $\left|F_v\right|+\left|F_e\right|\le 2n-5$, then it is proved that $Q_{n,k}-F_v-F_e$ contains a fault-free cycle of every odd length from $n-k+4$ to $2^n-2\left|F_v\right|-1$.

This paper is composed of four sections: In Section 2, I present and explore some basic terminology and lemmas related to hypercubes, i-partitions, and automorphism. The construction of the desired odd cycles is proposed in Section 3. Particularly, Section 3 focuses on the odd cycle embedding in the conditionally faulty $Q_{n,k}$, with $n\ge 5$ and k having different parity, when $\left|F_v\right|+\left|F_e\right|\ge 2n-5$, $\left|F_e\right|\ge 1$, or $\left|F_v\right|\le 2n-5$. Finally, Section 4 presents some conclusions and future work.

2. Preliminaries

For graph definitions and terminology not mentioned here, see [21]. A connected graph $G(V,E)$ can be used to model a network, where V denotes the vertex set of the graph G, and E is called the edge set of the graph G. Let the graph $G^{\prime }(V^{\prime },E^{\prime })$ be a subgraph of $G(V,E)$ if $V^{\prime }\subseteq V$ and $E^{\prime }\subseteq E$. If $V^{\prime }\subseteq V$ and $E^{\prime }\{(u,v)\in E:u,v\in V^{\prime }\}$, then we that say the graph $G^{\prime }(V^{\prime },E^{\prime })$ is a subgraph of $G(V,E)$ induced by $V^{\prime }$. We represent a mapping f from $V\left(G_1\right)$ to $V\left(G_2\right)$ such that $(u,v)\in E\left(G_1\right)$ if and only if $(f\left(u\right),f\left(v\right))\in E\left(G_2\right)$; then, we call the two graphs $G_1$ and $G_2$ isomorphic, denoted by $G_1\cong G_2$. We call x and y adjacent if $(x,y)\in E$. We use a sequence of adjacent vertices, $P[v_0,v_m]=[v_0,v_1,v_2,\dots ,v_m]$, to represent a path with the original vertex $v_0$ and end vertex $v_m$. The path is a cycle when $v_0=v_m$.

The n-dimensional hypercube can be denoted by $Q_n$, with $V\left(Q_n\right)=\left\{\right|u=x_1{x}_2\dots x_n,x_i$$=0$ or $1\}$ and $E\left(Q_n\right)=\left\{(u,v)\right|u=x_1{x}_2\dots x_{j-1}{x}_j{x}_{j+1}\dots x_n,v=y_1{y}_2\dots y_{j-1}{\overline{y}}_j{y}_{j+1}\dots y_n,1$$\le j\le n\}$. Clearly, $\left|V\left(Q_n\right)\right|=2^n$, $\left|E\left(Q_n\right)\right|=n·2^{n-1}$. We denote the ith dimensional edge set by $E_i=\left\{(u,v)\right|u=x_1{x}_2\dots x_{i-1}{x}_i{x}_{i+1}\dots x_n,v=y_1{y}_2\dots y_{i-1}{\overline{y}}_i{y}_{i+1}\dots y_n,1\le i\le n\}$. We use $d_G(u,v)$ to denote the distance between u and v, which corresponds to the shortest path between u and v in G.

Tzeng and Wei [9] proposed the enhanced hypercube $Q_{n,k}(1\le k\le n-1)$, which can be constructed from $Q_n$ by adding complementary edges. Let $E_c$ be the complementary edge set, given as $E_c=\left\{(u,\overline{u})\right|u=x_1{x}_2\dots x_{k-1}{x}_k{x}_{k+1}\dots x_n,\overline{u}=x_1{x}_2\dots x_{k-1}{\overline{x}}_k{\overline{x}}_{k+1}\dots {\overline{x}}_n,$$1\le k\le n-1\}$, where ${\overline{x}}_k$ is the complement of $x_k$—i.e., ${\overline{x}}_k=1-i$ iff $x_k=i$ for $i\in \{0,1\}$. Clearly, $V\left(Q_{n,k}\right)=V\left(Q_n\right)$ and $E\left(Q_{n,k}\right)=E\left(Q_n\right)\cup E_c$.

An automorphism of a graph G is a permutation $\sigma$ on $V\left(G\right)$ such that $(u,v)\in E\left(G\right)$ if and only if $\left(\sigma \right(u),\sigma (v\left)\right)\in E\left(G\right)$ for $\forall u,v\in V\left(G\right)$. For an undirected graph G, if for every pair of vertices $u,v\in V\left(G\right)$, there exists an automorphism of G that maps u to v, we say that the graph G is vertex-transitive, also known as vertex-symmetric. A graph G is edge-transitive if for any $(x,y),(u,v)\in E\left(G\right)$, there exists an automorphism $\sigma \in Aut\left(G\right)$ such that $\left\{\sigma \right(x),\sigma (y\left)\right\}=\{u,v\}$. It is well known that hypercubes are vertex-transitive and edge-transitive. However, enhanced hypercubes are vertex-transitive but not edge-transitive [22].

Definition 1

([13]). For $1\le k\le n-1$, an i-partition on $Q_{n,k}$, partitions $Q_{n,k}$ along dimension i to form two $(n-1)$-dimensional cubes, where $1\le i\le n$:

(i)

For $k\le i\le n$, $1\le k\le n-1$, we partition $Q_{n,k}(1\le k\le n-1)$ into two $(n-1)$-dimensional hypercubes $Q_{n-1}^L$ and $Q_{n-1}^R$, where $Q_{n-1}^L$(respectively, $Q_{n-1}^R$) is the subgraph of $Q_{n,k}$ induced by $V^{\prime }\subseteq V\left(Q_{n,k}\right)$, $V^{\prime }=\left\{v\right|v=x_1{x}_2\dots x_k\dots x_{i-1}0x_{i+1}\dots x_n\}$(respectively, $V^{\prime }=\left\{v\right|v=x_1{x}_2\dots x_k\dots x_{i-1}1x_{i+1}\dots x_n\}$). Thus, $E\left(Q_{n,k}\right)=E\left(Q_{n-1}^L\right)\cup E\left(Q_{n-1}^R\right)\cup E_c\cup E_i$.

(ii)

For $1\le i\le k-1$, $2\le k\le n-1$, we partition $Q_{n,k}(1\le k\le n-1)$ into two $(n-1)$-dimensional enhanced hypercubes $Q_{n-1,k-1}^L$ and $Q_{n-1,k-1}^R$, where $Q_{n-1,k-1}^L$(respectively, $Q_{n-1,k-1}^R$) is the subgraph of $Q_{n,k}$ induced by $V^{\prime }\subseteq V\left(Q_{n,k}\right)$, $V^{\prime }=\left\{v\right|v=x_1{x}_2\dots x_k\dots x_{i-1}$$0x_{i+1}\dots x_n\}$(respectively, $V^{\prime }=\left\{v\right|v=x_1{x}_2\dots x_k\dots x_{i-1}1x_{i+1}\dots x_n\}$). Thus, $E\left(Q_{n,k}\right)=E\left(Q_{n-1,k-1}^L\right)\cup E\left(Q_{n-1,k-1}^R\right)\cup E_i$.

Lemma 1

([14]). For $1\le k\le n-1$, let σ be an automorphism of $Q_{n,k}$ such that $\sigma \left(E_i\right)=E_j$ for any $i,$$j\in \{1,2,\dots ,n,c\}$. If $i\in \{1,2,\dots ,k-1\}$, then $Q_{n,k}-E_i=Q_{n-1,k-1}^L\cup Q_{n-1,k-1}^R$ is disconnected. If $i\in \{k,k+1,\dots ,n,c\}$, then $Q_{n,k}-E_i=Q_{n-1}^L\cup Q_{n-1}^R\cup E_c$.

Lemma 2

([5]). Let u and v be any two different vertices in $Q_n$, where $n\ge 2$. There exists a path of length l joining u and v with $d_{Q_n}(u,v)\le l\le 2^n-1$ and $2\left|\right(l-d_H(u,v))$.

Lemma 3

([6]). For $\left|F_e^{\prime }\right|+\left|F_v^{\prime }\right|\le n-2$ and $n\ge 3$, let u and v be any two distinct fault-free vertices in $Q_n$. Then, $Q_n-F_v^{\prime }-F_e^{\prime }$ contains a fault-free path $P[u,v]$ with length l, $d_{Q_n}(u,v)+2\le l\le 2^n-2\left|F_v^{\prime }\right|-1$ and $2\left|\right(l-d_{Q_n}(u,v))$.

Lemma 4

([7,23]). For $\left|F_e^{\prime }\right|+\left|F_v^{\prime }\right|\le n-2$ and $n\ge 3$, every fault-free edge of $Q_n$ lies on a fault-free even cycle with length l, $4\le l\le 2^n-2\left|F_v^{\prime }\right|$.

Lemma 5.

If $\left|F_e\right|+\left|F_v\right|\le n-2$, $1\le k\le n-1$ and $n\ge 3$, then every fault-free edge of $Q_{n,k}$ lies on a fault-free even cycle with length l, $4\le l\le 2^n-2\left|F_v\right|$.

Proof. 

Definition 1 indicates that $E\left(Q_{n,k}\right)=E\left(Q_n\right)\cup E_c$. We give e as a fault-free edge in $Q_{n,k}$:

(i)

$e\in E\left(Q_n\right)$. Lemma 4 ensures that the result is true.

(ii)

$e\in E_c$. By Lemma 1, for any arbitrary edge $e^{\prime }\in E\left(Q_n\right)$, we have an automorphism $\sigma \in Aut\left(Q_{n,k}\right)$ such that $\sigma \left(e^{\prime }\right)=e$. Since $e=\sigma \left(e^{\prime }\right)\in E\left(\sigma \left(Q_n\right)\right)$ and $\sigma \left(Q_n\right)$ is isomorphic to $Q_n$, $V\left(\sigma \left(Q_n\right)\right)=V\left(Q_n\right)=V\left(Q_{n,k}\right)$. Lemma 4 indicates that $e\in E(\sigma \left(Q_n\right)-F_v)$ lies on a fault-free cycle of every even length from 4 to $2^n-2\left|F_v\right|$.

By the above cases, the lemma holds. □

Lemma 6

([24]). For $n\ge 3$ and $\left|F_v^{\prime }\right|+\left|F_e^{\prime }\right|\le 2n-5$, every fault-free edge of the conditionally faulty $Q_n-F_v^{\prime }-F_e^{\prime }$ lies on a even cycle with length l, $6\le l\le 2^n-2\left|F_v^{\prime }\right|$.

Lemma 7

([7]). For $\left|F_e^{\prime }\right|\le n-2$, $\left|F_v^{\prime }\right|+\left|F_e^{\prime }\right|\le 2n-4$, and $n\ge 5$, $Q_n$ contains a fault-free even cycle with length from 4 to $2^n-2\left|F_v^{\prime }\right|$.

Lemma 8

([17]). If $\left|F{F}_v\right|+\left|F{F}_e\right|\le n-1$ and $n\ge 4$ is even, then $F{Q}_n-F{F}_v-F{F}_e$ contains a fault-free odd cycle with length l, $n+1\le l\le 2^n-2\left|F{F}_v\right|-1$.

3. Odd Cycle Embedding in Conditionally Faulty ${\mathit{Q}}_{\mathit{n},\mathit{k}}$

Firstly, we show two basic lemmas for $Q_{3,2}$ and $Q_{4,k}$, $k\in \{1,3\}$, which will be used as the basic cases of the proof of Theorem 1.

Lemma 9.

In conditionally faulty $Q_{3,2}$, if $\left|F_v\right|+\left|F_e\right|\le 1$, then we will find the fault-free odd cycles with length $3\le l\le 7-2|F_v|$.

Proof. 

In conditionally faulty $Q_{3,2}$, if $\left|F_v\right|+\left|F_e\right|=0$, the odd cycle with length $3\le l\le 7$ can be easily found.

If $\left|F_v\right|=1$ and $\left|F_e\right|=0$, by the vertex-transitivity of $Q_{3,2}$, we can assume that the vertex 000 is faulty. Let 000 be a faulty vertex. Thus, (010, 011, 001, 010), (001, 011, 111, 110, 010, 001), and (001, 011, 111, 101, 100, 110, 010, 001) form the desired cycles of lengths 3, 5, and 7 respectively.

If $\left|F_v\right|=0$ and $\left|F_e\right|=1$, by the edge-transitivity of $Q_{3,2}$, we hypothesize that the faulty edge is the 1-dimensional edge $(000,100)$, the 2-dimensional edge (000, 010), the 3-dimensional edge (000, 001), or the complementary edge (000, 011). We can construct the odd cycle with lengths 3, 5, and 7, respectively, the same as above. □

Lemma 10.

In conditionally faulty $Q_{4,k}(k\in \{1,3\})$, $\left|F_v\right|+\left|F_e\right|\le 3$, $Q_{n,k}-F_v-F_e$ contains fault-free odd cycles with length l, $n-k+2\le l\le 15-2\left|F_v\right|$.

Proof. 

By Lemma 8, $Q_{4,1}=F{Q}_4$ has a fault-free odd cycle with length $5\le l\le 15-2\left|F_v\right|$ when $\left|F_v\right|+\left|F_e\right|\le 3$. Now, we consider $Q_{4,3}$. If $\left|F_v\right|+\left|F_e\right|\le 2$, it is easy to construct the fault-free cycle of every odd length from 3 to $15-2\left|F_v\right|$. We suppose that $\left|F_v\right|+\left|F_e\right|=3$ in the following. Definition 1 indicates that we can partition $Q_{4,3}$ along an i-dimensional faulty edge, $i\in \{1,2,3,4,c\}$; then, it forms two subcubes satisfying that $Q_{n,k}-E_i$ is conditionally faulty.

Case 1. $\left|F_e\right|\ge 1$.

Case 1.1. $Q_{4,3}=Q_{3,2}^L\cup Q_{3,2}^R\cup E_i$, $i\in \{1,2\}$.

Let $F_i=E_i\cap F_e$, $F_e^L=F_e\cap Q_{3,2}^L$, $F_e^R=F_e\cap Q_{3,2}^R$, $F_v^L=F_v\cap Q_{3,2}^L$, and $F_v^R=F_v\cap Q_{3,2}^R$, for $i\in \{1,2\}$, where $E_i$ denotes the set of hypercube edges between $Q_{3,2}^L$ and $Q_{3,2}^R$. Since $Q_{4,3}-E_i\cong Q_{3,2}^L\cup Q_{3,2}^R$ is conditionally faulty, it follows that every vertex in $Q_{3,2}^L$ (resp. $Q_{3,2}^R$) connects at least two fault-free edges of $Q_{3,2}^L$ (resp. $Q_{3,2}^R$). It follows that $\left|F_i\right|\ge 1$, $\left|F_v^L\right|+\left|F_e^L\right|\le 2$, and $\left|F_v^R\right|+\left|F_e^R\right|\le 2$. Let us suppose that $\left|F_v^L\right|+\left|F_e^L\right|\le \left|F_v^R\right|+\left|F_e^R\right|$; then, $\left|F_v^L\right|+\left|F_e^L\right|\le \lfloor {\displaystyle \frac{3-1}{2}}\rfloor =1$, and $\left|F_v^R\right|+\left|F_e^R\right|\le 2$.

Case 1.1.1. $\left|F_v^R\right|+\left|F_e^R\right|\le 1$.

Note that $\left|F_i\right|\ge 1$ and $\left|F_v^L\right|+\left|F_e^L\right|\le 1$. Since $\left|F_v^R\right|+\left|F_e^R\right|\le 1$ and every vertex is incident to at least two fault-free vertices, by Lemma 9, there exists a fault-free cycle $C_R$ of every odd length from 3 to $7-2\left|F_v^1\right|$ in $Q_{3,2}^R-F_v^R-F_e^R$. Since $\left|F_v^L\right|+\left|F_e^L\right|+\left|F_i\right|\le 2$, we can always find an edge $(a,b)$ on cycle $C_R$ such that the edges in $\{(a,a^i),(b,b^i),(a^i,b^i)\}$ are all fault-free. Since $d_H(a^i,b^i)=1$, $\left|F_v^L\right|+\left|F_e^L\right|\le 1$, and $Q_{3,2}^L-E_c^L\cong Q_3$, by Lemma 3, there exists a fault-free path $P_L[a^i,b^i]$ of length from 3 to $7-2\left|F_v^0\right|$ in $Q_{3,2}^{i0}$, where $E_c^L$ is the set of complementary edges in $Q_{3,2}^L$. Note that the edge $(a^i,b^i)$ is fault-free. We have $1\le P_L[a^i,b^i]=l_0\le 7-2\left|F_v^0\right|$. $P_L[a^i,b^i]+(b^i,b)+C_R-(b,a)+(a,a^i)$ forms the desired cycle of every odd length with length $5\le l\le 15-2\left|F_v\right|$.

Case 1.1.2. $\left|F_v^R\right|+|F_e^R|=2$, i.e., $\left|F_i\right|=1$ and $\left|F_v^L\right|+\left|F_e^L\right|=0$.

If $\left|F_e^R\right|=2$ and $\left|F_i\right|=1$, we find an edge $(x,y)$ in $Q_{3,2}^R-F_e$ satisfying that $\{(x,x^i),(y,y^i),(x^i,$$y^i\left)\right\}\cap F_e=\varnothing$. Let us say that the edge $(x,y)$ is fault-free temporarily. Thus, the number of faulty elements is 1. Similarly to Case 1.1.1, we take $(x,y)$ instead of the edge $(a,b)$ in Case 1.1.1. If $\left|F_e^R\right|=1$ and there exists the faulty edge $(x,y)$ in $Q_{3,2}^R$ such that the edges in $\{(x,x^i),(y,y^i),(x^i,y^i)\}$ are all fault-free, the rest of the proof of this case is similar to Case 1.1.1. If either $\left|F_e^R\right|=1$ and the edges in $\{(x,x^i),(y,y^i),(x^i,y^i)\}$ are not all fault-free when $(x,y)$ is a faulty edge in $Q_{3,2}^R$ or $\left|F_v^R\right|=2$ and $\left|F_e^R\right|=0$, we will find the desired odd fault-free cycle as follows: Since $\left|F_i\right|=1$, by Lemma 9, we hypothesize that a vertex $x\in V\left(Q_{3,2}^R\right)\cap F_v$ is fault-free temporarily such that $C_R$ is a fault-free odd cycle with length from 3 to $7-2\left(\right|F_v^R|-1)$ containing the vertex x and $\{(z,z^i),(w,w^i),z^i,w^i\}\cap (F_v\cup F_e)=\varnothing$, where z and w are two adjacent vertices on the cycle $C_R$ in $Q_{3,2}^R-F_v^R-F_e^R+\left\{x\right\}$. Since $d_H(z^i,w^i)=2$ and $\left|F_v^L\right|+\left|F_e^L\right|=0$, by Lemma 2, $P_L[z^i,w^i]$ is a path with length $2\le l_L\le 6-2\left|F_v^L\right|$ in $Q_{3,2}^L$. $P_L[z^i,w^i]+(w^i,w)+C_R-(x,w)-(x,z)+(z,z^i)$ is an odd cycle with length $l=l_0+l_1+2$, $5\le l\le 15-2\left|F_v\right|$ in $Q_{4,3}$.

Case 1.2. $Q_{4,3}=Q_3^L\cup Q_3^R\cup E_c\cup E_i$, $i\in \{3,4,c\}$.

Let $F_i=E_i\cap F_e$, $F_e^L=F_e\cap Q_3^L$, $F_e^R=F_e\cap Q_3^R$, $F_v^L=F_v\cap Q_3^L$, and $F_v^R=F_v\cap Q_3^R$, for $i\in \{1,2\}$, where $E_i$ denotes the set of hypercube edges between $Q_3^L$ and $Q_3^R$. Then, we have $\left|F_i\right|\ge 1$, $\left|F_v^L\right|+\left|F_e^L\right|\le 2$ and $\left|F_v^R\right|+\left|F_e^R\right|\le 2$. Without loss of generality, we hypothesize that $\left|F_v^L\right|+\left|F_e^L\right|\le \left|F_v^R\right|+\left|F_e^R\right|$. Then, we have $\left|F_v^L\right|+\left|F_e^L\right|\le \lfloor {\displaystyle \frac{3-1}{2}}\rfloor =1$ and $\left|F_v^R\right|+\left|F_e^R\right|\le 2$.

Case 1.2.1. $\left|F_v^R\right|+\left|F_e^R\right|\le 1$.

Since $\left|F_v^R\right|+\left|F_e^R\right|\le 1$, by Lemma 4, $C_R$ is a fault-free even cycle with length $4\le l\le 8-2\left|F_v^R\right|$ in $Q_3^R-F_v^R-F_e^R$. Let us recall that $1\le \left|F_i\right|\le 3$ and $\left|E_i\right|+\left|E_c\right|=16$; we will select an edge $(a,b)$ on cycle $C_R$ satisfying that $\{(a,a^i),(b,\overline{b})\}\cap F_e=\varnothing$ (resp. $\{(a,\overline{a}),(b,b^i)\}\cap F_e=\varnothing$) and $d_H(a^i,\overline{b})=n-k-1=0$ (resp. $d_H(\overline{a},b^i)=n-k-1=0$) and choose an edge $(c,d)$ on cycle $C_R$ satisfying that $\{(c,c^i),(d,\overline{d}),c^i,\overline{d}\}\cap (F_v\cup F_e)=\varnothing )$ (resp. $\{(c,\overline{c}),(d,d^i),\overline{c},d^i\}\cap (F_v\cup F_e)=\varnothing )$ and $d_H(c^i,\overline{d})=n-k+1=2$. $P_R[a,\overline{a}]+(\overline{a},a^i)+(a^i,a)$ can form the desired cycle with lengths 3 and 5. Since $d_H(c^i,\overline{d})=2$ and $\left|F_v^L\right|+\left|F_e^L\right|\le 1$, by Lemma 3, there exists a fault-free path $P_L[c^i,\overline{d}]$ with length $4\le l_L\le 6-2\left|F_v^L\right|$ in $Q_3^L-F_v^L-F_e^L$. $P_L[c^i,\overline{d}]+(\overline{d},d)+C_R-(d,c)+(c,c^i)$ is an odd cycle with length $7\le l\le 15-2\left|F_v\right|$ in $Q_{4,3}$. Thus, $3\le l\le 15-2\left|F_v\right|$.

Case 1.2.2. $\left|F_v^R\right|+\left|F_e^R\right|=2$, i.e., $\left|F_i\right|=1$ and $\left|F_v^L\right|+\left|F_e^L\right|=0$.

If $\left|F_e^R\right|\ge 1$, we find a faulty edge $(x,y)$ and assume that $(x,y)$ is fault-free temporarily. Thus, $|(V\left(Q_3^R\right)\cup E\left(Q_3^R\right))\cap (F_v\cup F_e)|=1$. Since $\left|F_i\right|=1$ and $|F_v^L|+\left|F_e^L\right|=0$, the edges in $\{(x,x^i),(y,\overline{y}),x^i,\overline{y}\}$ or $\{(x,\overline{x}),(y,y^i),\overline{x},y^i\}$ are all fault-free. Similarly to Case 1.2.1, we take $(x,y)$ instead of the edges $(a,b)$ and $(c,d)$ in Case 1.2.1. If $\left|F_e^R\right|=0$, then $\left|F_v^R\right|=2$. We find a vertex x in $V\left(Q_3^R\right)\cap F_v$ and say that it is a fault-free vertex temporarily such that $C_R$ is an even cycle with length from 4 to $8-2\left(\right|F_v^R|-1)$ containing the vertex x and $\{(w,\overline{w}),(z,z^i))\}\cap F_e=\varnothing$ (resp. $\{(w,w^i),(z,\overline{z})\}$), where z and w are two adjacent vertices of x on cycle $C_R$ in $Q_3^R-F_v^R-F_e^R+\left\{x\right\}$. Since $\left|F_v^L\right|+\left|F_e^L\right|=0$ and $d_H(\overline{w},z^i)=1$ in $Q_3^L$, by Lemma 2, $P_L[\overline{w},z^i]$ is a path with length $1\le l_0\le 7-2\left|F_v^L\right|$ in $Q_3^L$. Hence, $P_L[\overline{w},z^i]+(z^i,z)+C_R-(z,w)+(w,\overline{w})$ forms the cycle with length $5\le l\le 17-2\left|F_v\right|$ in $Q_{4,3}$. Note that the fault-free cycle of length 3 in $Q_{4,3}$ is easy to find. Finally, $3\le l\le 15-2\left|F_v\right|$.

Case 2. $\left|F_e\right|=0$. i.e., $\left|F_v\right|=3$.

We choose two arbitrary faulty vertices which differ at the j-th position, where $j\in \{1,2,3,4\}$. We partition $Q_{4,3}$ along dimension j such that every 3-dimensional cube has at least one faulty vertex. If $Q_{4,3}=Q_{3,2}^L\cup Q_{3,2}^R\cup E_j$, the rest of the proof of this case is similar to that of the last part of Case 1.1.2. Similarly to Case 1.2.2, we can also find the odd cycles in $Q_{4,3}=Q_3^L\cup Q_3^R\cup E_j\cup E_c$.

In summary, the lemma is true. □

Now, we consider the embedding of odd cycles in conditionally faulty $Q_{n,k}$ when $n\ge 5$ and k have different parity, $F_e\ne \varnothing$, and $\left|F_v\right|+\left|F_e\right|\le 2n-5$. We first give a proof of the odd cycles in $Q_{5,k}$, $k\in \{2,4\}$, as follows.

Corollary 1.

In the conditionally faulty $Q_{5,k}$, $k\in \{2,4\}$, if $\left|F_e\right|=\left|F_i\right|+\left|F_c\right|=1$ and $\left|F_v\right|=4$, then there exists a fault-free odd cycle with length from $n-k+4$ to $2^5-2\left|F_v\right|-1$.

Proof. 

Since $\left|F_v^L\right|=2n-6=4$ for $n=5$ and $\left|F_i\right|+\left|F_c\right|=1$, we find two faulty vertices $x=x_1{x}_2\dots x_n$ and $y=y_1{y}_2\dots y_n$ which are different exactly ar the jth position, that is, $x_j+y_j=1$, where $j\in \{1,2,\dots ,5\}$, $j\ne i$. Now, we can partition $Q_{5,k}(k\in \{2,4\})$ along dimension j into two subcubes such that each subcube has at least one faulty vertex and $\left|F_j\right|=0$, where $|F_j|$ is the number of faulty edges between the two subcubes (see

Table 1. Cycle construction in the proof of Corollary 1 when $\left|F_e\right|=\left|F_i\right|+\left|F_c\right|=1$ and $\left|F_v\right|=4$ in $Q_{5,k}$, $k\in \{2,4\}$.

Cases	Distribution of Faulty Elements	Construction of Desired Cycles	Length l of Odd Cycles
$n=5$, $Q_{5,k}=Q_{4,k-1}^L\cup Q_{4,k-1}^R\cup E_j$,               $n=5$, $Q_{5,k}=Q_4^L\cup Q_4^R\cup E_j\cup E_c$	$\left|F_v^R\right|=3$, $\left|F_e^R\right|=1$ and $\left|F_v^L\right|=1$,           $\left|F_v^R\right|+F_e^R|=3$ and $\left|F_v^L\right|+\left|F_e^L\right|=2$,     $\left|F_v^R\right|=3$, $\left|F_e^R\right|=1$ and $\left|F_v^L\right|=1$, $\left|F_v^R\right|+F_e^R|=3$ and $\left|F_v^L\right|+\left|F_e^L\right|=2$	$C=P_L[a^j,b^j]+(b^j,b)+C_R[b,a]-(a,b)+(a,a^j)$, $C=P_L[z^j,w^j]+(w^j,w)+C_R[w,z]-(x,w)-(x,z)+(z,z^j)$.           $C=P_L[a^j,\overline{b}]+(\overline{b},b)+P_R[b,a]-(a,b)+(a,a^j)$ $C=P_L[a^j,\overline{a}]+(\overline{a},a)+(a,a^j)$;	$2^4-2|F_v^R|+3\le l=2^5-2\left|F_v\right|-1$, $2^4-2|F_v^R|+5\le l\le 2^5-2\left|F_v\right|-1$.     $n-k+2\le l\le 2^5-2\left|F_v\right|-1$,     $n-k+8\le l\le 2^5-2\left|F_v\right|-1$, $l=n-k+4$ and $l=n-k+6$ $n-k+4\le l\le 2^5-2\left|F_v\right|-1$.

).

• $Q_{5,k}=Q_{4,k-1}^L\cup Q_{4,k-1}^R\cup E_j$, $j\in \{1,2,\dots ,k-1\}$, $k\in \{2,4\}$.
  Let $F_v^L=F_v\cap V\left(Q_{4,k-1}^L\right)$, $F_e^L=F_e\cap E\left(Q_{4,k-1}^L\right)$ (resp. $F_v^R=F_v\cap V\left(Q_{4,k-1}^R\right)$, and $F_e^R=F_e\cap E\left(Q_{4,k-1}^R\right)$). We hypothesize that $\left|F_v^L\right|+\left|F_e^L\right|\le \left|F_v^R\right|+\left|F_e^R\right|$. Thus, $1\le \left|F_v^L\right|+\left|F_e^L\right|\le 2$ and $1\le \left|F_v^R\right|+\left|F_e^R\right|\le 4$. Since $\left|F_v^L\right|+\left|F_e^L\right|\le 2$, by Lemma 10, $C_L$ is a fault-free odd cycle with length $n-k+2\le l\le 2^4-2\left|F_v^L\right|-1$ in $Q_{4,k-1}^L$. Since every subcube has at least one faulty vertex and $\left|F_v\right|+\left|F_e\right|=5$, there exist at most three faulty vertices in $Q_{4,k-1}^R$ when $\left|F_v^R\right|+\left|F_e^R\right|=4$. Note that every vertex in $Q_{4,k-1}^L$ (resp. $Q_{4,k-1}^R$) connects at least two fault-free edges of $Q_{4,k-1}^L$ (resp. $Q_{4,k-1}^R$).

  (i)

  If $\left|F_v^R\right|=3$, $\left|F_e^R\right|=1$, and $\left|F_v^L\right|=1$, we will find an odd cycle with length from $2^4-2\left|F_v^L\right|+1$ to $2^5-2\left|F_v\right|-1$. The following apply: On one hand, if $(a,b)\in Fe\cap E\left(Q_{4,k-1}^R\right)$ and $\{a^j,b^j\}\cap F_v=\varnothing$, let us say that the edge $(a,b)$ is fault-free temporarily. Thus, $|(V\left(Q_{4,k}^R\right)\cup E\left(Q_{4,k}^R\right))\cap (F_e\cup F_v)|=3$. By Lemma 10, there exists a fault-free cycle $C_R$ containing the edge $(a,b)$ with odd length $2^4-2\left|F_v^R\right|-1$ in $Q_{4,k-1}^R+\left\{(a,b)\right\}-F_v^R-F_e^R$. Note that $d_H(a^j,b^j)=1$. As $\left|F_v^L\right|+\left|F_e^L\right|=1$ and $Q_{4,k-1}^L-E_c^L\cong Q_4$, Lemma 3 indicates that $P_L[a^j,b^j]$ is a path with odd length from 3 to $2^4-2\left|F_v^L\right|-1$. Hence, $P_L[a^j,b^j]+(b^j,b)+C_R[b,a]-(a,b)+(a,a^j)$ is an odd cycle with length $2^4-2\left|F_v^R\right|+3\le l\le 2^5-2\left|F_v\right|-1$. Since $\left|F_v^L\right|=3$ and $\left|F_v^R\right|=1$, it follows that $2^4-2\left|F_v^L\right|-1=2^4-2\left|F_v^R\right|+3$. Therefore, $n-k+2\le l\le 2^5-2\left|F_v\right|-1$. On the other hand, if $(a,b)\in F_e\cap E\left(Q_{4,k-1}^R\right)$ and $|\{a^j,b^j\}\cap F_v|=1$, we choose a vertex $x\in V\left(Q_{4,k-1}^R\right)\cap F_v$ and say that it is fault-free temporarily such that $\{z^j,w^j\}\cap F_v=\varnothing$ when there exists a cycle $C_R$ with length $2^4-2\left(\right|F_v^R|-1)-1$ containing the vertex x by Lemma 10, and the vertices z and w are adjacent to x on cycle $C_R$ in $Q_{4,k-1}^R+\left\{x\right\}-F_v^R-F_e^R$. Note that $d_H(z^j,w^j)=2$. Since $\left|F_v^L\right|+\left|F_e^L\right|=1$, by Lemma 3, $P_L[z^j,w^j]$ is a path with odd length $l_L$, $4\le l_L\le 2^4-2\left|F_v^l\right|-2$. Thus, $P_L[z^j,w^j]+(w^j,w)+C_R[w,z]-(x,w)-(x,z)+(z,z^j)$ is an odd cycle with length $2^4-2|F_v^R|+5\le l\le 2^5-2\left|F_v\right|-1$. Since $\left|F_v^R\right|=3$ and $\left|F_v^L\right|=1$, it follows that $2^4-2|F_v^R|+5=2^4-2\left|F_v^L\right|+1$. Therefore, $n-k+2\le l\le 2^5-2\left|F_v\right|-1$.

  (ii)

  If $\left|F_v^R\right|+\left|F_e^R\right|=3$ and $\left|F_v^L\right|+|F_e^L|=2$, we can use a similar method to the above to construct an odd cycle with length from $n-k+2$ to $2^5-2\left|F_v\right|-1$.

• $Q_{5,k}=Q_4^L\cup Q_4^R\cup E_j\cup E_c$, $j\in \{k,k+1,\dots 5\}$, $k\in \{2,4\}$.
  Let $F_v^L$ and $F_e^L$ (resp. $F_v^R$ and $F_e^R$) denote the set of faulty vertices and faulty edges in $Q_4^L$ (resp. $Q_4^R$), respectively. We hypothesize that $\left|F_v^L\right|+\left|F_e^L\right|\le \left|F_v^R\right|+\left|F_e^R\right|$. Thus, $1\le \left|F_v^L\right|+\left|F_e^L\right|\le 2$, and $1\le \left|F_v^R\right|+\left|F_e^R\right|\le 4$. Note that there exists only one faulty edge in $Q_{5,k}$ under the above condition. Hence, every vertex in $Q_4^L$ (resp. $Q_4^R$) connects at least two fault-free edges of $Q_4^L$ (resp. $Q_4^R$). The following apply:

  (i)

  If $\left|F_v^R\right|=3$, $\left|F_e^R\right|=1$, and $\left|F_v^L\right|=1$, we will find an odd cycle with length from $2^4-2\left|F_v^0\right|+1$ to $2^5-2\left|F_v\right|-1$. Let $(a,b)\in F_e\cap E\left(Q_{4,k}^R\right)$. Let us say that the edge $(a,b)$ is fault-free temporarily. Since $\left|F_v^L\right|=1$, either $\{a^j,\overline{b}\}\cap F_v=\varnothing$, or $\{\overline{a},b^j\}\cap F_v=\varnothing$. We hypothesize the former to be true. Now, the number of faulty elements in $Q_4^R$ is three, and by Lemma 7, there exists a fault-free even cycle $C_R$ containing the faulty edge $(a,b)$ whose length is from 4 to $2^4-2\left|F_v^R\right|$. Note that $d_H(a^j,\overline{b})=n-k-1$ or $d_H(a^j,\overline{b})=n-k+1$. Since $\left|F_v^L\right|=1$, by Lemma 3, $P_L[a^j,\overline{b}]$ is a path with length $n-k+3\le l_L\le 2^4-2\left|F_v^L\right|-2$ in $Q_4^L-F_v^L-F_e^L$. Thus, $P_L[a^j,\overline{b}]+(\overline{b},b)+P_R[b,a]-(a,b)+(a,a^j)$ is an odd cycle with length $n-k+8\le l\le 2^5-2\left|F_v\right|-1$. In addition, since $\left|F_v^L\right|=1$, we find a fault-free vertex $a\in V\left(Q_4^R\right)$ such that the vertices in $\{a^j,\overline{a}\}$ are both fault-free. Since $d_H(a^j,\overline{a})=n-k$, by Lemma 3, $P_L[a^j,\overline{a}]$ is a fault-free path with length $n-k+2$ and $n-k+4$ in $Q_4^L-F_v^L-F_e^l$. Hence, $P_L[a^j,\overline{a}]+(\overline{a},a)+(a,a^j)$ is an odd cycle with length $n-k+4$ and $n-k+6$. Thus, $n-k+4\le l\le 2^5-2\left|F_v\right|-1$.

  (ii)

  If $\left|F_v^R\right|+\left|F_e^R\right|=3$ and $\left|F_v^L\right|+\left|F_e^L\right|=2$, we can use a similar method to the above to find odd cycles with length from $n-k+4$ to $2^5-2\left|F_v\right|-1$.

□

Lemma 11.

In a conditionally faulty $Q_{n,k}$, if $n(\ge 5)$ and $k(1\le k\le n-1)$ have different parity, $|F_v|+|F_e|\le 2n-5$, and $\left|F_e\right|\ge 1$, then it contains a fault-free odd cycle with length l, $n-k+4\le l\le 2^n-2\left|F_v\right|-1$.

Proof. 

Since $\left|F_v\right|+|F_e|\le 2n-5$, $Q_{n,k}$ contains at most one vertex which is incident to exactly two fault-free edges. If not, $\left|F_e\right|\ge (n-1)+(n-1)-1=2n-3$, a contradiction.

Case 1. $F_e\cap E_i\ne \varnothing$, $i\in \{1,2,\dots ,k-1\}$. By Definition 1, we partition $Q_{n,k}$ along dimension $i\in \{1,2,\dots ,k-1\}$ of some faulty edge into two subcubes $Q_{n-1,k-1}^L$ and $Q_{n-1,k-1}^R$.

The proof of this case is by induction on n. By Lemma 10, the lemma is true if $n=4$. We hypothesize that the result is true for $4\le m<n$. We now consider that $m=n$.

Let $F_i=E_i\cap F_e$, $F_e^L=F_e\cap Q_{n-1,k-1}^L$, $F_e^R=F_e\cap Q_{n-1,k-1}^R$, $F_v^L=F_v\cap Q_{n-1,k-1}^L$, and $F_v^R=F_v\cap Q_{n-1,k-1}^R$, for $i\in \{1,2,\dots ,k-1\}$, where $E_i$ denotes the set of hypercube edges between $Q_{n-1,k-1}^L$ and $Q_{n-1,k-1}^R$. Then, we have $\left|F_i\right|\ge 1$, $\left|F_v^L\right|+\left|F_e^L\right|\le 2n-6$ and $\left|F_v^R\right|+\left|F_e^R\right|\le 2n-6$. Let us assume $\left|F_v^R\right|+\left|F_e^R\right|\le \left|F_v^L\right|+\left|F_e^L\right|$. It implies that $\left|F_v^R\right|+\left|F_e^R\right|\le \lfloor {\displaystyle \frac{\left(\right|F_v|+|F_e\left|\right)-|F_i|}{2}}\rfloor \le \lfloor {\displaystyle \frac{(2n-5)-1}{2}}\rfloor =n-3$ and $\left|F_v^L\right|+\left|F_e^L\right|\le 2n-6$. Since every vertex in $Q_{n,k}-E_i\cong Q_{n-1,k-1}^L\cup Q_{n-1,k-1}^R$ is incident to at least two fault-free edges, every vertex in $Q_{n-1,k-1}^L$ (respectively, $Q_{n-1,k-1}^R$) is incident to at least two fault-free edges of $Q_{n-1,k-1}^L$ (respectively, $Q_{n-1,k-1}^R$) (see

Table 2. Cycle construction in the proof of Lemma 11 when $F_e\cap E_i\ne \varnothing$, $i\in \{1,2,\dots ,k-1\}$.

Cases	Distribution of Faulty Elements	Construction of Desired Cycles	Length l of Odd Cycles
Case 1.1                   Case 1.2	$\left|F_v^L\right|+\left|F_e^L\right|\le 2n-7$               $\left|F_v^L\right|+\left|F_e^L\right|=2n-6$ $\left|F_e^L\right|\ge 2$;     $\left|F_v^L\right|\ge 2$.	$C_L$,     $C=C_L-(a,b)+(a,a^i)+(b,b^i)+(a^i,b^i)$, $C=C_L-(a,b)+(a,a^i)+(b,b^i)+C_R-(a^i,b^i)$.   $C_R$ $C=C_L-(x,y)+(x,x^i)+C_R-(x^i,y^i)+(y,y^i)$; $C=C_L-(x,w)-(x,z)+(w,w^i)+(z,z^i)+P_R[w^i,z^i]$.	$n-k+4\le l\le 2^{n-1}-2\left|F_v^L\right|-1$, $l=2^{n-1}-2\left|F_v^L\right|+1$,     $2^{n-1}-2|F_v^L|+3\le l\le 2^{n-1}-2\left|F_v\right|-1$.   $n-k+4\le l\le 2^{n-1}-1$, $2^{n-1}-2|F_v^L|+1\le l\le 2^n-2\left|F_v\right|-1$; $2^{n-1}-2|F_v^L+3|\le l\le 2^n-2\left|F_v\right|-1$.

).

Case 1.1. $\left|F_v^L\right|+\left|F_e^L\right|\le 2n-7$.

As $\left|F_v^L\right|+\left|F_e^L\right|\le 2n-7$, it implies that the conditionally faulty $Q_{n-1,k-1}^L$ has a fault-free odd cycle with length from $n-k+4$ to $2^{n-1}-2\left|F_v^L\right|-1$. Let $C_L$ be a fault-free cycle with length $2^{n-1}-2\left|F_v^L\right|-1$ in $Q_{n-1,k-1}^L$. Since $\lfloor {\displaystyle \frac{2^{n-1}-2\left|F_v^L\right|-1}{2}}\rfloor -|F_i|-|F_e^L|-|F_v^L|\ge 2^{n-2}-1-(2n-5)=2^{n-2}-(2n-4)\ge 2$ when $n\ge 5$, obviously, one can choose an edge $(a,b)$ in $C_L$ such that the edges in $\{(a,a^i),(b,b^i),(a^i,b^i)\}$ are all fault-free. Lemma 5 ensures that the edge $(a^i,b^i)$ lies on a fault-free cycle $C_R$ of every even length from 4 to $2^{n-1}-2\left|F_v^R\right|$ in $Q_{n-1,k-1}^R-F_v^R-F_e^R$. Thus, $C_L-(a,b)+(a,a^i)+(b,b^i)+(a^i,b^i)$ forms a cycle with length $2^{n-1}-2\left|F_v^L\right|+1$. 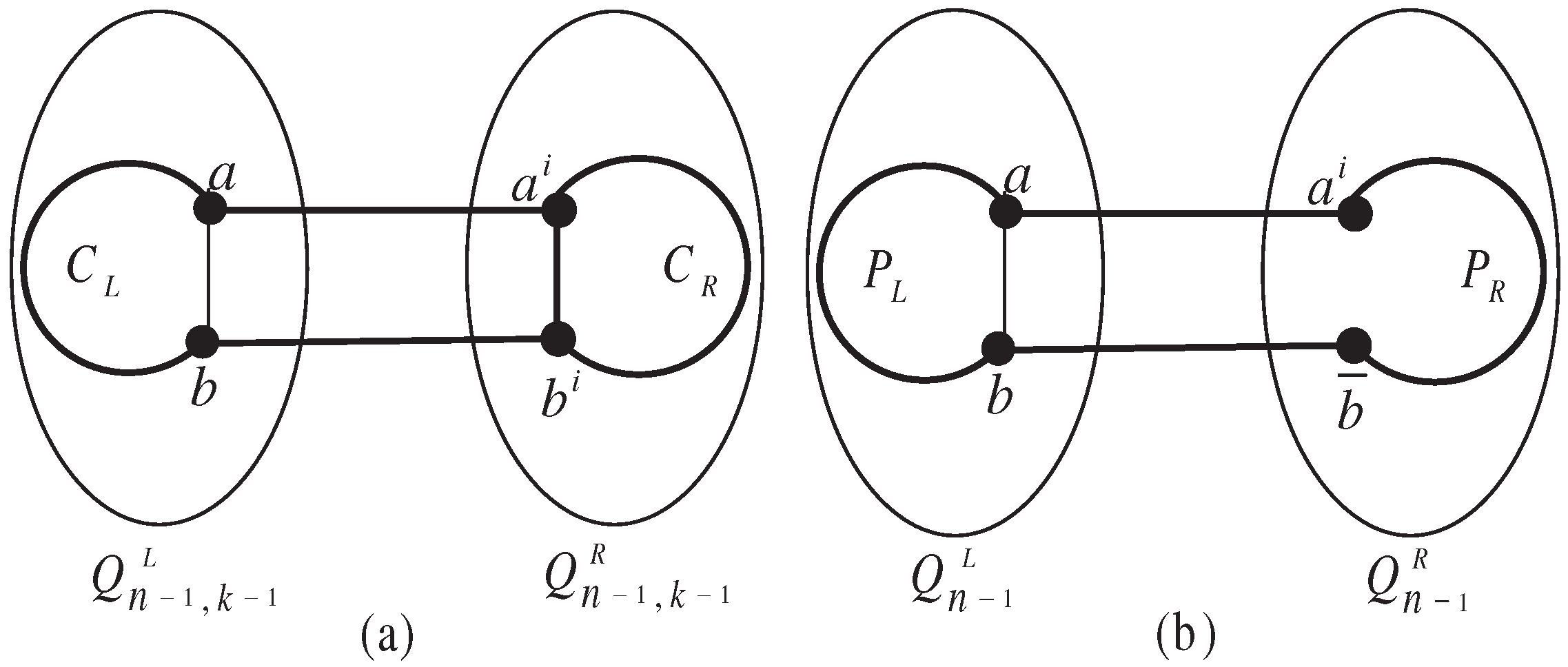$C_L-(a,b)+(a,a^i)+(b,b^i)+C_R-(a^i,b^i)$ forms a cycle whose length is from $2^{n-1}-2\left|F_v^L\right|+3$ to $2^n-2\left|F_v\right|-1$ (see Figure 1a). (In this paper, in the figures, we use the straight full line, the dotted line, and the curved line to denote the fault-free edge, the faulty edge, and the path, respectively. We use the bold lines to denote the selected desired fault-free cycle).

Case 1.2. $\left|F_v^L\right|+\left|F_e^L\right|=2n-6$.

Note that $\left|F_v^R\right|+\left|F_e^R\right|=0$. $Q_{n-1,k-1}^R$ has a fault-free odd cycle with length $n-k+4\le l\le 2^{n-1}-1$. The following apply:

• If $\left|F_e^L\right|\ge 2$, we can select a faulty edge $(x,y)\in E\left(Q_{n-1,k-1}^L\right)$ and suppose that it is fault-free temporarily such that the edges in $\{(x,x^i),(y,y^i),(x^i,y^i)\}$ are all fault-free. Thus, $Q_{n-1,k-1}^L-F_v^L-F_e^L+\left\{(x,y)\right\}$ contains a cycle $C_L$ with length $2^{n-1}-2\left|F_v^L\right|-1$ containing the edge $(x,y)$. We take $(x,y)$ instead of $(a,b)$ in Case 1.1. The rest of the construction of this case is similar to that of Case 1.1. Thus, we can construct the desired cycle as $C_L-(x,y)+(x,x^i)+(x^i,y^i)+(y,y^i)$ and $C_L-(x,y)+(x,x^i)+C_R-(x^i,y^i)+(y,y^i)$, and the length is from $2^{n-1}-2\left|F_v^L\right|+1$ to $2^n-2\left|F_v\right|-1$. Since $2^{n-1}-2\left|F_v^L\right|+1\le 2^{n-1}+1$, there exists the desired odd cycle with length l, $n-k+4\le l\le 2^n-2\left|F_v\right|-1$.
• If $\left|F_v^L\right|\ge 2$ when $\left|F_v^L\right|+\left|F_e^L\right|=2n-6\ge 4$ for $n\ge 5$, we find a faulty vertex $x\in V\left(Q_{n-1,k-1}^L\right)$, and there exists a fault-free cycle $C_L$ with odd length $2^{n-1}-2\left(\right|F_v^L|-1)-1$ containing the vertex x in $Q_{n-1,k-1}^L-F_v^L-F_e^L+\left\{x\right\}$ by the induction hypothesis, and the elements in $\{(z,z^i),(w,w^i),z^i,w^i\}$ are all fault-free, where z and w are two adjacent vertices of x on the cycle $C_L$. Since $\left|F_v^R\right|+\left|F_e^R\right|=0$ and $d_H(z^i,w^i)=2$, Lemma 2 ensures that there exists a path $P_R[z^i,w^i]$ whose length is every even integer from 2 to $2^{n-1}-2\left|F_v^1\right|-2$ in $Q_{n-1,k-1}^R$. Thus, $C_L-(x,w)-(x,z)+(w,w^i)+(z.z^i)+P_R[w^i,z^i]$ forms the desired cycle of length from $2^{n-1}-2\left|F_v^L\right|+3$ to $2^n-2\left|F_v\right|-1$ in $Q_{n,k}$. Since $|F_v^L\ge 2$, we have $2^{n-1}-2\left|F_v^L\right|+3<2^{n-1}+1$. Thus, $n-k+4\le l\le 2^n-2\left|F_v\right|-1$.

Case 2. $F_e\cap E_i\ne \varnothing$, $i\in \{k,k+1,\dots ,n,c\}$. Definition 1 ensures that we can partition $Q_{n,k}$ along dimension $i\in \{k,k+1,\dots ,n,c\}$ of some faulty edge into two subcubes $Q_{n-1}^L$ and $Q_{n-1}^R$.

For $i\in \{k,k+1,\dots ,n,c\}$, let $F_i=E_i\cap F_e$, $F_c=E_c\cap F_e$, $F_e^L=F_e\cap Q_{n-1}^L$, $F_e^R=F_e\cap Q_{n-1}^R$, $F_v^L=F_v\cap Q_{n-1}^L$, and $F_v^R=F_v\cap Q_{n-1}^R$. Then, we have $\left|F_i\right|+\left|F_c\right|\ge 1$, $\left|F_v^L\right|+\left|F_e^L\right|\le 2n-6$ and $\left|F_v^R\right|+\left|F_e^R\right|\le 2n-6$. We hypothesize that $\left|F_v^R\right|+\left|F_e^R\right|\le \left|F_v^L\right|+\left|F_e^L\right|$. Then, we have $\left|F_v^R\right|+\left|F_e^R\right|\le \lfloor {\displaystyle \frac{\left(\right|F_v|+|F_e\left|\right)-|F_i|}{2}}\rfloor \le \lfloor {\displaystyle \frac{(2n-5)-1}{2}}\rfloor =n-3$ and $\left|F_v^L\right|+\left|F_e^L\right|\le 2n-6$. Since every vertex of $Q_{n-1}^R$ connects $n-1$ edges and $\left|F_v^R\right|+\left|F_e^R\right|\le n-3$, it follows that $Q_{n-1}^R$ is conditionally faulty. Since every vertex in $Q_{n,k}-E_i$ connects at least two fault-free edges, $Q_{n,k}-E_i\cong Q_{n-1}^L\cup Q_{n-1}^R\cup E_c$ and $\left|F_v^L\right|+\left|F_e^L\right|\le 2n-6$, we can obtain that there exists at most one vertex in $Q_{n-1}^L$ which connects exactly only one fault-free edge in $Q_{n-1}^L$. If not, then $\left|F_v^L\right|+\left|F_e^L\right|\ge (n-2)+(n-2)-1=2n-5>2n-6$, a contradiction.

Case 2.1. $\left|F_v^L\right|+\left|F_e^L\right|\le 2n-7$.

Case 2.1.1. $l=n-k+4$.

Since there exist $2^{n-1}$ distinct vertices in $Q_{n-1}^L$ and $2^{n-1}>2n-5$ for $n\ge 5$, we choose a fault-free vertex $a\in V\left(Q_{n-1}^L\right)$ satisfying that the elements in $\{(a,a^i),(a,\overline{a}),a^i,\overline{a}\}$ are all fault-free. Note that $d_H(a^i,\overline{a})=n-k$. Since $\left|F_v^R\right|+\left|F_e^R\right|\le n-3$, by Lemma 3, there exists a fault-free path $P_R[a^i,\overline{a}]$ with odd length $l_R=n-k+2$ in $Q_{n-1}^R$. Hence, $P_R[a^i,\overline{a}]+(\overline{a},a)+(a,a^i)$ is a path with length $l=n-k+4$ (see

Table 3. Cycle construction in the proof of Lemma 11 when $F_e\cap E_i\ne \varnothing$, $i\in \{k,k+1,\dots ,n,c\}$ and $\left|F_v^L\right|+\left|F_e^L\right|\le 2n-7$.

Cases	Length l of Odd Cycles	Construction of Desired Cycles
Case 2.1.1   Case 2.1.2     $Q_{n-1}^L$ is conditionally faulty $\exists x\in V\left(Q_{n-1}^L\right)$ just satisfies $(x,z)\in E\left(Q_{n-1}^L\right)$.	$l=n-k+4$.   $n-k+6\le l\le 2^{n-1}-2\left|F_v^R\right|+1$,     $n-k+10\le l\le 2^n-2\left|F_v\right|-1$;     $2^{n-1}-2|F_v^R|+3\le l\le 2^n-2\left|F_v\right|-1$.	$P_R[a^i,\overline{a}]+(\overline{a},a)+(a,a^i)$.   $(a,b)+(b,\overline{b})+P_R[\overline{b},a^i]+(a^i,a)$, $P_L[a,b]+(b,\overline{b}),+P_R[\overline{b},a^i]+(a^i,a)$; $P_L[x,y]+(y,\overline{y}),+P_R[\overline{y},x^i]+(x^i,y)$.

).

Case 2.1.2. $n-k+6\le l\le 2^n-2\left|F_v\right|-1$.

Let us recall that $Q_{n-1}^L$ has $2^{n-2}$ vertex-disjoint edges and $2^{n-2}>2n-5$ when $n\ge 5$; we find a fault-free edge $(a,b)\in E\left(Q_{n-1}^L\right)$ such that the edges in $\{(a,a^i),(b,\overline{b})\}$ or $\{(a,\overline{a}),(b,b^i)\}$ are all fault-free edges. Let us say that the former is true. Note that $d_H(a^i,\overline{b})=n-k-1$ or $d_H(a^i,\overline{b})=n-k+1$. As $\left|F_v^R\right|+\left|F_e^R\right|\le n-3$, Lemma 3 implies that $P_R[a^i,\overline{b}]$ is fault-free and its length is every even integer $l_R$, $n-k+1\le l_R\le 2^{n-1}-2\left|F_v^R\right|-2$ or $n-k+3\le l_R\le 2^{n-1}-2\left|F_v^R\right|-2$. Thus, the cycle $(a,b)+(b,\overline{b})+P_R[\overline{b},a^i]+(a^i,a)$ has length l, $l=l_R+3$. That is, $n-k+4\le l\le 2^{n-1}-2\left|F_v^R\right|+1$ or $n-k+6\le l\le 2^{n-1}-2\left|F_v^R\right|+1$. The following apply:

• If each vertex in $Q_{n-1}^L$ connects at least two fault-free edges, since $\left|F_v^L\right|+\left|F_e^L\right|\le 2n-7$, by Lemma 6, $(a,b)$ lies on a fault-free cycle $C_L$ of every even length from 6 to $2^{n-1}-2\left|F_v^L\right|$ in $Q_{n-1}^L-F_v^L-F_e^L$. Thus, we have $P_L[a,b]+(b,\overline{b})+P_R[\overline{b},a^i]+(a^i,a)$ with length from $n-k+10$ to $2^n-2\left|F_v\right|-1$. Since $1\le k\le n-1$, $\left|F_v^R\right|\le n-3$ and $n\ge 5$, we can conclude that $2^{n-1}-2\left|F_v^R\right|+3\ge 2^{n-1}-2(n-3)+3>n-k+10$. Thus, we have $2^{n-1}-2|F_v^R|+3\le l\le 2^n-2\left|F_v\right|-1$ (see Figure 1b).
• If there is a vertex, say $x\in V\left(Q_{n-1}^L\right)$, that connects exactly one fault-free edge, say $(x,z)\in E\left(Q_{n-1}^L\right)$, as the vertex x connects $(n-2)$ faulty edges in $Q_{n-1}^L$, it implies that $|\{(x,x^i),(x,\overline{x})\}|\cap F_e\le 1$. Let us suppose that $(x,x^i)\notin F_e$. Since x is incident to $n-2$ faulty edges of $Q_{n-1}^L$ and $\left|F_v^R\right|+\left|F_e^R\right|\le n-3$, there exists at least one faulty edge incident to the vertex x, say $(x,y)$, such that $\left\{(y,\overline{y})\right\}\cap F_e=\varnothing$. Let us say that the edge $(x,y)$ is fault-free temporarily. Then, we can use t$(x,y)$ to replace t$(a,b)$ as in the above subcase. Similarly, we construct the path $P_L[x,y]+(y,\overline{y})+P_R[\overline{y},x^i]+(x^i,y)$, whose length is $2^{n-1}-2|F_v^R|+3\le l\le 2^n-2\left|F_v\right|-1$.

Case 2.2. $\left|F_v^L\right|+\left|F_e^L\right|=2n-6$.

Case 2.2.1. If $\left|F_e^L\right|=0$, then we have $\left|F_v^L\right|=2n-6$, $\left|F_i\right|+\left|F_c\right|=1$ and $\left|F_v^R\right|+\left|F_e^R\right|=0$ (see

Table 4. Cycle construction in the proof of Lemma 11 when $\left|F_e^L\right|=0$ or $\left|F_e^L\right|\ge 1$, and $\left|F_v^L\right|+\left|F_e^L\right|=2n-6$.

Cases	Distribution of Faulty Elements	Construction of Desired Cycles	Length l of Odd Cycles
Case 2.2.1   Case 2.2.2	$\left|F_e^L\right|=0$$\left|F_e^L\right|\ge 1$;	$C=(u,v)+(u,u^i)+P_R[u^i,\overline{v}]+(\overline{v},v)$ $C=P_L[x,y]+(x,x^i)+P_R[x^i,\overline{y}]+(\overline{y},y)$ $C=P_L[s,t]+(s,x^i)+P_R[s^i,\overline{t}]+(\overline{t},t)$	$n-k+2\le l\le 2^{n-1}-2\left|F_v^R\right|+1$ $2^{n-1}-2|F_v^R|+1\le l\le 2^n-2\left|F_v\right|-1$ $2^{n-1}-2|F_v^R|+1\le l\le 2^n-2\left|F_v\right|-1$

).

If $n=5$, then Corollary 1 indicates that it holds. Now, we consider $n\ge 6$. For $\left|F_v^L\right|=2n-6$, $\left|F_e^L\right|=0$, and $n\ge 6$, 7 helps to find an even cycle $C_L$ with length from 4 to $2^{n-1}-2\left|F_v^L\right|$ in $Q_{n-1}^L$. Clearly, we find an edge $(a,b)\in C_L$ such that $\{\overline{a},b^i\}\cap F_v=\varnothing$ or $\{a^i,\overline{b}\}\cap F_v=\varnothing$. We hypothesize the former to be true. As $\left|F_e^R\right|+\left|F_v^R\right|=0$, Lemma 2 implies that $P_R[\overline{a},b^i]$ is an even path with length $l_R$ in $Q_{n-1}^R$, where $n-k-1\le l_R\le 2^{n-1}-2\left|F_v^R\right|-2$ or $n-k+1\le 2^{n-1}-2\left|F_v^R\right|-2$. Finally, $P_L[a,b]+(b,b^i)+P_R[b^i,\overline{a}]+(\overline{a},a)$ is an odd cycle with length l, where $n-k+2\le l\le 2^n-2\left|F_v\right|-1$ or $n-k+4\le l\le 2^n-2\left|F_v\right|-1$.

Case 2.2.2. If $\left|F_e^L\right|\ge 1$, then $\left|F_v^L\right|\le 2n-7$, $\left|F_i\right|+\left|F_c\right|=1$, and $\left|F_v^R\right|+\left|F_e^R\right|=0$.

We select an edge $(u,v)$ in $Q_{n-1}^L$ satisfying that $\{(u,v),(u,u^i),(v,\overline{v})\}\cap F_e=\varnothing$. Lemma 2 indicates that $(u,v)+(u,u^i)+P_R[u^i,\overline{v}]+(\overline{v},v)$ is the desired path with length $n-k+2\le l\le 2^{n-1}-2\left|F_v^R\right|+1$. In the following, we choose a faulty edge $(x,y)$ in $Q_{n-1}^L$ such that the edges in $\{(x,x^i),(y,\overline{y})\}$ are fault-free and say that the edge $(x,y)$ is a fault-free edge temporarily. If every vertex in $Q_{n-1}^L$ is incident to at least two fault-free edges, Lemma 6 implies that $P_L[x,y]+(x,x^i)+P_R[x^i,\overline{y}]+(\overline{y},y)$ is the desired cycle with length $2^{n-1}-2|F_v^R|+1\le l\le 2^n-2\left|F_v\right|-1$. If there exists a vertex which connects exactly one edge in $E\left(Q_{n-1}^L\right)-F_e$, we can take s as the vertex and find an adjacent faulty edge $(s,t)$ satisfying $(s,s^i)\cap F_e=\varnothing$ when $(t,\overline{t})\cap F_e=\varnothing$ is fault-free. Finally, we find the odd cycle as $P_L[s,t]+(s,s^i)+P_R[s^i,\overline{t}]+(\overline{t},t)$ with length from $2^{n-1}-2\left|F_v^R\right|+1\le l\le 2^n-2\left|F_v\right|-1$.

In summary, the lemma is true. □

Lastly, we consider the embedding of odd cycles in conditionally faulty $Q_{n,k}$ where $n\ge 5$ and k have different parity, $F_e=\varnothing$, and $\left|F_v\right|+\left|F_e\right|\le 2n-5$.

Lemma 12.

In conditionally faulty $Q_{n,k}$, if $n(\ge 5)$ and $k(1\le k\le n-1)$ have different parity and $|F_v|\le 2n-5$, then $Q_{n,k}-F_v-F_e$ contains a fault-free odd cycle with l, where $n-k+4\le l\le 2^n-2\left|F_v\right|-1$.

Proof. 

Since $\left|F_v\right|\le 2n-5$, there exists at most one vertex which is adjacent to exactly two fault-free vertices. If not, we hypothesize that there exist two such vertices, and we have $\left|F_v\right|\ge 2(n-1)-1=2n-3>2n-5$, a contradiction. If every vertex in $Q_{n,k}$ is adjacent to at least two fault-free vertices, we choose two vertices $x=x_1{x}_2\dots x_n$ and $y=y_1{y}_2\dots y_n$ in $F_v$ to find an integer i satisfying that $1\le i\le n$, $x_i+y_i=1$. If there is a vertex $a=a_1{a}_2\dots a_i\dots a_n$ in $Q_{n,k}$ adjacent to exactly two fault-free vertices, we find a faulty vertex $a^{\prime }=a_1{a}_2\dots {\overline{a}}_i\dots a_n$ adjacent to the vertex a. Definition 1 indicates that $Q_{n,k}$ can be partitioned along dimension i into two $(n-1)$-dimensional cubes such that every cube has exactly one faulty vertex and every vertex connects at least two fault-free edges in $Q_{n,k}-E_i$.

Case 1. $i\in \{1,2,\dots ,k-1\}$, $Q_{n,k}=Q_{n-1,k-1}^L\cup Q_{n-1,k-1}^R\cup E_i$.

The proof of this case is by induction on n. By Lemma 10, the lemma holds when $n=4$. We hypothesize that the result is true for $4\le m<n$. We now consider that $m=n$. We can denote $F_v^L=F_v\cap Q_{n-1,k-1}^L$ and $F_v^R=F_v\cap Q_{n-1,k-1}^R$. Let us suppose that $\left|F_v^L\right|\le \left|F_v^R\right|$. We can conclude that $1\le \left|F_v^L\right|\le \lfloor {\displaystyle \frac{\left|F_v\right|-1}{2}}\rfloor =\lfloor {\displaystyle \frac{(2n-5)-1}{2}}\rfloor \le n-3$ and $1\le \left|F_v^R\right|\le 2n-6$.

Case 1.1. $1\le \left|F_v^L\right|\le n-3$ and $1\le \left|F_v^R\right|\le 2n-7$.

As $\left|F_v^R\right|\le 2n-7$ and every vertex in $Q_{n-1,k-1}^R$ is adjacent to at least two fault-free edges, $C_R$ is a cycle with length $n-k+4\le l\le 2^{n-1}-2\left|F_v^R\right|-1$ in $Q_{n-1,k-1}^R-F_v^R$.

Let $C_R$ be a fault-free cycle with length $2^{n-1}-2\left|F_v^R\right|-1$ in $Q_{n-1,k-1}^R$. As $\lfloor {\displaystyle \frac{2^{n-1}-2\left|F_v^R\right|-1}{2}}\rfloor$$\ge 2^{n-2}-(2n-7)-1>n-3$ for $n\ge 5$, we choose an edge $(a,b)\in E\left(Q_{n-1,k-1}^R\right)-F_e$ in cycle $C_R$ such that $\{a^i,b^i\}\cap F_v=\varnothing$. Since $\left|F_v^L\right|\le n-3$, Lemma 5 indicates that the edge $(a^i,b^i)$ lies on a fault-free cycle $C_L$ with every even length from 4 to $2^{n-1}-2\left|F_v^L\right|$ in $Q_{n-1,k-1}^L-F_v^L$. Therefore, $C_L[a^i,b^i]-(a^i,b^i)+(b^i,b)+C_R[b,a]-(b,a)+(a,a^i)$ forms the desired cycle with length from $2^{n-1}-2\left|F_v^R\right|+1$ to $2^n-2\left|F_v\right|-1$.

Case 1.2. $\left|F_v^0\right|=1$ and $\left|F_v^1\right|=2n-6$.

On one hand, since $\left|F_v^L\right|=1\le 2n-7$ for $n\ge 4$, $C_L$ is a cycle with every odd length $n-k+4\le 2^{n-1}-2\left|F_v^L\right|-1$ in $Q_{n-1,k-1}^L-F_v^L$. On the other hand, let $x\in V\left(Q_{n-1,k-1}^R\right)\cap F_v$. Let us say that the vertex x is fault-free temporarily. There exists a cycle $C_R$ containing the vertex x with length $2^{n-1}-2\left(\right|F_v^R|-1)-1$ in $Q_{n-1,k-1}^R+\left\{x\right\}-F_v^R$, and the vertices z and w are adjacent to the vertex x on the cycle $C_R$ such that the vertices in $\{z^i,w^i\}$ are both fault-free. Note that $d_H(z^i,w^i)=2$. Since $\left|F_v^L\right|=1$, by Lemma 3, $P_L[z^i,w^i]$ is a path with length $4\le l_L\le 2^{n-1}-2\left|F_v^L\right|-2$ in $Q_{n-1,k-1}^L-F_v^L$. Obviously, $P_L[z^i,w^i]+(z^i,z)+(w^i,w)+C_R-(x,z)-(x,w)$ forms the desired cycle with length l, $2^{n-1}-2\left|F_v^R\right|+5\le l\le 2^n-2\left|F_v\right|-1$. Since $\left|F_v^L\right|=1$, $\left|F_v^R\right|=2n-6$, and $n\ge 5$, $2^{n-1}-2|F_v^R|+5\le 2^{n-1}-2\left|F_v^L\right|+1$. Hence, $2^{n-1}-2|F_v^L|+1\le l\le 2^n-2\left|F_v\right|-1$ (see Figure 2a).

Case 2. $i\in \{k,k+1,\dots ,n,c\}$, $Q_{n,k}=Q_{n-1}^L\cup Q_{n-1}^R\cup E_i\cup E_c$.

We denote $F_v^L=F_v\cap Q_{n-1}^L$ and $F_v^R=F_v\cap Q_{n-1}^R$. Let us suppose that $\left|F_v^L\right|\le \left|F_v^R\right|$. Thus, $1\le \left|F_v^L\right|\le \lfloor {\displaystyle \frac{\left|F_v\right|-1}{2}}\rfloor =\lfloor {\displaystyle \frac{(2n-5)-1}{2}}\rfloor \le n-3$, and $1\le \left|F_v^R\right|\le 2n-6$.

Case 2.1. $l=n-k+4$.

Let us recall that $\left|V\left(Q_{n-1}^R\right)\right|=2^{n-1}$ and $2^{n-1}>2n-5$ for $n\ge 5$; we will find a vertex $a\in V\left(Q_{n-1}^R\right)\cap F_v$ satisfying that $\{(a,a^i),(a,\overline{a}),a^i,\overline{a}\}\cap F_e=\varnothing$. Note that $d_H(\overline{a},a^i)=n-k$. Since $|F_v^L|\le n-3$, by Lemma 3, there exists a fault-free path $P_L[\overline{a},a^i]$ with length $(n-k)+2$ in $Q_{n-1}^L-F_v^L$. Thus, $P_L[\overline{a},a^i]+(a^i,a)+(a,\overline{a})$ is an odd cycle with length $n-k+4$ in $Q_{n,k}$.

Case 2.2. $n-k+6\le l\le 2^n-2\left|F_v\right|-1$.

Case 2.2.1. $n=5$.

Since $1\le \left|F_v^R\right|\le 2n-6=4$ for $n=5$, by Lemma 7, $C_R$ is a fault-free even cycle with length $4\le l_R\le 2^4-2\left|F_v^R\right|$ in $Q_{n-1}^R-F_v^R$. Since $1\le \left|F_v^L\right|\le n-3=2$ for $n=5$ and the smallest length of cycle $C_R$ is 4, we choose an edge $(a,b)$ on the cycle $C_R$ such that the edges in $\{(a,a^i),(b,\overline{b})\}$ or $\{(a,\overline{a}),(b,b^i)\}$ are both fault-free. We hypothesize that the former is true. Thus, the path $P_R[a,b]$ is of length $1\le l_R\le 2^4-2\left|F_v^1\right|-1$. Since $\left|F_v^L\right|\le 2$, and $d_H(a^i,\overline{b})=n-k-1$ or $d_H(a^i,\overline{b})=n-k+1$, by Lemma 3, there exists a fault-free path $P_L[a^i,\overline{b}]$ with length $n-k+3\le l_L\le 2^4-2\left|F_v^L\right|-2$ in $Q_{n-1}^L-F_v^L$. $P_L[a^i,\overline{b}]+(\overline{b},b)+P_R[b,a]+(a,a^i)$ forms the desired cycle with length $n-k+6\le l\le 2^5-2\left|F_v\right|-1$ in $Q_{n,k}$.

Case 2.2.2. $n\ge 6$.

Since $\left|F_v^R\right|\le 2n-6$, $\left|F_e^R\right|=0\le n-2$, and $n-1\ge 5$ for $n\ge 6$, by Lemma 7, there exists a fault-free cycle $C_R$ of every even length from 4 to $2^{n-1}-2\left|F_v^R\right|$ in $Q_{n-1}^R-F_v^R$. Since $\left|F_v^L\right|\le n-3$ and $\left|F_v^R\right|\le 2n-6$, we find an edge $(a,b)$ in the cycle $C_R$ such that the vertices in $\{\overline{a},b^i\}$ or $\{a^i,\overline{b}\}$ are fault-free. If not, we have $\left|F_v^L\right|\ge \left({\displaystyle \frac{2^{n-1}-2\left|F_v^R\right|}{2}}\right)*2=2^{n-1}-2\left|F_v^R\right|\ge 2^{n-1}-(2n-6)>n-3$ for $n\ge 6$, which contradicts the fact that $\left|F_v^L\right|\le n-3$. We can assume that the former is true. Since $|F_e|=0$, we can obtain that $\{\overline{a},b^i,(a,\overline{a}),(b,b^i)\}\cap (F_v\cup F_e)=\varnothing$. Note that $d_H(\overline{a},b^i)=n-k-1$ or $d_H(\overline{a},b^i)=n-k+1$. Since $\left|F_v^L\right|\le n-3$, Lemma 3 implies that $P_L[\overline{a},b^i]$ is a path with length $n-k+1\le l_L\le 2^{n-1}-2\left|F_v^L\right|-2$ or $n-k+3\le l_L\le 2^{n-1}-2\left|F_v^L\right|-2$. Hence, $P_L[\overline{a},b^i]+(b^i,b)+C_R-(b,a)+(a,\overline{a})$ is an odd cycle with length $n-k+4\le l\le 2^n-2\left|F_v\right|-1$ or $n-k+6\le l\le 2^n-2\left|F_v\right|-1$ (see Figure 2b).

By combining the above cases, the lemma holds. □

Theorem 1.

In conditionally faulty $Q_{n,k}$, if $n(\ge 3)$ and $k(1\le k\le n-1)$ have different parity and $\left|F_v\right|+\left|F_e\right|\le 2n-5$, then $Q_{n,k}-F_v-F_e$ contains a fault-free odd cycle with length l, $n-k+4\le l\le 2^n-2\left|F_v\right|-1$.

Proof. 

By Lemma 9 and Lemma 10, the theorem holds in $Q_{3,2}$ and $Q_{4,k}$, $k\in \{1,3\}$, respectively. Lemma 11 indicates that the theorem holds in $Q_{n,k}$ when $n\ge 5$ and k have different parity, $\left|F_v\right|+\left|F_e\right|\le 2n-5$, and $|F_e|\ge 1$. In addition, by Lemma 12, the theorem holds in $Q_{n,k}$ when $n\ge 5$ and k have different parity and $|F_v|\le 2n-5$. In summary, Theorem 1 is true. □

4. Concluding Remarks

Network topology is an important issue in the design of computer networks since it is crucial to many key properties, such as symmetry and fault tolerance. In this article, we focus on the conditionally faulty enhanced hypercube $Q_{n,k}(1\le k\le n-1)$, where $n(\ge 3)$ and k have different parity. We prove that $Q_{n,k}-F_v-F_e$ contains a fault-free odd cycle with length from $n-k+4$ to $2^n-2\left|F_v\right|-1$ when $\left|F_v\right|+\left|F_e\right|\le 2n-5$. We believe that the structure constructed in this paper will be helpful in future research.

We will continue to investigate the fault tolerance of different networks under different faulty structures, such as the $K_{1,3}$ faulty structure, the $\{f_1,f_2\}$ faulty structure, and so on. To improve the efficiency of hypercube networks in structural fault-tolerant models, this seems to be a good direction for future research.