Enumerating the Number of Spanning Trees of Pyramid Graphs Based on Some Nonahedral Graphs
Abstract
1. Introduction
- Parallel edges: When two parallel edges in , each with conductances and , are merged into a single edge in with a conductance of , the count of spanning trees, , remains unchanged compared to .
- Serial edges: If two serial edges in , with conductances and , are combined into a single edge in with a conductance of , then can be calculated as multiplied by .
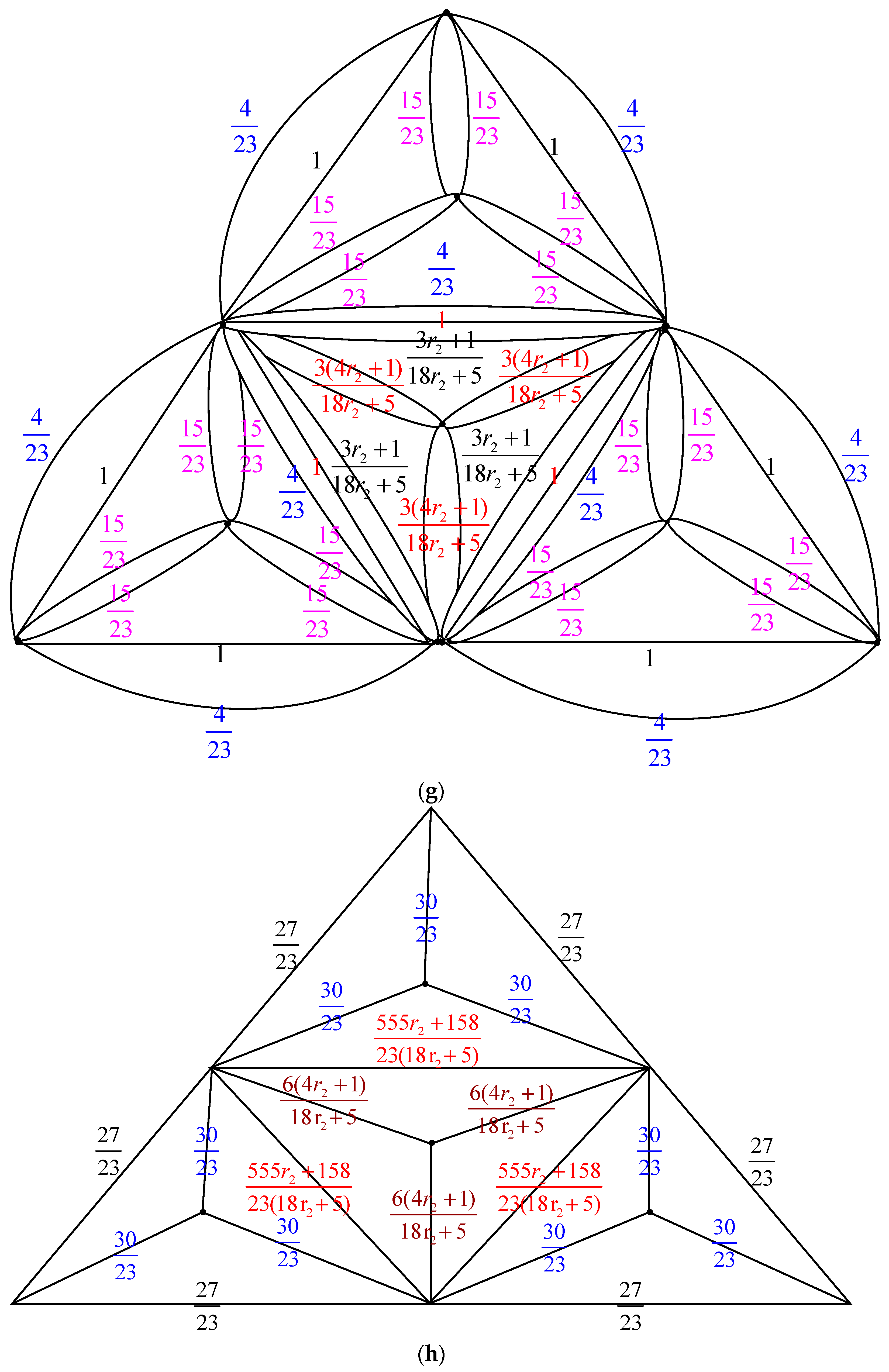
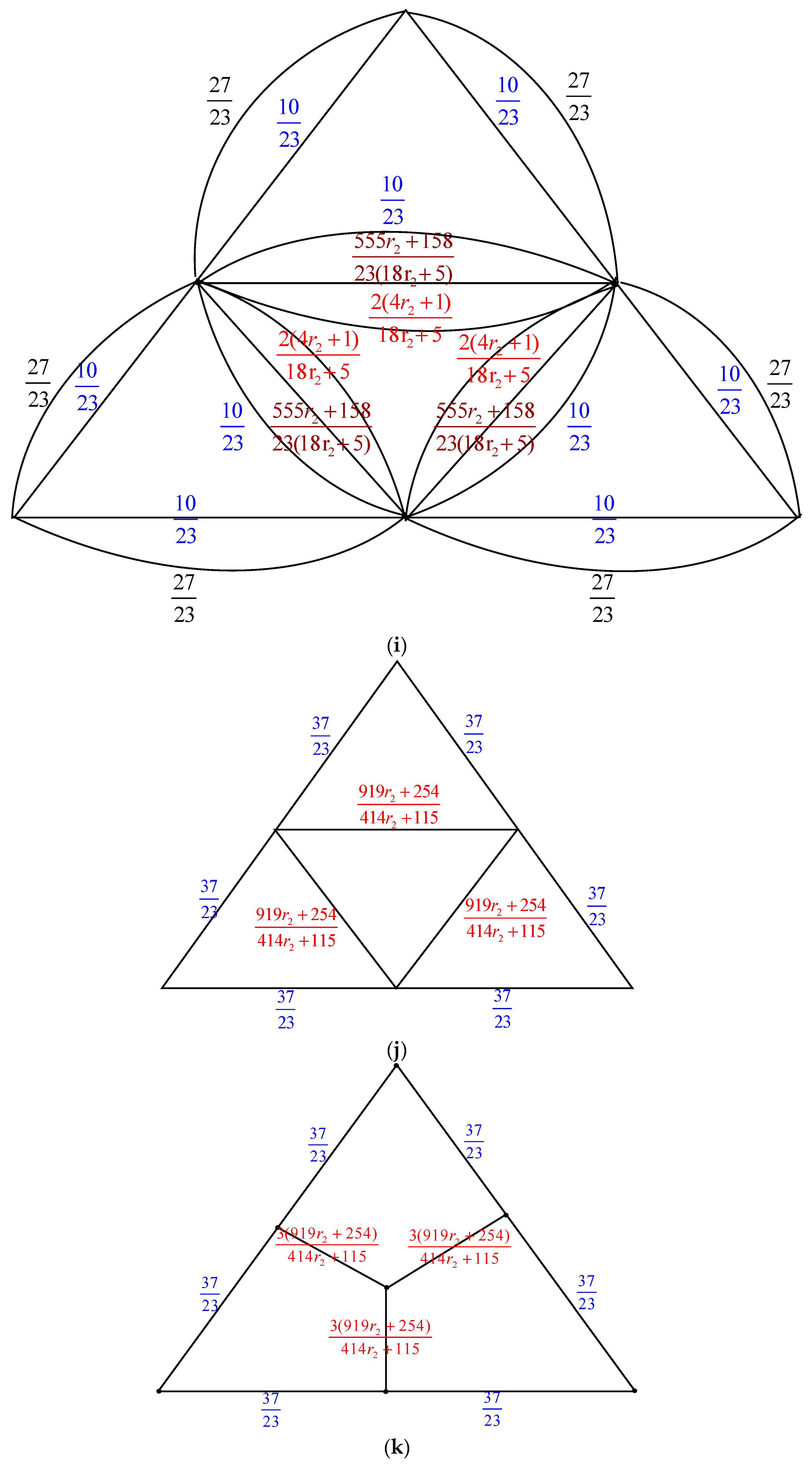
- Δ-Y Transformation: When a triangle in , with conductances , and is transformed into an electrically equivalent star graph in with conductances , and , the count of spanning trees in ,, can be determined as multiplied by .
- Y-Δ Transformation: If a star graph in , with conductances , and , is converted into an electrically equivalent triangle in with conductances , and , then is given by multiplied by .
2. Main Results
2.1. Number of Spanning Trees of Pyramid Graph Based on Nonahedron Graph
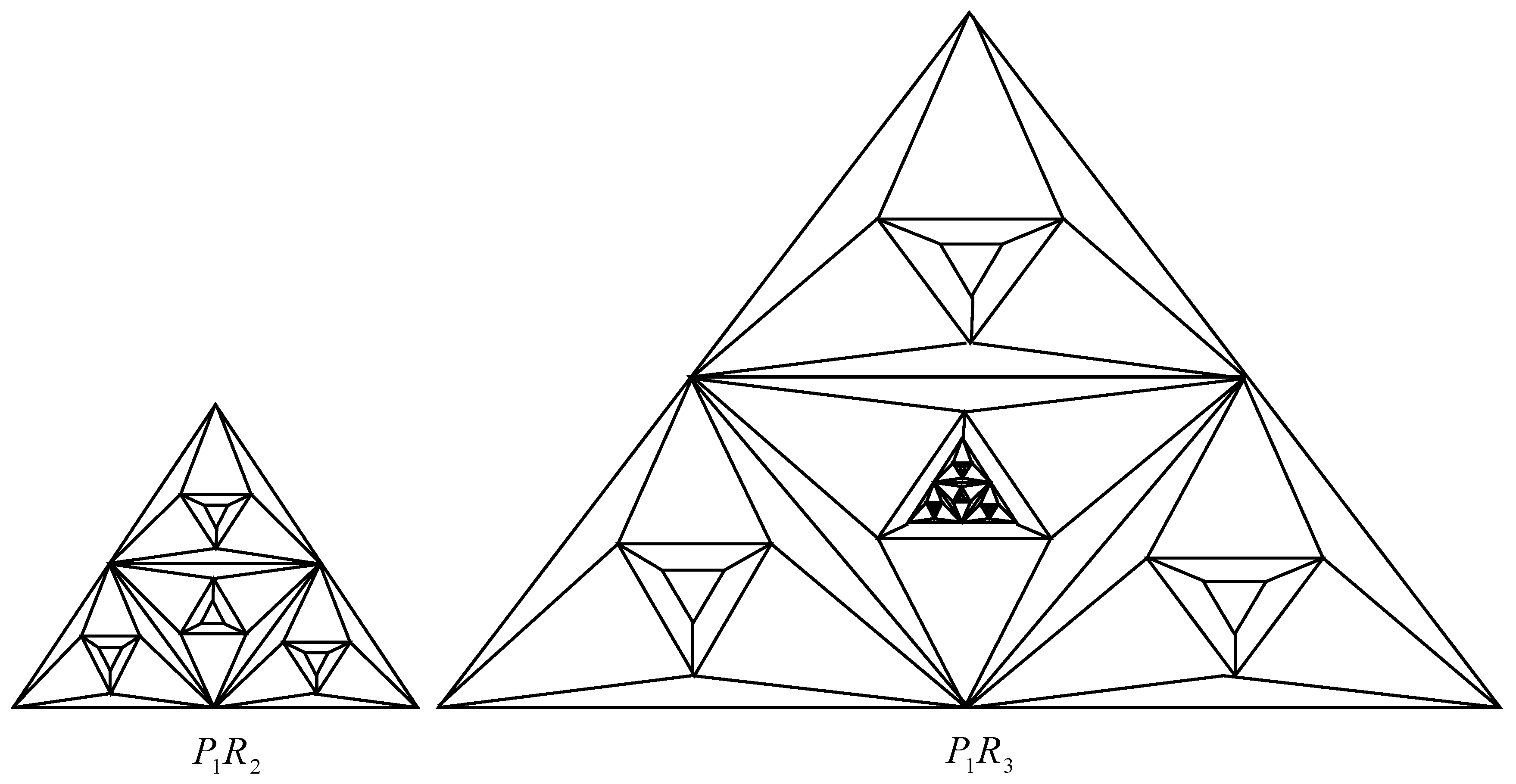
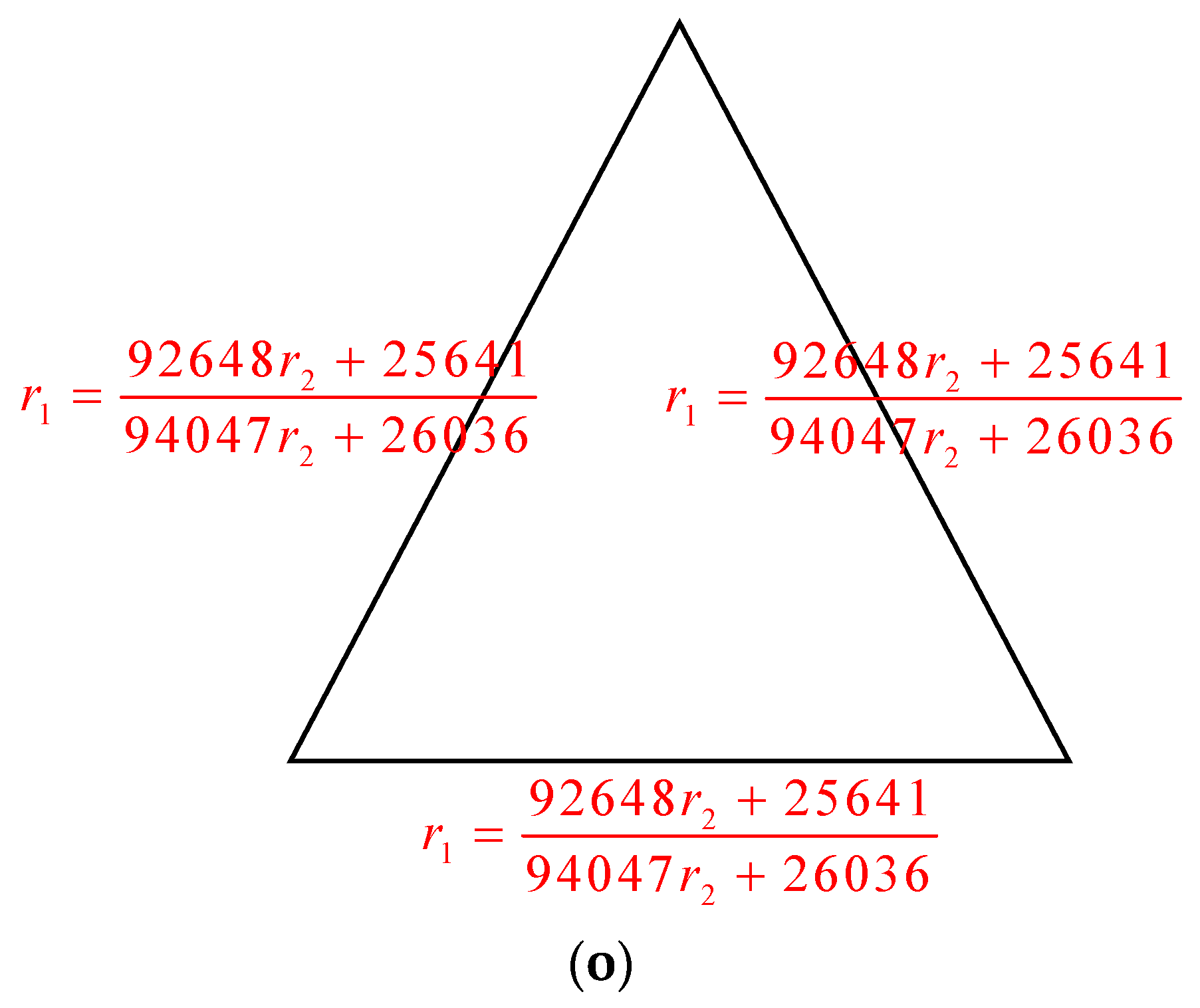
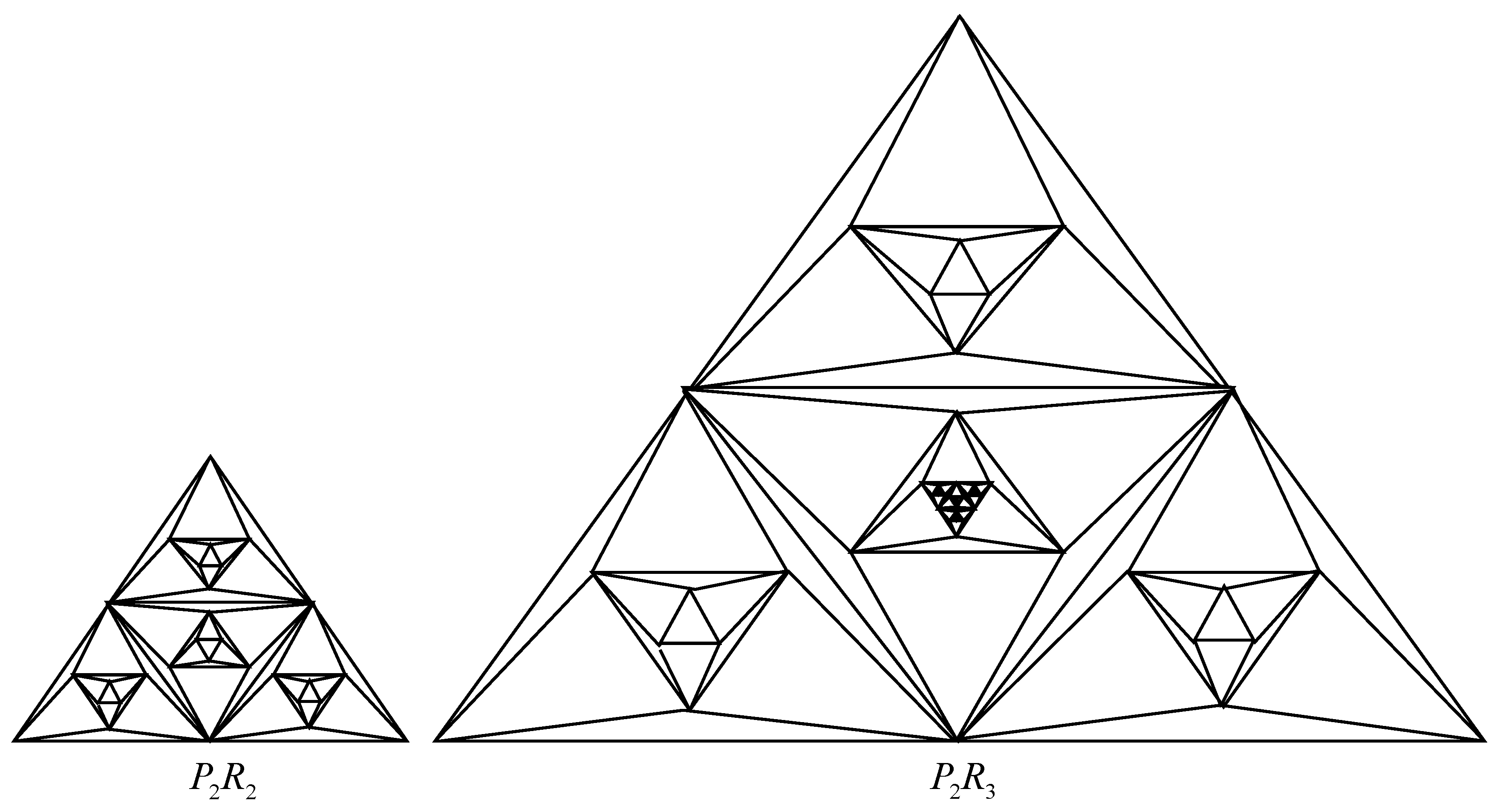
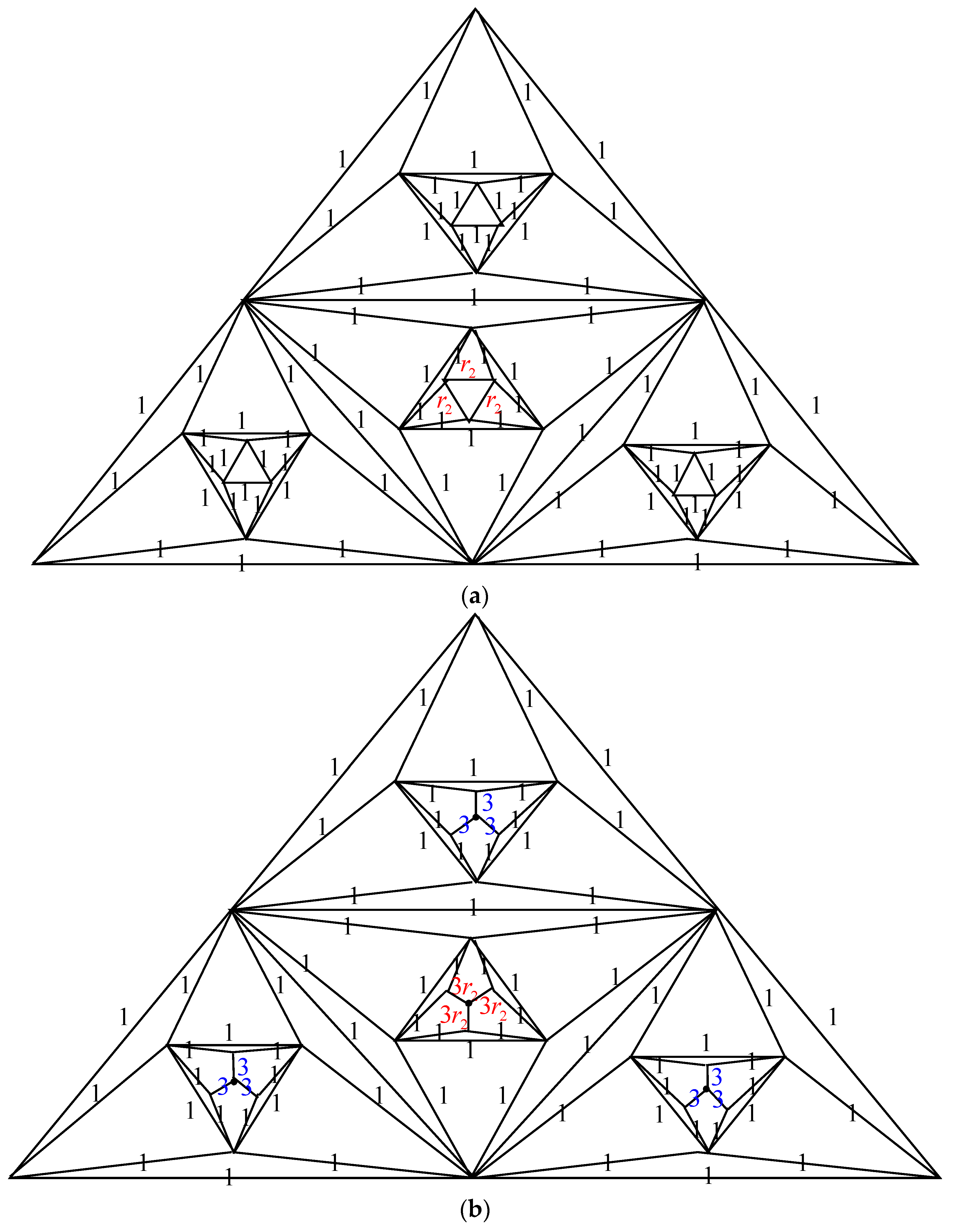
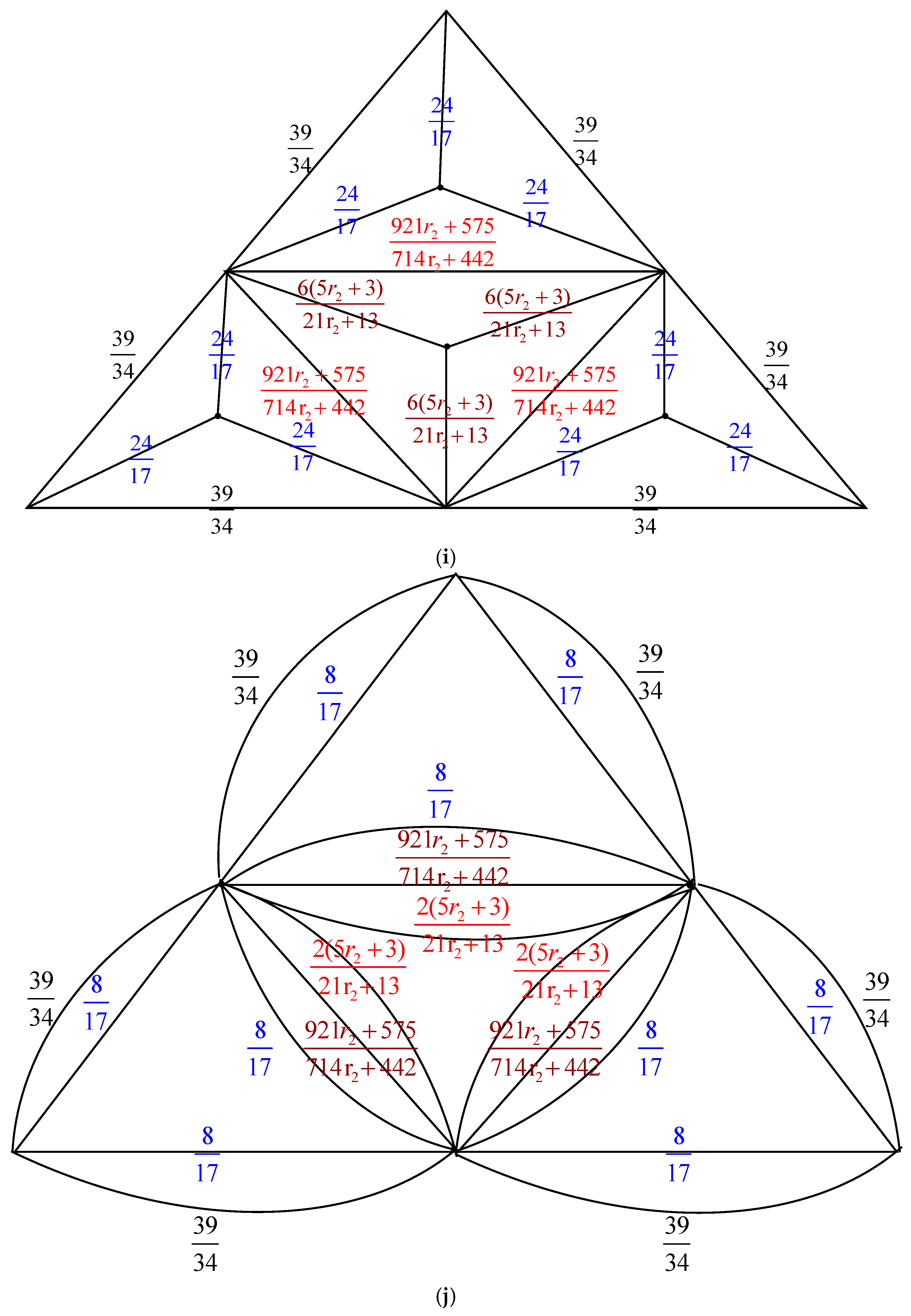
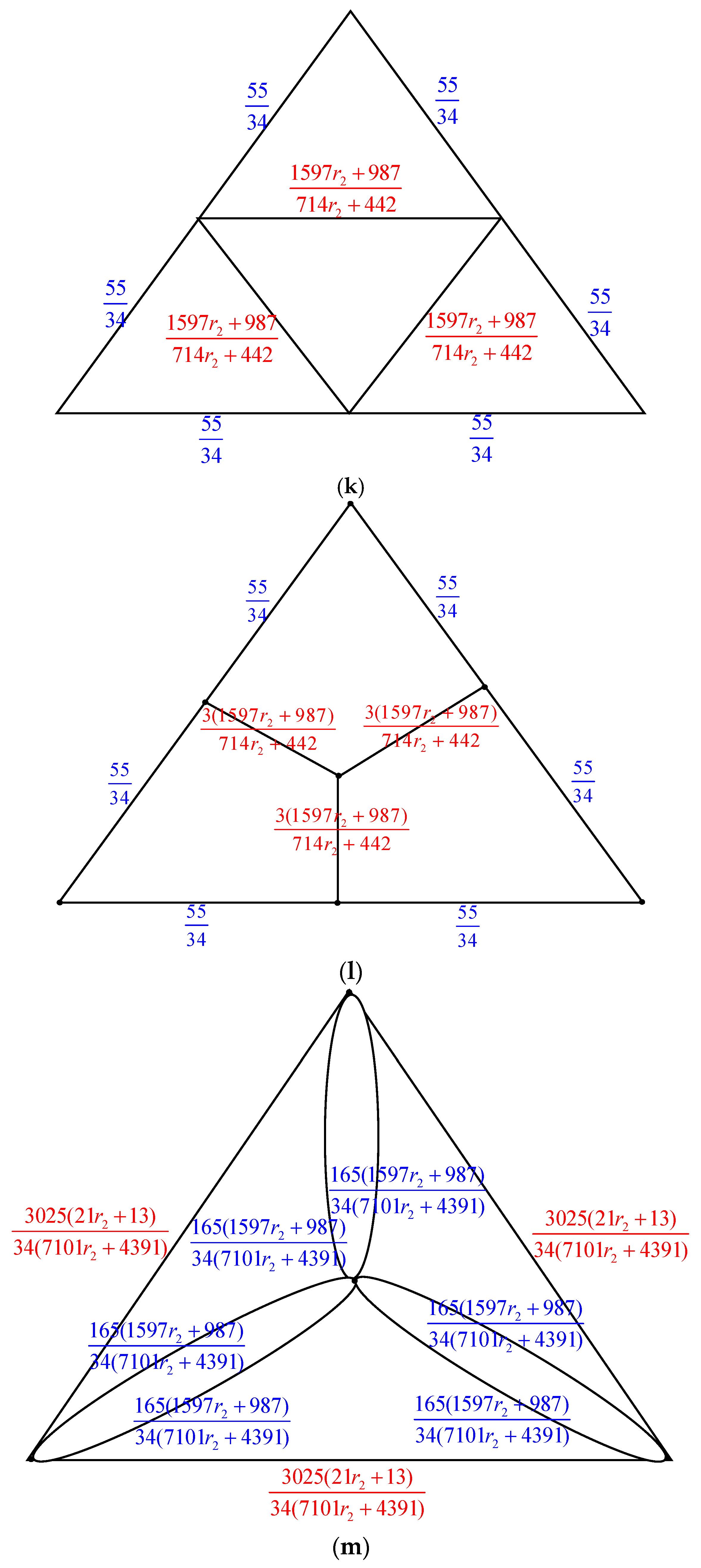
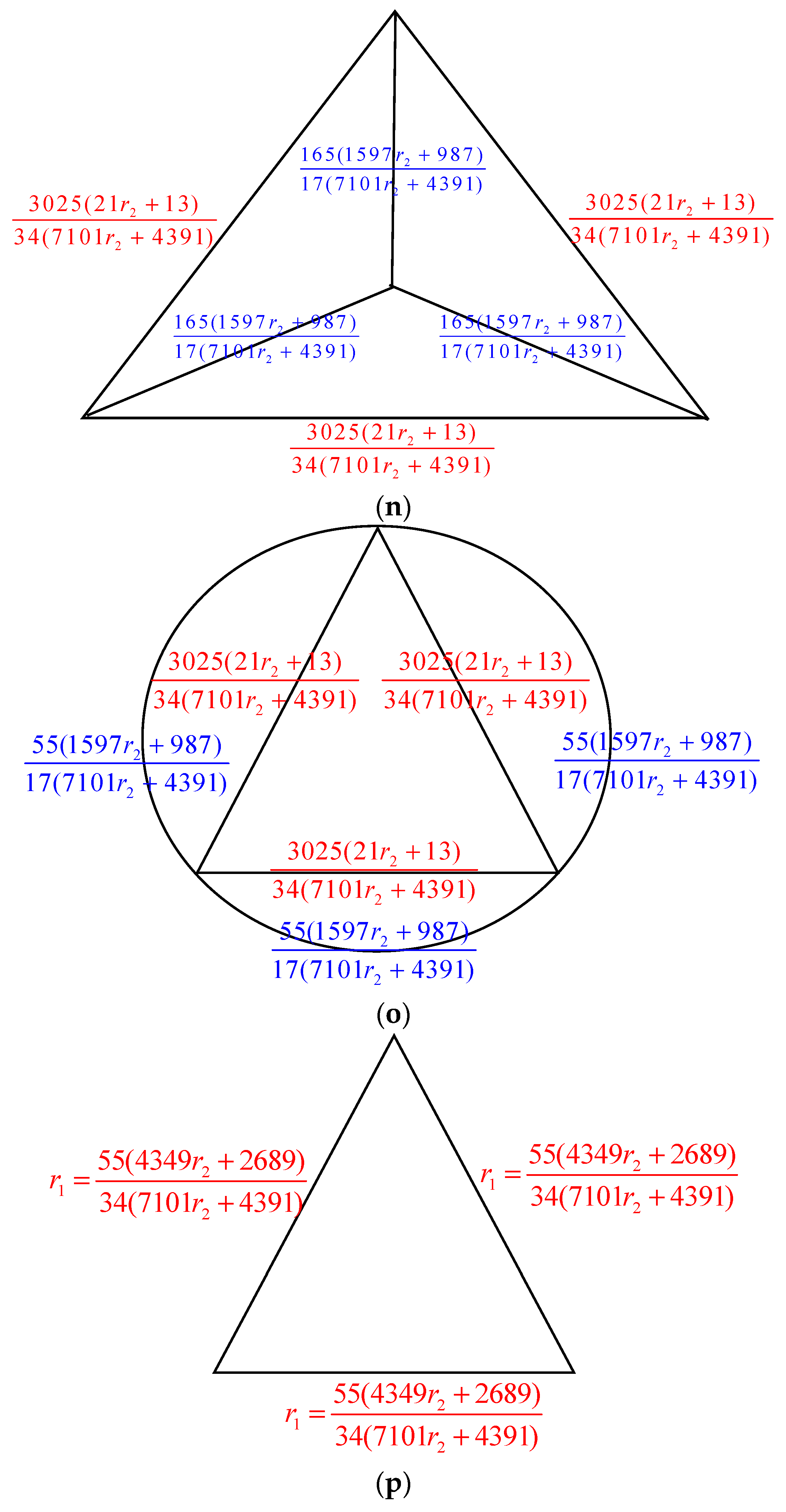
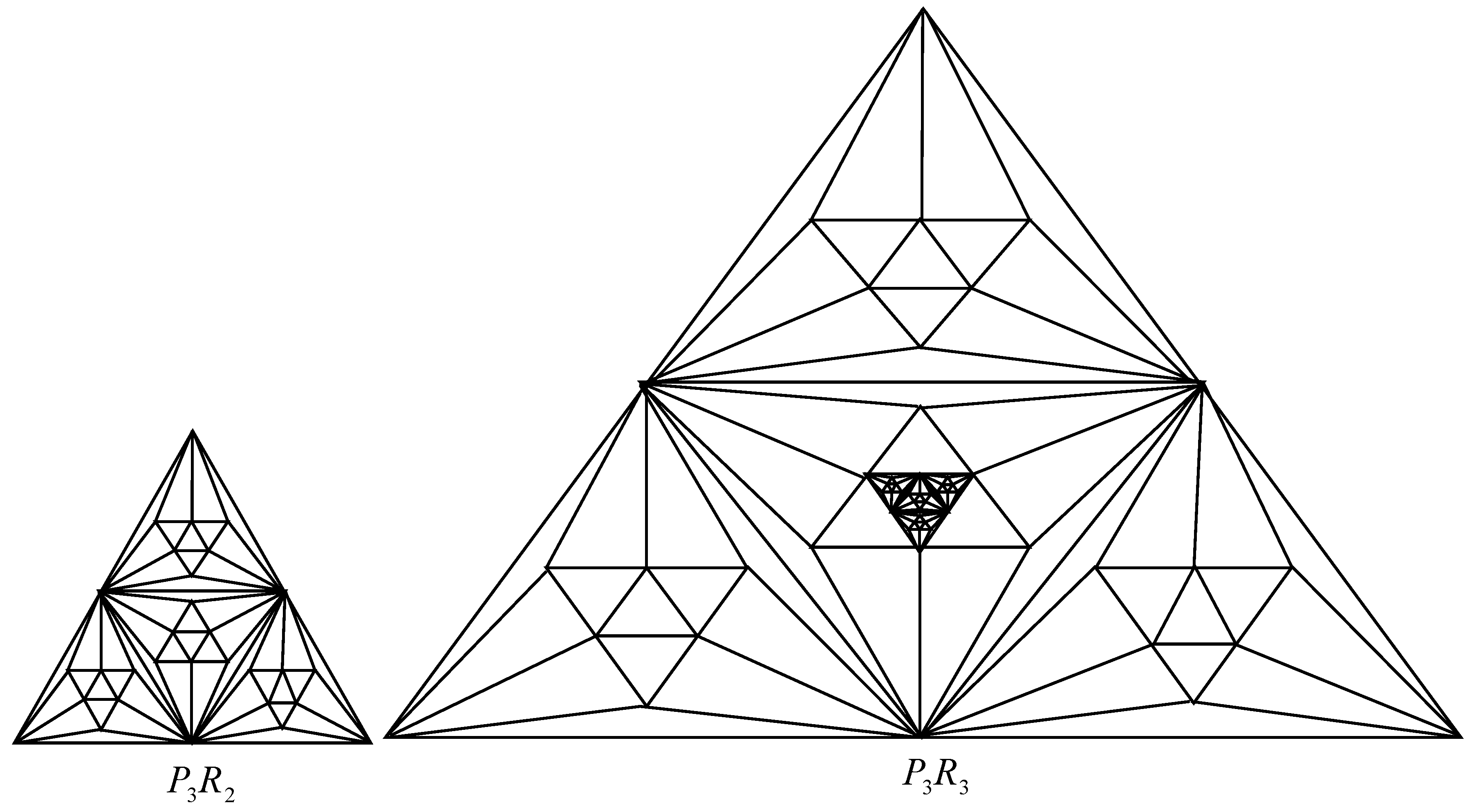
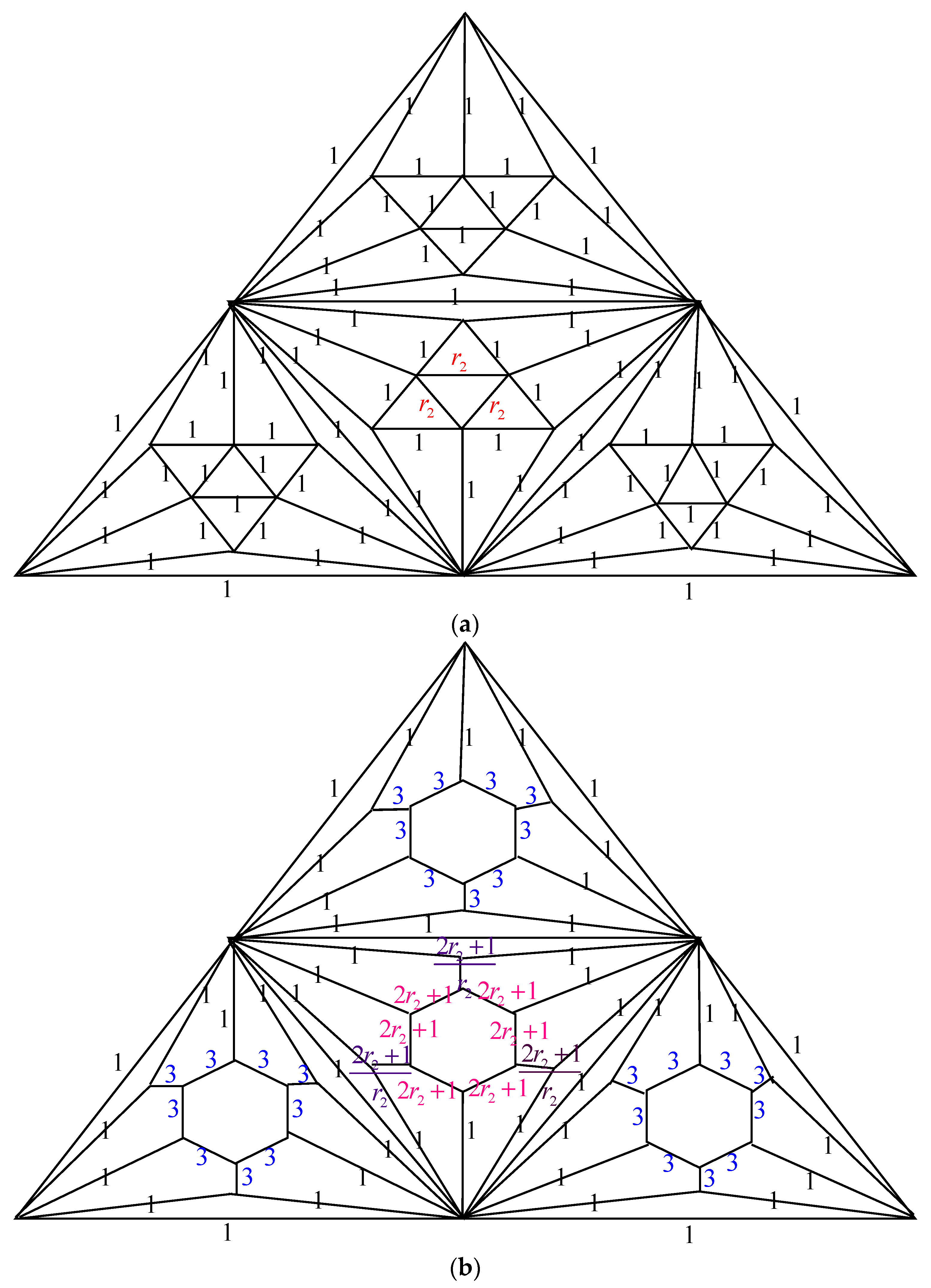
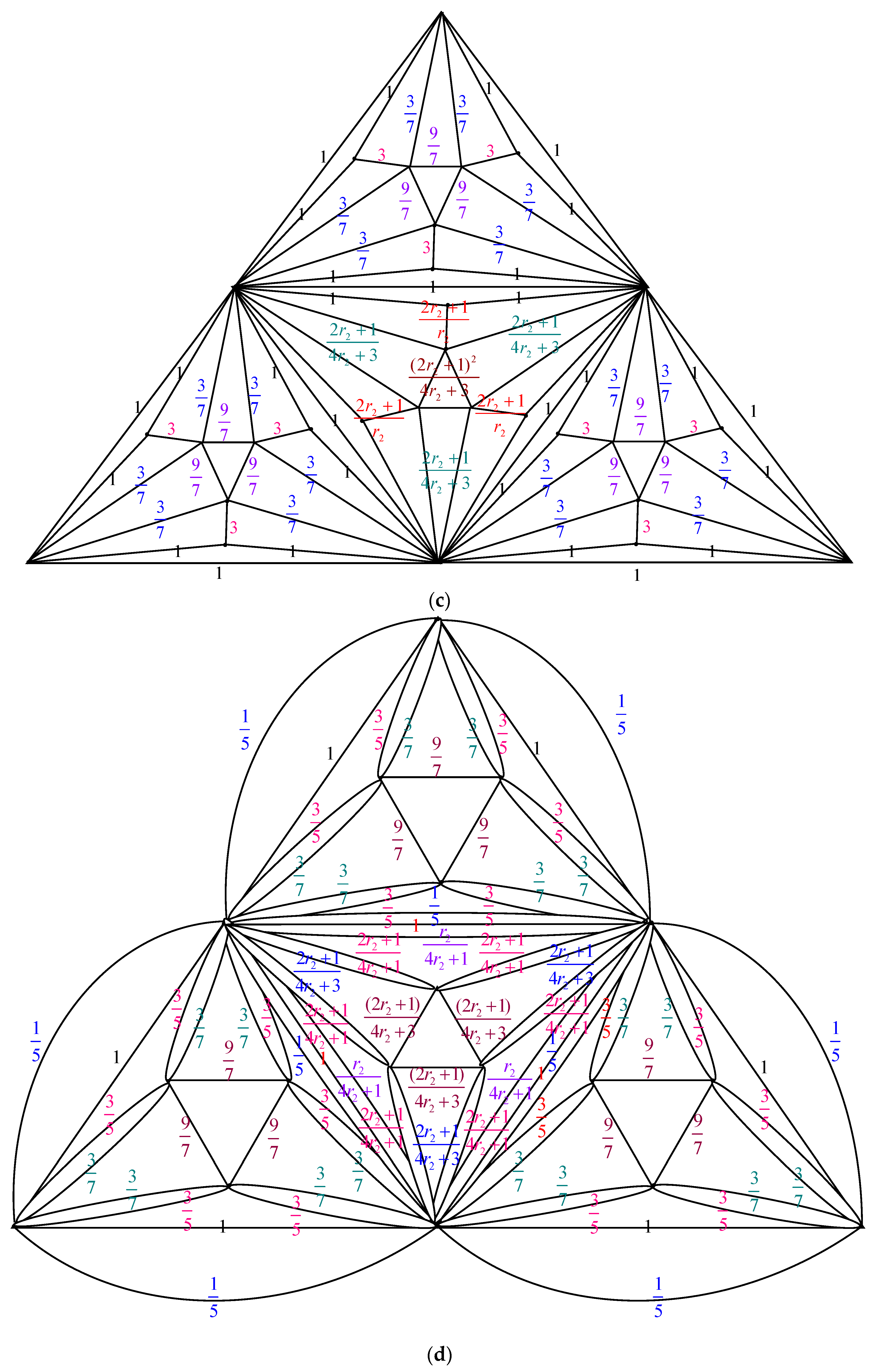
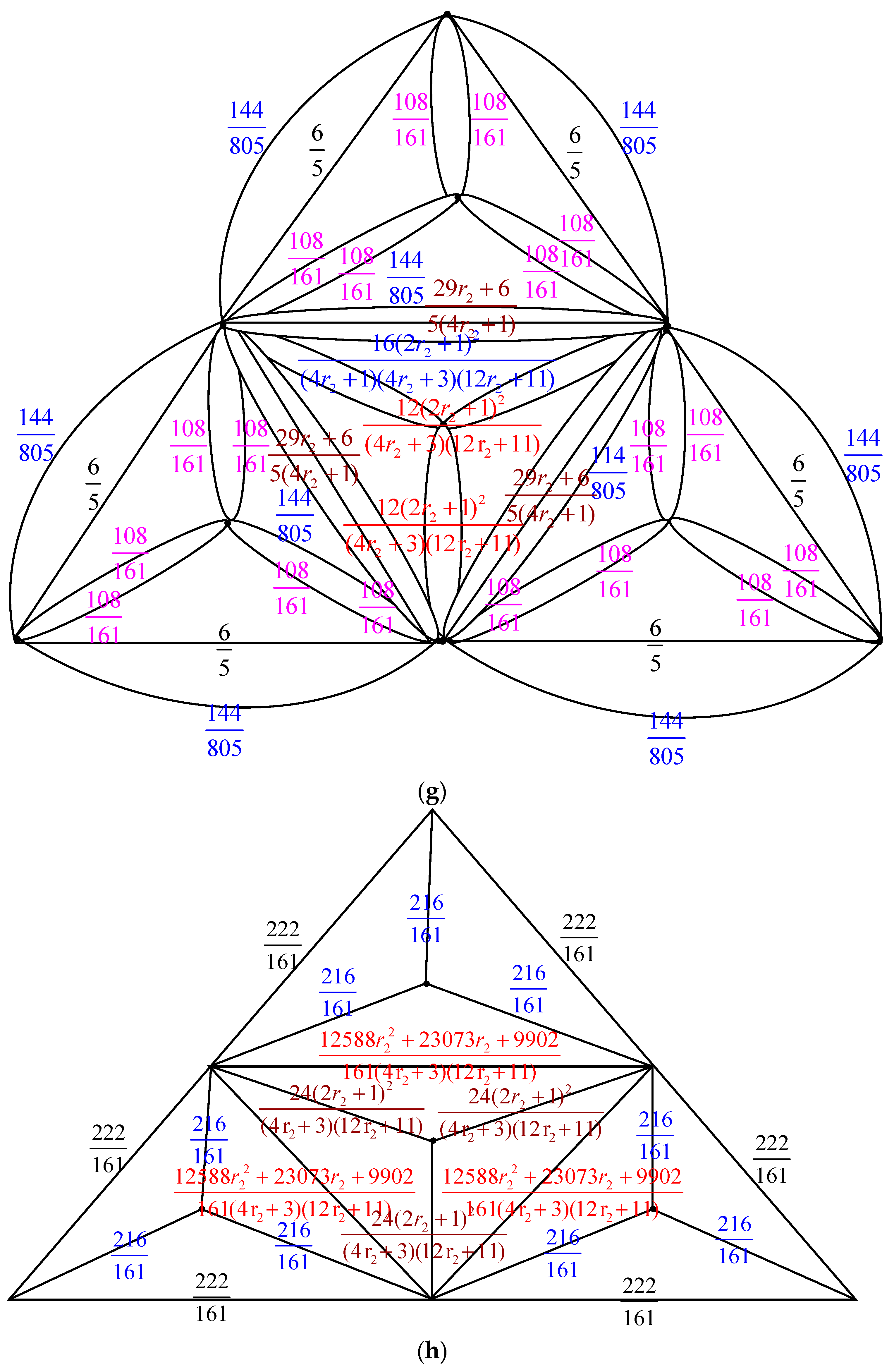
2.2. Number of Spanning Trees of Pyramid Graph Based on the Nonahedron
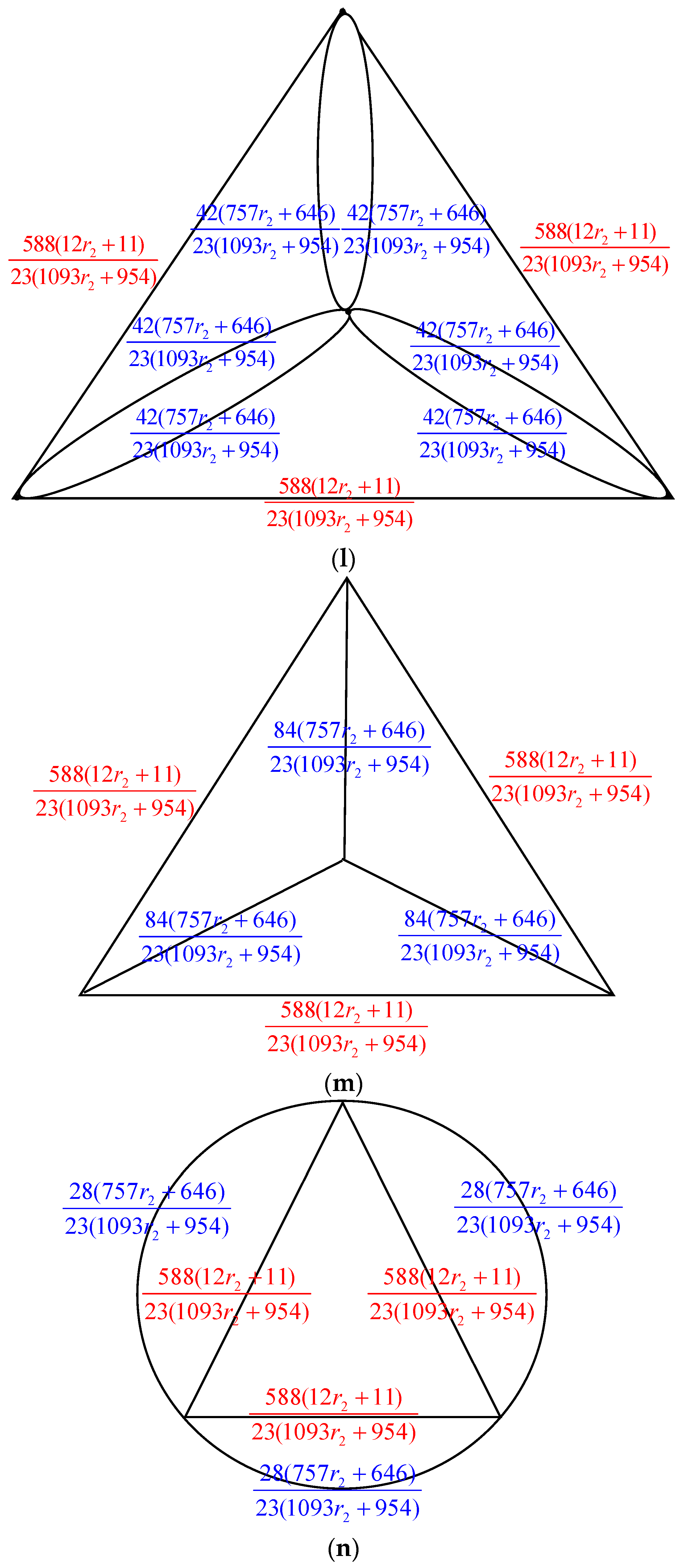
2.3. Number of Spanning Trees of Pyramid Graph Based on the Nonahedron Graph
3. Numerical Results
4. Spanning Tree Entropy
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhang, F.; Yong, X. Asymptotic enumeration theorems for the number of spanning trees and Eulerian trail in circulant digraphs & graphs. Sci. China Ser. A 1999, 43, 264–271. [Google Scholar]
- Zhang, H.; Zhang, F.; Huang, Q. On the number of spanning trees and Eulerian torus in iterated line digraph. Discret. Appl. Math. 1997, 73, 59–67. [Google Scholar]
- Letina, S.; Blanken, T.F.; Deserno, M.K.; Borsboom, D. Expanding network analysis tools in psychological networks: Minimal spanning trees, participation coefficients, and motif analysis applied to a network of 26 psychological attributes. Complexity 2019, 2019, 9424605. [Google Scholar] [CrossRef]
- Nikolopoulos, S.D.; Palios, L.; Papadopoulos, C. Counting spanning trees using modular decomposition. Theor. Comput. Sci. 2014, 526, 41–57. [Google Scholar] [CrossRef]
- Knopfmacher, J. Asymptotic isomer enumeration in chemistry. J. Math. Chem. 1998, 24, 61–69. [Google Scholar] [CrossRef]
- Cook, W.J.; Applegate, D.L.; Bixby, R.E.; Chvatal, V. The Traveling Salesman Problem: A Computational Study; Princeton University Press: Princeton, NJ, USA, 2006. [Google Scholar]
- Atajan, T.; Inaba, H. Network reliability analysis by counting the number of spanning trees. In Proceedings of the IEEE International Symposium on Communications and Information Technology, ISCIT 2004, Sapporo, Japan, 26–29 October 2004; pp. 601–604. [Google Scholar]
- Cașcaval, P. Approximate Method to Evaluate Reliability of Complex Networks. Complexity 2018, 2018, 596760. [Google Scholar] [CrossRef]
- Kirchhoff, G.G. Über die Auflösung der Gleichungen auf welche man bei der Untersucher der linearen Verteilung galuanischer Strome gefhrt wird. Ann. Phg. Chem. 1847, 72, 497–508. [Google Scholar] [CrossRef]
- Kelmans, A.K.; Chelnokov, V.M. A certain polynomial of a graph and graphs with an extremal number of trees. J. Comb. Theory B 1974, 16, 197–214. [Google Scholar] [CrossRef]
- Biggs, N.L. Algebraic Graph Theory, 2nd ed.; Cambridge University Press: Cambridge, UK, 1993; p. 205. [Google Scholar]
- Daoud, S.N. The Deletion—Contraction Method for Counting the Number of Spanning Trees of Graphs. Eur. Phys. J. Plus 2015, 130, 217. [Google Scholar] [CrossRef]
- Daoud, S.N. Complexity of Graphs Generated by Wheel Graph and Their Asymptotic Limits. J. Egypt. Math. Soc. 2017, 25, 424–433. [Google Scholar] [CrossRef]
- Daoud, S.N. Generating formulas of the number of spanning trees of some special graphs. Eur. Phys. J. Plus 2014, 129, 416. [Google Scholar] [CrossRef]
- Daoud, S.N. Number of Spanning Trees in Different Product of Complete and Complete Tripartite Graphs. Ars Comb. 2018, 139, 85–103. [Google Scholar]
- Liu, J.-B.; Daoud, S.N. Complexity of some of Pyramid Graphs Created from a Gear Graph. Symmetry 2018, 10, 689. [Google Scholar] [CrossRef]
- Daoud, S.N. Number of Spanning Trees of Cartesian and Composition Products of Graphs and Chebyshev Polynomials. IEEE Access 2019, 7, 71142–71157. [Google Scholar] [CrossRef]
- Teufl, E.; Wagner, S. Determinant identities for Laplace matrices. Linear Algebra Appl. 2010, 432, 441–457. [Google Scholar] [CrossRef]
- Daoud, S.N. Number of spanning Trees in the sequence of some Nonahedral graphs. Util. Math. 2020, 115, 1–18. [Google Scholar]
- Daoud, S.N.; Saleha, W. Complexity trees of the sequence of some nonahedral graphs generated by triangle. Heliyon 2020, 6, e04786. [Google Scholar] [CrossRef]
- Liu, J.-B.; Daoud, S.N. Number of Spanning Trees in the Sequence of Some Graphs. Complexity 2019, 2019, 4271783. [Google Scholar] [CrossRef]
- Wu, F.Y. Number of spanning trees on a lattice. J. Phys. A Math. Gen. 1977, 10, 113–115. [Google Scholar] [CrossRef]
- Lyons, R. Asymptotic enumeration of spanning trees. Combin. Probab. Comput. 2005, 14, 491–522. [Google Scholar] [CrossRef]
- Zhang, Z.; Wu, B.; Comellas, F. The number of spanning trees in Apollonian networks. Discret. Appl. Math. 2014, 169, 206–213. [Google Scholar] [CrossRef]
- Zhang, Z.; Liu, H.; Wu, B.; Zou, T. Spanning trees in a fractal scale—Free lattice. Phys. Rev. E 2011, 83, 016116. [Google Scholar] [CrossRef]










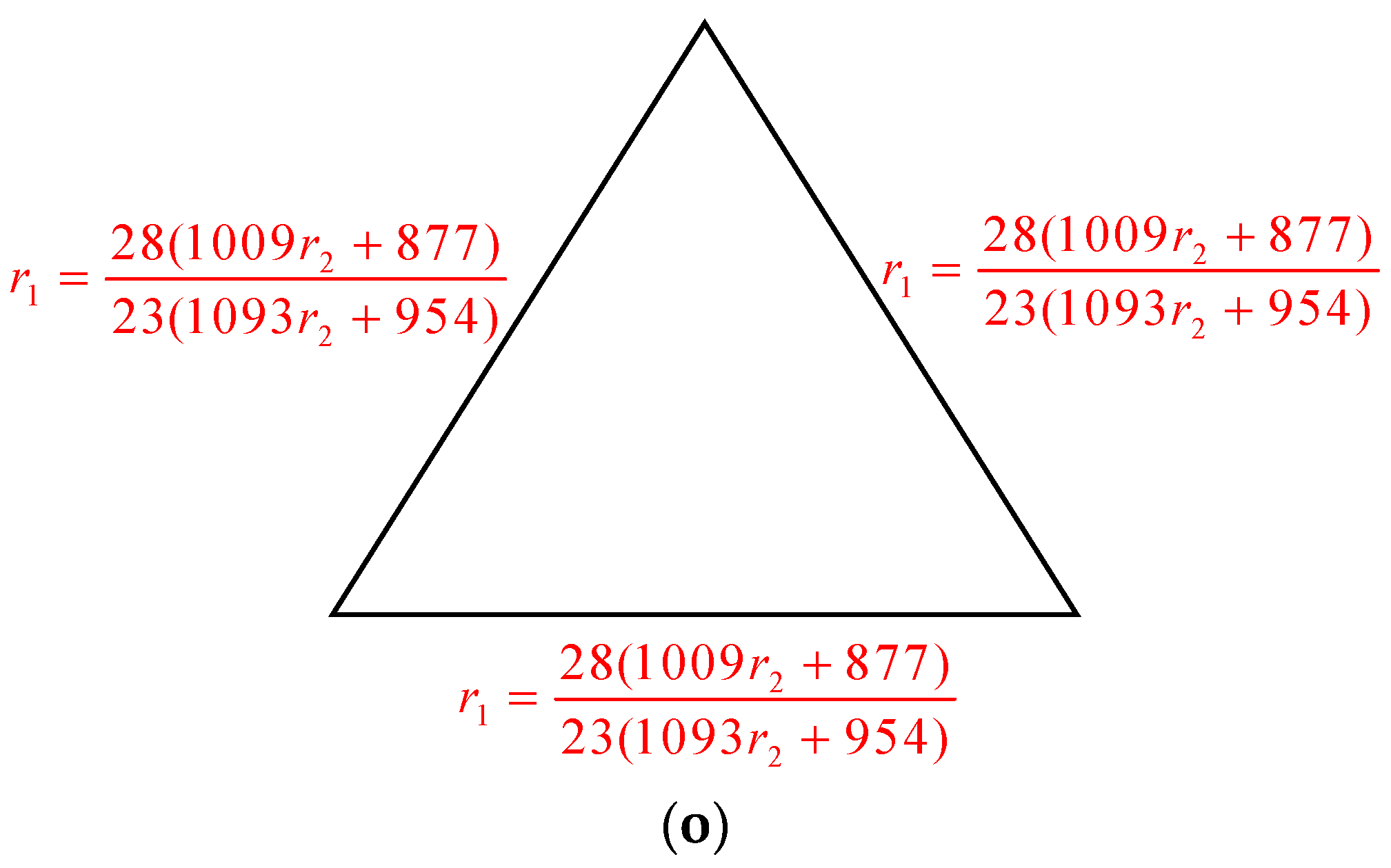
| 1 | |
| 2 | |
| 3 | |
| 4 | |
| 5 |
| 1 | |
| 2 | |
| 3 | |
| 4 | |
| 5 |
| 1 | |
| 2 | |
| 3 | |
| 4 | |
| 4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Asiri, A.; Daoud, S.N. Enumerating the Number of Spanning Trees of Pyramid Graphs Based on Some Nonahedral Graphs. Axioms 2025, 14, 148. https://doi.org/10.3390/axioms14030148
Asiri A, Daoud SN. Enumerating the Number of Spanning Trees of Pyramid Graphs Based on Some Nonahedral Graphs. Axioms. 2025; 14(3):148. https://doi.org/10.3390/axioms14030148
Chicago/Turabian StyleAsiri, Ahmad, and Salama Nagy Daoud. 2025. "Enumerating the Number of Spanning Trees of Pyramid Graphs Based on Some Nonahedral Graphs" Axioms 14, no. 3: 148. https://doi.org/10.3390/axioms14030148
APA StyleAsiri, A., & Daoud, S. N. (2025). Enumerating the Number of Spanning Trees of Pyramid Graphs Based on Some Nonahedral Graphs. Axioms, 14(3), 148. https://doi.org/10.3390/axioms14030148







