Comparative Analysis of Exact Methods for Testing Equivalence of Prevalences in Bilateral and Unilateral Combined Data with and without Assumptions of Correlation
Abstract
1. Introduction
2. Equal Correlation Coefficients Model
3. Individual Site Model without Considering Correlation
4. Methods for Individual Site Model
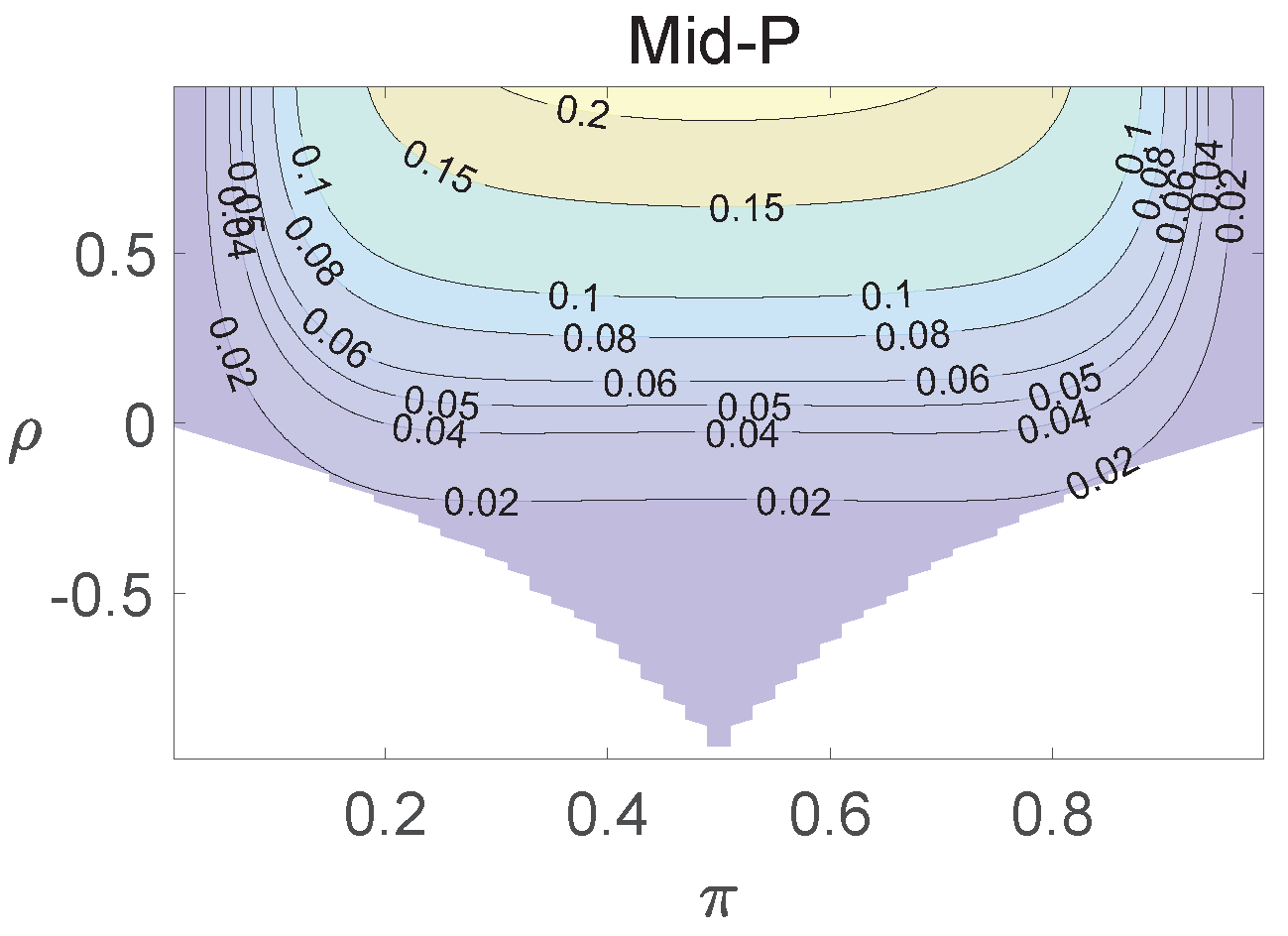
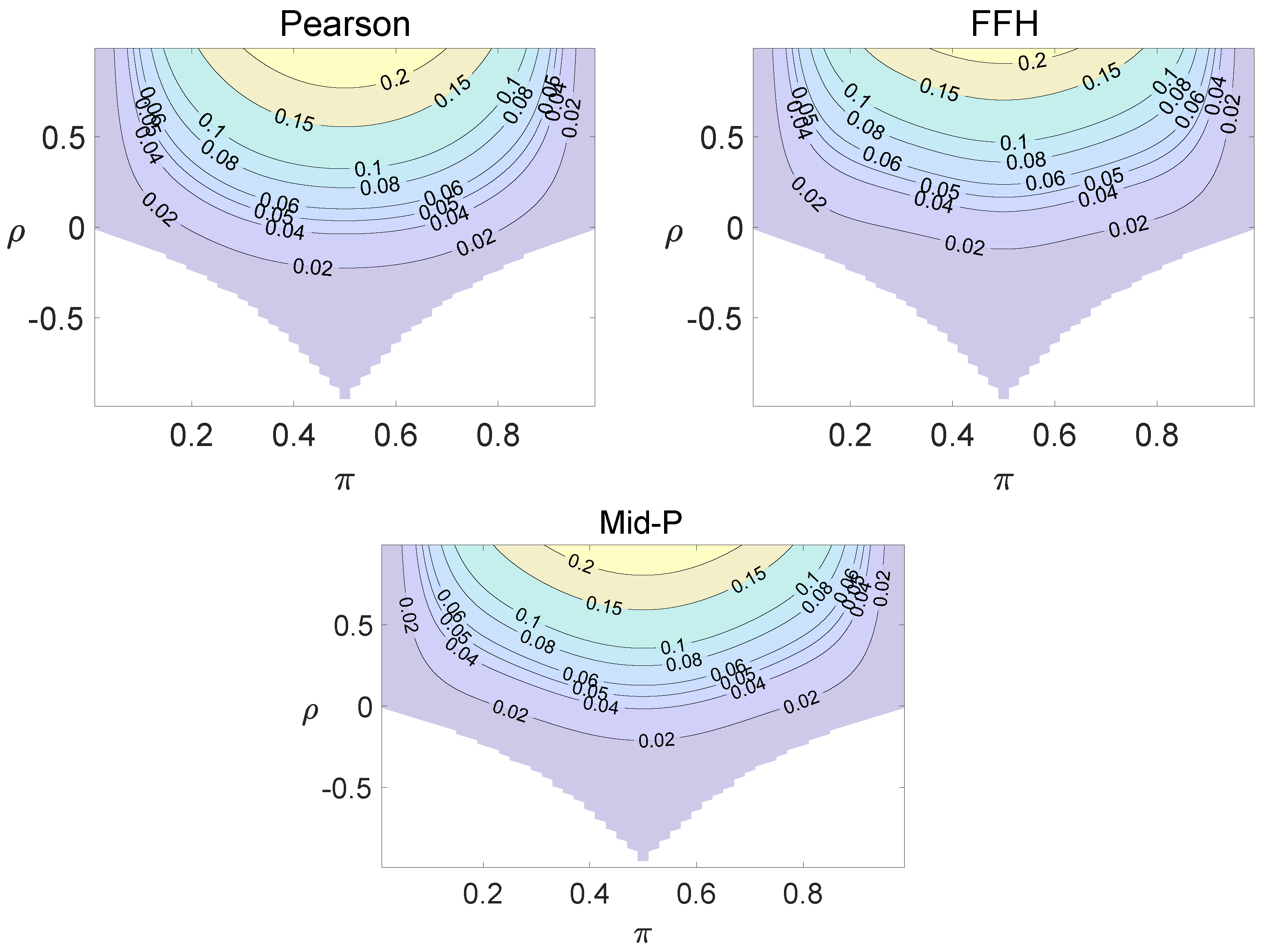
4.1. The Pearson Chi-Squared Test
4.2. The Fisher–Freeman–Halton Exact Test
4.3. The Mid-P Test
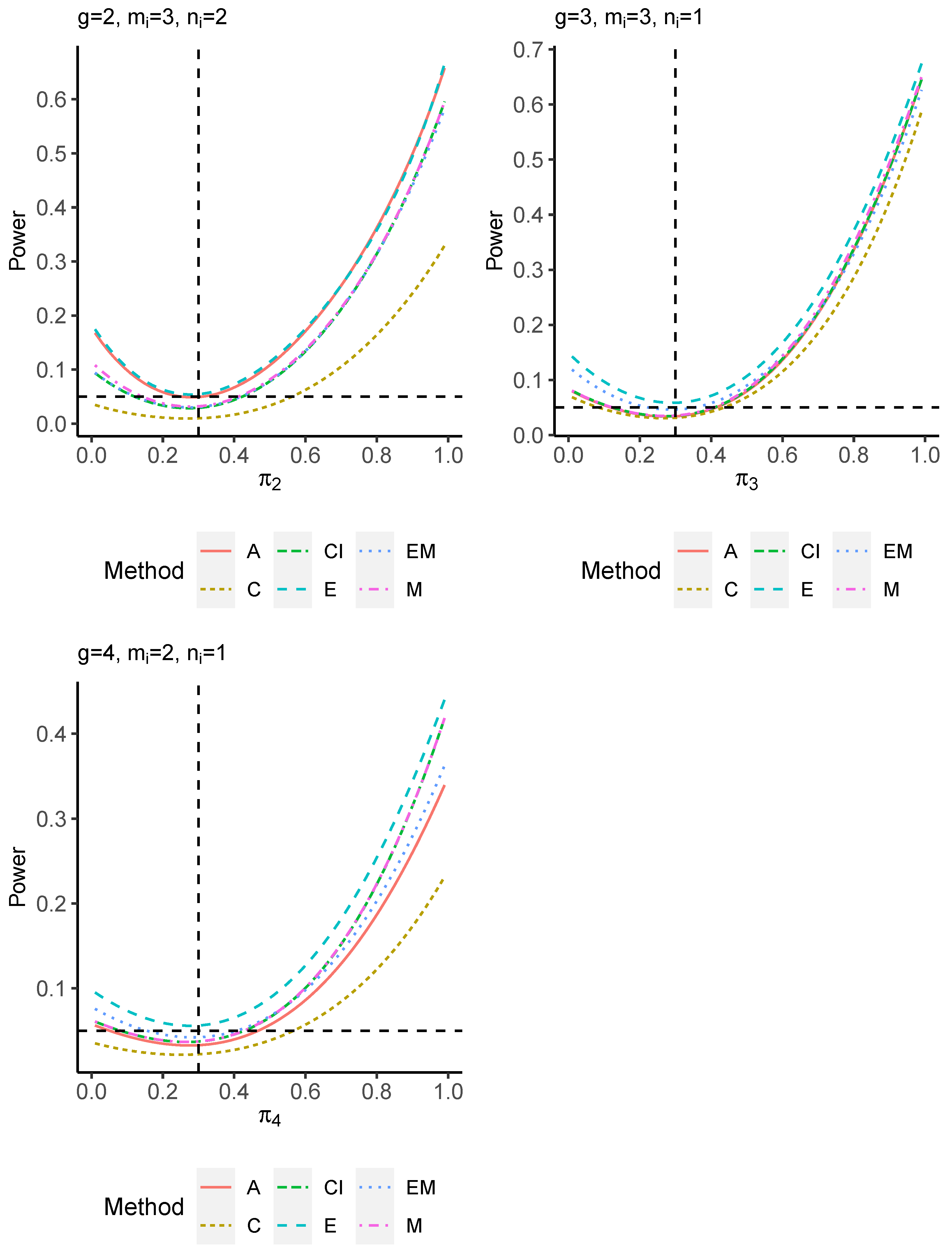
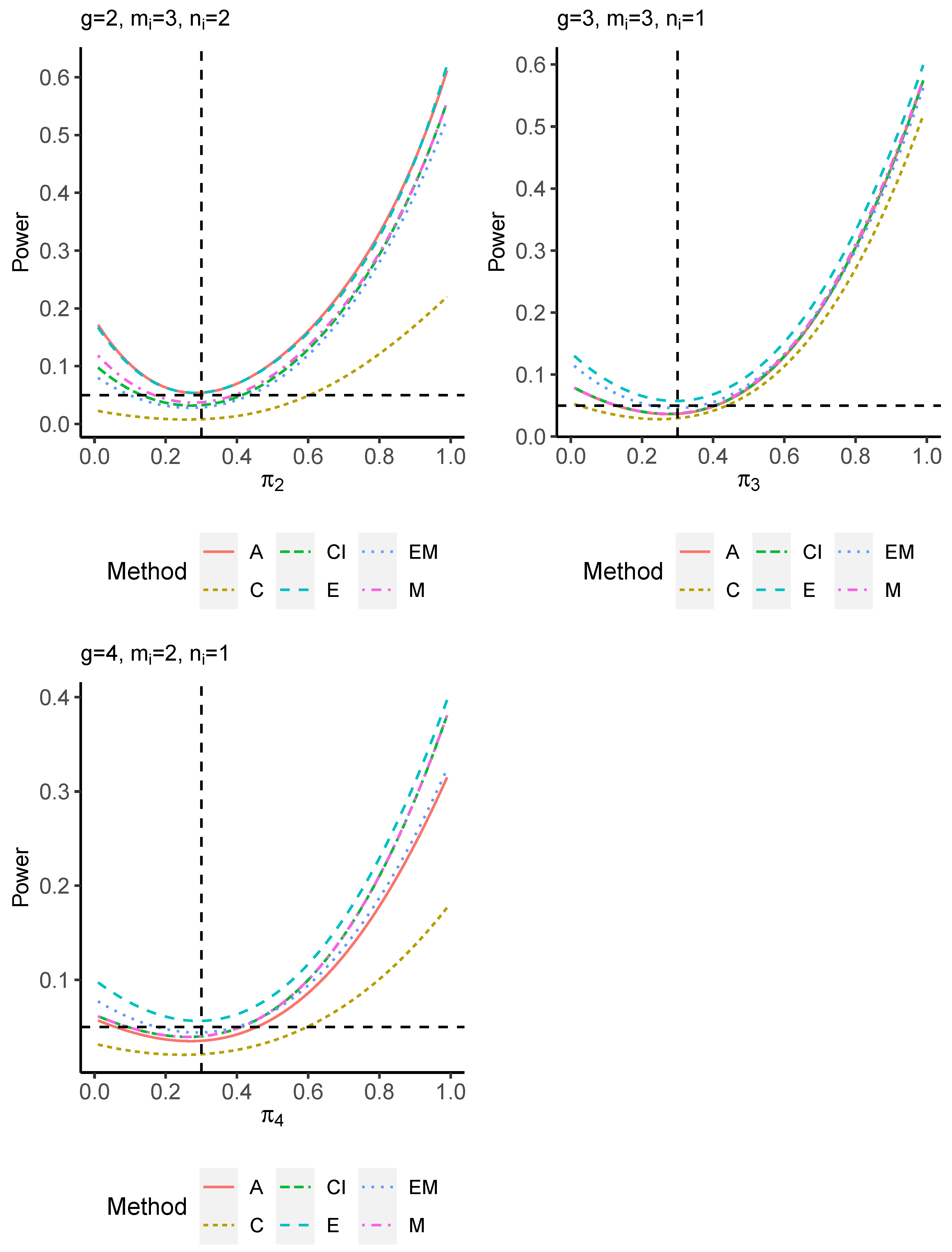
5. Methods for Equal Correlation Coefficients Model
5.1. Score Test
5.2. E Method
5.3. M Method
5.4. E + M Method
5.5. CI Method
- (1)
- Set as the starting point of , where is the constrained MLE of for . Initialize flag = 1 and stepsize = so that the updated upper bound does not prematurely exceed 1;
- (2)
- Update + flag × stepsize and calculate the conditional MLE given . Then, the score test statistic is given by ;
- (3)
- If , where is the quantile of the chi-square distribution with one degree of freedom, turn to the opposite searching direction by letting flag = −1 and reduce the stepsize by multiplying it by , then return to step (2). Otherwise, keep flag = 1 and return to step (2);
- (4)
- Repeat steps (2) and (3) until the stepsize is sufficiently small (e.g., ).
- (1)
- Set as the starting point of , where is the constrained MLE of for . Initialize flag = 1 and stepsize = ;
- (2)
- Update + flag × stepsize and calculate the conditional MLE given . Then, the score test statistic is given by ;
- (3)
- If , turn to the opposite searching direction by letting flag = −1 and reduce the stepsize by multiplying it by , then return to step (2). Otherwise, keep flag = 1 and return to step (2);
- (4)
- Repeat steps (2) and (3) until the stepsize is sufficiently small (e.g., ).
5.6. C Method
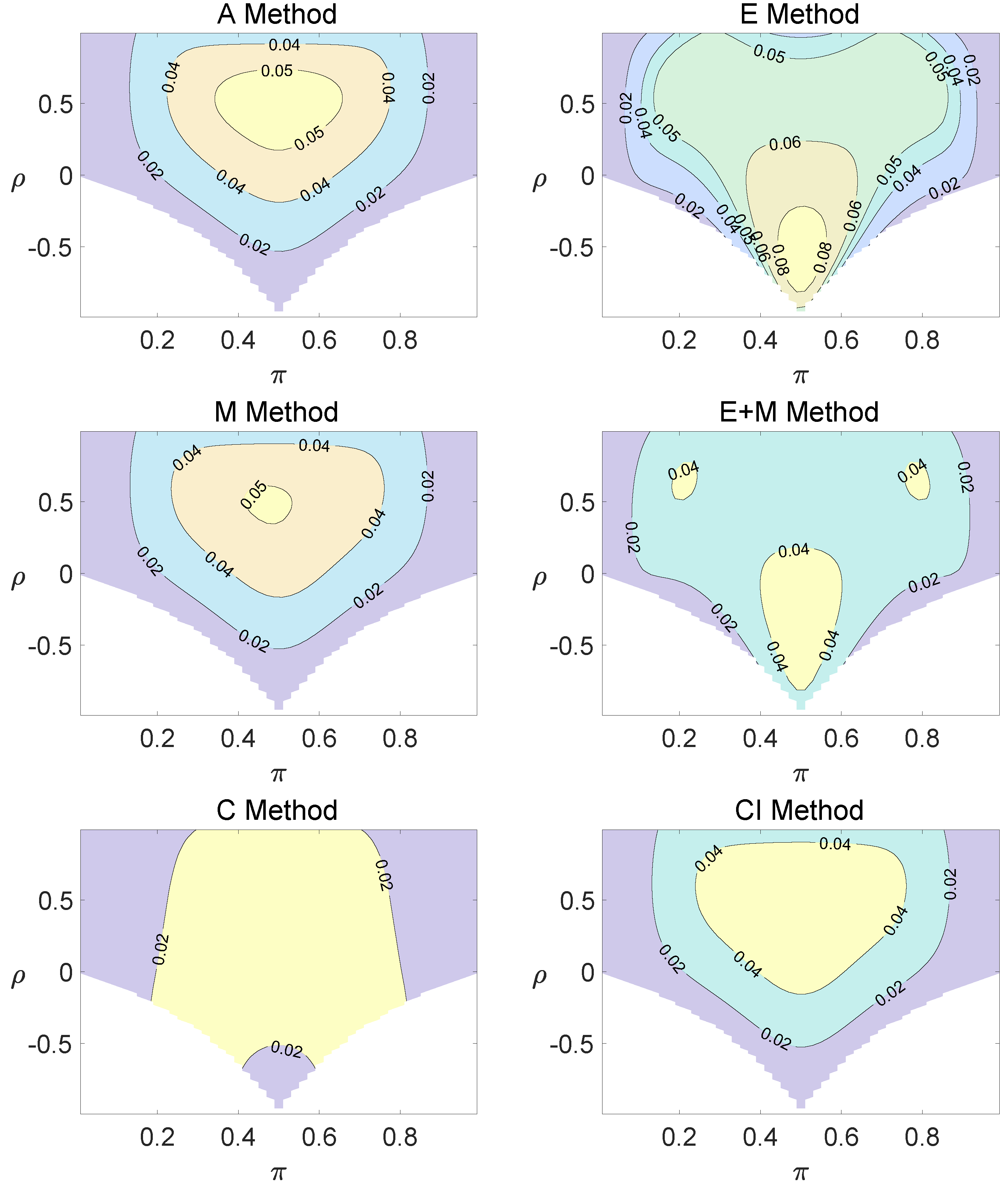
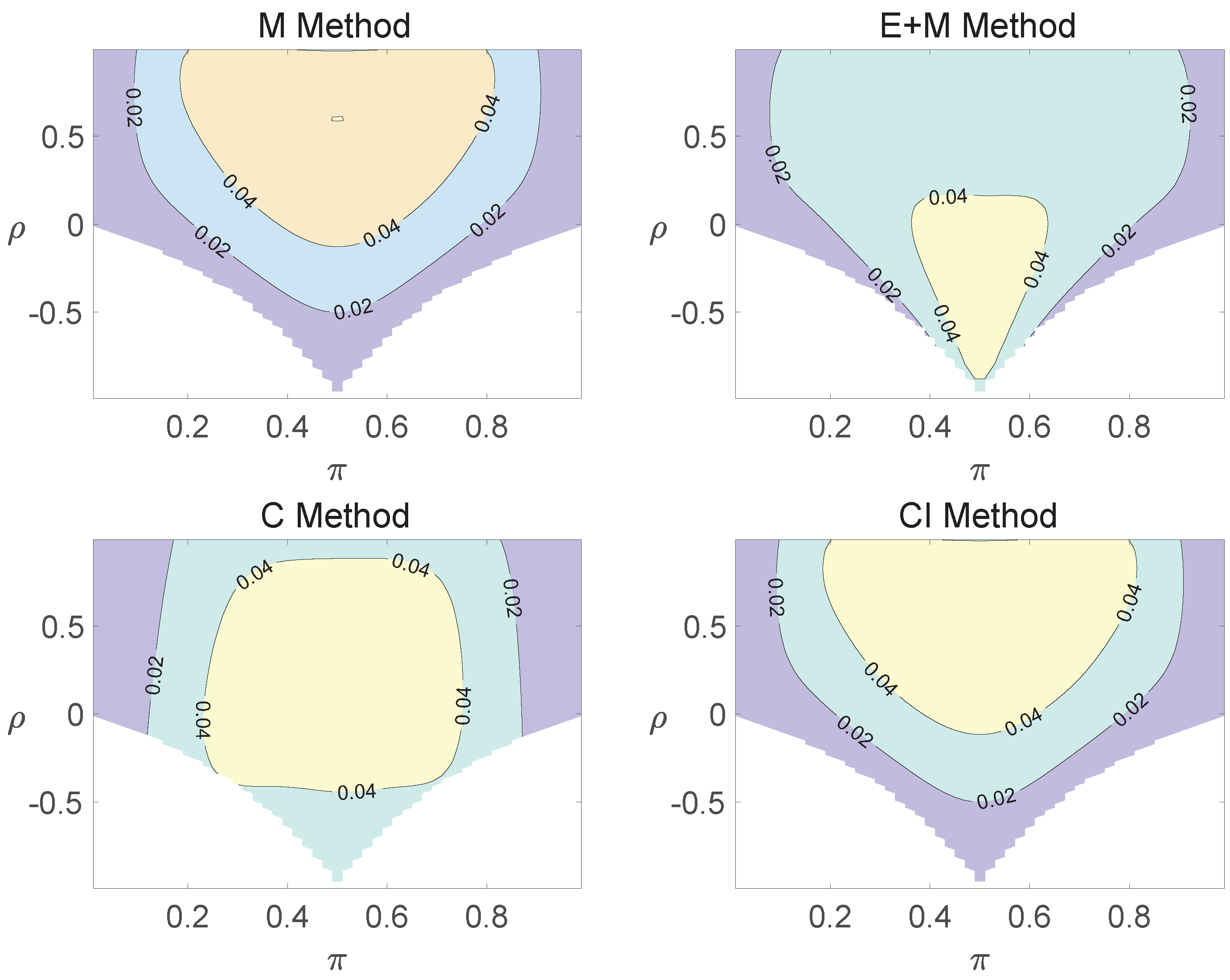
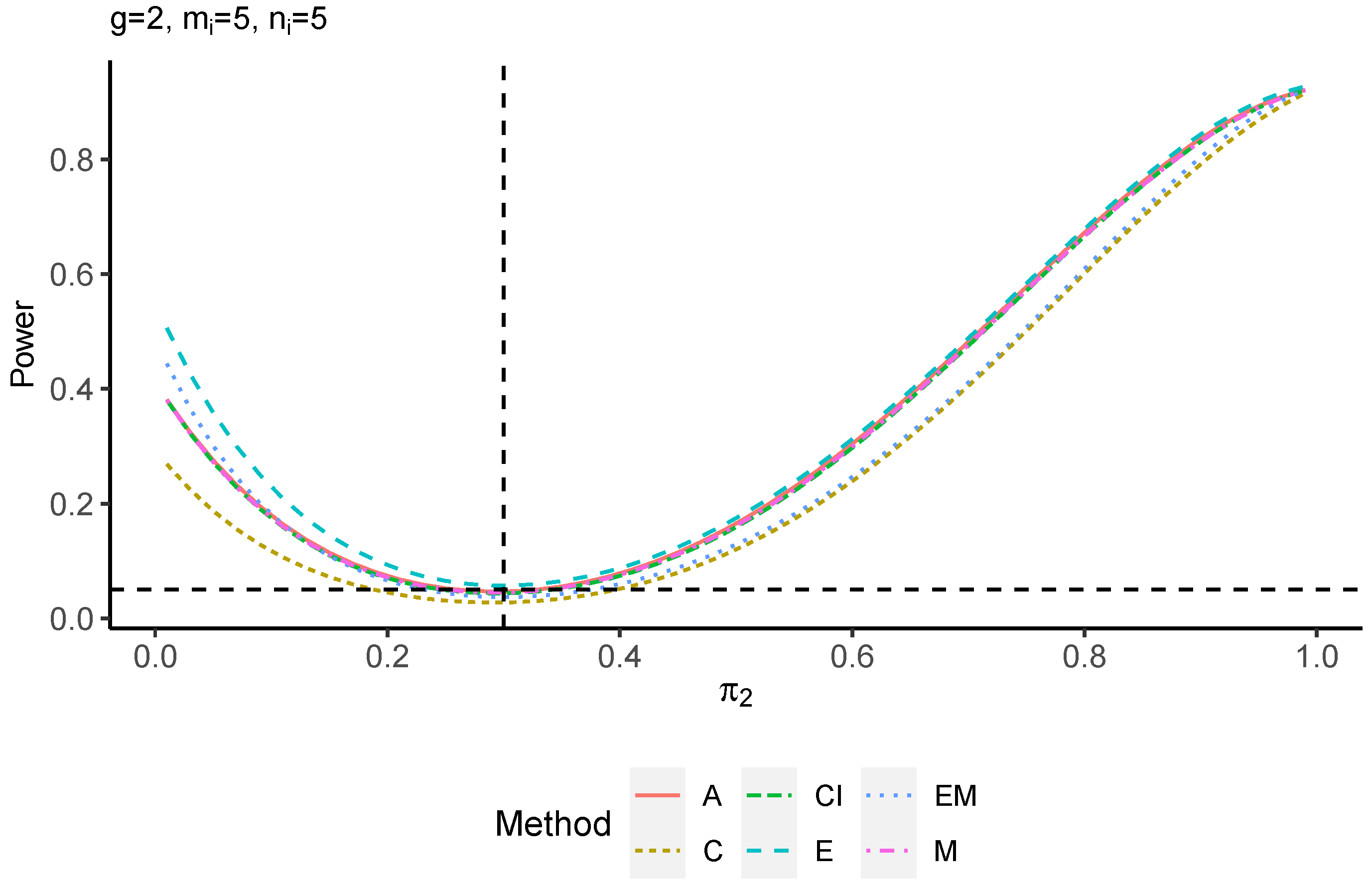
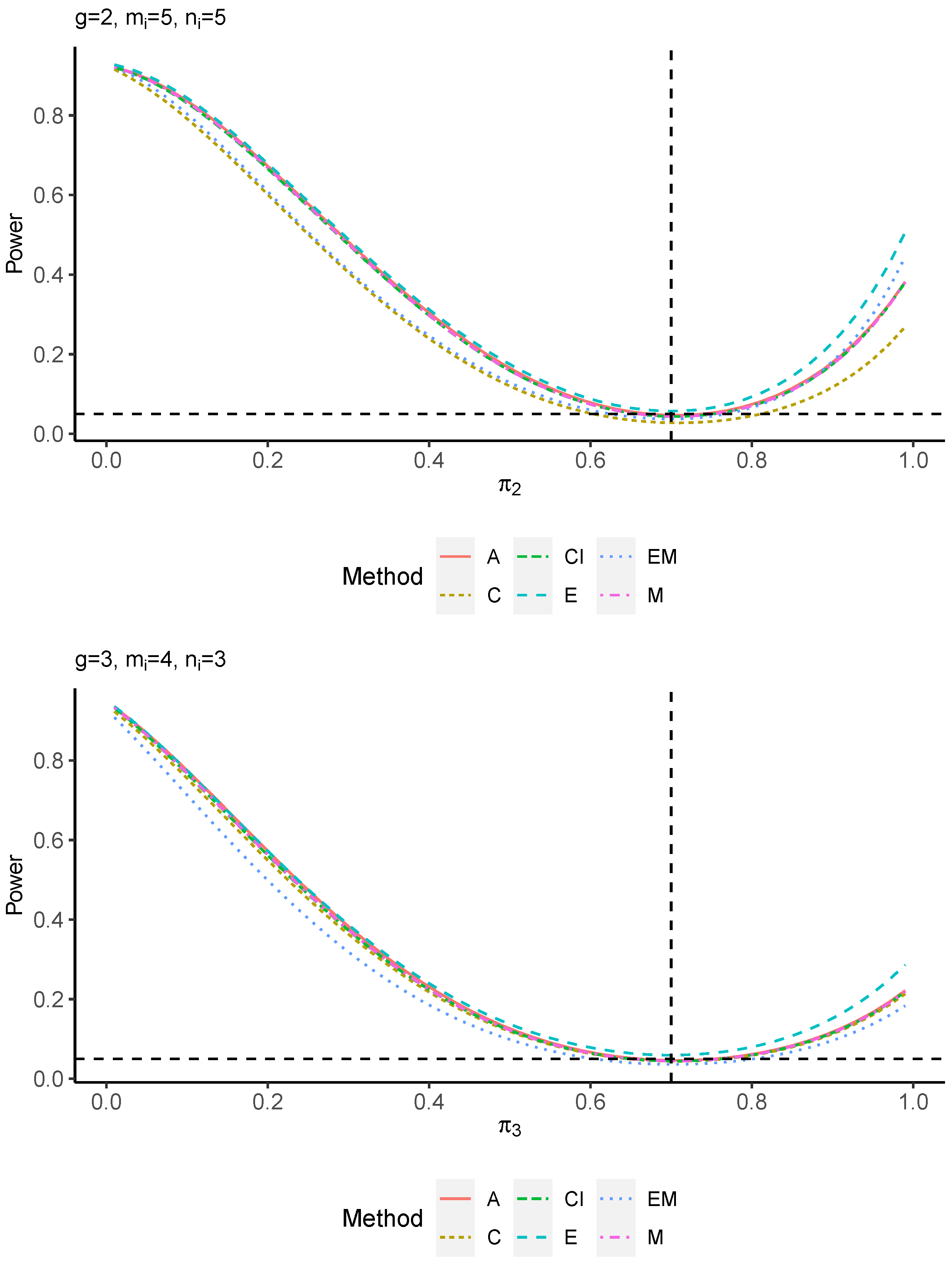
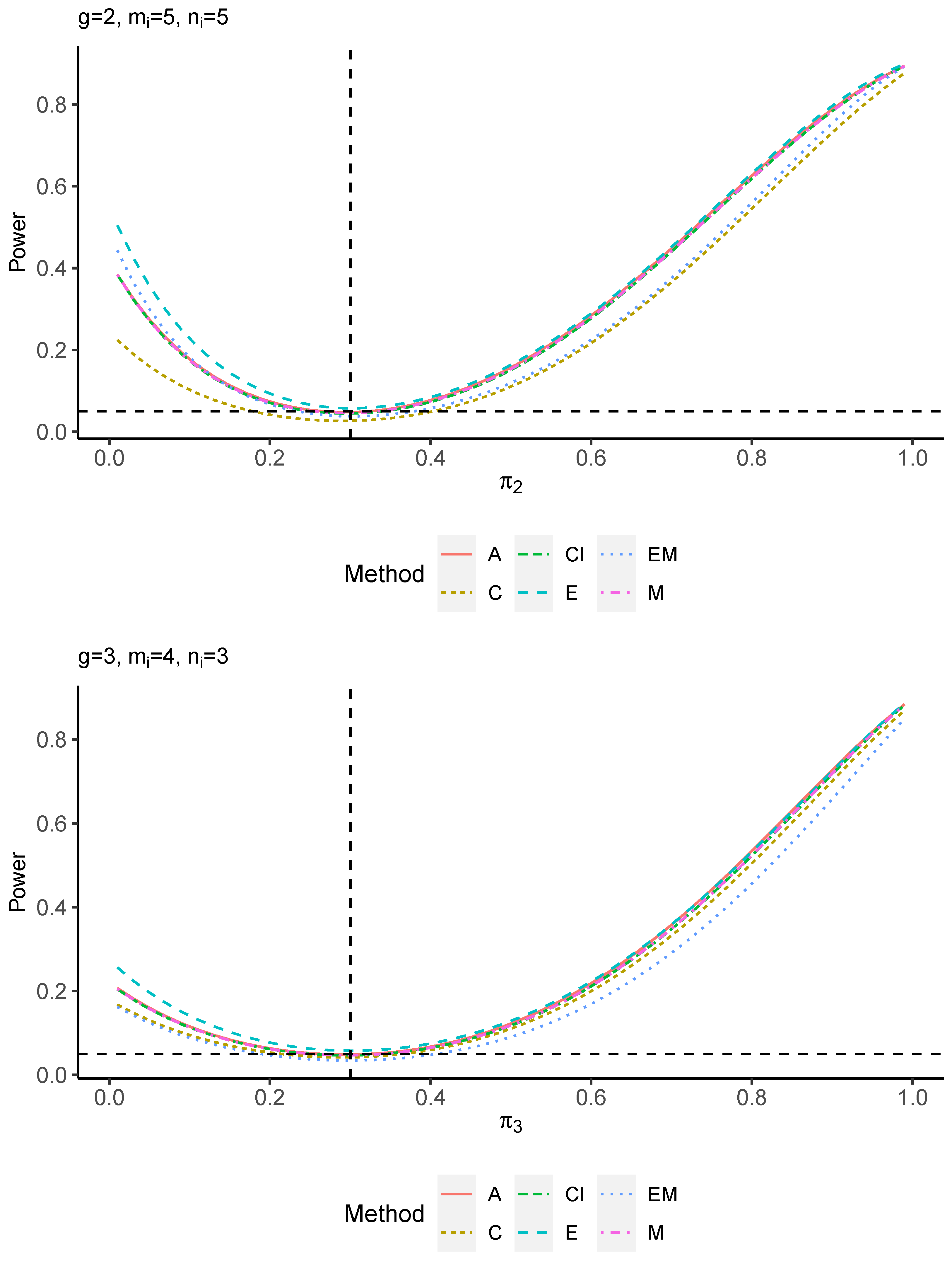
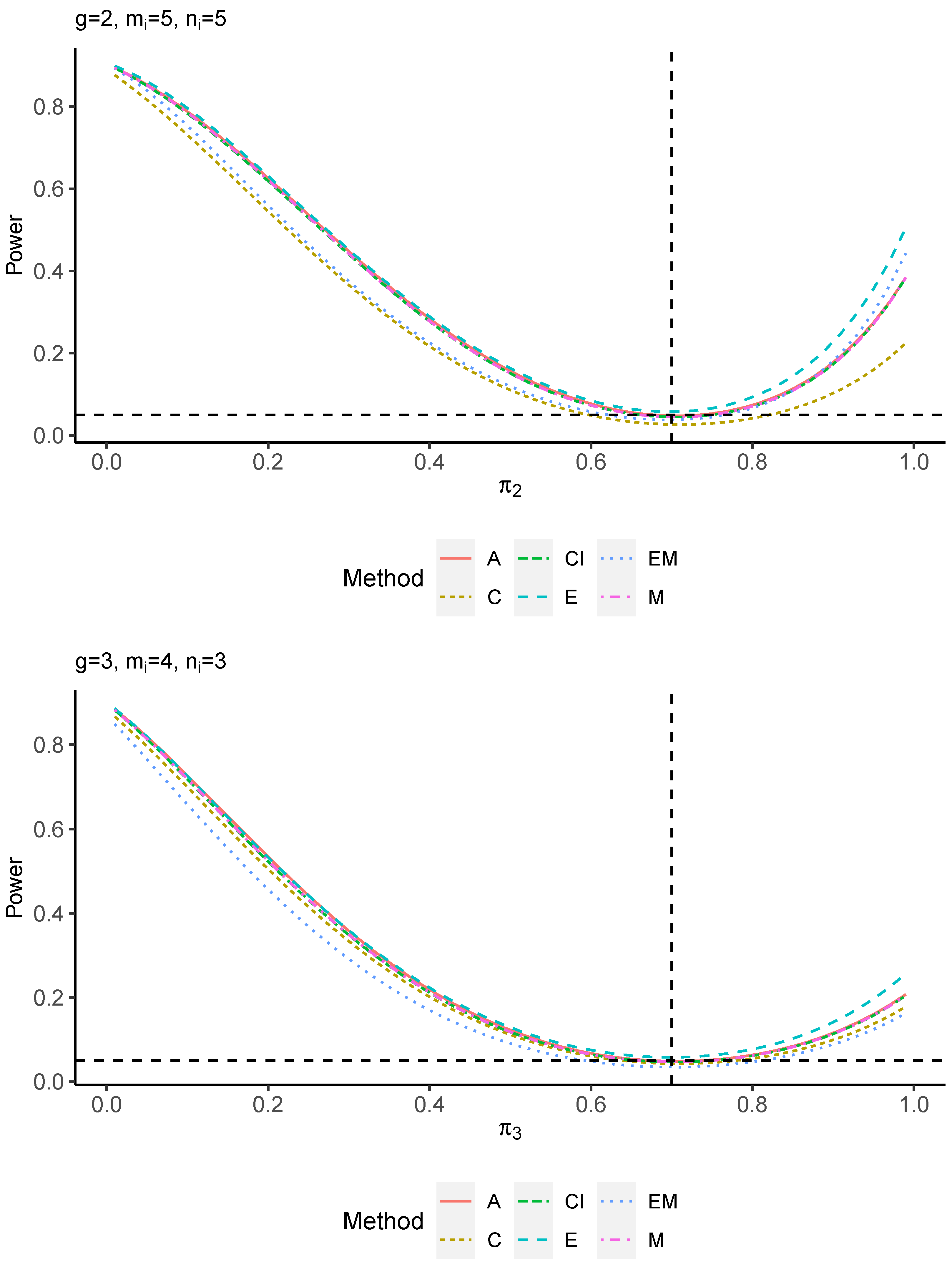
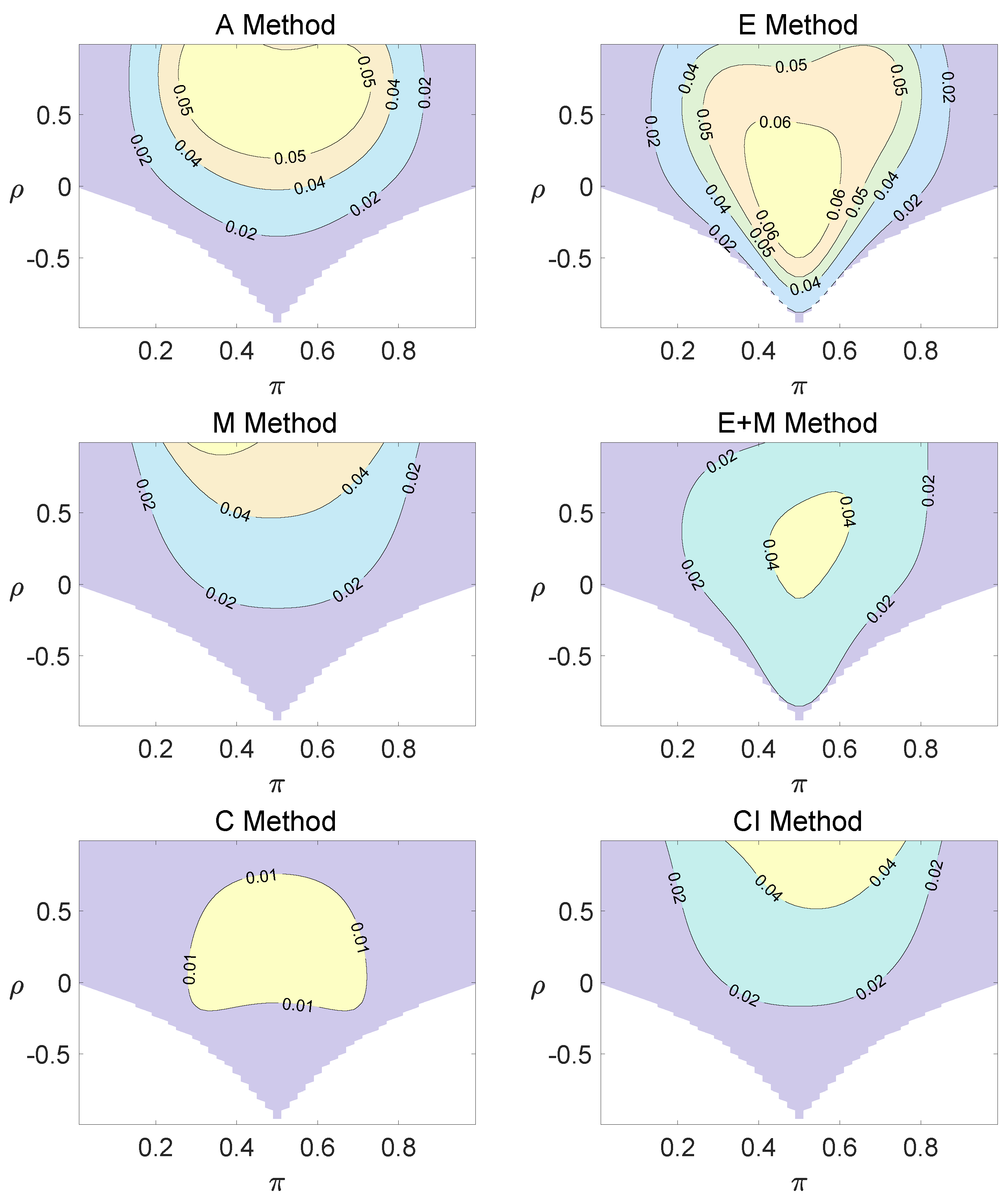
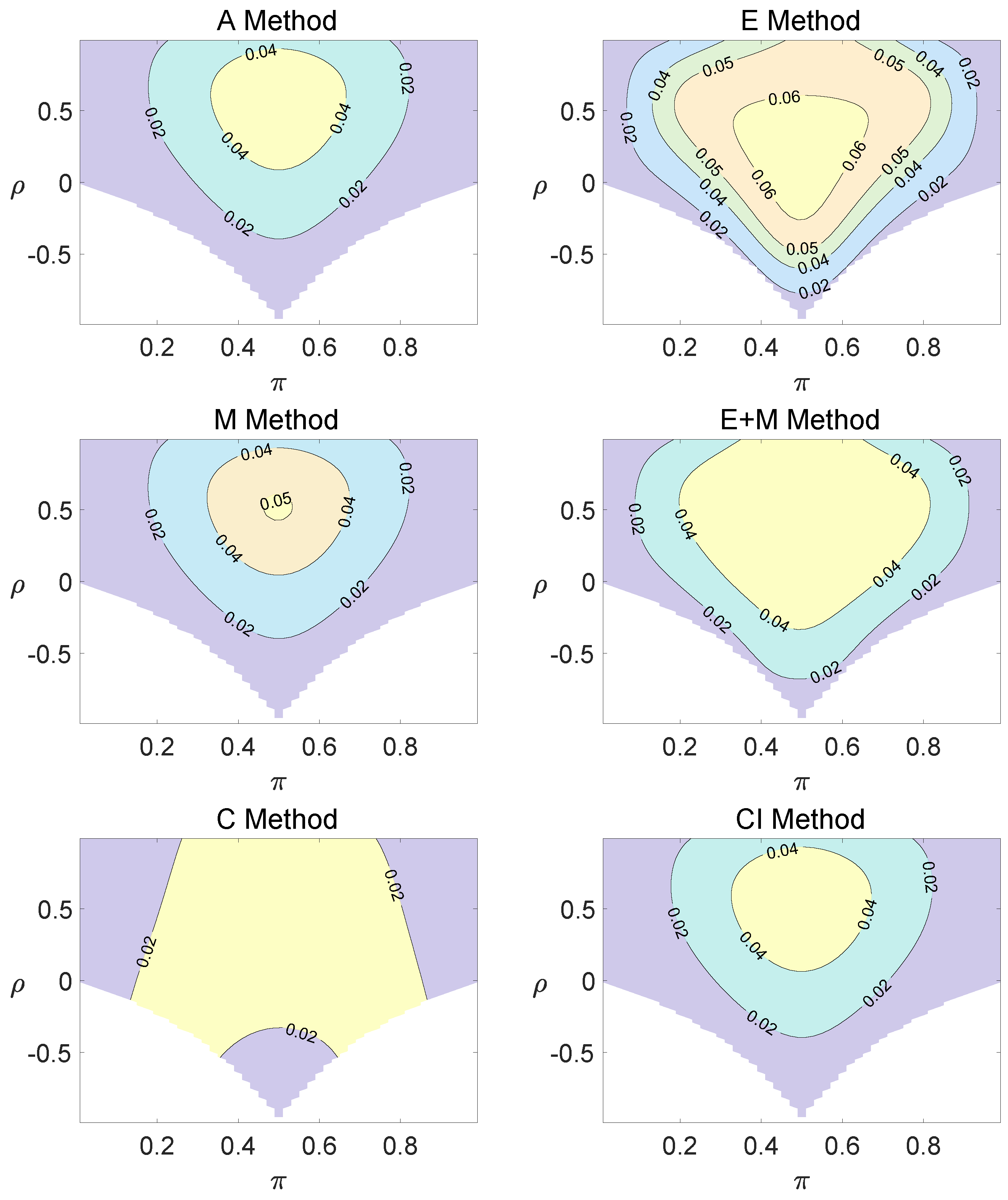
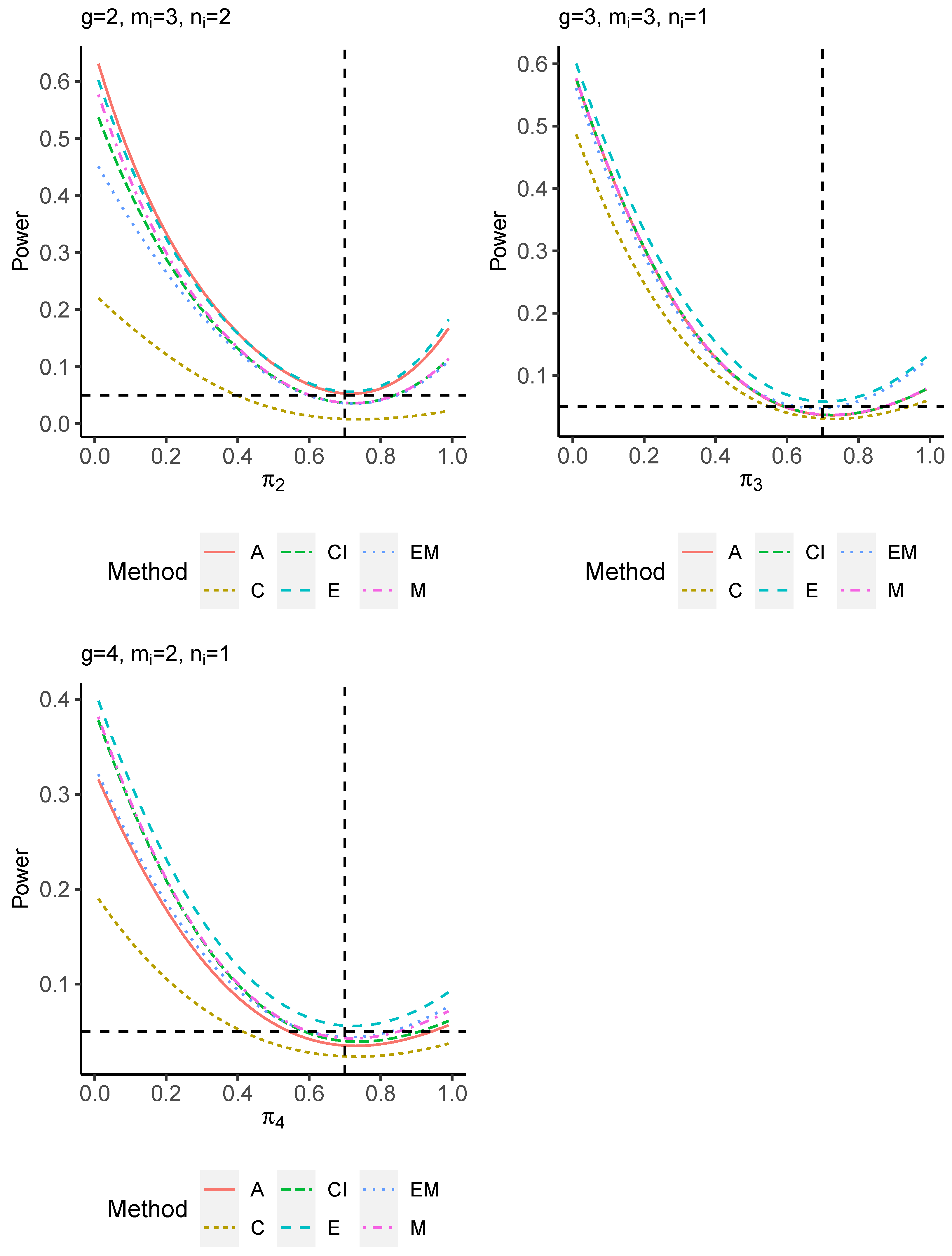
6. Numerical Study
7. Real Examples
8. Discussion
9. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B




References
- Zhang, H.G.; Ying, G.S. Statistical approaches in published ophthalmic clinical science papers: A comparison to statistical practice two decades ago. Br. J. Ophthalmol. 2018, 102, 1188–1191. [Google Scholar] [CrossRef] [PubMed]
- Pearson, K.X. On the criterion that a given system of deviations from the probable in the case of a correlated system of variables is such that it can be reasonably supposed to have arisen from random sampling. London, Edinburgh, Dublin Philos. Mag. J. Sci. 1900, 50, 157–175. [Google Scholar] [CrossRef]
- Rosner, B. Statistical methods in ophthalmology: An adjustment for the intraclass correlation between eyes. Biometrics 1982, 38, 105–114. [Google Scholar] [CrossRef] [PubMed]
- Donner, A.; Banting, D. Analysis of site-specific data in dental studies. J. Dent. Res. 1988, 67, 1392–1395. [Google Scholar] [CrossRef] [PubMed]
- Donner, A. Statistical methods in ophthalmology: An adjusted chi-square approach. Biometrics 1989, 45, 605–611. [Google Scholar] [CrossRef] [PubMed]
- Ma, C.X.; Liu, S. Testing equality of proportions for correlated binary data in ophthalmologic studies. J. Biopharm. Stat. 2017, 27, 611–619. [Google Scholar] [CrossRef] [PubMed]
- Mou, K.; Li, Z. Homogeneity Test of Many-to-One Risk Differences for Correlated Binary Data under Optimal Algorithms. Complexity 2021, 2021, 6685951. [Google Scholar] [CrossRef]
- Liu, X.; Yang, Z.; Liu, S.; Ma, C.X. Exact methods of testing the homogeneity of prevalences for correlated binary data. J. Stat. Comput. Simul. 2017, 87, 3021–3039. [Google Scholar] [CrossRef]
- Liddell, D. Practical Tests of 2Times2 Contingency Tables. J. R. Stat. Soc. Ser. D (Stat.) 1976, 25, 295–304. [Google Scholar]
- Basu, D. On the Elimination of Nuisance Parameters. J. Am. Stat. Assoc. 1977, 72, 279–290. [Google Scholar] [CrossRef]
- Lloyd, C.J. Exact P-Values Discret. Model. Obtained Estim. Maximization. Aust. N. Z. J. Stat. 2008, 50, 329–345. [Google Scholar] [CrossRef]
- Ma, C.X.; Wang, H. Testing the equality of proportions for combined unilateral and bilateral data under equal intraclass correlation model. Stat. Biopharm. Res. 2022, 15, 608–617. [Google Scholar] [CrossRef]
- Fagerland, M.; Lydersen, S.; Laake, P. Statistical Analysis of Contingency Tables; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Rao, C.R. Large sample tests of statistical hypotheses concerning several parameters with applications to problems of estimation. Math. Proc. Camb. Philos. Soc. 1948, 44, 50–57. [Google Scholar]
- Berger, R.L.; Boos, D.D. P values maximized over a confidence set for the nuisance parameter. J. Am. Stat. Assoc. 1994, 89, 1012–1016. [Google Scholar] [CrossRef]
- Silvapulle, M.J. A test in the presence of nuisance parameters. J. Am. Stat. Assoc. 1996, 91, 1690–1693. [Google Scholar] [CrossRef]
- Vexler, A. Valid P-Values Expect. p-Values Revisited.Ann. Inst. Stat. Math. 2021, 73, 227–248. [Google Scholar] [CrossRef]
- Tang, N.S.; Tang, M.L.; Qiu, S.F. Testing the equality of proportions for correlated otolaryngologic data. Comput. Stat. Data Anal. 2008, 52, 3719–3729. [Google Scholar] [CrossRef]
- Mandel, E.M.; Bluestone, C.D.; Rockette, H.E.; BLATTER, M.M.; Reisinger, K.S.; Wucher, F.P.; Harper, J. Duration of effusion after antibiotic treatment for acute otitis media: Comparison of cefaclor and amoxicillin. Pediatr. Infect. Dis. J. 1982, 1, 310–316. [Google Scholar] [CrossRef] [PubMed]
- Liang, S.; Fang, K.T.; Huang, X.W.; Xin, Y.; Ma, C. Homogeneity Tests and Interval Estimations of Risk Differences for Stratified Bilateral and Unilateral Correlated Data. arXiv 2023, arXiv:2304.00162. [Google Scholar] [CrossRef]
- Lu, W.; Ning, R.; Diao, K.; Ding, Y.; Chen, R.; Zhou, L.; Lian, Y.; McAlinden, C.; Sanders, F.W.; Xia, F.; et al. Comparison of two main orthokeratology lens designs in efficacy and safety for myopia control. Front. Med. 2022, 9, 798314. [Google Scholar] [CrossRef]
- Rose, L.V.; Schulz, A.M.; Graham, S.L. Use baseline axial length measurements in myopic patients to predict the control of myopia with and without atropine 0.01%. PLoS ONE 2021, 16, e0254061. [Google Scholar] [CrossRef] [PubMed]
- Berson, E.L.; Rosner, B.; Simonoff, E. Risk factors for genetic typing and detection in retinitis pigmentosa. Am. J. Ophthalmol. 1980, 89, 763–775. [Google Scholar] [CrossRef] [PubMed]
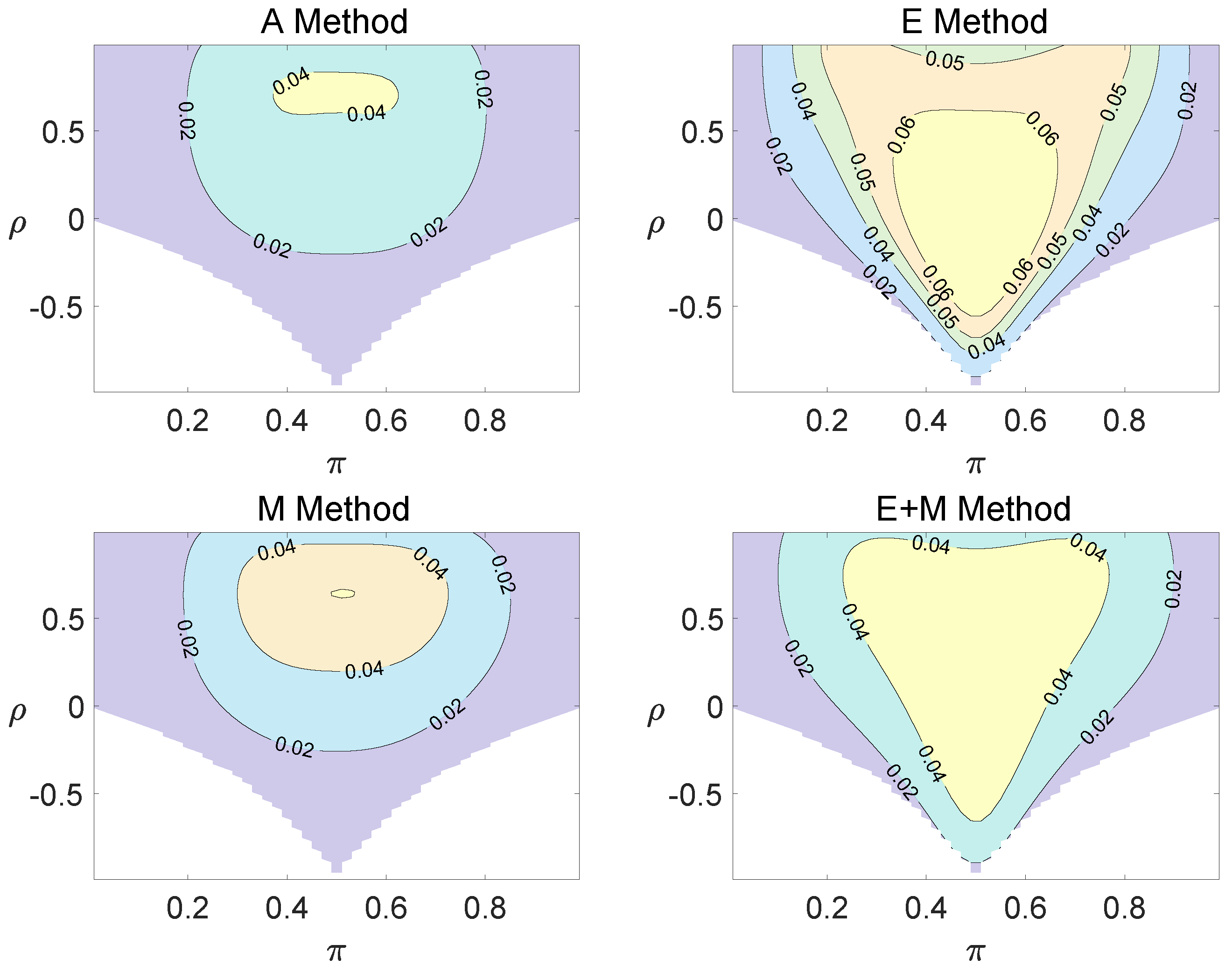
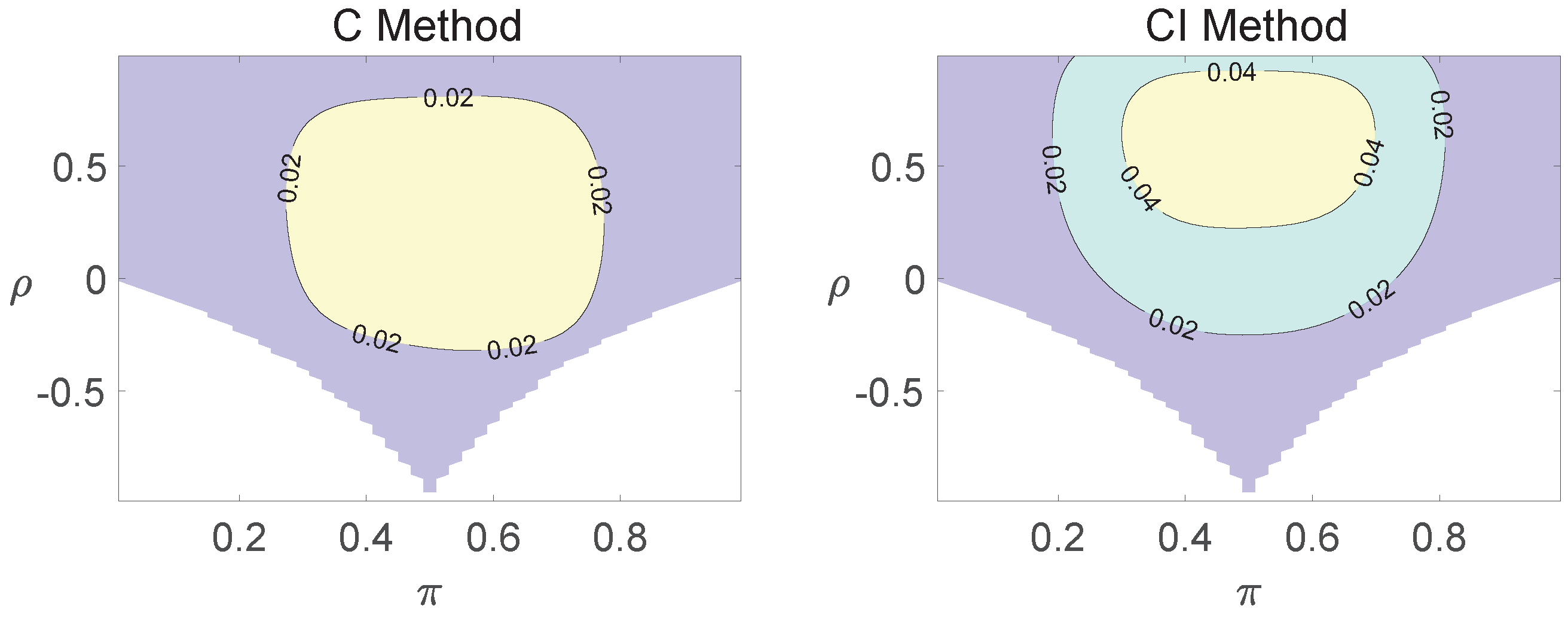
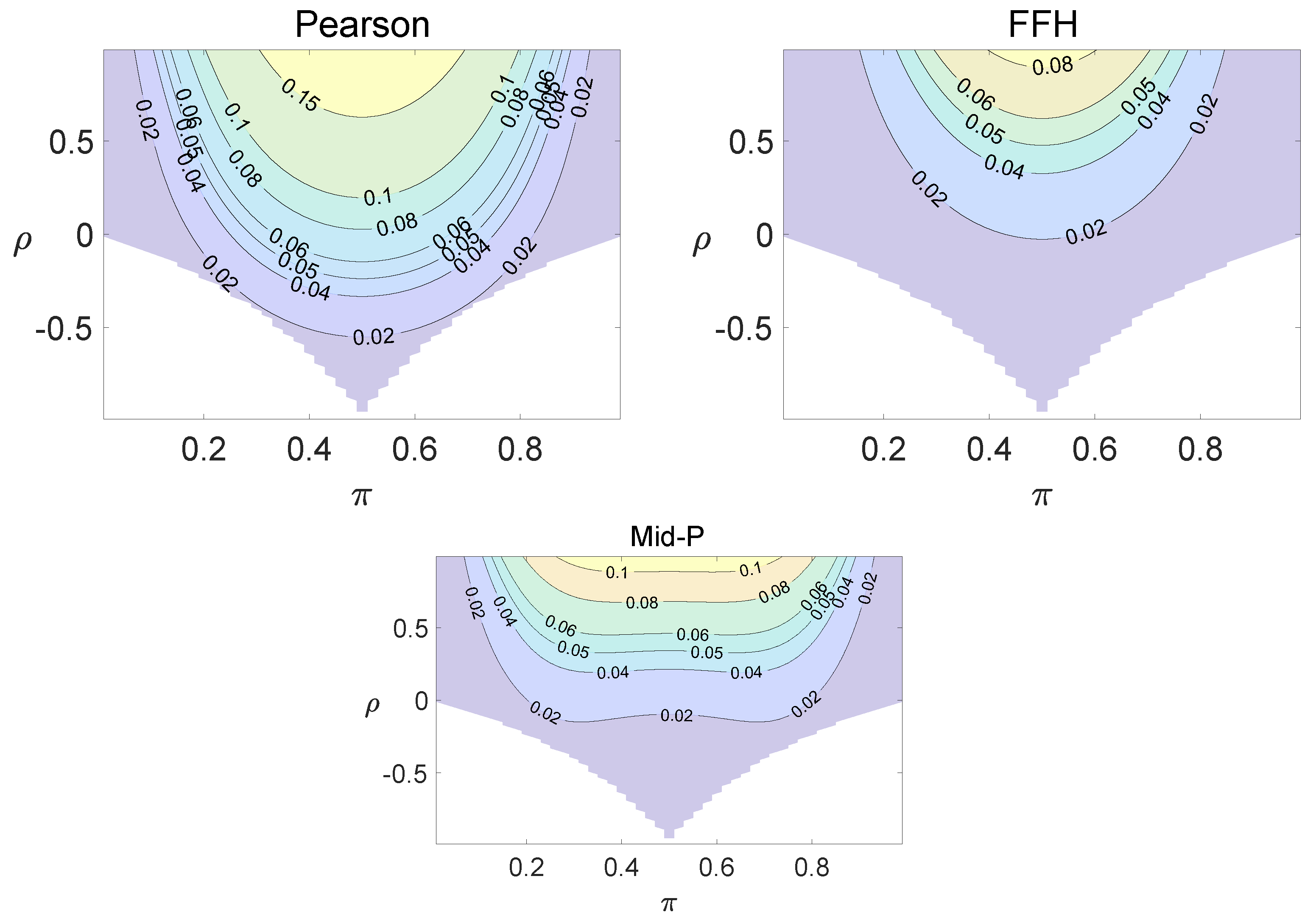
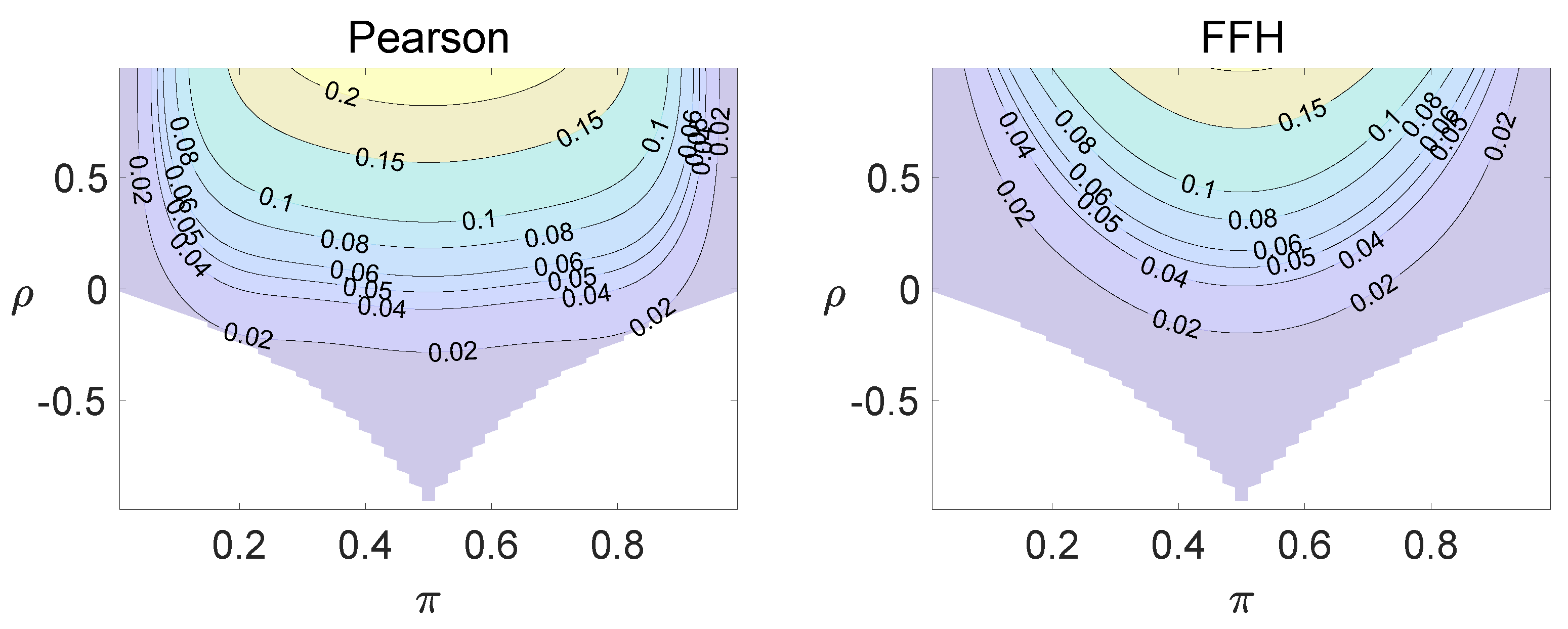

| Group (i) | 1 | 2 | … | g | Total | ||
|---|---|---|---|---|---|---|---|
| Bilateral | Response (r) | 0 | … | ||||
| 1 | |||||||
| 2 | |||||||
| Total | … | ||||||
| Unilateral | Response () | 0 | … | ||||
| 1 | |||||||
| Total | … | ||||||
| Group (i) | 1 | 2 | … | g | Total | |
|---|---|---|---|---|---|---|
| Response (e) | 0 | … | ||||
| 1 | … | |||||
| Total | … | T | ||||
| Treatment | Bilateral at Entry | Unilateral at Entry | |||
| No. of Cured Ears | No. of Cured Ears | ||||
| 0 | 1 | 2 | 0 | 1 | |
| Cefaclor | 0 | 1 | 3 | 8 | 11 |
| Amoxicillin | 1 | 0 | 6 | 7 | 11 |
| Approach | p-Value |
|---|---|
| A | 0.6629 |
| E | 0.6712 |
| M | 0.7218 |
| E + M | 0.8608 |
| C | 0.7667 |
| CI | 0.7197 |
| Design | Bilateral | Unilateral | |||
| No. of Eyes with Response | No. of Eyes with Response | ||||
| 0 | 1 | 2 | 0 | 1 | |
| VST | 9 | 3 | 7 | 2 | 1 |
| CRT | 7 | 0 | 0 | 0 | 0 |
| Approach | p-Value |
|---|---|
| A | 0.0188 |
| E | 0.0171 |
| M | 0.0220 |
| E + M | 0.0249 |
| C | 0.0265 |
| CI | 0.0219 |
| Design | Bilateral | Unilateral | |||
| No. of Eyes with Response | No. of Eyes with Response | ||||
| 0 | 1 | 2 | 0 | 1 | |
| DOM | 15 | 6 | 7 | 0 | 0 |
| AR | 7 | 5 | 9 | 0 | 0 |
| SL | 3 | 2 | 14 | 0 | 0 |
| Approach | p-Value |
|---|---|
| A | 0.0048 |
| E | 0.0042 |
| M | 0.0044 |
| E + M | NA |
| C | 0.0045 |
| CI | 0.0047 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, S.; Ma, C. Comparative Analysis of Exact Methods for Testing Equivalence of Prevalences in Bilateral and Unilateral Combined Data with and without Assumptions of Correlation. Axioms 2024, 13, 430. https://doi.org/10.3390/axioms13070430
Liang S, Ma C. Comparative Analysis of Exact Methods for Testing Equivalence of Prevalences in Bilateral and Unilateral Combined Data with and without Assumptions of Correlation. Axioms. 2024; 13(7):430. https://doi.org/10.3390/axioms13070430
Chicago/Turabian StyleLiang, Shuyi, and Changxing Ma. 2024. "Comparative Analysis of Exact Methods for Testing Equivalence of Prevalences in Bilateral and Unilateral Combined Data with and without Assumptions of Correlation" Axioms 13, no. 7: 430. https://doi.org/10.3390/axioms13070430
APA StyleLiang, S., & Ma, C. (2024). Comparative Analysis of Exact Methods for Testing Equivalence of Prevalences in Bilateral and Unilateral Combined Data with and without Assumptions of Correlation. Axioms, 13(7), 430. https://doi.org/10.3390/axioms13070430








