Extremal Bicyclic Graphs with Respect to Permanental Sums and Hosoya Indices
Abstract
1. Introduction
2. Some Preliminaries
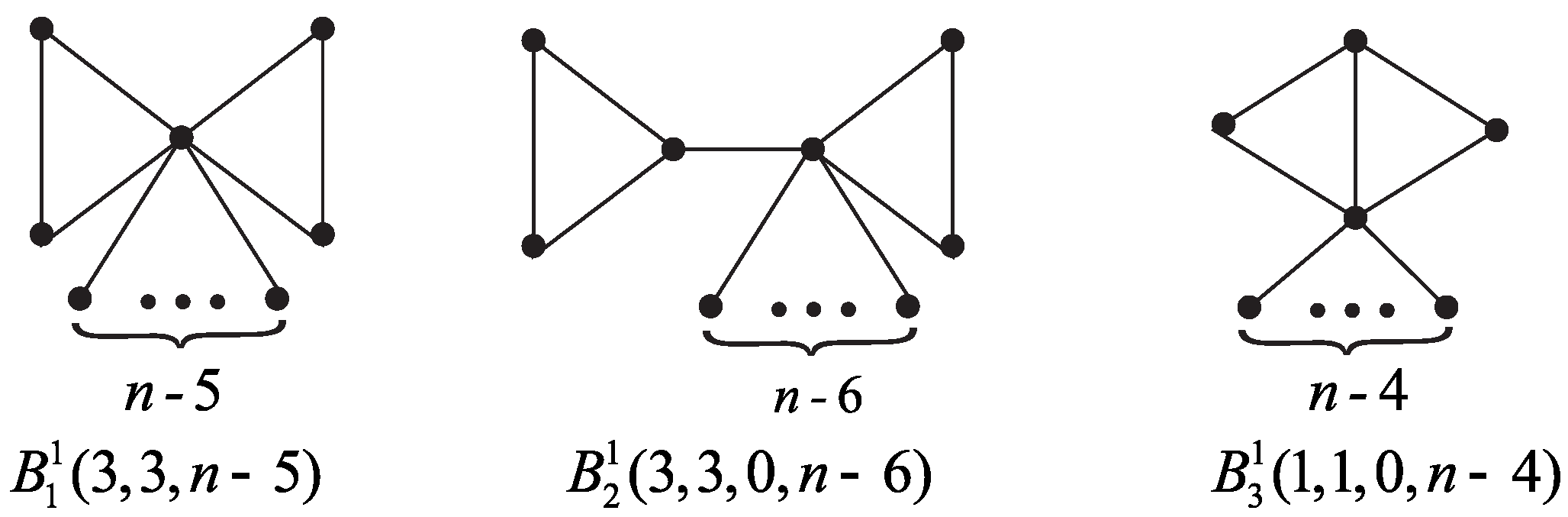
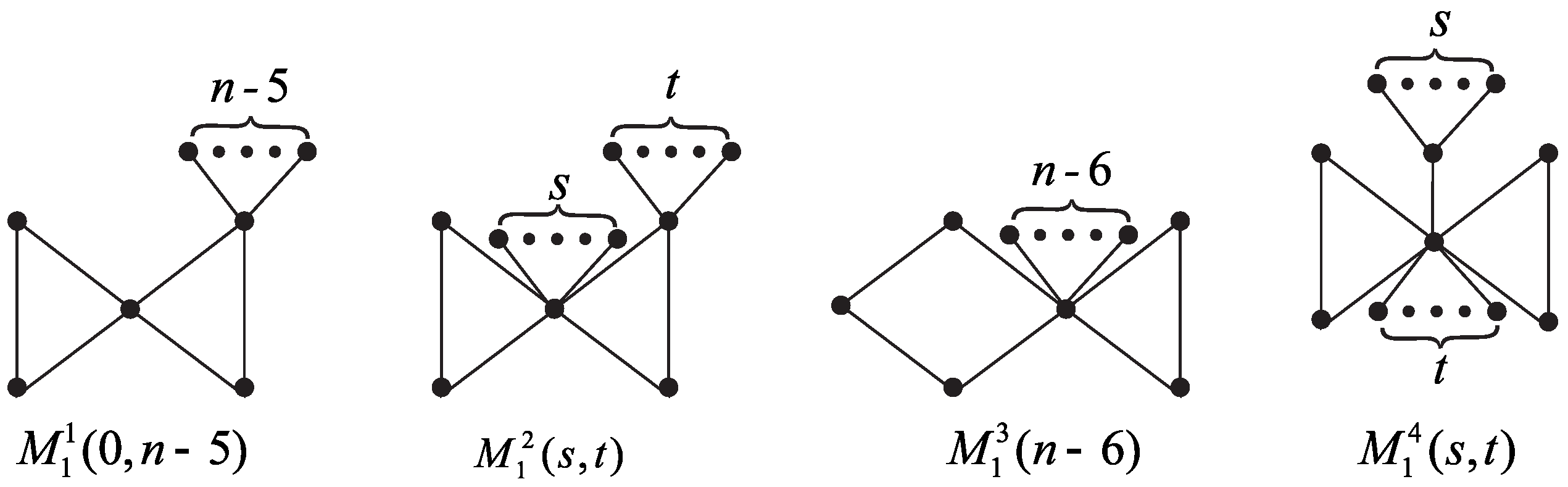
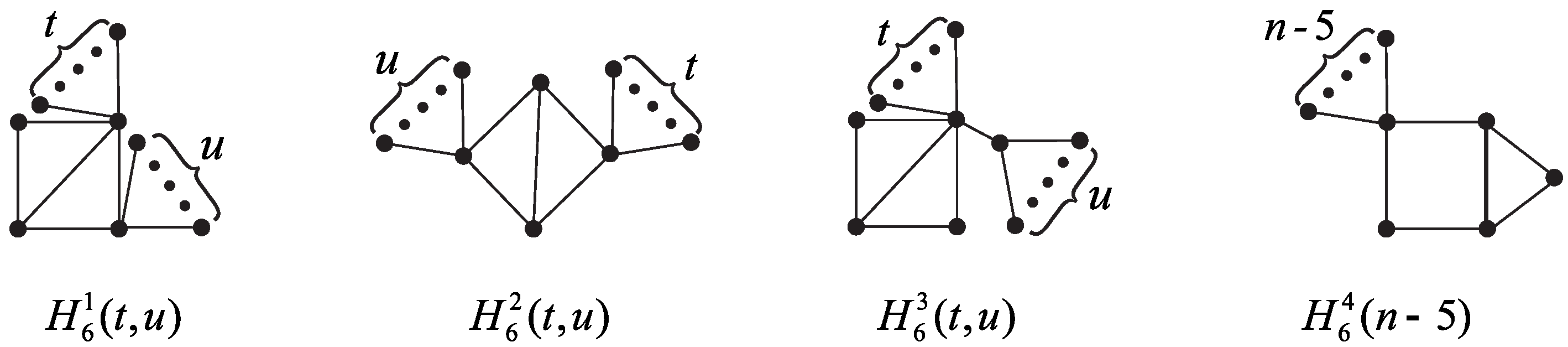
3. The Minimal Hosoya Indices of Bicyclics
3.1. The Second Minimal Hosoya Index of
3.2. The Minimal Hosoya Indices of
3.3. A Conclusion
4. The Minimal Permanental Sums of Bicyclic Graphs
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Valiant, L.G. The complexity of computing the permanent. Theoret. Comput. Sci. 1979, 8, 189–201. [Google Scholar] [CrossRef]
- Jerrum, M. Two dimensional monomer-dimer systems are computationally intractable. J. Statist. Phy. 1987, 48, 121–134. [Google Scholar] [CrossRef]
- Cash, G.G. The permanental polynomial. J. Chem. Inf. Comput. Sci. 2000, 40, 1203–1206. [Google Scholar] [CrossRef]
- Cash, G.G. Permanental polynomials of smaller fullerenes. J. Chem. Inf. Comput. Sci. 2000, 40, 1207–1209. [Google Scholar] [CrossRef] [PubMed]
- Kasum, D.; Trinajstić, N.; Gutman, I. Chemical graph theory. III. On permanental polynomial. Croat. Chem. Acta. 1981, 54, 321–328. [Google Scholar]
- Merris, R.; Rebman, K.R.; Watkins, W. Permanental polynomials of graphs. Linear Algebra Appl. 1981, 38, 273–288. [Google Scholar] [CrossRef]
- Wu, T.; So, W. Unicyclic graphs with second largest and second smallest permanental sums. Appl. Math. Comput. 2019, 351, 168–175. [Google Scholar] [CrossRef]
- Tong, H. Parallel Algorithms for Computing Permanents and Permanental Polynomials of Sparse Matrices. Ph.D. Thesis, Tsinghua University, Beijing, China, 2006. [Google Scholar]
- Xie, S.; Gao, F.; Lu, X.; Huang, R.B.; Wang, C.R.; Zhang, X.; Liu, M.L.; Deng, S.-L.; Zheng, L.-S. Capturing the labile Fullerene[50] as C50Cl10. Science 2004, 304, 699. [Google Scholar] [CrossRef]
- Wu, T.; Lai, H. On the permanental sum of graphs. Appl. Math. Comput. 2018, 331, 334–340. [Google Scholar] [CrossRef]
- Li, W.; Qin, Z.; Zhang, H. Extremal hexagonal chains with respect to the coefficients sum of the permanental polynomial. Appl. Math. Comput. 2016, 291, 30–38. [Google Scholar] [CrossRef]
- Li, S.; Wei, W. Extremal octagonal chains with respect to the coefficients sum of the permanental polynomial. Appl. Math. Comput. 2018, 328, 45–57. [Google Scholar] [CrossRef]
- Li, W.; Qin, Z.; Wang, Y. Enumeration of permanental sums of lattice graphs. Appl. Math. Comput. 2020, 370, 124–914. [Google Scholar] [CrossRef]
- Wu, T.; So, W. Permanental sums of graphs of extreme sizes. Discret. Math. 2021, 344, 112353. [Google Scholar] [CrossRef]
- Farrell, E.J. An introduction to matching polynomials. J. Combin. Theory Ser. B 1979, 27, 75–86. [Google Scholar] [CrossRef]
- Godsil, C.D.; Gutman, I. On the theory of the matching polynomial. J. Graph Theory 1981, 5, 137–144. [Google Scholar] [CrossRef]
- Huang, Y.; Shi, L.; Xu, X. The Hosoya index and the Merrifield-Simmons index. J. Math. Chem. 2018, 56, 3136–3146. [Google Scholar] [CrossRef]
- Wagner, S.; Gutman, I. Maxima and minima of the Hosoya index and the Merrifield– Simmons index: A survey of results and techniques. Acta Appl. Math. 2010, 112, 323–346. [Google Scholar] [CrossRef]
- Wu, T.; Jiu, X. Solution to a conjecture on the permanental sum. Axioms 2024, 13, 166. [Google Scholar] [CrossRef]
- Deng, H. The smallest Hosoya index in (n,n+1)-graphs. J. Math. Chem. 2008, 43, 119–133. [Google Scholar] [CrossRef]
- Wu, T.; Das, K. On the permanental sum of bicyclic graphs. Comput. Appl. Math. 2020, 39, 72–81. [Google Scholar] [CrossRef]
- Liu, H.; Lu, M. A unified approach to extremal cacti for different indices. MATCH Commun. Math. Comput. Chem. 2007, 58, 183–194. [Google Scholar]
- You, L.; Wei, C.; You, Z. The smallest Hosoya index of bicyclic graphs with given pendent vertices. J. Math. Res. Appl. 2014, 34, 12–32. [Google Scholar]
- Dolati, A.; Haghighat, M.; Golalizadeh, S.; Safari, M. The smallest Hosoya index of connected tricyclic graphs. MATCH Commun. Math. Comput. Chem. 2011, 65, 57–70. [Google Scholar]
- Ye, Y.; Pan, X.; Liu, H. Ordering unicyclic graphs with respect to Hosoya indices and Merrifield-Simmons indices. MATCH Commun. Math. Comput. Chem. 2008, 59, 191–202. [Google Scholar]
- Tepeh, A. Extremal bicyclic graphs with respect to Mostar index. Appl. Math. Comput. 2019, 355, 319–324. [Google Scholar] [CrossRef]
- Cruz, R.; Rada, J. Extremal values of the Sombor index in unicyclic and bicyclic graphs. J. Math. Chem. 2021, 59, 1098–1116. [Google Scholar] [CrossRef]
- Ilić, A.; Stevanović, D.; Feng, L.; Yu, G.; Dankelmann, P. Degree distance of unicyclic and bicyclic graphs. Discret. Appl. Math. 2011, 159, 779–788. [Google Scholar]
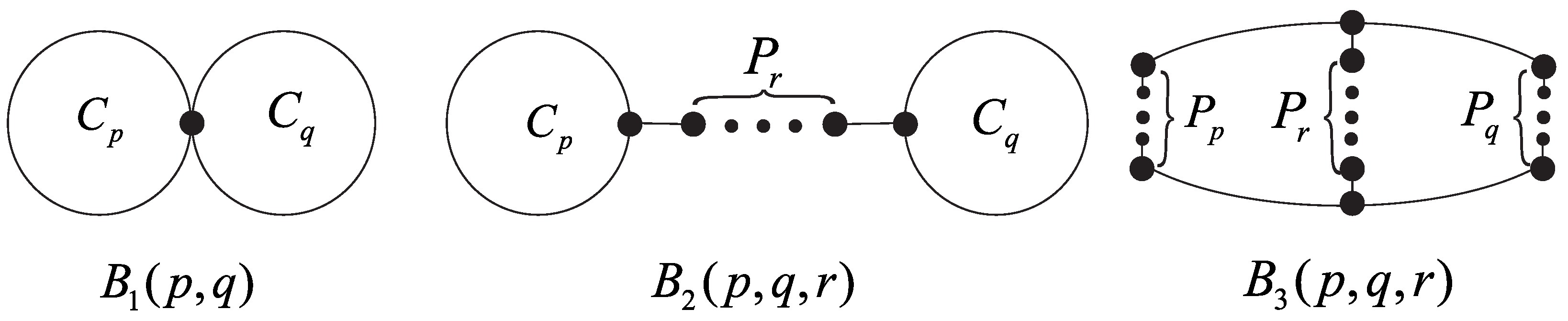
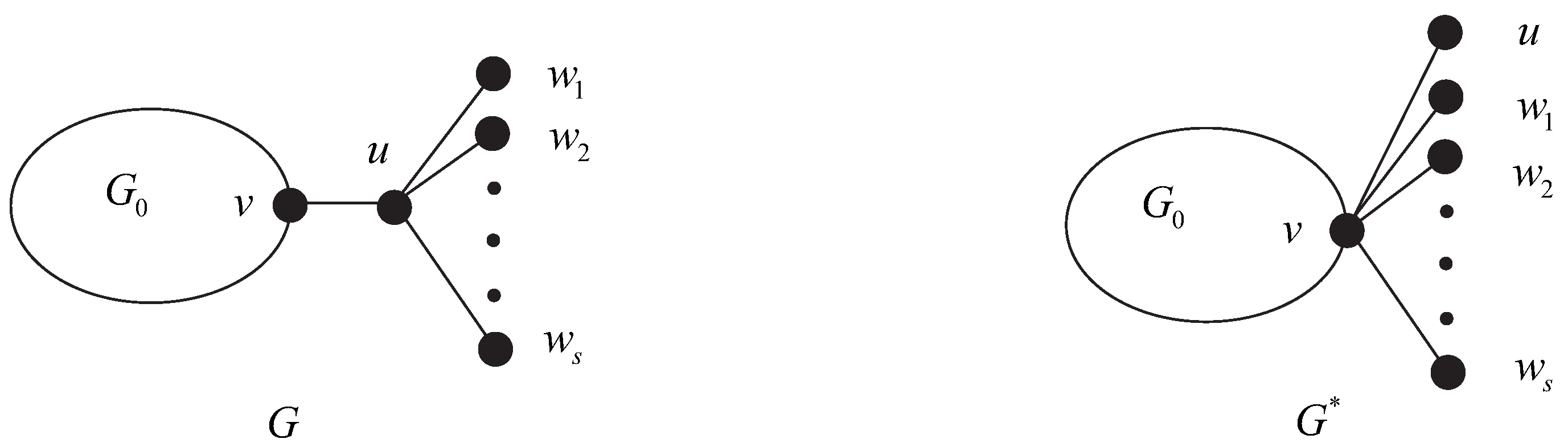
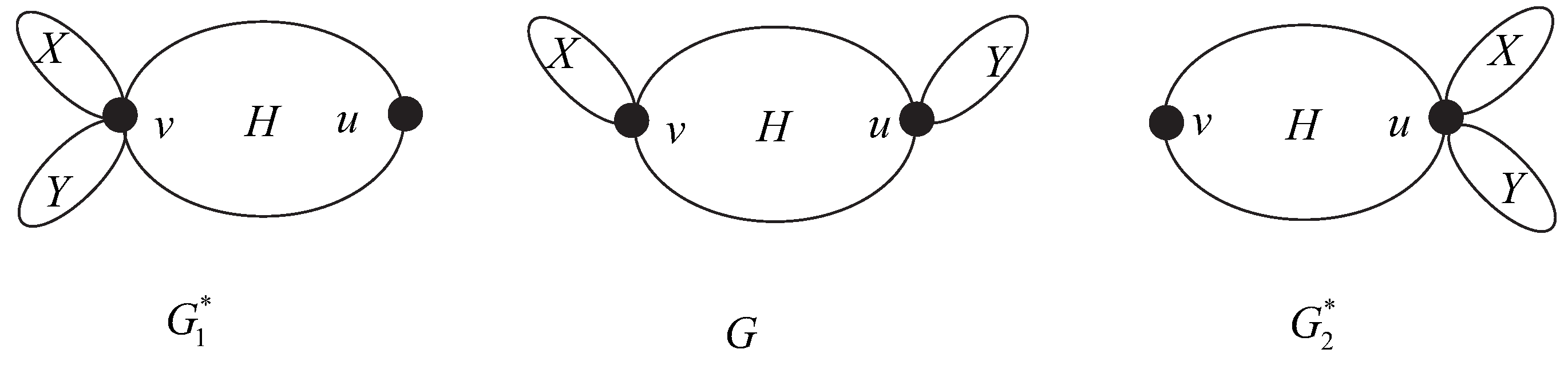

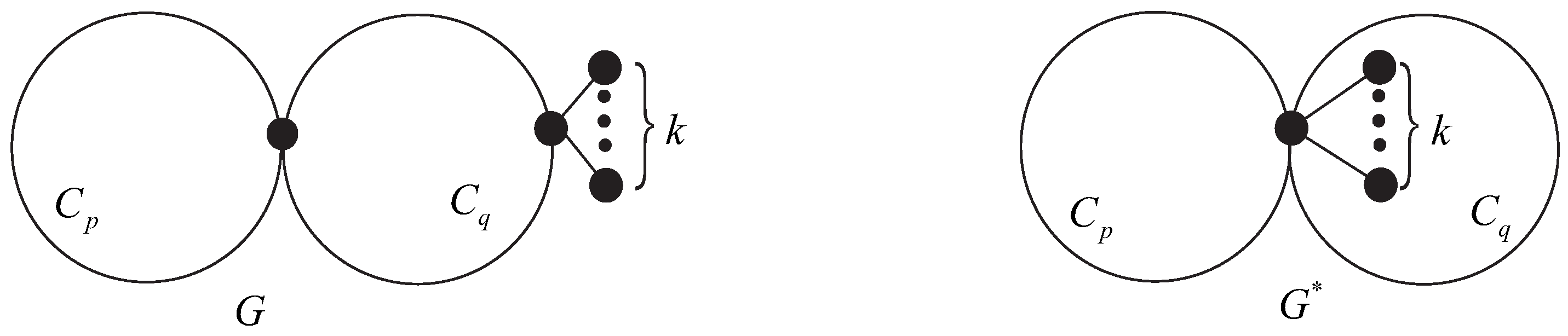




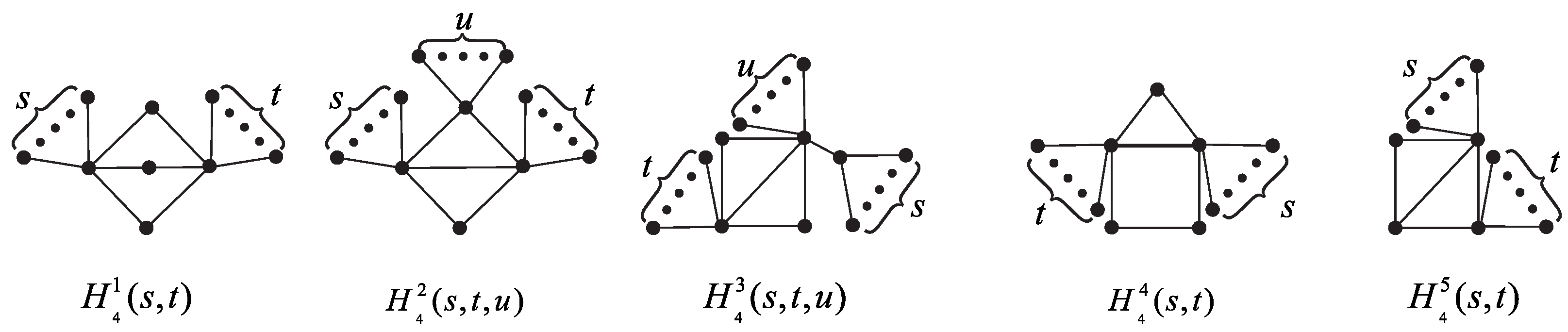
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, T.; Bai, Y.; Xu, S. Extremal Bicyclic Graphs with Respect to Permanental Sums and Hosoya Indices. Axioms 2024, 13, 330. https://doi.org/10.3390/axioms13050330
Wu T, Bai Y, Xu S. Extremal Bicyclic Graphs with Respect to Permanental Sums and Hosoya Indices. Axioms. 2024; 13(5):330. https://doi.org/10.3390/axioms13050330
Chicago/Turabian StyleWu, Tingzeng, Yinggang Bai, and Shoujun Xu. 2024. "Extremal Bicyclic Graphs with Respect to Permanental Sums and Hosoya Indices" Axioms 13, no. 5: 330. https://doi.org/10.3390/axioms13050330
APA StyleWu, T., Bai, Y., & Xu, S. (2024). Extremal Bicyclic Graphs with Respect to Permanental Sums and Hosoya Indices. Axioms, 13(5), 330. https://doi.org/10.3390/axioms13050330





